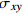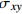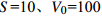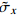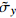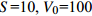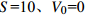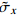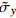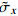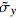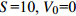基于广义混合有限元的压电复合材料层合板的数值分析

王聿航; 卿光辉

doi:10.13801/j.cnki.fhclxb.20210707.003

基于广义混合有限元的压电复合材料层合板的数值分析

王聿航,
卿光辉^,

中国民航大学　航空工程学院，天津 300300

基金项目: 国家自然科学基金(11502286)

详细信息

通讯作者:
卿光辉，博士，教授，硕士生导师，研究方向为广义混合有限元理论与方法、复合材料结构设计与修理　 E-mail：ghqing@cauc.edu.cn

中图分类号: O343.8
计量
- 文章访问数: 1262
- HTML全文浏览量: 502
- PDF下载量: 80
出版历程
- 收稿日期: 2021-05-10
- 修回日期: 2021-06-20
- 录用日期: 2021-06-29
- 网络出版日期: 2021-07-06
- 刊出日期: 2022-05-31

Analysis of piezoelectric composite laminates based on generalized mixed finite element

WANG Yuhang,
QING Guanghui^,

College of Aeronautical Engineering, Civil Aviation University of China, Tianjin 300300, China

摘要

摘要: 将纯弹性体的广义混合有限元法引入到压电材料的静力学分析中。由于采用了8节点六面体非协调实体单元对整体结构进行离散求解，摒弃了板壳理论中的诸多人为假设。非协调项的加入使该方法比同类协调元显示出更好的数值性能。本文方法将应力边界条件和位移边界条件同时考虑，并且求解过程中将层间应力和平面内应力分开处理，按每层的本构关系求解平面内应力，这样求得的层间应力和平面内应力都更加接近精确解。通过几个有代表性的层合板的数值算例说明了本文方法的精度，相较于传统的解析法和数值法，本文方法在适用性和有效性方面都具有优势。
- 压电复合材料 /
- 层合板 /
- 广义混合有限元 /
- 非协调 /
- 机电耦合
Abstract: Generalized mixed finite element method of pure elastomer was introduced into the static analysis of piezoelectric materials. Because the 8-node hexahedral noncompatible solid element was used to solve the whole structure discretely, many artificial assumptions in the theory of plate and shell were abandoned. The addition of noncompatible terms makes this method exhibit better numerical performance than the same kind of compatible elements. In this method, the stress boundary conditions and displacement boundary conditions were considered simultaneously, and the interlaminar stress and in-plane stress were treated separately in the solution process, and the in-plane stress was calculated according to the constitutive relation of each layer, so that the obtained interlaminar stress and in-plane stress are closer to the exact solution. The accuracy of the proposed method was illustrated by several representative numerical examples of laminates. Compared with the traditional analytical and numerical methods, the theoretical method presented in this paper has advantages in applicability and effectiveness.
- piezoelectric composite /
- laminated plates /
- generalized mixed finite element /
- noncompatible /
- coupling of mechanics and electricity

HTML全文

橡胶制品在日常生活中应用十分广泛，例如轮胎、传送带、密封垫圈等^[1]。硫化是将橡胶材料制成橡胶制品的关键工序，对产品的质量和寿命有着重要影响^[2]。尤其对于橡胶厚制品(厚度≥1.2 mm)来说，由于其本身的厚度较大，在硫化过程中易产生硫化不均的现象^[3-4]。针对这种问题，在橡胶配方中加入导热填料，如氧化石墨烯(GO)和多壁碳纳米管(MWCNTs)等，既可提高橡胶材料的导热性，从而提高硫化效率，同时也增强了橡胶的综合物理性能^[5]。但是仅将单一的导热填料添加到橡胶当中，往往要较大添加量才可达到使用要求，其填料自身的性能不能完全体现，并且由于其较大的添加量，导致其在橡胶中难以分散，增加了橡胶混炼的难度，降低了橡胶的多项物理性能。

MWCNTs结构稳定、各方面性能突出，使其在纳米材料应用领域备受关注^[6-7]。但由于MWCNTs易团聚、表面能低、各向异性等原因使其难以发挥自身优良的力学性能，因此常将MWCNTs与一些聚合物共混制备复合材料，如在橡胶、树脂、纤维等中加入MWCNTs可以同时发挥二者优势，使纳米复合材料的力学性能、耐磨性、导热、导电性能都得到较大提高^[8]。Kueseng等^[9]在天然橡胶中加入少量的MWCNTs制备MWCNTs/天然橡胶复合材料，发现橡胶的弹性模量和拉伸强度都得到了很大的提升。加入质量分数为1wt%MWCNTs时，胶料的拉伸强度从原来的0.28 MPa提升至0.56 MPa，断裂伸长率变化不大。在加入10wt%MWCNTs时，胶料的拉伸强度达到了2.55 MPa，但断裂伸长率下降明显，从原来的7.34%降低至2.94%。Falco等^[10]通过将MWCNTs与丁苯橡胶共混，发现MWCNTs的加入，会使得丁苯橡胶硫化速率降低。Das等^[11]在制备MWCNTs/丁苯-丁二烯复合材料时发现，当MWCNTs质量分数为4wt%时，复合材料的导热率由未加MWCNTs时的0.08 W/(m·K)提升至0.13 W/(m·K)。

GO表面含有丰富的含氧官能团，使得GO片层带负电荷，一些带有正电荷的阳离子会被吸引到相邻的片层之间，削弱片层之间的范德华力，片层间距随之变大，这有利于一些聚合物或者纳米填料粒子与GO相结合^[12]。近年来，随着人们对复合材料的需求越来越高，GO作为一种填料无论在聚合物基复合材料还是无机物基复合材料领域都颇受重视。谢苗等^[13]通过湿法混炼将GO与丁基胶乳(Isobutylene isoprene rubber, IIR) 共混制备GO/IIR复合材料，发现当GO质量分数为3wt%时得到的复合材料物理力学性能最佳。Allahbakhsh等^[14]通过将GO与三元乙丙橡胶共混，研究复合材料混炼胶的硫化性能。发现随着GO含量的适量增加，硫化活化能增加，加入更多的GO时，复合材料的导热率明显提高，导致硫化活化能降低。

导热橡胶按照导热原理分为本征型导热橡胶和填充型导热橡胶^[15]。填充型导热橡胶的导热性能主要来源于橡胶基团与导热填料之间形成的共同界面，分散在橡胶基体中填料粒子的形态主要有纤维状、片状、粒状，填料粒子形态结构不同，对其在橡胶基体中的分布状况也不同，形成的复合材料不管是在硫化性能、导热性能以及力学性能上都有所不同^[16]。本实验将具有一维线性结构的MWCNTs与二维平面结构的GO共同当作导热填料，添加到胎面胶配方中，通过二者的协同作用来搭建微观“导热桥梁”的填料网络，如图1所示，使胶料表现出优于其中任意一种单一填料的良好性能^[17]。并探究二者的配比对天然橡胶的硫化特性、导热性能与力学性能方面的影响^[18]。

图 1 氧化石墨烯/多壁碳纳米管 (GO/MWCNTs) 填料网络示意图

Figure 1. Schematic diagram of graphene oxide/multi-walled carbon nanotubes (GO/MWCNTs) packing network

下载: 全尺寸图片幻灯片

1. 实验材料及方法

1.1 原材料

天然橡胶 (Natural rubber，NR)，型号为RSS3，泰国进口；炭黑，型号为N330，卡博特（中国）有限公司；白炭黑，型号为1165MP，索尔维精细化工添加剂（青岛）有限公司；氧化锌 (ZnO)、防老剂4020、防老剂RD、硅烷偶联剂Si69、增塑剂A、硬脂酸、微晶蜡、防焦剂CTP、环保芳烃油、促进剂NS、硫磺均为市售工业级；多壁碳纳米管 (MWCNTs)，型号为JCMWC1，其物理参数为管径4~6 nm、长度10~20 μm、比表面积380~550，成都佳材科技有限公司；氧化石墨烯 (GO)，其物理参数为厚度≤1 μm、片径约为10 μm、单层，深圳粤创科技有限公司。

1.2 实验配方

基础配方：NR，100；炭黑，38.5；白炭黑，15；氧化锌，3.5；防老剂4020，2；防老剂RD，1.5；Si69，2；增塑剂A，2；硬脂酸，2；微晶蜡，1；防焦剂CTP，0.3；促进剂NS，1.25；硫磺，1。

在上述配方基础上，本次实验使用了6wt%的MWCNTs，同时配合了不同用量的GO，配方编号及GO的用量见表1。

1.3 混炼胶和硫化胶的制备

(1) NR塑炼

用宝轮精密检测仪器有限公司生产的BL-6157型双辊开炼机将取出的生胶进行塑炼，工艺步骤为：烘胶→破胶→薄通→放厚→下片；工艺参数设定为：冷却水温度为40~50℃，2 mm辊距破胶3次，0.2 mm辊距薄通15次，3 mm辊距下片3次。

表 1 GO/MWCNTs的不同配比

Table 1. Different ratios of GO/MWCNTs

Number	0#	1#	2#	3#	4#	5#	6#	7#
MWCNTs/wt%	0	6	6	6	6	6	6	6
GO/wt%	0	0	1	2	3	4	5	6

下载: 导出CSV

| 显示表格

(2) 密炼

将塑炼好的NR制成细长条加入上海科创橡塑机械设备有限公司生产的XSM-500型橡胶密炼机进行密炼。工艺参数设定为：填充系数为0.65，初始温度为室温25℃，上顶栓压力为0.6 MPa，冷却水温度为40℃，转子转速为80 r/min。混炼工艺步骤：密炼室内加入NR，计时45 s后加炭黑小料(硫磺、促进剂NS、防焦剂CTP除外)，计时20 s后提上顶栓后再落下，再计时20 s后加环保油、白炭黑，密炼室温度达140℃时提上顶栓翻胶后再落下，再计时60 s后提上顶栓开模，停机排胶、测试并记录胶温。

(3) 混炼胶开炼

开炼工艺步骤：压片、薄通后加硫磺、促进剂，左右割胶、打三角包轮换进行3~5次，下片，贴标签，覆膜静置12 h。

(4) 硫化胶试样制备

将晾放后的混炼胶试样按照每份29 g的标准进行裁样，将模具预热，按照开炼机上添加硫化体系时的压延方向将每份试样平坦地置于模腔中央，合上上下模具并放入平板硫化机中进行硫化。硫化时间为t_c90×1.3，硫化温度150℃，上模气压10 MPa。待平板硫化机达到设定时间自动开模后取出胶片贴上标签，晾放12 h。

1.4 表征

1.4.1 混炼胶硫化特性测试

使用台湾高铁检测仪器有限公司生产的M-2000-AN型无转子硫化仪来测试胶料的焦烧时间t_c10、正硫化时间t_c90以及最大扭矩M_H、最小扭矩M_L等硫化特性参数。将无转子硫化仪上下模预热至设定温度后进行测试，执行标准为GB/T 16584—1996^[19]测试参数为：温度为150℃，上模气压设定为0.6 MPa，测试时间为60 min，开模条件为达到测试时间或硫化曲线持平6 min。待测试完成后对所得数据进行记录保存。

1.4.2 硫化前、硫化后导热性能测试

实验所用仪器为德国耐驰公司生产的LFA447型激光闪射导热仪。实验分别对加入不同质量分数GO的混炼胶和硫化胶的导热率进行测试，测试温度从30℃递增至120℃，中间每隔30℃作为一个温度测试点对混炼胶的导热率进行测试，每个相同温度测三次，去掉极端值后取平均值作为该试样的导热率。

该仪器导热率的获得是通过测试材料的热扩散系数间接获得的，二者之间存在如下换算关系^[20]：

${\lambda }={\alpha }\times{C_{\rm{p}}}\times{\rho }$

(1)

其中： ${\lambda }$ 为胶料的导热率； ${C_{\rm{p}}}$ 为材料的比热； ${\rho }$ 为胶料的密度；α为热扩散系数。

(1) 热扩散系数的测量

仪器工作时会将氙灯作为加热源，由其瞬间发射出一束短脉冲打在胶料下表面，脉冲提供的热量会向试样的上表面传递，红外探测器此时会记录试样上表面的温度变化。实验开始时，需要对红外探测器的信号进行放大和矫正，得到温升曲线。曲线反应了测试过程中实验的温度变化，上表面温度表示试样几何尺度、热扩散系数与热损失之间的函数关系，在软件中选择合适的数学模型，计算得到试样的热扩散系数。

在软件中选择Cowan数学模型，通过确定无量纲数C、R来计算试样的热扩散系数。R值由程序根据导热仪所测试得到的数据计算而来，无热损时，R=2，C=0.1388。

$C=\frac{{\alpha }_{{t}_{50}}}{{d}^{2}};\;\;\;\;R=\frac{{\theta }_{5{t}_{50}}}{\theta_{{t}_{50}}}$

(2)

其中： ${\theta }_{{t}_{50}}$ 为时间为t₅₀时的温升； ${\theta }_{5{t}_{50}}$ 代表时间为5倍的 ${\theta }_{{t}_{50}}$ 时的温升；t₅₀为温度升到峰值50%所需时间； $\alpha_{t_{50}}$ 为时间为 t₅₀时的热扩散系数；d为试样厚度。

(2) 比热容的测量

试样的比热容是通过与标准试样(参比)的参数进行比较得到的，测试时要注意以下几点：

(i) 试样与参比的形状大小相同；

(ii) 试样表面需平整光滑；

(iii) 测试前需对试样进行石墨喷涂；

(iv) 试样与参比的热物性相似。

信号通过热损耗修正后得到的试样比热简化计算公式为

$\frac{{C}_{{\rm{pd}}}{m}_{{\rm{d}}}}{{C}_{{\rm{ps}}}{m}_{{\rm{s}}}}=\frac{{H}_{{\rm{s}}}}{{H}_{{\rm{d}}}}$

(3)

其中： ${C}_{{\rm{pd}}}$ 为橡胶复合材料的比热； ${m}_{{\rm{d}}}$ 为橡胶复合材料的质量； $H_{{\rm{d}}}$ 为橡胶复合材料的信号值； ${C}_{{\rm{ps}}}$ 为标准试样的比热； ${m}_{{\rm{s}}}$ 为标准试样的质量； $H_{{\rm{s}}}$ 为标准试样的信号值。公式(3)将已知比热参数的参比温升曲线的信号值与样品作反比，从而由已知参数的标样得到未知试样的比热。

(3)导热率测量

在得出试样的比热值后，可通过公式(3)计算材料的导热率。

1.4.3 混炼胶门尼黏度测试

本实验使用台湾优肯科技股份有限公司生产的 UM-2050 型门尼黏度计测试，执行标准为 GB/T 1232.1—2016^[21]。用符号 ML100℃1+4 表示，其中：M—门尼；L—大转子；1—预热时间 1 min；4—转动测试时间 4 min；100℃—试验测试温度。

1.4.4 硫化胶力学性能测试

本实验使用台湾优肯科技股份有限公司生产的TS 2005b型拉力试验机对所制备的硫化胶试样进行测试，测试项目包括定伸应力、断裂伸长率、拉伸强度、撕裂强度等。使用裁刀将所制备的硫化胶片裁出分别用于拉伸性能和撕裂性能测试的标准样条，各5片。试样两端对称夹紧于试验机夹具的上下两部分，试样长度方向轴线需与拉伸受力方向一致，并使试样处于自然无弯曲状态。按照GB/T 528—2009^[22]标准进行测试，在软件中输入每次测试对应的试样厚度，拉伸速率为500 mm/min，记录每次的测试值。本实验使用上海六菱仪器厂生产的LX-A型邵尔硬度计测试硫化胶的硬度，执行标准为GB/T 531.1—2008^[23]，测试要求：(1) 试样厚度≥6 mm，宽度≥15 mm，长度≥35 mm；(2) 试样表面应光滑、平整、无机械损伤及气泡存在，测试前用酒精擦拭表面。

2. 结果与讨论

图2(a)~2(d)分别为当MWCNTs添加量为6wt%时，GO含量对GO/MWCNTs橡胶复合材料最大扭矩M_H、交联密度ΔM、焦烧时间t_c10以及正硫化时间t_c90的影响。如图所示，当在基础配方中加入6wt%MWCNTs时，第二填料GO的加入使得胶料的硫化时间再次缩短，t_c10随着GO的增加基本呈现减小趋势，当GO的份数在3wt%时， t_c90取得最小值；最大扭矩M_H反映了胶料的剪切模量，与胶料的密度成正比^[24]。如图2(a)、2(b)所示，随着GO含量的增加，胶料的最大扭矩M_H和交联密度ΔM逐渐增大，这一方面是因为GO的插层作用以及较大的接触面积促进了交联反应；另一方面GO与MWCNTs之间形成的导热通路使得聚集的热量迅速扩散，形成的交联网络更加均匀^[25-26]；另外，越多的GO粒子存在于橡胶基体中，使得GO自身携带的自由基也会与橡胶分子链发生交联反应，使得橡胶的交联密度整体上得到提升^[27]。

图 2 GO含量对GO/MWCNTs橡胶复合材料最大扭矩M_H(a)、交联密度ΔM (b)、焦烧时间t_c10(c) 以及正硫化时间t_c90(d) 的影响

Figure 2. Influence of GO content of GO/MWCNTs rubber composite on the maximum torque M_H(a), crosslink density ΔM (b), scorching time t_c10 (c) and positive vulcanization time t_c90 (d)

下载: 全尺寸图片幻灯片

图 3 不同温度下GO/MWCNTs配比对GO/MWCNTs橡胶复合材料导热率的影响

Figure 3. Influence of GO/MWCNTs ratio on the thermal conductivity of GO/MWCNTs rubber composite at different temperature

下载: 全尺寸图片幻灯片

如图2(c)、2(d)所示，当GO与6wt%MWCNTs同时加入橡胶基体中，胶料的硫化效率在GO粒子含量较低时有一定的提高，这是因为GO未加入前，大部分MWCNTs在橡胶集体中处于分散状态，高度分散的MWCNTs粒子虽穿插于橡胶基体中，但是填料粒子间的接触并不完全；加入GO后，其特殊的平面结构会形成类似于MWCNTs的依附载体的形式。GO片径约为10 μm，而MWCNTs的管径只有5 nm，这会使得MWCNTs粒子的两端可以桥接在GO表面，二者的协同作用下形成导热通路，使得硫化过程中热量的传递效率提高，降低了硫化时间^[28]。另外，GO表面附有的丰富含氧基团使得GO整体呈弱酸性，这会吸收部分配方中的促进剂，抑制了硫化反应的发生^[29]。因此，GO含量在达到3wt%后，t_c90变化不再明显。由此可见，聚合物基体中存在良好的导热通路是提高橡胶硫化效率的重要影响因素，该作用规律对橡胶硫化过程的节能减耗具有指导意义。

2.1 GO/MWCNTs的配比对混炼胶及硫化胶导热性能的影响

图3(a)~3(d)分别为30℃、60℃、90℃、120℃时GO/MWCNTs配比对GO/MWCNTs橡胶复合材料导热率的影响。如图所示，当在基础配方中定量加入6wt%MWCNTs和不同含量的GO，无论是混炼胶还是硫化胶的导热率都有了明显的提升，当加入6wt%MWCNTs与6wt%GO 时，相较原基础配方，在120℃时(图3(d))两种导热填料的加入使得混炼胶的导热率从原来的0.168 W/(m·K)提高到0.309 W/(m·K)，硫化胶的导热率从原来的0.161 W/(m·K)提高到0.302 W/(m·K)，增幅分别达83.9%和87.6%；相较只加入6wt%MWCNTs而未加入GO时单一导热填料作用下胶料的导热性能而言，第二导热填料GO的加入使得前者导热率得到了进一步的提升，当GO含量为6wt%，在120℃时混炼胶及硫化胶的导热率增幅分别为25.1%和23.3%，说明在GO与MWCNTs多元导热填料的协同作用下，胶料的导热性能进一步得到了提高。

随着GO含量的增加，混炼胶及硫化胶的导热率增量越来越明显。究其原因，当橡胶基体中仅含有MWCNTs单一导热填料时，MWCNTs粒子细长的一维结构穿插于橡胶分子链之间，相邻填料粒子难以形成大面积接触对，MWCNTs自身优越的导热性能宏观上没有得到完全体现。由于GO的物理化学性能与MWCNTs具有相似性，因此当加入导热性能极好的GO粒子后，橡胶体系的宏观导热性能定会得到一定提升。另外，二维片层结构的GO粒子会将MWCNTs两端顺次相连，二者在微观状态下出现协同“架桥”作用^[30]。

图4展示了GO/MWCNTs的协同作用。如图所示，在橡胶基体中形成了良好的导热通道，热量传递过程中积聚的热量迅速传导开来，提高了体系的导热性能。并且，GO自身的片层结构使其在加入橡胶基体后容易堆叠，分散不均，而一维结构 MWCNTs粒子的存在能够在一定程度上抑制GO堆叠团聚，提高GO在橡胶基体中的分散性，使得热量传递更加均匀^[31]。

图 4 GO/MWCNTs的协同作用示意图

Figure 4. Diagram of synergy for GO/MWCNTs

下载: 全尺寸图片幻灯片

当添加3wt%GO和6wt%MWCNTs时，胶料硫化时间最短，对于节能意义最大，故分析此情况下混炼胶及硫化胶导热率随温度的变化情况，如图5所示。当GO添加量一定时，随着温度的增加，混炼胶及硫化胶的导热率呈增高趋势，且混炼胶的导热率要高于硫化胶导热率。这是因为温度越高，橡胶内部晶格振动越剧烈，振幅较大，相邻质点间的热量传递效率更高，橡胶导热率表现出升高趋势^[32]。随着温度升高至90~120℃，混炼胶及硫化胶的导热率差距越来越小，这是因为在测试温升过程中，混炼胶内部逐渐发生局部硫化，分子间化学键作用力使晶格运动受到束缚，难以从初始位置脱离来影响周围质点，抑制了橡胶体系中热量的传导，使混炼胶的热力学性质逐渐趋向于硫化胶。

图 5 温度对GO/MWCNTs橡胶复合材料导热率的影响

Figure 5. Influence of temperature on the thermal conductivity of GO/MWCNTs rubber composite

下载: 全尺寸图片幻灯片

2.2 GO/MWCNTs的配比对混炼胶门尼黏度的影响

表2为混炼胶的门尼黏度数据，由表可知，两种填料的同时加入使得胶料门尼黏度出现了再次降低趋势，这是因为胶料在门尼黏度仪的测试过程中，其内部一维结构的MWCNTs与二维平面GO粒子会根据受力方向发生取向，橡胶体系中的分子链段运动方向一致，增加了混炼胶中分子链的流动性^[33]。因此，GO/MWCNTs/NR复合材料的门尼黏度出现了持续降低的趋势，有利于提升复合材料的加工性能。

表 2 GO/MWCNTs橡胶复合材料混炼胶的门尼黏度

Table 2. Mooney viscosity for compounding of GO/MWCNTs rubber composites

MWCNTs /wt%	GO /wt%	Mooney viscosity/ ML100℃1+4
6	0	46.52
	1	45.23
	2	43.47
	3	42.49
	4	41.55
	5	40.98
	6	38.38
Notes: M—Mooney; L—Large rotor; 1—Preheating for 1 min; 4—Trial for 4 min.

下载: 导出CSV

| 显示表格

2.3 GO/MWCNTs的配比对硫化胶力学性能的影响

图6(a)~6(f)分别为GO/MWCNTs配比对GO/MWCNTs橡胶复合材料100%定伸应力、300%定伸应力、拉伸强度、拉断伸长率、撕裂强度和硬度的影响。如图所示，当MWCNTs与GO两种填料共同使用时，得到的MWCNTs/GO/NR复合材料的力学性能相较仅加入MWCNTs单一填料的胶料而言，随着GO添加量的不断增加，胶料的拉伸强度、撕裂强度与拉断伸长率均出现降低的趋势。胶料的100%定伸应力和300%定伸应力均出现先升高后降低的趋势，当GO含量为3wt%时，胶料的100%定伸应力和300%定伸应力均达到最佳。另外，GO作为第二补强填料的加入使胶料的硬度值也得到了提升。

图 6 GO/MWCNTs配比对GO/MWCNTs橡胶复合材料100%定伸应力 (a)、300%定伸应力 (b)、拉伸强度 (c)、拉断伸长率 (d)、撕裂强度 (e) 和硬度 (f) 的影响

Figure 6. Influence of GO/MWCNTs ratio on 100% constant elongation stress (a), 300% tensile stress (b), tensile strength (c), tesile elongation (d), tearing strength (e) and hardness (f) of GO/MWCNTs rubber composite

下载: 全尺寸图片幻灯片

如图6(a)和6(b)所示，当GO添加量较少时，在橡胶基体中分散均匀，GO具有较大比表面积和良好的力学性能，使得GO粒子与橡胶分子之间存在较强的范德华力，起到了物理交联的作用^[34]。另外，GO的加入使得橡胶导热率有所提升，促进了硫化的均匀反应，减少了橡胶基体中因硫化不均出现的“缺陷”；GO表面附有的一些极性含氧基团，比如羧基、羟基和环氧基等，可以与橡胶分子之间发生化学反应形成π-π键或者氢键，起到了化学交联的作用，使得体系的表观交联密度得到了提升，进而增强了胶料的定伸应力。当GO含量持续增加超过3wt%时，越来越多片层结构的GO发生堆叠现象，在橡胶基体中难以分散，分布不均，导致了内部应力集中现象的发生，降低了胶料的拉伸强度。MWCNTs与GO两种填料粒子很大程度上提升了体系的刚度与硬度，但同时会使得体系的脆性增强，导致拉伸强度、拉断伸长率与撕裂强度随之降低，其变化规律如图6(c)至6(f)所示。

从实际应用角度来讲，以胎面胶配方为基础通过添加GO/MWCNTs粒子来对NR改性，得到的复合材料的拉伸强度和撕裂强度并没有太大的现实意义，并且胎面胶正常工况下很难发生500%及以上的形变量。因此，对于工业生产，胶料的100%定伸应力和300%定伸应力的提高更具指导意义。综合来说，当GO与MWCNTs填料添加量分别为3wt%与6wt%时，胶料的综合性能表现最好。

3. 结论

由实验结果可知，多壁碳纳米管(MWCNTs)填料与氧化石墨烯(GO)填料二者存在协同作用，并且不同配比的MWCNTs与GO对胶料性能的影响也不同，当MWCNTs填料定量加入6wt%时，随着GO含量的增加：

(1) 硫化胶的最大转矩M_H与交联密度ΔM值呈增大趋势，表明GO与MWCNTs之间的协同作用再次提高了胶料的交联密度；混炼胶的门尼黏度持续降低，有助于改善橡胶的加工性能；

(2) 焦烧时间t_c10和正硫化时间t_c90先降低，在3wt%后t_c90略有回升，说明二者的协同作用能提高胶料的硫化效率，且当GO与MWCNTs填料添加量分别为3wt%与6wt%时，对硫化效率的提升最为明显；

(3) 混炼胶及硫化胶的导热率均有进一步的提高，当二者同时加入6wt%时，混炼胶与硫化胶的导热率分别提高了25.1%和23.3%。表明GO的加入使原单一导热填料配方体系出现了“架桥”现象，热量在粒子间更易传递；

(4) 硫化胶的100%定伸应力、300%定伸应力出现升高趋势，在 GO 含量为3wt%之后略微下降；拉伸强度、撕裂强度与拉断伸长率有所降低。

综合来看，当MWCNTs与GO添加量分别为6wt%与3wt%时，填料粒子对橡胶的补强效果最佳，其良好的导热性能增强了硫化反应的均匀性，实现了硫化过程的节能减耗。

图 1 锆钛酸铅压电陶瓷(PZT-4)压电层合板几何模型

Figure 1. Geometric model of lead zirconate titanate ceramic (PZT-4) piezoelectric laminates

下载: 全尺寸图片幻灯片

图 2 PZT-4压电层合板模型网格划分

Figure 2. Model meshing of PZT-4 piezoelectric laminates

下载: 全尺寸图片幻灯片

图 3 PZT-4压电层合板纵向位移u沿z轴的分布

Figure 3. Distribution of longitudinal displacement u of PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 7 工况1下PZT-4压电层合板平面内应力 ${\sigma }_{xy}$ 沿z轴的分布

Figure 7. Distribution of in-plane stress ${\sigma }_{xy}$ of PZT-4 piezoelectric laminates along the z axis under condition 1

下载: 全尺寸图片幻灯片

图 4 PZT-4压电层合板横向位移w沿z轴的分布

Figure 4. Distribution of the transverse displacement w of PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 5 PZT-4压电层合板层间应力 ${\sigma }_{z}$ 沿z轴的分布

Figure 5. Distribution of interlaminar stress ${\sigma }_{z}$ in PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 6 PZT-4压电层合板平面内应力 ${\sigma }_{x}$ 沿z轴的分布

Figure 6. Distribution of in-plane stress ${\sigma }_{x}$ of PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 8 PZT-4压电层合板层间应力 ${\sigma }_{xz}$ 沿z轴的分布

Figure 8. Distribution of interlaminar stress ${\sigma }_{xz}$ of PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 10 工况2下PZT-4压电层合板平面内应力 ${\sigma }_{xy}$ 沿z轴的分布

Figure 10. Distribution of in-plane stress ${\sigma }_{xy}$ of PZT-4 piezoelectric laminates along the z axis under condition 2

下载: 全尺寸图片幻灯片

图 9 PZT-4压电层合板电势φ沿z轴的分布

Figure 9. Distribution of the potential φ of PZT-4 piezoelectric laminates along the z axis

下载: 全尺寸图片幻灯片

图 11 聚偏氟乙烯(PVDF)压电层合板几何模型

Figure 11. Geometric model of polyvinylidene fluoride (PVDF) piezoelectric laminates

L—Length; H—Height

下载: 全尺寸图片幻灯片

图 12 PVDF压电层合板模型网格划分

Figure 12. Model meshing of PVDF piezoelectric laminates

下载: 全尺寸图片幻灯片

图 13 $S\text{=10}{\text{、}}V_{\text{0}}\text{=100}$ PVDF压电层合板平面内应力 ${\tilde \sigma _x}$ 、 ${\tilde \sigma _y}$ 沿z轴的分布

Figure 13. Distribution of in-plane stress ${\tilde \sigma _x}$ , ${\tilde \sigma _y}$ of PVDF piezoelectric laminates along the z axis when $S\text{=10}{\text{,}}\;V_{\text{0}}\text{=100}$

下载: 全尺寸图片幻灯片

图 14 $S\text{=10}{\text{、}}V_{\text{0}}\text{=0}$ PVDF压电层合板平面内应力 ${\tilde \sigma _x}$ 、 ${\tilde \sigma _y}$ 沿z轴的分布

Figure 14. Distribution of in-plane stress ${\tilde \sigma _x}$ , ${\tilde \sigma _y}$ of PVDF piezoelectric laminates along the z axis when $S\text{=10}{\text{,}}\;V_{\text{0}}\text{=0}$

下载: 全尺寸图片幻灯片

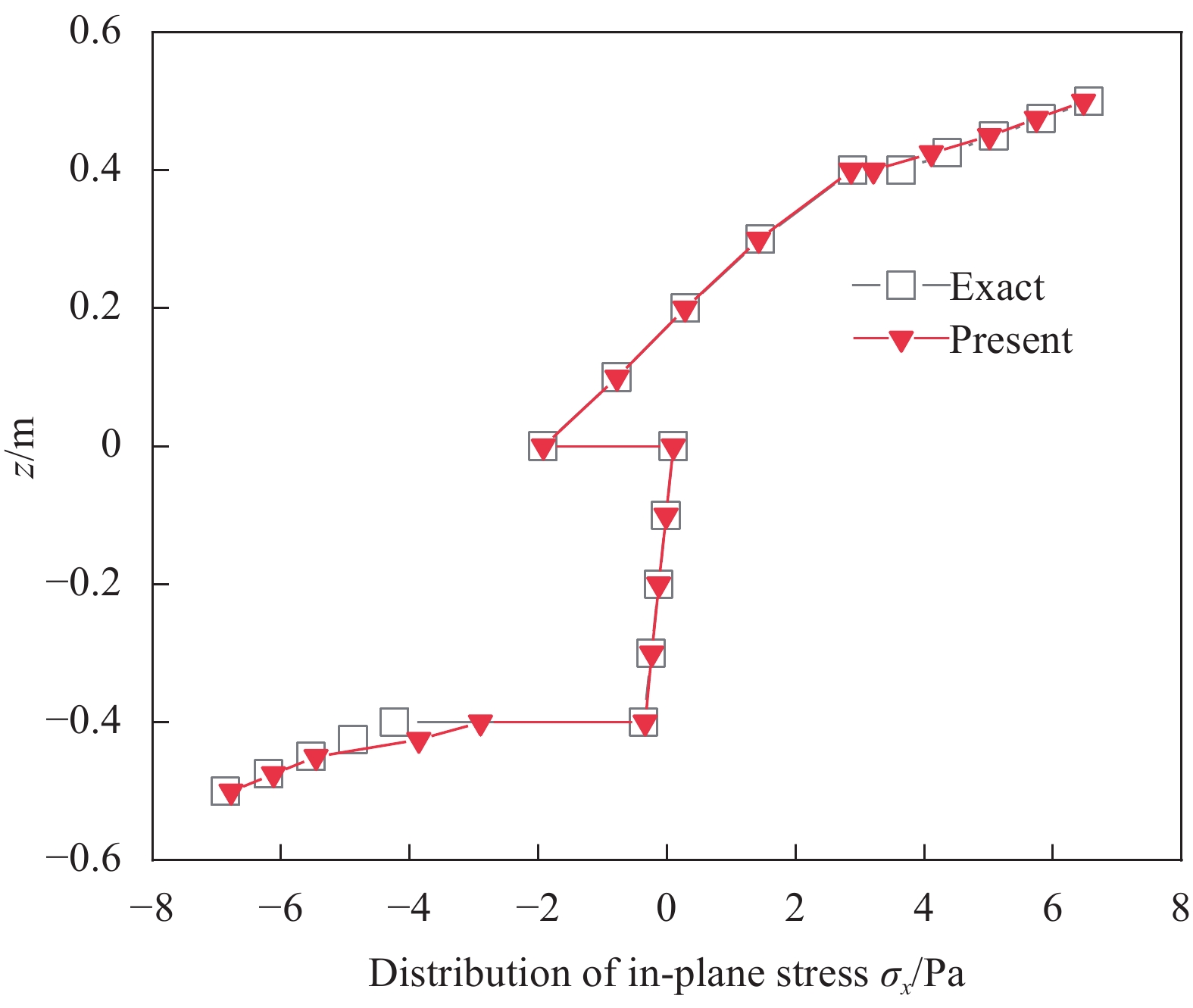
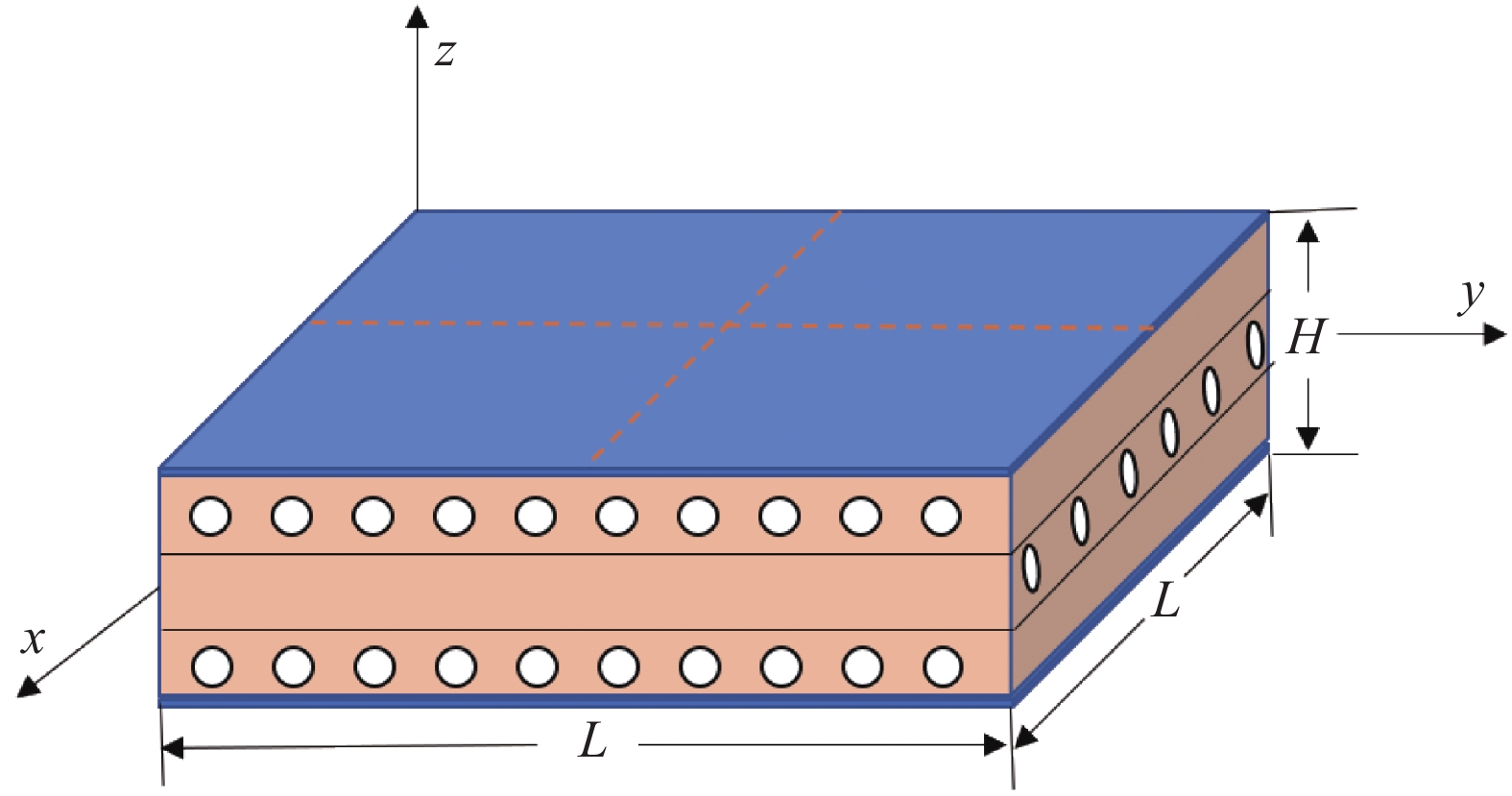
表 1 工况1下PZT-4压电层合板的各个物理参数值

Table 1 Each physical parameter value of PZT-4 piezoelectric laminates under working condition 1

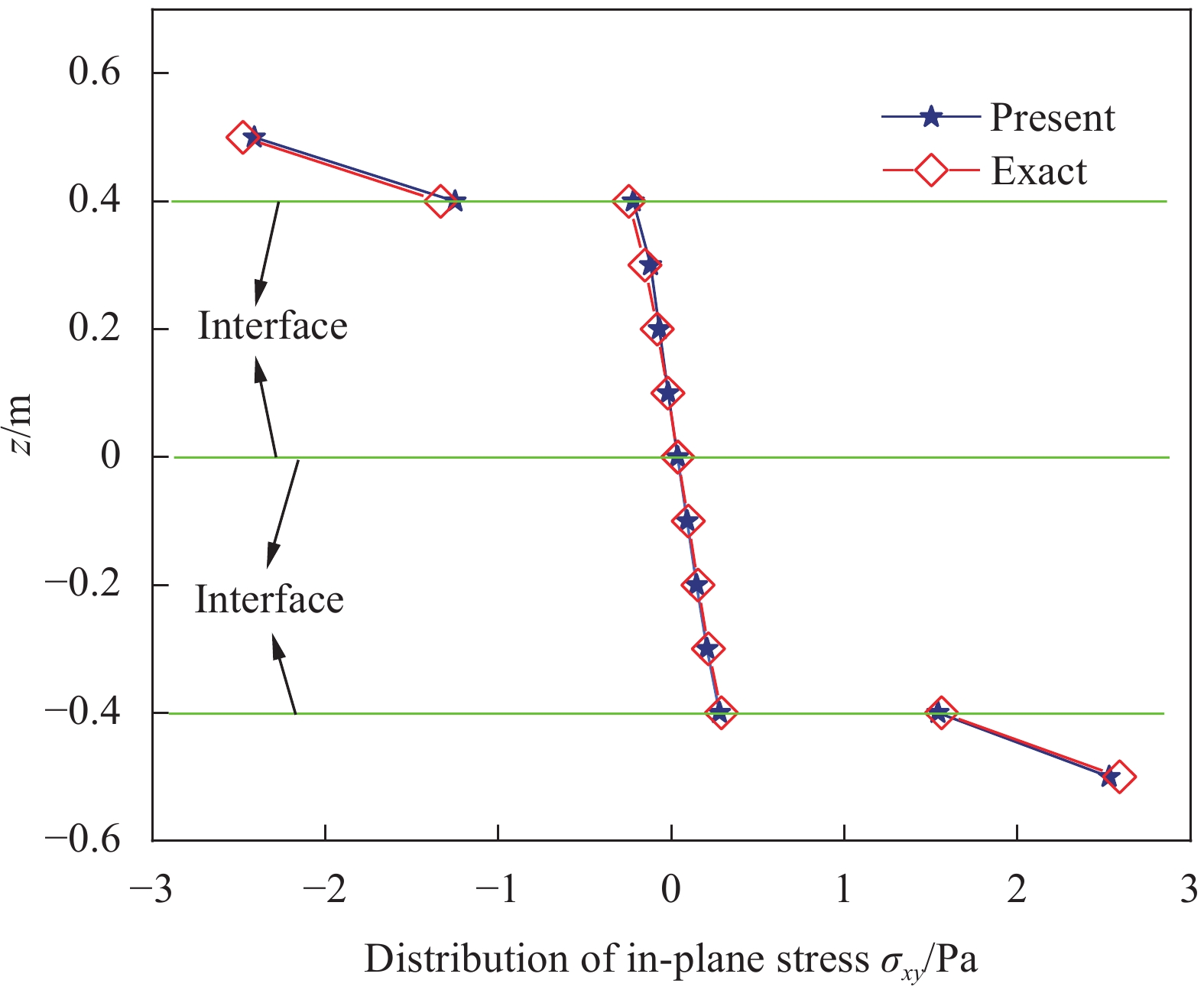
Reference solution	${}u\left({0,}\dfrac{L}{{2}}{,0}\right)$ ${\Bigr/1}{{0}}^{{-11}}\text{m}$	$\text{}w\left(\dfrac L{{2}}{,}\dfrac L{{2}}{,0}\right)$ ${\Bigr/}{1}{{0}}^{{-11}}\text{m}$	${\phi }\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right)$ ${\Bigr/}{1}{{0}}^{{-2}}\text{V}$	${\sigma }_{xz}\left({0,}\dfrac{L}{{2}}{,0}\right)\Bigr/{\rm{Pa}}$	${\sigma }_{x}\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right)\Bigr/{\rm{Pa}}$	${\sigma }_{z}\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right) \Bigr/{\rm{Pa}}$
Exact^[23]	20.392	30.027	0.611	—	6.5643	0.498
LW1^[24]	—	29.851	0.6032	0.7099	7.0132	0.579
Present	20.287	29.82	0.6065	0.691	6.46	0.497
Error/%	0.5	0.6	0.7	—	1.5	0.13
Notes: u—Longitudinal displacement; $w$ —Transverse displacement; $\phi$ —Electric potential; σ_z, σ_xz—Interlaminar stress; σ_x—In-plane stress.

下载: 导出CSV

表 2 工况2下PZT-4压电层合板的各个物理参数值

Table 2 Each physical parameter value of PZT-4 piezoelectric laminates under working condition 2

Reference solution	$w \left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0} }^{\rm{-11} }\rm{m}$	$\phi\left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-2}}\rm{V}$	${\sigma _{xz}} \left( {0,\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-3}}\rm{Pa}$	${\sigma _{z}} \left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-3}}\rm{Pa}$
Exact^[23]	−1.471	0.4476	−23.87	−14.612
LW3^[24]	−1.4707	0.4477	−22.70	−13.541
Present	−1.493	0.4475	−24.5	−15.469
Error/%	1.47	0.7	0.64	5.6

下载: 导出CSV

表 3 PVDF压电层合板的挠度和应力

Table 3 Deflection and stress of PVDF piezoelectric laminates

Load	L/H	Reference solution	$\tilde w\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,0}\right)$	${\tilde \sigma _x}\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,}\dfrac{h}{\text{2}}\right)$	${\tilde \sigma _y}\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,}\dfrac{h}{\text{6}}\right)$	${\tilde \tau _{xy}}\left(\text{0,0,}\dfrac{h}{\text{2}}\right)$	${\tilde \tau _{xz}} \left(\text{0,}\dfrac{L}{\text{2}}\text{,0}\right)$	${\tilde \tau _{yz}}\left(\dfrac{L}{\text{2}}\text{,0,0}\right)$
${p_0} = 1.0$ ${V_0} = 1.0$	10	Present	0.766	0.589	0.285	−0.0277	0.359	0.113
		Literature^[27]	0.764	0.502	0.290	−0.0269	0.371	0.137
		Literature^[5]	0.668	0.520	−	−	−	−
		Literature^[25]	0.774	0.589	0.284	−0.0287	0.358	0.123
	100	Present	0.445	0.540	0.180	−0.020	0.395	0.076
		Literature^[27]	0.434	0.539	0.181	−0.0212	0.390	0.076
		Literature^[5]	0.433	0.545	−	−	−	−
		Literature^[25]	0.471	0.538	0.181	−0.021	0.394	0.083
${p_0} = 1.0$ ${V_0} = 100$	10	Present	−2.0	−2.94	−2.16	0.210	−0.593	0.348
		Literature^[27]	−2.0	−2.73	−2.45	0.149	−0.710	0.450
		Literature^[5]	−1.91	−2.78	−	−	−	−
		Literature^[25]	−2.35	−3.12	−2.34	0.181	−0.683	0.336
	100	Present	0.424	0.489	0.084	−0.0192	0.384	0.081
		Literature^[27]	0.412	0.505	0.159	−0.0198	0.378	0.080
		Literature^[5]	0.411	0.510	−	−	−	−
		Literature^[25]	0.447	0.504	−0.158	−0.019	0.382	0.086
Notes: $\tilde w$ —Dimensionless transverse displacement; ${\tilde \sigma _x}$ , ${\tilde \sigma _y}$ , ${\tilde \tau _{xy}}$ —Dimensionless in-plane stress; ${\tilde \tau _{xz}}$ , ${\tilde \tau _{yz}}$ —Dimensionless interlaminar stress; $p_0$ —Peak mechanical load; V₀—Peak electric potential.

下载: 导出CSV

参考文献(27)

[1]	QING G H, MAO J, LIU Y. Generalized mixed finite element method for 3D elasticity problems[J]. Acta Mechanica Sinica,2018,34(2):1-10.
[2]	BENJEDDOU A. Advances in piezoelectric finite element modeling of adaptive structural elements: A survey[J]. Computers & Structures,2000,76(1-3):347-363.
[3]	REDDY J N. On laminated composite plates with integrated sensors and actuators[J]. Engineering Structures,1999,21(7):568-593. DOI: 10.1016/S0141-0296(97)00212-5
[4]	DETWILER D T, SHEN M H H, VENKAYYA V B. Finite element analysis of laminated composite structures containing distributed piezoelectric actuators and sensors[J]. Finite Elements in Analysis & Design,1995,20(2):87-100.
[5]	SHEIKH A H, TOPDAR P, HALDER S. An appropriate FE model for through thickness variation of displacement and potential in thin moderately thick smart laminates[J]. Composite Structures,2001,51:401-409. DOI: 10.1016/S0263-8223(00)00156-2
[6]	CARRERA E, BOSCOLO M. Classical and mixed finite elements for static and dynamic analysis of piezoelectric plates[J]. International Journal for Numerical Methods in Engineering,2010,70(10):1135-1181.
[7]	CARRERA E, BUETTNER A, NALI P. Mixed elements for the analysis of anisotropic multilayered piezoelectric plates[J]. Journal of Intelligent Material Systems & Structures,2010,21(7):701-717.
[8]	VIDAL P, GALLIMARD L, POLIT O. Modeling of piezoelectric plates with variables separation for static analysis[J]. Smart Materials and Structures,2016,25(5):055043.
[9]	PLAGIANAKOS T S, PAPADOPOULOS E G. Higher-order 2-D/3-D layerwise mechanics and finite elements for composite and sandwich composite plates with piezoelectric layers[J]. Aerospaceence & Technology,2015,40:150-163.
[10]	KORAYEM M H, HOMAYOONI A. The size-dependent analysis of multilayer micro-cantilever plate with piezoelectric layer incorporated voltage effect based on a modified couple stress theory[J]. European Journal of Mechanics-A/Solids,2017,61:59-72.
[11]	KPEKY, FESSAL, ABED-MERAIM, et al. Linear and quadratic solid-shell finite elements SHB8PSE and SHB20E for the modeling of piezoelectric sandwich structures[J]. Mechanics of Advanced Materials and Structures,2018,25(1-8):559-578.
[12]	MOLEIRO F, SOARES C, SOARES C, et al. Layerwise mixed models for analysis of multilayered piezoelectric composite plates using least-squares formulation[J]. Composite Structures,2015,119:134-149.
[13]	ZENKOUR A M, ALGHANMI R A. Stress analysis of a functionally graded plate integrated with piezoelectric faces via a four-unknown shear deformation theory[J]. Results in Physics,2018,12:268-277.
[14]	RAMEGOWDA P C, D ISHIHARA, NIHO T, et al. A novel coupling algorithm for the electric field-structure interaction using a transformation method between solid and shell elements in a thin piezoelectric bimorph plate analysis[J]. Finite Elements in Analysis and Design,2019,159:33-49.
[15]	LI W, SHEN H J. A layerwise finite element formulation of laminated composite cylindrical shells with piezoelectric layers[J]. Journal of Mechanical Science & Technology,2018,32(2):731-741.
[16]	KAPURIA S, AHMED A. A coupled efficient layerwise finite element model for free vibration analysis of smart piezo-bonded laminated shells featuring delaminations and transducer debonding[J]. International Journal of Mechanical Sciences,2021,194:106195. DOI: 10.1016/j.ijmecsci.2020.106195
[17]	刘艳红, 李锐. 含参数辛元与热弹性复合材料层合板分析[J]. 复合材料学报, 2019, 36(5):1306-1312. LIU Yanhong, LI Rui. Parametered symplectic element and analysis of thermoelastic composite laminates[J]. Acta Materiae Composite Sinica,2019,36(5):1306-1312(in Chinese).
[18]	赵直钦. 三类弹性体非协调辛有限元的理论研究[D]. 天津: 中国民航大学, 2020. ZHAO Zhiqin. Theoretical study on noncompatiable symplectic finite element of three elastomers[D]. Tianjin: Civil Aviation University of China, 2020(in Chinese).
[19]	PIAN T, WU C C. Hybrid and incompatible finite element methods[M]. New York: Chapman & Hall/CRC, 2006.
[20]	FELIPPA C A. Parametrized multifield variational principles in elasticity: I. Mixed functionals[J]. Communications in Applied Numerical Methods,2010,5(2):79-88.
[21]	FELIPPA C A. Parametrized multifield variational principles in elasticity: II. Hybrid functionals and the free formulation[J]. Communications in Numerical Methods in Engineering,2010,5(2):89-98.
[22]	沈观林, 胡更开, 刘彬. 复合材料力学[M]. 2版. 北京: 清华大学出版社, 2013. SHEN Guanlin, HU Gengkai, LIU Bin. Mechanics of composite materials[M]. 2th ed. Beijing: Tsinghua University Press, 2013(in Chinese).
[23]	HEYLIGER P. Static behavior of laminated elastic/piezoelectric plates[J]. AIAA Journal,1994,32(12):2481-2484. DOI: 10.2514/3.12321
[24]	CARRERA E, VALVANO S, KULIKOV G M. Multilayered plate elements with node-dependent kinematics for electro-mechanical problems[J]. International Journal of Smart and Nano Materials,2018,9(4):279-317. DOI: 10.1080/19475411.2017.1376722
[25]	RAY M C, BHATTACHARYA R, SAMANTA B. Exact solutions for static analysis of intelligent structures[J]. AIAA Journal,2012,31(9):1684-1691.
[26]	PAGANO N J, HATFIELD H J. Elastic behavior of multilayered bidirectional composites[J]. AIAA Journal,1972,10(7):931-933. DOI: 10.2514/3.50249
[27]	CEN S, SOH A K, LONG Y Q, et al. A new 4-node quadrilateral FE model with variable electrical degrees of freedom for the analysis of piezoelectric laminated composite plates[J]. Composite Structures,2002,58(4):583-599. DOI: 10.1016/S0263-8223(02)00167-8

施引文献(19)

期刊类型引用(12)

1.	张惠一，蒲云东，张逸舟，苏俊儒，袁小亚，胡兵兵. 氧化石墨烯助分散的碳纤维对水泥砂浆强度及电热性能的影响. 化工新型材料. 2025(03): 272-280 . 百度学术
2.	郑寒，董玉文，王宗波. 渗透结晶型防水剂对混凝土抗冻性能的影响. 水力发电. 2025(04): 104-110 . 百度学术
3.	曹济，陈晓庭，张文涛，孙绿林，宋永胜. 活性剂增强改性水泥基渗透结晶型防水材料抗渗试验. 粘接. 2024(02): 104-107 . 百度学术
4.	桂尊曜，蒲云东，张惠一，袁小亚，黎少伟，邵伟升. 石墨烯量子点对水泥砂浆流动度、强度和耐盐腐蚀性的影响. 复合材料学报. 2024(04): 2043-2054 . 本站查看
5.	李大芳，郭鑫，鲁义，施式亮，李贺. 煤层瓦斯抽采钻孔封孔材料研究进展与展望. 安全. 2024(05): 44-51 . 百度学术
6.	王黎，陶文彬. 反渗透自粘防水卷材对高层医院建筑工程的应用研究. 粘接. 2024(09): 27-29+40 . 百度学术
7.	陈宾，袁洋，胡杰铭，张召，张涛. 冲击荷载下氧化石墨烯改性珊瑚砂浆破碎分形特征研究. 兵器装备工程学报. 2024(10): 25-33 . 百度学术
8.	程文通，秦拥军，李东锦. 渗透结晶型防水材料活性母料制备与优化试验. 科学技术与工程. 2024(29): 12684-12690 . 百度学术
9.	陈韫钒. 活性剂增强改性水泥基渗透结晶型防水材料抗渗试验研究. 江西建材. 2024(08): 41-43 . 百度学术
10.	张惠一，桂尊曜，蒲云东，齐孟，曹蔚琦，袁小亚. 羟基化石墨烯对水泥基渗透结晶型防水材料力学性能的影响. 硅酸盐通报. 2023(05): 1569-1577+1588 . 百度学术
11.	陈宾，张涛，张召，袁洋，卢艺伟. 冲击荷载作用下氧化石墨烯改性珊瑚砂浆的动态力学特性与微观机制. 复合材料学报. 2023(08): 4682-4693 . 本站查看
12.	陈妤，董凯，张文杰，李国浩，李创创，唐丽. 干湿交替氯盐环境下氧化石墨烯水泥砂浆抗侵蚀研究. 硅酸盐通报. 2022(12): 4147-4153 . 百度学术