Numerical investigation on dynamic response of bio-inspired bi-directional corrugated lattice structure under impact loading
-
摘要:
为了探究仿生双正弦波纹点阵结构(Bio-inspired bi-directional corrugated lattice structure，BBCLS)的抗冲击性能，采用ANSYS/LSDYNA有限元分析软件建立了其在冲击荷载作用下的有限元数值模型，并基于已有的试验结果与数值模拟结果进行了对比，验证了该模型的有效性。在此基础上，研究了不同冲击速度对BBCLS的应力分布、变形模式、承载能力以及能量吸收特性的影响，并与传统体心立方点阵结构(BCC)进行了对比。同时利用该数值模型进一步分析了振幅、波纹数和胞壁厚度等微结构几何参数对BBCLS抗冲击性能的影响。研究结果表明：BBCLS在冲击荷载作用下的承载能力、吸能总量及比吸能均明显优于传统的BCC点阵结构。BBCLS的冲击动力学响应主要与冲击速度和微结构几何参数有关。在低速冲击时，BBCLS呈现整体变形模式；中高速冲击时，结构向局部变形模式转换。随着冲击速度的提高，增大振幅、波纹数、胞壁厚度均使结构在受到冲击载荷时应力分布均匀，有效增加了冲击端的平台应力。此外，微结构几何参数的改变对结构比吸能以及综合比吸能有显著影响。由于波纹数的增大，BBCLS的承载能力、刚度和吸能性均大幅度提高，当波纹数为8，冲击速度达到100 m/s，相比于波纹数为5，冲击速度为10 m/s比吸能提高201.36%。研究结果为研究仿生点阵结构的冲击变形失效和吸能效果提供了力学依据。
Abstract:In order to explore the impact resistance of bio-inspired bi-directional corrugated lattice structure (BBCLS), ANSYS/LSDYNA finite element analysis software was used to establish the finite element numerical model under the impact load, and the existing test results were compared with the numerical simulation results to verify the effectiveness of the model. On this basis, the effects of different impact velocities on the stress distribution, deformation mode, bearing capacity and energy absorption characteristics of BBCLS were studied, and compared with the traditional body-centered cubic lattice structure (BCC). The effects of the geometric parameters such as amplitude, ripple number and cell wall thickness on the impact resistance of BBCLS were further analyzed using the numerical model. The results show that the carrying capacity, total energy absorption and specific energy of BBCLS under impact load are obviously superior to the traditional BCC lattice structure. The impact dynamic response of BBCLS is mainly related to impact velocity and microstructure geometry parameters. At low speed impact, BBCLS presents an overall deformation pattern. The structure changes to the local deformation mode during the impact of medium and high speed. With the increase of impact velocity, the increase of amplitude, ripple number and cell wall thickness can make the stress distribution of the structure under impact load uniform, and effectively increase the platform stress at the impact end. In addition, the change of microstructure geometric parameters has a significant effect on the specific absorption energy of the structure and the overall specific absorption energy. As the number of ripples increases, the bearing capacity, stiffness and energy absorption of BBCLS are greatly improved. When the number of ripples is 8, the impact velocity reaches 100 m/s. Compared with the number of ripples, the impact velocity is 10 m/s, which is 201.36% higher than the energy absorption. The results provide a mechanical basis for the study of impact deformation failure and energy absorption effects of bionic lattice structures.
-
城市化及工业化的快速发展极大地促进了水泥制品的产出，随即引发的一系列环境问题，如巨大能源资源消耗、二氧化碳及其他温室气体排放等引起了全世界的强烈担忧[1]。为此，国内外学者做出了巨大的努力来降低其环境负面效应并寻求可替代方案。凭借绿色可持续性[2-3]，碱激发材料(Alkali-activated material，AAM)近年来获得了广泛关注[4-6]。然而，类似于普通混凝土，AAM脆性大及抗拉强度低等缺点从一定程度上限制了该材料的推广应用。
借鉴高延性水泥基复合材料的设计理念，在AAM体系中引入适量纤维，研制出了高延性碱激发纤维增强复合材料(Alkali-activated fiber reinforced composites，AAFRC)；相似地，伴随有多条细密裂缝开裂的应变硬化行为也是AAFRC的显著特征[7-9]。
赤泥(RM，也称铝土矿渣)是精制铝/氧化铝过程中产出的固体废物[10]，每生产1吨氧化铝就会产出1.0~1.5吨的RM[11]。大量RM被随机存储在蓄水库或干堆在田地中，并没有得到有效的再利用[12]，如何开发RM的潜在资源化利用已经成为一个亟需解决的问题。
尽管人们从不同角度对赤泥进行了大量的再利用尝试，包括作为吸收剂、中和剂、混凝剂和催化剂及从中回收铁、铝等有价金属和一些稀土元素[10,13]，且取得了一些进展，但由于一些技术或经济限制[14]，仅在这些领域回收利用如此大量的RM仍是不切实际的。考虑到RM潜在的火山灰效应[15]，土木工程领域的应用有望使其成为可能。近年来相关报道表明，RM已成功被用于制造水泥、砖和混凝土等建筑材料[16]；且也开展了以RM为原料生产AAM的研究。例如，He等[17]提出了一种基于混合RM和稻壳灰的新型AAM；Ye等[18]利用碱热RM合成了单组分AAM；Hu等[19]在低浓度NaOH激发下制备了一种RM-粉煤灰AAM。值得注意的是，现有RM-AAM的强度在大多数情况下无法与普通混凝土相比，即
⩽ 20 MPa [17-19]；而且类似于传统AAM的脆性缺点依然存在，这些弊端阻碍了该材料的实际工程应用。基于此，本文采用RM混合矿渣和硅灰并掺加聚乙烯(PE)纤维制备中高强、高延性的碱激发复合材料，通过单轴拉、压试验探究其宏观力学性能，结合三点抗弯与单裂缝拉伸等细观试验探究其高延性机制，并基于XRD及FTIR技术分析其水化产物，以期为RM的高附加值资源化利用提供新思路。
1. 试验材料及方法
1.1 原材料
赤泥(RM，山东魏桥创业集团有限公司)、矿渣(GGBS，河北天岩矿业公司)、硅灰(SF，山东温特实业有限公司)、普通河砂、自来水、碱激发剂及PE纤维(浙江全米特新材料科技有限公司)。其中，RM、GGBS及SF的主要化学组成见表1，其粒径分布见图1；碱激发剂由模数为3.3的水玻璃(山东优索化工有限公司)及99%的NaOH颗粒(国药集团化学药剂有限公司)配制而成；PE纤维性能参数如下：直径24 μm，长度12 mm，密度980 kg/m3，弹性模量110 GPa，抗拉强度3000 MPa，伸长率2%~3%。
表 1 赤泥(RM)、矿渣(GGBS)及硅灰(SF)的化学组成Table 1. Chemical compositions of red mud (RM), ground granulated blast furnace slag (GGBS) and silica fume (SF)wt% Material CaO SiO2 Al2O3 Fe2O3 MgO SO3 TiO2 Na2O K2O P2O5 RM 0.46 10.18 17.43 53.79 0.12 0.76 – 7.74 0.07 0.20 GGBS 44.39 33.20 13.20 0.38 6.31 – 0.82 0.33 0.28 – SF 0.49 92.26 0.89 1.97 0.96 – – 0.42 1.31 – 1.2 配合比及试件制备
基于前期预试验(0wt%~60wt%RM取代GGBS和SF)结果，选用40wt%RM掺量开展本研究。试件的具体配合比见表2，水胶比为0.39，PE/GGBS-SF为不含RM的对照组。
表 2 高延性碱激发纤维增强复合材料(AAFRC)配合比Table 2. Mixture proportion of alkali-activated fiber reinforced composites (AAFRC)Mixture RM/wt% SF/wt% GGBS/wt% Sand/wt% NaOH/wt% Na2SiO3/wt% Water/wt% Polyethylene
(PE) fiber/vol%PE/GGBS-SF 0.00 10.33 44.77 14.50 3.00 13.70 12.60 1.90 PE/RM-GGBS-SF 22.04 6.20 26.86 14.50 3.00 13.70 12.60 1.90 试件制备过程：将RM、GGBS、SF及砂按表2中配比倒入容量为5 L的水泥胶砂搅拌机慢速搅拌2~4 min，使原料混合均匀；将水和碱激发剂(Na2SiO3和NaOH提前配制冷却至室温)倒入，快速搅拌3~5 min直至浆体具有一定流动度；加入PE纤维，快速搅拌5~8 min，使纤维均匀分散。搅拌完毕后，立即将拌合物装入模具振捣密实，放置于实验室环境(温度为20℃~22℃、相对湿度为70%±5%)中养护24 h后脱模；试块表面覆盖保鲜膜，放置于80℃的烘箱中加热2 h后继续在室温中养护至28天。
1.3 试验方法
1.3.1 单轴拉伸及抗压试验
单轴拉伸试件为“狗骨状”[20]，中间标准段长度为80 mm，具体尺寸详见图2。采用济南川佰仪器设备有限公司生产的WDW-300型电子万能试验机，加载速度为0.3 mm/min。试验时，试件两边分别固定一套位移传感器(Linear variable displacement transducers，LVDT)记录标准段长度的变化，取两个测量结果的平均值来计算拉伸应变。
参考ASTM C109[21]，选用边长为50 mm的立方体试件进行抗压试验，加载速率为1.5 mm/min。注意保证试件承压表面的平整度，以最大程度地减少测试过程中的不确定性。
1.3.2 三点抗弯及单裂缝拉伸试验
为探究基体韧度对AAFRC拉伸延性的影响，根据ASTM E399[22]进行三点抗弯试验。首先依照表2配合比制作尺寸为354 mm×75 mm×40 mm的无纤维棱柱体试件，然后采用1.2中养护方法将试件养护至28天；试验前，用切割机在试件的中部底端切出一个30 mm深的切口(图3)，试验时试件底部跨径为300 mm，加载速率为0.3 mm/min。
通过单裂缝拉伸试验[23]，获得纤维最大桥接应力σoc和纤维桥接余能Jb′。试件形状为“小狗骨”(图4)；养护至28天后，在试件中心分别沿宽度、厚度方向切割6.5 mm、2 mm深的凹槽(宽度小于0.6 mm)，以便形成单裂缝。
1.3.3 水化产物分析
采用D8 Advance Bruker型XRD测试仪(赛默飞世尔(中国)科技有限公司)与Tensor-Bruker型光谱仪(德国布鲁克AXS有限公司)分析PE/RM-GGBS-SF的水化产物。为了避免砂和纤维的影响，根据表2配制不含砂和纤维的净浆试件，养护至28天后磨成粉末用于微观分析。
2. 结果与讨论
2.1 两种AAFRC拉伸性能
两种AAFRC的典型拉伸应力-应变曲线如图5所示。初裂强度(初始裂缝的拉伸应力)、抗拉强度(极限拉伸应力)及拉伸应变(极限拉伸应力对应的应变)详见表3。
可以看出，RM的引入导致AAFRC的初裂、抗拉强度均有所下降。与参照试件(PE/GGBS-SF)相比，PE/RM-GGBS-SF的初裂强度降低了12.1%，为(1.89±0.41) MPa；抗拉强度降低了26.4%，为(2.43±0.04) MPa。在碱激发体系中，CaO与SiO2是生成胶凝产物、贡献强度的重要组分。由表1可知，RM中CaO与SiO2的含量明显低于GGBS和SF (即CaO：0.46wt% vs 44.39wt%+0.49wt%，SiO2：10.18wt% vs 33.20wt%+92.26wt%)；且RM中的SiO2主要以石英相的形式存在[16]。RM的掺入会明显减少体系内Ca、Si含量，从而减少水化凝胶产物的生成，导致强度降低。同时，由图1可知，绝大多数RM的粒径大于GGBS和SF，RM的引入可能会降低基体的密实度，从而对强度不利。另一方面，RM中包含较多的碱金属(Na2O+K2O=7.74%+0.07%)，导致复合材料体系内的碱性得以提升，有利于强度的发展[24]。当前试验结果可认为由上述机制共同作用产生，强度的降低则说明，本研究中反应组分减少及RM较大粒径带来的不利影响可能占主导地位。
表 3 AAFRC的拉伸性能Table 3. Tensile properties of AAFRCMixture Initial cracking strength/MPa Tensile strength/MPa Tensile strain/% PE/GGBS-SF 2.15±0.13 3.30±0.13 3.07±0.32 PE/RM-GGBS-SF 1.89±0.41 2.43±0.04 3.58±0.11 对比二者拉伸应变发现，RM的引入提高了AAFRC的拉伸延性；相比参照试件，PE/RM-GGBS-SF的拉伸应变增加了16.6%，高达3.5%。纤维增强脆性基体复合材料的拉伸延性受基体初裂强度的影响，通常情况下，初裂强度越低，获得较大应变的可能性越大[23]。仅从这点来看，当前的应变结果可以通过基体初裂强度的变化合理地得以解释。不过，具体的应变机制比较复杂，更多的参数(包括基体、纤维、基体-纤维界面特性等)都影响着试件的拉伸行为，进一步的讨论将在2.3节(基于细观试验[25])给出。
2.2 两种AAFRC抗压强度
表4为两种AAFRC的抗压强度，其中PE/GGBS-SF抗压强度为(69.13±2.16) MPa，PE/RM-GGBS-SF抗压强度为(48.83±1.86) MPa。掺入RM后，抗压强度降低了29.4%。抗压强度与初裂、抗拉强度的变化相似，表明上述强度变化机制可能也同样适用于抗压强度。也即，反应组分减少导致胶凝产物含量降低及较大粒径RM引入导致基体的不致密，可能也是抗压强度减少的主要原因。总体来看，尽管RM的引入使AAFRC的强度有所下降，但仍处于较高水平，近50 MPa的抗压强度可满足大部分工程的使用要求；且拉伸应变水平与高延性水泥基复合材料近乎相当[26]。因此，可以认为RM制备高延性碱激发复合材料具有一定的可行性，这为实现其大规模、高附加值的资源化利用提供了新的思路。
表 4 AAFRC抗压强度Table 4. Compressive strength of AAFRCMixture Compressive strength/MPa PE/GGBS-SF 69.13±2.16 PE/RM-GGBS-SF 48.83±1.86 2.3 AAFRC高延性机制
纤维增强脆性基体复合材料产生应变硬化行为需要满足两个核心准则[23]：(1) 强度准则：基体初始开裂强度σcr必须小于纤维最大桥接应力σoc；(2) 能量准则：基体断裂能Jtip不得超过纤维桥接余能Jb′。此外，两个应变硬化性能指数PSHS(强度指数，σoc/σcr)和PSHE(能量指数，Jb′/Jtip) 可用来评估复合材料的应变硬化潜力，饱和应变硬化行为的实现需要满足两个更严格的条件：(1) PSHS
\geqslant 1.20~1.35；(2) PSHE\geqslant 2.7~3.0[27-28]。理论上，PSH指标越大，越容易获取较高的拉伸延性[25]。基于三点抗弯试验得到的峰值荷载FQ，按照以下各式可计算出基体断裂韧度Km和基体断裂能Jtip：
{K}_{\mathrm{m}}=\frac{1.5\left({F}_{\mathrm{Q}}+\dfrac{mg}{2} \times{10}^{-3}\right)\times {10}^{-3} S {{a}_{0}}^{0.5}}{t{h}^{2}}f \left(\alpha \right) (1) f \left(\alpha \right)=\frac{1.99-\alpha \left(1-\alpha \right)\left(2.15-3.93\alpha +2.7{\alpha }^{2}\right)}{\left(1+2\alpha \right){\left(1-\alpha \right)}^{\frac{3}{2}}} (2) \alpha ={a}_{0}/h (3) {J}_{\mathrm{t}\mathrm{i}\mathrm{p}}=\frac{{{K}_{\mathrm{m}}}^{2}}{{E}_{\mathrm{m}}} (4) 式中：m为试件质量；S为试件跨度；a0为切槽深度；t和h分别为试件宽度和高度；Em为拉伸试验获得的弹性模量；f(α)为形状参数；g=9.8 N/kg。
结果详见表5。可以看出，相比于对照试件，PE/RM-GGBS-SF的Km表现出下降趋势，与基体初裂强度的变化相一致；基体断裂能Jtip也从(5.05±0.23) J·m−2减小至(4.28±0.75) J·m−2，较小的Jtip有利于获得较大的
{J}_{\mathrm{b}}{{{'}}}/{J}_{\mathrm{tip}} ，这从一定程度上反映了PE/RM-GGBS-SF获取较高拉伸应变(延性)的潜能，或者认为RM的掺入使基体趋于多缝开裂：{J}_{\mathrm{b}}{{{'}}}={\sigma }_{\mathrm{o}\mathrm{c}}{\delta }_{\mathrm{o}\mathrm{c}}-{\int }_{0}^{{\delta }_{\mathrm{o}\mathrm{c}}}\sigma \left(\delta \right)\mathrm{d}\delta (5) 式中：σoc和δoc分别为纤维最大桥接应力与对应的裂缝开口位移；σ(δ)为纤维桥接应力。
表 5 AAFRC的基体断裂韧度与基体断裂能Table 5. Matrix fracture toughness and fracture energy of AAFRCMixture Mass m/kg Peak load FQ/kN Km/(MPa·m1/2) Jtip/(J·m−2) PE/GGBS-SF 1.99±0.06 0.27±0.03 0.30±0.05 5.05±0.23 PE/RM-GGBS-SF 2.03±0.05 0.23±0.04 0.26±0.07 4.28±0.75 Notes: Km—Fracture toughness of matrix; Jtip—Fracture energy of matrix. 单裂缝拉伸试验得到应力-裂缝开口位移曲线如图6所示，由此可得到纤维最大桥接应力σoc和相应的裂缝开口位移δoc，进而根据式(5)可计算出纤维桥接余能
{J}_{\mathrm{b}}{{{'}}} 。结合已经得到的基体初裂强度σcr和基体断裂能Jtip，即可计算出PSHS及PSHE性能指数，结果如表6所示。![]()
图 6 AAFRC的单裂缝拉伸应力-开口位移曲线Figure 6. Stress-crack opening displacement curves of AAFRCfrom single crack tensile tests表 6 AAFRC单裂缝拉伸试验结果与强度指数PSHS及能量指数PSHETable 6. Results from single crack tensile test and strength index PSHS and energy index PSHE of AAFRCMixture Peak stress σoc/MPa Crack opening δoc/mm Jb′/(J·m−2) PSHS PSHE PE/GGBS-SF 3.14±0.05 0.24±0.04 50.23±37.33 1.47±0.11 10.30±7.86 PE/RM-GGBS-SF 3.03±0.09 0.20±0.07 63.58±24.83 1.72±0.39 16.37±8.67 Notes: Jb′—Complementary energy of fibers. 由表6可看出，本研究中两种AAFRC的PSH指数均大于1 (即σoc>σcr，且Jb′>Jtip)，表明产生应变硬化行为的两个核心准则均得到满足，这合理地解释了两种试件表现出明显应变硬化行为(图5)的原因。进一步注意到，两种试件的PSH指数均满足饱和应变硬化条件：PE/GGBS-SF的PSHS指数略大于1.30，PSHE指数远大于3.0；PE/RM-GGBS-SF的两个性能指数均明显大于各自建议值。基于此，两种试件获得较高拉伸应变性能(3%以上)的结果得到了较好的印证。此外，对比PE/GGBS-SF 与PE/RM-GGBS-SF拉伸应变与PSH指数之间的关系，发现随着RM引入后拉伸应变的增加，PSHS和PSHE指数均表现出增加趋势。这从一定程度上说明，使用PSH指数理解本研究中碱激发复合材料体系的拉伸延性行为是可行的。
2.4 AAFRC的水化产物
图7(a)、7(b)分别为矿粉、RM及两种AAFRC试样的XRD图谱。可以看出，矿粉的主要衍射峰为白云石相(CaMg(CO3)2)，RM则为赤铁矿(Fe2O3)和针铁矿(FeO(OH))相。随着碱激发反应的进行，两种AAFRC体系内均未出现白云石相；而PE/RM-GGBS-SF的XRD衍射峰则含有明显的赤铁矿和针铁矿相，这主要与RM的引入密切相关[22]。而且，RM及PE/RM-GGBS-SF的XRD图谱中均出现了较多的赤铁矿衍射峰，这也很好地对应了RM化学组分中较高的Fe2O3含量(表1)。值得注意，两种AAFRC试样的XRD图谱均在20°~36°之间出现了明显的隆起，这是无定形(或类凝胶状)地聚合反应产物的典型衍射模式[29]。即碱激发体系内生成了与地聚合反应产物相似的胶凝物相。不过，引入RM后，隆起部位趋于平缓，这从一定程度上说明了PE/RM-GGBS-SF体系中胶凝产物量的减少，很好地对应了宏观力学强度降低的试验结果。
![]()
图 7 RM和GGBS (a) 及AAFRC (b) 的XRD图谱Figure 7. XRD patterns of RM, GGBS (a) and AAFRC (b)对比图8两种AAFRC的FTIR图谱，发现两种试样的波带起伏状态基本保持一致、不同特征波峰出现的位置没有明显差异，这说明RM的掺入并没有导致新物质的产生。3415 cm−1与1630 cm−1处的波峰分别与(H)—OH基团的伸缩振动、弯曲振动有关[30]，(H)—OH基团可能源自于样品表面的物理吸附水[29]，也可能来自于反应产物中的化学结合水[31]。这两个波峰峰强均比参照试样弱，结合XRD图谱分析结果，可以认为波峰减弱的主要原因为水化产物的减少。1402 cm−1与875 cm−1处的波峰分别对应CO32−基团中C—O—C键的不对称伸缩[31]与平面外弯曲振动[32]，说明两种材料体系内均发生了碳化。根据Yu等[32]和Zhang等[33]的研究，1035 cm−1处波峰的出现归因于Si—O—Al/Si键的不对称伸缩振动，表明体系内有铝硅酸盐无定型产物，例如水化硅铝酸钙(C-A-S-H)及碱性硅铝酸盐(N-A-S-H)凝胶。465 cm−1处的波峰与Si—O—Si键的弯曲振动有关，与其相邻且在较高波数位置(近600 cm−1)波峰的出现则意味着体系内存在有水化硅酸钙(C-S-H)或C-A-S-H凝胶[31]。进一步注意到，RM的掺入导致1035 cm−1及600 cm−1处的波峰峰强均有所减弱，这意味着对应胶凝产物的减少，与XRD分析结果相一致。
3. 结 论
(1) 聚乙烯纤维增强赤泥-碱矿渣复合材料(PE/RM-GGBS-SF)的抗拉强度可以达到2.4 MPa，同时具有与高延性水泥基复合材料相当的高拉伸应变性能，为3.5%；抗压强度可以高达近50 MPa。
(2) 三点抗弯和单裂缝拉伸等细观试验结果可以很好地解释本研究中碱激发纤维增强复合材料(AAFRC)的高延性行为。两种体系均满足实现拉伸应变硬化的两个核心(强度及能量)准则；且PE/RM-GGBS-SF试件的强度指数(PSHS)和能量指数(PSHE)均大于参照试件，很好地对应了其表现出的较大拉伸应变。
(3) XRD和FTIR分析结果显示：赤泥(RM)的引入导致碱激发体系内出现明显的针铁矿及赤铁矿衍射峰；除水化硅酸钙(C-S-H)或水化硅铝酸钙(C-A-S-H)凝胶外，PE/RM-GGBS-SF水化产物中也存在有与地聚合反应产物相似的物相，即碱性硅铝酸盐(N-A-S-H)凝胶。
-
![]()
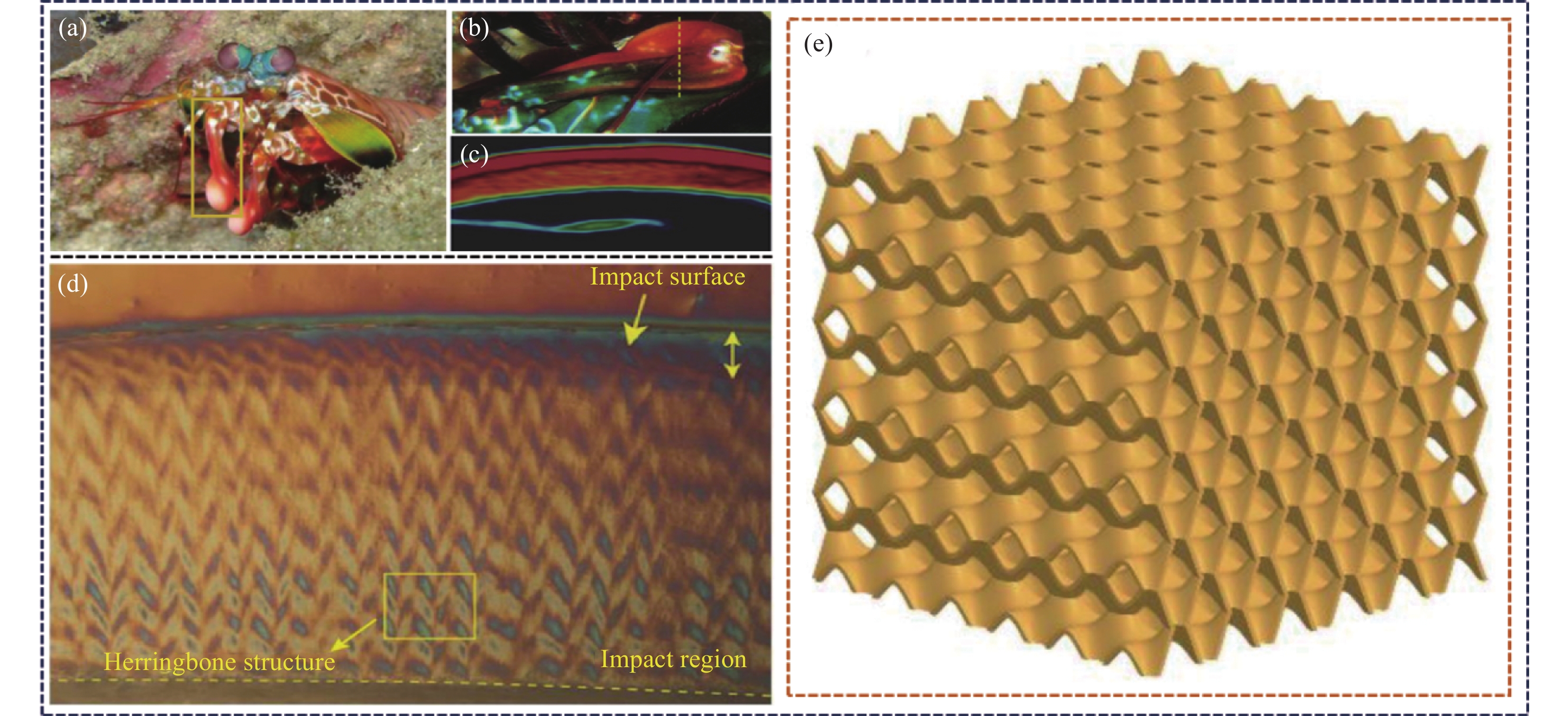
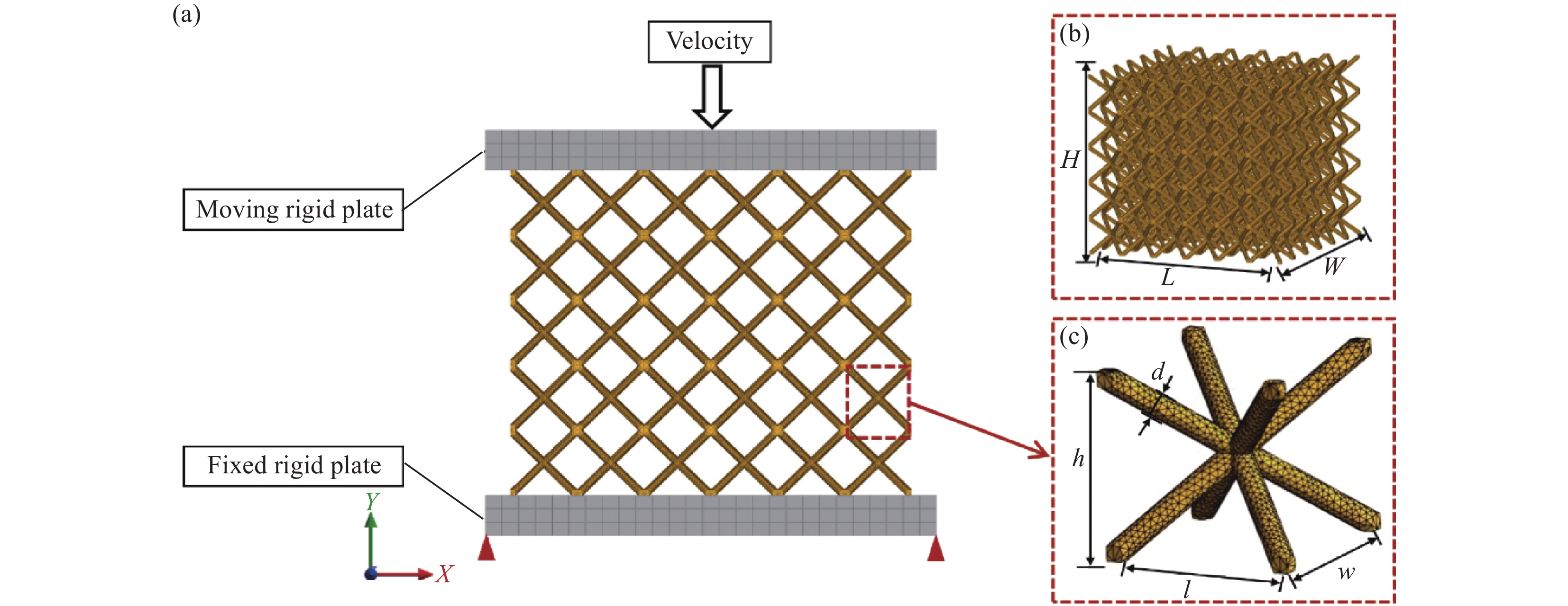
图 1 仿生双正弦波纹点阵结构(BBCLS)示意图：(a)雀尾螳螂虾[25]；(b)虾鳌[27]；(c)抗冲击区域[27]；(d)虾螯抗冲击区域微观结构[27]；(e) BBCLS[32]
Figure 1. Schematic diagram of bio-inspired bi-directional corrugated lattice structure (BBCLS): (a) Odontodactylus scyllarus[25]; (b) Shrimp chela[27];(c) Impact zone[27]; (d) Macro-microstructure of shrimp chela[27]; (e) BBCLS[32]
![]()
图 2 BBCLS有限元模型：(a)准静态压缩荷载下BBCLS计算模型；(b) BBCLS单层模型；(c) BBCLS胞元模型
Figure 2. BBCLS finite element model: (a) Computational model of BBCLS under quasi-static compression loading; (b) BBCLS single-layer model; (c) BBCLS single cell model
A—Amplitude; N—Wave number; t—Cell wall thickness
![]()
图 3 BBCLS试验与模拟破坏形态对比图
Figure 3. Comparison between experimental and simulated failure state of BBCLS
![]()
图 4 BBCLS的力-位移曲线试验与模拟结果对比
Figure 4. Comparison of experimental and simulated force-displacement curves of BBCLS
FEM—Finite element model; EXP—Experiment
![]()
图 5 不同冲击速度下的BBCLS在典型应变水平下的变形图
Figure 5. Deformation diagram of BBCLS under typical strain levels at different impact velocities
![]()
图 6 不同冲击速度下的BBCLS在冲击端和固定端的名义应力-应变曲线
Figure 6. Nominal stress-strain curves on impact end and fixed end of BBCLS under different impact velocities
![]()
图 7 不同冲击速度下的BBCLS能量吸收效率
Figure 7. Energy absorption efficiency of BBCLS at different impact velocities
![]()
图 8 不同冲击速度下的BBCLS平台应力及密实化应变
Figure 8. Plateau stress and densification strain curves of BBCLS at different impact velocities
![]()
图 9 不同冲击速度下BBCLS的比吸能ESEA对比
Figure 9. Comparison of specific energy absorption ESEA of BBCLS at different impact velocities
![]()
图 10 静态荷载与冲击荷载下BBCLS比吸能对比
Figure 10. Comparison of BBCLS ESEA under static load and dynamic load
![]()
图 11 体心立方点阵结构(BCC)有限元模型：(a) 冲击荷载作用下BCC计算模型；(b) BCC三维模型；(c) BCC胞元模型
Figure 11. Body-centered cubic lattice structure (BCC) finite element model: (a) Computational model of BCC under impact load; (b) BCC three-dimensional model; (c) BCC single cell model
H, h—Height; L, l—Length; W, w—Width; d—Diameter
![]()
图 13 两种点阵结构在冲击荷载下的力-位移曲线
Figure 13. Force-displacement curves of two lattice structure under impact loading
![]()
图 14 两种点阵结构在冲击荷载下的能量吸收指标
Figure 14. Energy absorption indicators of two lattice structure under impact loading
![]()
图 15 两种点阵结构的应力云图：(a) BCC；(b) BBCLS
Figure 15. Stress cloud of two lattice structures: (a) BCC; (b) BBCLS
![]()
图 16 不同振幅A下BBCLS固定端的名义应力-应变曲线
Figure 16. Nominal stress-strain curves on fixed end of BBCLS under different amplitudes A
![]()
图 19 不同振幅的BBCLS在0.6应变下的比吸能
Figure 19. ESEA at 0.6 strain of BBCLS under different amplitudes
![]()
图 17 不同振幅下BBCLS冲击端的名义应力-应变曲线
Figure 17. Nominal stress-strain curves on impact end of BBCLS under different amplitudes
![]()
图 18 不同振幅的BBCLS比吸能对比
Figure 18. Comparison of ESEA of BBCLS under different amplitudes
![]()
图 20 不同波纹数N下BBCLS在固定端的名义应力-应变曲线
Figure 20. Nominal stress-strain curves on fixed end of BBCLS under different wave number N
![]()
图 23 不同波纹数的BBCLS在0.6应变下的比吸能
Figure 23. ESEA at 0.6 strain of BBCLS under different wave number
![]()
图 21 不同波纹数下BBCLS在冲击端的名义应力-应变曲线
Figure 21. Nominal stress-strain curves on impact end of BBCLS under different wave number
![]()
图 22 不同波纹数的BBCLS比吸能对比
Figure 22. Comparison of ESEA of BBCLS under different wave number
![]()
图 24 不同厚度t下BBCLS在固定端的名义应力-应变曲线
Figure 24. Nominal stress-strain curves on fixed end of BBCLS under different thicknesses t
![]()
图 27 不同厚度的BBCLS在0.6应变下的比吸能
Figure 27. ESEA at 0.6 strain of BBCLS under different thicknesses
![]()
图 25 不同厚度下BBCLS在冲击端的名义应力-应变曲线
Figure 25. Nominal stress-strain curves on impact end of BBCLS under different thicknesses
![]()
图 26 不同厚度的BBCLS比吸能对比
Figure 26. Comparison of ESEA of BBCLS under different thicknesses
表 1 BBCLS初始峰值力仿真与试验误差对比
Table 1 Comparison between simulation and test error of BBCLS contact force peak value
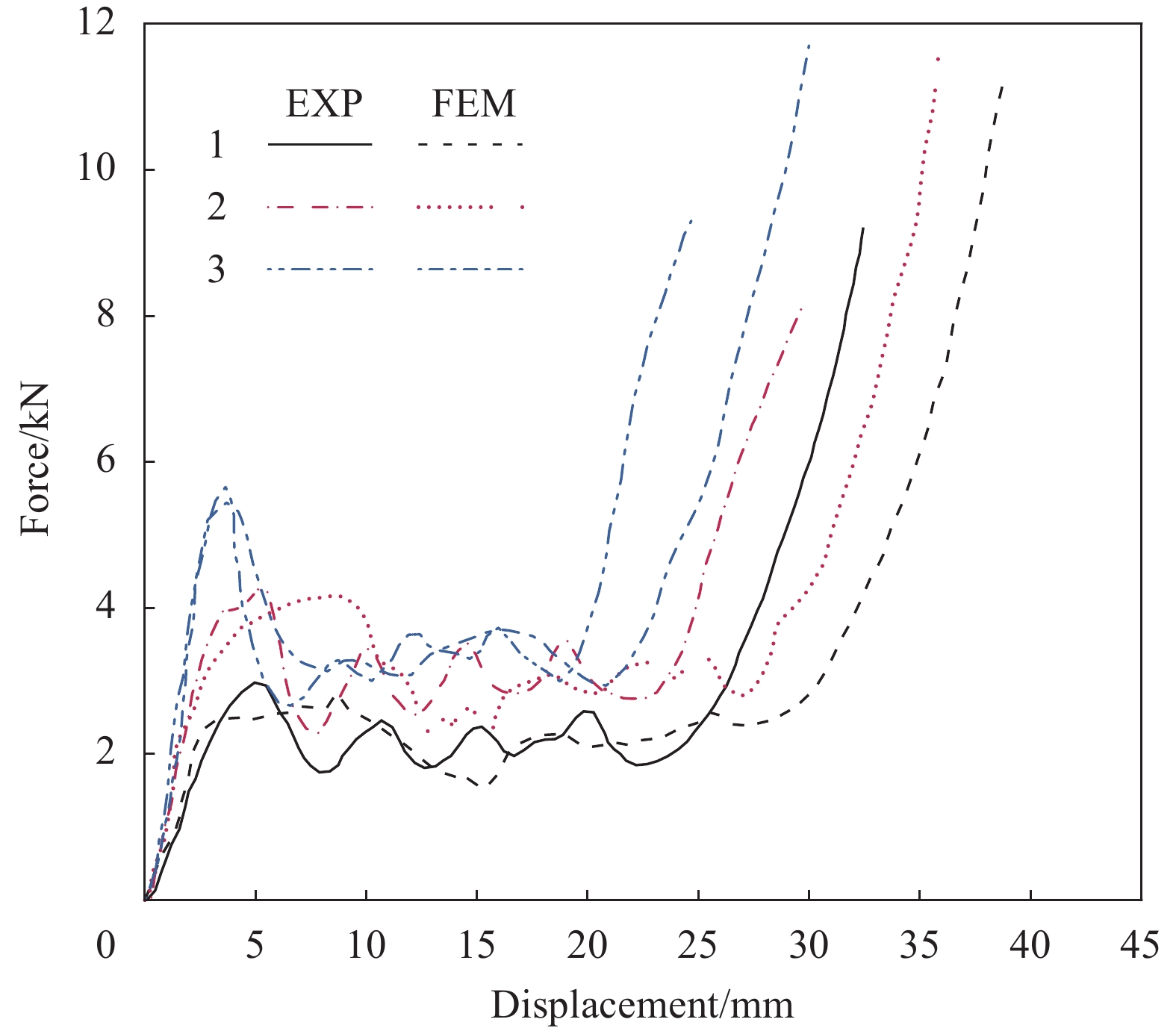
Sample name Wave number Relative density Test value[32]/kN Simulated value/kN Absolute error/kN Relative error/% 1 5 0.13303 2.97 2.84 0.13 4.38 2 6 0.14662 4.25 4.17 0.08 1.88 3 7 0.16201 5.65 5.46 0.19 3.36 
下载: 导出CSV
-
[1] BAROUTAJI A, SAJJIA M, OLABI A G. On the crashworthiness performance of thin-walled energy absorbers: Recent advances and future developments[J]. Thin-Walled Structures, 2017, 118: 137-163. DOI: 10.1016/j.tws.2017.05.018
[2] QIU X M, YU T X. Some topics in recent advances and applications of structural impact dynamics[J]. Applied Mechanics Reviews, 2011, 64(3): 1-12.
[3] 余同希, 朱凌, 许骏. 结构冲击动力学进展(2010~2020)[J]. 爆炸与冲击, 2021, 41(12): 4-64. YU Tongxi, ZHU Ling, XU Jun. Progress of structural impact dynamics (2010—2020)[J]. Explosion and Shock Waves, 2021, 41(12): 4-64(in Chinese).
[4] YANG J, CHEN X, SUN Y, et al. Compressive properties of bidirectionally graded lattice structures[J]. Materials & Design, 2022, 218: 110683.
[5] YE J, SUN Z, DING Y, et al. The deformation mechanism, energy absorption behavior and optimal design of vertical-reinforced lattices[J]. Thin-Walled Structures, 2023, 190: 110988. DOI: 10.1016/j.tws.2023.110988
[6] 张武昆, 谭永华, 高玉闪, 等. 周期性轻质多孔结构在能量吸收和振动方面的研究进展[J]. 振动与冲击, 2023, 42(8): 1-19. ZHANG Wukun, TAN Yonghua, GAO Yushan, et al. Research progress on energy absorption and vibration of periodic lightweight porous structures[J]. Journal of Vibration and Shock, 2023, 42(8): 1-19(in Chinese).
[7] XU P, GUO W, YANG L, et al. Crashworthiness analysis of the biomimetic lotus root lattice structure[J]. International Journal of Mechanical Sciences, 2024, 263: 108774. DOI: 10.1016/j.ijmecsci.2023.108774
[8] 韩剑, 孙士勇, 牛斌, 等. 树脂基复合材料点阵结构的制造技术研究进展[J]. 航空学报, 2023, 44(9): 47-67. HAN Jian, SUN Shiyong, NIU Bin, et al. Research progress in manufacturing technology of resin matrix composites with lattice structure[J]. Acta Aeronautica et Astronautica Sinica, 2023, 44(9): 47-67(in Chinese).
[9] XU Z, RAZAVI S M J, AYATOLLAHI M R. Functionally graded lattice structures: Fabrication methods, mechanical properties, failure mechanisms and applications[M]. Amsterdam: Comprehensive Structural Integrity, 2022: 433-466.
[10] DONG G, WIJAYA G, TANG Y, et al. Optimizing process parameters of fused deposition modeling by Taguchi method for the fabrication of lattice structures[J]. Additive Manufacturing, 2018, 19: 62-72. DOI: 10.1016/j.addma.2017.11.004
[11] CUI Z, ZHAO J, XU R, et al. Mechanical design and energy absorption performances of novel plate-rod hybrid lattice structures[J]. Thin-Walled Structures, 2023, 194(10): 111349.
[12] YANG J, CHEN X, SUN Y, et al. Rational design and additive manufacturing of grain boundary-inspired, multi-architecture lattice structures[J]. Materials & Design, 2023, 235: 112448.
[13] XIAO L, FENG G, LI S, et al. Mechanical characterization of additively-manufactured metallic lattice structures with hollow struts under static and dynamic loadings[J]. International Journal of Impact Engineering, 2022, 169: 104333. DOI: 10.1016/j.ijimpeng.2022.104333
[14] MA X, ZHANG N, CHANG Y, et al. Analytical model of mechanical properties for a hierarchical lattice structure based on hierarchical body-centered cubic unit cell[J]. Thin-Walled Structures, 2023, 193: 111217. DOI: 10.1016/j.tws.2023.111217
[15] LEE J J, MOHAMMED A A, PULLEN A, et al. Mechanical characterisation of 3D printed lightweight lattice structures with varying internal design alterations[J]. Materials Today Communications, 2023, 36: 106456. DOI: 10.1016/j.mtcomm.2023.106456
[16] XIAO L, SONG W. Additively-manufactured functionally graded Ti-6Al-4V lattice structures with high strength under static and dynamic loading: Experiments[J]. International Journal of Impact Engineering, 2018, 111: 255-272. DOI: 10.1016/j.ijimpeng.2017.09.018
[17] HA N S, LU G. A review of recent research on bio-inspired structures and materials for energy absorption applications[J]. Composites Part B: Engineering, 2020, 181: 107496. DOI: 10.1016/j.compositesb.2019.107496
[18] PLESSIS A, BROECKHOVEN C, YADROITSAVA I, et al. Beautiful and functional: A review of biomimetic design in additive manufacturing[J]. Additive Manufacturing, 2019, 27: 408-427. DOI: 10.1016/j.addma.2019.03.033
[19] AHAMED M K, WANG H, HAZELL P J. From biology to biomimicry: Using nature to build better structures—A review[J]. Construction and Building Materials, 2022, 320: 126195. DOI: 10.1016/j.conbuildmat.2021.126195
[20] SIDDIQUE S H, HAZELL P J, WANG H, et al. Lessons from nature: 3D printed bio-inspired porous structures for impact energy absorption—A review[J]. Additive Manufacturing, 2022, 58: 103051. DOI: 10.1016/j.addma.2022.103051
[21] ZHANG W, YIN S, YU T X, et al. Crushing resistance and energy absorption of pomelo peel inspired hierarchical honeycomb[J]. International Journal of Impact Engineering, 2019, 125: 163-172. DOI: 10.1016/j.ijimpeng.2018.11.014
[22] 冀旭晖. 仿生蜘蛛网点阵结构设计及其吸能效果研究[D]. 哈尔滨: 哈尔滨理工大学, 2022. JI Xuhui. Study on bionic spider web lattice structure design and energy absorption effect[D]. Harbin: Harbin University of Science and Technology, 2022(in Chinese).
[23] MENG L, SHI J, YANG C, et al. An emerging class of hyperbolic lattice exhibiting tunable elastic properties and impact absorption through chiral twisting[J]. Extreme Mechanics Letters, 2020, 40: 100869. DOI: 10.1016/j.eml.2020.100869
[24] PATEK S N, KORFF W L, CALDWELL R L. Deadly strike mechanism of a mantis shrimp[J]. Nature, 2004, 428(6985): 819-820. DOI: 10.1038/428819a
[25] WEAVER J C M G W, MISEREZ A, EVANS-LUTTERODT K, et al. The stomatopod dactyl club: A formidable damage-tolerant biological hammer[J]. Science, 2012, 336(6086): 1275-1280. DOI: 10.1126/science.1218764
[26] PATEK S N, CALDWELL R L. Extreme impact and cavitation forces of a biological hammer: Strike forces of the peacock mantis shrimp Odontodactylus scyllarus[J]. Journal of Experimental Biology, 2005, 208(19): 3655-3664. DOI: 10.1242/jeb.01831
[27] YARAGHI N A, GUARÍN-ZAPATA N, GRUNENFELDER L K, et al. A sinusoidally architected helicoidal biocomposite[J]. Advanced Materials, 2016, 28(32): 6835-6844. DOI: 10.1002/adma.201600786
[28] YANG X, MA J, SHI Y, et al. Crashworthiness investigation of the bio-inspired bi-directionally corrugated core sandwich panel under quasi-static crushing load[J]. Materials & Design, 2017, 135: 275-290.
[29] HAN Q, SHI S, LIU Z, et al. Study on impact resistance behaviors of a novel composite laminate with basalt fiber for helical-sinusoidal bionic structure of dactyl club of mantis shrimp[J]. Composites Part B: Engineering, 2020, 191: 107976. DOI: 10.1016/j.compositesb.2020.107976
[30] HUANG H, YANG X, YAN Q, et al. Crashworthiness analysis and multiobjective optimization of bio-inspired sandwich structure under impact load[J]. Thin-Walled Structures, 2022, 172(3): 108840.
[31] CUI C Y, CHEN L, FENG S, et al. Novel cuttlebone-inspired hierarchical bionic structure enabled high energy absorption[J]. Thin-Walled Structures, 2023, 186: 110693. DOI: 10.1016/j.tws.2023.110693
[32] LI B, LIU H, ZHANG Q, et al. Crushing behavior and energy absorption of a bio-inspired bi-directional corrugated lattice under quasi-static compression load[J]. Composite Structures, 2022, 286: 115315. DOI: 10.1016/j.compstruct.2022.115315
[33] HUNT C J, MORABITO F, GRACE C, et al. A review of composite lattice structures[J]. Composite Structures, 2022, 284: 115120. DOI: 10.1016/j.compstruct.2021.115120
[34] ZHU J, ZHOU H, WANG C, et al. A review of topology optimization for additive manufacturing: Status and challenges[J]. Chinese Journal of Aeronautics, 2021, 34(1): 91-110. DOI: 10.1016/j.cja.2020.09.020
[35] CLAVERIE T, CHAN E, PATEK S N. Modularity and scaling in fast movements: Power amplification in mantis shrimp[J]. Evolution, 2011, 65(2): 443-461. DOI: 10.1111/j.1558-5646.2010.01133.x
[36] 黄晗, 许述财, 陈姮. 仿生波纹夹层结构耐撞性分析及优化[J]. 爆炸与冲击, 2021, 41(8): 36-46. DOI: 10.11883/bzycj-2020-0275 HUANG Han, XU Shucai, CHEN Heng. Impact resistance analysis and optimization of biomimetic corrugated sandwich structures[J]. Explosion and Shock Waves, 2021, 41(8): 36-46(in Chinese). DOI: 10.11883/bzycj-2020-0275
[37] 魏路路, 余强, 赵轩, 等. 内凹-反手性蜂窝结构的面内动态压溃性能研究[J]. 振动与冲击, 2021, 40(4): 261-269. WEI Lulu, YU Qiang, ZHAO Xuan, et al. Research on in-plane dynamic crushing behavior of concave and backhanded honeycomb structures[J]. Journal of Vibration and Shock, 2021, 40(4): 261-269(in Chinese).
[38] GIBSON L J, ASHBY M F. Cellular solids[M]. Cambridge: Cambridge University Press, 1997: 2-12.
[39] 刘伟洛. 增材制造三周期极小曲面结构的力学性能研究[D]. 广州: 广州大学, 2021. LIU Weiluo. Research on mechanical properties of additive manufacturing three-period minimal curved surface structures[D]. Guangzhou: Guangzhou University, 2021(in Chinese).
[40] 徐向聪, 高佳丽, 郝云波. 304不锈钢多层梯度点阵结构压缩性能及梯度率影响研究[J]. 机械强度, 2023, 45(6): 1318-1325. XU Xiangcong, GAO Jiali, HAO Yunbo. Research on compression properties and influence of gradient rate of 304 stainless steel multilayer gradient lattice structure[J]. Mechanical Strength, 2023, 45(6): 1318-1325(in Chinese).
[41] MASKERY I, ABOULKHAIR N T, AREMU A O, et al. A mechanical property evaluation of graded density Al-Si10-Mg lattice structures manufactured by selective laser melting[J]. Materials Science and Engineering: A, 2016, 670: 264-274. DOI: 10.1016/j.msea.2016.06.013
[42] 李晓丹, 朱庆丰, 孔淑萍, 等. 3D打印AlSi10Mg合金组织性能研究[J]. 材料科学与工艺, 2019, 27(2): 16-21. DOI: 10.11951/j.issn.1005-0299.20180138 LI Xiaodan, ZHU Qingfeng, KONG Shuping, et al. Study on microstructure and properties of 3D printed AlSi10Mg alloy[J]. Materials Science and Technology, 2019, 27(2): 16-21(in Chinese). DOI: 10.11951/j.issn.1005-0299.20180138
[43] 陈剑勇. 静动态载荷下三周期极小曲面多孔结构响应特性研究[D]. 武汉: 华中科技大学, 2023. CHEN Jianyong. Study on response characteristics of three-period minimal curved porous structures under static and dynamic loads[D]. Wuhan: Huazhong University of Science and Technology, 2023(in Chinese).
[44] MACONACHIE T, LEARY M, TRAN P, et al. The effect of topology on the quasi-static and dynamic behaviour of SLM AlSi10Mg lattice structures[J]. The International Journal of Advanced Manufacturing Technology, 2021, 118(11-12): 4085-4104.
[45] 厉雪, 肖李军, 宋卫东. 3D打印梯度Gyroid结构的动态冲击响应[J]. 高压物理学报, 2021, 35(3): 90-99. DOI: 10.11858/gywlxb.20210701 LI Xue, XIAO Lijun, SONG Weidong. Dynamic impact response of 3D printed gradient gyroid structure[J]. Journal of High Pressure Physics, 2021, 35(3): 90-99(in Chinese). DOI: 10.11858/gywlxb.20210701
[46] 卢子兴, 王欢, 杨振宇, 等. 星型-箭头蜂窝结构的面内动态压溃行为[J]. 复合材料学报, 2019, 36(8): 1893-1900. LU Zixing, WANG Huan, YANG Zhenyu, et al. In-plane dynamic crushing behavior of star-arrow honeycomb structures[J]. Acta Materiae Compositae Sinica, 2019, 36(8): 1893-1900(in Chinese).
[47] 张晓楠, 晏石林, 欧元勋, 等. 负泊松比内凹蜂窝结构梯度设计与动态冲击响应[J]. 振动与冲击, 2023, 42(3): 193-198. ZHANG Xiaonan, YAN Shilin, OU Yuanxun, et al. Gradient design and dynamic shock response of concave honeycomb structures with negative Poisson's ratio[J]. Journal of Vibration and Shock, 2023, 42(3): 193-198(in Chinese).
[48] 李成兵, 李锐, 张吉涛, 等. 多阶式层级梯度蜂窝结构的共面冲击响应[J]. 高压物理学报, 2023, 37(3): 121-132. LI Chengbing, LI Rui, ZHANG Jitao, et al. Coplanar shock response of multistage hierarchical gradient honeycomb structures[J]. Journal of High Pressure Physics, 2023, 37(3): 121-132(in Chinese).
[49] 纪小刚, 张建安, 栾宇豪, 等. 仿皮肤三维多孔点阵结构压缩吸能性能研究[J]. 机械工程学报, 2021, 57(15): 222-230. DOI: 10.3901/JME.2021.15.222 JI Xiaogang, ZHANG Jian'an, LUAN Yuhao, et al. Study on compressive energy absorption performance of three-dimensional porous lattice structure modeled on skin[J]. Chinese Journal of Mechanical Engineering, 2021, 57(15): 222-230(in Chinese). DOI: 10.3901/JME.2021.15.222
[50] 李心远, 宋卫东, 陈键. 3D打印TPMS多孔材料力学性能数值仿真[J]. 太原理工大学学报, 2019, 50(3): 386-393. LI Xinyuan, SONG Weidong, CHEN Jian. Numerical simulation of mechanical properties of 3D printed TPMS porous materials[J]. Journal of Taiyuan University of Technology, 2019, 50(3): 386-393(in Chinese).
[51] 肖江海, 侯俊玲, 李群. 基于MJF的极小曲面结构力学行为及吸能特性研究[J]. 固体力学学报, 2024, 45(2): 201-212. XIAO Jianghai, HOU Junling, LI Qun. Study on mechanical behavior and energy absorption characteristics of minimal surface structures based on MJF[J]. Journal of Solid Mechanics, 2024, 45(2): 201-212(in Chinese).
[52] 赵众豪, 池瑜莉, 冯峻良, 等. 新型CFCB点阵夹芯结构面外压缩载荷下能量吸收特性研究[J]. 振动与冲击, 2023, 42(17): 166-174. ZHAO Zhonghao, CHI Yuli, FENG Junliang, et al. Study on energy absorption characteristics of novel CFCB lattice sandwich structures under out-of-plane compression loads[J]. Journal of Vibration and Shock, 2023, 42(17): 166-174(in Chinese).
[53] 虞科炯, 徐峰祥, 华林. 正弦曲边负泊松比蜂窝结构面内冲击性能研究[J]. 振动与冲击, 2021, 40(13): 51-59. YU Kejiong, XU Fengxiang, HUA Lin. Research on in-plane impact performance of sinusoidal curved curved negative Poisson's ratio honeycomb[J]. Journal of Vibration and Shock, 2021, 40(13): 51-59(in Chinese).
-
期刊类型引用(3)
1. 程超，张晨宇，裴志磊，陈正国，周飞，周金利，张辉，孙泽玉，余木火. 双环戊二烯单体预聚增粘及其碳纤维增强复合材料性能评价. 复合材料学报. 2024(01): 155-169 . 
本站查看
2. 郝励. 碳纤维对SiC陶瓷基材料的导热性能影响研究. 化学与粘合. 2024(03): 235-239 . 百度学术
3. 柯锋，王朝恩. 热压制备的碳纤维复合材料不同温度的机械性能测试. 粘接. 2023(10): 112-114 . 百度学术
其他类型引用(1)
-
其他相关附件
-
目的
点阵结构作为一种新型轻质多功能结构，因其具有能量吸收能力好、比强度和比刚度高等诸多优异的性能，常被用于保护人员或结构免受冲击载荷的影响。与准静态荷载下的失效模式不同，在冲击荷载作用下，点阵结构一般会发生动态失稳，结构内部发生大的塑性/弹性变形。此外，点阵结构在空间上有材料与结构双重多尺度特征，冲击载荷作用又增加了时间多尺度特征。本研究旨在探究一种新型仿生双正弦波纹点阵结构(Bio-inspired bi-directional corrugated lattice structure, BBCLS)在冲击荷载作用下的力学性能和吸能特性。
方法首先采用ANSYS/LSDYNA有限元分析软件建立了其在冲击荷载作用下的有限元数值模型，并基于已有的试验结果与数值模拟结果进行了对比，验证了该模型的有效性。在此基础上，研究了不同冲击速度对BBCLS的应力分布、变形模式、承载能力以及能量吸收特性的影响，并与传统体心立方点阵结构(BCC)进行了对比。此外，系统探究了冲击速度及振幅、波纹数、胞壁厚度等微结构几何参数对BBCLS抗冲击特性的影响规律。
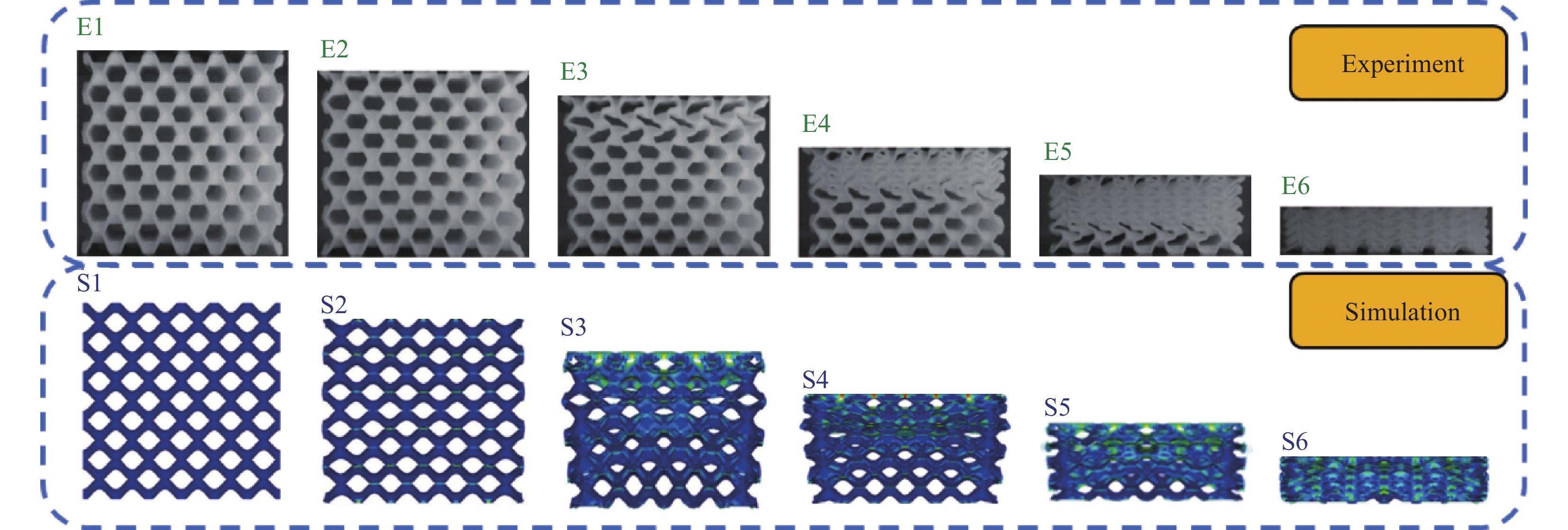
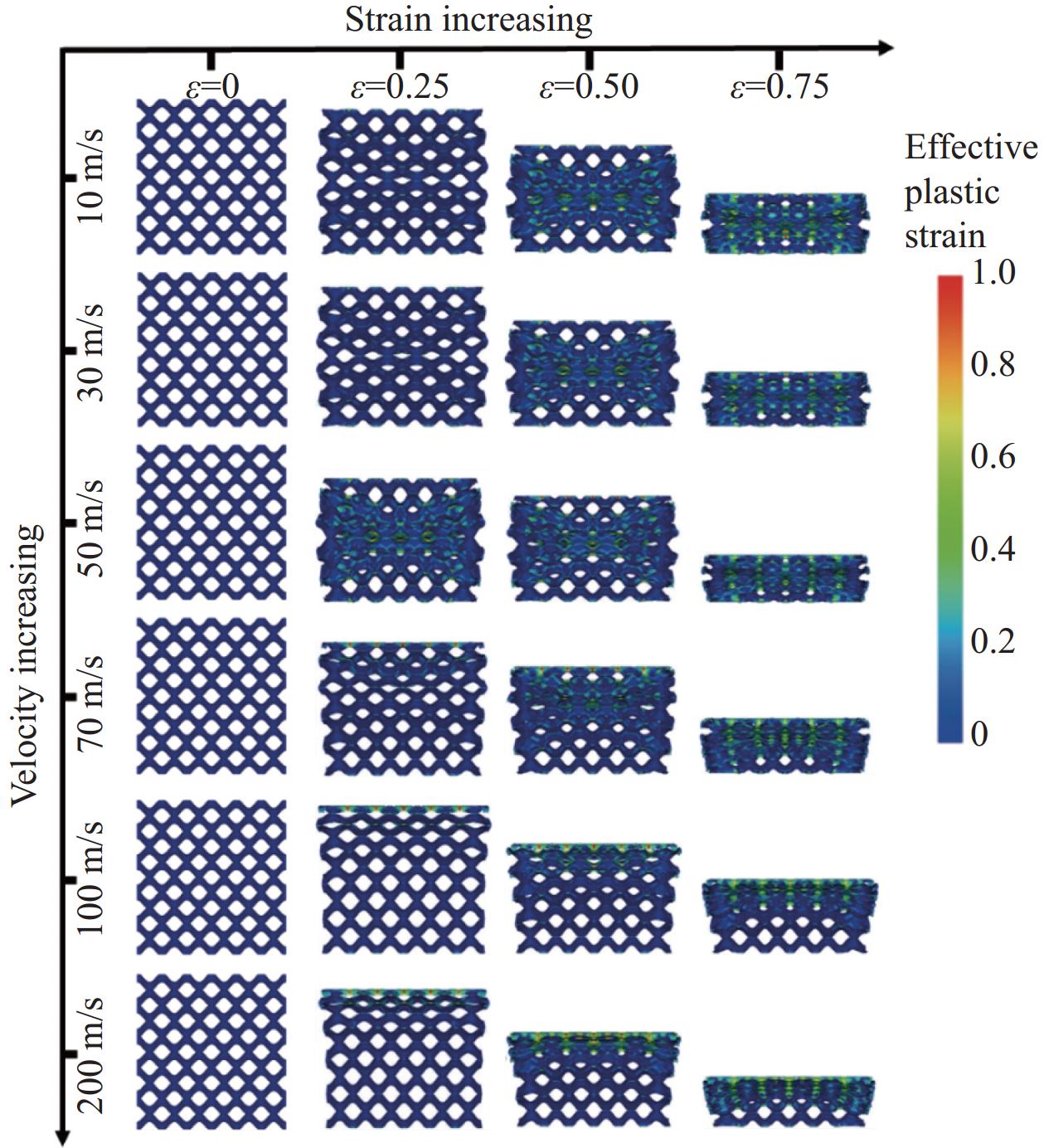
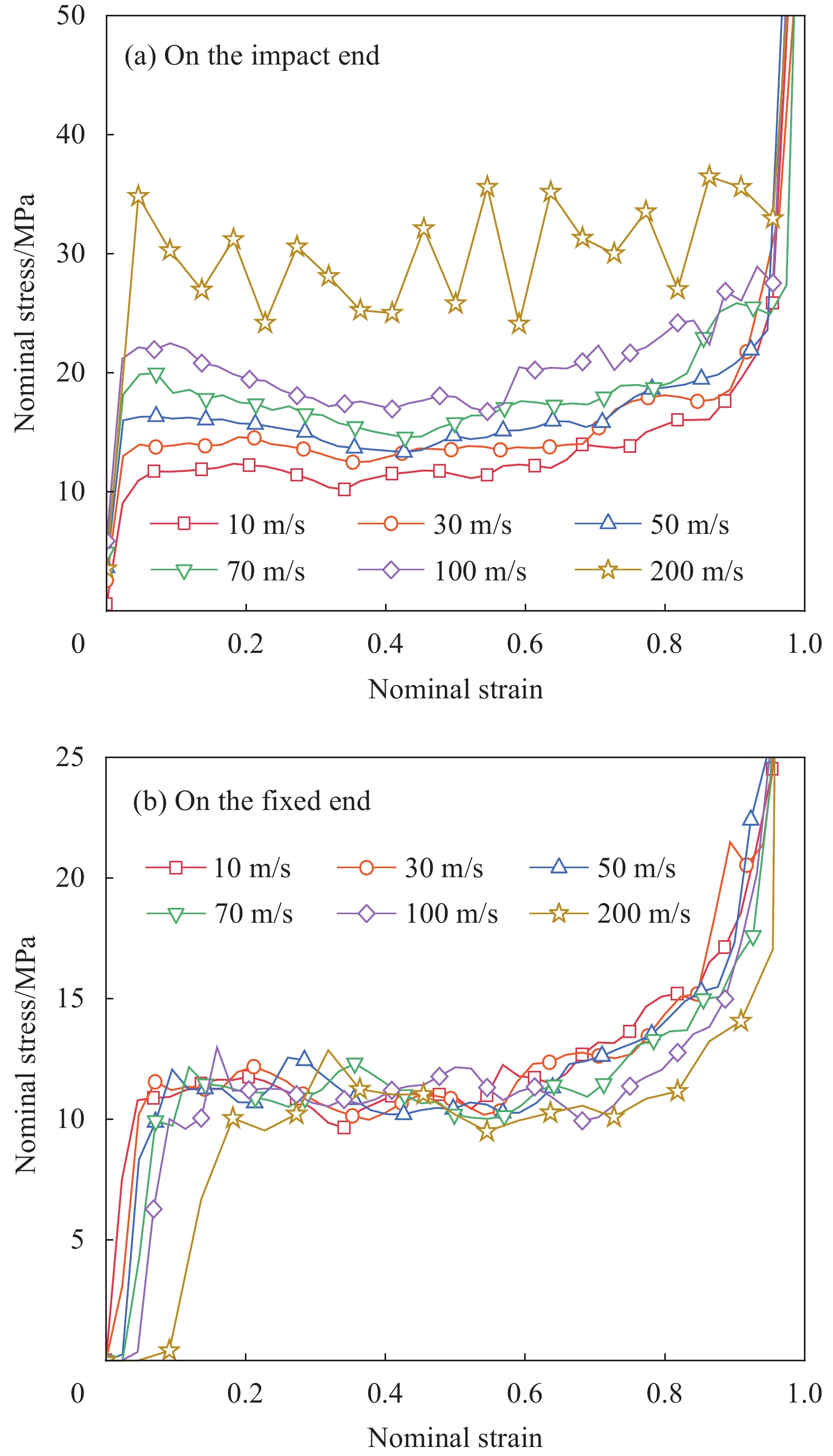
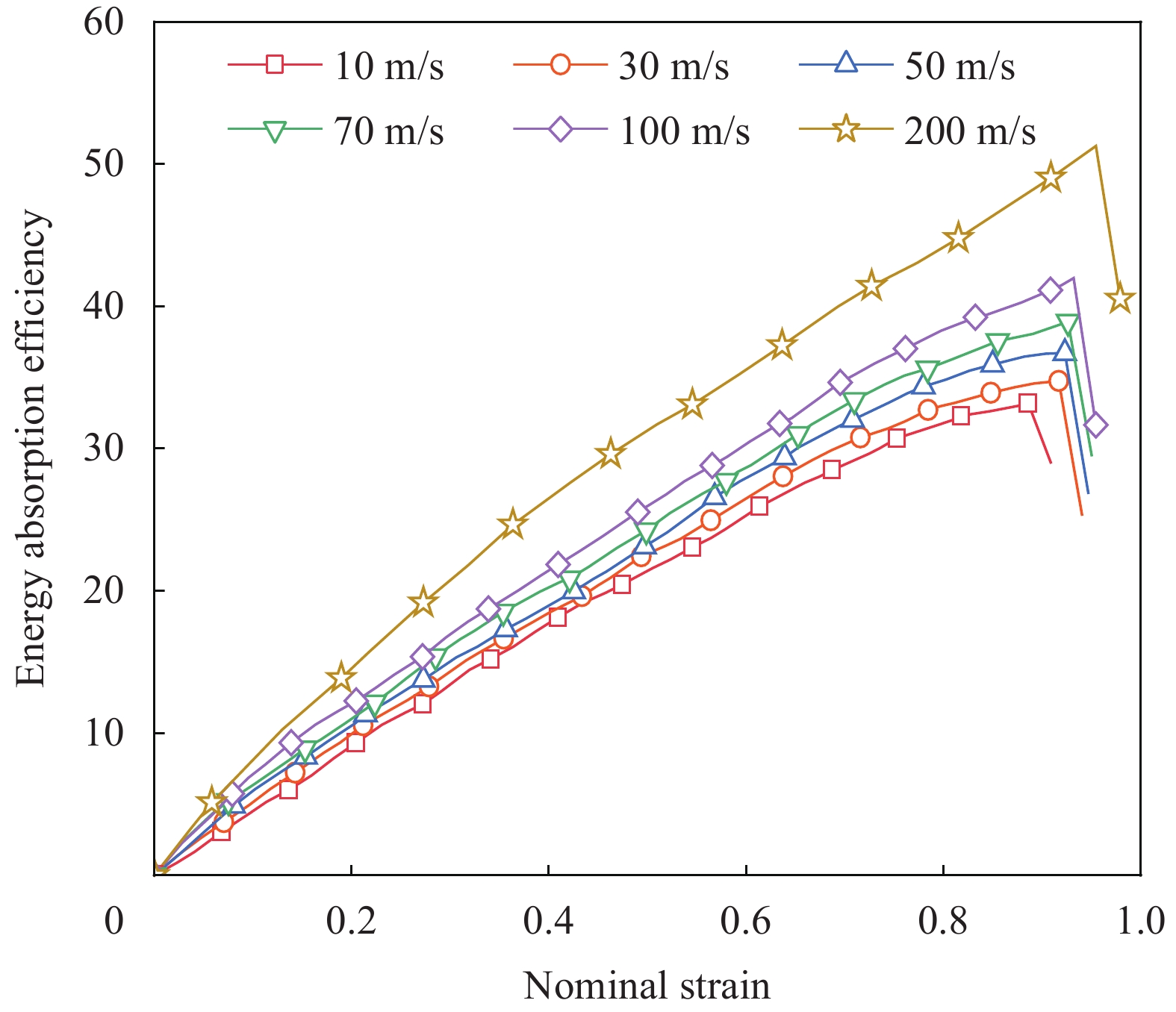
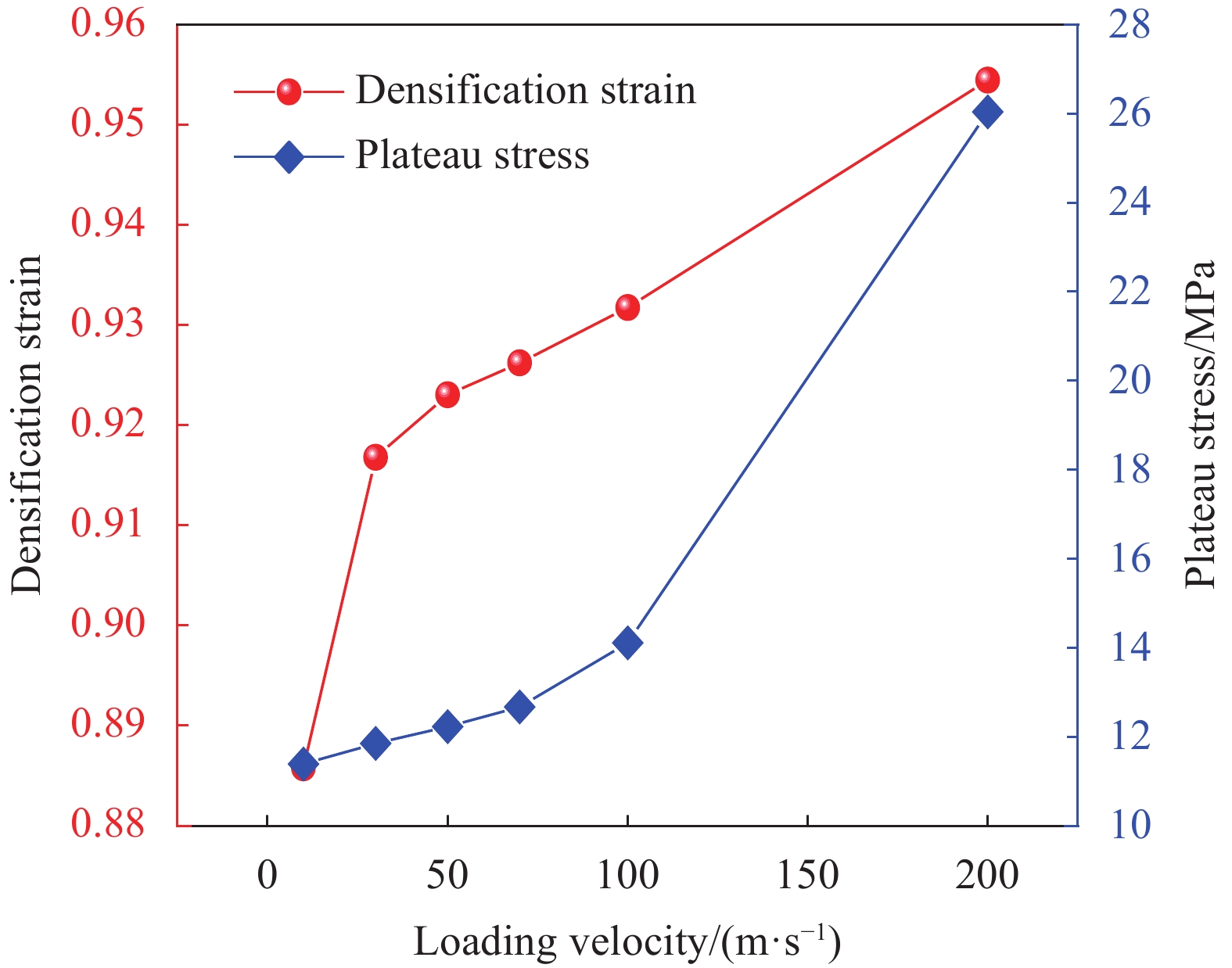
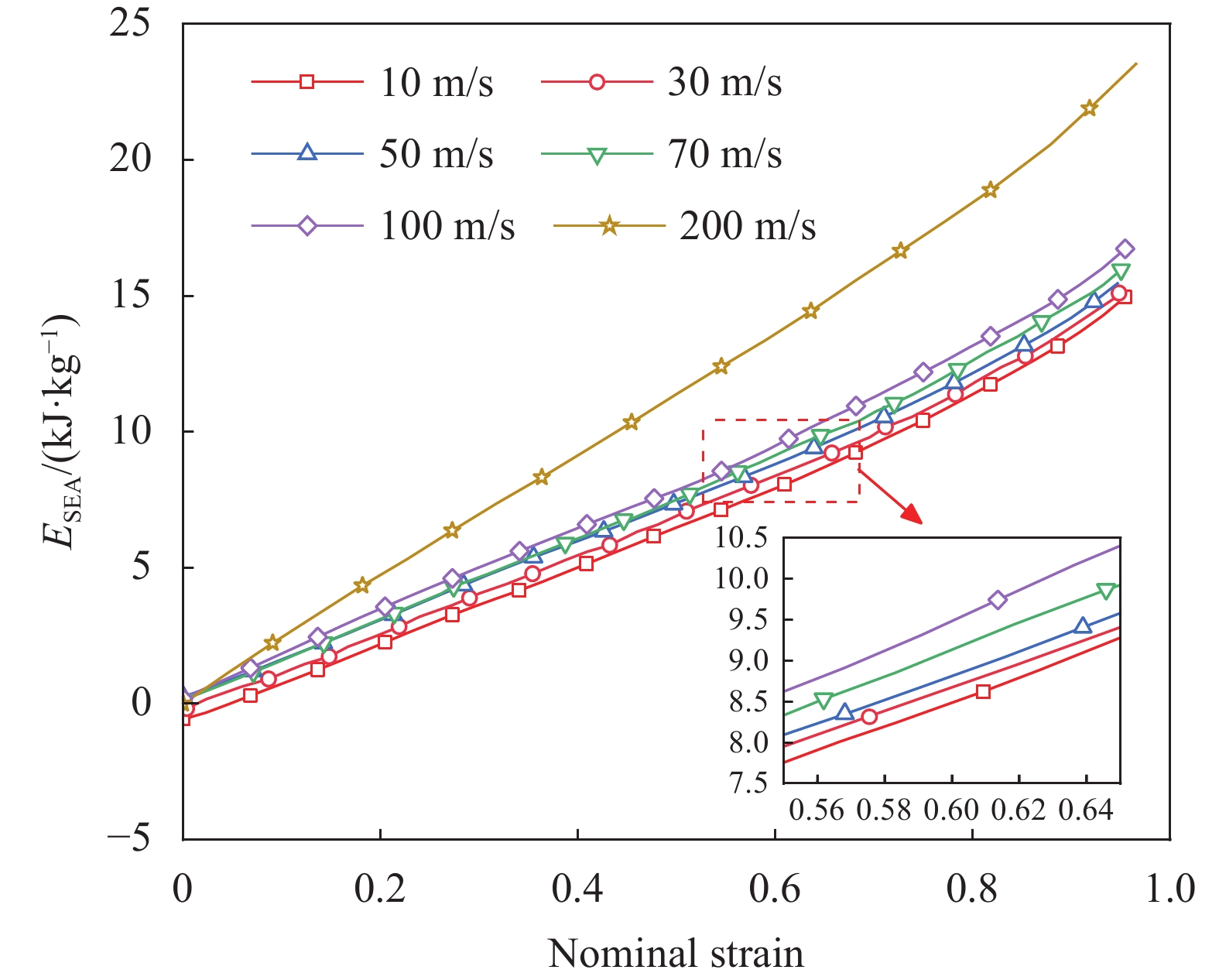
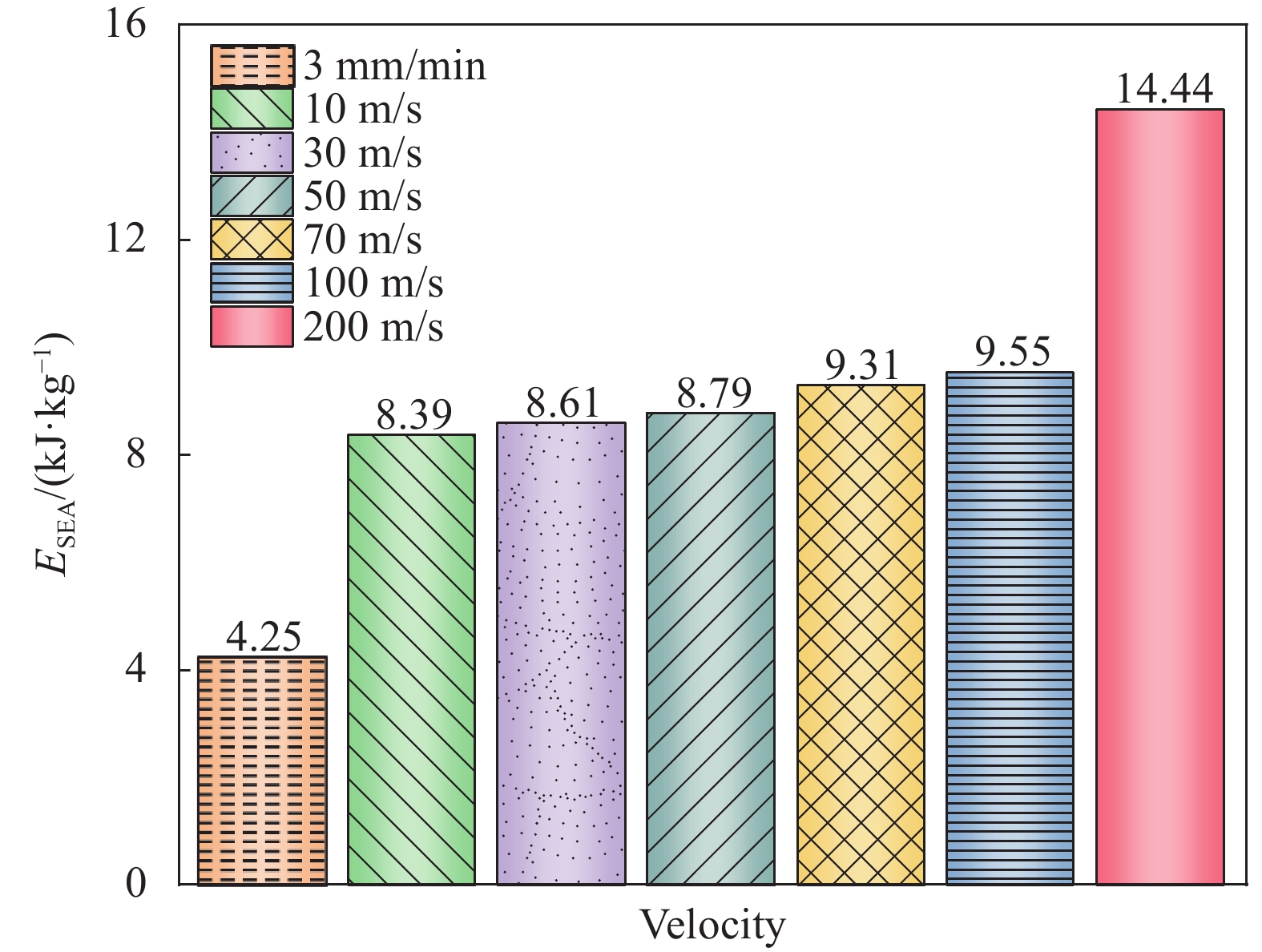
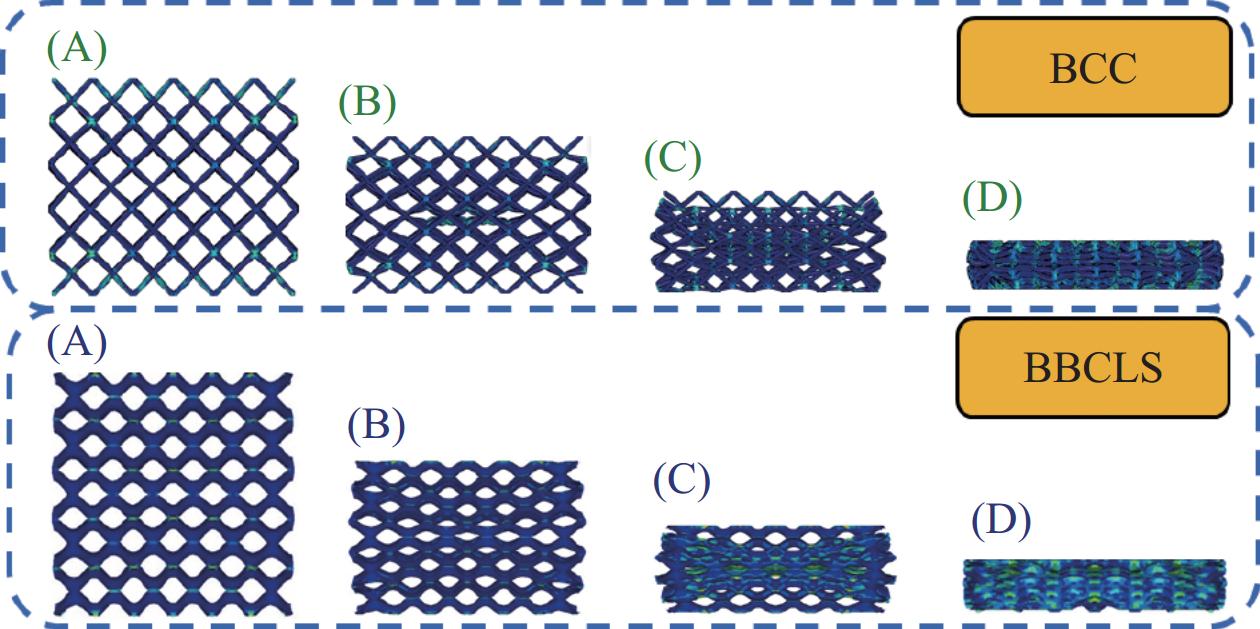
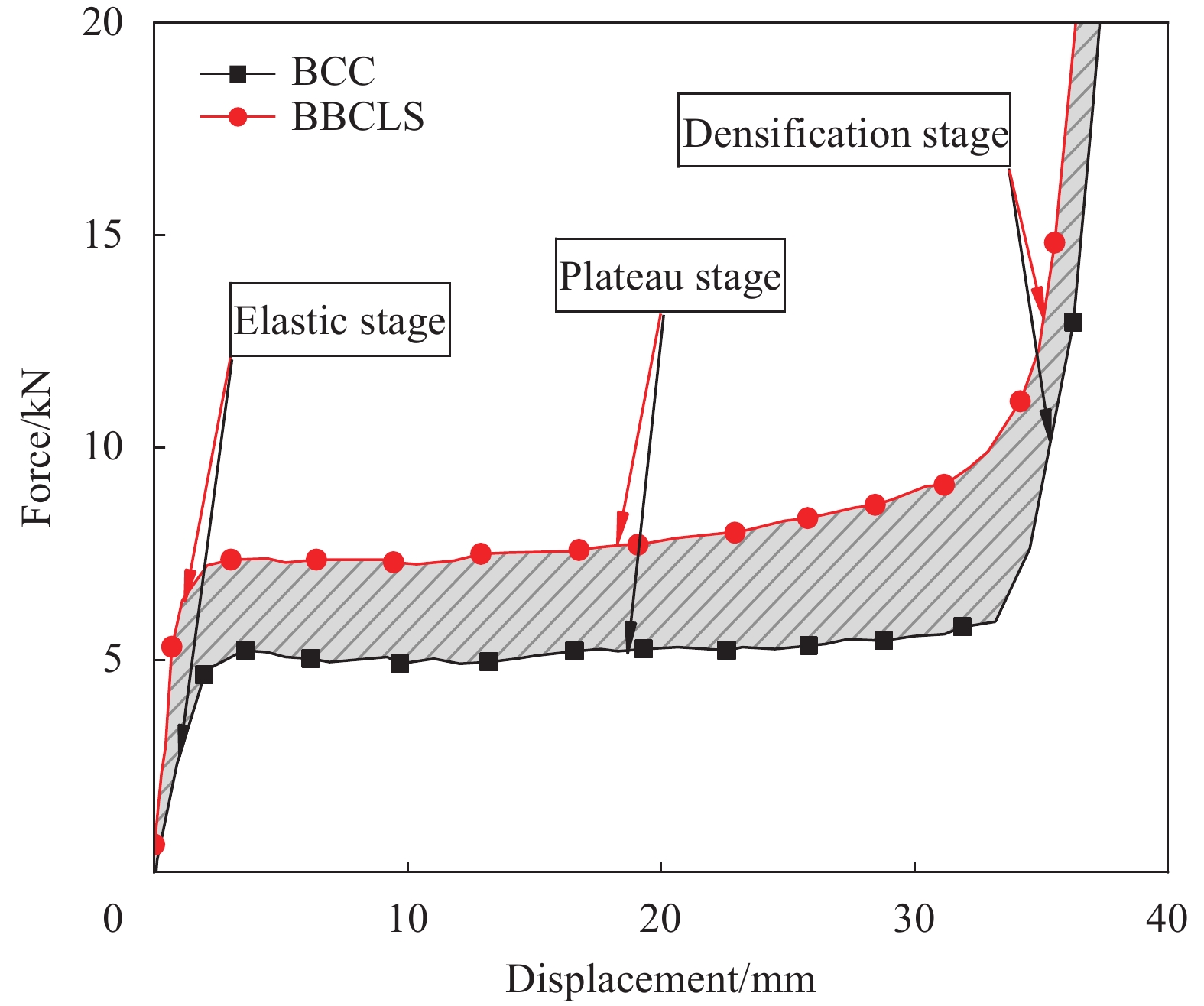
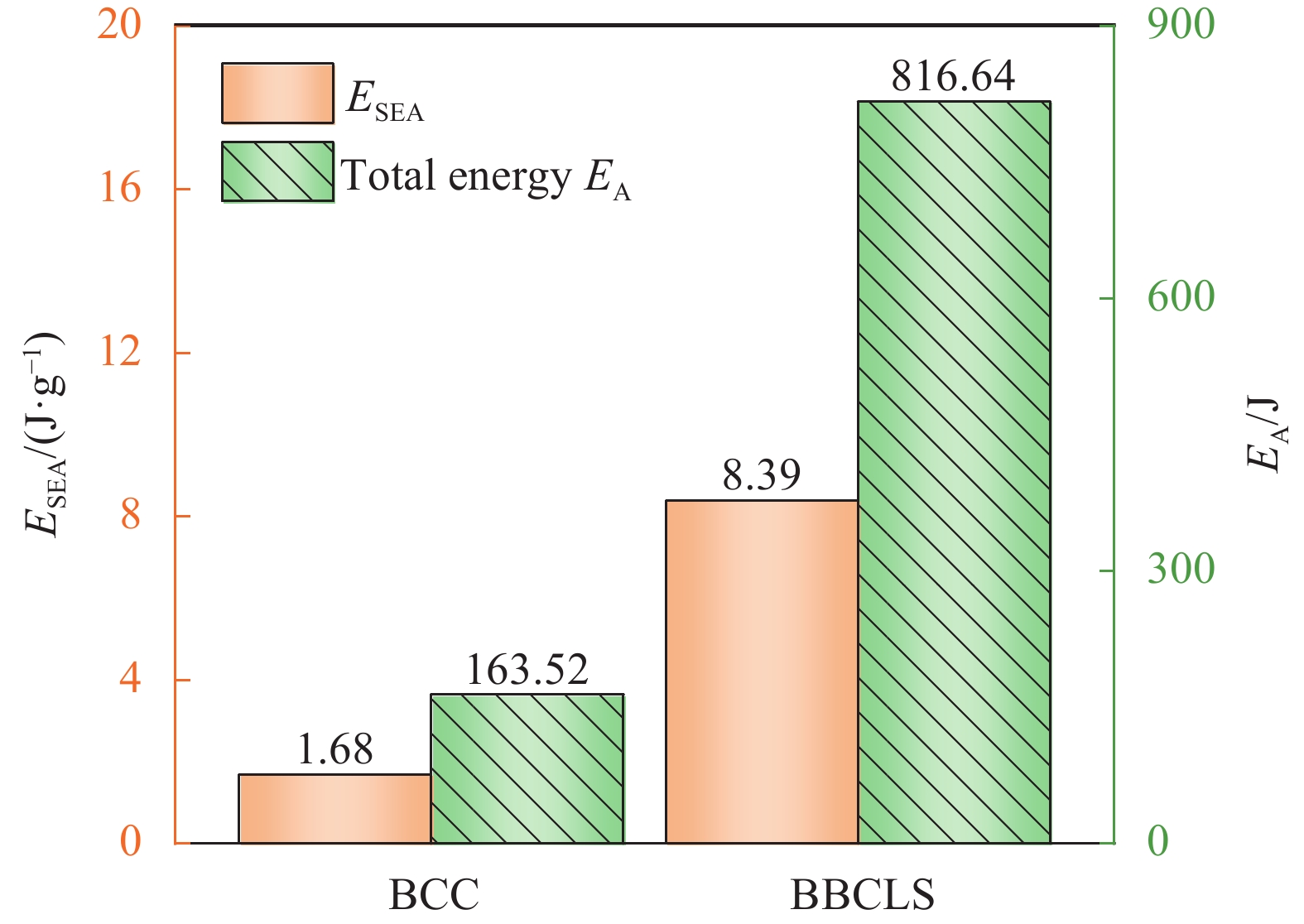
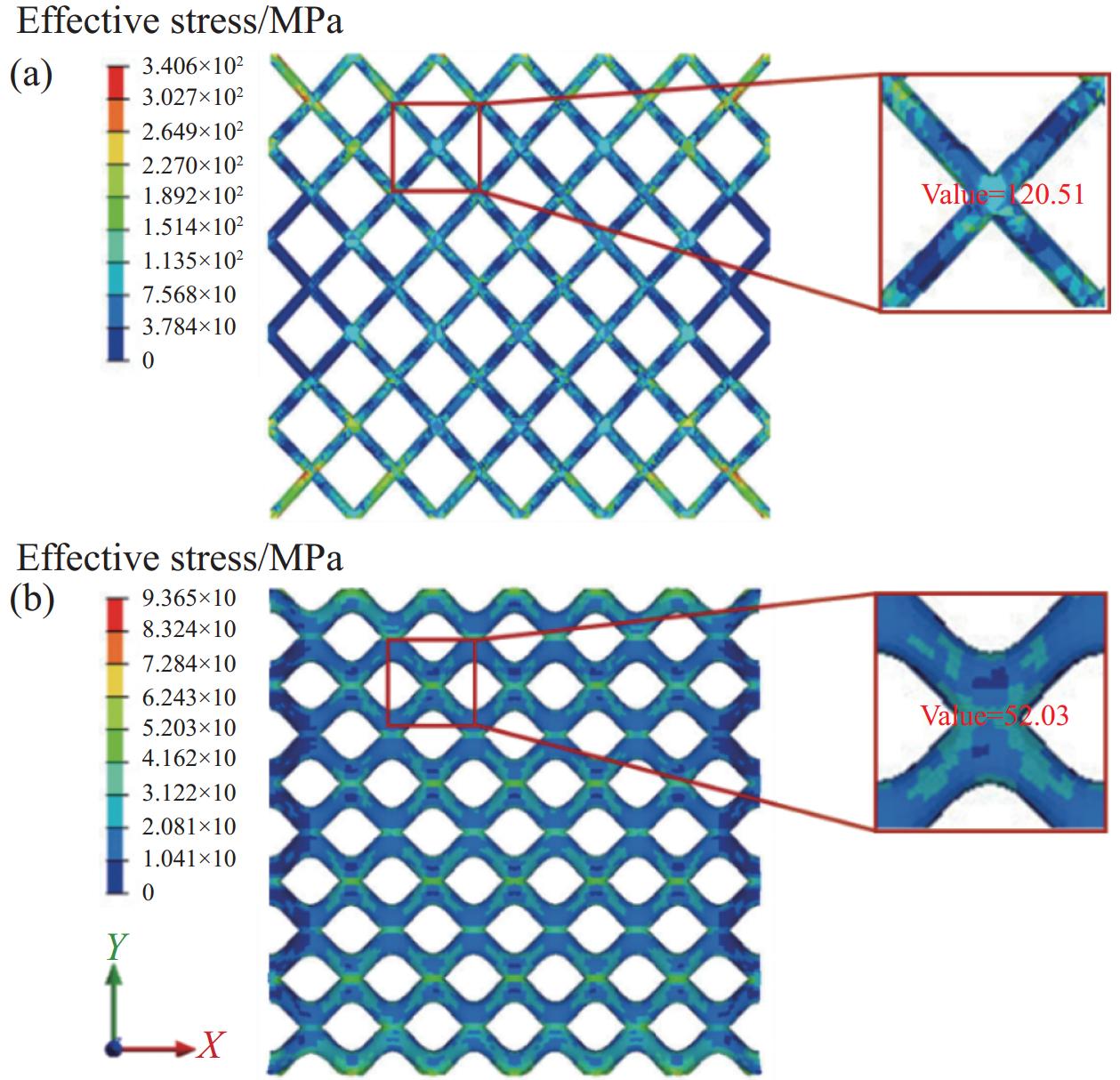
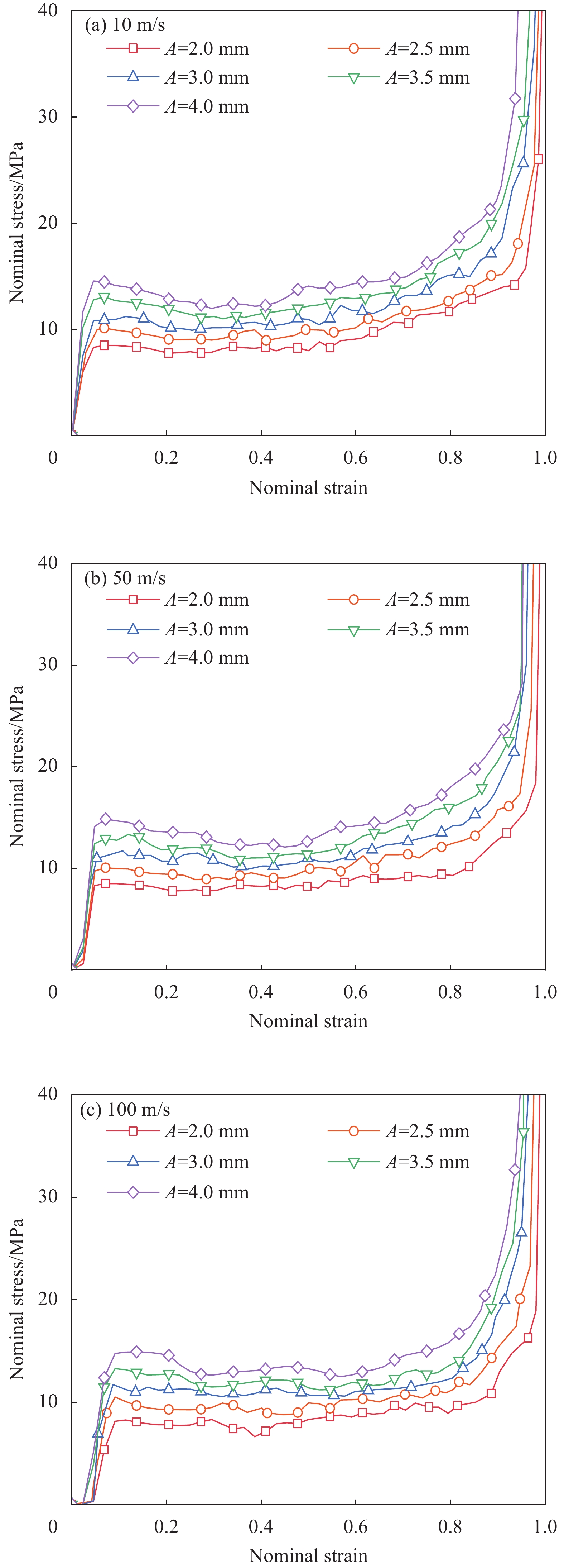
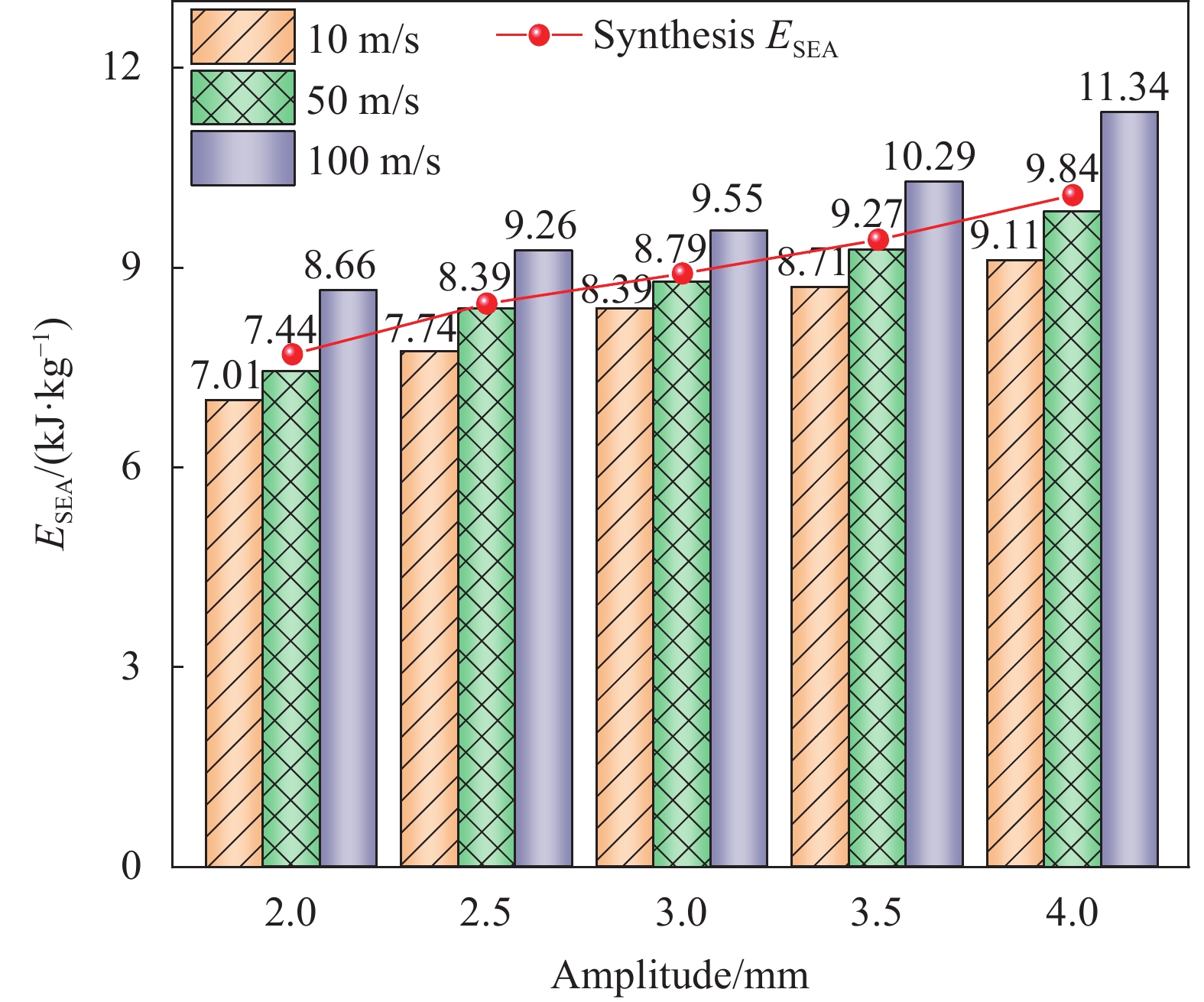
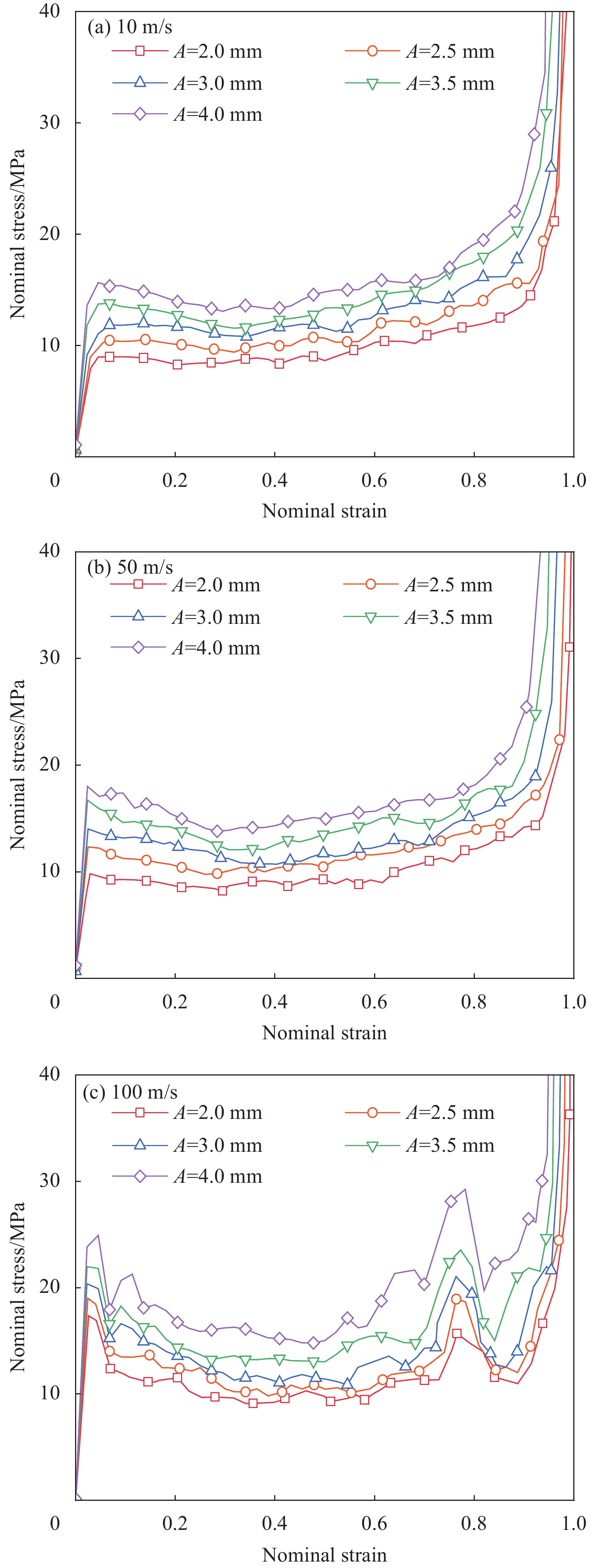
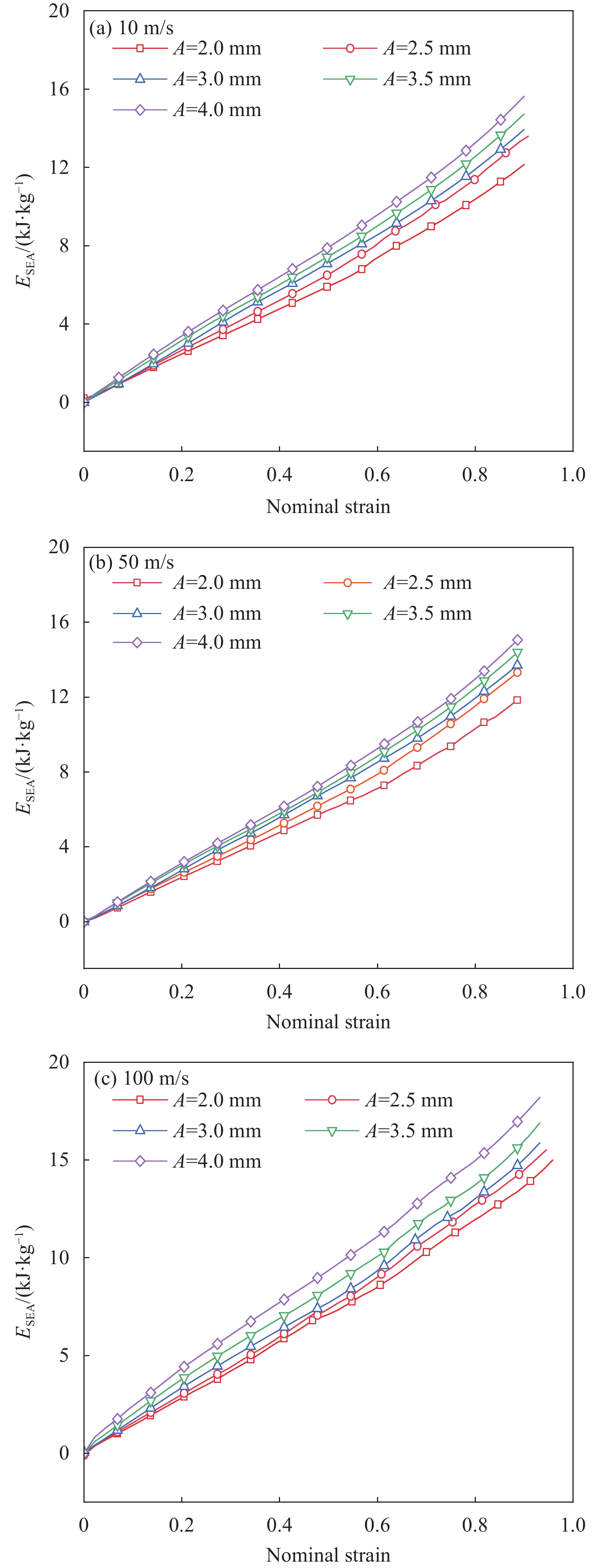
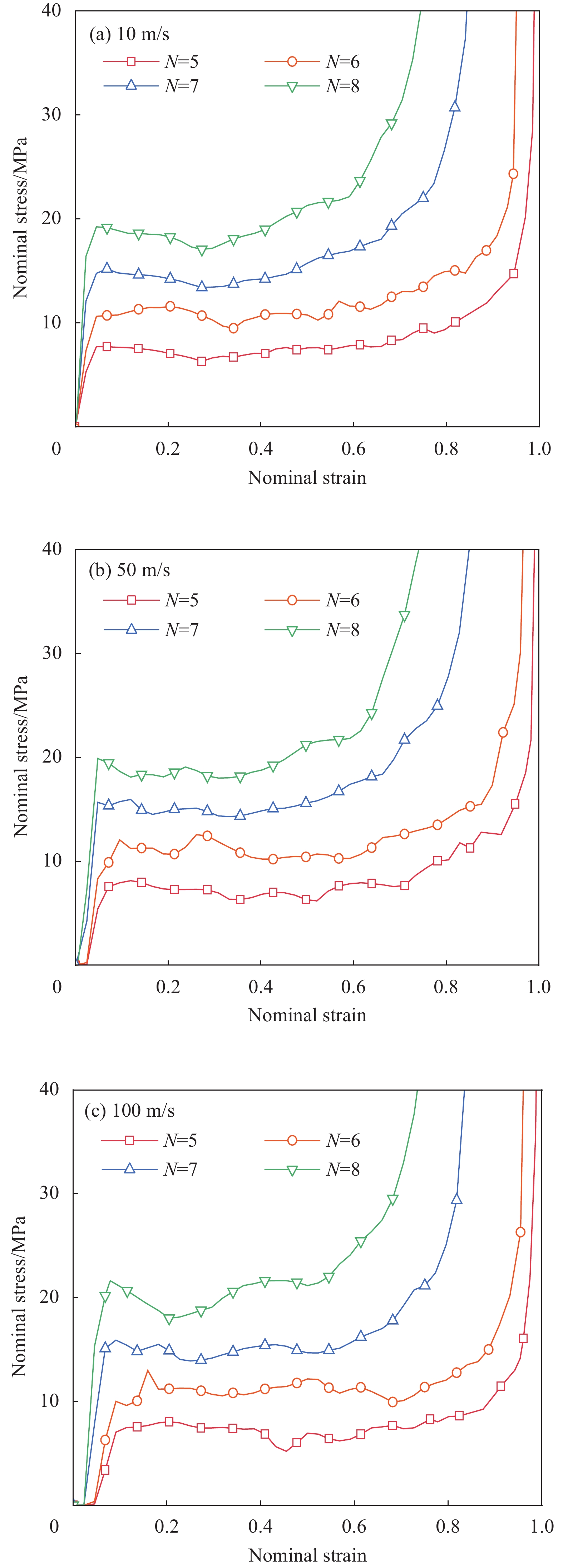
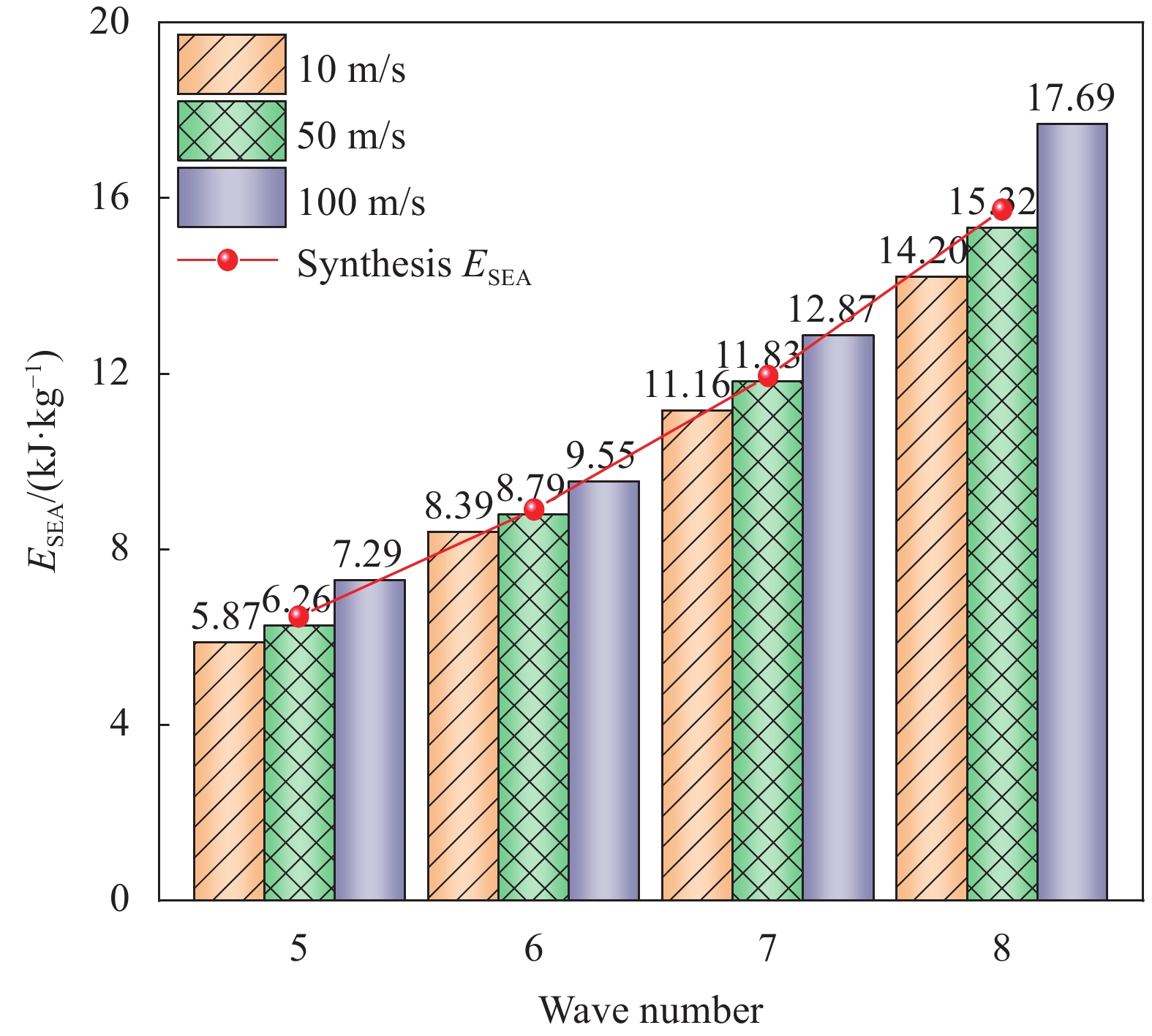
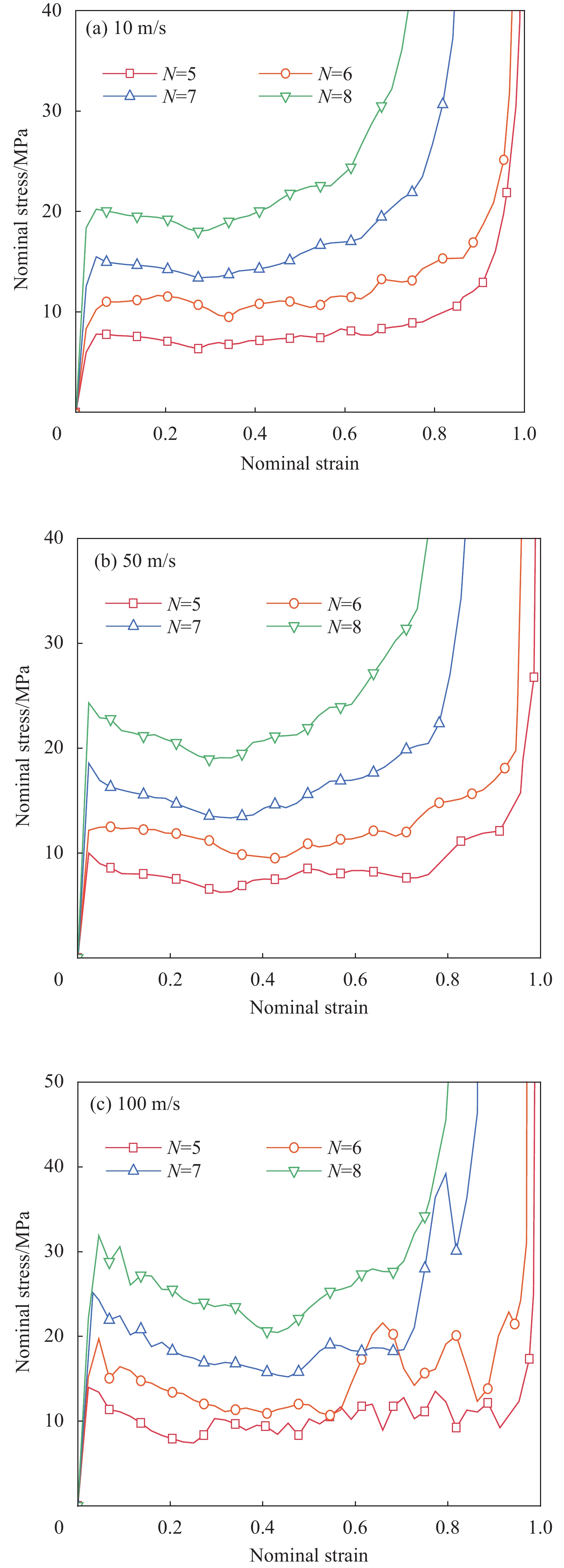
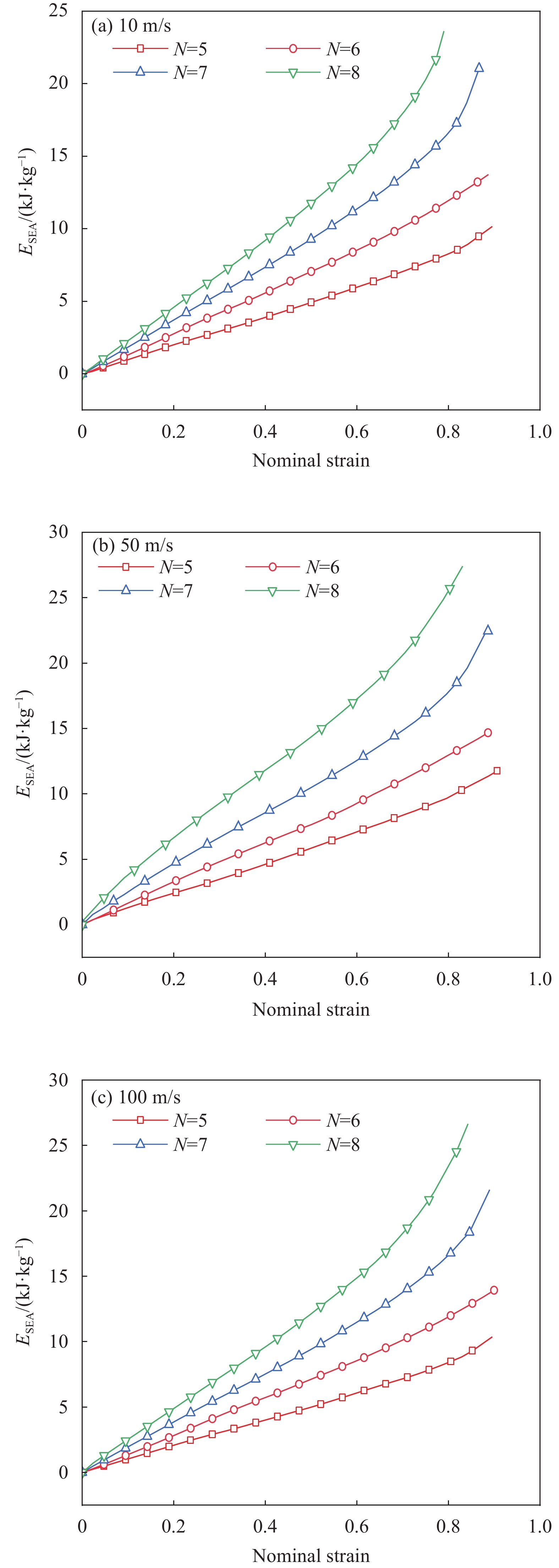
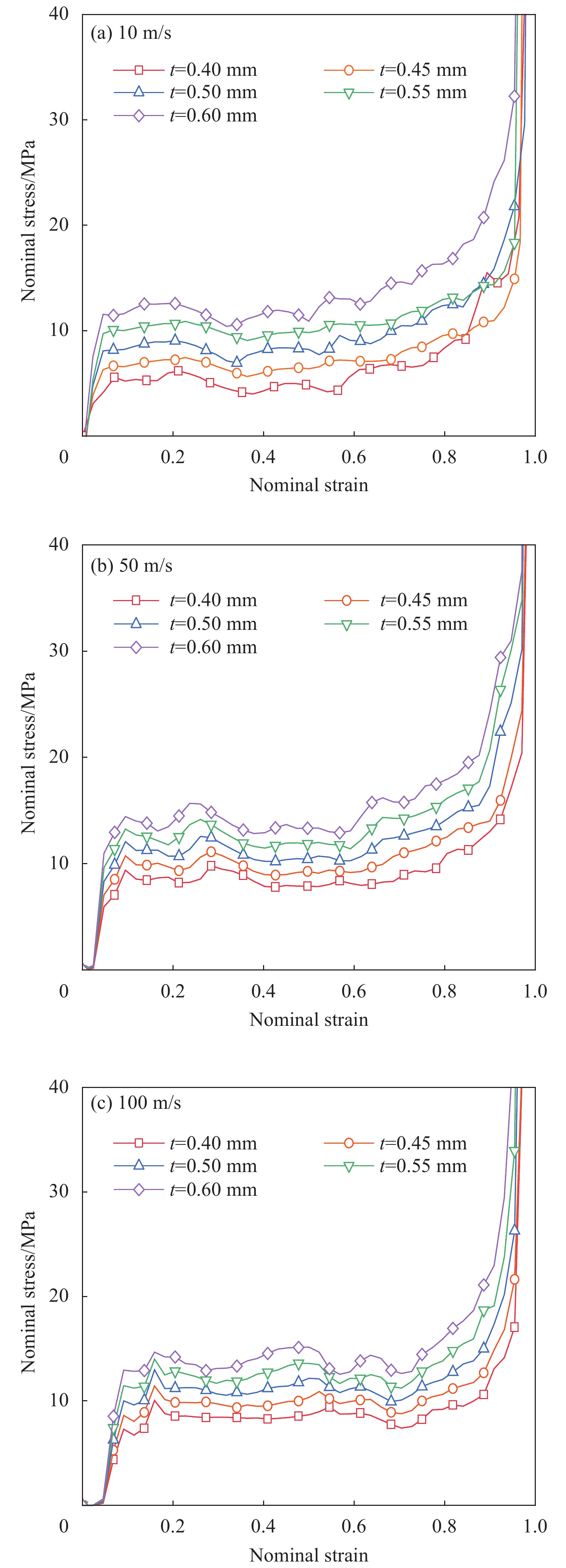
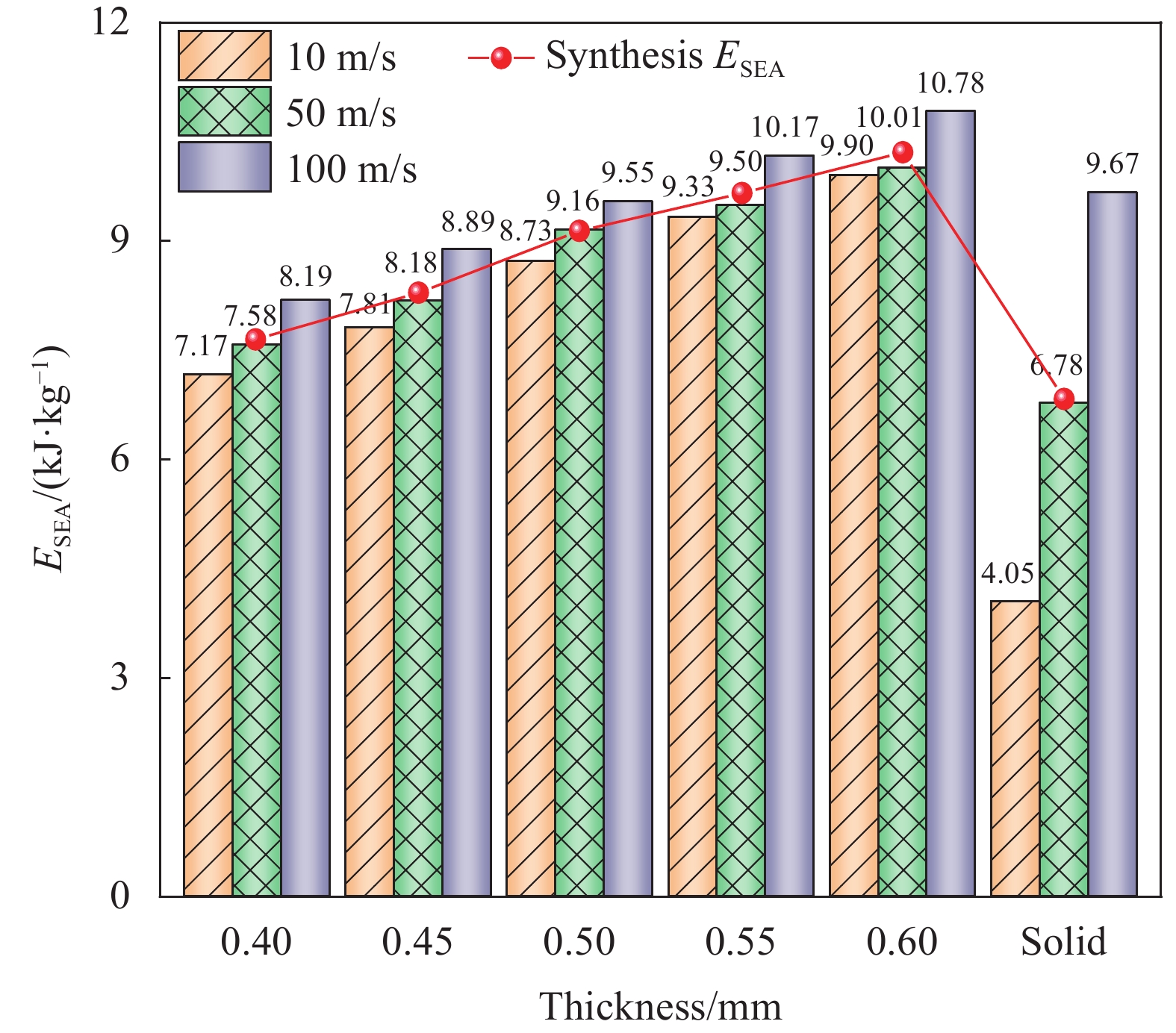
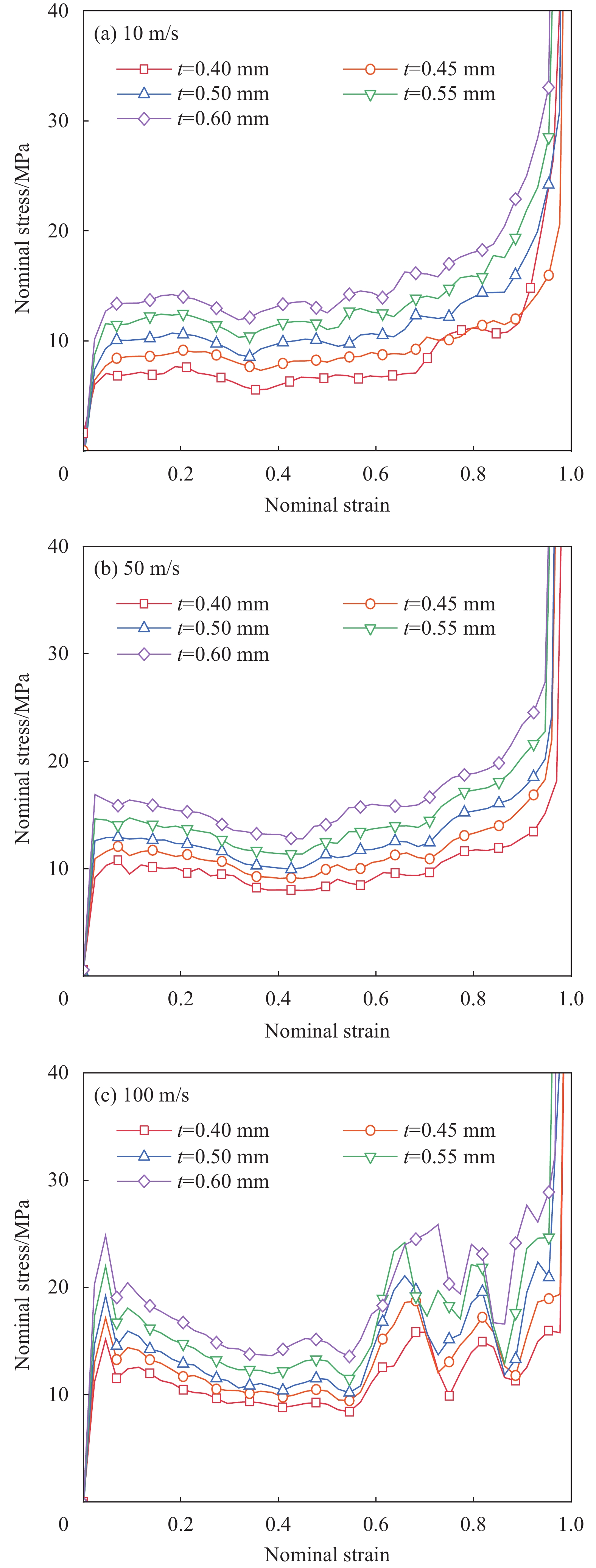
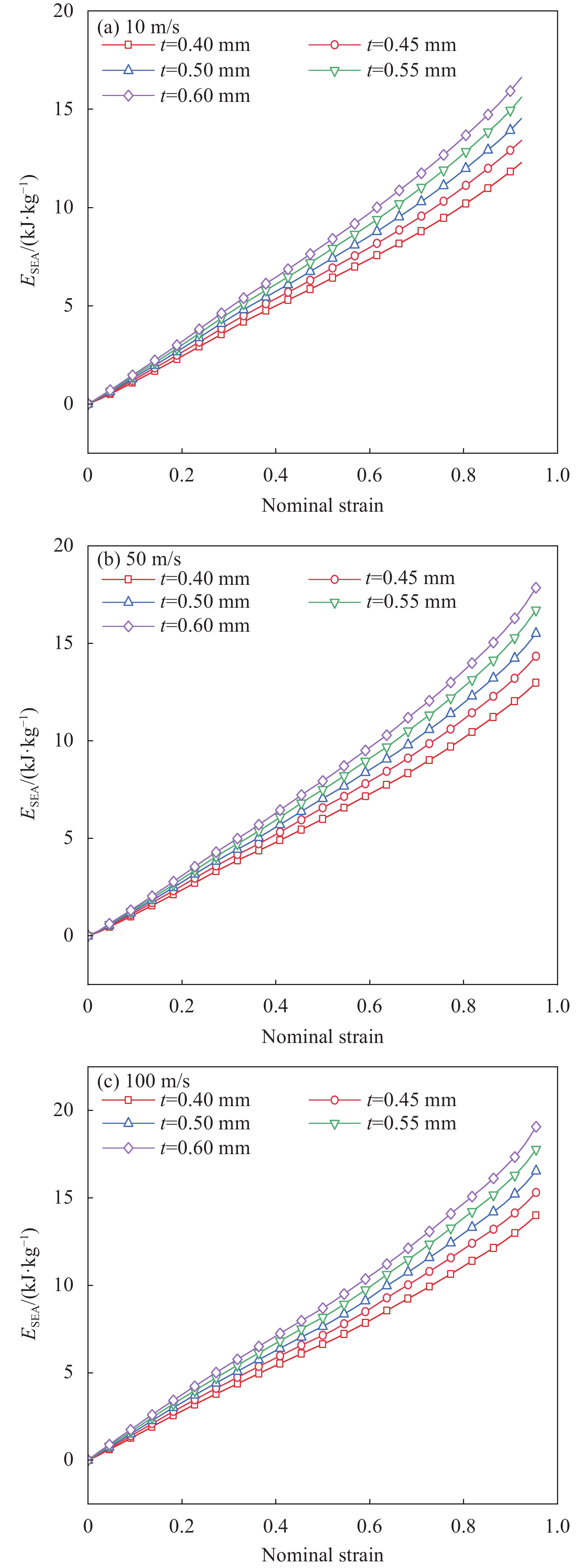
结果①数值模拟得到的压缩荷载作用下BBCLS整体变形模式与试验结果基本一致；同时从BBCLS力-位移曲线的试验和模拟对比结果，可以看出数值模拟结果变化趋势与试验结果吻合较好，其中初始峰值力与试验结果误差较小，为1.88%~4.38%，这也验证了数值模拟的可靠性。②BBCLS的动态吸能性能要优于其准静态压缩，这主要由于BBCLS的变形模式与冲击速度密切相关，随着冲击速度增加，惯性效应逐渐增强，更多胞元均匀协调地参与变形，使BBCLS抗压溃能力更强，同时更早到达密实阶段。此外，通过对BBCLS在不同冲击速度下冲击端及固定端名义应力-应变曲线的分析可知，BBCLS可以降低传递到被保护件的应力值，从而有效地保护结构不被破坏。冲击速度对于BBCLS平台应力有显著影响，冲击速度越大，冲击端平台应力越大，BBCLS受到冲击时吸能效果更好。与平台应力的变化相同，密实化应变与冲击速度的变化呈线性增大关系。然而，不同冲击速度作用下BBCLS的密实化应变相差不大(10m/s与200m/s冲击时仅差0.068)。这表明冲击速度对于BBCLS密实应变没有影响，而密实应变主要受结构内部胞元的影响。此外，不同冲击载荷下BBCLS均呈现出比准静态载荷下更优异的能量吸收能力，说明BBCLS更加适用于冲击载荷下的能量吸收。③通过与冲击荷载作用下传统的BCC对比发现，BBCLS表现出了优越的吸收能量特性，BBCLS的能量吸收总量与比能量吸收均为BCC型的5倍左右，显著优于BCC，且极大的缓解了BCC点阵结构节点处应力集中问题，这是由于BBCLS为曲面型结构，胞元之间由连续且光滑的表面过渡和连接，有效增加了结构实体部分应力分布的面积，使得应力可以迅速均匀地分布到结构内部，并且由于BBCLS内部存在孔隙，使得结构充分发挥出减震抗冲击性能。④随着冲击速度的提高，增大振幅、波纹数、胞壁厚度等微结构几何参数均使结构在受到冲击载荷时应力分布均匀，有效增加了冲击端的平台应力。此外，微结构几何参数的改变对结构比吸能以及综合比吸能有显著影响。
结论①与准静态压缩荷载变形模式不同，BBCLS在冲击载荷作用下的冲击过程可分为三种模式：低速冲击荷载下的均匀变形模式；中速冲击荷载下的过渡变形模式；高速冲击荷载下的动态变形模式。随着冲击速度的增大，BBCLS的初始应力峰值、平台应力和密实应变越大，能量吸收能力越强。②BBCLS在冲击荷载作用下的承载能力、吸能总量及比吸能均明显优于传统的BCC点阵结构，BBCLS不仅改善了传统BCC点阵结构在节点处应力集中的问题，且在承载能力与能量吸收性能方面表现出明显的优势。③随着冲击速度的增大，相同振幅的BBCLS冲击端平台应力会随着冲击速度提升而增大，且速度越高增幅越明显。④由于波纹数的增大，BBCLS的承载能力、刚度和吸能性均大幅度提高，BBCLS在相同冲击速度下的平台应力逐渐升高，波纹数为8时结构的平台应力最大。当波纹数为8时，冲击速度达到100m/s，相比于波纹数为5，冲击速度为10m/s结构在应变达到0.6的SEA提高201.36%。⑤随着胞壁厚度和冲击速度的增大，BBCLS的比吸能明显增大。其中胞壁厚度对BBCLS比吸能的增大作用更为显著。在一定程度上增大胞壁厚度可使结构冲击变形更加均匀，能够有效地提高BBCLS在不同冲击载荷作用下的吸能能力。在实际应用中，需要综合考虑结构轻量化设计不可盲目增大相对密度以达到更强的承载效果。
-
点阵结构具有良好抗冲击能力和能量吸收能力，在工程领域具有广泛的应用前景。尽管目前有关点阵结构的制备、设计和力学性能等方面取得了一定的研究进展，但是研究人员仍在不断探索结构更加复杂、抗冲击性能更加优越的点阵结构。近年来，仿生结构因在能量吸收能力上表现出比传统结构更加优异的性能，引起国内外学者广泛关注。其中，受雀尾螳螂虾螯抗冲击区域微观结构启发而提出的仿生双正弦波纹点阵结构(Bio-inspired bi-directional corrugated lattice structure，BBCLS)，在静态压缩性能和吸能方面展现出独特的优势。但是，目前对于其在冲击载荷下的变形失效机理尚不清楚。为了探究BBCLS的抗冲击性能，本文采用ANSYS/LSDYNA有限元分析软件建立了其在冲击荷载作用下的有限元数值模型，并基于已有的试验结果与数值模拟结果进行了对比，验证了该模型的有效性。在此基础上，研究了不同冲击速度对BBCLS的应力分布、变形模式、承载能力以及能量吸收特性的影响，并与传统体心立方点阵结构(BCC)进行了对比。同时利用该数值模型进一步分析了振幅、波纹数和胞壁厚度等微结构几何参数对BBCLS抗冲击性能的影响。研究结果表明：BBCLS在冲击荷载作用下的承载能力、吸能总量及比能量均明显优于传统的BCC点阵结构。BBCLS的冲击动力学响应主要与冲击速度和微结构几何参数有关。在低速冲击时，BBCLS呈现整体变形模式；中高速冲击时，结构向局部变形模式转换。随着冲击速度的提高，增大振幅、波纹数、胞壁厚度均使结构在受到冲击载荷时应力分布均匀，有效增加了冲击端的平台应力。研究结果为研究仿生点阵结构的冲击变形失效和吸能效果提供了力学依据。
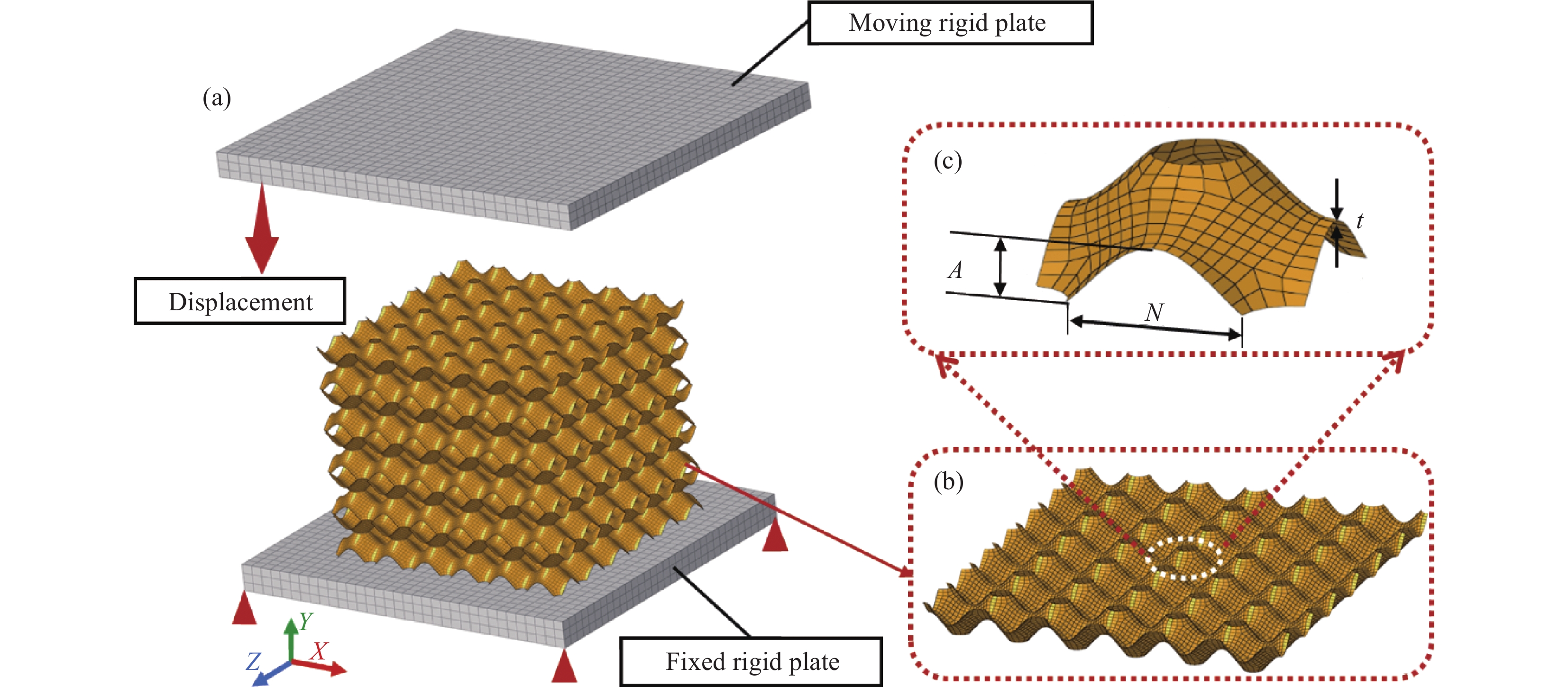
BBCLS有限元模型
不同冲击速度下的BBCLS在典型应变水平下的变形图
计量
- 文章访问数: 236
- HTML全文浏览量: 112
- PDF下载量: 12
- 被引次数: 4
