Design and mechanical analysis of a novel chiral honeycomb structure
-
摘要:
当前手性蜂窝结构的研究除了关注结构本身所用材料以外,通过改变单元内部拓扑组合以提升力学性能成为绝大部分研究的重点,而大部分现有的手性蜂窝结构中都存在既会带来更大的结构刚度、同时也会增加整体结构重量的刚性大中心节点。针对现状,本文提出了一种易变形、延展性好的新型四手性细胞结构,通过能量法理论推导了梁结构力学性能的数值解,并用有限元方法进行了数值验证。通过参数分析,讨论了该结构的力学性能。结果表明:该负泊松比结构具有优异的力学表现,等效弹性模量低至10−6,且拥有最低为−5.5的大拉剪耦合系数范围。其等效弹性模量最低仅有V型梁结构的10%,等效剪切模量低于ATCS结构2个数量级;力学性能调节范围也接近于ATCS的1.5至2倍。作为一种新型手性结构,更低的等效弹性模量与范围更广的拉剪耦合系数在航空航天、船舶、医疗等领域有着巨大的应用潜力。
Abstract:The current research on chiral honeycomb structures not only focuses on the materials used for the structure itself but also emphasizes improving mechanical performance by altering the internal topological arrangements of the units. Most existing chiral honeycomb structures feature rigid central nodes that increase both structural stiffness and overall weight. Addressing this situation, this paper proposed a novel tetra-chiral cell structure characterized by easy deformability and good extensibility. Theoretical deductions of beam structure mechanics using energy methods were presented, along with numerical validations using finite element analysis. Through parameter analysis, the mechanical performance of this structure was discussed. Results indicate that this structure, with a negative Poisson's ratio, demonstrates excellent mechanical properties. It exhibits an equivalent elastic modulus as low as 10−6 and a large range of shear coupling coefficients as low as −5.5. The equivalent elastic modulus is only 10% of that of a V-beam structure, and the equivalent shear modulus is lower by two orders of magnitude compared to an ATCS structure. The range of mechanical performance adjustment is also approximately 1.5 to 2 times that of the ATCS structure. As a new type of chiral structure, its lower equivalent elastic modulus and wider range of shear coupling coefficients present significant potential applications in aerospace, maritime, medical, and other fields.
-
目前,大量常规材料(木材、塑料、纤维等)存在易燃问题,需要进行阻燃处理。此外,这些常规材料在使用过程中无从感知外界情况,火灾发生时,无法及时向外界发出警报,需要依靠外界安装的火灾预警系统实现报警。2015年我国发布《建筑设计防火规范》,规定相关场所和部位必须安装火灾预警系统。目前商业火灾预警系统发展迅速,主要是基于传感器检测火灾现场温度、湿度、光照强度和物体燃烧后的烟气数据变化从而对现场进行监控,但也存在不少问题。由于火灾传感器往往与建筑材料分离,距离起火点有一定距离,导致火灾警报响应时间往往大于100 s[1],无法为火灾的及时扑救和人员撤离提供最佳时间。由于此类传感器无法面对大雨、大风、灰尘和腐蚀性湿气等苛刻外界环境,因此其在室外及开放公共场所的应用受到较大限制。鉴于上述问题,有必要缩短火灾预警系统的预警时间,实现超早期火灾探测报警。智能材料是一类具有内置或内在传感器、致动器和控制机构的材料,其能够以预定的方式和程度在短时间响应外界刺激,并在刺激被移除后迅速恢复到其原始状态[2]。智能材料打破了传统结构材料和功能材料的界限,应用领域广泛[3-6],前景诱人。随着智能材料的发展,智能涂层引起了研究者们极大的兴趣。智能涂层是一种人造的、能够对外部刺激有选择地提供最佳反应的涂层系统。将智能涂层引入传统建材领域,赋予各种材料智能响应功能,使其在使用过程中主动对外界“火灾”做出响应,将极大程度提高建筑的可靠性,对保障人员的生命及财产安全具有重大研究意义。
在各种基底上构建具有阻燃预警功能的纳米涂层,一方面大大降低了所需阻燃剂的添加量,避免了对聚合物基体性能的破坏;另一方面,火灾发生时,涂层对可燃材料起到有效的阻隔作用,使可燃材料隔热、隔氧,同时涂层可以智能地响应火灾时外部环境变化,从而大大提高火焰检测响应速度,有效改善火灾预警系统的可靠性。本文综述并讨论了近年来阻燃预警涂层的火灾预警机制、构筑策略及目前的研究进展。
1. 涂层火灾预警及阻燃机制
商业化的火灾预警依赖于火灾传感器对火灾现场环境的检测和反馈,现有阻燃预警涂层研究主要依赖于对火灾发生时温度变化的“识别和响应”,并将温度信号转化为可接收的电信号传递给外界,因此在涂层中构建能够只在材料着火时接通的通电回路是非常必要的(如图1所示)。目前,阻燃预警涂层主要是向涂层中引入具有热致响应变化的粒子,如氧化石墨烯(GO)、金属氧化物半导体纳米粒子等,依据功能粒子的差异,涂层的火灾预警机制可分为两大类,即化学反应型和物理型。
1.1 化学反应型涂层火灾预警机制
燃烧是可燃材料剧烈氧化的过程,材料表面达到着火温度时,许多化学物质会发生氧化还原反应,产生新的结构和物质。通过合理选择负载的功能粒子,便可在涂层表面着火时触发“扳机”,连通导电回路,实现对火灾的及时预警。
GO是氧化还原法合成石墨烯过程中的中间产物,其结构式如图2(a)所示,表面接枝了羟基、羧基、环氧基等含氧官能团[7-8],具有很好的水溶性和化学活性,由于在氧化过程中原本的石墨烯共轭网络受到严重官能化,GO的电阻显著高于石墨烯。当GO处于高温条件下时,其表面会脱去含氧官能团发生还原反应,从而使电阻降低,因此GO具有优异的温度-电阻变化,具有火灾预警潜力。
Xiao等[9]较早研究了热处理升温速率对GO结构及导电性的影响,结果表明,升温速率对GO结构有很大影响,且温度越高,GO结构越接近石墨结构,导电性越好,当热处理温度高于180℃时,处理后材料电导率可达1 S/m。侯若男等[10]研究了热还原温度对GO能带结构和电阻-温度特性变化规律的影响,首先在不同温度下(100~450℃)还原GO,得到不同还原程度的还原氧化石墨烯(rGO),以期制备不同还原程度的rGO阻温元件,并对其结构属性的变化进行表征分析,测试了其电阻-温度特性。结果表明,随着还原温度由100℃提高到450℃,GO含氧官能团逐渐脱失减少,缺陷增多;禁带宽度减小,由3.04 eV减小到2.4 eV;导电性增强,电阻由696.3 kΩ降至8 Ω,表现出负电阻-温度特性。阻温元件的电阻随着测试温度的升高而减小,表现出明显的半导体行为,且电导率与温度关系符合阿伦尼乌斯定理。
由于GO优秀的负电阻-温度变化机制,其在火灾预警涂层中具有优异表现,如图2(a)所示。将GO引入功能涂层后并在涂层中添加电极构成火灾预警回路。在正常情况下,由于GO较高的电阻率,导电回路并不连通;一旦火灾发生,材料表面温度达到着火温度(220~500℃)[11-12],涂层中的GO受热发生还原反应,表面含氧官能团(羟基、羧基等)逐渐脱失,着火点附近电极间的电阻迅速降低,导电回路接通,从而可实现对于火灾的及时预警[8]。
除GO外,一些其他物质的氧化还原反应同样可以构建阻燃预警涂层。Liu等[13]将聚乙烯醇(PVA)/AgNO3复合材料嵌入三聚氰胺海绵中(如图2(b)所示)。该海绵暴露在火焰中后,PVA还原AgNO3形成单分散性的银纳米颗粒,使整个材料由绝缘状态变成导电状态,可以迅速实现火灾报警。
表面活性剂是一类低添加量时即可显著降低溶液表面张力并改变体系界面状态的物质。通过在导电纳米粒子表面构建一层脂肪酸盐类的表面活性剂,可以有效改善纳米粒子的分散性。再将处理后的纳米粒子引入功能涂层后,也可以避免导电回路的形成。当火灾发生时,导电纳米粒子表面的脂肪酸盐分解,导电纳米粒子相互接触构成导电网络,可以有效实现火灾预警功能。Yu等[14]利用(3-氨基丙基)三乙氧基硅烷和二甲基十八烷基[3-(三甲氧基硅基)丙基]氯化铵与壬基酚聚氧乙烯醚硫酸钠的离子交换反应对导电多壁碳纳米管(MWCNT)进行接枝改性,制备活性MWCNT纳米流体,并将其涂覆于棉织物表面,成功构建了具有阻燃预警功能的涂层(响应时间约为21 s),如图2(c)所示。理论上,任何掺杂在涂料中可以形成导电回路的纳米粒子均可以采用表面活性剂处理以实现预警功能。
1.2 物理型涂层火灾预警机制
化学反应型阻燃预警涂层主要利用化学物质在高温下的反应来实现“一次性”触发的阻燃预警回路,与其作用机制不同,以金属氧化物半导体为主的半导体热敏材料是一类可以将温度信号转换为电信号的半导体材料,在温度探测和火灾监控方面具有很大潜力。
半导体材料是一类具有半导体性能(导电能力介于导体与绝缘体之间,电阻率约在10−3 Ω·cm~109 Ω·cm范围内),可用来制作半导体器件和集成电路的电子材料。以Fe、Mn、Co、Ni、Cu等过渡族金属的氧化物为例,如图3所示[15],此类材料均具有半导体性质,类似于Ge、Si晶体材料。低温情况下,此类材料体内的载流子(电子和空穴)数目少,电阻较高;随着温度升高,体内载流子数目增加,电阻值降低,表现为负温度系数热敏电阻特性。通过选择适当的金属氧化物半导体纳米粒子,将其引入功能涂层,在火灾发生时,随材料表面温度上升,作为热敏电阻的金属氧化物半导体电阻迅速减小,导电回路接通,从而实现对火灾的及时预警。
1.3 涂层阻燃机制
燃烧是一种剧烈的氧化过程,需要来自大气的O2作为氧化剂,可燃的基体作为燃料,这一过程才能持续进行下去。阻燃的关键在于阻止火焰与聚合物界面的降解行为,而这一目标往往通过向易燃材料添加阻燃剂实现。目前,阻燃剂的添加方式主要包括三类:(1)采用物理共混手段将阻燃剂掺入聚合物基体中,具有成本低、混合速度快的优点,但阻燃剂的有效载荷通常过高,会对材料的强度和弹性模量产生不利影响;(2)将含有阻燃功能的基团通过化学反应结合到基体上,使阻燃功能基团成为聚合物链的一个组成部分,该方法有利于提高阻燃效率并获得更高的耐久性;(3)采用防火涂料在材料表面构建阻燃涂层,该阻燃方式在各种商业应用中得到广泛应用。
常见的GO及金属氧化物半导体基火灾预警涂层,除了具有优异的热致响应变化特性,还具备优异的阻隔性能,能够同步赋予基体优异的阻燃性能。在涂层阻燃方面,GO具有突出的富碳结构,以GO为主的碳基纳米材料在阻燃方面具有优异表现。GO具有特殊的二维片层结构,将其添加到阻燃涂层后,可以形成致密的物理隔绝层,在燃烧过程中能够有效隔绝基底与热量和O2的接触。同时,GO在高温情况下热解,生成CO2和水蒸气,并形成更加致密连续的炭层结构,从而能够有效提高材料的阻燃性能。李洪飞等[16]以水性丙烯酸乳液为成膜物质,钛白粉为颜料,加入不同质量GO纳米粒子作为协效阻燃/抑烟剂配制膨胀型防火涂料,对涂层的阻燃和抑烟效果进行研究。结果表明,GO能有效提高涂层的耐燃时间并降低峰值生烟速率(pSPR)。当GO添加量达到涂料总量的0.025%时,涂层耐燃时间增加了59.5%。对涂层燃烧后炭层的结构形貌进行分析发现,具有片状结构的GO在涂料受热膨胀过程中会使自身和基体分子链取向,进而在聚合物炭化过程中形成骨架结构,增加炭层强度,达到阻燃和抑烟的目的。
金属氧化物半导体在聚合物阻燃方面同样具有优异表现,过渡族金属纳米粒子已经被证明有促进聚合物基体催化成炭的作用,同时具有良好的抑烟效果[17]。同时,为实现涂层的火灾预警功能,在材料表面构建出的致密金属氧化物涂层也能够很好地起到物理屏障作用,有效改善基体的阻燃性能。但考虑到单纯地添加金属纳米粒子对于材料的阻燃效力不足,过渡金属的纳米粒子(如硼酸锌、氧化镍等)常被作为磷氮系阻燃剂、碳基阻燃剂的协效剂使用,用于同步抑烟和提高阻燃效果[18-19]。
2. 基体的选择与涂层构建方式
2.1 涂层基体与传统涂层技术
建筑阻燃及火灾预警的智能涂层往往需要构建在木材、纺织品、塑料等易燃的高分子材料表面,而这些材料的表面特性差异对涂层构筑策略存在重大影响。木材、纺织品等天然高分子材料包含大量纤维素等天然多糖和天然蛋白,表面存在大量极性基团,能够轻易在表面构建牢固的涂层,而不需要考虑对基底进行复杂的预处理。而以聚烯烃为代表的塑料等合成高分子具有低的表面能和弱的界面层,表面难以浸润,缺乏构建涂层的反应位点,在其表面构建牢固涂层较困难,往往需要通过表/界面改性手段来增加表面能,改变界面的物理或化学组成,改善界面的几何性质和结晶形态,或是清除杂质和弱边界层,才能成功构建涂层。常见的表/界面改性手段包括化学处理[20]、等离子体处理[21]、交联聚合[22]等,用以提高材料表面能或额外赋予材料表面活性基团,从而成功构建涂层,处理较为繁琐复杂。
以木材为例,目前常见的功能涂层可以分为三类(如图4所示),包括:
(1)有机聚合物涂层,主要包括丙烯酸酯类、聚氨酯类有机涂料,通过共聚自身发生交联聚合,形成复杂的网状结构,可牢固地附着在木材表面,成膜性和黏附性较好,成膜方式简便易行,构成木材表面的保护层,还可以赋予木材抗紫外、防水、高强度、耐磨、装饰性等优点。从溶剂介质来看,此类涂料又可以分为溶剂型和水性涂料,水性涂料在涂覆固化过程中不会释放有机挥发成分,更符合环保需求。从涂覆工艺上看,此类涂层可以采用简单的涂刷、喷涂工艺,施工过程简单。从成膜工艺上看,在工业化生产过程中,此类涂层主要通过紫外光固化,结合强度牢固可靠。
(2)纳米粒子/聚合物复合涂层,通过向聚合物基体中添加纳米粒子,可以制备得到纳米粒子/聚合物复合涂层。相对于单一的有机聚合物涂层,通过选择性地引入纳米粒子构建复合涂层,材料的耐磨性、硬度、韧性和强度均得到一定程度的提高,同时还可赋予其抗老化、自清洁、抗菌防霉等新特性。包括SiO2、ZnO、TiO2、纳米黏土等多种纳米粒子均可作为功能粒子制备纳米粒子/聚合物复合涂层[23-26]。但无机纳米粒子在聚合物基底中的分散始终是一个问题。
Auclair等[27]通过预先将ZnO纳米颗粒分散在水中,从而改善与聚合物涂层的相容性。Zhao等[28]采用纳米晶纤维素(NCC)模板,通过原位聚合法合成纳米纤维素和纳米SiO2杂化胶体(NCC-SiO2)。将制备的NCC-SiO2杂化胶体引入水性聚丙烯酸酯(PAA)涂层。NCC模板可抑制纳米SiO2的聚集,使其均匀分布在PAA涂层中。此外,氢键交联网络的形成改善了NCC、SiO2和PAA界面相容性。与单独的NCC和纳米SiO2相比,NCC-SiO2杂化胶体对水性PAA涂层的力学性能和透光率有明显改善。
(3)无机纳米材料涂层,无机纳米粒子本身不具备很好的成膜性,因此无法通过简单的涂刷-固化过程将其构建在木材表面。目前在木材表面通过溶胶-凝胶法[29]和水热法[30]生长无机纳米粒子涂层的工艺较成熟。由于木材表面具有大量的羟基和管胞空隙,更利于无机纳米材料的附着和生长。但该方法工艺繁琐,处理成本昂贵,同时不适用于大尺寸试件的涂覆,因此当下无机纳米材料涂层的实用价值不高。
2.2 阻燃预警涂层构筑工艺
智能涂层的构筑不仅要考虑涂层基底的性质,还要考虑涂层成分的特性,两者的共同作用决定了阻燃预警涂层的构筑策略。材料表面涂层效果的好坏,除与基体和涂料本身的性能有关外,也与涂覆的工艺有直接联系。在这些工艺性能中,最重要的是涂层的附着力问题,包括涂层与基体的附着力及涂层内部的凝聚力。关于涂层与基体材料表面附着的原理很多,其中比较流行的理论有机械咬合黏接理论、静电理论、吸附理论、扩散理论、酸碱使用理论和化学键理论等。总之,附着力是机械连接、静电吸引和化学键合共同作用的结果。为提供与基体间更好的附着力,主要通过表面改性手段提高基底的表面能,赋予其更多的附着位点,从而实现牢固的涂覆。
目前阻燃预警涂层主要通过负载GO、金属氧化物半导体等纳米粒子来实现阻燃目的。尽管以有机聚合物为主体,功能粒子负载的涂层技术已经相对成熟,但考虑到上述功能粒子在有机涂层中的分散性及阻燃预警涂层的结构设计要求,上述的预警功能粒子不仅仅作为功能粒子,更是作为涂层的主体部分参与涂层的构建。为了在材料表面构建以功能粒子为连续相组分,且能与涂层基底牢固结合的阻燃预警涂层,涂层的构筑工艺不仅要考虑到功能粒子与基底附着力的问题,涂层本身的凝聚力问题也是关键。
从涂层功能粒子特性的角度出发,作为最常见的阻燃预警涂层功能粒子,GO表面存在大量电荷和活性基团,以其为主体的阻燃预警涂层有必要使这些电荷和活性基团参与到涂层的组装过程中。基于电荷驱动的层层自组装技术在阻燃预警涂层技术在构筑阻燃预警涂层方面表现出众。以GO为例,其表面存在大量电荷,可以通过电荷作用与基底形成离子键的作用,形成牢固涂层。在构筑含GO基的智能涂层方面具有突出优势。层层自组装涂层的制备通常涉及基底的表面电荷。首先,将基底暴露于含有聚合物或纳米粒子的水溶液中,利用基底表面电荷作用吸附溶液中带有能够与基底特定结合的聚合物或纳米粒子,时间从几秒到几分钟不等。然后,进行洗涤和干燥步骤,以去除任何松散黏附的材料。使一个单层沉积在一个表面上,这个表面也逆转了有效的表面相互作用,允许一种互补材料被沉积。此过程形成一个双层,可以根据需要重复多次以获得所需的厚度或性能。GO具有大量负电荷,且具有大量活性基团,将其与带有相反电荷的纳米粒子进行复配,通过层层自组装技术便可成功在基底表面构建含有GO的功能涂层。Wang等[31]利用层层自组装技术,将GO和PVA组装得到超薄多层薄膜。GO可以通过氢键作用与PVA交替自组装到石英衬底上,紫外-可见光谱和XRD结果表明,组装过程定量可重复。考虑到石墨烯具有优异的电性能、热性能和力学性能,可作为传统涂层填料的替代品,降低填料的添加量。Kulkarni等[32]通过层层自组装技术将GO构建进入厚度约为50 nm的聚电解质多层膜中。当GO添加量达到8%时,涂层的弹性模量由1.5 GPa增加至20 GPa,力学性能得到显著改善。由于层层自组装技术可以使GO以纳米涂层的形式均匀转移到基体表面,大大减少了所需GO的量,组装条件温和简单。但目前层层组装过程需要进行较多循环才能达到所需涂层厚度,过程较为繁琐,在GO基阻燃预警涂层的应用中存在一定局限。
GO特殊的片层结构上存在大量活性官能团,利用硅烷偶联剂接枝GO纳米片上的活性基团,可以利用化学键实现GO纳米片的定向交联,同时还能获得优于纯GO的物理性能,在构建GO基功能涂层方面非常常见。沈凯燕[33]将GO与几种硅烷偶联剂进行复合,对获得的复合材料进行一系列表征,发现在GO和硅烷偶联剂形成的复合材料中,复合材料具有良好的分散性,且在普通的布氏漏斗过滤下可得到纸状薄膜,复合材料的层间距增大,力学性能优于纯GO。楚景慧等[34]将羟基化处理后的镁合金交替在1,2-双(三乙氧基硅基)乙烷(BTSE)和GO溶液中反复浸渍,得到致密的多层自组装涂层,由于硅烷偶联剂与GO之间可以通过共价键和氢键连接,有效提高了GO层片之间的结合力,使涂层致密,显著改善了镁合金的耐腐蚀和耐磨性。此外,由于C、Si元素存在协效阻燃作用,因此硅烷偶联剂改性法制备的GO基阻燃预警涂层在阻燃方面具有更出众的表现。
金属氧化物纳米粒子易团聚,在涂料中分散不佳,且成膜性差,常规条件下无法单独形成功能涂层,无法通过常规方式直接转移到涂层中实现预期的阻燃预警功能。因此,以金属氧化物半导体为主的阻燃预警涂层主要通过溶胶-凝胶及水热合成法直接在基体表面“生成”。通过溶胶-凝胶法在预先经过处理的材料表面构建金属氧化物纳米粒子,可以有效避免纳米粒子分散性不佳带来的问题。Wang等[35]利用γ-甲基丙烯氧基丙基三甲氧基硅烷(MAPS)的辐射接枝聚合(RIGP)和随后的溶胶-凝胶原位矿化,在聚对苯二甲酸乙二醇酯(PET)织物上制备高耐久性、高强度的ZnO涂层。涂层的界面层则由Zn—O—Si和Si—O—Si共价键组成,不仅可以改善纳米ZnO与聚合物基体间的结合,也克服了无机粒子成膜能力差的缺点。图5为在材料表面构筑阻燃预警智能涂层的策略和方法。
3. 阻燃预警涂层的应用
随着智能材料概念的出现,阻燃预警涂层日益受到重视。基于GO、金属氧化物半导体等的阻燃预警涂层得到了广泛研究应用。
3.1 GO基阻燃预警涂层
GO兼具优异的阻燃和负电阻-温度特性,同时GO表面携带大量负电荷,具有亲水性,可以简单利用电荷组装技术或混入水性涂料将其构建入功能涂层中。操作方式简单、过程温和、环保,以GO为首的阻燃预警涂层出现较早,国内外均开展了广泛研究。图6为GO 基阻燃预警涂层。
最近,Wu等[36]在GO基阻燃预警涂层方面开展了一系列研究,基于硅烷偶联剂改性GO的构筑策略,在各种易燃材料(聚氨酯、棉织物、木材)表面构筑多层次的有机硅/GO涂层,并连接低压安全电源(<36 V)和报警灯,制备了火焰快速探测/预警传感器装置,涂层具有明显的温度响应电阻变化,可用于探测异常高温环境的传感器,从而在可燃材料着火温度以下实现防火。该涂层具有超疏水(水接触角约为156°)、良好的结构稳定性(火焰燃烧90 s后结构基本不变)、快速火焰探测响应时间(2~3 s)、优异的温度响应性(低于易燃材料的着火点温度)和出色的协同阻燃等特性。此外,上述涂层具有良好的耐候性能,将一些水滴滴到涂层表面(模拟下雨天),警报的响应时间几乎不发生变化,即便将这些样品放置在恶劣外界环境下长达180天后,警报时间也几乎不变,在公共场所和室外条件下的应用显示出相当潜力。由于GO基阻燃预警涂层的出色表现,Huang等[37]进一步以不同含烷氧基的硅烷分子在水溶液中通过水解和缩合与GO反应,组装成硅烷GO纸。与纯GO纸相比,硅烷GO纸具有良好的机械柔韧性、强的耐酸碱性、优异的阻燃性和较低硅烷含量下的热稳定性。此外,硅烷GO纸的火焰探测响应时间为1.6 s,附着在热敏电阻上时的火焰探测响应时间为5 s。此外,针对目前GO基阻燃预警涂层响应温度较高的问题,Zhang等[38]通过向涂层中引入抗坏血酸分子,用于改善涂层的火灾预警性能,成功实现涂层在较低温度下(120℃)的火灾预警功能。此外,为进一步改善涂层的阻燃性能,Guo等[39]还制造了一种聚磷酸铵(APP)/GO/四氢全氟癸基三甲氧基硅烷(TFTS)杂化涂料。由于在燃烧过程中形成了一层均匀覆盖且致密的含有P-Si的还原石墨烯层,这种涂料对于高温火焰表现出极为敏感的温度响应电阻变化。同时,硅烷和APP分子在涂层中呈现选择性分布,从而形成具有低水亲和力的微/纳米粗糙表面,可实现超疏水性(水接触角约为158.4°),是一种绿色多功能涂料。Huang等[40]进一步通过选择硅烷偶联剂3-巯基丙基三甲氧基硅烷(MPTS)与GO搭配制备MPTS-GO纸。研究发现,在高温火焰下,MPTS-GO纸表面形成了一层致密的纳米SiO2微粒层,这层纳米SiO2层可阻挡外界热量向内部GO的传递,同时促进GO层碳化。由于含有巯基的MPTS分子促进了高温下GO的热还原,MPTS-GO可在1 s内反应并触发火灾警报装置,同时,将MPTS-GO纸暴露在室外一年或放在水中几分钟,依旧可以在短时间内触发火灾报警,表明MPTS-GO纸在恶劣环境下依旧可以显示出优良的火灾预警功能和结构稳定性。
基于电荷作用驱动的层层自组装技术在构筑含GO基的智能涂层同样研究广泛。Xie等[8]在羟丙基甲基纤维素(HMC)上接枝脲基嘧啶酮(UPy)基团,合成了功能性纤维素(FC)。随后,利用GO和FC通过一步自组装制备新型多功能阻燃纳米涂层。制备出珍珠状纳米涂层赋予多种可燃材料(包括聚丙烯、聚氨酯泡沫和木材)特殊的防火安全性。在燃烧实验中,所有涂覆材料均实现自熄灭。当遇到火灾时,纳米涂层迅速形成稳定的多条导电路径,并在3 s内触发火灾报警灯。此外,由于UPy基团的引入,涂层在常温下同时具备自愈合性能。但基于GO/石墨烯的火灾报警电路只有在两电极之间的GO纳米片快速受热还原时才能显示灵敏的火灾报警响应。受此限制,目前的火灾预警涂层仍限于小尺寸使用,难以满足实际需求。为解决这一问题,Xie等[41]进一步采用喷涂法制备了一种基于GO、银纳米线和氟化物聚乙烯醇缩丁醛(FPVB)的具有超灵敏火灾报警和超疏水性能的三明治型纳米阻燃涂料,通过将银纳米线和FPVB溶于丁醇,预先在沉积GO/FC前将该溶液喷涂于基底上,并在阻燃预警涂层沉积完成后再次喷涂一层含银纳米粒子的导电层,构建了三明治型的多层阻燃预警涂层。通过在GO/FC阻燃预警涂层上下两面附加含有银纳米线的导电涂层,相当于将原本阻燃预警涂层报警回路的电极安装在整个涂层上,火灾发生时任意着火点两端电极的距离均为微米级,从而解决了阻燃预警涂层大尺寸化的问题,同时在更大的基体表面(300 mm×50 mm)实现更灵敏的火灾预警(0.83 s),此外疏水的保护层也有效解决了原本涂层的亲水流失问题,如图6(c)所示。
Chen等[42]采用简单的逐层组装方法,在木浆纸(WPP)表面构建了苯氧基环磷腈功能化GO (FGO)和壳聚糖功能化碳纳米管(CNTs)的多层涂层,FGO/CNTs杂化结构在不降低WPP内在柔韧性的前提下,具有良好的力学性能和阻燃性能。同时,FGO/CNTs杂化结构的电阻对火焰和温度高度敏感,使其成为理想的火焰传感器。柔性FGO/CNTs杂化结构包覆WPP传感器在乙醇火焰或热处理条件下燃烧时具有良好的形态保持能力和阻燃性能,在点燃可燃材料前几秒钟内可感测到温度的升高。此外,在构建复合导电网络时,可以通过改变CNTs含量来调节火灾响应时间。
最近,Chen等[43]以超长羟基磷灰石纳米线(HNs)和GO混合制备了耐火无机火灾智能报警墙纸,室温下,GO处于电绝缘状态,当遭遇火灾时,高温使GO发生还原反应,壁纸则发生由电绝缘到导电状态的改变,从而触发报警装置。通过使用聚多巴胺作为还原剂和封端剂,GO热敏传感器的灵敏度和阻燃性得到提高,同时实现在低温(126.9℃)下的火灾快速响应(2 s)及长时间报警(5 min)的优异特性。
3.2 金属氧化物半导体阻燃预警涂层
金属氧化物半导体在阻燃预警涂层方面的研究值得关注。Zhang等[15]采用溶胶-凝胶法,在聚丙烯无纺布表面逐层组装Fe3O4和鱼鳞状银纳米片,成功构建了具有阻燃预警功能的阻燃预警涂层,可实现低温下的火灾响应(<100℃),同时具有灵敏的火灾预警速度(2 s),持续响应时间可达15 min,同时涂覆后,织物具有较高的机械灵活性和坚固性,可以容易地裁剪成各种形状而不影响其阻燃预警性能,作为商业化的火灾预警系统存在一定研究价值。
3.3 其他阻燃预警涂层
Yu等[14]采用(3-氨基丙基)三乙氧基硅烷和二甲基十八烷基[3-(三甲氧基硅基)丙基]氯化铵同时对MWCNT进行表面改性,然后将其与壬基酚聚氧乙烯醚进行离子交换反应,成功合成了具有软玻璃流变性的MWCNT纳米流体,并通过简单喷涂将MWCNT纳米流体涂覆于棉织物表面。结果表明,MWCNT纳米流体的加入在提高棉织物导热性的同时,还保持了棉织物的电绝缘性。MWCNT纳米流体/棉织物的最大导热系数是棉织物的2.42倍。表面接枝的非导电硅烷分子和MWCNT的有机离子盐阻碍了MWCNT的相互接触,形成了保持电绝缘的导电网络。此外,在棉织物燃烧过程中,表面接枝的MWCNT纳米流体有机分子开始分解,从而促进MWCNT导电网络的形成,表现为电流的存在,作为一种低压直流电源在火灾报警传感器中具有潜在应用价值。
最近,Liu等[44]利用蚕丝纤维蛋白和钙离子的螯合作用制备了一种环保的水凝胶。常温条件下,该水凝胶具有较高的电阻率,当温度上升(<200℃)时,水和钙离子在凝胶中的迁移速度增加,凝胶电阻迅速减小,从而可以快速触发火灾报警系统(约2 s),同时水凝胶具有优异的阻燃性能,当钙离子含量达到25%时,水凝胶的极限氧指数为43%,UL-94等级为V-0级。此种水凝胶材料具有优异的附着性能,可作为涂层涂覆在多种材料(纸张、木材、纺织品)表面,为阻燃预警涂层的构筑提供新的思路。
4. 阻燃预警涂层的耐候和耐磨性能
随着阻燃预警涂层研究的不断发展,其在公共场所和室内环境的应用逐渐提上议程。作为智能涂层,涂层的破损和老化即意味着涂层保护和预警功能的丧失,因此有必要探讨阻燃预警涂层使用过程中的耐老化和耐磨擦性能,但目前关于阻燃预警涂层耐候和耐磨性能仍缺乏系统研究。根据阻燃预警功能粒子的差异,以GO、金属氧化物半导体等为基体的阻燃预警涂层在耐老化和耐磨擦性能方面具有不同表现。
GO为具有单原子厚度的二维碳原子晶体,机械性能优异,摩擦系数低,具有电绝缘性,常被用于改善各类材料的耐磨性能[45-46],具有出众的表现。Zhang等[45]采用微弧氧化法在镁锂合金表面制备了含GO涂层,含GO涂层的致密性、厚度和硬度均高于游离GO涂层。当在不锈钢球上滚动时,其表现出优异的抗磨擦和耐磨损性能。为获得更加优异的耐磨性能,GO常与硅烷偶联剂混合使用在材料表面制备耐磨涂层。如上文所述,汤龙程课题组[36-40]以硅烷偶联剂改性GO在多种材料表面制备了阻燃预警涂层,此类涂层具有优秀的耐候性能,即使是经过水浸或暴露在室外长达1年的时间,仍能保持其原本的阻燃预警性能。虽未对涂层的耐磨性能进行测试,但参考硅烷偶联剂改性GO在其他材料表面的耐磨作用,硅烷偶联剂改性GO基阻燃预警涂层的耐磨性能应当具有出色表现。与之相对的,基于层层自组装技术得到的GO基阻燃预警涂层的构筑过程主要依靠电荷相互作用实现,涂层的牢固程度比不上硅烷偶联剂改性GO基阻燃预警涂层,且涂层存在亲水流失问题,其耐候和耐磨损表现相对并不出众。Xie等[41]通过在此类涂层表面额外构建保护层的方式,改善了涂层的流失性,一定程度上改善了其耐候性能。
在材料表面均匀生长而成的金属氧化物纳米涂层具有优异的耐磨性能。Xue等[47]借助等离子体增强原子层沉积辅助水热表面工程技术在3D订制耳塞的表面构建了均匀排列的纳米ZnO阵列,在使用数周后,大部分ZnO纳米阵列仍保持均匀致密,仅出现少量小面积裂纹,表明所得涂层具有优异的耐磨性能。在耐候性能方面,金属氧化物性能同样值得期待。高鹤等[48]采用提拉涂膜法,采用ZnO溶胶及TiO2溶胶在杨木表面构建了保护性纳米金属氧化物涂层,紫外老化试验表明,涂层显著改善了木材的耐候性能。可以预见,通过合理选择金属氧化物在材料表面构建致密的涂层,将可以在实现阻燃预警功能的同时改善材料的耐候性能和耐磨性能。
5. 结束语
阻燃预警涂层技术应用前景诱人,目前基于碳基纳米材料的阻燃预警涂层已有较多研究,基于表面活性剂和半导体等的阻燃预警技术也相应被研发出来。但受限于阻燃预警涂层本身的结构特点,大尺寸涂层的响应仍然十分困难。此外,阻燃预警涂层对涂层本身在使用过程中的稳定性提出更高要求。
综合考虑成本及不同领域对阻燃预警涂层的需求,当今及未来阻燃预警涂层技术仍将关注以下几点:
(1)遴选适宜的阻燃预警功能粒子,简化阻燃预警涂层涂覆工艺,降低生产加工成本,使其满足工业化大规模生产的需求;
(2)实现阻燃预警涂层的多功能化,通过额外引入功能粒子或调整涂层工艺及结构,赋予涂层大尺寸化响应、自修复、耐磨损等性能,进一步推动其工业化进程;
(3)进一步探明阻燃预警涂层的响应原理及调控机制,推动智能涂层技术的发展;
(4)将阻燃预警涂层构建于其他智能材料表面,探索多种智能材料间的协同效果,探索制备复合智能材料;
(5)结合阻燃预警涂层技术和物联网技术,进一步推动其在智能家居和公共安全领域的应用。
-
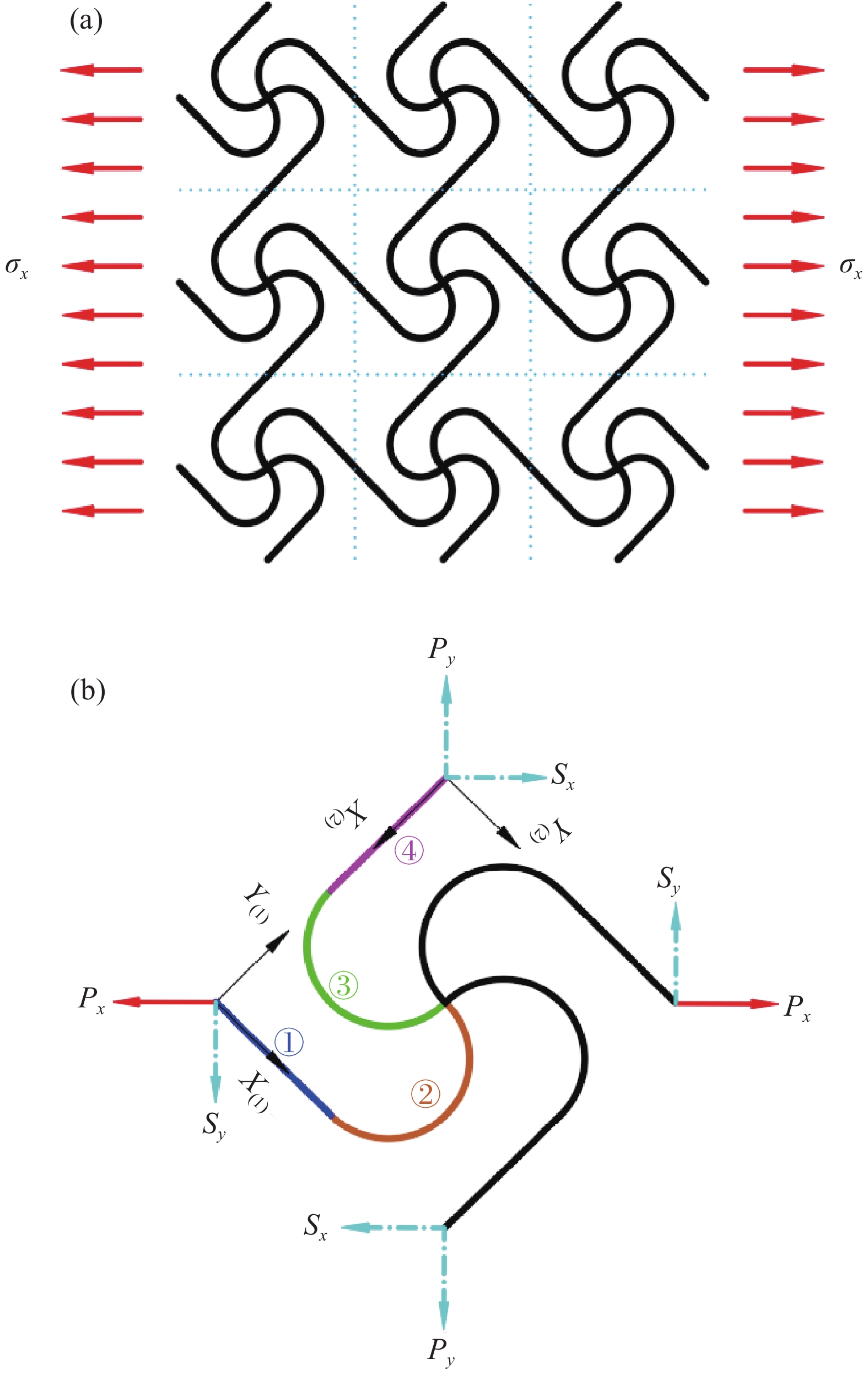
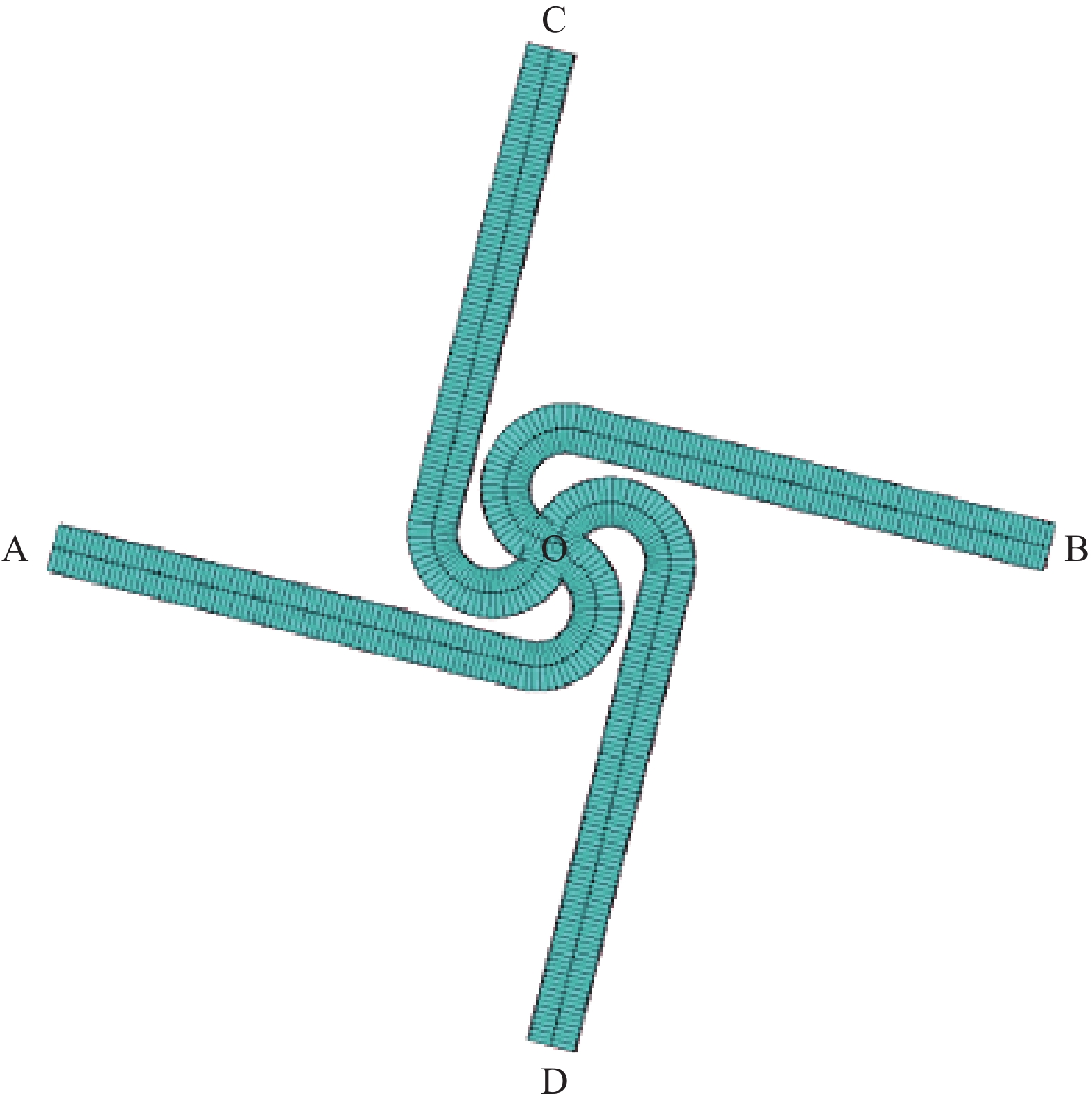
表 1 有限元仿真载荷与边界条件
Table 1 Load and boundary conditions used in the finite element simulation
Conditions Tensile load in the X direction Shear load Load condition Ux(A)=−εx2×H
Ux(B)=εx2×H
Uy(O)=0Ux(C)=−εx2×H
Ux(D)=εx2×H
Uy(O)=0z-direction SYMM Periodic condition Ux(C)=Ux(D)
Uy(C)=Uy(D)
θz(A)=θz(B)
θz(C)=θz(D)Uy(A)=Uy(B)
θz(A)=θz(B)
θz(C)=θz(D) -
[1] Daynes S, Weaver P M. Review of shape-morphing automobile structures: concepts and outlook[J]. Proceedings of the Institution of Mechanical Engineers, Part D: Journal of Automobile Engineering, 2013, 227(11): 1603-1622. DOI: 10.1177/0954407013496557
[2] Bubert E A, Woods B K S, Lee K, et al. Design and fabrication of a passive 1D morphing aircraft skin[J]. Journal of intelligent material systems and structures, 2010, 21(17): 1699-1717. DOI: 10.1177/1045389X10378777
[3] Qiu K, Wang Z, Zhang W. The effective elastic properties of flexible hexagonal honeycomb cores with consideration for geometric nonlinearity[J]. Aerospace Science and Technology, 2016, 58: 258-266. DOI: 10.1016/j.ast.2016.08.026
[4] 张大海. 冲击载荷下蜂窝及其夹芯结构力学行为研究[D]. 东南大学, 2018. Zhang Dahai. Study on the Mechanical Behavior of Honeycomb and Its Sandwich Structure under Impact Load[D]. Southeast University, 2018(in Chinese).
[5] 吴鹤翔, 刘颖. 梯度变化对密度梯度蜂窝材料力学性能的影响[J]. 爆炸与冲击, 2013, 33(2): 163-168. DOI: 10.3969/j.issn.1001-1455.2013.02.008 Wu Hexiang, Liu Ying. The Influence of Gradient Variation on the Mechanical Properties of Density Gradient Honeycomb Materials[J]. Explosion and Impact, 2013, 33(2): 163-168(in Chinese). DOI: 10.3969/j.issn.1001-1455.2013.02.008
[6] 赵畅, 周丽, 邱涛. 柔性铰可变形蜂窝结构的面内拉伸性能[J]. 复合材料学报, 2024, 42: 1-9. Zhao Chang, Zhou Li, Qiu Tao. In-plane tensile properties of flexible Hinge deformable honeycomb structures[J]. Journal of Composites, 2024, 42: 1-9(in Chinese).
[7] 吴文旺, 肖登宝, 孟嘉旭, 等. 负泊松比结构力学设计、抗冲击性能及在车辆工程应用与展望[J]. 力学学报, 2021, 53(3): 611-638. DOI: 10.6052/0459-1879-20-333 Wu Wenwang, Xiao Dengbao, Meng Jiaxu, et al. Mechanical Design, Impact Resistance Performance, and Prospects for Application in Vehicle Engineering of Negative Poisson's Ratio Structures[J]. Acta Mechanica Sinica, 2021, 53(3): 611-638(in Chinese). DOI: 10.6052/0459-1879-20-333
[8] Ajaj R M, Parancheerivilakkathil M S, Amoozgar M, et al. Recent developments in the aeroelasticity of morphing aircraft[J]. Progress in Aerospace Sciences, 2021, 120: 100682. DOI: 10.1016/j.paerosci.2020.100682
[9] Qiu K, Wang Z, Zhang W. The effective elastic properties of flexible hexagonal honeycomb cores with consideration for geometric nonlinearity[J]. Aerospace Science and Technology, 2016, 58: 258-266. DOI: 10.1016/j.ast.2016.08.026
[10] 刘旭畅, 李爽, 杨金水. 一种新型手性负泊松比结构的减振性能[J]. 复合材料学报, 2024, 41(1): 477-484 DOI: 10.13801/j.cnki.fhclxb.20230609.001 Liu Xuchang, Li Shuang, Yang Jinshui. A new type of structure of chiral negative poisson's ratio of vibration reduction performance[J]. Journal of composite materials, 2024, 41(1): 477-484 DOI: 10.13801/j.cnki.fhclxb.20230609.001
[11] Papka S D, Kyriakides S. In-plane crushing of a polycarbonate honeycomb[J]. International Journal of Solids and Structures, 1998, 35(3-4): 239-267. DOI: 10.1016/S0020-7683(97)00062-0
[12] Ashby M F, Gibson L J. Cellular solids: structure and properties[J]. Press Syndicate of the University of Cambridge, Cambridge, UK, 1997: 175-231.
[13] Masters I G, Evans K E. Models for the elastic deformation of honeycombs[J]. Composite structures, 1996, 35(4): 403-422. DOI: 10.1016/S0263-8223(96)00054-2
[14] Xu M, Liu D, Wang P, et al. In-plane compression behavior of hybrid honeycomb metastructures: Theoretical and experimental studies[J]. Aerospace Science and Technology, 2020, 106: 106081. DOI: 10.1016/j.ast.2020.106081
[15] M Wagner, T Chen, et al. Large Shape Transforming 4D Auxetic Structures[J]. 3D Printing and Additive Manufacturing, 2017, 4(3): 133-141. DOI: 10.1089/3dp.2017.0027
[16] Gang X Z , Xin R , Wei J , et al. A novel auxetic chiral lattice composite: Experimental and numerical study[J]. Composite Structures, 2022, 282.
[17] Yi Z , Xin R , Wei J , et al. In-plane compressive properties of assembled auxetic chiral honeycomb composed of slotted wave plate[J]. Materials & Design, 2022, 221.
[18] Dirrenberger J, Forest S, Jeulin D. Effective elastic properties of auxetic microstructures: anisotropy and structural applications[J]. International Journal of Mechanics and Materials in Design, 2013, 9: 21-33. DOI: 10.1007/s10999-012-9192-8
[19] Tabacu S, Negrea R F, Negrea D. Experimental, numerical and analytical investigation of 2D tetra-anti-chiral structure under compressive loads[J]. Thin-Walled Structures, 2020, 155: 106929. DOI: 10.1016/j.tws.2020.106929
[20] 任鑫, 张相玉, 谢亿民. 负泊松比材料和结构的研究进展[J]. 力学学报, 2019, 51(3): 656-687. DOI: 10.6052/0459-1879-18-381 Ren Xin, ZHANG Xiangyu, Xie Yimin. Research progress of materials and structures with negative Poisson ratio[J]. Chinese Journal of Mechanical Mechanics, 2019, 51(3): 656-687(in Chinese). DOI: 10.6052/0459-1879-18-381
[21] Ren X , Das R , Tran P , et al. Auxetic metamaterials and structures: a review[J]. Smart Materials and Structures, 2018, 27(2):
[22] Sha Z D, She C M, Xu G K, et al. Metallic glass-based chiral nanolattice: Light weight, auxeticity, and superior mechanical properties[J]. Materials Today, 2017, 20(10): 569-576. DOI: 10.1016/j.mattod.2017.10.001
[23] Lorato A, Innocenti P, Scarpa F, et al. The transverse elastic properties of chiral honeycombs[J]. Composites Science and Technology, 2010, 70(7): 1057-1063. DOI: 10.1016/j.compscitech.2009.07.008
[24] Lu X, Tan V B C, Tay T E. Auxeticity of monoclinic tetrachiral honeycombs[J]. Composite Structures, 2020, 241: 112067. DOI: 10.1016/j.compstruct.2020.112067
[25] Yang P , Gang X Z , Dong H , et al. The out-of-plane compressive behavior of auxetic chiral lattice with circular nodes[J]. Thin-Walled Structures, 2023, 182(PA):
[26] Chen Y J, Scarpa F, Liu Y J, et al. Elasticity of anti-tetrachiral anisotropic lattices[J]. International Journal of Solids and Structures, 2013, 50(6): 996-1004. DOI: 10.1016/j.ijsolstr.2012.12.004
[27] Ma Q, Cheng H, Jang K I, et al. A nonlinear mechanics model of bio-inspired hierarchical lattice materials consisting of horseshoe microstructures[J]. Journal of the Mechanics and Physics of Solids, 2016, 90: 179-202. DOI: 10.1016/j.jmps.2016.02.012
[28] Liu W, Li H, Yang Z, et al. In-plane elastic properties of a 2D chiral cellular structure with V-shaped wings[J]. Engineering Structures, 2020, 210: 110384. DOI: 10.1016/j.engstruct.2020.110384
[29] Weidong L, Zhendong Y, Jiong Z. The in-plane tensile and shear properties of novel chiral cellular structures[J]. Mechanics of Advanced Materials and Structures, 2022, 29(27): 5933-5952. DOI: 10.1080/15376494.2021.1969607
[30] Mousanezhad D, Haghpanah B, Ghosh R, et al. Elastic properties of chiral, anti-chiral, and hierarchical honeycombs: A simple energy-based approach[J]. Theoretical and Applied Mechanics Letters, 2016, 6(2): 81-96. DOI: 10.1016/j.taml.2016.02.004
[31] Zhu Y, Jiang S, Lu F, et al. A novel enhanced anti-tetra-missing rib auxetic structure with tailorable in-plane mechanical properties[J]. Engineering Structures, 2022, 262: 114399. DOI: 10.1016/j.engstruct.2022.114399
[32] Yang C, Yang K, Tian Y, et al. Theoretical analysis on the stiffness of compression–torsion coupling metamaterials[J]. Extreme Mechanics Letters, 2021, 46: 101336. DOI: 10.1016/j.eml.2021.101336
[33] Zhang Z W, Tian R L, Zhang X L, et al. A novel butterfly-shaped auxetic structure with negative Poisson’s ratio and enhanced stiffness[J]. Journal of Materials Science, 2021, 56(25): 14139-14156. DOI: 10.1007/s10853-021-06141-4
[34] 王雪松, 刘卫东, 刘典. 新型反四手性蜂窝结构的面内拉伸弹性[J]. 复合材料学报, 2023, 40(8): 4849-4861. Wang Xuesong, LIU Weidong, LIU Dian. New four chiral honeycomb structure of in-plane tensile elasticity[J]. Journal of composite materials, 2023, 40(8): 4849-4861 (in Chinese).
-
期刊类型引用(7)
1. 刘瑜,盛德星,王辉,王玲,李茜,胡涛,李永. 紫外-冷凝老化对碳纤维/环氧树脂复合材料性能的影响. 装备环境工程. 2025(01): 196-202 .  百度学术
百度学术
2. 肖瀚瑶,张勇,王安东,樊伟杰. SO_2盐雾与冲击载荷交替作用下AerMet100钢损伤研究. 装备环境工程. 2025(02): 1-11 .  百度学术
百度学术
3. 秦国锋,秦旺招,糜沛纹,李铭,范秋寒. 复合材料在湿-热-载荷作用下的加速老化与自然老化研究综述. 交通运输工程学报. 2024(05): 173-194 .  百度学术
百度学术
4. 张柱柱,陈跃良,姚念奎,卞贵学,张勇,张杨广. 冲击载荷作用下38CrMoAl渗氮钢损伤机理和耐腐蚀性能. 航空学报. 2021(05): 199-210 .  百度学术
百度学术
5. 王安东,卞贵学,张勇,陈跃良,张柱柱,张杨广. 海洋环境下G814/3233复合材料的老化机理及加速老化与自然老化的相关性. 航空学报. 2021(05): 250-262 .  百度学术
百度学术
6. 张勇,王安东,陈跃良,樊伟杰,马瑞民. CF8611/AC531复合材料的实验室加速老化行为研究. 装备环境工程. 2020(05): 116-121 .  百度学术
百度学术
7. 高超干,周储伟. 复合材料环境当量等效加速老化试验方法. 工程塑料应用. 2020(08): 103-107+117 .  百度学术
百度学术
其他类型引用(2)
-
其他相关附件
-
本文图文摘要
点击下载
-
-
目的
当前手性蜂窝结构的研究除了关注结构本身所用材料以外,通过改变单元内部拓扑组合以提升力学性能成为绝大部分研究的重点,而大部分现有的手性蜂窝结构中都存在既会带来更大的结构刚度、同时也会增加整体结构重量的刚性大中心节点,所以在对结构柔性需求较高的工程领域,仍需开发具有更高变形能力的结构。针对现状,本文提出了一种易变形、延展性好的新型四手性细胞结构,研究分析该结构的力学性能。
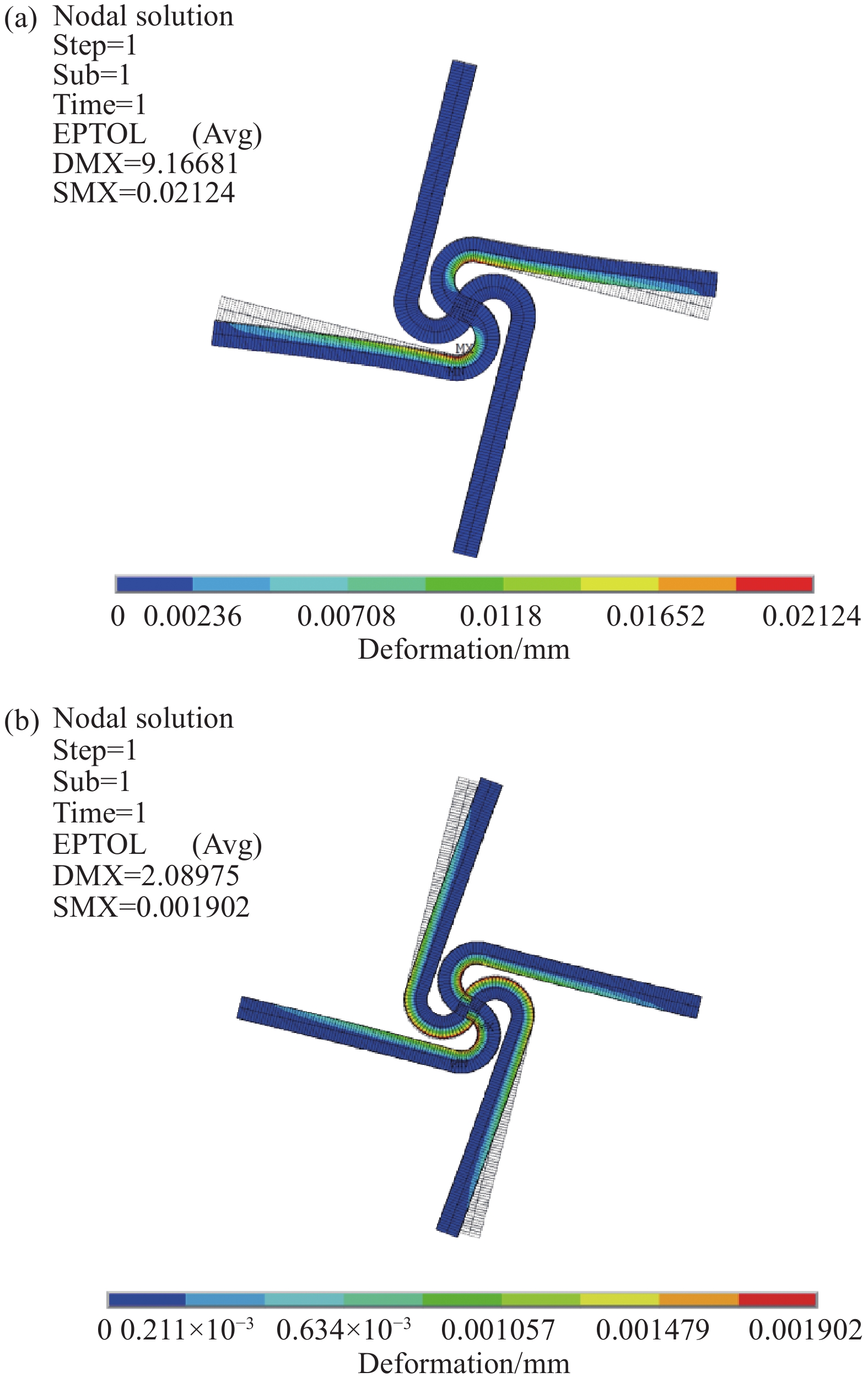
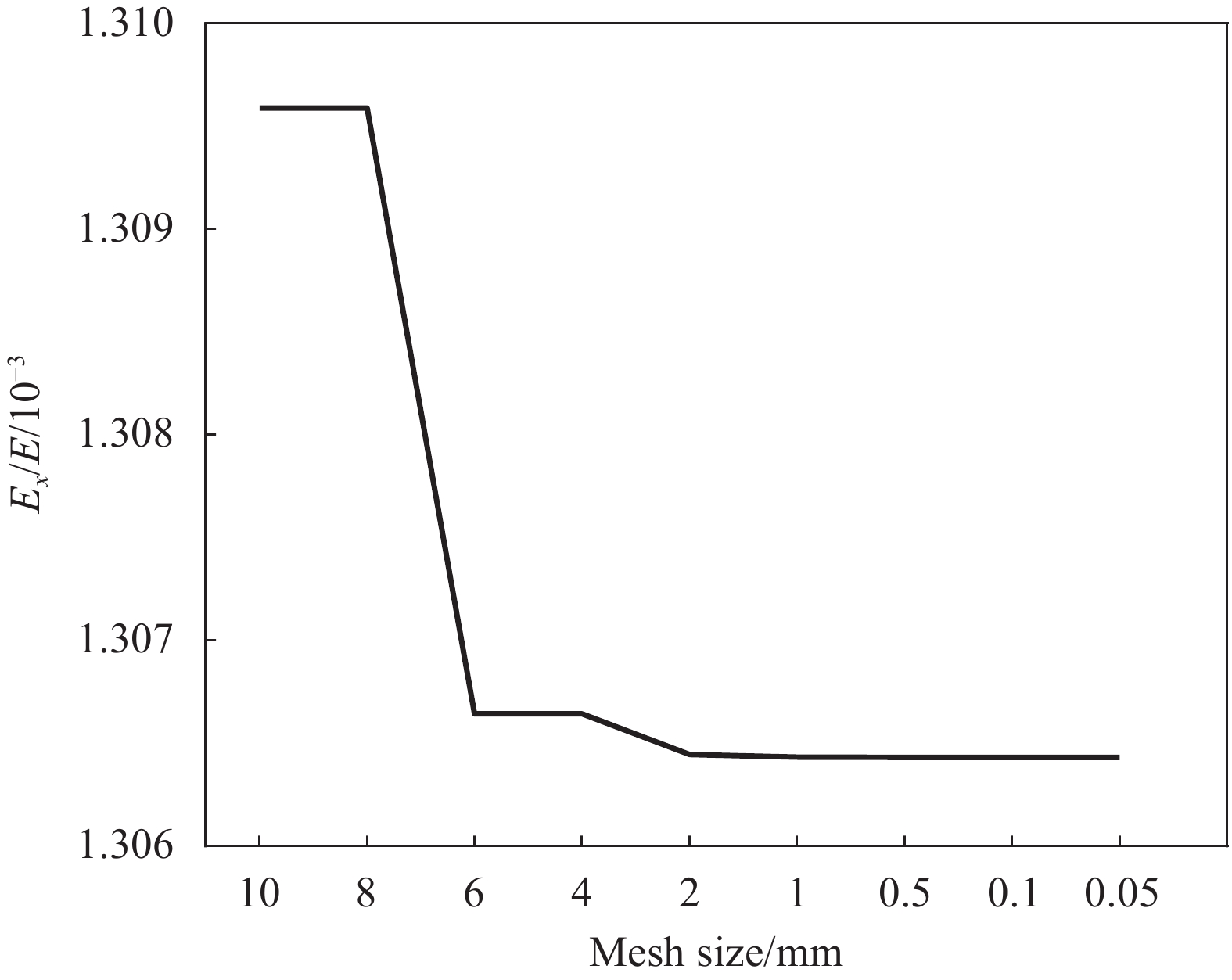
方法通过能量法理论推导了梁结构力学性能的数值解,并用有限元方法进行了数值验证。通过参数分析,讨论了该结构的力学性能。在理论推导方面,根据截面法对单元结构进行受力分析,建立结构分别在拉伸载荷、剪切载荷下的应变能方程;根据卡氏第二定理推导出结构的形变、应变等,得到结构力学特性的理论数值解。在数值验证方面,利用软件ANSYS18.0建立相应的有限元模型,在网格无关性的验证后,进行网格划分、仿真模拟,得出相应力学性能系数。最后,在同等相对密度条件下,将本文所提结构与V型梁(下文简称VS)、anti-tetra-chiral structures(后面简称ATCS)进行对比,揭示了所提结构的力学性能优势。
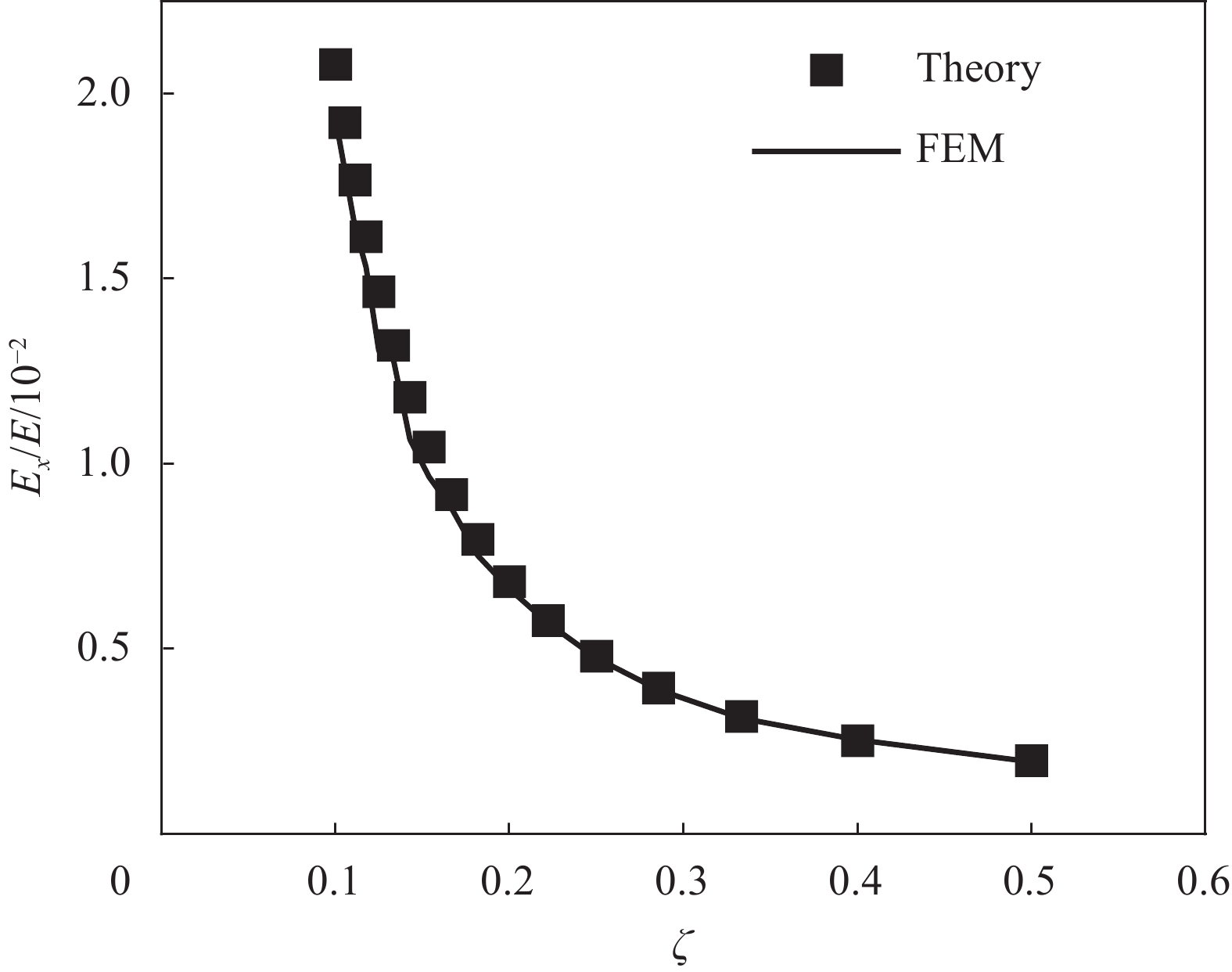
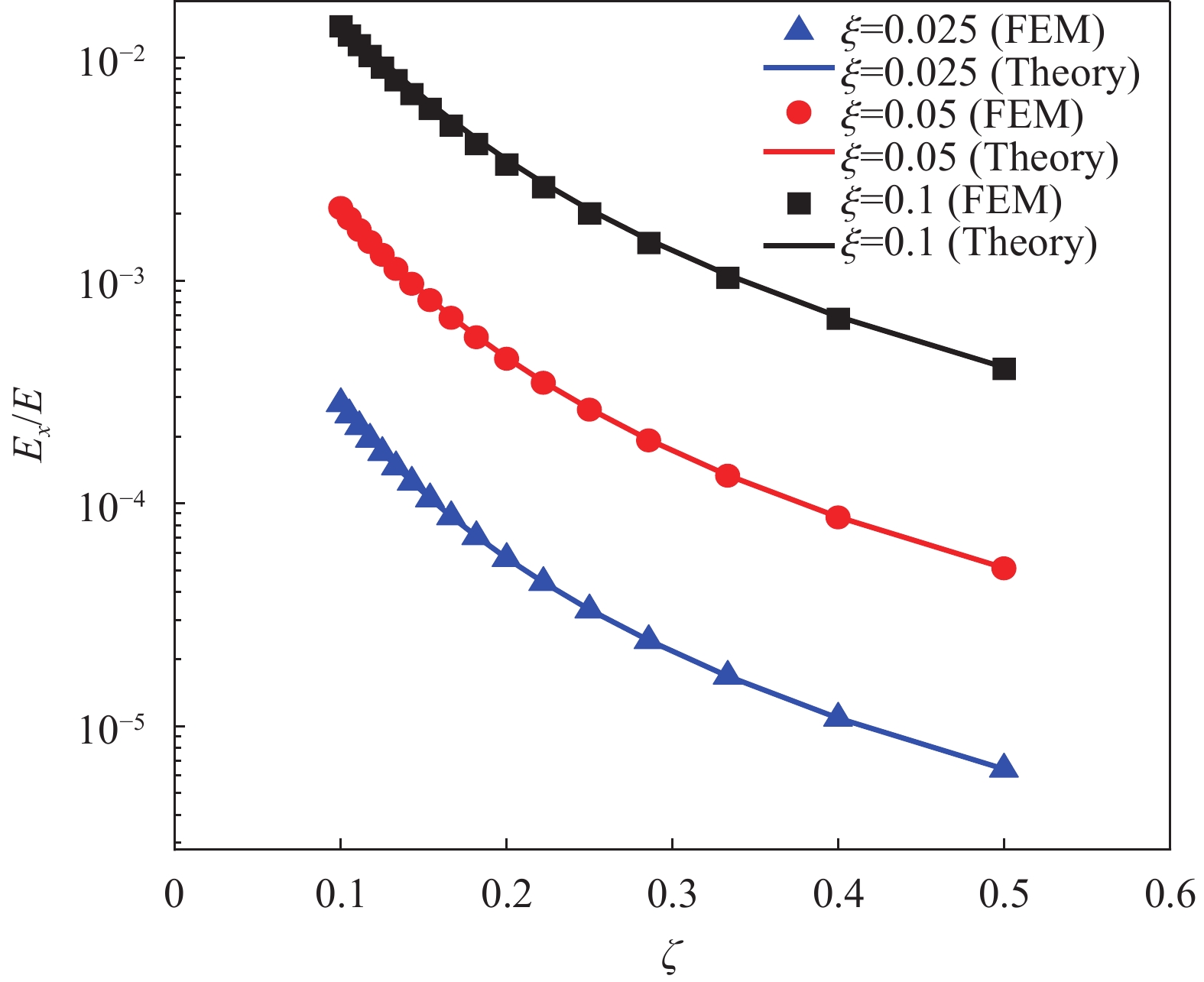
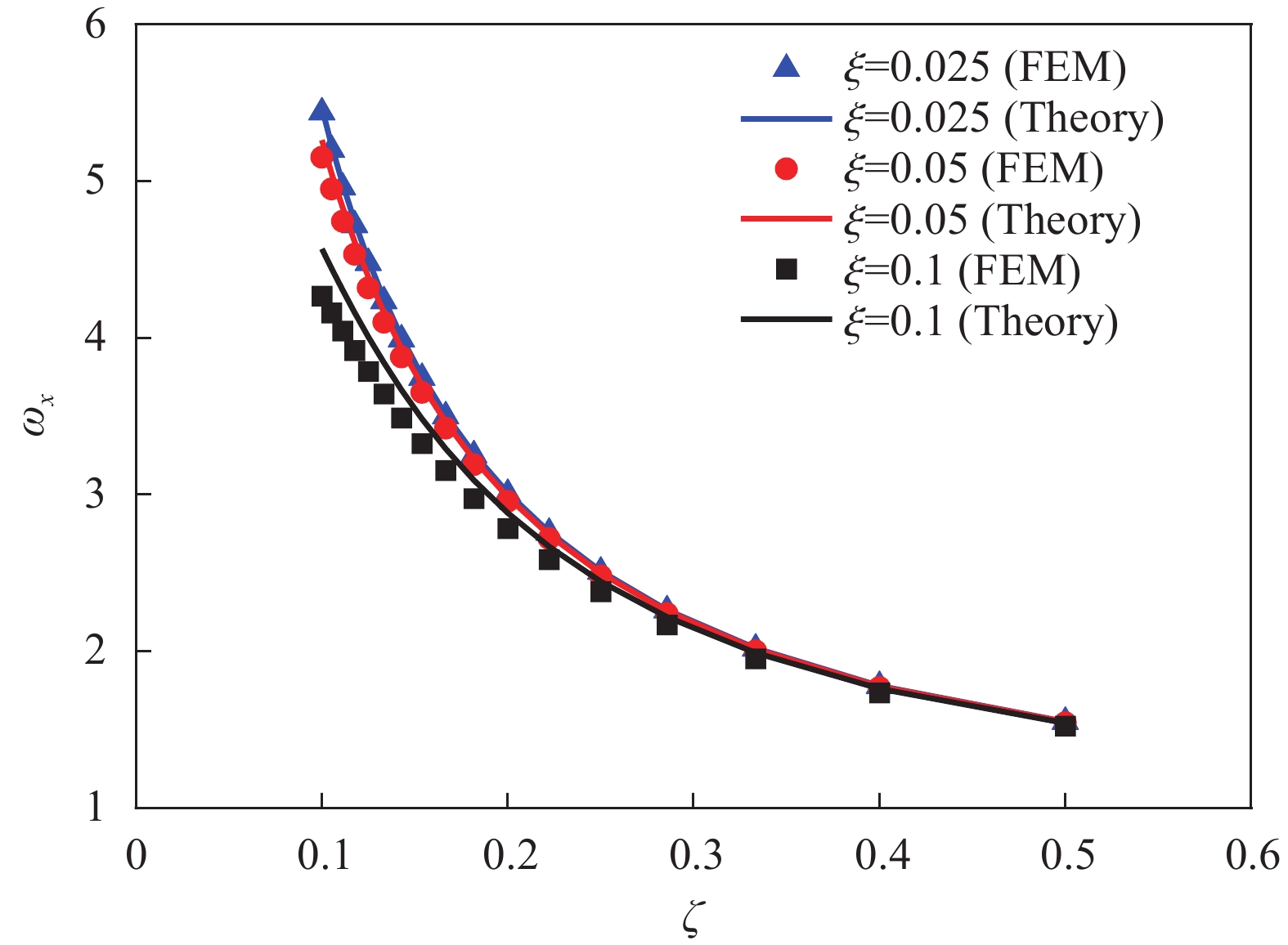
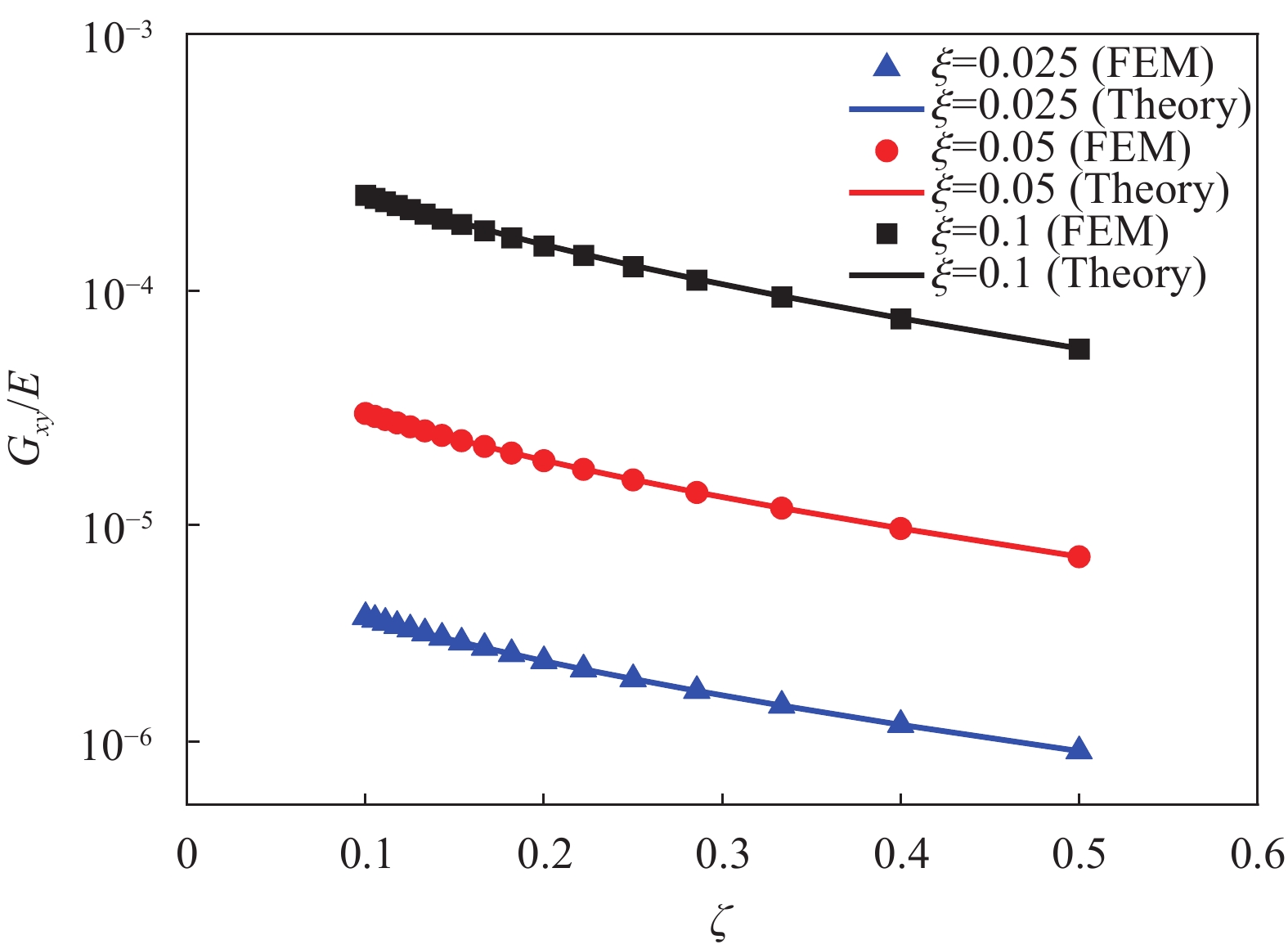
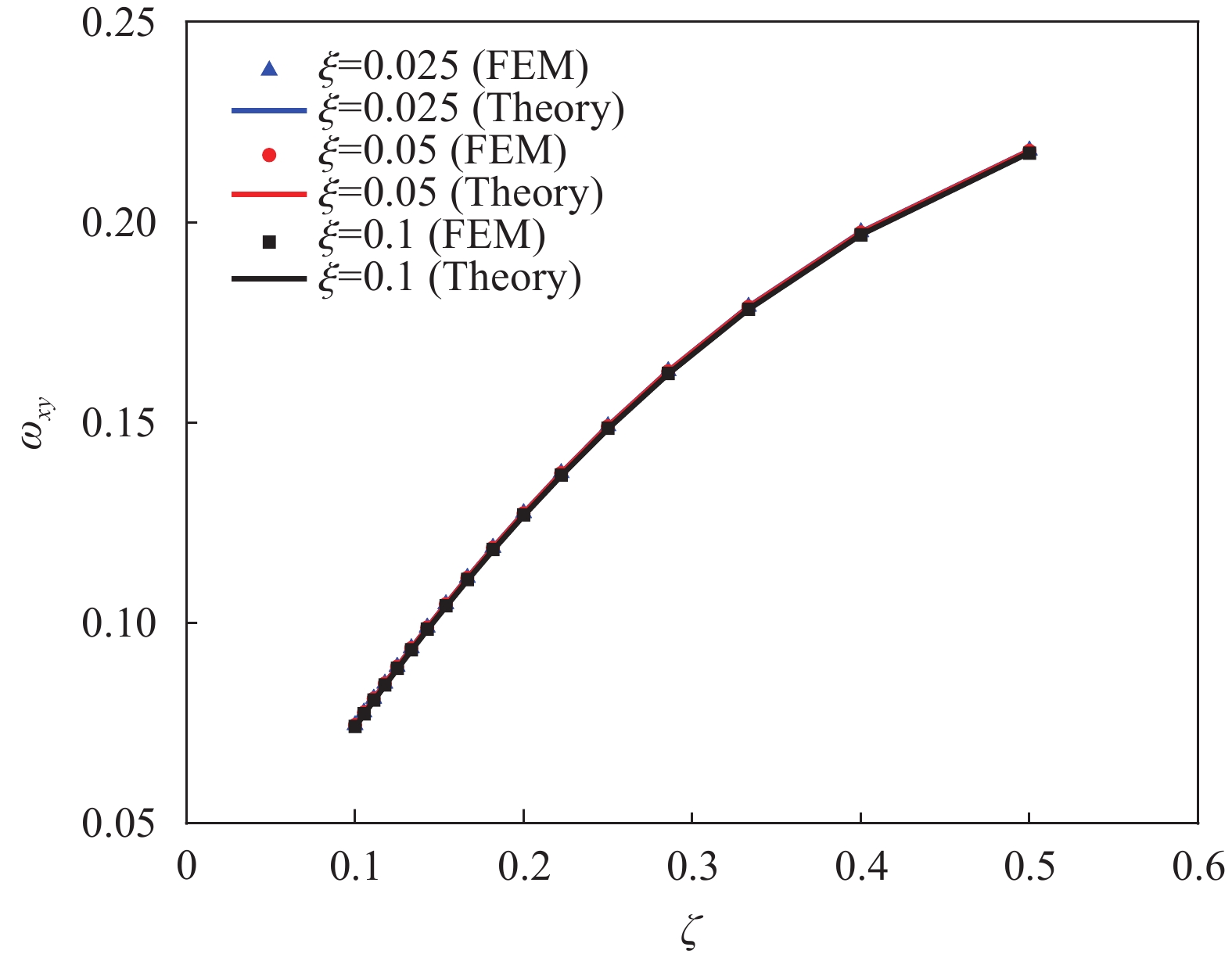
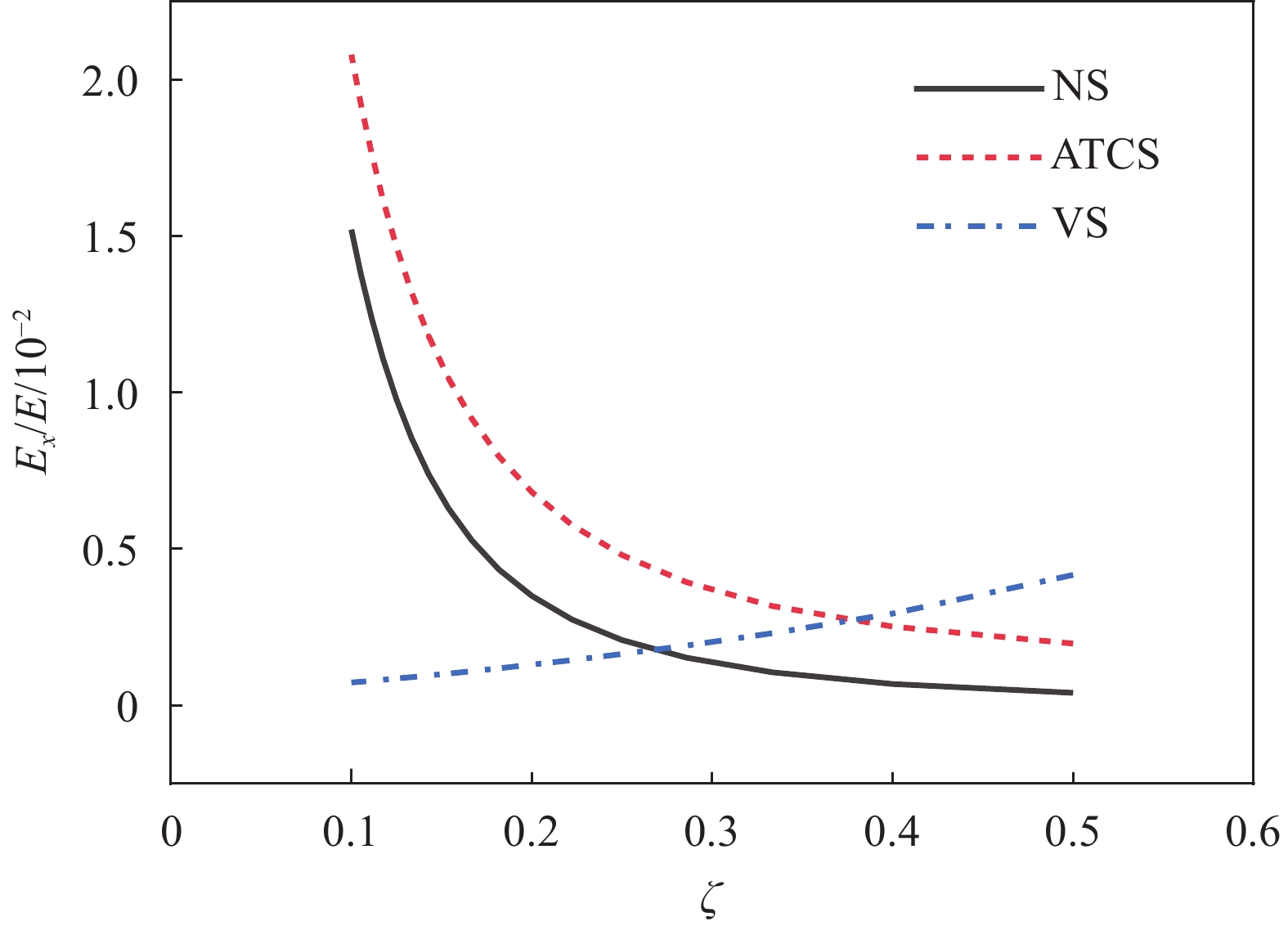
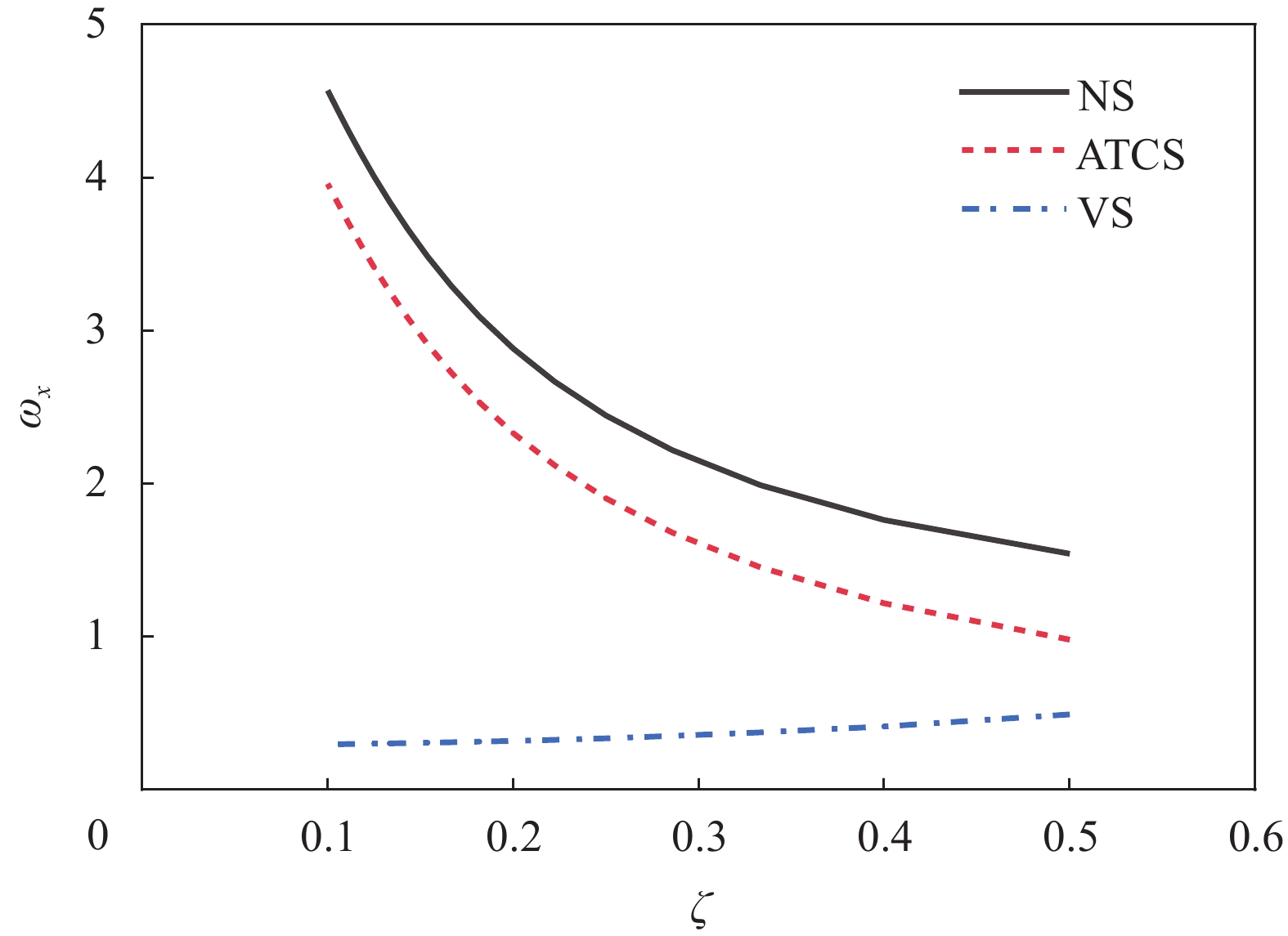
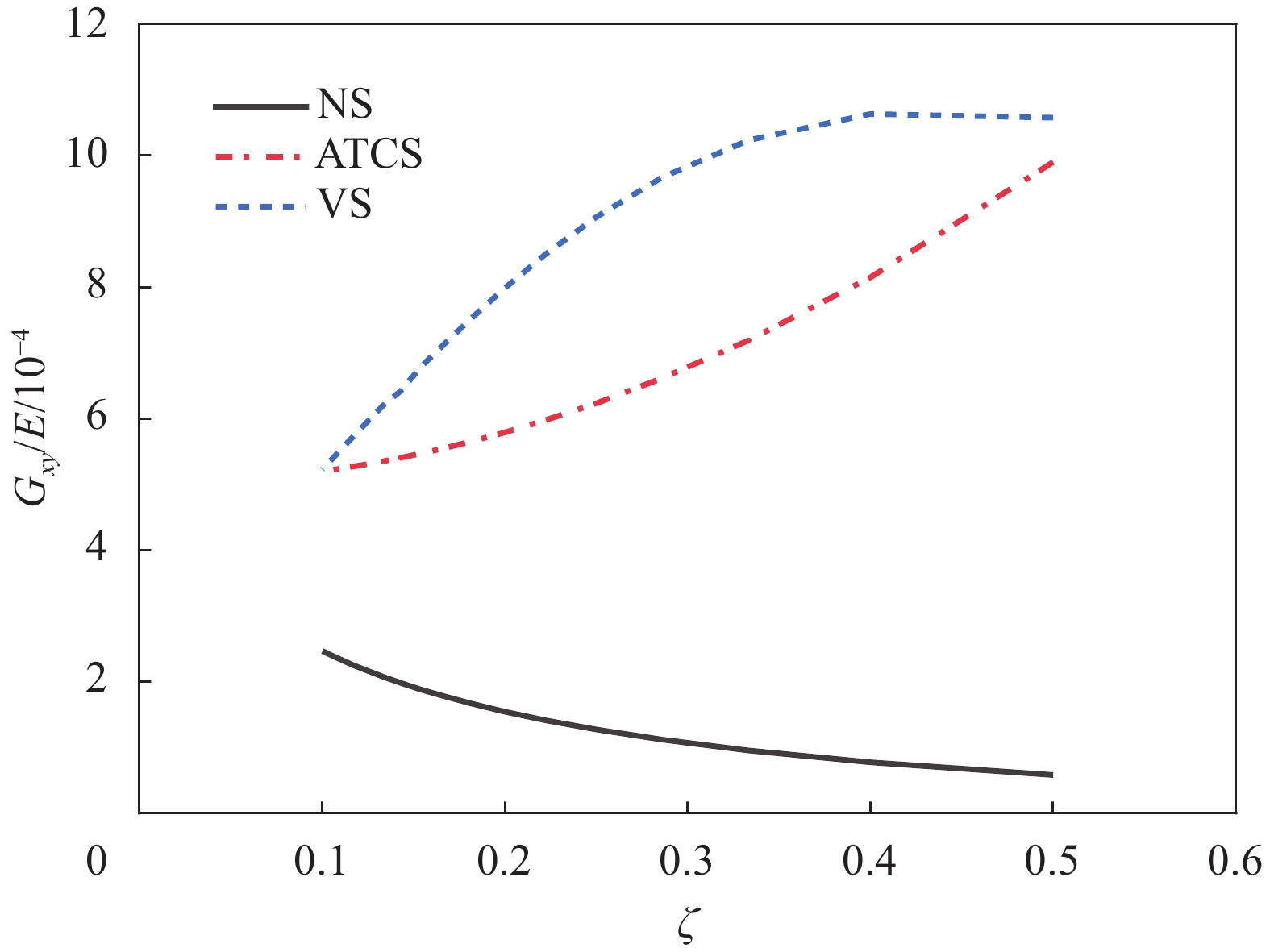
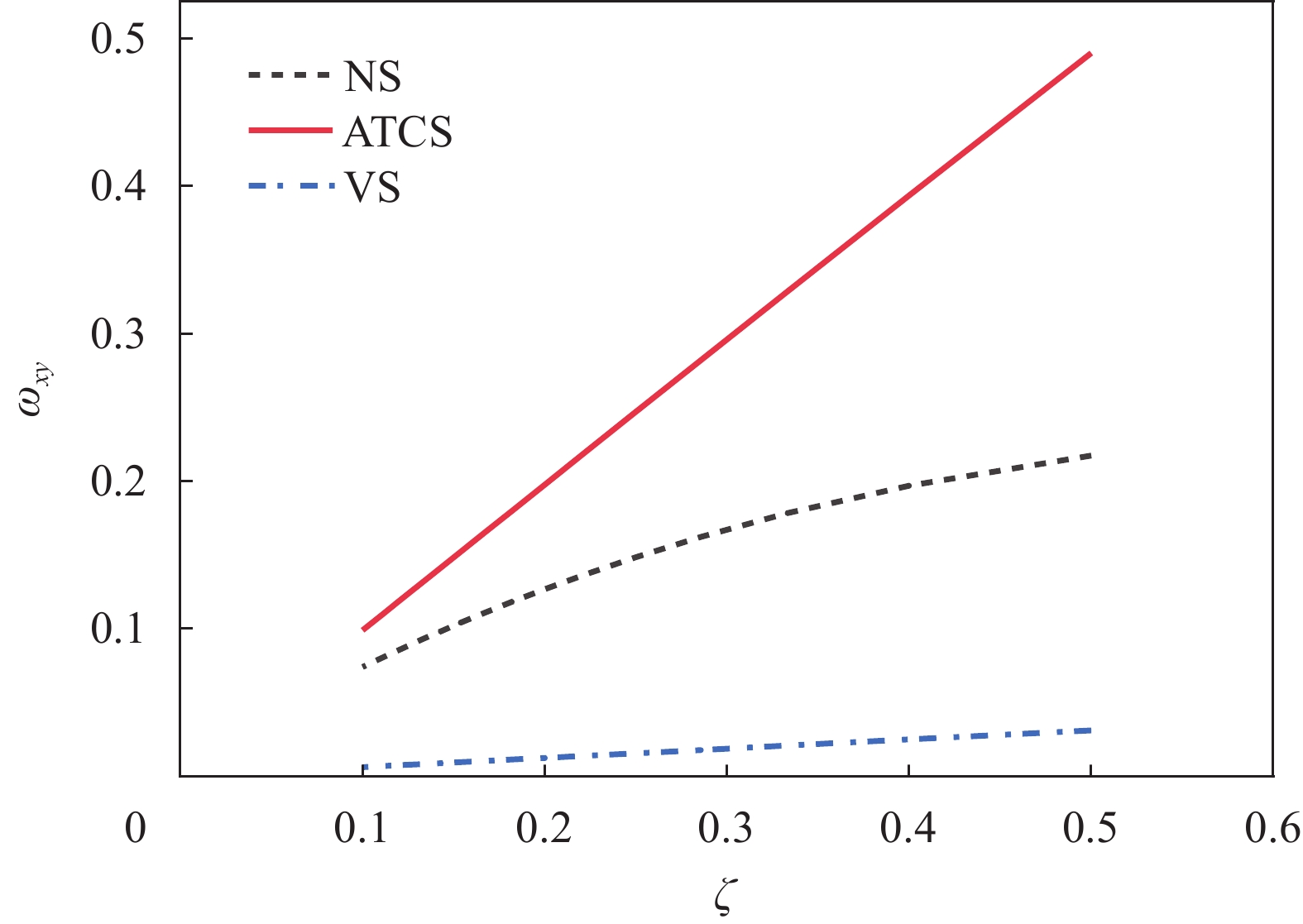
结果从不同参数、下力学性能参数的理论预测、有限元仿真结果以及三种结构的理论预测结果图中可以看出,ϕ本研究推导得到的结构的理论预测性能与有限元实验仿真结果吻合较好。κ新型手性蜂窝结构的等效弹性模量低至10,且拥有较大范围的拉剪耦合系数,最低可达到-5.5。λ与ATCS和VS相比,新型手性蜂窝结构的等效弹性模量低至ATCS的73%,VS的10%;拉剪耦合效应高达ATCS的157%,VS的1544%;等效剪切模量与ATCS相比,可达2个数量级的降低;剪拉耦合效应最低可达ATCS的44%。μ新型手性蜂窝结构具有较宽的力学性能调节范围,约为ATCS和VS结构1.5~2倍,能满足更多状态下的结构力学性能需求。
结论本文所提结构作为一种由半圆弧以及直韧带梁组成的曲-直混合梁无刚性中心节点新型四手性蜂窝结构,相比传统手性结构具有高柔性、大拉剪耦合系数范围等特点。ϕ较长的直韧带对所提结构具有大柔韧性有重要影响,圆弧韧带的旋转对所提结构的耦合效应具有重要影响。κ该新型手性蜂窝结构可用于变体机翼、船舶、医疗支架等领域,且在变体与防护结构上拥有良好的应用前景。λ本研究在线弹性假设条件下开展,未考虑材料或结构的非线性。此外,本文所提新型手性蜂窝结构属于负泊松比结构,目前该结构在准静态与动态平压下的缓冲性能尚不明确,后续可对此开展深入研究。其他:本文在进行有限元仿真时,为了提高获取力学性能参数的效率,利用Matlab自动生成不同参数数值的APDL输入文件,并调用ANSYS进行模拟仿真以及输出结果,从而实现了有限元仿真输入输出的“自动化”。
-
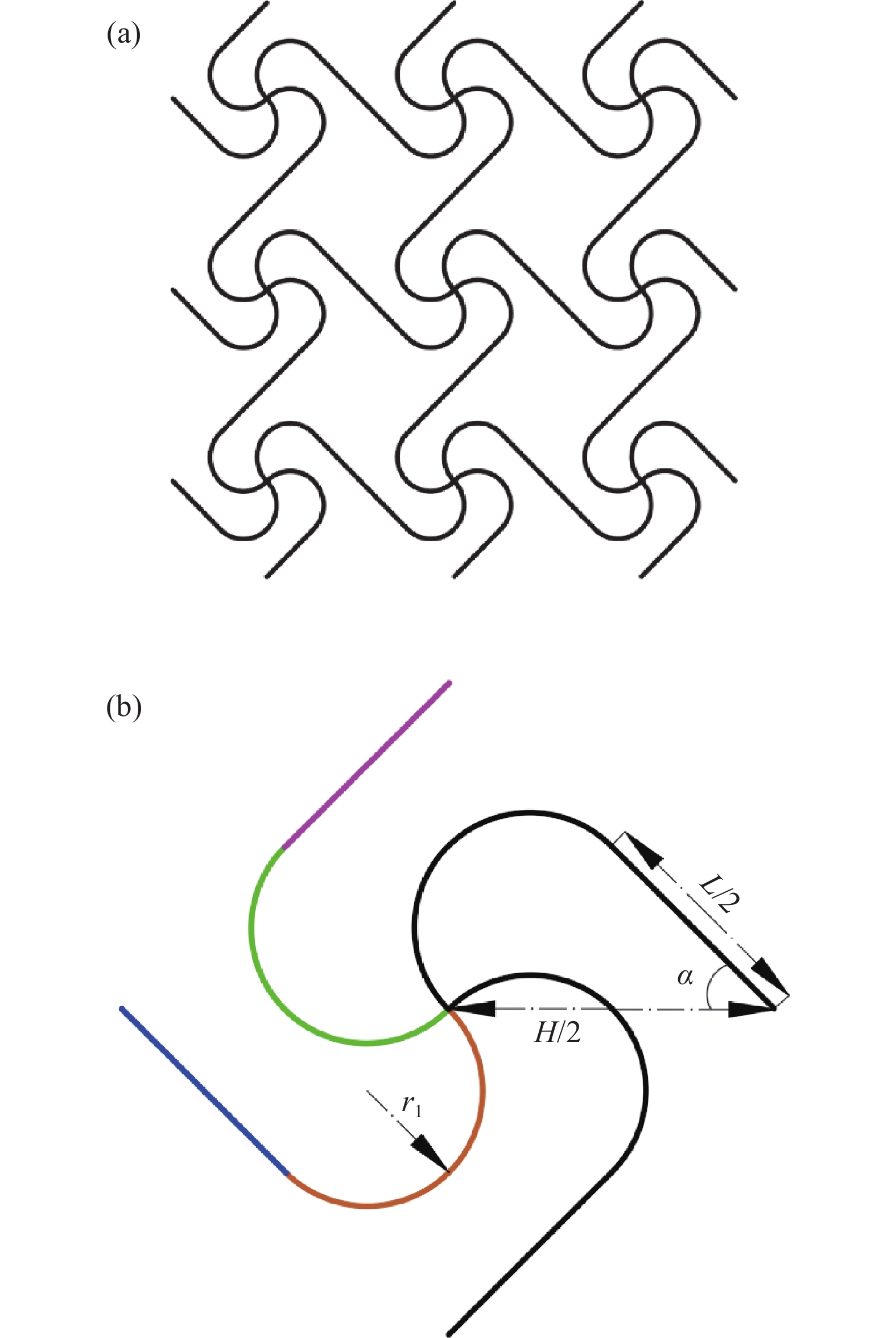
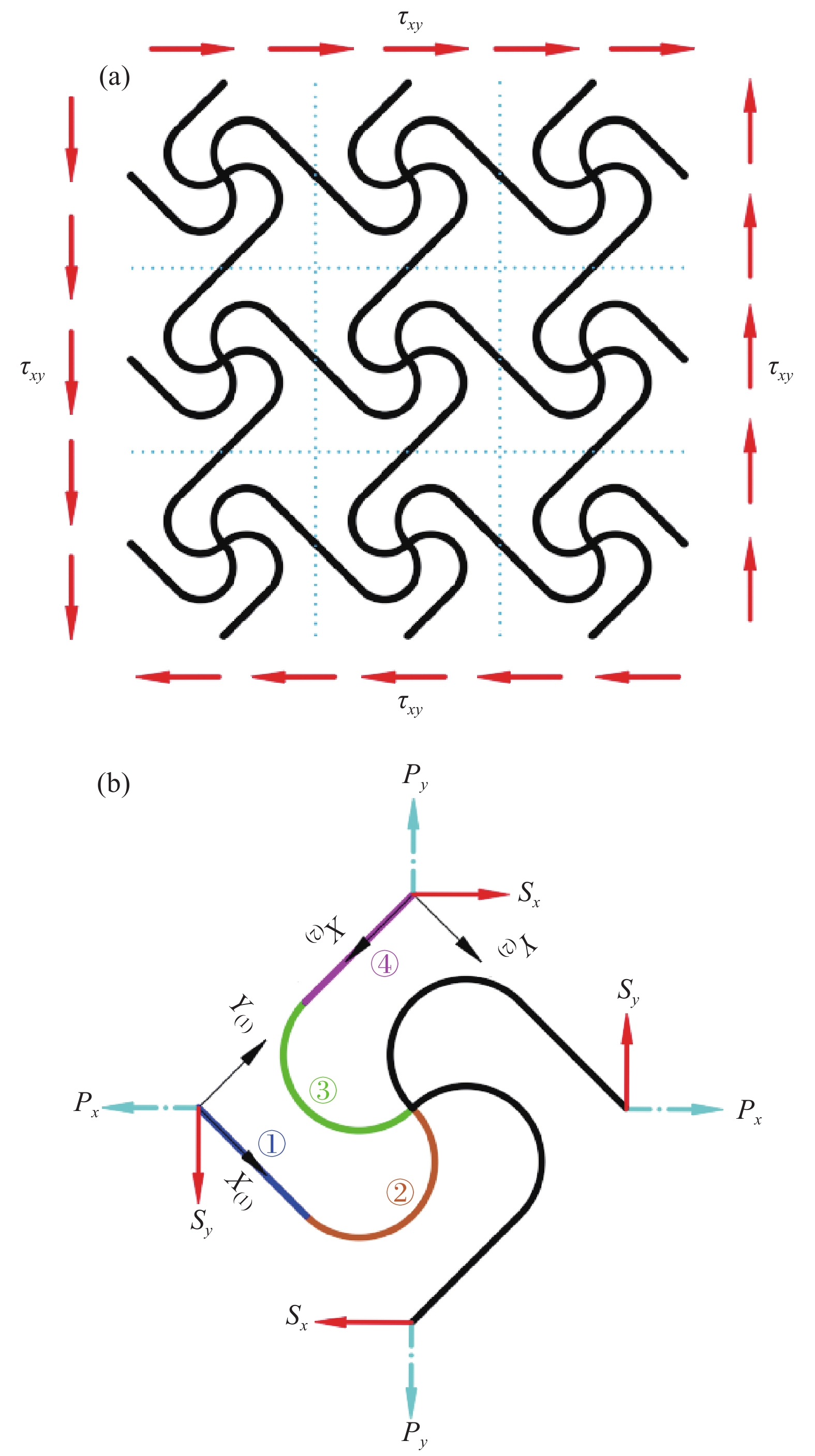
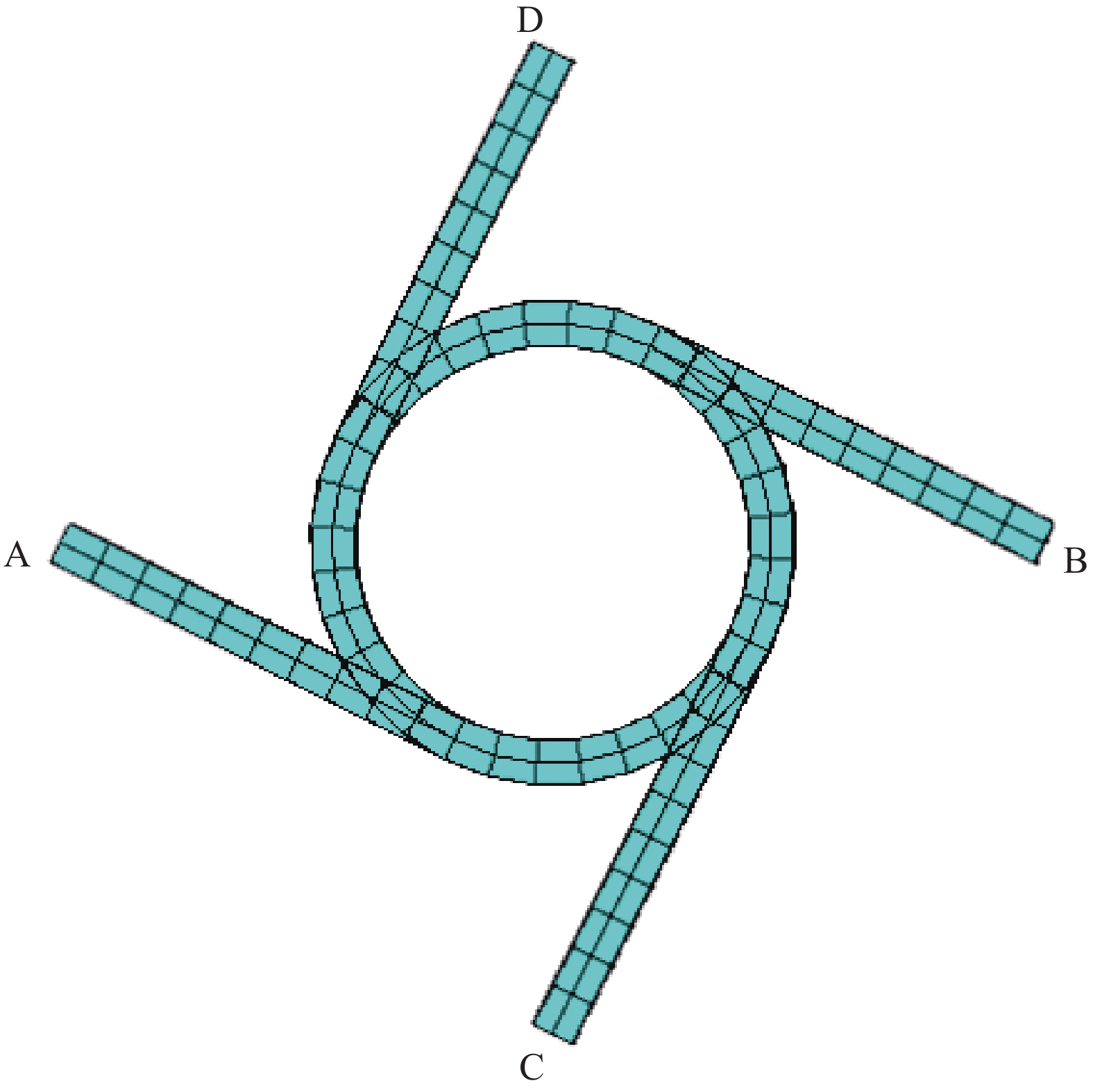
手性蜂窝结构因其极其优异的力学性能表现以及广阔的应用市场前景受到极大关注。虽然已有较多的手性蜂窝结构被提出,但是它们大多都是直梁和刚性中心大节点的组合,这样的主流组合往往会因刚性中心大节点的存在而带来更大的结构刚度,同时也会消耗更多的材料。
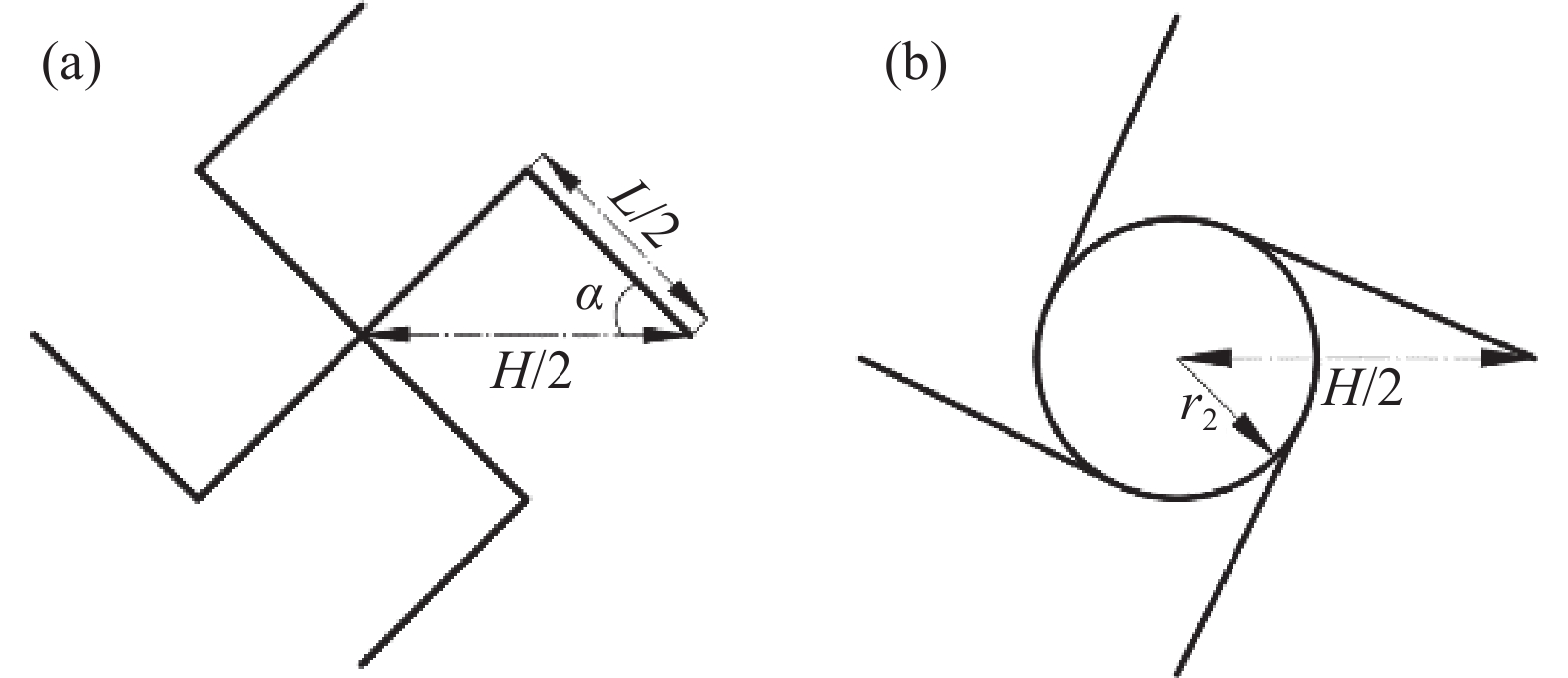
本文提出了一种易变形、延展性好的新型四手性细胞结构,如图1(a)所示,将刚性中心大结点转化为半圆弧韧带与直韧带组合,提高了整体结构的一体化程度,且大幅提升了结构的柔性,在实际制造中也能做到材料的节省。通过能量法理论推导梁结构力学性能的数值解,并用有限元方法进行了数值验证。结果表明:本文所提结构的等效弹性模量仅有VS、ATCS的10%、73%,剪切弹性模量低于ATCS结构2个数量级;拉剪耦合系数范围也接近于ATCS的1.5至2倍。
图1(a)左侧为含有刚性中心大结点的传统手性结构,右侧为本文提出的新型手性蜂窝结构;图1(b)为部分力学性能系数的比较图。
ATCS单元与新型手性蜂窝单元结构以及部分力学性能系数对比






 下载:
下载: