Optimization technology for composite flexible skin based on negative Poisson's ratio honeycomb
-
摘要: 针对由负泊松比蜂窝夹芯和弹性表皮构成的复合式柔性蒙皮,提出了柔性蒙皮几何参数优化方法。首先,将柔性蒙皮的胞壁长度、胞壁夹角、蜂窝高度、胞壁厚度、表皮厚度等几何参数作为变量,设计了柔性蒙皮结构参数化建模方法;其次,通过均实验设计方法获取样本点,利用参数化方法构建柔性蒙皮结构有限元模型,并通过仿真获取各几何参数组合状态对应的结构性能;然后,基于响应面方法构造柔性蒙皮几何参数与结构性能关系的近似模型,并对近似模型的精度进行了定量评估;最后,以近似模型为基础,以柔性蒙皮几何参数为优化变量,综合考虑面内应变、泊松比、面外挠度、质量等因素,采用加权系数法构造了综合优化目标函数,并采用遗传算法进行优化,得到了柔性蒙皮结构综合性能最优的构型。构建了复合式柔性蒙皮有限元模型,进行优化方法验证,结果显示,优化后的构型与初始构型相比,面内变形能力提升30.73%,面外承载能力提高30%,结构质量减轻22.77%,泊松比降低20.73%。Abstract: The geometric parameter optimization method of flexible skin composed of negative Poisson's ratio honeycomb core and elastic skin was proposed. Firstly, a parameterized modeling method for the flexible skin structure was designed, with geometric parameters such as cell wall length, cell wall Angle, honeycomb height, cell wall thickness and skin thickness. Secondly, the sample points were obtained by uniform experimental design method, the finite element models of flexible skin structure were constructed using the parameterized method, and the structural performance corresponding to various combinations of geometric parameters was obtained through simulation. Then, an approximate model for the relationship between the geometric parameters of the flexible skin and the structural performance was constructed using the response surface method, and the accuracy of the approximate model was quantitatively evaluated. Finally, based on the approximate model, taking the geometric parameters of the flexible skin as optimization variables, and considering the factors such as internal strain, Poisson's ratio, out-of-plane deflection, and mass, a comprehensive optimization objective function was constructed using the weighted coefficient method, and the optimal configuration of the flexible skin structure was obtained by genetic algorithm. A finite element model of the composite flexible skin was constructed to validate the optimization. The results show that compared with the initial configuration, the in-plane deformation capacity is increased by 30.73%, the out-of-plane load capacity is increased by 30%, the structural mass is reduced by 22.77%, and the Poisson’s ratio is reduced by 20.73%.
-
随着航空航天、武器装备及轨道交通等领域对轻质合金材料需求的不断增加,对高质量、多用途铝合金的需求也相应增加[1-4]。铝基复合材料已经成为国防、航空航天及民用领域极为重要的集结构和功能的一体化材料[5]。轻质6系铝合金具有良好的导电性、高的比强度、良好的加工性能、耐腐蚀等特点[6],比7系铝合金具有更高的疲劳强度和比2系铝合金具有更高的耐腐蚀性,适用于制作精密的摩擦接触部件[7]。基于对载流摩擦副材料的综合考虑,单一的轻质6系铝合金无法满足服役需求,研究出优异承载电流、耐摩擦磨损的高强度铝基复合材料,是降低载流摩擦副在服役过程中材料损伤和失效的关键。
载流摩擦工况下,机械磨损和电气磨损同时发生,载流摩擦副的界面材料磨损消耗是导致其性能下降和失效的重要原因之一,主要磨损机制为磨粒磨损、粘着磨损、氧化磨损和电弧侵蚀等[3, 8-12]。铝基复合材料的耐磨性能主要取决于增强相种类、尺寸、形状以及与基体的结合强度[3, 13, 14]。通常在铝基体中加入颗粒、纤维、晶须等类型的增强相,使铝基复合材料具有更好的力学性能和耐摩擦磨损性能[3, 15]。TiB2p等硬质陶瓷颗粒由于具有高硬度、高模量、高耐磨性等优点在金属基复合材料领域得到了广泛的应用[16-18]。同时,碳纤维、碳纳米管、石墨等碳材料因其优异的力学性能、耐高温、抗摩擦和其自润滑性,被认为是耐摩擦磨损复合材料的重要增强相材料[5, 19-28]。作为固体润滑剂,碳的存在可以平稳摩擦过程、降低摩擦系数和磨损率等[29, 30]。晶须材料也可以提升铝基复合材料的耐磨性,其中SiCw是已合成晶须中硬度最高、模量最大的晶须[5, 25]。SiCw增强铝基复合材料具有高强度高耐磨性等特点[31]。Zhong等[32]制备了40 vol% (TiB2p-TiCp)/Al-Cu-Mg复合材料,发现亚微米级的TiB2p具有较好的界面结合能力,可以保持复合材料的刚性,抵抗塑性流动。Cao等[30]制备了碳纤维增强AA5052块体复合材料,磨擦试验表明,铝基复合材料的磨损过程更加稳定,磨损体积损失降低70%以上。碳纤维在铝基体中可以抑制微裂纹的形核和扩展,有效防止材料在磨损过程中的剥落,从而提高摩擦学性能。Rouhi等[17]制备了10 vol.%的SiCw/Al复合材料,进行参数为5 N、0.1 m/s、
1000 m的销-盘式磨损实验,发现其磨损率较基体下降90%,表现出更稳定的摩擦性能,耐磨性的提高是由于在磨损表面形成了保护性的机械混合层。可见,降低接触界面的磨损程度是提高载流摩擦副可靠性和稳定性的关键[8]。陶瓷颗粒的加入对降低磨损率有显著效果,但摩擦时材料的磨粒磨损机制不利于摩擦过程,会增大磨损率,后续研究应该尽量避免。目前对于铝基复合材料载流摩擦磨损研究主要集中于单一增强相改变其体积分数或表面涂层对材料干摩擦磨损性能的影响[13, 33-35],而针对载流工况下增强相种类对复合材料载流摩擦磨损性能的研究较少。本文选用颗粒(TiB2p)、纤维(Cf)和晶须(SiCw)作为增强相,采用放电等离子烧结(SPS)和热挤压的工艺结合制备5 vol.%的6063Al基复合材料,并对其微观组织和基础性能进行对比分析,同时探究铝基复合材料在载流工况下的摩擦系数、磨损率、磨损形貌并总结分析其磨损机制,为载流摩擦应用下铝合金的摩擦磨损行为研究提供一定的思路和理论依据。
1. 实验材料及方法
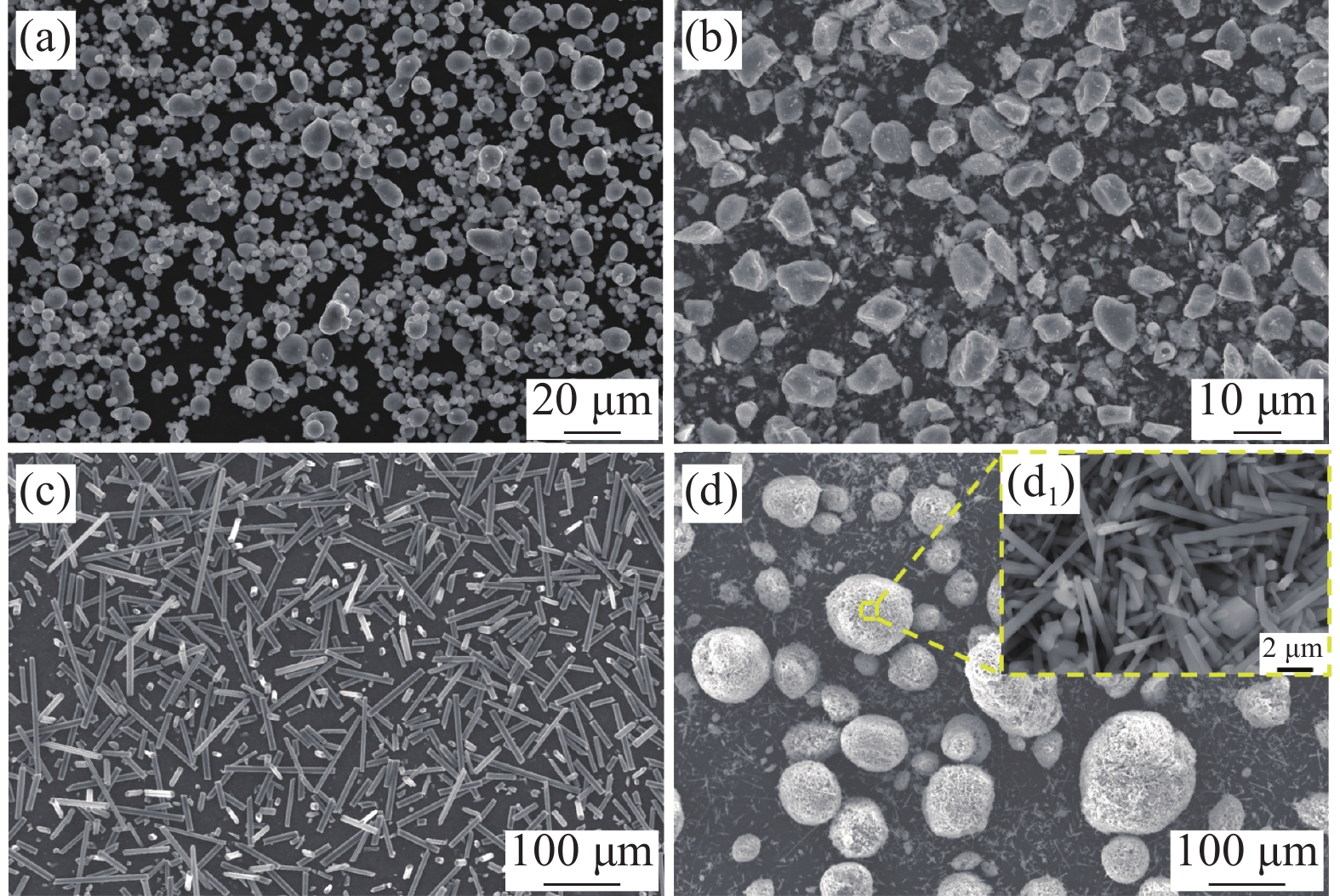
本实验所用基体材料为6063铝合金粉(平均粒径为6~9 μm,长沙天久金属材料有限公司,其名义成分如表1所示),增强相材料为TiB2颗粒(平均粒径为2 μm,上海卜微应用技术有限公司);碳纤维(Cf) (平均长度为150 μm,平均直径为7~8 μm,上海新翱复合材料科技研发中心),SiC晶须(平均长度为5~10 μm,平均直径为0.5 μm)。其微观形貌如图1所示。
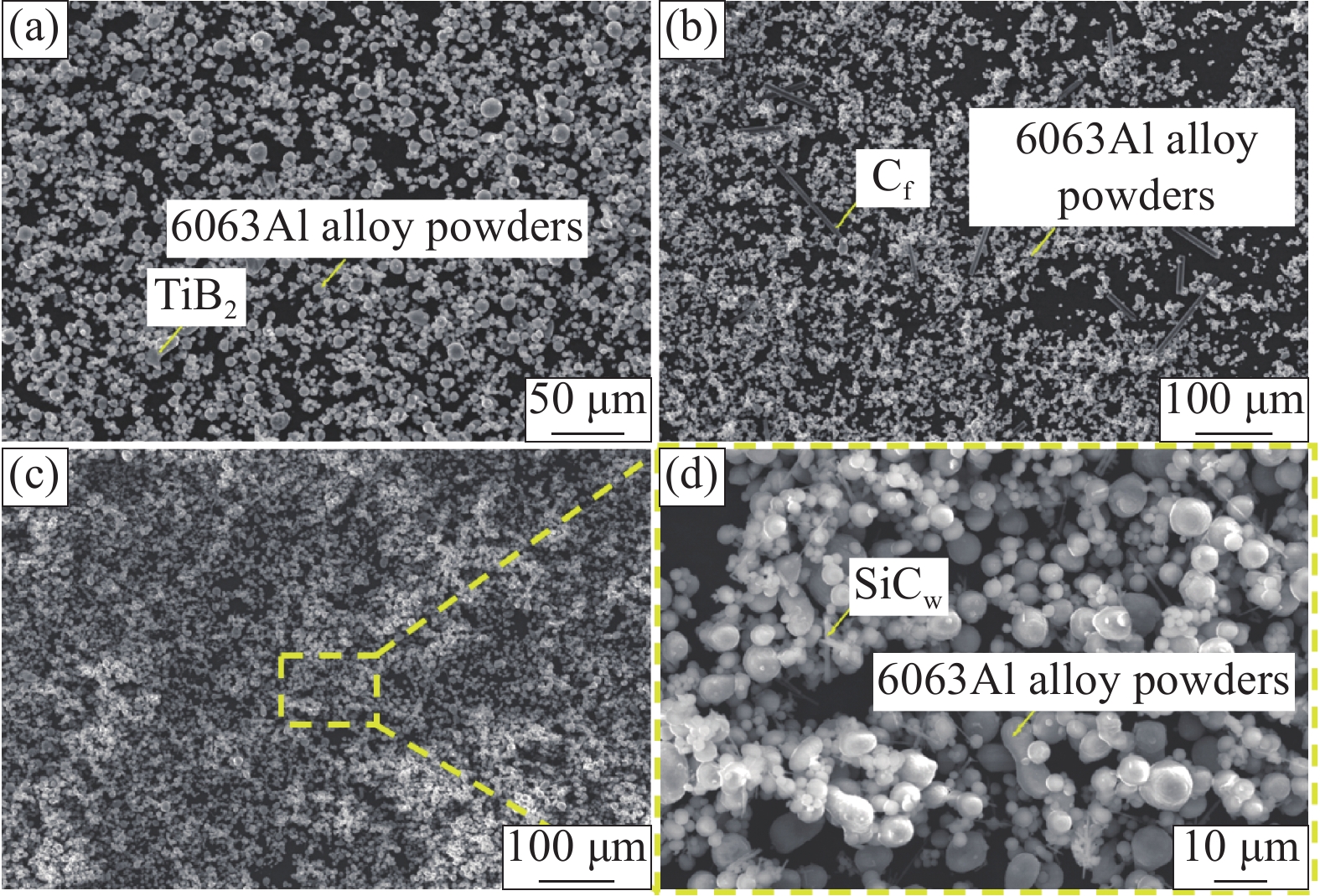
表 1 6063铝合金的名义成分表Table 1. Nominal composition list of 6063Al alloy6063Al alloy Al Mg Si Fe Cu Mn Cr Ti Zn Content/wt.% Bal. 0.45~0.90 0.20~0.60 0.35 0.10 0.10 0.10 0.10 0.10 采用SPS烧结法和热挤压法相结合制备TiB2p/6063Al、Cf/6063Al、SiCw/6063Al三种复合材料,同时,6063Al基体作为对比材料采用相同工艺进行制备,其制备工艺过程如下:(1) 翻转式混粉:将6063铝合金粉、增强相粉末和适量玛瑙球按比例装入球磨罐中(增强相体积分数为5 vol.%),大中小规格的玛瑙球质量比为1∶3∶6,球料比为1∶1。将球磨罐固定在卧式机床上,设置翻转转速为45 r/min,混合时间8 h。图2为混粉结束后复合粉末的微观形貌。可以看出,TiB2p、Cf和SiCw均在基体粉末中均匀分布,这有利于后续烧结体的高致密性。(2) SPS烧结:利用FHP-828型快速热压烧结炉烧结成型,烧结温度为500℃,烧结压力为30 MPa,保温保压5 min,制成Φ40 mm × 30 mm的圆柱形试样。(3) 热挤压:复合材料在450℃的温度下保温3 h后,利用四柱液压机(YA32-315A)对烧结坯进行热挤压成型,挤压比约为7∶1,最终制成直径为Φ15 mm的棒材。
采用钨灯丝扫描电子显微镜(SEM,JSM-IT100,JEOL,Japan)对6063Al基复合材料的微观组织形貌进行表征。采用Sigma2008B1涡流电导率仪测试复合材料的导电率。采用320HBS-3000型数显布氏硬度计测试复合材料的硬度(压头为Φ5 mm的碳化钨硬质合金球,测试载荷为250 kgf,保压时间为30 s)。采用阿基米德排水法测量计算复合材料的致密度,测量湿重时介质为去离子水(25℃)。根据公式(1)计算材料的实际密度[36]:
ρ实际=M干M干−M湿(ρ水−ρ空)+ρ空 (1) 式中,ρ实际为材料的实际密度(g/cm3),M干和M湿分别是材料在空气和液体介质中测得的质量(g),ρ水为常温去离子水的密度(g/cm3),ρ水为空气的密度(g/cm3)。用实际密度除以理论密度即为材料的致密度。
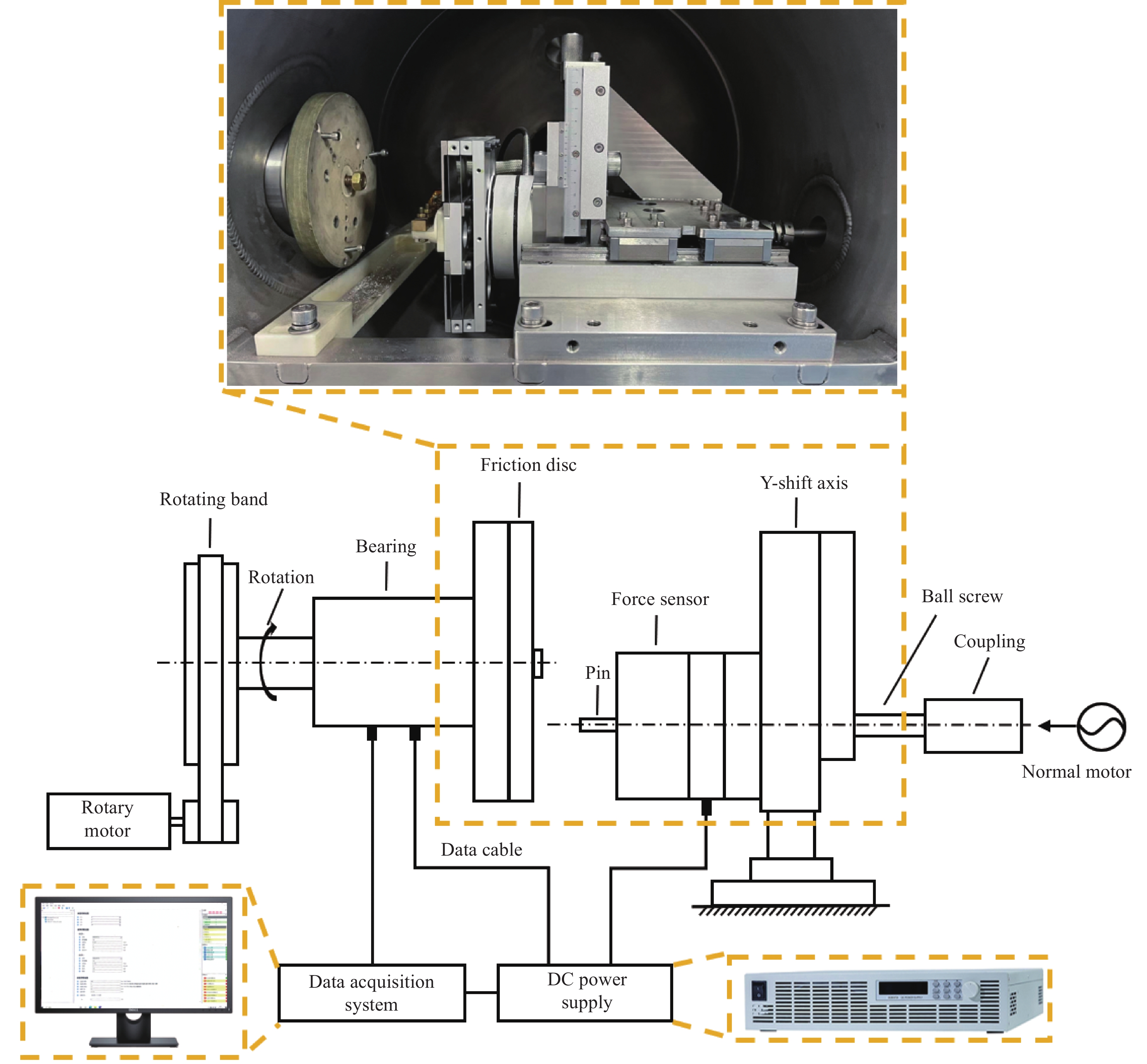
采用MTF-CF200特种材料电损伤测试系统对6063Al基复合材料的载流摩擦磨损性能进行测试,测试系统示意图如图3所示。该设备为销-盘式摩擦试验系统,本试验所用的销材料为6063Al基复合材料,尺寸为Φ6 mm × 15 mm,盘材料为CuCrZr合金,其尺寸为Φ130 mm × 10 mm。在试验前将销试样的摩擦面加工出半径为0.5 mm、角度为45°的倒角,此时销试样的截面积约为19.63 mm2。工作参数设定:线速度为12 m/s、法向力为30 N、加载电流和电压分别为20 A和22 V。此时摩擦面的电流密度约为1.02 A/mm2。试验前后使用精度为0.1 mg的高精度电子天平(LS220 A)测量销试样的质量。根据公式(2)计算磨损前后材料的线磨损率[37]:
W=ΔGvt (2) 式中,W为线磨损率(mg/m),ΔG为磨损前后复合材料的质量损失(mg),v为线速度(m/s),t是磨损时间(s)。
根据公式(3)和(4)计算材料的载流效率,公式(3)、(5)和(6)计算其载流稳定性[37]:
ˉI=1nn∑i=1xi (3) η=ˉII0×100% (4) σA=√1nn∑i=1(xi−ˉI)2 (5) δ=σAˉI×100% (6) 式中,ˉI为试验过程中实际电流的平均值(A),xi为瞬时电流值(A),η为载流效率(%),I0为设定电流值(A),σA为实际电流的标准差(A),δ为载流稳定性(%)。
采用钨灯丝扫描电子显微镜(SEM,JSM-IT100,JEOL,Japan)观察磨损后销试样表面的微观形貌;采用奥林巴斯OLS5100激光共聚集显微镜观察磨损后销试样表面的三维形貌。
2. 结果及讨论
2.1 6063Al基复合材料微观组织
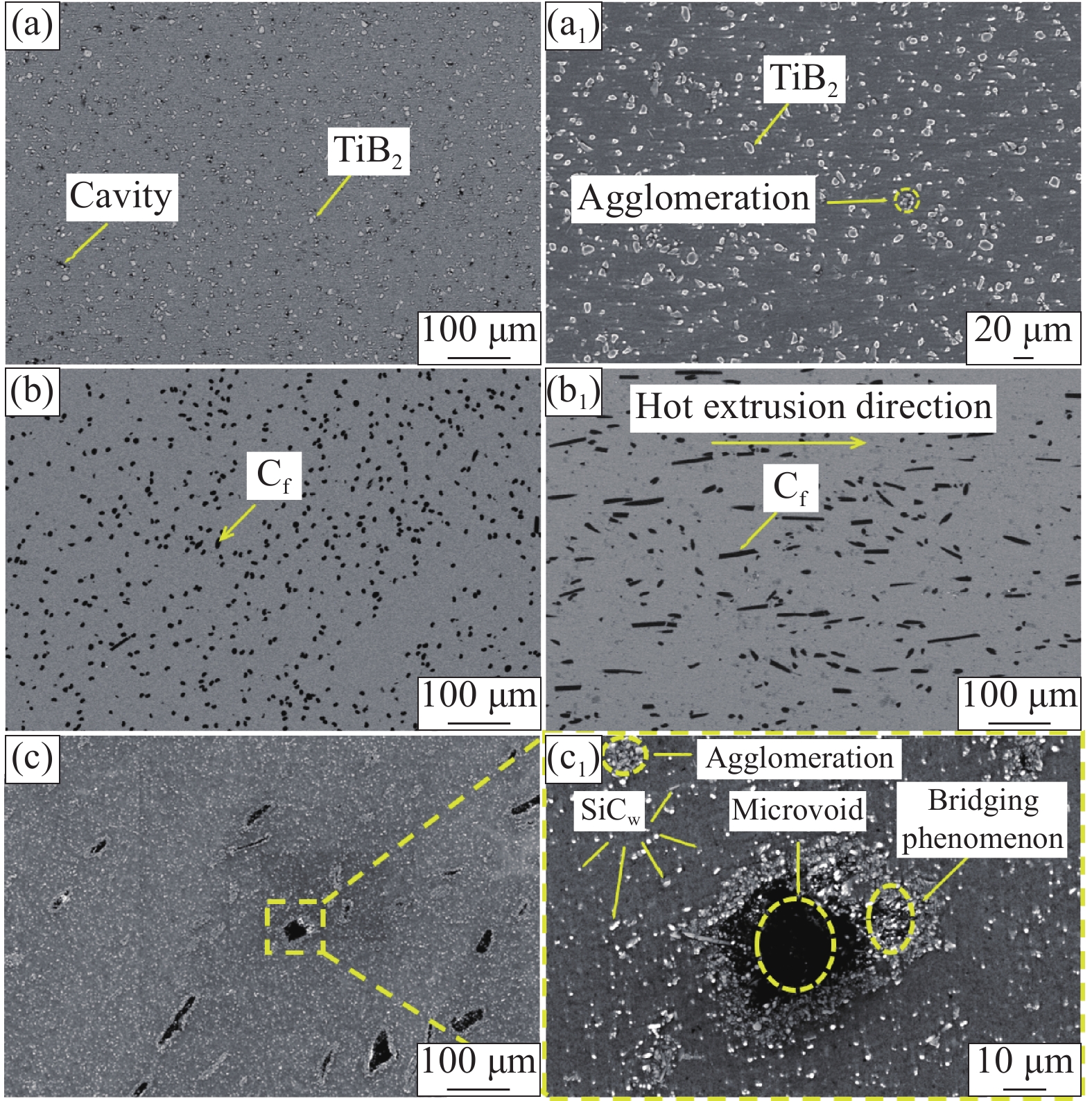
图4为6063Al基复合材料的SEM微观组织。可以看出,图4(a)中的白色颗粒为TiB2p,大部分均匀地分布在6063Al基体(灰色区域)中,表现为与基体之间良好的界面结合。TiB2p/6063Al复合材料表面存在小部分的空洞缺陷(黑色区域),这些缺陷一部分是在磨样抛光过程中TiB2p脱落造成的,一部分是TiB2p少量聚集使得颗粒之间没有基体填充造成的(见图4(a1))。图4(b)中的圆形黑点为Cf,可以观察到Cf在6063Al基体中分布较均匀,与6063Al基体之间结合紧密,未见空洞、裂纹等缺陷。图4b1为Cf/6063Al复合材料沿挤压方向剖面的微观组织,可以观察到大部分Cf沿着平行于热挤压的方向择优排布。图4c中的亮白色部分为SiCw,黑色部分的中心及周围是SiCw出现大量团聚的区域。将团聚区域放大观察(见图(4c1)),发现SiCw聚集区域的中心形成约20 μm的微孔,并且微孔的边缘部分出现晶须之间的相互搭桥现象。这是由于聚集区域周围的SiCw与6063Al基体的界面结合最薄弱,裂纹容易在此区域形成和扩展。试样在进行打磨抛光时,试样受力方向与晶须接触处垂直,缺陷处会形成微小的裂纹,随着裂纹扩展,团聚区域的中心材料容易脱离,形成微孔。而基体中团聚的晶须也会导致材料内部中出现许多孔隙等微缺陷。
2.2 6063Al基复合材料的基础性能
表2为6063Al基复合材料的致密度、硬度和导电率。可以看出,不同增强相的添加会对6063Al的硬度有不同程度的提升作用,而对其致密度和导电率会有不同程度的降低作用。其中,Cf/6063Al复合材料和6063Al基体的致密度均达到99%以上,材料较致密。TiB2p/6063Al和SiCw/6063Al复合材料的致密度分别在98%和97%左右,这是由于两种增强相与基体之间的界面结合相对较弱,且在基体中存在增强相的部分聚集,使得基体与增强相之间不能完全啮合,复合材料中的局部孔隙数目增加,导致复合材料的致密度降低。6063Al基复合材料的硬度均高于6063Al基体,这是由于增强相作为第二相,可以阻碍位错运动,增强了复合材料的抗塑性变形能力,从而提高了6063Al基复合材料的硬度。其中,TiB2p/6063Al的硬度最高,其值为68.38 HB,较6063Al基体提升11.55%;Cf/6063Al和SiCw/6063Al的硬度分别较6063Al基体提升3.43%和10.51%。这是由于TiB2p属于硬质陶瓷相,硬度较高的细小颗粒均匀分布在6063Al基体中,对复合材料硬度的提升效果优于另外两种增强相。另外,SiCw/6063Al复合材料的硬度虽然也较高,但材料表面和内部的微孔缺陷较多,且复合材料的致密度较低,这对复合材料的力学性能有一定的影响。
表 2 6063Al基复合材料的基础性能Table 2. Basic properties of 6063Al matrix compositesAluminum matrix and composites Relative density/% Hardness/HB Electrical conductivity/% IACS TiB2p/6063Al 98.63 68.38(±0.56) 44.52(±0.18) Cf/6063Al 99.41 63.40(±0.71) 48.40(±0.07) SiCw/6063Al 97.57 67.74(±1.28) 45.65(±0.16) 6063Al 99.87 61.30(±0.48) 50.40(±0.06) 增强相的加入导致6063Al基复合材料中电子传输的界面增多,对电子的散射作用增强,金属中自由电子运动的阻力增大,导致导电率的下降[9],因此,6063Al基复合材料的导电率均低于6063Al基体。其中,Cf/6063Al的导电率(48.40% IACS)下降最少,较6063Al基体降低3.97%;TiB2p/6063Al和SiCw/6063Al的导电率分别较6063Al基体下降11.67%和9.42%。这是由于Cf/6063Al复合材料的致密度优于另外两种复合材料,材料内部的缺陷较少,对电子的散射作用相对较弱;Cf长径比约为16,其电阻率具有各向异性,沿轴向的电阻率较低,而Cf在基体中大部分沿挤压方向择优排布(图4b1),这缓解了界面增多带来的铝基复合材料导电率下降现象。TiB2p虽与Cf的整体电阻率相差不大[38],但TiB2p的粒径较小,在相同体积分数下分布在基体中,TiB2p/6063Al比Cf/6063Al电子传输的界面数量多,对电子散射作用较强,故TiB2p/6063Al的导电率较低。在SiCw/6063Al复合材料中SiCw存在团聚,团聚处有空洞等缺陷会影响其导电率。综上,当三种增强相以相同的体积分数添加时,TiB2p/6063Al复合材料的硬度最高,Cf/6063Al复合材料的导电率和致密度受增强相的影响最小。
2.3 6063Al基复合材料的载流摩擦磨损性能
2.3.1 摩擦系数和磨损率
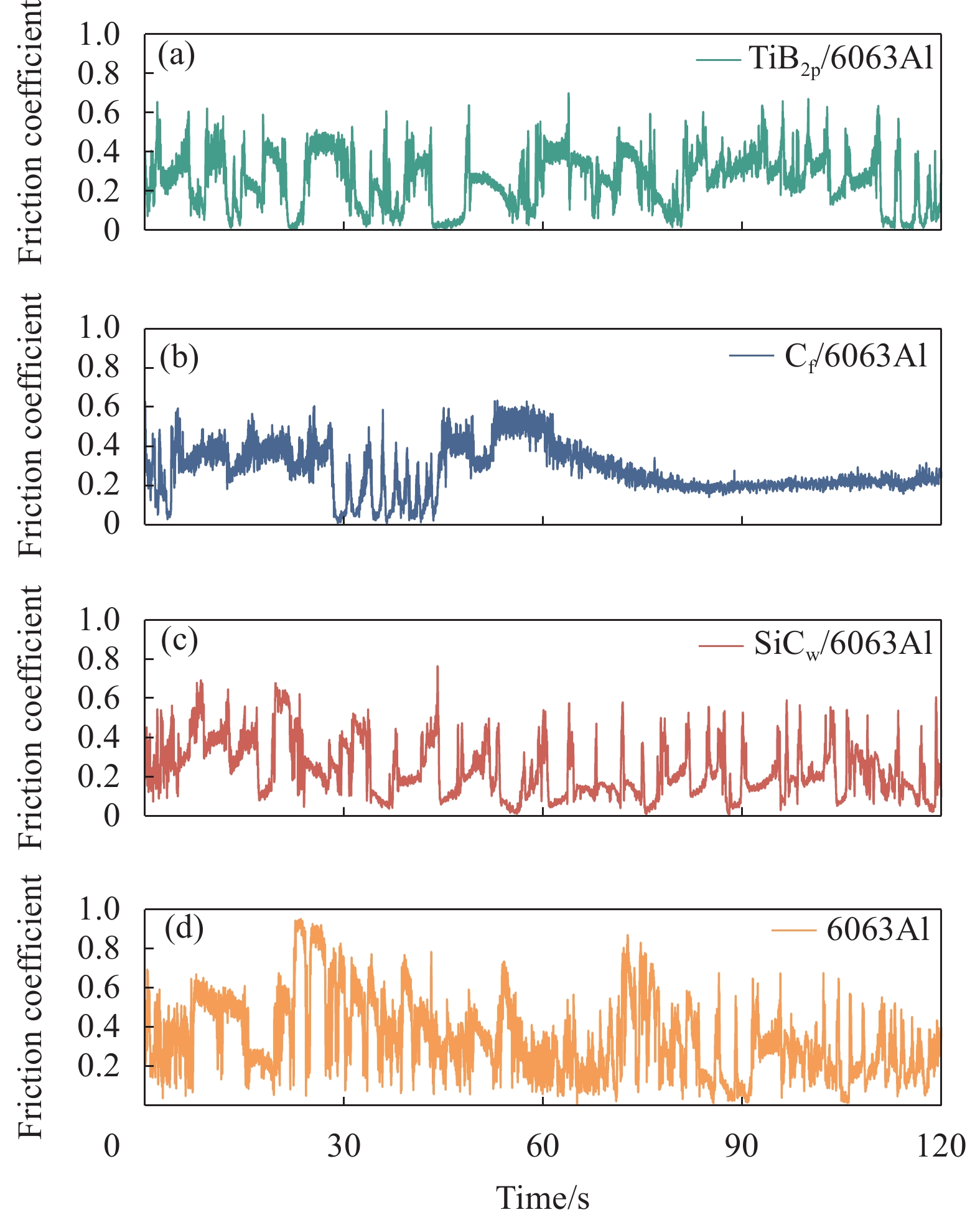
图5和图6分别显示了6063Al基复合材料载流摩擦系数的瞬时波动值、均值和线磨损率。由图5和图6中的摩擦系数均值可以看出,不同增强相类型对6063Al基复合材料载流摩擦系数的影响不同。由图5(a)可以看出,当摩擦时间为0 s~60 s时,TiB2p/6063Al复合材料的摩擦系数波动较大;60 s后,摩擦系数逐渐平稳。整体来看,TiB2p/6063Al复合材料的摩擦系数整体变化趋势较6063Al基体平稳,平均摩擦系数(0.309)较6063Al基体降低8.85%,这说明添加TiB2p可降低摩擦系数,使摩擦过程更加平稳。由图5(b)可以看出,当摩擦时间为0 s~30 s时,Cf/6063Al复合材料的摩擦系数呈现小范围的波动;30 s~45 s之间,摩擦系数出现较大波动,这可能是由于摩擦形成的机械层的剥落导致的;60 s后,摩擦系数在0.24左右的极小数值范围内波动,出现平稳滑动阶段,其平均摩擦系数(0.257)较6063Al基体降低24.19%,说明Cf的添加极大地提升了复合材料的摩擦性能。由图5(c)可以看出,SiCw/6063Al复合材料的摩擦系数整体波动较其余材料最为明显,但是其平均摩擦系数(0.235)最小,说明SiCw的添加对降低复合材料的载流摩擦系数具有一定的效果,但是对摩擦过程的稳定性提升并不明显。
由摩擦系数分析可知,Cf/6063Al复合材料的摩擦性能最优。在经历约60 s磨合阶段后即进入平稳摩擦阶段,这得益于Cf本身属于良好的润滑相,且在摩擦时可以在磨损表面形成一层碳膜[39]。碳润滑膜的存在可以减少接触和粘着,随着摩擦时间的延长,摩擦表面温度升高,此时摩擦系数的降低和平稳主要得益于这层碳膜的润滑作用[37]。此外,Cf/6063Al复合材料的导电率较6063Al基体下降最少,材料传输电流的阻力较小,这也是摩擦系数较稳定的原因之一。TiB2p/6063Al复合材料的硬度最高,在摩擦时颗粒支撑点较多,凸起的TiB2p减小了基体与磨盘之间的接触面积,使摩擦阻力减小,并且支撑点的存在可以加快热量的扩散,使得摩擦系数减小,但随时变化的接触面积使得其承载电流的能力减弱,摩擦稳定性较低[10]。SiCw/6063Al复合材料表面存在较多微孔缺陷,缺陷中的SiCw容易脱落,缺陷增大,裂纹随之扩展。随着磨损的增加,SiCw脱落的越来越多,机械层也更易被磨掉成磨屑飞出,使得摩擦系数波动较大。同时,脱离基体的大量SiCw未与基体形成磨屑掉落前,在摩擦面上SiCw的嵌入、垂直在基体中分布和脱落的材料在摩擦面上的滚动可能对摩擦副间的摩擦具有正向作用,故其摩擦系数整体较小。
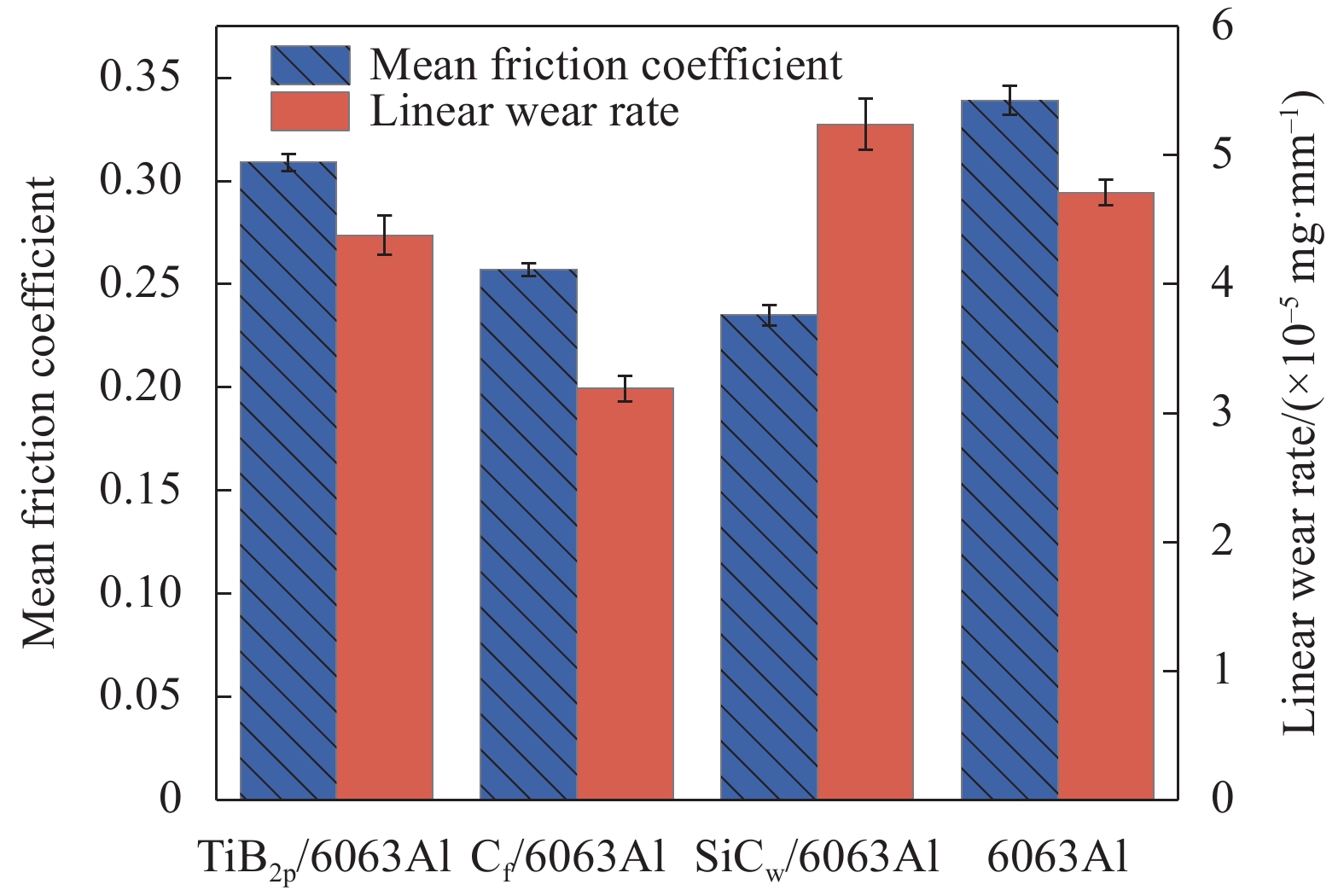
由图6中6063Al基复合材料的线磨损率可以看出,6063Al基体的线磨损率为4.71 × 10−5 mg/mm。三种复合材料中,Cf/6063Al复合材料的线磨损率(3.19 × 10−5 mg/mm)最低,较6063Al基体降低了32.27%。TiB2p/6063Al的线磨损率较6063Al基体降低7.01%,而SiCw/6063Al的线磨损较6063Al基体升高11.25%。结合摩擦系数可以看出,除了SiCw/6063Al复合材料外,其余材料的摩擦系数与磨损率基本呈正相关,这与Zhai等人的研究结果一致[40]。在空载和室温条件下,电流对晶格间原子排列的影响相对较小,但摩擦会引起晶粒的塑性变形,产生大量的层错、位错等缺陷,导致原子间的不规则排列,这阻碍了电流的分布和流动,使得局部的焦耳热增加,晶粒在一定程度上软化,随着热量的积累和磨损加剧,基体材料会因软化、熔化发生粘着磨损而被消耗[41]。在磨损过程中,接触表面即将脱落的增强相材料与软化的铝基体继续参与磨损,在力的作用形成了一层机械混合层,对复合材料的次表面起到好的保护作用。
随着磨损的增加,原有的机械层被破坏,磨损率增加,进而形成新的机械层[9]。Cf/6063Al复合材料的线磨损率最小,耐磨损性能最优。碳纤维轴向力学性能的各向异性使得Cf/6063Al复合材料在摩擦时纤维能够承担主要的载荷,这可以抑制裂纹的形成和扩展,有效地防止材料在磨损过程中的脱落,从而减小材料的磨损。在摩擦时,Cf/6063Al复合材料受到机械磨损和电磨损的共同作用,基体材料逐渐损耗,碳材料在磨损表面聚集、研磨、破碎,形成一层碳润滑相[42],这能有效减少材料的机械磨损。同时,Cf具有一定的分散和稳定电弧的作用,可使材料避免受到集中的电弧侵蚀,有效减少了材料的电磨损[37]。TiB2p/6063Al复合材料在摩擦时,随着6063Al基体的损耗,硬质颗粒会暴露出来直接与摩擦盘接触滑动。TiB2p/6063Al复合材料的硬度虽然最高,在一定程度上可以增强材料抵抗塑性变形的能力,但TiB2p与6063Al之间的界面结合属于机械结合,结合强度不高,在摩擦时TiB2p容易被拖拽出形成磨粒,导致磨损表面的粗糙度增大,摩擦副之间的接触面积减少,电流密度增大,这会使接触面“起弧”,加重电磨损的程度,从而使得其线磨损率较6063Al基体仅降低了7.01%。SiCw/6063Al复合材料的表面存在大量的微孔等缺陷,在电流的条件下,微孔等缺陷处电流密集,会产生严重的电弧侵蚀。与基体结合不牢固的SiCw脱落时,摩擦表面会形成大量的电火花,同时产生大量热能对表面进行侵蚀熔化。由于SiCw/6063Al复合材料的内部缺陷较多,使得磨损表面材料剥落速度加快,这严重影响了复合材料的耐磨损性能。
2.3.2 载流质量
载流质量包括载流效率和载流稳定性,载流效率是载流摩擦过程中电信号传导的效率,该值越大,载流能力越强;载流稳定性是衡量载流摩擦副传输电流稳定性的特征参数,该值越小,代表载流越稳定[9, 10]。
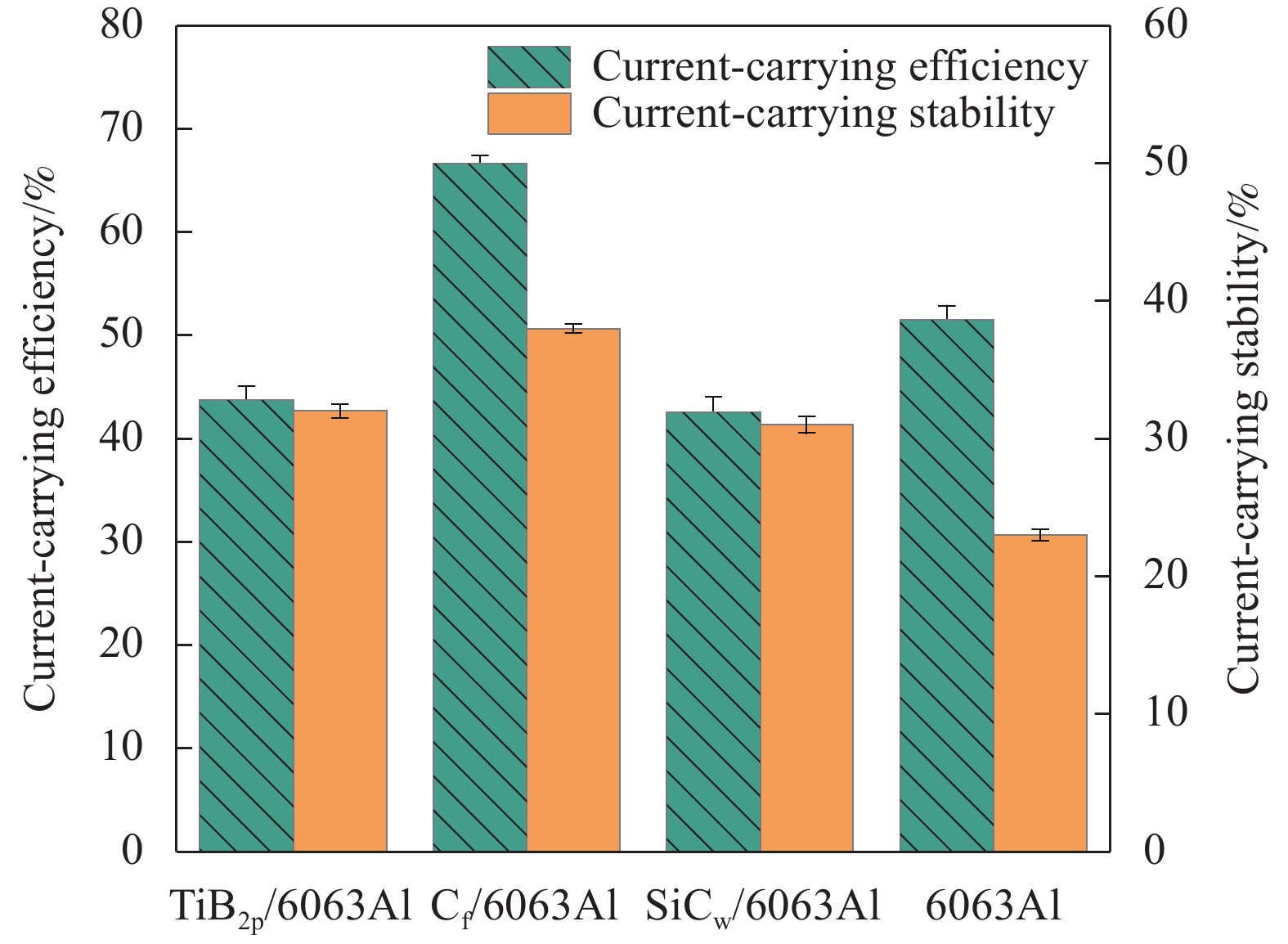
图7是6063Al基复合材料的载流效率和载流稳定性。可以看出,6063Al基复合材料的载流效率均不超过70%,其中Cf/6063Al复合材料的载流效率(66.61%)最高,较6063Al基体提升29.44%,TiB2p/6063Al和SiCw/6063Al复合材料的载流效率分别较6063Al基体降低15.02%和17.39%。载流效率与摩擦接触面的平整程度、接触情况及材料的导电率有关[9, 20, 42]。6063Al基体的导电率最高,增强相加入后,复合材料的导电率下降,这是6063Al基体的载流效率比复合材料的载流效率高的重要原因之一。其中,Cf/6063Al复合材料的载流效率较6063Al基体提升较多,这是由于:(1) Cf/6063Al复合材料的导电率下降最少,承载和传输电子的效率较高;(2) 磨损表面的碳材料,不仅起到分散电弧的作用,而且增加了摩擦接触面的润滑性,破碎的纤维填充在软化的基体表面,使
得摩擦副接触面积的增大和电流更加均匀的分布;(3) 由瞬时摩擦系数的稳定阶段可知,摩擦副之间的接触情况较好这均是Cf/6063Al复合材料载流效率较6063Al基体得到了大幅度提升的原因。然而,当Cf被磨断、破碎或拔出重新分布在基体表面时,引起磨损表面的凹凸会影响材料的载流稳定性。在摩擦时,TiB2p/6063Al复合材料中的TiB2p会降低摩擦面的平整度,使得摩擦副的实际接触面积减小,电流密度增加,电阻增大,接触面间的状态不稳定,导致载流效率和稳定性较差。在摩擦时,SiCw/6063Al复合材料的磨损最严重,同时材料表面的缺陷会引起大量微小电弧的产生,加剧磨损,这都导致SiCw/6063Al复合材料的载流质量最差。
2.4 6063Al复合材料磨损微观形貌及磨损机制
2.4.1 磨损微观形貌
图8是6063Al基复合材料磨损表面的微观形貌。可以看出,6063Al基复合材料的磨损表面均以熔融烧蚀、片层状磨损、犁沟等形貌为主。
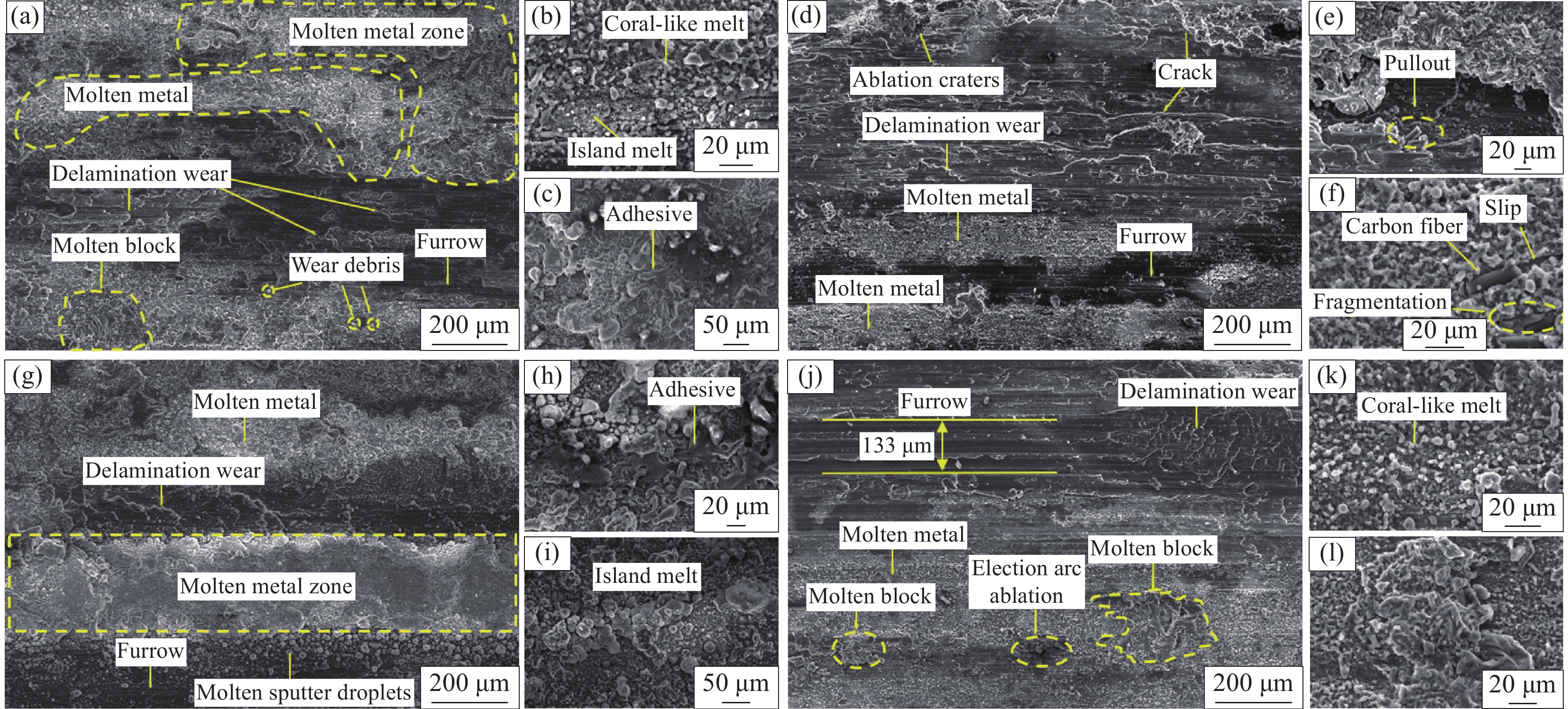
由图8(a)可以看出,TiB2p/6063Al复合材料的磨损表面存在少许磨粒、大面积的金属熔融区、大量的金属熔融块和局部片状磨损区,犁沟区域比6063Al基体磨损表面浅。放大倍数观察发现(见图8(b、c)),熔融区域存在珊瑚状熔融物和尺寸较小的岛状熔融物,粘着痕迹明显,磨损机制主要以磨粒磨损、粘着磨损和电弧侵蚀为主。在摩擦磨损过程中,电流的加入使销试样和摩擦盘之间产生电弧,接触面温度升高,最终热量累积导致磨损表面被烧蚀产生烧蚀坑和金属熔融物。
由图8(d)可以看出,Cf/6063Al复合材料的磨损表面较其余铝基复合材料更平整,可以清晰地观察到金属熔化区形成的熔体较小,犁沟深度较6063Al材料的浅,并伴随有裂纹产生。由图8(e、f)可以看出,Cf裸露在磨损表面,一部分Cf被破碎和拔出,粘着现象减轻。可以看到明显的剥落坑(见图8(e))和纤维在基体表面轻微的滑动现象(见图8(f)),磨损机制主要以磨粒磨损和电弧侵蚀为主。摩擦过程中,Cf起主要的承载作用[43, 44],随着材料的损耗,碳纤维在磨损表面研磨和破碎,形成碳润滑层[39, 43],这极大地降低了复合材料的摩擦系数和磨损率。
由图8(g)可以看出,SiCw/6063Al复合材料的磨损表面的片层状磨损区域较小,存在大面积的金属熔融区和小尺寸熔块,出现严重的电弧侵蚀。从图8(h、i)可以看出,熔融区域周围伴随有许多岛状熔融金属冷却后的凝固液滴,电弧烧蚀导致磨损表面局部熔化严重,粘着痕迹较多,存在许多粘着块,其磨损机制主要以粘着磨损、磨粒磨损和电气磨损为主。
由图8(j)可以看出,6063Al基体材料的磨损表面沿摩擦方向存在较深且数量较多的犁沟和大范围的片层状磨损区。犁沟区域附近存在机械混合层,在摩擦时容易产生粘着磨损。电流作用下,6063Al基体材料出现电弧烧蚀区,金属熔化形成尺寸较大的熔融区,该区域附近存在许多珊瑚状熔融物(见图8(k))和大尺寸的熔块(见图8(l))。此外,磨损表面清晰可见一条宽约133 μm左右的犁沟带,这可能是熔融液态金属铝在电弧烧蚀后产生的液滴沿着摩擦方向发生瞬时冷焊,形成的磨粒造成磨粒磨损的结果。磨损机制主要以粘着磨损、磨粒磨损和电弧侵蚀为主。
2.4.2 磨损三维形貌
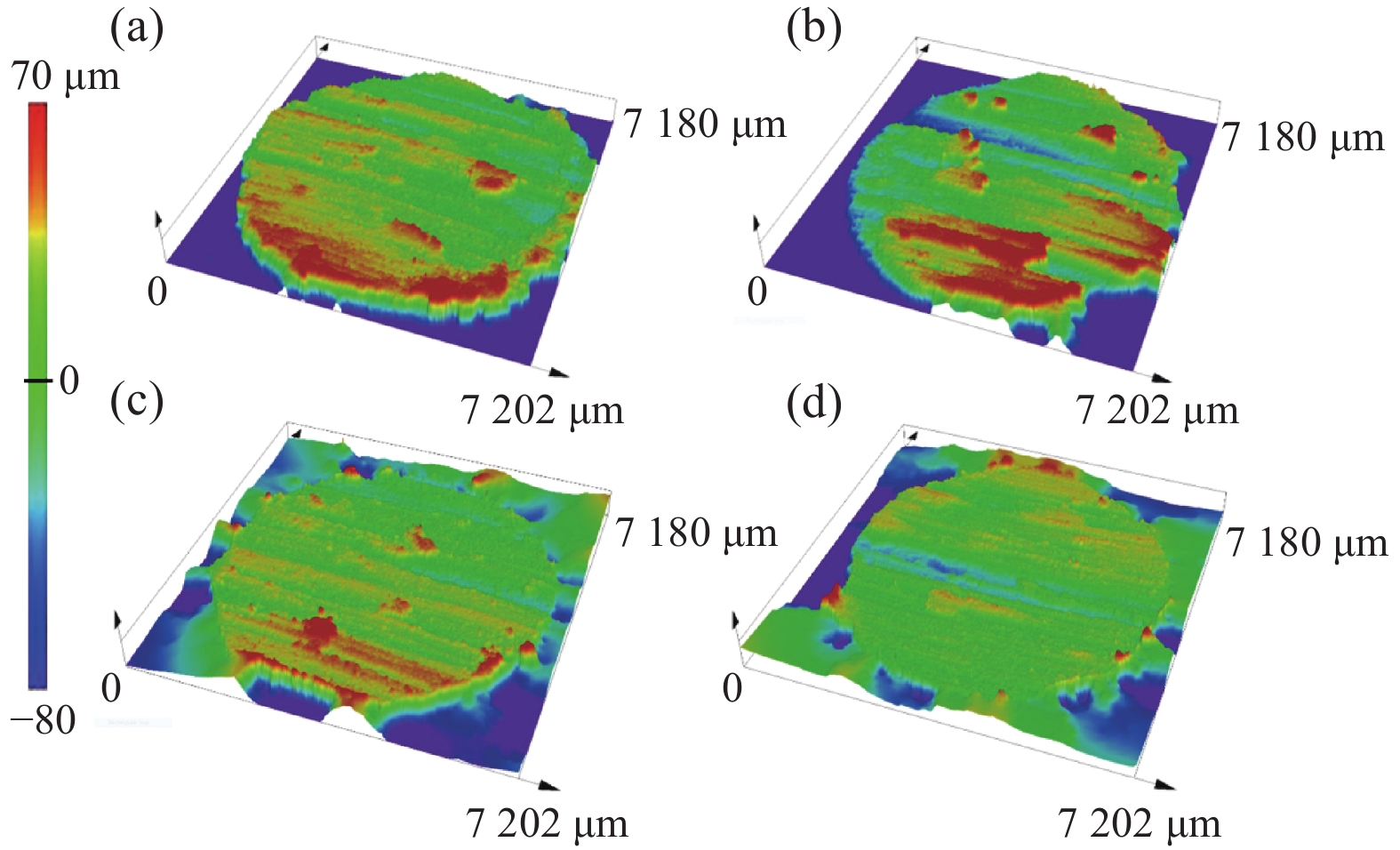
图9是6063Al基复合材料磨损表面的三维高度示意图。可以看出,磨损率较高的6063Al基体材料及SiCw/6063Al复合材料的表面存在较少的熔融金属残留物,磨损率较低的TiB2p/6063Al和Cf/6063Al复合材料表面的表面凸起较多。这是由于6063Al基体在摩擦时粘着磨损较严重,没有增强相的支撑和承载作用,表面软化和熔化的部分会更易形成磨屑被磨损消耗,使得最终的磨损面高度差较小。SiCw/6063Al复合材料在摩擦时由于SiCw的易脱落性、复合材料表面和内部的缺陷使得材料容易脱离飞溅形成磨屑,其磨损表面存在一部分凸起。在摩擦时,TiB2p和Cf阻碍基体的剥落作用更好,机械层维持时间较长。值得注意的是,Cf对基体的承载作用最佳,机械层留在Cf/6063Al复合材料磨损表面的面积更大、时间更久,这有利于载流摩擦磨损的进行,同时降低磨损率。
2.4.3 磨损机制
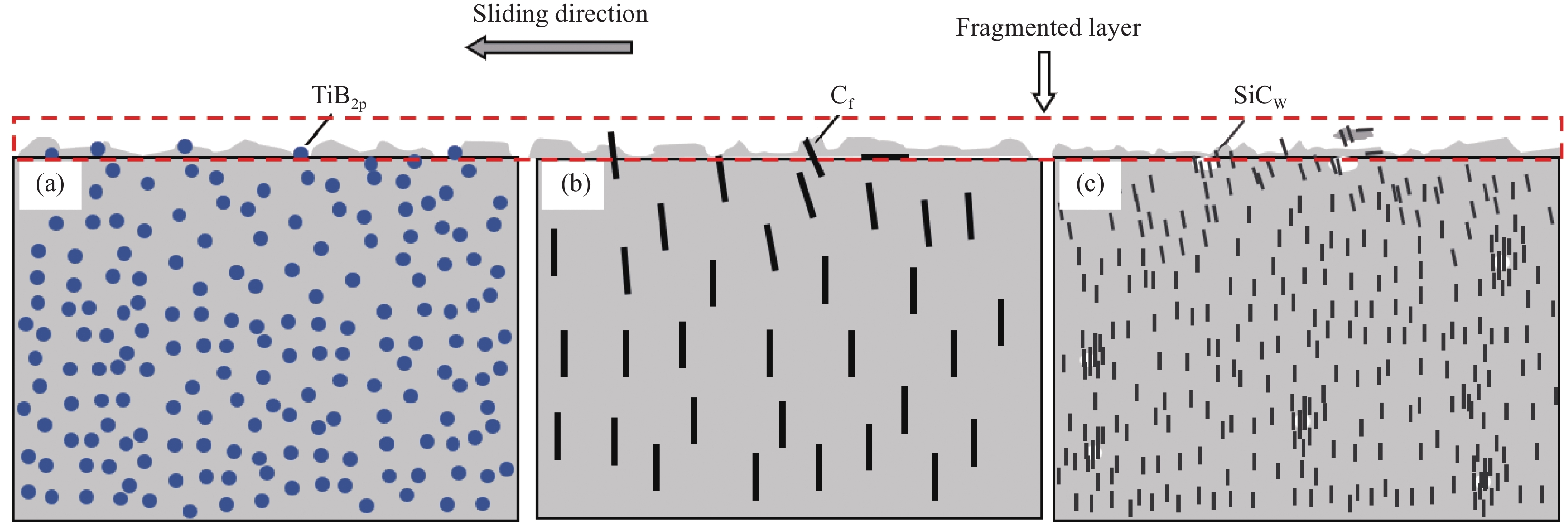
图10是6063Al基复合材料的磨损机制示意图。由磨损表面的微观形貌分析可知,TiB2p/6063Al复合材料的磨损机制主要以磨粒磨损、粘着磨损和电弧侵蚀为主。由图10(a)可以看出,由于TiB2p/6063Al复合材料的硬度较高,在摩擦过程中,TiB2p起到支撑作用,有利于加快摩擦表面的热量散失,减少了粘着磨损痕迹。同时,TiB2p的存在提高基体中增强相周围的位错密度[10, 45],减少裂纹的萌生和扩展,也阻碍了基体材料在摩擦高热时的金属流动。当基体软化时,TiB2p嵌入到基体中继续参与磨损,从而阻碍材料的磨损脱落,降低了磨损率。Cf/6063Al复合材料的磨损机制主要以磨粒磨损和电弧侵蚀为主。由图10(b)可以看出,Cf作为减磨和润滑材料,摩擦时起到承担主要载荷和分散电弧的作用,随着磨损的进行,破碎、裸露和拔出(见图8(b1))的Cf在基体表面形成一层碳润滑膜[37],不仅起到固体润滑的作用,而且阻止了摩擦副金属间的直接接触,可有效地改善材料的摩擦系数和降低磨损率。SiCw/6063Al复合材料的磨损机制主要以粘着磨损、磨粒磨损和电气磨损为主。由图10(c)可以看出,SiCw/6063Al复合材料的硬度虽然比基体材料高,但是SiCw与基体材料结合较弱,且表面及内部存在大量缺陷,导致摩擦时缺陷处的SiCw会大量脱落,销试样在摩擦盘表面上的反复挤压和摩擦面上的切削力使磨损更加严重,在电流和摩擦力的作用下,与熔化后飞溅的金属基体一同脱落,这使得SiCw/6063Al复合材料的整体摩擦系数不稳定,磨损率也高于基体材料。
3. 结 论
(1)相同体积分数下,TiB2p和Cf在6063Al基体中分布较均匀,而SiCw/6063Al复合材料中的SiCw存在部分团聚现象。不同类型增强相对6063Al基体的硬度有不同幅度的提升效果,而致密度和导电率均有不同程度的降低。其中,TiB2p/6063Al复合材料的硬度最高,其值为68.38 HB,较6063Al提升11.55%,Cf/6063Al和SiCw/6063Al的硬度较基体分别提升3.43%和10.51%。
(2)不同类型增强相的添加使6063Al基复合材料摩擦系数的稳定性和均值有不同程度的降低,对磨损率和载流质量的影响不同。综合来看,Cf/6063Al复合材料的载流摩擦磨损性能最优异,载流摩擦系数的波动性最小,出现稳定摩擦滑动阶段。同时,其线磨损率最小、载流效率最高,线磨损率(3.19 × 10−5 mg/mm)较6063Al减少32.27%,载流效率(66.61%)较6063Al基体提升29.44%。
(3)载流摩擦条件下,Cf/6063Al复合材料的磨损表面拥有更平整的微观形貌,较少的金熔融属物。Cf承担主要载荷,并在磨损表面形成一层碳润滑膜,磨损机制主要以磨粒磨损和电弧侵蚀为主。TiB2p/6063Al复合材料的磨损机制主要以磨粒磨损、粘着磨损和电弧侵蚀为主,SiCw/6063Al复合材料主要以粘着磨损、电气磨损为主。
-
表 1 聚二甲基硅氧烷(PDMS)-Nylon6复合材料力学性能
Table 1 Mechanical properties of Polydimethylsiloxane (PDMS)-Nylon6 composite materials
Material Elastic modulus/
MPaPoisson's ratio Density/
(kg⋅mm−3)Nylon6 1.06 0.35 1.14×10−6 PDMS 1.8 0.49 2.33×10−6 表 2 PDMS-Nylon6复合材料柔性蒙皮模型边界条件
Table 2 Boundary conditions of the PDMS-Nylon6 composite flexible skin model
Boundary Boundary of in-plane
load conditionsBoundary of out-plane load conditions A ux=uy=uz=0
γx=γy=γz=0ux=uy=uz=0
γx=γy=γz=0B Fx
γx=γy=γz=0ux=uy=uz=0
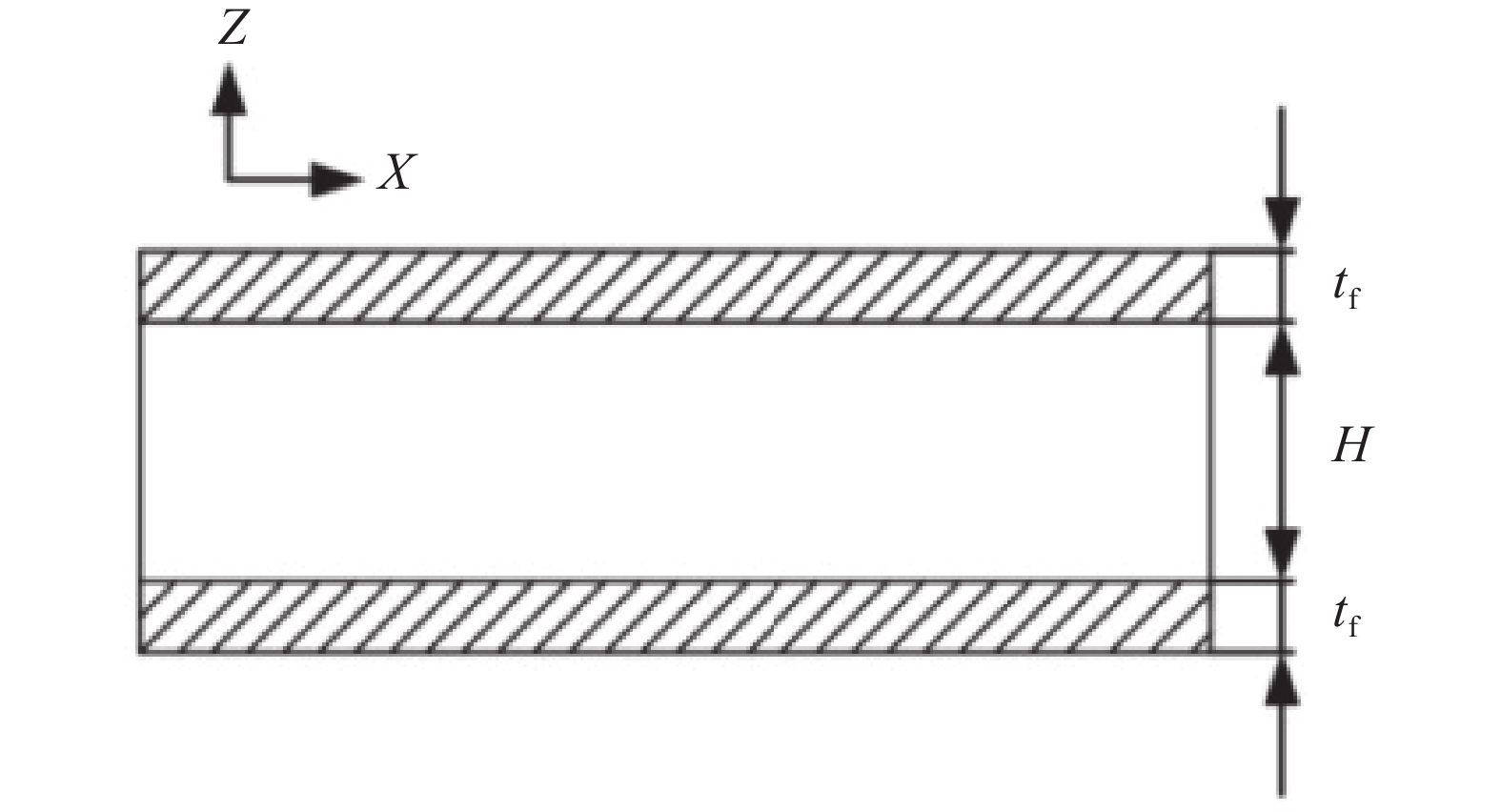
γx=γy=γz=0C Free Free D Free Free E Free Free F Free Pn=0.001MPa 表 3 PDMS-Nylon6复合材料柔性蒙皮均匀实验设计
Table 3 Uniform experimental design for PDMS-Nylon6 composite flexible skin
Parameters Levels 1 2 3 4 5 L1/mm 4.5 4.75 5 5.25 5.5 L2/ mm 6.75 7.125 7.5 7.875 8.25 θ /(°) 31.5 33.25 35 36.75 38.5 H /mm 1.8 1.9 2 2.1 2.2 t /mm 0.45 0.475 0.5 0.525 0.55 tf/ mm 0.9 0.95 1 1.05 1.1 F / N 450 475 500 525 550 Notes: F is the external load. 表 4 PDMS-Nylon6复合材料柔性蒙皮近似模型的评估
Table 4 Evaluation of the approximated model for PDMS-Nylon6 composite flexible skin
εx μ ω G R 0.9996 0.9999 0.9999 1.0 ˉe 0.99% 0.32% 0.83% 0.05% σe 1.20% 0.24% 0.67% 0.06% Notes: εx is the in-plane tensile strain; μ is the Poisson’s ratio; ω is the out-of-plane deflection; G is the structural mass; R is the correlation coefficient; ˉe is the mean of the relative error; σe is the standard deviation of the relative error. 表 5 优化前后PDMS-Nylon6复合材料柔性蒙皮的几何参数对比
Table 5 Comparison of geometric parameters of PDMS-Nylon6 composite flexible skin before and after optimization
Variable Initial value Optimized value L1/mm 5 4.5 L2/ mm 7.5 7.3164 θ /(°) 35 38.5 H /mm 2 2.2 t /mm 0.65 0.45 tf/ mm 1 0.9 表 6 优化前后PDMS-Nylon6复合材料柔性蒙皮的性能对比
Table 6 Comparison of PDMS-Nylon6 composite flexible skin performance before and after optimization
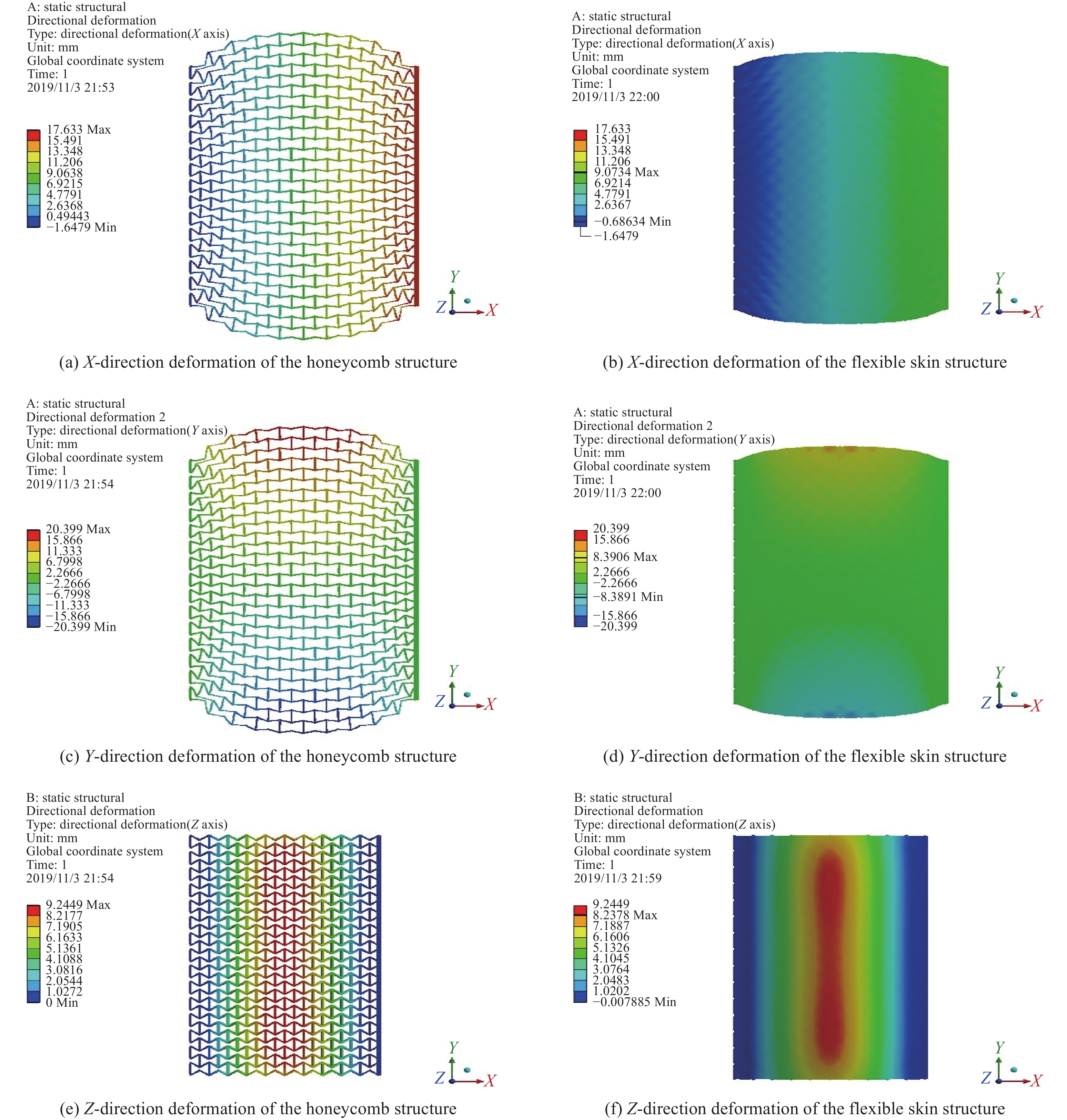
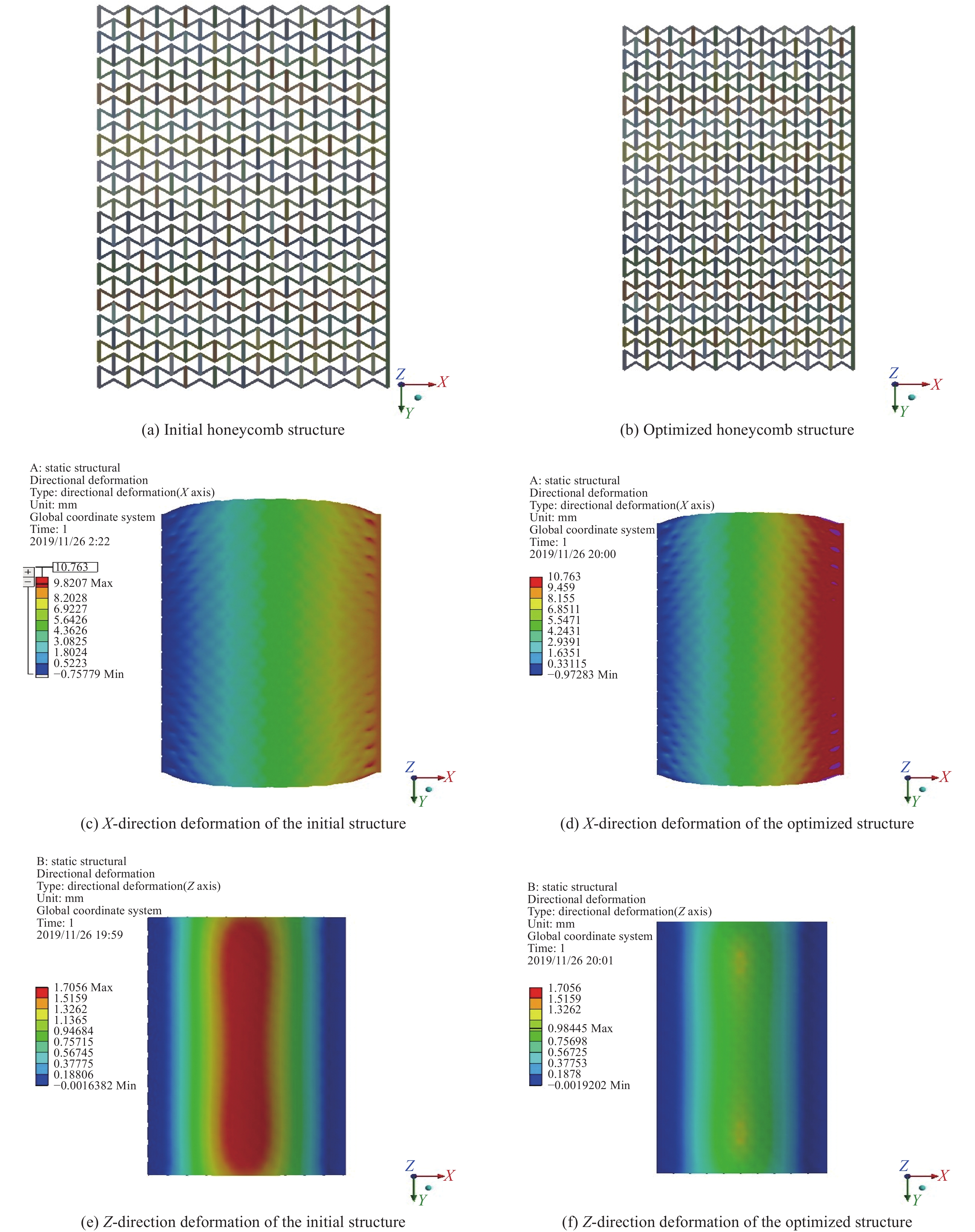
Variable Initial value Optimized value Percent change εx 23.92% 31.27% 30.73% μ 1.3886 1.1008 -20.73% ω 1.6% 1.12% -30% G 0.0584 0.0451 -22.77% F(x) 0.3522 0.0740 -78.99% -
[1] Weisshaar T A. Morphing aircraft systems: historical perspectives and future challenges[J]. Journal of Aircraft, 2013, 50(2): 337-353. DOI: 10.2514/1.C031456
[2] Sofla A, Meguid S A, Tan K T, et al. Shape morphing of aircraft wing: status and challenges[J]. Materials and Design, 2010, 31(3): 1284-1292. DOI: 10.1016/j.matdes.2009.09.011
[3] Joshi S P, Tidwell Z, Crossley W A, et al. Comparison of morphing wing strategies based upon aircraft performance impacts[J]. Proceedings of the 45th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference, Palm Springs, CA: AIAA, 2004, 1722: 1-7.
[4] Jha A K, Kudva J N. Morphing aircraft concepts, classifications, and challenges. Anderson E H, Proc. SPIE 5388, Smart Structures and Materials 2004: Industrial and Commercial Applications of Smart Structures Technologies, San Diego, CA: International Society for Optics and Photonics, 2004: 213-224.
[5] Reich G, Sanders B. Introduction to morphing aircraft research[J]. Journal of Aircraft, 2007, 44(4): 1059. DOI: 10.2514/1.28287
[6] 任鑫, 张相玉, 谢亿民. 负泊松比材料和结构的研究进展[J]. 力学学报, 2019, 51(3): 656-687. DOI: 10.6052/0459-1879-18-381 Ren Xin, Zhang Xiangyu, Xie Yimin. Research progress in auxetic materials and structures[J]. Chinese Journal of Theoretical and Applied Mechanics, 2019, 51(3): 656-687(in Chinese). DOI: 10.6052/0459-1879-18-381
[7] 沈元, 昂海松, 刘卫东. 用于变形机翼夹心式柔性伸缩蒙皮的梯形蜂窝支撑结构[J]. 复合材料学报, 2015, 32(3): 8158-22. She Y, Ang HS, Liu W D. Trapezoidal cellular support structure applied to flexible telescopic sandwich skin of morphing wing[J]. Acta Materiae Compositae Sinica, 2015, 32(3): 8158-22(in Chinese).
[8] Olympio K R, Gandhi F. Flexible skins for morphing aircraft using cellular honeycomb cores[J]. Journal of Intelligent Material Systems and Structures, 2010, 21(17): 1719-1735. DOI: 10.1177/1045389X09350331
[9] Olympio K R, Gandhi F. Zero Poisson’s ratio cellular honeycombs for flex skins undergoing one-dimensional morphing[J]. Journal of intelligent material systems and structures, 2010, 21(17): 1737-1753. DOI: 10.1177/1045389X09355664
[10] Bubert E A, Woods B K, Lee K, et al. Design and fabrication of a passive 1D morphing aircraft skin[J]. Journal of Intelligent Material Systems and Structures, 2010, 21(17): 1699-1717. DOI: 10.1177/1045389X10378777
[11] Murugan S, Saavedra Flores E I, Adhikari S, et al. Optimal design of variable fiber spacing composites for morphing aircraft skins[J]. Composite Structures, 2012, 94(5): 1626-1633 DOI: 10.1016/j.compstruct.2011.12.023
[12] Olympio K R, Gandhi F. Skin designs using multi-objective topology optimization[J]. Proceedings of the 49th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Schaumburg, IL: AIAA, 2008, 1793: 1-26.
[13] Liu Weidong, Zhu Hua, Zhou Shengqiang, Bai Yalei, Zhao Chunsheng. Topology Optimization of Support Structure of Telescope Skin Based on Bit-matrix Representation NSGA-II[J]. Chinese Journal of Aeronautics, 2013, 26(6): 1422-1429 DOI: 10.1016/j.cja.2013.07.046
[14] 刘明, 李永新, 吴金玺, 等. 基于响应面法的负泊松比蜂窝芯结构多目标优化[J]. 现代制造工程, 2015, (10): 1-4. DOI: 10.3969/j.issn.1671-3133.2015.10.001 Liu Ming, Li Yongxin, Wu Jinxi, et al. Structure multi-objective optimization of negative Poisson’s ratio honeycomb core based on response surface method[J]. Modern Manufacturing Engineering, 2015, (10): 1-4(in Chinese). DOI: 10.3969/j.issn.1671-3133.2015.10.001
[15] De shanShan, Qiao Li, InamullahKhan, et al. A novel finite element mo del updating method based on substructure and response surface model[J]. Enginee ring Structures, 2015, 103.
[16] Henikar G, Palmer G M. Response-Surface Methodology-Revisited[J]. Cereal Food Science, 1976, 21: 432-445.
[17] Henikar G, Use of Response-Surface Methodology in Sensory Evaluation[J]. Food Technology, 1982, 36: 96-101.
[18] Bucher C G, Bourgund U. A Fast and Efficient Response Surface Approach for Structural Reliability Problems[J]. Structural Safety, 1990, 7(1): 57-66. DOI: 10.1016/0167-4730(90)90012-E
[19] Goldberg D E, Holland J H. Genetic algorithms and machine learning[J]. machine learning, 1988, 3(2): 95-99.
[20] Wright A H. Genetic algorithms for real parameter optimization[J]. Foundations of Genetic Algorithms, 1991, 1: 205-218.
[21] 胡春幸, 侯玉亮, 铁瑛, 等. 基于遗传算法的碳纤维增强树脂复合材料层合板单搭胶接结构的多目标优化[J]. 复合材料学报, 2021, 38(6): 1847-1858. HU Chunxing, HOU Yuliang, TIE Ying, et al. Multi-objective optimization of adhesively bonded single-lap joints of carbon fiber reinforced polymer laminates based on genetic algorithm[J]. Acta Materiae Compositae Sinica, 2021, 38(6): 1847-1858(in Chinese).
-
-
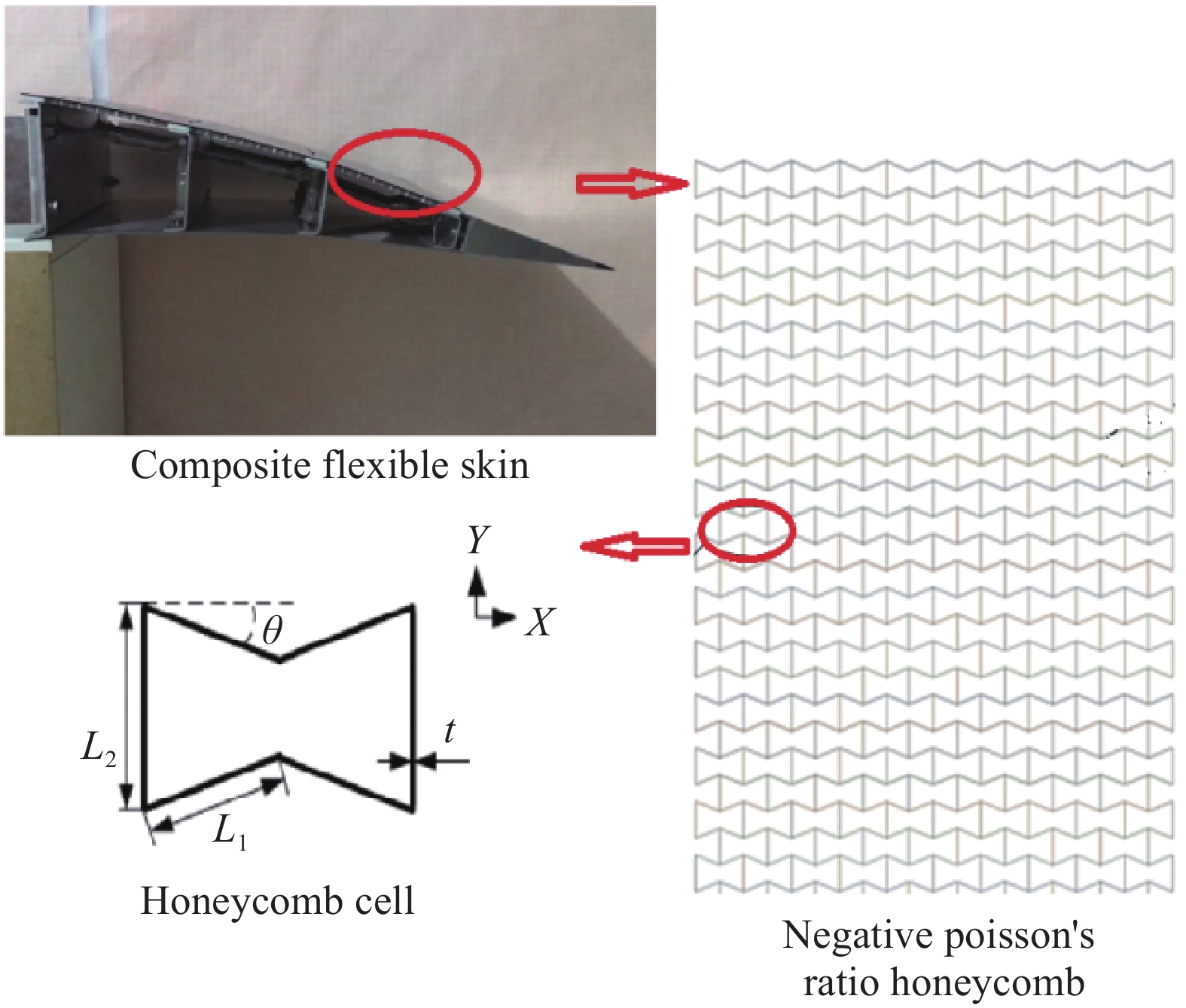
柔性蒙皮技术是变体飞机机翼的关键技术之一,其主要作用是在变形时承受并传递气动载荷,保证机翼表面光滑。由蜂窝夹芯的和弹性表皮构成的复合式柔性蒙皮,是当前重要的柔性蒙皮构型之一。对于此类柔性蒙皮,同时考虑面内大变形、面外大刚度、结构质量轻等目标,实现柔性蒙皮结构的优化设计,具有较大的技术挑战。
针对由负泊松比蜂窝夹芯和弹性表皮构成的复合式柔性蒙皮,提出了柔性蒙皮几何参数优化方法。首先,将柔性蒙皮的胞壁长度、胞壁夹角、蜂窝高度、胞壁厚度、表皮厚度等几何参数作为变量,设计了柔性蒙皮结构参数化建模方法;其次,通过均实验设计方法获取样本点,利用参数化方法构建柔性蒙皮结构有限元模型,并通过仿真获取各几何参数组合状态对应的结构性能;然后,基于响应面方法构造柔性蒙皮几何参数与结构性能关系的近似模型,并对近似模型的精度进行了定量评估;最后,以近似模型为基础,以柔性蒙皮几何参数为优化变量,综合考虑面内应变、泊松比、面外挠度、质量等因素,采用加权系数法构造了综合优化目标函数,并采用遗传算法进行优化,得到了柔性蒙皮结构综合性能最优的构型。构建了复合式柔性蒙皮有限元模型,进行优化方法验证,结果显示,优化后的构型与初始构型相比,面内变形能力提升30.73%,面外承载能力提高30%,结构质量减轻22.77%,泊松比降低20.73%。

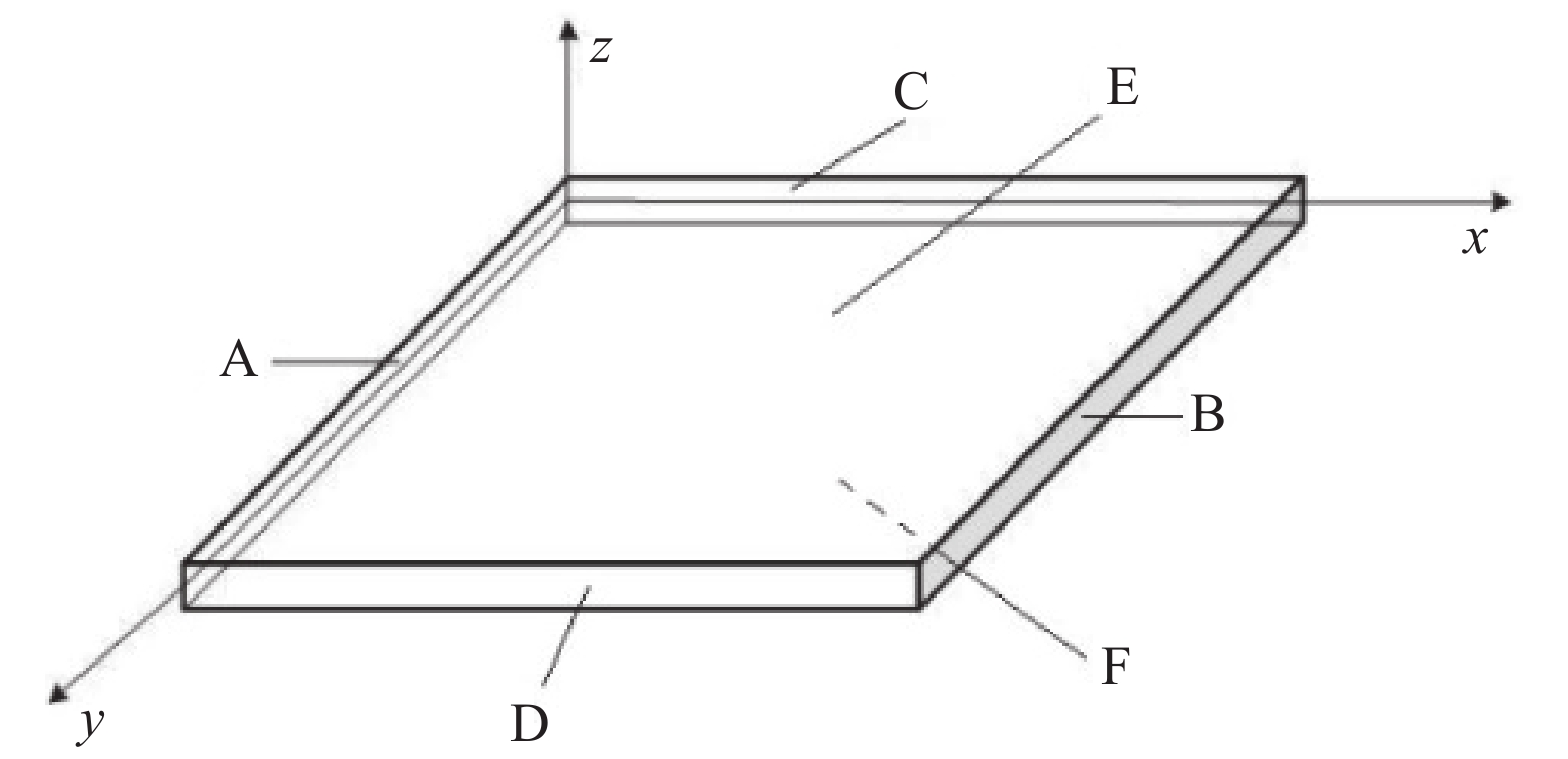
柔性蒙皮及蜂窝胞元示意图

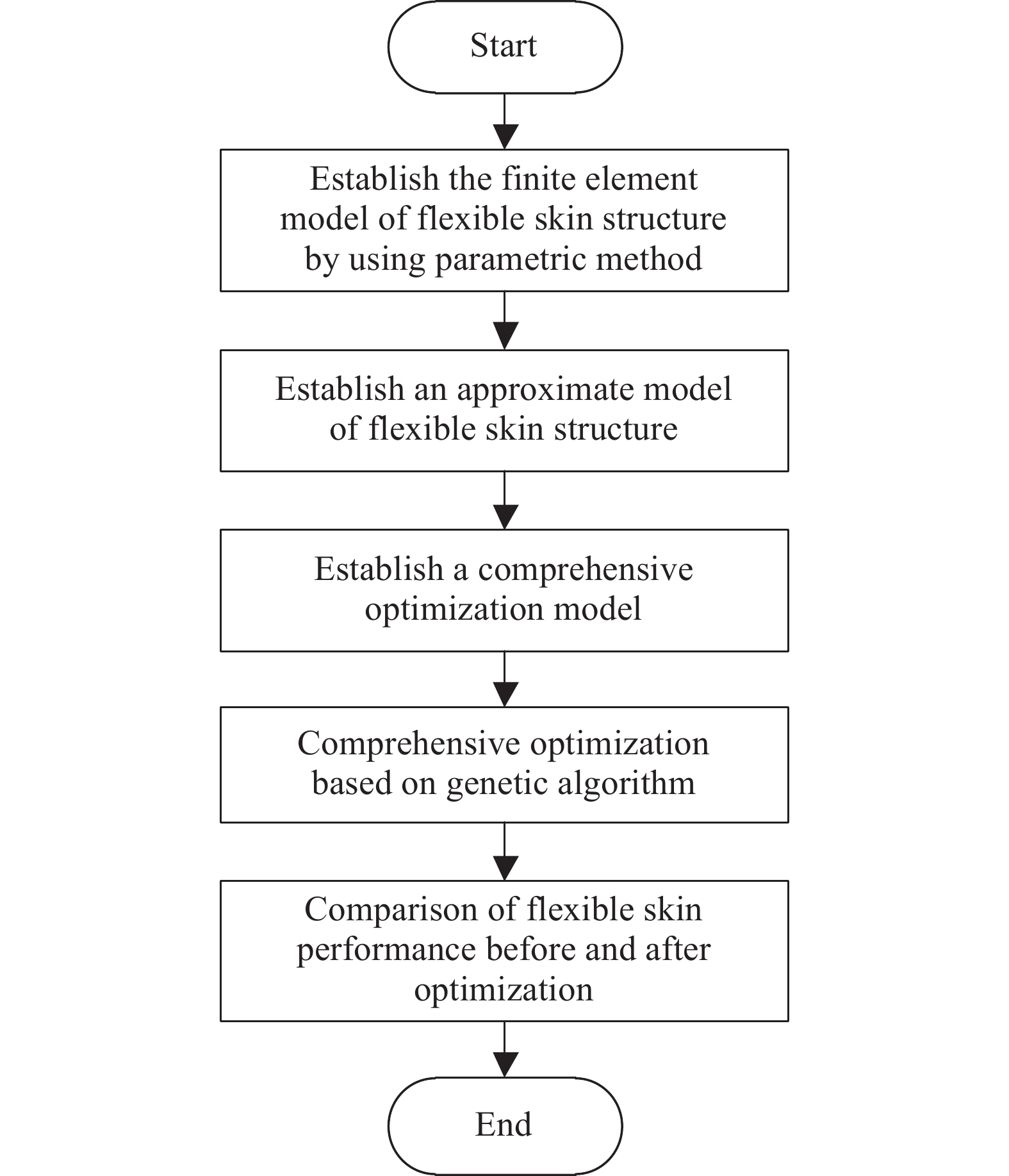
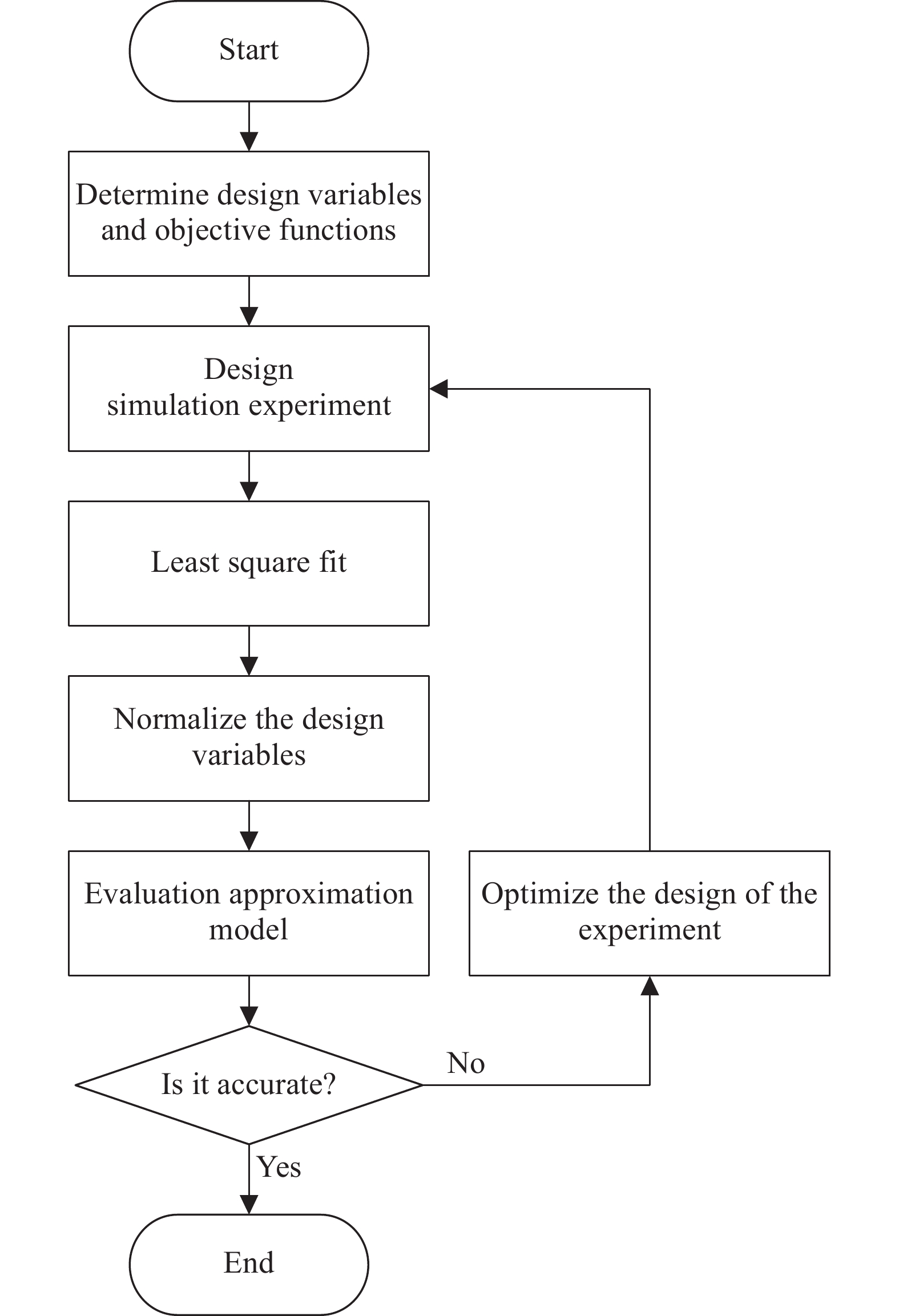
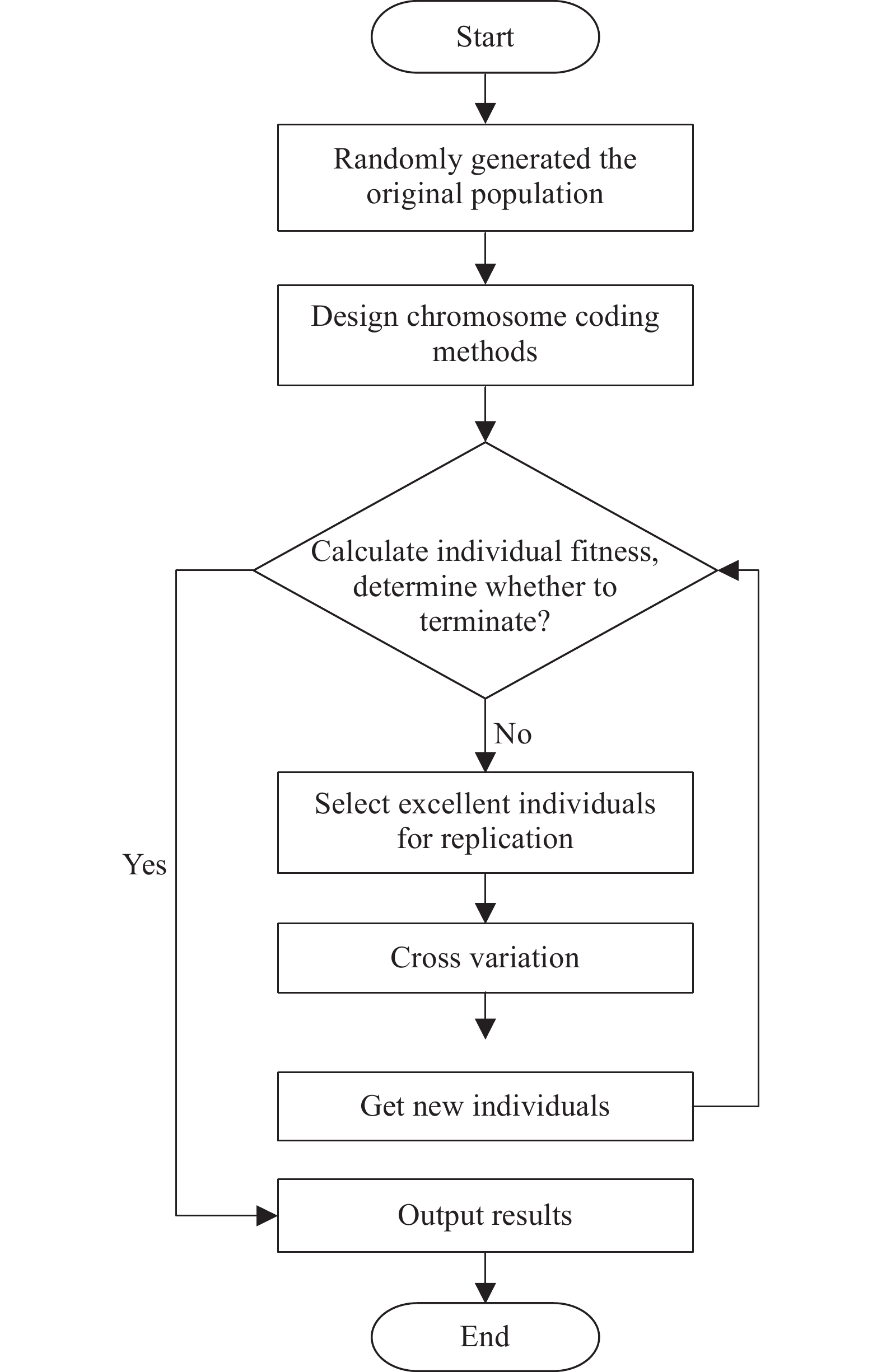
柔性蒙皮结构综合优化流程图

优化前后柔性蒙皮位移云图对比




 下载:
下载: