Study on plastic yield strength of Gyroid lattice structures based on functional principleson
-
摘要: 点阵结构与密实结构存在的力学性能差异之一表现在塑性屈服响应上，因此，研究其屈服行为可为点阵结构的设计和应用提供重要的理论依据。首先，对Gyroid点阵结构进行简化，并基于变形体功能原理建立其力学模型，得到Gyroid点阵结构塑性屈服强度与体积分数之间的映射关系；然后，基于有限元分析软件Abaqus对Gyroid点阵结构准静态压缩过程开展仿真实验，初步验证理论模型的准确性；最后，通过选择性激光熔化(SLM)制备不同体积分数316L不锈钢Gyroid点阵结构，进行单轴压缩实验，分析其变形机制与力学性能。结果表明：理论推导、有限元仿真结果与实验结果相比，误差在25%以内，且根据3种方法结果拟合得到的Gibson-Ashby模型系数具有较好的一致性，表明本文基于理论推导建立的Gyroid点阵结构塑性屈服强度预测模型的有效性。理论模型的构建方法可以转化到其他复杂类型点阵结构中，为快速核算点阵结构力学性能，并将其应用在工程装备中提供理论依据。Abstract: One of the differences in mechanical properties between lattice structures and dense structures lies in the plastic yield response. Therefore, studying their yield behavior can provide important theoretical basis for the design and application of lattice structures. Firstly, The Gyroid lattice structure was simplified and its mechanical model was established based on the principle of deformable body function, obtaining the mapping relationship between the plastic yield strength and volume fraction of the Gyroid lattice structure. Then, based on the finite element analysis software Abaqus, simulation experiments were conducted on the quasi-static compression process of Gyroid lattice structures to preliminarily verify the accuracy of the theoretical model. Finally, different volume fractions of 316L stainless steel Gyroid lattice structures were prepared by selective laser melting (SLM), and uniaxial compression experiments were conducted to analyze their deformation mechanism and mechanical properties. The results show that the error between theoretical derivation, finite element simulation results and experimental results is within 25%, and the coefficients of the Gibson-Ashby model fitted based on the results of the three methods have good consistency, indicating the effectiveness of the Gyroid lattice structure plastic yield strength prediction model established based on theoretical derivation. The construction method of theoretical models can be transformed into other complex types of lattice structures, providing a theoretical basis for quickly calculating the mechanical properties of lattice structures and applying them in engineering equipment.
-
渗透性是水泥基材料耐久性能研究的关键内容，材料的渗透性与其耐久性劣化过程密切相关，对水泥基材料的长期性能有重要影响[1]。目前，水泥基材料的抗渗测试有抗水渗透试验和抗氯离子渗透试验两种标准[2]。这两种方法仅能提供水或离子的最终渗透结果，无法全面分析渗透介质在基体中的渗透过程，因此缺乏对水泥基材料抗渗性能影响机理的数据支持。1H低场核磁共振技术是利用1H作为探针分析水泥基材料中水的分布和含量，具有灵敏、快速和无侵入的独特优势，为研究水泥基材料中水分的迁移、渗透提供新的研究方法。目前，相关研究主要集中于水泥基材料的孔结构表征、物相表征和水分动力学。Valckenborg等[3]通过低场核磁研究了水泥砂浆干燥过程中的水分布情况，发现干燥过程中水分首先从毛细孔散失。Heijden 等[4]用核磁共振技术成像分析了混凝土在加热干燥过程中水分的变化情况。Bohris等[5]基于梯度回波成像采集了静态水分布数据，并通过成像计算了毛细吸水扩散速率。Ai Zhang等[6]通过低场核磁研究发现白水泥干燥过程中自由水从大孔隙蒸发到小孔。
修补材料的服役过程中，材料的含水率决定侵蚀介质的迁移。因此，深入地研究修补材料中水的渗透和分布是分析修补材料耐久性能的关键。核磁共振可以通过获取正比于水含量的氢核的核磁信号来计算水分的含量，利用横向弛豫时间分布可以得到水泥基材料中水分的分布[7]。通过低场核磁检测不同的应力状态下水的横向弛豫时间和渗水量，可以有效地反映水泥基材料的抗渗透性能。通过分析修补材料中水分渗透的变化，可以有效揭示不同组分对抗渗性能的影响机理。
聚合物改性水泥基材料是一种典型的有机-无机复合材料，具有韧性强、粘结强度高、耐久性好等优点，在结构修补领域具有广阔的应用前景[8]。聚合物有乳液和可再分散乳胶粉(以下简称聚合物)两种形式。可再分散乳胶粉是聚合物乳液经过特殊处理、喷雾干燥后，得到“壳-核”结构的聚合物颗粒，性能与乳液相似，但具有不影响水灰比、使用方便的优点。相关研究表明由于干燥失水和水泥水化消耗水，分散在水泥浆体中的聚合物粒子经历逐渐浓缩、密实堆积、团聚变形、融合成膜等过程，最终在水泥浆体孔隙、C-S-H凝胶间、氢氧化钙表面和界面过渡区形成连续的聚合物膜，提高水泥基材料的抗渗性能[9,10]。同时，聚合物膜可以有效地提高水泥基材料的抗折和粘结强度[11]。梅军鹏等[12]研究发现纳米二氧化钛与苯丙乳液复合改善乳液引起的早期强度降低及对水化的延迟作用，提高水泥基材料的抗渗性。李国忠等[13]研究发现醋酸乙烯酯-乙烯共聚聚合物能够改善了水泥基路面裂缝灌浆材料的可灌性，有效提高了材料的黏结性能及抗渗性能。
本文通过1H低场核磁共振技术测试了渗透压力、渗透时间和轴压对聚合物修补砂浆中可蒸发水的横向弛豫时间和渗水量，并以此表征修补砂浆的抗渗性。通过孔结构和微观形貌分析了聚合物对修补砂浆横向弛豫时间和渗水量的影响机理，为水泥基材料的抗渗性研究提供了一种新的思路。
1. 实验材料及方法
1.1 原材料
采用抚顺水泥股份有限公司生产的P·I 42.5水泥，表1和表2分别为水泥的化学成分和物理力学性能；聚合物采用德国瓦克公司生产的5044N型可再分散乳胶粉(图1)，其主要成分是醋酸乙烯酯-乙烯聚合物；减水剂选用瑞士西卡530P改性聚羧酸盐减水剂；消泡剂选用临沂市凯奥化工有限公司的金属皂类消泡剂；砂为ISO标准砂；水为自来水。
表 1 水泥的化学组成Table 1. Chemical compositions of cementSiO2 Al2O3 Fe2O3 CaO MgO SO3 Na2O f-CaO Loss 20.6 4.57 3.29 63.27 2.59 2.11 0.55 0.76 2.15 表 2 水泥物理力学性能Table 2. Physical and mechanical properties of cementSetting time/min Flexural strength/MPa Compressive strength/MPa Initial set Final set 3 d 7 d 28 d 3 d 7 d 28 d 128 196 5.3 6.4 8.5 26 35.9 45.6 1.2 配合比
修补砂浆的胶砂比为1∶2，水灰比为0.35，减水剂选用聚羧酸减水剂，掺量为0.2%，同时采用掺量为0.5%消泡剂调整砂浆含气量。水泥质量的0、3%、6%、9%、12%、15%的聚合物改性修补砂浆的抗渗性能，依次标记为K0、K3、K6、K9、K12、K15，砂浆具体的配合比见表3。
1.3 试验方法
1.3.1 制备工艺
按照试验配合比将水泥、聚合物、减水剂和标准砂在砂浆搅拌机中搅拌2 min。将预先溶有减水剂的水加入到搅拌锅中，慢搅2 min(自转(140±5) r/min，公转(62±5) r/min)使浆体混合均匀，然后浇筑于模具。试样成型1 d后脱模，并在温度(20 ± 2)℃、相对湿度95%环境中养护到指定龄期。
1.3.2 力学性能
参照GB/T 17671-2021《水泥胶砂强度检验方法》[14]测试修补材料的3 d、7 d和28 d的抗折、抗压强度。采用界面弯拉强度表征修补砂浆粘结强度，首先制备40 mm×40 mm×80 mm的标准砂浆试块作为粘结基体。在标准养护条件下养护28 d，然后将标准水泥砂浆试块放入40 mm×40 mm×160 mm模中的一侧，另一侧倒入聚合物改性修补砂浆，在养护到指定龄期后测试三点抗折强度：
R=1.5FL/b3 (1) 式中：R为粘结强度(MPa)；F为断裂时的荷载(N)；L为支撑圆柱的距离(100 mm)；b为棱柱体正方形截面边长(40 mm)。
表 3 聚合物改性修补砂浆配合比Table 3. Mix proportion of polymer modified repair mortarSpecimen No. Cement/g Sand/g Superplasticizer/g Defoamer/g Water/g Polymer/g K0 100 200 0.2 0.5 35 0 K3 100 200 0.2 0.5 35 3 K6 100 200 0.2 0.5 35 6 K9 100 200 0.2 0.5 35 9 K12 100 200 0.2 0.5 35 12 K15 100 200 0.2 0.5 35 15 1.3.3 抗渗性能
修补材料抗渗性采用φ50 mm ×100 mm的圆柱形试样。实验仪器为苏州纽迈分析仪器股份有限公司生产的PQ-001三轴核磁共振检测系统，磁体为永磁体，恒定磁场0.49 T，主频率21 MHz。在2英寸三轴加持探头(图2)采用CPMG脉冲序列测试不同条件下试样内部氢核的横向弛豫，得到横向弛豫时间T2分布和含水量(体积比)。渗水过程中的含水量减去试块的初始含水量得到渗水量。测试中，等待时间
4000 ms，回波个数18000 ，累加次数8。砂浆抗渗性试验在试样养护到28 d后直接放入三轴夹具中进行测试(不真空饱水)，采用热缩膜隔离围压液和试块，并依次施加围压、轴压和渗透压。研究渗透压变化时，围压和轴压分别固定为8 MPa和0 MPa；研究时间变化时，围压、轴压和渗透压分别固定为8 MPa、0 MPa和2 MPa；研究轴压变化时，围压、渗透压分别固定为4 MPa和2 MPa。试样在50 MPa轴压作用后，采用纽迈核磁成像系统进行成像，分析试样破坏形貌。聚合物中存在的氢质子可能会影响低场核磁共振对聚合物改性砂浆的测试结果。本文测试了纯聚合物的横向弛豫时间分布，并与测试试样K9进行对比(图3)。聚合物主要成分醋酸乙烯酯-乙烯聚合物的氢信号存在于聚合物链条内，低场核磁信号难以捕捉。经试验在本实验测试参数下检测到聚合物的氢信号相对于测试试样极低，故本实验忽略聚合物中氢信号对试样低场核磁测试的影响。
![]()
图 3 聚合物和测试试样横向弛豫分布对比Figure 3. Comparison of transverse relaxation distribution between polymer and test sample1.3.4 微观结构分析
孔结构测试采用与抗渗性能测试相同的设备、程序和测试参数。通过真空高压充分饱水后的水含量表征孔隙率。φ50 mm ×100 mm的试样养护到28 d后真空饱水12 h。放置到50 mm氢测试探头线圈进行孔结构测试，测试过程中不施加任何的应力。
双组分快速交换模型是低场核磁共振弛豫测试孔结构最常用的模型[15]。对于多孔介质，水分子与孔隙表面的相互作用是影响驰豫的主要因素。对于孔体积为V和表面积为S的孔道，横向驰豫时间T2可以用下式表示[16]：
1T2=ρ2⋅SV (2) 式中ρ2为表面弛豫效率，是低场核磁表征砂浆孔结构的重要参数。根据相关文献，该研究中ρ2取值12 nm/ms[17,18]。假设为圆柱形孔，则横向弛豫时间T2与孔隙的等效半径r之间近似满足线性关系下式：
r=2ρ2T2 (3) 采用捷克TESCAN MIRA LMS型场发射扫描电镜分析了修补材料的微观形貌和元素分布。将养护到指定龄期的砂浆试样使用无水乙醇终止水化。测试前，将中止水化的样品在真空干燥箱中60℃烘干2 h。试验中观察新鲜断裂面，并喷金120 s增加试样的导电性。SEM图像分辨率为 0.9 nm@15 kV，能谱Maping拍摄时加速电压为 15 kV。
2. 结果与分析
2.1 聚合物对修补砂浆力学性能的影响
图4是聚合物掺量对修补砂浆力学性能的影响。由图4(a)可知，随着聚合物掺量的增加，修补砂浆的抗折强度先增大后减小。当聚合物的掺量为12%时，修补材料的抗折强度最大为9.6 MPa，比对照组提高了16.93%。这表明聚合物提高了砂浆的弯曲韧性。聚合物在砂浆中形成交织的网状膜，这种网状膜结构是典型的柔性结构，降低了水泥砂浆的弹性模量，提高了修补砂浆的韧性[19]。随着掺量的增加，聚合物逐渐形成连续的聚合物膜，改善了砂浆内部缺陷[20]。因此12%掺量时抗折强度最大。但是，聚合物掺量继续增加，聚合物引气作用会在水泥基体中引入空气形成较多的闭合孔，降低了砂浆的抗折强度[21]。
![]()
图 4 聚合物掺量对修补砂浆力学性能的影响Figure 4. Influence of polymer content on mechanical properties of repairing mortar粘结强度是修补材料重要的力学指标，采用界面弯拉强度表征修补砂浆粘结强度。由图4(b)可知，随着聚合物掺量的增加修补砂浆的粘结强度先增减后减小，最优掺量为9%，此时修补砂浆的28 d粘结强度可达5.1 MPa，相对于对照组提高了57.45%。聚合物会渗透进入修补基体中的孔隙中，失水成膜后在砂浆基体中形网状膜结构，能够与修补体形成良好地“桥接”作用。同时聚合物良好的黏结性能可显著提高修补材料与基体的黏附强度。
由图4(c)可知，聚合物降低了修补砂浆的抗压强度，聚合物掺量在9%以内时，抗压强度降低不明显，修补材料的28 d抗压强度为49.9 MPa，相对于对照组下降了9.8%。聚合物会吸附在水泥颗粒表面，并最终逐渐成膜，延缓水泥水化，降低水泥的水化速率。
2.2 基于低场核磁技术的聚合物改性修补砂浆抗渗性研究
通过分析聚合物对修补砂浆力学性能的影响，选取K0和K9抗渗试验作为实验组。通过修补砂浆的渗水量表征修补砂浆的抗渗性能，试样的横向弛豫时间变化反应水的渗透过程。
2.2.1 渗透时间对聚合物改性修补砂浆抗渗性的影响
图5是渗透时间对试样横向弛豫时间的影响(测试条件：渗透压2 MPa、围压8 MPa)。当渗透压稳定在2 MPa后开始采集数据，因此在渗透时间为0 min时渗透已经开始。从图中可以看出试样的横向弛豫时间分布呈现出三峰分布的模式，且三个峰的位置基本相同，根据相关研究表明凝胶层间水、凝胶孔隙水、水化产物间孔和毛细孔的弛豫时间T2分别为0.08~0.12 ms、0.25~0.5 ms和0.8~1 ms和＞10 ms[14]。第一个峰主要是凝胶孔隙水，第二和三峰主要是大毛细孔水。随着时间的增加水不断的渗入砂浆中，K0修补砂浆横向弛豫时间分布的第一个峰和第三个峰逐渐增加。这表明水分逐渐渗透入砂浆的凝胶孔和毛细孔。由于聚合物膜填充和阻隔作用，K9试样的横向弛豫时间变化不明显。
![]()
图 5 不同渗透时间修补砂浆横向弛豫时间分布Figure 5. Transverse relaxation time distribution of repaired mortar at different penetration time表4是不同时间渗透时间修补砂浆中的渗水率。在2 MPa的渗透压下，试样中的渗水量随着时间的增加逐渐增大，因此在试验测试的过程中要留够充足的稳定时间，本实验中每次测试至少保持0.5 h。试样的渗水率与横向弛豫时间分布变化相似，K0试样的孔隙率较大并且不具有阻水功能的膜结构，所以随时间延长试样的含水率变化明显。相对的，K9试样的含水率随时间变化不明显。原因在于聚合物可以填充修补砂浆中的微小孔隙，阻断联通毛细孔隙，优化了修补砂浆的孔结构。伴随水泥的水化过程，聚合物颗粒吸在水化产物、孔隙和界面过渡区之间，并失水形成均匀、柔性的聚合物膜阻断水的渗透通道。因此，在相同的渗透压下水渗入砂浆内部的能力减弱。
表 4 不同渗透时间修补砂浆渗水量Table 4. Seepage amount of repaired mortar at different times5 min 10 min 15 min 20 min 25 min 30 min K0 0.4% 0.71% 1.02% 1.29% 1.54% 1.72% K9 0.05% 0.13% 0.18% 0.22% 0.24% 0.27% 2.2.2 渗透压对聚合物改性修补砂浆抗渗性的影响
图6是不同渗透压下试样的横向弛豫时间分布。从图中可以看出在不同的渗透压下试样的横向弛豫时间分布呈现出三峰分布的模式，这可能是因为随着渗透压增大的过程，水逐渐的渗入修补材料的孔隙中，但是并不改变修补材料的孔结构。随着渗透压的增大，K0和K9试样的横向弛豫时间分布强度呈现出增大的趋势，并且不同渗透压下K9试样的横向弛豫时间分布差别较小，这是因为聚合物在水泥基材料中成膜有效的提高了修补材料的抗渗性。渗透压相对于时间对凝胶孔的峰值的提升明显。修补材料中小孔更难以渗透，渗透压是影响凝胶孔渗水量的主要因素。水泥浆体中，聚合物胶粉先吸水分散在水中，并吸附在水泥颗粒表面。伴随水泥水化和水分蒸发，聚合物逐渐失水、破乳，并在水化产物表面成膜，然后逐渐形成网络膜结。聚合物膜覆盖水化产物表面有效的阻碍了凝胶孔渗水通道；与此同时，较大的聚合物颗粒还可以填充水泥基体中的孔隙，增强修补砂浆致密性从而提高抗渗性能。因此在不同的渗透压作用下阻碍作用和堵塞作用分别造成了K9试样横向弛豫分布第一个峰和第三个峰的变化幅度的降低。
![]()
图 6 不同渗透压修补砂浆横向弛豫时间分布Figure 6. Transverse relaxation time distribution of repaired mortar at different penetration pressure从表5可以看出随着渗透压的增大，试样中的渗水量逐渐增加，而且对于没有互穿网络结构的K0试样含水量增加明显。渗透压是影响横向弛豫时间分布和渗水量的主要因素，通过低场核磁测试砂浆的抗渗性时，渗透压是主要的控制因素。
表 5 不同渗透压修补砂浆渗水量Table 5. Seepage amount of repaired mortar at different infiltration times1 MPa 2 MPa 3 MPa 4 MPa 5 MPa 6 MPa K0 1.59% 3.1% 3.9% 4.26% 4.68% 5.34% K9 0.57% 0.97% 1.48% 1.79% 2.02% 2.28% 2.2.3 三轴加载下聚合物改性修补砂浆抗渗性性能
图7是不同轴压下的横向弛豫时间分布。在轴向压力作用下，修补砂浆中的孔隙被压缩变小，因此第一个峰的峰值略有增。K0试样在轴压50 MPa时，横向弛豫时间分布发生了较为明显的变化，K9试样未发生明显变化。图8是试验轴压作用下的破坏形貌，K0试样在轴压和渗透压的作用下产生裂纹，因此在轴压到达50 MPa时间横向弛豫时间分布突变。由于K9试样中加入了聚合物，试样的韧性增强，试样产生明显的压缩变形未产生大裂纹，因此试样的T2弛豫分布没有突变。通过对50 MPa轴压下的试样进行低场核磁共振成像发现，K0试样内部出现明显的交叉裂缝，聚合物改善了修补砂浆的韧性，K9试样未出现明显的优势渗透通道。聚合物改性使得骨料与水化产物、水化产物和水化产物之间黏合更加密实。聚合物的弹性模量远小于水泥和骨料，因此聚合物降低了修补砂浆的弹性模量，同时聚合物在颗粒与裂缝之间的桥接作用提高了砂浆的断裂韧性，使受力状态下砂浆试样中应力集中大大减小，聚合物改性砂浆由脆性破坏逐渐向塑性变形破坏模式转变。当修补砂浆受到外力时，贯穿于修补砂浆内部的聚合物膜不仅可以抑制内部微裂缝的进一步扩展，避免快速形成渗透通道，而且可以吸收一部分冲击力，提高修补砂浆的韧性。
![]()
图 7 不同轴压修补砂浆横向弛豫时间分布Figure 7. Transverse relaxation time distribution of repaired mortar under different coaxial pressures![]()
图 8 三轴试验试样的破坏形貌(a)K0;(b)K9Figure 8. Failure morphology of triaxial test samples(a)K0;(b)K9表6是不同轴压作用下修补材料的渗水量。在围压和轴压的作用下，修补砂浆被缓慢的压缩，因此试样中的渗水量有小幅度减小。在修补砂浆产生明显的裂纹破坏前，轴压并不影响砂浆的抗渗性能，当产生破坏裂纹时修补砂浆的抗渗性能会产生突变。聚合物明显提高了修补材料的韧性，在复杂应力下服役时可有效地减少裂缝的产生，防止修补材料的抗渗性突变。
表 6 不同轴压修补砂浆渗水量Table 6. Seepage amount of repairing mortar under different coaxial pressure20 MPa 30 MPa 40 MPa 50 MPa K0 0.03% −0.03% −0.11% −0.38% K9 −0.08% −0.13% −0.12% −0.03% 2.3 聚合物改性修补砂浆抗渗性微观机理
采用1H低场核磁共振测孔技术和扫描电镜分析了聚合物改性修补砂浆的微观结。图9是聚合物掺量对修补砂浆孔结构的影响。1H低场核磁共振测试所得孔径主要呈现双峰模式，主要分为0.001~0.07 μm、2~100 μm两个范围，孔径尺寸跨越了5个数量级，第一个峰的峰极值和面积远大于第二个峰，占据优势地位。聚合物降低0.001~0.07 μm范围的峰值和面积，这可能是聚合物成膜封闭和充填了大的孔隙，降低了孔的尺寸。当聚合物的掺量过多时，试样中2~100 μm的孔会增多，聚合物的增稠作用导致修补砂浆中毛细孔增多。梅塔和蒙泰罗的研究表明毛细管孔隙(>50 nm)对于水泥基材料的抗渗性更具影响力[22]，因此聚合物的掺量应控制9%以内。从表7中的数据可知，随着聚合物掺量的增加，修补砂浆的孔隙率呈现先减小后增大的趋势，聚合物掺量为9%时，修补砂浆的孔隙率最小为7.45%。
![]()
图 9 聚合物掺量对修补砂浆孔结构的影响Figure 9. Influence of polymer content on pore structure of repair mortar表 7 聚合物掺量对修补砂浆孔隙率的影响Table 7. Influence of polymer content on porosity of repair mortarK0 K3 K6 K9 K12 K15 Porosity/% 10.73 9.25 8.53 7.45 8.49 9.84 图10是聚合物修补砂浆SEM微观形貌。K0试样中可以看到大量的孔隙，这些孔隙极大的影响砂浆的抗渗性能，同时砂浆中还存在针棒状钙矾石、片状氢氧化钙和凹凸不平的C-S-H凝胶。K9试样相对于K0的孔隙明显减少，这与孔结构数据相符。致密的聚合物膜附着在水化产物表面，并逐渐形成交互的网络膜结构，封堵了砂浆孔隙，提高了修补砂浆的抗渗水能力[23]。因此聚合物明显降低了修补砂浆的横向弛豫时间分布强度和渗水量。图11是K0和K9试样在2000倍下断面EDS面扫图谱和元素含量。K0试样断面的孔隙处可见几微米的片状产物，元素分布图中相应的部分钙元素富集、硅元素缺失，因此可推断为氢氧化钙，其他区域主要以C-S-H凝胶为主。K9试样的碳含量为17.7 At%，相对K0的9.5 At%明显增加，这是因为聚合物中含有大量的碳元素。碳元素广泛地分布于试样各区域，表明聚合物在水泥基体中分布均匀，可以形成良好的阻水交互网络薄膜。
![]()
图 10 不同聚合物掺量修补砂浆的断面SEM图像Figure 10. Cross-section SEM images of repair mortar with different polymer contents![]()
图 11 修补砂浆的断面EDS面扫图图谱和元素分布Figure 11. Sectional EDS scan map and element distribution of the repair mortar水泥基材料的抗渗性与孔隙结构密切相关。聚合物成膜后粘附在水化产物表面并分割、填充了水泥基材料的孔隙，改善了修补砂浆的微观结构。水泥和聚合物组成的胶凝体系水化产物之间可以相互交融、相互填充，具有更高的密实度[24]。这使得水更难进入水泥基体内部，抗渗性能优异。另一方面，聚合物膜粘附在水化产物表面，并贯穿于水泥水化物之间，有效的阻挡了水化产物内部凝胶孔的运移通道，像一道道“闸门”截断了毛细管道，阻断了材料内部与外界的联系，有效提高水泥基材料的抗渗性能。
3. 结 论
(1)聚合物改善了修补砂浆的力学性能，掺量9%时砂浆的抗折强度和粘结强度分别提高16.99%和57.45%，增加了砂浆的韧性和界面性能。
(2)通过横向弛豫时间分布和渗水量有效的表征聚合物改性修补砂浆抗渗性能。随渗透压增高，修补砂浆凝胶孔隙和毛细孔隙水增加，聚合物明显降低两者的增长趋势。
(3)在砂浆发生结构破坏前，轴压对修补砂浆的抗渗性能基本无影响，砂浆产生交叉裂缝破坏后抗渗性能会产生突变。聚合物改性修补砂浆产生明显的压缩变形未产生明显裂纹，试样的横向弛豫时间分布没有突变。
(4)聚合物优化了砂浆的孔结构和微观形貌，孔隙率最大降低了3.28%。聚合物薄膜的阻隔和填充效应降低了凝胶孔和毛细孔的通道运移能力，减少了修补砂浆的横向弛豫强度、渗水量，提高了砂浆的抗渗性能。
-
![]()
图 1 Gyroid点阵结构设计与制造示意图
Figure 1. Schematic diagram of Gyroid lattice structure design and manufacturing
![]()
图 2 Gyroid点阵结构晶胞简化示意图
Figure 2. Simplified schematic diagram of Gyroid lattice structure cell
d—Diameter; l—Branching rod length; a—Spatial length of a cell
![]()
图 3 Gyroid晶胞受力分析
Figure 3. Analysis of Gyroid crystal cell stress
σ—Stress; F—Force; θ—Angle between pole and horizontal plane; M1—Torque at the node
![]()
图 5 压缩模拟载荷与边界条件
Figure 5. Compression simulation load and boundary conditions
A—Cross sectional area of lattice structure; L—Side length of the lattice structure; u—Displacement of upper plane nodes
![]()
图 6 不同单元尺寸的模型收敛性分析
Figure 6. Convergence analysis of models with different element sizes
![]()
图 7 有限元模拟获得的Gyroid点阵结构应力-应变曲线
Figure 7. Stress-strain curves of Gyroid lattice structure obtained from finite element simulation
![]()
图 8 Gyroid点阵结构应力/应变云图
Figure 8. Stress/strain distribution of Gyroid lattice structure
![]()
图 11 Gyroid点阵结构理论与实测尺寸对比
Figure 11. Comparison of theoretical and actual dimensions of Gyroid lattice structure
![]()
图 12 Gyroid点阵结构理论与实测质量对比
Figure 12. Comparison of theoretical and actual mass of Gyroid lattice structure
![]()
图 13 Gyroid点阵结构表面微观形貌
Figure 13. Microscopic morphologies of Gyroid lattice structure surface
![]()
图 14 Gyroid点阵结构压缩实验过程
Figure 14. Experimental process of Gyroid lattice structure compression
![]()
图 15 Gyroid点阵结构压缩变形机制
Figure 15. Compression deformation mechanism of Gyroid lattice structure
![]()
图 16 压缩实验获到的Gyroid点阵结构应力-应变曲线
Figure 16. Stress-strain curves of Gyroid lattice structure obtained from compression experiments
![]()
图 17 简化前后Gyroid点阵结构体积分数对比
Figure 17. Comparison of volume fractions of Gyroid lattice structures before and after simplification
![]()
图 18 Gyroid点阵结构塑性屈服强度Gibson-Ashby拟合
Figure 18. Gibson-Ashby fitting of plastic yield strength of Gyroid lattice structure
σG—Plastic yield strength of lattice structure; σs—Yield strength of base metal; Vv—Volume fraction
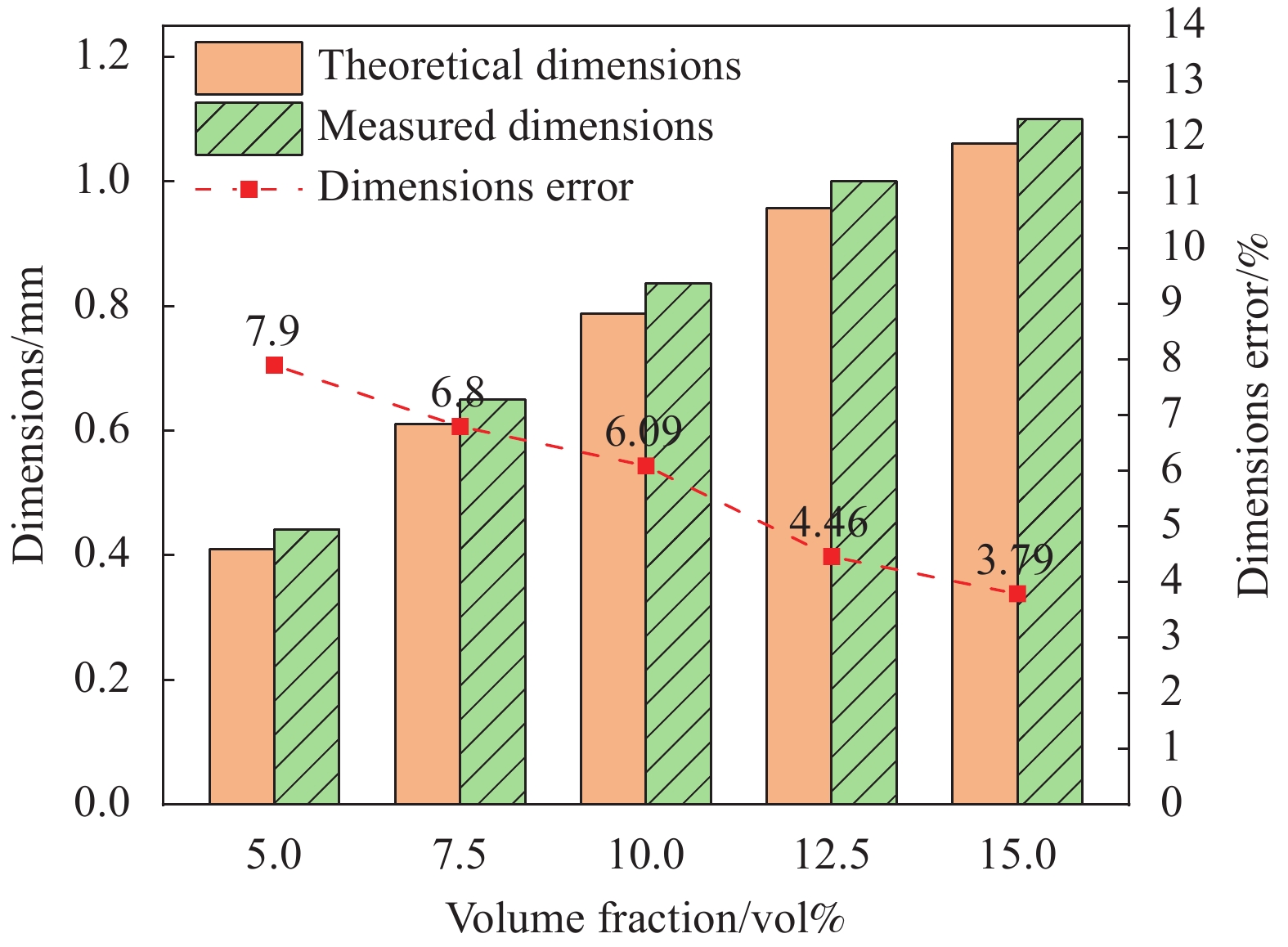
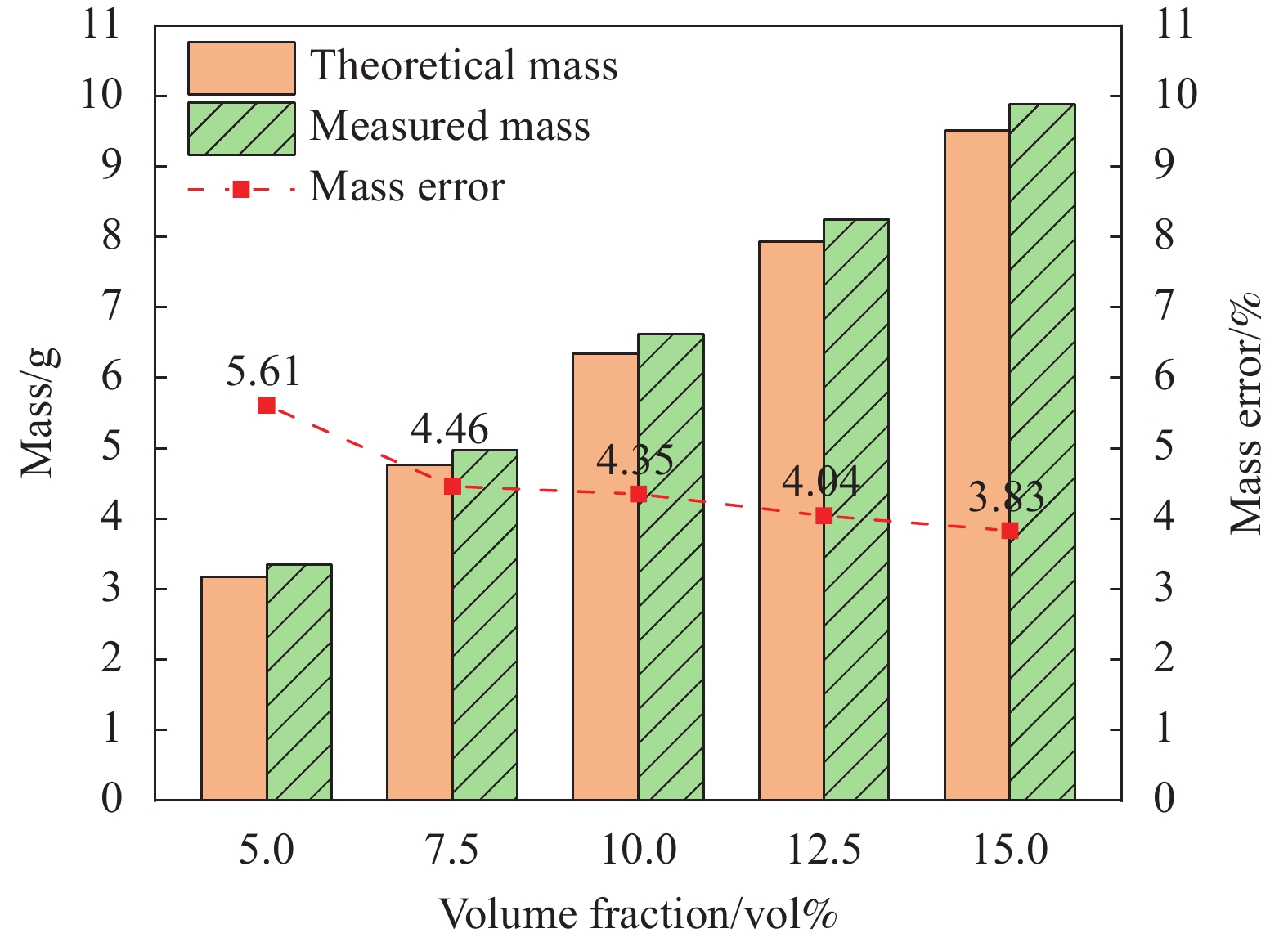
表 1 Gyroid点阵结构理论推导、有限元和实验结果对比
Table 1 Comparison of theoretical derivation, finite element and experimental results of Gyroid lattice structure
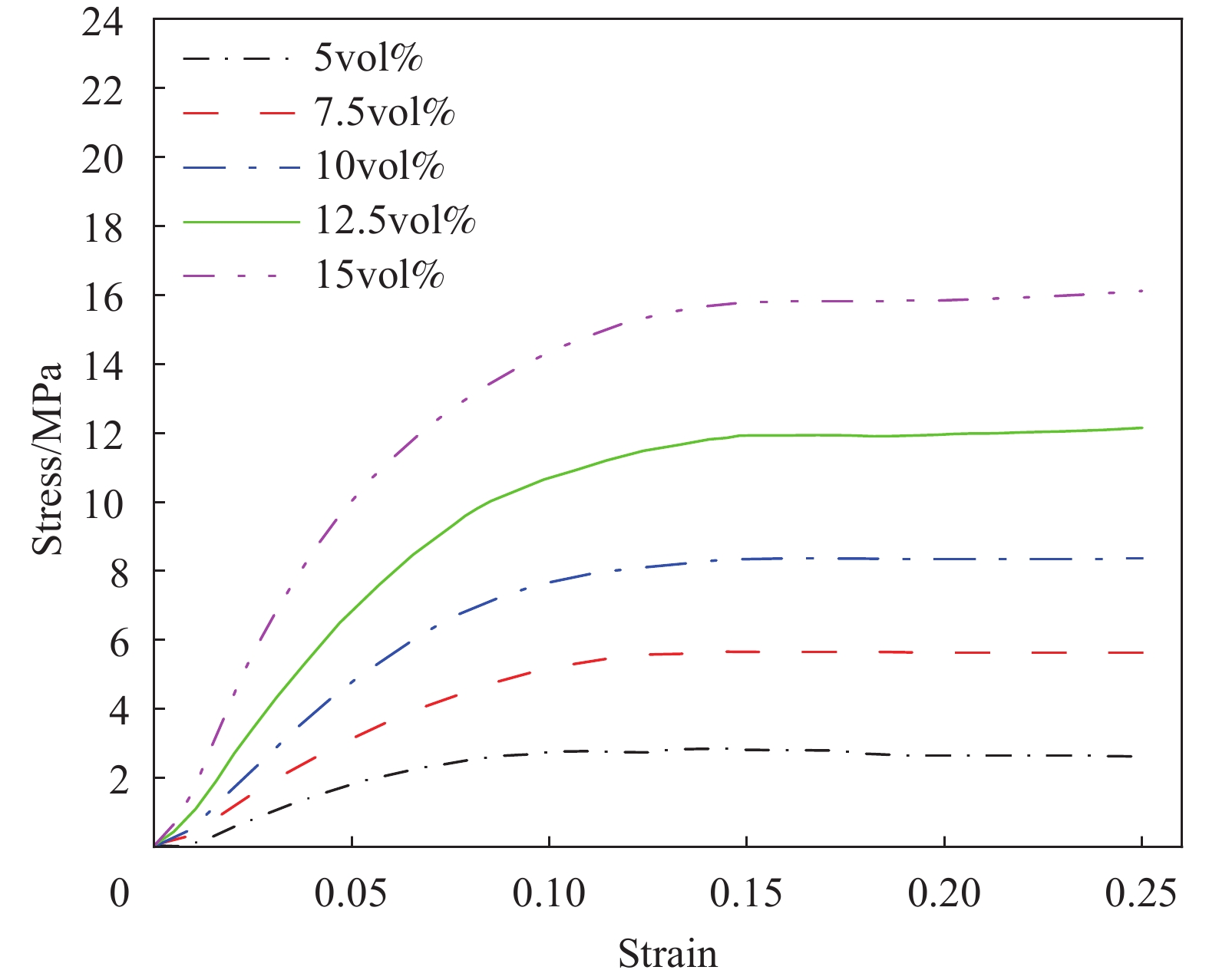
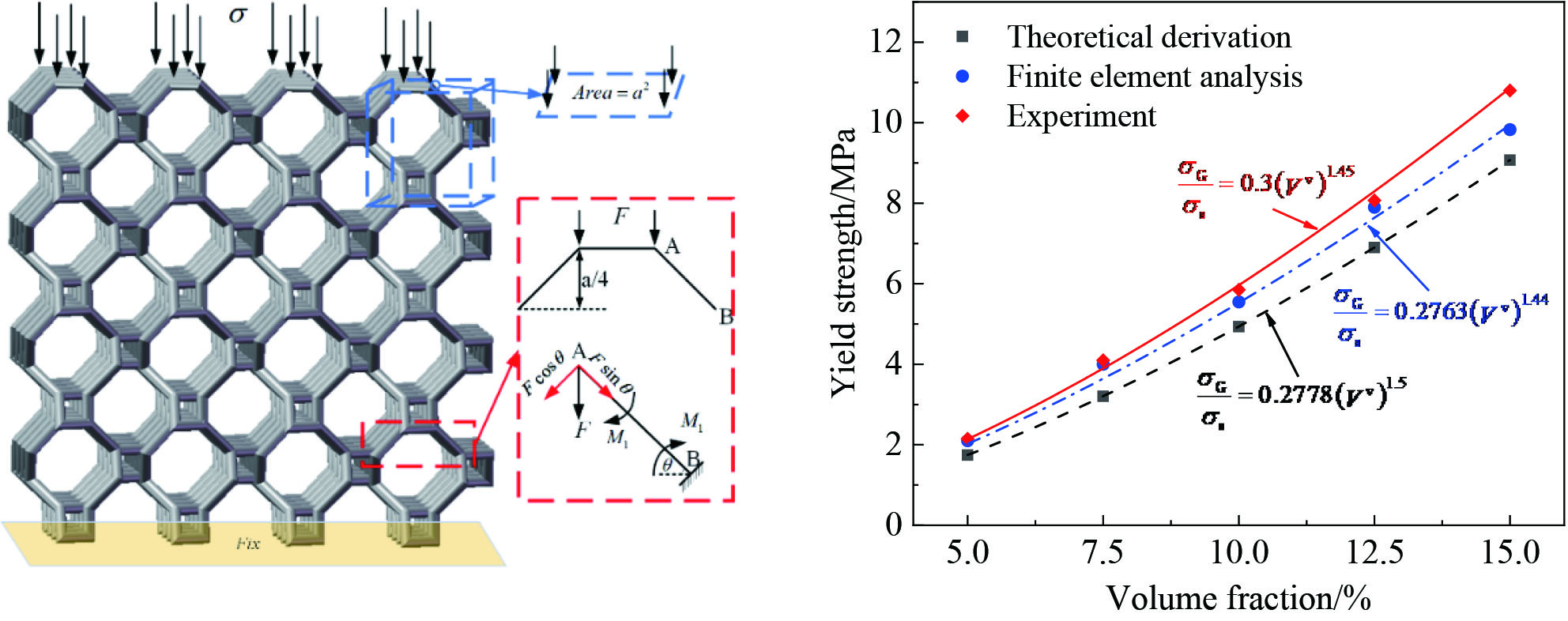
Volume fraction/vol% Plastic yield strength/MPa Theoretical derivation Finite element analysis Experimental 5 1.48 1.78 1.83 7.5 2.72 3.40 3.48 10 4.19 4.72 4.97 12.5 5.85 6.71 6.86 15 7.69 8.36 9.18 
下载: 导出CSV
-
[1] 程树良, 吴灵杰, 孙帅, 等. X型点阵夹芯结构受局部冲击时动态力学性能试验与数值模拟[J]. 复合材料学报, 2022, 39(7): 3641-3651. CHENG Shuliang, WU Lingjie, SUN Shuai, et al. Experiment and numerical simulation of dynamic mechanical properties of X-lattice sandwich structure under local impact[J]. Acta Materiae Compositae Sinica, 2022, 39(7): 3641-3651(in Chinese).
[2] WU F H, LIAN H, PEI G B, et al. Design and optimization of variable-density lattice structure based on load paths[J]. Facta Universitatis-Series Mechanical Engineering, 2023, 21(2): 273-292. DOI: 10.22190/FUME220108017W
[3] SCHAEDLER T A, JACOBSEN A J, CARTER W B. Toward lighter, stiffer materials[J]. Science, 2013, 341(6151): 1181-1182. DOI: 10.1126/science.1243996
[4] 梁栋, 陈伟, 胡勇, 等. 点阵单元类型和排布角度对传热特性的影响[J]. 航空动力学报, 2022, 37(1): 26-35. LIANG Dong, CHEN Wei, HU Yong, et al. Influences of lattice unit type and arrangement orientation on heat transfer characteristics[J]. Journal of Aerospace Power, 2022, 37(1): 26-35(in Chinese).
[5] 李永欣, 郭长春, 李凯伦, 等. SLM制备的小长径比四棱锥点阵填充轻量化研究[J]. 机械工程学报, 2021, 57(24): 132-138. DOI: 10.3901/JME.2021.24.132 LI Yongxin, GUO Changchun, LI Kailun, et al. Filling lightweight research on pyramid lattice with small slenderness ratio fabricated by SLM[J]. Journal of Mechanical Engineering, 2021, 57(24): 132-138(in Chinese). DOI: 10.3901/JME.2021.24.132
[6] CHOUHAN G, BALA MURALI G. Designs, advancements, and applications of three-dimensional printed Gyroid structures: A review[J]. Proceedings of the Institution of Mechanical Engineers, Part E: Journal of Process Mechanical Engineering, 2024, 238(2): 965-987.
[7] FENG J W, FU J Z, YAO X H, et al. Triply periodic minimal surface (TPMS) porous structures: From multi-scale design, precise additive manufacturing to multidisciplinary applications[J]. International Journal of Extreme Manufacturing, 2022, 4(2): 022001. DOI: 10.1088/2631-7990/ac5be6
[8] Al-KETAN O, ROWSHAN R, Al-RUB R K A. Topology-mechanical property relationship of 3D printed strut, skeletal, and sheet based periodic metallic cellular materials[J]. Additive Manufacturing, 2018, 19: 167-183. DOI: 10.1016/j.addma.2017.12.006
[9] DEVALK T, LAKES R. Poisson's ratio and modulus of Gyroid lattices[J]. Physica Status Solidi B-Basic Solid State Physics, 2021, 258(12): 2100081. DOI: 10.1002/pssb.202100081
[10] 张明康. 隐式曲面梯度多孔结构优化设计及激光选区熔化成形力学性能研究[D]. 广州: 华南理工大学, 2020. ZHANG Mingkang. Optimal design and mechanical properties of implicit surface gradient porous structure manufactured by selective laser melting[D]. Guangzhou: South China University of Technology, 2020(in Chinese).
[11] JONES A, LEARY M, BATEMAN S, et al. Parametric design and evaluation of TPMS-like cellular solids[J]. Materials & Design, 2022, 221: 110908.
[12] LU Y T, ZHAO W Y, CUI Z T, et al. The anisotropic elastic behavior of the widely-used triply-periodic minimal surface based scaffolds[J]. Journal of the Mechanical Behavior of Biomedical Materials, 2019, 99: 56-65. DOI: 10.1016/j.jmbbm.2019.07.012
[13] KHADERI S N, DESHPANDE V S, FLECK N A. The stiffness and strength of the Gyroid lattice[J]. International Journal of Solids and Structures, 2014, 51(23-24): 3866-3877. DOI: 10.1016/j.ijsolstr.2014.06.024
[14] MASKERY I, STURM L, AREMU A O, et al. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing[J]. Polymer, 2018, 152: 62-71. DOI: 10.1016/j.polymer.2017.11.049
[15] XING P, HUANG Q Y, ZHANG G X, et al. Compensating the anisotropic mechanical properties of electron beam melting-based Gyroid scaffolds using structural design[J]. International Journal of Mechanical Sciences, 2022, 226: 107442. DOI: 10.1016/j.ijmecsci.2022.107442
[16] KELLY C N, FRANCOVICH J, JULMI S, et al. Fatigue behavior of as-built selective laser melted titanium scaffolds with sheet-based Gyroid microarchitecture for bone tissue engineering[J]. Acta Biomaterialia, 2019, 94: 610-626. DOI: 10.1016/j.actbio.2019.05.046
[17] YANG L, YAN C Z, CAO W C, et al. Compression-compression fatigue behaviour of Gyroid-type triply periodic minimal surface porous structures fabricated by selective laser melting[J]. Acta Materialia, 2019, 181: 49-66. DOI: 10.1016/j.actamat.2019.09.042
[18] VIET N V, ZAKI W. Free vibration and buckling characteristics of functionally graded beams with triply periodic minimal surface architecture[J]. Composite Structures, 2021, 274: 114342. DOI: 10.1016/j.compstruct.2021.114342
[19] USHIJIMA K, CANTWELL W J, MINES R A W, et al. An investigation into the compressive properties of stainless steel micro-lattice structures[J]. Journal of Sandwich Structures & Materials, 2011, 13(3): 303-329.
[20] ATAEE A, LI Y C, BRANDT M, et al. Ultrahigh-strength titanium Gyroid scaffolds manufactured by selective laser melting (SLM) for bone implant applications[J]. Acta Materialia, 2018, 158: 354-368. DOI: 10.1016/j.actamat.2018.08.005
[21] YANG X, YANG Q, SHI Y S, et al. Effect of volume fraction and unit cell size on manufacturability and compressive behaviors of Ni-Ti triply periodic minimal surface lattices[J]. Additive Manufacturing, 2022, 54: 102737. DOI: 10.1016/j.addma.2022.102737
[22] ZADPOOR A A, HEDAYATI R. Analytical relationships for prediction of the mechanical properties of additively manufactured porous biomaterials[J]. Journal of Biomedical Materials Research Part A, 2016, 104(12): 3164-3174. DOI: 10.1002/jbm.a.35855
[23] FU J, DING J H, QU S, et al. Improved light-weighting potential of SS316L triply periodic minimal surface shell lattices by micro laser powder bed fusion[J]. Materials & Design, 2022, 222: 111018.
[24] YOO D. Computer-aided porous scaffold design for tissue engineering using triply periodic minimal surfaces[J]. International Journal of Precision Engineering and Manufacturing, 2011, 12(1): 61-71. DOI: 10.1007/s12541-011-0008-9
[25] PENG X, HUANG Q, ZHANG Y, et al. Elastic response of anisotropic Gyroid cellular structures under compression: Parametric analysis[J]. Materials & Design, 2021, 205: 109706.
[26] 杨磊. 增材制造三周期极小曲面点阵结构的力学性能研究[D]. 武汉: 华中科技大学, 2020. YANG Lei. Research on mechanical properties of additive manufactured triply periodic minimal surface lattice structures[D]. Wuhan: Huazhong University of Science and Technology, 2020(in Chinese).
[27] 迟静, 李敏, 王淑峰, 等. Ti-C含量对多孔TiC/FeAl复合材料孔型结构和力学性能的影响[J]. 复合材料学报, 2018, 35(9): 2503-2511. CHI Jing, LI Min, WANG Shufeng, et al. Effects of Ti-C contents on the pore structures and mechanical properties of porous TiC/FeAl composites[J]. Acta Materiae Compositae Sinica, 2018, 35(9): 2503-2511(in Chinese).
[28] ZHANG L, FEIH S, DAYNES S, et al. Energy absorption characteristics of metallic triply periodic minimal surface sheet structures under compressive loading[J]. Additive Manufacturing, 2018, 23: 505-515. DOI: 10.1016/j.addma.2018.08.007
[29] ZHANG C, ZHENG H, YANG L, et al. Mechanical responses of sheet-based Gyroid-type triply periodic minimal surface lattice structures fabricated using selective laser melting[J]. Materials & Design, 2022, 214: 110407.
[30] MACONACHIE T, LEARY M, LOZANOVSKI B, et al. SLM lattice structures: Properties, performance, applications and challenges[J]. Materials & Design, 2019, 183: 108137.
[31] WANG N Y, MEENASHISUNDARAM G K, CHANG S, et al. A comparative investigation on the mechanical properties and cytotoxicity of cubic, octet, and TPMS Gyroid structures fabricated by selective laser melting of stainless steel 316L[J]. Journal of the Mechanical Behavior of Biomedical Materials, 2022, 129: 015151.
[32] RAVICHANDER B B, JAGDALE S H, KUMAR G. Surface morphology, compressive behavior, and energy absorption of graded triply periodic minimal surface 316L steel cellular structures fabricated by laser powder bed fusion[J]. Materials, 2022, 15(23): 8294. DOI: 10.3390/ma15238294
[33] YANG K V, ROMETSCH P, JARVIS T, et al. Porosity formation mechanisms and fatigue response in Al-Si-Mg alloys made by selective laser melting[J]. Materials Science and Engineering A-Structural Materials Properties Microstructure and Processing, 2018, 712: 166-174. DOI: 10.1016/j.msea.2017.11.078
-
目的
点阵结构与密实结构存在的力学性能差异之一表现在塑性屈服响应上，因此，研究其屈服行为可为点阵结构的设计和应用提供重要的理论依据。
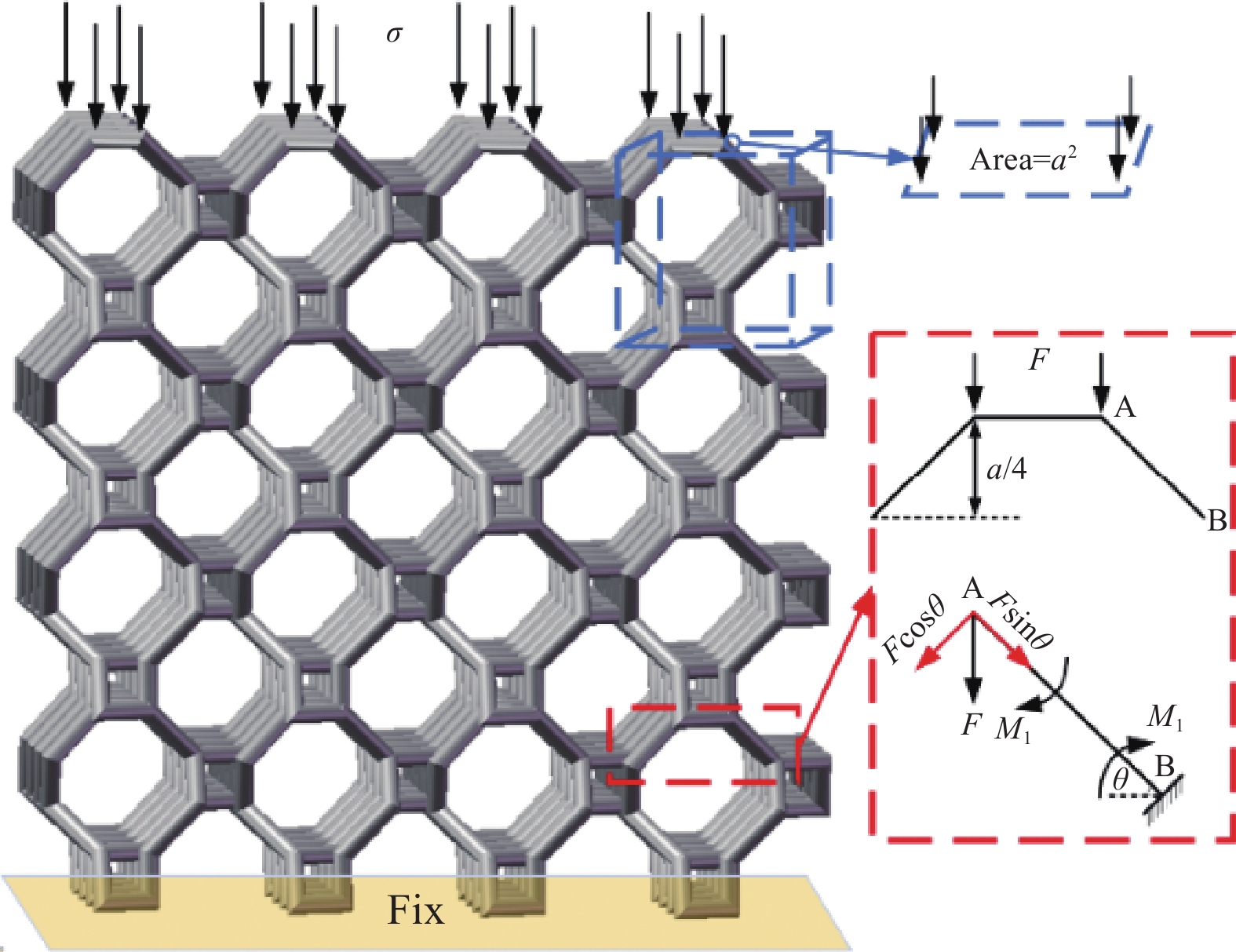
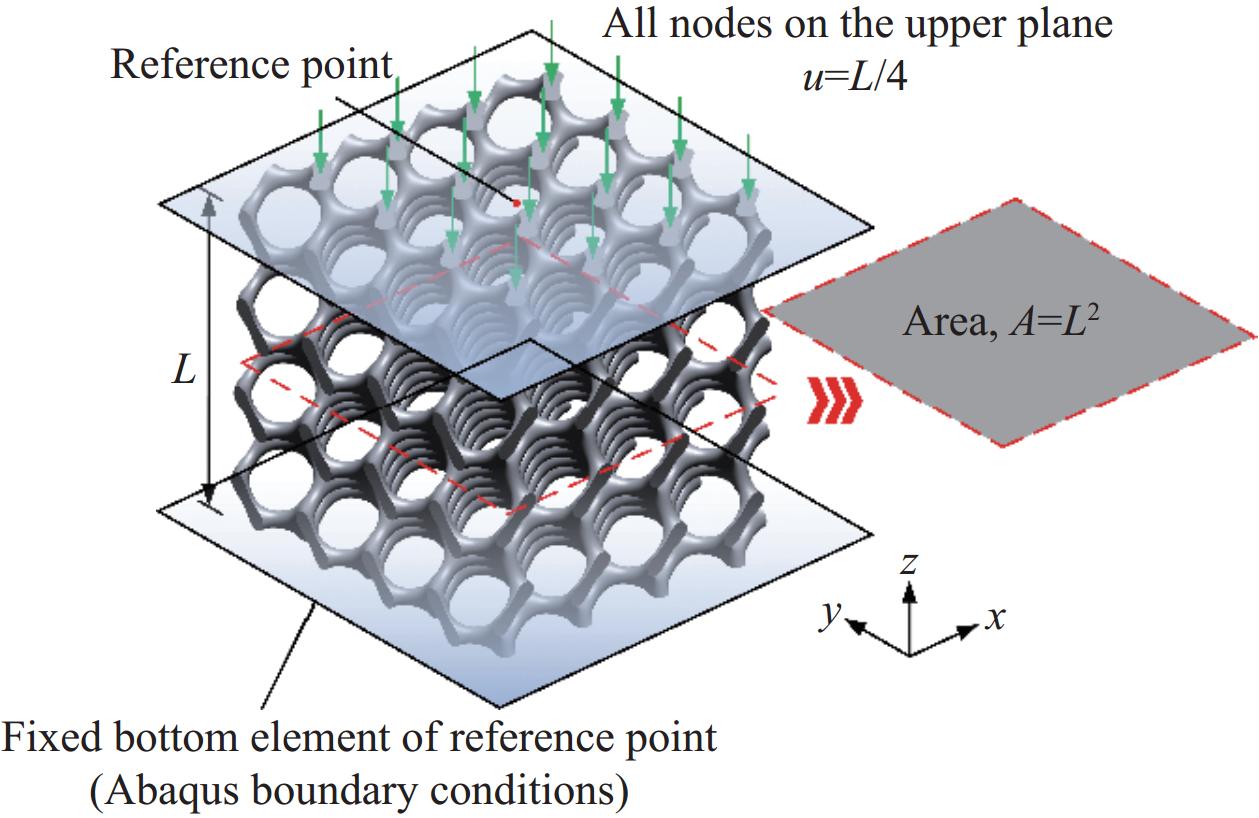
方法对Gyroid点阵结构进行简化，并基于变形体功能原理建立其力学模型，得到Gyroid点阵结构塑性屈服强度与体积分数之间的映射关系。然后，基于有限元分析软件Abaqus对Gyroid点阵结构准静态压缩过程开展仿真实验，初步验证理论模型的准确性。最后，通过选择性激光熔化(Selective Laser Melting，SLM)制备不同体积分数316L不锈钢Gyroid点阵结构，进行单轴压缩实验，分析其变形机制与力学性能。
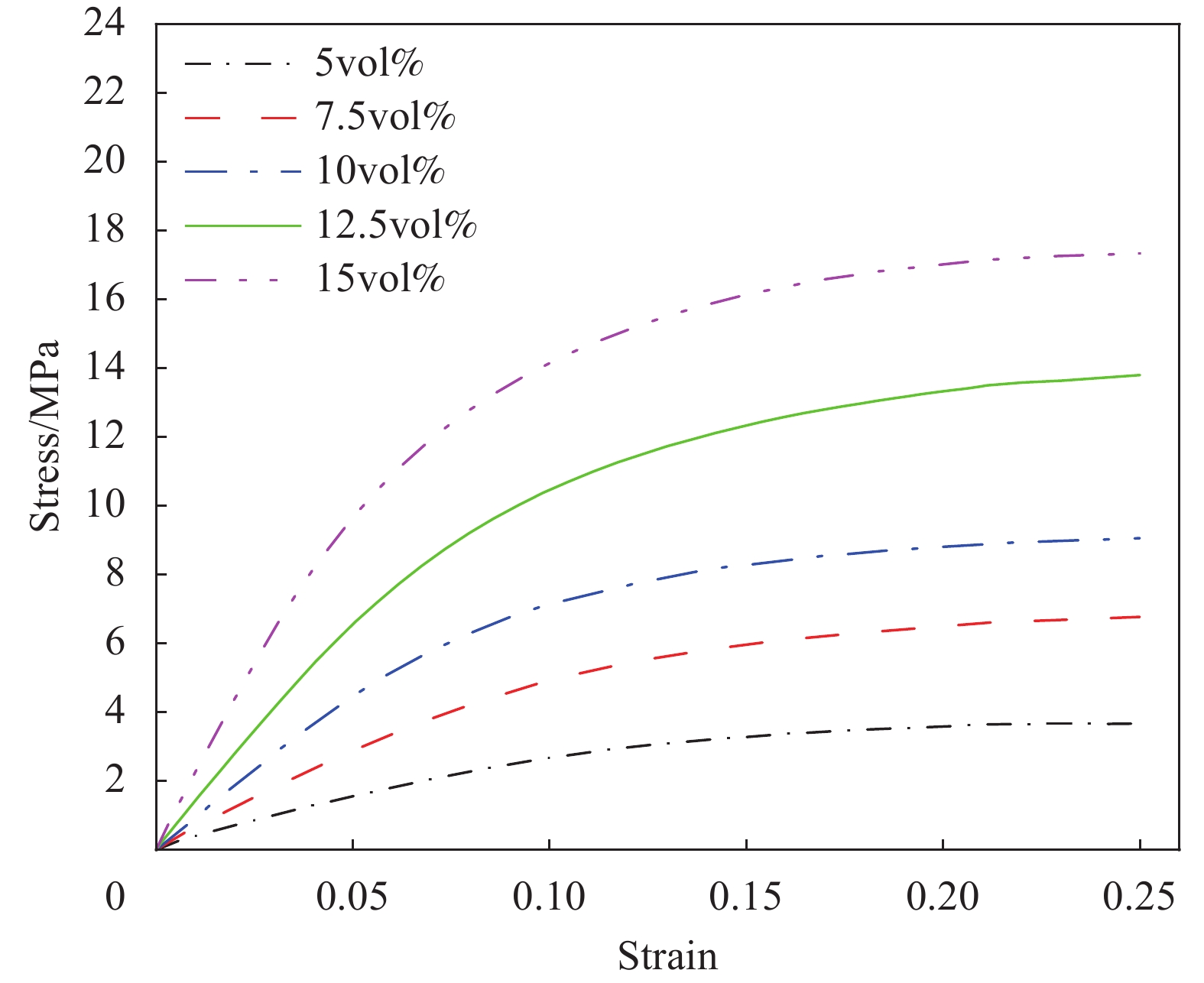
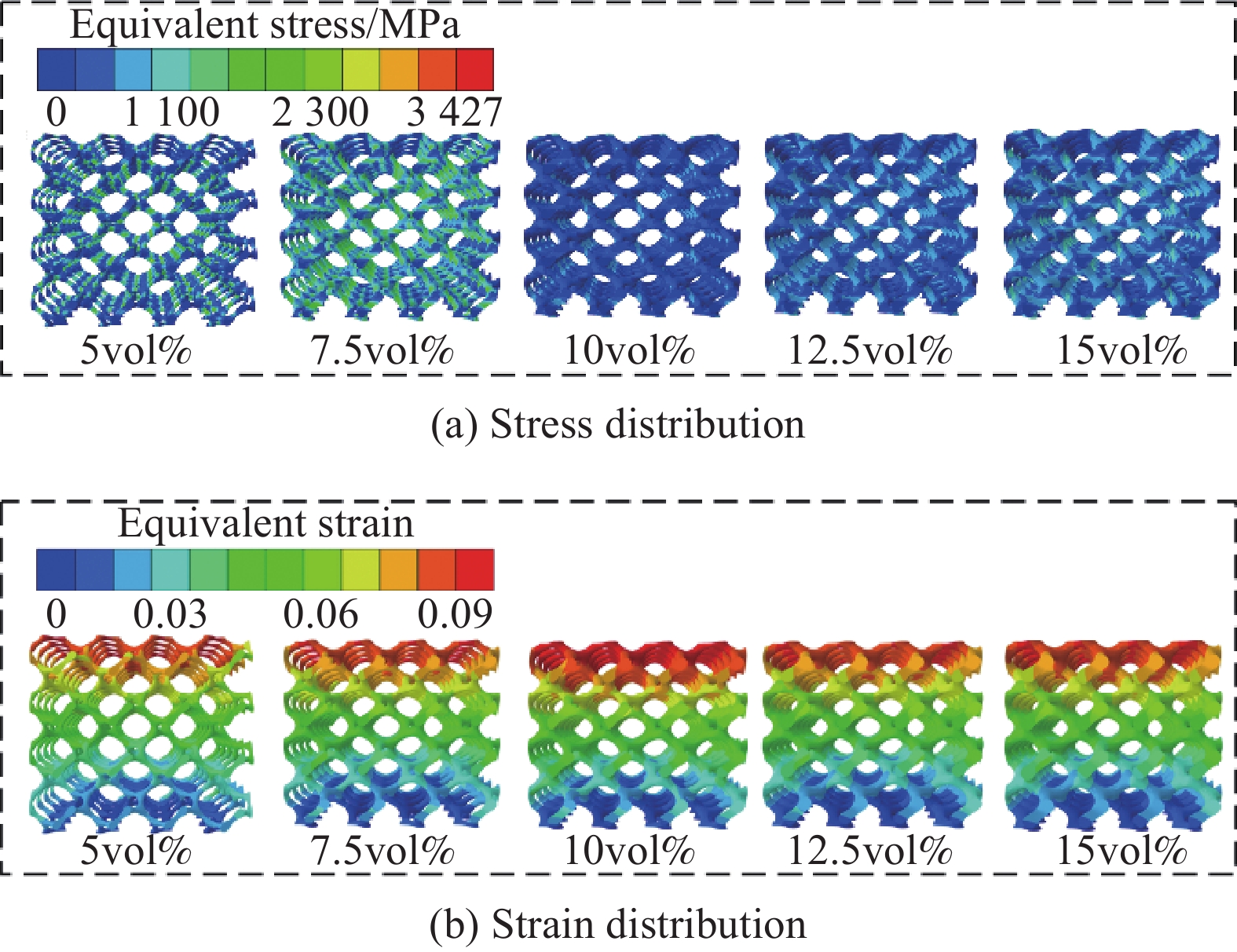
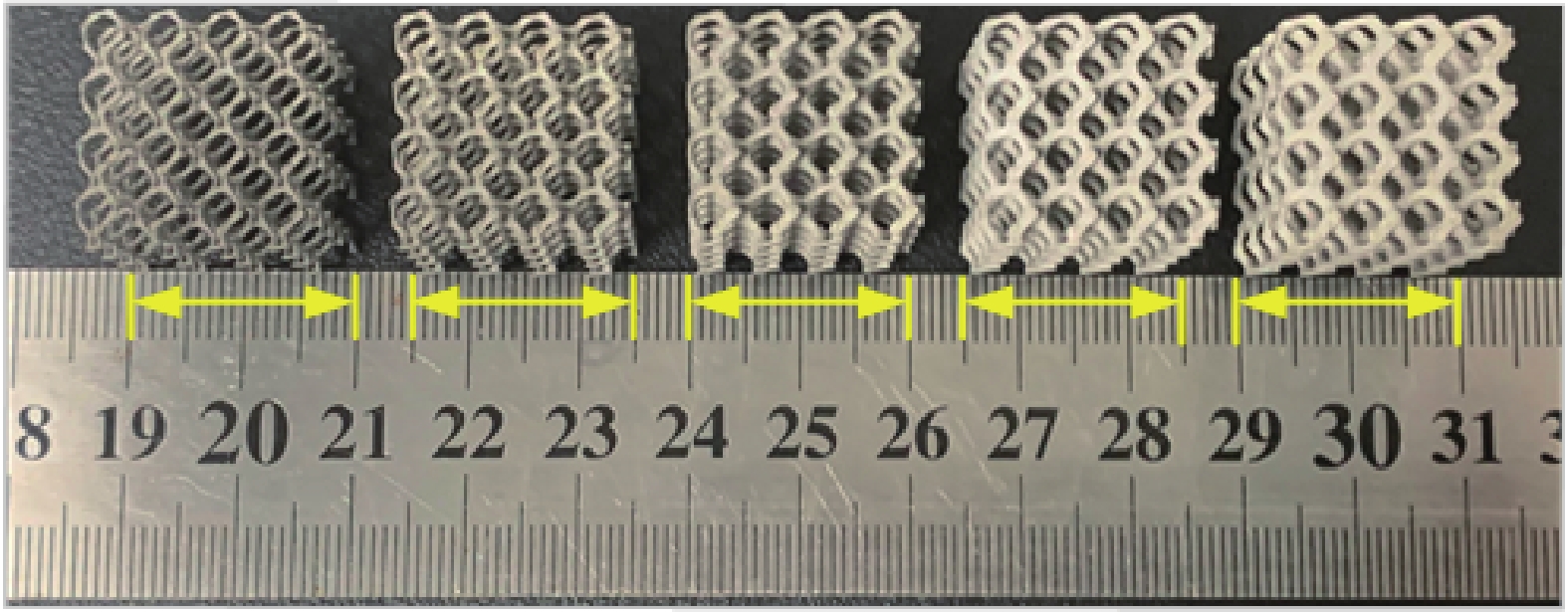
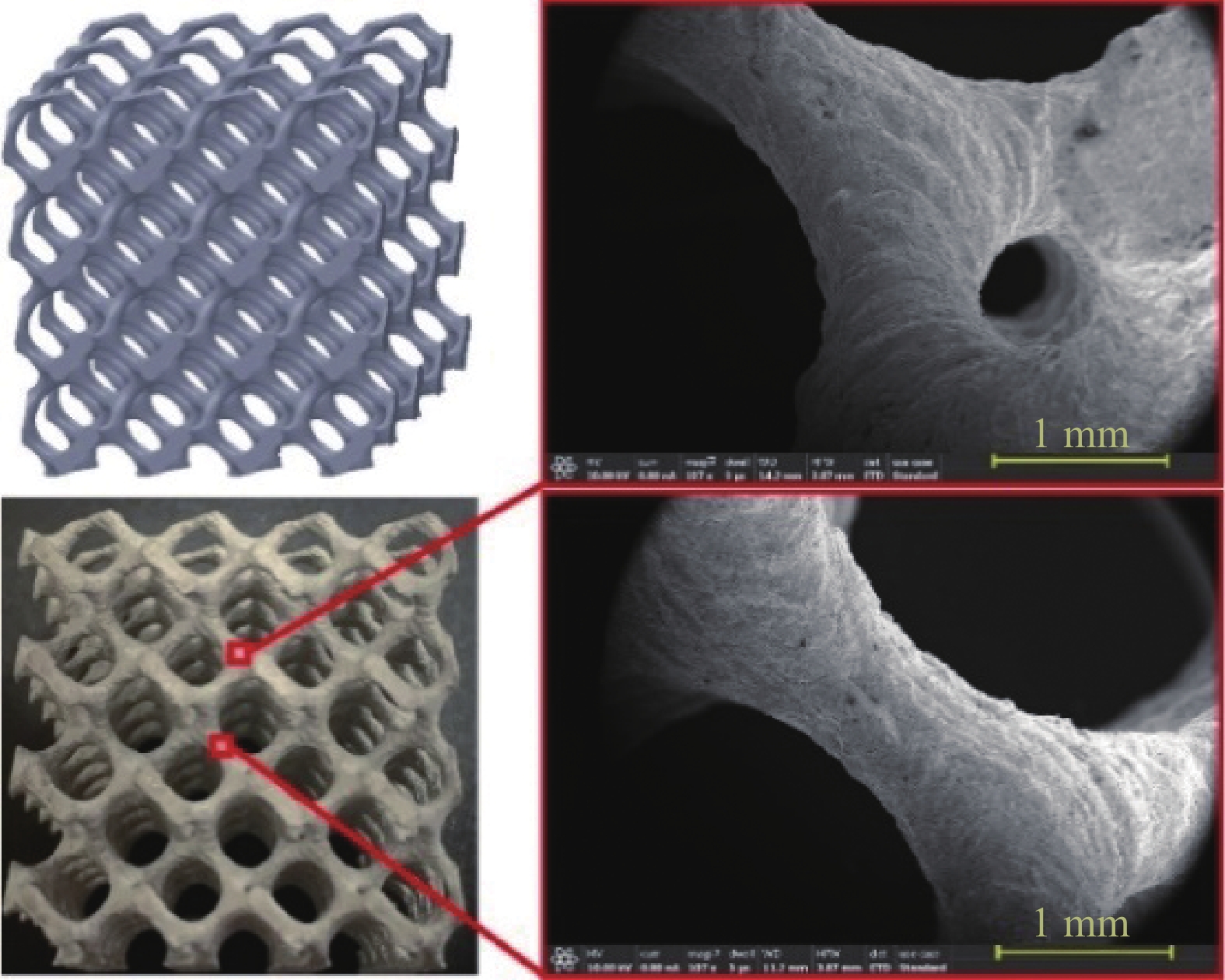
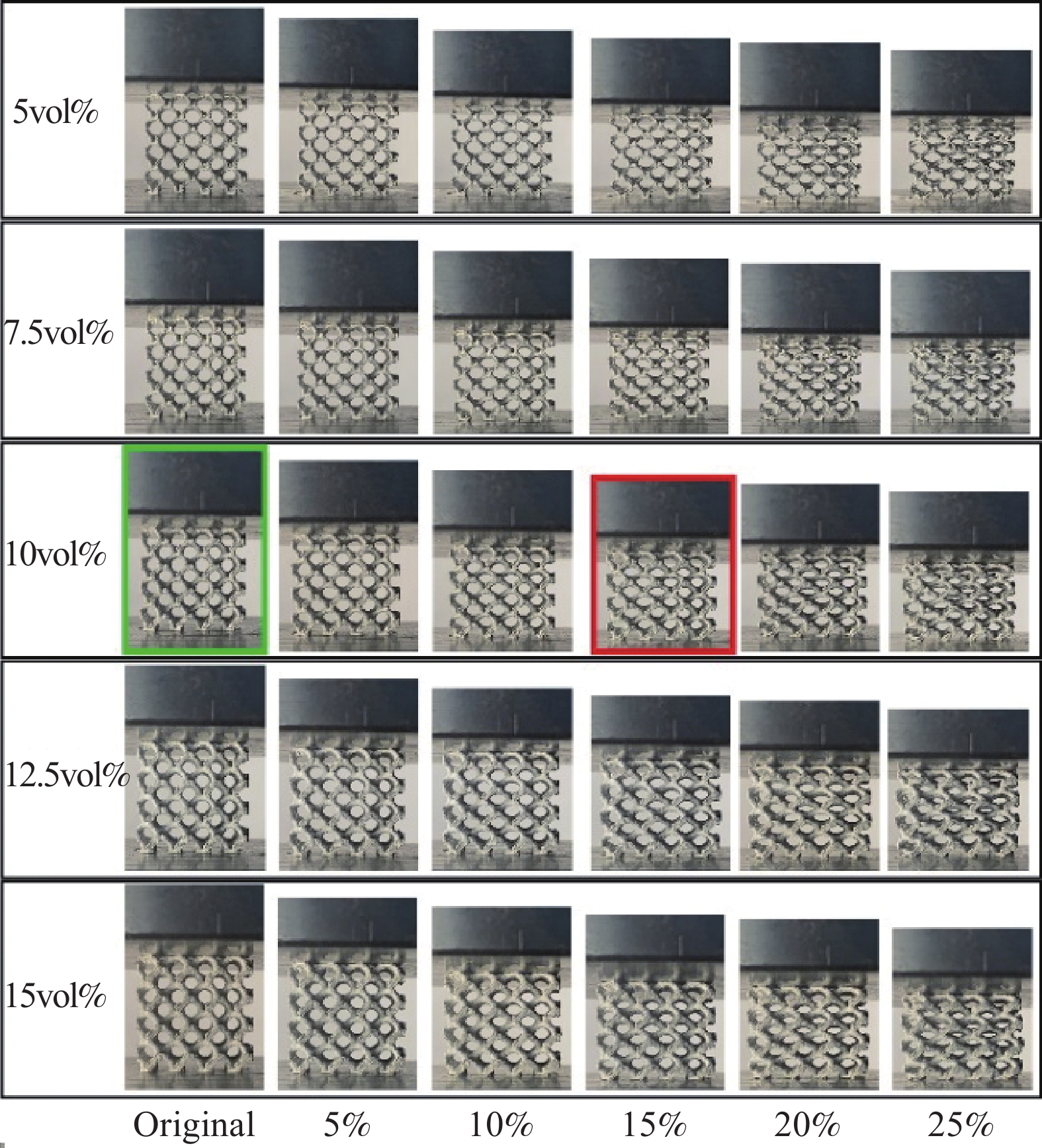
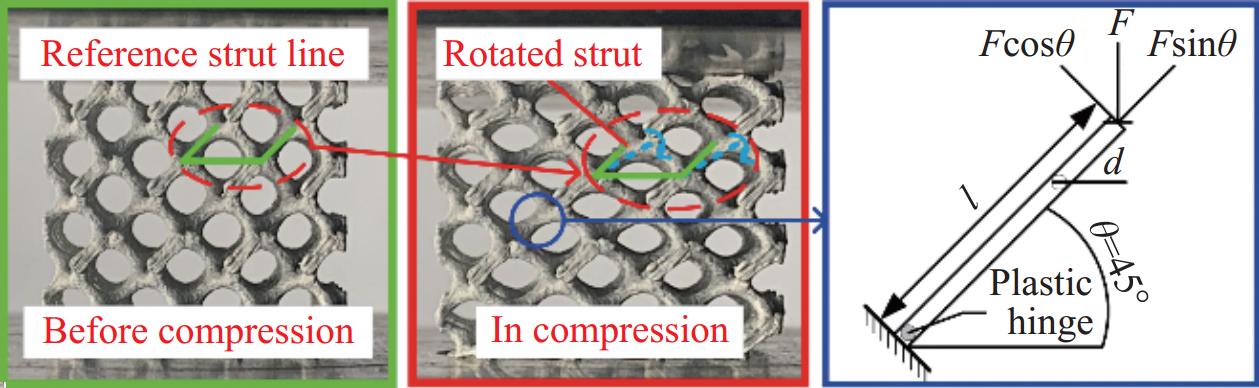
结果(1)通过理论推导发现，对于母体材料确定的Gyroid点阵结构，其塑性屈服强度与体积分数的1.5次方成正比。(2)有限元分析得到的应力-应变曲线表明，Gyroid点阵结构压缩过程中以弯曲变形为主，在单胞内部，应力/应变最大值集中在斜杆中间位置，因此斜杆中心呈现较高的屈服趋势。(3)通过观察Gyroid点阵结构压缩实验过程可以看出塑性铰的位置以及变形机制，发现与理论推导中支杆的变形行为与承载形式一致，进一步验证理论推导中所用方法的适用性，且得到的应力-应变曲线形式与有限元模拟得到的具有较好的一致性。(4)基于理论推导和有限元模拟得到的Gyroid点阵结构塑性屈服强度结果与实验结果存在一定偏差，但总体趋势基本一致，且三者的Gibson-Ashby拟合模型系数接近。对比发现，理论推导和有限元模拟得到的塑性屈服强度均低于实验结果值，原因是点阵结构打印过程中由于激光加减速导致更多能量输入，SLM打印会有更大的熔池，使得试件尺寸和质量略大于设计值，这都会提高Gyroid点阵结构力学性能，导致实验屈服强度数值偏大，但总体看理论推导与实验偏差在25%以内，证明本文基于理论推导得到的点阵结构塑性屈服强度与体积分数之间的数学关系模型具有适用性。
结论本文基于理论推导结果拟合得到的Gibson-Ashby模型可用于任意体积分数Gyroid点阵结构的塑性屈服强度预测。本文的方法为工程上快速核算复杂点阵结构力学行为提供理论指导，为点阵结构设计提供重要的理论支撑。
-
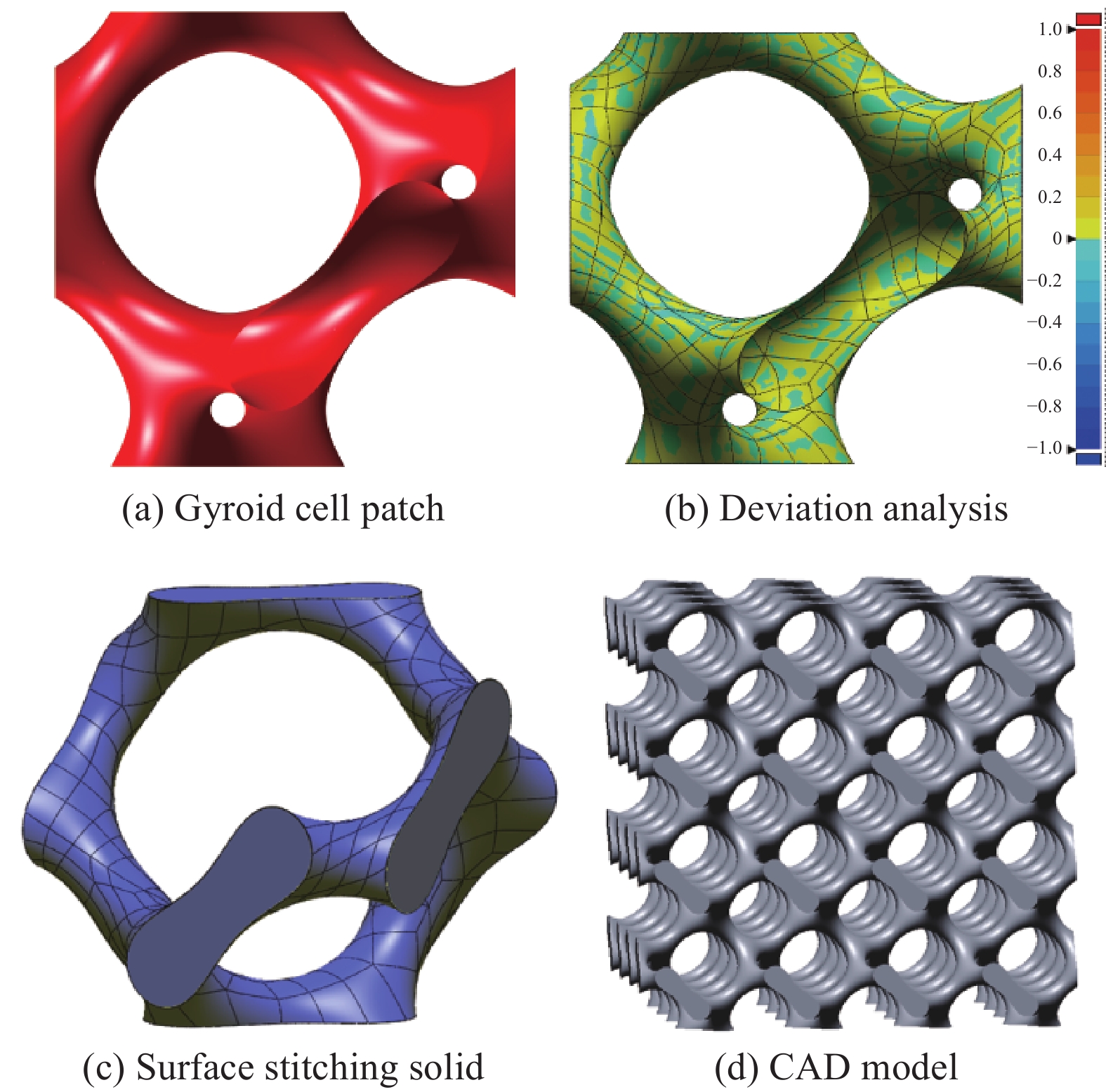
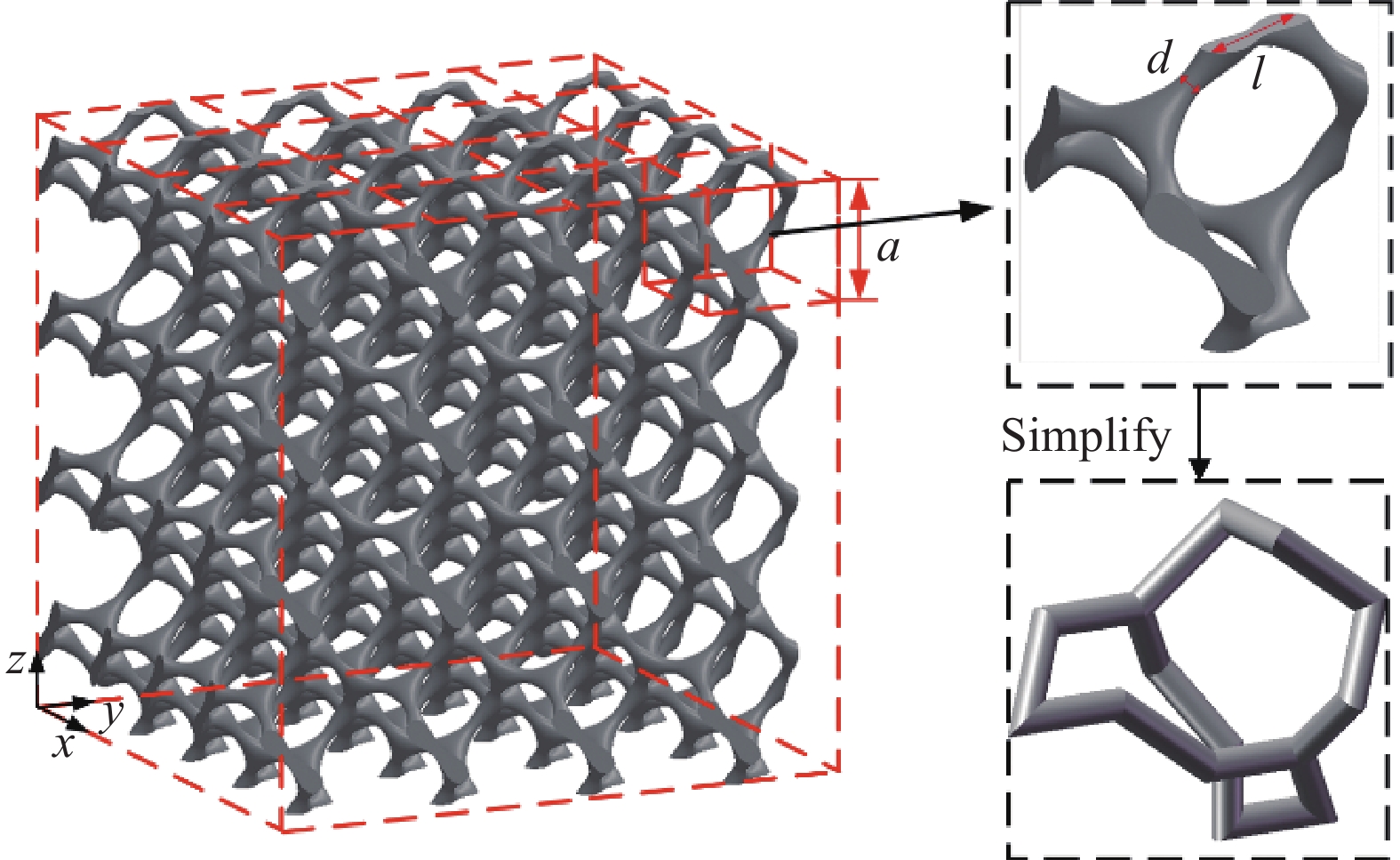
Gyroid隐式曲面点阵结构表面光滑且模型内部全连通，不存在微桁架相邻杆件连接处应力集中的问题，在点阵结构晶胞构型的应用上已被证明具有较大的优势和潜力。塑性屈服强度是点阵结构与密实结构存在的力学性能差异之一，研究其屈服行为可为点阵结构的设计和应用提供重要的理论依据。然而，对Gyroid隐式曲面点阵结构屈服强度研究大多基于仿真和实验，缺乏理论支撑。
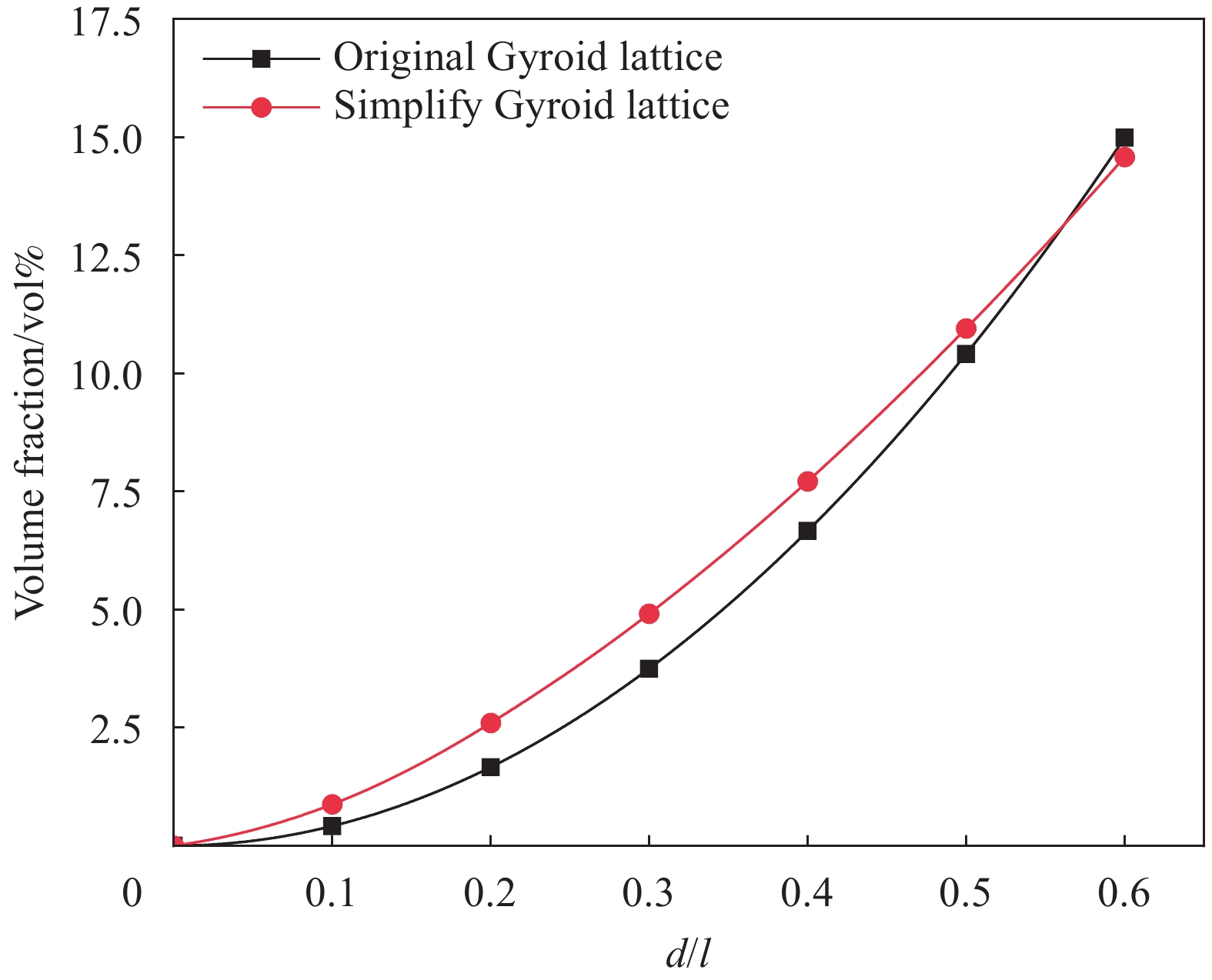
本文考虑Gyroid点阵结构的几何特性，在受力分析基础上基于变形体功能原理建立其塑性本构模型，得到点阵结构塑性屈服强度与体积分数之间的映射关系，来预测任意体积分数Gyroid点阵结构的屈服强度；然后，基于有限元分析软件Abaqus对Gyroid点阵结构准静态压缩过程开展仿真实验，初步验证理论模型的准确性；最后，通过选择性激光熔化制备不同体积分数316L不锈钢Gyroid点阵结构，进行单轴压缩实验，分析其变形机制与力学性能。
结果表明，理论推导、有限元仿真结果与实验结果相比，误差在25%以内，且根据三种方法结果拟合得到的Gibson-Ashby模型系数具有较好的一致性，表明本文基于理论推导建立的Gyroid点阵结构塑性屈服强度预测模型的有效性。理论模型的构建方法可以转化到其他复杂类型点阵结构中，为快速核算点阵结构力学性能，并将其应用在工程装备中提供理论依据。
Gyroid点阵结构塑性屈服强度研究
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
