SiC/AZ91D复合材料中孔隙缺陷对裂纹萌生和扩展行为的影响

李步炜; 尧军平; 陈国鑫; 李怡然; 梁超群

doi:10.13801/j.cnki.fhclxb.20230711.002

SiC/AZ91D复合材料中孔隙缺陷对裂纹萌生和扩展行为的影响

南昌航空大学　航空制造工程学院，南昌 330000

基金项目: 国家自然科学基金(52065046；51661024)；江西省科技重点研发计划(20202BBEL53024)；研究生创新专项(2030009101050)

详细信息

通讯作者:
尧军平，博士，教授，硕士生导师，研究方向为金属基复合材料　E-mail: yyyjpsz@126.com

中图分类号: TB331
计量
- 文章访问数: 812
- HTML全文浏览量: 384
- PDF下载量: 58
出版历程
- 收稿日期: 2023-05-23
- 修回日期: 2023-06-27
- 录用日期: 2023-06-30
- 网络出版日期: 2023-07-10
- 刊出日期: 2024-02-29

Effect of porosity defects on crack initiation and propagation behavior in SiC/AZ91D composites

School of Aeronautical Manufacturing Engineering, Nanchang Hangkong University, Nanchang 330000, China

Funds: National Natural Science Foundation of China (52065046; 51661024); Jiangxi Provincial Science and Technology Key Research and Development Program (20202BBEL53024); Postgraduate Innovation Special Fund Project (2030009101050)

摘要

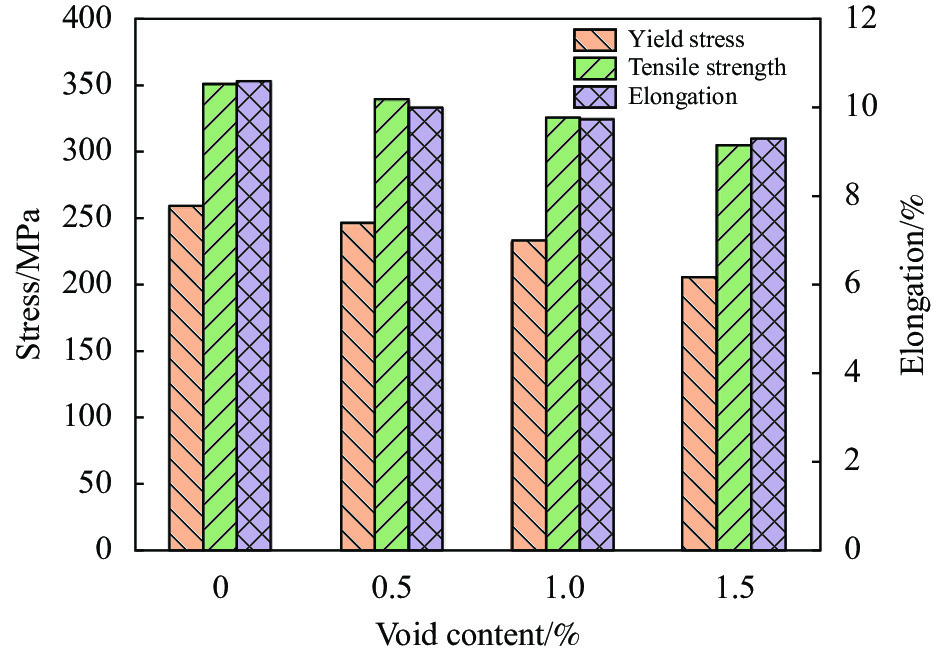
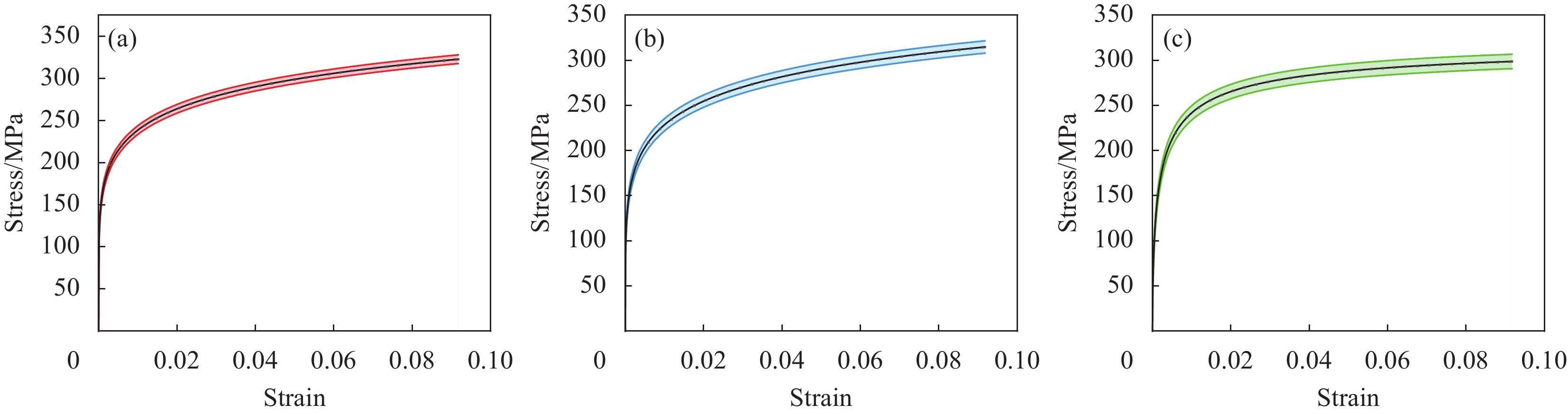
摘要: 采用内聚力模型及有限元分析方法，在含真实形貌SiC颗粒增强AZ91D镁基复合材料中引入孔隙缺陷，分析不同孔隙率及孔隙形状在单轴拉伸过程中对SiC/AZ91D复合材料力学行为的影响。结果表明：孔隙长径比为1时，孔隙率为0%、0.5%、1.0%、1.5%的复合材料的抗拉强度分别为351.214 MPa、339.452 MPa、325.735 MPa、306.791 MPa，抗拉强度随孔隙率的增加逐渐降低，复合材料中裂纹萌生和裂纹扩展时间均随孔隙率增加而提前。孔隙长径比越大，其尖端部位应力集中越严重，复合材料抗拉强度也越低。无孔隙缺陷的SiC/AZ91D复合材料裂纹萌生扩展机制是颗粒与基体交界处萌生微裂纹，微裂纹相互连接形成主裂纹绕开颗粒进行扩展致使材料断裂，含孔隙的SiC/AZ91D复合材料裂纹萌生扩展机制为微裂纹在孔隙周围萌生，与颗粒和基体交界处产生的微裂纹相互连接，汇集成主裂纹绕开颗粒扩展使材料断裂。
- SiC/AZ91D复合材料 /
- 裂纹萌生 /
- 裂纹扩展 /
- 有限元分析 /
- 孔隙率 /
- 内聚力模型
Abstract: Using the finite element analysis method, this study introduced porosity defects into SiC/AZ91D magnesium matrix composites with realistic SiC particle morphology, and analyzed the influence of different porosity rates and shapes on the mechanical behavior of SiC/AZ91D composites during uniaxial tensile process. The results show that when the aspect ratio of the pore length to width is 1, the tensile strengths of the composites with void contents of 0%, 0.5%, 1.0%, and 1.5% are 351.214 MPa, 339.452 MPa, 325.735 MPa and 306.791 MPa, respectively. The tensile strength gradually decreases with the increase of porosity rate, and the initiation and propagation time of cracks in the composite material advances with the increase of porosity rate. As the aspect ratio of the pore length to width increases, the stress concentration at the tip of the pore becomes more severe, resulting in lower tensile strength of the composite material. The crack initiation and propagation mechanism in the SiC/AZ91D composite material without porosity defects involves the initiation of microcracks at the particle-matrix interface, followed by their interconnection to form a main crack, which propagates around the particles leading to material fracture. In the case of SiC/AZ91D composites with porosity, microcracks initiate around the pores and interconnect with microcracks generates at the particle-matrix interface, ultimately converging into a main crack that propagates around the particles, causing material fracture.
- SiC/AZ91D composites /
- crack initiation /
- crack extension /
- finite element analysis /
- porosity /
- cohesive model

HTML全文

复合材料由于具有高比强度、高比刚度、抗疲劳、抗腐蚀及可设计性等优点，被广泛地运用在航空航天、船舶、汽车、能源等领域^[1-5]。随着使用范围的逐渐扩大和应用场景的不断丰富，明确不同环境下复合材料的力学性能至关重要^[6]。水分是复合材料面临的一个主要环境因素，当复合材料长期暴露在水分中时，由于吸湿作用，复合材料基体、纤维和基体界面及复合材料层间会出现不同程度的损伤，导致复合材料的力学性能退化^[7]。实际服役过程中，复合材料受到水分影响的同时还面临不同载荷工况的作用，外载荷可能会在复合材料中诱发微裂纹和界面脱粘，从而影响复合材料的吸湿行为，进而导致复合材料的力学行为和失效机制更加复杂^[8]。因此，研究长期水分-载荷作用下复合材料的力学行为对复合材料的安全评估具有重要意义。

复合材料的基体为高分子材料，对水分较敏感，研究者最先关注的是复合材料的吸湿性能。Pradip等^[9]研究了不同纤维类型及混杂纤维复合材料的吸湿性能，同时应用ANSYS仿真模拟了水分扩散过程。Rocha等^[10]选取纤维随机分布材料的代表性体积单元研究了界面对水分扩散过程的影响。国内学者同样通过试验研究了不同型号复合材料在各种湿热环境下的吸湿性能，并应用有限元分析、理论计算等方法对复合材料吸湿性能进行了模拟预测^[11-12]。吴以婷等^[13]研究了碳纤维增强环氧树脂基复合材料层合板的吸湿特性，发现材料在脱湿和吸湿过程中均呈现出两段式的特点。此外，材料吸湿后的力学性能退化也备受关注。Shetty等^[14]围绕吸湿后复合材料性能退化的机制进行研究，发现经过湿热老化后碳纤维/环氧树脂复合材料层合板的层间剪切强度降低近1/3。张裕恒等^[15]测试了碳纤维增强乙烯基树脂复合材料在水箱中老化90天后的压缩强度和层间剪切强度，二者分别下降了7.6%和12.3%。对于酚醛树脂复合材料和环氧树脂复合材料，吸湿后性能同样会发生退化^[16-17]。

上述的研究仅考虑了水分单独作用的情况，未考虑吸湿过程中载荷的影响。研究表明拉伸预应力对单向带复合材料的吸湿特性影响明显，能加速水分的扩散。Humeau等^[18]在吸湿过程中给±45°铺层的层合板施加了静态拉伸载荷，发现拉伸载荷对试样的吸湿特性影响明显。李彪等^[19]还研究了海水浸泡与拉伸应力耦合下碳纤维增强复合材料单向层合板的剩余强度，指出预应力的存在会造成复合材料的抗拉强度和弹性模量的进一步退化，且应力水平越高，这种退化现象越明显。当复合材料层合板受到弯曲载荷-水分作用时，其吸湿特性和剩余强度也会受到影响，当弯曲应力为60%弯曲强度时，吸湿量上升最快，材料强度下降最严重，达 21.15%^[20]。

相较于单向复合材料，编织复合材料中编织物增强体的纤维相互缠结，显示出较强的整体性，使其具有更好的抗冲击性和损伤容限等优点，常作为主传力或次传力结构参与结构的载荷传递过程^[21]。在承受载荷的同时，编织复合材料同样受到水分等环境因素的影响。而关于水分-载荷耦合作用下编织复合材料吸湿行为和性能退化机制的研究较匮乏。因此，本文针对碳纤维增强环氧树脂(T300/H69)二维(2D)编织复合材料在长期水分-载荷作用后的力学行为展开研究，设计了水分-拉伸载荷耦合作用下的加载装置，开展了不同拉伸预应力下的吸湿试验和强度性能退化试验，研究拉伸预应力对其吸湿特性的影响，分析不同水分-载荷作用下T300/H69编织复合材料弹性模量及失效强度的退化规律，并结合宏微观形貌分析，揭示长期水分-载荷耦合作用下T300/H69编织复合材料力学性能的退化机制，最后建立水分-载荷作用下复合材料的剩余强度预测方法。

1. 试验方法

1.1 原材料与制备

选取平纹编织的碳纤维增强环氧树脂基复合材料(T300/H69)进行研究。其中每10 mm×10 mm的面积内，经纱和纬纱各铺设5条，每条纱束中包含碳纤维单丝约3000根。通过真空袋成型工艺制备T300/H69编织复合材料，固化压力为0.16 MPa，材料的铺层方式为[(±45°)₁₀]，单层厚度为0.18 mm。T300平纹编织复合材料的基本力学性能参数如表1所示。

表 1 碳纤维增强环氧树脂(T300/H69)复合材料的基本力学性能参数

Table 1. Basic mechanical properties of carbon fiber reinforced epoxy resin (T300/H69) composites

Engineering constant	Value	Engineering constant	Value
E₁/GPa	55.90	X_T/MPa	602
E₂/GPa	54.60	X_C/MPa	431
E₃/GPa	7.220	Y_T/MPa	597
G₁₂/GPa	3.812	Y_C/MPa	422
G₁₃/GPa	3.122	Z_T/MPa	60
G₂₃/GPa	3.122	Z_C/MPa	152
$\nu _{12}$	0.068	S₁₂/MPa	83
$\nu _{13}$	0.310	S₁₃/MPa	49
$\nu_{23}$	0.310	S₂₃/MPa	49
Notes: E₁, E₂ and E₃—Elastic modulus in different directions; G₁₂, G₁₃ and G₂₃—Shear modulus in different directions; $\nu_{12}$ , $\nu_{13}$ and $\nu_{23}$ —Poisson's ratio of the material; X_T, X_C, Y_T, Y_C, Z_T, Z_C—Axial tension, axial compression, transverse tension, transverse compression, normal tension, normal tension; S₁₂, S₁₃, S₂₃—In-plane shear strength.

下载: 导出CSV

| 显示表格

固化完成后，参考GB/T 1447—2005^[22]切割成试样，切割完成的T300/H69编织复合材料尺寸如图1(b)所示。

图 1 拉伸预应力施加系统

Figure 1. Tension prestressing application system

R—Radius

下载: 全尺寸图片幻灯片

1.2 水分-载荷环境试验

为了实现水分-载荷耦合环境的作用，设计了如图1所示的夹具及载荷施加装置。整套装置主要包含穿心千斤顶(配有液压泵及泵表)、应力支撑型材架、传力杆、夹板。其中穿心千斤顶用于施加拉伸载荷，通过观察液压泵表的数值控制手动泵的行程，可以实现不同大小拉伸载荷的施加，利用传力杆将载荷传递到试样上。试样两端通过不锈钢夹板进行夹持，并且通过多个夹板实现试样串联，通过螺丝固定在应力支撑型材架上。参考标准HB 7401—2020^[23]，通过浸泡法进行吸湿试验，吸湿量通过下式进行计算：

$M_t=\left|\frac{W_t-W_0}{W_0}\right|\times100\%$

(1)

式中：W₀为干燥试样的质量；W_t为t时间材料试样的质量；M_t为t时刻试样的吸湿量。

将夹持好的试样放置于亚克力水槽中进行浸泡，水温为室温。定期将试样取出，用吸水纸擦干水分后记录试样质量，称重完放回水槽继续浸泡，每组试样包含4件重复试验件。通过静力试验获得材料的屈服强度σ_s和弹性模量E。以屈服强度为参考，分别设置低应力状态80%σ_s、标准应力状态100%σ_s、高应力状态120%σ_s、140%σ_s这4种预应力水平来分析T300/H69编织复合材料力学性能的退化行为。

1.3 拉伸失效试验

对干燥试样和环境处理后的试样进行拉伸失效测试，以研究水分单独和水分-载荷耦合作用下复合材料的强度变化。考虑到不同时间下材料的吸湿量不同，对材料性能的影响也不同。因此选取144 h的时间间隔，分别以0 h、144 h、288 h、432 h为试验工况进行吸湿后的拉伸性能测试，当浸泡时间达到144 h、288 h、432 h后将试样取出称重，之后立即开展静力试验。同时以屈服应力为界，设置仅有预应力作用的对照组。试验矩阵如表2所示。

表 2 试验矩阵

Table 2. Test matrix

Pretreated	Specimen group	Test time/h
Untreated	Dry	0
Moisture	Wet	144, 288, 432
Load	D-80%σ_s	288
	D-110%σ_s	288
	D-120%σ_s	288
Moisture-load	W-80%σ_s	144, 288, 432
	W-100%σ_s	144, 288, 432
	W-120%σ_s	144, 288, 432
	W-140%σ_s	144, 288, 432
Notes: D and W—Dry state and moisture absorption state of the material; σ_s—Yield stress.

下载: 导出CSV

| 显示表格

采用深圳万测试验设备有限公司制造的TSE 504C电子万能试验机进行拉伸试验，试验中通过引伸计测试试件的变形，按1 mm/min的加载速度进行试验，每类工况重复测试4件试样。试验均在室温下进行。

1.4 微观形貌表征

从受水分-载荷耦合作用后的复材试样的破坏区域切割出长宽各10 mm的小方块型试样，通过ZEISS公司制造的SUPRA 55场发射扫描电子显微镜 (SEM) 观察试样拉伸断口处纤维和基体的损伤形貌。观察前对试样进行喷金处理。

2. 结果与讨论

2.1 T300/H69编织复合材料的吸湿特性分析

通过浸泡吸湿试验获得了T300/H69编织复合材料的吸湿量与吸湿时间的关系，如图2中的散点所示。

图 2 不同预应力下T300/H69编织复合材料的吸湿量-时间变化曲线

Figure 2. Moisture absorption-time curves of T300/H69 braided composites under different prestresses

下载: 全尺寸图片幻灯片

可以看出在不同的拉伸预应力下，T300/H69编织复合材料的吸湿规律基本一致。在吸湿前期，吸湿量随着吸湿时间迅速增大，随着吸湿时间不断增加，吸湿量的增加速率有所减缓。此外，拉伸预应力对吸湿量有明显影响。比如吸湿432 h后，5种拉伸预应力下材料的吸湿量分别为0.404%、0.543%、0.656%、0.881%、1.240%，说明在相同的浸泡时间下，预应力越大，编织复合材料的吸湿量越大，相较于无应力状态的吸湿量，140%σ_s状态下的吸湿量增加了207%。

进一步分析拉伸预应力对材料吸湿过程的影响，选取144 h、288 h、432 h这3个典型时刻的吸湿量，得到吸湿量随拉伸预应力的变化曲线如图3所示。可知，在水分-载荷耦合环境下，T300/H69编织复合材料的吸湿量与拉伸预应力总体上呈现正相关。而且以屈服应力为界限，当拉伸预应力小于材料的屈服应力时，吸湿量增加相对平缓，越靠近屈服应力，吸湿量的增加幅度越明显，一旦拉伸预应力超过材料的屈服应力，T300/H69编织复合材料的吸湿量显著提高。

图 3 不同预应力下T300/H69编织复合材料的吸湿量

Figure 3. Moisture absorption of T300/H69 braided composites under different prestresses

下载: 全尺寸图片幻灯片

Fick模型^[24-25]常被用于表征水分在材料中的扩散过程，如下式所示：

$\frac{M_t}{M_{\infty}}=\left\{1-\frac{8}{\text{π}^2}\sum\limits_{j=0}^{\infty}\frac{\exp\left[-\left(2j+1\right)^2\text{π}^2\left(Dt/h^2\right)\right]}{\left(2j+1\right)^2}\right\}$

(2)

在实际工作中，常常对Fick模型进行数学简化，简化结果如下式所示：

$\frac{{{M_t}}}{{{M_\infty }}} = 1 - \exp \left[ { - 7.3{{\left( {\frac{{Dt}}{{{h^2}}}} \right)}^{0.75}}} \right]$

(3)

式中：h为材料的厚度；t为试样的吸水时间；D为试样的水分扩散系数；M_∞为平衡吸湿量。

针对图2中的试验点，将水分扩散系数D和平衡吸湿量M_∞看作未知参数，采用式(3)对其进行拟合，得到的水分扩散系数和平衡吸湿量汇总于表3。此外Fick模型的拟合曲线如图2中实线所示，可以看出Fick模型可以很好地描述水分-载荷作用下编织复合材料的吸湿行为。

表 3 Fick吸湿模型的相关参数

Table 3. Relevant parameters of Fick moisture absorption

Tensile prestress	Diffusion coefficient D/(mm²·h⁻¹)	Balanced moisture content M_∞/%
0	0.00175	0.464
80%σ_s	0.00192	0.612
100%σ_s	0.00213	0.706
120%σ_s	0.00229	0.917
140%σ_s	0.00257	1.297

下载: 导出CSV

| 显示表格

由表3可知，对于T300/H69编织复合材料而言，在432 h试验测量的吸湿量均小于Fick模型预测的结果，表明试样未到达吸湿平衡。从表中可以看出吸湿后的T300/H69编织复合材料的吸湿扩散系数和平衡吸湿量均与拉伸预应力正相关，随拉伸预应力的增大逐渐增大，表现出明显的非线性关系。这是由于拉伸预应力会使材料产生一定程度的微裂纹等缺陷，导致水分进入材料的速率变快，T300/H69编织复合材料对水分的吸收和容纳能力变强。

2.2 T300/H69编织复合材料的剩余力学性能

2.2.1 载荷-位移分析

对于施加相同预应力的试样，其在不同时间环境处理后的载荷-位移曲线变化规律一致，因此选取作用288 h的试样进行研究。分别给出干态、仅预应力、仅吸湿、水分-载荷耦合作用下的载荷-位移曲线进行分析，如图4所示。

对于本文研究的 [(±45°)₁₀]铺层的T300/H69编织复合材料，从图4(a)和图4(e)可看出，干态和水分单独作用后其载荷-位移曲线具有相同的变化趋势，均表现明显的双线性特征；从图4(b)~图4(d)可以看出，仅预应力也会对材料的拉伸性能产生劣化影响，当预应力超过屈服应力时，双线性特征不再明显。对于水分-载荷耦合作用的试样，从图4(f)~图4(i)可以看出，引入水分作用后，材料性能劣化程度会进一步加剧，当应力水平小于100%σ_s时，其载荷-位移曲线也具有明显的双线性特征，但当拉伸预应力超过100%σ_s时，这种双线性特征受到的影响更加显著，在120%σ_s时两阶段曲线的界限已不再分明，当预应力为140%σ_s时，载荷-位移曲线已无双线性变化规律。对于144 h和432 h的工况均存在同样的现象。

图 4 不同工况下作用288 h后T300/H69编织复合材料的载荷-位移曲线

Figure 4. Load-displacement curves of T300/H69 braided composites after 288 h under different working conditions

下载: 全尺寸图片幻灯片

上述结果表明，相对于水分单独作用和预应力单独作用，水分-载荷耦合对T300/H69编织复合材料拉伸变形造成的影响更明显。

2.2.2 模量及强度分析

进一步分析水分-载荷耦合作用对T300/H69编织复合材料失效强度(σ_f)和弹性模量(E)的影响，结果如表4和图5所示。

表 4 T300/H69编织复合材料的弹性模量及强度

Table 4. Elastic modulus and strength of T300/H69 braided composites

Category		144 h		288 h		432 h
Category		E/GPa	σ_f/GPa	E/GPa	σ_f/GPa	E/GPa	σ_f/GPa
Moisture	Average	13.721	0.195	13.627	0.193	13.586	0.192
Moisture	CV	2.13%	2.31%	4.61%	2.01%	1.09%	1.82%
D-80%σ_s	Average	—	—	13.204	0.191	—	—
D-80%σ_s	CV	—	—	2.61%	2.30%	—	—
D-100%σ_s	Average	—	—	12.715	0.187	—	—
D-100%σ_s	CV	—	—	1.89%	1.6%	—	—
D-120%σ_s	Average	—	—	10.643	0.180	—	—
D-120%σ_s	CV	—	—	3.64%	1.78%	—	—
W-80%σ_s	Average	12.873	0.190	12.682	0.184	12.535	0.181
W-80%σ_s	CV	2.05%	0.74%	3.01%	1.36%	2.43%	1.88%
W-100%σ_s	Average	11.947	0.182	11.516	0.174	11.419	0.169
W-100%σ_s	CV	4.19%	2.58%	3.32%	2.82%	5.08%	3.02%
W-120%σ_s	Average	10.404	0.162	9.506	0.151	8.975	0.143
W-120%σ_s	CV	3.27%	3.33%	4.12%	3.88%	5.39%	2.66%
W-140%σ_s	Average	9.232	0.151	7.521	0.138	6.136	0.128
W-140%σ_s	CV	4.78%	2.72%	5.66%	3.38%	7.12%	4.11%
Notes: E—Elastic modulus of the material; σ_f—Failure strength of the material; CV—Coefficient of variation.

下载: 导出CSV

| 显示表格

图 5 不同环境中T300/H69编织复合材料的弹性模量(a)及失效强度(b)

Figure 5. Elastic modulus (a) and failure strength (b) of T300/H69 braided composites in different environments

下载: 全尺寸图片幻灯片

从表4和图5中可以看出，对于所有的工况，弹性模量及失效强度的变异系数(CV)均不超过10%，表明试验数据具有较好的重复性，且失效强度和弹性模量随着吸湿时间的增加均逐渐下降。水分单独作用下，材料的弹性模量及强度下降幅度较低，此时两者最大分别仅下降了2.34%和3.03%。预应力单独作用时，模量和强度均会随应力水平的增加而降低。水分-载荷耦合作用下，材料的弹性模量及强度的下降幅度更大，例如在288 h，D-120%σ_s工况下的弹性模量和失效强度分别下降了23%和9.1%，W-120%σ_s工况下弹性模量和失效强度分别下降了31.7%和 23.7%。这说明水分-载荷耦合效应会导致材料内部损伤加剧。

进一步分析失效强度与预应力的变化关系，如图6所示。可知，不论是干态还是水分-应力耦合作用，只要预应力超过屈服应力，材料的强度均会有明显下降。下降幅度与拉伸预应力相关，应力水平越高，弹性模量及强度越低，两者最大下降达55.9%和35.4%。同时，以屈服应力为界限，当预应力小于材料的屈服应力时，材料强度的下降幅度较平缓，一旦预应力超过屈服应力，材料强度的下降趋势加剧。这表明超过屈服应力的预加载应力会使材料的潜在损伤更加剧烈。

图 6 T300/H69编织复合材料的预应力-失效强度曲线

Figure 6. Prestress-failure strength curves of T300/H69 braided composites

下载: 全尺寸图片幻灯片

在长期的水分-载荷环境作用中，吸湿量是能够直接检测的参数，也是反映材料受到环境影响的重要指标。因此，图7进一步分析不同工况下T300/H69编织复合材料弹性模量及失效强度随吸湿量的演变规律。

可以看出，在任意应力水平下，T300/H69编织复合材料的弹性模量和强度均随着吸湿量的增大而减小，而且表现出明显线性关系。随着拉伸预应力的增大，拟合曲线的斜率增加，表明材料的弹性模量和强度劣化更严峻。此外，对比图7(a)和图7(b)还可以发现，T300/H69编织复合材料弹性模量的下降程度明显大于失效强度，该规律不受预应力水平的影响。

2.3 T300/H69编织复合材料的失效机制

为了揭示水分-载荷作用后编织复合材料力学性能的退化机制，分别对破坏后的宏观失效模式和微观形貌进行分析。

2.3.1 宏观失效模式分析

不同工况下编织复合材料的宏观失效模式基本一致，选取432 h的失效照片和干燥情况对比，如图8所示，可以看出，断裂位置全部位于试验件的中央标距段内，断口均呈现出V字型，断口较齐整，左右两侧断口区域能完全重叠，并且绕断裂中心处旋转对称，断裂面沿着纱束的走向进行扩展，并可见一定数量的断裂纤维。由断口形状可知，水分-载荷耦合作用对T300/H69编织复合材料的宏观破坏模式影响较小。

图 7 T300/H69编织复合材料的弹性模量(a)及失效强度(b)随吸湿量的变化

Figure 7. Elastic modulus (a) and failure strength (b) of T300/H69 braided composites change with moisture absorption

下载: 全尺寸图片幻灯片

图 8 T300/H69编织复合材料宏观断口形貌

Figure 8. Macroscopic fracture surface of T300/H69 braided composites

下载: 全尺寸图片幻灯片

2.3.2 微观形貌分析

图9为干燥和吸湿432 h试样的SEM结果对比。可以看出：(1)干燥状态下试样断口的树脂较光滑，树脂内裂纹较少，如图9(a)所示；(2)当水分单独作用时，图9(b)显示树脂基体表面较粗糙，出现大量的“褶皱”条纹，表明在长期的水分作用下树脂基体发生了塑化，此外，还能观察到少量的基体微裂纹；(3)对于水分-载荷耦合作用的试样也出现了吸湿塑化，从图9(c)~9(f)中同样能观察到粗糙的基体断面。与此同时，受预应力作用的基体微裂纹数量明显增多。此外，当拉伸预应力为100%σ_s时，观察到纤维-基体界面脱粘，当拉伸预应力为120%σ_s和140%σ_s，纤维-基体界面脱粘的区域显著增加。故而基体微裂纹和纤维-基体界面脱粘程度的加剧是水分-载荷作用后编织复合材料剩余强度进一步劣化的主要诱因。

图 9 T300/H69编织复合材料试样拉伸断口的SEM图像

Figure 9. SEM images of tensile fracture of T300/H69 braided composites specimen

下载: 全尺寸图片幻灯片

2.4 T300/H69编织复合材料的剩余强度预测

虽然通过试验能获得T300/H69编织复合材料在水分-载荷耦合作用下力学性能的变化规律，但试验成本较高，试验周期较长。因此，建立水分-载荷耦合作用下复合材料剩余强度的有效预测方法，对于复合材料的工程应用具有重要意义。

Shiva等^[26]认为复合材料的剩余强度与水分和外部载荷均呈现指数型下降规律，如下式所示：

$\frac{{{\sigma _t}}}{{{\sigma _{{\mathrm{dry}}}}}} = \exp \left[ { - {{\left( {\frac{D}{\phi }} \right)}^2}At} \right]\exp \left[ { - {{\left( {\frac{D}{\phi }} \right)}^2}B{\sigma _{{\mathrm{prestress}}}}t} \right]$

(4)

式中：σ_t为t小时后复合材料的抗拉强度；σ_dry为干燥条件下的抗拉强度；σ_prestress为拉伸预应力；t为水分-载荷耦合作用时间；A为水分老化常数，由吸湿老化后的试验结果获得；B为应力系数，可从材料在水分-载荷耦合作用后的强度退化结果获得； $\phi$ 为几何参数，取材料表面积之和与上下表面面积之和的比值。

Shiva模型中假设吸湿老化时间较长时，复合材料的剩余强度为0，这一假设与实际工程情况存在差异。在无穷时间吸湿后，材料往往还具有一定的剩余力学性能。因此，考虑到无穷老化时间后复合材料仍具有残余性能这一特性，本文对Shiva剩余强度模型做了修正，引入水分-载荷耦合作用无穷时间后的剩余强度σ_∞，得到水分-载荷耦合作用下T300/H69编织复合材料剩余强度预测模型，如下式所示：

${ {\sigma _t} = \left( {{\sigma _{{\mathrm{dry}}}} - {\sigma _\infty }} \right)\exp \left( { - {{\left( {\frac{D}{\phi }} \right)}^2}At} \right)\exp \left( { - {{\left( {\frac{D}{\phi }} \right)}^2}B{\sigma _{{\mathrm{prestress}}}}t} \right) + {\sigma _\infty } }$

(5)

从试验测量的吸湿量可以发现，试样并未达到吸湿平衡，但从图7可知材料的强度和吸湿量存在线性关系，因此结合图7中的线性关系和表3中的平衡吸湿量(1.297%)对σ_∞进行预测，得到σ_∞为119 MPa，基于该值对强度进行预测，结果如图10所示。

图 10 T300/H69编织复合材料不同模型下的拟合结果

Figure 10. Fitting results under different models of T300/H69 braided composites

下载: 全尺寸图片幻灯片

可以看出，对于受水分-载荷耦合作用的T300/H69编织复合材料而言，当拉伸预应力不超过屈服应力时，Shiva剩余强度模型的预测效果较好，而一旦拉伸预应力超过屈服应力，其预测精度明显较低，尤其是在高应力水平时，误差较大，对于W-140%σ_s 工况下的试样，在432 h的预测误差达7.5%。较Shiva剩余强度模型而言，本文建立的模型能够很好地预测不同应力水平下复合材料的剩余强度，最大相对误差仅约3.2%，预测精度高于Shiva模型。

为了进一步验证本文提出的理论模型，对文献[20]中30%弯曲抗拉强度、60%弯曲抗拉强度下受弯吸湿层合板的剩余弯曲强度数据进行了拟合，拟合结果如图11所示。

图 11 文献[20]数据拟合

Figure 11. Literature [20] data fitting

下载: 全尺寸图片幻灯片

可知，两种弯曲载荷下，预测值与试验数据的最大相对误差仅为1.85%。进一步验证了本文建立的剩余强度模型的有效性，可指导受水分-载荷作用后复合材料的安全评估。

3. 结论

本文通过试验和理论研究了二维(2D)编织复合材料在水分-载荷长期耦合作用下的力学性能退化行为。主要结论如下:

(1)碳纤维增强环氧树脂(T300/H69)编织复合材料的吸湿量随拉伸预应力的增加而增大，以屈服应力为界限，当拉伸预应力小于材料的屈服应力时，吸湿量增加相对平缓，一旦拉伸预应力超过材料的屈服应力，材料的吸湿量显著提高。Fick吸湿模型能够较好地描述材料在水分-载荷下的吸湿特性；

(2)长期水分-载荷环境作用对T300/H69编织复合材料力学性能影响显著，随着应力水平的增加，其弹性模量和强度的退化越明显，且弹性模量的下降程度大于失效强度，在140%σ_s (σ_s为屈服强度)预应力下作用432 h后下降分别达55.9%和35.4%。此外，T300/H69编织复合材料的弹性模量和强度退化比例与吸湿量存在线性关系；

(3)微观形貌分析表明吸湿会引起基体塑化，同时预应力导致基体微裂纹和纤维-基体界面脱粘程度加剧，揭示了水分-载荷作用后编织复合材料力学性能的下降机制；

(4)对Shiva剩余强度理论进行修正，建立了复合材料在水分-载荷耦合作用下的剩余强度预测模型，实现了良好预测。

图 1 SiC颗粒的SEM图像及其建模

Figure 1. SEM images and modeling of SiC particles

下载: 全尺寸图片幻灯片

图 2 不同孔隙率的SiC/AZ91D镁基复合材料模型：(a) VC=0 (理想状况)；(b) VC=0.5%；(c) VC=1.0%；(d) VC=1.5%

Figure 2. Modeling diagram of SiC/AZ91D magnesium-based composite materials with different void contents: (a) VC=0 (Ideal condition); (b) VC=0.5%; (c) VC=1.0%; (d) VC=1.5%

下载: 全尺寸图片幻灯片

图 3 SiC/AZ91D镁基复合材料模型及网格划分和载荷施加：(a) SiC/AZ91D模型；(b) 网格划分；(c) 载荷施加

Figure 3. Modeling, meshing and load application of SiC/AZ91D magnesium-based composite materials: (a) SiC/AZ91D model; (b) Meshing; (c) Load application

下载: 全尺寸图片幻灯片

图 4 不同孔隙形状的SiC/AZ91D镁基复合材料模型：(a) 长径比r=1；(b) r=2；(c) r=4

Figure 4. Model of SiC/AZ91D magnesium-based composite materials with different pore shapes: (a) Aspect ratio of the pore length to width r=1; (b) r=2; (c) r=4

下载: 全尺寸图片幻灯片

图 5 内聚力单元受力变形模型

Figure 5. Force-deformation model of cohesive element

下载: 全尺寸图片幻灯片

图 6 双线性内聚力模型

Figure 6. Bilinear cohesive zone model

${\delta }_{\mathrm{m}}^{\text{max}}$ —Maximum value of the effective displacement; ${\delta }_{\mathrm{m}}^{\mathrm{f}}$ —Effective displacement at complete failure; ${\delta }_{\mathrm{m}}^{0}$ —Effective displacement at the initiation of damage; ${\tau }_{\mathrm{m}}^{0}$ —Maximum separation stress; K—Elasticity coefficient or spring constant; D—Damage amount

下载: 全尺寸图片幻灯片

图 7 拉伸过程中含不同孔隙率SiC/AZ91D镁基复合材料应力-应变曲线

Figure 7. Stress-strain curves of SiC/AZ91D magnesium-based composite materials with different void content during tensile process

下载: 全尺寸图片幻灯片

图 8 拉伸过程中不同孔隙率SiC/AZ91D复合材料屈服应力值、抗拉强度和伸长率

Figure 8. Yield stress, tensile strength and elongation of SiC/AZ91D composite materials with different void contents during the tensile process

下载: 全尺寸图片幻灯片

图 9 孔隙周围A、B、C方向示意图

Figure 9. Schematic diagram of the A, B, and C directions around the void

S—Quivalent stress (MPa)

下载: 全尺寸图片幻灯片

图 10 孔隙周围基体A、B、C 方向应力随施载时间变化曲线

Figure 10. Stress variation curves with respect to loading time in the A, B, and C directions of the matrix around the void

下载: 全尺寸图片幻灯片

图 11 含不同孔隙率 SiC/AZ91D 复合材料裂纹长度随时间变化曲线

Figure 11. Crack length variation curves with respect to time in SiC/AZ91D composite materials with different void contents

下载: 全尺寸图片幻灯片

图 12 不同孔隙率的SiC/AZ91D复合材料裂纹扩展路径：(a) VC=0%；(b) VC=0.5%；(c) VC=1.0%；(d) VC=1.5%

Figure 12. Crack propagation paths in SiC/AZ91D composite materials with different void contents: (a) VC=0%; (b) VC=0.5%; (c) VC=1.0%; (d) VC=1.5%

下载: 全尺寸图片幻灯片

图 13 含不同孔隙形状的SiC/AZ91D复合材料的拉伸应力-应变曲线

Figure 13. Stress-strain curves during tension of SiC/AZ91D composite materials with different void shapes

下载: 全尺寸图片幻灯片

图 14 拉伸过程中含不同孔隙形状SiC/AZ91D 复合材料抗拉强度和伸长率

Figure 14. Tensile strength and elongation of SiC/AZ91D composite materials with different void shapes during the tensile process

下载: 全尺寸图片幻灯片

图 15 含不同形状孔隙的复合材料同一区域的应力场：(a) r=1；(b) r=2；(c) r=4

Figure 15. Stress field in the same region of composite materials with different void shapes: (a) r=1; (b) r=2; (c) r=4

下载: 全尺寸图片幻灯片

图 16 含不同孔隙形状 SiC/AZ91D 复合材料裂纹长度随时间变化曲线

Figure 16. Crack length variation curves with respect to time in SiC/AZ91D composite materials with different void shapes

下载: 全尺寸图片幻灯片

图 17 含不同形状孔隙的SiC/AZ91D复合材料的裂纹扩展路径：(a) r=1；(b) r=2；(c) r=4

Figure 17. Crack propagation paths in SiC/AZ91D composite materials with different void shapes: (a) r=1; (b) r=2; (c) r=4

下载: 全尺寸图片幻灯片

图 18 拉伸片试样

Figure 18. Tensile sheet sample

下载: 全尺寸图片幻灯片

图 19 实验拉伸片试样尺寸

Figure 19. Experimental tensile sheet sample size

R—Circle radius

下载: 全尺寸图片幻灯片

图 20 实验使用的WDW-E100D微机控制电子万能试验机

Figure 20. WDW-E100D microcomputer-controlled electronic universal testing machine used in the experiment

下载: 全尺寸图片幻灯片

图 21 含不同孔隙率的SiC/AZ91D复合材料仿真与实验拉伸应力-应变曲线对比

Figure 21. Comparison of simulated and experimental stress-strain curves during tensile testing of SiC/AZ91D composite materials with different void contents

下载: 全尺寸图片幻灯片

图 22 含不同孔隙率SiC/AZ91D复合材料拉伸应力-应变误差带图：(a) VC=0.5%；(b) VC=1.0%；(c) VC=1.5%

Figure 22. Tensile stress-strain error band diagram of SiC/AZ91D composite materials with different void contents: (a) VC=0.5%; (b) VC=1.0%; (c) VC=1.5%

下载: 全尺寸图片幻灯片

表 1 排水法测量的SiC/AZ91D复合材料孔隙率(VC)

Table 1 Void content (VC) measurement for SiC/AZ91D composite material using drainage method

Sample serial number	VC/%
1	1.56
2	0.84
3	0.85
4	1.62
5	0.83
6	0.41
7	1.65
8	0.94
9	0.48
10	1.18

下载: 导出CSV

表 2 AZ91D镁合金和SiC颗粒的基本参数

Table 2 Basic parameters of AZ91D magnesium alloy and SiC particles

Material	$\rho$ /(kg·m⁻³)	E/GPa	$\mu$	σ_b/MPa
AZ91D	1800	45	0.33	164
SiC	3215	450	0.17	2000
Notes: $\rho$ —Material density; E—Modulus of elasticity; $\mu$ —Poisson's ratio; σ_b—Tensile strength.

下载: 导出CSV

表 3 SiC/AZ91D颗粒-界面的本构模型参数

Table 3 Constitutive model parameters of SiC/AZ91D particle-interface

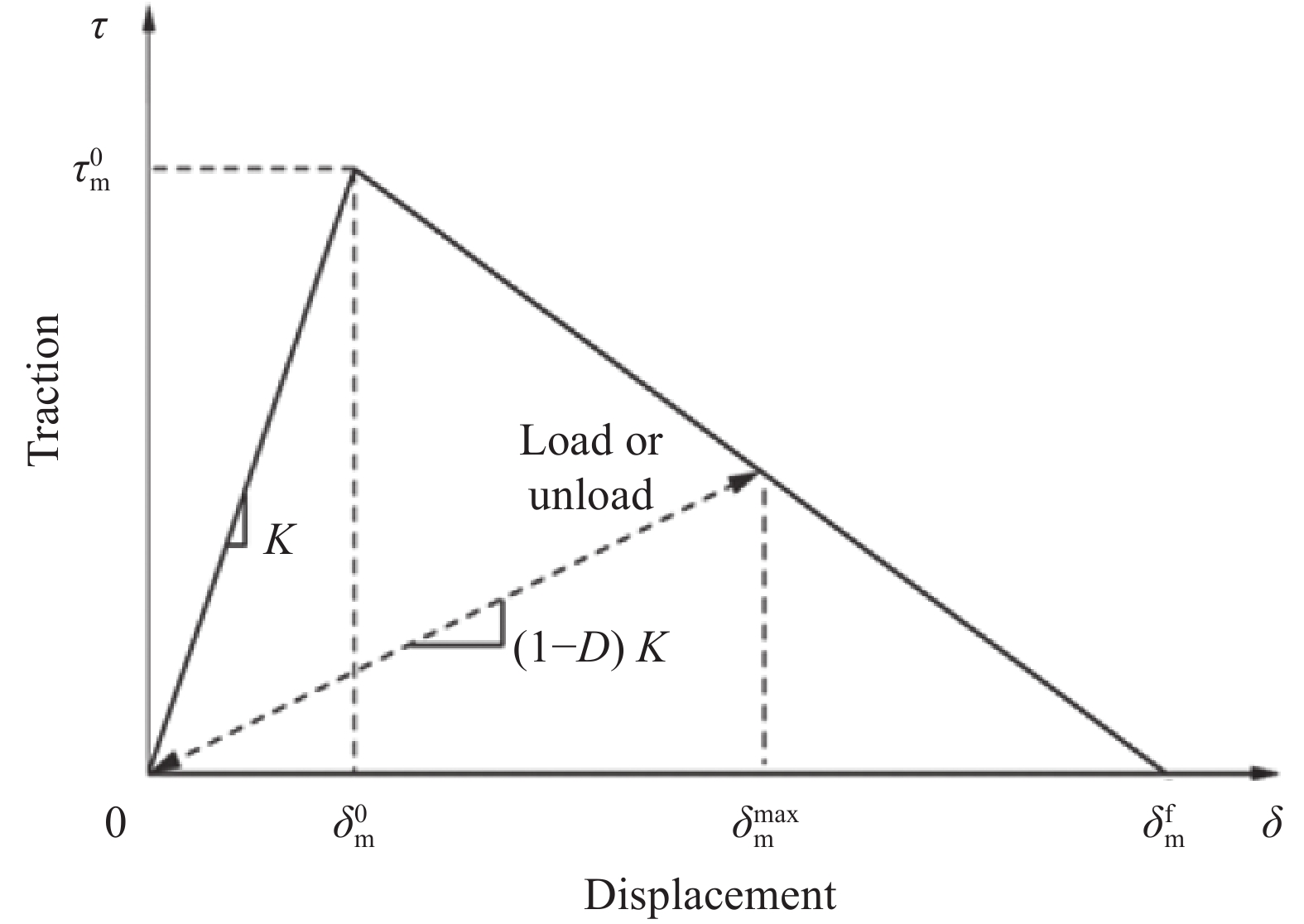
${t}_{\mathrm{n}}/\mathrm{M}\mathrm{P}\mathrm{a}$	${t}_{\mathrm{t}}/\mathrm{M}\mathrm{P}\mathrm{a}$	${\delta }_{\mathrm{m}\mathrm{a}\mathrm{x}}/\mathrm{m}\mathrm{m}$	${\delta }_{\mathrm{f}}/\mathrm{m}\mathrm{m}$
400	400	0.00015	0.00005
Notes: ${t}_{\mathrm{n}}$ —Interface normal nominal stress; ${t}_{\mathrm{t}}$ —Interfacial tangential nominal stress; ${\delta }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ —Destruction displacement; ${\delta }_{\mathrm{f}}$ —Material complete failure separation.

下载: 导出CSV

表 4 AZ91D镁合金的Johnson-Cook (J-C)本构参数

Table 4 Johnson-Cook (J-C) constitutive model parameters for AZ9ID magnesium alloy

A/MPa	B/MPa	n	C	${u}_{{\rm{f}}}^{\mathrm{p}\mathrm{l} }/{\rm{mm} }$
164	600	0.283	0.021	0.00015
Notes: A—Yield strength of AZ91D matrix under static load; B—Hardening constant; n—Hardening exponent; C—Strain rate constant; ${u}_{\mathrm{f}}^{\mathrm{p}\mathrm{l}}$ —Failure displacement.

下载: 导出CSV

表 5 SiC颗粒本构模型及失效参数

Table 5 Constitutive model and failure parameters of SiC particles

Parameter	Value
G/GPa	193
A₀	0.96
B₀	0.35
C₀	0.009
M	1
N	0.65
T₁/MPa	750
S_FMAX/MPa	1300
L_HE/MPa	11700
P_HEL/MPa	5130
D₁	0.48
D₂	0.48
K₁	220000
K₂	361000
K₃	0
Notes: G—Shear modulus; A₀—Strength parameter before damage; B₀—Strength parameter when damage occurs; C₀—Strain rate constant; M—Pressure index when damage occurs; N—Pressure index when no damage occurs; T₁—Cut-off pressure; S_FMAX—Maximum fracture strength; L_HE—Hugoniot elastic limit; P_HEL—Hugoniot elastic limit pressure; D₁, D₂—Fracture constant; K₁, K₂, K₃—Material parameter.

下载: 导出CSV

参考文献(34)

[1]	褚兵强. 低能量冲击对含孔隙CFRP层合板力学性能的影响研究[D]. 哈尔滨: 哈尔滨工业大学, 2011. CHU Bingqiang. The effects of low-energy impact on the mechanical properties of carbon fiber reinforced polymer laminates with voids[D]. Harbin: Harbin Institute of Technology, 2011(in Chinese).
[2]	李钊. 碳纤维复合材料孔隙率超声检测与评价技术研究[D]. 杭州: 浙江大学, 2014. LI Zhao. Research on ultrasonic detection and evaluation technique for porosity of carbon fiber composites[D]. Hangzhou: Zhejiang University, 2014(in Chinese).
[3]	李亚星, 李洪双, 陈普会. 考虑孔隙随机性的SiC/SiC复合材料力学性能参数概率分析[J/OL]. 应用力学学报: 1-12[2024-01-08]. LI Yaxing, LI Hongshuang, CHEN Puhui. Probability analysis of mechanical properties parameters of SiC/SiC composites considering pore randomness[J/OL]. Chinese Journal of Applied Mechanics: 1-12[2024-01-08](in Chinese).
[4]	HONG Y, WANG W J, LIU J Q, et al. Effect of porosity and interface structures on thermal and mechanical properties of SiCp/6061Al composites with high volume fraction of SiC[J]. Transactions of Nonferrous Metals Society of China,2019,29(5):941-949. DOI: 10.1016/S1003-6326(19)65003-X
[5]	GAO M Q, GUO E Y, CHEN Z N, et al. Revealing the role of micropore defects in tensile deformation of a B₄Cp/Al composite using an actual three-dimensional model[J]. Journal of Materials Research and Technology,2023,22:3146-3155. DOI: 10.1016/j.jmrt.2022.12.145
[6]	CHAMBERS A R, EARL J S, SQUIRES C A, et al. The effect of voids on the flexural fatigue performance of unidirectional carbon fibre composites developed for wind turbine applications[J]. International Journal of Fatigue,2006,28(10):1389-1398. DOI: 10.1016/j.ijfatigue.2006.02.033
[7]	KOSMANN N, KARSTEN J M, SCHUETT M, et al. Determining the effect of voids in GFRP on the damage behaviour under compression loading using acoustic emission[J]. Composites Part B: Engineering,2015,70:184-188.
[8]	张阿樱. 孔隙率对碳纤维/环氧树脂层压板力学性能的影响[J]. 中国机械工程, 2010, 21(24):3014-3018. ZHANG A'ying. Experimental characterization of porosity and mechanical properties of carbon/epoxy composite laminates[J]. China Mechanical Engineering,2010,21(24):3014-3018(in Chinese).
[9]	张阿樱, 张东兴. 不同孔隙率 CFRP 层合板冲击后力学性能试验表征[J]. 材料科学与工艺, 2014, 46(3): 54-60. ZHANG A'ying, ZHANG Dongxing. Research on the static mechanical properties of CFRP laminates with different void contents[J]. Materials Science and Technology, 2014, 46(3): 54-60(in Chinese).
[10]	RICOTTA M, QUARESIMIN M, TALREJA R. Mode I strain energy release rate in composite laminates in the presence of voids[J]. Composites Science and Technology,2008,68(13):2616-2623. DOI: 10.1016/j.compscitech.2008.04.028
[11]	SUO Y Y, WANG B, JIA P R, et al. The effect of fabrication defects on the mechanical behaviors of metal matrix composites[J]. Materialstoday Communications,2022,25:101663. DOI: 10.1016/j.mtcomm.2020.101663
[12]	乔海燕, 任学冬, 史亦韦, 等. GH4169高温合金涡轮盘表面径轴向裂纹的渗透检测可行性[J]. 航空材料学报, 2016, 36(6):92-96. DOI: 10.11868/j.issn.1005-5053.2016.6.015 QIAO Haiyan, REN Xuedong, SHI Yiwei, et al. Feasibility of penetrant detection of radial and axial cracks on the surface of GH4169 superalloy turbine disk[J]. Journal of Aeronautical Materials,2016,36(6):92-96(in Chinese). DOI: 10.11868/j.issn.1005-5053.2016.6.015
[13]	李灼华. 射线检测在复合材料无损检测中的应用[J]. 科技传播, 2014, 6(3): 179, 175. LI Zhuohua. Application of radiographic testing in nondestructive testing of composite materials[J]. Science and Technology Communication, 2014, 6(3): 179, 175(in Chinese).
[14]	陈文玮. 超声检测技术在金属材料焊接成型中的应用[J]. 山西冶金, 2022, 45(3):264-265. DOI: 10.16525/j.cnki.cn14-1167/tf.2022.03.108 CHEN Wenwei. Application of ultrasonic testing technology in welding and forming of metal materials[J]. Shanxi Metallurgy,2022,45(3):264-265(in Chinese). DOI: 10.16525/j.cnki.cn14-1167/tf.2022.03.108
[15]	孟祥姝, 李武胜. 单向复合材料孔隙率测试技术与分布规律研究[J]. 高科技纤维与应用, 2021, 46(4):52-55. DOI: 10.3969/j.issn.1007-9815.2021.04.008 MENG Xiangshu, LI Wusheng. Research on porosity testing technology and distribution law of unidirectional composite materials[J]. High-tech Fiber and Application,2021,46(4):52-55(in Chinese). DOI: 10.3969/j.issn.1007-9815.2021.04.008
[16]	凌振宝, 邹得宝, 张堃, 等. 岩矿石孔隙度测量方法[J]. 吉林大学学报(地球科学版), 2011, 41(3):921-924. DOI: 10.13278/j.cnki.jjuese.2011.03.032 LING Zhenbao, ZOU Debao, ZHANG Kun, et al. Measuring method of rock ore porosity[J]. Journal of Jilin University (Earth Science Edition),2011,41(3):921-924(in Chinese). DOI: 10.13278/j.cnki.jjuese.2011.03.032
[17]	耿昆. SiCp/Al复合材料基于微细观的有限元建模拟实[D]. 上海: 上海交通大学, 2017. GENG Kun. Finite element modeling and simulation of SiCp/Al composites based on micro view[D]. Shanghai: Shanghai Jiao Tong University, 2017(in Chinese).
[18]	邱鑫. 挤压铸造SiCp/AZ91镁基复合材料的显微结构与性能[D]. 哈尔滨: 哈尔滨工业大学, 2006. QIU Xin. Microstructure and properties of squeeze cast SiCp/AZ91 magnesium matrix composites[D]. Harbin: Harbin Institute of Technology, 2006(in Chinese).
[19]	王涛. 高体积分数SiCp/Al复合材料高速铣削基础研究[D]. 北京: 北京理工大学, 2015. WANG Tao. Fundamental study on high speed milling of high volume fraction SiCp/Al composite[D]. Beijing: Beijing Institute of Technoloy, 2015(in Chinese).
[20]	TANG J M, DENG Q C. Experiment and simulation of SiC particle-reinforced aluminum matrix composites fabricated by friction stir processing[J]. The International Journal of Advanced Manufacturing Technology,2022,122(2):895-910. DOI: 10.1007/s00170-022-09967-7
[21]	张磊刚. SiCp/Mg 多级纳米复合材料的压缩断裂特性研究[D]. 成都: 西南交通大学, 2018. ZHANG Leigang. Research on compressive fracture properties of SiCp/Mg hierarchical nanocomposites[D]. Chengdu: Southwest Jiaotong University, 2018(in Chinese).
[22]	SU Y S, LI Z, YU Y, et al. Structural modeling and tensile simulation of graphene-reinforced metal matrix composites[J]. Science China Materials,2018,61(1):112-124. DOI: 10.1007/s40843-017-9142-2
[23]	梁超群, 尧军平, 李怡然, 等. SiC/AZ91D镁基复合材料单轴压缩过程中裂纹萌生扩展机制[J]. 复合材料学报, 2023, 40(7): 4282-4293. LIANG Chaoqun, YAO Junping, LI Yiran, et al. Mechanism of crack initiation and propagation during uniaxial compression of SiC/AZ91D magnesium matrix composites[J]. Acta Materiae Compositae Sinica, 2023, 40(7): 4282-4293(in Chinese).
[24]	夏聪聪. 裂纹尖端拘束对单边缺口拉伸试样裂纹扩展阻力曲线影响研究[D]. 天津: 天津大学, 2021. XIA Congcong. Research on the effect of crack tip constraint on the crack propagation resistance curve of unilateral notched tensile specimens[D]. Tianjin: Tianjin University, 2021(in Chinese).
[25]	ZHANG J, OUYANG Q B, GUO Q, et al. 3D microstructure-based finite element modeling of deformation and fracture of SiCp/Al composites[J]. Composites Science and Technology,2016,123:1-9. DOI: 10.1016/j.compscitech.2015.11.014
[26]	魏俊磊. 颗粒增强镁基复合材料力学行为的研究[D]. 太原: 太原理工大学, 2018. WEI Junlei. Study on mechanical behavior of particle reinforced magnesium matrix composites[D]. Taiyuan: Taiyuan University of Technology, 2018(in Chinese).
[27]	卢子兴. 复合材料界面的内聚力模型及其应用[J]. 固体力学学报, 2015, 36(S1):85-94. DOI: 10.19636/j.cnki.cjsm42-1250/o3.2015.s1.014 LU Zixing. The cohesion model of composite material interface and its application[J]. Journal of Solid Mechanics,2015,36(S1):85-94(in Chinese). DOI: 10.19636/j.cnki.cjsm42-1250/o3.2015.s1.014
[28]	翁琳. 基于微观结构的颗粒增强复合材料力学性能数值分析[D]. 上海: 上海交通大学, 2015. WENG Lin. Numerical analysis of mechanical properties of particle-reinforced composites based on microstructure[D]. Shanghai: Shanghai Jiao Tong University, 2015(in Chinese).
[29]	张炯, 屈展, 黄其青, 等. 基于内聚力模型的圆形夹杂与基体界面渐进脱粘分析[J]. 西安石油大学学报(自然科学版), 2014, 29(3):106-110, 12. ZHANG Jiong, QU Zhan, HUANG Qiqing, et al. Progressive debonding analysis of circular inclusion and matrix interface based on cohesion model[J]. Journal of Xi'an Shiyou University (Natural Science Edition),2014,29(3):106-110, 12(in Chinese).
[30]	周圣杰. B4C/2024Al复合材料变形断裂行为研究[D]. 哈尔滨: 哈尔滨工业大学, 2020. ZHOU Shengjie. Research on deformation and fracture behavior of B4C/2024Al composites[D]. Harbin: Harbin Institute of Technology, 2020(in Chinese).
[31]	周爽. 纳米增强体镁基复合材料力学性能数值模拟[D]. 沈阳: 沈阳工业大学, 2017. ZHOU Shuang. Numerical simulation of mechanical properties of nano-reinforced magnesium matrix composites[D]. Shenyang: Shenyang University of Technology, 2017(in Chinese).
[32]	JOHNSON G R, HOLMQUIST T J. An improved computational constitutive model for brittle materials[J]. AIP Conference Proceedings, 1994, 309(1): 981-984.
[33]	XIANG D H, SHI Z L, FENG H, et al. Finite element analysis of ultrasonic assisted milling of SiCp/Al composites[J]. The International Journal of Advanced Manufacturing Technology,2019,105:3477-3488.
[34]	张世强. 曲线回归的拟合优度指标的探讨[J]. 中国卫生统计, 2002, 19(1):9-11. DOI: 10.3969/j.issn.1002-3674.2002.01.003 ZHANG Shiqiang. Study on the goodness-of-fit in dex of curve regression[J]. China Health Statistics,2002,19(1):9-11(in Chinese). DOI: 10.3969/j.issn.1002-3674.2002.01.003

施引文献

资源附件(0)

长摘要

目的
SiC/AZ91D镁基复合材料广泛应用于航空制造，汽车生产，电子封装等领域，其在制造成型过程中不可避免会出现孔隙，孔隙的存在对复合材料力学性能产生不利影响，影响其损伤过程中的裂纹萌生和扩展。本文采用有限元分析方法建立含孔隙缺陷的真实SiC/AZ91D镁基复合材料的有限元模型，研究不同孔隙率及孔隙形状对SiC/AZ91D镁基复合材料裂纹萌生和扩展的影响。
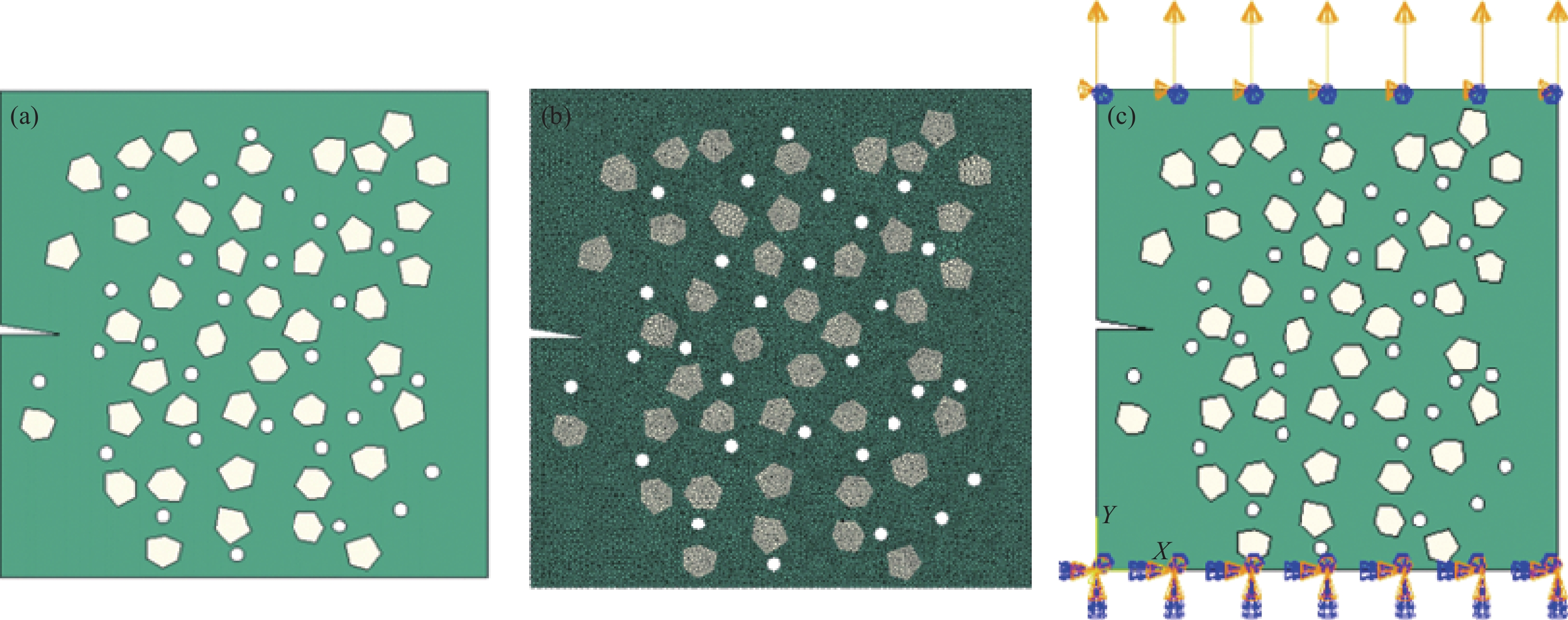
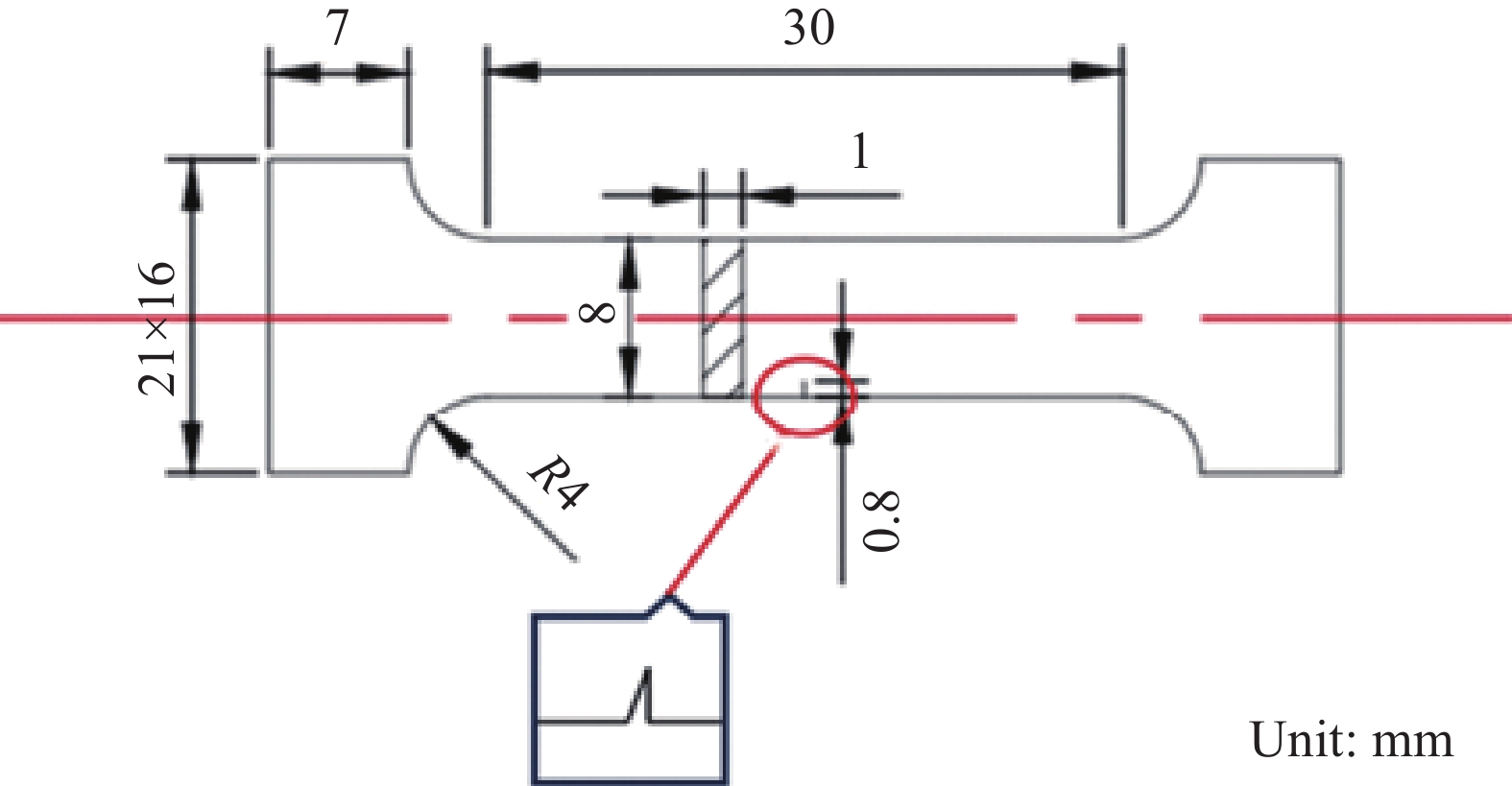
方法
首先在模型建立部分，根据SiC颗粒的SEM图像建立SiC颗粒的真实形貌模型，使用有限元分析软件Abaqus ，采用随机分布算法将颗粒与孔隙在基体模型中生成，得到不同孔隙含量以及不同孔隙形状的SiC/AZ91D镁基复合材料模型。其次在模拟加载部分，于颗粒与基体的界面处引入内聚力模型来模拟其在拉伸过程中裂纹萌生扩展的过程，内聚力单元的引入能够解决裂纹尖端存在奇异性的弊端；在模型上端施加拉伸位移载荷，下端为完全固定约束，侧向为自由边界条件。最后在实验验证部分采用带有预制裂纹的拉伸片试样，试样长度和宽度均远远大于其厚度，用来表征二维特征，实验加载过程中，试样的边界条件处于无约束状态，与模拟边界条件一致。
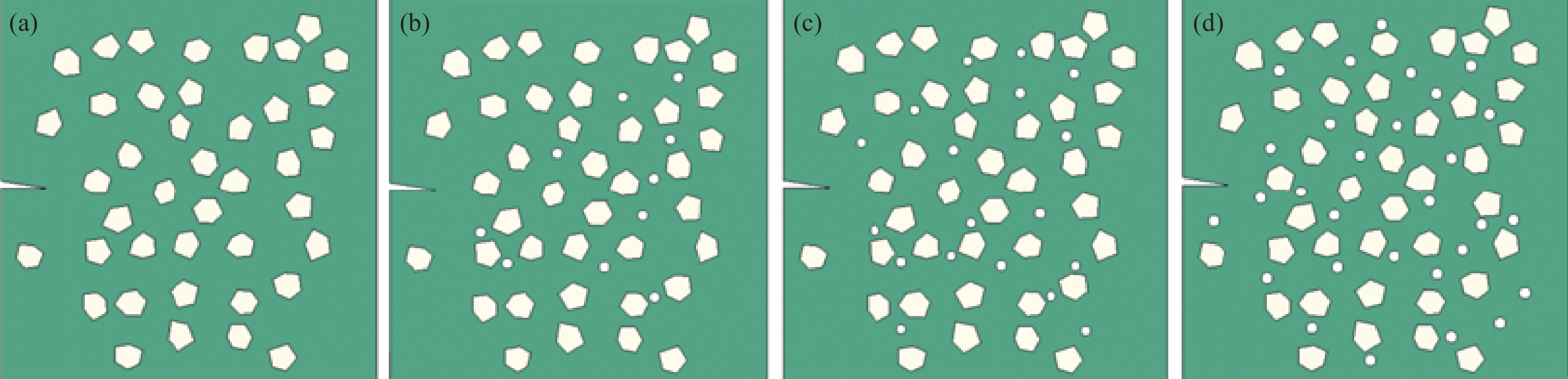
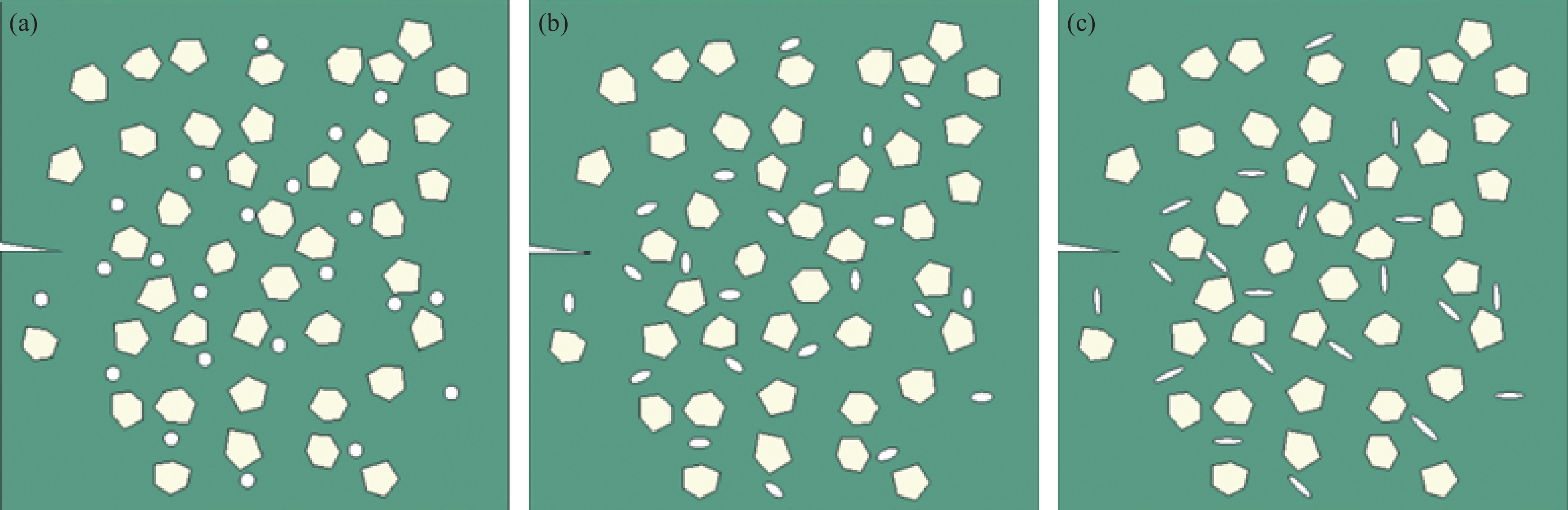
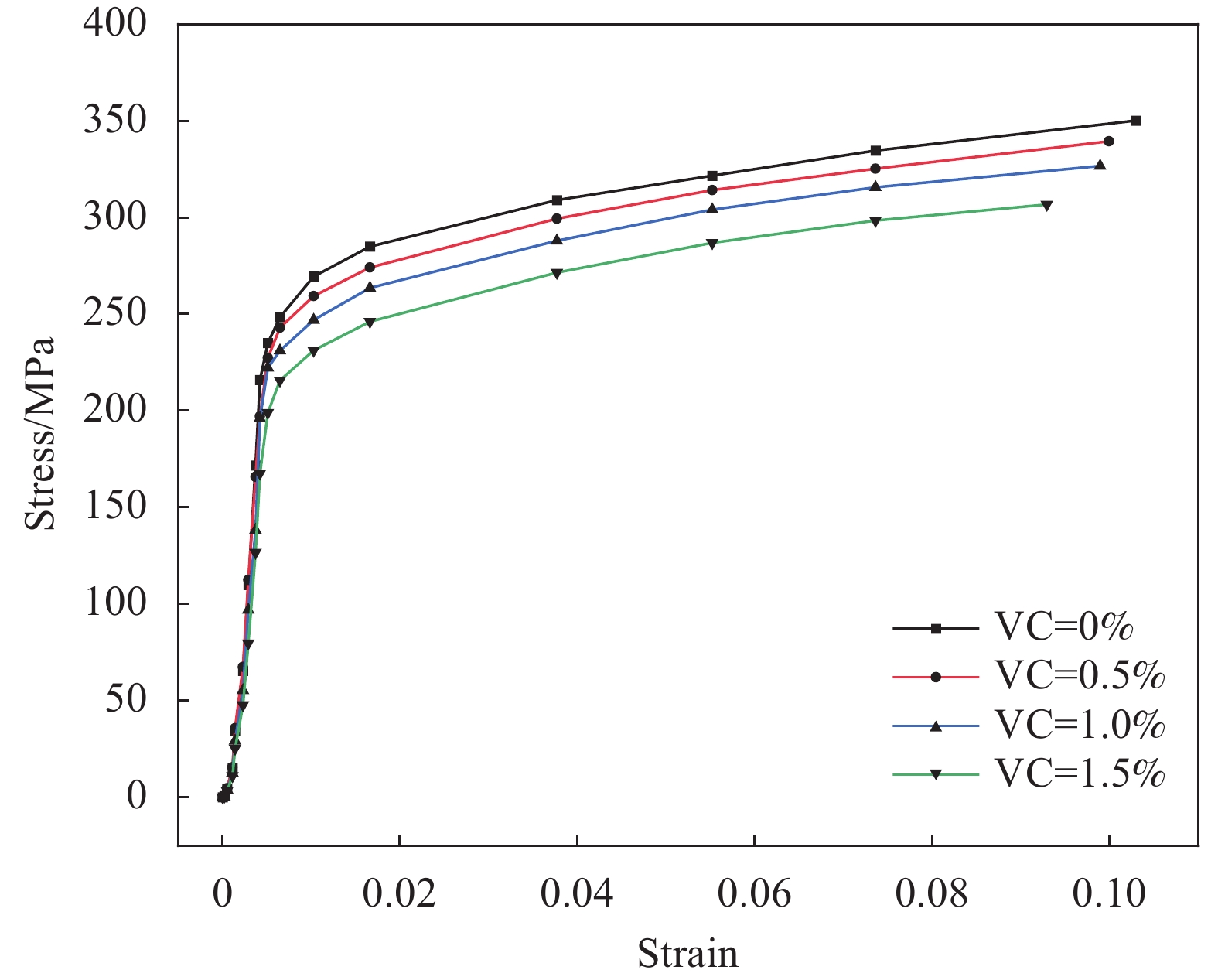
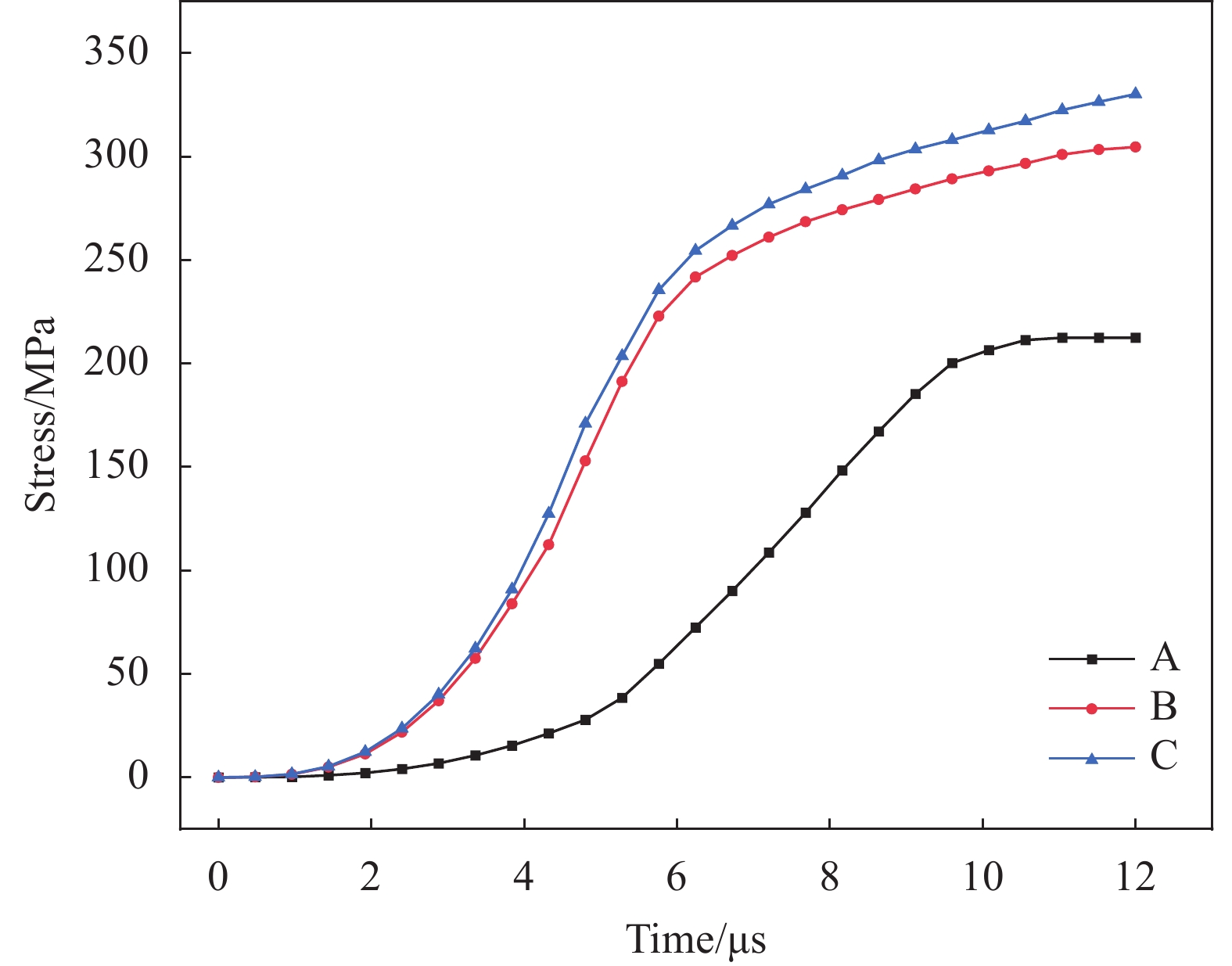
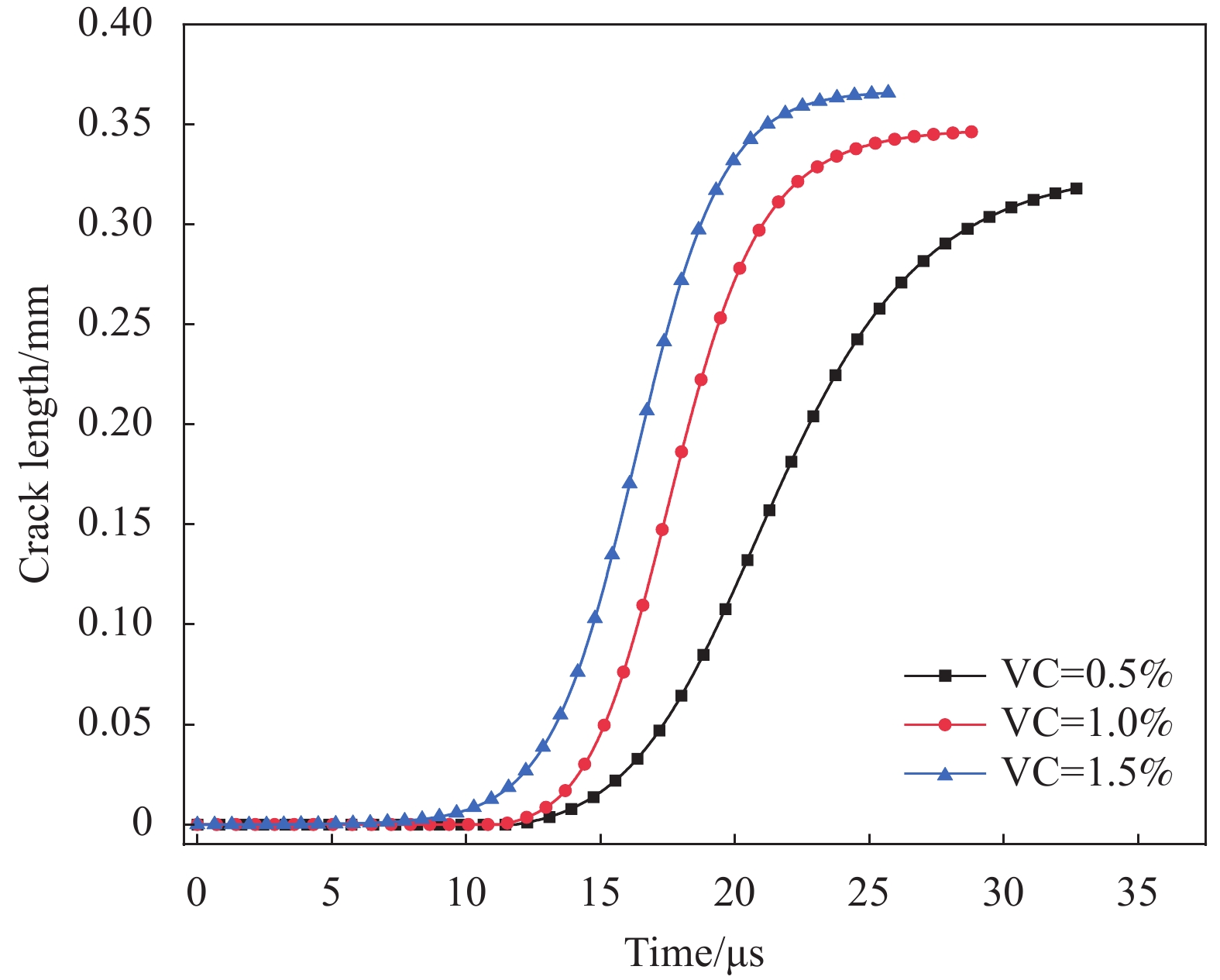
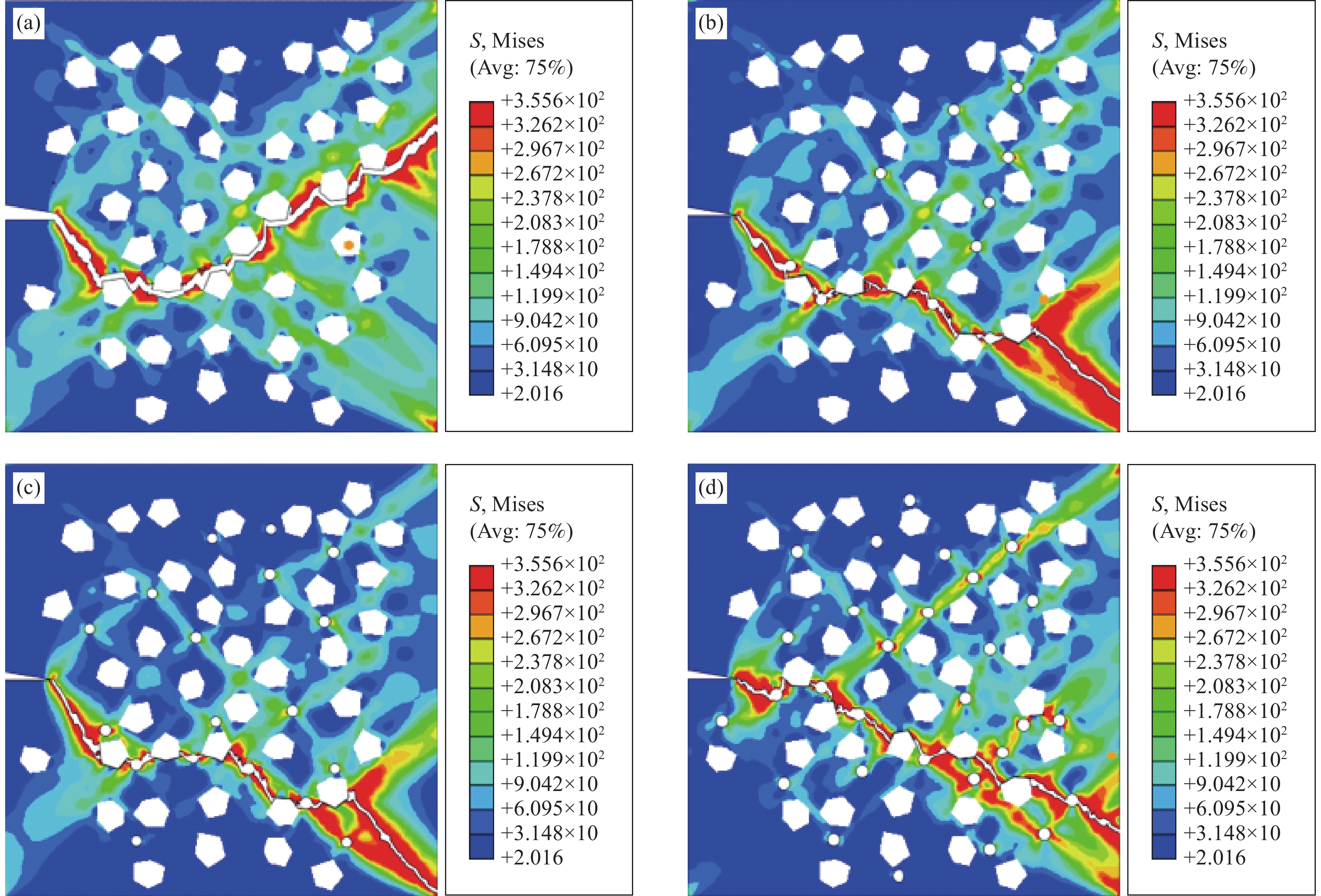
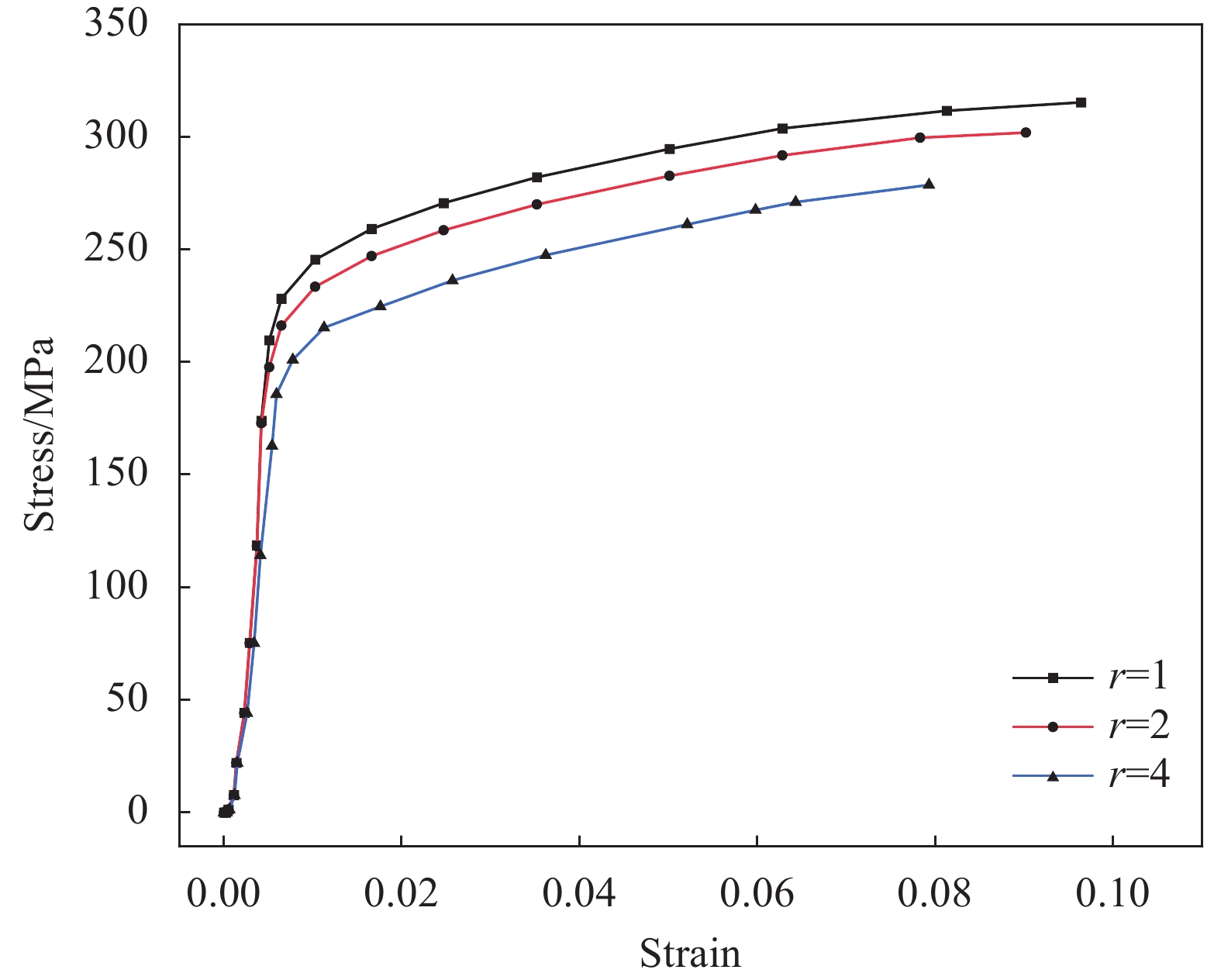
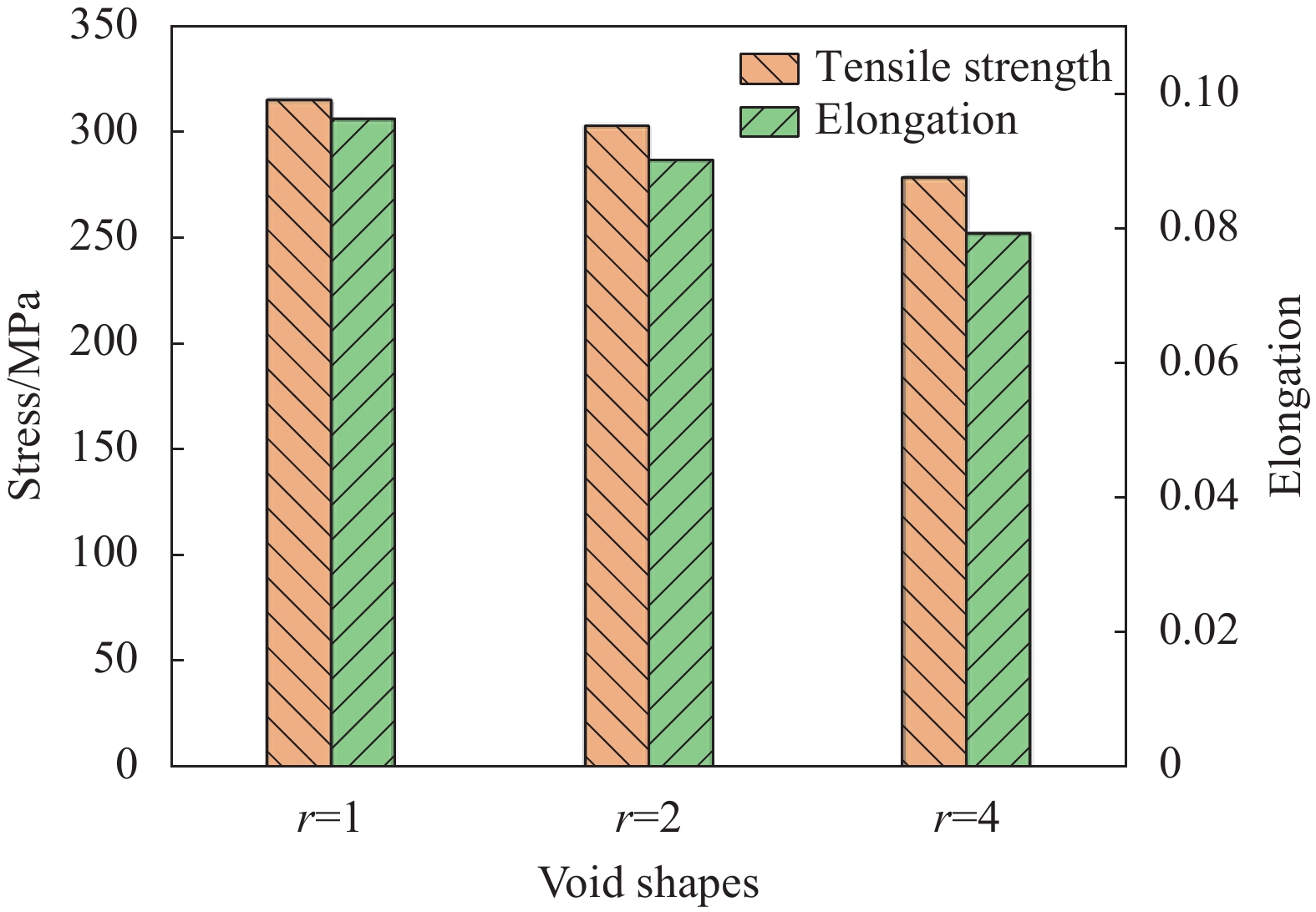
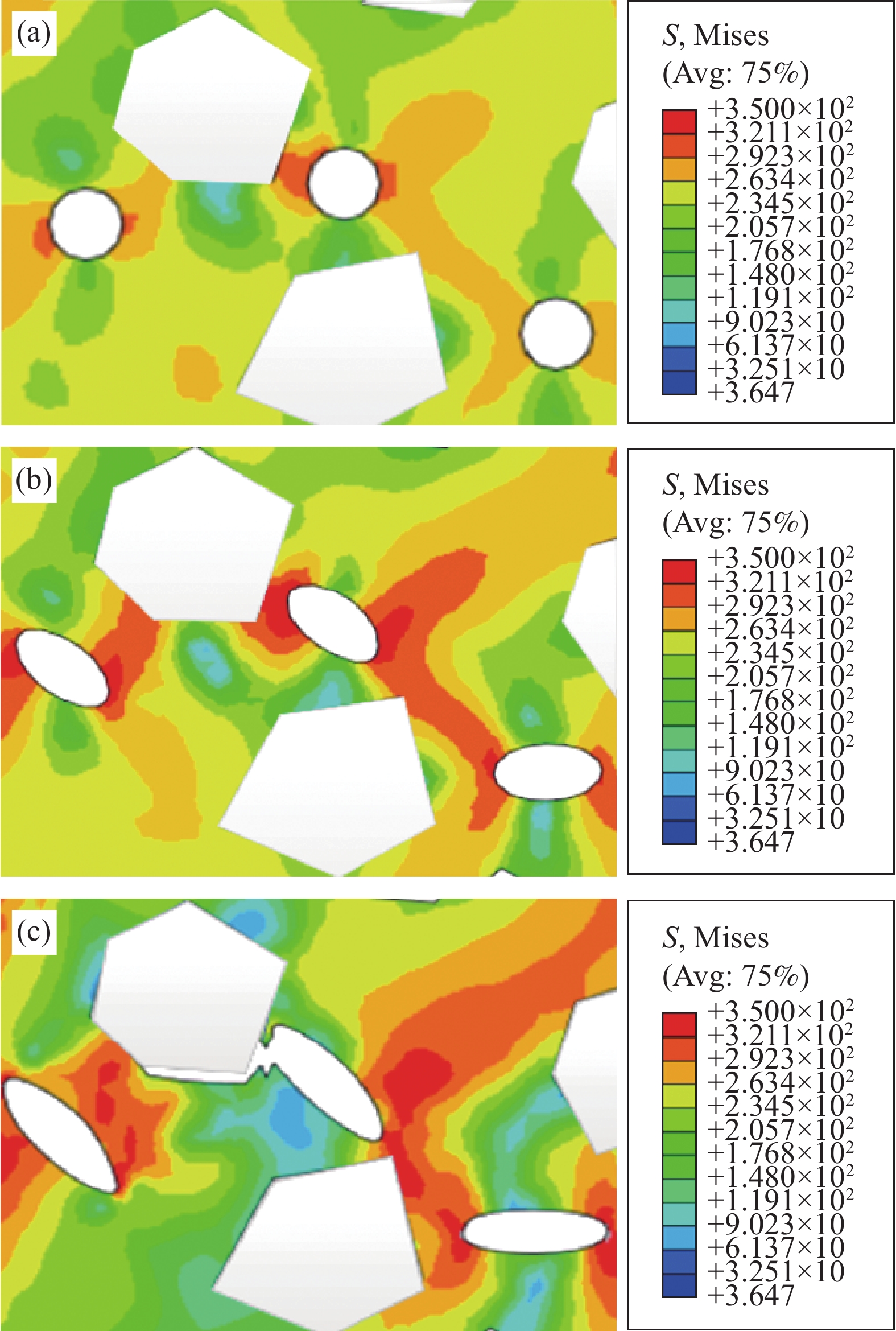
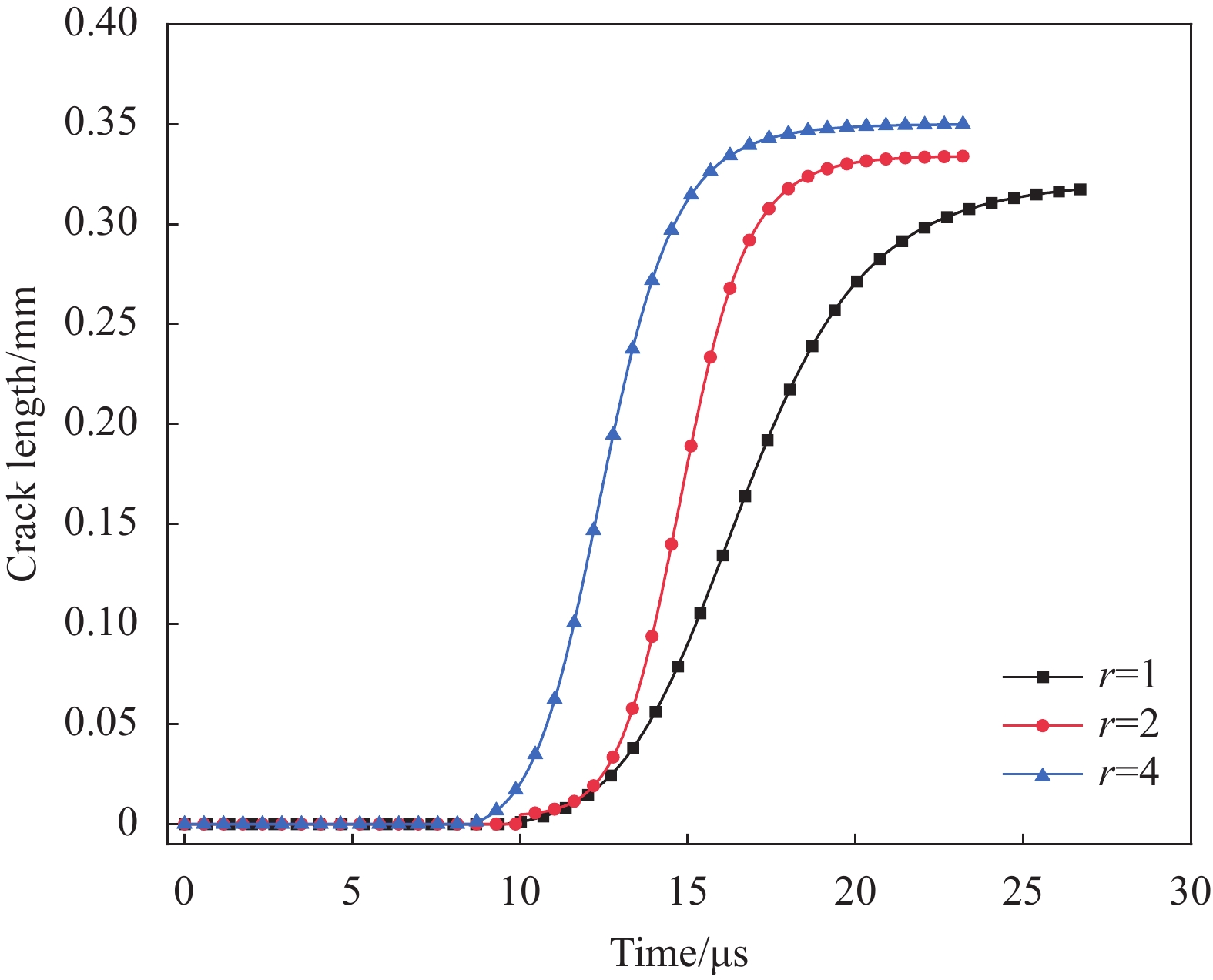
结果
从有限元模拟得出的结果分析可得出孔隙缺陷对复合材料整体力学性能影响，可分为以下几点：①不同孔隙率SiC/AZ91D复合材料性能各不相同，孔隙率越大，其屈服应力值，抗拉强度及伸长率越小；②含不同孔隙形状的SiC/AZ91D复合材料性能各不相同，孔隙长径比r越大，其抗拉强度及伸长率越小。孔隙缺陷对SiC/AZ91D复合材料裂纹萌生扩展的影响可分为以下几点：①孔隙存在会改变其所在区域的应力场分布，孔隙周围垂直于施载方向和与施载呈45°夹角方向的应力随时间增长较快，均远大于平行于施载方向的应力值，微裂纹易在此两处产生；②随着材料孔隙率的增加，裂纹萌生和断裂的时间均逐渐提前，孔隙率越大，其促进裂纹萌生和扩展作用越明显；③随着孔隙长径比r增大，裂纹萌生和断裂的时间均逐渐提前，孔隙长径比越大，其对裂纹萌生扩展的促进作用越明显；④含孔隙的SiC/AZ91D复合材料裂纹萌生扩展机制为微裂纹在孔隙周围萌生，与颗粒和基体交界处产生的微裂纹相互连接，汇集成主裂纹绕开颗粒扩展使材料断裂。从实验验证部分可得实验与模拟所得数据较为吻合，存在较小的偏差可能是由于模型中未考虑在复合材料微观组织存在缺陷造成的，根据实验与模拟结果所绘误差带图分析可知孔隙率1.5%的复合材料误差最大；孔隙率1.0%的复合材料误差次之；孔隙率0.5%的复合材料误差最小。
结论
通过内聚力模型方法，可对含不同孔隙的SiC/AZ91D复合材料进行细致的有限元分析，能够从模拟中得出复合材料裂纹萌生扩展的具体过程。不同孔隙率及孔隙形状对复合材料的影响各不相同，在选用过程中应优先选用孔隙含量较低的复合材料以减少孔隙缺陷对其造成的不利影响。

创新点

颗粒增强金属基复合材料广泛应用于航空制造，汽车生产，电子封装等领域，复合材料成型过程中不可避免会出现孔隙，孔隙的存在对复合材料力学性能产生不利影响，同时影响其损伤过程中的裂纹萌生和扩展。研究孔隙对复合材料力学行为影响的方法主要有实验和数值模拟。实验能够提供准确的数据，但需要大量重复实验，且受到随机因素的影响，存在误差和离散性，而数值方法具有可重复性和计算效率高等优点。
本文通过排水法测得复合材料孔隙率数据，并将其引入复合材料模型中。在模型中，使用基于真实形貌的SiC颗粒，在颗粒与界面处引入内聚力单元来模拟裂纹的萌生和扩展过程，以避免裂纹尖端出现奇异性。同时，采用适用于大变形和大断裂的JH2本构作为增强体SiC颗粒的本构模型。从图1可以观察到裂纹扩展路径，即孔隙周围的微裂纹与颗粒-基体交界处的微裂纹相互汇合形成主裂纹。图2中的模拟结果与实验测试结果进行对比，误差均在5%以内，验证了本文分析的可信度和可靠性。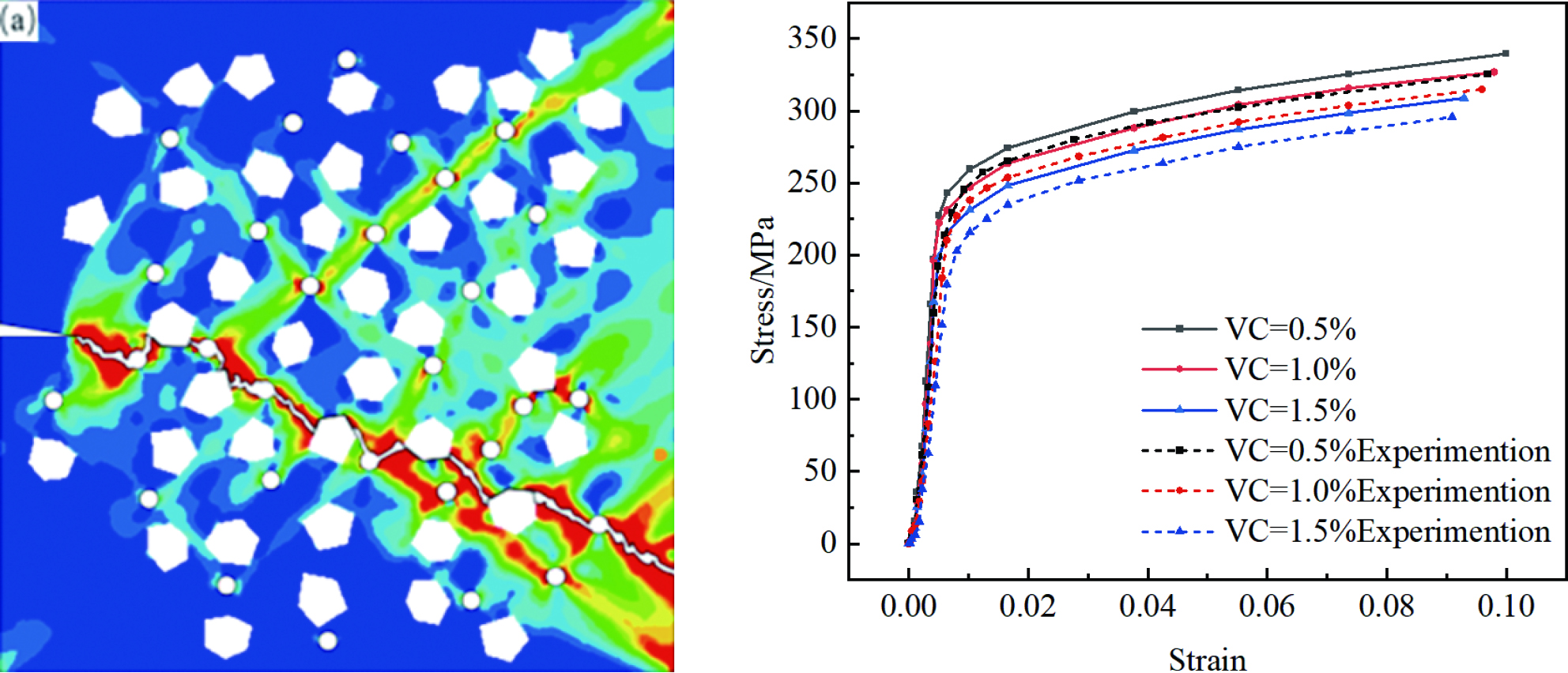
含孔隙的SiC/AZ91D复合材料在单轴拉伸过程中(a)裂纹路径(b)模拟结果与实验对比

图(22) / 表(5)

计量

文章访问数: 812
HTML全文浏览量: 384
PDF下载量: 58
被引次数: 0

1. 试验方法
1.1 原材料与制备
1.2 水分-载荷环境试验
1.3 拉伸失效试验
1.4 微观形貌表征
2. 结果与讨论
2.1 T300/H69编织复合材料的吸湿特性分析
2.2 T300/H69编织复合材料的剩余力学性能
2.2.1 载荷-位移分析
2.2.2 模量及强度分析
2.3 T300/H69编织复合材料的失效机制
2.3.1 宏观失效模式分析
2.3.2 微观形貌分析
2.4 T300/H69编织复合材料的剩余强度预测
3. 结论

1. 试验方法
1.1 原材料与制备
1.2 水分-载荷环境试验
1.3 拉伸失效试验
1.4 微观形貌表征
2. 结果与讨论
2.1 T300/H69编织复合材料的吸湿特性分析
2.2 T300/H69编织复合材料的剩余力学性能
2.2.1 载荷-位移分析
2.2.2 模量及强度分析
2.3 T300/H69编织复合材料的失效机制
2.3.1 宏观失效模式分析
2.3.2 微观形貌分析
2.4 T300/H69编织复合材料的剩余强度预测
3. 结论

参考文献(34)

施引文献

资源附件(0)

长摘要

创新点

SiC/AZ91D复合材料中孔隙缺陷对裂纹萌生和扩展行为的影响

通讯作者: 尧军平，博士，教授，硕士生导师，研究方向为金属基复合材料 E-mail: yyyjpsz@126.com

计量

出版历程

Effect of porosity defects on crack initiation and propagation behavior in SiC/AZ91D composites

1. 试验方法

1.1 原材料与制备

1.2 水分-载荷环境试验

1.3 拉伸失效试验

1.4 微观形貌表征

2. 结果与讨论

2.1 T300/H69编织复合材料的吸湿特性分析

2.2 T300/H69编织复合材料的剩余力学性能

2.2.1 载荷-位移分析

2.2.2 模量及强度分析

2.3 T300/H69编织复合材料的失效机制

2.3.1 宏观失效模式分析

2.3.2 微观形貌分析

2.4 T300/H69编织复合材料的剩余强度预测

3. 结 论

计量

出版历程

目录

1. 试验方法

1.1 原材料与制备

1.2 水分-载荷环境试验

1.3 拉伸失效试验

1.4 微观形貌表征

2. 结果与讨论

2.1 T300/H69编织复合材料的吸湿特性分析

2.2 T300/H69编织复合材料的剩余力学性能

2.2.1 载荷-位移分析

2.2.2 模量及强度分析

2.3 T300/H69编织复合材料的失效机制

2.3.1 宏观失效模式分析

2.3.2 微观形貌分析

2.4 T300/H69编织复合材料的剩余强度预测

3. 结 论

通讯作者:
尧军平，博士，教授，硕士生导师，研究方向为金属基复合材料　E-mail: yyyjpsz@126.com

3. 结论

3. 结论