基于单元级损伤指标的纤维增强复合材料层合板损伤识别方法

石庆贺; 杨颖; 孙伟; 王昊; 胡可军

doi:10.13801/j.cnki.fhclxb.20230710.002

基于单元级损伤指标的纤维增强复合材料层合板损伤识别方法

石庆贺^1, ,,
杨颖¹,
孙伟²,
王昊¹,
胡可军¹

1.
江苏理工学院　材料工程学院，常州 213001
2.
中国航空研究院，北京 100000

基金项目: 国家自然科学基金(12102156；52205157)；国家重点研发计划(2020YFB1506703)；江苏省(校)研究生实践创新计划项目(XSJCX23_61)

详细信息

通讯作者:
石庆贺，博士，讲师，硕士生导师，研究方向为结构健康监测、损伤识别、复合材料结构优化设计　E-mail: shiqinghe34@126.com

中图分类号: TB332
计量
- 文章访问数: 711
- HTML全文浏览量: 303
- PDF下载量: 35
出版历程
- 收稿日期: 2023-05-07
- 修回日期: 2023-06-16
- 录用日期: 2023-06-29
- 网络出版日期: 2023-07-10
- 刊出日期: 2024-02-29

Damage identification method for fiber-reinforced composite laminates based on element-level damage indicators

1.
School of Materials Engineering, Jiangsu University of Technology, Changzhou 213001, China
2.
China Academy of Aeronautical Research, Beijing 100000, China

Funds: National Natural Science Foundation of China (12102156; 52205157); National Key Research and Development Program (2020YFB1506703); Postgraduate Research & Practice Innovation Program of Jiangsu Province (XSJCX23_61)

摘要

摘要: 本文针对复合材料层合板结构提出了一种能够综合反映其承载能力缺失的单元级损伤指标−层合单元损伤指标，该指标既可以反映层合板面内外方向刚度的损伤情况，又具有参数数量较少、较易识别的优点。为保证所提损伤指标的合理性，本文利用数学和力学算子对单元级损伤指标与材料级损伤指标进行了等效，并比较了不同损伤指标在表征损伤程度之间的差异性。并提出了基于单元级损伤参数的复合材料结构损伤识别流程，即首先利用单元应变能差值指标对损伤单元进行筛选，然后利用优化方法对候选单元的损伤程度进行辨识。本文所提方法通过数值算例和一个试验进行了验证，分析了单元级损伤参数各个元素之间的相关性，并验证了基于单元级损伤参数的复合材料层合板结构损伤识别流程。本文的研究成果补充了现有复合材料结构健康监测理论。
- 复合材料 /
- 损伤识别 /
- 单元级损伤参数 /
- 层合板结构 /
- 优化 /
- 损伤定位
Abstract: An element-level damage index, damage index of laminated element, was proposed for composite laminate structures that can comprehensively reflect the degradation of load-bearing capacity, which can reflect the damage of stiffness in both internal and external directions and has the advantages of fewer parameters and easier identification. In order to ensure the rationality of the proposed damage index, mathematical and mechanical operators was used to equate the element-level damage index with the material-level damage index, and the differences between different damage indexes in characterizing the degree of damage were compared. The damage identification process of composite structures based on element-level damage parameters was also proposed, i.e., the damage elements were firstly screened by using modal strain energy change ratio, and then the damage degree of the candidate elements was identified by using optimization methods. The proposed method was validated by numerical examples and an experiment work, and the correlation between the elements of the element-level damage parameters was analyzed and the damage identification process of the composite laminate structure based on the element-level damage parameters was validated. The results of this paper complement the existing theory of health monitoring of composite structures.
- composite material /
- damage identification /
- element-level damage parameters /
- laminate structure /
- optimization /
- damage location

HTML全文

聚四氟乙烯(Polytetrafluoroethylene, PTFE)具有摩擦系数低、耐腐蚀和热稳定性优异等特点，是轴承、齿轮等传动结构件的理想基础材料，但是，纯PTFE的磨损率较高且机械强度低，往往需要填充纤维进行改性以提高其综合性能^[1]。

在多种PTFE的纤维填充相中，碳纤维(Carbon Fibre, CF)因其突出的轻质高强高弹模特性受到了广泛关注^[2]。但是，CF表面类石墨结构使其反应活性较低，很难与PTFE基体产生牢固的界面结合，因此需要对其进行表面处理以提高CF与PTFE之间的浸润性^[3]。近期研究发现，将纳米尺度的碳纳米管(Carbon Nanotube, CNT)粒子接枝于微米尺度的CF表面构建CF-CNT微纳多尺度增强体，可以提高纤维表面粗糙度与化学活性，改善CF与树脂基体的浸润性能^[4]。在相关研究中，化学接枝法因其成本低廉、能耗低的特点成为了CF-CNT微纳多尺度增强体的主要制备方法^{[4, 5]}。但是，化学接枝法主要基于剧烈氧化反应(强酸、强氧化剂等)，易损伤CF表面、降低纤维力学性能并产生环境污染^{[6, 7]}。而且，当前研究主要聚焦于CF-CNT多尺度增强体对树脂基复合材料力学性能的影响，而关于其对复合材料摩擦学性能影响的研究较少，尤其对PTFE基复合材料性能影响的研究还未见报道。

除此之外，在PTFE及其复合材料的摩擦学机制研究方面，已有研究对转移膜形成过程^[8-10]、摩擦化学反应^[11-13]等进行了深入探讨，但是，这些研究一般聚焦于摩擦试验结果(摩擦系数与磨损率)，而对摩擦过程各个阶段，尤其是与起始摩擦阶段密切相关的材料表面能的细致讨论较少。

针对以上问题，本研究采用不损伤纤维表面的稀土处理方法制备CF-CNT微纳多尺度增强体，将其用于增强PTFE基复合材料并研究了材料表面性能及往复摩擦学性能，随后探讨了多尺度增强体对复合材料摩擦过程各个阶段的影响机制、以及试验参数对材料摩擦学行为的影响。本研究提出的CF-CNT微纳多尺度增强体制备方法具有显著的工艺优势和强化效果：(1)增强体制备方法不损伤纤维表面且无污染，相对传统化学法更加温和环保；(2)CF-CNT多尺度增强PTFE基复合材料的磨损率相对纯PTFE降低75.4%，相比同类研究有较大提高。此外，研究中对于摩擦过程各个阶段尤其是起始摩擦阶段的分析也丰富了PTFE基复合材料摩擦学理论体系。

1. 实验材料及方法

1.1 实验材料

CNT(纯度99%、长度0.5~2 μm、直径30~50 nm)购自南京先丰纳米材料科技有限公司，CF(纯度99%、长度<75 μm、直径7 μm)购自沧州中丽新材料科技有限公司，乙醇(AR)和尿素(AR)购自国药化学试剂有限公司，LaCl₃(AR)购自上海展云化工有限公司，粒径15~260 μm的PTFE粉末，购自中昊晨光化工研究院有限公司。

1.2 多尺度增强体的合成

首先将CF浸入0.015 mol/L的LaCl₃-乙醇溶液中，搅拌1小时左右，在室温下放置约6小时，然后过滤并在100℃干燥6小时得到RCF。然后，将CNT超声分散到0.015 mol/L的LaCl₃-乙醇溶液中，并参考本团队已有研究结果向溶液中添加适量尿素^{[14, 15]}，再在室温静置约6小时。随后，将CNT过滤并在100℃下干燥6小时以获得RCNT。CNT与RCNT的沉降实验结果如图1所示，可见RCNT在乙醇中的分散性显著提高，这是由于其表面吸附有较多尿素分子^[15]。接着将RCF涂布在玻璃基板上，再将RCNT超声分散在乙醇中得到浓度为0.4 g/L的分散液，使用滴管将分散液逐滴滴蘸到RCF表面，然后将所得混合物在100℃加热6小时。最后，将干燥后的样品在乙醇中超声清洗以去除多余的RCNT，并在60℃的真空烘箱中干燥12小时，得到RCF-RCNT多尺度增强体。为揭示RCF-RCNT多尺度增强体对复合材料的强化机制，本研究还使用CF和CNT，根据RCF-RCNT增强体的滴蘸制备方法制备了CF-CNT样品，并且，为保留CF表面的CNT，CF-CNT样品未经过超声清洗。

图 1 超声30 min所得浓度为1.0 g/L的分散系静置不同时刻的沉降结果对比图：碳纳米管(CNT)-乙醇分散系静置1 h (a)及24 h (b)，RCNT-乙醇分散系静置1 h (c)及24 h (d)

Figure 1. Comparison of the sedimentation results of the dispersion system with a concentration of 1.0 g/L obtained by ultrasonicating for 30 min at different times: Carbon nanotube (CNT)-ethanol dispersion system kept still for 1 h (a) and 24 h (b), RCNT-ethanol dispersion system kept still for 1 h (c) and 24 h (d)

下载: 全尺寸图片幻灯片

1.3 PTFE基复合材料的制备

首先，将增强体以5wt.%的比例与PTFE粉料进行机械共混。然后，将混合粉料倒入金属模具中，缓慢加压至35 MPa，保持5分钟，然后缓慢卸掉压力，取出压制成型的坯料。接着，将坯料放入烘箱中，以约60℃/小时的速率升温到370℃并保持1小时进行烧结，然后停止加热，冷却至室温后取出试件。最后，通过机械加工将试件制备成测试所需试样形状。本研究中，以CF、RCF、CF-CNT、RCF-RCNT为填充相制得的复合材料分别记为CF/PTFE、RCF/PTFE、CF-CNT/PTFE、RCF-RCNT/PTFE。

1.4 表征与测试

使用FEI Sirion 200扫描电子显微镜(Scanning Electron Microscope, SEM)观察样品微观形貌，使用532 nm Ar激光源的Renishaw RM2000拉曼光谱仪进行拉曼光谱分析，使用德清盛泰芯电子科技有限公司生成的数显邵氏硬度计测量复合材料硬度，使用Rigaku Ultima IV型X射线衍射仪(Cu Kα辐射，λ = 0.154 nm，扫描步长0.02°)表征复合材料晶体结构，使用KRUSS DSA100液滴形状分析系统测量复合材料对水和二碘甲烷的接触角。使用兰州中科凯华公司生产的HSR-2M型往复摩擦磨损试验机，测量复合材料的摩擦系数及体积磨损率，对磨球材质为GCr15、直径6 mm，往复行程5 mm，摩擦时间为30分钟，实验时环境温度19~21℃，湿度42%~66%。使用Keyence VHX-500F数码显微镜观察摩擦后的对磨球表面形貌。

2. 结果与讨论

2.1 多尺度增强体表征

图2(a, b)为RCF-RCNT多尺度增强体微观形貌，可见RCNT在纤维表面均匀分布，形成了多尺度结构。图2(c)为三种样品的拉曼光谱，其中，1590 cm⁻¹(G波段)和1350 cm⁻¹(D波段)附近的吸收峰分别代表sp²杂化碳的面内振动和不定形碳，两吸收峰强度比I_D/I_G通常用于衡量CF表面石墨结构的无序程度，其值越高代表不定形碳越多^[16]。本研究采用Gaussian-Lorentzian拟合计算I_D/I_G数值^{[17, 18]}，结果表明CF的I_D/I_G=1.84，与已有研究相近^{[18, 19]}。一般认为，制备CF-CNT多尺度增强体的传统化学接枝法中，对CF采用的高温、强酸、电化学氧化改性方法会破坏CF表面石墨结构，引入无定形碳，从而使I_D/I_G数值升高^{[20, 21]}。然而，本研究中RCF的I_D/I_G=1.83，略小于CF中的值，这表明LaCl₃-乙醇处理不会引起明显的纤维表面损伤，同时，本研究中多尺度增强体的合成主要采用低毒低污染的LaCl₃、尿素和乙醇^[22]，并不涉及强酸强氧化剂等有害物质的使用，因此相对传统化学法具有绿色环保的工艺优势。此外，在RCF-RCNT多尺度增强体中I_D/I_G=1.61，这是由于增强体中含有石墨化程度更高的CNT^{[23, 24]}。

图 2 (a, b) RCF-RCNT多尺度增强体形貌；(c) CF、RCF、RCF-RCNT的拉曼光谱

Figure 2. (a, b) Morphology of RCF-RCNT multiscale reinforcer; (c) Raman spectra of CF, RCF and RCF-RCNT.

下载: 全尺寸图片幻灯片

2.2 复合材料表面性能分析

2.2.1 硬度及晶体结构

本研究中PTFE复合材料的硬度如表1所示，可见，硬质的CF和CF-CNT填充相的加入使复合材料硬度明显提高，且提高程度相当。但是，RCF、RCF-RCNT填充相的加入使复合材料的硬度相对CF/PTFE和CF-CNT/PTFE进一步增加，这表明RCF/PTFE和RCF-RCNT/PTFE中可能出现了其他硬度较高的新相。因此，本研究对复合材料的晶体结构进行了分析。

表 1 不同复合材料的硬度

Table 1. Hardness of different composites

Sample	Hardness (Shore D)
PTFE	57.0±2.9
CF/PTFE	61.7±1.5
RCF/PTFE	62.3±0.6
CF-CNT/PTFE	61.3±1.4
RCF-RCNT/PTFE	62.7±0.2

下载: 导出CSV

| 显示表格

图3(a)为不同复合材料的XRD光谱，2θ=18°、32°、37°、41°附近的衍射峰分别对应PTFE晶体中的(100)、(110)、(200)、(108)晶面^[25]。图3(b)中2θ=16°附近的驼峰对应PTFE中的无定形晶粒，根据该驼峰强度和2θ=18.14°附近衍射主峰强度可计算得到复合材料结晶度 ${X}_{\mathrm{c}}$ ，本研究采用文献^[26]所述方法计算结晶度，所得结果如图3(a)所示。可见，CF和CF-CNT填充相的加入使PTFE结晶度从35%上升到38%和41%，这是由CF及CNT的加入使液态PTFE形核结晶的位点增加，发生多点形核所致^[27]。RCF、RCF-RCNT增强体的加入使结晶度进一步提高到44%，这是由于增强体合成过程中在表面吸附了La(III)，而La(III)的络合能力较强，在PTFE结晶过程中具有成核剂的作用，这使成核率提高、结晶速率加快，因此结晶度明显增大^{[28, 29]}。除此之外，由图3(c)可见，CF/PTFE (17.94°)及RCF/PTFE (17.94°)的主衍射峰相对纯PTFE (18.14°)明显左移，这是由于纤维增强体的加入在基体中产生了较大的内应力，引起晶格膨胀晶面间距增大，而CF-CNT和RCF-RCNT的加入使衍射峰分别右移至18.10°和18.30°，这是因为纳米级CNT粒子插入PTFE分子链，进而引起晶格收缩使晶面间距减小^{[25, 30]}。

图 3 不同复合材料的X射线衍射光谱及结晶度

${X}_{\mathrm{c}}$ (a)及2θ=16° (b),18.14° (c)处的放大图

Figure 3. XRD pattern and crystallinity

${X}_{\mathrm{c}}$ of different composites (a) and enlarged view at 2θ=16° (b) and 18.14° (c)

下载: 全尺寸图片幻灯片

综上所述，填充相使PTFE复合材料硬度提高的原因有二，其一是碳材料本身为硬质相，其二是填充相的加入提高了复合材料结晶度，因此CF和CF-CNT的加入使复合材料硬度变大，而RCF和RCF-RCNT的加入使硬度进一步提高。

2.2.2 接触角与表面能

材料表面能与其摩擦磨损性能，尤其是初始摩擦阶段的摩擦学行为密切相关，但是，当前研究对纤维增强复合材料的表面性能研究较少，针对这一问题，本研究采用Fowkes-Owens-Wendt-Kaelble模型^[31-33]计算复合材料的表面能，在该模型中，复合材料表面能 ${\gamma }_{s}$ 由下式给出

${\gamma }_{s}={\gamma }_{s}^{p}+{\gamma }_{s}^{d}$

(1)

${\gamma }_{l}(1+\mathrm{cos}\theta )=2\left(\sqrt{{\gamma }_{l}^{p}{\gamma }_{s}^{p}}+\sqrt{{\gamma }_{l}^{d}{\gamma }_{s}^{d}}\right)$

(2)

其中，

${\gamma }^{p}$ 由分子极性引起的吸引力

${\gamma }^{d}$ 范德瓦尔斯力等其他力的和

$\theta$ 接触角

$l$ 接触角测量时所用的液相

$s$ 接触角测量时所用的复合材料

本研究中采用蒸馏水和二碘甲烷测量复合材料接触角，所得结果如表2所示。

表 2 不同复合材料的接触角

Table 2. Contact angle of different composites

Sample	Contact angle/(°)
Sample	Water	Diiodomethane
PTFE	109.0±4.4	78.1±5.9
CF/PTFE	120.8±0.5	76.4±1.8
RCF/PTFE	118.4±2.9	79.0±1.3
CF-CNT/PTFE	119.1±2.5	75.8±2.1
RCF-RCNT/PTFE	110.2±4.4	78.4±0.6

下载: 导出CSV

| 显示表格

两种不同液相的表面能 ${\gamma }_{l}$ 及分量 ${\gamma }_{l}^{p}$ 、 ${\gamma }_{l}^{d}$ 由参考文献^[34]给出，如表3所示。

表 3 所用液相的表面能

Table 3. Surface tension of the used test liquid

Wetting liquids	Surface tension ${\gamma }_{l}$ /(mJ·m⁻²)	Dispersion component ${\gamma }_{l}^{d}$ /(mJ·m⁻²)	Polar component ${\gamma }_{l}^{p}$ /(mJ·m⁻²)
Water	72.8	21.8	51.0
Diiodomethane	50.8	48.5	2.3

下载: 导出CSV

| 显示表格

将测量所得接触角及液滴参数代入式(1~2)计算得到的复合材料表面能如图4所示，可见CF和CF-CNT的加入使复合材料的表面能逐渐升高，这是由于CF与CNT的表面能相对PTFE较高^{[35, 36]}。而RCF和RCF-RCNT的加入使复合材料表面能相对CF/PTFE和CF-CNT/PTFE明显降低，这是由于RCF/PTFE和RCF-RCNT/PTFE的结晶度较高，因此表面具有较多的惰性PTFE晶体结构。一般而言，复合材料表面能越低，在摩擦过程中与对磨件表面之间的黏着作用越弱、摩擦阻力越小。RCF-RCNT的加入大大降低了PTFE复合材料表面能，这将对PTFE复合材料产生显著的减摩降磨作用。

图 4 不同复合材料的表面能

Figure 4. Surface energy of different composites

下载: 全尺寸图片幻灯片

2.3 复合材料的摩擦学性能

2.3.1 多尺度增强PTFE复合材料的摩擦学性能

一起始摩擦系数分析

不同复合材料的摩擦系数实时变化曲线图如图5所示。由图5(a)可知，PTFE、CF/PTFE、RCF/PTFE、CF-CNT/PTFE、RCF-RCNT/PTFE的起始摩擦系数分别为0.096、0.200、0.162、0.206、0.090。

图 5 不同复合材料的摩擦系数实时变化曲线图：(a) 2 min前(放大图)；(b) 2 min后(往复频率6 Hz，载荷16 N)

Figure 5. Real-time coefficient of friction curve of different composites: (a) magnified view before 2 min; (b) after 2 min (reciprocating frequency: 6 Hz, load: 16 N)

下载: 全尺寸图片幻灯片

在无润滑条件下，材料起始摩擦系数与摩擦副表面能密切相关，一般认为，较低的表面能意味着两摩擦副之间相对较弱的黏着作用以及较小的起始摩擦系数^[37]，Sinha等^{[37, 38]}在Bradly^[39]和Tabor^[40]的研究基础上进一步提出了高分子薄膜起始摩擦系数与摩擦副表面能之间的下述关系：

${\mu }_{\mathrm{i}}=\frac{{C}_{1}{C}_{2}\mathrm{exp}\left(n{F}_{\mathrm{o}}\right)}{{F}^{0.25}}+\alpha$

(3)

${\mathrm{F}}_{\mathrm{o}}=2{\text{π}} R({\gamma }_{1}+{\gamma }_{2}-{\gamma }_{12})$

(4)

${\gamma }_{12}={\left(\sqrt{{\gamma }_{1}}-\sqrt{{\gamma }_{2}}\right)}^{2}$

(5)

其中，

${\mu }_{\mathrm{i}}$ 起始摩擦系数

F 载荷

${C}_{1},{C}_{2},\alpha ,n$ 常数

${F}_{\mathrm{o}}$ 牵引力

$R$ 圆形接触区域的半径

${\gamma }_{1},{\gamma }_{2}$ 两摩擦副材料表面能

${\gamma }_{12}$ 两摩擦副材料之间的界面能

将式(4~5)代入式(3)，有

${\mu }_{\mathrm{i}}=\frac{{C}_{1}{C}_{2}\mathrm{exp}\left(4{\text{π}} nR\sqrt{{\gamma }_{1}{\gamma }_{2}}\right)}{{F}^{0.25}}+\alpha$

(6)

由式(6)可知，起始摩擦系数 ${\mu }_{\mathrm{i}}$ 与两摩擦副表面能乘积 ${\gamma }_{1}{\gamma }_{2}$ 大致成正相关关系。本研究中对磨球均为同种材料， ${\gamma }_{2}$ 可视为不变，而根据前文表面能测试结果知复合材料表面能大小顺序为：γ(CF-CNT/PTFE) ≈ γ(CF/PTFE) > γ(RCF/PTFE) > γ(PTFE) ≈ γ(RCF-RCNT/PTFE)，这正与五种材料的起始摩擦系数大小顺序相同。

二摩擦学性能对比

由图5(b)可见，纯PTFE的摩擦系数在摩擦过程中先缓慢增加至0.17左右，在第20 min时突然升高至0.21左右，其平均摩擦系数为0.16。加入CF后，复合材料的摩擦系数先是缓慢升高到0.29左右，在第13.5 min后突然降为0.22左右，维持7.5 min后，在第21 min时又突然升高到0.29左右，而后在第28 min时再次出现相似的突变。与CF/PTFE相似的是，CF-CNT/PTFE复合材料的摩擦曲线也是先缓慢升高到0.3左右，而后在第6 min突然降低，但是此后一直维持在0.22左右不再发生突变。与PTFE、CF/PTFE、CF-CNT/PTFE三种材料不同的是，RCF/PTFE与RCF-RCNT/PTFE的摩擦系数始终未发生突变，其摩擦曲线经历前期的磨合后很快稳定，且RCF/PTFE达到稳定的时间较短。就整个摩擦过程而言，复合材料的平均摩擦系数及磨损率如图6所示。

图 6 不同复合材料的摩擦系数及磨损率

Figure 6. Coefficient of friction and wear rate of different composites

下载: 全尺寸图片幻灯片

由图6知，RCF-RCNT/PTFE复合材料的磨损率为79.0×10⁻⁶ mm³·N⁻¹·m⁻¹，相对于纯PTFE降低了75.4%。这一降磨效果与同类研究的对比如表4所示，可见，本研究中的降磨效果优于同类研究。

为揭示复合材料摩擦磨损机制，本研究对摩擦表面微观形貌进行了分析。

表 4 相关研究中PTFE基复合材料摩擦学性能对比

Table 4. Tribological performance comparison of PTFE based composites in similar studies

Ref.	Composites	Type of friction	Test conditions	Wear rate / (×10⁻⁶ mm³·N⁻¹·m⁻¹)	Decrement of wear rate/%
[41]	PTFE	Reciprocating sliding	Counterface: φ=10 mm steel ball, load: 10 N, frequency: 10 Hz	304.8	−48.1
[41]	30vol.% h-BN/AO/PTFE	Reciprocating sliding		158.2	−48.1
[42]	PTFE/AP	Reciprocating sliding	Counterface: φ=9 mm Si₃N₄ ball, load: 10 N, frequency: 1 Hz	3.2	−44.1
[42]	6wt.% modified CF/PTFE/AP	Reciprocating sliding	Counterface: φ=9 mm Si₃N₄ ball, load: 10 N, frequency: 1 Hz	1.79	−44.1
[43]	PTFE	Reciprocating sliding	Counterface: φ=5 mm Si₃N₄ ball, load: 3 N, frequency: 2 Hz	2.5	−44.0
[43]	5wt.% CF/5wt.%BP/PTFE	Reciprocating sliding	Counterface: φ=5 mm Si₃N₄ ball, load: 3 N, frequency: 2 Hz	1.4	−44.0
[2]	PTFE	Continuous sliding	Counterface: φ=6 mm steel ball, load: 10 N, rotational speed: 200 r/min	390	−29.7
[2]	20wt.%CF/PTFE	Continuous sliding		274	−29.7
[1]	PTFE	Reciprocating sliding	Counterface: 5×5×25 mm steel pin, load: 50 N, frequency: 2 Hz	0.21	—
	15wt.% AF/PTFE			0.23	+9.5
	15wt.% CF/PTFE			0.24	+14.3
	15wt.% GF/PTFE			0.18	−14.3
	0.5wt.% BF/PTFE			0.15	−28.6
	0.5wt.% BF+ 10wt.%MoS₂/PTFE			0.17	−19.1
[20]	PI	Reciprocating sliding	Counterface: φ=4 mm steel ball, load: 4.5 N, velocity: 0.083 m/s	6.44	−72.2
[20]	5wt.% CF-CNT reinforcer/PI	Reciprocating sliding		1.79	−72.2
This study	PTFE	Reciprocating sliding	Counterface: φ=6 mm steel ball, load: 16 N, frequency: 6 Hz	321.6	−75.4
This study	5wt.% RCF-RCNT reinforcer/PTFE	Reciprocating sliding	Counterface: φ=6 mm steel ball, load: 16 N, frequency: 6 Hz	79.0	−75.4
Notes: h-BN—hexagonal boron nitride; AO—alumina particles; AP—aluminum dihydrogen phosphate; BP—black phosphorus; AF—aramid fiber; GF—glass fiber; BF—basalt fiber; PI—polyimide.

下载: 导出CSV

| 显示表格

三磨损面形貌分析

图7为复合材料的磨损面典型形貌。如图7(a)所示，低倍率下的纯PTFE的磨损面较为光滑，仅有些许细小的碎屑，光滑的表面意味着较低的摩擦阻力，这与纯PTFE较低的摩擦系数相符。相比之下，CF/PTFE及CF-CNT/PTFE的磨损面显得较为粗糙。CF/PTFE中出现了大块磨屑，如图7(d)所示，在高倍率下可见CF与基体部分脱粘的现象，如图7(e, f)所示，这表明二者间结合性能较差。CF-CNT/PTFE的磨损面出现了因CF拔出而留下的凹坑，如图7(j)所示，在高倍率下可见部分拔出后裸露的CF，但其并未与基体脱粘，这表明纤维与基体结合性能有一定提高。CF/PTFE及CF-CNT/PTFE中，脱粘后裸露、甚至脱落的硬质CF，在摩擦过程中容易对基体形成犁削，加剧材料磨损。相比之下，RCF/PTFE及RCF-RCNT/PTFE的磨损面较为光滑，视野中未见大块磨粒及CF脱落形成的凹坑。高倍率下可见RCF/PTFE中CF与基体结合紧密，基本未出现脱粘现象，如图7(h)所示，而RCF-RCNT/PTFE中CF则牢固镶嵌于基体中，如图7(n, o)所示，这都表明了纤维与基体之间良好的结合性能。

图 7 复合材料磨损面典型形貌：(a~c) PTFE, (d~f) CF/PTFE, (g~i) RCF/PTFE, (j~l) CF-CNT/PTFE, (m~o) RCF-RCNT/PTFE

Figure 7. Typical worn surface morphology of composites: (a~c) PTFE, (d~f) CF/PTFE, (g~i) RCF/PTFE, (j~l) CF-CNT/PTFE, (m~o) RCF-RCNT/PTFE

下载: 全尺寸图片幻灯片

图8为复合材料对磨球的典型表面形貌。在图8(a)中，纯PTFE的对磨球表面可见由PTFE黏着形成的转移膜，且犁沟并不明显，这表明其磨损机制主要为黏着磨损。其余四种复合材料对磨球照片中，转移膜表面均出现了明显的犁沟，犁沟主要由摩擦过程中从基体脱落的磨屑尤其是硬质纤维刮擦所致，这表明四种复合材料的磨损机制包括黏着磨损及磨料磨损。此外，从图8(b)可见CF/PTFE中的犁沟较宽且较深，这是由于CF/PTFE中产生了大块磨粒及纤维脱粘现象严重，CF-CNT/PTFE和RCF/PTFE中的犁沟相对较浅，RCF-RCNT/PTFE的犁沟最浅最窄，这也与复合材料磨损率变化趋势相符。

图 8 复合材料对磨球典型表面形貌：(a) PTFE, (b) CF/PTFE, (c) RCF/PTFE, (d) CF-CNT/PTFE, (e) RCF-RCNT/PTFE

Figure 8. Typical GCr15 ball surface morphology of (a) PTFE, (b) CF/PTFE, (c) RCF/PTFE, (d) CF-CNT/PTFE, (e) RCF-RCNT/PTFE after tribological test

下载: 全尺寸图片幻灯片

四摩擦磨损机制研究

基于以上分析，本研究提出以下的摩擦磨损机制：即五种复合材料的摩擦过程可分为(1)表面粘附阶段、(2)表面变形阶段、(3)转移膜形成阶段，由于CF/PTFE及CF-CNT/PTFE复合材料的对磨球中形成的转移膜与钢球结合力较弱，因此它们的摩擦过程还包括(4)转移膜脱落及再形成阶段。以上各阶段在摩擦曲线中的对应关系如图9所示，摩擦过程中磨粒脱落过程如图10所示。

图 9 不同复合材料摩擦过程各阶段及其摩擦磨损机制简图

Figure 9. Diagram of the proposed friction and wear stage and corresponding mechanism of different composites

下载: 全尺寸图片幻灯片

图 10 复合材料磨粒脱落过程简图：(a) CF/PTFE, (b) CF-CNT/PTFE, (c) RCF/PTFE, (d) RCF-RCNT/PTFE

Figure 10. Schematic illustration of the wear debris generation process of CF/PTFE (a), CF-CNT/PTFE (b), RCF/PTFE (c), RCF-RCNT/PTFE (d) composites

下载: 全尺寸图片幻灯片

(1)表面粘附阶段：此阶段主要包括摩擦过程起始阶段的数秒，此时摩擦系数与材料表面能基本成正相关关系。

(2)表面变形阶段：此阶段主要包括摩擦曲线中摩擦系数上升最快的部分，此时在对磨球的挤压下，材料变形使表面凹凸不平，摩擦阻力迅速增大。由于五种材料的硬度整体相对较低，形变过程迅速，此阶段的持续时间也较短。

(3)转移膜形成阶段：此阶段主要包括摩擦曲线中摩擦系数逐渐上升到最高值的部分。随着摩擦过程的进行，对磨球的反复挤压犁削及产生的摩擦热逐渐使表面材料脱落形成磨粒，并将磨粒软化、压平，然后黏着于钢球表面形成转移膜，转移膜的形成避免了对磨球与表面材料的直接接触，因此摩擦系数上升速度降低。

(4)转移膜脱落及再形成阶段：由于纯PTFE、CF/PTFE和CF-CNT/PTFE中形成的转移膜强度较低、与对磨球结合力较弱，因此摩擦一段时间后转移膜会被磨穿或发生脱落，这就导致了CF/PTFE摩擦曲线中的突然上升和下降。转移膜被磨穿或脱落后，对于纯PTFE，磨损机制由黏着磨损转变为磨料磨损，因此摩擦系数突然变大^[44]；对于CF/PTFE和CF-CNT/PTFE，磨损机制由黏着磨损—磨料磨损的混合磨损转变为以磨料磨损为主，使摩擦系数突然降低^{[45, 46]}。

在摩擦过程中，纯PTFE表面摩擦产生的PTFE碎屑较软且有润滑作用，因此其全过程的摩擦系数均相对较小。CF/PTFE复合材料因为界面结合强度较低，纤维易与基体发生脱粘进而形成硬质磨粒，如图7(f)所示，进而产生三体磨料磨损，如图10(a)所示。CF-CNT/PTFE界面性能有所提高，但仍然会发生基体脱落使硬质纤维裸露，如图7(l)所示，从而易造成严重的磨粒磨损如图10(b)所示。但是，CF/PTFE和CF-CNT/PTFE的磨损率相对纯PTFE有明显下降，这主要是由于耐磨性较好的CF和CNT对基体的强化作用，以及CF和CF-CNT提高了PTFE结晶度使基体硬度也有所增加。

对于RCF/PTFE复合材料而言，其摩擦系数快速达到平稳阶段，且在整个摩擦过程始终未发生突变。这是由于RCF表面存在的La(III)可与PTFE基体分子中的F原子发生配位反应^[47]，从而提高界面结合性能，如图7(i)所示，减少了摩擦过程中从基体脱粘形成的纤维硬质点，而且La(III)还可提高钢球表面所形成转移膜的强度和稳定性^[48-50]，使其在整个摩擦过程中不易被磨穿或发生脱落，避免了三体磨料磨损，如图10(c)所示。同时，RCF的加入提高了复合材料硬度，使其挤压变形量较小且不易被硬质磨粒犁削。因此RCF/PTFE复合材料的摩擦系数及磨损率相对CF/PTFE和CF-CNT/PTFE均有所降低。

而RCF-RCNT的加入使得复合材料耐磨减摩性能进一步提高。这是由于RCF-RCNT增强体的多尺度结构使其与基体产生机械啮合作用，更加有效提高了界面结合性能，如图7(o)所示，而且RCF-RCNT表面存在更多La(III)，更有效提高了转移膜强度和稳定性。这使纤维不易脱粘形成的硬质磨粒、转移膜不易被磨穿或脱落，进而避免了三体磨料磨损，如图10(d)所示。同时，复合材料的硬度也进一步提高，因此RCF-RCNT/PTFE复合材料的摩擦系数及磨损率进一步降低。

2.3.2 载荷对复合材料摩擦学行为的影响

图11为RCF-RCNT/PTFE复合材料在不同载荷下的摩擦系数、摩擦力、磨损率及磨损量，随着载荷的上升，RCF-RCNT/PTFE复合材料摩擦系数和磨损率均未产生明显的变化趋势，因此本研究从摩擦力和磨损量两方面进行分析。可见，摩擦力先上升后降低，磨损量则持续上升。关于载荷对摩擦力的影响，本研究从以下两方面考虑，即：

图 11 不同载荷下的RCF-RCNT/PTFE复合材料摩擦系数、摩擦力、磨损率及磨损量

Figure 11. Coefficient of friction, frictional force, wear rate and wear loss of RCF-RCNT/PTFE composites under different load

下载: 全尺寸图片幻灯片

(1)载荷提高使对磨球与PTFE复合材料的接触面扩展，能量耗散及摩擦力显著变大，根据库仑定律可知此时摩擦系数上升。其中，根据Johnson-Kendall-Roberts接触模型知接触区半径 $a$ 为^[51]：

$a={\left[\frac{3R}{8}\left(\sqrt{\frac{8{\gamma }_{12}{\text{π}} }{{E}^{*}}}+\sqrt{\frac{8{\gamma }_{12}{\text{π}} }{{E}^{*}}+\frac{16}{3R{E}^{*}}F}\right)\right]}^{\frac{2}{3}}$

(7)

其中，

$F$ 载荷

$R$ 对磨球半径

${E}^{*}$ 有效弹性模量

${\gamma }_{12}$ 两摩擦副材料之间的界面能

(2)载荷提高使摩擦面上的凸起点被压平、粗糙度降低，摩擦力减小。

在载荷从12 N提高到18 N的过程中，摩擦副接触面扩展，同时载荷较小不足以压平摩擦表面的凸起点，因素(1)占主要地位，因此摩擦力上升。在载荷从18 N增加到20 N的过程中，由于载荷较大，摩擦面表面的凸起点逐渐被压平，同时，由式7知，载荷 $F$ 较大时，增加载荷产生的接触半径 $a$ 增长较小，因此因素(2)此时占据主要地位，摩擦力减小。

关于载荷对磨损量的影响，根据Johnson-Kendall-Roberts接触模型知接触区变形深度 $d$ 为^[51]：

$d=\frac{{a}^{2}}{R}+\sqrt{\frac{2{\gamma }_{12}{\text{π}} a}{{E}^{*}}}$

(8)

联立式(7)和式(8)知接触区半径 $a\propto {F}^{1/3}$ ，变形深度 $d\propto {F}^{2/3}$ ，同时，磨损量 $\Delta V\propto \left(d\cdot a\right)$ ，即 $\Delta V\propto F$ ，因此RCF-RCNT/PTFE复合材料的磨损量随载荷的增大而增大。另外，材料体积磨损率 $W=\Delta V/(F\cdot L)$ ( $L$ 为摩擦长度)，因此， $W\propto {F}^{0}$ ，即，由于本研究中载荷提高并未造成磨损机制及材料物性(弹性模量等)的变化，所以不同载荷下的磨损率无明显变化趋势。

2.3.3 往复频率对复合材料摩擦学行为的影响

图12为不同往复频率下的RCF-RCNT/PTFE复合材料摩擦系数、磨损率及磨损量，图13为不同频率下的对磨球典型表面形貌。RCF-RCNT/PTFE复合材料摩擦系数随着往复频率从0.21先升高到0.26后降低到0.20。这是由于，往复频率较低时，随着频率提高，摩擦生热逐渐变快，摩擦面快速升温软化，加剧了钢球表面转移膜与基体材料的黏着磨损，从而导致4 Hz到8 Hz时的摩擦系数增大。但是，由图13可知，往复频率为10 Hz时对磨球表面转移膜相对6 Hz时较为连续且未见较深的犁沟，这表明当往复频率进一步升高时摩擦面继续升温，高温使磨粒快速软化，在摩擦副之间形成了连续的转移膜，阻碍了对摩球与表面材料的直接接触，从而使8 Hz到12 Hz时的摩擦系数降低。

图 12 不同往复频率下的RCF-RCNT/PTFE复合材料摩擦系数、磨损率及磨损量

Figure 12. Coefficient of friction, wear rate and wear loss of RCF-RCNT/PTFE composites under different reciprocating frequency

下载: 全尺寸图片幻灯片

图 13 往复频率为6 Hz (a)、10 Hz (b)，载荷为16 N时RCF-RCNT/PTFE复合材料摩擦学试验中的GCr15对磨球典型形貌

Figure 13. Typical worn surface morphology of GCr15 grinding ball after the tribological test of RCF-RCNT/Ep under 16 N and 6 Hz (a), 10 Hz (b)

下载: 全尺寸图片幻灯片

此外，RCF-RCNT/PTFE复合材料磨损率随往复频率升高逐渐降低，磨损量随往复频率从4 Hz升高到12 Hz逐渐增加。摩擦过程是对磨球对磨合材料的磨削过程，较大的往复频率意味着较快的摩擦速度，这一方面增大了摩擦长度，提高了磨损量，另一方面也使摩擦表面温度升高，材料升温软化后粘附在钢球表面阻碍了钢球对复合材料的直接磨削，因此磨损量 $\Delta V$ 随往复频率 $f$ 提高的增加程度并不大，由于磨损率 $W=\Delta V/(F\cdot f\cdot {L}_{0}\cdot t)(f$ 为往复频率， $F,{L}_{0},t$ 分别为载荷、往复行程和摩擦时间)，因此发生了磨损率的下降。

3. 结论

本研究采用绿色合成法制备碳纤维(Carbon Fibre, CF)-碳纳米管(Carbon Nanotube, CNT)微纳多尺度增强体，将其用于提高聚四氟乙烯(Polytetrafluoroethylene, PTFE)基复合材料摩擦学性能，从硬度、结晶度、表面能、界面结合性能等方面分析了增强体对复合材料起始摩擦系数、摩擦曲线和磨损率的影响机制，并探究了摩擦学试验中的往复频率及载荷对多尺度增强PTFE基复合材料摩擦学行为的影响。所得主要结论如下：

(1)本研究所提出的RCF-RCNT微纳多尺度增强体制备方法不损伤纤维，相对传统化学接枝法具有绿色环保的工艺优势。

(2)RCF-RCNT多尺度增强体提高了PTFE基复合材料的硬度，原因在于硬质纤维相不但能提高硬度，还能增加PTFE形核结晶位点，而且RCF-RCNT增强体表面稀土La(III)具有成核剂的作用，这使复合材料结晶度提高。

(3)PTFE基复合材料的起始摩擦系数与其表面能成正相关关系，RCF-RCNT/PTFE因其较高的结晶度而具有较低的表面能和起始摩擦系数。

(4)RCF-RCNT/PTFE复合材料的磨损率相对纯PTFE降低了75.4%，这一减磨程度优于同类研究，其原因在于：RCF-RCNT增强体的多尺度结构使其与基体产生机械啮合作用，而且其表面存在的La(III)与PTFE基体分子中的F原子发生配位反应，显著提高了界面结合性能及转移膜强度和稳定性，使纤维不易脱粘形成硬质点、转移膜不易脱落导致三体磨料磨损。同时，RCF-RCNT/PTFE复合材料硬度较高，挤压变形量较小且不易被硬质磨粒犁削。

(5)在RCF-RCNT/PTFE复合材料的往复摩擦学实验中，随着载荷增加，摩擦面扩展速度减缓但摩擦表面逐渐被压平，因此摩擦力先增大后减小，同时由于磨损机制及材料物性无明显改变，所以磨损量维持线性增长，磨损率并未产生明显变化；随着往复频率增加，软化的基体和磨料逐渐使钢球表面转移膜连续，磨损机制逐渐由黏着磨损与磨料磨损的混合磨损形式向单一黏着磨损转变，因此摩擦系数先增大后减小，而磨损率持续减小。总体而言，RCF-RCNT/PTFE复合材料在高往复频率、低载荷下的磨损率更低。

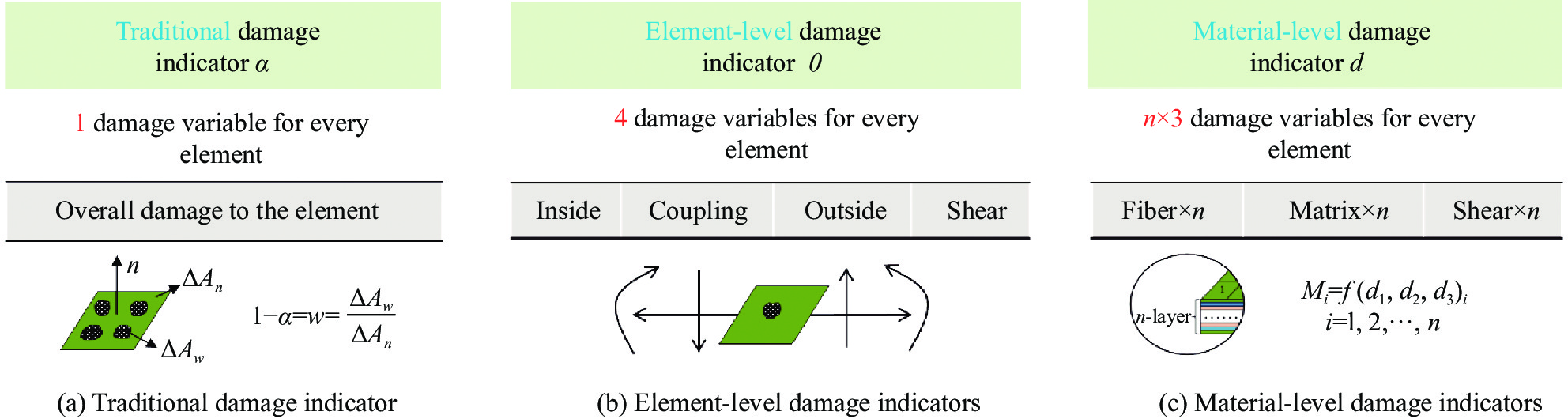
图 1 3类损伤指标所需损伤变量个数及特点

Figure 1. Number of damage variables required for the three types of damage indicators and their characteristics

w—Traditional damage variable; ΔA_n—Normallized area; ΔA_w—Damaged area; M_i—Vector containing material-level damage indicators;f(d₁, d₂, d₃)_i—Function of material-level damage indicators

下载: 全尺寸图片幻灯片

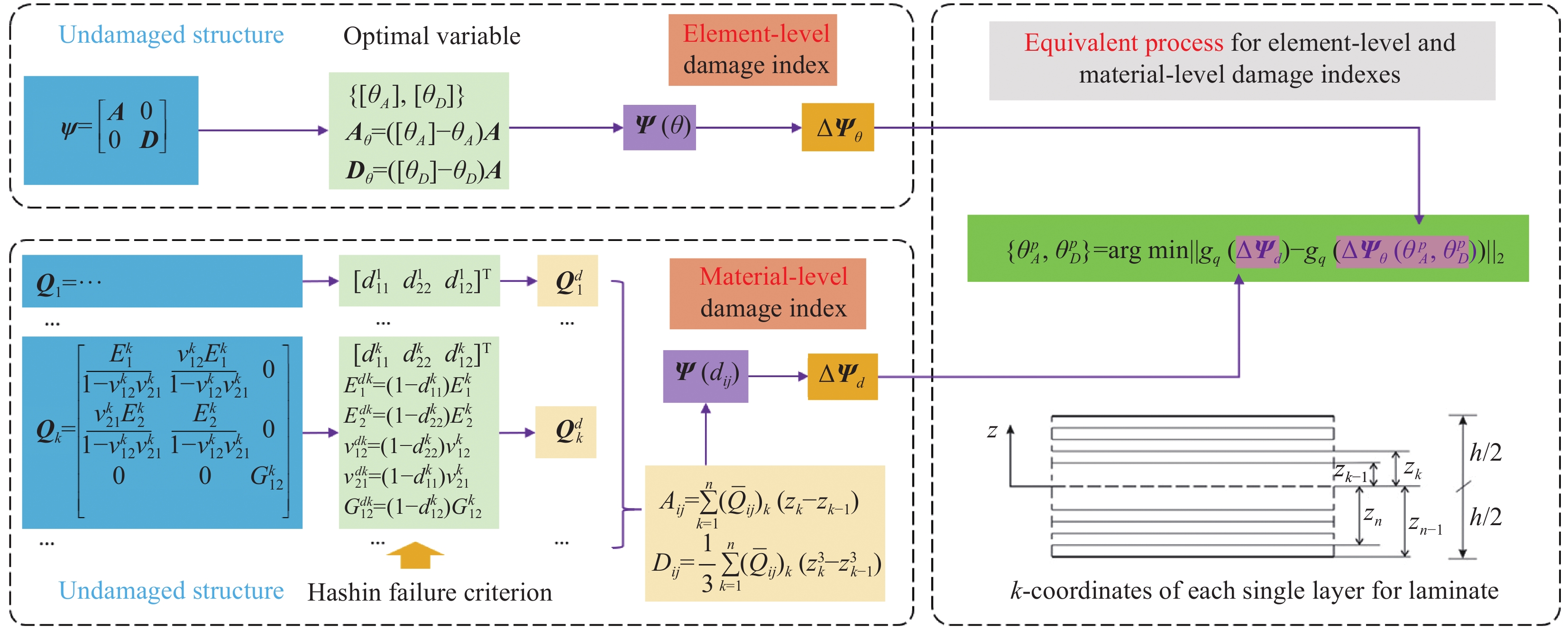
图 2 材料级和单元级损伤指标等效过程示意图

Figure 2. Schematic diagram of equivalent process for material-level and element-level damage indicators

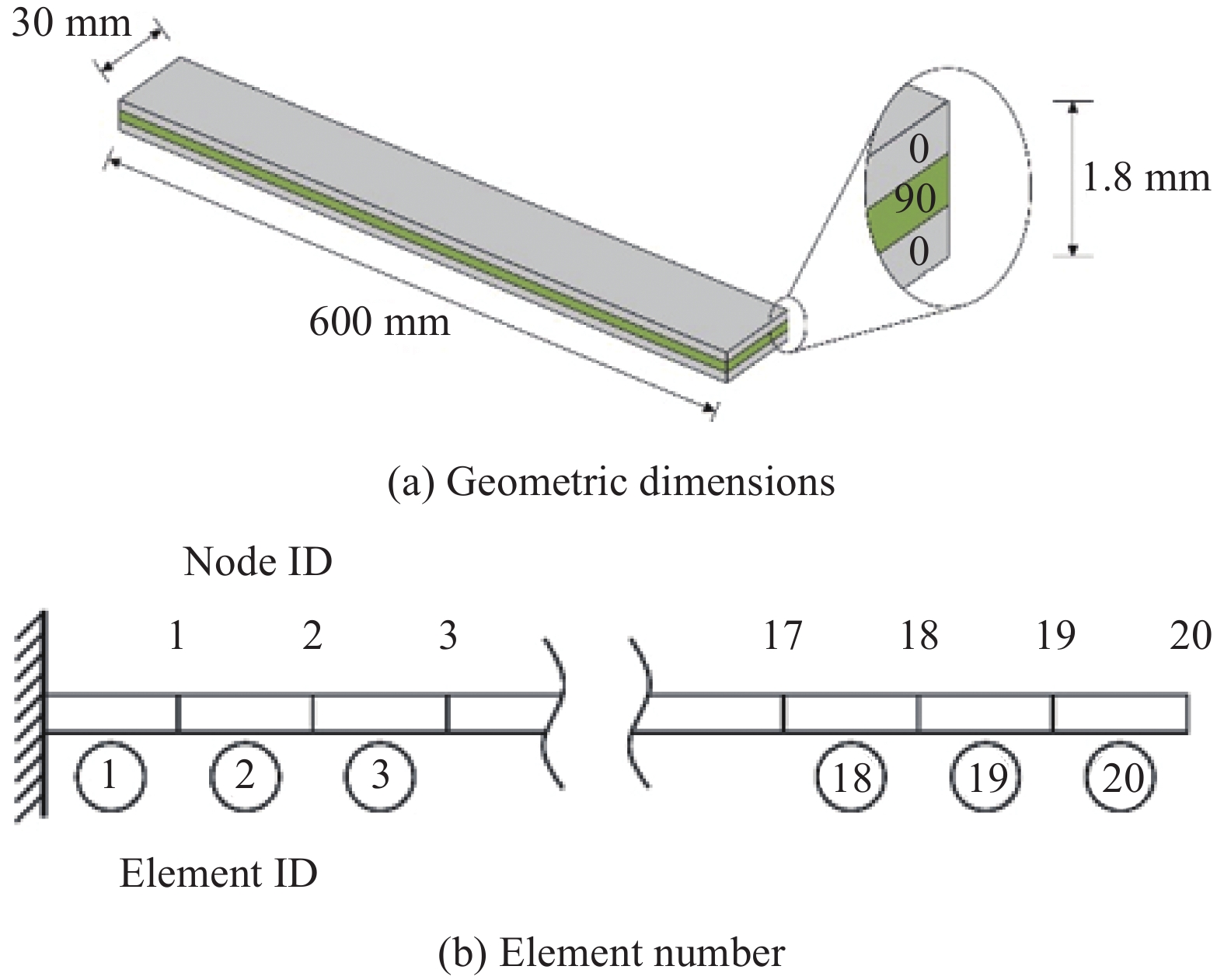
${\boldsymbol{\varPsi }}$ —Stiffness matrix of symmetric laminates; A—Tensile stiffness matrix; D—Bending stiffness matrix; Q₁—Stiffness matrix of the 1st layer; Q₁ ^d—Stiffness matrix of the 1st layer of the damaged structure; Q_k—Stiffness matrix of the kth layer; Q_k ^d—Stiffness matrix of the kth layer of the damaged structure; $E_1^{dk}, E_{\rm{2}}^{dk}, v_{12}^{dk}, v_{{\rm{21}}}^{dk}, G_{{\rm{12}}}^{dk}$ —Longitudinal, transverse elastic moduli, Poisson's ratio in both in-face directions, and shear modulus of the kth layer of the damaged structure; $E_1^{k}, E_{\rm{2}}^{k}, v_{12}^{k}, v_{{\rm{21}}}^{k}, G_{{\rm{12}}}^{k}$ —Longitudinal, transverse elastic moduli, Poisson's ratio in both in-face directions, and shear modulus of the kth layer of the undamaged structure; $d_{11}^k, d_{22}^k, d_{{\rm{12}}}^k$ —Material-level damage parameters in fiber direction, matrix direction and shear direction for the kth layer; ${\boldsymbol{\varPsi }}\left( \theta \right)$ —Stiffness matrix expressed by element-level damage parameters; $\Delta {\boldsymbol{\varPsi }}_\theta ^{}$ —Residual stiffness matrix expressed by element-level damage parameters; ${\boldsymbol{\varPsi }}\left( {d_{ij}^{}} \right)$ —Stiffness matrix expressed by material-level damage parameters; $\Delta{\boldsymbol{ \varPsi}} _d^{}$ —Residual stiffness matrix expressed by material-level damage parameters; $\theta _A^p, \theta _D^p$ —In-plane and out-of-plane element-level damage indicators under the pth failure mode; $g_q^{}$ —The qth kind of operator; $\left\| \cdot \right\|_2$ —The 2-norm of matrix; h—Thickness of laminates; ${z_k}$ —Midplane coordinates of the kth layer

下载: 全尺寸图片幻灯片

图 3 层合板面内载荷系数退化曲线

Figure 3. In-plane loading coefficient degradation curves of laminates

下载: 全尺寸图片幻灯片

图 4 层合板拉弯组合工况系数退化曲线

Figure 4. Combined tension-bending condition coefficient degradation curves of laminates

下载: 全尺寸图片幻灯片

图 5 层合板的面内面外损伤系数

Figure 5. In-plane and out-plane damage factors of laminates

下载: 全尺寸图片幻灯片

图 6 四边固支板示意图

Figure 6. Diagram of four sides of the fixed support plate

下载: 全尺寸图片幻灯片

图 7 损伤工况1损伤定位指标

Figure 7. Damage condition 1 damage positioning index

NMSECR—Normalized modal strain energy change ratio

下载: 全尺寸图片幻灯片

图 8 损伤工况2损伤定位指标

Figure 8. Damage condition 2 damage positioning index

下载: 全尺寸图片幻灯片

图 9 识别参数平均值(MV)随噪声水平变化曲线

Figure 9. Curves of mean value (MV) of identification parameterswith noise level

A—In-plane damage; D—Out-plane damage

下载: 全尺寸图片幻灯片

图 10 识别参数标准差(std)随噪声水平变化曲线

Figure 10. Curve of standard deviation (std) of identification parameters with noise level

下载: 全尺寸图片幻灯片

图 11 悬臂梁模型信息

Figure 11. Cantilever beam model information

下载: 全尺寸图片幻灯片

图 12 模态测试设备示意图

Figure 12. Schematic diagram of the modal test equipment

DAE—Data acquisition equipment

下载: 全尺寸图片幻灯片

图 13 梁模态测试试验设备

Figure 13. Modal test equipment for beam

下载: 全尺寸图片幻灯片

图 14 预制的贯穿裂纹损伤

Figure 14. Prefabricated penetration crack damage

下载: 全尺寸图片幻灯片

图 15 未损伤和损伤悬臂梁的模态信息

Figure 15. Modal information of undamaged and damaged cantilever beams

下载: 全尺寸图片幻灯片

图 16 悬臂梁的测点位置

Figure 16. Location of measurement points of cantilever beam

下载: 全尺寸图片幻灯片

图 17 悬臂梁的损伤定位指标

Figure 17. Damage localization index of cantilever beam

下载: 全尺寸图片幻灯片

图 18 重构单元

Figure 18. Rebuilt elements

下载: 全尺寸图片幻灯片

图 19 计算等效刚度的工况

Figure 19. Working conditions for calculating equivalent stiffness

M_y—Moment of force coupling around the y axis

下载: 全尺寸图片幻灯片

图 20 等效刚度计算结果和位移云图

Figure 20. Equivalent stiffness calculation results and displacement nephogram

K_xz ^u —Rotational stiffness around the y direction in the undamaged state; K_xz ^d—Rotational stiffness around the y direction in the damaged state

下载: 全尺寸图片幻灯片

表 1 复合材料层合板材料属性

Table 1 Material parameters of composite laminate

E₁/GPa	E₂/GPa	G₁₂/GPa	ν₁₂	X_t/GPa	X_c/GPa	Y_t/GPa	Y_c/GPa	S₁₂/GPa
140	10	5	0.3	1500	1200	50	250	70
Notes: E₁ and E₂—Longitudinal and transverse elastic moduli; G₁₂—In-plane shear modulus; v₁₂—Poisson's ratio; X_t and X_c—Longitudinal tensile and compressive strengths; Y_t and Y_c—Transverse tensile and compressive strengths; S₁₂—In-plane shear strength.

下载: 导出CSV

表 2 仿真工况

Table 2 Simulation conditions

Number	Description of working conditions and load vectors	[Nx; Ny; Nxy]	[Mx; My; Mxy]
1	Tensile	[100; 100; 0]	[0; 0; 0]
2	Tensile and bending	[100; 100; 0]	[100; 100; 0]
Notes: [Nx; Ny; Nxy]—Combined internal force per element width of laminate;[Mx; My; Mxy]—Combined internal moment per element width of laminate.

下载: 导出CSV

表 3 层合板两种载荷的失效路径

Table 3 Failure paths of two loads of laminates

No.	Failure paths
1	1MT, 3MT, 5MT, 7MT→2MT, 6MT→4MT→1FT, 2FT, 3FT, 4FT, 5FT, 6FT, 7FT
2	6MT, 7MT→1FC, 7FT→5MT→1MC→3MT→2MC→4MT, 5FT, 6FT→3FC→1MT, 4FT
Notes: MT and MC—Tensile and compression of matrix; FT and FC—Tensile and compression of fiber.

下载: 导出CSV

表 4 损伤工况设置

Table 4 Damage condition settings

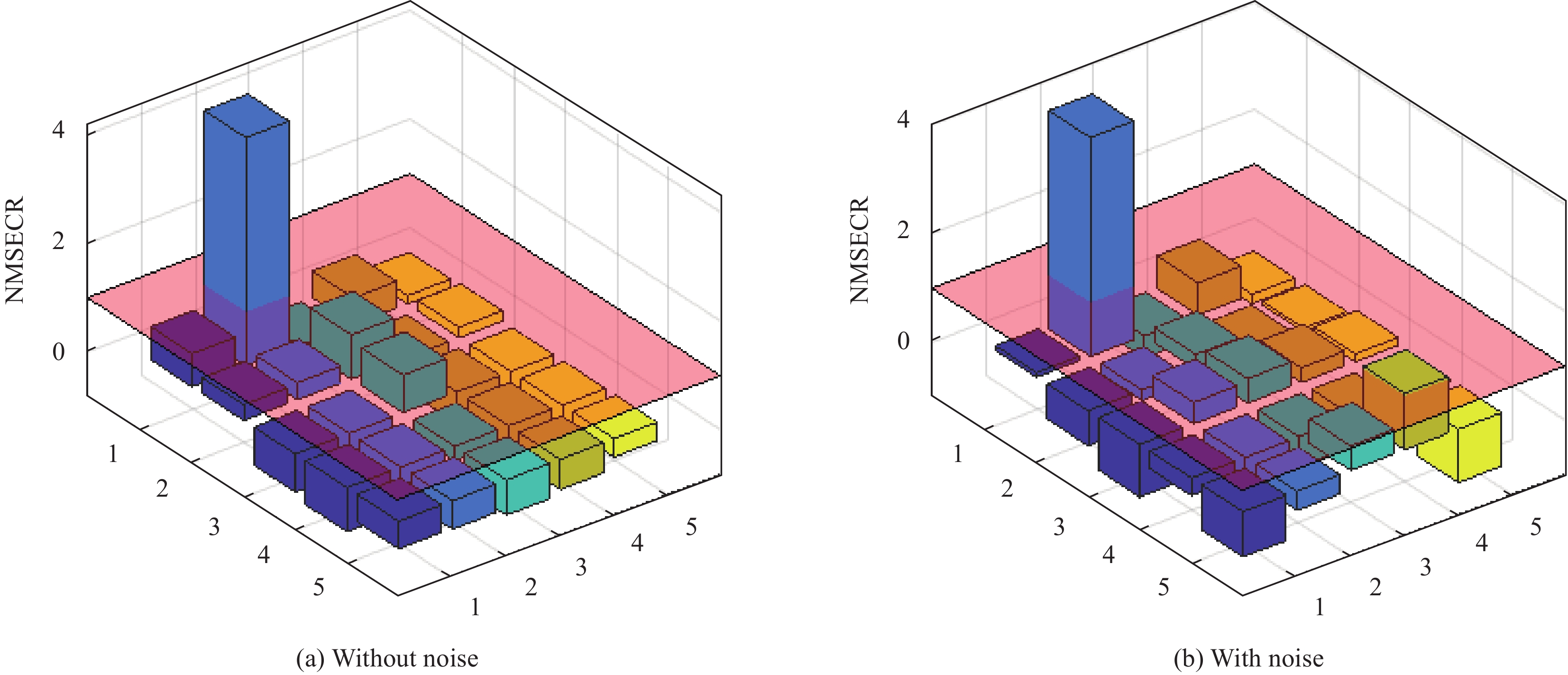
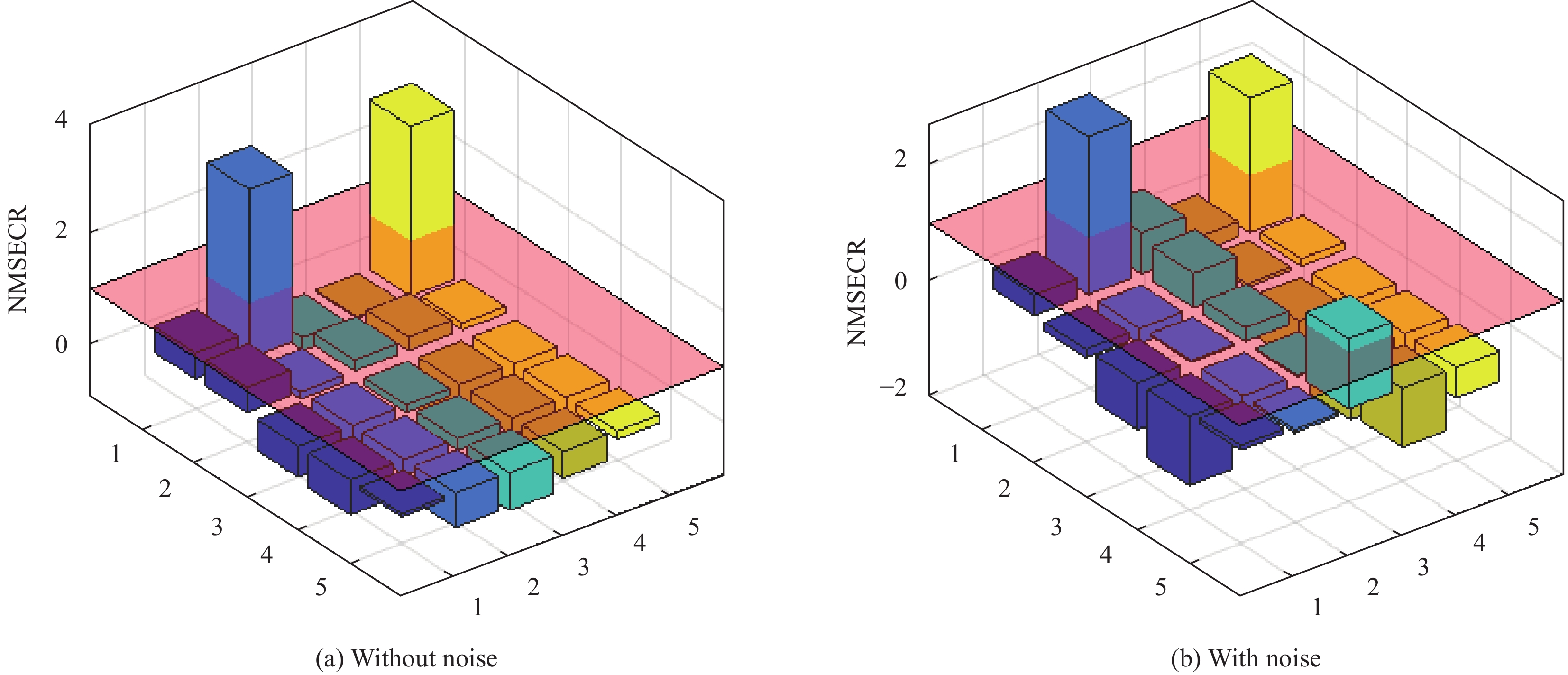
Damage condition	Damage element	Extent of damage
Damage condition	Damage element	θ_A	θ_D
1	2	0.3	0.25
2	2	0.3	0.25
2	5	0.2	0.25

下载: 导出CSV

表 5 无噪声工况识别结果

Table 5 Identification results without noise condition

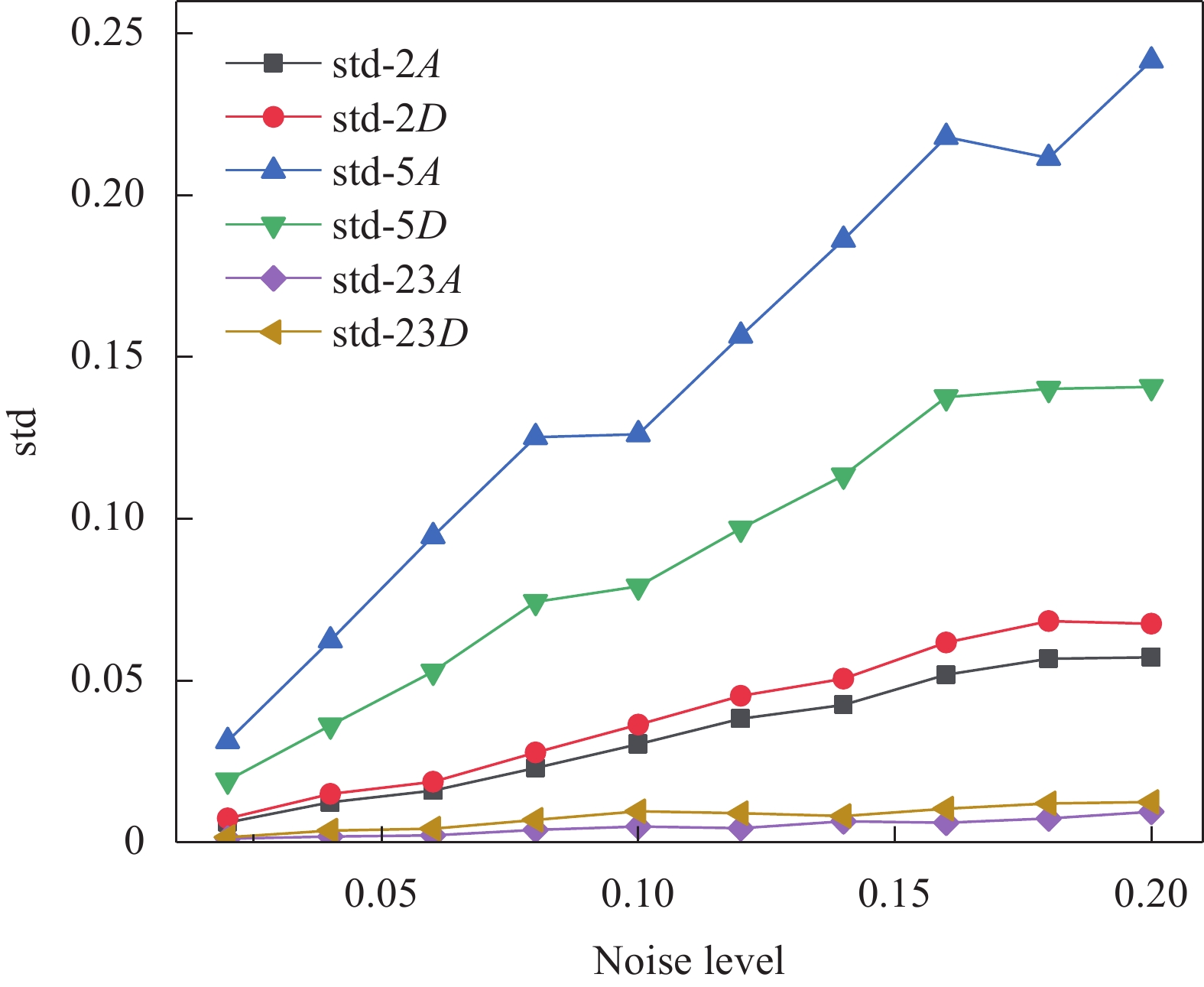
Damage conditions	Damaged element	θ_A		θ_D
Damage conditions	Damaged element	Identified value	Error	Identified value	Error
Single damage	2	0.3	0	0.25	0
Single damage	24	0	—	0	—
Multi-damage	2	0.3	0	0.25	0
	5	0.2	0	0.25	0
	23	0	—	0	—

下载: 导出CSV

表 6 含噪声工况识别结果

Table 6 Identification results with noise conditions

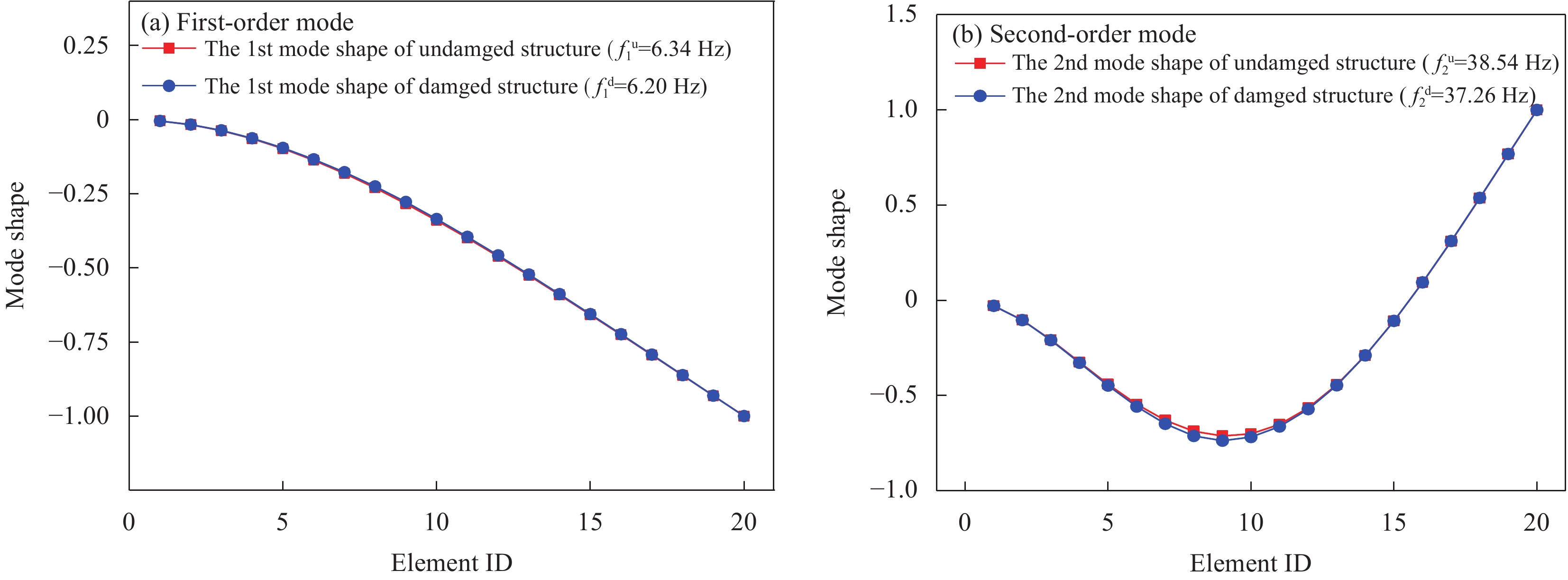
Damage conditions	Damaged element	θ_A		θ_D
Damage conditions	Damaged element	Identified value	Error	Identified value	Error
Single damage	2	0.2993	0.20%	0.2513	−0.52%
Single damage	24	0	—	0	—
Multi-damage	2	0.3002	0.07%	0.2491	−0.36%
	5	0.1902	−4.90%	0.2401	−3.96%
	23	0.0001	—	0	—

下载: 导出CSV

表 7 悬臂梁修正后的固有频率

Table 7 Corrected modal frequency of cantilevered beam

Modal order	Simulation values/Hz	Test values/Hz	Relative error/%
1	6.47	6.34	2.21
2	38.72	38.54	0.49
3	96.01	96.57	−0.53
4	188.76	190.91	−1.13
5	336.17	339.77	−1.06

下载: 导出CSV

表 8 悬臂梁修正后的弹性参数

Table 8 Elastic parameters of cantilever beam after model updating

E₁₁/GPa	E₂₂/GPa	G₁₂/GPa	v₁₂	ρ/(kg·m⁻³)
119.57	8.79	5.70	0.30	1794.67
Note: ρ—Density.

下载: 导出CSV

参考文献(27)

[1]	陈雪峰, 杨志勃, 田绍华, 等. 复合材料结构损伤识别与健康监测展望[J]. 振动测试与诊断, 2018, 38(1):1-10, 202. CHEN Xuefeng, YANG Zhibo, TIAN Shaohua, et al. A review of the damage detection and health monitoring for composite structures[J]. Journal of Vibration, Measurement & Diagnosis,2018,38(1):1-10, 202(in Chinese).
[2]	陈健, 袁慎芳. 加筋复合材料结构分层损伤的贝叶斯诊断及预测[J]. 复合材料学报, 2021, 38(11):3726-3736. DOI: 10.13801/j.cnki.fhclxb.20210202.003 CHEN Jian, YUAN Shenfang. Bayesian diagnosis and prognosis of delamination damage in the stiffened composite structure[J]. Acta Materiae Compositae Sinica,2021,38(11):3726-3736(in Chinese). DOI: 10.13801/j.cnki.fhclxb.20210202.003
[3]	徐浩, 沙刚刚, 李腾腾, 等. 基于二维连续小波变换与数据融合技术的逆有限元法-伪激励法结构损伤识别方法[J]. 复合材料学报, 2021, 38(10):3554-3562. DOI: 10.13801/j.cnki.fhclxb.20210115.001 XU Hao, SHA Ganggang, LI Tengteng, et al. Structure damage identification method of inverse finite element method-pseudo-excitation method based on 2D continuous wavelet transform and data fusion technology[J]. Acta Materiae Compositae Sinica,2021,38(10):3554-3562(in Chinese). DOI: 10.13801/j.cnki.fhclxb.20210115.001
[4]	颜津玮, 曹善成, 徐超. 基于低秩与稀疏分解的层合板损伤定位[J]. 振动测试与诊断, 2022, 42(6):1220-1225, 1250. YAN Jinwei, CAO Shangcheng, XU Chao. Damage localization of composite laminated plates based on low-rank and sparse decomposition[J]. Journal of Vibration, Measurement & Diagnosis,2022,42(6):1220-1225, 1250(in Chinese).
[5]	黄斌, 郭文豪, 鲁溢, 等. 随机梁式结构静力损伤识别的一种改进方法[J]. 计算力学学报, 2018, 35(4): 451-457. HUANG Bin, GUO Wenhao, LU Yi, et al, An improved method of damage identification of random beam structures[J]. Chinese Journal of Computational Mechanics , 2018, 35(4): 451-457(in Chinese).
[6]	闫天红, 王凤山, 姜民政, 等. 基于模态参数灵敏度分析的结构损伤识别研究[J]. 机械设计, 2019, 36(12): 79-83 YAN Tianhong, WANG Fengshan, JIANG Minzheng, et al, Identification of structural damage based on the sensitivity analysis of modal parameters[J]. Journal of Machine Design, 2019, 36(12): 79-83(in Chinese).
[7]	SHI Q H, WANG X J, WANG L, et al. Set-membership identification technique for structural damage based on the dynamic responses with noises[J]. Structural Control & Health Monitoring,2017,24(2):e1868.
[8]	DU Y, ZHOU S, JING X, et al. Damage detection techniques for wind turbine blades: A review[J]. Mechanical Systems and Signal Processing,2020,141:106445. DOI: 10.1016/j.ymssp.2019.106445
[9]	WANG X J, SHI Q H, WANG L, et al. Anisotropic reduction factor-based damage identification method for fiber-reinforced composite laminates[J]. Structural Control and Health Monitoring,2018,25(11):e2253. DOI: 10.1002/stc.2253
[10]	邱家波, 李华东. 复合材料帽型加筋层合板典型板元的等效刚度计算[J]. 复合材料科学与工程, 2020(7):33-39. DOI: 10.3969/j.issn.1003-0999.2020.07.005 QIU Jiabo, LI Huadong. Equivalent stiffness calculation of typical plante element of composite cap stiffened laminates[J]. Journal of Composites Science and Engineering,2020(7):33-39(in Chinese). DOI: 10.3969/j.issn.1003-0999.2020.07.005
[11]	朱秀杰, 熊超, 殷军辉, 等. 基于复合材料层合箱梁改进解析模型计算等效刚度[J]. 复合材料学报, 2020, 37(6):1483-1495. DOI: 10.13801/j.cnki.fhclxb.20190706.001 ZHU Xiujie, XIONG Chao, YIN Junhui, et al. Equivalent stiffness calculation based on refined analytical model of composite laminated box beam[J]. Acta Materiae Compositae Sinica,2020,37(6):1483-1495(in Chinese). DOI: 10.13801/j.cnki.fhclxb.20190706.001
[12]	刘航, 杜国君, 冯岩. 构造上正交各向异性半球形凸起凹凸板等效刚度研究[J]. 应用数学和力学, 2020, 41(1):70-80. LIU Hang, DU Guojun, FENG Yan. Study on equivalent stiffnesses of orthotropic hemi-spherical convex plates[J]. Applied Mathematics and Mechanics,2020,41(1):70-80(in Chinese).
[13]	黄炳生, 秦雨生, 黄泰杰. 基于等效刚度的矩形孔蜂窝梁钢框架结构内力计算方法[J]. 力学季刊, 2023, 44(1):218-226. DOI: 10.15959/j.cnki.0254-0053.2023.01.022 HUANG Bingsheng, QIN Yusheng, HUANG Taijie. Internal force calculation of steel frame structure containing castellated beams with rectangular holes based on equivalent stiffness method[J]. Chinese Quarterly of Mechanics,2023,44(1):218-226(in Chinese). DOI: 10.15959/j.cnki.0254-0053.2023.01.022
[14]	GHODRATI AMIRI G, ZARE HOSSEINZADEH A, JAFARIAN ABYANEH M. A new two-stage method for damage identification in linear-shaped structures via grey system theory and optimization algorithm[J]. Journal of Rehabilitation in Civil Engineering,2015,3(2):45-58.
[15]	VO-DUY T, HO-HUU V, DANG-TRUNG H, et al. A two-step approach for damage detection in laminated composite structures using modal strain energy method and an improved differential evolution algorithm[J]. Composite Structures,2016,147:42-53. DOI: 10.1016/j.compstruct.2016.03.027
[16]	郭惠勇, 李正良. 基于应变能等效指标的结构损伤识别技术研究[J]. 固体力学学报, 2013, 34(3):286-291. DOI: 10.3969/j.issn.0254-7805.2013.03.009 GUO Huiyong, LI Zhengliang. Structural damage identification method based on strain energy equivalence parameter[J]. Chinese Journal of Solid Mechanics,2013,34(3):286-291(in Chinese). DOI: 10.3969/j.issn.0254-7805.2013.03.009
[17]	XIA Y, HAO H, BROWNJOHN J M W, et al. Damage identification of structures with uncertain frequency and mode shape data[J]. Earthquake Engineering & Structural Dynamics,2002,31(5):1053-1066.
[18]	FANG Y, LIU X, XING J, et al. Substructure damage identification based on sensitivity of power spectral density[J]. Journal of Sound and Vibration,2023,545:117451. DOI: 10.1016/j.jsv.2022.117451
[19]	JALALI S S, MAHZOON M, MOHAMMADI H. Identification of damage properties of glass/epoxy laminates using machine learning models[J]. International Journal of Impact Engineering, 2023, 177: 104510.
[20]	XU D, LIU P F, CHEN Z P, et al. Achieving robust damage mode identification of adhesive composite joints for wind turbine blade using acoustic emission and machine learning[J]. Composite Structures,2020,236:111840. DOI: 10.1016/j.compstruct.2019.111840
[21]	DINH-CONG D, NGUYEN-THOI T. A chaos game optimization-based model updating technique for structural damage identification under incomplete noisy measurements and temperature variations[J]. Structures,2023,48:1271-1284. DOI: 10.1016/j.istruc.2023.01.032
[22]	HUANG M, CHENG X, LEI Y. Structural damage identification based on substructure method and improved whale optimization algorithm[J]. Journal of Civil Structural Health Monitoring,2021,11(2):351-380. DOI: 10.1007/s13349-020-00456-7
[23]	STUBBS N, BROOME T H, OSEGUEDA R. Nondestructive construction error detection in large space structures[J]. AIAA Journal,1990,28(1):146-152. DOI: 10.2514/3.10365
[24]	刘康. 基于模型修正的结构损伤识别优化方法研究[D]. 武汉: 武汉理工大学, 2018. LIU Kang. The optimized method for structural damage identification based on model updating technique[D]. Wuhan: Wuhan University of Technology, 2018(in Chinese).
[25]	YAM L, YAN Y, JIANG J. Vibration-based damage detection for composite structures using wavelet transform and neural network identification[J]. Composite Structures,2003,60(4):403-412. DOI: 10.1016/S0263-8223(03)00023-0
[26]	BEGAMBRE O, LAIER J E. A hybrid particle swarm optimization-simplex algorithm (PSOS) for structural damage identification[J]. Advances in Engineering Software,2009,40(9):883-891. DOI: 10.1016/j.advengsoft.2009.01.004
[27]	HASHIN Z. Failure criteria for unidirectional fiber composites[J]. Journal of Applied Mechanics,1980,47(2):329-334. DOI: 10.1115/1.3153664

施引文献(2)

期刊类型引用(1)

付强，李浩明，彭磊，李智，林木松，张丽，谢松瑜，唐征海，郭宝春. 填充型导热绝缘硅橡胶复合材料的研究进展. 绝缘材料. 2024(09): 1-14 .

百度学术

其他类型引用(1)

资源附件(0)

长摘要

目的
复合材料层合板结构具有纤维断裂、基体开裂、剪切失效、分层等多种失效模式。目前存在传统损伤指标和材料级损伤指标对复合材料层合板的刚度损伤进行定义。前者具有损伤参数少，识别较为容易的优点，但是能够反映的损伤信息有限；后者能够对复合材料层合板的失效模式进行表征，但是损伤参数数量巨大，识别起来较为困难。基于此，本文提出了单元级损伤指标，即基于经典层合板理论对层合板单元的面内刚度和面外刚度的折减进行了表征。为保证所提损伤指标的合理性，本文利用数学和力学算子对单元级损伤指标与材料级损伤指标进行了等效，比较了不同损伤指标在表征损伤程度之间的差异性，并提出了面向单元级损伤指标的复合材料层合板损伤识别流程。
方法
从数学和力学两方面建立材料级损伤参数和单元级损伤参数的等效标的数学物理量。提出了能够反映层合板单元损伤指标的单元级结构损伤指标，并对材料级与单元级损伤指标建立“等效”关系，该指标具有识别参数较少、包含损伤信息较多的优势。此外，本文优化了单元级损伤指标的损伤识别流程，即第一步利用损伤前后的模态应变能指标筛选损伤位置，第二步利用优化方法识别单元级损伤指标大小。
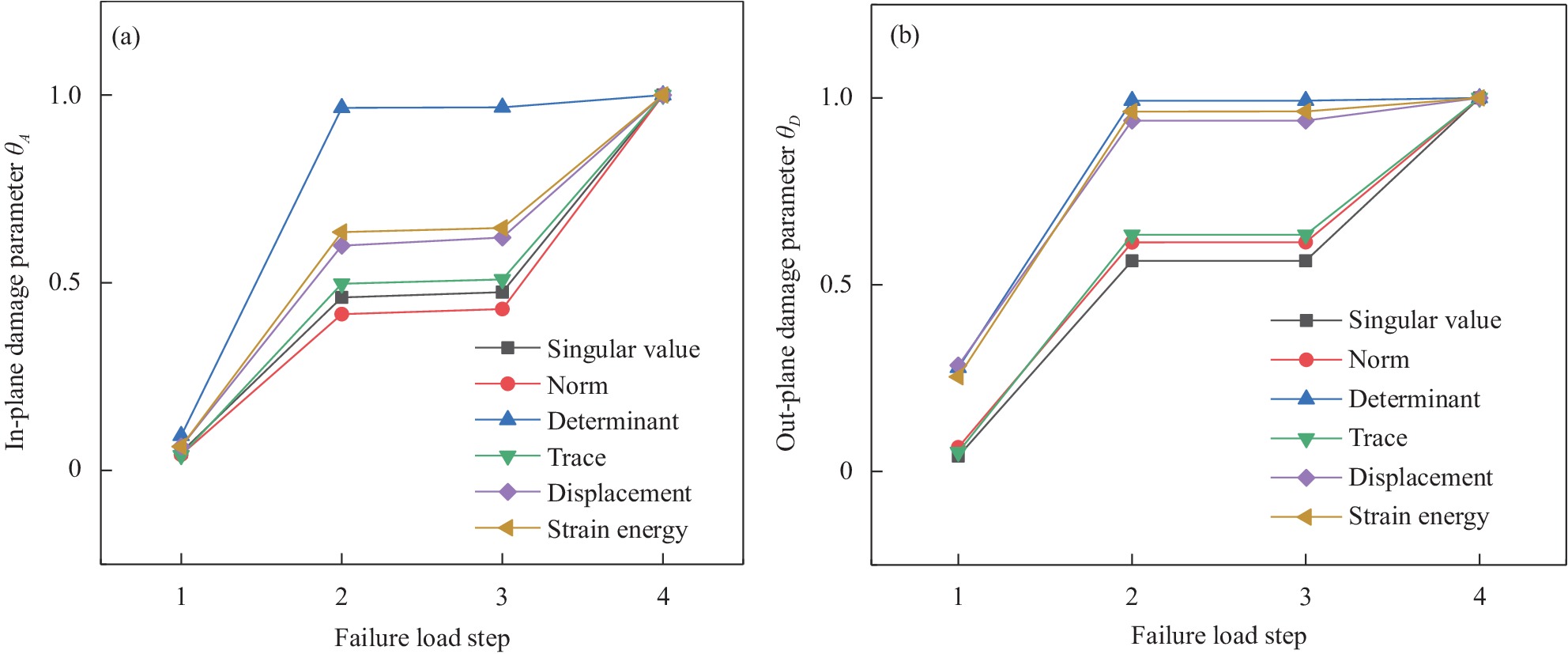
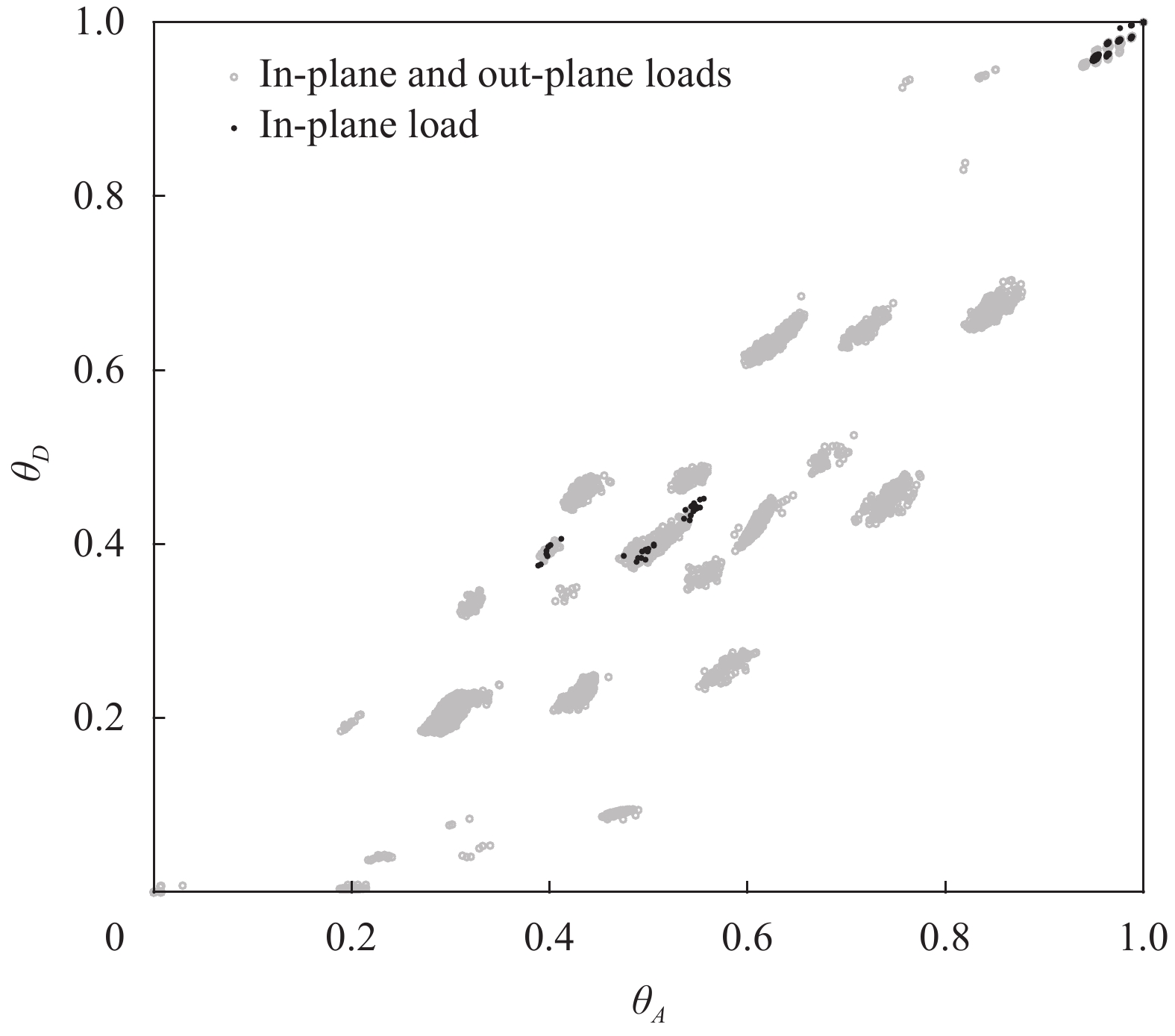
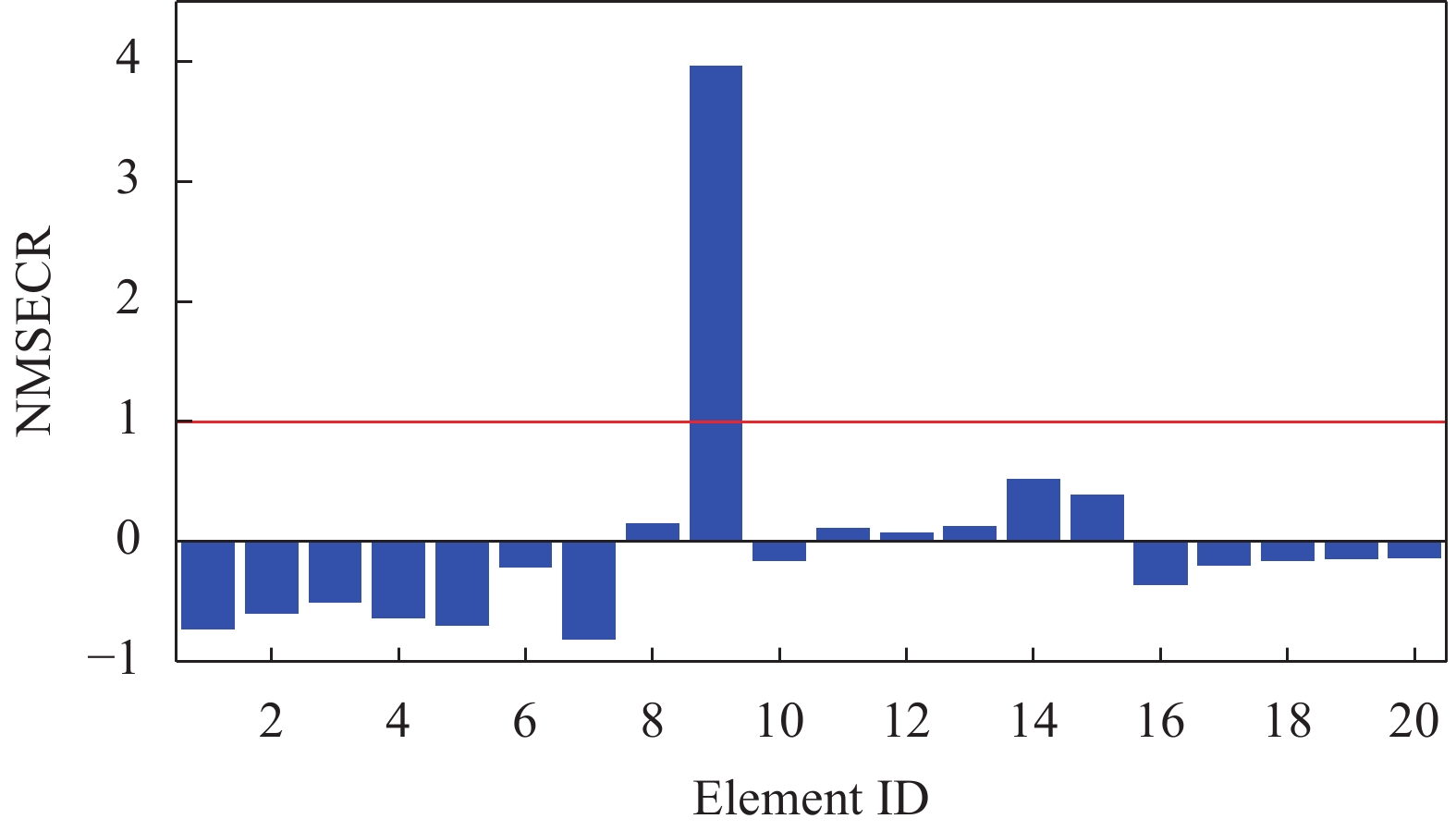
结果
通过比较不同等效算子计算出的损伤指标，发现各种指标随载荷的增加也会单调增加，各损伤指标的增长趋势趋于一致，并分析了面内和面外损伤参数之间的相关性。范数、奇异值和迹三种指标的等效结果近似相等，行列式和力学等效指标等效结果较大。这是由于前三类指标在数学上对损伤的定义较为接近，均是将损伤结构刚度矩阵的奇异值向包含单元级损伤参数的刚度矩阵进行“逼近”。在进行损伤定位时，无噪声条件下可以清晰地分辨出损伤和未损伤单元，在噪声条件下有可能会出现误判，但是这种误判可以通过第二步损伤定量识别来进行消除。此外，本文还分析了噪声水平对识别结果误差的影响，发现随着噪声水平的增加，不同位置的单元级损伤识别误差呈现不同的增长趋势。这是由于不同单元的刚度系数对噪声的敏感程度不同，因此造成差异化的影响趋势。另外，因单元关联的测点信息越多，受到噪声的影响就越小，这是因为不同测点的噪声会有一定的抵消作用。
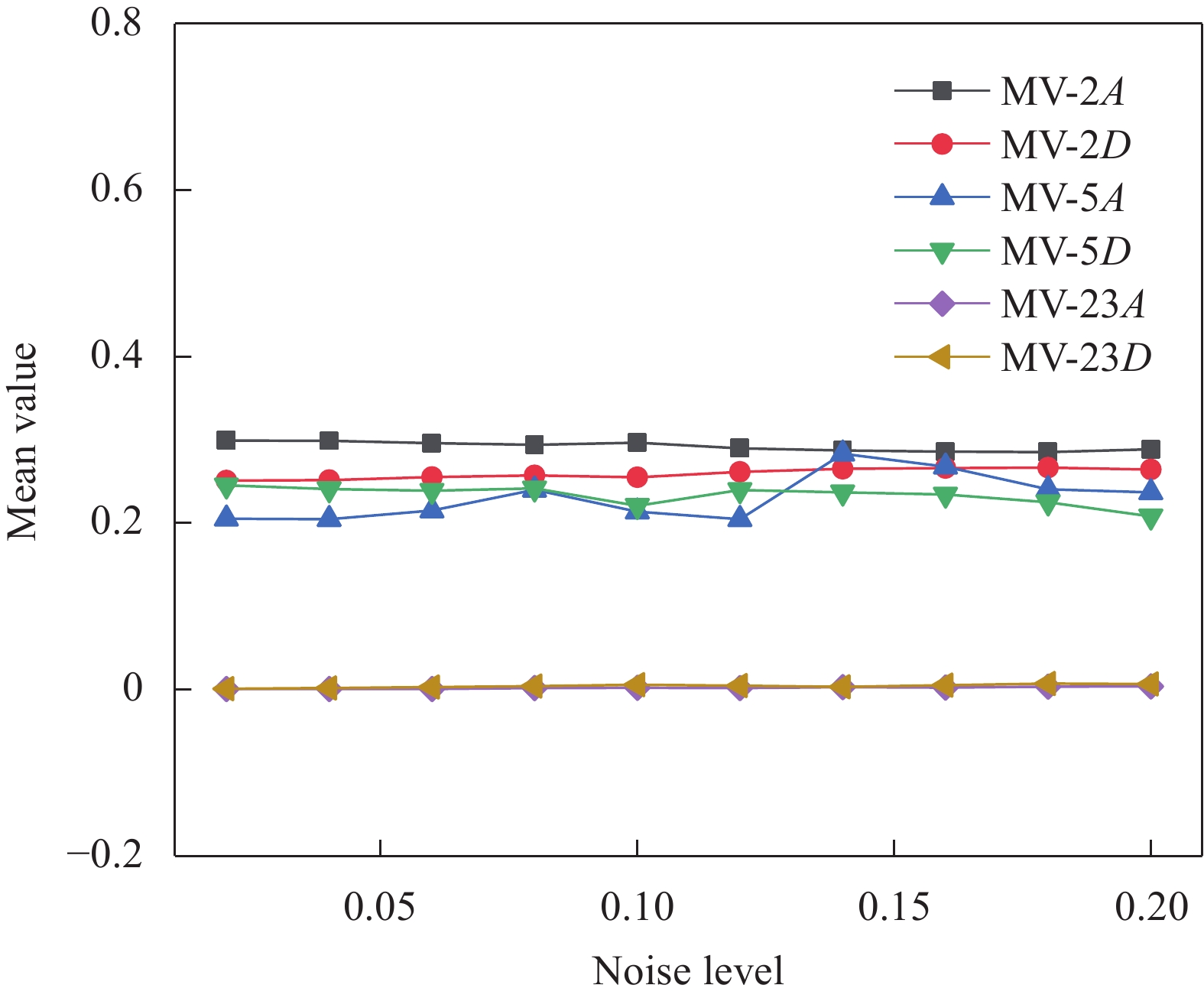
结论
本文针对复合材料层合板结构建立了一类新的损伤指标——单元级损伤指标，该指标既能够反映面内和面外的损伤程度，又具有参数数量少的优势。以材料级损伤参数为基准，本文建立了单元级损伤指标与材料级损伤指标的映射关系，从力学和数学两个角度建立了等效指标。提出了基于单元级损伤参数的复合材料层合板的分步损伤识别流程，即首先利用模态应变能指标进行损伤定位，筛选出损伤单元，其次利用优化方法对损伤单元的损伤程度进行识别。

创新点

由于复合材料层合板结构存在其各向异性和复杂加工工序等原因，导致其具有纤维断裂、基体开裂、剪切失效、分层等多种失效模式。目前存在两种损伤指标来对复合材料层合板的刚度损伤进行定义，一种是传统损伤指标，它采用一个标量来表示单元整体的损伤情况。一种是材料级损伤指标，它对层合板每个单层的各向承载能力损失进行表征。第一种指标具有损伤参数少，识别较为容易的优点，但是能够反映的损伤信息有限；第二类损伤指标能够对复合材料层合板的失效模式进行表征，但是损伤参数数量巨大，识别起来较为困难。基于此，本文提出了单元级损伤指标——层合单元损伤指标，即基于经典层合板理论对层合板单元的面内刚度和面外刚度的折减进行了表征。以壳单元为例，三种损伤指标具有的损伤变量数量对比如下图1所示。从图中可以看出该损伤指标具有损伤参数较少，又能描述层合板结构的损伤状态，能够实现计算效率和损伤完备表征的合理平衡。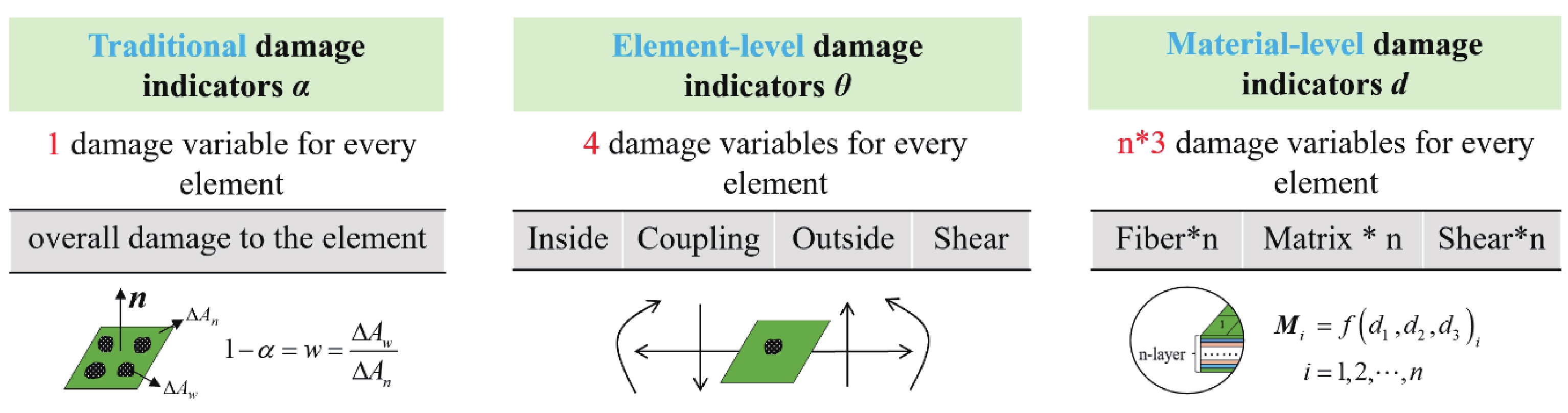
三类损伤指标所需损伤变量个数以及特点
本文基于该损伤指标提出了复合材料层合板的两步损伤识别方法，第一步通过应变能指标差值指标对结构损伤位置进行辨识，第二步通过优化方法对结构的层合单元损伤指标进行定量识别。并对噪声对损伤识别结果误差的影响进行了定量分析，如下图2所示。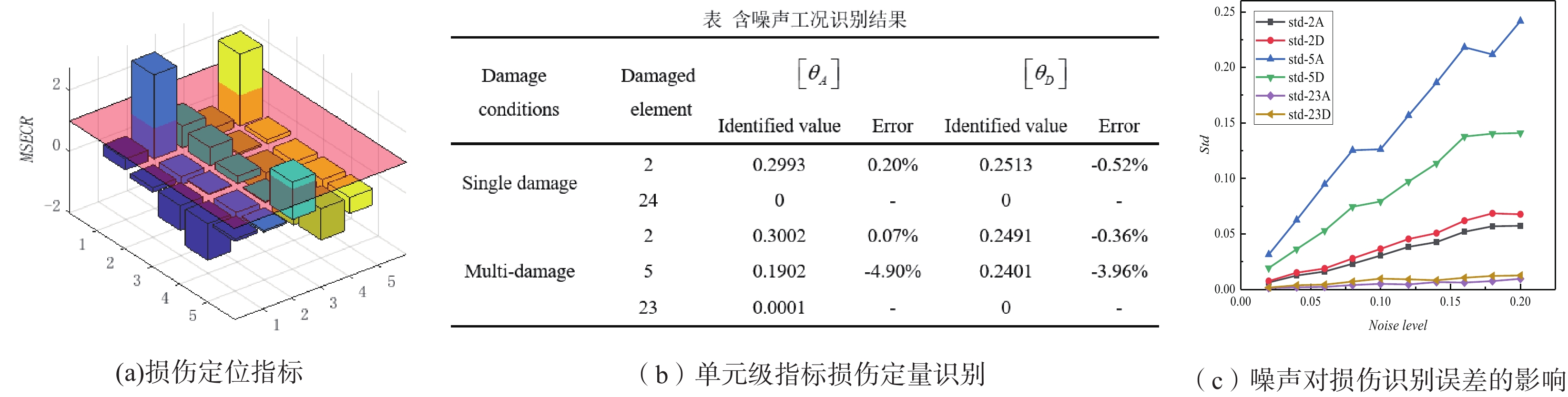
基于层合单元损伤指标的识别流程以及噪声对识别结果误差的影响

图(20) / 表(8)

计量

文章访问数: 711
HTML全文浏览量: 303
PDF下载量: 35
被引次数: 2

1. 实验材料及方法
1.1 实验材料
1.2 多尺度增强体的合成
1.3 PTFE基复合材料的制备
1.4 表征与测试
2. 结果与讨论
2.1 多尺度增强体表征
2.2 复合材料表面性能分析
2.2.1 硬度及晶体结构
2.2.2 接触角与表面能
2.3 复合材料的摩擦学性能
2.3.1 多尺度增强PTFE复合材料的摩擦学性能
一起始摩擦系数分析
二摩擦学性能对比
三磨损面形貌分析
四摩擦磨损机制研究
2.3.2 载荷对复合材料摩擦学行为的影响
2.3.3 往复频率对复合材料摩擦学行为的影响
3. 结论

1. 实验材料及方法
1.1 实验材料
1.2 多尺度增强体的合成
1.3 PTFE基复合材料的制备
1.4 表征与测试
2. 结果与讨论
2.1 多尺度增强体表征
2.2 复合材料表面性能分析
2.2.1 硬度及晶体结构
2.2.2 接触角与表面能
2.3 复合材料的摩擦学性能
2.3.1 多尺度增强PTFE复合材料的摩擦学性能
一起始摩擦系数分析
二摩擦学性能对比
三磨损面形貌分析
四摩擦磨损机制研究
2.3.2 载荷对复合材料摩擦学行为的影响
2.3.3 往复频率对复合材料摩擦学行为的影响
3. 结论

参考文献(27)

施引文献(2)

资源附件(0)

长摘要

创新点

基于单元级损伤指标的纤维增强复合材料层合板损伤识别方法

通讯作者: 石庆贺，博士，讲师，硕士生导师，研究方向为结构健康监测、损伤识别、复合材料结构优化设计 E-mail: shiqinghe34@126.com

计量

出版历程

Damage identification method for fiber-reinforced composite laminates based on element-level damage indicators

1. 实验材料及方法

1.1 实验材料

1.2 多尺度增强体的合成

1.3 PTFE基复合材料的制备

1.4 表征与测试

2. 结果与讨论

2.1 多尺度增强体表征

2.2 复合材料表面性能分析

2.2.1 硬度及晶体结构

2.2.2 接触角与表面能

2.3 复合材料的摩擦学性能

2.3.1 多尺度增强PTFE复合材料的摩擦学性能

一 起始摩擦系数分析

二 摩擦学性能对比

三 磨损面形貌分析

四 摩擦磨损机制研究

2.3.2 载荷对复合材料摩擦学行为的影响

2.3.3 往复频率对复合材料摩擦学行为的影响

3. 结 论

期刊类型引用(1)

其他类型引用(1)

计量

出版历程

目录

1. 实验材料及方法

1.1 实验材料

1.2 多尺度增强体的合成

1.3 PTFE基复合材料的制备

1.4 表征与测试

2. 结果与讨论

2.1 多尺度增强体表征

2.2 复合材料表面性能分析

2.2.1 硬度及晶体结构

2.2.2 接触角与表面能

2.3 复合材料的摩擦学性能

2.3.1 多尺度增强PTFE复合材料的摩擦学性能

一 起始摩擦系数分析

二 摩擦学性能对比

三 磨损面形貌分析

四 摩擦磨损机制研究

2.3.2 载荷对复合材料摩擦学行为的影响

2.3.3 往复频率对复合材料摩擦学行为的影响

3. 结 论

通讯作者:
石庆贺，博士，讲师，硕士生导师，研究方向为结构健康监测、损伤识别、复合材料结构优化设计　E-mail: shiqinghe34@126.com

一起始摩擦系数分析

二摩擦学性能对比

三磨损面形貌分析

四摩擦磨损机制研究

3. 结论

一起始摩擦系数分析

二摩擦学性能对比

三磨损面形貌分析

四摩擦磨损机制研究

3. 结论