Probabilistic residual strength model for composite materials considering stress levels
-
摘要: 针对当前大多数复合材料剩余强度模型通用化程度低、试验成本高的问题，本文提出了一个计及应力水平效应且独立于应力水平的剩余强度概率模型。首先，给出归一化强度储备的定义，并根据归一化强度储备推导出确定性剩余强度模型。然后，将一个疲劳寿命概率模型耦合进确定性剩余强度模型，进而衍生出一个新的剩余强度概率模型。最后，利用文献中的恒幅与变幅剩余强度试验数据对所提出的剩余强度概率模型的准确性和适用性进行验证。结果表明：几乎所有的恒幅试验数据点都分布在预测曲线的95%置信上限与5%置信下限之间，且50%可靠度的预测曲线对试验数据具有高拟合优度值：0.94、0.84及0.97。所提出的模型在充分考虑了复合材料剩余强度统计特征的前提下，仅用一组模型参数即可准确描述多个应力水平下的强度退化。在变幅工况下，所提出模型在升序与降序变幅加载中的预测值与试验值的相对误差均低于6%。Abstract: To address the problems of low generalization and high testing costs of most current residual strength models for composites, a probabilistic residual strength model that accounts for the effect of stress level and is independent of stress level was proposed. Firstly, the normalized strength reserve was defined and a deterministic residual strength model was derived based on the normalized strength reserve. Then, a fatigue life probability model was coupled into the deterministic residual strength model, and then a new residual strength probability model was derived. Finally, the accuracy and applicability of the proposed probabilistic residual strength model was verified using constant-amplitude and variable-amplitude residual strength experimental data from the open literatures. The results show that almost all the constant amplitude experimental data points are distributed between the upper 95% confidence limit and lower 5% confidence limit of the prediction curves, and the prediction curves with 50% reliability have high goodness-of-fit values for the experimental data: 0.94, 0.84 and 0.97. The proposed model accurately describes strength degradation at multiple stress levels using only one set of model parameters, with sufficient consideration of the statistical characteristics of the residual strength of the composite. The relative error between the predicted values of the proposed model and experimental values for both ascending and descending variable-amplitude loading is less than 6%.
-
Keywords:
- composite /
- strength reserve /
- residual strength /
- probability /
- fatigue life /
- constant and variable amplitude /
- stress level
-
基于金属卤化物钙钛矿吸光材料的钙钛矿太阳能电池(Perovskite solar cells，PSCs)，经过十多年的发展，目前已经达到了25.5%的能量转换效率(Power conversion efficiency，PCE)[1-4]，且具有理论上的低成本、易制备等优点，是当前材料、能源领域最为活跃的研究方向之一。根据钙钛矿太阳能电池中功能薄膜的沉积制备顺序，一般可以将其分为正置结构(透明导电基底/电子传输层/钙钛矿层/空穴传输层/顶电极)和倒置结构(透明导电基底/空穴传输层/钙钛矿层/电子传输层/顶电极)两大类。上述功能层之中，钙钛矿吸光层和电子、空穴传输层对PSCs性能的影响最为显著[5]。这些关键功能层的制备和优化可以通过低温溶液法实现。这一方面，有利于PSCs的低成本、简单制备；但另一方面，也意味着这些功能层材料，易于在溶剂环境或高温条件下被破坏。这就对器件制备过程中的最后一步—顶电极的沉积，提出了一些要求。
自从2012年全固态的PSCs结构基本成形以来，大多数研究工作中，都是使用真空蒸镀的Au或Ag薄膜来作为标准的顶电极，以避免沉积过程中引入溶剂和高温环境[2-3]。但由于PSCs中的离子迁移会腐蚀蒸镀金属电极从而破坏器件的稳定性[6]，以及真空设备尺寸放大后的成本、维护问题，研究者们一直关注并探索着适合于大面积制备和大规模应用的PSCs顶电极材料及其制备方法。
电子产业领域，特别是柔性电子领域中的一些低温导电材料，可以为PSCs的顶电极制备提供一些非真空的技术方法。例如，基于弱极性、低沸点溶剂的导电碳浆料或纳米金属导电油墨，对下层功能薄膜的破坏程度较低，可以通过多种易于放大的涂布方式来制备顶电极。实际上，导电碳浆和纳米金属导电油墨分别在无空穴传输层的正置结构器件中和半透明器件中有较多的应用研究。
除了涂布法之外，转移法也是制备顶电极的一种途径。具体而言，转移法制备顶电极是先将合适的材料预制成导电膜，再通过一定的工艺方法，将其转移到仅缺省顶电极层的PSCs半电池结构上，并使其与下层薄膜直接形成良好的界面欧姆接触，即可得到完整的电池器件。理论上，预制成的膜状电极，可以具有比原位沉积制备的顶电极更好的导电性。同时可以在较低的温度、无溶剂或极少量溶剂的条件下实现转移，从而避免制备过程对器件性能的负面影响。
1. 转移法顶电极的介绍
目前，已经有若干种属于不同体系的电极材料，被以多种不同的转移方式，制备成了PSCs的顶电极。将已报道的转移法顶电极技术，按照电极的厚度形态进行分类，可大致上归结为以下两种情况。
其一是，当转移电极为薄膜状态的情况(厚度1微米以下)。此时，电极薄膜往往无法形成自支撑的结构，或者虽然可以实现自支撑，但是难以对其单独进行取样、裁剪、拉平、放置等操作。这种类型具体来说，包括超薄导电聚合物膜[7]、碳纳米管膜[8]、石墨烯膜[9]、金属薄膜[10]、超薄金属箔[11]等电极材料。其工艺流程一般是先将需要转移的电极材料制备或放置于特定的基底上，再利用转移电极与基底、转移电极与器件下层薄膜之间的表面结合力差异，实现从基底到电池上的转移。因此，选用的基底一般是聚四氟乙烯(PTFE)[12]、聚二甲基硅氧烷(PDMS)[7]等低表面能、具有脱模性的材料，或者滤膜这种粗糙多孔、与转移电极接触面积较少的材料[11]，以减少电极与基底之间的结合力。而在转移的过程中，一般要通过基底向电极和器件的接触面施加一定的压力，以实现电极与器件的结合。有时还需要引入加热条件[10]或者少量溶剂的辅助作用[8]，来促进电极/器件界面的形成。由于电极除去基底后自身厚度很小，电极材料与器件下层的界面结合力足以克服电极薄膜自身的起伏状态，使电极服帖。如图1(a)所示，薄膜的转移过程实际上类似于印刷工业中的转印技术。
![]()
图 1 钙钛矿太阳能电池中基于转移法的薄膜顶电极(a)和厚膜顶电极(b)制备流程示意图Figure 1. Schematic diagrams of the transfer process for thin film (a) and thick film (b) top electrode for PSCs其二是，当转移电极为厚膜状态的情况。此时，电极可以自支撑独立存在，并保持原本的状态整体转移到器件上。不同于薄膜电极天然的服帖性，厚膜电极往往需要依赖于自带的、具有压敏性的胶类物质参与电极界面的结合。具体来说，商品导电胶带[13]和一些自制的导电胶膜[14]可以通过这种形式用作顶电极。如图1(b)所示，厚膜的转移过程实际上类似于电子设备生产中的贴合技术。
2. 转移法顶电极的材料
近年来已有多种不同的电极材料被用于转移法顶电极，综合考虑电极的结构、电极的主体材料和电极与器件界面处的材料，将其分为导电聚合物电极、碳材料电极、金属电极和复合电极这4种类型，依次进行介绍。
2.1 导电聚合物电极
聚3,4-乙烯二氧噻吩(PEDOT)是性能最好的导电聚合物材料之一，并常以水分散的聚苯乙烯磺酸盐(PEDOT:PSS)形式，应用在有机太阳能电池(Organic photovoltaics，OPV)和PSCs中作为空穴传输材料和透明电极材料。用于顶电极时，PEDOT:PSS仍然具有透明的效果，可以实现电池的双面光照工作状态，甚至半透明的器件效果。
2015年，华中科技大学的周印华团队将一种OPV中使用的转移法PEDOT:PSS应用于PSCs中。他们将PEDOT:PSS分散液旋涂在PDMS、保鲜膜等基底上成膜，然后转移到OPV[15-16]或者PSCs[7,17]上，最后再剥离基底得到PEDOT:PSS顶电极。在正置结构的钙钛矿太阳能电池中，电极转移前需要对空穴传输层进行等离子体处理[7]或者滴加少量的异丙醇[17]，用来促进PEDOT:PSS薄膜与有机空穴传输材料Spiro-OMeTAD(2,2',7,7'-四[N,N-二(4-甲氧基苯基)氨基]-9,9'-螺二芴)的界面结合。不过由于这种PEDOT:PSS薄膜的方阻较大，当器件的有效面积从0.06 cm2增大到1 cm2时，PCE会从10.1%显著下降到2.9%。这种基于PEDOT:PSS顶电极的器件不仅可以从顶电极方向透光，还可以通过对电极薄膜厚度的调控和选用不同的空穴传输材料，改变薄膜的光学反射特性。由此可实现如图2所示的彩色PSCs，根据颜色的不同，器件PCE在11%到15%的范围内变化[18]。
![]()
图 2 (a)基于转移法聚苯乙烯磺酸盐(PEDOT:PSS)顶电极的钙钛矿太阳能电池结构示意图；(b)彩色钙钛矿太阳能电池照片[18]Figure 2. (a) Device structure of transferred polystyrene sulfonate (PEDOT:PSS) electrode based PSCs; (b) Photographs of colorful PSCs[18]TCO—Transparent conductive oxide; ETL—Electron transporting layer; HTL—Hole transporting layer; PEDOT:PSST—Transferred PEDOT:PSS韩国科学技术院的Park团队[19]也发展了一种干法转移PEDOT:PSS顶电极的技术，并将其应用于柔性的半透明PSCs中。如图3(a)中所示，他们使用带有聚氨酯丙烯酸酯(PUA)涂层的聚碳酸酯(PC)膜作为旋涂制备PEDOT:PSS薄膜的基底，并利用聚乙烯亚胺(PEI)溶液来调控PEDOT:PSS电极的功函数，使其与倒置结构器件相匹配。经过100℃下的压印处理并剥离PUA/PC基底，如图3(b)中照片所示，PEDOT:PSS薄膜就可以转印到器件中，与电子传输材料PCBM(碳60衍生物)之间形成良好的结合。最终得到图3(c)中的柔性半透明倒置PSCs，在1 cm2的较大有效面积上获得超过13%的有效PCE。另外值得一提的是，在这种方法中，PEDOT:PSS薄膜在转移之前已经进行了充分的真空干燥，有利于钙钛矿器件的稳定。
![]()
图 3 干法转印PEDOT∶PSS顶电极的流程图(a)、PEDOT:PSS转印顶电极(b)和半透明钙钛矿电池(c)的照片[19]Figure 3. Schematic diagrams of the dry stamping transfer process of the PEDOT:PSS layer for PSCs (a), photographs of transferred PEDOT:PSS top electrode layer onto the device (b) and semitransparent PSCs (c) [19]PUA—Polyurethane acrylate; PEI—Polyethyleneimine; ITO—Indium tin oxide2.2 碳材料电极
导电碳材料，包括石墨、炭黑、碳纳米管(Carbon nanotube，CNT)、石墨烯等，都在PSCs顶电极领域得到了应用。碳材料固有的化学稳定性和疏水性，有利于实现钙钛矿器件的稳定。
香港科技大学的杨世和团队[20]较早进行了转移法碳电极的研究。他们通过收集蜡烛烟灰得到了海绵状多孔的碳材料，然后将其直接干法转印到钙钛矿薄膜上。经过高温除杂处理蜡烛烟灰碳，并通过10 MPa压强下的辊压转移，可以在无空穴传输层结构的PSCs中得到最高5.4%的PCE。但是研究发现，这种把碳材料转移到钙钛矿表面方法获得的器件性能，劣于将碳材料嵌入钙钛矿层的器件性能，该技术缺少后续的研究报道。
碳纳米管和石墨烯因其优异的力学性能和电学性能，而常用于透明导电基底，特别是柔性透明导电基底的制备。在PSCs领域，也可以将其预制成导电薄膜，并通过转移法制备成顶电极。例如利用化学气相沉积法(CVD)制备碳纳米管，并直接在反应器下游的滤膜上收集，就可以得到CNT薄膜。2014年，新加坡南洋理工大学的Wong和Mathews团队[8]首先将这种CNT薄膜用于PSCs中。他们将这种可自支撑的薄膜直接用镊子从滤膜基底上剥离(图4(a))，并借助甲苯的辅助，转移制备到钙钛矿吸光层上。这种可双面透光的无空穴传输层结构PSCs(图4(b))，可以达到6.3%的PCE。更重要的是，这种由1D材料形成的网络状薄膜，存在着大量的孔隙结构以供填充。他们进一步利用溶液旋涂工艺，将Spiro-OMeTAD填充进CNT薄膜中，得到空穴传输层和顶电极一体化的结构。空穴传输材料Spiro-OMeTAD的引入改善了钙钛矿器件的异质结特性，将器件PCE提升至9.9%。
![]()
此后，这类CNT薄膜材料及其转移工艺，被清华大学、日本东京大学等部分国内外的研究团队继续发展，目前已经应用在了柔性器件、全碳电极器件等多种类型的PSCs中[21-22]。通过对器件的系统性优化，包括使用2D/3D结合的钙钛矿吸光层组分、利用三氟甲磺酸蒸汽对CNT薄膜进行掺杂等，目前这种电极可以实现最高17.6%的PCE[23]。此外，类似于PEDOT:PSS薄膜的情况，可以通过掺杂SnO2、PEI等来调控CNT薄膜的功函数，使其与倒置结构PSCs的电子传输层匹配，相应的倒置器件PCE可以达到14%[24-25]。
作为一种2D材料，石墨烯具有天然的薄膜属性。目前，高质量的石墨烯薄膜一般是在铜箔表面CVD生长出来的，因此在使用的过程中往往需要经过覆盖PMMA聚合物、溶解铜箔、溶解聚合物层等一系列的复杂操作，最后才能将其从刻蚀液中湿法转移到其他基底上。鉴于钙钛矿器件中不便使用湿法转移，一些研究工作通过干法转移，将铜箔上的石墨烯制备到钙钛矿吸光层和Spiro-OMeTAD空穴传输层上，得到可工作的钙钛矿光电器件[26-27]。香港理工大学的严锋团队[9]将铜箔上的石墨烯先转移至PDMS/PMMA基底上，然后在其表面旋涂一层PEDOT:PSS辅助粘结，最后把多层膜整体贴合到正置结构PSCs的Spiro-OMeTAD层上作为顶电极。得到如图5所示的半透明钙钛矿器件，最高PCE可以达到12%。
![]()
单层石墨烯材料具有极高的电导率，但是实际用作电极材料时，其方块电阻大于100 Ω/sq，显然难以满足大面积光伏器件的要求。韩国釜山大学的Lee团队[28]利用铜箔生长的石墨烯和碳纳米管干纺技术，制备了可自支撑的多层混合薄膜，并在氯苯和压力的辅助下将其转移到正置结构的PSCs中。通过调控石墨烯和CNT的层数，将石墨烯/CNT多层混合薄膜的方阻降低到51 Ω/sq，最终得到了超过15%的器件PCE。
2.3 金属电极
金属电极具有导电性好的优点，钙钛矿太阳能电池中常规的金属顶电极，是利用真空环境下的蒸镀、溅射等物理气相沉积工艺制备的。利用预制的金属薄膜材料转移制备顶电极，有望在保有金属电极高性能的前提下，突破真空镀膜设备的尺寸限制、降低设备成本。
基于金属纳米线的网络结构，是一类具有潜在应用价值的透明导电材料。美国斯坦福大学的McGehee团队和Christoforo等[29]一起，将Ag纳米线应用在钙钛矿/晶硅电池(或铜铟镓硒电池)的四端叠层光伏系统中。溶剂分散的Ag纳米线在聚对苯二甲酸乙二酯(PET)塑料基底上喷涂成膜后干燥，再将形成的纳米导电网络转印到正置结构PSCs的Spiro-OMeTAD层上。因为金属纳米线材料的力学特性，转移过程需要在PET上加盖薄载玻片，再用滚珠来施加转印的压力。基于这种电极的半透明钙钛矿器件和相应的叠层光伏系统，可以分别实现12.7%和18.6%的PCE。非贵金属材料的使用，对于PSCs的低成本制备十分重要，但是非贵金属纳米材料的表面配体和氧化层会影响其导电性。韩国延世大学的Moon团队[30]利用喷涂在聚酰亚胺膜上的Cu纳米线@乳酸核壳结构，并在200℃下加热产生还原性氛围，即可获得互联性良好的纯Cu纳米线网络。再同样通过滚珠的压力作用(图6(a))，将Cu纳米导电网络转印至钙钛矿器件中(图6(b))，可获得9.9%的PCE。
![]()
利用预制的蒸镀金属薄膜再转移，是蒸镀金属顶电极工艺直接拓展，且可以通过多次转移来突破蒸镀设备尺寸的限制。但是到目前为止，这方面仅有少量Au薄膜转移制备电极的研究报道。新加坡材料研究与工程研究院的Li和Wang等[10]使用PDMS作为金薄膜的基底，并在90℃下层压两个小时，最后剥离PDMS膜，实现了Au电极在正置结构PSCs上的转移制备以及13.7%的器件PCE。另外值得注意的是，该研究认为相对于蒸镀的金电极，转移法Au电极中的Au原子不容易扩散进下方的功能层，因此具有更好的器件稳定性。武汉理工大学的肖俊彦等[12]则选用脱模性更好的PTFE膜作为转印介质，通过图7所示的室温下辊压方式，将蒸镀在PTFE上的Au层转移至钙钛矿器件上，获得了17.1%的PCE，作为对照组的标准蒸镀Au电极器件PCE为19.3%。
![]()
金的单质或合金材料还具有非常优异的延展性，可以被加工成百纳米级别厚度的超薄箔片。将用于包金装饰的金箔直接转移制备成PSCs的顶电极，可以实现15%的PCE[31]。日本京都大学的Matsuda团队[11]选用了厚度约100 nm的商用Au35Ag65合金箔来制备器件。他们用硝酸腐蚀其中的Ag来实现脱合金化，从而得到多孔结构的Au箔。通过无水乙醇的辅助，将多孔Au箔沉积在钙钛矿器件上，获得了高达19%的PCE(对照的蒸镀Au电极器件PCE为20.4%)。同时，在柔性PSCs中也获得了17.3%的PCE，并且在1000次弯折后(曲率半径为5 mm)保持了初始PCE的98.5%。此外，如图8所示，这种自支撑的多孔Au箔还可以在溶去电池的其他功能层后重复循环使用。
![]()
2.4 复合电极
以上3类电极材料的已报道研究结果，与实际应用还有着较远的距离。具体而言，有如下几点问题：导电聚合物、石墨烯电极的导电性能难以满足大面积器件的需求；高质量石墨烯、碳纳米管薄膜、金薄膜的材料成本高；非贵金属纳米材料在钙钛矿器件中的化学稳定性存疑。而基于多种材料体系复合而成的顶电极，理论上可以结合这3类材料的优点，例如金属的高导电性、导电聚合物的空间连续性和碳材料的化学稳定性。同时，还可以利用非导电性的粘合剂，来增强顶电极和器件下层材料之间的界面结合。这类复合电极通常是由高导电性的基底和含有导电性填料与粘合剂的导电胶层构成的，总厚度达到数十微米，可以直接通过压力贴合的方式制备到到器件上。
实际上，工业上用于电子连接或实验室用于电镜制样的导电胶带，就具有这种复合电极的特性。美国内布拉斯加-林肯大学的黄劲松团队[13]将一种电镜使用的导电胶带用在了钙钛矿太阳能电池中。这种胶带使用Cu箔作为基底，方阻低至0.001 Ω/sq，并使用微米金属Ni颗粒和丙烯酸压敏胶作为导电胶层，Z轴方向电阻低至0.005 Ω/in。通过70℃加热软化丙烯酸胶，并控制辊压贴合工艺的压强，他们把导电胶带制备在倒置结构PSCs的电子传输层PCBM上，可以达到超过12%的PCE。
由于目前的商品导电胶带并不是为PSCs设计的，在贴合的过程中，较低的操作压强会降低电极的有效接触面积，而过大的操作压强则可能导致金属导电颗粒刺穿器件的下层结构。利用PEDOT:PSS作为导电填料的一些实验室自制导电胶膜，可以更好地与钙钛矿器件匹配。英国斯旺西大学的Worsley团队[14]使用嵌有金属Ni微网的PET薄膜作为导电基底，1.75%的PEDOT:PSS与丙烯酸压敏胶混合作为导电胶层，制备了一种透明导电胶膜(图9(a)~9(b))。他们在钙钛矿器件的Spiro-OMeTAD层上喷涂一种几乎不含水PEDOT:PSS以降低界面电阻，再通过简单的手指按压，就完成了这种透明复合顶电极的制备。在如图9(c)所示的器件结构里，PCE可以达到15.5%，其光电流密度(21 mA/cm2)甚至高于作为对比的标准Au电极器件(19 mA/cm2)。在他们的后续研究中，进一步将这种电极用于Ti箔基底的PSCs上作为透明顶电极，得到了PCE为10.3%的柔性PSCs[32]。德国埃尔朗根-纽伦堡大学的Brabec团队与其合作者[33]以AgNWs/PET为导电基底，用PEDOT:PSS和40%的山梨糖醇混合制备导电胶层，应用在OPV和PSCs中。其中对于倒置结构的钙钛矿器件，可以通过60℃下的压力贴合，得到9.8%的PCE。值得一提的是，他们还将这种电极用于激光刻蚀加工过的小面积(30 mm2)PSCs串联组件中，组件与单块电池的性能相当，这对于未来的实际应用有重要的参考价值。
![]()
石墨、炭黑等廉价导电碳材料，一般需要与粘合剂混合后制成导电碳浆使用，因此把基于碳浆的电极分类为复合电极。具体而言，除了涂布在器件中再原位干燥制备成电极之外，也可以把碳浆预先涂布在其他基底上干燥成膜后，再转移制备成顶电极。2015年，中国科学院物理研究所的孟庆波团队[34]首先报道了一种自支撑的热塑性导电碳膜电极。他们利用热塑性粘合剂和导电碳粉混合成的碳浆，刮涂在PTFE上，干燥后将其从基底上剥离即得到热塑性碳膜。将无空穴传输层的半电池、碳膜和铝箔一起热压贴合，可以实现13.5%PCE的无空穴传输层结构PSCs。为了避免热压过程对器件中Spiro-OMeTAD材料的影响，他们2018年又报道了一种具有常温下自粘性的导电碳膜技术。基于商品导电碳浆和溶剂置换干燥处理(图10(a)~10(b))，得到具有微观大孔结构和一定压敏粘性的碳膜，再将其压力转移到常规的正置结构PSCs中，PCE可达到19.2%(对照的蒸镀Au电极器件PCE为20%)[35]。溶剂置换干燥法在提供表面粘性的同时，使得这种自粘性碳膜的导电性明显下降，因此难以在大面积的器件中应用。武汉理工大学的肖俊彦等[36-37]尝试将多种高导电性基底与自粘性碳膜结合，以提高大面积转移电极的性能。他们发现，具有一定可压缩性的石墨纸基底和高度柔性的导电布基底性能较好，分别在大面积(1 cm2)的刚性和柔性PSCs中可获得17%和14%以上的转换效率。虽然这类碳膜电极缺少蒸镀Au电极那样的光学反射特性，但可以与器件的Spiro-OMeTAD层之间实现高质量的界面接触(图10(c))，所以能够达到与标准Au电极器件非常接近的性能。
![]()
对于适用转移法的复合电极材料，粘合剂其实并不总是必需的。大连理工大学的史彦涛团队[39]发展了一类顶电极可拆卸的堆叠式钙钛矿太阳能电池。他们使用PEDOT:PSS[38]和多种碳材料粉末，同时喷涂在正置结构半电池和导电玻璃、金属箔等导电基底上形成几微米厚的涂层。再将半电池和导电基底的涂层面直接堆叠组装，得到可正常工作的电池器件(图11)。这种顶电极的转移和使用方式非常类似于染料敏化太阳电池中的对电极。在传统的染料敏化太阳电池中，是依靠液态电解质的流动性来实现固-液界面的有效电接触；而在这种堆叠式钙钛矿电池中，良好的固-固界面电接触是依靠导电聚合物和碳材料的适当弹塑性来实现的[40]。经过优化，基于掺杂PEDOT:PSS和石墨烯基碳材料粉末作为顶电极接触材料的堆叠式器件，PCE分别可以达到14.6%和18.6%(对照的蒸镀Ag电极器件PCE为19.4%)，而且经过数百次的反复拆卸和组装过程，器件性能能够一直基本保持不变。此外，高导电性基底的引入，还可以解决PEDOT:PSS和石墨烯薄膜材料电阻较大的问题，相应的平方厘米级大面积钙钛矿器件PCE可达到13.5%和16.4%。
![]()
3. 转移法顶电极的展望
综上所述，多种类型的电极材料都可以通过转移法作为正置结构和倒置结构、刚性器件和柔性器件、透明电池和不透明电池、单块器件和串联组件的顶电极应用在钙钛矿太阳能电池中(表1)。这些电极材料的转移工艺具有操作简单、不受真空设备尺寸限制、对器件下层结构影响小等优点，为高性能器件的大面积制备技术中的顶电极部分，提供了一种解决方案。
表 1 几种代表性的转移法顶电极技术总结Table 1. Summary of several transferred top electrode techniquesTop electrode Substrate Transfer process PCE/% Device Ref. PEDOT:PSS Plastic wrap
(peel off)Assisted with one drop of isopropanol 10.1
2.9 lConventional
Semitransparent[17] PEDOT:PSS PDMS(peel off) Assisted with O2 plasma for 5 s 15.1 Conventional
Colorful[18] PEI doped PEDOT:PSS PUA/PC
(peel off)Roll press under 100℃ 13.6 f, l Inverted
Semitransparent[19] Doped CNT film Membrane film(peel off) Assisted with 200 μL spiro-OMeTAD 17.56 Conventional [23] CNT film Si wafer Assisted with droplets of chlorobenzene 11.9 f Conventional [21] CNT film Si wafer Assisted with 100 μL PEI-isopropanol solution 10.8 Inverted [25] Graphene/
PEDOT:PSSPDMS/PMMA Roll press under 65℃ 12.37 Conventional
Semitransparent[9] Ag nanowire film PET(peel off) Ball bearing press under 500 g force 12.7 Conventional
Semitransparent[29] Au PTFE(peel off) Roll press 17.14 Conventional [12] Nanoporous Au Membrane film(peel off) Assisted with 200 μL anhydrous ethanol 19.0
17.3 fConventional [11] Ni particles in acrylic Cu foil Roll press under 70℃, 980 Pa 12.5 Inverted [13] PEDOT:PSS in acrylic Ni mesh embedded PET Press onto PEDOT:PSS covered semi-cell 15.5 Conventional
Semitransparent[14] Carbon paste film Flat press under 0.7 MPa 19.2 Conventional [35] Carbon paste film Conductive cloth Flat press 19.36
14.05 f, lConventional [37] PEDOT:PSS Al foil, Cu foil, FTO etc. Stack with PEDOT:PSS covered semi-cell 14.6 Conventional [38] Graphene Al foil, FTO Stack with graphene covered semi-cell 18.65
16.42 lConventional [40] Notes: Upper script "f" represents flexible device, and "l" represents large device area about 1 cm2; PDMS, PC, PET, PMMA, PTFE are abbreviations for common polymer materials. 在未来的研究中，转移法顶电极还有一些材料、工艺方面问题，需要更深入地去探索并解决。
(1) 转移法顶电极器件的能量转换效率还需要提升。目前小面积PSCs的认证PCE已经超过了25%，而基于转移法顶电极的钙钛矿器件，文献报道的最佳结果还未达到20%。为此，首先需要将转移法顶电极技术与钙钛矿吸光层和电荷传输层的最新进展结合使用。如果能够采用标准蒸镀Au电极的器件PCE超过22%的半电池，多孔金箔、自粘性大孔碳膜、石墨烯基堆叠式等高效率型的顶电极技术应该可以实现20%以上的PCE。其次，需要基于转移法顶电极的特性，对下层半电池进行优化。目前的透明和碳基非透明型转移法顶电极，往往缺少光学反射特性，如果能够通过调控吸光层、引入陷光结构等方式增强对入射光的利用，那么转移法顶电极器件与标准蒸镀Au电极器件之间的性能差距应该可以进一步缩小。
(2) 转移法顶电极器件的稳定性需要得到检验。稳定性问题可以算是金属卤化物钙钛矿材料和PSCs应用中的最大阻碍，而转移法顶电极器件的稳定性问题还未有详细的讨论。为此，在材料层面上，需要研究电极材料本身及其转移法工艺应用在器件后的化学稳定性问题，例如PEDOT:PSS材料含水的影响、金属材料化学反应和离子迁移的影响等。在器件层面上，则需要关注转移法顶电极与下层薄膜的界面稳定性问题，例如在光照、电场、变温、弯折等条件下，界面能否长时间保持有效电接触。此外，目前几种高效率的转移法顶电极技术，都是基于以Spiro-OMeTAD为空穴传输层的正置结构PSCs的，因此还需要验证这些顶电极技术与更稳定的倒置结构器件、聚合物基正置结构器件的匹配性。
(3) 转移法顶电极的成本需要进行合理的核算。包括转移法在内的各种替代蒸镀贵金属电极技术，最吸引人的就是成本优势，但是目前缺少严格的成本分析。例如，转移法中的大面积石墨烯、碳纳米管薄膜、多孔金箔、金属纳米线等电极材料都不是通用材料，需要评估大规模使用中的成本。而对于复合电极，除了各种原材料的成本，还要计算预制备过程的成本和损耗。总之，最终应该通过核算单位面积下的各种顶电极技术成本，而非单位质量下的各种电极材料价格，来分析技术在成本上的可行性。
(4) 转移法顶电极的制备需要实现标准化、自动化。目前已报道的转移法顶电极技术，缺少不同团队之间的相互验证，甚至在同一团队内也难以保证研究的延续性。这里一个可能的原因是，已报道的转移法顶电极技术，往往高度依赖于个人操作，导致转移过程的温度、压强及其实现方法因人而异。所以不仅实验的重现性没有保障，而且操作简单的优点也只是停留在理论上。为此，需要更多地利用通用性的设备来制备电极材料、转移顶电极，以实现整个工艺流程的标准化和自动化。对这方面的研究探索将有助于解决转移法顶电极在大面积、串联组件中的应用问题，甚至实现电极-封装一体化的功能，推动整个钙钛矿太阳能电池领域的发展。
-
![]()
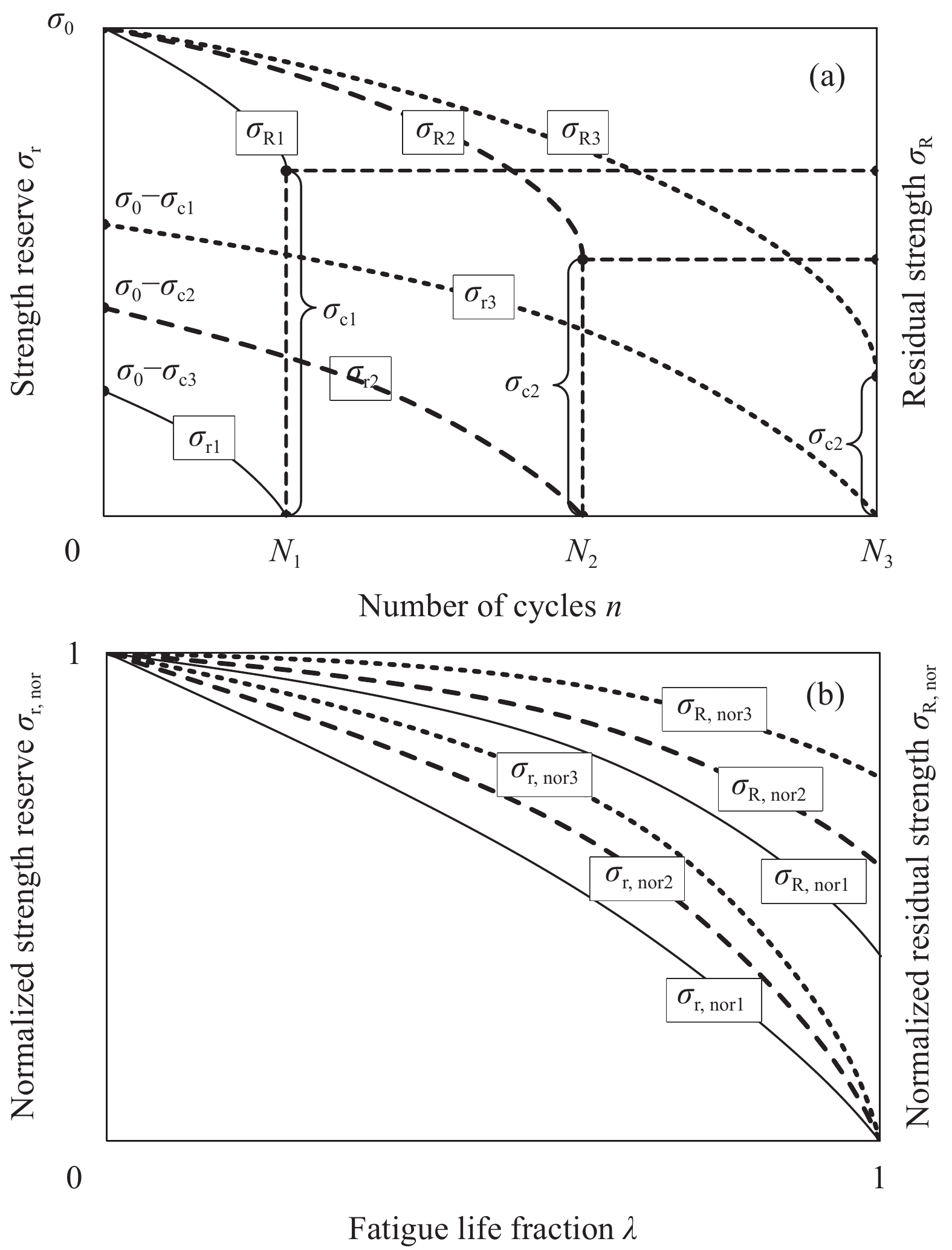
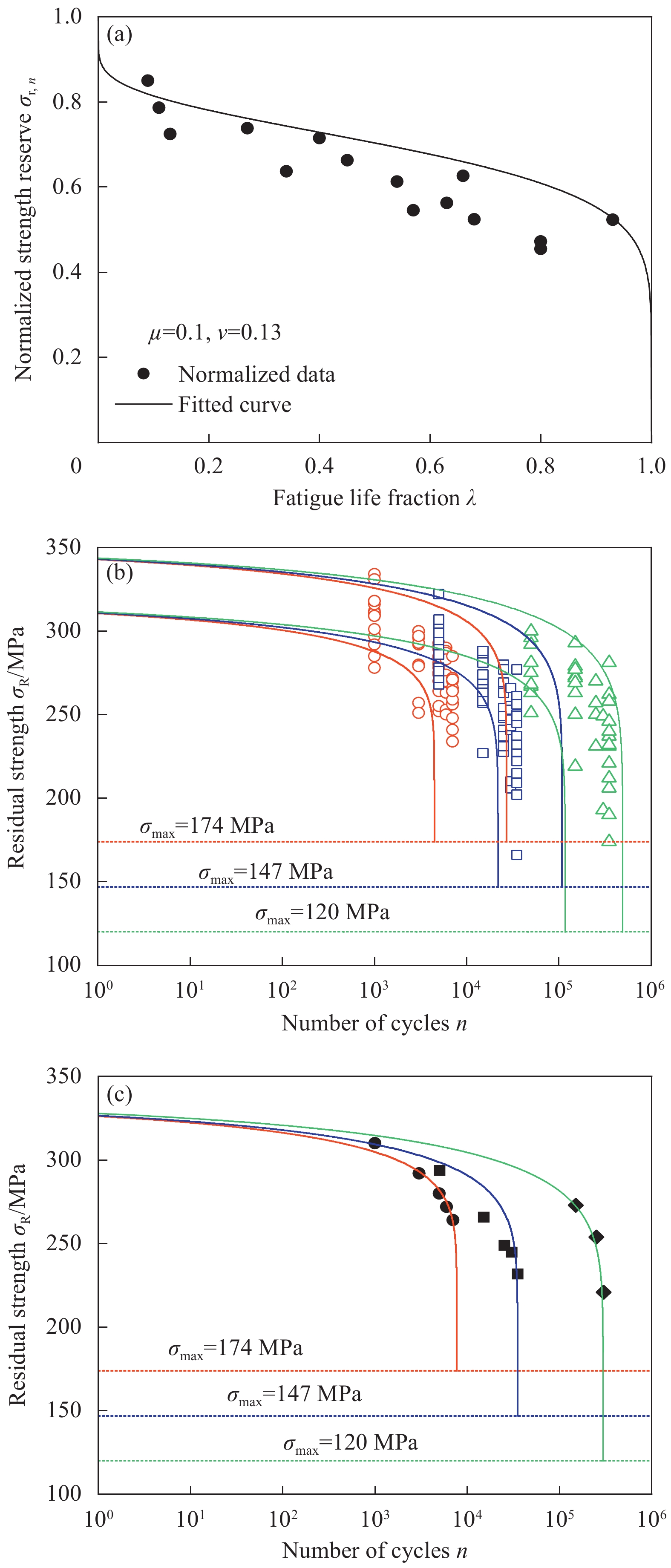
图 1 归一化强度储备的概念：(a) 剩余强度与强度储备；(b) 归一化剩余强度与归一化强度储备
Figure 1. Concept of normalized strength reserve: (a) Residual strength and strength reserve; (b) Normalized residual strength and normalized strength reserve
σ0—Tensile static strength; σc—Critical strength, numerically equal to the peak stress; N—Fatigue life
![]()
图 2 两个连续载荷块的加载示意图
Figure 2. Loading diagram of two continuous load blocks
σmax,i—Peak stress of the ith load block; σmax,i+1—Peak stress of the (i+1)th load block; ni—Cycle counts of the ith load block; ni+1—Cycle counts of the (i+1)th load block
![]()
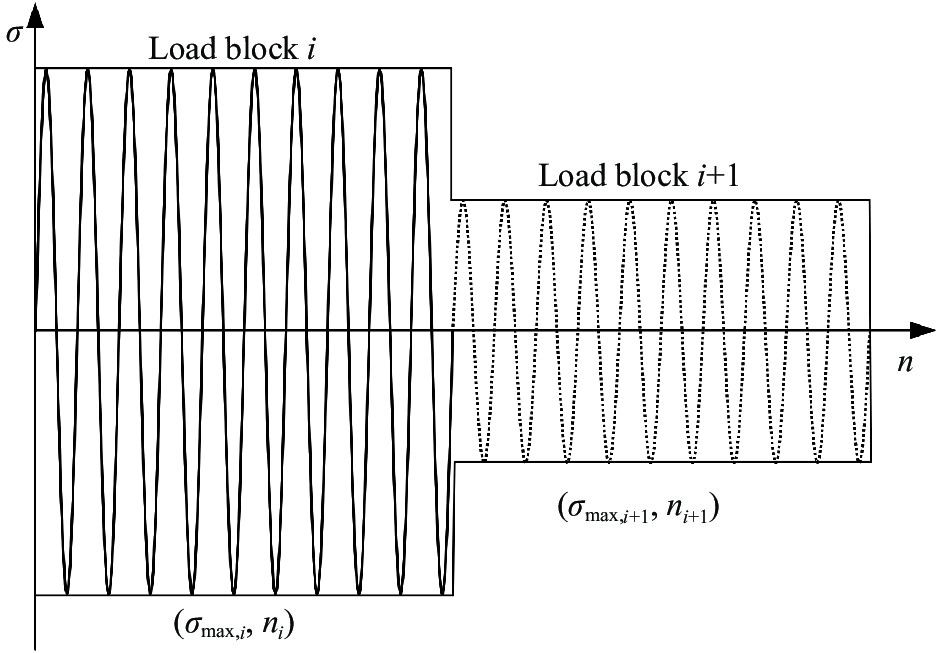
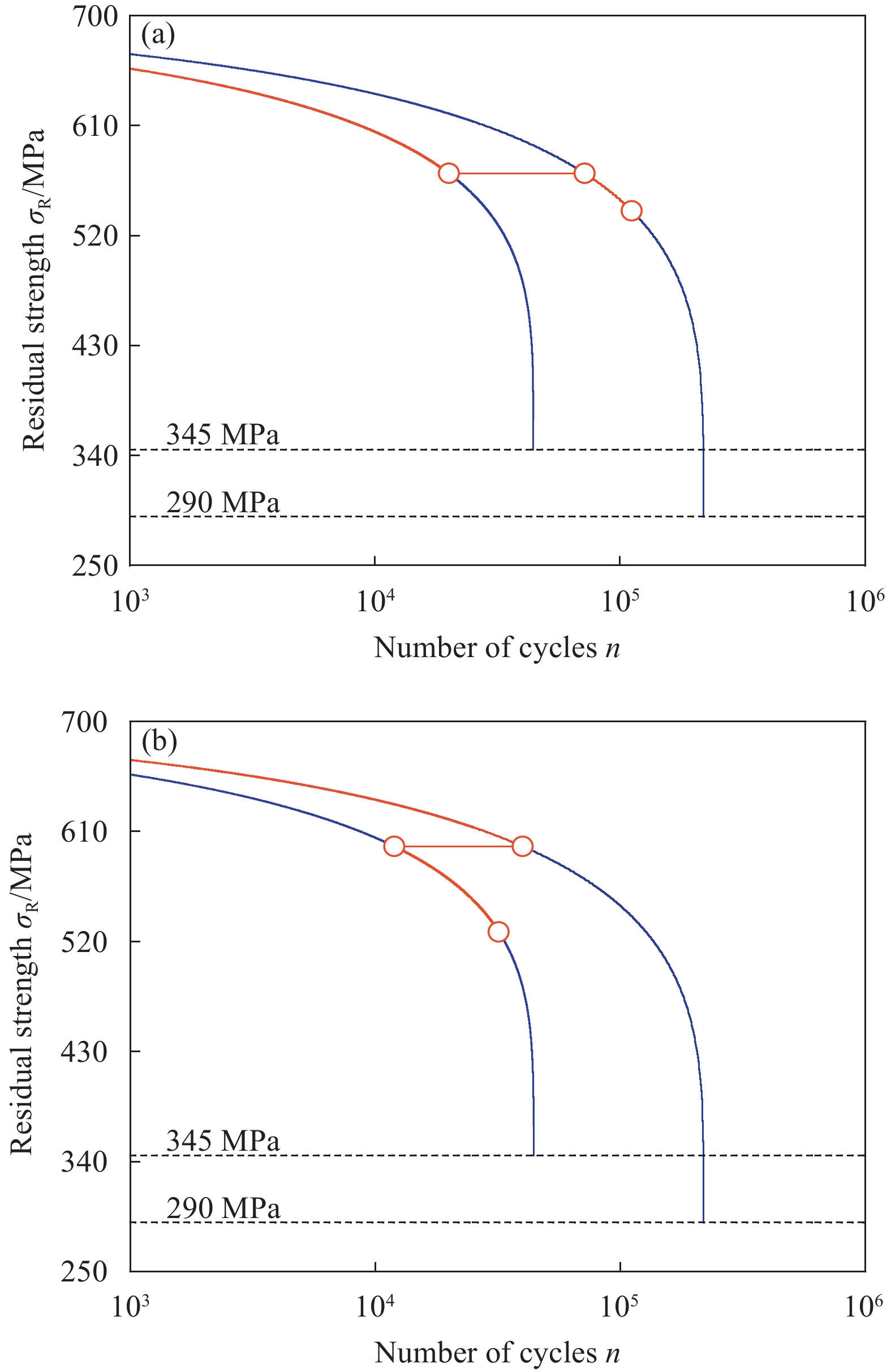
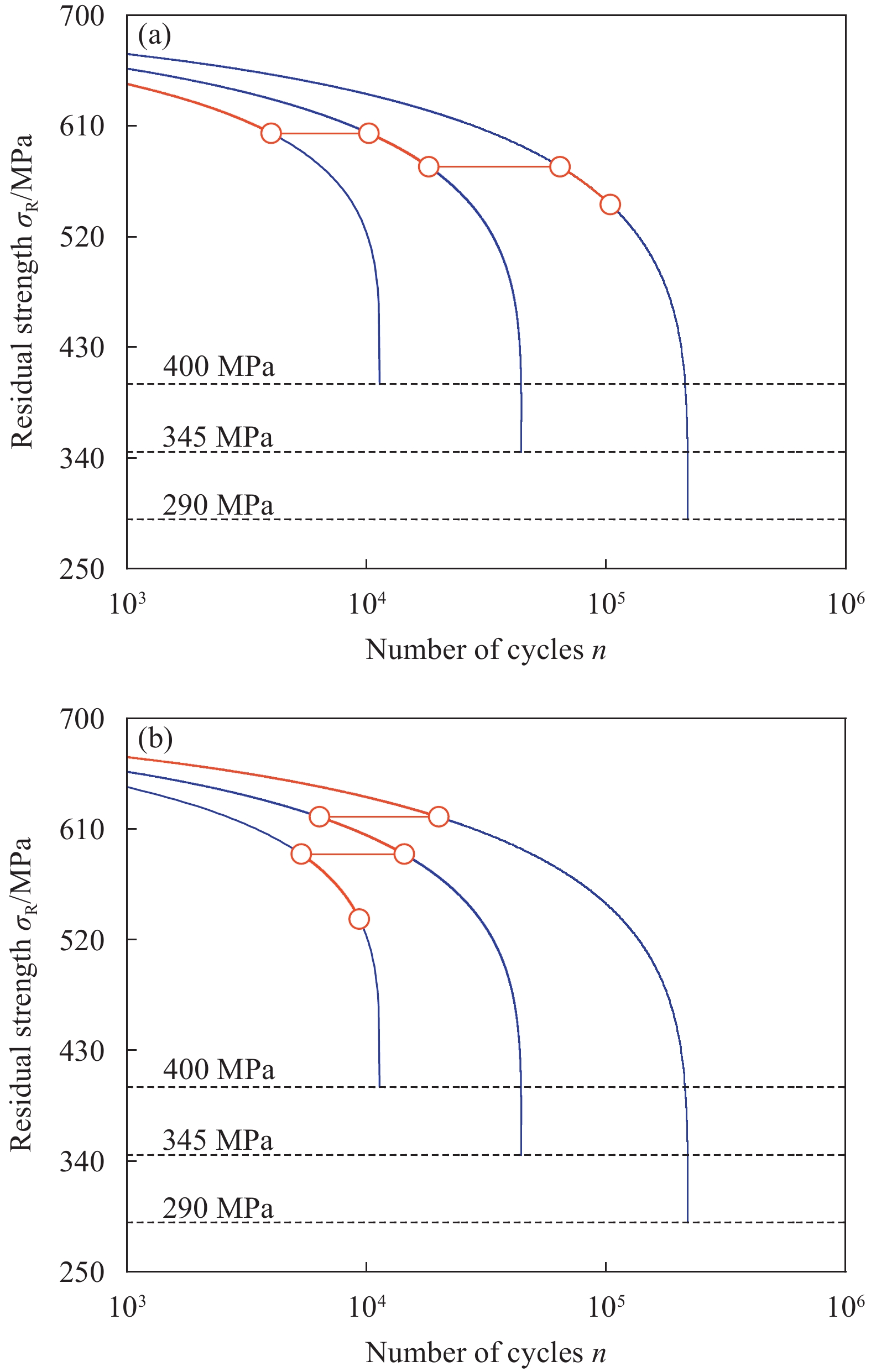
图 3 变幅加载下的剩余强度退化路径：(a) 低-高 (L-H)；(b) 高-低 (H-L)
Figure 3. Residual strength degradation paths under variable amplitude loading: (a) Low-high (L-H); (b) High-low (H-L)
mi—Number of stress cycles in the first load block; mi+1,i—Equivalent number of cycles of the first load block mi in the second load block
![]()
![]()
![]()
图 6 模型参数拟合与VARTM E-玻璃/乙烯基酯的剩余强度预测曲线：(a) 归一化强度储备拟合曲线与试验数据[22]；(b) 置信区间为5%~95%的剩余强度预测区间与试验数据[22]；(c) 失效概率为50%的剩余强度预测曲线与试验数据[22]
Figure 6. Model parameter fitting and residual strength prediction curves for VARTM E-glass/vinyl ester: (a) Normalized strength reserve fitting curve vs. experimental data[22]; (b) Residual strength prediction band with confidence interval of 5%-95% vs. experimental data[22]; (c) Residual strength prediction curves with 50% failure probability vs. experimental data[22]
μ, ν—Model parameters for the normalized strength reserve model
![]()
图 7 二级应力水平变幅加载的T300/934石墨/环氧树脂剩余强度预测：(a) 高-低；(b) 低-高
Figure 7. Residual strength prediction of T300/934 graphite/epoxy under variable amplitude loading containing 2 stress levels: (a) H-L; (b) L-H
![]()
图 8 三级应力水平变幅加载的T300/934石墨/环氧树脂剩余强度预测：(a) 高-低；(b) 低-高
Figure 8. Residual strength prediction of T300/934 graphite/epoxy under variable amplitude loading containing 3 stress levels: (a) H-L; (b) L-H
![]()
图 9 22级应力水平加载的VARTM E-玻璃/乙烯基酯剩余强度预测曲线与试验数据[22]：(a) 50%失效概率的剩余强度预测；(b) 5%失效概率的剩余强度预测
Figure 9. Residual strength prediction curves of VARTM E-glass/vinyl ester for 22 stress level loading vs. experimental data[22]: (a) Residual strength prediction for 50% probability of failure; (b) Residual strength prediction for 5% probability of failure
表 1 铺层顺序为[0/+45/90/−45/0]s的VARTM E-玻璃/乙烯基酯的静拉伸强度[22]
Table 1 Static tensile strength of VARTM E-glass/vinyl ester with a lay-up sequence of [0/+45/90/−45/0]s[22]
Test number σ0/MPa Test number σ0/MPa 1 338 11 343 2 342 12 333 3 350 13 333 4 333 14 327 5 321 15 323 6 328 16 338 7 326 17 331 8 353 18 332 9 341 19 341 10 332 20 321 
下载: 导出CSV
表 2 铺层顺序为[0/45/90/−452/90/45/0]2的T300/934石墨/环氧树脂的静拉伸强度[26]
Table 2 Static tensile strength of T300/934 graphite/epoxy with a lay-up sequence of [0/45/90/−452/90/45/0]2[26]
Test number σ0/MPa Test number σ0/MPa 1 427.49 14 481.96 2 444.04 15 481.96 3 445.42 16 486.79 4 445.42 17 486.79 5 449.55 18 491.61 6 455.07 19 492.30 7 455.07 20 495.06 8 466.10 21 496.44 9 468.86 22 500.58 10 477.82 23 503.33 11 477.82 24 511.61 12 477.80 25 519.88 13 480.58
下载: 导出CSV
表 3 T300/934石墨/环氧树脂的非线性累积损伤与线性累积损伤的对比[7]
Table 3 Non-linear cumulative damage of T300/934 graphite/epoxy in comparison to linear cumulative damage[7]
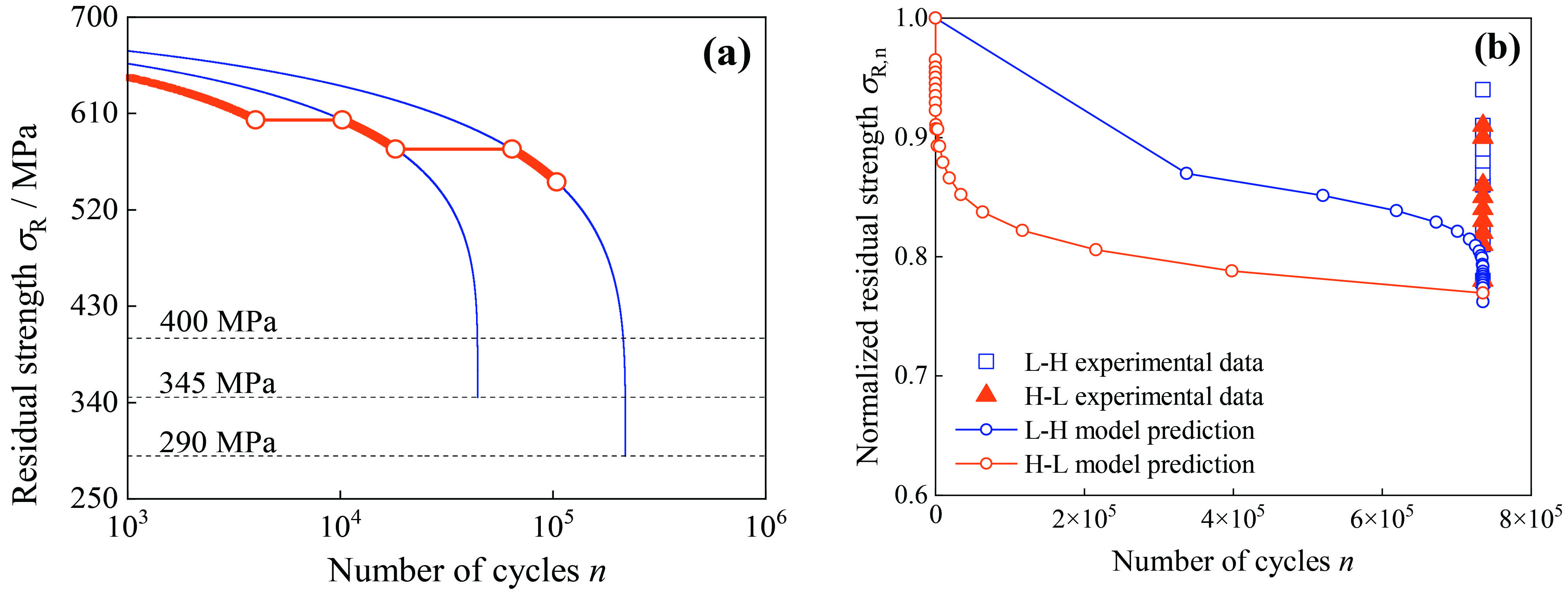
σ1 σ2 σ3 n1 n2 n3 Ref. [7] Eq.(14) Eq.(15) H-L 345 290 — 20000 40000 — 0.76 0.63 0.45 L-H 290 345 — 40000 20000 — 0.67 0.63 0.50 H-L 400 345 290 4000 8000 20000 0.86 0.62 0.48 L-H 290 345 400 20000 8000 4000 0.70 0.62 0.46 Notes: σ1—Peak stress of the first loading; σ2—Peak stress of the second loading; σ3—Peak stress of the third loading; n1—Number of cycles for the first loading; n2—Number of cycles for the second loading; n3—Number of cycles for the third loading.
下载: 导出CSV
表 4 VARTM E-玻璃/乙烯基酯的变幅测试载荷谱[22]
Table 4 Load spectra for variable amplitude testing of VARTM E-glass/vinyl ester[22]
Load cycle Stress/MPa Load cycle Stress/MPa 337535 74 394 157 182664 81 213 150 98852 88 115 164 53496 95 62 170 28950 102 34 177 15667 109 18 183 8478 116 10 190 4588 123 5 196 2483 130 3 203 1344 144 2 209 727 137 1 216
下载: 导出CSV
表 5 两个算例(VARTM E-玻璃/乙烯基酯和T300/934石墨/环氧树脂)涉及的模型参数
Table 5 Model parameters involved in the two cases (VARTM E-glass/vinyl ester and T300/934 graphite/epoxy)
α β γ δ μ ν Case 1 0.0544 0.1730 490 20 0.2 0.25 Case 2 0.1909 0.1932 340.6 20.99 0.1 0.13 Notes: α, β—Model parameters of the S-N curve; γ—Scale parameter of the Weibull distribution of static strength; δ—Shape parameter of the Weibull distribution of static strength.
下载: 导出CSV
-
[1] SHABANI P, TAHERI-BEHROOZ F, SAMAREH-MOUSAVI S S, et al. Very high cycle and gigacycle fatigue of fiber-reinforced composites: A review on experimental approaches and fatigue damage mechanisms[J]. Progress in Materials Science,2021,118:100762. DOI: 10.1016/j.pmatsci.2020.100762
[2] 吴涛, 姚卫星, 黄杰. 纤维增强树脂基复合材料超高周疲劳研究进展[J]. 材料导报, 2022, 36(6):214-222. WU Tao, YAO Weixing, HUANG Jie. Recent development of research on very high cycle fatigue of fiber reinforced plastic[J]. Materials Reports,2022,36(6):214-222(in Chinese).
[3] 程小全, 杜晓渊. 纤维增强复合材料疲劳寿命预测及损伤分析模型研究进展[J]. 北京航空航天大学学报, 2021, 47(7):1311-1322. DOI: 10.13700/j.bh.1001-5965.2020.0229 CHEN Xiaoquan, DU Xiaoyuan. Research development of fatigue life prediction and damage analysis model of fiber-reinforced composite[J]. Journal of Beijing University of Aeronautics and Astronautics,2021,47(7):1311-1322(in Chinese). DOI: 10.13700/j.bh.1001-5965.2020.0229
[4] WU F Q, YAO W X. A fatigue damage model of composite materials[J]. International Journal of Fatigue,2010,32(1):134-138. DOI: 10.1016/j.ijfatigue.2009.02.027
[5] 李顶河, 李梁轶, 郭巧荣, 等. 基于离散损伤模型的复合材料双悬臂梁分层疲劳扩展分析[J]. 复合材料学报, 2022, 39(7):3603-3615. LI Dinghe, LI Liangyi, GUO Qiaorong, et al. Fatigue delamination analysis of composite double cantilever beams based on discrete damage zone model[J]. Acta Materiae Compositae Sinica,2022,39(7):3603-3615(in Chinese).
[6] HUANG J Z, GUO L C, CHEN L L, et al. Damage evolution of 3D woven carbon/epoxy composites under the tension-compression fatigue loading based on multi damage information[J]. International Journal of Fatigue,2022,154:106566. DOI: 10.1016/j.ijfatigue.2021.106566
[7] D'AMORE A, GRASSIA L. A method to predict the fatigue life and the residual strength of composite materials subjected to variable amplitude (VA) loadings[J]. Composite Structures,2019,228:111338. DOI: 10.1016/j.compstruct.2019.111338
[8] 高建雄, 安宗文, 白学宗. 随机载荷下风电叶片复合材料剩余强度概率模型[J]. 太阳能学报, 2018, 39(8):2169-2175. GAO Jianxiong, AN Zongwen, BAI Xuezong. Probabilistic model of residual strength of composite materials for wind turbine bldae under random load[J]. Acta Energiae Solaris Sinica,2018,39(8):2169-2175(in Chinese).
[9] D'AMORE A, GIORGIO M, GRASSIA L. Modeling the residual strength of carbon fiber reinforced composites subjected to cyclic loading[J]. International Journal of Fatigue,2015,78:31-37. DOI: 10.1016/j.ijfatigue.2015.03.012
[10] MA H D, MA Q, BAI X Z, et al. A material property degradation model of composite laminates considering stress level[J]. Mechanics of Advanced Materials and Structures, 2023, 30(2): 272-283.
[11] CHOU P C, CROMAN R. Residual strength in fatigue based on the strength-life equal rank assumption[J]. Journal of Composite Materials,1978,12(2):177-194. DOI: 10.1177/002199837801200206
[12] BROUTMAN L, SAHU S. A new theory to predict cumula-tive fatigue damage in fiber glass reinforced plastics[M]. West Conshohocken: ASTM International, 1972: 170-188.
[13] FANG G W, GAO X G, ZHANG S, et al. A residual strength model for the fatigue strengthening behavior of 2D needled CMCs[J]. International Journal of Fatigue,2015,80:298-305. DOI: 10.1016/j.ijfatigue.2015.06.019
[14] GANESAN C, JOANNA P. Modeling the residual strength and fatigue life of carbon fiber composites under constant amplitude loading[J]. Mechanics of Advanced Materials and Structures,2020,27(21):1840-1848. DOI: 10.1080/15376494.2018.1526353
[15] STOJKOVIĆ N, FOLIĆ R, PASTERNAK H. Mathematical model for the prediction of strength degradation of composites subjected to constant amplitude fatigue[J]. International Journal of Fatigue,2017,103:478-487. DOI: 10.1016/j.ijfatigue.2017.06.032
[16] 赵晟, 张继文. 一种基于复合材料剩余强度的衍生疲劳损伤模型[J]. 复合材料学报, 2020, 37(10):2473-2481. ZHAO Sheng, ZHANG Jiwen. A derivative fatigue damage model based on residual strength of composite[J]. Acta Materiae Compositae Sinica,2020,37(10):2473-2481(in Chinese).
[17] HALPIN J C, JOHNSON T A, WADDOUPS M E. Kinetic fracture models and structural reliability[J]. International Journal of Fracture Mechanics,1972,8(4):465-468. DOI: 10.1007/BF00191112
[18] TSERPES K I, PAPANIKOS P, LABEAS G, et al. Fatigue damage accumulation and residual strength assessment of CFRP laminates[J]. Composite Structures,2004,63(2):219-230. DOI: 10.1016/S0263-8223(03)00169-7
[19] PASSIPOULARIDIS V A, PHILIPPIDIS T P. Strength degradation due to fatigue in fiber dominated glass/epoxy composites: A statistical approach[J]. Journal of Composite Materials,2009,43(9):997-1013. DOI: 10.1177/0021998308097738
[20] SHOKRIEH M M, LESSARD L B. Multiaxial fatigue behaviour of unidirectional plies based on uniaxial fatigue experiments—I. Modelling[J]. International Journal of Fatigue,1997,19(3):201-207. DOI: 10.1016/S0142-1123(96)00074-6
[21] POST N L, CASE S W, LESKO J J. Modeling the variable amplitude fatigue of composite materials: A review and evaluation of the state of the art for spectrum loading[J]. International Journal of Fatigue,2008,30(12):2064-2086. DOI: 10.1016/j.ijfatigue.2008.07.002
[22] POST N, CAIN J, MCDONALD K, et al. Residual strength prediction of composite materials: Random spectrum loading[J]. Engineering Fracture Mechanics,2008,75(9):2707-2724. DOI: 10.1016/j.engfracmech.2007.03.002
[23] VASSILOPOULOS A, NIJSSEN R. Fatigue life prediction of composite materials under realistic loading conditions (variable amplitude loading)[M]//Fatigue life prediction of composites and composite structures. Second Editon. Sawston: Woodhead Publishing, 2010: 293-333.
[24] PAEPEGEM W V, DEGRIECK J. Effects of load sequence and block loading on the fatigue response of fiber-reinforced composites[J]. Mechanics of Advanced Materials and Structures,2002,9(1):19-35. DOI: 10.1080/153764902317224851
[25] YANG J, JONES D. Load sequence effects on the fatigue of unnotched composite materials[J]. Fatigue of Fibrous Composite Materials,1981,723:213-232.
[26] RYDER J, WALKER E. Ascertainment of the effect of compressive loading on the fatigue lifetime of graphite epoxy laminates for structural applications[R]. Burbank: Lockheed-California co Burbank Rye Canyon Research Lab, 1976.
-
期刊类型引用(1)
1. 杨元林，李英，陈丽佳，牛连斌. 钙钛矿太阳能电池中顶电极的研究进展. 功能材料. 2022(07): 7040-7057 . 
百度学术
其他类型引用(1)
-
目的
疲劳作为评估工程结构可靠性与耐久性的关键指标，对于轻量化、高性能的复合材料也必须纳入考量。疲劳建模是复合材料结构疲劳寿命评估、预测的关键一环，面对工况复杂的航空、航天及风电用复合材料而言，疲劳模型仍然存在成本高昂、适用工况单一(恒幅)的问题。本文试图通过试验数据信息聚集的方法来建模复合材料疲劳的应力水平依赖性，以期降低研究复合材料疲劳性能、确定疲劳模型参数所需的试验成本，并将新模型应用于变幅加载工况，探究多级应力水平下的复合材料疲劳寿命预测问题。
方法首先，给出强度储备与归一化强度储备的定义与公式，并根据该归一化强度储备的定义与公式对剩余强度数据进行转换和聚集，即先将所有应力水平下的剩余强度试验数据转换为强度储备数据，再根据试验静强度将强度储备数据归一换算至[0,1]区间，从而得到归一化强度储备数据。其次，依据复合材料试样强度-寿命等秩假设，推导出试样疲劳寿命分布函数，再从该寿命分布函数中反解出寿命，即可得到给定失效概率的疲劳寿命计算公式，进而将该概率寿命模型耦合进剩余强度模型，最终得到了一个新的概率型剩余强度模型。最后，利用归一化强度储备公式对归一化强度储备数据进行最小二乘拟合，得到一组模型参数，再带入概率型剩余强度模型中去预测多个应力水平下的强度退化过程。此外，结合前一载荷水平的应力加载结束时的剩余强度与后一载荷水平的应力加载开始时的剩余强度值相等的原则，便可基于所提出的概率型剩余强度模型开展多级变幅加载时的复合材料剩余强度预测。
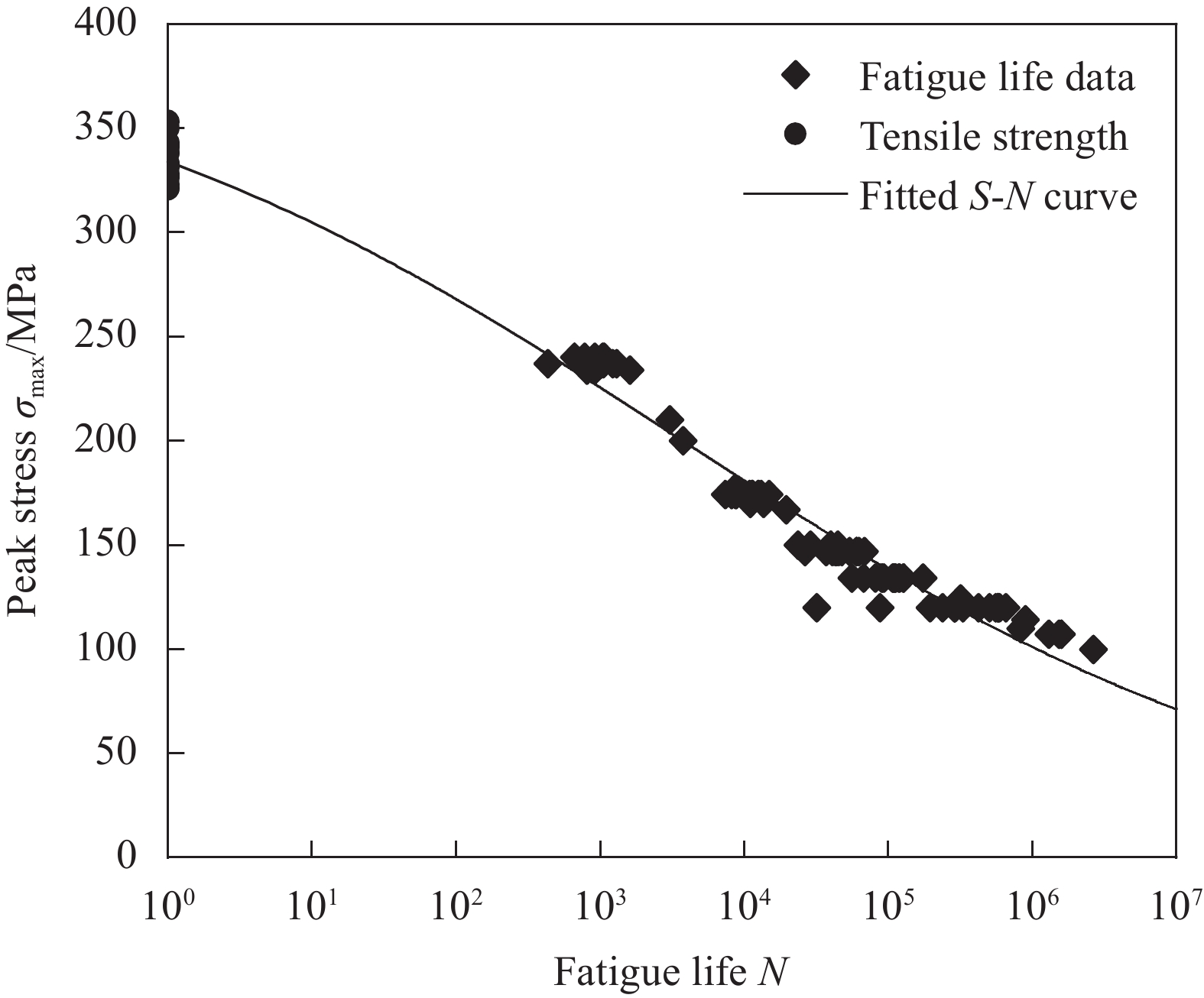
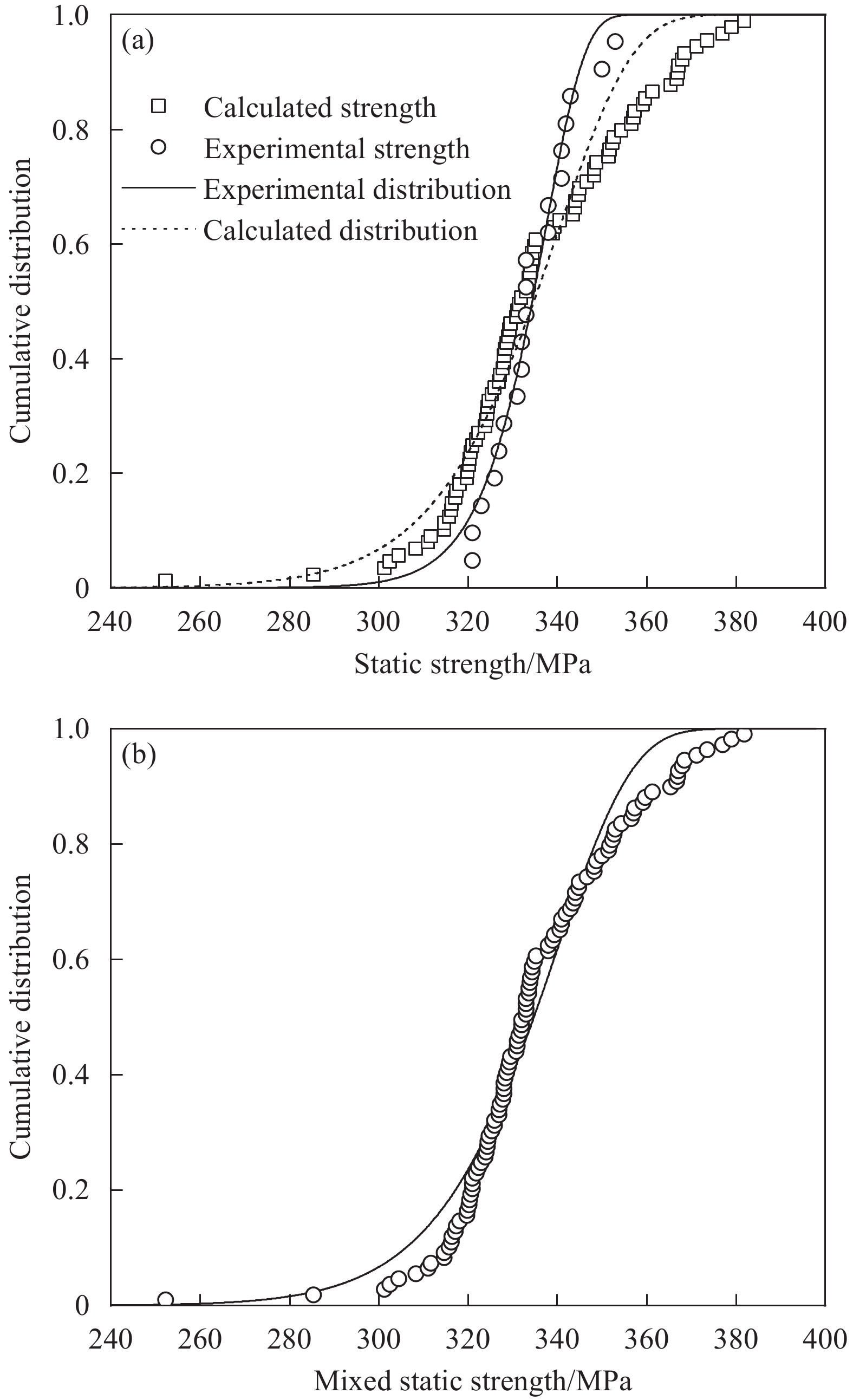
结果在对静强度进行威布尔分布拟合时存在两类静强度值：一类是根据拉伸试验获得的试验静强度，另一类是根据强度-寿命等秩假设，从疲劳寿命计算而来的计算静强度。理论上讲，根据试验静强度和计算静强度进行威布尔拟合得到的两条曲线应该是重合的，而两者实际不重合，究其根本原因是疲劳测试和静力测试间的加载应变率差异明显且未被考虑，试验中疲劳测试的加载频率为10 Hz，应力水平跨度从100 MPa到240 MPa，则应力水平从谷值到峰值的加载时间为0.05 s，平均加载率的跨度区间为2000-4800 MPa⋅s，进而根据给定的材料刚度为25000 MPa，可确定疲劳测试时的平均应变率为0.08-0.192 s。另一方面，静力测试时的加载速率为667 N⋅s，根据试样的横截面积(150 mm)即可确定平均加载率为4.45 MPa⋅s，则相应的平均应变率为1.78×10 s。由此可见，静力测试的平均应变率较疲劳测试的平均应变率低了3个数量级，进而导致了这种差异。采用文献中的数据对所提出模型的考虑应力水平的能力进行了验证，归一化强度储备曲线的拟合优度为 = 0.8031，将归一化强度储备拟合得到的一组模型参数代入3个应水平的剩余强度模型，得到了3个应力水平的剩余强度预测曲线与试验数据的对比，几乎所有的试验数据点都准确地散落在置信分布带之内。此外，为了更明确地证明模型的预测精度，给出了在50%失效概率下的模型预测曲线与试验数据中值的对比，3个应力水平下(即174、147及120 MPa)的拟合优度分别为：0.94、0.84及0.97。2级应力水平的变幅加载算例验证中，升序加载造成的损伤0.5大于降序加载造成的损伤0.45，而在3个应力水平的变幅加载算例验证中，升序加载造成的损伤0.46小于降序加载造成的损伤0.48。最后在22级应力水平变幅加载的算例验证中，当选择失效概率为50%时，升序加载的模型预测剩余强度值为272 MPa，试验中值为289 MPa，相对误差为5.9%；降序加载的模型预测剩余强度值为278 MPa，试验中值为285 MPa，相对误差为2.5%。当选择失效概率为5%时，升序加载的模型预测剩余强度值为255 MPa，试验最小值为260 MPa，相对误差为2%；降序加载的模型预测剩余强度值为257 MPa，试验最小值为262 MPa，相对误差为1.9%。
结论试验静强度与计算静强度的威布尔统计分布存在明显差异的原因是静力测试和疲劳测试时的应变率相差过大；所提出概率型剩余强度模型能在显著降低模型参数依赖性的同时，准确地刻画试样的剩余强度退化过程；当考虑强度的固有分散性时，在仅有两到三级应力加载的情况下，单调升序与单调降序对复合材料试样寿命的影响可以忽略不计；所提出模型的预测值与试验值之间的相对误差均不超过6%，且预测精度随着可靠度的增加而有所提高。
-
剩余强度模型被广泛用于复合材料疲劳寿命预测，然而除最简单的线性模型之外，其余模型的预测精度都依赖大量的疲劳试验，尤其是在更符合实际工况的变幅加载条件下，采用剩余强度模型预测寿命之前必须借助疲劳试验确定试样在每个应力水平下的强度退化规律，这是一个极度耗时、成本高昂的过程。此外，复合材料固有的各向异性、成型工艺的多样性以及加工缺陷的不确定性，必然导致疲劳试验数据具有强分散性，因此复合材料的疲劳表征应采用统计学方式。
为了降低预测复合材料变幅疲劳行为所需的试验投入，首先根据归一化强度储备与剩余强度间的定量关系，给出了计及应力水平效应的确定性剩余强度模型，再基于强度-寿命等秩假设，通过将疲劳寿命耦合进确定性剩余强度模型，衍生出一个考虑应力水平效应且独立于应力水平的剩余强度概率模型。
图1 所示的恒幅工况算例验证表明，所提出的模型仅用1组模型参数就可准确预测3个应力水平下的强度退化；图2 (a)所示为3级应力水平变幅工况下的剩余强度预测，遵循前一载荷块加载结束时的剩余强度与后一载荷块加载开始时的剩余强度值相等的原则，即可执行不同应力水平间的加载次数等效转换，进而完成变幅工况下的剩余强度预测；图2 (b)所示为22级应力水平变幅工况下的剩余强度预测，降序预测误差为1.9%，升序预测误差为2%。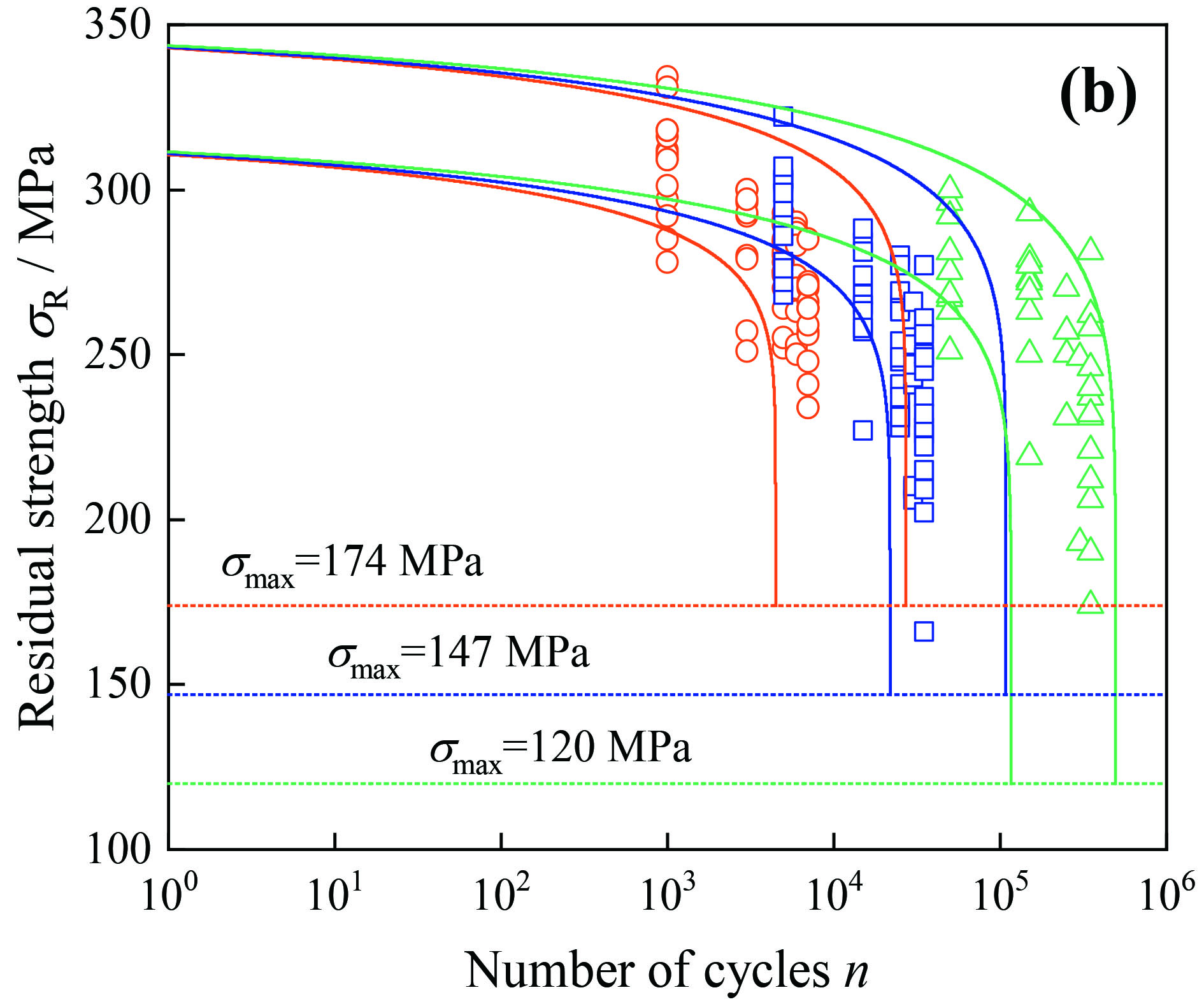
恒幅工况下的应力水平独立性算例验证
变幅工况算例验证：(a) 3级变幅预测；(b) 22级变幅预测结果
计量
- 文章访问数: 746
- HTML全文浏览量: 323
- PDF下载量: 51
- 被引次数: 2
