Bionic design of wood cell wall based on 3D printing
-
摘要: 木材中起骨架作用的纤维素是以不同螺旋结构的微纤丝形式存在于细胞壁中。本文通过将3D打印技术与仿真模拟相结合，研究木材细胞壁的纤维螺旋增强结构。使用微晶纤维素(MCC)/聚乳酸(PLA)复合材料，在对MCC/PLA复合材料各项性能进行测试的基础上，借助3D打印技术构建木材细胞壁螺旋结构，通过改变纤维取向和纤维孔状结构编程合成结构的力学功能。有限元仿真则用于强调纤维在刚性单元之间的载荷传递机制中的关键作用。结果表明：通过编程纤维的取向和结构可以宏观调控结构的性能，其中纤维的交叉结构作为一种优化设计可以用于提高结构成型制品的力学性能。这些结构可以被组装成更大的系统，用于构建具有优化特定功能的模块化复合材料；在异质结构设计和新型复合材料制造领域中均具有潜在的应用价值。Abstract: Cellulose, which plays the role of skeleton in wood, exists in the cell wall in the form of microfibrils with different helical structures. In this paper, the fiber spiral reinforced structure of wood cell wall was studied by combining 3D printing technology with simulation. Using microcrystalline cellulose (MCC)/polylactic acid (PLA) composites, based on the testing of the properties of MCC/PLA composites, the spiral structure of wood cell wall was constructed with the help of 3D printing technology, and the mechanical function of the structure was programmed by changing the fiber orientation and fiber pore structure. Finite element simulation was used to emphasize the key role of fiber in the load transfer mechanism between rigid elements. The results show that the properties of the structure can be controlled by programming the orientation and structure of the fiber, and the cross structure of the fiber can be used to improve the mechanical properties of the structural molded products as an optimal design. These structures can be assembled into larger systems for building modular composites with optimized specific functions. It has potential application value in the field of hetero structure design and new composite material manufacturing.
-
Key words:
- fused deposition molding /
- cellulose /
- bionic design /
- mechanical properties /
- finite element
-
图 1 木材细胞壁S2层微纳结构的仿生模型图
Figure 1. Bionic model diagram of micro-nano structure in S2 layer of wood cell wall
图 2 3D打印试件截面图
(a) Upper surface structure diagram of circular 0° structure; (b) Comparison with upper surface structure diagram of structure
Figure 2. Cross section of 3D printed specimen
图 3 压缩后试件图
(a) Circular 45° compression specimen; (b) Circular 45° cross structure compression specimen
Figure 3. Specimen drawing after compression
图 4 纯PLA与MCC/PLA复合材料脆断面的SEM图像
Figure 4. SEM images of brittle sections of pure PLA and MCC/PLA composites
图 5 纯PLA与MCC/PLA复合材料的DSC曲线图
Figure 5. DSC curves of pure PLA and MCC/PLA composites materials
图 7 纯PLA与MCC/PLA复合材料的拉伸强度与拉伸杨氏模量对比
Figure 7. Comparison of Young's modulus and tensile strength of pure PLA and MCC/PLA composites
图 8 MCC/PLA圆形、矩形、多边形结构及基体PLA对照结构的杨氏模量与压缩强度对比
Figure 8. Comparison of Young's modulus and compressive strength of MCC/PLA circular, rectangular, polygonal structure and matrix PLA control structure
图 9 MCC/PLA圆形结构螺旋角度0°~60°的弹性模量与压缩强度对比
Figure 9. Comparison of elastic modulus and compressive strength of MCC/PLA circular structure with spiral angle of 0°-60°
图 10 MCC/PLA圆形0°结构及MCC/PLA圆形交叉结构弹性模量与压缩强度对比
Figure 10. Comparison of elastic modulus and compressive strength of MCC/PLA circular 0° structure and MCC/PLA circular cross structure
图 11 多边形结构有限元分析应力分布云图
S—Von Mises stress
Figure 11. Finite element analysis results of polygonal porous structure
图 12 圆形结构(a)与矩形结构(b)的应力分布云图
Figure 12. Von-Mises of circular structure (a) and rectangular structure (b)
图 13 三种结构的截面形状：(a)多边形；(b)圆形；(c)矩形
Figure 13. Sectional shapes of three structures: (a) Polygonal; (b) Circular; (c) Rectangular
图 14 15°~60°的交叉填充螺旋结构在3000 N集中力下的应力分布云图
Figure 14. Stress distribution cloud diagram of the filled spiral structure with 15°-60° under 3000 N concentrated force
图 15 15°~60°交叉螺旋结构的应变柱状图
Figure 15. Strain histogram of 15°-60° cross helical structure
图 16 15°~60°的螺旋结构在90°扭转角下的应力分布云图
Figure 16. Stress distribution nephogram of the spiral structure with 15°-60° under 90° torsion angle
图 17 15°~60°的螺旋结构在90°扭转角下的扭转角-扭矩图
Figure 17. Torsion angle-torque diagram of 15°-60° helical structure under 90° torsion angle
图 18 圆形15°交叉结构与圆形结构的应力-应变曲线
Figure 18. Stress-strain curves of circular 15° cross structure and circular structure
表 1 复合材料的命名
Table 1. Name of composite materials
Sample MCC/wt% 10%MCC/PLA 10 20%MCC/PLA 20 30%MCC/PLA 30 Notes: MCC—Microcrystalline cellulose; PLA—Polylactic acid. 
下载: 导出CSV
表 2 纯PLA与 MCC/PLA复合材料的DSC曲线特征值
Table 2. DSC curve characteristic values of pure PLA and MCC/PLA
Glass transition temperature/℃ Crystallization temperature/℃ Crystallinity/% Melting temperature/℃ Pure PLA 64.92 115.92 2.26 169.925 10%MCC/PLA 65.91 110.91 2.25 169.917 20%MCC/PLA 64.92 109.92 4.68 169.921 30%MCC/PLA 64.92 109.92 5.37 169.923
下载: 导出CSV
表 3 纯PLA与MCC/PLA复合材料的TG曲线特征值
Table 3. Characteristic values of TG curves of pure PLA and MCC/PLA composites
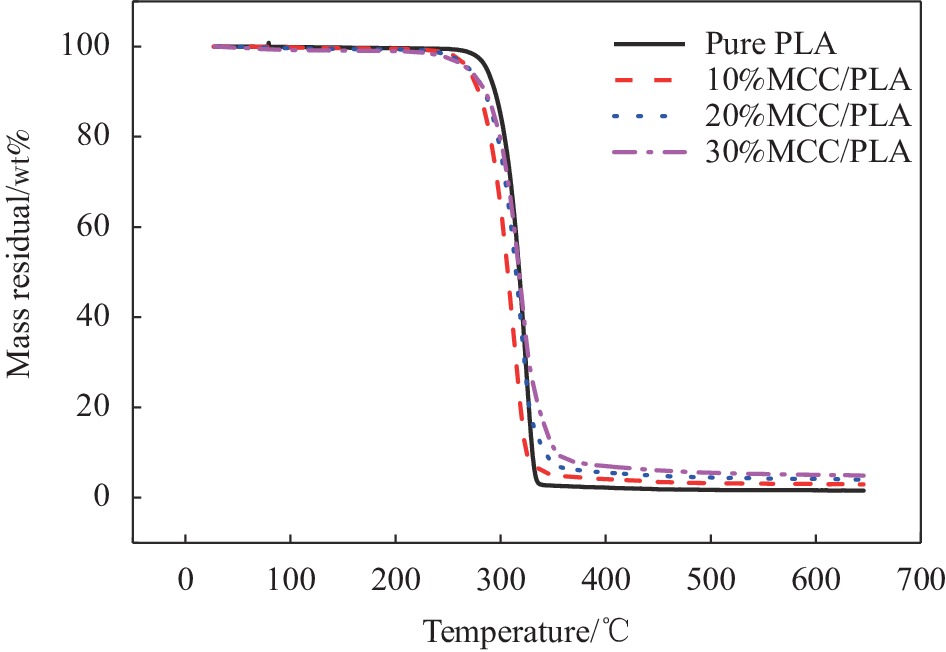
Inflection point temperature/℃ 210℃ residual/wt% 600℃ residual/wt% Pure PLA 323.91 99.61 1.64 10%MCC/PLA 312.66 99.47 3.01 20%MCC/PLA 319.42 99.28 4.16 30%MCC/PLA 320.11 98.91 5.01
下载: 导出CSV
表 4 材料的有限元参数设定
Table 4. Finite element parameter setting of materials
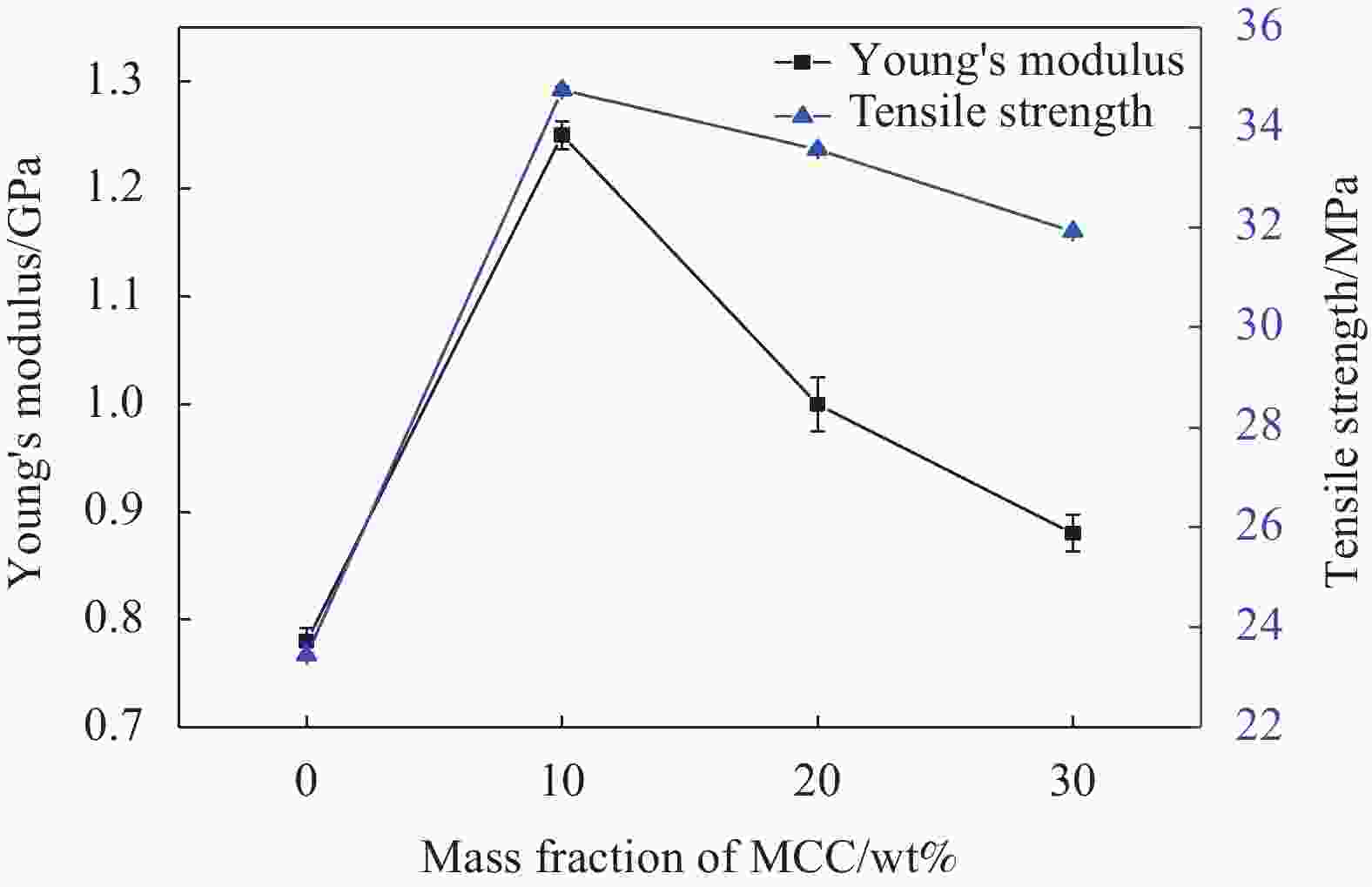
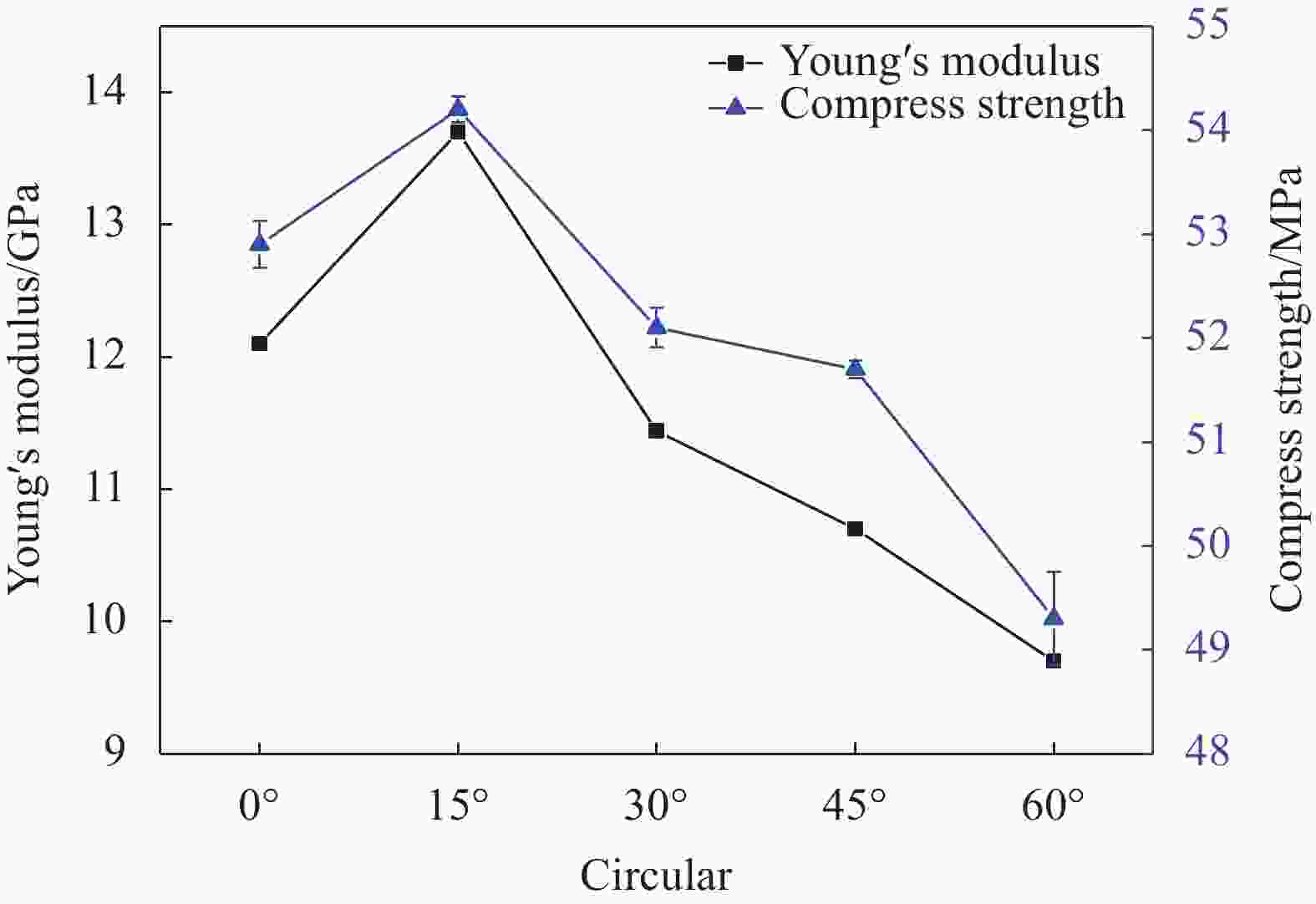
Modulus of elasticity/GPa Poisson's ratio Yield strength/MPa Filling structure 1.20 0.30 34.70 Matrix structure 0.78 0.35 25.40
下载: 导出CSV
表 5 结构力学性能的有限元分析结果与压缩实验结果
Table 5. Finite element analysis results and stress experimental results of mechanical properties of structures
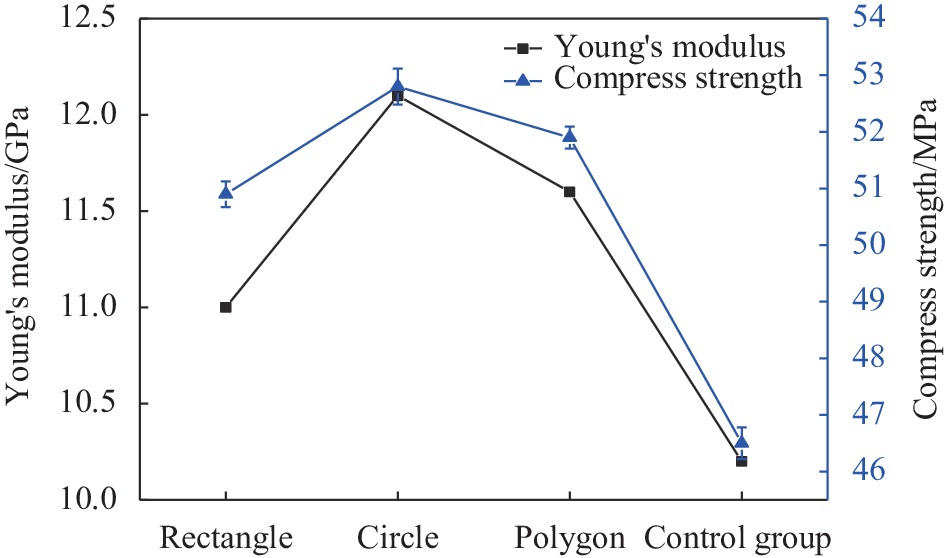
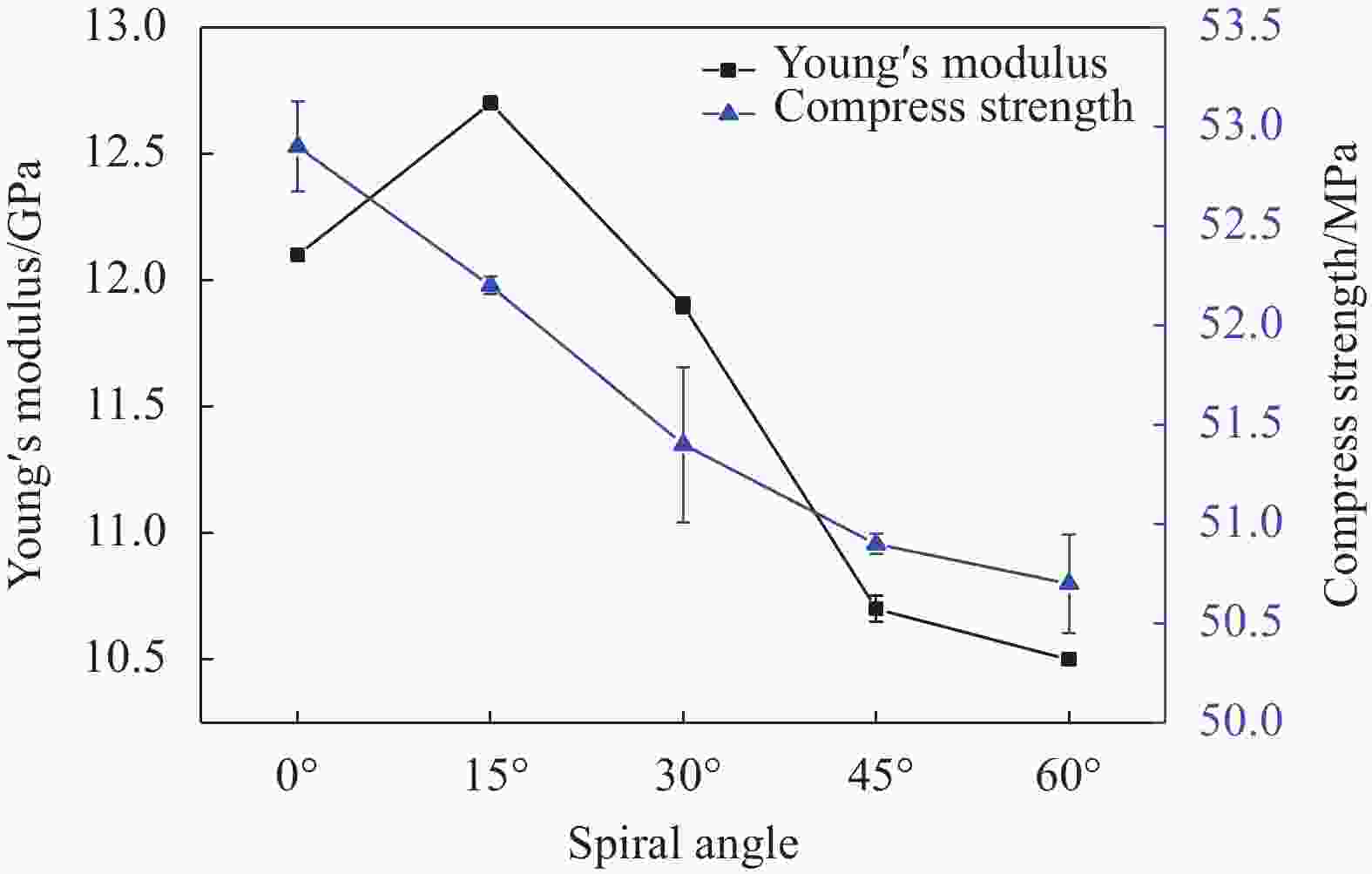
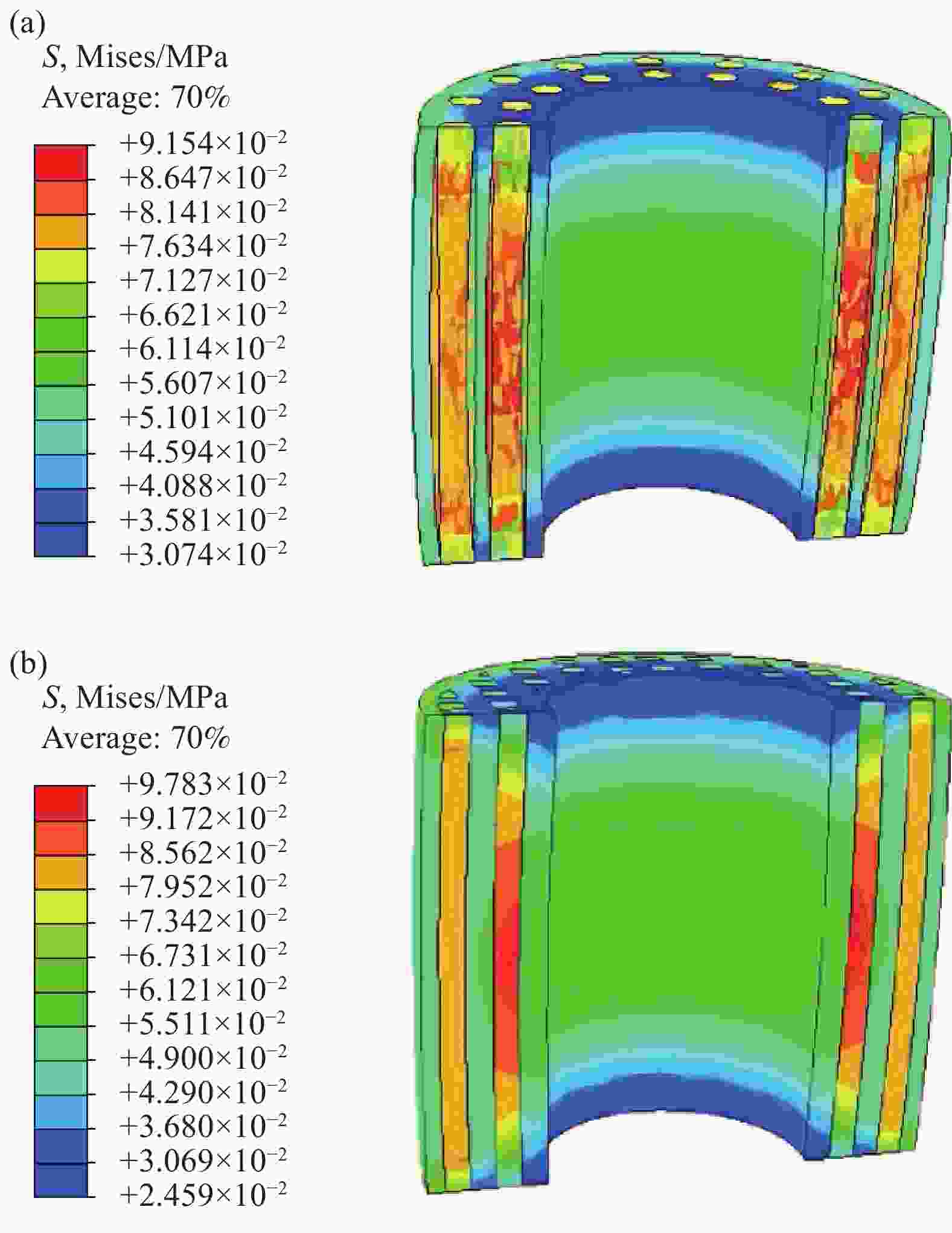
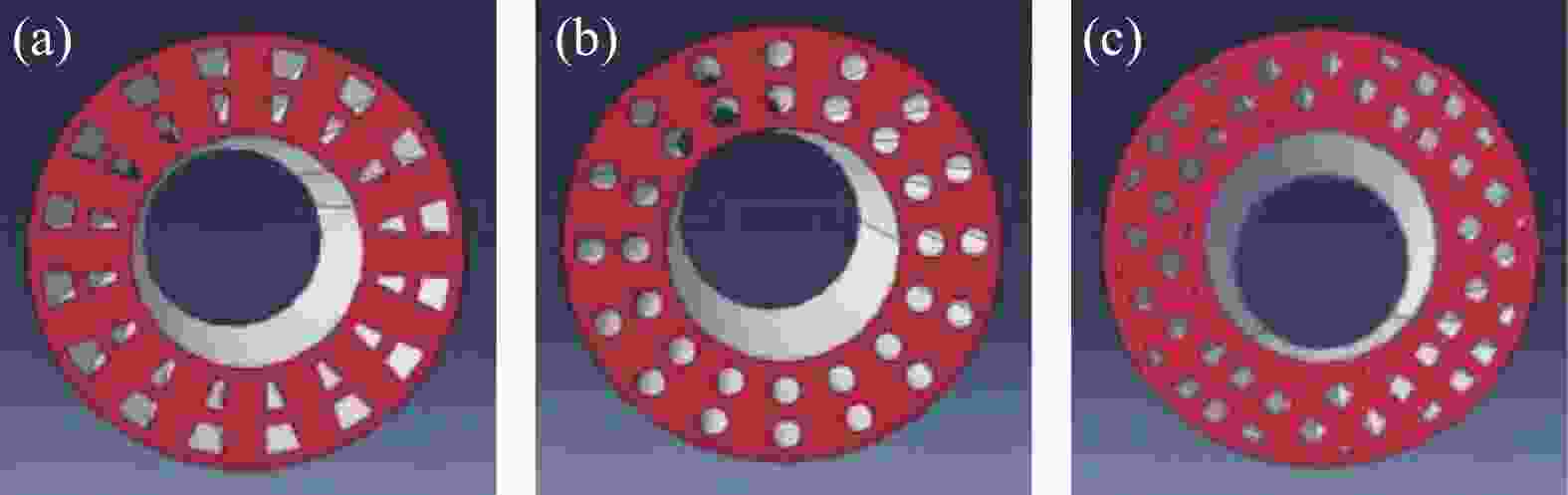
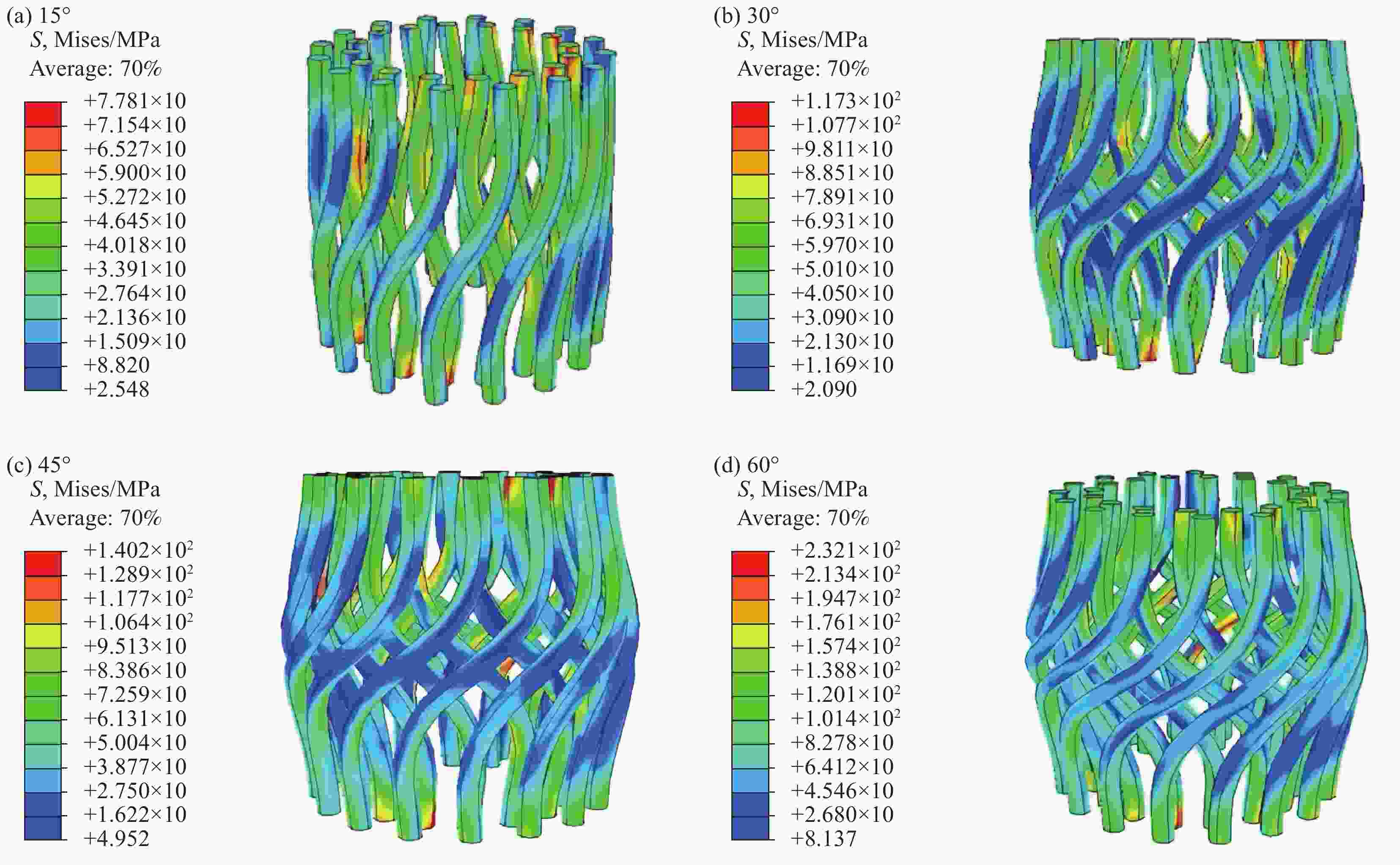
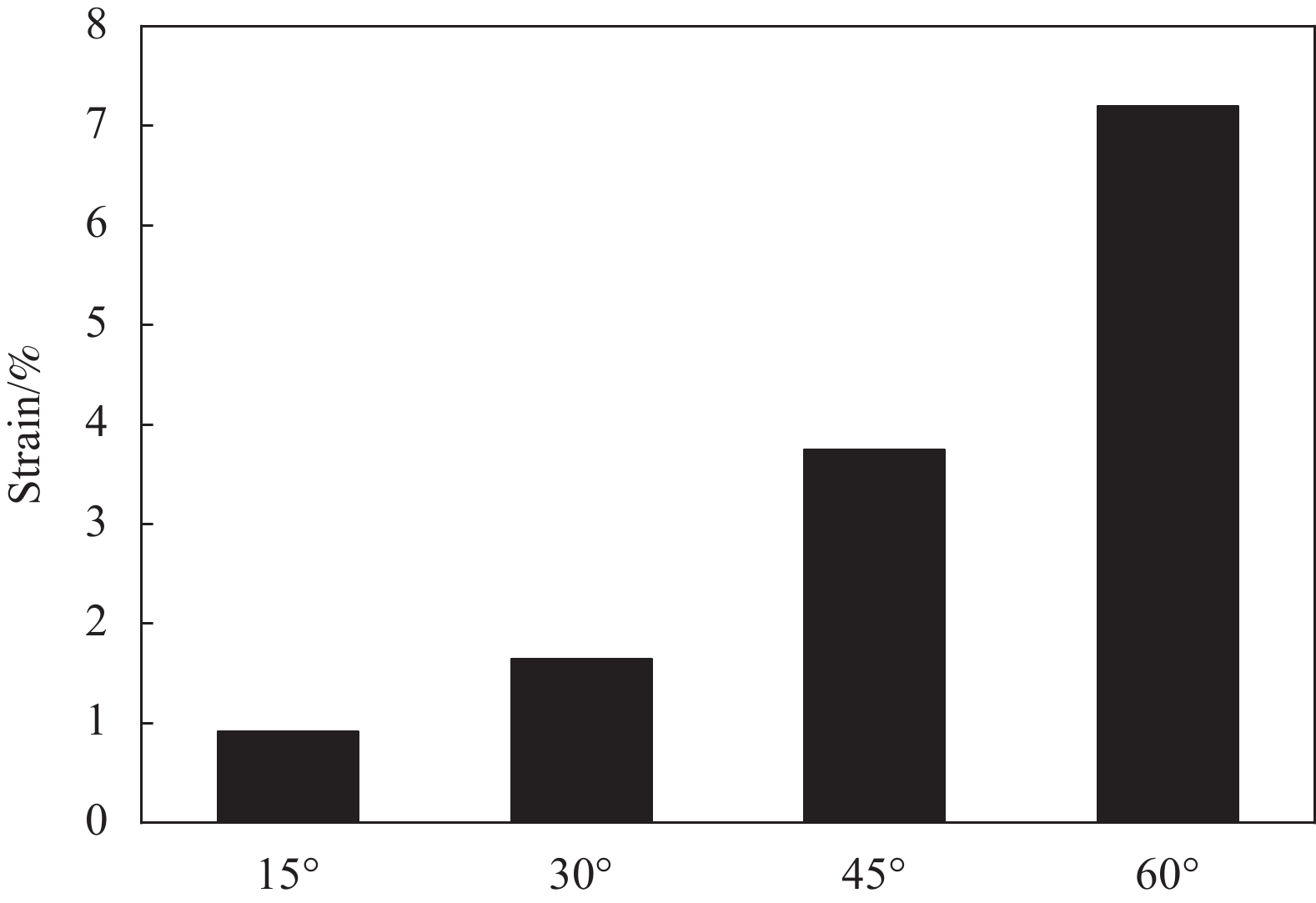
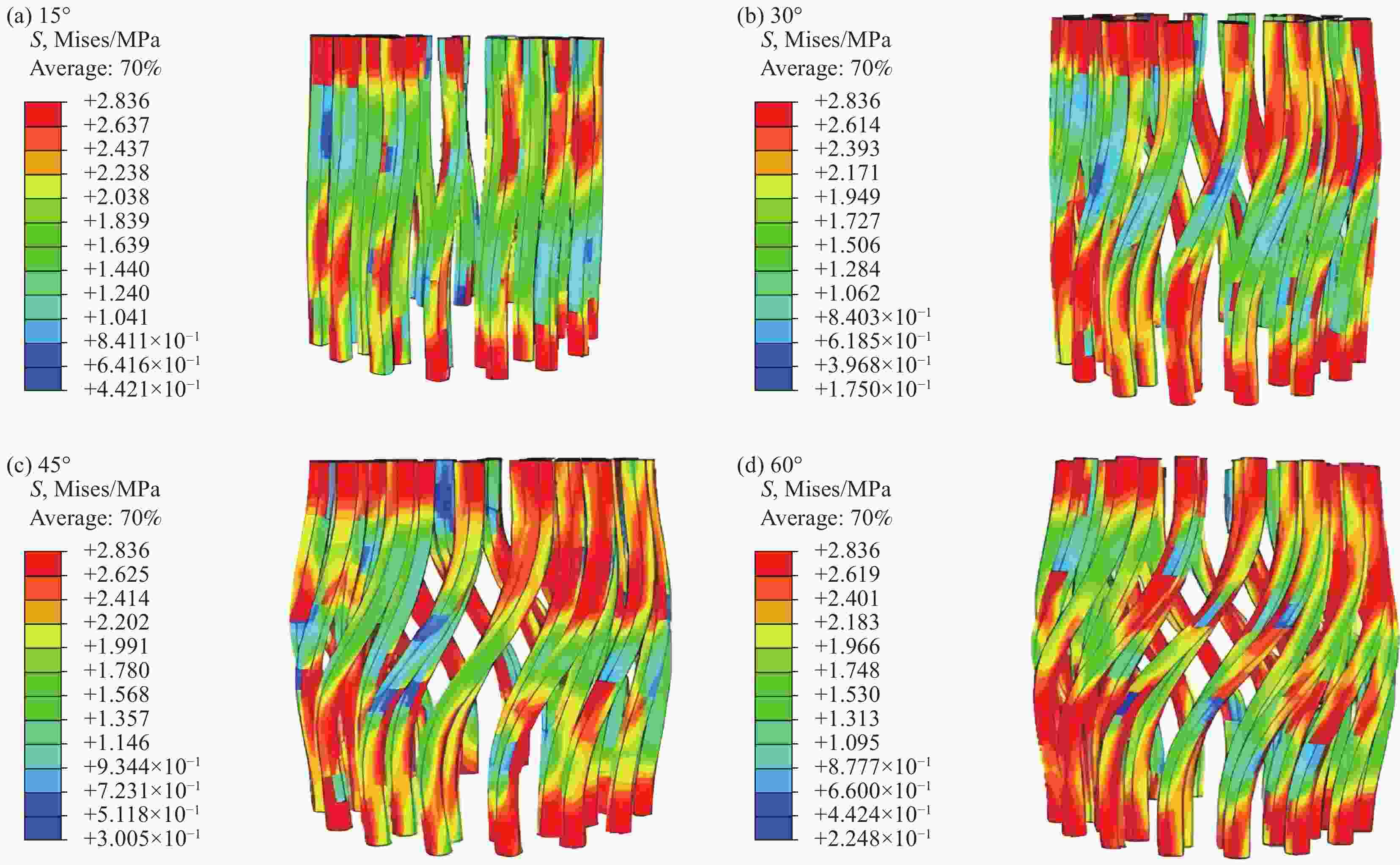
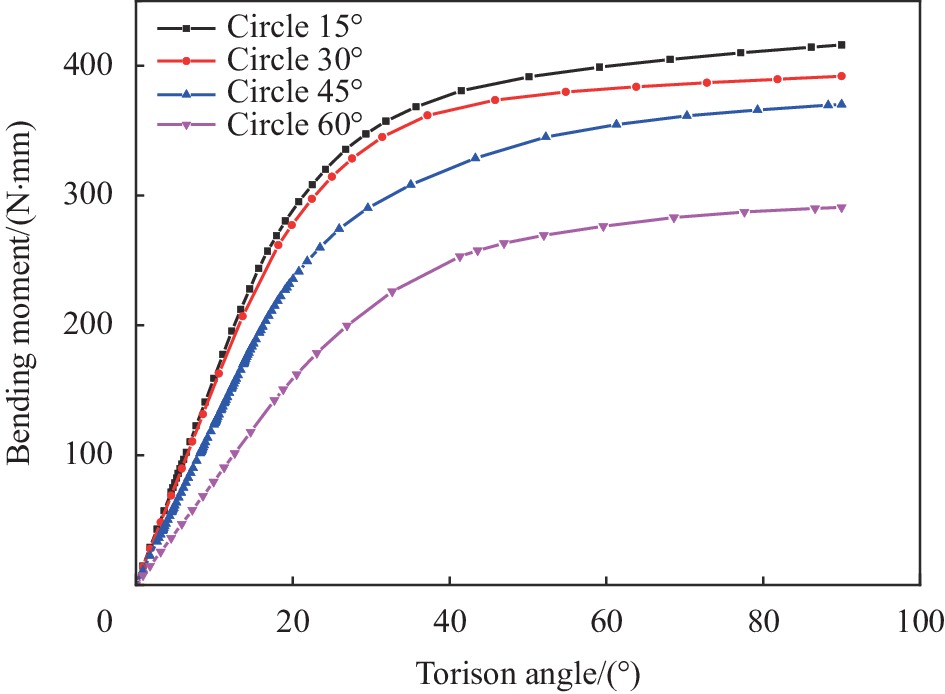
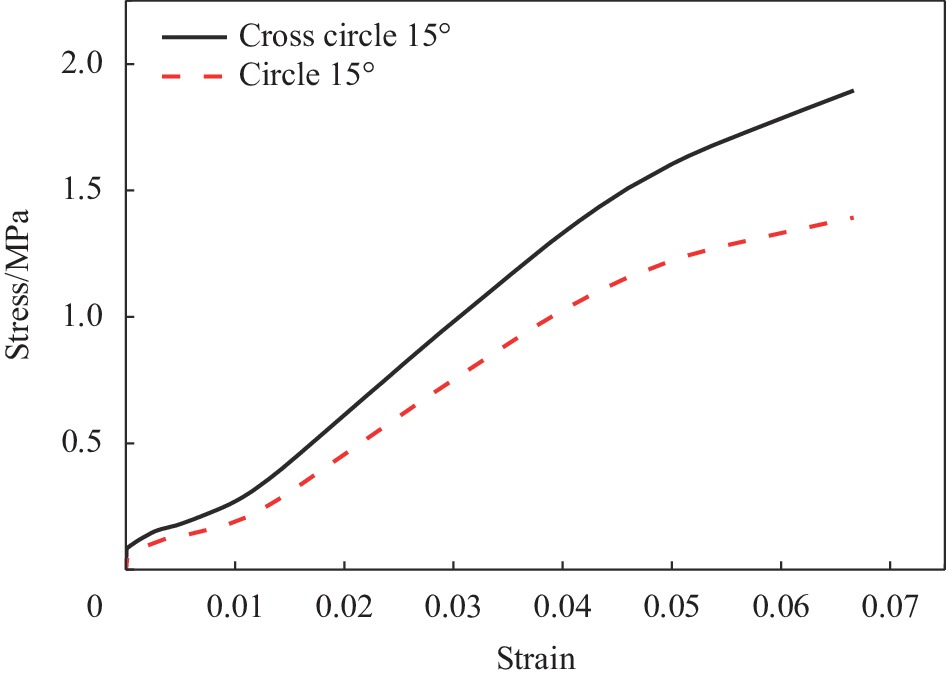
Stress/MPa Modulus of elasticity/GPa Relative error/% Theoretical value Actual value Theoretical value Actual value Stress Modulus of elasticity Rectangle 46.2 50.9 13.46 11.0 10.17 18.27 Polygon 47.4 51.9 13.23 11.6 9.49 12.32 Circle 0° 49.2 52.8 13.80 12.1 7.32 12.32 Circle 15° 49.6 52.2 13.40 12.7 5.65 5.22 Circle 30° 48.0 51.4 13.24 11.9 7.08 10.12 Circle 45° 47.3 50.9 13.11 10.7 7.61 18.38 Circle 60° 46.5 50.7 12.82 10.5 9.03 18.10
下载: 导出CSV
-
[1] 杨蕊, 曹清华, 梅长彤, 等. 高孔隙率三维结构木材构建功能复合材料的研究进展[J]. 复合材料学报, 2020, 37(8):1796-1804.YANG Rui, CAO Qinghua, MEI Changtong, et al. Research progress of functional composites constructed from three-dimensional structural wood with high porosity[J]. Acta Materiae Compositae Sinica,2020,37(8):1796-1804(in Chinese). [2] 郭宇, 李超, 李英洁, 等. 木材细胞壁与木材力学性能及水分特性之间关系研究进展[J]. 林产工业, 2019, 46(8):14-18.GUO Yu, LI Chao, LI Yingjie, et al. Research progress on the relationship between wood cell wall and wood mechanical properties and water properties[J]. Forest Products Industry,2019,46(8):14-18(in Chinese). [3] 曾其蕴, 李世红, 周本濂. 生物复合材料的特征及仿生的探讨[J]. 复合材料学报, 1993(1):1-7.ZENG Qiyun, LI Shihong, ZHOU Benlian. Discussion on and bionics of biological composites[J]. Acta Materiae Compositae Sinica,1993(1):1-7(in Chinese). [4] 朱越骅. 中山杉木材宏观与微观特征及湿热形变机理[D]. 南京: 南京林业大学, 2020.ZHU Yuehua. Macro and micro characteristics and hygrothermal deformation mechanism of Zhongshan fir wood[D]. Nanjing: Nanjing Forestry University, 2020(in Chinese). [5] 安鑫. 毛竹纤维细胞壁微纤丝取向与超微构造研究[D]. 北京: 中国林业科学研究院, 2016.AN Xin. Study on microfibril orientation and ultrastructure of fiber cell wall in moso bamboo[D]. Beijing: Chinese Academy of Forestry, 2016(in Chinese). [6] 孙海燕. 杉木无性系木材力学性质及其与微观结构相关性研究[D]. 北京: 中国林业科学研究院, 2019.SUN Haiyan. Study on wood mechanical properties of Chinese firclones and their correlation with microstructure[D]. Beijing: Chinese Academy of Forestry Sciences, 2019(in Chinese). [7] SCHULGASSER K, WITZTUM A. On the strength of herbaceous vascular plant stems[J]. Annals of Botany,1997,80(1):35-44. doi: 10.1006/anbo.1997.0404 [8] REITERER A, LICHTENEGGER H, TSCHEGG S, et al. Experimental evidence for a mechanical function of the cellulose microfibril angle in wood cell walls[J]. Philosophical Magazine A,1999,79(9):2173-2184. doi: 10.1080/01418619908210415 [9] 李坚, 甘文涛, 王立娟. 木材仿生智能材料研究进展[J]. 木材科学与技术, 2021, 35(4):1-14.LI Jian, GAN Wentao, WANG Lijuan. Research progress of wood bionic intelligent materials[J]. Wood Science and Technology,2021,35(4):1-14(in Chinese). [10] 方文彬, 林云, 罗建举, 等. 火炬松速生材构造变异规律的研究[J]. 中南林学院学报, 1995(1):13-19.FANG Wenbin, LIN Yun, LUO Jianju, et al. Study on structural variation of fast-growing wood of loblolly pine[J]. Journal of Central South Forestry University,1995(1):13-19(in Chinese). [11] KOSEI A, MAYU M, KEISUKE T, et al. Dependence of Poisson’s ratio and Young’s modulus on microfibril angle (MFA) in wood[J]. Holzforschung,2018,72(4):321-327. doi: 10.1515/hf-2017-0091 [12] 赵彻. 异质材料与微结构耦合仿生设计及其3D打印[D]. 长春: 吉林大学, 2017.ZHAO Che. Bionic design and 3D printing of heterogeneous materials and microstructure coupling[D]. Changchun: Jilin University, 2017(in Chinese). [13] FRATZL P, BURGERT I, KECKES J. Mechanical model for the deformation of the wood cell wall[J]. Zeitschrift Für Metallkunde,2004,95(7):579-584. [14] DENG Q, LI S, CHEN Y. Mechanical properties and failure mechanism of wood cell wall layers[J]. Computational Materials Science,2012,62:221-226. doi: 10.1016/j.commatsci.2012.05.050 [15] YE W G, DOU H, CHENG Y Y, et al. Self-sensing properties of 3D printed continuous carbon fiber-reinforced PLA/TPU honeycomb structures during cyclic compression[J]. Materials Letters,2022,317:132077. doi: 10.1016/j.matlet.2022.132077 [16] 房鑫卿. 3D打印技术的发展历程及应用前景[J]. 轻工科技, 2019(5):77-78.FANG Xinqing. Development process and application prospect of 3D printing technology[J]. Light Industry Science and Technology,2019(5):77-78(in Chinese). [17] 刘俊, 孙璐姗, 王钱钱, 等. 3D打印生物质基复合材料研究进展及应用前景[J]. 生物产业技术, 2017(3): 68-81.LIU Jun, SUN Lushan, WANG Qianqian, et al. Research progress and application prospect of 3D printing biomass matrix composites[J]. Biotechnology, 2017(3): 68-81(in Chinese). [18] 郭少豪, 吕振. 3D打印改变世界的新机遇新浪潮[J]. 中国科技信息, 2013, 484(23):147.GUO Shaohao, LV Zhen. New opportunities and new wave of 3D printing changing the world[J]. China Science and Technology Information,2013,484(23):147(in Chinese). [19] 赵萍, 蒋华, 周芝庭. 熔融沉积快速成型工艺的原理及过程[J]. 机械制造与自动化, 2003(5):17-18. doi: 10.3969/j.issn.1671-5276.2003.05.006ZHAO Ping, JIANG Hua, ZHOU Zhiting. Principle and process of melt deposition rapid prototyping[J]. Mechanical Manufacturing and Automation,2003(5):17-18(in Chinese). doi: 10.3969/j.issn.1671-5276.2003.05.006 [20] 李仲阳. 回转式FDM连续挤出喷头[J]. 机械制造, 2002(2):29-30. doi: 10.3969/j.issn.1000-4998.2002.02.012LI Zhongyang. Rotary FDM continuous extrusion nozzle[J]. Machinery Manufacturing,2002(2):29-30(in Chinese). doi: 10.3969/j.issn.1000-4998.2002.02.012 [21] QIN D X, SANG L, ZHANG Z H, et al. Compression performance and deformation behavior of 3D-printed PLA-based lattice structures[J]. Polymers,2022,14(5):1062. doi: 10.3390/polym14051062 [22] 周意诚, 刘爱红, 赵巧玲, 等. 3维打印用聚乳酸材料的改性研究进展[J]. 化工新型材料, 2021, 49(3):216-220.ZHOU Yicheng, LIU Aihong, ZHAO Qiaoling, et al. Research progress on modification of polylactic acid materials for 3D printing[J]. New Chemical Materials,2021,49(3):216-220(in Chinese). [23] 颜家强, 戢德贤, 杨桂花, 等. 微晶纤维素的制备方法及其应用领域概述[J]. 中华纸业, 2021, 42(10):8-13.YAN Jiaqiang, YUAN Dexian, YANG Guihua, et al. Overview of preparation methods and application fields of microcrystalline cellulose[J]. Zhonghua Paper,2021,42(10):8-13(in Chinese). [24] XIAN X J, WANG X F, ZHU Y C, et al. Effects of MCC content on the structure and performance of PLA/MCC biocomposites[J]. Journal of Polymers and the Environment, 2018, 26: 3484-3492. [25] American Society for Testing and Materials. Standard test method for tensile properties of plastics: ASTM D638-03[S]. West Conshohocken: ASTM International, 2004. [26] 中国国家标准化管理委员会. 塑料压缩性能试验方法: GB/T 1041—2008[S]. 北京: 中国标准出版社, 2009.Standardization Administration of the People's Republic of China. Test method for compressive properties of plastics: GB/T 1041—2008[S]. Beijing: China Standards Press, 2009(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 1287
- HTML全文浏览量: 600
- PDF下载量: 64
- 被引次数: 0
