Numerical study on the effect of void content on hygrothermal performances of carbon fiber reinforced polyamide 6 composites
-
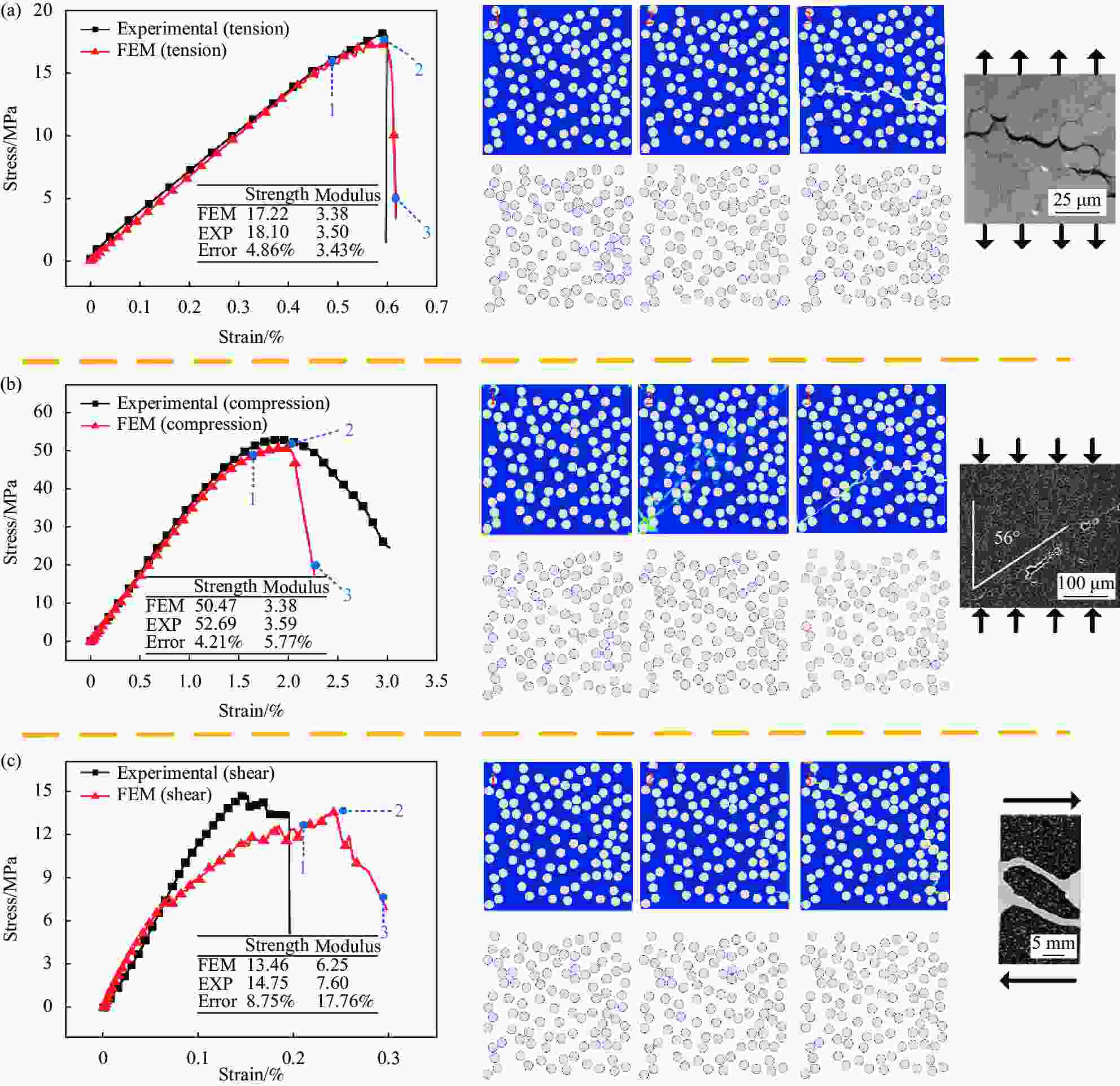
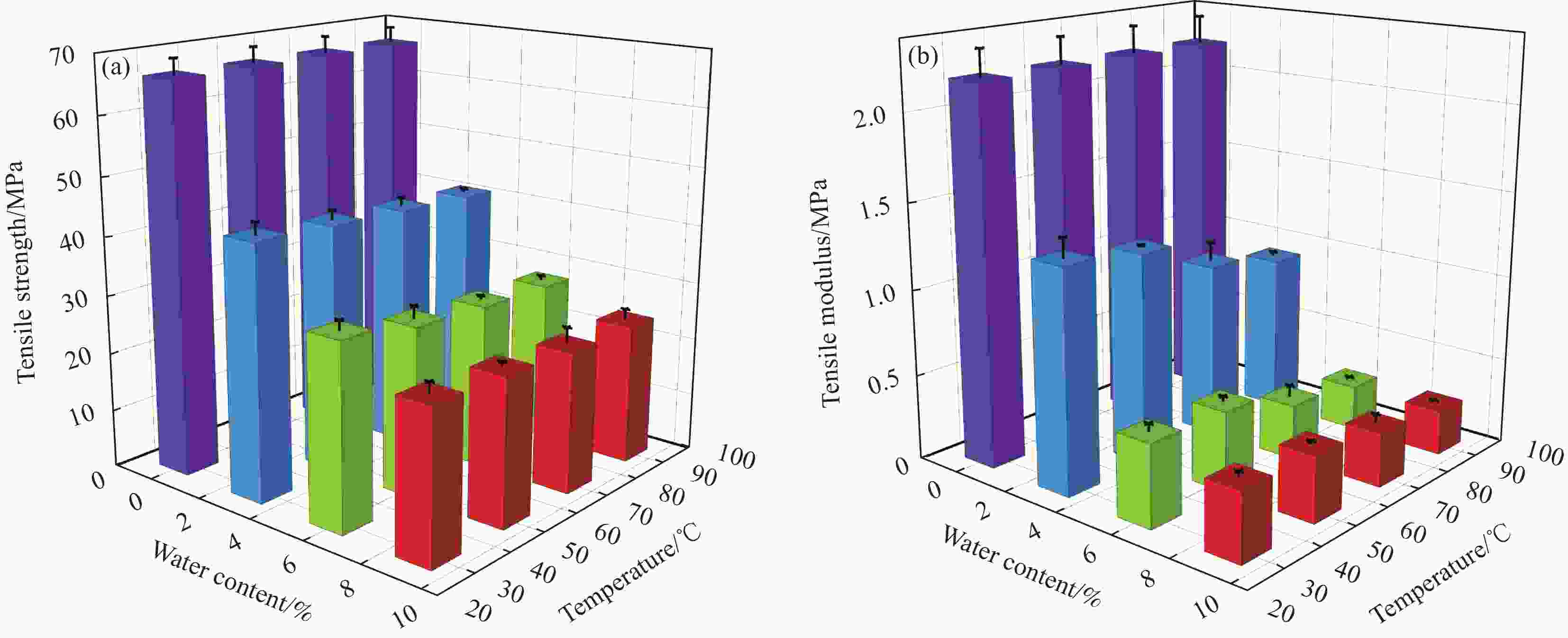
摘要: 孔隙在复合材料制造过程中广泛存在，在湿热环境下孔隙的存在会改变应力场和水分场，进而影响复合材料的吸湿性能与力学老化性能。对碳纤维/尼龙6(Carbon fiber reinforced polyamide 6，CF/PA6)复合材料在不同温度浸水环境下吸湿老化后的力学性能测试，研究了温度与吸湿量对其力学性能的影响及强度与模量等力学参数的演化规律，建立吸湿参数与力学参数的关联函数。基于随机顺序吸附法算法(Random sequential adsorption，RSA)，建立了纤维、界面和孔隙随机分布的代表性体积单元(Representative volume element，RVE)模型。在本构模型中引入依赖于吸湿量的退化因子，研究了孔隙含量对复合材料横向拉伸、压缩、剪切强度和模量的影响，揭示了湿热老化前后不同的失效机制。结果表明：在热湿老化前，由于应力集中，孔隙会导致复合材料力学性能下降，孔隙率含量每增加1%，横向拉伸强度降低6.4%；湿热老化后，基体吸湿塑化效应是复合材料力学性能降低主要因素，对应降低率为3.86%。Abstract: Voids are comment defects generated during the manufacturing process and highly sensitive to moisture in the hygrothermal environment. The presence of voids will change the stress field and moisture field, which has deleterious effects on the mechanical performances. The mechanical properties of carbon fiber reinforced polyamide 6 (CF/PA6) composites after hygrothermal aging under different temperature immersion environment were tested to study the effects of temperature and water absorbed content on the mechanical properties. The correlation function between water content and mechanical parameters was established. Based on the random sequential adsorption algorithm (RSA), the representative volume element (RVE) model with random distribution of fibers, interfaces and voids was established. The quantitative effects of voids content on strength and modulus under the loading of transverse tension, compression and shear were investigated, by introducing a degradation factor dependent on water content into the constitutive model, and the failure mechanisms before and after hygrothermal aging were revealed. Conclusively, before hygrothermal aging, voids induce the decrease of mechanical properties due to stress concentration, and every 1% increase in the voids content results in a 6.4% decrease of transverse tensile strength. However, matrix degradation due to the absorbed water content after hygrothermal aging is the dominant factor, and the corresponding rate is 3.86%.
-
Key words:
- voids content /
- hygrothermal aging /
- RVE /
- failure mechanism /
- numerical simulation
-
图 1 吸湿量与老化温度对尼龙6(PA6)基体拉伸力学性能影响
Figure 1. Effect of absorbed water content and temperature on the tensile properties of polyamide 6 (PA6)
图 2 吸湿量与老化温度对碳纤维(CF)/PA6横向拉伸力学性能的影响
Figure 2. Effect of absorbed water content and temperature on the transverse tensile properties of carbon fiber (CF)/PA6
图 3 吸湿量对PA6基体和CF/PA6复合材料拉伸强度与弹性模量的影响
Figure 3. Effect of water content on the tensile strength and elastic modulus of PA6 resin and CF/PA6 composites
图 4 不同孔隙含量的CF/PA6复合材料代表性体积单元(RVE)模型
Figure 4. Representative volume element (RVE) models with different contents of voids for CF/PA6 composites
图 5 CF/PA6复合材料RVE模型在横向拉伸 (a)、横向压缩 (b) 和横向剪切 (c) 荷载作用下的渐进失效
FEM—Finite element analysis; EXP—Experimental
Figure 5. Failure progression in RVE model of CF/PA6 composites under the loading of tension (a), compression (b) and shearing (c)
图 6 CF/PA6复合材料预测模型与数值模拟计算结果对比
Figure 6. Comparison of prediction models and numerical simulation results of CF/PA6 composites
图 7 CF/PA6复合材料孔隙含量对吸湿性能的影响
Figure 7. Effect of voids content on the water absorption performance of CF/PA6 composites
图 8 孔隙含量对CF/PA6未老化试样力学性能的影响：(a) 强度；(b) 弹性模量
σt, σc, σs—Tensile strength, compressive strength and shear strength of CF/PA6 composites before hygrothermal aging; Et, Ec, Es—Tensile modulus, compressive modulus and shear modulus of CF/PA6 composites before hygrothermal aging
Figure 8. Effect of voids content on the mechanical performance for unaged CF/PA6 samples: (a) Strength; (b) Elastic modulus
图 9 孔隙含量对CF/PA6复合材料湿热老化模型力学性能的影响：(a)强度；(b)弹性模量
σt', σc', σs'—Tensile strength, compressive strength and shear strength of CF/PA6 composites after hygrothermal aging; Et', Ec', Es'—Tensile modulus, compressive modulus and shear modulus of CF/PA6 composites after hygrothermal aging
Figure 9. Effect of voids content on the mechanical performance for hygroscopic saturated CF/PA6 composite: (a) Strength; (b) Elastic modulus
图 10 CF/PA6复合材料未老化模型在横向拉伸(a)、横向压缩(b)及横向剪切(c)模式下的失效模式
Figure 10. Failure patterns of unaged CF/PA6 composite in the tension (a), compression (b) and shear simulation (c)
图 11 CF/PA6复合材料湿热老化模型在横向拉伸(a)、横向压缩(b)及横向剪切(c)模式下的失效模式
Figure 11. Failure patterns of hygroscopic saturated CF/PA6 composite in the tension (a), compression (b) and shear simulation (c)
表 1 CF/PA6复合材料各组分材料在50℃浸水环境的吸湿参数
Table 1. Water absorption parameters for component materials in the CF/PA6 composites immersed at 50℃ water bath
Parameter Diffusivity/(106 mm2·s−1) Water content/% PA6 4.64 9.40 CF 0.00 0.00 Voids 46.40 23.50 CF/PA6 2.16 5.26 
下载: 导出CSV
表 2 CF/PA6复合材料力学性能
Table 2. Mechanical properties for CF/PA6 composites
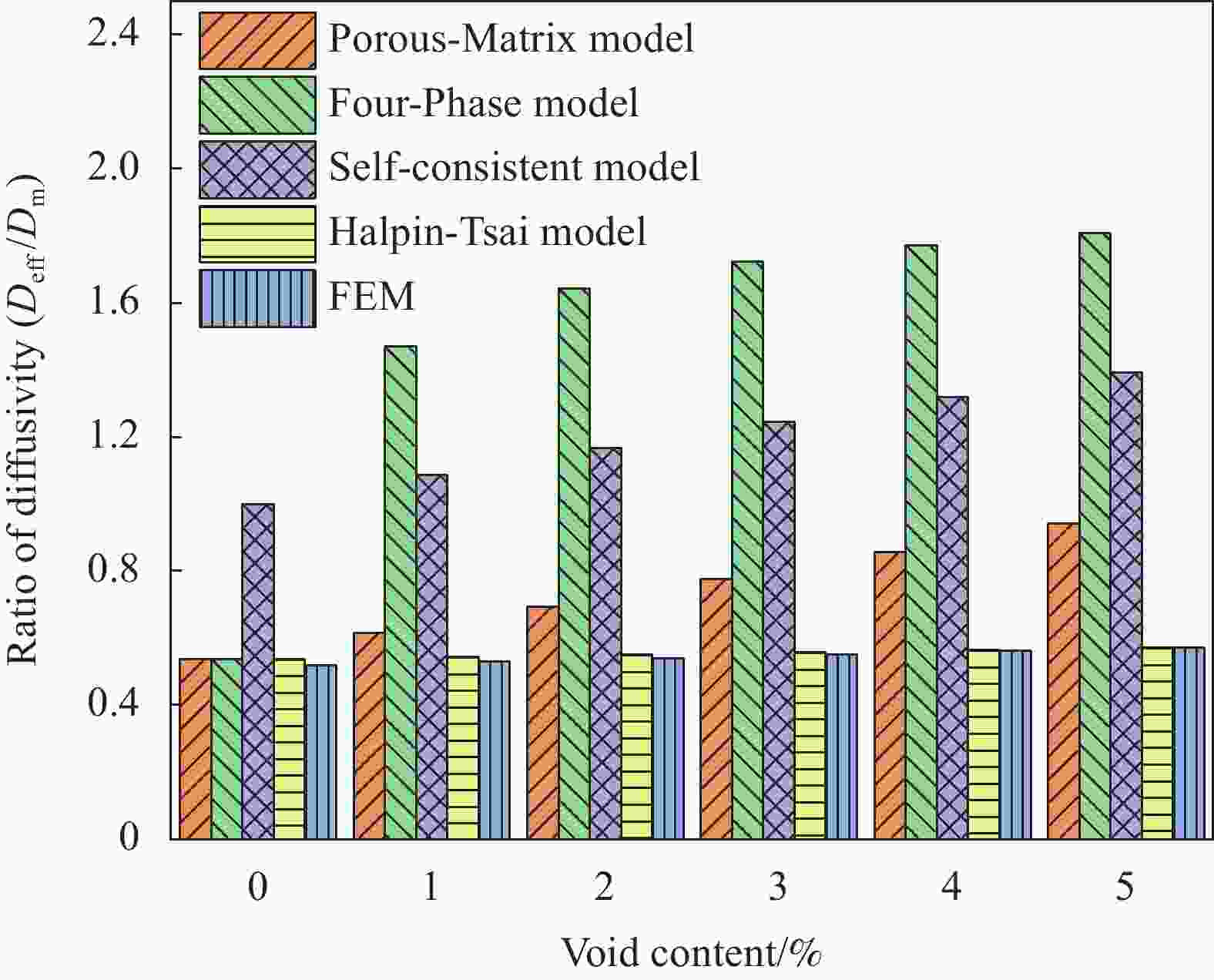
Fiber $E_2^{\rm f}/{\text{GPa}}$ $\mu _{23}^{\rm f}$ $\alpha^{\mathrm{f}} /\left(10^{-6} {\text{℃}}^{-1}\right)$ $ {\beta ^{\rm f}} $ ${\rho ^{\rm f}}/({\text{kg} } \cdot { {\text{m} }^{ {\text{-3} } } })$ 16.54 0.25 −0.83 0 1810 Matrix $ {E^{\rm m}}/{\text{GPa}} $ ${\mu ^{\rm m}}$ ${\sigma _{y{\rm t}}}/{\text{MPa}}$ $ {\sigma _{y{\rm c}}}/{\text{MPa}} $ $\alpha^{\mathrm{m}} /\left(10^{-6} {\text{℃}} ^{-1}\right)$ 2.19 0.34 25 50 4 $ {\beta ^{\rm m}} $ ${\rho ^{\rm m}}/({\text{kg}} \cdot {{\text{m}}^{{{ - 3}}}})$ 0.1 1080 Interface $ K_{\rm n}^0/({\text{N}} \cdot {\text{m}}{{\text{m}}^{{{ - 3}}}}) $ $K_{\rm s}^0/({\text{N}} \cdot {\text{m}}{{\text{m}}^{{{ - 3}}}})$ $K_{\rm t}^0/({\text{N}} \cdot {\text{m}}{{\text{m}}^{{{ - 3}}}})$ $t_{\rm n}^0/{\text{MPa}}$ $t_{\rm s}^0/{\text{MPa}}$ $3.13 \times {10^4}$ $5.0 \times {10^4}$ $5.0 \times {10^4}$ 17.11 40.67 $t_{\rm t}^0/{\text{MPa}}$ $G_{\rm n}^{\rm c}{\text{/(N}} \cdot {\text{m}}{{\text{m}}^{ - 1}}{\text{)}}$ $G_{\rm s}^{\rm c}{\text{/(N}} \cdot {\text{m}}{{\text{m}}^{ - 1}}{\text{)}}$ $G_{\rm t}^{\rm c}{\text{/(N}} \cdot {\text{m}}{{\text{m}}^{ - 1}}{\text{)}}$ 40.67 0.22 0.23 0.23 Notes: $E_2^{\rm f}$ and $ {E^{\rm m}} $—Tensile modulus of the carbon fiber and the PA6 matrix; $\mu _{23}^{\rm f}$ and ${\mu ^{\rm m}}$—Poisson's ratio of the carbon fiber and the PA6 matrix; $ {\alpha ^{\rm f}} $ and $ {\alpha ^{\rm m}} $—Coefficient of thermal expansion for the carbon fiber and the PA6 matrix; $ {\beta ^{\rm f}} $ and $ {\beta ^{\rm m}} $—Coefficient of moisture expansion for the carbon fiber and the PA6 matrix; ${\rho ^{\rm f}}$ and ${\rho ^{\rm m}}$—Density of the carbon fiber and the PA6 matrix; ${\sigma _{y{\rm t}}}$ and $ {\sigma _{y{\rm c}}} $—Tensile and compressive yield strength of the PA6 matrix; $ K_{\rm n}^0 $, $K_{\rm s}^0$ and $K_{\rm t}^0$—Interfacial stiffness; $t_{\text{n}}^{\text{0}}$, $t_{\text{s}}^{\text{0}}$ and $t_{\text{t}}^{\text{0}}$—Interfacial strength; $G_{\text{n}}^{\text{c}}$, $G_{\text{s}}^{\text{c}}$ and $G_{\text{t}}^{\text{c}}$—Fracture toughness.
下载: 导出CSV
表 3 含孔隙缺陷复合材料的相对扩散系数的预测模型[17]
Table 3. Several theoretical models for calculating effective diffusion coefficient of composites containing voids[17]
Model Expression Porous-Matrix model $ \dfrac{{{D_{{\rm{eff}}}}}}{{{D_{\rm{m}}}}} = \left[ {1 + 2{\alpha _{{\rm{mv}}}}{V_{\rm{v}}}\dfrac{{\left( {{{{D_{\rm{v}}}}/ {{D_{\rm{m}}}}}} \right) - 1}}{{\left( {{{{D_{\rm{v}}}} / {{D_{\rm{m}}}}}} \right) + 1}}} \right]\dfrac{{1 - {V_{\rm{f}}}}}{{1 + {V_{\rm{f}}}}} $，
${\alpha _{ {\rm{mv} } } } = \dfrac{ { {\rho _{ {\rm{water} } } } } }{ { {M_{\rm{m} } }{\rho _{\rm{m}}}(1 - {v_{ {\rm{void} } } })} }$Four-Phase
model$ \dfrac{{{D_{{\rm{eff}}}}}}{{{D_{\rm{m}}}}} = \dfrac{{(1 - k{V_{\rm{f}}})(1 + k) + \varPhi (1 + k{V_{\rm{f}}})(k - 1)}}{{(1 + k{V_{\rm{f}}})(1 + k) + \varPhi (1 - k{V_{\rm{f}}})(k - 1)}} $，
$ k = {{({V_{\rm{v}}} + {V_{\rm{f}}})} / {{V_{\rm{f}}}}} $, $ \varPhi = {\alpha _{{\rm{mv}}}}({{{D_{\rm{v}}}} / {{D_{\rm{m}}}}}) $Self-consistent model $ \dfrac{{{D_{{\rm{eff}}}}}}{{{D_{\rm{m}}}}} = \dfrac{1}{{2(1 + k)}}\left( {\lambda + \sqrt {4\dfrac{{{D_{\rm{v}}}}}{{{D_{\rm{m}}}}}({k^2} - 1) + {\lambda ^2}} } \right) $，
$ \lambda = \dfrac{{{D_{\rm{v}}}}}{{{D_{\rm{m}}}}}\left( {1 + k\left( {2{V_{\rm{v}}} - 1} \right)} \right) + \left( {1 + k\left( {1 - 2{V_{\rm{v}}}} \right)} \right) $Notes: $ {D_{\rm{v}}} $, $ {D_{\rm{m}}} $ and $ {D_{{\rm{eff}}}} $—Diffusivity of voids, matrix and composites; $ {V_{\rm{f}}} $ and Vv—Volume fraction of carbon fiber and voids in CF/PA6 composites; $ {\alpha _{{\rm{mv}}}} $, $ k $, $ \varPhi $ and $ \lambda $—Intermediate variable; Vvoid—Void content in the matrix; ρm and ρwater—Density of matrix and water; Mm—Absorbed water content in the matrix.
下载: 导出CSV
表 4 CF/PA6复合材料不同孔隙率RVE模型的湿热老化前后的裂纹数量对比
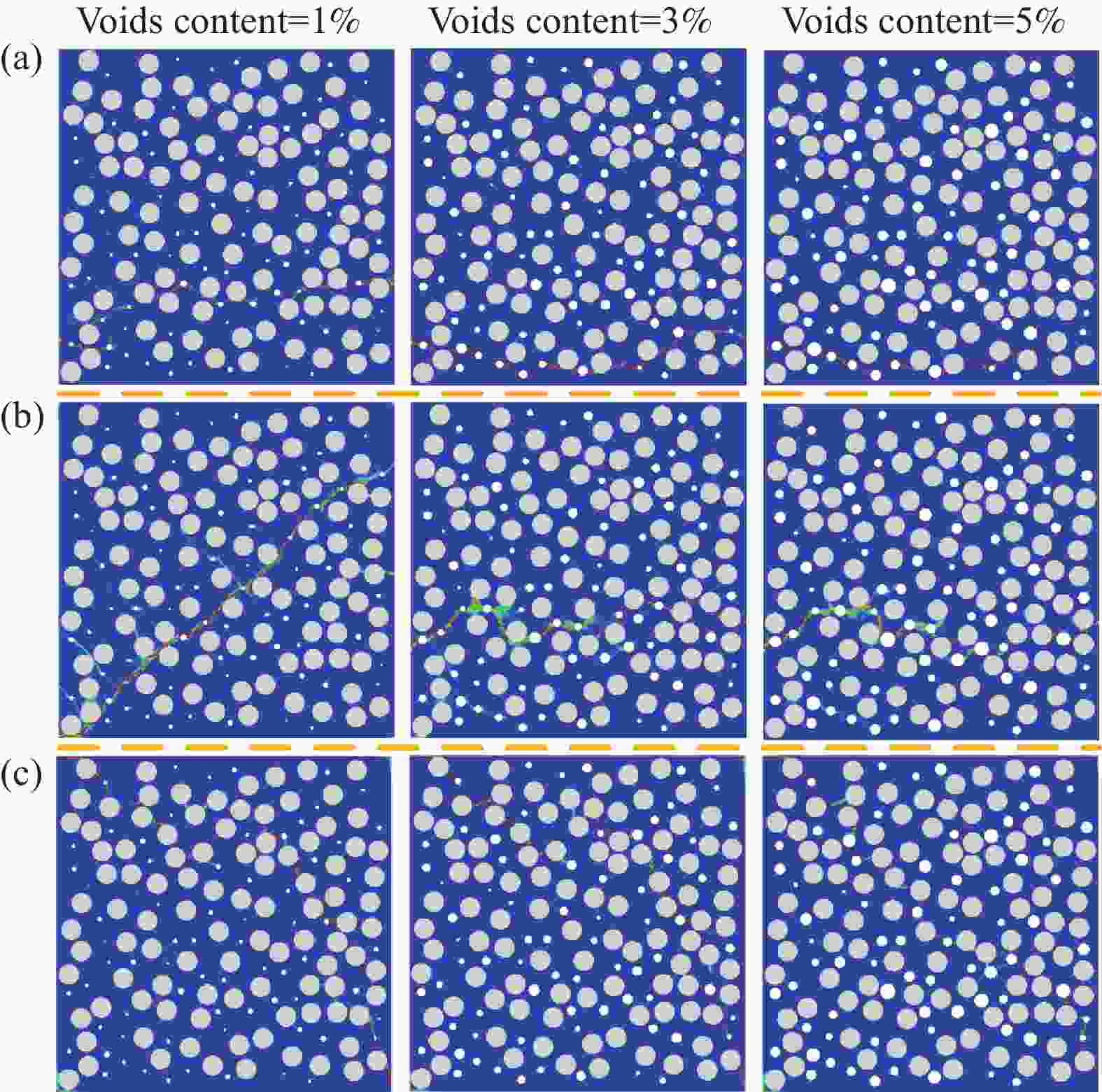
Table 4. Comparison of the number of cracks in RVE models of CF/PA6 composites with different porosities before and after hygrothermal aging
Porosity/% Unaged Aged Tension Compression Shear Tension Compression Shear 1 4 2 3 9 10 5 2 5 2 2 10 9 3 3 1 3 3 9 15 6 4 1 2 2 7 14 6 5 2 2 2 10 14 5
下载: 导出CSV
-
[1] GRUNENFELDER L K, NUTT S R. Void formation in compo-site prepregs–Effect of dissolved moisture[J]. Composites Science and Technology,2010,70(16):2304-2309. doi: 10.1016/j.compscitech.2010.09.009 [2] MEHDIKHANI M, STRAUMIT I, GORBATIKH L, et al. Detailed characterization of voids in multidirectional carbon fiber/epoxy composite laminates using X-ray micro-computed tomography[J]. Composites Part A: Applied Science and Manufacturing,2019,125:105532. doi: 10.1016/j.compositesa.2019.105532 [3] LUO L, ZHANG B, LEI Y, et al. Identification of voids and interlaminar shear strengths of polymer-matrix compo-sites by optical microscopy experiment and deep learning methodology[J]. Polymers for Advanced Technologies,2021,32(4):1853-1865. doi: 10.1002/pat.5226 [4] COSTA M L, DE ALMEIDA S F M, REZENDE M C. The influence of porosity on the interlaminar shear strength of carbon/epoxy and carbon/bismaleimide fabric laminates[J]. Composites Science and Technology,2001,61(14):2101-2108. doi: 10.1016/S0266-3538(01)00157-9 [5] LITTLE J E, YUAN X, JONES M I. Characterisation of voids in fibre reinforced composite materials[J]. NDT & E International,2012,46:122-127. [6] MANTA A, GRESIL M, SOUTIS C. Infrared thermography for void mapping of a graphene/epoxy composite and its full-field thermal simulation[J]. Fatigue & Fracture of Engineering Materials & Structures,2019,42(7):1441-1453. [7] TRETIAK I, SMITH R A. A parametric study of segmentation thresholds for X-ray CT porosity characterisation in composite materials[J]. Composites Part A: Applied Science and Manufacturing,2019,123:10-24. doi: 10.1016/j.compositesa.2019.04.029 [8] 李波, 万小朋, 赵美英. 孔隙率对复合材料单向板横向力学性能的影响[J]. 玻璃钢/复合材料, 2017(6):33-38.LI Bo, WAN Xiaopeng, ZHAO Meiying. The influence of void contents on transverse mechanical properties of unidirectional composites[J]. Fiber Reinforced Plastics/Composites,2017(6):33-38(in Chinese). [9] 梁向雨, 林莉, 陈军, 等. 孔隙尺寸离散度大的碳纤维增强复合材料随机孔隙建模方法研究[J]. 航空材料学报, 2013, 33(3):81-85.LIANG Xiangyu, LIN Li, CHEN Jun, et al. Random void modeling for carbon fibre reinforced composite with highly dispersed void size[J]. Journal of Aeronautical Materials,2013,33(3):81-85(in Chinese). [10] HUANG H, TALREJA R. Effects of void geometry on elastic properties of unidirectional fiber reinforced composites[J]. Composites Science and Technology,2005,65(13):1964-1981. doi: 10.1016/j.compscitech.2005.02.019 [11] NIKOPOUR H. A virtual frame work for predication of effect of voids on transverse elasticity of a unidirectionally reinforced composite[J]. Computational Materials Science,2013,79:25-30. doi: 10.1016/j.commatsci.2013.05.049 [12] ASHOURI VAJARI D, GONZÁLEZ C, LLORCA J, et al. A numerical study of the influence of microvoids in the transverse mechanical response of unidirectional composites[J]. Composites Science and Technology,2014,97:46-54. doi: 10.1016/j.compscitech.2014.04.004 [13] DONG C. Effects of process-induced voids on the properties of fibre reinforced composites[J]. Journal of Materials Science & Technology,2016,32(7):597-604. [14] WANG M, ZHANG P, FEI Q, et al. Computational evaluation of the effects of void on the transverse tensile strengths of unidirectional composites considering thermal residual stress[J]. Composite Structures,2019,227:111287. doi: 10.1016/j.compstruct.2019.111287 [15] MEHDIKHANI M, PETROV N A, STRAUMIT I, et al. The effect of voids on matrix cracking in composite laminates as revealed by combined computations at the micro- and meso-scales[J]. Composites Part A: Applied Science and Manufacturing,2019,117:180-192. doi: 10.1016/j.compositesa.2018.11.009 [16] HYDE A, HE J, CUI X, et al. Effects of microvoids on strength of unidirectional fiber-reinforced composite materials[J]. Composites Part B: Engineering,2020,187:107844. doi: 10.1016/j.compositesb.2020.107844 [17] GUERIBIZ D, RAHMANI M, JACQUEMIN F, et al. Homogenization of moisture diffusing behavior of composite materials with impermeable or permeable fibers—Application to porous composite materials[J]. Journal of Compo-site Materials,2009,43(12):1391-1408. doi: 10.1177/0021998308104229 [18] BOURENNANE H, GUERIBIZ D, FRÉOUR S, et al. Modeling the effect of damage on diffusive behavior in a polymeric matrix composite material[J]. Journal of Reinforced Plastics and Composites,2019,38(15):717-733. doi: 10.1177/0731684419845479 [19] LEI Y, ZHANG J, ZHANG T, et al. Water diffusion in carbon fiber reinforced polyamide 6 composites: Experimental, theoretical, and numerical approaches[J]. Journal of Reinforced Plastics and Composites,2019,38(12):578-587. doi: 10.1177/0731684419835034 [20] SANG L, WANG C, WANG Y, et al. Effects of hydrothermal aging on moisture absorption and property prediction of short carbon fiber reinforced polyamide 6 composites[J]. Composites Part B: Engineering,2018,153:306-314. doi: 10.1016/j.compositesb.2018.08.138 [21] SANG L, WANG Y, WANG C, et al. Moisture diffusion and damage characteristics of carbon fabric reinforced polyamide 6 laminates under hydrothermal aging[J]. Composites Part A: Applied Science and Manufacturing,2019,123:242-252. doi: 10.1016/j.compositesa.2019.05.023 [22] American Society of Testing Materials. Standard test method for moisture absorption properties and equilibrium conditioning of polymer matrix composite materials: ASTM D5229M-14[S]. West Conshohocken: American Standards Press, 2014. [23] American Society of Testing Materials. Standard test method for tensile properties of plastics: ASTM D638-14[S]. West Conshohocken: American Standards Press, 2014. [24] American Society of Testing Materials. Standard test method for tensile properties of polymer matrix composite materials: ASTM D3039-14[S]. West Conshohocken: American Standards Press, 2014. [25] ALTENBACH H, BOLCHOUN A, KOLUPAEV V A. Phenomenological yield and failure criteria[M]. Springer: Plasticity of Pressure-sensitive Materials, 2014: 49-152. [26] BRÜNIG M, CHYRA O, ALBRECHT D, et al. A ductile damage criterion at various stress triaxialities[J]. International Journal of Plasticity,2008,24(10):1731-1755. doi: 10.1016/j.ijplas.2007.12.001 [27] PARÍS F, CORREA E, CAÑAS J. Micromechanical view of failure of the matrix in fibrous composite materials[J]. Composites Science and Technology,2003,63(7):1041-1052. doi: 10.1016/S0266-3538(03)00017-4 [28] GONZÁLEZ C, LLORCA J. Mechanical behavior of unidirectional fiber-reinforced polymers under transverse compression: Microscopic mechanisms and modeling[J]. Composites Science and Technology,2007,67(13):2795-2806. doi: 10.1016/j.compscitech.2007.02.001 [29] JIA L, YU L, ZHANG K, et al. Combined modelling and experimental studies of failure in thick laminates under out-of-plane shear[J]. Composites Part B: Engineering,2016,105:8-22. doi: 10.1016/j.compositesb.2016.08.017 [30] HUANG Z M. Micromechanical failure analysis of unidirectional composites[M]. England: Failure Analysis, IntechOpen, 2018: 43. [31] AFFDL J C H, KARDOS J L. The Halpin-Tsai equations: A review[J]. Polymer Engineering and Science,1976,16(5):344-352. doi: 10.1002/pen.760160512 -
下载:
点击查看大图
计量
- 文章访问数: 1194
- HTML全文浏览量: 757
- PDF下载量: 79
- 被引次数: 0
