石墨烯/聚甲基丙烯酸甲酯纳米复合材料的拉伸性能：粗粒化分子动力学模拟

侯国珍; 陈小明; 丁鹏; 马河川; 张洁; 邵金友; 吴建洋

doi:10.13801/j.cnki.fhclxb.20210707.004

石墨烯/聚甲基丙烯酸甲酯纳米复合材料的拉伸性能：粗粒化分子动力学模拟

侯国珍^1,,
陈小明^1, ,,
丁鹏^1,,
马河川^1,,
张洁^2,,
邵金友^1,,
吴建洋^3,

1.
西安交通大学　机械工程学院，西安 710049
2.
西安交通大学　电子与信息工程学院，西安 710049
3.
厦门大学　物理科学与技术学院，厦门 361005

基金项目: 国家自然科学基金(51705411)

详细信息

通讯作者:
陈小明，博士，教授，博士生导师，研究方向为多功能微纳复合材料　E-mail：xiaomingchen@xjtu.edu.cn

中图分类号: TB332
计量
- 文章访问数: 1414
- HTML全文浏览量: 441
- PDF下载量: 112
出版历程
- 收稿日期: 2021-05-17
- 修回日期: 2021-06-26
- 录用日期: 2021-06-28
- 网络出版日期: 2021-07-06
- 刊出日期: 2022-05-31

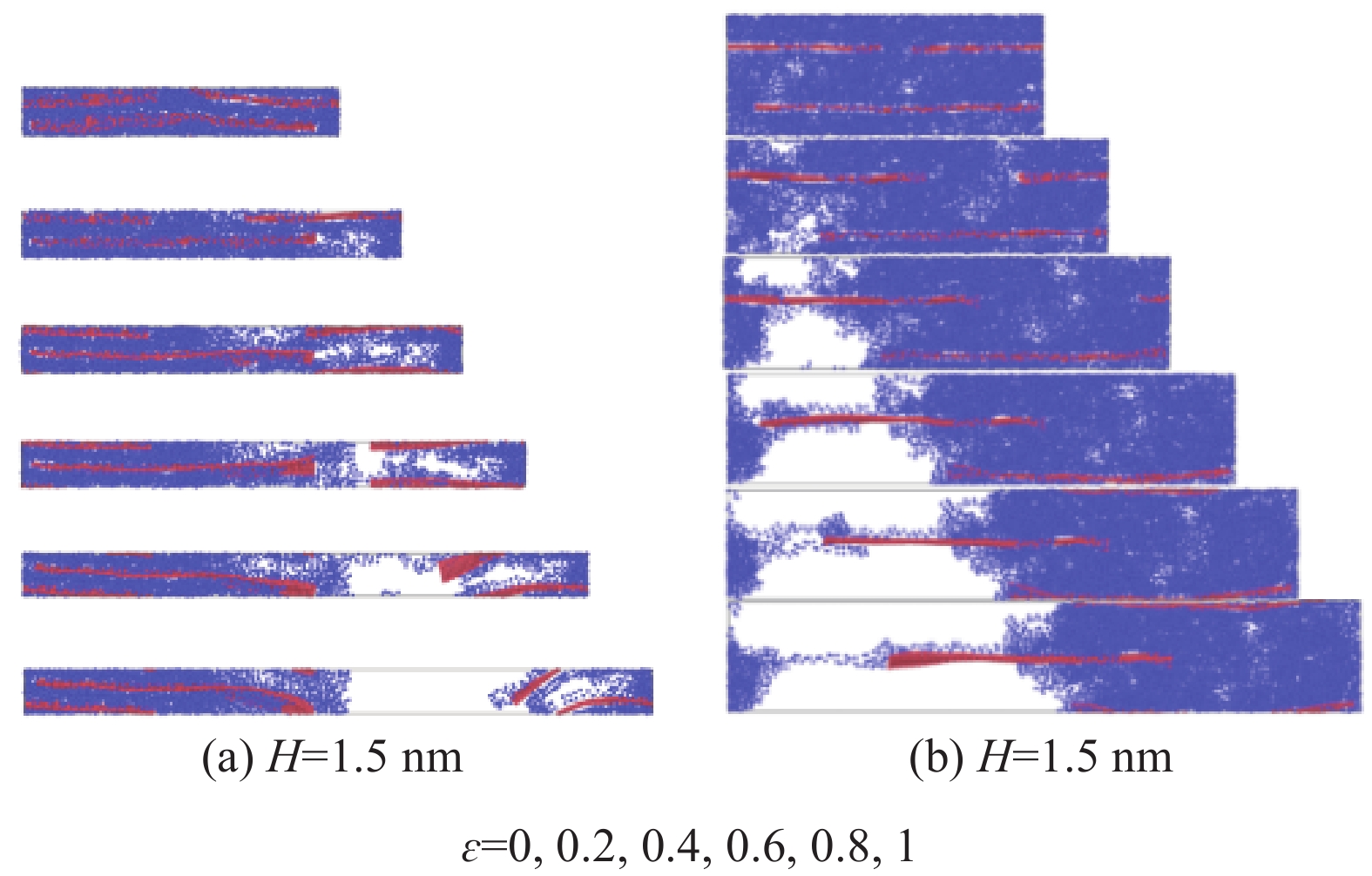
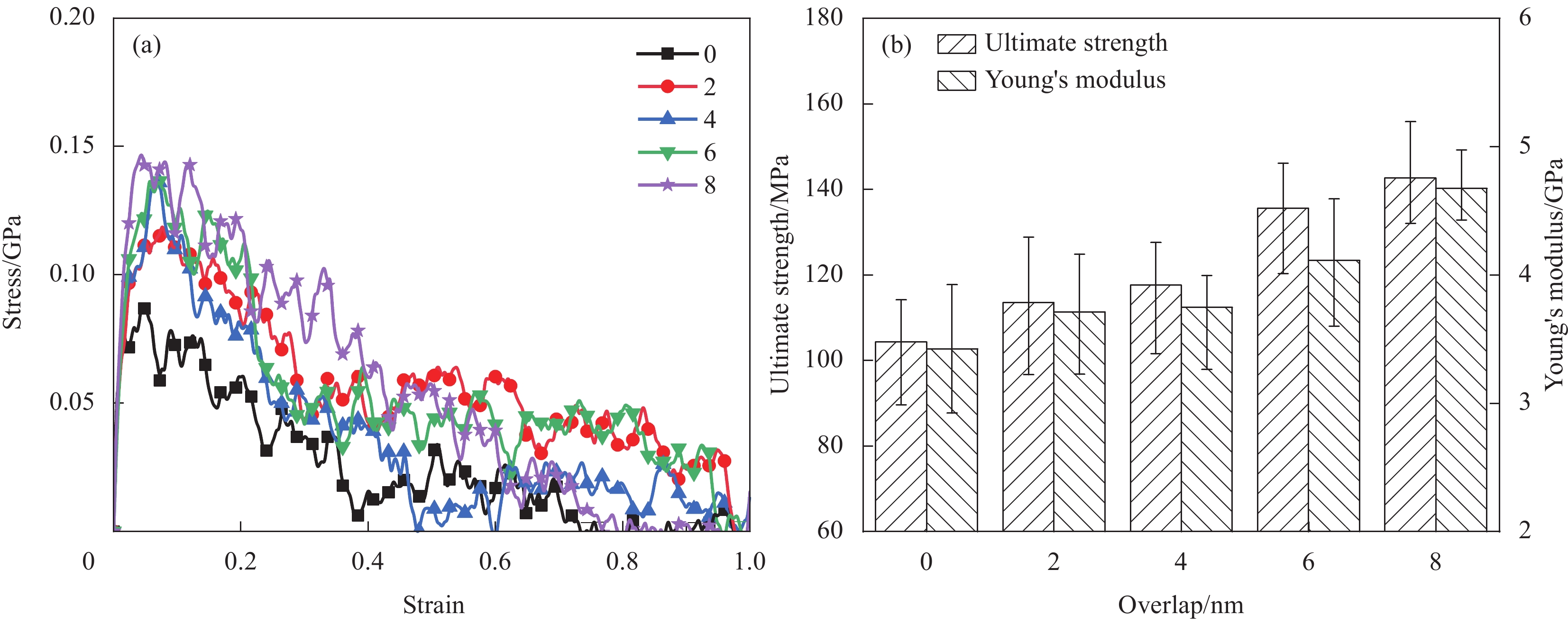
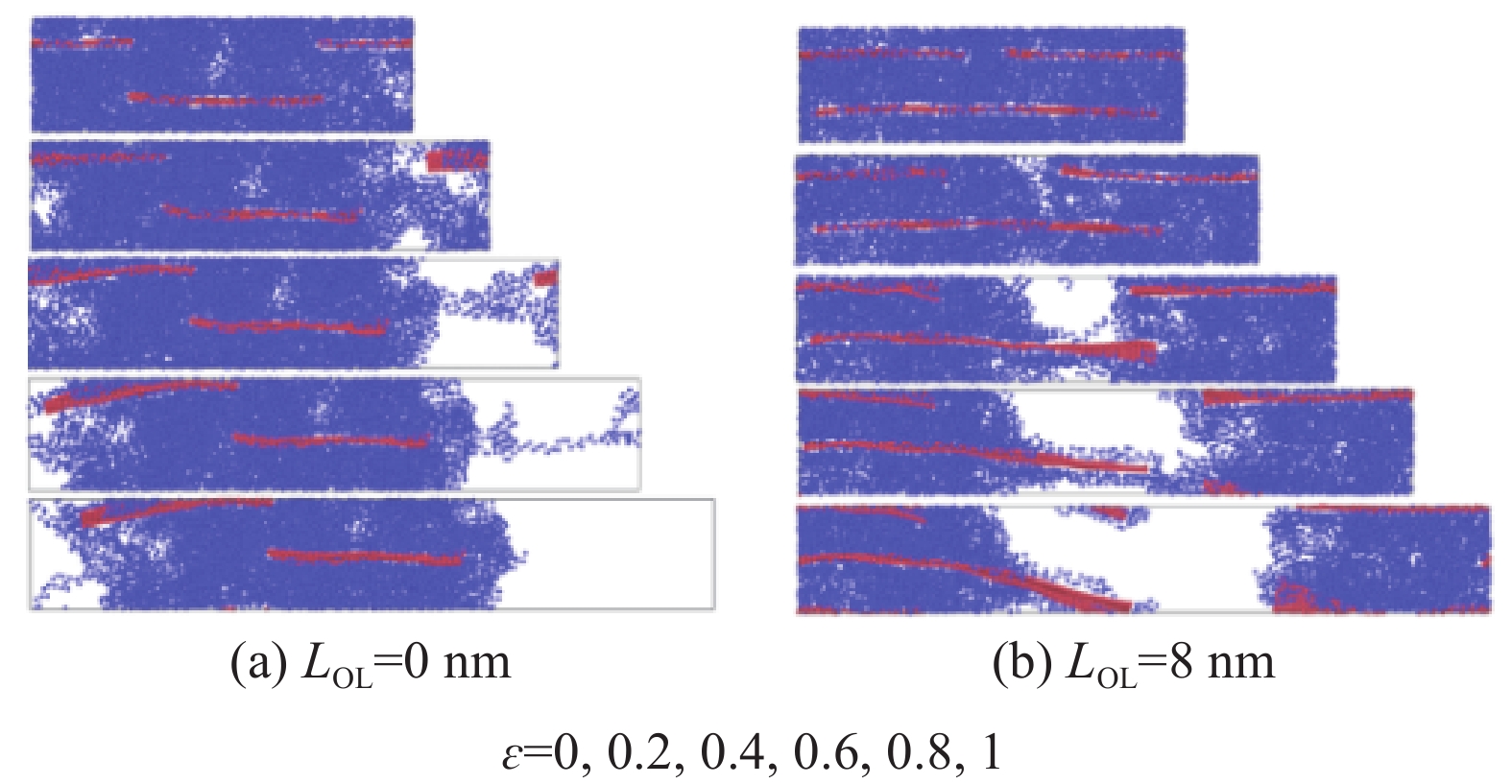
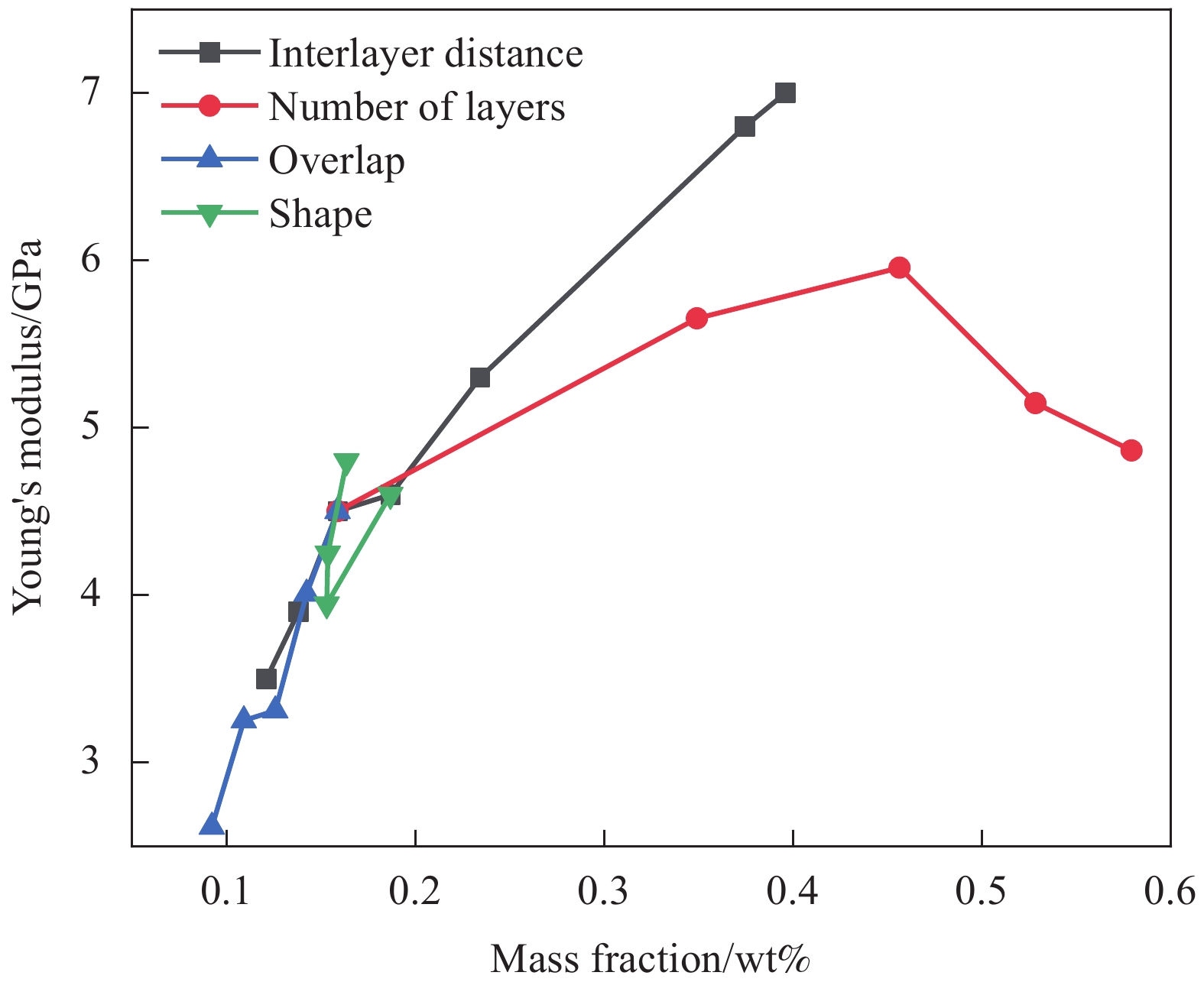
Mechanical performance of graphene/polymethyl-methacrylate nano-composites under tension loads: A coarse-grained molecular dynamic simulation

1.
School of Mechanical Engineering, Xi’an Jiaotong University, Xi’an 710049, China
2.
School of Eletronics and Information Engineering, Xi’an Jiaotong University, Xi’an 710049, China
3.
School of Physical Science and Technology, Xiamen University, Xiamen 361005, China

摘要

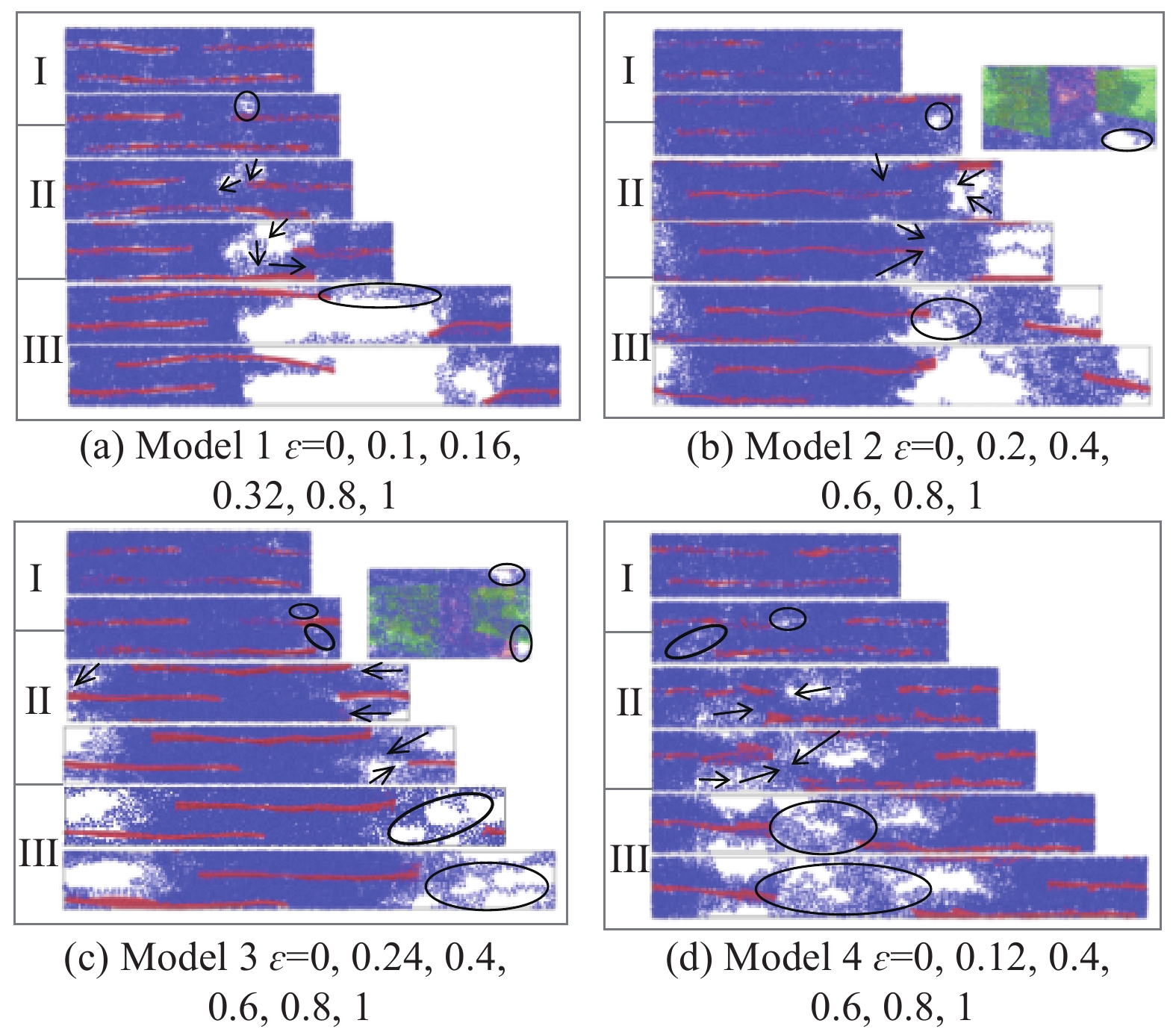
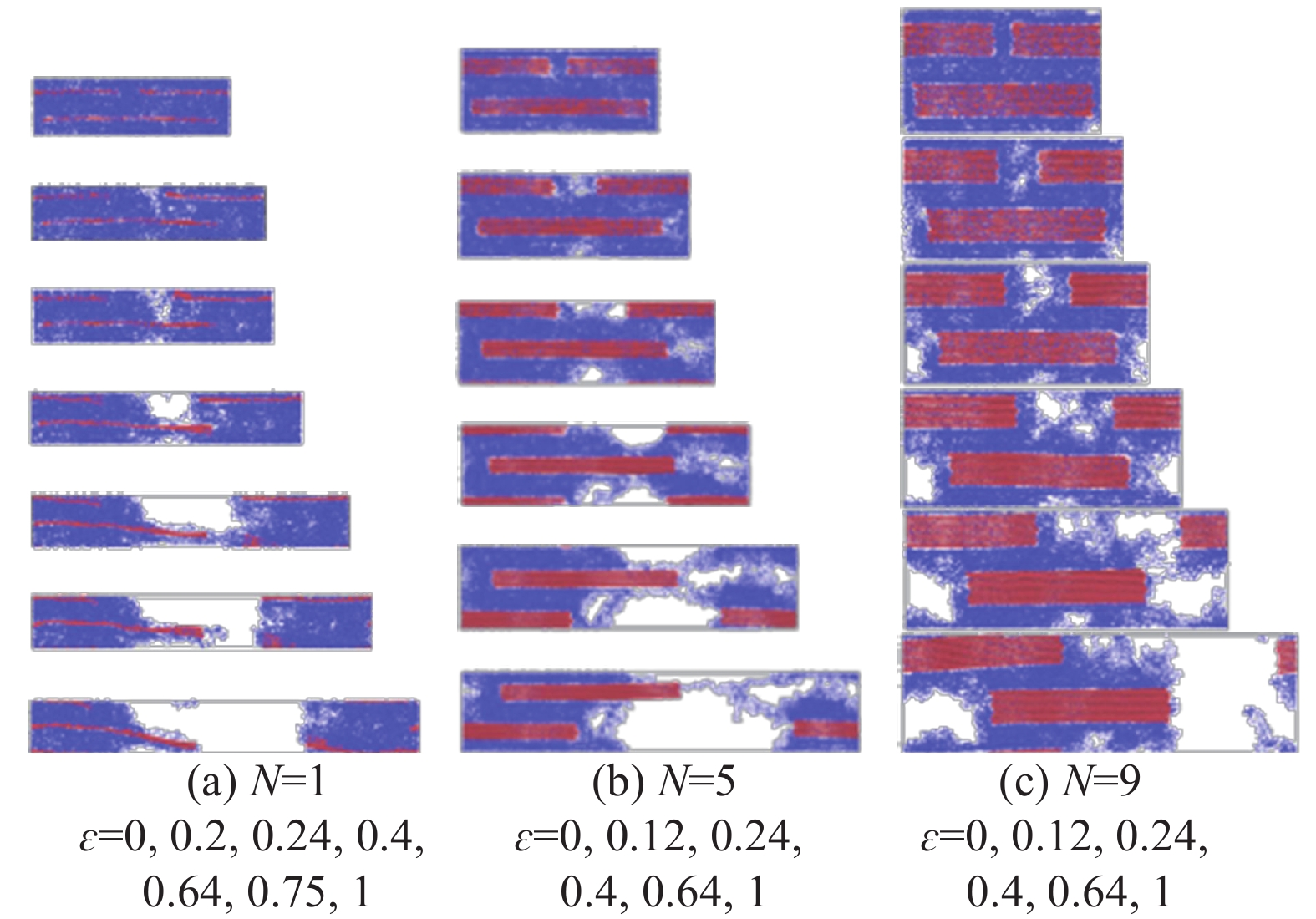
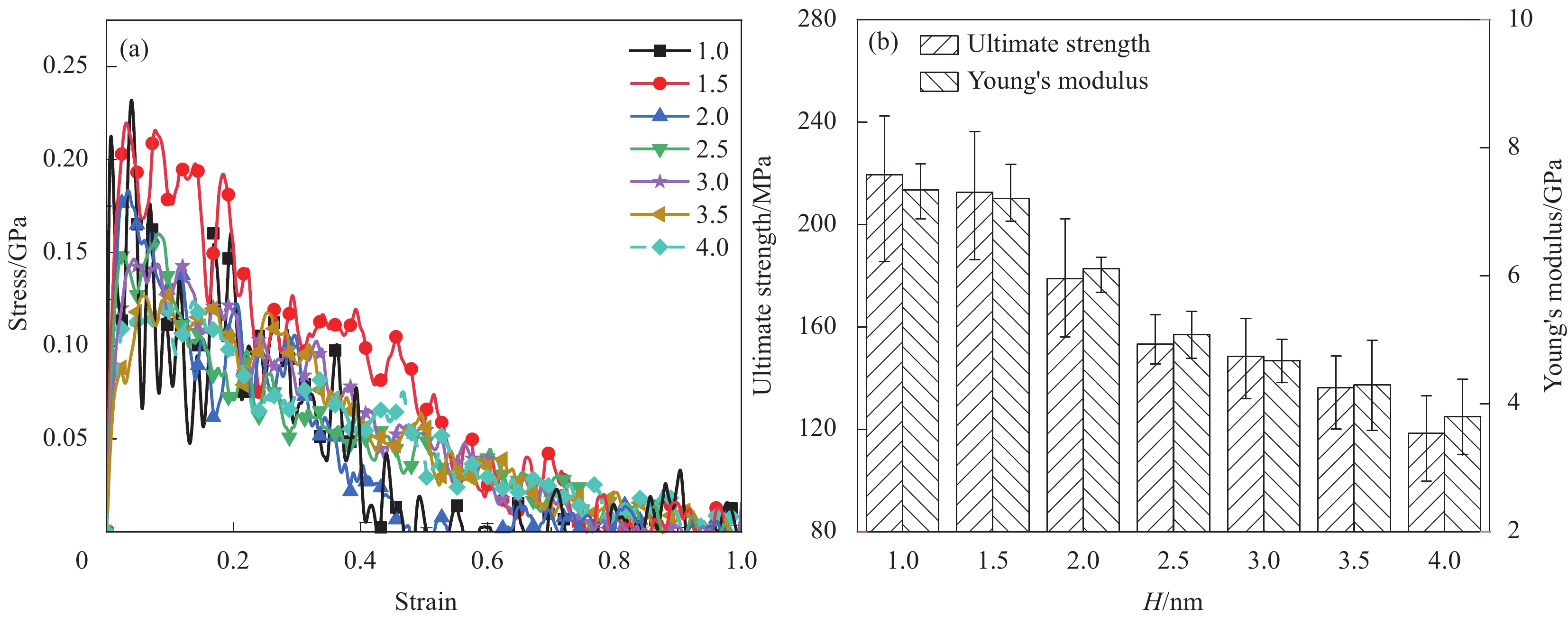
摘要: 高强度是复合材料设计追求的重要目标，自然界中的珍珠层具有优异的力学性能，受其复杂的层次结构的启发，设计了一种石墨烯交错排布增强聚甲基丙烯酸甲酯的纳米复合材料。利用粗粒化分子动力学模拟，系统地研究了拉伸载荷作用下石墨烯的二维几何形状、层数、空间排布对纳米复合材料整体力学性能的影响。结果表明，不同几何形状的石墨烯对复合材料的增强效果有很大的差异，其中，矩形与锯齿形接近，都强于梯形石墨烯；存在最佳的石墨烯层数使复合材料的整体拉伸力学性能最强；减少石墨烯层间距离或增加重叠距离，都可提升其力学性能。总之，现有的研究结果揭示了各个因素的影响规律及微观机制，为设计具有目标性能的纳米复合材料提供了理论指导。
- 粗粒化分子动力学 /
- 石墨烯/聚甲基丙烯酸甲酯纳米复合材料 /
- 拉伸 /
- 几何形状 /
- 力学性能
Abstract: Strength is a important factor to consider when designing high-performance composite materials. Inspired by the excellent mechanical properties and complex hierarchical structure of nacre, a nanocomposite was designed, in which the graphene layers were interlaced in the polymethyl methacrylate matrix. Coarse-grained molecular dynamics simulations were used to investigate the effect of various geometrical variations on the mechanical properties under tension loads, including the two-dimensional geometric shapes of graphene, the number of graphene layers, the interlayer distance of the graphene sheets and the overlap length of the graphene sheets. The simulation results show that the strengthening effects of different geometries of graphenes on composites are very different, among which, the rectangle and sawtooth shapes are close to each other and are stronger than the trapezoidal graphene. There is an optimal number of graphene layers to make the composite have the strongest overall mechanical properties. The mechanical properties of graphene can be improved by reducing the interlayer distance of the graphene sheets or increasing the overlap length of the graphene sheets. Overall, this paper systematically studies the influencing factors of graphene-reinforced polymer composites and reveals the influence rules and microscopic mechanisms of each factor. This study provides a useful guidance for the design of nanocompo-sites with targeted properties.
- coarse-grained molecular dynamics /
- graphene/polymethyl-methacrylate nanocomposites /
- tensile /
- geometric shapes /
- mechanical properties

HTML全文

随着我国桥梁建设的快速发展，交通量的增加，桥梁结构遭遇火灾情况也时有发生^[1-4]，2007年10月广东广深高速虎门大桥，油罐车爆炸引发大火，拉索和桥墩都被大火湮灭；2014年，湖南郴州在建赤石特大桥在主跨合拢前6号桥墩左幅塔顶突发大火，事故导致6号桥墩左幅9根斜拉索断裂，这些火灾事故对缆索的受力性能构成了极大的考验。文献[5-8]对钢丝缆索的高温力学性能进行研究，在火灾高温下钢丝力学性能会明显下降，导致缆索的承载能力急剧下降。

采用轻质、高强、耐腐蚀、抗疲劳的碳纤维增强树脂复合材料(Carbon fiber reinforced polymer，CFRP)用于桥梁缆索，可提高桥梁跨径，从根本上解决钢质拉索的腐蚀及疲劳问题。但CFRP索内的CFRP筋遇到火灾后环氧树脂会燃烧分解，影响其极限承载性能，对桥梁结构的安全造成影响。文献[9-12]通过试验研究发现，高温下CFRP筋的力学性能下降十分明显。付成龙等^[11]研究了温度对CFRP筋弯曲强度和压缩强度的影响，研究显示温度对试样弯曲强度和压缩强度的影响较大，CFRP筋的强度保留率随温度升高而降低。方志等^[12]对较高玻璃化转变温度T_g(T_g >200℃)的CFRP筋高温后力学性能进行研究，处理温度为100℃时，筋材静力性能与常温试件相比未发生明显变化，筋材经历200℃和300℃温升作用后，其抗拉强度、弹性模量和极限拉应变均有所下降。

文献[13-15]对桥梁缆索的阻燃防火措施做了一些研究。李艳等^[13]在索体外表面设置一种导热系数很低的耐高温防火涂层，从而降低火源热辐射传给索体的温度。张凯等^[14]研究了带砂浆包覆层CFRP筋的高温力学性能，在砂浆包覆层保持完好未爆裂的情况下，包覆层为CFRP筋提供了较好的隔氧环境，CFRP筋在长时间高温作用后具有较高的残余强度。徐玉林等^[15]对外包陶瓷纤维防火层的CFRP索的耐火性进行了火灾试验研究，对CFRP 缆索外包陶瓷纤维防火层可大幅提高缆索的临界安全耐火时长。

综上所述，目前已有一些缆索的阻燃防火措施，如外包砂浆或陶瓷纤维防火层，但这些措施会大幅度增大索体直径，严重影响索体外表面的空气动力学特性。本文针对桥梁缆索用CFRP筋在高温下的力学性能及CFRP索的阻燃防火措施进行系统研究，研制开发具有阻燃防火特性的CFRP索，避免火灾带来的风险，保障应用安全，有助于CFRP索的推广应用。

1. CFRP筋高温力学性能

CFRP筋采用拉挤成型工艺制备，为了便于锚固，筋材表面带有螺旋肋，筋材底径7 mm，纤维体积分数为72vol%，密度为1.52 g/cm³，玻璃化转变温度T_g为120℃。

图1为CFRP筋高温拉伸试验。可见，筋材两端采用粘结型锚固方式，筋材锚固后穿过试验台架，在筋材中间自由段部位外套金属铝筒，金属铝筒外缠绕加热带对筒内空气进行加热，采用热电偶监测空气温度，采用温度继电器控制温度，使金属铝筒内温度保持设定温度，采用千斤顶加载，加载速度不超过300 MPa/min。筋材拉伸强度为筋材破断时压力传感器载荷读数除以筋材承载面积。

图 1 碳纤维增强树脂复合材料(CFRP)筋高温拉伸试验

Figure 1. High temperature tensile test of carbon fiber reinforced polymer (CFRP) tendon

下载: 全尺寸图片幻灯片

1.1 CFRP筋不同加热温度下力学性能

对筋材中间自由段部位进行加热，加热至指定温度，保温2 h后进行破断拉伸试验，获得筋材在高温下的拉伸强度。

图2为不同温度下保温 2 h后的CFRP筋材抗拉强度。可以看出，随着试验温度的升高，筋材拉伸强度呈线性下降趋势，270℃加热2 h，筋材强度降为2000 MPa左右，210℃加热2 h，筋材强度最低为2245.8 MPa，比初始强度下降26.13%。图3为保温2 h后筋材高温拉伸破断照片。可以看出，筋材发生了散丝状断裂。

图 2 不同温度下保温 2 h后的CFRP筋材抗拉强度

Figure 2. Tensile strength of CFRP tendons at different temperatures with heat preservation 2 h

下载: 全尺寸图片幻灯片

图 3 CFRP筋材高温拉伸破断状态

Figure 3. Tensile fracture state of CFRP tendons at high temperature

下载: 全尺寸图片幻灯片

1.2 CFRP筋不同加热时间下力学性能

对筋材中间自由段部位进行加热，加热至210℃，分别保温1、2、3 h后进行破断拉伸试验，获得筋材在高温下的拉伸强度。图4为210℃不同保温时间下的CFRP筋材抗拉强度。

图 4 210℃不同保温时间下的CFRP筋材抗拉强度

Figure 4. Tensile strength of CFRP tendons with different holding time at 210℃

下载: 全尺寸图片幻灯片

可以看出，筋材高温拉伸强度仅与试验温度有关，当筋材芯部温度达到保温温度时，筋材的高温拉伸强度与保温时间无关，210℃的高温3 h内，筋材剩余拉伸强度均能达到2245.8 MPa以上。

1.3 CFRP筋加热冷却后力学性能

对筋材中间自由段部位进行加热，加热至指定温度，保温2 h，待筋材充分冷却至室温后进行破断拉伸试验，获得筋材经历高温冷却后的拉伸强度，如图5所示。可以看出，筋材高温加热冷却后继续进行拉伸试验，拉伸强度会存在一定的可逆性恢复，且恢复后的剩余强度均能达到2800 MPa以上，但最终剩余拉伸强度较原始强度呈略微下降趋势，且加热温度越高，剩余拉伸强度越低，最大下降幅度为6.13%。

图 5 经历不同温度加热2 h冷却后CFRP筋材抗拉强度

Figure 5. Tensile strength of CFRP tendons after heating at different temperatures for 2 h and cooling

下载: 全尺寸图片幻灯片

2. CFRP索阻燃防火措施

分别采用石棉布、陶瓷纤维布及阻燃防火涂层材料来研究对CFRP筋/索的阻燃防火效果。

2.1 石棉布、陶瓷纤维布阻燃防火效果

对在持荷状态下的7 mm直径CFRP筋试验件中间部位用火焰温度1000℃的高温火焰枪进行灼烧，如图6所示，其中图6(a)中筋材无保护，图6(b)中筋材包裹陶瓷纤维布，观测不同时间筋材的受力状态及筋材表面的温度变化，灼烧2 h后，进行破断拉伸试验，获得剩余强度。

表1为不同防护措施下筋材温度及持荷性能。可以看出，在无任何防护条件下，对拉伸应力水平1170 MPa条件下的CFRP筋用火焰温度1000℃的高温火焰枪进行灼烧，25 min后，筋材灼烧部位树脂热解，筋材断裂；采用45 mm厚度陶瓷纤维布与石棉包裹筋材，施加1170 MPa拉伸应力，经过1000℃火焰灼烧2 h，筋材表面温度最高分别为562℃与635℃，筋材高温部位树脂发生热解，没有发生断裂(图7)，剩余强度分别为1646 MPa与1249 MPa，图8为其破断试样；采用60 mm厚度石棉包裹筋材，施加1170 MPa拉伸应力，经过1000℃火焰灼烧2 h，筋材表面温度最高为170℃，筋材完好，没有发生断裂，剩余强度为3121 MPa，筋材基本没有发生损伤。

图 6 持荷条件下CFRP筋阻燃防火措施对比

Figure 6. Comparison on fire retardant measures of CFRP tendons under load conditions

下载: 全尺寸图片幻灯片

表 1 不同防护类型下CFRP筋材温度及持荷性能

Table 1. Temperature and load carrying capacity of CFRP tendons under different protection types

Protection type	Protection thickness/mm	Burning time/min	CFRP tendons temperature/℃	Stress level/MPa	Test result	Resident strength/MPa
—	—	25	1000	1170	Resin pyrolysis, tendon tensile fracture	—
Ceramic fiber cloth	45	120	562	1170	Resin pyrolysis, tendon is not fracture	1646
Asbestos	45	120	635	1170	Resin pyrolysis, tendon is not fracture	1249
Asbestos	60	120	170	1170	The tendon is not damaged	3121

下载: 导出CSV

| 显示表格

图 7 CFRP筋材高温下树脂热解(562℃，2 h)

Figure 7. Resin pyrolysis of tendons at high temperature (562℃, 2 h)

下载: 全尺寸图片幻灯片

图 8 树脂热解后CFRP筋材极限拉伸破断

Figure 8. Ultimate tensile fracture of CFRP tendons after resin pyrolysis

下载: 全尺寸图片幻灯片

以上试验研究可以看出，包裹60 mm厚的石棉可以起到很好的阻燃防火效果，但是过厚的石棉必然影响索体直径，给CFRP索的盘卷带来困难，同时会改变索体表面原有的空气动力学特性，不方便应用。

2.2 阻燃防火涂层

选用一种阻燃防火涂层，刷在CFRP索股索体双层聚乙烯(PE)护套外表面，其中索股直径61 mm，PE护套厚度6 mm，阻燃防火涂层厚度2 mm，如图9所示。所用阻燃防火涂料层由基料丙烯酸乳液、膨胀催化剂聚磷酸铵、碳化剂季戊四醇、膨胀发泡剂三聚氰胺与氯化石蜡、颜料钛白粉、成膜助剂醇酯等组成。

图 9 刷有阻燃防火涂层的CFRP索股

Figure 9. CFRP cable strand coated with fire retardant coating

下载: 全尺寸图片幻灯片

在PE表面刷有2 mm阻燃防火涂层，并在索体PE内表面预埋测温线，用火焰温度1000℃的高温火焰枪对索股局部进行长达2 h的高温灼烧试验(图10)，阻燃防火涂料层发生膨胀并形成均匀而致密蜂窝状碳化层，保护双层PE护套不发生燃烧，使得缆索具有阻燃防火特性，PE护套仅发生软化。无阻燃防火涂层保护的索体5 min内PE护套燃烧殆尽，漏出索体(图11)。图12为2 mm阻燃防火涂层温度-时间曲线。可以看出，2 h灼烧索股PE内表面最高温度为206℃。

图 10 阻燃防火涂层遇火焰发泡

Figure 10. Fire retardant coating foams when expose to fire

下载: 全尺寸图片幻灯片

图 11 无阻燃防火涂层聚乙烯(PE)燃烧

Figure 11. Combustion of polyethylene (PE) sheath without fire retardant coating

下载: 全尺寸图片幻灯片

图 12 2 mm厚阻燃防火涂层温度-时间曲线

Figure 12. Temperature-time curve of 2 mm thickness fire retardant coating

下载: 全尺寸图片幻灯片

2.3 CFRP索股表层不同位置处温度测定

为探究发生火灾时CFRP索股内部PE内筋材温度，将测温线置于不同位置处测量灼烧试验时各位置的温度(图13)，分别为索股PE内表面、距离PE内表面7 mm、距离PE内表面14 mm。图14为灼烧2 h索股内部不同位置处温度-时间曲线。可以看出，紧贴PE内表面的温度最高，为206℃，其次是测温线与PE内表层间隔7 mm处的温度(次外层筋材)，为156℃，温度最低的是与PE内表层距离14 mm处的温度(第三层筋材)，为100℃。

图 13 CFRP索股测温位置

Figure 13. Temperature measurement position of CFRP cable strand

下载: 全尺寸图片幻灯片

图 14 CFRP索股不同位置处温度-时间曲线

Figure 14. Temperature-time curves at different positions of CFRP cable strand

下载: 全尺寸图片幻灯片

3. 阻燃防火涂层耐火时间

针对阻燃防火涂层的不同厚度，试验研究在1000℃火焰灼烧下阻燃防火效果的持续性，索股规格同2.2节。图15为不同厚度阻燃防火涂层温度-时间曲线。可知无阻燃防火涂层防护，索股PE层5 min燃烧殆尽；0.3 mm厚度阻燃防火涂层可保护索股PE层20 min；1.4 mm厚度阻燃防火涂层可保护索股PE层160 min；刷有2 mm厚度阻燃防火涂层的索股在长达360 min的火焰灼烧下，PE内表面最高温度为245℃，PE层未发生破坏，仅发生软化，建议阻燃防火涂层厚度为2 mm。

图 15 不同厚度阻燃防火涂层的温度-时间曲线

Figure 15. Temperature-time curves of fire retardant coating with different thickness

下载: 全尺寸图片幻灯片

图16为2 mm厚度阻燃防火涂层的索股燃烧360 min试验过程的发泡过程。可以看出，随着火焰灼烧时间的增长，发泡层高度逐渐增大，发泡尺寸也逐渐增大，6 h熄火后形成一个6 cm×8 cm、高4 cm的发泡层，长达6 h的灼烧试验，PE内表面最高温度为245℃，熄火后，拨开厚厚的发泡层，PE护套仅发生软化。结合图15与图16，可以看出，燃烧前20 min为快速发泡升温阶段，发泡层快速增大，PE内表面温度从室温上升到196℃；20~140 min为稳定阶段，发泡层缓慢增大，PE内表面温度维持在203~209℃之间；140~360 min为动态平衡阶段，继续燃烧温度缓慢升高，燃烧至180 min，PE内表面温度达到216℃，阻燃防火涂层内层达到发泡温度开始发泡，发泡层高度增加，PE内表面温度下降，燃烧至240 min，PE内表面温度降至200℃，燃烧至280 min左右，发泡层表层开始发生热解，PE内表面温度升高至230℃左右，阻燃防火涂层内层达到发泡温度进一步发泡，发泡层高度持续增加，PE内表面温度下降，但随着发泡层表层热解，PE内表面温度又缓慢上升。

图 16 2 mm厚度阻燃防火涂层的CFRP索股膨胀发泡过程

Figure 16. Intumescent process of CFRP cable strand coated with 2 mm thickness fire retardant coating

下载: 全尺寸图片幻灯片

4. 结论

(1) 碳纤维增强树脂复合材料(Carbon fiber reinforced polymer，CFRP)筋材高温剩余强度随温度升高呈线性下降趋势，210℃加热3 h，剩余强度最低为2245.8 MPa，比初始强度下降26.13%。

(2) CFRP筋材高温加热冷却后强度存在一定程度的可逆性恢复，剩余强度均能达到2800 MPa以上，但较原始强度略微下降，且经历温度越高剩余强度越低，最大下降幅度为6.13%。

(3) 对比3种阻燃防火措施，阻燃防火涂层具有较好的阻燃防火效果，2 h灼烧索股聚乙烯(PE)内表面最高温度为206℃，次外层筋材最高温度为156℃，第三层筋材最高温度为100℃，火灾2 h内，索股仍可承载，剩余强度≥2245 MPa。

(4) 阻燃防火涂层越厚防护时间越长，2 mm厚阻燃防火涂层的索股在长达360 min的火焰灼烧下，PE内表面最高温度为245℃，PE层未发生破坏，仅发生软化，建议阻燃防火涂层的厚度为2 mm。

图 1 石墨烯/PMMA纳米复合材料分子动力学(MD)模型示意图

Figure 1. Schematic diagram of molecular dynamics (MD) model of graphene/PMMA nanocomposites

H—Distance between graphene layers; N—Number of graphene layers; L_OL—Graphene overlap distance; α—Angle of model, 75°

下载: 全尺寸图片幻灯片

图 2 不同石墨烯几何形状的石墨烯/PMMA纳米复合材料与纯PMMA的拉伸应力-应变曲线(a)和杨氏模量与极限强度(b)

Figure 2. Tensile stress-strain curves (a) and Young's modulus and ultimate strength (b) of graphene/PMMA nanocomposites with different graphene geometries and pure PMMA

下载: 全尺寸图片幻灯片

图 3 不同石墨烯几何形状的石墨烯/PMMA纳米复合材料的拉伸变形过程((b)和(c)内的插图分别为xy平面应变为0.2和0.24的快照)

Figure 3. Tensile deformation processes of graphene/PMMA nanocomposites with different graphene geometries (Illustrations in (b) and (c) are snapshots of the xy plane when the strains are 0.2 and 0.24)

ε—Strain

下载: 全尺寸图片幻灯片

图 4 PMMA链与石墨烯的相互作用能变化曲线及聚合物链的构型变化

Figure 4. Variation of the interaction energy between PMMA chain and graphene and the configurational changes of polymer chains

下载: 全尺寸图片幻灯片

图 5 不同石墨烯层数的石墨烯/PMMA纳米复合材料的应力-应变曲线 (a)、杨氏模量与极限强度 (b)和z方向的密度分布 (c)

Figure 5. Tensile stress-strain curves (a), Young's modulus and ultimate strength (b) and the mass density distribution in the z direction(c) of graphene/PMMA nanocomposites with different number of graphene layers

下载: 全尺寸图片幻灯片

图 6 不同石墨烯层数的石墨烯/PMMA纳米复合材料的拉伸变形过程

Figure 6. Tensile deformation processes of graphene/PMMA nanocomposites with different number of graphene layers

下载: 全尺寸图片幻灯片

图 7 不同石墨烯层间距离的石墨烯/PMMA纳米复合材料的应力-应变曲线 (a) 和杨氏模量与极限强度 (b)

Figure 7. Tensile stress-strain curves (a) and Young's modulus and ultimate strength (b) of graphene/PMMA nanocomposites with different interlayer distances between graphene layers

下载: 全尺寸图片幻灯片

图 8 不同石墨烯层间距离的石墨烯/PMMA纳米复合材料的拉伸变形过程

Figure 8. Tensile deformation processes of graphene/PMMA nanocomposites with different interlayer distances between graphene layers

下载: 全尺寸图片幻灯片

图 9 不同石墨烯重叠距离的石墨烯/PMMA纳米复合材料的应力-应变曲线 (a)、杨氏模量和极限强度 (b)

Figure 9. Tensile stress-strain curves (a) and Young's modulus and ultimate strength (b) of graphene/PMMA nanocomposites with different overlap distances between graphene layers

下载: 全尺寸图片幻灯片

图 10 不同石墨烯重叠距离的石墨烯/PMMA纳米复合材料的拉伸变形过程

Figure 10. Tensile deformation processes of graphene/PMMA nanocomposites with different overlap distances between graphene layers

下载: 全尺寸图片幻灯片

图 11 石墨烯/PMMA纳米复合材料杨氏模量与石墨烯质量分数的关系

Figure 11. Variation of the Young’s modulus with the mass fraction of graphene of graphene/PMMA nanocomposites

下载: 全尺寸图片幻灯片

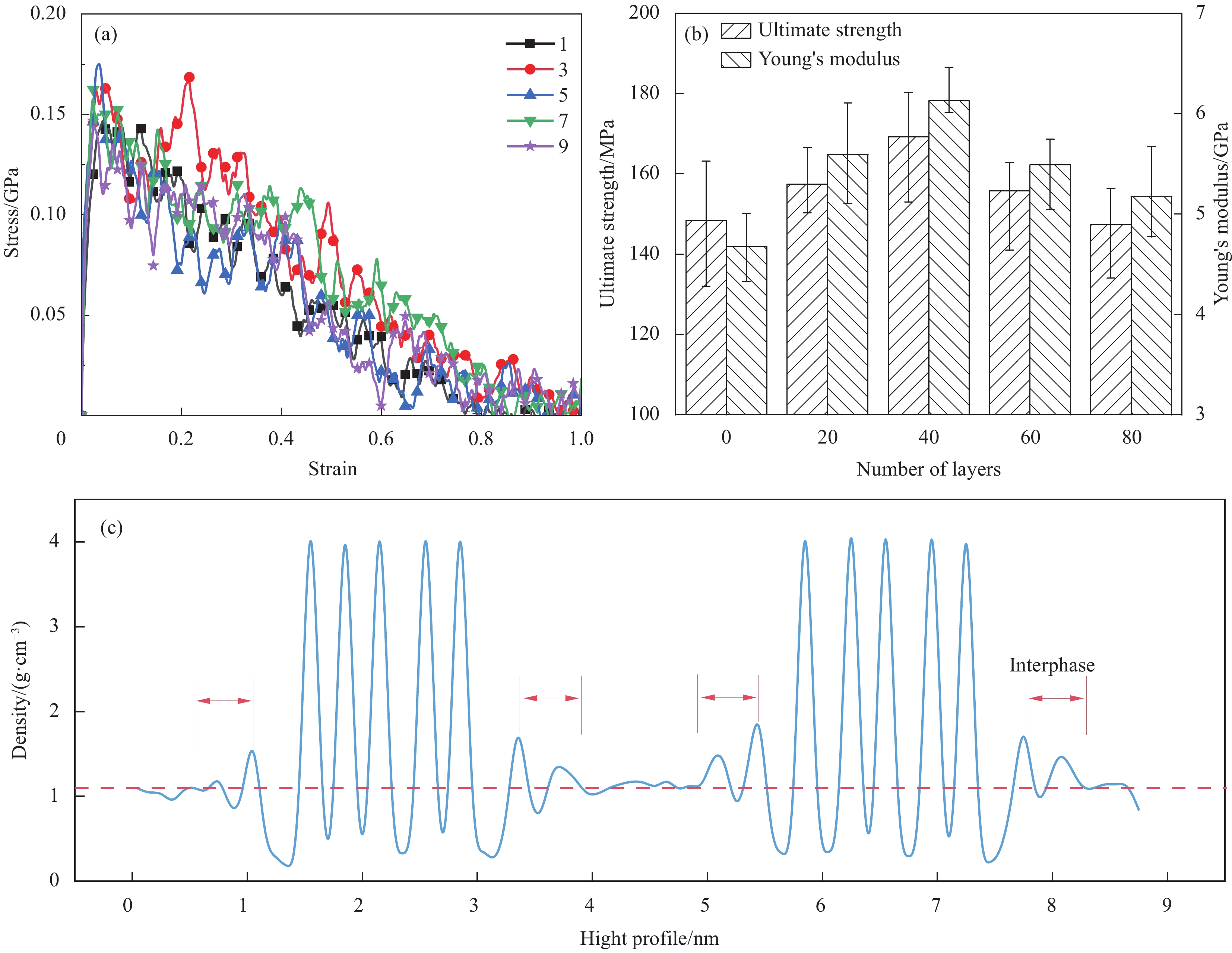
表 1 粗粒化石墨烯势函数及参数^[41]

Table 1 Functional form and parameters of the force filed for the coarse-grained graphene^[41]

Potential	Function	Parameter
Bond	${V_{{\rm{bond}}}} = {k_{\rm{b}}}{(1 - {{\rm{e}}^{ - \partial (d - {d_0})}})^2}$	${k_{\rm{b}}} = 194.61\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}};\;\;{d_0} = 0.28\;{\rm{nm}};\;\;\partial = 0.155\;{\rm{n}}{{\rm{m}}^{ - 1}}$
Angle	${V_{ {\rm{angle} }} } = {k_{\rm{\theta } } }{(\theta - {\theta _0})^2}$	${k_{\rm{\theta }}} = 409.40\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}};\;\;{\theta _0} = 120^\circ$
Dihedral	${V_{{\rm{dihedral}}}} = {k_\Phi }(1 - \cos (2\phi ))$	${k_\Phi } = 4.15\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}$
Non-bonded	${V_{ {\rm{nb} } } } = 4\varepsilon \left[ { { {\left( {\dfrac{\sigma }{r} } \right)}^6} - { {\left( {\dfrac{\sigma }{r} } \right)}^{12} } } \right],\;\;r < {r_{ {\rm{cut} } } }$	$\varepsilon = 0.82\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}};\;\;{r_{{\rm{cut}}}} = 1.2\;{\rm{nm;}}\;\;\sigma = {\rm{0}}{\rm{.346\;nm}}$
Notes: V_bond, V_angle, V_dihedral and V_nb—Sum over the energies of all the bonds, angles, dihedrals, and pair-wise non-bonded interactions of the system respectively; d, θ and ϕ—Bond stretching, bond angle bending and dihedral angle torsion; k_b and ∂—Depth and a parameter related to the width of the potential well of the bond respectively; d₀—Equilibrium distance of the bond; k_θ—Spring constant of the angle interaction; θ₀—Equilibrium angle; k_Φ—Spring constant of the dihedral interaction; r—Distance between two atoms; ε—Depth of the Lennard-Jones potential well for non-bonded interactions; σ—Lennard-Jones parameter associated with the equilibrium distance between two non-bonded beads; r_cut—Cutoff distance of the non-bonded interactions.

下载: 导出CSV

表 2 粗粒化聚甲基丙烯酸甲酯(PMMA)势函数及参数^[42]

Table 2 Functional form and parameters of the force filed for the coarse-grained polymethyl methacrylate (PMMA)^[42]

Potential	Function	Parameters
Bond	${V_{{\rm{bond}}}} = \dfrac{{{k_{\rm{d}}}}}{2}{(d - d{}_0)^2}$	${k_{\rm{d}}} = 19461\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}} \cdot {\rm{n}}{{\rm{m}}^{ - 2}};\;\;{d_0} = 0.402\;{\rm{nm}}$
Angle	${V_{{\rm{angle}}}} = \dfrac{{{k_{\rm{\theta }}}}}{2}{(\theta - {\theta _0})^2}$	${k_{\rm{\theta }}} = 794.89\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}} \cdot {\rm{ra}}{{\rm{d}}^{{\rm{ - 2}}}};\;\;{\theta _0} = 89.6^\circ$
Dihedral	${V_{{\rm{dihedral}}}} = \dfrac{{{k_\Phi }}}{2}(1 - \cos (2\phi ))$	${k_\Phi } = 42.05\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}$
Non-bonded	${V_{{\rm{nb}}}} = {D_0}\left[ {{{\left( {\dfrac{{{r_0}}}{r}} \right)}^{12}} - 2{{\left( {\dfrac{{{r_0}}}{r}} \right)}^6}} \right]$	${D_0} = 1.34\;{\rm{kcal}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}{\rm{;}}\;\;{r_0} = 0.653\;{\rm{nm}}$
Notes: k_d—Spring constant of the bond length; D₀ and r₀—Associated with the equilibrium well depth and the equilibrium distance of the non-bonded interactions.

下载: 导出CSV

参考文献(50)

[1]	RITCHIE R O. The conflicts between strength and toughness[J]. Nature Materials,2011,10(11):817-822. DOI: 10.1038/nmat3115
[2]	ESPINOSA H D, JUSTER A L, LATOURTE F J, et al. Tablet-level origin of toughening in abalone shells and translation to synthetic composite materials[J]. Nature Communications,2011,2(1):1-9.
[3]	RHO J Y, KUHN-SPEARING L, ZIOUPOS P. Mechanical properties and the hierarchical structure of bone[J]. Medical Engineering & Physics,1998,20(2):92-102.
[4]	SONG J, CHEN C, ZHU S, et al. Processing bulk natural wood into a high-performance structural material[J]. Nature,2018,554(7691):224-228. DOI: 10.1038/nature25476
[5]	BENZAIT Z, TRABZON L. A review of recent research on materials used in polymer-matrix composites for body armor application[J]. Journal of Composite Materials,2018,52(23):3241-3263. DOI: 10.1177/0021998318764002
[6]	吕志杰, 邓莉莉, 田清波, 等. 仿生结构复合陶瓷刀具材料力学性能及显微结构[J]. 稀有金属材料与工程, 2018, 47(12):3848-3852. LV Zhijie, DENG Lili, TIAN Qingbo, et al. Microstructure and mechanical properties of biomimetic composite ceramic tool materials[J]. Rare Metal Materials and Engineering,2018,47(12):3848-3852(in Chinese).
[7]	孙娜, 吴俊涛, 江雷. 贝壳珍珠层及其仿生材料的研究进展[J]. 高等学校化学学报, 2011, 32(10):2231-2239. SUN Na, WU Juntao, JIANG Lei. Research progress of nacre and biomimetic synthesis of nacre-like materials[J]. Chemical Journal of Chinese Universities-Chinese,2011,32(10):2231-2239(in Chinese).
[8]	SUN X, HUANG C, WANG L, et al. Recent progress in graphene/polymer nanocomposites[J]. Advanced Mater-ials,2020,33:2001105.
[9]	WAN S, PENG J, JIANG L, et al. Bioinspired graphene-based nanocomposites and their application in flexible energy devices[J]. Advanced Materials,2016,28(36):7862-7898. DOI: 10.1002/adma.201601934
[10]	WANG Y, XIA S, LI H, et al. Unprecedentedly tough, folding-endurance, and multifunctional graphene-based artificial nacre with predesigned 3D nanofiber network as matrix[J]. Advanced Functional Materials,2019,29(38):1903876. DOI: 10.1002/adfm.201903876
[11]	MAO L B, GAO H L, YAO H B, et al. Synthetic nacre by predesigned matrix-directed mineralization[J]. Science,2016,354(6308):107-110. DOI: 10.1126/science.aaf8991
[12]	MEYERS M A, LIN A Y M, CHEN P Y, et al. Mechanical strength of abalone nacre: Role of the soft organic layer[J]. Journal of the Mechanical Behavior of Biomedical Mater-ials,2008,1(1):76-85. DOI: 10.1016/j.jmbbm.2007.03.001
[13]	SHEN X, ZHENG Q, KIM J K. Rational design of two-dimensional nanofillers for polymer nanocomposites toward multifunctional applications[J]. Progress in Materials Science,2021,115:100708. DOI: 10.1016/j.pmatsci.2020.100708
[14]	YAO H B, GE J, MAO L B, et al. 25th anniversary article: Artificial carbonate nanocrystals and layered structural nanocomposites inspired by nacre: Synthesis, fabrication and applications[J]. Advanced Materials,2014,26(1):163-188. DOI: 10.1002/adma.201303470
[15]	张学骜, 王建方, 吴文健, 等. 贝壳珍珠层生物矿化及其对仿生材料的启示[J]. 无机材料学报, 2006, 21(2):257-266. DOI: 10.3321/j.issn:1000-324X.2006.02.001 ZHANG Xueao, WANG Jianfang, WU Wenjian, et al. Advances in biomineralization of nacreous layer and its inspiration for biomimetic materials[J]. Journal of Inorganic Materials,2006,21(2):257-266(in Chinese). DOI: 10.3321/j.issn:1000-324X.2006.02.001
[16]	WANG R Z, SUO Z, EVANS A G, et al. Deformation mechanisms in nacre[J]. Journal of Materials Research,2001,16(9):2485-2493. DOI: 10.1557/JMR.2001.0340
[17]	柏嵩, 沈小平. 石墨烯基无机纳米复合材料[J]. 化学进展, 2010, 22(11):2106-2118. BAI Song, SHEN Xiaoping. Graphene-based inorganic nanocomposites[J]. Progress in Chemistry,2010,22(11):2106-2118(in Chinese).
[18]	STANKOVICH S, DIKIN D A, DOMMETT G H B, et al. Graphene-based composite materials[J]. Nature,2006,442(7100):282-286. DOI: 10.1038/nature04969
[19]	KUMAR A, SHARMA K, DIXIT A R. A review on the mechanical properties of polymer composites reinforced by carbon nanotubes and graphene[J]. Carbon Letters,2020,31(9):149-165.
[20]	张力, 吴俊涛, 江雷. 石墨烯及其聚合物纳米复合材料[J]. 化学进展, 2014, 26(4):560-571. ZHANG Li, WU Juntao, JIANG Lei. Graphene and its polymer nanocomposites[J]. Progress in Chemistry,2014,26(4):560-571(in Chinese).
[21]	SALEEM A, ZHANG Y, GONG H, et al. Enhanced thermal conductivity and mechanical properties of a GNP reinforced Si3N4 composite[J]. Rsc Advances,2019,9(68):39986-39992. DOI: 10.1039/C9RA09286B
[22]	SINGH J, WANI M F. Fretting wear of spark plasma sintered Ti₃SiC₂/GNP ceramic composite against Si₃N₄[J]. Ceramics International,2021,47(4):5648-5655. DOI: 10.1016/j.ceramint.2020.10.150
[23]	MONTAZERI A, MOBARGHEI A. Nanotribological behavior analysis of graphene/metal nanocomposites via MD simulations: New concepts and underlying mechanisms[J]. Journal of Physics and Chemistry of Solids,2018,115:49-58. DOI: 10.1016/j.jpcs.2017.12.012
[24]	TANG W, ZHANG J, WU J, et al. Mechanical properties and enhancement mechanisms of titanium-graphene nanocomposites[J]. Acta Mechanica Sinica,2020,36(4):855-865. DOI: 10.1007/s10409-020-00968-x
[25]	周登山. 功能化石墨烯/聚合物体系的分子间相互作用力的分子动力学模拟研究[D]. 青岛: 中国石油大学(华东), 2010. ZHOU Dengshan. Molecular dynamics simulation investigation of intermolecular interactions for functionalized graphene sheets/polymers system[D]. Qingdao: China University of Petroleum (East China), 2010(in Chinese).
[26]	FANG M, WANG K, LU H, et al. Covalent polymer functionalization of graphene nanosheets and mechanical properties of composites[J]. Journal of Materials Chemistry,2009,19(38):7098-7105. DOI: 10.1039/b908220d
[27]	WAN Y J, TANG L C, GONG L X, et al. Grafting of epoxy chains onto graphene oxide for epoxy composites with improved mechanical and thermal properties[J]. Carbon,2014,69:467-480. DOI: 10.1016/j.carbon.2013.12.050
[28]	ZAMAN I, KUAN H C, MENG Q, et al. A facile approach to chemically modified graphene and its polymer nanocomposites[J]. Advanced Functional Materials,2012,22(13):2735-2743. DOI: 10.1002/adfm.201103041
[29]	CHENG Q, WU M, LI M, et al. Ultratough artificial nacre based on conjugated cross-linked graphene oxide[J]. Angewandte Chemie-International Edition,2013,52(13):3750-3755. DOI: 10.1002/anie.201210166
[30]	CUI W, LI M, LIU J, et al. A strong integrated strength and toughness artificial nacre based on dopamine cross-linked graphene oxide[J]. ACS Nano,2014,8(9):9511-9517. DOI: 10.1021/nn503755c
[31]	WAN S, PENG J, LI Y, et al. Use of synergistic interactions to fabricate strong, tough, and conductive artificial nacre based on graphene oxide and chitosan[J]. ACS Nano,2015,9(10):9830-9836. DOI: 10.1021/acsnano.5b02902
[32]	LIU N, ZENG X, PIDAPARTI R, et al. Tough and strong bioinspired nanocomposites with interfacial cross-links[J]. Nanoscale,2016,8(43):18531-18540. DOI: 10.1039/C6NR06379A
[33]	XIA W, SONG J, MENG Z, et al. Designing multi-layer graphene-based assemblies for enhanced toughness in nacre-inspired nanocomposites[J]. Molecular Systems Design & Engineering,2016,1(1):40-47.
[34]	LIU N, PIDAPARTI R, WANG X. Mechanical performance of graphene-based artificial nacres under impact loads: A coarse-grained molecular dynamic study[J]. Polymers,2017,9(4):134-145.
[35]	DING P, WU J, ZHANG J, et al. Role of geometric shapes on the load transfer in graphene-PMMA nanocomposites[J]. Computational Materials Science,2020,184:109863. DOI: 10.1016/j.commatsci.2020.109863
[36]	GUO Z, SONG L, CHAI G B, et al. Multiscale finite element analyses on mechanical properties of graphene-reinforced composites[J]. Mechanics of Advanced Materials and Structures,2019,26(20):1735-1742. DOI: 10.1080/15376494.2018.1447176
[37]	HONARVAR F M, POURABBAS B, HOSSEINI M S, et al. Molecular dynamics simulation: The effect of graphene on the mechanical properties of epoxy based photoresist: SU8[J]. Scientia Iranica,2018,25(3):1879-1890.
[38]	SANTHAPURAM R R, MULLER S E, NAIR A K. Nanoscale bending properties of bio-inspired Ni-graphene nanocomposites[J]. Composite Structures,2019,220:798-808. DOI: 10.1016/j.compstruct.2019.03.093
[39]	WANG F, LIU K, LI D, et al. Fracture toughness of biological composites with multilevel structural hierarchy[J]. Journal of Applied Mechanics-Transactions of the Asme,2020,87(7):071004. DOI: 10.1115/1.4046845
[40]	PELITI L. Self-avoiding walks[J]. Physics Reports-Review Section of Physics Letters,1984,103(1-4):225-231.
[41]	RUIZ L, XIA W, MENG Z, et al. A coarse-grained model for the mechanical behavior of multi-layer graphene[J]. Carbon,2015,82:103-115. DOI: 10.1016/j.carbon.2014.10.040
[42]	ARASH B, PARK H S, RABCZUK T. Mechanical properties of carbon nanotube reinforced polymer nanocomposites: A coarse-grained model[J]. Composites Part B: Engineering,2015,80:92-100. DOI: 10.1016/j.compositesb.2015.05.038
[43]	LORENTZ H A. Ueber die anwendung des satzes vom virial in der kinetischen theorie der gase[J]. Annalen Der Physik,1881,248(1):127-136. DOI: 10.1002/andp.18812480110
[44]	PLIMPTON S. Fast parallel algorithms for short-range molecular-dynamics[J]. Journal of Computational Physics,1995,117(1):1-19. DOI: 10.1006/jcph.1995.1039
[45]	STUKOWSKI A. Visualization and analysis of atomistic simulation data with OVITO-the open visualization tool[J]. Modelling and Simulation in Materials Science and Engineering,2010,18(1):015012. DOI: 10.1088/0965-0393/18/1/015012
[46]	GAO H J, JI B H, JAGER I L, et al. Materials become insensitive to flaws at nanoscale: Lessons from nature[J]. Proceedings of the National Academy of Sciences of the United States of America,2003,100(10):5597-5600. DOI: 10.1073/pnas.0631609100
[47]	JI B H, GAO H J. Mechanical properties of nanostructure of biological materials[J]. Journal of the Mechanics and Physics of Solids,2004,52(9):1963-1990. DOI: 10.1016/j.jmps.2004.03.006
[48]	HU K, GUPTA M K, KULKARNI D D, et al. Ultra-robust graphene oxide-silk fibroin nanocomposite membranes[J]. Advanced Materials,2013,25(16):2301-2307. DOI: 10.1002/adma.201300179
[49]	LIN F, XIANG Y, SHEN H S. Temperature dependent mechanical properties of graphene reinforced polymer nanocomposites-A molecular dynamics simulation[J]. Compo-sites Part B: Engineering,2017,111:261-269. DOI: 10.1016/j.compositesb.2016.12.004
[50]	LIU F, HU N, NING H, et al. Molecular dynamics simulation on interfacial mechanical properties of polymer nanocomposites with wrinkled graphene[J]. Computational Materials Science,2015,108:160-167. DOI: 10.1016/j.commatsci.2015.06.023

施引文献(3)

期刊类型引用(2)

1.	李友明，景昭，吴增文，李冰垚，刘琛，葛敬冉，梁军. 随机疲劳下复合材料剩余刚度-剩余强度关联模型及寿命预测. 强度与环境. 2024(01): 23-30 . 百度学术
2.	马帅，金珊珊. 碳纤维增强复合材料对钢筋混凝土的加固作用. 材料导报. 2022(S1): 252-256 . 百度学术