Analysis of piezoelectric composite laminates based on generalized mixed finite element
-
摘要: 将纯弹性体的广义混合有限元法引入到压电材料的静力学分析中。由于采用了8节点六面体非协调实体单元对整体结构进行离散求解，摒弃了板壳理论中的诸多人为假设。非协调项的加入使该方法比同类协调元显示出更好的数值性能。本文方法将应力边界条件和位移边界条件同时考虑，并且求解过程中将层间应力和平面内应力分开处理，按每层的本构关系求解平面内应力，这样求得的层间应力和平面内应力都更加接近精确解。通过几个有代表性的层合板的数值算例说明了本文方法的精度，相较于传统的解析法和数值法，本文方法在适用性和有效性方面都具有优势。Abstract: Generalized mixed finite element method of pure elastomer was introduced into the static analysis of piezoelectric materials. Because the 8-node hexahedral noncompatible solid element was used to solve the whole structure discretely, many artificial assumptions in the theory of plate and shell were abandoned. The addition of noncompatible terms makes this method exhibit better numerical performance than the same kind of compatible elements. In this method, the stress boundary conditions and displacement boundary conditions were considered simultaneously, and the interlaminar stress and in-plane stress were treated separately in the solution process, and the in-plane stress was calculated according to the constitutive relation of each layer, so that the obtained interlaminar stress and in-plane stress are closer to the exact solution. The accuracy of the proposed method was illustrated by several representative numerical examples of laminates. Compared with the traditional analytical and numerical methods, the theoretical method presented in this paper has advantages in applicability and effectiveness.
-
图 1 锆钛酸铅压电陶瓷(PZT-4)压电层合板几何模型
Figure 1. Geometric model of lead zirconate titanate ceramic (PZT-4) piezoelectric laminates
图 3 PZT-4压电层合板纵向位移u沿z轴的分布
Figure 3. Distribution of longitudinal displacement u of PZT-4 piezoelectric laminates along the z axis
图 7 工况1下PZT-4压电层合板平面内应力
$ {\sigma }_{xy} $ 沿z轴的分布Figure 7. Distribution of in-plane stress
$ {\sigma }_{xy} $ of PZT-4 piezoelectric laminates along the z axis under condition 1
图 4 PZT-4压电层合板横向位移w沿z轴的分布
Figure 4. Distribution of the transverse displacement w of PZT-4 piezoelectric laminates along the z axis
图 5 PZT-4压电层合板层间应力
$ {\sigma }_{z} $ 沿z轴的分布Figure 5. Distribution of interlaminar stress
$ {\sigma }_{z} $ in PZT-4 piezoelectric laminates along the z axis
图 6 PZT-4压电层合板平面内应力
$ {\sigma }_{x} $ 沿z轴的分布Figure 6. Distribution of in-plane stress
$ {\sigma }_{x} $ of PZT-4 piezoelectric laminates along the z axis
图 8 PZT-4压电层合板层间应力
$ {\sigma }_{xz} $ 沿z轴的分布Figure 8. Distribution of interlaminar stress
$ {\sigma }_{xz} $ of PZT-4 piezoelectric laminates along the z axis
图 10 工况2下PZT-4压电层合板平面内应力
$ {\sigma }_{xy} $ 沿z轴的分布Figure 10. Distribution of in-plane stress
$ {\sigma }_{xy} $ of PZT-4 piezoelectric laminates along the z axis under condition 2
图 9 PZT-4压电层合板电势φ沿z轴的分布
Figure 9. Distribution of the potential φ of PZT-4 piezoelectric laminates along the z axis
图 11 聚偏氟乙烯(PVDF)压电层合板几何模型
Figure 11. Geometric model of polyvinylidene fluoride (PVDF) piezoelectric laminates
L—Length; H—Height
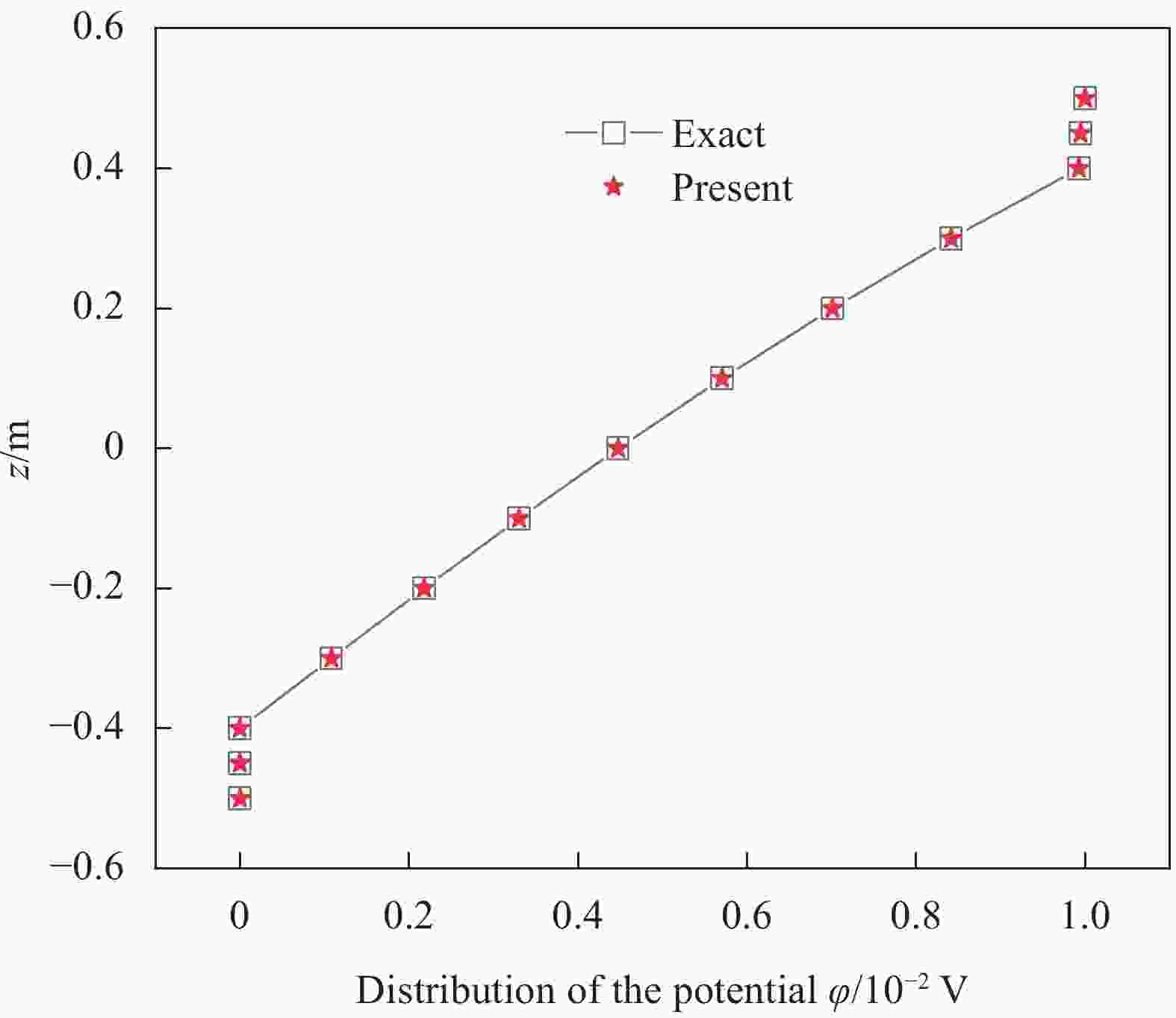
图 13
$S\text{=10}{\text{、}}V_{\text{0}}\text{=100}$ PVDF压电层合板平面内应力${\tilde \sigma _x}$ 、${\tilde \sigma _y}$ 沿z轴的分布Figure 13. Distribution of in-plane stress
${\tilde \sigma _x}$ ,${\tilde \sigma _y}$ of PVDF piezoelectric laminates along the z axis when$ S\text{=10}{\text{,}}\;V_{\text{0}}\text{=100} $ 
图 14
$S\text{=10}{\text{、}}V_{\text{0}}\text{=0}$ PVDF压电层合板平面内应力${\tilde \sigma _x}$ 、${\tilde \sigma _y}$ 沿z轴的分布Figure 14. Distribution of in-plane stress
${\tilde \sigma _x}$ ,${\tilde \sigma _y}$ of PVDF piezoelectric laminates along the z axis when$ S\text{=10}{\text{,}}\;V_{\text{0}}\text{=0} $ 表 1 工况1下PZT-4压电层合板的各个物理参数值
Table 1. Each physical parameter value of PZT-4 piezoelectric laminates under working condition 1
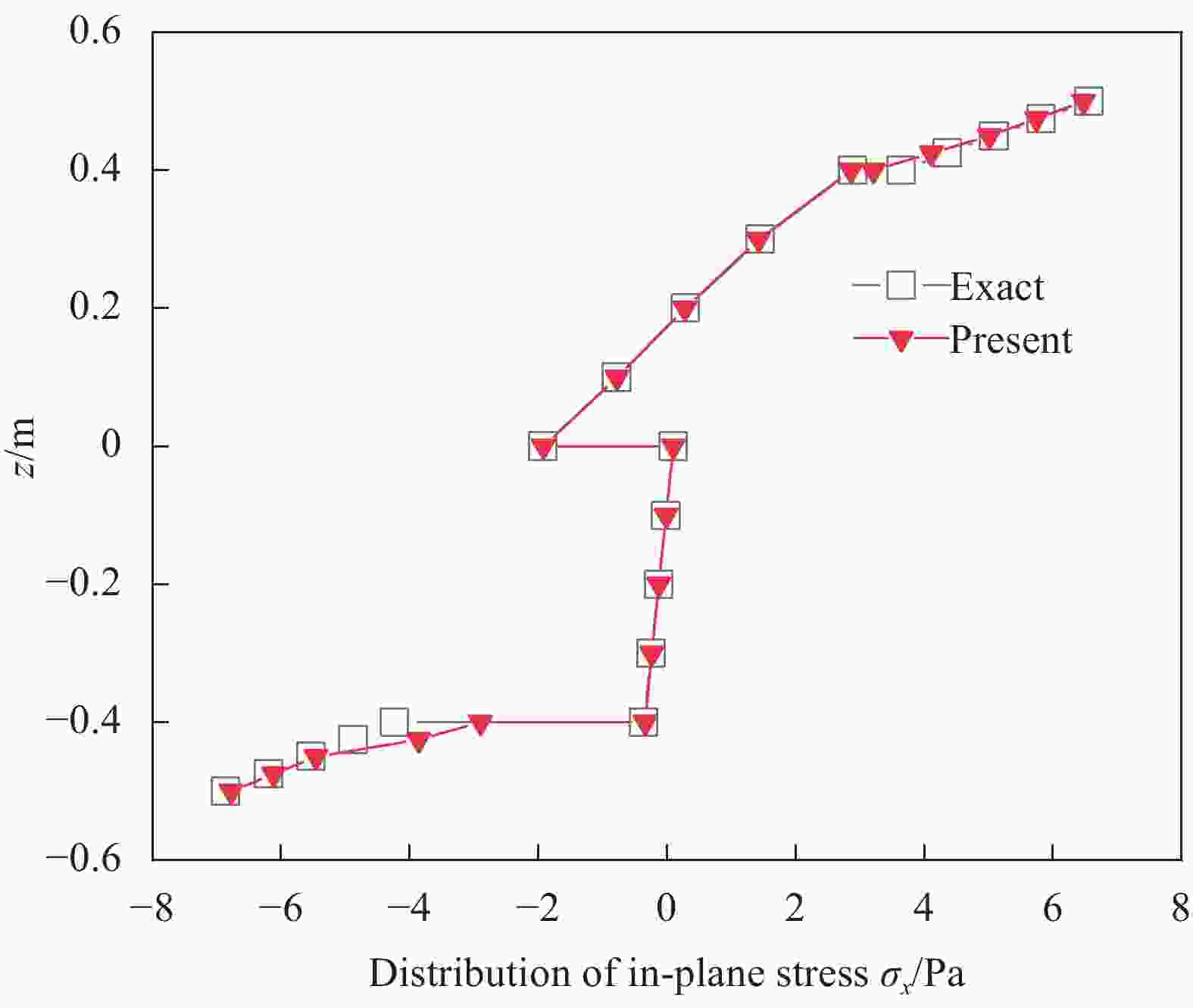
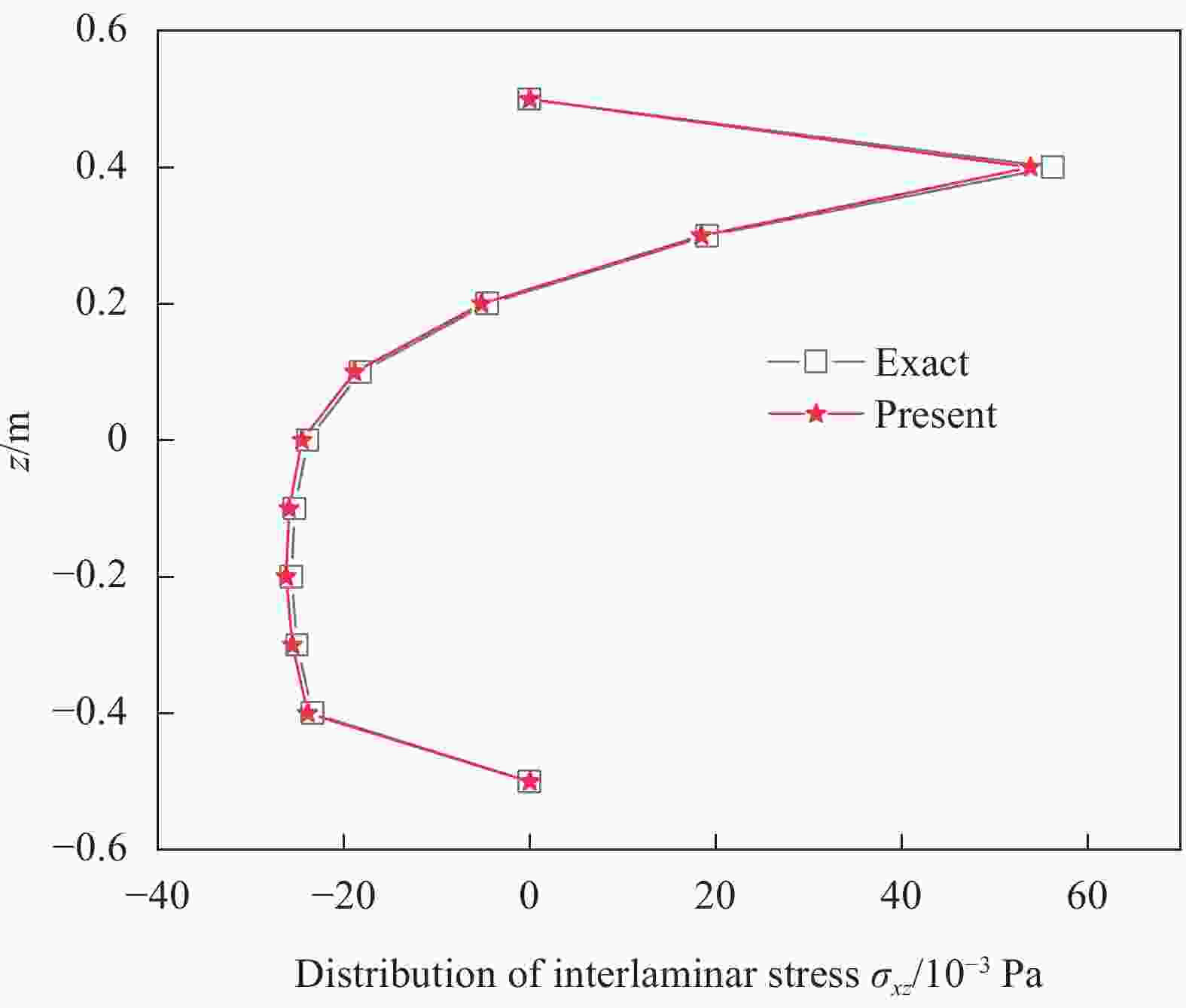
Reference solution $ {}u\left({0,}\dfrac{L}{{2}}{,0}\right) $$ {\Bigr/1}{{0}}^{{-11}}\text{m} $ $ \text{}w\left(\dfrac L{{2}}{,}\dfrac L{{2}}{,0}\right) $$ {\Bigr/}{1}{{0}}^{{-11}}\text{m} $ $ {\phi }\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right) $$ {\Bigr/}{1}{{0}}^{{-2}}\text{V} $ $ {\sigma }_{xz}\left({0,}\dfrac{L}{{2}}{,0}\right)\Bigr/{\rm{Pa}} $ $ {\sigma }_{x}\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right)\Bigr/{\rm{Pa}} $ $ {\sigma }_{z}\left(\dfrac{L}{{2}}{,}\dfrac{L}{{2}}{,0}\right) \Bigr/{\rm{Pa}}$ Exact[23] 20.392 30.027 0.611 — 6.5643 0.498 LW1[24] — 29.851 0.6032 0.7099 7.0132 0.579 Present 20.287 29.82 0.6065 0.691 6.46 0.497 Error/% 0.5 0.6 0.7 — 1.5 0.13 Notes: u—Longitudinal displacement; $w $—Transverse displacement; $\phi $—Electric potential; σz, σxz—Interlaminar stress; σx—In-plane stress. 
下载: 导出CSV
表 2 工况2下PZT-4压电层合板的各个物理参数值
Table 2. Each physical parameter value of PZT-4 piezoelectric laminates under working condition 2
Reference solution $w \left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0} }^{\rm{-11} }\rm{m}$ $\phi\left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-2}}\rm{V} $ $ {\sigma _{xz}} \left( {0,\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-3}}\rm{Pa} $ $ {\sigma _{z}} \left( {\dfrac{L}{2},\dfrac{L}{2},0} \right) \rm{\Bigr/1}{\rm{0}}^{\rm{-3}}\rm{Pa} $ Exact[23] −1.471 0.4476 −23.87 −14.612 LW3[24] −1.4707 0.4477 −22.70 −13.541 Present −1.493 0.4475 −24.5 −15.469 Error/% 1.47 0.7 0.64 5.6
下载: 导出CSV
表 3 PVDF压电层合板的挠度和应力
Table 3. Deflection and stress of PVDF piezoelectric laminates
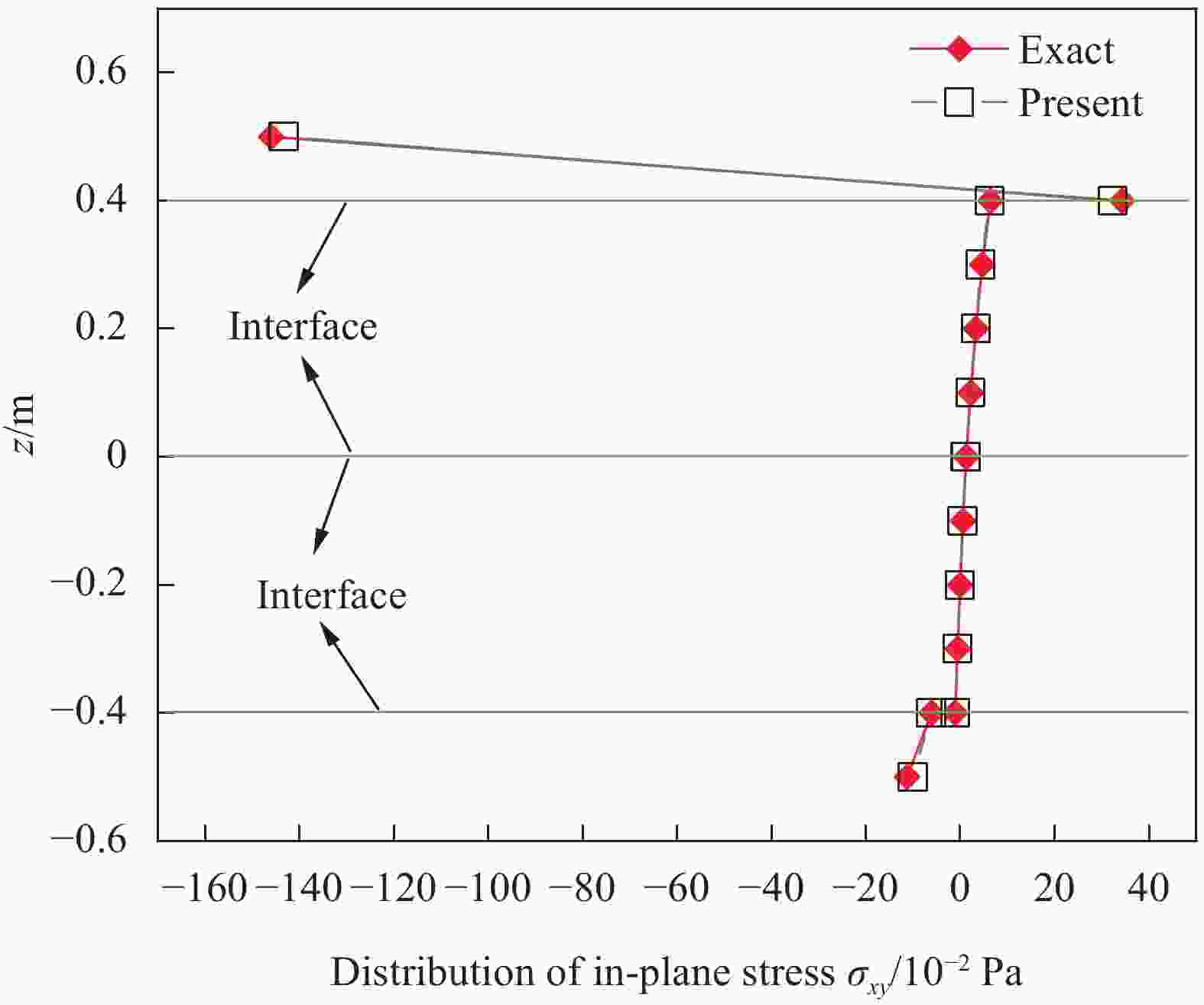
Load L/H Reference
solution$\tilde w\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,0}\right) $ ${\tilde \sigma _x}\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,}\dfrac{h}{\text{2}}\right) $ ${\tilde \sigma _y}\left(\dfrac{L}{\text{2}}\text{,}\dfrac{L}{\text{2}}\text{,}\dfrac{h}{\text{6}}\right) $ ${\tilde \tau _{xy}}\left(\text{0,0,}\dfrac{h}{\text{2}}\right) $ ${\tilde \tau _{xz}} \left(\text{0,}\dfrac{L}{\text{2}}\text{,0}\right) $ ${\tilde \tau _{yz}}\left(\dfrac{L}{\text{2}}\text{,0,0}\right) $ ${p_0} = 1.0$${V_0} = 1.0$ 10 Present 0.766 0.589 0.285 −0.0277 0.359 0.113 Literature[27] 0.764 0.502 0.290 −0.0269 0.371 0.137 Literature[5] 0.668 0.520 − − − − Literature[25] 0.774 0.589 0.284 −0.0287 0.358 0.123 100 Present 0.445 0.540 0.180 −0.020 0.395 0.076 Literature[27] 0.434 0.539 0.181 −0.0212 0.390 0.076 Literature[5] 0.433 0.545 − − − − Literature[25] 0.471 0.538 0.181 −0.021 0.394 0.083 ${p_0} = 1.0$${V_0} = 100$ 10 Present −2.0 −2.94 −2.16 0.210 −0.593 0.348 Literature[27] −2.0 −2.73 −2.45 0.149 −0.710 0.450 Literature[5] −1.91 −2.78 − − − − Literature[25] −2.35 −3.12 −2.34 0.181 −0.683 0.336 100 Present 0.424 0.489 0.084 −0.0192 0.384 0.081 Literature[27] 0.412 0.505 0.159 −0.0198 0.378 0.080 Literature[5] 0.411 0.510 − − − − Literature[25] 0.447 0.504 −0.158 −0.019 0.382 0.086 Notes: $\tilde w $—Dimensionless transverse displacement; ${\tilde \sigma _x}$, $ {\tilde \sigma _y}$, $ {\tilde \tau _{xy}}$—Dimensionless in-plane stress; ${\tilde \tau _{xz}}$, ${\tilde \tau _{yz}}$—Dimensionless interlaminar stress; $ p_0$—Peak mechanical load; V0—Peak electric potential.
下载: 导出CSV
-
[1] QING G H, MAO J, LIU Y. Generalized mixed finite element method for 3D elasticity problems[J]. Acta Mechanica Sinica,2018,34(2):1-10. [2] BENJEDDOU A. Advances in piezoelectric finite element modeling of adaptive structural elements: A survey[J]. Computers & Structures,2000,76(1-3):347-363. [3] REDDY J N. On laminated composite plates with integrated sensors and actuators[J]. Engineering Structures,1999,21(7):568-593. doi: 10.1016/S0141-0296(97)00212-5 [4] DETWILER D T, SHEN M H H, VENKAYYA V B. Finite element analysis of laminated composite structures containing distributed piezoelectric actuators and sensors[J]. Finite Elements in Analysis & Design,1995,20(2):87-100. [5] SHEIKH A H, TOPDAR P, HALDER S. An appropriate FE model for through thickness variation of displacement and potential in thin moderately thick smart laminates[J]. Composite Structures,2001,51:401-409. doi: 10.1016/S0263-8223(00)00156-2 [6] CARRERA E, BOSCOLO M. Classical and mixed finite elements for static and dynamic analysis of piezoelectric plates[J]. International Journal for Numerical Methods in Engineering,2010,70(10):1135-1181. [7] CARRERA E, BUETTNER A, NALI P. Mixed elements for the analysis of anisotropic multilayered piezoelectric plates[J]. Journal of Intelligent Material Systems & Structures,2010,21(7):701-717. [8] VIDAL P, GALLIMARD L, POLIT O. Modeling of piezoelectric plates with variables separation for static analysis[J]. Smart Materials and Structures,2016,25(5):055043. [9] PLAGIANAKOS T S, PAPADOPOULOS E G. Higher-order 2-D/3-D layerwise mechanics and finite elements for composite and sandwich composite plates with piezoelectric layers[J]. Aerospaceence & Technology,2015,40:150-163. [10] KORAYEM M H, HOMAYOONI A. The size-dependent analysis of multilayer micro-cantilever plate with piezoelectric layer incorporated voltage effect based on a modified couple stress theory[J]. European Journal of Mechanics-A/Solids,2017,61:59-72. [11] KPEKY, FESSAL, ABED-MERAIM, et al. Linear and quadratic solid-shell finite elements SHB8PSE and SHB20E for the modeling of piezoelectric sandwich structures[J]. Mechanics of Advanced Materials and Structures,2018,25(1-8):559-578. [12] MOLEIRO F, SOARES C, SOARES C, et al. Layerwise mixed models for analysis of multilayered piezoelectric composite plates using least-squares formulation[J]. Composite Structures,2015,119:134-149. [13] ZENKOUR A M, ALGHANMI R A. Stress analysis of a functionally graded plate integrated with piezoelectric faces via a four-unknown shear deformation theory[J]. Results in Physics,2018,12:268-277. [14] RAMEGOWDA P C, D ISHIHARA, NIHO T, et al. A novel coupling algorithm for the electric field-structure interaction using a transformation method between solid and shell elements in a thin piezoelectric bimorph plate analysis[J]. Finite Elements in Analysis and Design,2019,159:33-49. [15] LI W, SHEN H J. A layerwise finite element formulation of laminated composite cylindrical shells with piezoelectric layers[J]. Journal of Mechanical Science & Technology,2018,32(2):731-741. [16] KAPURIA S, AHMED A. A coupled efficient layerwise finite element model for free vibration analysis of smart piezo-bonded laminated shells featuring delaminations and transducer debonding[J]. International Journal of Mechanical Sciences,2021,194:106195. doi: 10.1016/j.ijmecsci.2020.106195 [17] 刘艳红, 李锐. 含参数辛元与热弹性复合材料层合板分析[J]. 复合材料学报, 2019, 36(5):1306-1312.LIU Yanhong, LI Rui. Parametered symplectic element and analysis of thermoelastic composite laminates[J]. Acta Materiae Composite Sinica,2019,36(5):1306-1312(in Chinese). [18] 赵直钦. 三类弹性体非协调辛有限元的理论研究[D]. 天津: 中国民航大学, 2020.ZHAO Zhiqin. Theoretical study on noncompatiable symplectic finite element of three elastomers[D]. Tianjin: Civil Aviation University of China, 2020(in Chinese). [19] PIAN T, WU C C. Hybrid and incompatible finite element methods[M]. New York: Chapman & Hall/CRC, 2006. [20] FELIPPA C A. Parametrized multifield variational principles in elasticity: I. Mixed functionals[J]. Communications in Applied Numerical Methods,2010,5(2):79-88. [21] FELIPPA C A. Parametrized multifield variational principles in elasticity: II. Hybrid functionals and the free formulation[J]. Communications in Numerical Methods in Engineering,2010,5(2):89-98. [22] 沈观林, 胡更开, 刘彬. 复合材料力学[M]. 2版. 北京: 清华大学出版社, 2013.SHEN Guanlin, HU Gengkai, LIU Bin. Mechanics of composite materials[M]. 2th ed. Beijing: Tsinghua University Press, 2013(in Chinese). [23] HEYLIGER P. Static behavior of laminated elastic/piezoelectric plates[J]. AIAA Journal,1994,32(12):2481-2484. doi: 10.2514/3.12321 [24] CARRERA E, VALVANO S, KULIKOV G M. Multilayered plate elements with node-dependent kinematics for electro-mechanical problems[J]. International Journal of Smart and Nano Materials,2018,9(4):279-317. doi: 10.1080/19475411.2017.1376722 [25] RAY M C, BHATTACHARYA R, SAMANTA B. Exact solutions for static analysis of intelligent structures[J]. AIAA Journal,2012,31(9):1684-1691. [26] PAGANO N J, HATFIELD H J. Elastic behavior of multilayered bidirectional composites[J]. AIAA Journal,1972,10(7):931-933. doi: 10.2514/3.50249 [27] CEN S, SOH A K, LONG Y Q, et al. A new 4-node quadrilateral FE model with variable electrical degrees of freedom for the analysis of piezoelectric laminated composite plates[J]. Composite Structures,2002,58(4):583-599. doi: 10.1016/S0263-8223(02)00167-8 -
下载:
点击查看大图
计量
- 文章访问数: 1185
- HTML全文浏览量: 477
- PDF下载量: 76
- 被引次数: 0
