CFRP防撞梁低速碰撞渐进损伤及优化

黄德明; 朱孙科; 王秋林; 孙永刚; 郑佳秋

doi:10.13801/j.cnki.fhclxb.20210702.002

CFRP防撞梁低速碰撞渐进损伤及优化

doi: 10.13801/j.cnki.fhclxb.20210702.002

1.
重庆交通大学　机电与车辆工程学院，重庆 400074
2.
成都航空职业技术学院，成都 610100
3.
桂林电子科技大学　机电工程学院，桂林 541010

基金项目: 重庆市自然科学基金(cstc2016jcyjA0467；cstc2017jcyjAX0248)；重庆市教委科学技术研究项目(KJQN201800718)

详细信息

通讯作者:
朱孙科，博士，副教授，研究方向为复合材料结构损伤　E-mail：suncobest@163.com

中图分类号: TH113.2；TB33
计量
- 文章访问数: 1324
- HTML全文浏览量: 733
- PDF下载量: 83
- 被引次数: 0
出版历程
- 收稿日期: 2021-05-24
- 修回日期: 2021-06-22
- 录用日期: 2021-06-27
- 网络出版日期: 2021-07-02
- 刊出日期: 2022-06-01

Progressive damage and optimization of CFRP anti-collision beams in low-speed collision

1.
School of Mechatronics and Vehicle Engineering, Chongqing Jiaotong University, Chongqing 400074, China
2.
Chengdu Vocational and Technical College of Aviation, Chengdu 610100, China
3.
School of Mechanical and Electrical Engineering, Guilin University of Electronic Technology, Guilin 541010, China

摘要

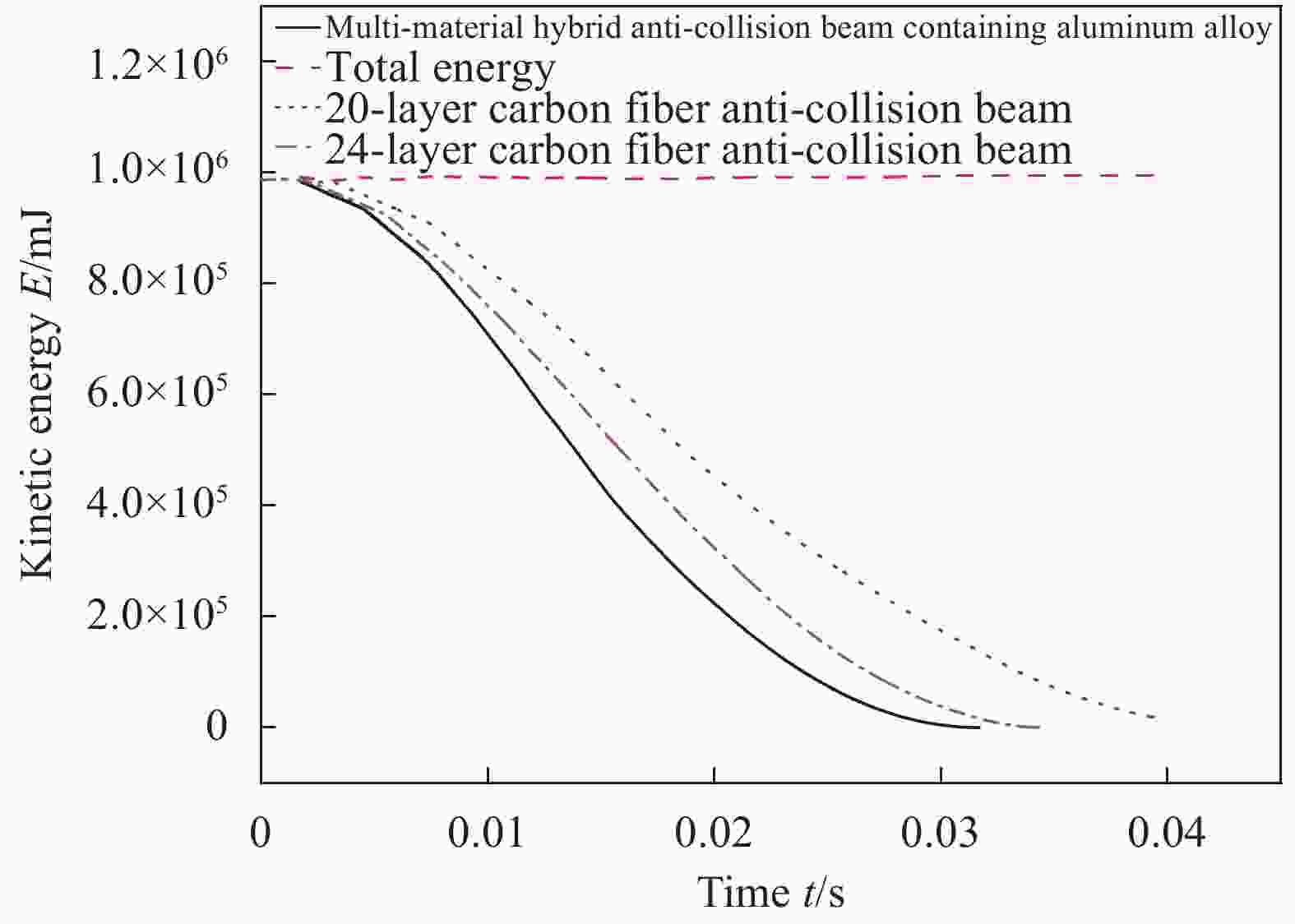
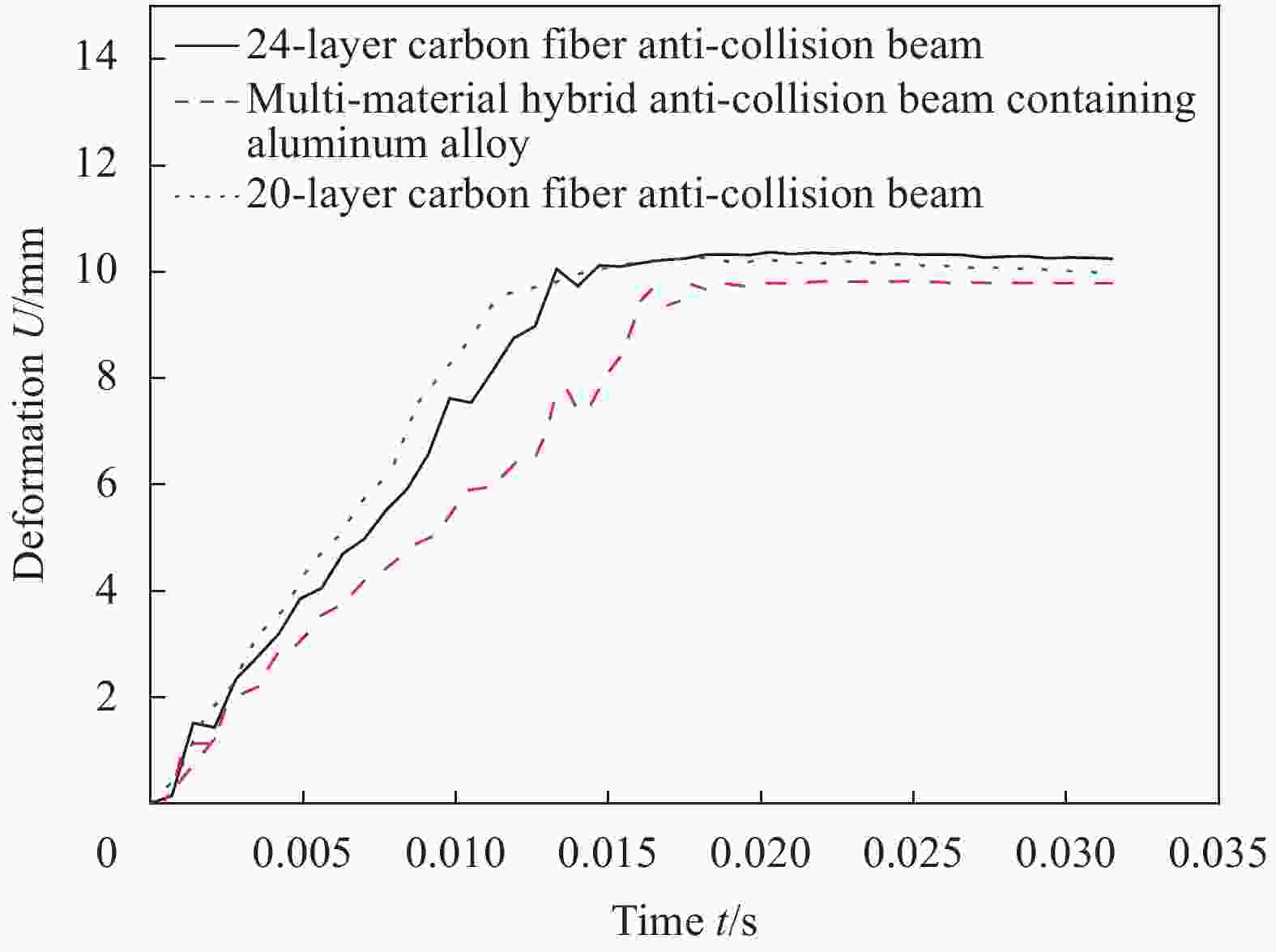
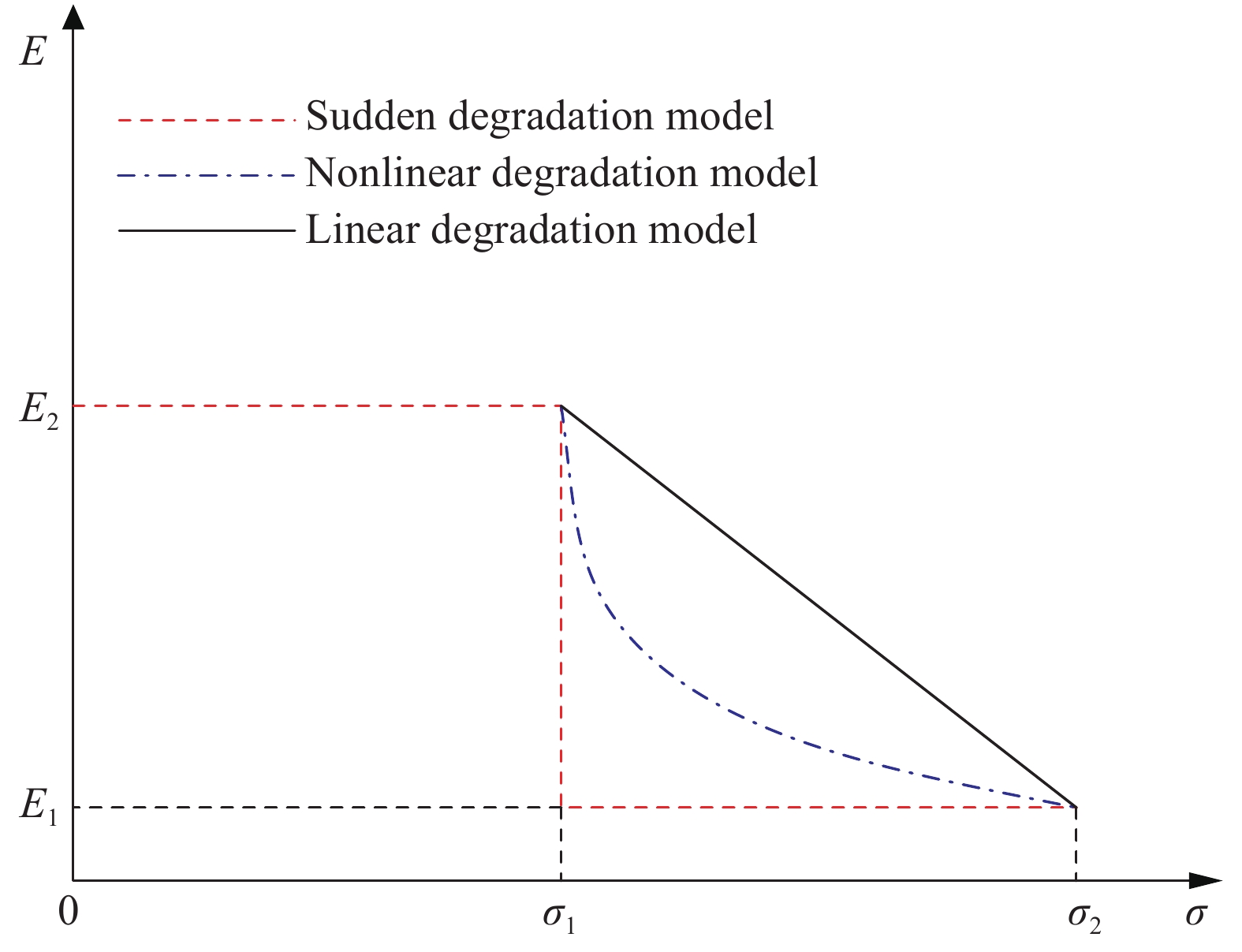
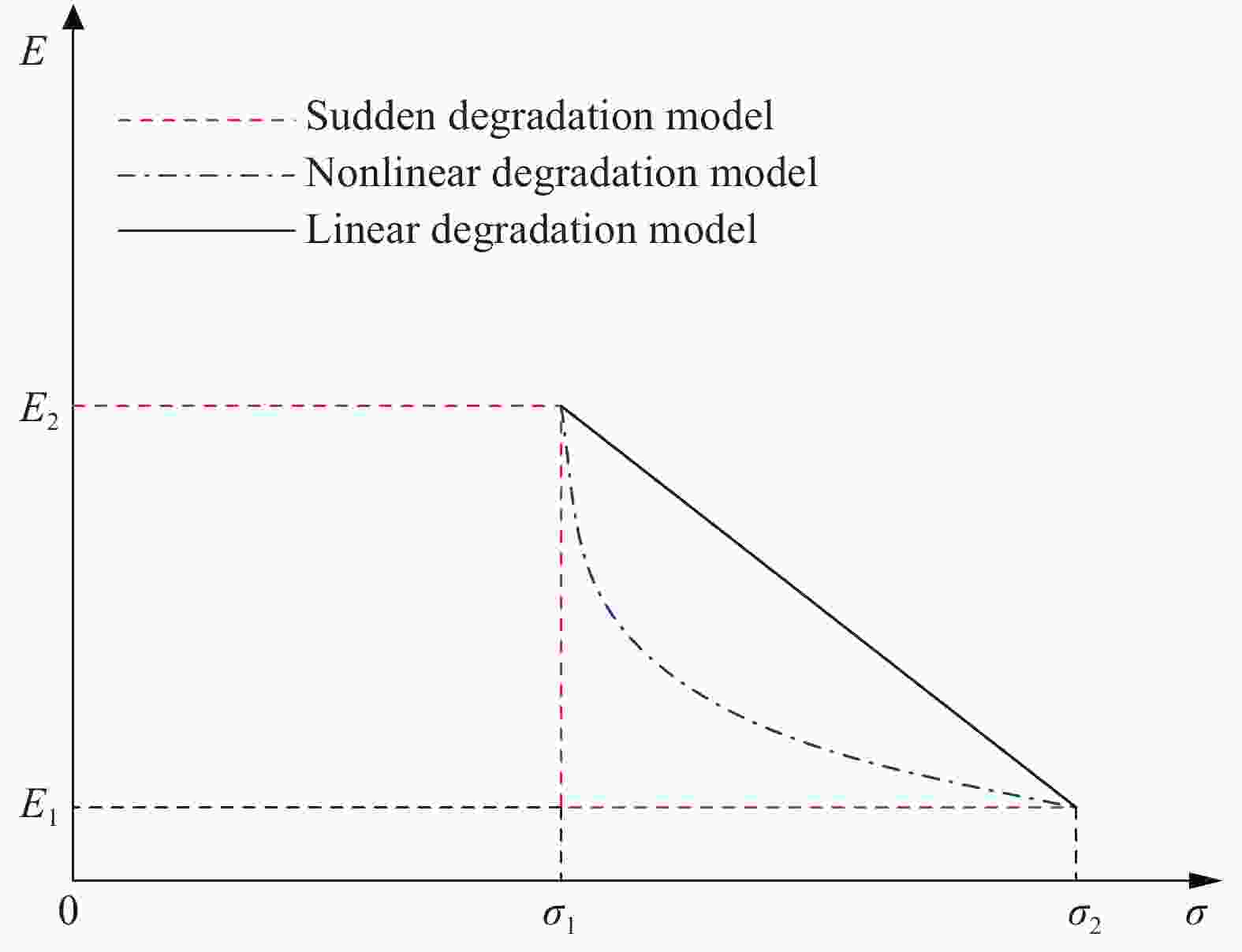
摘要: 为预测和控制低速碰撞中碳纤维增强树脂复合材料(CFRP)防撞梁损伤程度，建立了含CFRP防撞梁的有限元显式动力学碰撞模型，防撞梁层内采用实体复合材料模拟其力学特性，采用Cohesive单元模拟CFRP层间相互作用。发展了基于Tsai-Wu张量理论的VUSDFLD子程序用于判定碰撞过程中复合材料单元6个方向损伤，失效单元按照突降退化模型进行刚度折减，利用Johnson-Cook本构模型模拟铝合金强化层碰撞损伤，其失效单元采用线性连续退化模型进行刚度折减。通过[±45°/45°/0°/0°/90°/−45°/0°/0°/90°]_s和[±45°/45°/0°/0°/0°/−45°/90°/−45°/0°/0°/90°]_s两种CFRP防撞梁铺层结构碰撞结果与含铝合金强化层CFRP防撞梁碰撞结果对比可知，在层内单元数相同的情况下，CFRP防撞梁增设4层复合材料铺层后，失效单元数量降低明显；碰撞过程中含铝合金强化层的多材料混合防撞梁结构在质量基本不变的情况下，失效单元数显著降低。结果表明，所开发的VUSDFLD子程序能够用于复合材料防撞梁的显式动力学碰撞损伤模拟，基于碰撞损伤的计算结果为CFRP防撞梁的结构设计提供参考。
- CFRP /
- 显式动力学 /
- Tsai-Wu失效准则 /
- 铝合金材料 /
- 碰撞损伤
Abstract: In order to predict and control the damage degree of carbon fiber reinforced polymer (CFRP) anti-collision beams in low speed collision, a finite element explicit dynamic collision model of CFRP anti-collision beams was established. Mechanical properties of CFRP anti-collision beams were simulated by solid composite materials, and the interlayer interaction of CFRP was simulated by cohesive element. A VUSDFLD subroutine based on Tsai-Wu tensor theory was developed to determine the damage of composite elements in six directions during the collision process. The stiffness of the failure elements was reduced according to the sudden degradation model. The Johnson-Cook constitutive model was used to simulate the impact damage of reinforced aluminum alloy layers. The stiffness reduction of the failure element was carried out by linear continuous degradation model. The collision results of two CFRP laminates ([±45°/45°/0°/0°/90°/45°/0°/0°/90°]_s and [±45°/45°/0°/0°/0°/45°/90°/45°/0°/0°/90°]_s) were compared with the collision results of CFRP anti-collision beam containing aluminum alloy reinforced layer. It can be seen that when the number of elements in the layer is the same, the number of failure elements decreases obviously by adding four layers of composite laminates to CFRP anti-collision beam. The number of failure elements of the multi-material hybrid anti-collision beam structure with reinforced aluminum alloy layer is significantly reduced under the condition that the mass of the beam is basically unchanged. The results show that the developed VUSDFLD subroutine can be used for the explicit dynamic collision damage simulation of composite anti-collision beams, and the results based on the collision damage simulation can provide a reference for the structural design of CFRP anti-collision beams.
- CFRP /
- explicit dynamics /
- Tsai-Wu failure criterion /
- aluminum alloy material /
- impact damage

HTML全文

图 1 复合材料刚度折减方式

Figure 1. Composite material stiffness reduction method

E—Elasticity modulus; σ—Stress vector

下载: 全尺寸图片幻灯片

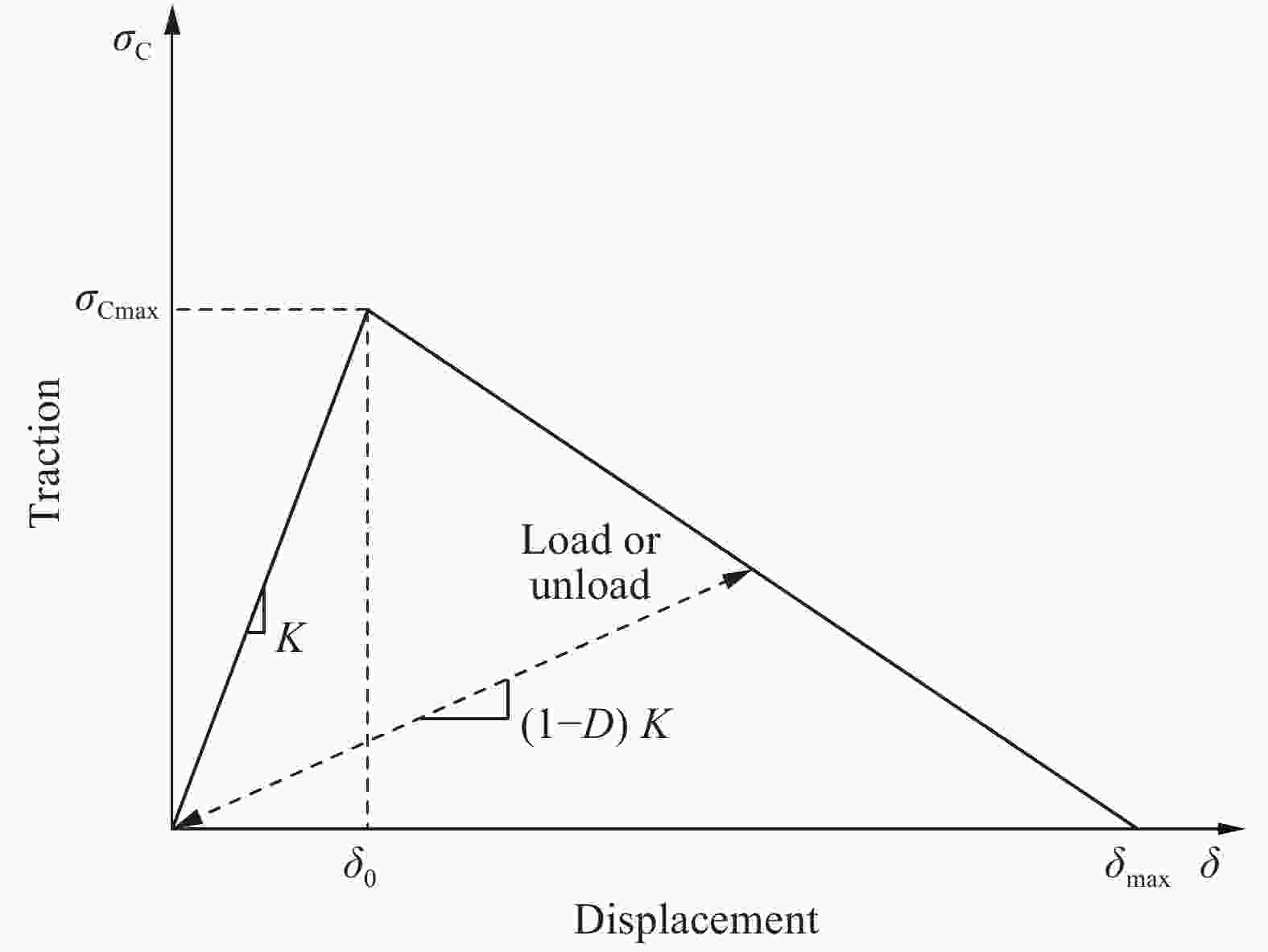
图 2 内聚力单元双线性本构关系

${\sigma }_{\mathrm{C}\mathrm{m}\mathrm{a}\mathrm{x}}$—Maximum traction; $ {\delta }_{0} $—Displacement at the beginning of the damage; $ {\delta }_{\mathrm{m}\mathrm{a}\mathrm{x}} $—Displacement at break; D—Degradation parameter of the cohesion unit

Figure 2. Bilinear constitutive relationship of cohesion unit

下载: 全尺寸图片幻灯片

图 3 Johnson-Cook模型的损伤演化规律

Figure 3. Damage evolution law of Johnson-Cook model

U—Displacement; U_max—Maximum displacement; $\bar D $—Damage parameter

下载: 全尺寸图片幻灯片

图 4 CFRP试件载荷-时间曲线

Figure 4. Load-time curve of CFRP specimen

下载: 全尺寸图片幻灯片

图 5 CFRP试件应力-时间曲线

Figure 5. Stress-time curve of CFRP specimen

下载: 全尺寸图片幻灯片

图 6 CFRP防撞梁低速碰撞有限元模型

Figure 6. Finite element model of low-speed collision of CFRP anti-collision beam

下载: 全尺寸图片幻灯片

图 7 低速碰撞碰撞器尺寸标准

Figure 7. Low-speed collision collider size standard

R—Radius

下载: 全尺寸图片幻灯片

图 8 CFRP防撞梁第1层复合材料FV3方向损伤演化过程

Figure 8. Damage evolution process in FV3 direction of the first layer of composite material of CFRP anti-collision beam

下载: 全尺寸图片幻灯片

图 9 CFRP防撞梁第2层复合材料FV3方向损伤演化过程

Figure 9. Damage evolution process in the FV3 direction of the second layer of composite material of CFRP anti-collision beam

下载: 全尺寸图片幻灯片

图 10 多材料混合防撞梁第21层复合材料FV3方向损伤演化过程

Figure 10. Damage evolution process in FV3 direction of the 21st layer composite of multi-material hybrid anti-collision beam

下载: 全尺寸图片幻灯片

图 11 CFRP防撞梁24层复合材料防撞梁出现损伤时间对比

Figure 11. Comparison of damage time of 24-layer CFRP anti-collision beam

下载: 全尺寸图片幻灯片

图 12 嵌入铝合金材料防撞梁出现损伤时间对比

Figure 12. Comparison of damage time of anti-collision beams embedded in aluminum alloy materials

下载: 全尺寸图片幻灯片

图 13 三种防撞梁结构碰撞动能曲线对比

Figure 13. Comparison of collision kinetic energy curves of three anti-collision beam structures

下载: 全尺寸图片幻灯片

图 14 三种防撞梁结构弧形顶点处变形对比

Figure 14. Comparison of deformations at the arc vertices of three anti-collision beam structures

下载: 全尺寸图片幻灯片

表 1 碳纤维/环氧复合材料防撞梁材料参数

Table 1. Material parameters of carbon fiber/epoxy composite anti-collision beam

Elastic modulus/GPa	Poisson’s ratio	Density/(kg·$ {\mathrm{m}}^{-3} $)	Strength/MPa
$ {E}_{1} $=114	$ {\gamma }_{12} $=$ {\gamma }_{13} $=0.3	$ \rho $=1780	$ {X}_{\mathrm{T}} $=2688	$ {Z}_{\mathrm{T}} $=55.5
$ {E}_{2} $=$ {E}_{3} $=8.61	$ {\gamma }_{23} $=0.45		$ {X}_{\mathrm{C}} $=1458	$ {Z}_{\mathrm{C}} $=175
$ {G}_{12} $=$ {G}_{13} $=4.16			$ {Y}_{\mathrm{T}} $=69.5	$ {S}_{12} $=$ {S}_{13} $=136
$ {G}_{23} $=3.0			$ {Y}_{\mathrm{C}} $=236	$ {S}_{23} $=95.6

下载: 导出CSV

表 2 碳纤维增强树脂复合材料(CFRP)性能退化方式

Table 2. Performance degradation methods of carbon fiber reinforced polymer (CFRP)

Breaking mode	Breaking rule	Stiffness reduction
1 direction stretch	$ {\sigma }_{1}>{X}_{\mathrm{T}} $	$ E=0.01\mathrm{\%}E $ $ G=0.01\mathrm{\%}G $ $ v =1\mathrm{\%}v $
1 direction compression	$ -{\sigma }_{1}>{X}_{\mathrm{C}} $	$ E=0.01\mathrm{\%}E $ $ G=0.01\mathrm{\%}G $ $ v =1\mathrm{\%}v $
2 direction stretch	$ {\sigma }_{2}>{Y}_{\mathrm{T}} $	$ {E}_{2}=1\%{E}_{2} $ $ {G}_{12}=20\%{G}_{12} $ $ {G}_{13}=20\%{G}_{13} $
2 direction compression	$ -{\sigma }_{2}>{Y}_{\mathrm{C}} $	$ {E}_{2}=1\%{E}_{2} $ $ {G}_{12}=20\%{G}_{12} $ $ {G}_{13}=20\%{G}_{13} $
3 direction stretch	$ {\sigma }_{3}>{Z}_{\mathrm{T}} $	$ {E}_{3}=1\%{E}_{3} $ $ {G}_{12}=20\%{G}_{12} $ $ {G}_{13}=20\%{G}_{13} $
3 direction compression	$ {-\sigma }_{3}>{Z}_{\mathrm{C}} $	$ {E}_{3}=1\%{E}_{3} $ $ {G}_{12}=20\%{G}_{12} $ $ {G}_{13}=20\%{G}_{13} $
1-2 in-plane shear	$ \left\|{\tau }_{12}\right\|>{S}_{12} $	$ {E}_{2}=1\%{E}_{2} $ $ {G}_{12}=1\%{G}_{12} $
1-3 in-plane shear	$ \left\|{\tau }_{13}\right\|>{S}_{13} $	$ {E}_{3}=1\%{E}_{3} $ $ {G}_{13}=1\%{G}_{13} $
2-3 in-plane shear	$ \left\|{\tau }_{23}\right\|>{S}_{23} $	$ {E}_{2}=1\%{E}_{2}{E}_{3}=1\%{E}_{3} $ $ G=1\mathrm{\%}G $
Notes: E includes $ {E}_{1} $, $ {E}_{2} $ and $ {E}_{3} $; G includes $ {G}_{12} $, $ {G}_{13} $ and $ {G}_{23} $; $ v $ includes $ {v }_{1} $, $ {v }_{2} $ and $ { v }_{3} $.

下载: 导出CSV

表 3 黏结层Cohesive单元材料参数

Table 3. Material parameters of the cohesive element of the bonding layer

Parameter	$ {K}_{1}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-3}) $	$ {K}_{2}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-3}) $	$ {K}_{3}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-3}) $	$ N/\mathrm{M}\mathrm{P}\mathrm{a} $	$ S/\mathrm{M}\mathrm{P}\mathrm{a} $	$ T/\mathrm{M}\mathrm{P}\mathrm{a} $	$ {G}_{1\mathrm{c}}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-1}) $	$ {G}_{2\mathrm{c}}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-1}) $	$ {G}_{3\mathrm{c}}/(\mathrm{N}·{\mathrm{m}\mathrm{m}}^{-1}) $
Value	24000	24000	24000	60	70	70	3.84	3.84	1.88
Notes: K—Stiffness coefficients in different directions; N, S, T—Intensity in different directions; $ {G}_{\mathrm{c}} $—Fracture energies in different directions.

下载: 导出CSV

表 4 6061-T6铝合金材料参数

Table 4. 6061-T6 aluminum alloy material parameters

Parameter	Value	Parameter	Value	Parameter	Value
Density$ \rho $/(t·$ {\mathrm{m}\mathrm{m}}^{-3} $)	2.7×10⁻⁹	Material parameter c	0.2215	Damage parameter $ {D}_{2} $	1.45
Elastic modulus $ E/\mathrm{M}\mathrm{P}\mathrm{a} $	7×10⁴	Material parameter n	0.34	Damage parameter $ {D}_{3} $	−0.47
Poisson’s ratio $ \gamma $	0.33	Material parameter m	1	Damage parameter $ {D}_{4} $	0.011
Material parameter $ A/\mathrm{M}\mathrm{P}\mathrm{a} $	265	Reference strain rate $ {\dot{\epsilon }}_{0}/\mathrm{S} $	1.34	Damage parameter $ {D}_{5} $	1.6
Material parameter B$ /\mathrm{M}\mathrm{P}\mathrm{a} $	426	Damage parameter $ {D}_{1} $	−0.77	Reference strain rate $ {\dot{\epsilon }}_{0}/\mathrm{S} $	1

下载: 导出CSV

表 5 6个CFRP试件的数据

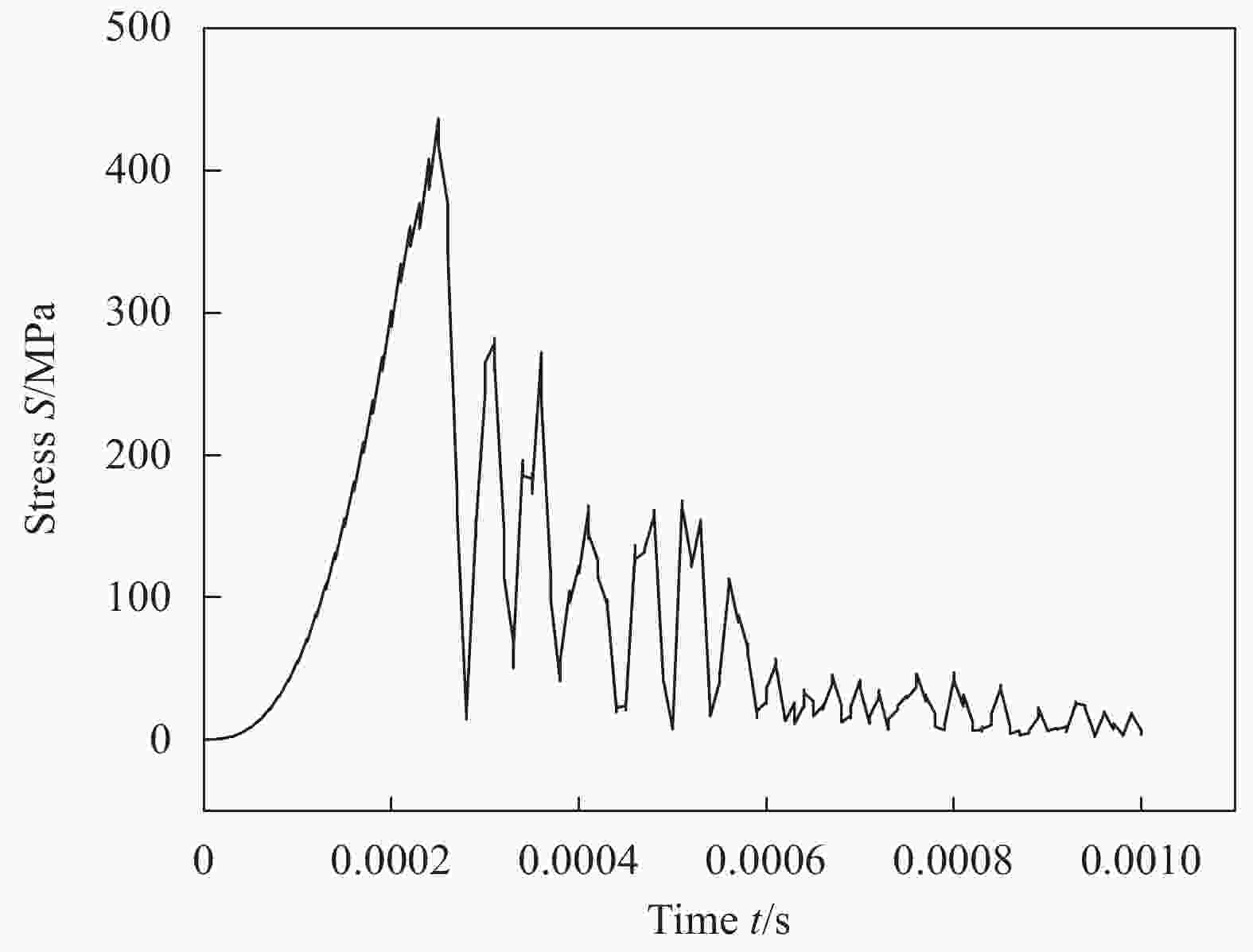
Table 5. Data of 6 experimental CFRP specimens

Number	Maximum load/kN	Residual strength/MPa
1	56.92	419
2	54.39	400
3	57.22	421
4	55.87	406
5	61.04	441
6	54.19	399

下载: 导出CSV

表 6 20层CFRP防撞梁复合材料层失效单元数统计

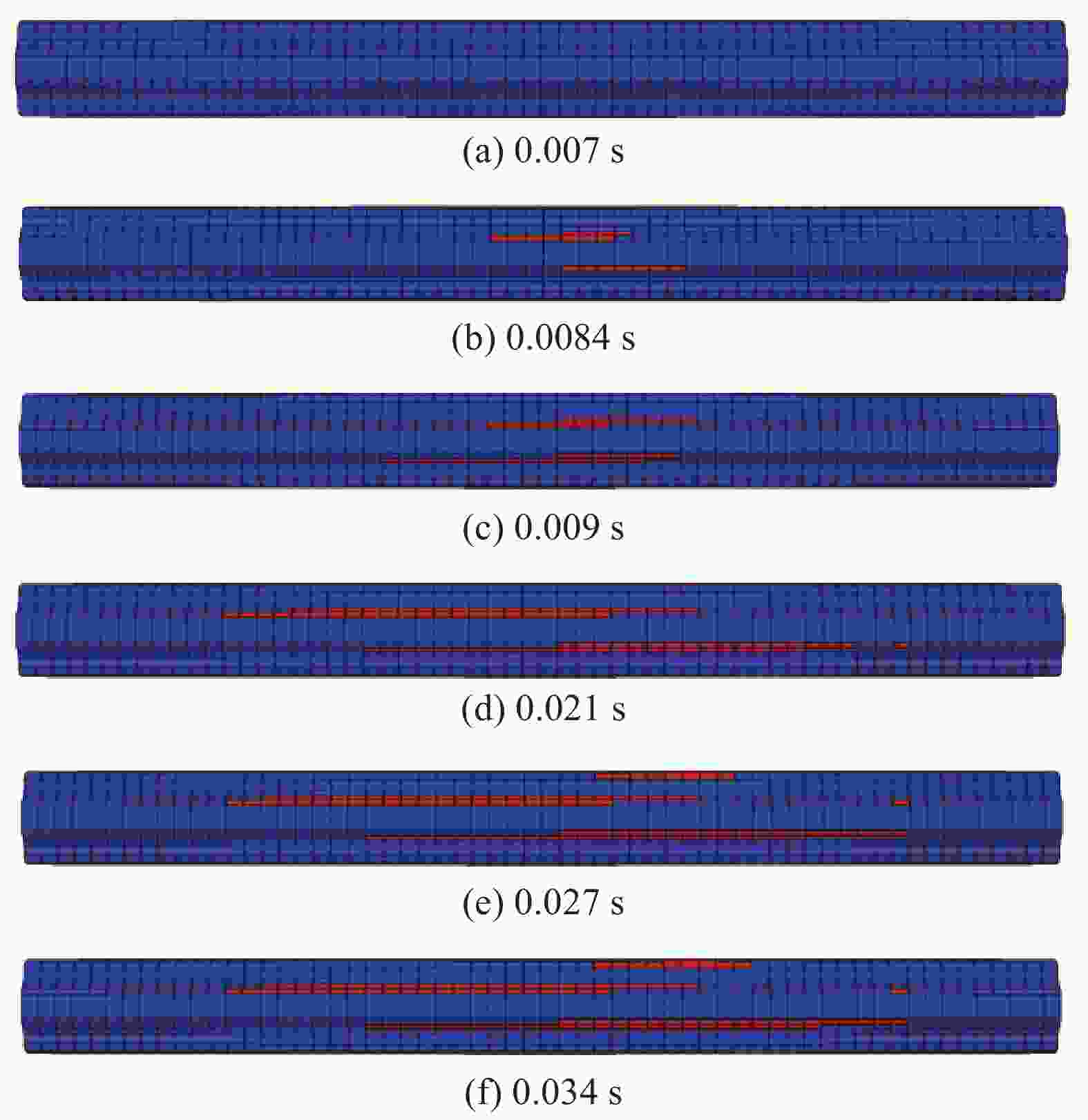
Table 6. Statistics on the number of failure units of 20 composite material layers of CFRP anti-collision beam

Concrete layer number	FV1	FV2	FV3	FV4	FV5	FV6	Total	Angle/(°)
1	0	187	213	0	5	202	607	45
2	0	147	227	0	5	178	557	−45
3	0	129	164	0	7	187	487	45
4	0	184	54	7	2	106	353	0
5	0	178	52	7	2	108	347	0
6	0	0	236	42	57	41	376	90
7	0	102	198	0	0	151	451	−45
8	0	181	91	13	5	108	398	0
9	0	184	102	14	4	90	394	0
10	0	0	122	44	56	119	341	90
11	0	0	134	45	54	133	366	90
12	0	136	103	10	6	97	352	0
13	0	129	97	10	6	105	347	0
14	0	138	236	0	11	193	578	−45
15	0	0	171	38	47	141	397	90
16	0	86	103	8	10	109	316	0
17	0	90	104	8	8	109	319	0
18	0	85	116	0	8	207	416	45
19	0	68	183	0	13	235	499	−45
20	0	112	159	0	15	218	504	45

下载: 导出CSV

表 7 24层CFRP防撞梁复合材料层失效单元数统计

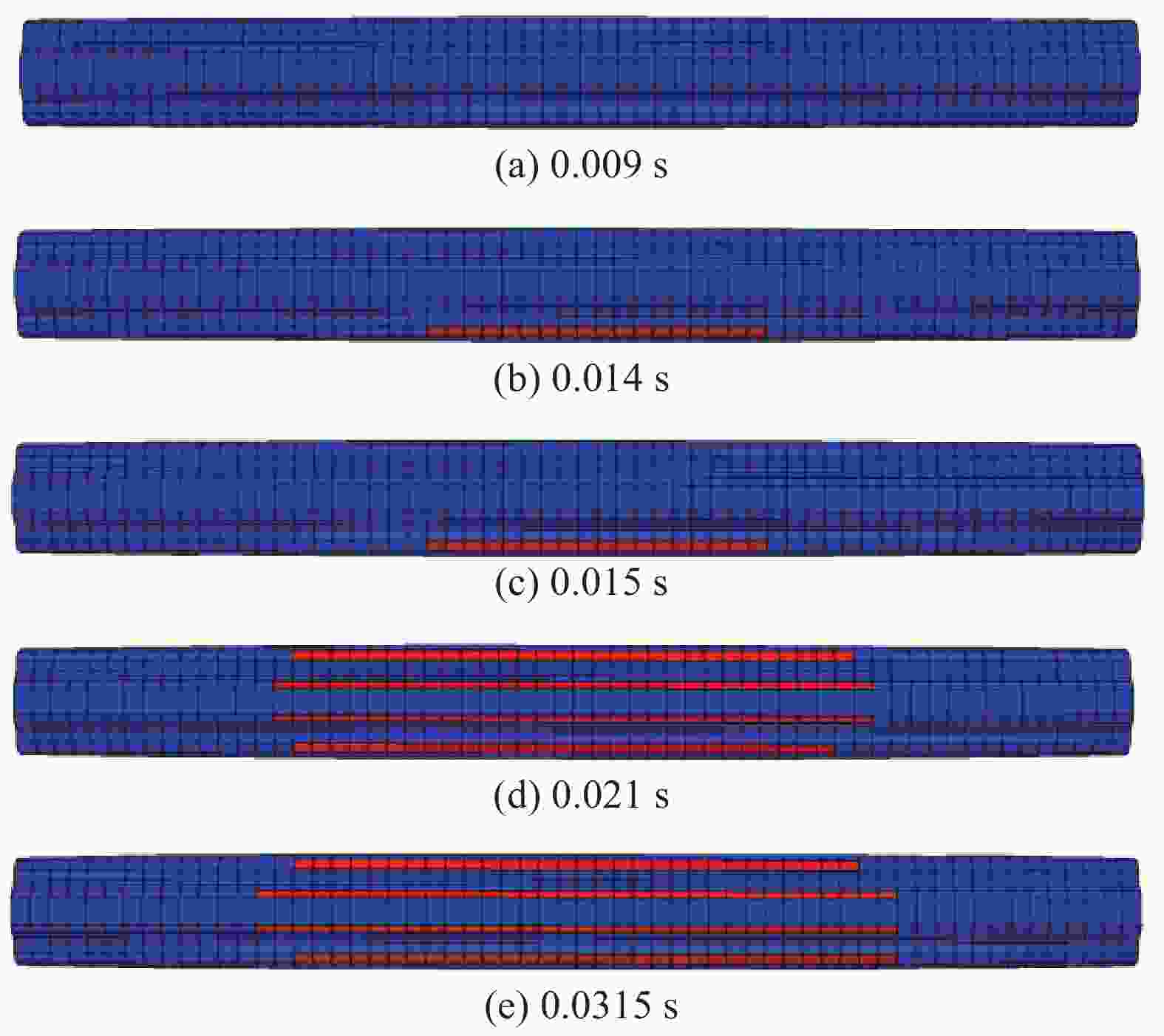
Table 7. Statistics on the number of failure units of 24 layers of composite material of CFRP anti-collision beam

Concrete layer number	FV1	FV2	FV3	FV4	FV5	FV6	Total	Angle/(°)
1	0	171	221	0	0	175	567	45
2	0	176	197	0	1	197	571	−45
3	0	145	171	0	2	177	495	45
4	0	226	65	9	6	20	326	0
5	0	207	51	8	8	21	295	0
6	0	185	36	9	4	20	254	0
7	0	152	143	0	2	87	384	−45
8	0	0	143	14	0	58	215	90
9	0	125	134	0	3	58	320	−45
10	0	184	42	5	7	25	263	0
11	0	178	52	3	7	28	268	0
12	0	0	106	15	0	112	233	90
13	0	0	15	13	0	155	183.	90
14	0	374	138	6	3	11	532	0
15	0	149	99	5	8	28	289	0
16	0	80	28	0	2	100	210	−45
17	0	0	12	6	0	153	171	90
18	0	66	20	0	0	123	209	−45
19	0	170	21	3	11	35	240	0
20	0	181	34	2	12	32	261	0
21	0	225	58	2	11	32	328	0
22	0	77	33	0	3	205	318	45
23	0	79	24	0	0	192	295	−45
24	0	90	53	0	3	213	359	45

下载: 导出CSV

表 8 铝合金层和CFRP层失效单元数统计

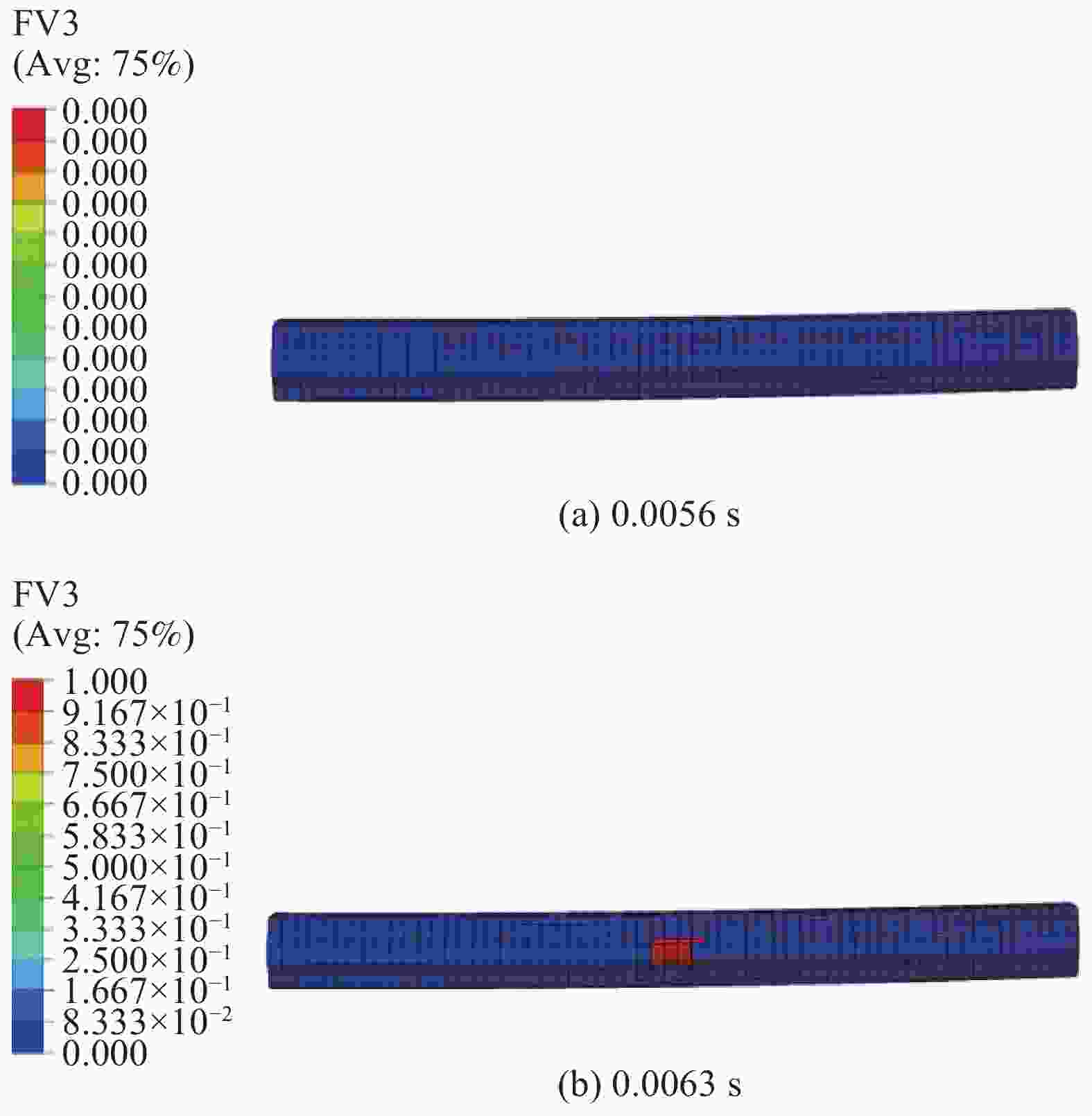
Table 8. Statistics of the number of failure units of the aluminum alloy layer and the CFRP layer

Concrete layer number	FV1	FV2	FV3	FV4	FV5	FV6	Total	Angle/(°)
1	0	0	0	0	0	0	0(SDEG)	Aluminum alloy
2	0	0	0	0	0	0	0(SDEG)	Aluminum alloy
3	0	0	0	0	0	0	0(SDEG)	Aluminum alloy
4	0	103	5	4	2	23	137	0
5	0	17	1	4	2	20	44	0
6	0	1	1	4	2	20	28	0
7	0	36	26	0	0	30	92	−45
8	0	0	0	0	0	62	62	90
9	0	43	33	0	0	70	146	−45
10	0	71	41	0	4	17	133	0
11	0	80	0	1	5	17	103	0
12	0	0	0	0	0	173	173	90
13	0	0	0	0	0	176	176	90
14	0	0	0	0	0	0	0(SDEG)	Aluminum alloy
15	0	134	4	1	6	16	161	0
16	0	51	10	0	0	139	200	−45
17	0	0	5	0	0	198	203	90
18	0	55	13	0	0	140	208	−45
19	0	224	126	0	6	20	376	0
20	0	226	129	0	5	21	381	0
21	0	262	133	0	8	20	423	0
22	0	58	30	0	3	155	246	45
23	0	61	78	0	0	164	303	−45
24	0	73	47	0	3	197	320	45
Note: SDEG—Scalar stiffness degradation, when SDEG>1, the material fails.

下载: 导出CSV

参考文献(23)

[1]	WANG Kangkang, ZHAO Libin, HONG Haiming, et al. A strain-rate-dependent damage model for evaluating the low velocity impact induced damage of composite laminates[J]. Composite Structures,2018,201:995-1003. doi: 10.1016/j.compstruct.2018.06.046
[2]	CAMINERO M A, GARCÍA-MORENO I, RODRÍGUEZ G P. Damage resistance of carbon fibre reinforced epoxy laminates subjected to low velocity impact: Effects of laminate thickness and ply-stacking sequence[J]. Polymer Testing,2017,63:530-541. doi: 10.1016/j.polymertesting.2017.09.016
[3]	AL-HAJAJ Z, SY B L, BOUGHERARA H, et al. Impact properties of a new hybrid composite material made from woven carbon fibres plus flax fibres in an epoxy matrix[J]. Composite Structures,2019,208:346-356.
[4]	PAPA I, BOCCARUSSO L, LANGELLA A, et al. Carbon/glass hybrid composite laminates in vinylester resin: Bending and low velocity impact tests[J]. Composite Structures,2020,232:111571.
[5]	HE B, WANG B, WANG Z, et al. Mechanical properties of hybrid composites reinforced by carbon fiber and high-strength and high-modulus polyimide fiber[J]. Polymer,2020,204:122830.
[6]	ZHOU J, LIAO B, SHI Y, et al. Low-velocity impact behavior and residual tensile strength of CFRP laminates[J]. Composites,2019,161:300-313.
[7]	PUSHPARAJA M, BALAGANESAN G, VELMURUGAN R, et al. Energy absorption characteristics of carbon/epoxy nano filler dispersed composites subjected to localized impact loading[J]. Procedia Engineering,2017,173(Complete):175-181.
[8]	SAMAL S, MARVALOVA B, PETRIKOVA I, et al. Impact and post impact behavior of fabric reinforced geopolymer composite[J]. Construction & Building Materials,2016,127(nov. 30):111-124.
[9]	CAMINERO M A, GARCÍA-MORENO I, RODRIGUEZ G P, et al. Experimental study of the influence of thickness and ply-stacking sequence on the compression after impact strength of carbon fibre reinforced epoxy laminates[J]. Polymer Testing,2018,66:360-370.
[10]	SALVETTI M, SBARUFATTI C, GILIOLI A, et al. On the mechanical response of CFRP composite with embedded optical fibre when subjected to low velocity impact and CAI tests[J]. Composite Structures,2017,179:21-34.
[11]	FOTOUHI M, JALALVAND M, WISNOM M R. High performance quasi-isotropic thin-ply carbon glass hybrid composites with pseudo-ductile behaviour in all fibre orientations[J]. Composites Science and Technology,2017,152:101-110.
[12]	王莉, 熊舒, 肇研, 等. T800级碳纤维复合材料抗冲击性能[J]. 航空材料学报, 2018, 38(5):147-151. WANG Li, XIONG Shu, ZHAO Yan, et al. Impact resistance of T800 grade carbon fiber composites[J]. Journal of Aeronautical Materials,2018,38(5):147-151(in Chinese).
[13]	任明伟, 洪治国, 周玉敬, 等. 复合材料防撞梁低速碰撞优化设计[J]. 复合材料学报, 2022, 39(2): 854-862. REN Mingwei, HONG Zhiguo, ZHOU Yujing, et al. Low-speed collision optimization design of composite anti-collision beams[J]. Acta Materiae Compositae Sinica, 2022, 39(2): 854-862(in Chinese).
[14]	陈静, 唐傲天, 田凯, 等. 碳纤维复合材料防撞梁轻量化设计[J]. 汽车工程, 2020, 42(3):390-395. CHEN Jing, TANG Aotian, TIAN Kai, et al. Lightweight design of carbon fiber composite anti-collision beam[J]. Automotive Engineering,2020,42(3):390-395(in Chinese).
[15]	张超, 方鑫, 刘建春. 复合材料层板冰雹高速冲击损伤预测及失效机理分析[J/OL]. 北京航空航天大学学报: 1-14[2021-05-20]. https://doi.org/10.13700/j.bh.1001-5965.2020.0636. ZHANG Chao, FANG Xin, LIU Jianchun. High-speed impact damage prediction and failure mechanism analysis of composite laminates with hail[J/OL]. Journal of Beijing University of Aeronautics and Astronautics: 1-14[2021-05-20]. https://doi.org/10.13700/j.bh.1001-5965.2020.0636 (in Chinese).
[16]	周俊杰, 王生楠. 复合材料层合板低速冲击渐进损伤模型[J]. 西北工业大学学报, 2021, 39(1):37-45. doi: 10.3969/j.issn.1000-2758.2021.01.005 ZHOU Junjie, WANG Shengnan. Progressive damage model of composite laminates under low-velocity impact[J]. Journal of Northwestern Polytechnical University,2021,39(1):37-45(in Chinese). doi: 10.3969/j.issn.1000-2758.2021.01.005
[17]	拓宏亮, 马晓平, 卢智先. 基于连续介质损伤力学的复合材料层合板低速冲击损伤模型[J]. 复合材料学报, 2018, 35(7):202-212. TUO Hongliang, MA Xiaoping, LU Zhixian. Low-velocity impact damage model of composite laminates based on continuum damage mechanics[J]. Acta Materiae Compo-sitae Sinica,2018,35(7):202-212(in Chinese).
[18]	孔祥宏, 王志瑾. 基于Abaqus的有限元渐进损伤强度分析[J]. 计算机应用与软件, 2012, 29(10):236-240. KONG Xianghong, WANG Zhijin. Finite element progressive damage analysis based on Abaqus[J]. Computer Applications and Software,2012,29(10):236-240(in Chinese).
[19]	王涛, 侯玉亮, 铁瑛, 等. 基于ECPL模型的平纹机织复合材料低速冲击多尺度模拟[J]. 振动与冲击, 2020, 39(20):295-304. WANG Tao, HOU Yuliang, TIE Ying, et al. Multi-scale simulation of low-velocity impact of plain woven composites based on ECPL model[J]. Journal of Vibration and Shock,2020,39(20):295-304(in Chinese).
[20]	周琳. 金属材料新的动态本构模型[D]. 合肥: 中国科学技术大学, 2019. ZHOU Lin. New dynamic constitutive model of metal materials[D]. Hefei: University of Science and Technology of China, 2019(in Chinese).
[21]	崔俊杰, 郭章新, 朱明, 等. 表面带金属层的复合材料层合板低速冲击数值模拟[J]. 材料导报, 2021, 35(4):4150-4158. CUI Junjie, GUO Zhangxin, ZHU Ming, et al. Numerical simulation of low-velocity impact of composite laminates with metal layers on the surface[J]. Materials Reports,2021,35(4):4150-4158(in Chinese).
[22]	孔祥宏, 王志瑾. 复合材料层压板压缩剩余强度分析[J]. 飞机设计, 2014, 34(6):42-47, 52. KONG Xianghong, WANG Zhijin. Analysis of compression residual strength of composite laminates[J]. Aircraft Design,2014,34(6):42-47, 52(in Chinese).
[23]	肖金涛, 张帅, 徐锐良, 等. 碳纤维复合材料防撞梁与铝合金吸能盒低速碰撞轻量化设计优化[J]. 河南科技大学学报(自然科学版), 2021, 42(3):25-32, 33. XIAO Jintao, ZHANG Shuai, XU Ruiliang, et al. Low-speed collision lightweight design optimization of carbon fiber composite anti-collision beam and aluminum alloy energy-absorbing box[J]. Journal of Henan University of Science and Technology: Natural Science,2021,42(3):25-32, 33(in Chinese).