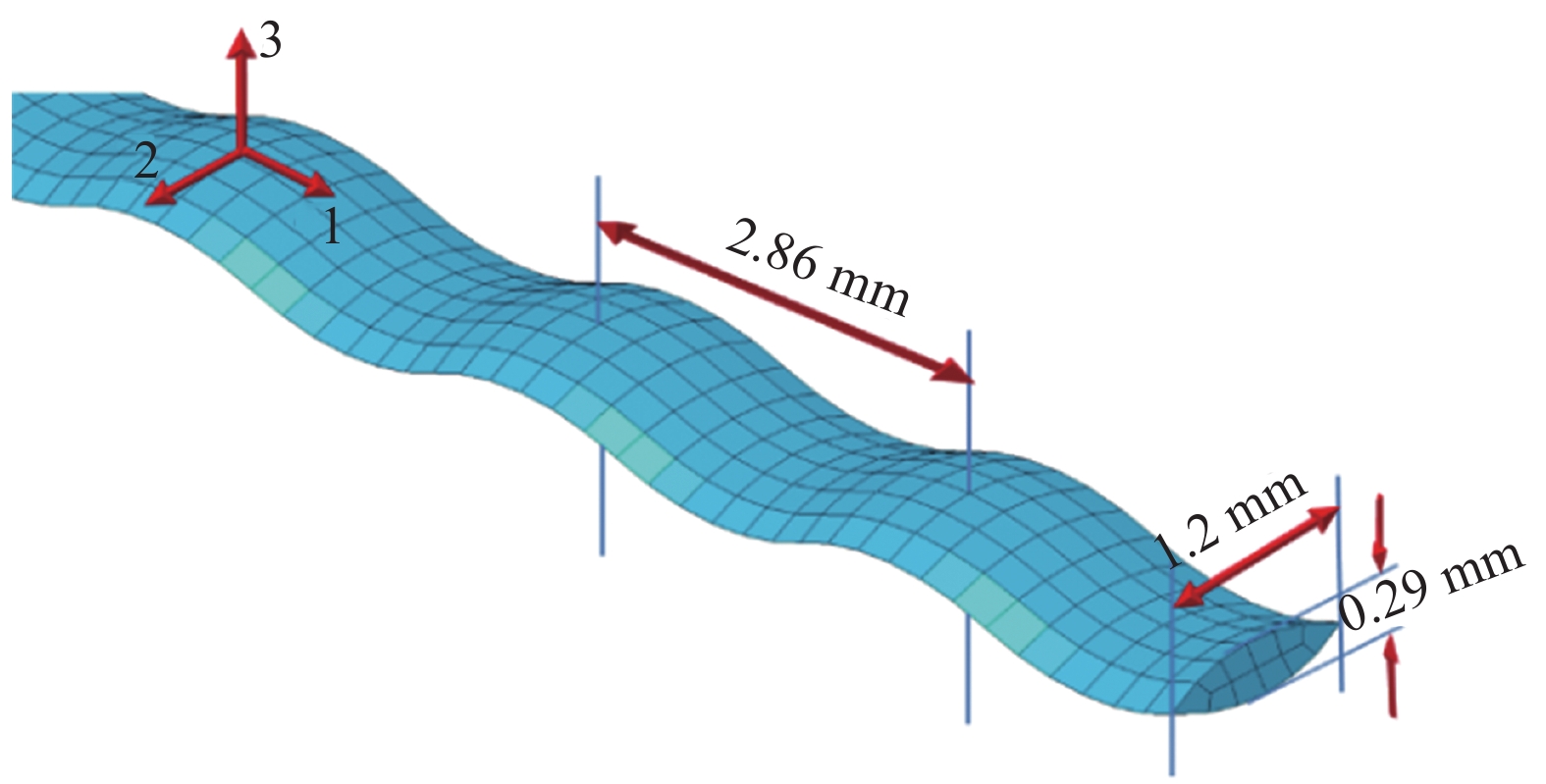
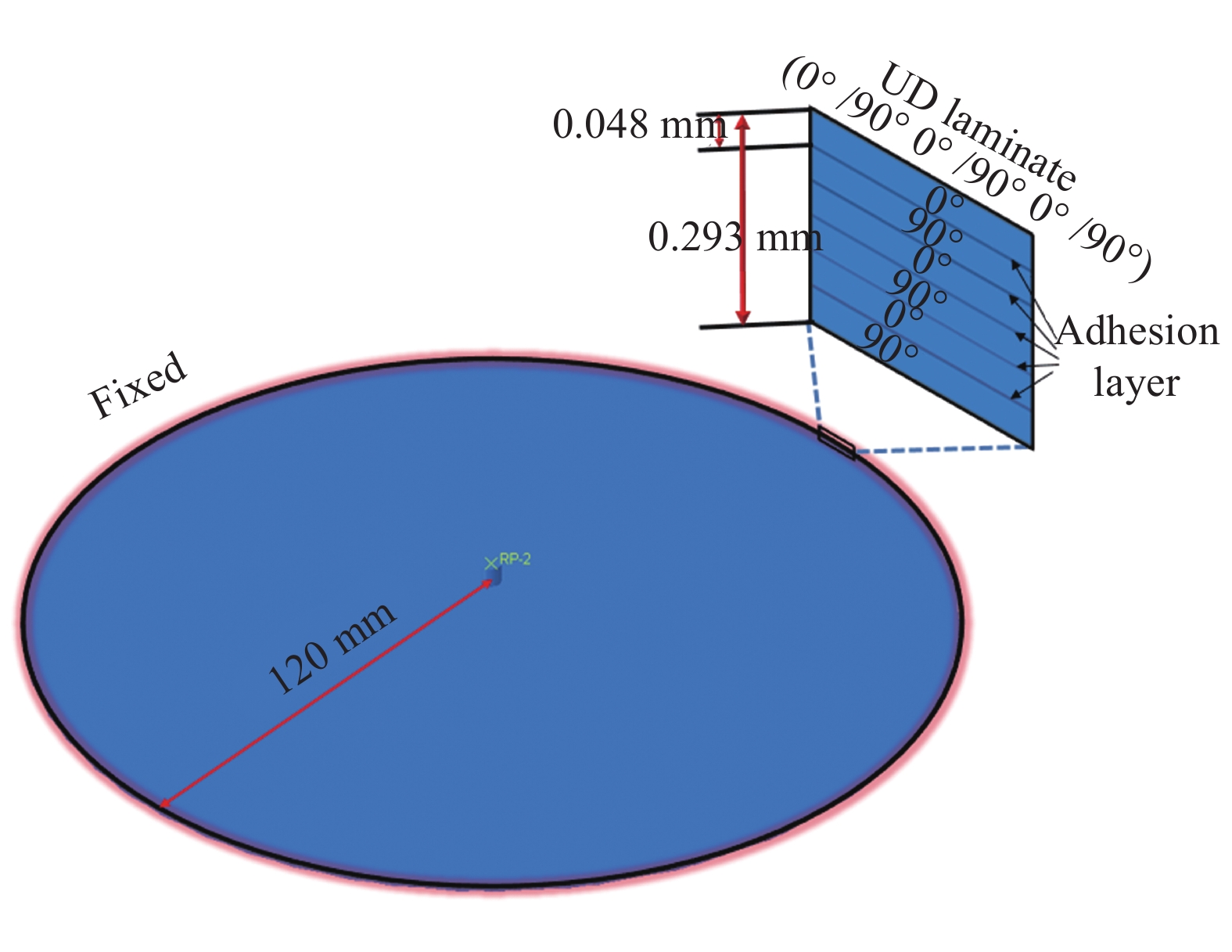
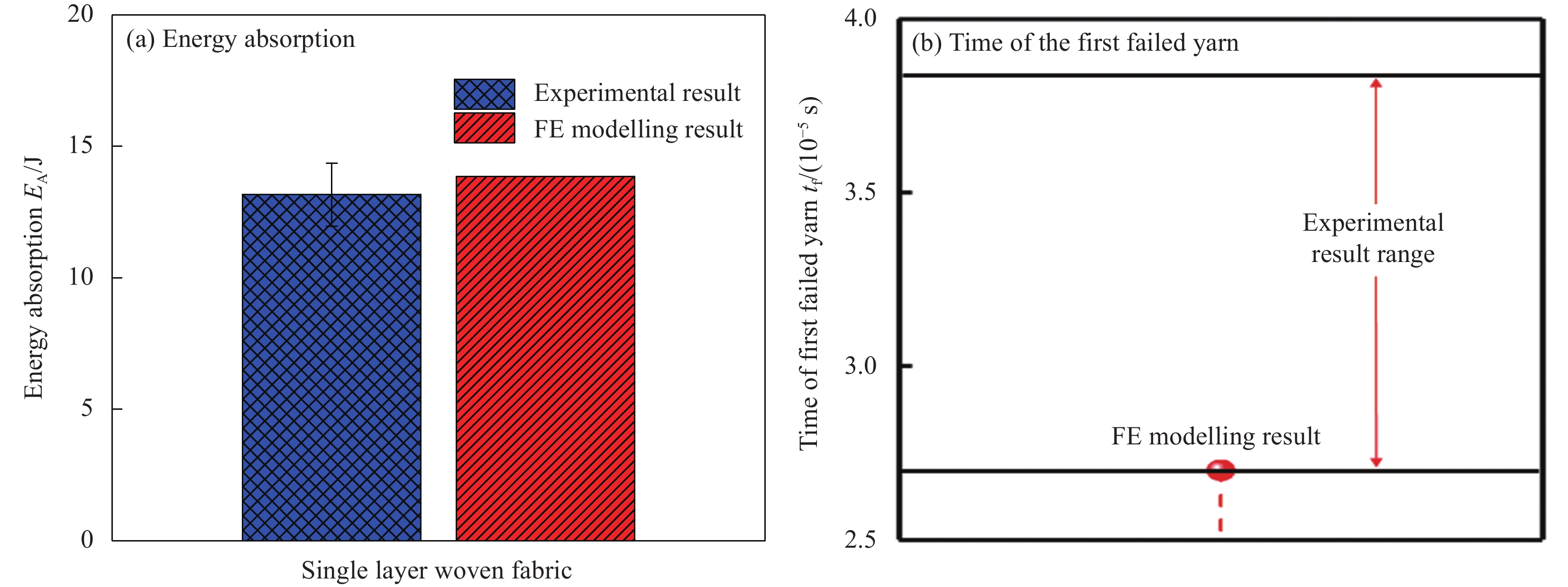
Ballistic mechanism of the hybrid panels with UHMWPE woven fabrics and UD laminates
-
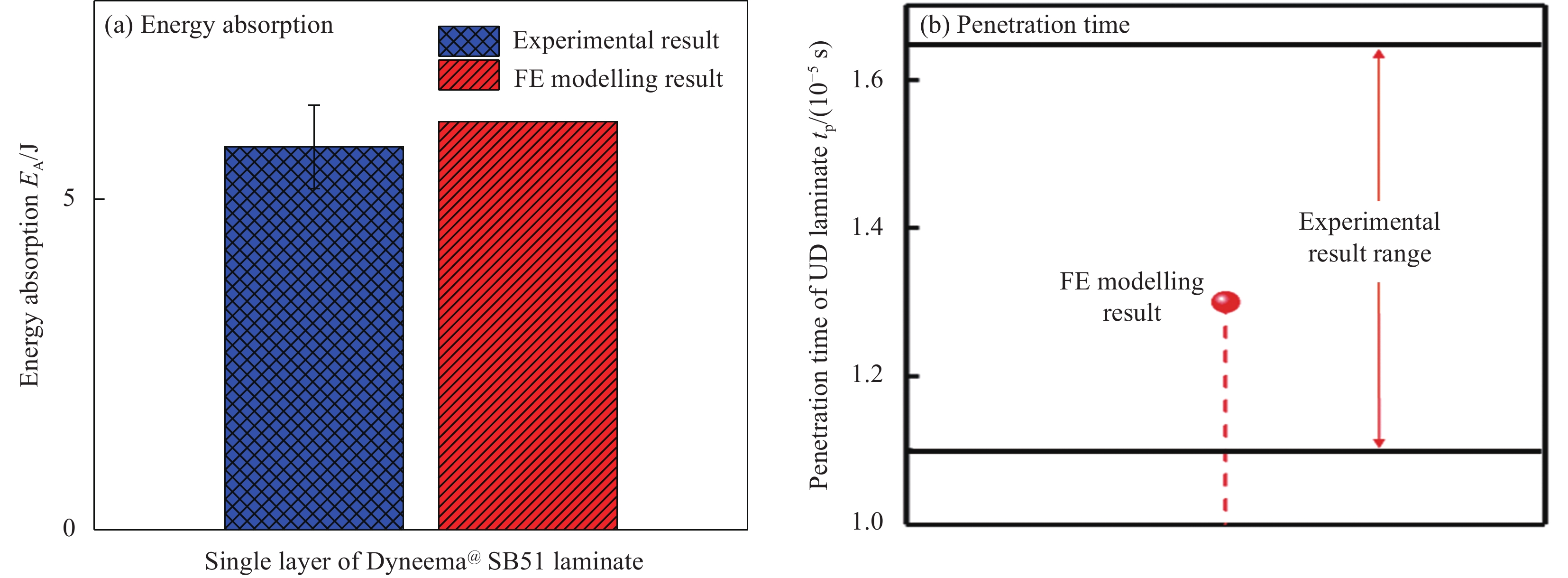
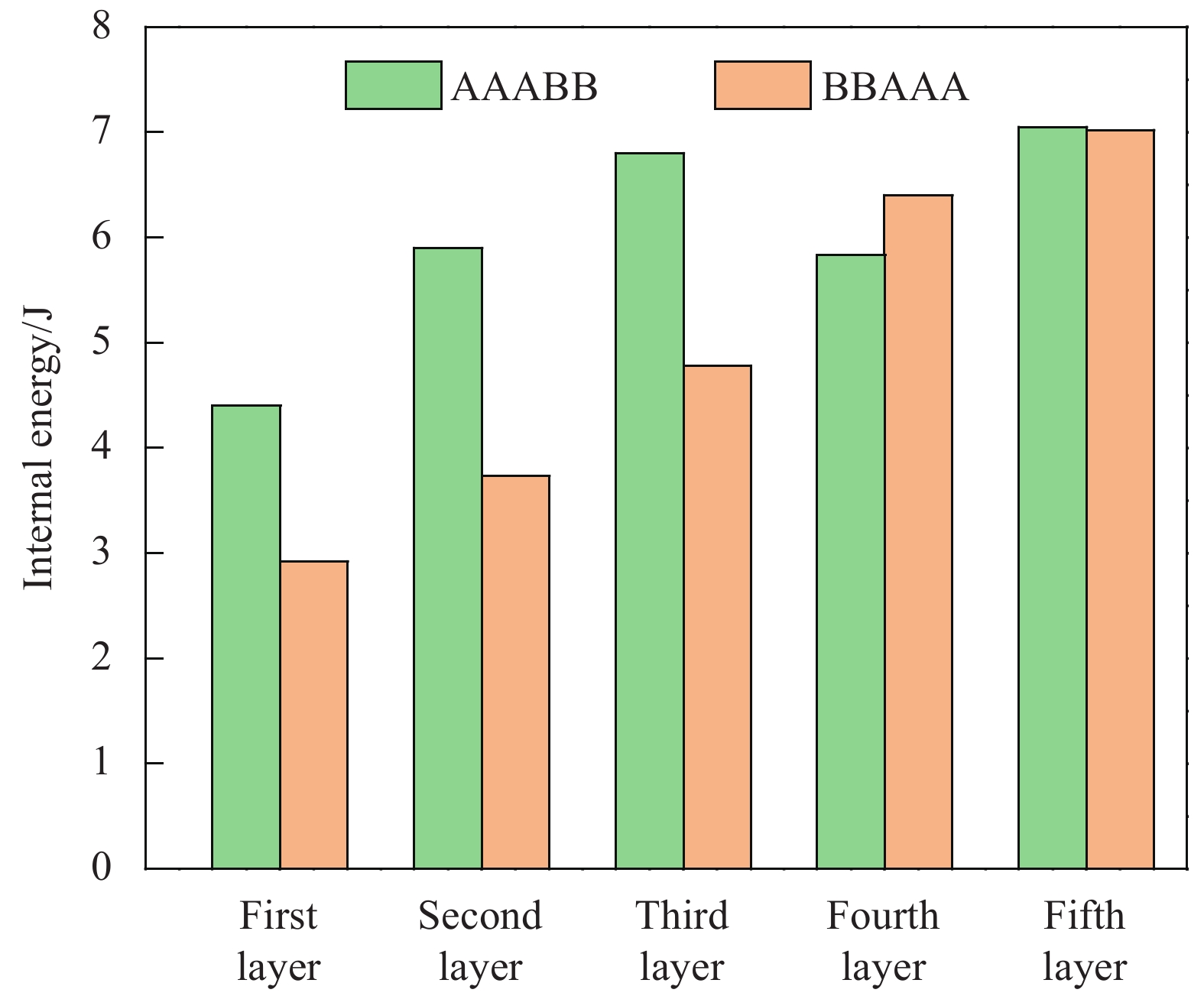
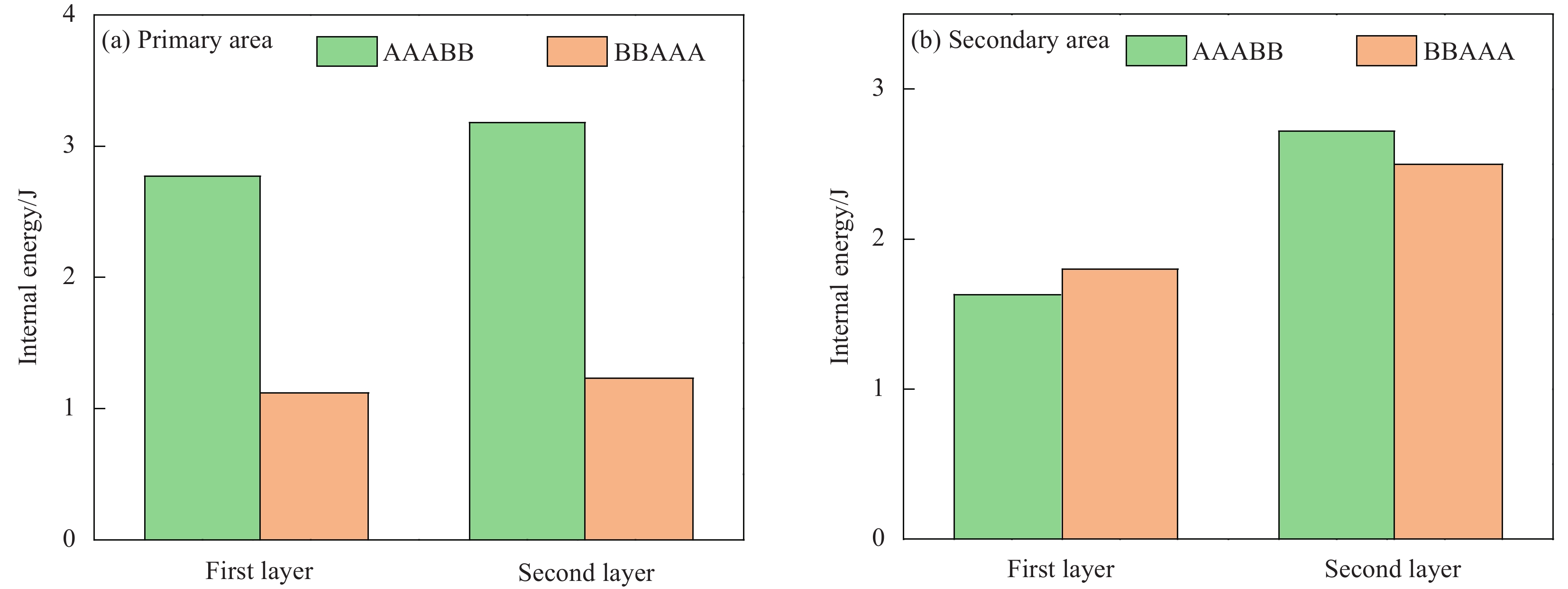
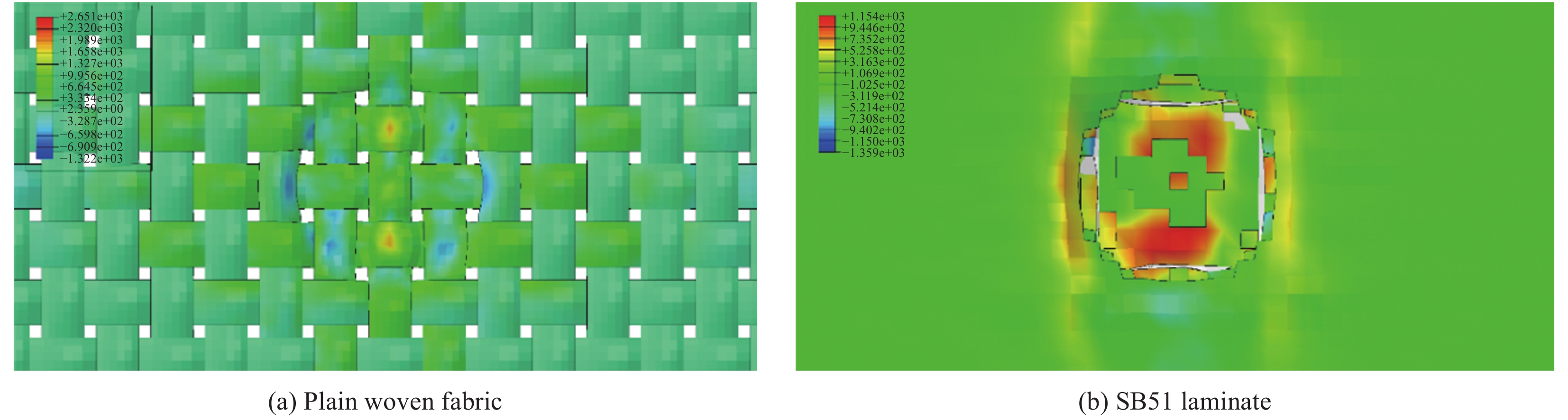
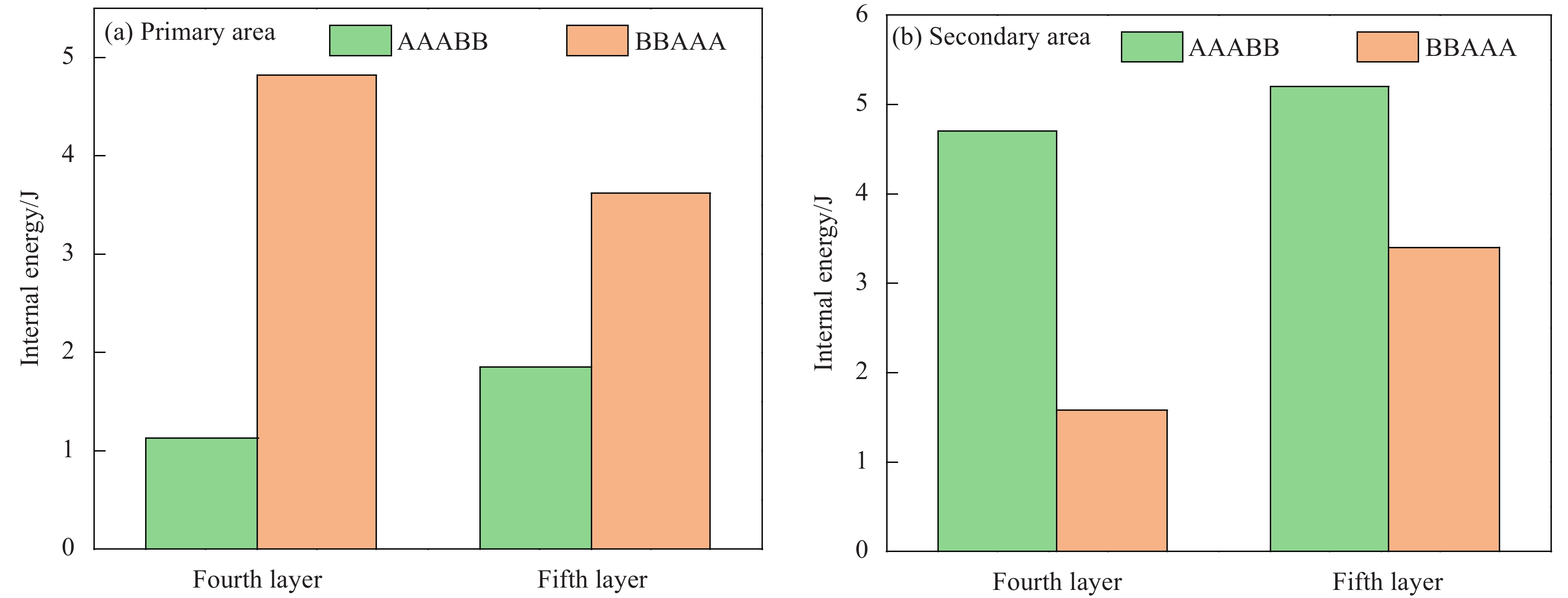
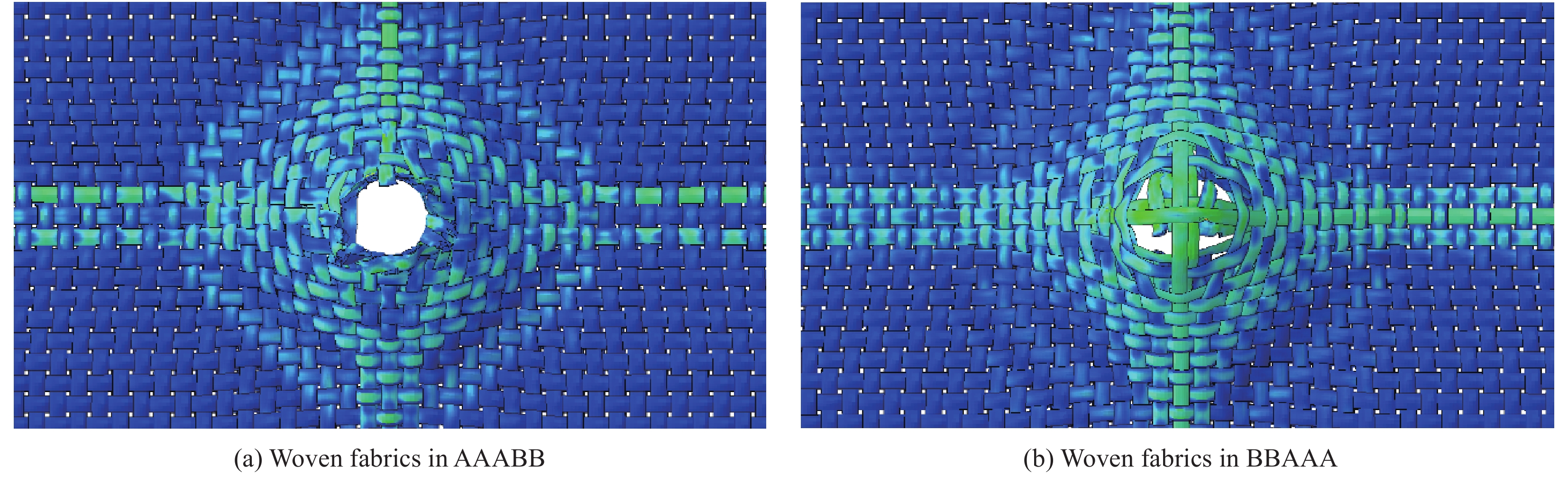
摘要: 柔性防弹衣具有隐蔽性好、穿着舒适的优点，而采用平纹与单向(UD)布杂化结构具有更好的防护效果。本文采用三层超高分子量聚乙烯(UHMWPE)纤维平纹织物(A)和两层Dyneema® SB51 UD布(B)组成AAABB和BBAAA两种混合靶板，通过弹道实验比较两种排列方式的防弹性能差异。结果表明，将平纹织物在前UD布在后能大幅提升整块板的防弹性能，能量吸收比后者高约20%。进一步采用有限元模拟来阐明其防弹机制，模拟结果表明将平纹织物放在面层不易被切断，使得平纹织物层发生更大的形变，也使后面的UD布发生大面积形变，吸收大量能量。而UD布放在前面层易产生的切力破坏，失去对后面层的作用。而平纹织物在后层容易发生滑移，且形变纵深过大，不利于防弹保护。该研究结果阐明了平纹织物和UD布不同顺序堆叠时的防弹机制，为进一步优化设计该类柔性防弹衣提供了坚实的理论基础。Abstract: Soft body amour has the advantages of good concealment and high comfortability. Recent studies demonstrate that soft body amours made up of layers of woven fabrics and unidirectional (UD) laminates show better ballistic performance. However, the mechanism behind has not been clarified. This work utilized three layers of woven fabrics (A) made by ultrahigh molecular weight polyethylene (UHMWPE) yarns and two layers of Dyneema® SB51 UD laminates (B) to compose two types of hybrid panels, viz. AAABB and BBAAA. Ballistic tests were carried out to evaluate their performance. The results show that the first type panel absorbs around 20% more energy than the second counterpart does. The finite element modelling is used to clarify the ballistic mechanism. The results illustrate that when the woven fabrics in front, they are not easier to be failed by shear, which results in more deformation of the fabric and therefore more deformation of the UD laminates behind, thereby giving rise to higher energy absorption. In contrast, when the UD laminates in front, they are easier to be damaged by the shear stress, failing to act on the followed layers. Moreover, when the woven fabrics at the rear, they tend to slip and meanwhile bring about more deformation in depth, which is detrimental to the protection. This study sheds light on the ballistic mechanism of hybrid panels with laying woven fabrics and UD laminates in different sequences. Such results, theoretically, pave the way for the design of the hybrid soft body amours.
-
传统的固体火箭发动机复合材料壳体在筒身段往往采用的是等直径形式，便于缠绕线型的设计和工艺制备，以及简化壳体结构设计和强度校核工作。但直筒壳体在其他方面的表现也存在不足，例如燃烧效率低，燃烧不均匀，较大冗余质量等问题。带锥度壳体相对传统壳体来说，在保证结构强度和刚度的情况下，可以更多的降低壳体重量和缩减结构外形尺寸，提高载荷能力和整体性能以及减小气动阻力[1]。因此，锥柱于一体化复合材料壳体逐步成为固体火箭发动机复合材料壳体的一个研究热点。
在复合材料壳体结构受力形式研究方面，Wagner等[2]研究了锥形壳体结构在外压载荷下的稳定性，获得了壳体的外压临界载荷、屈曲失稳波形、轴向位移以及径向位移随外压载荷的变化规律；李靖[3]研究了直筒壳体应力形式，并基于网格理论研究了壳体直筒结构爆破压强及爆破形式；祖磊等[4]基于板壳力学建立的复合材料壳体有矩模型探究了壳体封头复杂的内部机理，获得了典型直筒复合材料壳体内压作用下的应力响应分布规律；张行等[5]建立了封头逐层预测方法研究了厚度变化对封头的影响。复合材料壳体因其受结构上金属接头尺寸、开口比、封头形状等，以及材料上金属、树脂基复合材料等的综合影响，导致其封头内压载荷呈现复杂受力模式[6]。为此，工程上常采用补强方式实现其封头结构的增强，在此方面诸多学者结合封头应力分布状态特征开展了复合材料壳体封头补强方面[7-9]的研究，得到了补强层厚度以及补强区间对壳体性能的影响，并考虑到不同补强方式的影响，以及纵向和环向补强对封头性能提升的效果[10]。
在复合材料壳体结构损伤失效研究方面，有学者基于采用剪切滞后理论和能量法建立的损伤模型探明了基体开裂[11]和分层损伤[12]分别对壳体承载性能的影响。也有学者利用参数化设计语言分析了纤维缠绕复合材料压力容器的渐进失效过程，并基于Hashin准则构建了复合材料壳体的渐进失效模型，预测了其损伤类型、演化以及最终爆破压力。同时，也有学者基于Abaqus UMAT分析了壳体内压下的破坏主要是为环向层纤维、纵向层基体破坏，其中，喻琳峰[13]构建了复合材料壳体封头精细化缠绕模型，探究了其可能得破坏原因以及关键影响参数。Lin等[14]基于PUCK准则开展渐进失效分析，通过爆压试验验证了其复合材料结构渐进损伤本质；另外，考虑到各种前置结构形式、工艺等对纤维缠绕增材制造结构件承载性能和损伤演化过程的影响，Lokesh等[15]对典型的复合材料壳体进行了失效模式影响分析和失效模式影响与临界度分析，以研究所有潜在的失效模式及其严重程度对壳体最终性能的影响。Liu[16]基于细化有限元模型的薄壁复合材料壳体二次加载过程损伤分析方法，研究了薄壁复合材料壳体二次加载过程造成的损伤和壳体性能下降规律。Weerts[17]研究了直筒结构压力容器在不同内压载荷下的抗接触破坏强度研究，以及其对结构剩余强度的影响。Tales[18]研究不同缠绕模式的直筒结构筒身在径向压力作用下的损伤失效行为，获得了不同缠绕线型对径向外载的应力响应及损伤演化规律。
综上所述，国内对于复合材料壳体的研究主要以直筒为主，国外主要在理论上和失效模式等方面对其结构强度和结构进行分析，对该类锥柱一体化纤维缠绕复合材料壳体的线型设计、结构设计以及损伤失效演化过程方面并未展开过多的研究。为此，本文针对锥柱一体化复合材料壳体开展缠绕线型、补强结构、损伤失效研究，基于非测地线理论和连分数方式开展其缠绕线型研究，得到了实现其均匀布满的合适切点数和滑移系数；运用网格理论开展壳体直筒段初始铺层设计,引入轴对称单元实现壳体应力分布特征快速获取以及补强结构的快速确定；结合Hashin准则开展其典型损伤失效过程分析研究，得到了该锥柱一体复合材料壳体结构典型失效模式和损伤演化过程。
1. 锥柱一体化壳体缠绕线型设计
本文所研究的锥柱一体化复合材料壳体结构如图1所示，其结构主要包括绝热层内衬、前后金属接头、前后金属堵盖、复合材料层。壳体封头采用的是椭球封头，其中锥段封头长半轴90 mm，椭球比为1.7，极孔半径为30 mm，直筒段封头长半轴100 mm，椭球比为1.7，极孔半径为30 mm, 前后接头的肩宽比为1.8，前后接头尺寸为M24×3的内螺纹通孔，前后堵头为M24×3外螺纹。
![]()
图 1 锥柱一体化纤维缠绕壳体结构(单位：mm)Figure 1. Fiber wound structure of the cone-cylinder integrated composite case (Unit: mm)针对上述锥柱一体化复合材料壳体具有结构上的特殊性、缠绕线型上的多样性、应力响应上的复杂性，因此本文采用如图2所示锥柱一体化复合材料壳体分析流程，实现满足其承载能力要求的结构设计和结构承载过程中的损伤失效行为演化研究。首先，获得直筒段缠绕角度分布范围，为锥段和直筒段角度非测地线连续变化提供设计裕度。其次，根据直筒段缠绕角度计算得到初始铺层参数，获得未补强壳体应力分布状态和结构危险区域。最后，根据补强结构受力状态分析其承载过程中的损伤演化过程。
![]()
图 2 锥柱一体化复合材料壳体缠绕线型、铺层结构设计、补强及损伤失效分析流程Figure 2. Analysis flow of the trajectory patterns design, laminate design, reinforcement structure design, and damage and failure of the cone-cylinder integrated composite case1.1 纤维缠绕壳体均匀布满设计
由于锥柱一体化壳体结构封头形状以及筒身形状变化，采用测地线单一缠绕模式无法适应锥柱一体化壳体稳定缠绕及均匀布满要求，为此需用非测地线[19-20]模式进行壳体结构设计以满足稳定缠绕要求，其缠绕角和中心转角的微分方程可表述为
{dαdz=λ[(1+r′2)sin2α−rr″ (1) 其中：w 为纤维缠绕整数圈，d 为是时序相邻两根纱束之间跨过的距离，D 为筒身直径，B 为纱片宽度，α 为缠绕角度，z为轴向坐标，θ为 芯模中心转角，λ 为滑移系数，r 封头平行圆半径，r'和r''分别为封头处平行圆半径方程的一阶导数和二阶导数，N为以纱带宽度等分周长数
调整不同的滑移系数可以得到不同缠绕线型，较多切点数会导致封头架空较多，导致封头强度下降。为此，本文通过优化滑移系数确定了直筒段封头滑移系数为
0.01966 ，锥段封头滑移系数为0.02227 ，保证了缠绕线型的稳定实现。考虑缠绕线型从左极孔出发经过筒身，穿过左赤道圆、锥柱交接区、锥段封头赤道并到达右极孔的线型必须要是连续的，缠绕线型设计中需保留直筒段和锥段封头在赤道圆处所共有部分。结合上述角度控制方式和中心转角公式计算得到当前中心转角范围，进而得到当前缠绕壳体在该设定参数下的m、k (m是纱带宽等分赤道圆周长的整数，k是赤道处相邻两次缠绕的间距)。考虑到较大的缠绕切点数会在封头部位形成较多的架空缺陷，影响封头整体承载能力，较小的缠绕切点数又会使得极孔附近堆积较厚，工艺常采用不大于10切点进行缠绕制备。为此本文采用中位数5切点数缠绕线型进行后续得研究工作。芯模转角为488.85°，因此丝嘴来回一次对应的主轴转数488.85/360=1.3579 =2−0.6421 ，其中该壳体缠绕采用的带宽B=10 mm，筒身直筒段缠绕角设定为17.5°，求得等分数m=59~62，跨距k=0.6421 N，取整后得到k=37~39。为满足壳体均匀布满规律引用连分数法[21]以确定纤维缠绕工艺所需参数。将上述列表中的一系列k/m值转化为连分数形式，得到缠绕线型对应的切点数、级数、总循环圈数、小车往返一次的中心转角等一系列缠绕线型参数，具体内容如表1所示。
表 1 不同k/m对应的线型切点数及实际转角Table 1. Count of tangent points and actual rotation angle of the trajectories corresponding to different k/mNo. k/m Tangent point count/series Rotation angle of meridian/(°) Error/(°) 1 37/59 8/4 494.24 5.39 2 38/59 14/4 488.14 −0.71 3 39/59 3/3 482.03 −6.82 4 37/60 13/6 498.00 9.15 5 37/61 28/6 501.64 12.79 6 38/61 8/5 495.74 6.89 7 39/61 25/5 489.84 0.99 8 37/62 5/3 505.16 16.31 9 39/62 27/5 493.55 4.7 Notes：k is the distance between two adjacent points at the equator circle; m is the integer of equator circle splitting count by bandwidth. 1.2 壳体锥段缠绕线型设计
由于缠绕线型在芯模上是连续的，为实现封头和直筒段角度变化连续，且锥段不发生滑纱现象，需调整锥段滑移系数使得缠绕角从锥段的大端17.5°稳定过渡到小端的19.5°，结合该壳体尺寸得到锥段滑移系数为0.035，该部分对应的缠绕角度、缠绕厚度分布变化如图3所示。可知，与直筒身结构相比锥段的缠绕厚度和缠绕角度呈现非线性特征，且随着锥角的增大，缠绕角度非线性逐渐增强。
![]()
图 3 锥柱一体化复合材料壳体锥段缠绕角与缠绕层厚度分布：(a)缠绕角度分布；(b)缠绕厚度分布Figure 3. Winding angle and layer thickness distribution of the cone-cylinder integrated composite case: (a) Fiber wound angle distribution; (b) Thickness distribution为使得直筒段受力一致，其环向缠绕层滑移系数设定为0，但对于锥段的环向缠绕层而言，由于其存在3°的坡度，因此必须给环向缠绕线型的设计增加滑移系数以保证不发生滑砂。锥段不同位置的滑移系数可按下式进行计算：
\left. \begin{gathered} {k_0} = ({R_1} - {R_2})/(0 - L) \\ {h_y} = - k(r\sin (a) - {R_1}\sin ({\alpha _0}))/ \\ ((r - {R_1})\sin (a)\sin ({\alpha _0})) \\ \end{gathered} \right\} (2) 式中：k0表示曲线方程一阶导，hy表示锥段不同位置滑移系数，L为锥段总长，r表示锥段母线上不同位置其对应的平行圆半径，R1表示锥段大段的半径，R2表示锥段小段的半径，α表示其缠绕角，α0表示直筒段缠绕角。根据式(2)即可由锥柱交接处的连续性条件得到锥段滑移系数为-0.05。根据缠绕线型计算结果开展缠绕线型切根及均匀布满分析，得到其线型仿真结果如图4所示，可知螺旋层和环向层均可以很好的覆盖直筒段、直筒段和锥段封头、锥段。
![]()
图 4 锥柱一体化复合材料壳体结构缠绕线型仿真结果示意图：(a)螺旋层；(b)环向层Figure 4. Trajectories simulation results of the cone-cylinder integrated structure: (a) Telical layer; (b) Hoop layer综上，除筒身直段外均采用非测地线缠绕方式可以很好的实现缠绕线型的均匀布满，且先确定两侧封头及直筒段的均匀布满要求，在进一步调整筒身锥段的缠绕线型方式可以很好地满足锥柱一体结构缠绕线型设计。
2. 锥柱一体化复合材料壳体结构设计及损伤失效分析
2.1 未补强壳体模型建立及应力分析
考虑到复合材料结构在承载过程中存在的损伤及渐进失效过程，本文引入Hashin 失效准则[22]以判定宏观结构是否达到损伤状态，并结合Camanho退化方式建立了纤维缠绕复合材料刚度退化模型。所用材料参数值实验室自测结果如表2所示。壳体封头厚度采用三次样条公式实现其厚度分布预测[23]。筒身段螺旋层、环向层纤维单层厚度分别为0.133 mm、0.125 mm，考虑到壳体封头螺旋层强度难以高效发挥，引入应力平衡系数的方式来提高封头纵向层的承载能力[24]，防止其低压爆破，取值为0.75。得到其直筒段基于网格理论的得到的初始铺层参数为6层螺旋7层环向，铺层顺序定为[±17.5°/90°/±17.5°/90°2/±17.5°/90°3]。对于锥段变厚度变角度特征，采用和封头相同的建模方法，通过分割几何模型并为不同模型赋予离散正轴属性，实现各个模型离散角度、刚度信息表达。
表 2 复合材料力学性能参数Table 2. Mechanical property parameters of composite materialsType Parameters Value Strength/
MPaXT (0° tension) hoop layer 3556.00 XT (0° tension) helical layer 2600 Composite
materialXC (0° compression) 1354.00 YT (90° tension) 84.31 YC (90°compression) 190.00 S(shear) 60.67 Modulus/
GPaExt (0°Tension) 170.00 Exc (0°compression) 140.00 Eyt (90° tension) 8 Eyc (90° compression) 7.61 µxy 0.33 µyz 0.35 µxz 0.33 Gxy 4 Gyz 2.5 Gzx 4 EPDM E 0.9 μ 0.47 30 CrMnSiA E 196 μ 0.3 Notes：E is the modulus, μ is the Poisson's ratio, G is the shear modulus. 表 3 碳布补强层材料参数Table 3. Material parameters of carbon cloth reinforcement layerModulus/GPa Poisson’s ratio Shear modulus/GPa Strength/MPa Ex Ey Ez µxy µyz µxz Gxy Gyz Gzx 1250 50 50 8 0.055 0.35 0.35 4.3 3.5 3.5 锥柱一体化复合材料壳体结构为轴对称回转体结构形式，本文自研软件中采用轴对称四边形单元作为基本单元，其节点自由度只有两个(轴向位移+周向位移)，相比于三维实体单元，节点数少、计算快，其单元形式如图5(a)所示。考虑到壳体在承受内压载荷是存在较大的变形、几何非线性现象，在该轴对称单元中引入非线性应变位移关系[25]，以解决当前壳体受载中存在的小应变、大挠度和有限转角的问题。另外，考虑单元形状导致刚度奇异，致使单元存在沙漏模式和零能现象问题，引入人工沙漏刚度法[26]获得了的复合材料四边形单元刚度数值较为精确解，并使得所构建单元通过了patch测试[27]。
![]()
图 5 本文轴对称四边形单元：(a)位移模式；(b)沙漏模式Figure 5. Axisymmetric quadrilateral element: (a) Displacement mode; (b) Hourglass mode纤维纱束按照设定好的切点数、缠绕角度、缠绕顺序一层一层堆叠在芯模上，后续铺层依此规律逐层生成缠绕复材层的节点以及网格单元。而接头和绝热层采用Gmsh生成其对应的节点及单元号。环向缠绕层只存在于筒身区域，赤道圆处存在几何过度问题，几何建模中引入楔形处理方式实现缠绕层以及补强层的顺利过渡。在后处理方面，引入OpenGL开放图形库实现锥柱一体化复合材料壳体结构的前后处理过程可视化，使用彩虹图(Rainbow)以及RGB值进行可视化渲染得到壳体失效云图，最终全局的应力、位移、应变、损伤等参数的最大值、最小值显示。基于Hashin失效准则的损伤自定义变量SDV1~4分别表示基体拉伸、压缩，纤维拉伸、压缩损伤失效因子。
不考虑材料损伤折减时，未补强锥柱一体化复合材料壳体在35 MPa时其螺旋、环向缠绕层纤维方向应力云图及曲线如图7所示。可知，由于在直筒段的受力形式符合柱壳薄膜应力形式，其环向和轴向应力满足2∶1比例关系，且环向应力和螺旋向应力保持平稳。在锥柱交接区，由前述缠绕角度和厚度分布知其整体刚度发生变化，该处的径向位移协调由附加弯矩和附加剪应力贡献，发生弯曲变形时内外层纤维方向应力存在差异，且螺旋缠绕层内外层较环向缠绕层明显，说明此时轴向附加弯矩和剪应力较环向较大[4]。另外，由直筒段、锥段封头应力分布曲线可知，锥柱一体化复合材料壳体封头结构应力存在较大差异，且直筒段赤道圆附近应力最大最小值应力差大于锥段小端所在赤道圆。根据当前螺旋层强度参数数据，为研究筒身锥柱区域影响该锥柱一体化复合材料壳体直筒段封头需要进行补强。
![]()
图 6 螺旋和环向缠绕层、补强层结构收尾过渡形式Figure 6. Structure form at transition area of the helical, hoop and reinforcement layer![]()
图 7 锥柱一体化复合材料壳体螺旋和环向缠绕层纤维方向应力：(a)螺旋层；(b)环向层Figure 7. Stress in the fiber orientation of the cone-cylinder integrated composite case: (a) Stress distribution of the helical layer; (b) Stress distribution of the hoop layer2.2 锥柱一体化复合材料壳体渐进损伤失效演化分析
由上述分析可知，锥柱一体化复合材料壳体结构在两侧封头和中部锥柱交接处均存在应力突变现象，为探究其对复合材料层损伤演化过程的影响，以及最终导致结构件发生破坏性失效的机理，本文开展复合材料壳体渐进失效研究，基于Hashin失效准则追踪该异型结构件在承载过程中的损伤形成和扩展过程，以探究造成其破坏失效的内部机理。为了避免直筒段封头结构因变角度、变曲率、变厚度、变缺陷等结构特征导致附加应力，导致壳体出现低压破坏问题，本文采用碳布补强的工艺方式为该锥柱一体化复合材料壳体封头结构进行增强处理，以降低封头螺旋层应力损伤状态。根据上述研究可知，直筒段封头存在较大的应力，而锥段封头应力相对较小，因此本文采用前封头两层碳布补强，后封头不补强方式开展研究工作。补强后铺层方案为[±17.5°/90°/前封头全补1层/±17.5°/90°/前封头全补1层/±17.5°/90°3]。
考虑到弹性结构体在载荷作用下必然会产生弹性变形，为研究该锥柱一体化复合材料壳体结构在承载过程中的损伤失效过程，首先需要分析其在载荷下的变形过程，以了解其全局变形状态以及变形过程中在拉伸、弯曲等变形形式对应力重分布以及损伤的贡献权重。提取内压载荷下纤维方向应变(LE11)、垂直纤维方向应变(LE22)进行分析，提取各层应变数据如图8所示。可知，总体上看从15~32 MPa的打压过程中，纤维方向应变与垂直纤维方向应变均呈现整体平移形式，纤维方向应变中环向层与螺旋缠绕层间隔距离随载荷增加逐渐拉大，说明其承载过程中发生了载荷重分配现象，使得环向缠绕层工作环境逐渐恶化。由垂直纤维方向应变的变化过程可知，从15~30 MPa之间螺旋缠绕层和环向缠绕层应变数值间距在逐渐拉大，但是从30~32 MPa变化的过程中，其间距却减小了。从该过程前后可知，30 MPa时螺旋缠绕层已经接近变形破坏极限，32 MPa时螺旋缠绕层应变增量很小，而环向缠绕层应变却有一个较大的增加，表明此过程中，环向缠绕层发生了破坏，其2方向在当前30~32 MPa过程中获得了较大的应力重分配数值，也预示着该锥柱一体化复合材料壳体结构的损伤失效过程发生在该载荷作用带宽内，为后续结合Hashin失效准则研究其失效模式奠定基础。
![]()
图 8 锥柱一体化复合材料壳体不同载荷下纤维方向应变和垂直纤维方向应变结果(横坐标表示从左极孔到右极孔的轴向坐标，纵坐标LE11和LE22分别表示沿纤维方向和垂直纤维方向应变)Figure 8. Fiber directional and perpendicular fiber directional strain results of the cone-cylinder integrated composite case under different pressure loads (The horizontal axis represents the axis-directional coordinate from the left polar hole to the right polar hole, the vertical axis represents the fiber orientation and transverse fiber orientation strain)另外，螺旋层靠近极孔两侧垂直纤维方向应变相对较大，且纤维方向应变在15~32 MPa过程中也发生了较大幅度的增加，表明复合材料壳体结构容器在承受内压过程中，极孔处出现了一定程度的胀口现象。对比直筒段封头和锥段封头变形曲线以及图7所示未补强壳体应力分布可知，封头补强工艺会很大幅度的降低其应力水平，但同时也会使得复合材料结构最内、最外缠绕层在金属接头外圆附近存在较大的应力、应变差距。相比而言，锥段封头的内外层应力、应变水平较低，且整体较为平稳，说明锥段对壳体应力水平改善明显。
在上述研究结构整体随载荷增加作用下的纤维方向和垂直纤维方向应变单一变化规律后，还需研究其在轴向和环向双向变形作用下的失效过程，以明晰其内在变形之间的耦合作用机制。由于锥柱一体化复合材料壳体为轴对称回转体结构形式，其在承受内压载荷时主要表现为承受拉弯产生的正应力。因此，本文提取该锥柱一体化复合材料壳体结构在不同内压载荷下的纤维方向拉伸损伤和垂直纤维方向损伤因子结果如图9所示。由图可知，总体来看，载荷从15 MPa增加到32 MPa的过程中，SDV1、SDV3所表征的复合材料微元损伤程度逐渐增加。其损伤演化过程可以分为基体损伤、基体和纤维同步同时损伤、结构失效3个阶段。
![]()
图 9 锥柱一体化复合材料壳体不同载荷下渐进损伤演化结果(横坐标表示从左极孔到右极孔的轴向坐标，纵坐标SDV1和SDV3分别表示沿纤维方向和垂直纤维方向损伤因子)Figure 9. Progressive damage results of the cone-cylinder integrated composite case under different pressure loads (The horizontal axis represents the axis-directional coordinate from the left polar hole to the right polar hole, the vertical axis SDV1 and SDV3 represents the fiber orientation and transverse fiber orientation damage factor)随着载荷逐渐增加拉大了筒身区域螺旋缠绕层和环向缠绕层的SDV1间距，表明承载过程中随着垂直纤维方向的初始损伤形成，其单元应力发生重分配，使得环向缠绕层结构承担了更多的载荷，加速了环向缠绕层的损伤进程。与直筒结构的复合材料壳体不同，15~30 MPa过程中SDV3结果所示锥柱一体化复合材料壳体交接区的横向剪力、轴向弯矩对基体损伤的贡献作用逐渐明显，使得该处的基体拉伸损伤集中程度逐步加剧，最终使得该处结构发生沿轴向的撕裂并引发局部环向纤维断裂，该损伤模式与后续实验结果吻合。
3. 锥柱一体化复合材料壳体试验验证
由以上分析结果可知，锥柱一体化复合材料壳体结构在筒身锥柱交界处和直筒段赤道圆处存在应力突变现象，为探明损伤破坏模式与实际的差异，开展锥柱一体化复合材料壳体水压爆破试验，其破坏模式如图10所示，可知当前壳体主要破坏位置为锥柱交接处和直筒段赤道圆处，且靠近直筒段赤道圆处环向缠绕层的纤维断裂，锥柱交界处基体轴向开裂和部分环向缠绕层的断裂。
通常，复合材料壳体结构在的渐进失效过程会出现材料刚度降级，在筒身段出现径向位移突变现象，为此，本文通过获取直筒段径向位移随载荷变化参数，获得了仿真分析结构失效点。并结合上述得到的水压爆破试验爆破压强、爆破位置及失效模式，验证了仿真模型计算失效演化过程的正确性，计算结果对比如图11所示。可知，当前仿真计算爆破压强为32 MPa，与实际爆破压强30.4 MPa误差为5.3%，该误差在允许范围内，计算结果符合实际。
![]()
图 10 锥柱一体化复合材料壳体爆破试验过程及实验结果Figure 10. Experiment test result of the cone-cylinder integrated composite case![]()
图 11 锥柱一体化复合材料壳体径向位移与载荷的关系Figure 11. Radial displacement versus pressure load of cone-cylinder integrated composite case4. 结 论
针对传统固体火箭发动机壳体结构主要以直筒结构为主，对于新兴锥柱一体化复合材料壳体结构缠绕线型设计、受力分布、损伤失效过程及失效模式研究较少。为此，本文针对锥柱一体化复合材料壳体缠绕线型和损伤失效方面的研究相关结论如下：
(1) 与直筒身结构相比，锥柱一体化复合材料壳体结构缠绕线型从筒身到赤道圆呈现非线性特征，且随着锥段角度的增加其缠绕厚度和缠绕角度非线性现象逐渐明显；
(2) 与直筒形式相比锥柱交界处复合材料层结构存在横向剪力和弯矩，致使内外层存在应力差距，使得螺旋层层内外差距大于环向缠绕层。另外，锥角的存在降低了锥柱一体化复合材料壳体锥段小端的赤道圆及封头的应力水平，使得该封头部位相较于直筒段赤道圆和封头安全；
(3) 补强后壳体损伤主要集中在直筒段赤道圆、锥柱交接区、锥段赤道圆附近，且随压力增加锥柱交接区的基体在横向剪力和弯矩作用下出现拉伸破坏且集中程度逐渐加剧，加剧了该处应力分布状况，最终造成交接区和直筒段环向层纤维断裂。
-
![]()
图 1 两组超高分子量聚乙烯(UHMWPE)纤维平纹织物-单向(UD)布杂化结构示意图
Figure 1. Schematic diagram of two groups of hybrid structures with ultrahigh molecular weight polyethylene (UHMWPE) yarns woven fabrics and unidirectional (UD) laminates
A—Woven fabrics; B—UD laminates
![]()
图 2 纱线模型尺寸、方向定义与网格划分
Figure 2. Size of yarn model, definition of yarn directions, and mesh development
![]()
图 3 平纹织物模型尺寸、边缘条件设置和主区域和副区域定义
Figure 3. Size of plain woven fabric model, setting of boundary condition, and definition of primary and secondary areas
![]()
图 4 UD布模型尺寸和边缘条件设置
Figure 4. Dimension of UD laminate model and setting of boundary condition
![]()
图 6 单层UHMWPE纤维平纹织物能量吸收和第一根纱线断裂的实验和模拟数据对比
Figure 6. Energy absorption and time of first failed yarn of single UHMWPE fiber woven fabric from experiments and modelling
![]()
图 7 单层Dyneema@ SB51 UD布能量吸收和穿透时间的实验和模拟数据对比
Figure 7. Energy absorption and penetration time of single Dyneema@ SB51 UD fabric from experiments and modelling
![]()
图 9 两组UHMWPE纤维平纹织物-UD布混合板每层的内能吸收
Figure 9. Energy absorption of internal energy of each layer in the two hybrid panels with UHMWPE yarn woven fabrics and UD laminates
![]()
图 10 两组UHMWPE纤维平纹织物-UD布混合板前两层主区域和副区域的内能吸收
Figure 10. Internal energy of primary area and secondary area of the first two layers in the two hybrid panels with UHMWPE yarn woven fabrics and UD laminates
![]()
图 11 在1×10−6 s时AAABB中第一层与BBAAA中第一层所受拉应力分散图
Figure 11. Contours of tensile stress distributions on the first layer of AAABB and BBAAA at 1×10−6 s
![]()
图 12 两组UHMWPE纤维平纹织物-UD布混合板后两层主区域和副区域的内能吸收
Figure 12. Internal energy of primary and secondary areas of the first two layers in the two hybrid panels with UHMWPE yarn woven fabrics and UD laminates
![]()
图 13 两组UHMWPE纤维平纹织物-UD布混合板中每层平纹织物层的内能吸收
Figure 13. Internal energy of each layer in the two hybrid panels with UHMWPE yarn woven fabrics and UD laminates
![]()
图 14 两组UHMWPE纤维平纹织物-UD布混合板中的平纹织物Mises应力分散图
Figure 14. Contours of Mises stress distributions of woven fabrics in the two hybrid panels with UHMWPE yarn woven fabrics and UD laminates
表 1 UHMWPE纱线模型弹性性质
Table 1 Elastic properties of UHMWPE yarn in modelling
E1/MPa E2/MPa E3/MPa ν12 ν13 ν23 G12/MPa G13/MPa G23/MPa 88000 1210 1210 0.2 0.2 0.4 370 370 1500 Notes: E1, E2, E3—Young’s modulus in the 1, 2, 3-directions of the UHMWPE yarn model, respectively; ν12—Poisson’s ratio that characterizes the transverse strain in the 2-direction, when the yarn model is stressed in the 1-direction; ν13—Poisson’s ratio that characterizes the transverse strain in the 3-direction, when the yarn model is stressed in the 1-direction; ν23—Poisson’s ratio that characterizes the transverse strain in the 3-direction, when the yarn model is stressed in the 2-direction; G12, G13, G23—Shear modulus in 12, 13, 23-directions of the yarn model. 
下载: 导出CSV
表 2 UD布模型弹性性质
Table 2 Elastic properties of a UD ply
E1/MPa E2/MPa E3/MPa ν12 ν13 ν23 G12/MPa G13/MPa G23/MPa 110000 3600 3600 0.07 0.07 0.07 420 420 420
下载: 导出CSV
表 3 粘结层的弹性性质
Table 3 Elastic properties of the adhesive ply
E/Enn/MPa G1/Ess/MPa G2/Ett/MPa 850 850 850 Notes: E/Enn—Nominal traction in the normal direction; G1/Ess and G2/Ett—Nominal tractions in the two local shear directions.
下载: 导出CSV
-
[1] CHEESEMAN B A, BOGETTI T A. Ballistic impact into fabric and compliant composite laminates[J]. Composite Structures,2003,61(1-2):161-173. DOI: 10.1016/S0263-8223(03)00029-1
[2] 顾伯洪. 织物弹道贯穿性能分析计算[J]. 复合材料学报, 2002, 19(6):92-96. DOI: 10.3321/j.issn:1000-3851.2002.06.017 GU Bohong. Analytical modelling of woven fabric under ballistic perforation[J]. Acta Materiae Compositae Sinica,2002,19(6):92-96(in Chinese). DOI: 10.3321/j.issn:1000-3851.2002.06.017
[3] 朱德举, 彭恋. SiC-超高分子量聚乙烯仿生柔性叠层结构防弹性能关键影响因素的仿真与试验[J]. 复合材料学报, 2020, 37(11):2928-2940. ZHU Deju, PENG Lian. Simulation and experiment of key influencing factors on ballistic performance of SiC-ultra-high molecular weight polyethylene biomimetic flexible laminated structure[J]. Acta Materiae Compositae Sinica,2020,37(11):2928-2940(in Chinese).
[4] GU B. Analytical modeling for the ballistic perforation of planar plain-woven fabric target by projectile[J]. Compo-sites Part B: Engineering,2003,34(4):361-371. DOI: 10.1016/S1359-8368(02)00137-3
[5] 何业茂, 焦亚男, 周庆, 等. 弹道防护用先进复合材料弹道响应的研究进展[J]. 复合材料学报, 2021, 38(5):1331-1348. HE Yemao, JIAO Ya'nan, ZHOU Qing, et al. Research progress on ballistic response of advanced composite for ballistic protection[J]. Acta Materiae Compositae Sinica,2021,38(5):1331-1348(in Chinese).
[6] 陈晓钢. 纺织基防弹防穿刺材料的研究回顾[J]. 纺织学报, 2019, 40(6):158-164. CHEN Xiaogang. Trend of research in textile-based protective materials against ballistic and stabbing[J]. Journal of Textile Research,2019,40(6):158-164(in Chinese).
[7] 周熠, 陈晓钢, 张尚勇, 等. 超高分子量聚乙烯平纹织物在柔性防弹服中的应用[J]. 纺织学报, 2016, 37(4):60-64. ZHOU Yi, CHEN Xiaogang, ZHANG Shangyong, et al. Application of ultra-high molecular-weight polyethylene plain weave on soft body armour[J]. Journal of Textile Research,2016,37(4):60-64(in Chinese).
[8] TABIEI A, NILAKANTAN G. Ballistic impact of dry woven fabric composites: A Review[J]. Applied Mechanics Reviews,2008,61(1):1-13.
[9] NILAKANTAN G, GILLESPIE J W. Ballistic impact modeling of woven fabrics considering yarn strength, friction, projectile impact location, and fabric boundary condition effects[J]. Composite Structures,2012,94(12):3624-3634. DOI: 10.1016/j.compstruct.2012.05.030
[10] CHEN X, ZHU F, WELL G. An analytical model for ballistic impact on textile based body armour[J]. Composites Part B: Engineering,2013,45(1):1508-1514. DOI: 10.1016/j.compositesb.2012.08.005
[11] YANG Y, CHEN X. Study of energy absorption and failure modes of constituent layers in body armour panels[J]. Composites Part B: Engineering,2016,98:250-259. DOI: 10.1016/j.compositesb.2016.04.071
[12] YANG Y, CHEN X. Determination of materials for hybrid design of 3D soft body armour panels[J]. Applied Compo-site Materials,2018,25(4):861-875. DOI: 10.1007/s10443-018-9716-y
[13] CHEN X, ZHOU Y, WELL G. Numerical and experimental investigations into ballistic performance of hybrid fabric panels[J]. Composites Part B: Engineering,2014,58:35-42. DOI: 10.1016/j.compositesb.2013.10.019
[14] BAJYA M, MAJUMDAR A, BUTOLA B, et al. Ballistic performance and failure modes of woven and unidirectional fabric based soft armour panels[J]. Composite Structures,2021,255:112941. DOI: 10.1016/j.compstruct.2020.112941
[15] GUO Z, CHEN W. A merit parameter to determine the stacking order of heterogeneous diphasic soft armor systems[J]. Composite Structures,2020,241:112086. DOI: 10.1016/j.compstruct.2020.112086
[16] WANG Y, CHEN X, YOUNG R, et al. An experimental study of the effect of ply orientation on ballistic impact perfor-mance of multi-ply fabric panels[J]. Textile Research Jour-nal,2016,86(1):34-43. DOI: 10.1177/0040517514566110
[17] LU Z, YUAN Z, CHEN X, et al. Evaluation of ballistic performance of STF impregnated fabrics under high velocity impact[J]. Composite Structures,2019,227:111208. DOI: 10.1016/j.compstruct.2019.111208
[18] YUAN Z, CHEN X, ZENG H, et al. Identification of the elastic constant values for numerical simulation of high velocity impact on dyneema® woven fabrics using orthogonal experiments[J]. Composite Structures,2018,204:178-191. DOI: 10.1016/j.compstruct.2018.07.024
[19] WANG Y, CHEN X, YOUNG R, et al. A numerical and experimental analysis of the influence of crimp on ballistic impact response of woven fabrics[J]. Composite Structures,2016,140:44-52. DOI: 10.1016/j.compstruct.2015.12.055
[20] HUANG W, WANG Y, XIA Y. Statistical dynamic tensile strength of UHMWPE-fibers[J]. Polymer,2004,45(11):3729-3734. DOI: 10.1016/j.polymer.2004.03.062
[21] PISANOVA E, ZHANDAROV S, MÄDER E. How can adhesion be determined from micromechanical tests?[J]. Composites Part A: Applied Science and Manufacturing,2001,32(3):425-434.
[22] GRUJICIC M, ARAKERE G, HE T, et al. A ballistic material model for cross-plied unidirectional ultra-high molecular-weight polyethylene fiber-reinforced armor-grade compo-sites[J]. Materials Science & Engineering A,2008,498(1-2):231-241.
[23] YUAN Z, WANG K, QIU J, et al. A numerical study on the mechanisms of Dyneema® quasi-isotropic woven panels under ballistic impact[J]. Composite Structures,2020,236:111855. DOI: 10.1016/j.compstruct.2020.111855
[24] MIN S, CHU Y, CHEN X. Numerical study on mechanisms of angle-plied panels for ballistic protection[J]. Materials & Design,2016,90:896-905.
[25] VAN DINGENEN J L J. High performance dyneema fibres in composites[J]. Materials & Design,1989,10(2):101-104.
[26] ONG CW, BOEY CW, HIXSON R S, et al. Advanced layered personnel armor[J]. International Journal of Impact Engineering,2011,38(5):369-383. DOI: 10.1016/j.ijimpeng.2010.12.003
[27] ATTWOOD J P, FLECK N A, WADLEY H N G, et al. The compressive response of ultra-high molecular weight polyethylene fibres and composites[J]. International Journal of Solids and Structures,2015,71:141-155. DOI: 10.1016/j.ijsolstr.2015.06.015
-
计量
- 文章访问数: 1401
- HTML全文浏览量: 690
- PDF下载量: 163
