Functional element topology optimization method based on multiple evaluation points for metamaterial design with zero Poisson’s ratio
-
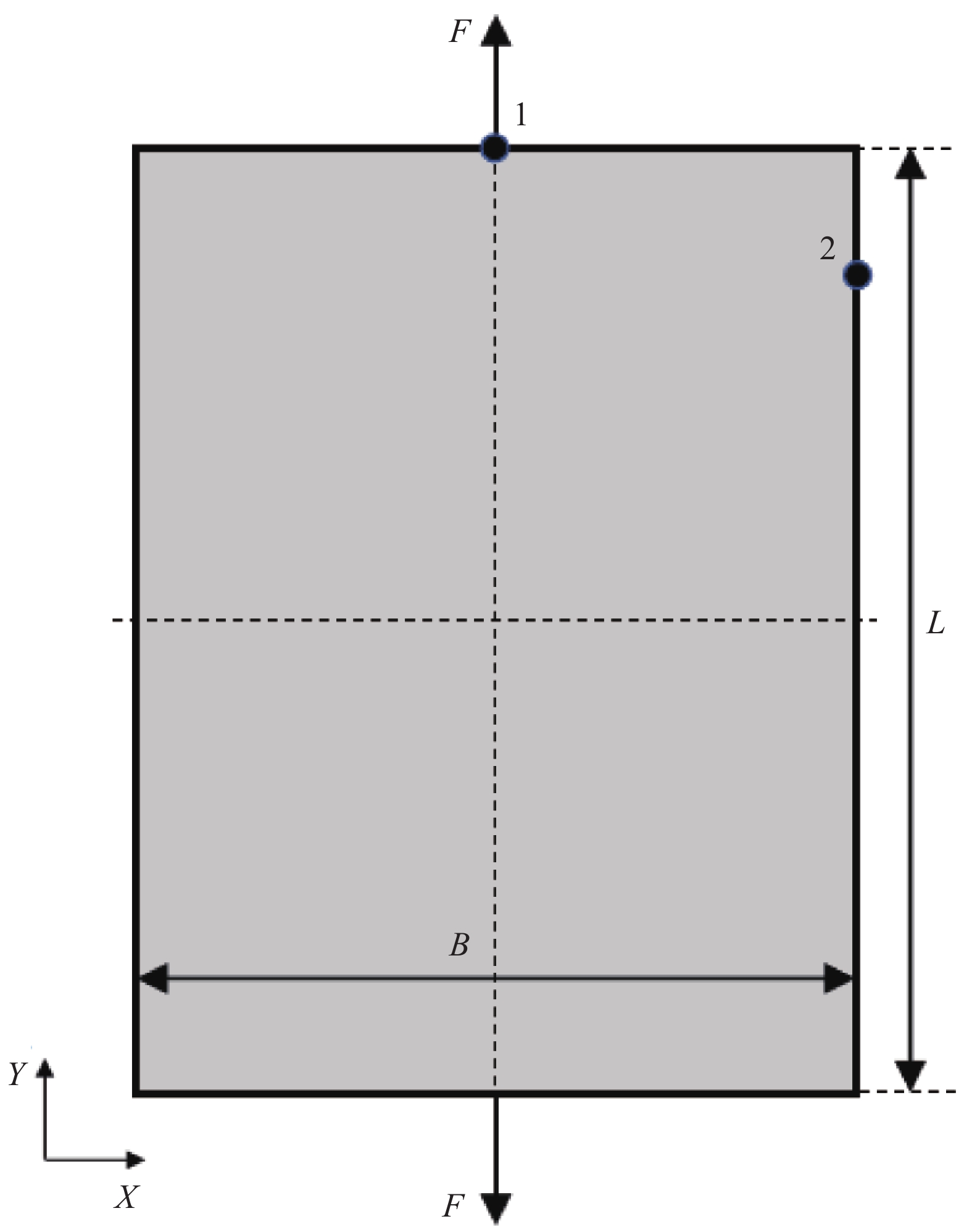
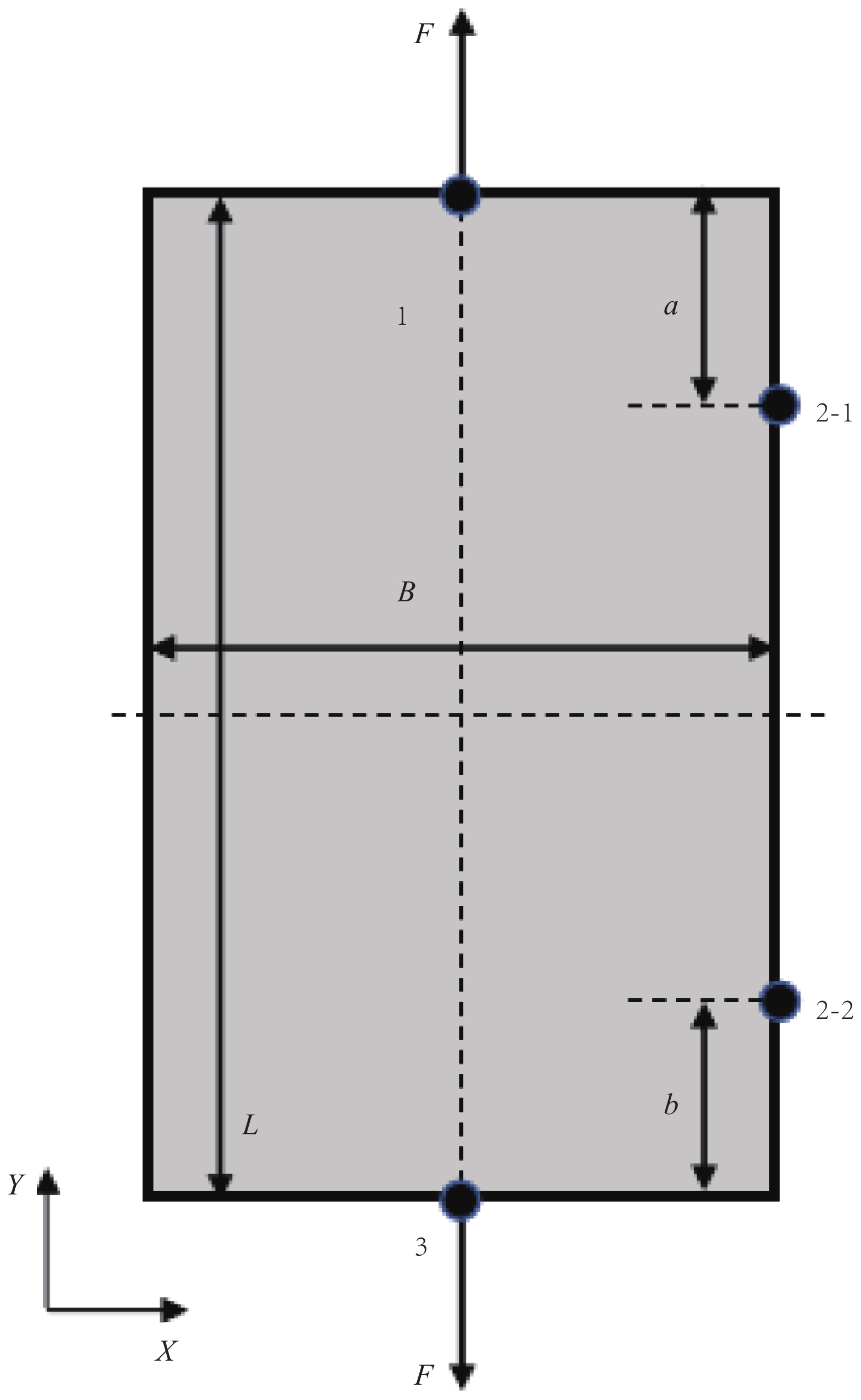
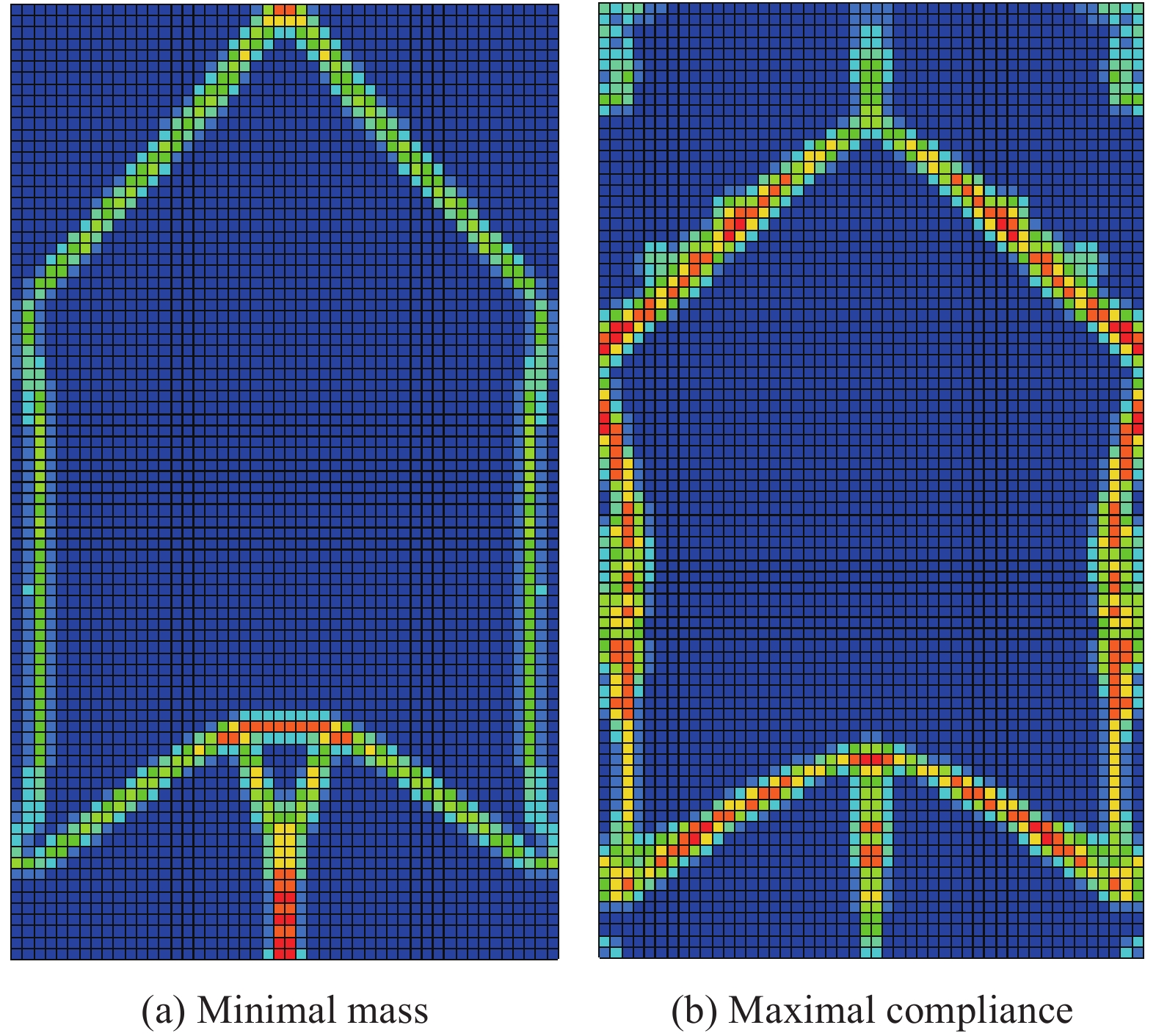
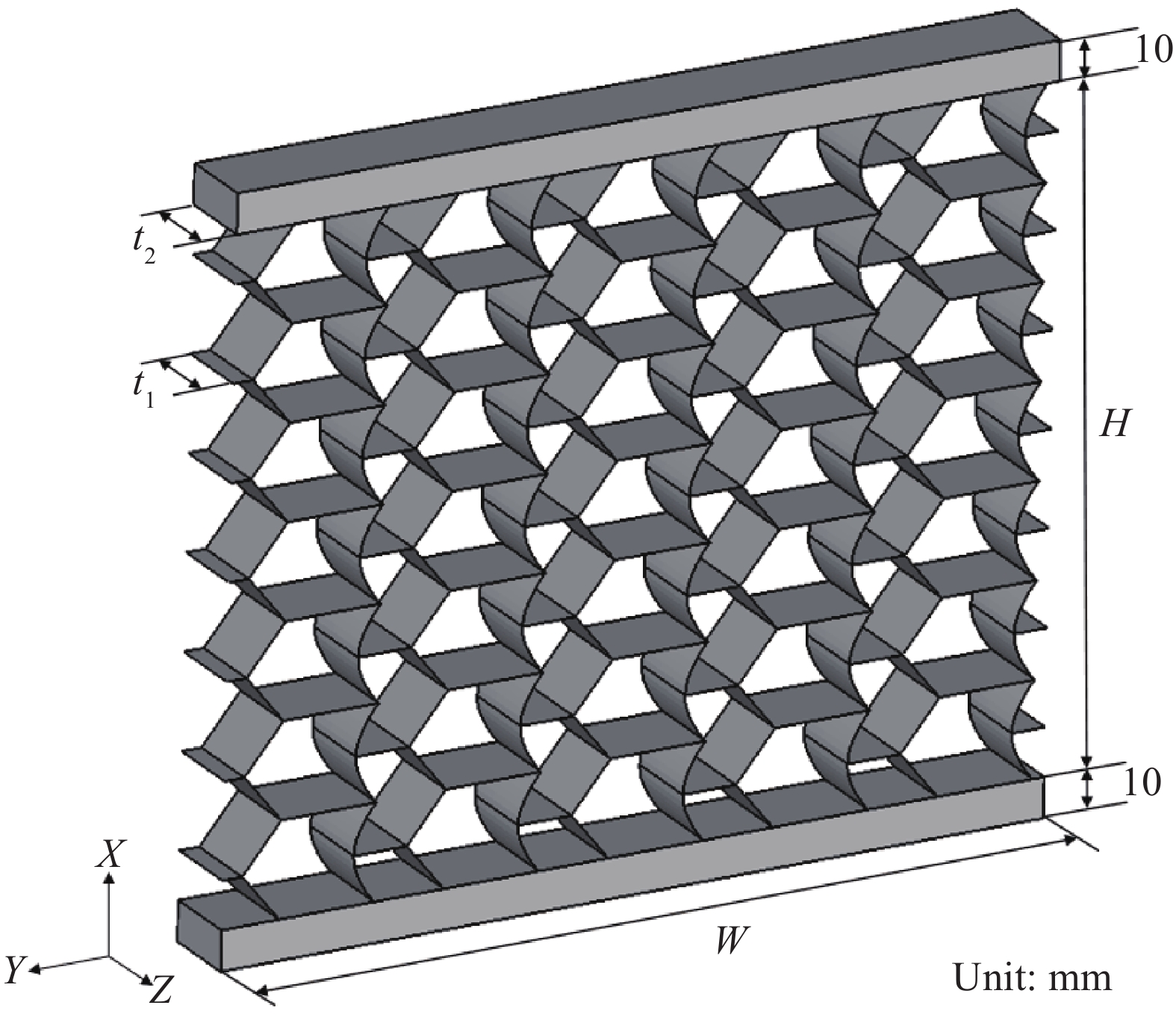
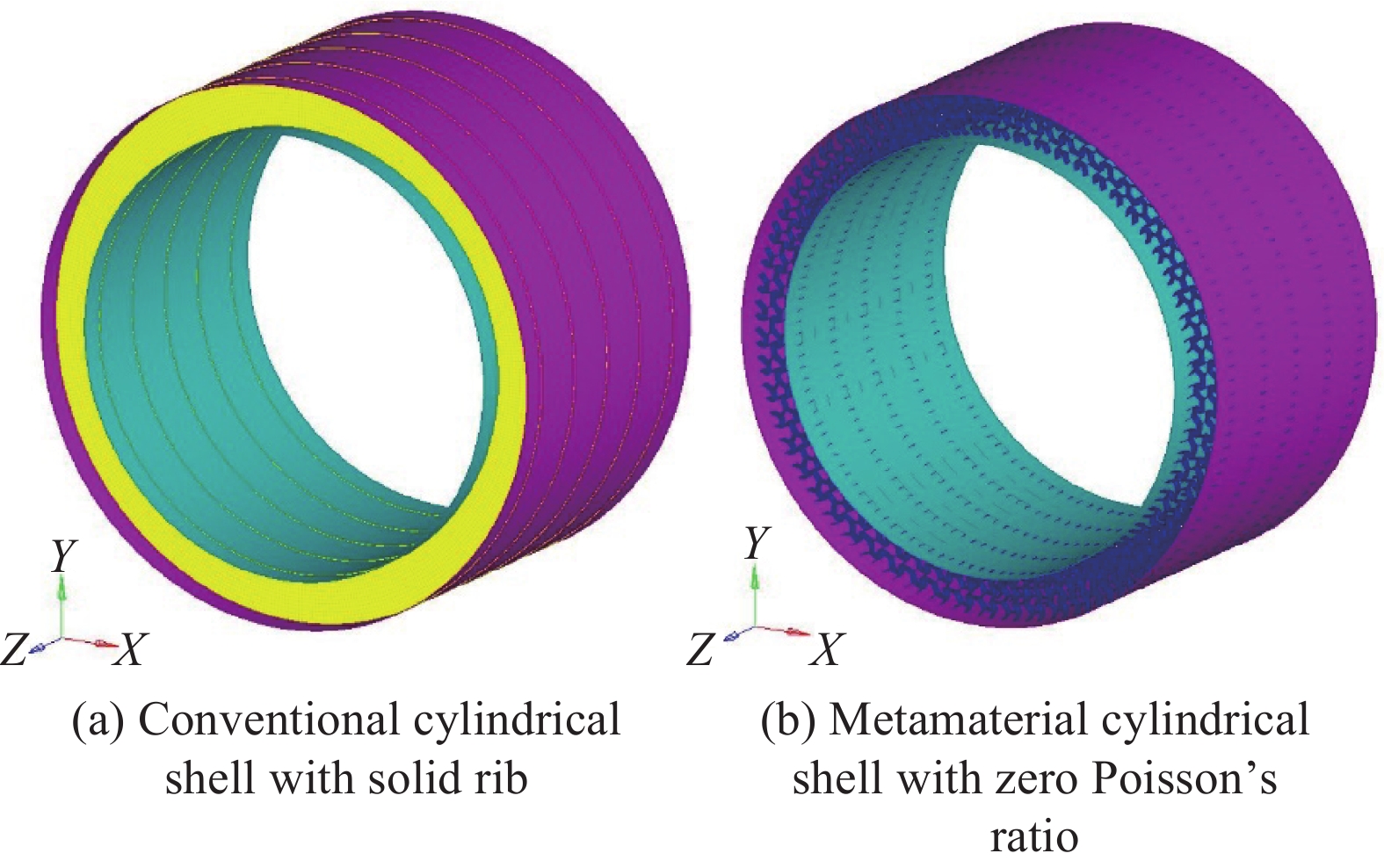
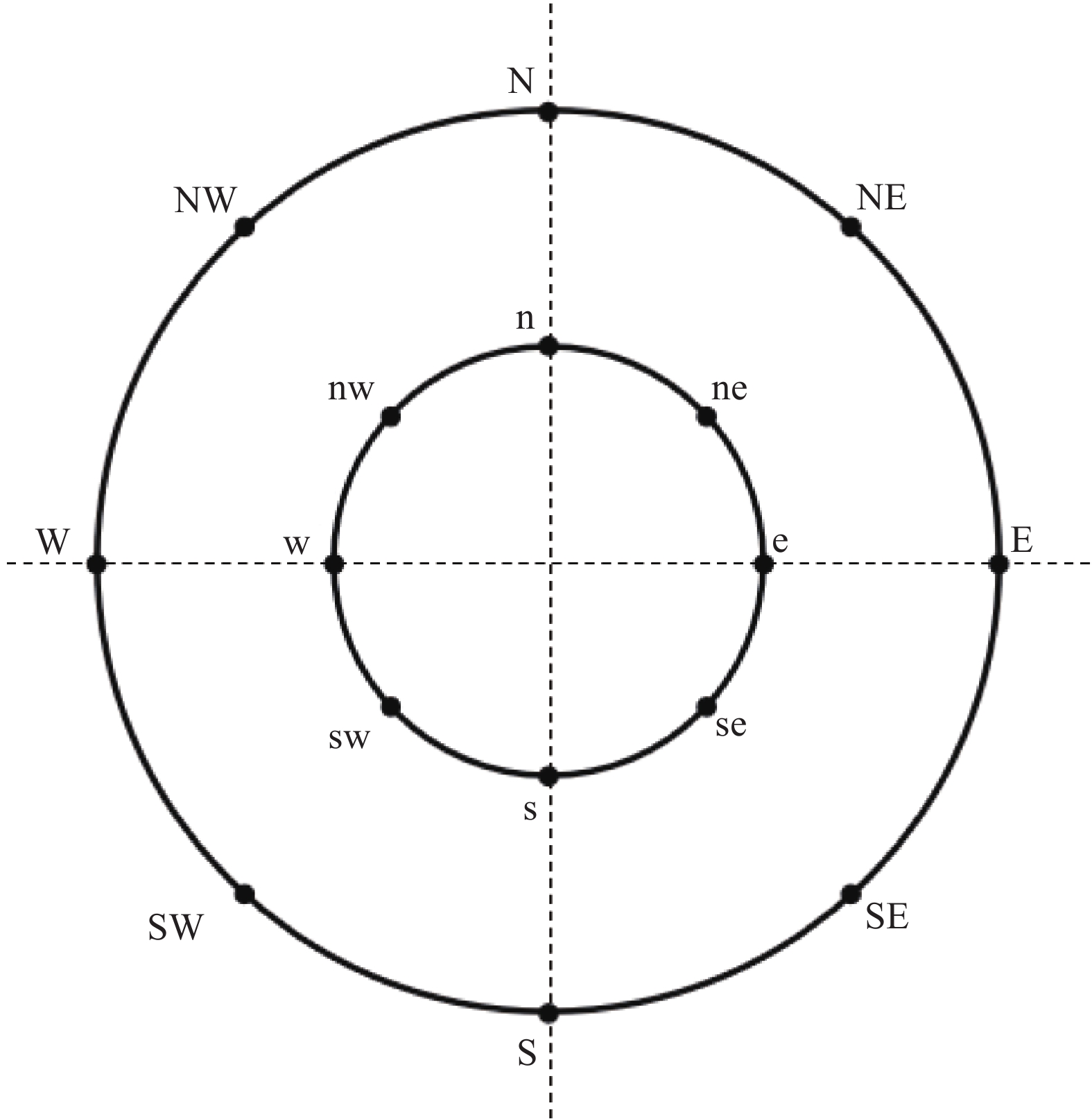
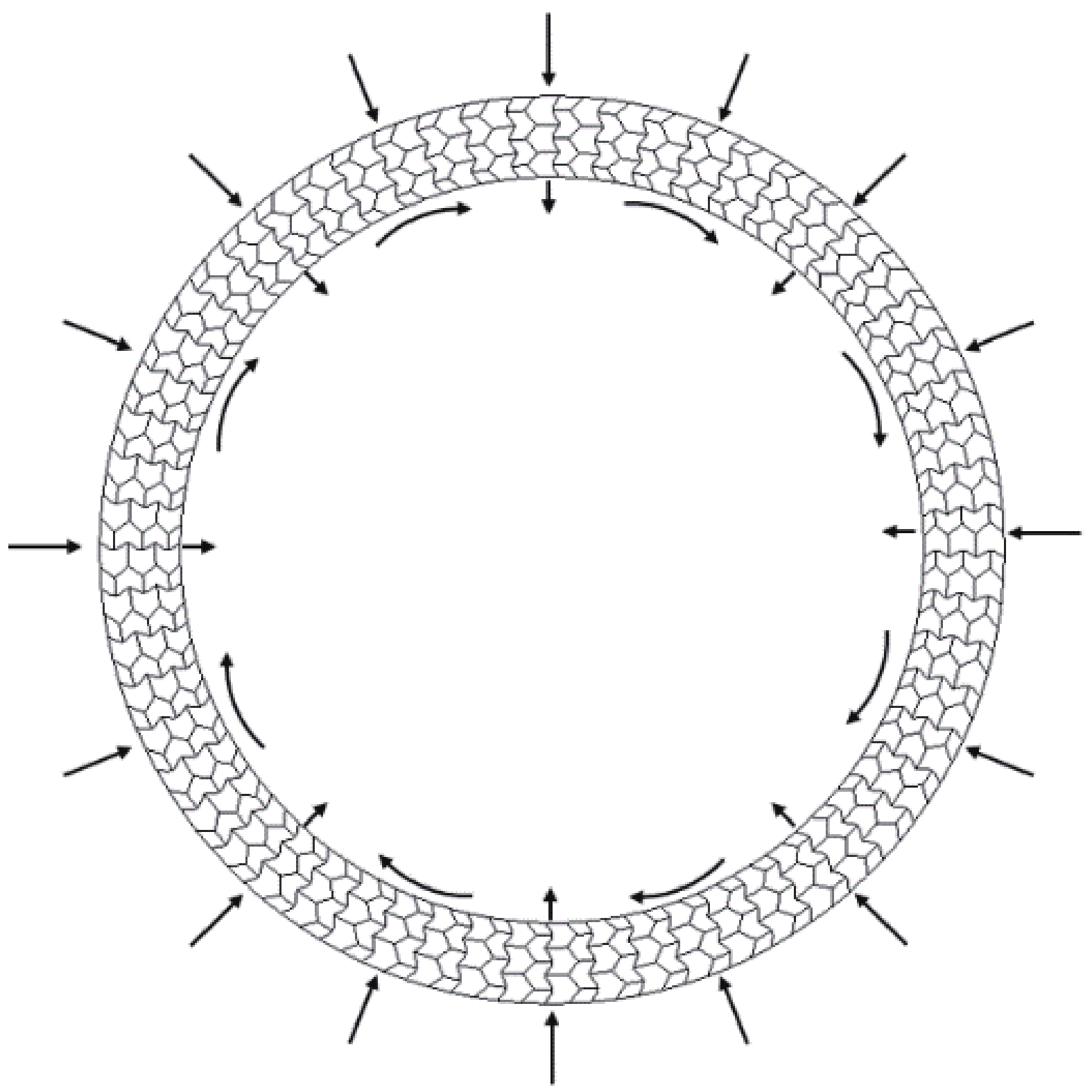
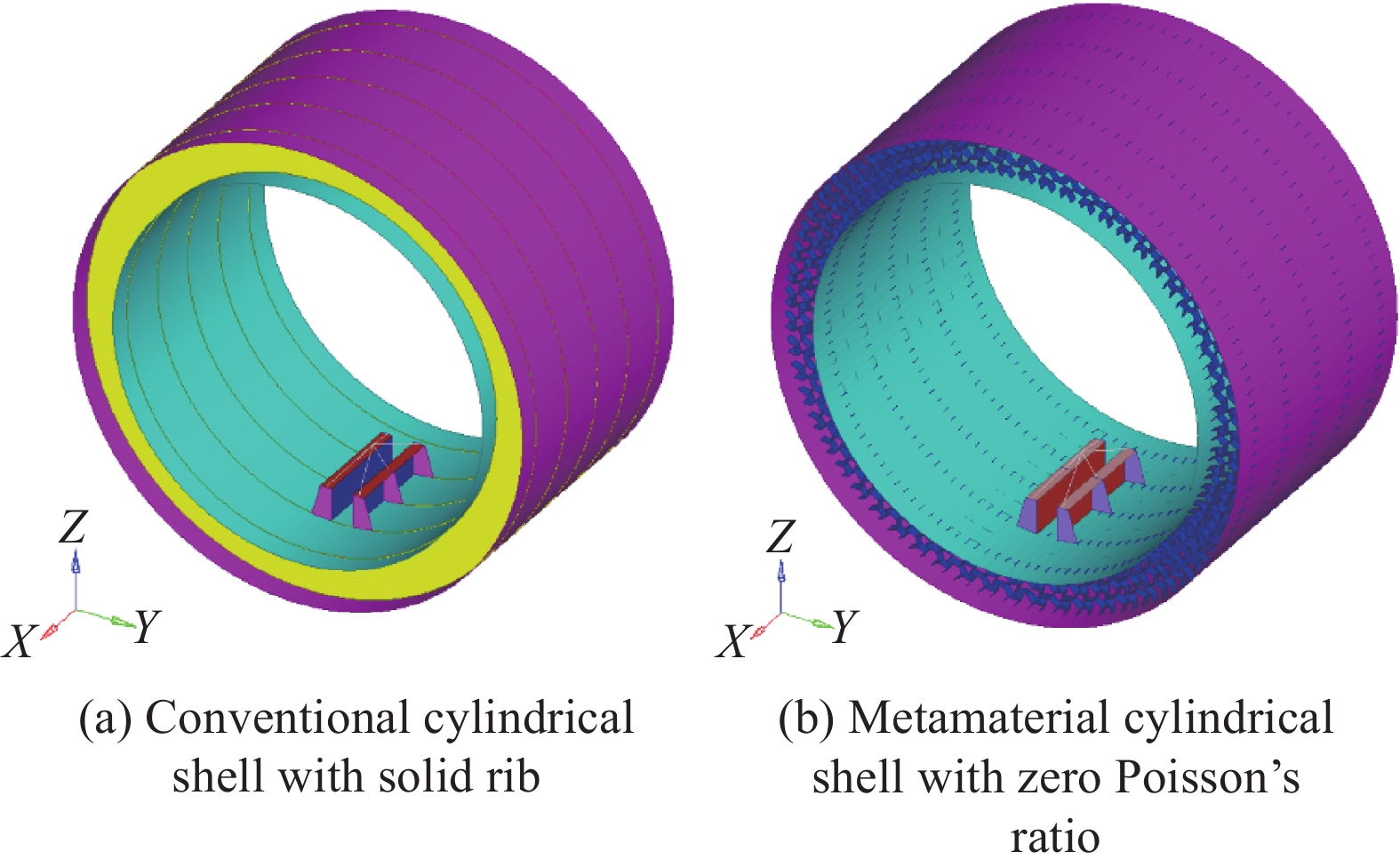
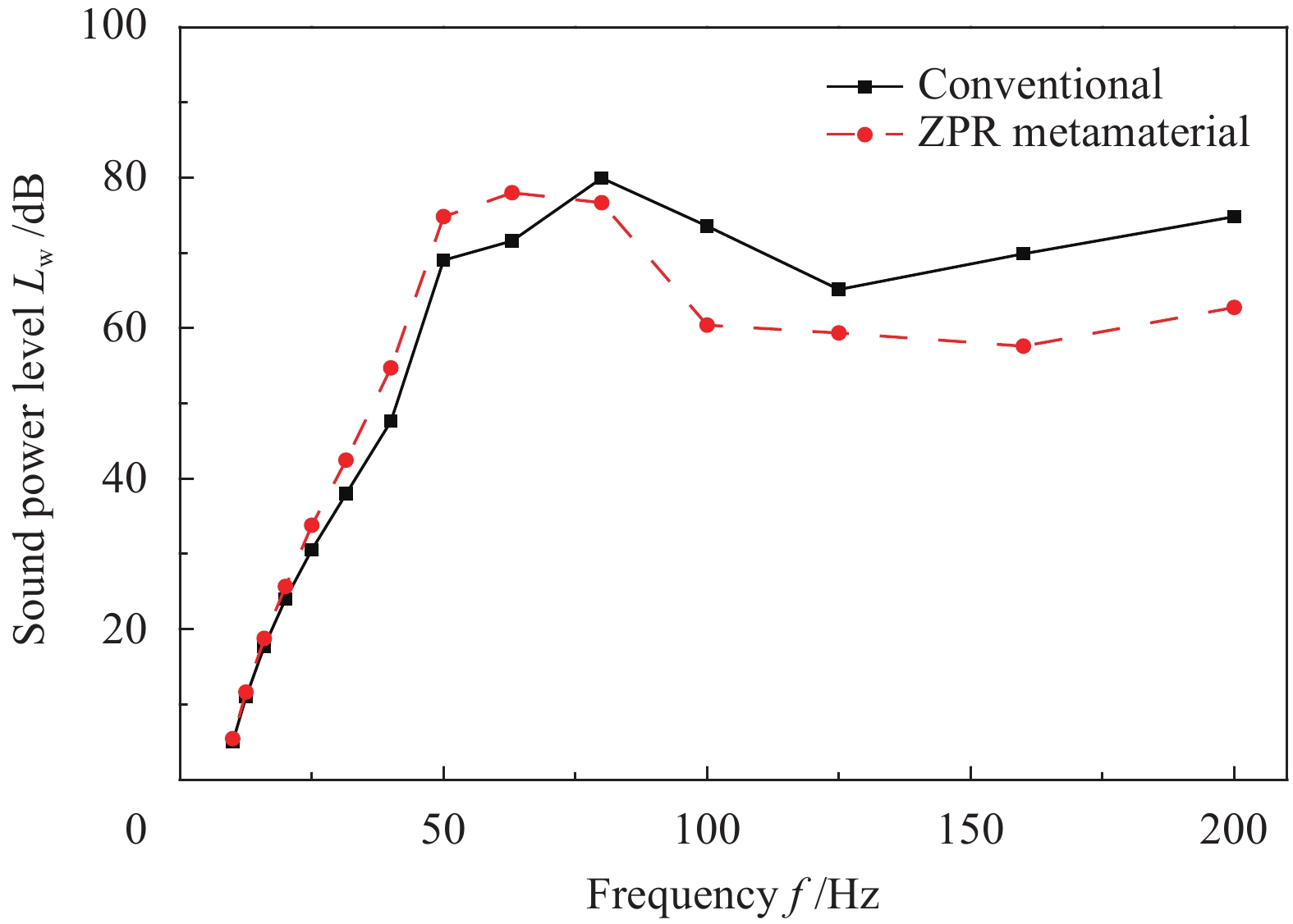
摘要: 提出基于多评价点约束的零泊松比超材料功能基元拓扑优化设计方法。在同一功能基元拓扑基结构中，通过建立对于多个评价点的正、负泊松比约束，实现胞元零泊松比效应。分别采用最小质量和最大柔度目标函数拓扑优化模型优化设计出与半内六角蜂窝相似的零泊松比功能基元最优拓扑构型。提取功能基元最优构型并周期性序构了零泊松比超材料试件，通过有限元方法验证了该功能基元的零泊松比效应，并分析超材料试件的静、动力学特性。计算结果表明，最大柔度目标函数设计的功能基元构型的泊松比更接近于零，且具有更好的承载与隔振性能。设计了零泊松比超材料环肋双层圆柱壳结构，进行外壳静压和内部设备激振下壳体水下辐射噪声分析。研究表明，零泊松比超材料环肋可将外壳压缩变形转换为内外壳间环肋旋转，实现耐压壳内壳的保形，且具有较好的降噪性能。Abstract: The functional element topology optimization method based on multiple evaluation points was proposed to design metamaterial with zero Poisson’s ratio. The zero Poisson’s ratio effect of a cell was achieved through multiple evaluation points that defined positive and negative Poisson’s ratio constraints in one topological ground structure. Topology optimization models were established by minimal mass and maximal compliance objective functions, and corresponding functional element configurations with zero Poisson’s ratio were optimized and designed, which were similar to semi re-entrant hexagonal honeycomb. The optimal functional element configurations were extracted and periodic arranged as metamaterial structure with zero Poisson’s ratio. The finite element method (FEM) was used to verify the Poisson’s ratio of these functional elements. The static and dynamic characteristics of metamaterial structures were also analyzed through finite element models. The results show that the metamaterial structure based on maximal compliance objective has better in-plane specific stiffness and vibration isolation performance, and its Poisson’s ratio is closer to zero compared with minimal mass objective model. The structures of double-layered cylindrical shells with conventional solid ring-rib and zero Poisson’s ratio metamaterial ring-rib were designed, and the analysis under static pressure from outer shell and underwater radiation noise caused by internal equipment were conducted. By converting the compression deformation of the outer shell into the rotation of the inner shell, the zero Poisson’s ratio metamaterial ring-rib achieves shape conservation of the inner shell. The metamaterial ring-rib can also reduce the underwater radiation noise from cylindrical shell.
-
图 1 不同混合型零泊松比（ZPR）蜂窝及其周期微元
Figure 1. Different periodic elements of hybrid honeycombs with zero Poisson’s ratio (ZPR)
图 2 超材料设计的功能基元拓扑优化(FETO)法示意图
Figure 2. Schematic of the functional element topology optimization (FETO) method of metamaterial design
图 3 任意正/负泊松比超材料设计的矩形拓扑基结构
Figure 3. Rectangular topological ground structure of metamaterial with arbitrary positive/negative Poisson’s ratio
图 5 不同目标函数下ZPR功能基元构型
Figure 5. Configurations of functional element with ZPR in different objective functions
图 9 ZPR超材料结构振动评价点位置
Figure 9. Position of evaluation points for calculating vibration of metamaterial structure with ZPR
图 10 不同ZPR超材料结构的频响曲线
Figure 10. Frequency response curves of metamaterial structure with ZPR in different finite element models
图 11 不同ZPR超材料结构的加速度振级落差
Figure 11. Acceleration vibration level difference of metamaterial structure with ZPR in different finite element models
图 12 ZPR超材料环肋圆柱壳
Figure 12. Ring rib of metamaterial structure with ZPR in cylindrical shell
图 14 常规圆柱壳与ZPR超材料圆柱壳
Figure 14. Conventional cylindrical shell and metamaterial cylindrical shell with ZPR
图 15 双层圆柱壳静力学评价点位置
Figure 15. Position of evaluation points of double cylindrical shell in static analysis
图 16 不同双层圆柱壳的应力分布
Figure 16. Stress distribution of different double cylindrical shells
图 17 ZPR超材料圆柱壳静压变形示意图
Figure 17. Schematic of displacement in metamaterial cylindrical shell with ZPR under static pressure
图 18 包含基座的双层圆柱壳FEM模型
Figure 18. FEM model of double cylindrical shell with base structure
图 19 双层圆柱壳声学边界元模型
Figure 19. Acoustic boundary element model of double cylindrical shell
图 20 不同圆柱壳的水下辐射声功率级
Figure 20. Underwater radiation noise power level of different cylindrical shells
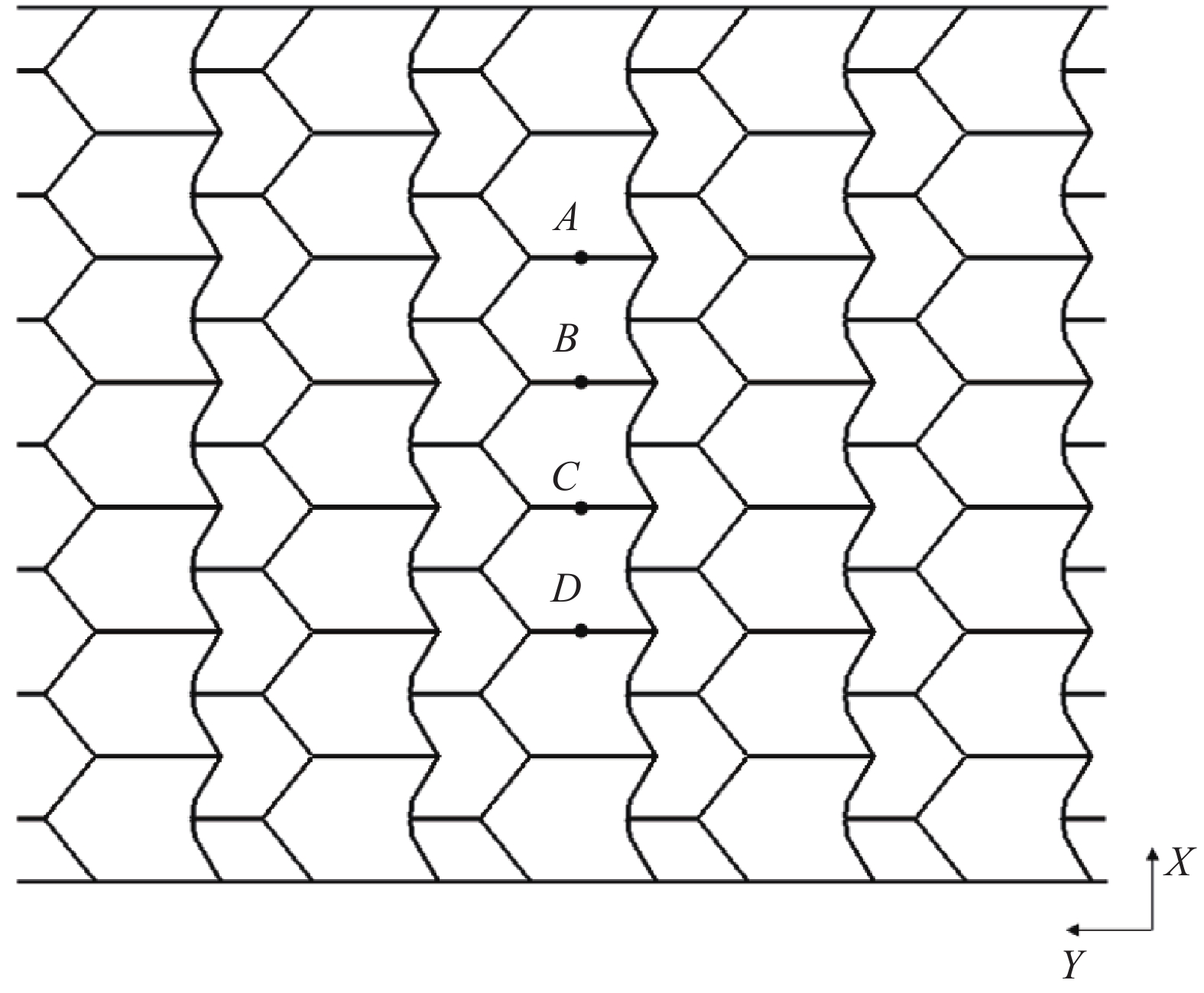
表 1 ZPR功能基元的验证
Table 1. Verification of functional element with ZPR
FEM model Objective function Orientation Strain Poisson’s ratio Beam element Minimal mass X −1.17×10−4 0.091 Y 1.06×10−5 Maximal compliance X −7.84×10−5 −0.0091 Y −7.14×10−7 Shell element Minimal mass X −1.07×10−5 0.095 Y 1.01×10−6 Maximal compliance X −6.95×10−6 −0.012 Y −8.53×10−8 Note: FEM—Finite element model. 
下载: 导出CSV
表 2 ZPR超材料结构宏观等效弹性模量和面内比模量
Table 2. Macroscopic equivalent elastic modulus and in-plane specific stiffness of metamaterial structures with ZPR
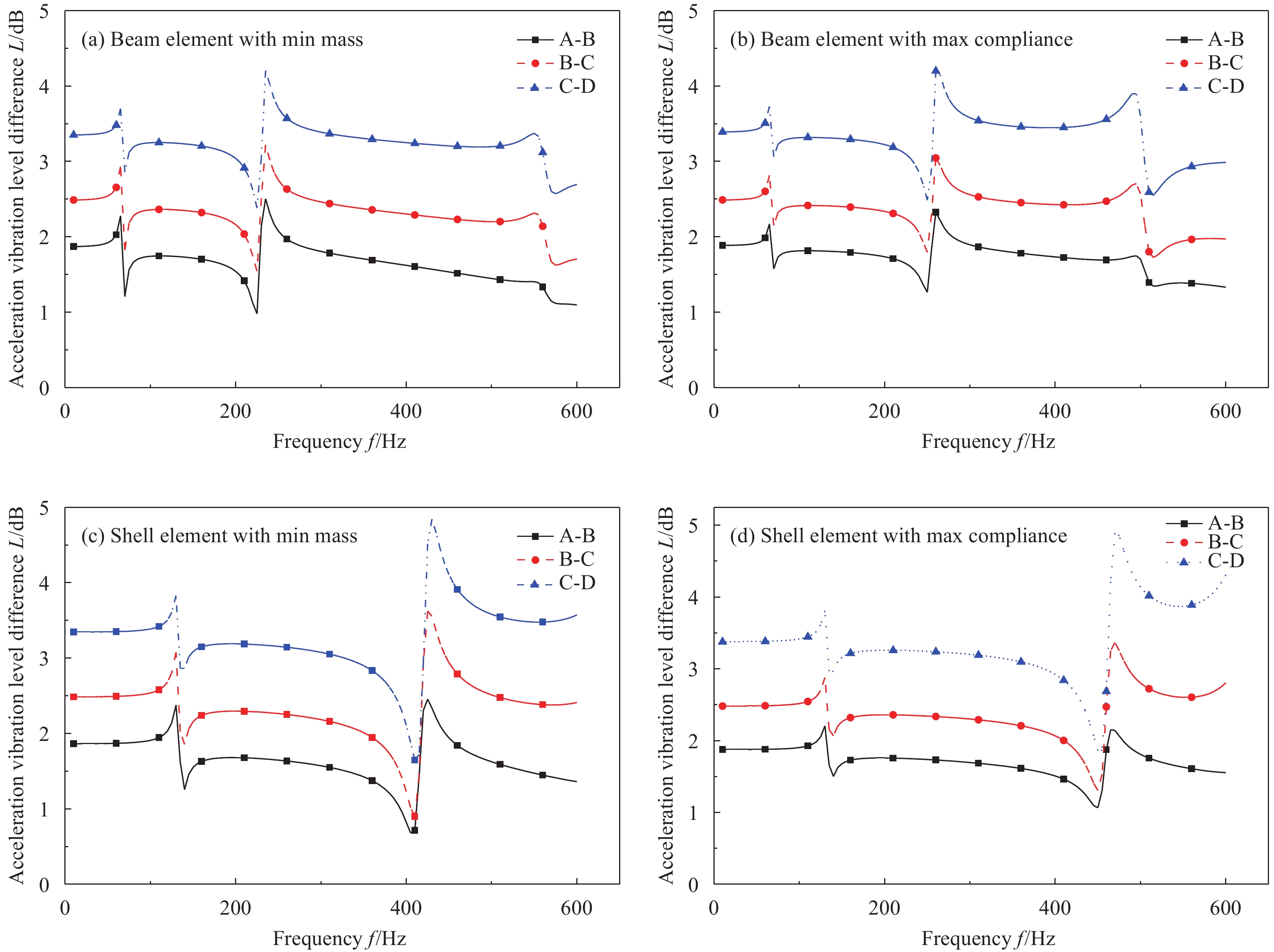
FEM model Objective function P/N △X/mm H/mm W/mm t1/mm A/mm2 Ee/MPa Ks/(m2·s−2) Beam element Minimal mass 106 0.37 168 210 2 420 115.53 146 117.42 Maximal compliance 106 0.27 168 210 2 420 158.21 220 660.68 Shell element Minimal mass 105 0.033 168 210 20 4 200 126.13 159 517.11 Maximal compliance 106 0.024 168 210 20 4 200 178.90 249 129.51 Notes：P—Load amplitude; △X—Overall deformation of metamaterial structure; H—Height of metamaterial structure; W—Width of metamaterial structure; t1—Thickness of metamaterial structure; A—Area of the plane perpendicular to the load in metamaterial structure; Ee and Ks—Macroscopic equivalent elastic modulus and in-plane specific stiffness of metamaterial structures.
下载: 导出CSV
表 3 ZPR超材料结构的总加速度振级落差
Table 3. Total acceleration VLD of metamaterial structure with ZPR
FEM model Objective function Evaluation points Acceleration VLD/dB Beam element Minimal mass A-B 1.60 B-C 2.21 C-D 3.09 Maximal compliance A-B 1.70 B-C 2.30 C-D 3.18 Shell element Minimal mass A-B 1.42 B-C 2.00 C-D 2.85 Maximal compliance A-B 1.74 B-C 2.60 C-D 3.66 Note: VLD—Vibration level difference.
下载: 导出CSV
表 4 不同双层圆柱壳的径向位移
Table 4. Radial displacement of different double cylindrical shells
Evaluation point Conventional cylindrical shell Metamaterial cylindrical shell Inner shell/mm Outer shell/mm In-out ratio Inner shell/mm Outer shell/mm In-out ratio N 1.58 1.73 0.913 0.885 2.69 0.329 NE 1.58 1.73 0.913 0.817 2.73 0.300 E 1.59 1.74 0.914 0.889 2.69 0.330 SE 1.56 1.71 0.913 0.740 2.69 0.275 S 1.57 1.72 0.908 0.841 2.65 0.318 SW 1.54 1.69 0.913 0.755 2.64 0.286 W 1.56 1.72 0.908 0.842 2.65 0.318 NW 1.56 1.70 0.913 0.782 2.69 0.291 Average 1.57 1.72 0.912 0.819 2.68 0.306
下载: 导出CSV
表 5 不同双层圆柱壳的内壳周向位移
Table 5. Circumferential displacement of inner shell in different double cylindrical shells
Evaluation point Conventional cylindrical shell/mm Metamaterial cylindrical shell/mm N 4.38×10−3 −0.193 NE 4.26×10−3 −0.218 E 2.64×10−3 −0.241 SE −1.12×10−3 −0.253 S −9.50×10−4 −0.245 SW −1.50×10−3 −0.225 W 2.64×10−4 −0.197 NW 4.69×10−3 −0.187 Average 1.58×10−3 −0.220 Ratio to radial displacement 1.01×10−3 0.269 Note：Positive and negative values in the table mean counterclockwise and clockwise circumferential displacements respectively.
下载: 导出CSV
-
[1] 金忠谋. 材料力学[M]. 北京: 机械工业出版社, 2012.JIN Zhongmou. Mechanics of materials[M]. Beijing: China Machine Press, 2012(in Chinese). [2] 程文杰, 周丽, 张平, 等. 零泊松比十字形混合蜂窝设计分析及其在柔性蒙皮中的应用[J]. 航空学报, 2015, 36(2):680-690.CHENG Wenjie, ZHOU Li, ZHANG Ping, et al. Design and analysis of a zero Poisson’s ratio mixed cruciform honeycomb and its application in flexible skin[J]. Acta Aeronautica et Astronautica Sinica,2015,36(2):680-690(in Chinese). [3] ALDERSON K L, ALDERSON A, SMART G, et al. Auxetic polypropylene fibres Part 1-Manufacture and characterisation[J]. Plastics Rubber and Composites,2002,31(8):344-349. doi: 10.1179/146580102225006495 [4] EVANS K E, NKANSAH M A, HUTCHINSON I J, et al. Molecular network design[J]. Nature,1991,353(6340):124. doi: 10.1038/353124a0 [5] GIBSON L J, ASHBY M F. Cellular solids: Structure and properties[M]. London: Cambridge University Press, 1997. [6] 卢子兴, 赵亚斌. 一种有负泊松比效应的二维多胞材料力学模型[J]. 北京航空航天大学学报, 2006, 32(5):594-597. doi: 10.3969/j.issn.1001-5965.2006.05.022LU Zixing, ZHAO Yabin. Mechanical model of two-dimensional cellular materials with negative Poisson’s ratio[J]. Journal of Beijing University of Aeronautics and Astronautics,2006,32(5):594-597(in Chinese). doi: 10.3969/j.issn.1001-5965.2006.05.022 [7] OLYMPIO K R, GANDHI F. Zero Poisson's ratio cellular honeycombs for flex skins undergoing one-dimensional morphing[J]. Journal of Intelligent Material Systems and Structures,2010,21(17):1737-1753. doi: 10.1177/1045389X09355664 [8] 鲁超, 李永新, 董二宝, 等. 零泊松比蜂窝芯等效弹性模量研究[J]. 材料工程, 2013(12):80-84.LU Chao, LI Yongxin, DONG Erbao, et al. Equivalent elastic modulus of zero Poisson’s ratio honeycomb core[J]. Journal of Materials Engineering,2013(12):80-84(in Chinese). [9] 李杰锋, 沈星, 陈金金. 零泊松比胞状结构的单胞面内等效模量分析及其影响因素[J]. 航空学报, 2015, 36(11):3616-3629.LI Jiefeng, SHEN Xing, CHEN Jinjin. Single cells’ in-plane equivalent moduli analysis of zero Poisson’s ratio cellular structure and their effects factor[J]. Acta Aeronautica et Astronautica Sinica,2015,36(11):3616-3629(in Chinese). [10] GRIMA J N, OLIVERI L, ATTARD D, et al. Hexagonal honeycombs with zero Poisson's ratios and enhanced stiffness[J]. Advanced Engineering Materials,2010,12(9):855-862. doi: 10.1002/adem.201000140 [11] GRIMA J N, ATTARD D. Molecular networks with a near zero Poisson's ratio[J]. Physica Status Solidi B-Basic Solid State Physics,2011,248(1):111-116. doi: 10.1002/pssb.201083979 [12] ATTARD D, GRIMA J N. Modelling of hexagonal honeycombs exhibiting zero Poisson's ratio[J]. Physica Status Solidi B-Basic Solid State Physics,2011,248(1):52-59. doi: 10.1002/pssb.201083980 [13] SOMAN P, FOZDAR D Y, LEE J W, et al. A three-dimensional polymer scaffolding material exhibiting a zero Poisson's ratio[J]. Soft Matter,2012,8(18):4946-4951. doi: 10.1039/c2sm07354d [14] GONG X, HUANG J, SCARPA F, et al. Zero Poisson's ratio cellular structure for two-dimensional morphing applications[J]. Composite Structures,2015,134:384-392. doi: 10.1016/j.compstruct.2015.08.048 [15] HUANG J, GONG X, ZHANG Q, et al. In-plane mechanics of a novel zero Poisson's ratio honeycomb core[J]. Compo-sites Part B: Engineering,2016,89:67-76. doi: 10.1016/j.compositesb.2015.11.032 [16] QIN H, YANG D, REN C. Modelling theory of functional element design for metamaterials with arbitrary negative Poisson's ratio[J]. Computational Materials Science,2018,150:121-133. doi: 10.1016/j.commatsci.2018.03.056 [17] QIN H, YANG D, REN C. Design method of lightweight metamaterials with arbitrary Poisson's ratio[J]. Materials,2018,11(9):1574. [18] 秦浩星, 杨德庆. 任意负泊松比超材料结构设计的功能基元拓扑优化法[J]. 复合材料学报, 2018, 35(4):1014-1023.QIN Haoxing, YANG Deqing. Functional element topology optimal method of metamaterial design with arbitrary negative Poisson’s ratio[J]. Acta Materiae Compositae Sinica,2018,35(4):1014-1023(in Chinese). [19] NIU B, YAN J, CHENG G. Optimum structure with homogeneous optimum cellular material for maximum fundamental frequency[J]. Structural and Multidisciplinary Optimization,2009,39(2):115-132. doi: 10.1007/s00158-008-0334-4 [20] WANG B, CHENG G D. Design of cellular structures for optimum efficiency of heat dissipation[J]. Structural and Multidisciplinary Optimization,2005,30(6):447-458. doi: 10.1007/s00158-005-0542-0 [21] 阎军, 邓佳东, 程耿东. 基于柔顺性与热变形双目标的多孔材料与结构几何多尺度优化设计[J]. 固体力学学报, 2011, 32(2):119-132.YAN Jun, DENG Jiadong, CHENG Gengdong. Multi geometrical scale optimization for porous structure and material with muti-objective of structural compliance and thermal deformation[J]. Chinese Journal of Solid Mechanics,2011,32(2):119-132(in Chinese). [22] 张卫红, 孙士平. 多孔材料/结构尺度关联的一体化拓扑优化技术[J]. 力学学报, 2006, 38(4):522-529. doi: 10.3321/j.issn:0459-1879.2006.04.012ZHANG Weihong, SUN Shiping. Integrated design of porous materials and structures with scale-coupled effect[J]. Chinese Journal of Theoretical and Applied Mechanics,2006,38(4):522-529(in Chinese). doi: 10.3321/j.issn:0459-1879.2006.04.012 [23] XIA L, BREITKOPF P. Design of materials using topology optimization and energy-based homogenization approach in Matlab[J]. Structural and Multidisciplinary Optimization,2015,52(6):1229-1241. doi: 10.1007/s00158-015-1294-0 [24] 李洪泉. 关于比强度和比模量单位的使用辨析[J]. 宇航材料工艺, 2012, 42(1):112. doi: 10.3969/j.issn.1007-2330.2012.01.026LI Hongquan. Analysis on the use of specific stength and specific modulus units[J]. Aerospace Materials & Technology,2012,42(1):112(in Chinese). doi: 10.3969/j.issn.1007-2330.2012.01.026 -
下载:
点击查看大图
计量
- 文章访问数: 1192
- HTML全文浏览量: 710
- PDF下载量: 149
- 被引次数: 0
