基于自动铺放技术的高精度变刚度复合材料层合板屈曲性能

卫宇璇; 张明; 刘佳; 刘硕; 崔志刚

doi:10.13801/j.cnki.fhclxb.20200218.001

基于自动铺放技术的高精度变刚度复合材料层合板屈曲性能

doi: 10.13801/j.cnki.fhclxb.20200218.001

北京卫星制造厂有限公司，北京 100094

详细信息

通讯作者:
张明，博士，研究员，博士生导师，研究方向为航天器复合材料结构设计　E-mail：nanwang20041208@sina.com

中图分类号: TB332
计量
- 文章访问数: 1527
- HTML全文浏览量: 648
- PDF下载量: 86
- 被引次数: 0
出版历程
- 收稿日期: 2019-12-18
- 录用日期: 2020-01-22
- 网络出版日期: 2020-02-19
- 刊出日期: 2020-11-15

Buckling performance of high-precision variable stiffness composites laminate based on automatic placement technology

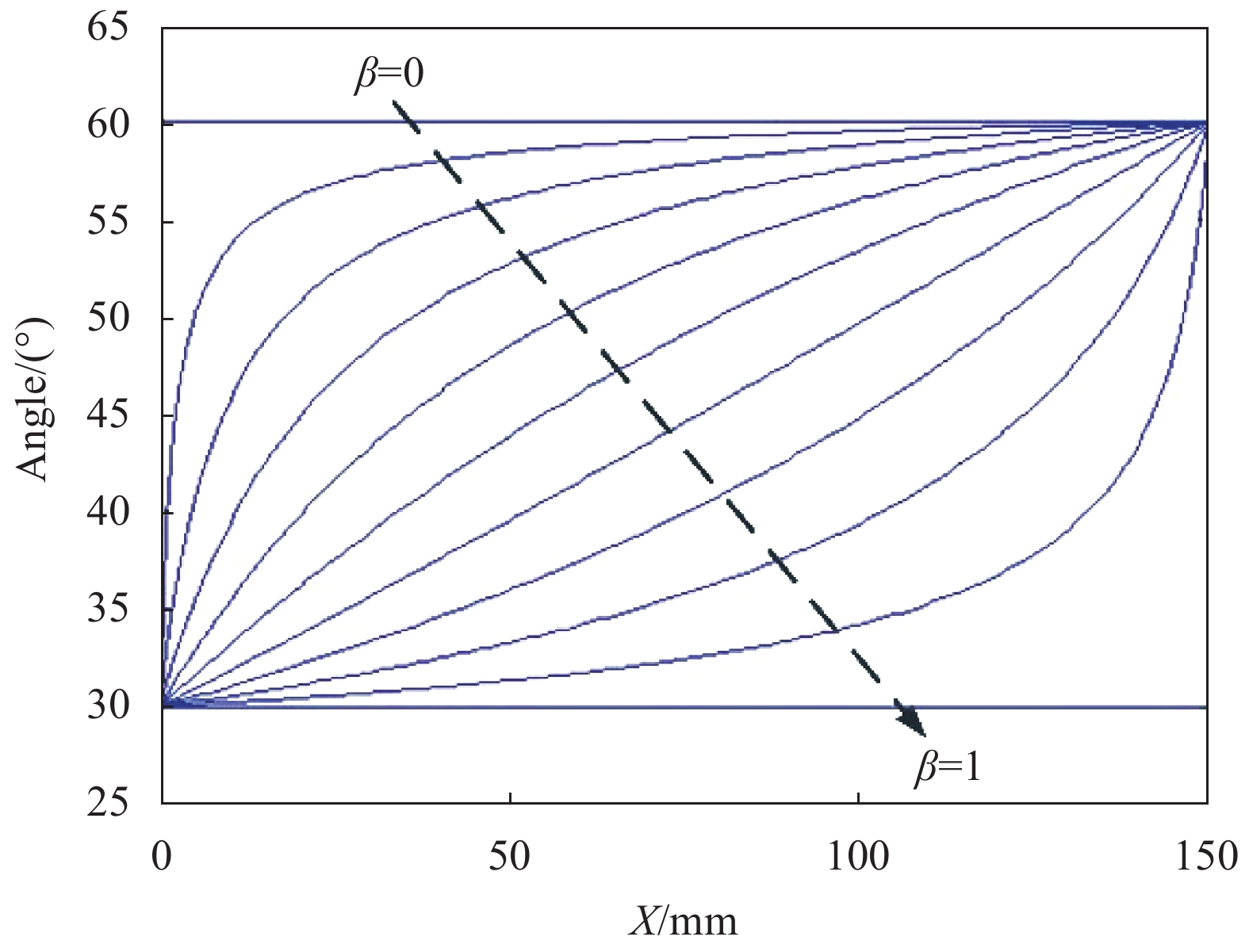
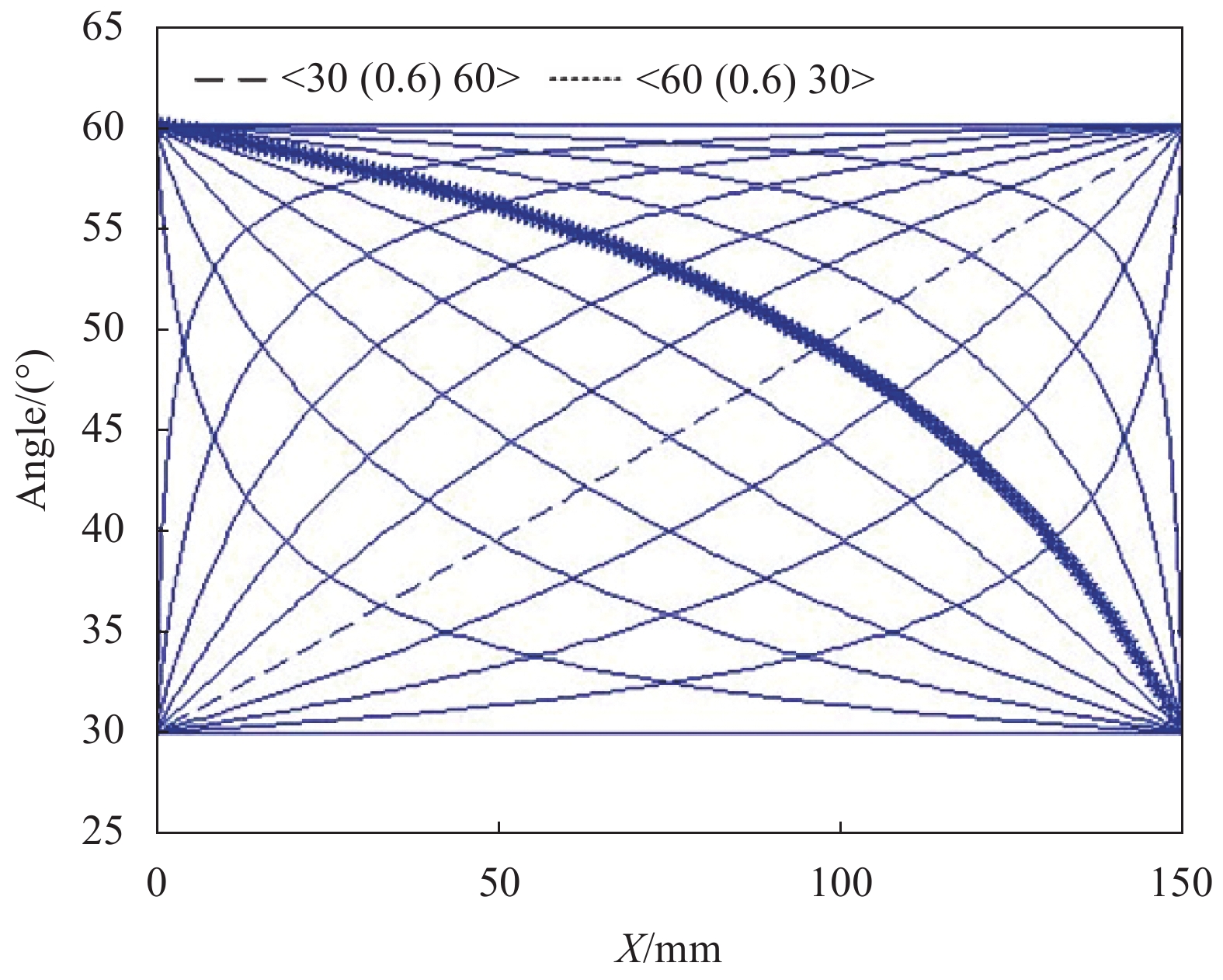
Beijing Spacecraft, Beijing 100094, China

摘要

摘要: 基于自动铺放技术制备的曲线变刚度复合材料层合板，通过定制面内刚度，可有效提高结构的抗屈曲性能。在铺放过程中，铺放轨迹的路径规划是实现变刚度设计的关键技术之一。鉴于此，本文分别以纤维角度线性变化曲线、等曲率曲线及二次Bezier曲线构成的纤维轨迹为研究对象，对其压缩屈曲性能进行参数化分析。并利用有限元模型研究了铺丝头上丝带宽度对层合板型面精度和抗屈曲力学性能的影响。结果表明：在压缩工况下，二次Bezier曲线路径的抗屈曲性能最佳，等曲率曲线路径受曲率约束的影响最小。铺丝头丝束宽度一定，丝带宽度与重叠区域面积和抗屈曲性能呈负相关。使用最大的丝带宽度可最大程度地减小重叠区域面积，提高结构的型面精度，同时保证结构屈曲性能提高37.3%。
- 变刚度 /
- 屈曲特性 /
- 自动铺放 /
- Bezier曲线 /
- 纤维丝束
Abstract: The buckling resistance of the variable stiffness composite laminates laid by the automatic placement can be effectively improved by tailoring the in-plane stiffness. During the placement process, laying the trajectory path planning is one of the key technologies to achieve variable stiffness design. So, the fiber trajectories composed of angle linear variation, constant-curvature curve and quadratic Bezier curve were taken as the research object, and the parametric analysis of its compression buckling performance was carried out. Finally, the influence of tape width on the surface profile accuracy and buckling resistance mechanical properties was studied using a finite element model. The results show that under the compression condition, the secondary Bezier curve has the best buckling resistance, and the equal curvature curve is least affected by the curvature constraint. The width of the tape is negatively correlated with the area of the overlap area and the buckling resistance, when the tow width of the fiber placement head is constant. Using the largest tape size can minimize the overlapping area and improve the profile accuracy of the composite structure. At the same time, it is guaranteed that the buckling performance of the structure is improved by 37.3%.
- variable stiffness /
- buckling performance /
- automatic placement /
- Bezier curve /
- fiber tow

HTML全文

图 1 纤维角度线性变化

Figure 1. Fiber angle linear variation

T₀—Starting angle; T₁—Ending angle; d—Characteristic length

下载: 全尺寸图片幻灯片

图 2 角度线性变化曲线纤维路径示意图

Figure 2. Schematic of fiber path of fiber angle linear variation curve

$ \phi $—Rotation angle; d—Characteristic length

下载: 全尺寸图片幻灯片

图 3 二次Bezier曲线纤维路径示意图

Figure 3. Schematic of fiber path of quadratic Bezier curve

T₀—Starting angle; T₁—Ending angle; β—Connection parameter; a—Length

下载: 全尺寸图片幻灯片

图 4 丝束宽度为3.175 mm的碳纤维/环氧树脂(M40J/4211)复合材料丝束临界曲率

Figure 4. Critical curvature of carbon fiber/epoxy (M40J/4211) composite tow with tow width of 3.175 mm

下载: 全尺寸图片幻灯片

图 5 M40J/4211复合材料变刚度层合板有限元模型

Figure 5. Finite element model of M40J/4211 composite variable stiffness laminated plate

下载: 全尺寸图片幻灯片

图 6 纤维角度线性变化曲线轨迹满足曲率约束的M40J/4211复合材料变刚度层合板屈曲特性

Figure 6. Buckling performance of M40J/4211 composite variable stiffness laminated plate with linear trajectory of fiber angle satisfying curvature constraint

下载: 全尺寸图片幻灯片

图 7 等曲率曲线轨迹满足曲率约束的M40J/4211复合材料变刚度层合板屈曲特性

Figure 7. Buckling performance of M40J/4211 composite variable stiffness laminated plate with constant-curvature curve trajectory satisfying curvature constraints

下载: 全尺寸图片幻灯片

图 8 二次Bezier曲线轨迹满足曲率约束的M40J/4211复合材料变刚度层合板屈曲特性

Figure 8. Buckling performance of M40J/4211 composite variable stiffness laminated plate with quadratic Bezier curve trajectory satisfying curvature constraints

下载: 全尺寸图片幻灯片

图 9 Bezier曲线纤维角度变化过程

Figure 9. Process of quadratic Bezier curve fiber variable angle

下载: 全尺寸图片幻灯片

图 10 Bezier曲线曲率约束非对称性验证

Figure 10. Bezier curve curvature constraint asymmetry verification

<30 (0.6) 60>—T₀=30, β=0.6, T₁=60; <60 (0.6) 30>—T₀=60, β=0.6, T₁=30

下载: 全尺寸图片幻灯片

图 11 不同丝带宽度的自动纤维铺放技术(AFP)有限元模型

Figure 11. Finite element models of automatic fiber placement (AFP) with different tape width

下载: 全尺寸图片幻灯片

图 12 丝带宽度对M40J/4211复合材料变刚度层合板屈曲性能的影响

Figure 12. Influence of tape width variation on buckling performance of M40J/4211 composite variable stiffness laminated plate

下载: 全尺寸图片幻灯片

图 13 丝带宽度对M40J/4211复合材料变刚度层合板平均厚度的影响

Figure 13. Influence of tape width variation on average thickness of M40J/4211 composite variable stiffness laminated plate

下载: 全尺寸图片幻灯片

表 1 算法程序有效性验证

Table 1. Algorithm validity verification

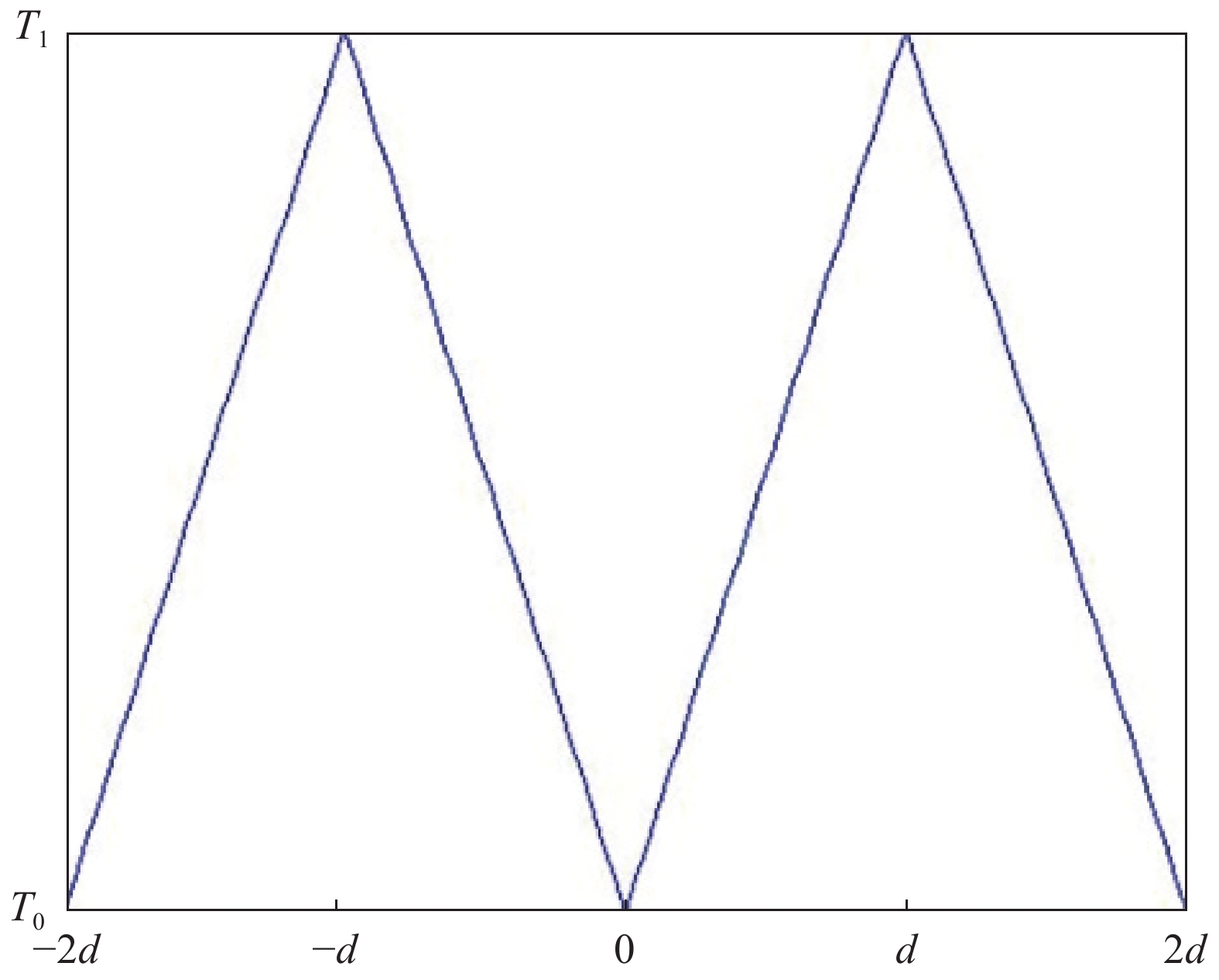
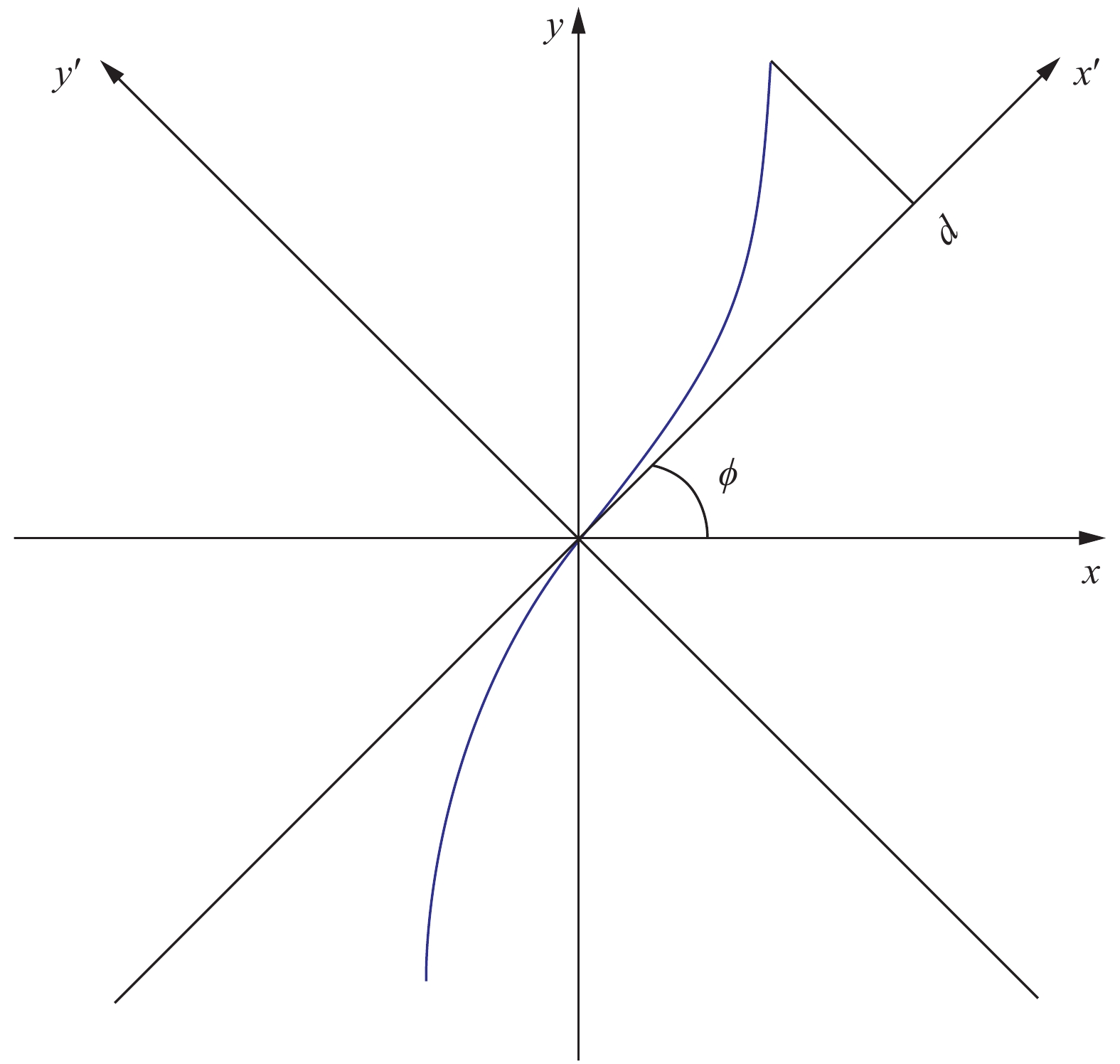
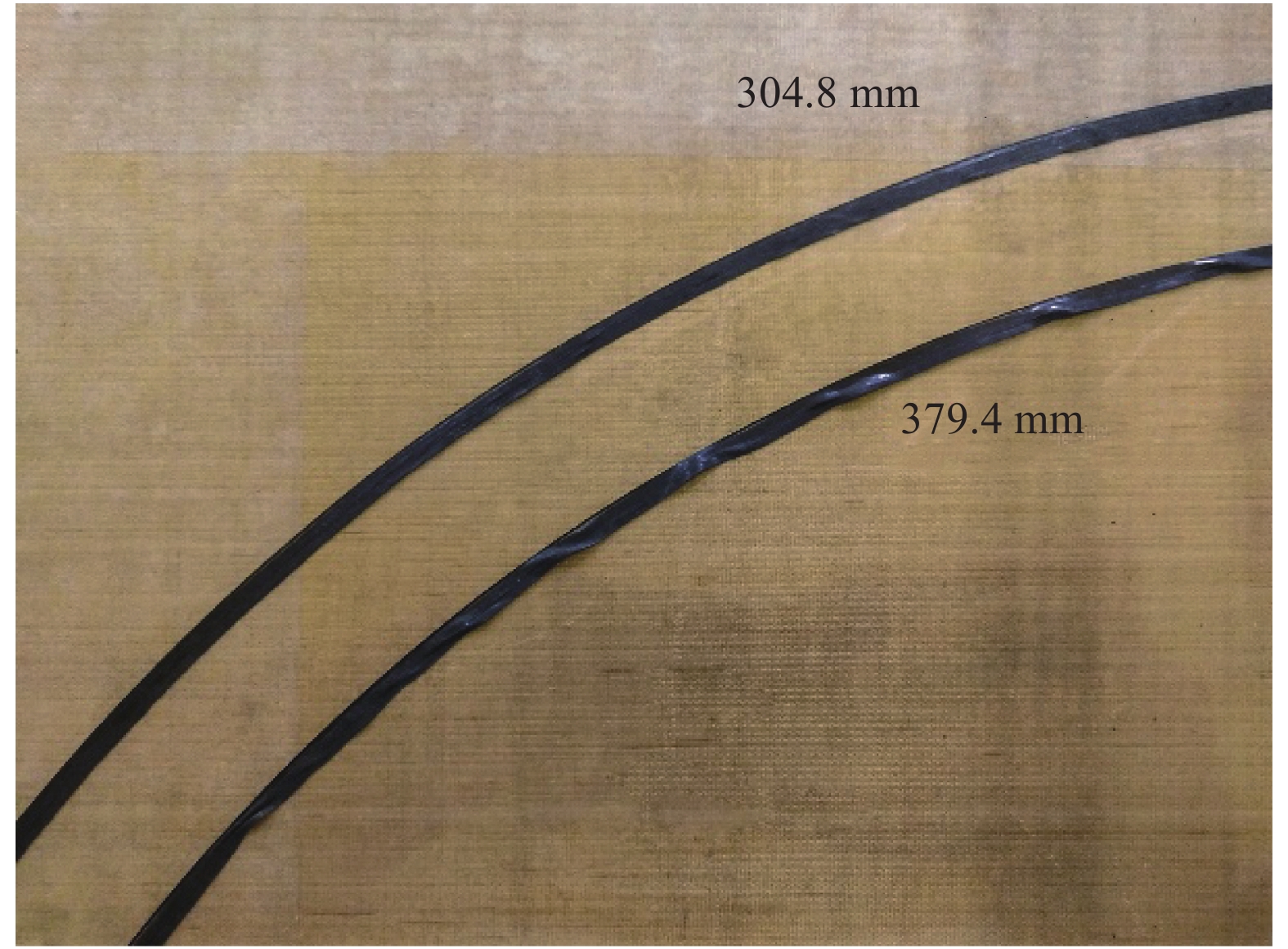
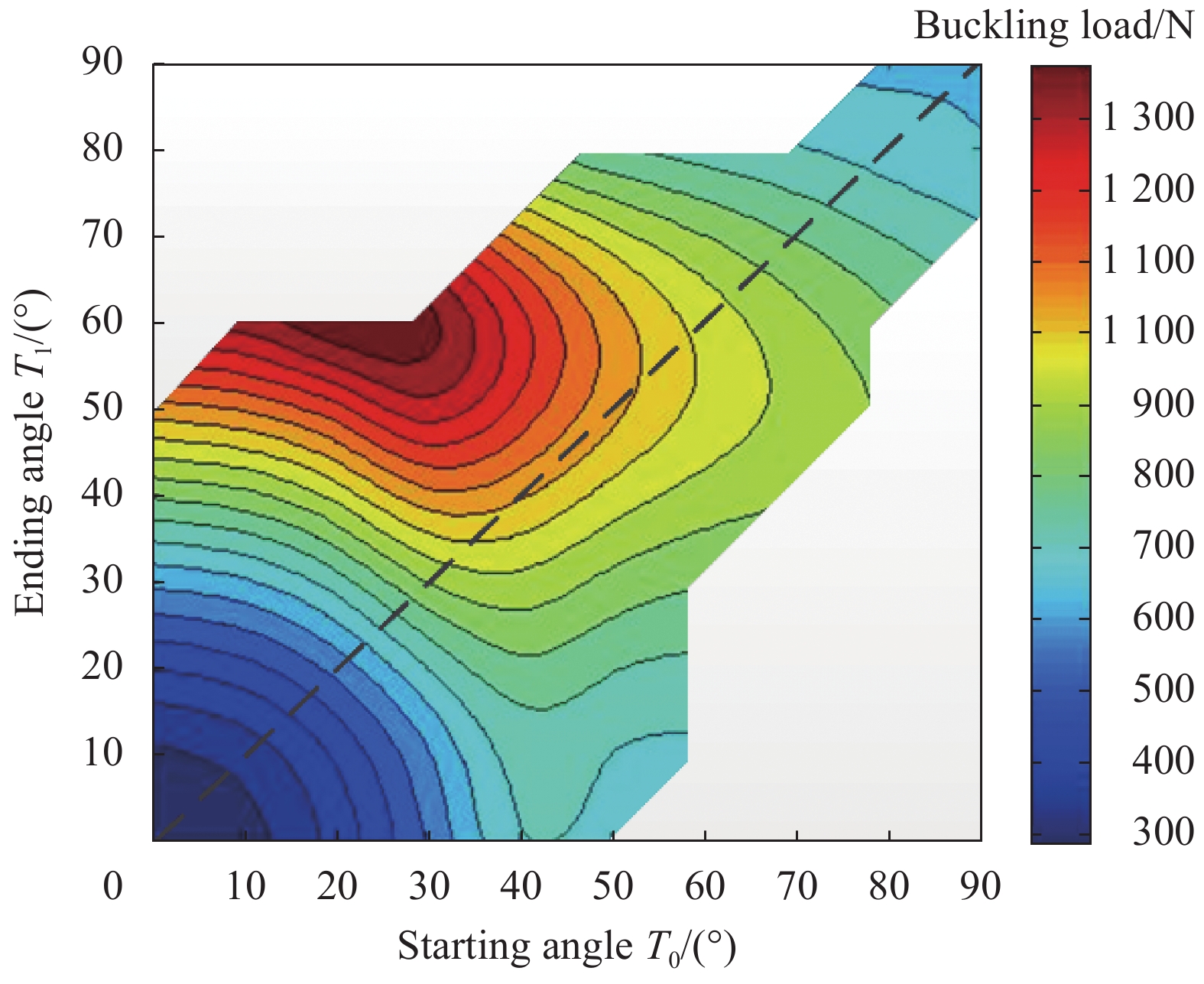
Curve placement example	This article/N	Literature[6]/N	Error/%
${\left[ { \pm 45/0 \pm {{\left\langle {45\|60} \right\rangle }_2}/0 \pm {{\left\langle {30\|15} \right\rangle }_2}} \right]_{\rm{S}}}$	15 689.5	15 641	0.30
${\left[ { \pm 45/0 \pm \left\langle {30\|45} \right\rangle /0 \pm {{\left\langle {45\|60} \right\rangle }_2}/0 \pm \left\langle {30\|15} \right\rangle } \right]_{\rm{S}}}$	16 558.0	16 514	0.27

下载: 导出CSV

表 2 丝带宽度对M40J/4211复合材料变刚度层合板型面精度及结构抗屈曲效率的影响

Table 2. Effect of tape width on profile accuracy and structure buckling efficiency of M40J/4211 composite variable stiffness laminated plate

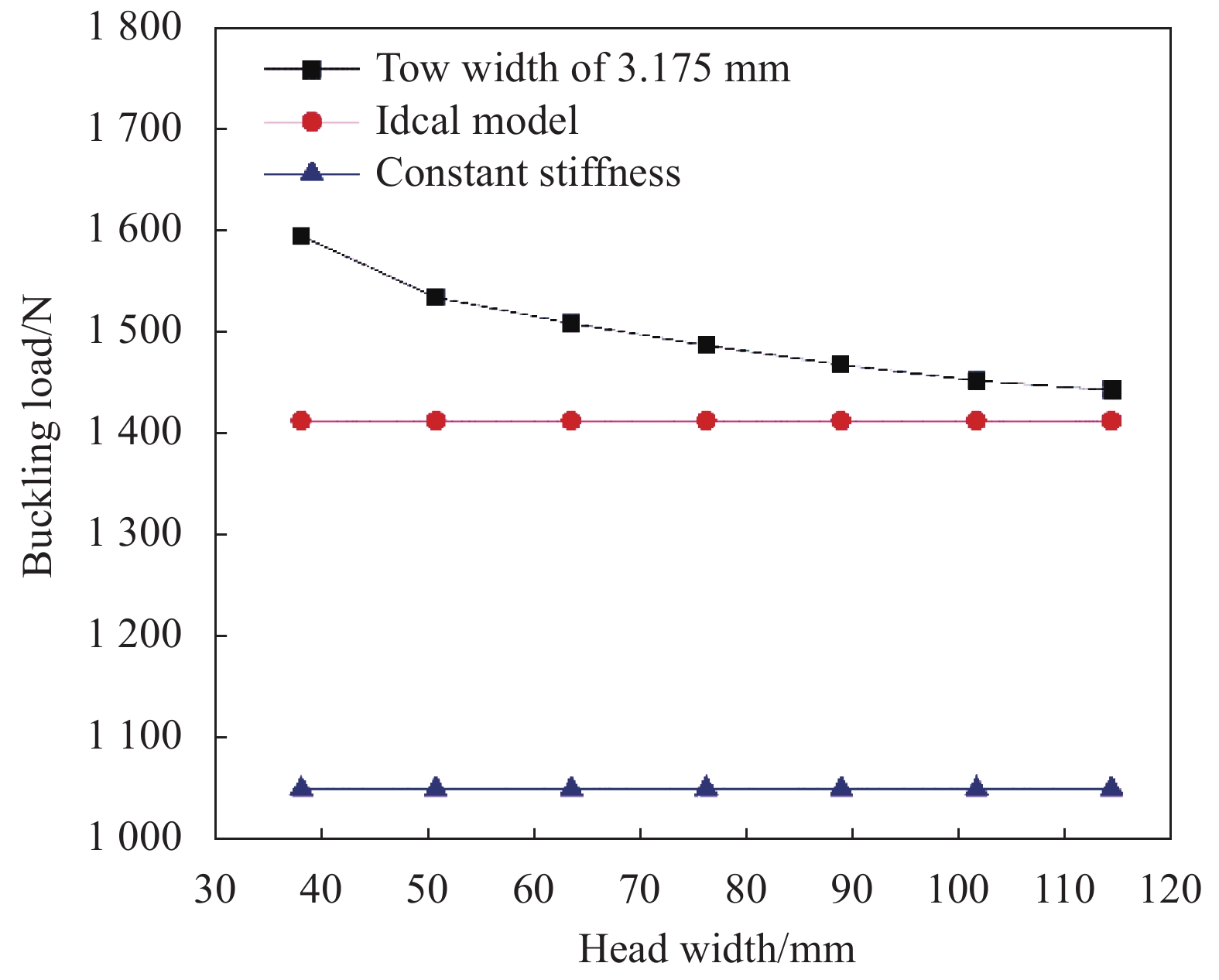
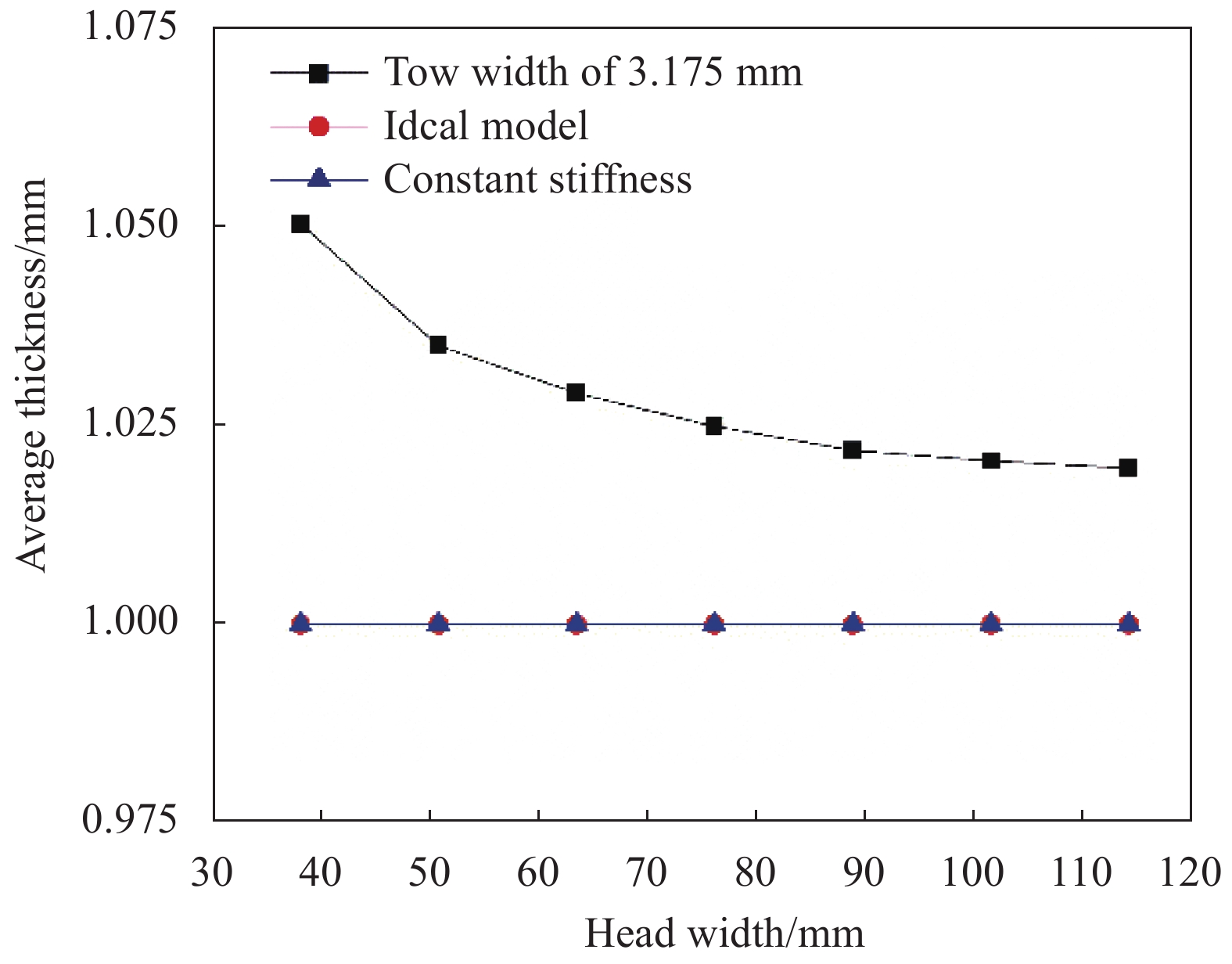
Tape width/mm	Number of tow	Average thickness/mm	Critical buckling load/N	Improved structural efficiency/%
38.1	12	1.0505	1 594	44.4
50.8	16	1.0352	1 534	41.0
63.5	20	1.0292	1 508	39.5
76.2	24	1.0250	1 487	38.0
88.9	28	1.0219	1 468	36.7
101.6	32	1.0206	1 452	35.4
114.3	36	1.0197	1 443	34.7

下载: 导出CSV

参考文献(20)

[1]	文立伟, 肖军, 王显峰, 等. 中国复合材料自动铺放技术研究进展[J]. 南京航空航天大学学报, 2015, 47(5):637-649. WEN Liwei, XIAO Jun, WANG Xianfeng, et al. Process of automated placement technology for composites in China[J]. Journal of Nanjing University of Aeronautics & Astronautics,2015,47(5):637-649(in Chinese).
[2]	周晓芹, 曹正华. 复合材料自动铺放技术的发展及应用[J]. 航空制造技术, 2009(s1):1-3, 7. ZHOU Xiaoqin, CAO Zhenghua. Development and application of automated placement technology for composites[J]. Aeronautical Manufacturing Technology,2009(s1):1-3, 7(in Chinese).
[3]	SHIRINZADEH B, CASSIDY G, OETOMO D, et al. Trajectory generation for open-contoured structures in robotic fibre placement[J]. Robotics and Computer-Integrated Manufacturing,2007,23(4):380-394. doi: 10.1016/j.rcim.2006.04.006
[4]	ABDALLA M M, SETOODEH S, GÜRDAL Z. Design of variable stiffness composite panels for maximum fundamental frequency using lamination parameters[J]. Composite Structures,2007,81(2):283-291. doi: 10.1016/j.compstruct.2006.08.018
[5]	SETOODEH S, ABDALLA M M, GÜRDAL Z. Design of variable-stiffness laminates using lamination parameters[J]. Composites Part B: Engineering,2006,37(4-5):301-309. doi: 10.1016/j.compositesb.2005.12.001
[6]	马永前, 张淑杰, 许震宇. 纤维曲线铺放的变刚度复合材料层合板的屈曲[J]. 玻璃钢/复合材料, 2009(5):31-35. MA Yongqian, ZHANG Shujie, XU Zhenyu. The buckling of variable-stiffness composite panels with curvilinear fiber format[J]. Fiber Reinforced Plastics/Composites,2009(5):31-35(in Chinese).
[7]	GÜRDAL Z, OLMEDO R. In-plane response of laminates with spatially varying fiber orientations: Variable stiffness concept[J]. AIAA Journal,1993,31(4):751-758. doi: 10.2514/3.11613
[8]	GÜRDAL Z, TATTING B F, WU C K. Variable stiffness composite panels: Effects of stiffness variation on the in-plane and buckling response[J]. Composites Part A: Applied Science and Manufacturing,2008,39(5):911-922. doi: 10.1016/j.compositesa.2007.11.015
[9]	WU K C, GÜRDAL Z, STARNES J H. Structural response of compression loaded, tow placed, variable stiffness panels[C]//Proceedings of 43rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference. Denver: AIAA, 2002: 2002-1512.
[10]	JEGLRY D C, TATTING B F, GÜRDAL Z. Tow-steered panels with holes subjected to compression or shear loading[C]//Proceedings of 46th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference. Austin: AIAA, 2005: 2005-2081.
[11]	GÜRDAL Z, TATTING B F, WU K C. Tow-placement technology and fabrication issues for laminated composite structures[C]//Proceedings of 46th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference. Austin: AIAA, 2005: 2005-2017.
[12]	富宏亚, 曹忠亮, 杜霖, 等. Bezier曲线变角度层合板设计及屈曲特性分析[J]. 复合材料学报, 2017, 34(8):1729-1735. FU Hongya, CAO Zhongliang, DU Lin, et al. Design of Bezier curve variable angle laminates and analysis on buckling property[J]. Acta Materiae Compositae Sinica,2017,34(8):1729-1735(in Chinese).
[13]	牛雪娟, 杨涛, 杜宇. 基于流场函数的变刚度层合板铺放设计[J]. 固体火箭技术, 2014, 37(6):848-855. NIU Xuejuan, YANG Tao, DU Yu. Path planning of variable stiffness laminates based on the flow field function[J]. Journal of Solid Rocket Technology,2014,37(6):848-855(in Chinese).
[14]	张雁, 张峤, 梁珂, 等. 变刚度复合材料机翼设计与优化[C]//2015中国力学大会. 上海: 中国力学学会, 2015. ZHANG Yan, ZHANG Qiao, LIANG Ke, et al. Design and optimization of variable stiffness composite wing[C]//2015 The Chinese Congress of Theoretical and Applied Mechanics. Shanghai: Chinese Society of Theoretical and Applied Mechanics, 2015(in Chinese).
[15]	FALCÓ O, MAYUGO J A, LOPES C S, et al. Variable-stiffness composite panels: As-manufactured modeling and its influence on the failure behavior[J]. Composites Part B: Engineering,2014,56:660-669. doi: 10.1016/j.compositesb.2013.09.003
[16]	PEETERS D M J, LOZANO G G, ABDALLA M M. Effect of steering limit constraints on the performance of variable stiffness laminates[J]. Computers & Structures,2018,196:94-111.
[17]	MAROUENE A, BOUKHILI R, CHEN J, et al. Effects of gaps and overlaps on the buckling behavior of an optimally designed variable-stiffness composite laminates: A numerical and experimental study[J]. Composite Structures,2016,140:556-566. doi: 10.1016/j.compstruct.2016.01.012
[18]	杜霖. 变刚度层合板力学性能分析及铺放成型技术研究[D]. 哈尔滨: 哈尔滨工业大学, 2016. DU Lin. Study on mechanical behavior of variable stiffness laminates and its manufacturing technology with fiber placement[D]. Harbin: Harbin Institute of Technology, 2016(in Chinese).
[19]	CAO Z, FU H, HAN Z. Comparative study on bezier curve and linear variable angle method for variable stiffness laminates[J]. Polymer Composites,2019,40(3):952-960.
[20]	顾杰斐, 陈普会, 孔斌, 等. 考虑制造因素的变刚度层合板的抗屈曲铺层优化设计[J]. 复合材料学报, 2018, 35(4):866-875. GU Jiefei, CHEN Puhui, KONG Bin, et al. Layup optimization for maximum buckling load of variable stiffness laminates considering manufacturing factors[J]. Acta Materiae Compositae Sinica,2018,35(4):866-875(in Chinese).