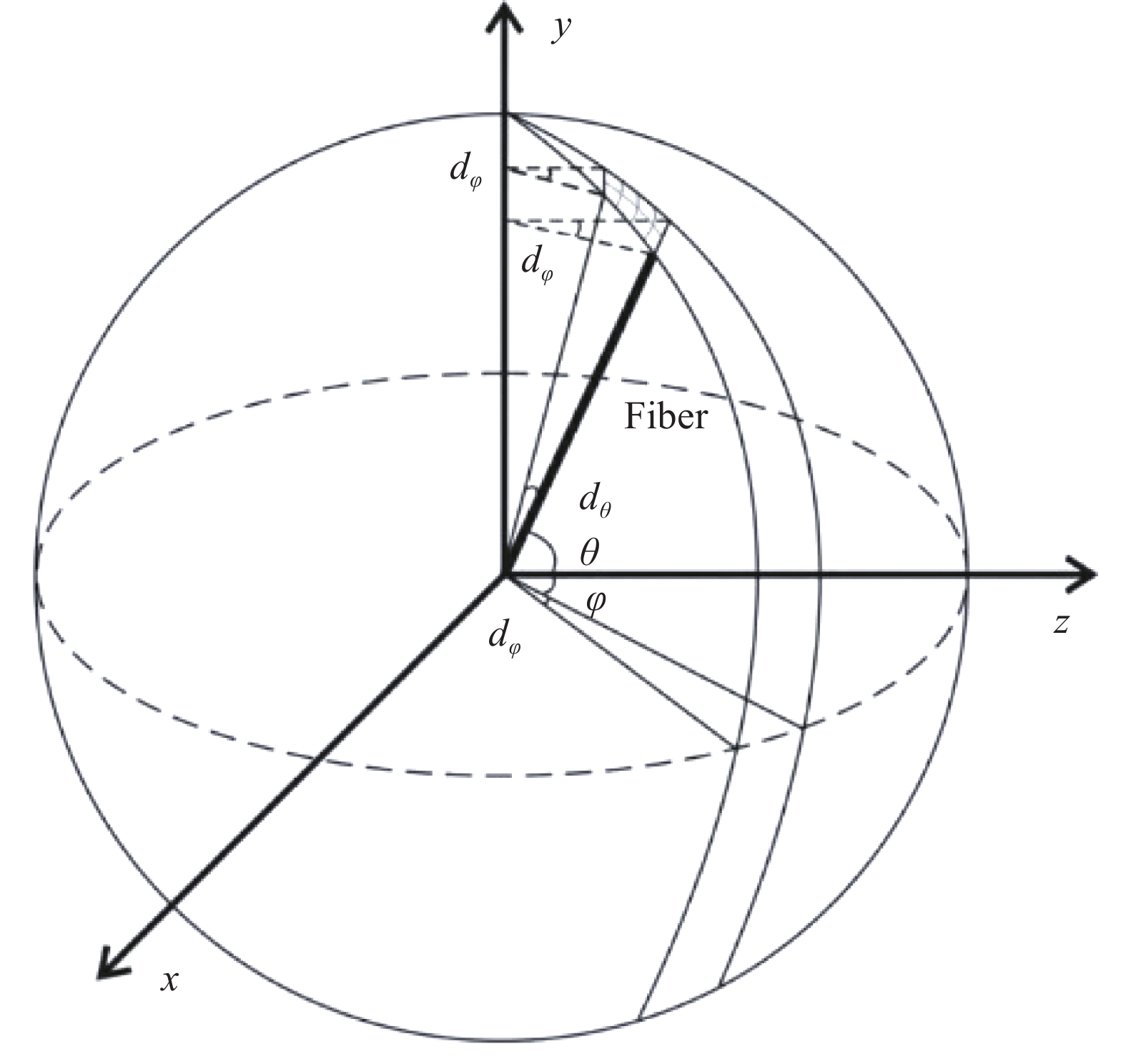
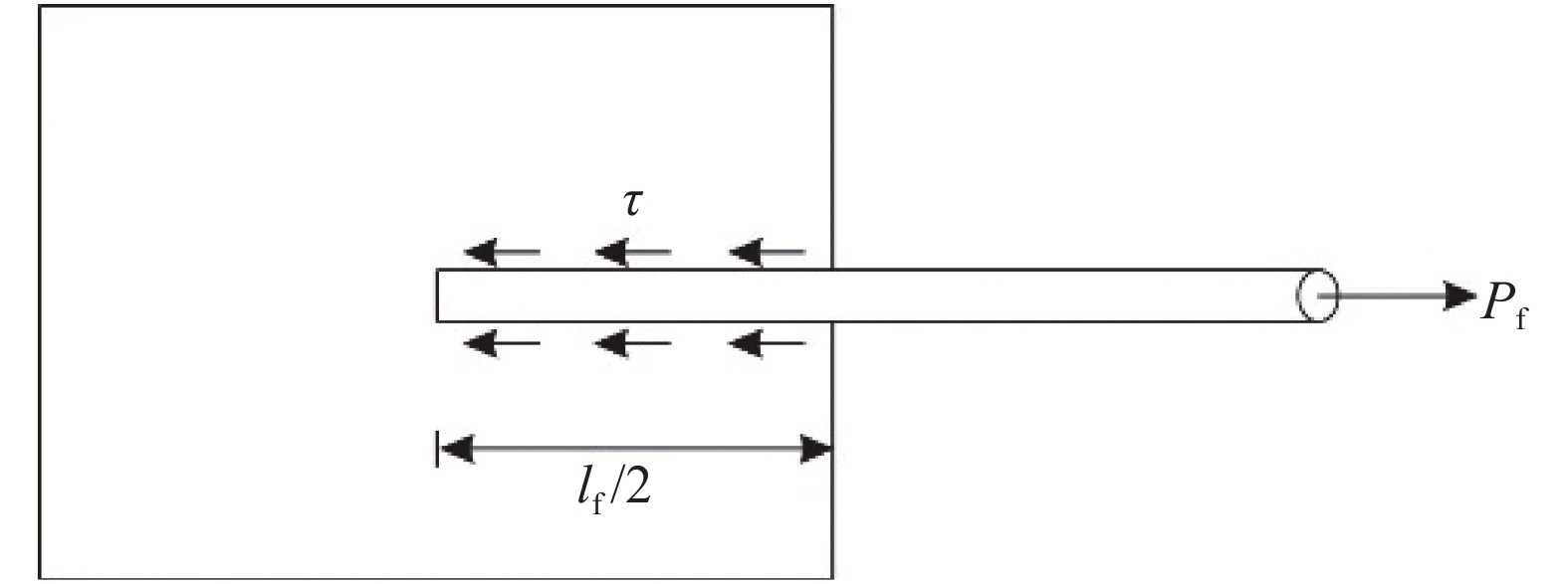
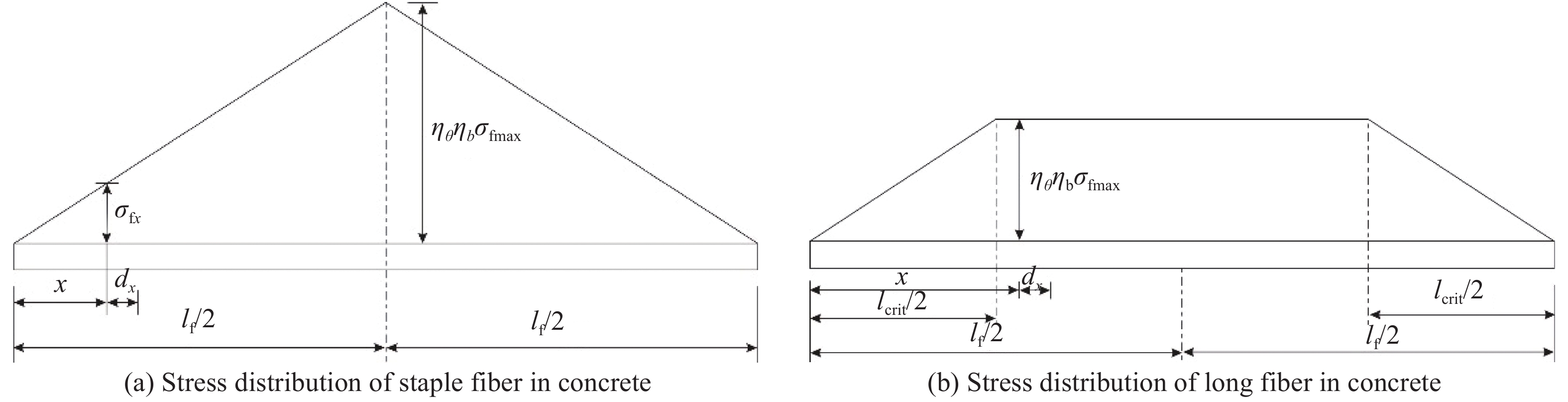
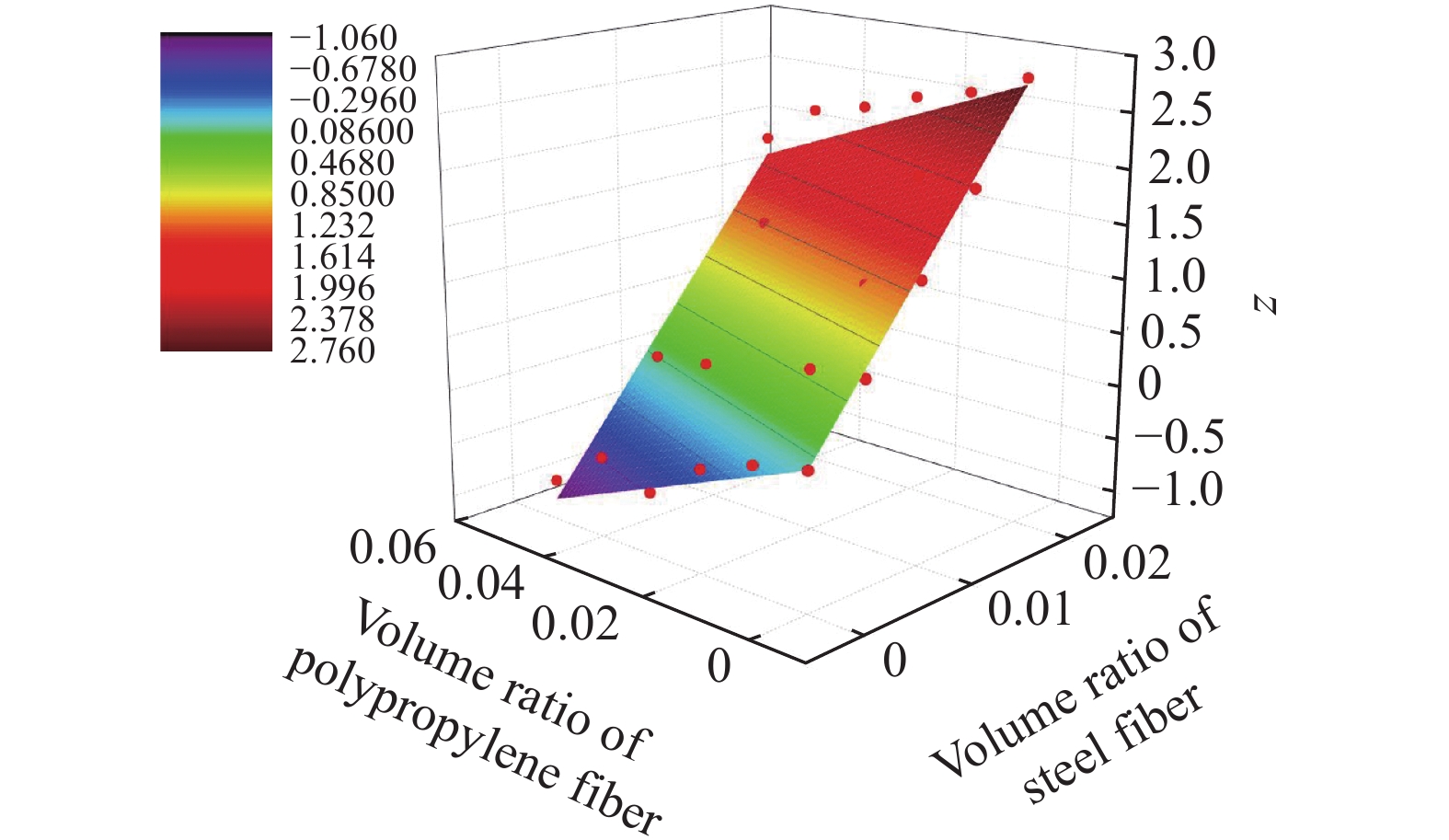
Calculation of bearing capacity of polypropylene-steel fiber reinforced concrete column under large eccentric loading
-
摘要: 对270个聚丙烯纤维掺量(体积分数)分别为0vol%、0.1vol%、0.2vol%、0.3vol%、0.4vol%、0.5vol%、钢纤维掺量(体积分数)分别为0vol%、0.5vol%、1vol%、1.5vol%、2vol%的聚丙烯-钢纤维/混凝土试块进行立方体抗压试验、轴心抗压试验和劈裂抗拉试验，基于复合材料力学理论，考虑纤维的取向系数、长度有效系数和界面黏结系数，对其建立强度预测模型并进行机制分析，同时选取掺量分别为0vol%、0.1vol%、0.3vol%的聚丙烯纤维、掺量分别为0vol%、1.5vol%的钢纤维制作6根聚丙烯-钢纤维/混凝土柱，对其进行大偏心受压试验，在强度预测模型的基础上进行承载力计算，提出聚丙烯-钢纤维/混凝土承载力计算方法。结果表明：钢纤维对聚丙烯-钢纤维/混凝土立方体抗压强度、轴心抗压强度和劈裂抗拉强度均有提高；聚丙烯纤维可提高聚丙烯-钢纤维/混凝土的劈裂抗拉强度，但不能提高聚丙烯-钢纤维/混凝土的抗压强度；聚丙烯-钢混杂纤维加入混凝土柱可有效提高其极限承载力。Abstract: The cube compressive test, axial compressive test and splitting tensile test were conducted on 270 polypropylene-steel fiber/concrete test blocks with polypropylene fiber content (volume fraction) of 0vol%, 0.1vol%, 0.2vol%, 0.3vol%, 0.4vol%, 0.5vol% and steel fiber content (volume fraction) of 0vol%, 0.5vol%, 1vol%, 1.5vol% and 2vol% respectively. Based on the mechanical theory of polypropylene-steel fiber/concrete composites, the strength prediction model was established considering the fiber orientation coefficient, effective length coefficient and interfacial bond coefficient. The mechanism was also analyzed. Six polypropylene-steel fiber/concrete columns were made from polypropylene fiber with a content of 0vol%, 0.1vol%, 0.3vol% and steel fiber with a content of 0vol%, 1.5vol% to perform large eccentric compression test. On the basis of strength prediction model, the bearing capacity of polypropylene-steel fiber/concrete was calculated. The results show that steel fiber can improve the compressive strength, axial compressive strength and splitting tensile strength of polypropylene-steel fiber/concrete. Polypropylene fiber can improve the splitting tensile strength of polypropylene-steel fiber/concrete, but can’t improve the compressive strength. The ultimate bearing capacity of concrete columns can be effectively increased by adding polypropylene-steel fibers.
-
工程用水泥基复合材料(Engineered cementitious composites，ECC)是一种通过微观力学和断裂力学原理对材料体系进行系统设计和优化的短纤维乱向水泥基复合材料[1-2]，国内外学者围绕ECC的受拉、受弯等基本力学性能开展了大量相关研究工作，并取得了众多优异成果[3-8]。然而ECC的抗拉强度仍然有限，用于结构加固时对构件的抗拉、抗弯承载力和抗裂性能的增强效果有限，仍需与性能优异的增强材料共同使用，为此，众多学者相继开展筋材、网材等用于增强ECC受力性能的研究。
Fischer等[9]、Mihashi等[10]进行了钢筋增强ECC单轴拉伸试验，结果表明，钢筋增强ECC试件裂缝分布更加均匀，减小了裂缝宽度，提高了变形能力。郑宇宙等[11]、朱忠锋等[12]对纤维增强ECC复合材料进行了单轴拉伸试验，结果表明，增强材料可以明显改善ECC的受拉性能，其轴向刚度、极限承载力也得到了显著提高。但是已研究的增强材料中，采用普通钢筋时，ECC的优越性能不能完全发挥，使用纤维编织网增强材料又降低了经济性。而高强不锈钢绞线抗拉强度高，具有良好的耐腐蚀性能和经济性。相关学者对高强不锈钢绞线加固混凝土结构的研究表明，其能有效提高加固构件的抗弯、抗剪承载力和刚度[13-17]。目前该加固技术已成功应用于实际工程，并取得了优异的效果[18-19]。
综上分析，高强不锈钢绞线网是由不锈钢绞线编制而成，本身具有很强的耐腐蚀、抗锈蚀能力；ECC具有良好的裂缝分散能力，正常使用状态裂缝宽度很小[1-10]，二者结合后，可以使ECC开裂后钢绞线不发生锈蚀，耐久性好。因此，朱俊涛等[20]提出了新型高性能复合材料“高强不锈钢绞线网增强ECC”。已对高强不锈钢绞线网与ECC的粘结锚固性能[21-22]进行了试验和理论研究，结果表明，在试验所得的粘结滑移曲线中，平均粘结应力达到峰值开始微下降至峰值的85%左右之后，即进入延性强化段，该阶段平均粘结应力不再降低，仅滑移量呈水平线增长，说明高强不锈钢绞线网与ECC具有良好的粘结锚固性能。为了尽快使该复合材料在实际工程中得到应用，本文对高强不锈钢绞线网增强ECC的受拉性能进行试验和理论研究。
1. 试验概况
1.1 试件设计及材性试验
为了研究ECC抗拉强度、高强不锈钢绞线网受拉方向配筋率及高强不锈钢绞线网增强ECC试件宽度三个影响因素对高强不锈钢绞线增强ECC抗拉性能的影响，共设计了9组试件，每组3个共27个试件，各组试件参数见表1。为了避免试件在加载过程中在变截面处应力集中破坏，本试验受拉试件均采用哑铃型试件，试件变截面处为弧形，试件示意图如图1所示。试验中ECC的两种配合比见刘伟康[5]研究成果，试件标准养护28天。对预留的ECC受拉薄板试件(280 mm×40 mm×15 mm)进行拉伸试验，得到两种ECC配合比的抗拉性能，各组试件的ECC抗拉性能见表2。高强不锈钢绞线直径均为2.4 mm，对其进行拉伸试验(3根)，得到高强不锈钢绞线应力-应变曲线如图2所示。
表 1 高强不锈钢绞线网增强工程水泥基复合材料(ECC)试件参数Table 1. Parameters of engineered cementitious composites(ECC) reinforced by high-strength stainless steel wire mesh specimensGroup number Test section width bc /mm Steel strand spacing/mm Reinforcement ratio of steel strand TC12 80 50 0.0028 TC22 70 40 0.0032 TC32 60 30 0.0037 TC42 50 20 0.0048 TC52 90 30 0.0037 TD12 80 50 0.0028 TD22 70 40 0.0032 TD32 60 30 0.0037 TD42 50 20 0.0048 ![]()
图 1 高强不锈钢绞线网增强ECC试件设计详图(虚线表示钢绞线)Figure 1. Design details of ECC reinforced by high-strength stainless steel wire mesh specimens (Dotted line indicates steel strand)表 2 ECC受拉试验结果Table 2. Tensile test results of ECCGroup number Tensile strength/MPa Ultimate tensile strain Cracking stress/MPa Cracking strain TC12 3.53 0.0179 2.45 0.000204 TC22 3.53 0.0179 2.45 0.000204 TC32 3.53 0.0179 2.45 0.000204 TC42 3.53 0.0179 2.45 0.000204 TC52 3.53 0.0179 2.45 0.000204 TD12 3.46 0.0297 2.39 0.000189 TD22 3.46 0.0297 2.39 0.000189 TD32 3.46 0.0297 2.39 0.000189 TD42 3.46 0.0297 2.39 0.000189 ![]()
图 2 高强不锈钢绞线应力-应变曲线Figure 2. Stress-strain curves of high-strength stainless steel strand1.2 加载及测试方法
单轴拉伸试验采用100 kN电液伺服万能试验机。试验加载采用位移控制，加载速率为0.2 mm/min。试件正反面分别粘贴应变片，测试区段两侧面分别布置量程为30 mm的位移计。
1.3 试验现象及机制分析
高强不锈钢绞线网增强ECC试件受拉试验的加载装置如图3(a)所示。当荷载达到极限荷载的25%~30%时，在试件接近中间位置表面出现一条细微裂缝(如图3(b)所示)，宽度仅为0.02 mm。随着荷载的增加，试件表面不断出现新的裂缝，已有的裂缝宽度缓慢增大；当荷载增加到极限荷载的85%~90%，裂缝数量达到饱和状态(如图3(c)所示)，试件表面布满相互平行的细微裂缝，此时各试件的最大裂缝宽度为0.2~0.28 mm，该状态的荷载远远超过了规范GB 50010—2010[23]规定的正常使用极限状态。继续加载，试件表面不再出现新裂缝，已有裂缝变宽。达到极限荷载时，试件表面的某条裂缝发展成主裂缝，同时伴随着明显的纤维拔出和断裂声，随后主裂缝处的纵向高强不锈钢绞线拉断。试件破坏形态如图3(d)所示。
![]()
图 3 高强不锈钢绞线网增强ECC试件受拉破坏过程Figure 3. Tensile failure process of ECC reinforced by high-strength stainless steel wire mesh specimens分析上述试验现象发现，高强不锈钢绞线的加入增加了ECC裂缝分散能力，其机制主要是钢绞线表面的螺旋进一步限制了ECC裂缝的开展，使受拉试件在达到极限承载力的85%~90%时，最大裂缝宽度依然满足规范GB 50010—2010[23]中正常使用极限状态裂缝要求。充分表明了本文研究的新型复合材料高强不锈钢绞线网增强ECC具有良好的抗裂性能；验证了高强不锈钢筋网和ECC具有很好的粘结性能。
2. 试验结果及分析
2.1 试验结果
各组高强不锈钢绞线网增强ECC试件拉伸试验结果见表3 (试验值均取同一组试件的平均值，应力为试验拉力除以受力面积)。
表 3 高强不锈钢绞线网增强ECC试件拉伸试验结果Table 3. Tensile test results of ECC reinforced by high-strength stainless steel wire mesh specimensGroup number Cracking stress/MPa Cracking strain Ultimate tensile stress/MPa Ultimate tensile strain Elastic modulus/MPa TC12 2.20 0.000156 7.09 0.030874 14 471 TC22 2.25 0.000162 7.94 0.034056 14 122 TC32 2.41 0.000171 8.77 0.030581 14 492 TC42 2.56 0.000182 10.65 0.034815 14 578 TC52 2.54 0.000181 8.82 0.033683 14 642 TD12 1.97 0.000145 6.41 0.031929 14 216 TD22 1.99 0.000149 7.81 0.043660 14 727 TD32 2.07 0.000139 8.62 0.041116 14 460 TD42 2.19 0.000159 10.30 0.035051 14 496 2.1.1 试件宽度对高强不锈钢绞线网增强ECC受拉性能的影响
对比表3中TC32组和TC52组试件，结果表明，增大截面宽度对受拉试件的开裂应力和极限应力几乎没有影响。
2.1.2 高强不锈钢绞线配筋率对高强不锈钢绞线网增强ECC受拉性能的影响
对比表3中TC12~TC42及TD12~TD42试件的试验结果可知，随着高强不锈钢绞线配筋率的增大，受拉试件的开裂应力略有增大，极限应力明显增大。
2.1.3 ECC抗拉强度对高强不锈钢绞线网增强ECC受拉性能的影响
对比表3中TC12组与TD12组、TC22组与TD22组、TC32组与TD32组及TC42组与TD42组试件结果可知，随着ECC抗拉强度的增大，受拉试件的开裂应力和极限应力均明显增大。
2.2 高强不锈钢绞线网增强ECC受拉应力-应变曲线
高强不锈钢绞线网增强ECC试件在单轴荷载作用下的受拉应力-应变曲线如图4所示。可知，高强不锈钢绞线网增强ECC试件受拉应力-应变曲线呈现出明显的两阶段特征，故可将其分为两个阶段：(1)可表征为弹性阶段，从试件开始加载到试件开裂为止，为峰值的25%~30%。在该阶段ECC和不锈钢绞线均处于弹性工作状态；应力-应变曲线接近为直线；(2)可表征为弹塑性阶段，对应于试件ECC开裂至完全破坏(纵向高强不锈钢绞线拉断)。该阶段应力-应变曲线为非线性关系，试件表现出明显的弹塑性性质。此阶段主要是ECC裂缝出现和发展，由于裂缝处的聚乙烯醇(PVA)纤维尚未从水泥基中拔出或拉断，而高强不锈钢绞线表面的螺旋增大了与ECC的粘结力。因此，该阶段ECC和高强不锈钢绞线依然共同承担拉力，亦是高强不锈钢绞线网增强ECC受拉的主要阶段。由图4还可以看出，极限应力是开裂应力的3~5倍，极限应变为开裂应变的200倍以上，达到3%~5%，超过了本文采用的ECC和高强不锈钢绞线本身的极限拉应变，显示了该新型高性能复合材料优越的变形能力。
![]()
图 4 高强不锈钢绞线网增强ECC试件受拉应力-应变曲线Figure 4. Tensile stress-strain curves of ECC reinforced by high-strength stainless steel wire mesh specimens3. 高强不锈钢绞线网增强ECC受拉本构模型
3.1 模型提出
由图4可知，高强不锈钢绞线网增强ECC受拉应力-应变曲线可分为弹性阶段(OA段)和应变硬化阶段(AB段)。弹性阶段即认为应力-应变为线性关系；弹塑性(应变硬化)阶段应力由ECC和钢绞线共同承担，其本构模型由二者应力-应变关系组合而成。同时考虑该阶段高强不锈钢绞线网和ECC可能产生相对滑移，用γ表示钢绞线的应力发挥系数。本文提出了高强不锈钢绞线网增强ECC受拉本构模型，如图5所示。其表达式如下：
![]()
图 5 高强不锈钢绞线网增强ECC受拉应力-应变曲线模型Figure 5. Tensile stress-strain curve model of ECC reinforced by high-strength stainless steel wire meshσse={Eseεse(εse⩽ (1) 式中：σse、σse,cr和σse,u分别为高强不锈钢绞线网增强ECC试件的受拉应力、开裂应力和极限拉应力；σe和σs分别为ECC和高强不锈钢绞线的应力；Ese为高强不锈钢绞线网增强ECC的弹性模量；εse、εse,cr和εse,u分别为高强不锈钢绞线网增强ECC试件的受拉应变、开裂应变和极限拉应变；γ为高强不锈钢绞线的应力发挥系数；ρs为高强不锈钢绞线的配筋率。
3.2 模型参数分析
3.2.1 弹性阶段
高强不锈钢绞线网增强ECC受拉试件开裂前，由高强不锈钢绞线和ECC共同承担外部荷载；该阶段高强不锈钢绞线网与ECC间无滑移。根据应变协调原理得出弹性阶段高强不锈钢绞线网增强ECC受拉试件的弹性模量Ese如下式：
{E_{\rm{se}}} = {E_{\rm{e}}} + {E_{\rm{s}}}\frac{{{A_{\rm{s}}}}}{{{A_{\rm{se}}}}} (2) 式中：Ee和Es分别为ECC和高强不锈钢绞线的弹性模量；Ase和As分别为高强不锈钢绞线网增强ECC和高强不锈钢绞线的截面面积。
3.2.2 弹塑性阶段
首先建立高强不锈钢绞线受拉本构模型。对本试验得到的高强不锈钢绞线应力-应变曲线(图2)采用三次多项式进行拟合，如下式：
\frac{{{\sigma _{\rm{s}}}}}{{{\sigma _{\rm{s,u}}}}} = a\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}} + (3 - 2a){\left( {\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}}} \right)^2} + (a - 2){\left( {\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}}} \right)^3}\tag{3a} (3a) 式中：σs,u为高强不锈钢绞线的极限拉应力；εs和εs,u分别为高强不锈钢绞线应变和极限拉应变。
根据式(3a)，基于最小二乘法对试验数据进行回归分析，得到待定常数a的值。对三组数据进行拟合，其值分别为3.37、3.26和3.36。由拟合结果可知，各组值较接近，故可取其平均值a=3.33。将a代入式(3a)，则有：
\frac{{{\sigma _{\rm{s}}}}}{{{\sigma _{\rm{s,u}}}}} = 3.33\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}} - 3.66{\left( {\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}}} \right)^2} + 1.33{\left( {\frac{{{\varepsilon _{\rm{s}}}}}{{{\varepsilon _{\rm{s,u}}}}}} \right)^3}\tag{3b} (3b) ECC的受拉应力-应变关系采用刘伟康[5]的研究成果，如下式：
\!\!\!\!\!\!\quad{\sigma _{\rm{e}}} = \left\{ {\begin{array}{*{20}{l}} {\dfrac{{{\sigma _{\rm{e,cr}}}}}{{{\varepsilon _{\rm{e,cr}}}}}{\varepsilon _{\rm{e}}}}&{\;\;\left( {{\varepsilon _{\rm{e}}} \leqslant {\varepsilon _{\rm{e,cr}}}} \right)}\\ {\left( {0.31\dfrac{{{\varepsilon _{\rm{e}}}}}{{{\varepsilon _{\rm{e,u}}}}} + 0.69} \right){\sigma _{\rm{e,u}}}}&{\left( {{\varepsilon _{\rm{e,cr}}} \leqslant {\varepsilon _{\rm{e}}} \leqslant {\varepsilon _{\rm{e,u}}}} \right)} \end{array}} \right. (4) 式中：σe,cr和σe,u为ECC的开裂应力和极限拉应力；εe、εe,cr和εe,u为ECC的应变、开裂应变和极限拉应变。
3.2.3 高强不锈钢绞线应力发挥系数γ
将式(2)、式(3b)和式(4)代入式(1)，则高强不锈钢绞线网增强ECC受拉本构关系可表示为
{\sigma _{\rm{se}}}{\rm{ = }}\left\{ {\begin{array}{*{20}{l}} {\left( {{E_{\rm{e}}} + {E_{\rm{s}}}\dfrac{{{A_{\rm{s}}}}}{{{A_{\rm{se}}}}}} \right){\varepsilon _{\rm{se}}}} \\ \begin{array}{l} \!\!\!\!\!\!\!\!\gamma \left[ {{\rm{3}}{\rm{.33}}\dfrac{{{\varepsilon _{\rm{se}}}}}{{{\varepsilon _{\rm{se,u}}}}}{\rm{ - 3}}{\rm{.66}}{{\left( {\dfrac{{{\varepsilon _{\rm{se}}}}}{{{\varepsilon _{\rm{se,u}}}}}} \right)}^2}{\rm{ + 1}}{\rm{.33}}{{\left( {\dfrac{{{\varepsilon _{\rm{se}}}}}{{{\varepsilon _{\rm{se,u}}}}}} \right)}^3}} \right]{\sigma _{\rm{s,u}}}\dfrac{{{A_{\rm{s}}}}}{{{A_{\rm{se}}}}} +\\ \!\! \left( {{\rm{0}}{\rm{.31}}\dfrac{{{\varepsilon _{\rm{se}}}}}{{{\varepsilon _{\rm{se,u}}}}} + 0.69} \right){\sigma _{\rm{e,u}}} \\ \end{array} \end{array}} \right. (5) 基于应变协调，高强不锈钢绞线网增强ECC的开裂应变与ECC相等，即εse,cr=εe,cr；同时高强不锈钢绞线网增强ECC受拉试件的破坏形态为纵向高强不锈钢绞线拉断破坏，其极限应变主要取决于高强不锈钢绞线的极限应变大小，即εse,u=εs,u。
分别将开裂状态和破坏状态的应变值代入式(5)，利用MATLAB软件进行分析，得到式(5)中高强不锈钢绞线应力发挥系数γ的值，如表4所示。可知，各组值较接近，故取其平均值(0.725)作为式(5)中γ的值。
表 4 高强不锈钢绞线应力发挥系数γ取值Table 4. Value of stress development coefficient γ of high-strength stainless steel strandGroup number γ R2 TC12 0.73 0.9267 TC22 0.72 0.9459 TC32 0.76 0.9607 TD12 0.71 0.9157 TD22 0.72 0.9755 TD32 0.71 0.9325 3.2.4 开裂应力和抗拉强度公式
基于高强不锈钢绞线网增强ECC受拉试件的本构关系模型(式(5))，推导出高强不锈钢绞线网增强ECC受拉试件的开裂应力和极限拉应力即抗拉强度的计算公式：
\left\{ {\begin{array}{*{20}{l}} {{\sigma _{\rm{se,cr}}} = \left( {{E_{\rm{e}}} + {E_{\rm{s}}}\dfrac{{{A_{\rm{s}}}}}{{{A_{\rm{se}}}}}} \right){\varepsilon _{\rm{se,cr}}}} \\ {{f_{\rm{se,u}}} = 0.725{\sigma _{\rm{s,u}}}\dfrac{{{A_{\rm{s}}}}}{{{A_{\rm{se}}}}} + {\sigma _{\rm{e,u}}}} \end{array}} \right. (6) 式中，fse,u为高强不锈钢绞线网增强ECC受拉试件的极限应力，即抗拉强度。
3.3 模型验证
为验证本文提出的高强不锈钢绞线网增强ECC受拉本构模型，将试验得出的应力-应变曲线与式(5)计算的应力-应变曲线进行对比，如图6所示。将试验得出的开裂应力和极限拉应力(抗拉强度)与式(6)计算的结果进行对比，如表5所示。
表 5 试验与式(6)计算得到的高强不锈钢绞线网增强ECC试件开裂应力和极限应力的对比Table 5. Comparison of cracking stress and ultimate tensile stress of ECC reinforced by high-strength stainless steel wire mesh specimens obtained by test and equation (6)Group number Cracking stress/MPa Ultimate tensile stress/MPa T C R T C R TC12 2.20 2.26 0.97 7.09 6.79 1.04 TC22 2.25 2.27 0.99 7.94 7.25 1.10 TC32 2.41 2.28 1.06 8.77 7.87 1.11 TC42 2.56 2.30 1.11 10.65 9.19 1.16 TC52 2.54 2.28 1.11 8.82 7.87 1.12 TD12 1.97 1.94 1.01 6.41 6.58 0.97 TD22 1.99 1.95 1.02 7.81 7.03 1.11 TD32 2.07 1.96 1.06 8.62 7.63 1.13 TD42 2.19 1.98 1.11 10.30 8.90 1.16 Notes: T—Test value; C—Calculated value; R—T/C. ![]()
图 6 高强不锈钢绞线网增强ECC受拉本构模型验证Figure 6. Verification of tensile constitutive model of ECC reinforced by high-strength stainless steel wire mesh从图6可看出，本文提出的本构模型与试验应力-应变曲线整体趋势完全一致。其中，本文所提的受拉本构关系曲线的弹性阶段与试验值基本重合，符合良好；其弹塑性阶段与试验曲线弯曲度均吻合较好(TD42稍有差别)，仅试验在ECC开裂后，荷载有微小波动，属于正常情况。因此，本文提出的高强不锈钢绞线网增强ECC受拉本构模型能较准确地预测受拉应力-应变关系全曲线。表5中的高强不锈钢绞线网增强ECC受拉试件的开裂应力试验值与计算值比值的平均值为1.05，标准差为0.05，变异系数为0.05；极限应力试验值与计算值比值的平均值为1.10，标准差为0.06，变异系数为0.05，说明本文提出的式(6)计算结果和试验值吻合良好。
综上所述，本文提出的高强不锈钢绞线网增强ECC受拉本构模型和开裂应力、抗拉强度计算公式可用于反映高强不锈钢绞线网增强ECC受拉状态的应力-应变关系和分析其受拉性能。
4. 结 论
对高强不锈钢绞线网增强工程水泥基复合材料(Engineered cementitious composites，EEC) 的受拉性能进行试验和理论研究，提出了其受拉本构关系模型。
(1)高强不锈钢绞线表面的螺旋进一步限制了ECC裂缝的开展，增加了ECC裂缝的分散能力，使受拉试件在达到极限承载力的85%时，最大裂缝宽度依然小于规范GB 50010—2010[23]中正常使用极限状态裂缝要求。充分显示了高强不锈钢绞线网增强ECC的良好抗裂性能。
(2)高强不锈钢绞线网增强ECC受拉应力-应变曲线分为弹性阶段和弹塑性阶段，弹塑性阶段是其主要受拉阶段，该阶段是ECC和钢绞线共同受拉至钢绞线拉断，极限应变可达到3%~5%，显示了该新型复合材料优越的变形能力。
(3)随着ECC抗拉强度提高和纵向钢绞线配筋率增大，高强不锈钢绞线网增强ECC的开裂应力和极限拉应力(抗拉强度)均增大。
(4)所建立的受拉本构模型及模型参数计算公式与试验结果吻合良好，能够较好地反映高强不锈钢绞线网增强ECC的受拉应力-应变关系，预测其受力状态；所提出的高强不锈钢绞线网增强ECC受拉开裂应力、抗拉强度的计算公式与试验结果吻合良好，可以用于分析该新型复合材料受拉性能。
-
![]()
图 3 聚丙烯-钢纤维/混凝土柱配筋
Figure 3. Reinforcement drawing of polypropylene-steel fiber/concrete column
![]()
图 4 聚丙烯-钢纤维/混凝土柱大偏心受压试验
Figure 4. Large eccentric compression test of polypropylene-steel fiber/concrete column
![]()
图 7 混凝土-纤维界面黏结力与纤维拉应力模型
Figure 7. Concrete-fiber interfacial bonding force and fiber tensile stress model
![]()
图 8 混凝土中不同长度纤维的应力分布
Figure 8. Stress distribution of fibers of different lengths in concrete
![]()
图 9 聚丙烯-钢纤维/混凝土劈裂抗拉强度拟合模型
Figure 9. Splitting tensile strength fitting model of polypropylene-steel fiber/concrete
![]()
图 10 聚丙烯-钢纤维/混凝土抗压强度拟合模型
Figure 10. Compressive strength fitting model of polypropylene-steel fiber/concrete
![]()
图 12 聚丙烯-钢纤维/混凝土拉-压本构模型
Figure 12. Tension-compression constitutive model of polypropylene-steel fiber/concrete
![]()
图 13 聚丙烯-钢纤维/混凝土柱不同阶段正截面受力情况
Figure 13. Stress of normal section of polypropylene-steel fiber/concrete column at different stages
表 1 锂渣的化学成分
Table 1 Chemical composition of lithium slag
wt% SiO2 Al2O3 Fe2O3 CaO MgO SO3 K2O Na2O Li2O 54.39 19.83 1.40 7.98 0.24 8.30 0.14 0.26 0.77 
下载: 导出CSV
表 2 聚丙烯纤维和钢纤维的主要参数
Table 2 Parameters of polypropylene fibers and steel fibers
Fiber type Length/mm Diameter/μm Density/(g·cm−3) Elasticity modulus /GPa Tensile strength/MPa Steel fiber 33 300 7.82 210 ≥600 Polypropylene fiber 19 33 0.91 >3.5 530
下载: 导出CSV
表 3 混凝土配比
Table 3 Mix proportions of concrete
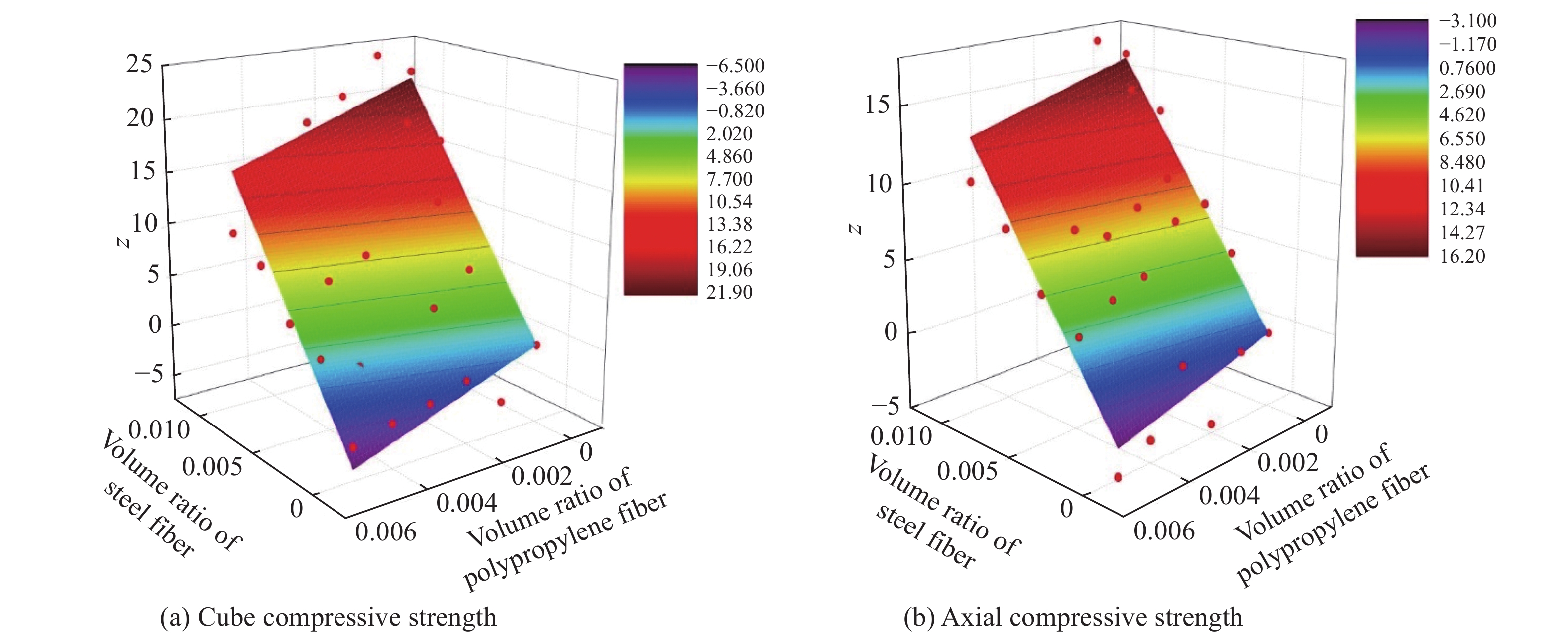
kg·m–3 Water Cement Sand Cobblestone Water reducer Lithium slag C50 concrete composition 150 530 656 1 170 5.3 — Concrete composition used in test 150 424 656 1 170 5.3 106
下载: 导出CSV
表 4 聚丙烯-钢纤维/混凝土劈裂抗拉强度试验值及计算值
Table 4 Test values and calculation values of splitting tensile strength of polypropylene-steel fiber/concretes
Test number Volume fraction of polypropylene fiber/vol% Volume fraction of steel fiber/vol% Test value of split tensile strength/MPa Calculated value of split tensile strength/MPa Test value/Calculated value P0S0 0 0 2.92 2.92 1.00 P0S1 0 0.5 3.51 3.59 0.98 P0S2 0 1 4.19 4.27 0.98 P0S3 0 1.5 4.84 4.94 0.98 P0S4 0 2 5.68 5.61 1.01 P1S0 0.1 0 3.07 2.97 1.03 P1S1 0.1 0.5 3.72 3.64 1.02 P1S2 0.1 1 4.30 4.31 1.00 P1S3 0.1 1.5 5.11 4.99 1.02 P1S4 0.1 2 5.74 5.66 1.01 P2S0 0.2 0 3.14 3.02 1.04 P2S1 0.2 0.5 3.44 3.69 0.93 P2S2 0.2 1 4.11 4.36 0.94 P2S3 0.2 1.5 5.01 5.04 0.99 P2S4 0.2 2 5.88 5.71 1.03 P3S0 0.3 0 3.03 3.07 0.99 P3S1 0.3 0.5 4.02 3.74 1.07 P3S2 0.3 1 4.17 4.41 0.95 P3S3 0.3 1.5 4.78 5.09 0.94 P3S4 0.3 2 5.97 5.76 1.04 P4S0 0.4 0 3.46 3.12 1.11 P4S1 0.4 0.5 4.22 3.79 1.11 P4S2 0.4 1 4.38 4.46 0.98 P4S3 0.4 1.5 5.17 5.13 1.01 P4S4 0.4 2 6.12 5.81 1.05 P5S0 0.5 0 3.35 3.16 1.06 P5S1 0.5 0.5 3.49 3.84 0.91 P5S2 0.5 1 4.03 4.51 0.89 P5S3 0.5 1.5 5.05 5.18 0.97 P5S4 0.5 2 6.03 5.86 1.03
下载: 导出CSV
表 5 聚丙烯-钢纤维/混凝土立方体抗压强度试验值及计算值
Table 5 Test values and calculated values of cube compressive strength of polypropylene-steel fiber/concrete
Test number Volume fraction of polypropylene fiber/vol% Volume fraction of
steel fiber/vol%Test value of cube compressive strength/MPa Calculated value of cube compressive strength/MPa Test value/
Calculated valueP0S0 0 0 62.8 62.80 1.00 P0S1 0 0.5 64.2 67.96 0.94 P0S2 0 1 69.0 73.12 0.94 P0S3 0 1.5 78.3 78.28 1.00 P0S4 0 2 84.1 83.44 1.01 P1S0 0.1 0 58.2 61.45 0.95 P1S1 0.1 0.5 69.2 66.61 1.04 P1S2 0.1 1 74.2 71.77 1.03 P1S3 0.1 1.5 80.5 76.93 1.05 P1S4 0.1 2 86.0 82.09 1.05 P2S0 0.2 0 61.2 60.10 1.02 P2S1 0.2 0.5 66.3 65.26 1.02 P2S2 0.2 1 69.1 70.42 0.98 P2S3 0.2 1.5 74.8 75.58 0.99 P2S4 0.2 2 82.4 80.74 1.02 P3S0 0.3 0 60.0 58.76 1.02 P3S1 0.3 0.5 62.0 63.91 0.97 P3S2 0.3 1 70.4 69.07 1.02 P3S3 0.3 1.5 77.3 74.23 1.04 P3S4 0.3 2 80.3 79.39 1.01 P4S0 0.4 0 59.2 57.41 1.03 P4S1 0.4 0.5 62.5 62.57 1.00 P4S2 0.4 1 68.7 67.73 1.01 P4S3 0.4 1.5 69.5 72.88 0.95 P4S4 0.4 2 77.2 78.04 0.99 P5S0 0.5 0 58.1 56.06 1.04 P5S1 0.5 0.5 64.1 61.22 1.05 P5S2 0.5 1 65.5 66.38 0.99 P5S3 0.5 1.5 69.3 71.54 0.97 P5S4 0.5 2 70.8 76.70 0.92
下载: 导出CSV
表 6 聚丙烯-钢纤维/混凝土轴心抗压强度试验值及计算值
Table 6 Test values and calculated values of axial compressive strength of polypropylene-steel fiber/concretes
Test
numberVolume fraction of polypropylene fiber/vol% Volume fraction of steel fiber/vol% Test value of axial compressive strength/MPa Calculated value of axial compressive strength/MPa Test value/
Calculated valueP0S0 0 0 43.3 43.30 1.00 P0S1 0 0.5 47.7 47.13 1.01 P0S2 0 1 50.4 50.97 0.99 P0S3 0 1.5 55.7 54.80 1.02 P0S4 0 2 58.9 58.63 1.00 P1S0 0.1 0 42.8 42.64 1.00 P1S1 0.1 0.5 51.6 46.47 1.11 P1S2 0.1 1 52.4 50.31 1.04 P1S3 0.1 1.5 57.5 54.14 1.06 P1S4 0.1 2 60.1 57.97 1.04 P2S0 0.2 0 38.8 41.98 0.92 P2S1 0.2 0.5 51.0 45.81 1.11 P2S2 0.2 1 51.0 49.65 1.03 P2S3 0.2 1.5 53.3 53.48 1.00 P2S4 0.2 2 55.8 57.31 0.97 P3S0 0.3 0 43.5 41.32 1.05 P3S1 0.3 0.5 48.1 45.15 1.07 P3S2 0.3 1 49.7 48.99 1.01 P3S3 0.3 1.5 51.0 52.82 0.97 P3S4 0.3 2 53.0 56.65 0.94 P4S0 0.4 0 39.6 40.66 0.97 P4S1 0.4 0.5 47.3 44.49 1.06 P4S2 0.4 1 50.7 48.33 1.05 P4S3 0.4 1.5 50.3 52.16 0.96 P4S4 0.4 2 54.7 55.99 0.98 P5S0 0.5 0 38.2 40.00 0.96 P5S1 0.5 0.5 45.7 43.83 1.04 P5S2 0.5 1 47.3 47.67 0.99 P5S3 0.5 1.5 50.4 51.50 0.98 P5S4 0.5 2 52.5 55.33 0.95
下载: 导出CSV
表 7 聚丙烯-钢纤维/混凝土柱极限承载力试验值及计算值
Table 7 Test values and calculation values of ultimate bearing capacity of polypropylene-steel fiber/concrete column
Test
numberVolume fraction of
polypropylene
fiber/vol%Volume fraction
of steel
fiber/vol%Equivalent
compression
zone height/mmTest value
of bearing
capacity/kNCalculated value
of bearing
capacity/kNTest value/
Calculated
valueP0S0 0 0 80.43 564.6 529.2 0.94 P1S0 0 0.001 81.60 572.0 561.4 0.98 P3S0 0 0.003 84.00 600.2 603.5 1.01 P0S3 0.015 0 78.95 638.4 576.8 0.91 P1S3 0.015 0.001 79.81 654.2 605.0 0.93 P3S3 0.015 0.003 81.58 651.7 609.5 0.94
下载: 导出CSV
-
[1] 徐礼华, 夏冬桃, 夏广政, 等. 钢纤维和聚丙烯纤维对高强混凝土强度的影响[J]. 武汉理工大学学报, 2007, 29(4):58-60, 98. DOI: 10.3321/j.issn:1671-4431.2007.04.018 XU Lihua, XIA Dongtao, XIA Guangzheng, et al. Effect of steel fiber and polypropylene fiber on the strength of high strength concrete[J]. Journal of Wuhan University of Technology,2007,29(4):58-60, 98(in Chinese). DOI: 10.3321/j.issn:1671-4431.2007.04.018
[2] 高丹盈, 赵军, 汤寄予. 掺有纤维的高强混凝土劈拉性能试验研究[J]. 土木工程学报, 2005, 38(7):21-26. DOI: 10.3321/j.issn:1000-131X.2005.07.005 GAO Danying, ZHAO Jun, TANG Jiyu. An experimental study on the behavior of fiber reinforced high-strength concrete under splitting tension[J]. China Civil Engineering Journal,2005,38(7):21-26(in Chinese). DOI: 10.3321/j.issn:1000-131X.2005.07.005
[3] 潘慧敏, 马云朝. 钢纤维混凝土抗冲击性能及其阻裂增韧机理[J]. 建筑材料学报, 2017, 20(6):956-961. DOI: 10.3969/j.issn.1007-9629.2017.06.021 PAN Huimin, MA Yunchao. Impact resistance of steel fiber reinforced concrete and its mechanism of crack resistance and toughening[J]. Journal of Building Materials,2017,20(6):956-961(in Chinese). DOI: 10.3969/j.issn.1007-9629.2017.06.021
[4] 李传习, 聂洁, 石家宽, 等. 纤维类型对混凝土抗压强度和弯曲韧性的增强效应及变异性的影响[J]. 土木与环境工程学报(中英文), 2019, 41(2):147-158. LI Chuanxi, NIE Jie, SHI Jiakuan, et al. Effect of fiber type on compressive strength and flexural toughness of concrete and analysis of variability[J]. Journal of Civil and Environmental Engineering,2019,41(2):147-158(in Chinese).
[5] 赵秋山, 徐慎春, 刘中宪. 钢纤维增强超高性能混凝土抗压性能的细观数值模拟[J]. 复合材料学报, 2018, 35(6):1661-1673. ZHAO Qiushan, XU Shenchun, LIU Zhongxian. Microscopic numerical simulation of the uniaxial compression of steel fiber reinforced ultra-high performance concrete[J]. Acta Materiae Compositae Sinica,2018,35(6):1661-1673(in Chinese).
[6] 赵纪生, 陶夏新, 师黎静, 等. 钢纤维混凝土弯压构件承载力试验与理论分析[J]. 土木工程学报, 2005, 38(3):12-16. DOI: 10.3321/j.issn:1000-131X.2005.03.003 ZHAO Jishen, TAO Xiaxin, SHI Lijin, et al. Experimental and theoretical studies on the bearing capacities of steel-fiber reinforced structural members under compressive bending[J]. China Civil Engineering Journal,2005,38(3):12-16(in Chinese). DOI: 10.3321/j.issn:1000-131X.2005.03.003
[7] 徐礼华, 黄乐, 韦翠梅, 等. 钢-聚丙烯混杂纤维混凝土柱抗震承载力试验研究[J]. 建筑结构学报, 2014, 35(8):95-103. XU Lihua, HUANG Le, WEI Cuimei, et al. Experimental Tests of seismic bearing capacity of steel-polypropylene hybrid fiber reinforced concrete columns[J]. Journal of Building Structures,2014,35(8):95-103(in Chinese).
[8] 朱海堂, 程晟钊, 高丹盈, 等. BFRP筋钢纤维高强混凝土梁受弯承载力试验与理论[J]. 复合材料学报, 2018, 35(12):3313-3323. ZHU Haitang, CHENG Shengzhao, GAO Danyin, et al. Exprimental and theoretical study on the flexural capacity on high-strength concrete beams reinforced with BFRP bars and steel fiber[J]. Acta Materiae Compositae Sinica,2018,35(12):3313-3323(in Chinese).
[9] TOKGOZ S, DUNDAR C, TANRIKULU A K. Experimental behaviour of steel fiber high strength reinforced concrete and composite columns[J]. Journal of Constructional Steel Research,2012,74:98-107. DOI: 10.1016/j.jcsr.2012.02.017
[10] 中国工程建设标准化协会. 纤维混凝土试验方法标准: CECS 13∶2009[S]. 北京: 中国计划出版社, 2010. China Association for Engineering Construction Standardization. Standard test methods for fiber reinforced concrete: CECS 13∶2009[S]. Beijing: China Planning Press, 2010(in Chinese).
[11] 中华人民共和国住房和城乡建设部. 普通混凝土力学性能试验方法标准: GB/T 50081—2002[S]. 北京: 中国建筑工业出版社, 2003. Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for test method of mechanical properties of ordinary concrete: GB/T 50081—2002[S]. Beijing: China Architecture & Building Press, 2003(in Chinese).
[12] GANESAN N, INDIRA P V, SABEENA M V. Bond stress slip response of bars embedded in hybrid fiber reinforced high performance concrete[J]. Construction and Building Materials,2014,50:108-115. DOI: 10.1016/j.conbuildmat.2013.09.032
[13] 中国工程建设标准化协会. 纤维混凝土结构技术规程: CECS 38∶2004[S]. 北京: 中国计划出版社, 2005. China Association for Engineering Construction Standardization. Technical specification for fiber reinforced concrete structures: CECS 38∶2004[S]. Beijing: China Planning Press, 2005(in Chinese).
[14] 李秋义, 樊红, 赵景海. 三维乱向分布钢纤维方向效能系数的理论值[J]. 哈尔滨建筑大学学报, 1998, 31(1):83-86. LI Qiuyi, FAN Hong, ZHAO Jinghai. Theoretical value of efficiency coefficient of three dimensional random distribution steel fiber direction[J]. Journal of Harbin University of Civil Engineering and Architecture,1998,31(1):83-86(in Chinese).
[15] 慕儒, 马艳奉, 李辉, 等. 定向钢纤维混凝土中的钢纤维分布X-ray CT分析[J]. 电子显微学报, 2015, 34(6):487-491. DOI: 10.3969/j.issn.1000-6281.2015.06.007 MU Ru, MA Yanfeng, LI Hui, et al. Analysis of the distribution of steel fiber in aligned steel fiber reinforced concrete using digital X-ray CT scanning[J]. Journal of Chinese Electron Microscopy Society,2015,34(6):487-491(in Chinese). DOI: 10.3969/j.issn.1000-6281.2015.06.007
[16] 俞家欢, 刘琼阳. 水泥基复合材料中纤维拉拔的变位约束细观力学模型[J]. 复合材料学报, 2008, 25(5):147-150. DOI: 10.3321/j.issn:1000-3851.2008.05.024 YU Jiahuan, LIU Qiongyang. Meso-scale variable engagement model for single fiber reinforced concrete under uniaxial tension[J]. Acta Materiae Compositae Sinica,2008,25(5):147-150(in Chinese). DOI: 10.3321/j.issn:1000-3851.2008.05.024
[17] 罗洪林, 杨鼎宜, 周兴宇, 等. 不同长径比聚丙烯纤维增强混凝土的力学特性[J]. 复合材料学报, 2019, 36(8):1935-1948. LUO Hongling, YANG Dingyi, ZHOU Xingyu, et al. Mechanical properties of polypropylene fiber reinforced concrete with different aspect ratios[J]. Acta Materiae Compositae Sinica,2019,36(8):1935-1948(in Chinese).
[18] 王力, 徐礼华, 邓方茜, 等. 波纹型钢纤维-混杂纤维混凝土界面粘结性能[J/OL]. 建筑材料学报:1-15 [2020-08-10]. 网址: kns.cnki.net/kcms/detail/31.1764.TU.20190716.1323.009.html. WANG Li, XU Lihua, DENG Fangqian, et al. The bonding performance of corrugated steel fiber-hybrid fiber concrete matrix interface[J]. Journal of Building Materials:1-15 [2020-08-10]. Website: kns.cnki.net/kcms/detail/31.1764.TU.20190716.1323.009.html(in Chinese).
[19] 李秋义, 李家和, 袁杰. SFRC兼有阻裂作用的复合理论[J]. 哈尔滨建筑大学学报, 2002, 35(4):81-83, 107. LI Qiuyi, LI Jiahe, YUAN Jie. Composite theory of SFRC accompanied with action of crack arrest[J]. Journal of Harbin University of Civil Engineering and Architecture,2002,35(4):81-83, 107(in Chinese).
[20] 郑建岚, 郑作樵. 钢纤维钢筋高强混凝土柱的变形与延性计算[J]. 工程力学, 1999, 16(1):56-66. ZHENG Jianlan, ZHENG Zuoqiao. Calculation of deformation and ductility of steel fiber reinforced high strength concrete columns[J]. Engineering Mechanics,1999,16(1):56-66(in Chinese).
-
期刊类型引用(16)
1. 王新玲，李世伟，金蕾蕾，范家俊. 高强不锈钢绞线网/ECC约束高强混凝土受压本构模型. 工程力学. 2023(09): 153-160 . 
百度学术
2. 王新玲，罗鹏程，钱文文，李可. 高强不锈钢绞线网增强工程水泥基复合材料薄板受弯承载力研究. 建筑结构学报. 2022(01): 164-172 . 百度学术
3. 姚淇耀，陆宸宇，彭林欣，滕晓丹，罗月静. 氯盐侵蚀作用下BFRP筋增强海砂ECC的拉伸及弯曲性能试验. 复合材料学报. 2022(03): 1215-1227 . 本站查看
4. 朱俊涛，张凯，邹旭岩，李可，张普. 钢绞线与水泥基复合材料局部黏结滑移模型. 哈尔滨工程大学学报. 2022(04): 541-548 . 百度学术
5. 王新玲，李赟璞，李苗浩夫，范家俊. 高强不锈钢绞线网增强ECC加固RC短柱轴心受压试验. 复合材料学报. 2022(05): 2308-2317 . 本站查看
6. 朱俊涛，张凯，王新玲，李可. 高强钢绞线网增强ECC与混凝土界面黏结-滑移关系研究. 工程力学. 2022(09): 204-214 . 百度学术
7. 李可，王宇，李志强，朱俊涛. 高强钢绞线网增强ECC加固无损RC梁受弯承载力研究. 建筑结构学报. 2022(12): 82-90 . 百度学术
8. 李可，李思辰，周擎威，王新玲. 高强钢绞线与工程水泥基复合材料黏结性能试验. 沈阳工业大学学报. 2022(06): 706-713 . 百度学术
9. 惠迎新，王文炜，朱忠锋. FRP-ECC复合约束混凝土圆柱反复受压力学性能. 复合材料学报. 2022(11): 5586-5598 . 本站查看
10. 王新玲，陈永杰，钱文文，李可，朱俊涛. 高强不锈钢绞线网增强工程水泥基复合材料弯曲性能试验. 复合材料学报. 2021(04): 1292-1301 . 本站查看
11. 王春红，左祺，支中祥，徐磊，SARANI Zakaria，SHERAZ Hussain Siddique Yousfani. 聚乙烯醇乳液改性对汉麻秸秆纤维/水泥基复合材料性能的影响. 复合材料学报. 2021(05): 1567-1575 . 本站查看
12. 朱俊涛，赵奎，邹旭岩，王新玲，李可，张哲. 高强不锈钢绞线网在ECC中的搭接性能. 土木与环境工程学报(中英文). 2021(03): 174-182 . 百度学术
13. 朱俊涛，侯晶强，王新玲，李可. 基于损伤演化的钢绞线网/ECC黏结滑移关系. 华中科技大学学报(自然科学版). 2021(06): 103-108 . 百度学术
14. 范建伟，李可，王新玲. 高性能复合材料“高强钢绞线/ECC”板受弯试验分析. 建筑科学. 2021(09): 130-136 . 百度学术
15. 王新玲，卫垚鑫，范建伟，朱俊涛. 新型复合材料“高强钢绞线网/ECC约束素混凝土”受压性能试验研究. 复合材料学报. 2021(11): 3904-3911 . 本站查看
16. 王新玲，赵要康，周擎威，朱俊涛. 高强不锈钢绞线与ECC黏结滑移性能研究. 华中科技大学学报(自然科学版). 2020(12): 108-113 . 百度学术
其他类型引用(2)
-
计量
- 文章访问数: 1252
- HTML全文浏览量: 441
- PDF下载量: 36
- 被引次数: 18
