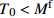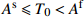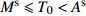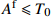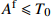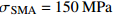形状记忆合金在复合材料损伤监测中的应用

张亚楠; 刘亚冬; 刘兵飞

doi:10.13801/j.cnki.fhclxb.20201111.001

形状记忆合金在复合材料损伤监测中的应用

1.
中国民航大学　机场学院，天津 300300
2.
中国民航大学　中欧航空工程师学院，天津 300300
3.
中国民航大学　航空工程学院，天津 300300

基金项目: 国家自然科学基金(11502284)；中科院重点部署项目(KFZD-SW-435)；中央高校基金(3122015C019)

详细信息

通讯作者:
刘兵飞，博士，副教授，硕士生导师，研究方向为智能材料与结构力学　 E-mail：bingfeiliu2@126.com

中图分类号: TG139.6；TB33
计量
- 文章访问数: 01281
- HTML全文浏览量: 0414
- PDF下载量: 094
出版历程
- 收稿日期: 2020-09-02
- 录用日期: 2020-10-27
- 网络出版日期: 2020-11-10
- 刊出日期: 2021-04-07

Application of shape memory alloy in damage monitoring of composite materials

1.
Airport College, Civil Aviation University of China, Tianjin 300300, China
2.
Sino-European Institute of Aviation Engineering, Civil Aviation University of China, Tianjin 300300, China
3.
Faculty of Aerospace Engineering, Civil Aviation University of China, Tianjin 300300, China

摘要

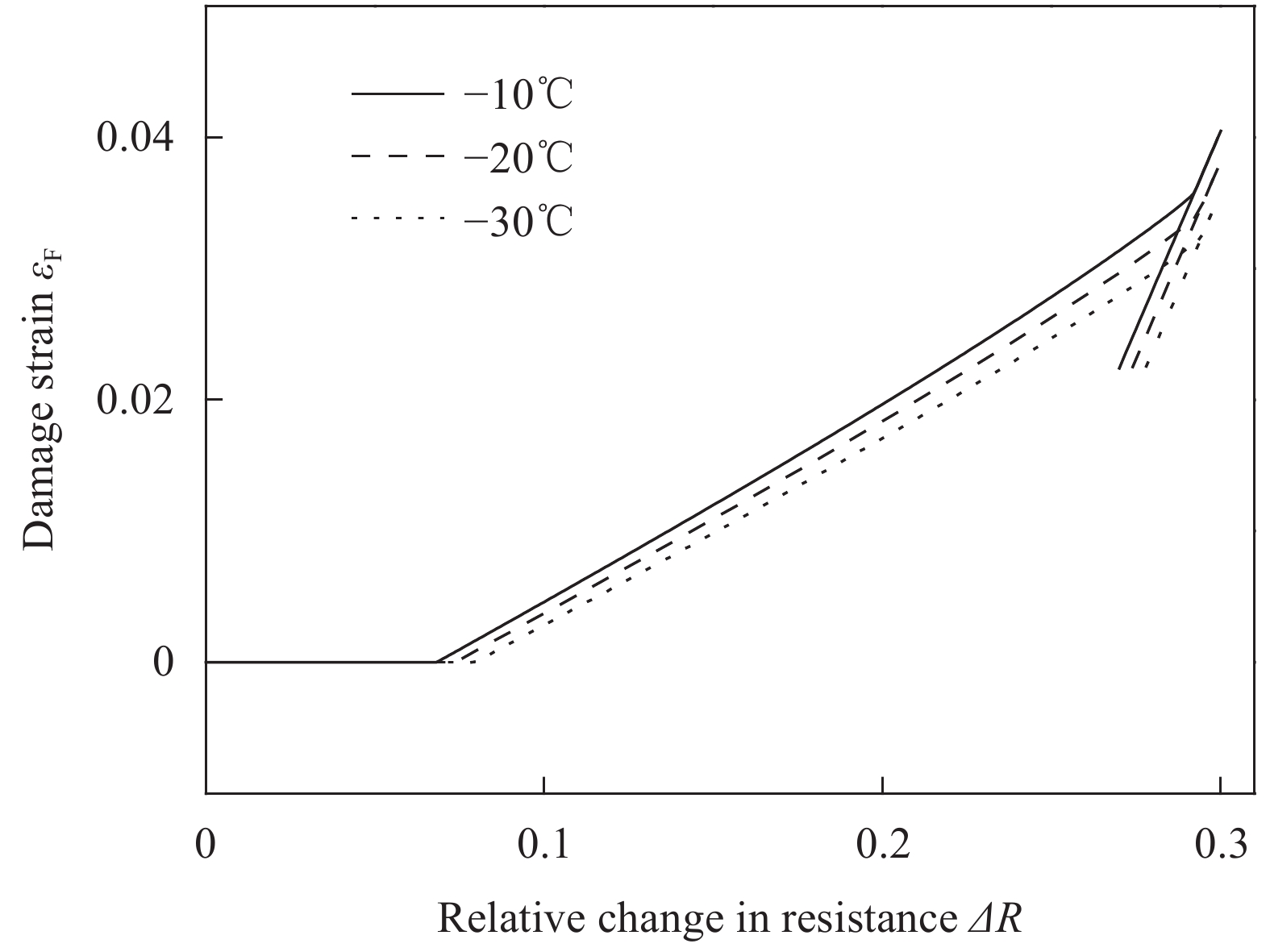
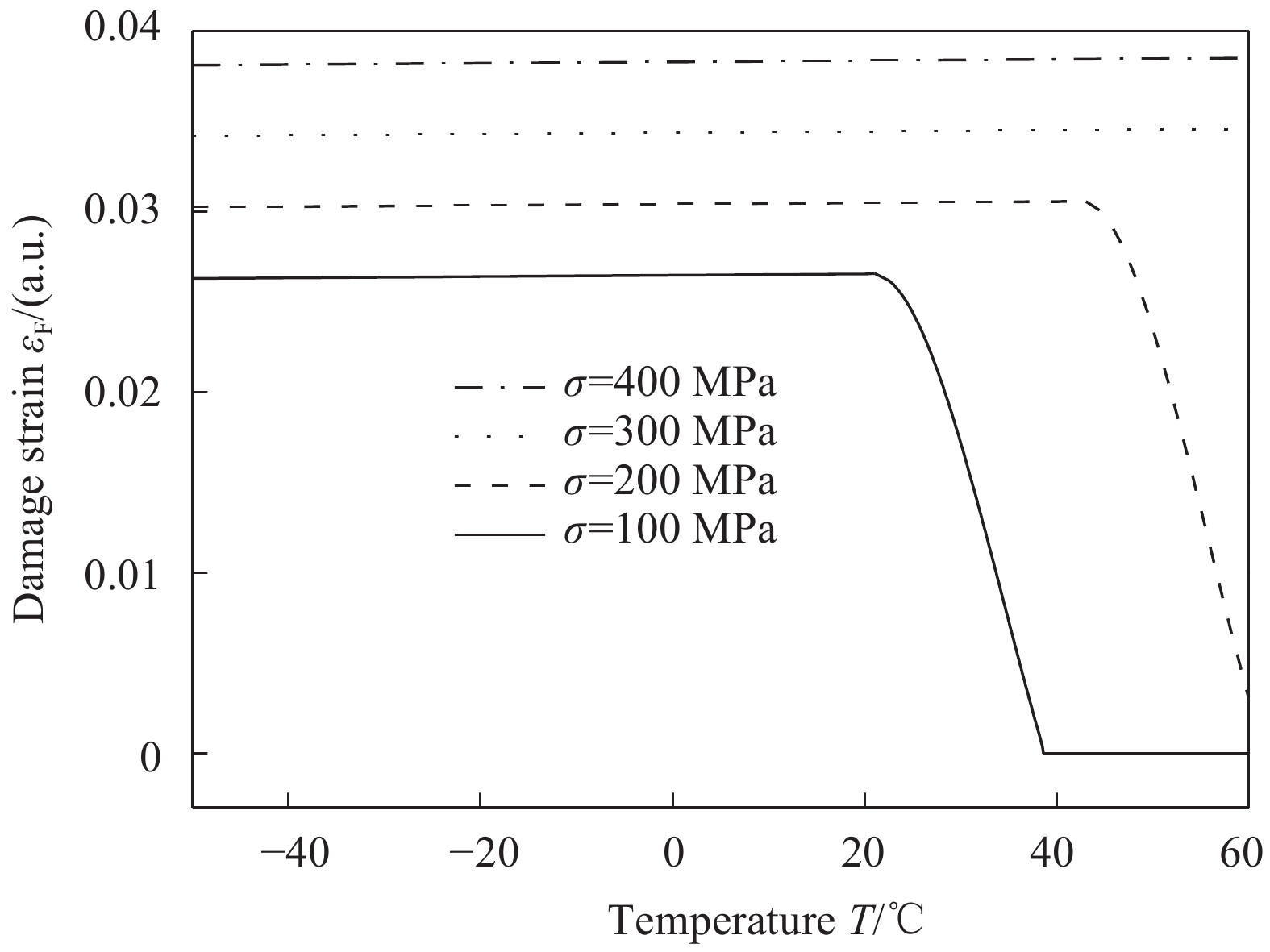
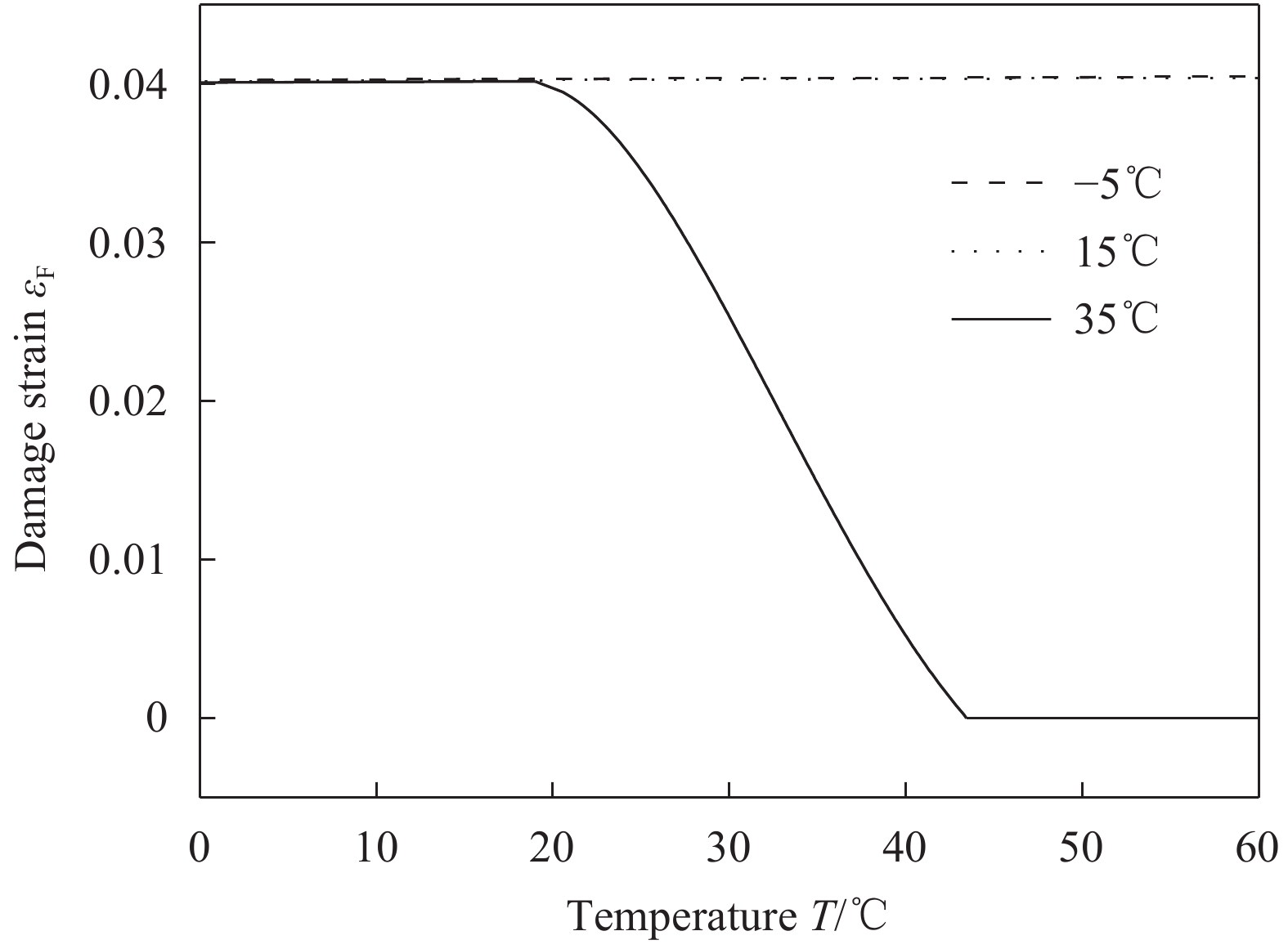
摘要: 复合材料的损伤会对结构的可靠性和安全性造成威胁，近年来引起国内外专家和学者的广泛关注。本文将形状记忆合金(SMA)埋入复合材料试件中，通过对SMA电阻变化与复合材料应变之间的关系进行讨论，建立不同监测条件下复合材料的损伤监测理论模型。基于该模型讨论了不同初始状态下SMA材料的损伤监测行为。研究结果表明：复合材料的损伤与SMA电阻变化呈线性关系，温度荷载在SMA未发生相变时对损伤监测影响较小，在SMA发生相变时对损伤监测影响较大。本研究可为基于SMA的复合材料损伤监测理论的进一步工程应用提供理论指导。
- 形状记忆合金 /
- 复合材料 /
- 损伤监测 /
- 变温 /
- 相变
Abstract: The damage of composite materials poses a threat to the reliability and safety of the structure, and has attracted widespread attention from domestic and foreign experts and scholars in recent years. This study embeded the shape memory alloy (SMA) in the composite material specimen, and discussed the relationship between the SMA resistance change and the composite material strain, established theoretical models of composite damage monitoring under different monitoring conditions. Based on this model, the damage monitoring behavior of SMA materials in different initial states was discussed. The research results show that the damage of the composite material has a linear relationship with the resistance change of the SMA. The temperature load has little influence on the damage monitoring when the SMA does not undergo a phase change, and has a greater influence on the damage monitoring when the SMA undergoes a phase change. The research can provide theoretical guidance for the further engineering application of SMA-based composite damage monitoring theory.
- shape memory alloy /
- composite materials /
- damage monitoring /
- change temperature /
- phase tran-sition

HTML全文

图 1 埋入形状记忆合金(SMA)的复合材料示意图

Figure 1. Schematic diagram of composite material embedded with shape memory alloys (SMA)

下载: 全尺寸图片幻灯片

图 2 对低温马氏体相升温过程中 (a) 和高温奥氏体相降温过程中 (b) 相变或重定向临界应力与温度之间的关系

Figure 2. Relationship between phase transformation or reorientation critical stress and temperature during the heating process of low-temperature martensite phase (a) cooling process of high-temperature austenite phase (b)

下载: 全尺寸图片幻灯片

图 3 ${T_0} < {M^{\rm{f}}}$ 时SMA损伤应变与电阻相对变化的关系

Figure 3. Relationship between the damage strain and the relative change of resistance at ${T_0} < {M^{\rm{f}}}$ for SMA

下载: 全尺寸图片幻灯片

图 4 ${A^{\rm{s}}} \leqslant {T_0} < {A^{\rm{f}}}$ 时损伤应变与电阻相对变化的关系

Figure 4. Relationship between the damage strain and the relative change of resistance at ${A^{\rm{s}}} \leqslant {T_0} < {A^{\rm{f}}}$

下载: 全尺寸图片幻灯片

图 5 ${M^{\rm{s}}} \leqslant {T_0} < {A^{\rm{s}}}$ 时SMA损伤应变与电阻相对变化的关系

Figure 5. Relationship between the damage strain and the relative change of resistance at ${M^{\rm{s}}} \leqslant {T_0} < {A^{\rm{s}}}$ for SMA

下载: 全尺寸图片幻灯片

图 6 ${A^{\rm{f}}} \leqslant {T_0}$ 时SMA损伤应变与电阻相对变化的关系

Figure 6. Relationship between the damage strain and the relative change of resistance at ${A^{\rm{f}}} \leqslant {T_0}$ for SMA

下载: 全尺寸图片幻灯片

图 7 ${T}_{0}={\rm{-}}80$ ℃时不同应力下SMA损伤应变与温度的关系

Figure 7. Relationship between damage strain and temperature under different stresses and ${T}_{0}={\rm{-}}80$ ℃ for SMA

下载: 全尺寸图片幻灯片

图 8 ${\sigma _{{\rm{SMA}}}} = 150\;{\rm{MPa}}$ 时不同初始温度下SMA损伤应变与温度的关系

Figure 8. Relationship between damage strain and temperature at different initial temperatures and ${\sigma _{{\rm{SMA}}}} = 150\;{\rm{MPa}}$ for SMA

下载: 全尺寸图片幻灯片

图 9 ${T}_{0}={\rm{-}}5$ ℃时加载阶段不同应力下SMA损伤应变与温度的关系

Figure 9. Relationship between damage strain and temperature under different stresses during loading stage and ${T}_{0}={\rm{-}}5$ ℃ for SMA

下载: 全尺寸图片幻灯片

图 10 ${T}_{0}={\rm{-}}5$ ℃时卸载阶段不同应力下SMA损伤应变与温度的关系

Figure 10. Relationship between damage strain and temperature under different stresses during unloading stage and ${T}_{0}={\rm{-}}5$ ℃ for SMA

下载: 全尺寸图片幻灯片

图 11 ${\sigma _{{\rm{SMA}}}} = 450\;{\rm{MPa}}$ 时加载阶段不同初始温度下SMA损伤应变与温度的关系

Figure 11. Relationship between damage strain and temperature at different initial temperatures in the loading stage and ${\sigma _{{\rm{SMA}}}} = 450\;{\rm{MPa}}$ for SMA

下载: 全尺寸图片幻灯片

图 12 ${\sigma _{{\rm{SMA}}}} = 300\;{\rm{MPa}}$ 时卸载阶段不同初始温度下SMA损伤应变与温度的关系

Figure 12. Relationship between damage strain and temperature at different initial temperatures during unloading stage and ${\sigma _{{\rm{SMA}}}} = 300\;{\rm{MPa}}$ for SMA

下载: 全尺寸图片幻灯片

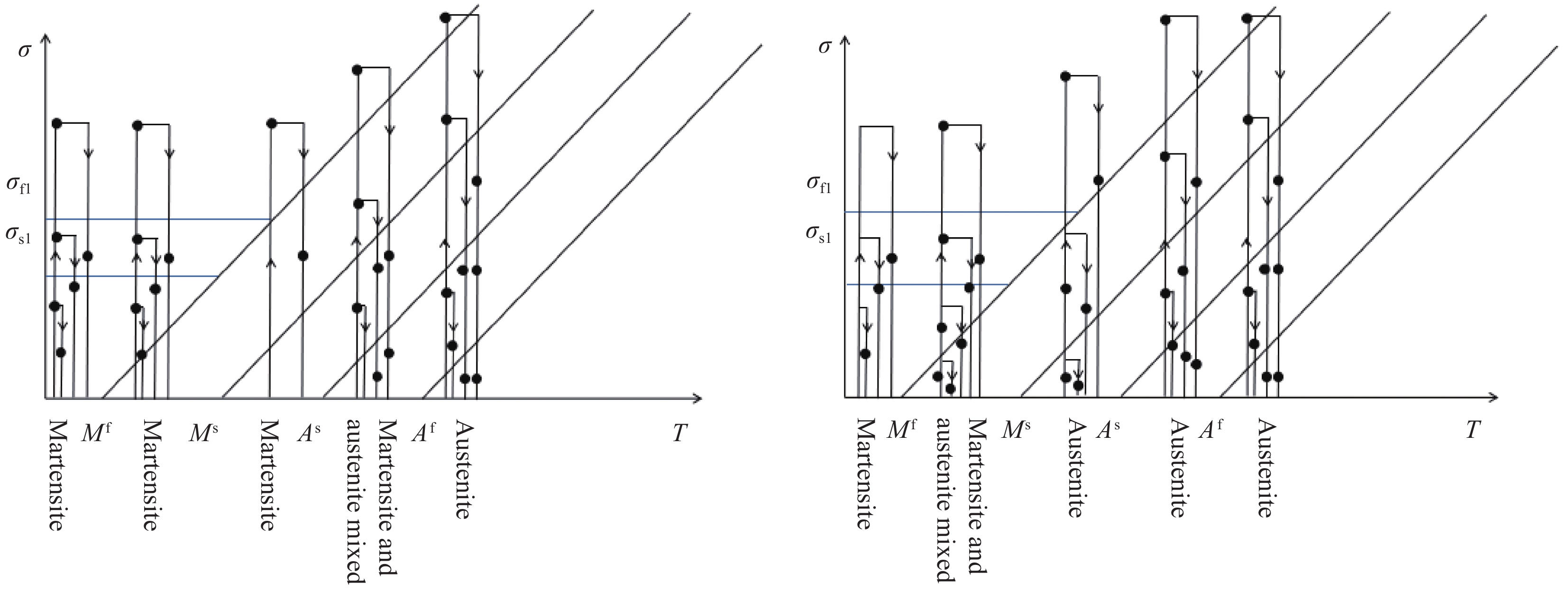
表 1 对初始状态为低温马氏体相的SMA升温过程中的情况分类

Table 1 Classification of the conditions during the heating process of the SMA whose initial state is low-temperature martensite phase

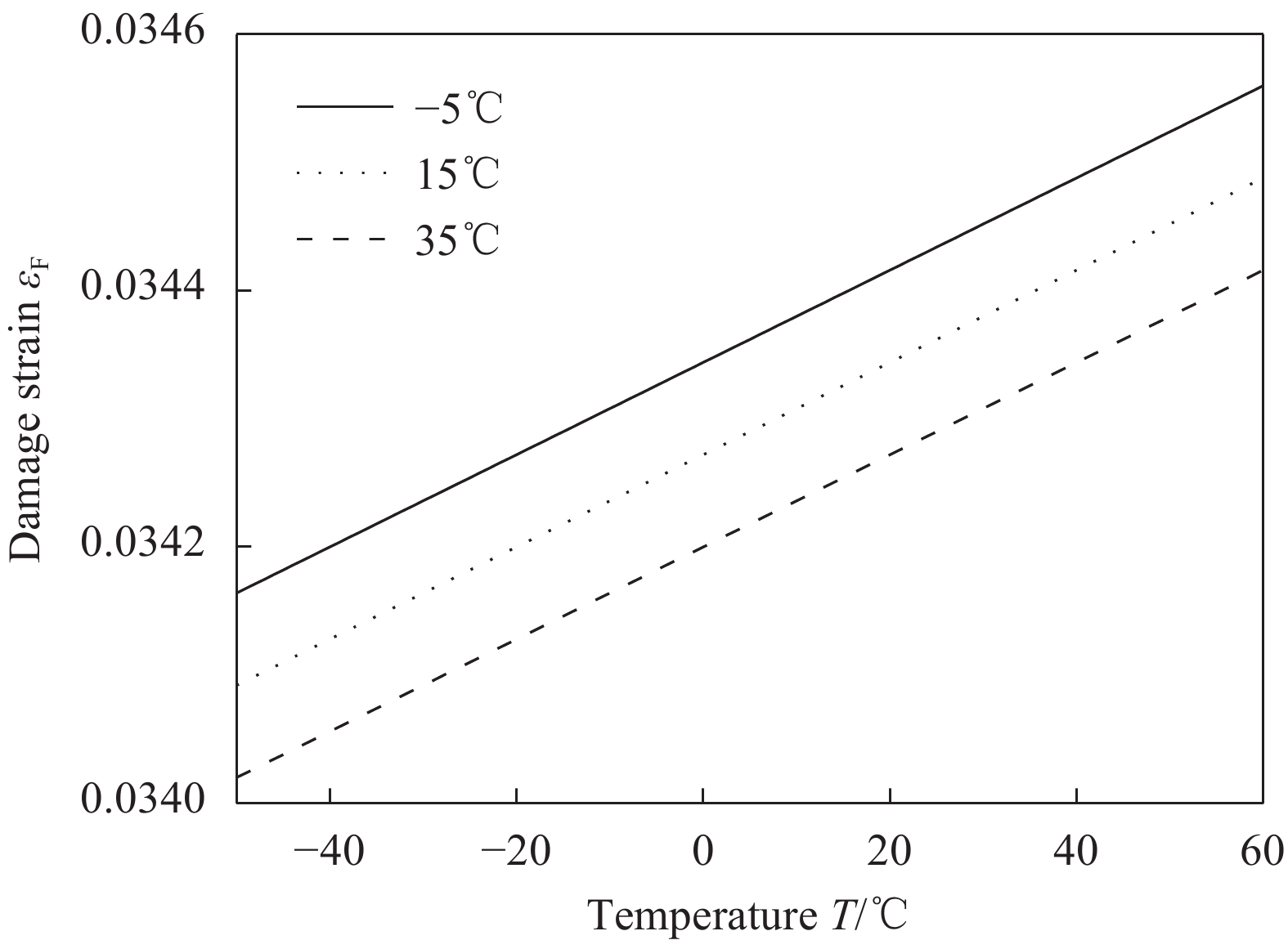
Temperature section	SMA initial state	Loading phase	Composite material	Uninstall phase
${T_0} < {M^{\rm{f}}}$	Martensite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s1}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
			Damaged
		${\sigma _{{\rm{s1}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f1}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${M^{\rm{f}}} \leqslant {T_0} < {M^{\rm{s}}}$	Martensite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s1}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
			Damaged
		${\sigma _{{\rm{s1}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f1}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${M^{\rm{s}}} \leqslant {T_0} < {A^{\rm{s}}}$	Martensite	$0 \leqslant {\sigma _{{\rm{SMA}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
${M^{\rm{s}}} \leqslant {T_0} < {A^{\rm{s}}}$	Martensite	$0 \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
${A^{\rm{s}}} \leqslant {T_0} < {A^{\rm{f}}}$	Martensite and austenite mixed	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s4}}}}$	No damage	${\sigma _{{\rm{SMA}}}} \geqslant {\sigma _{{\rm{As4}}}}$		$0 \leqslant {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{As4}}}}$
			Damaged
		${\sigma _{{\rm{s4}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f4}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f4}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f4}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${A^{\rm{f}}} \leqslant {T_0}$	Austenite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s5}}}}$	No damage	$\begin{array}{l} {\sigma _{{\rm{SMA}}}} \\ \geqslant {\sigma _{{\rm{As5}}}} \end{array}$	$\begin{array}{l} {\sigma _{{\rm{Af5}}}} < {\sigma _{{\rm{SMA}}}} \\ < {\sigma _{{\rm{As5}}}} \end{array}$		$\begin{array}{l} 0 \leqslant {\sigma _{{\rm{SMA}}}} \\ \leqslant {\sigma _{{\rm{Af5}}}} \end{array}$
			Damaged
		${\sigma _{{\rm{s5}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f5}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f5}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f5}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
Notes: M^f−Martensitic transformation completion temperature; M^s−Martensitic transformation start temperature; A^s−Austenite transformation start temperature; A^f−Austenite transformation completion temperature; σ_SMA—Stress of the SMA; ${\sigma _{{\rm{s1}}}},{\sigma _{{\rm{f1}}}}$ —SMA begins and completes redirection to critical stress; ${\sigma _{{\rm{f2}}}}$ —SMA completes the critical stress of martensitic transformation in M^f≤T₀＜M^s; ${\sigma _{{\rm{s3}}}},{\sigma _{{\rm{f3}}}}$ —Critical stress for the initiation and completion of martensitic transformation in M^f≤T₀＜A^s of SMA; ${\sigma _{{\rm{s4}}}},{\sigma _{{\rm{f4}}}}$ , ${\sigma _{{\rm{As}}4}},{\sigma _{{\rm{Af4}}}}$ —SMA begins and completes martensitic transformation and austenitic transformation in A^s≤T₀＜A^f section; ${\sigma _{{\rm{s5}}}},{\sigma _{{\rm{f5}}}},{\sigma _{{\rm{As5}}}},{\sigma _{{\rm{Af5}}}}$ —SMA begins and completes martensitic transformation and austenitic transformation in A^f≤T₀ section.

下载: 导出CSV

表 2 对初始状态为高温奥氏体相的SMA降温过程中的情况分类

Table 2 Classification of the conditions during the cooling process of the SMA whose initial state is high-temperature austenite phase

Temperature section	SMA initial state	Loading phase	Composite material	Uninstall phase
${T_0} < {M^{\rm{f}}}$	Martensite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s1}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
			Damaged
		${\sigma _{{\rm{s1}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f1}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f1}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${M^{\rm{f}}} \leqslant {T_0} < {M^{\rm{s}}}$	Martensite and austenite mixed	$0 \leqslant {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f2}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
			Damaged
		${\sigma _{{\rm{f2}}}} \leqslant {\sigma _{{\rm{SMA}}}} < \sigma '_{{\rm{s2}}}$	No damage
			Damaged
		$\sigma' _{{\rm{s2}}} \leqslant {\sigma _{{\rm{SMA}}}} < \sigma' _{{\rm{f2}}}$	No damage
			Damaged
		$\sigma' _{{\rm{f2}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		$\sigma' _{{\rm{f2}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${M^{\rm{s}}} \leqslant {T_0} < {A^{\rm{s}}}$	Austenite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s3}}}}$	No damage	$0 \leqslant {\sigma _{{\rm{SMA}}}}$
			Damaged
		${\sigma _{{\rm{s3}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f3}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f3}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f3}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${A^{\rm{s}}} \leqslant {T_0} < {A^{\rm{f}}}$	Austenite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s4}}}}$	No damage	${\sigma _{{\rm{SMA}}}} \geqslant {\sigma _{{\rm{As4}}}}$		$0 \leqslant {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{As4}}}}$
			Damaged
		${\sigma _{{\rm{s4}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f4}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f4}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f4}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged
${A^{\rm{f}}} \leqslant {T_0}$	Austenite	$0 \leqslant {\sigma _{{\rm{SMA}}}} \leqslant {\sigma _{{\rm{s5}}}}$	No damage	$\begin{array}{l} {\sigma _{{\rm{SMA}}}} \\ \geqslant {\sigma _{{\rm{As5}}}} \end{array}$	$\begin{array}{l} {\sigma _{{\rm{Af5}}}} < {\sigma _{{\rm{SMA}}}} \\ < {\sigma _{{\rm{As5}}}} \end{array}$		$\begin{array}{l} 0 \leqslant {\sigma _{{\rm{SMA}}}} \\ \leqslant {\sigma _{{\rm{Af5}}}} \end{array}$
			Damaged
		${\sigma _{{\rm{s5}}}} < {\sigma _{{\rm{SMA}}}} < {\sigma _{{\rm{f5}}}}$	No damage
			Damaged
		${\sigma _{{\rm{f5}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	No damage
		${\sigma _{{\rm{f5}}}} \leqslant {\sigma _{{\rm{SMA}}}}$	Damaged

下载: 导出CSV

表 3 与玻璃纤维/不饱和树脂和SMA有关的材料参数^{[16, 18-19]}

Table 3 Material parameters related to glass fiber/unsaturated resin and SMA^{[16, 18-19]}

M^f/℃	M^s/℃	A^s/℃	A^f/℃	E^A/MPa	E^M/MPa	${\alpha ^{\rm{A}}}$ /℃⁻¹	${\alpha ^{\rm{M}}}$ /℃⁻¹	H/%
−85	−52	0.2	28.4	60 000	25 400	1.1×10⁻⁵	6.6×10⁻⁶	3.5
E_G/MPa	C(MPa·℃⁻¹)	${\sigma _{\rm{G}}}$ /MPa	$\sigma _{\rm{s}}^{{\rm{cr}}}$ /MPa	$\sigma _{\rm{f}}^{{\rm{cr}}}$ /MPa	${\alpha_{\rm{G}} }$ /℃⁻¹
33 400	5	417.1	100	170	3×10⁻⁶
Notes: E^A—Elastic modulus of SMA austenite; E^M—Elastic modulus of SMA martensite; ${\alpha ^{\rm{A}}}$ —Thermal expansion coefficient of SMA austenite; ${\alpha ^{\rm{M}}}$ —Thermal expansion coefficient of SMA martensite; H—Maximum recoverable residual strain; E_G—Elastic modulus of glass fiber unsaturated resin; C—Stress influence factor; ${\sigma _{\rm{G}}}$ —Stress on glass fiber unsaturated resin; $\sigma _{\rm{s}}^{{\rm{cr}}}$ —SMA reorientation starts critical stress; $\sigma _{\rm{f}}^{{\rm{cr}}}$ —SMA reorientation ends critical stress; ${\alpha_{\rm{G}} }$ —Thermal expansion coefficient of glass fiber unsaturated resin.

下载: 导出CSV

表 4 与电阻率有关的材料参数^[20]

Table 4 Material parameters related to resistivity^[20]

${C_{{\rm{M1}}}}$ /(Ω·m·℃⁻¹)	${C_{{\rm{M2}}}}$ /(Ω·m)	${C_{{\rm{A1}}}}$ /(Ω·m·℃⁻¹)	${C_{{\rm{A2}}}}$ /(Ω·m)
7×10⁻¹⁰	0.87×10⁻⁶	8×10⁻¹⁰	0.72×10⁻⁶
Note: ${C_{{\rm{M1}}}}$ , ${C_{{\rm{M2}}}}$ , ${C_{{\rm{A1}}}}$ , ${C_{{\rm{A2}}}}$ —Material parameters related to resistivity.

下载: 导出CSV

参考文献(20)

[1]	杜善义. 先进复合材料与航空航天[J]. 复合材料学报, 2007, 24(1):1-12. DOI: 10.3321/j.issn:1000-3851.2007.01.001 DU S Y. Advanced composite materials and aerospace engineering[J]. Acta Materiae Compositae Sinica,2007,24(1):1-12(in Chinese). DOI: 10.3321/j.issn:1000-3851.2007.01.001
[2]	WU F, YAO W X. A fatigue damage model of composite materials[J]. International Journal of Fatigue,2010,32(1):134-138. DOI: 10.1016/j.ijfatigue.2009.02.027
[3]	EI-SABBAGH A, STEUERNAGEL L, ZIEGMANN G. Characterisation of flax polypropylene composites using ultrasonic longitudinal sound wave technique[J]. Composites: Part B,2013,45:1164-1172. DOI: 10.1016/j.compositesb.2012.06.010
[4]	FREEMANTLE R J, HANKINSON N, BROTHERHOOD C J. Rapid phased array ultrasonic imaging of large area composite aerospace structures[J]. Insight,2005,47(3):129-132. DOI: 10.1784/insi.47.3.129.61315
[5]	RYU C H, PARK S H, KIM D H, et al. Nondestructive evaluation of hidden multi-delamination in a glass-fiber-reinforced plastic composite using terahertz spectroscopy[J]. Composite Structures,2016,156:338-347. DOI: 10.1016/j.compstruct.2015.09.055
[6]	HOSOI A, YAMAGUCHI Y, JU Y, et al. Detection and quantitative evaluation of defects in glass fber reinforced plastic laminates by microwaves[J]. Composite Structures, 2015, 128: 134-144.
[7]	KALYANAVALLI V, ABILASHA R T K, SASTIKUMAR D. Long pulse thermography investigations of basalt fiber reinforced composite[J]. NDT & E International,2018,100:84-91.
[8]	周玉敬, 任明伟, 刘刚, 等. 基于FBG传感技术的复合材料T型加筋板低速冲击损伤监测[J]. 复合材料学报, 2019, 36(10):2266-2274. ZHOU Y J, REN M W, LIU G, et al. Low-velocity impact damage monitoring of composite T-stiffened panels based on FBG sensors[J]. Acta Materiae Compositae Sinica,2019,36(10):2266-2274(in Chinese).
[9]	ZHAO H T, ZHANG B M, WU Z J, et al. Infrastructure study for optical fiber grating in smart composite materials[J]. Transducer and Microsystem Technologies,2007,26(12):27-30.
[10]	KAN Q, KANG G. Constitutive model for uniaxial transformation ratchetting of super-elastic NiTi shape memory alloy at room temperature[J]. International Journal of Plasticity,2010,26(3):441-465. DOI: 10.1016/j.ijplas.2009.08.005
[11]	SUN Q P, HWANG K C. Micromechanics modeling for the constitutive behavior of polycrystalline shape memory alloy[J]. Journal of the Mechanics and Physics of Solids,1993,41(1):1-33. DOI: 10.1016/0022-5096(93)90060-S
[12]	WU X D, FAN Y Z, WU J S. A study on the variations of the electrical resistance for NiTi shape memory alloy wires during the thermo-mechanical loading[J]. Materials & Design,2000,21(6):511-515.
[13]	狄生奎, 韩全治, 李慧, 等. SMA在结构健康监测中的应用研究[J]. 低温建筑技术, 2008(4):58-60. DOI: 10.3969/j.issn.1001-6864.2008.04.027 DI S K, HAN Q Z, LI H, et al. Research on the shape memory alloy applied in structural health monitoring[J]. Low Temperature Architecture Technology,2008(4):58-60(in Chinese). DOI: 10.3969/j.issn.1001-6864.2008.04.027
[14]	狄生奎, 花尉攀, 汲生伟, 等. 约束态SMA混凝土梁的裂缝监测及自修复[J]. 建筑材料学报, 2010, 13(2):237-242. DOI: 10.3969/j.issn.1007-9629.2010.02.021 DI S K, HUA W P, JI S W, et al. Self-monitoring and self-repairing of crack in concrete beam with constraint super-elastic SMA[J]. Journal of Building Materials,2010,13(2):237-242(in Chinese). DOI: 10.3969/j.issn.1007-9629.2010.02.021
[15]	袁江, 邱自学, 邵建新, 等. 基于无线射频识别传感标签的形状记忆合金增强复合结构低速冲击响应监测[J]. 机械工程学报, 2012, 48(18):89-96. DOI: 10.3901/JME.2012.18.089 YUAN J, QIU Z X, SHAO J X, et al. Low speed impact test for shape memory alloy-reinforced composite structure based on radio frequency idebtification sensortags[J]. Journal of Mechanical Engineering,2012,48(18):89-96(in Chinese). DOI: 10.3901/JME.2012.18.089
[16]	王庆菲. SMA智能混凝土的裂缝监测与自修复理论研究[D]. 天津: 中国民航大学. 2020. WANG Q F. Theoretical research on crack monitoring and self-repairing mechanism for SMA intelligent concrete materials[D]. Tianjin: Civil Aviation University of China, 2020(in Chinese).
[17]	IKUTA K, TSUKAMOTO M, HIROSE S. Institute of electric and electronic engineer. mathematical model and experimental verification of shape memory alloy for designing micro actuator[C]//Micro Electro Mechanical Systems, 1991, MEMS '91, Proceedings. An Investigation of Micro Structures, Sensors, Actuators, Machines and Robots. IEEE. 1991: 103-108.
[18]	BRINSON L C. One-dimensional constitutive behavior of shape memory alloys: Thermomechanical derivation with non-constant material functions and redefined martensite internal variable[J]. Journal of Intelligent Material Systems and Structures,1993,4(2):229-242. DOI: 10.1177/1045389X9300400213
[19]	詹瑒. 纤维增强复合材料(FRP)格构柱基本性能研究[D]. 南京: 东南大学. 2016. ZHAN Y. Performance evaluation of lattice columns using pultruded fiber-reinforced polymer(FRP) structurak profiles[D]. Nanjing: Southeast University, 2016(in Chinese).
[20]	崔迪, 李宏男, 宋钢兵. NiTi形状记忆合金电阻特性研究[J]. 建筑材料学报, 2008(5):68-74. CUI D, LI H N, SONG G B. Study on electrical resistance properties of NiTi shape memory alloy[J]. Journal of Building Materials,2008(5):68-74(in Chinese).

施引文献(6)

期刊类型引用(2)

1.	李滋阳，王思佳，邓文举. 陶瓷颗粒增强金属基复合材料研究进展. 轻工科技. 2021(04): 41-44 . 百度学术
2.	陈亚楠，金云学，牛牧野，陈洪美，杜文栋. Ni_3Al(Cr)合金室温干滑动摩擦磨损性能研究. 江苏科技大学学报(自然科学版). 2019(05): 18-26 . 百度学术