Research on composite mechanical properties of larch under perpendicular-to-grain tension/compression and transversal shear
-
摘要:
实际木结构中木材大多同时受到顺纹正应力与横向剪应力复合作用，掌握其复合受力性能是木结构受力分析的重要基础。研发并制作了一种用于测试木材拉/压-剪切复合受力性能的杠杆加载装置与试件，基于此开展了木材顺纹拉/压、横纹剪切以及顺纹正应力与横向剪应力复合加载试验，其中，剪切荷载由单轴试验机提供、拉/压加载由杠杆装置实现。考察了不同拉/压-剪切应力复合作用下木材试件的破坏形态、应力-应变曲线、拉/压-剪切复合强度特性，进一步考察了经典正交各向异性强度准则对落叶松材拉/压-剪切复合受力强度的表征效果，结果表明，Hill准则、Hasebe准则的适用性最好。研究结果可为木结构的精细化受力分析提供重要借鉴。
Abstract:The majority of wood within the actual wooden structure experiences a combination of normal stress along the grain and transverse shear stress. A lever-loading device and specimen were developed and manufactured to assess the composite mechanical properties of wood under tension/compression and shear stress. Experiments were conducted to evaluate longitudinal tension/compression, transverse shear, longitudinal normal stress, and transverse shear stress under combined loading conditions. The uniaxial testing machine supplied the shear load, while the lever device facilitated tension/compression loading. The investigation delved into failure modes, stress-strain curves, and composite strength characteristics of wood specimens under various normal-shear combined stresses. Furthermore, the study explored the efficacy of classical orthotropic strength criteria in determining the normal-shear composite strength of wood. Results indicate that Hill criterion and Hasebe criterion exhibited superior performance.These findings serve as crucial reference for the precise stress analysis of wooden structures.
-
可再生能源的间隙性和波动性为能量的安全、高效利用带来较大挑战，已经成为经济可持续发展面临的重要议题[1]。钒液流电池(Vanadium liquid flow battery，VRB)可将电能高效储存，具有本征安全、响应速度快和运行寿命长等特点，相对于其他类型储能技术，钒电池技术在大规模、长时储能领域显示出优越的发展前景[1-3]。
作为钒电池的关键部件，质子交换膜(Proton exchange membrane，PEM)需具有如下特性[4-5]：(1)高离子选择性，即高质子传导率和低钒离子渗透率；(2)高拉伸强度和穿刺强度；(3)良好的理化稳定性；(4)较好的经济性。目前，基于全氟磺酸树脂的PEM在VRB中的应用最为广泛，如杜邦公司Nafion系列膜，但其溶胀性和离子选择性等与VRB的严格要求仍存在差距，优化膜的离子渗透性是本领域的研究热点[6-8]。实验表明，向膜中引入具有适宜孔道结构和表面性质的功能材料是改善其离子选择性的有效手段[5]，如金属-有机框架(Metal-organic frameworks，MOFs)材料[9-11]。
在MOFs材料中，UIO-66 (Zr-MOF)由六核氧化锆簇作为二级构建单元和1, 4-苯二甲酸接头构建[12]，具有刚性结构和较强的耐酸性，同时拥有介于水和离子(＜0.3 nm)和钒离子(＞0.6 nm)的孔道尺寸[13]。UIO-66主要通过水热合成法制备，但在合成过程中，烘箱加热较难提供均匀的受热环境，存在合成时间长、材料结构均一性差等问题[14]。微波加热可促进反应物中分子或离子直接耦合，实现能量的快速传导，具有反应速率快和产率高等优点，已被广泛用于多种纳米材料的合成[14-16]。Zhai等[6]通过在磺化聚醚醚酮(SPEEK)中掺入15wt% 的传统水热法UIO-66-NH2来改善复合膜的质子传导率，在120 mA·cm−2电流密度下，电池的能量效率(Energy efficiency，EE)为77.3%。Lu等[17]制备高质子选择性的聚多巴胺(PDA)@MOF-808，并将其掺入SPEEK中以优化复合膜的性能，在120 mA·cm−2电流密度下，该膜的EE为83.9%。贾儒等[13]和Wan等[14]也分别对UIO-66的合成和应用展开研究，均采用耗时较长的传统水热法。
鉴于此，本文采用不同加热方式制备氨基官能化的UIO-66 (UIO-66-NH2)，验证微波加热在UIO-66-NH2材料的合成效率、结构优化方面的优势，并将其与Nafion 掺杂，通过溶液浇筑法制备复合膜，对膜的理化性质和电池性能进行表征，探讨新型膜材料对钒电池性能的影响。
1. 实验部分
1.1 原材料
氯化锆(ZrCl4，98%)、2-氨基对苯二甲酸(BDC-NH2，99%)，上海麦克林生化科技有限公司；Nafion溶液(固含量5%)，美国杜邦公司；无水乙醇，沈阳试剂二厂；N, N-二甲基甲酰胺(DMF)、MgSO4、硫酸氧钒(VOSO4·5H2O)、浓硫酸(98%)、乙酸(99%)，国药集团化学试剂有限公司。
1.2 UIO-66-NH2的制备
UIO-66-NH2合成原料摩尔比：ZrCl4∶BDC-NH2 = 1∶1。首先，将二者分别溶于20 mL DMF，再在配体溶液中加入2.3 mL乙酸，超声波辅助下混合20 min后得均匀的合成液。随后，将适量上述合成液倒入微波合成罐中，在不同温度下合成15 min，离心后获得棕黄色粉末。将上述粉末分散在无水乙醇中，多次更新乙醇下保持48 h，完成产物清洗。最后，在60℃下干燥12 h，获得产物UIO-66-NH2，记为M-U66-NH2。作为对比，采用同样的合成液，利用传统烘箱加热，在120℃条件下反应24 h制备UIO-66-NH2，经过相同的清洗、干燥处理，获得产物UIO-66-NH2，记为T-U66-NH2。具体操作流程和样品编号见图1和表1。
1.3 复合质子交换膜的制备
以溶液浇筑法制备复合膜[18]：称取一定质量的M-U66-NH2，在超声辅助下分散在Nafion树脂溶液(固含量5%)中，在450 r/min下搅拌10 h后获得铸膜液。将适量铸膜液倒入带有凹槽的玻璃板上，在140℃真空烘箱中处理4 h，除去溶剂后得到复合膜，在恒温、恒湿条件下保存、备用。将掺杂M-U66-NH2的复合膜记作M/N-X，X=1、2、3、6和9，X表示Nafion树脂中M-U66-NH2的质量分数。将掺杂T-U66-NH2的复合膜记作T/N-X，X=3。同时，采用相似工艺制备无M-U66-NH2掺杂的纯树脂膜，记作P-N。具体操作流程和样品编号见图1和表1。
![]()
图 1 微波合成氨基官能化的UIO-66 (UIO-66-NH2)及Nafion复合膜的制备过程示意图Figure 1. Schematic diagram of the preparation process of UIO-66-NH2 and Nafion composite membraneM-U66-NH2—UIO-66-NH2 prepared by microwave assisted method; UIO-66-NH2—Zr-MOF when the preparation method does not need to be distinguished; BDC-NH2—2-aminoterephthalic Acid; DMF—N, N-dimethylformamide; HAc—Acetic acid表 1 复合质子交换膜的命名Table 1. Naming of composite proton exchange membranesSample name Instruction M-U66-NH2 "M" represents microwave heating; "U66" refers to the metal-organic framework material UIO-66; Overall, it indicates the UIO-66-NH2 sample prepared by microwave heating. T-U66-NH2 "T" represents traditional oven heating; Overall, it indicates the UIO-66-NH2 sample prepared by oven heating. M/N-X "M/N" represents the composite membrane with M-U66-NH2 and Nafion resin; "X" represents the percentage content of M-U66-NH2 in the membrane. T/N-X "T/N" represents the composite membrane with T-U66-NH2 and Nafion resin; "X" represents the percentage content of T-U66-NH2 added to the membrane. P-N A pure, unmodified Nafion membrane N212 Commercial Nafion 212 membrane from DuPont (USA) 1.4 形貌、结构表征和性能测试
采用X射线衍射仪(XRD，Bruker D8)和傅里叶红外光谱(FTIR，Nicolet 380，扫描范围：
4000 ~750 cm−1)评价粉末结构；扫描电子显微镜(SEM，SU8010，Hitachi)和扫描电子能谱(EDS)表征样品的微观形貌和元素分布；万能拉伸试验机(Instron 1186，加载速度为10 mm/min)检测膜的力学性能。膜面积溶胀率的计算公式如下：
SR = (Swet−Sdry)Sdry×100% (1) 式中：Swet为湿膜面积(cm2)；Sdry为干膜面积(cm2)。
吸水率的计算公式如下：
WU = (Wwet−Wdry)Wdry×100% (2) 式中：Wwet为湿膜质量(g)；Wdry为干膜质量(g)。
应用紫外分光光度计(TU-1810，Beijing Purcell General Instrument Co., Ltd.)测试膜的VO2+渗透性[7]，取样间隔12 h，钒离子渗透率计算公式如下：
VBd(CB(t))dt=APL(CA−CB(t)) (3) 式中：CA为VOSO4溶液侧VO2+浓度；CB(t)为t时刻MgSO4溶液侧VO2+浓度；VB为MgSO4溶液体积；P为钒离子渗透率；L为膜厚度；A为膜的有效面积(1.77 cm2)。
应用电化学工作站(CHI660E，上海辰华)测试膜的离子电导率(σ)，计算公式如下：
σ=DRb (4) 式中：Rb为膜的面电阻；D为膜厚度。
采用新威电池测试系统表征VRB的电池性能，电流密度范围为100~200 mA·cm−2。循环测试的电流密度为150 mA·cm−2，循环200次。测试膜的放电容量衰减率，计算公式如下：
容量衰减率=Qdis200Qdis1×100% (5) 式中：Qdis200为循环200次后的放电容量；Qdis1为第一次循环前放电容量。
单次衰减=容量衰减率循环次数 (6) 2. 结果分析与讨论
2.1 UIO-66-NH2的形貌与结构
为了优化UIO-66-NH2的合成参数，考察微波合成温度对材料结构的影响。如图2(a)所示，合成时间为15 min，当温度较低时(60℃)，晶体结构较规则，颗粒尺寸约为1 μm，但产率仅为60%。随着合成温度的升高，晶体结构特征逐渐增强。如图2(c)所示，100℃下所合成样品具有明显的近八面体的晶体形貌[18]。当温度升至120℃时，晶体结构更加完整，此时粒径约为200 nm (图2(d))，分散性较好，且产率接近90%。由图2(e)可见，通过普通水热法也可以制备出形貌规则的UIO-66-NH2材料，只是所需的合成时间更长[19]。
![]()
图 2 不同温度下微波加热15 min ((a)~(d))、烘箱加热24 h (e)制备UIO-66-NH2的SEM图像及微波加热15 min样品的XRD图谱(f)Figure 2. SEM images of UIO-66-NH2 by microwave heating for 15 min ((a)-(d)), oven heating for 24 h (e) and XRD patterns of UIO-66-NH2 by microwave heating for 15 min (f)XRD图谱反映样品的结晶度，如图2(f)所示，样品在2θ=7.40°和8.66°处出现了较明显的衍射峰，对应UIO-66-NH2的(111)和(002)晶面，在2θ=26.22°和31.86°等处出现强度略低的衍射峰，以上峰位置和强度与文献报道结果基本一致[20]，证明本实验成功合成出UIO-66-NH2晶体。而且，随着合成温度的升高，晶体的衍射峰强度逐渐增加，即使在60℃下仍能合成出晶体。主要原因在于微波作用下反应釜内晶体前驱体溶液能够快速、均匀受热，微波的折射和反射诱导晶体快速成核、生长[21]，合成效率得到显著提高。
2.2 质子交换膜的形貌与结构
为了更清晰地观察复合膜的微观形貌以及膜厚度，本实验对膜样品进行SEM表征。如图3(a)~3(c)所示，不同膜样品均呈现出较均匀、致密的形貌特征，M-U66-NH2的掺杂量对膜形貌的影响较小。图3(d)~3(f)中，不同膜的厚度相近，约为40 μm。P-N膜的截面更加平滑，随着M-U66-NH2掺杂量的增加，复合膜截面的粗糙度逐渐增加，但晶体的掺入未明显改变复合膜的结构。图3(g)~3(j)清晰地展现出M-U66-NH2在M/N-3膜表面的元素分布情况，与M-U66-NH2相关的Zr、N元素及与Nafion树脂相关的F、S元素在膜表面均匀分布，说明复合膜中M-U66-NH2的分散性良好，未发生明显的团聚现象，这为功能材料充分发挥其表面性质和孔道尺寸等优势提供了条件。
![]()
图 3 P-N (a)、M/N-1 (b)、M/N-3 (c) 膜的表面SEM图像；P-N (d)、M/N-1 (e)、M/N-3 (f)膜的截面SEM图像；M/N-3膜的表面EDS元素分布图((g) N；(h) Zr；(i) F；(j) S)Figure 3. Surface SEM images of P-N (a), M/N-1 (b) and M/N-3 (c); Cross-section SEM images of P-N (d), M/N-1 (e) and M/N-3 (f); EDS element images of M/N-3 ((g) N; (h) Zr; (i) F; (j) S)为了探究高M-U66-NH2含量对复合膜结构的影响，本实验制备了M/N-6和M/N-9膜。如图4(a)所示，当掺杂量为6wt%时，复合膜截面出现较多尺寸约为2~3 μm的孔洞，这是由于较高掺杂量下，强相互作用促使M-U66-NH2粒子团聚，导致膜内出现缺陷。当掺杂量增至9wt%时，复合膜截面呈现出非对称结构，膜层中可以观察到大尺寸的M-U66-NH2团聚体。产生该现象的原因在于成膜过程中随着溶剂的缓慢挥发，较大尺寸的团聚体会逐渐下沉，并在玻璃板侧富集，形成高M-U66-NH2含量的多孔、疏松区域，此结构不利于UIO-66-NH2功能的充分发挥。同时，产生的缺陷会加速钒离子的跨膜渗透，降低质子交换膜的离子选择性和力学性能[14]。
![]()
图 4 M/N-6 ((a), (c))和M/N-9 ((b), (d))膜的截面SEM图像Figure 4. Cross-sectional SEM images of M/N-6 ((a), (c)) and M/N-9 ((b), (d))通过FTIR分析M-U66-NH2、P-N、M/N-3膜样品的结构特征，如图5所示。M-U66-NH2在
3458 、3356 和1436 cm−1处具有明显的特征峰，分别对应N—H的对称和非对称伸缩振动及N—H的剪切伸缩振动，与文献报道一致[22]。P-N膜在1207 、1151 cm−1处为C—F伸缩振动峰，1053 cm−1处为O=S=O振动峰及980 cm−1处为C—F特征峰，此结果与文献中Nafion树脂的特征峰一致[18]。M/N-3膜的FTIR图谱可以近似为M-U66-NH2和P-N图谱的叠加，表明UIO-66-NH2材料在膜中稳定存在，制膜过程并未破坏其微观结构。![]()
图 5 M-U66-NH2、P-N膜和M/N-3膜的红外图谱Figure 5. FTIR spectra of M-U66-NH2, P-N and M/N-3 membranes2.3 复合质子交换膜的理化性质
吸水率和溶胀率是质子交换膜的重要性能指标。如图6(a)所示，基于M-U66-NH2的亲水性和丰富孔道，复合膜的吸水率和溶胀率均随掺入量的提高而增大[23]。同时，M-U66-NH2的刚性结构赋予膜较低的溶胀率(<4%)[24]。膜的吸水率和溶胀率直接影响其离子传导性能，由图6(b)可见，随着UIO-66-NH2掺杂量的提高，膜的质子传导率逐渐增大，而面电阻逐渐变小，如M/N-3的质子传导率达到122.18 mS·cm−1，此改善效果得益于膜中—NH2和—SO3H形成的酸/碱对及存在的氢键网络[25]。当掺杂量超过6wt%时，M-U66-NH2在膜内团聚而缺陷产生，膜的质子传导率较低。比较可见，M/N-3膜的吸水率、溶胀率和质子传导率均高于T/N-3膜，这与UIO-66-NH2的尺寸均一性和完整结构相关。
![]()
图 6 复合膜的吸水率和溶胀率(a)、面电阻和质子传导率(b)、力学性能(c)和应力-应变曲线(d)Figure 6. Water uptake and swelling ratio (a), area resistance and conductivity (b), mechanical properties (c) and stress-strain curves of different membranes (d)N212—Nafion 212 commercial membrane, DuPont, USA通过拉伸测试评价膜的机械强度。如图6(c)和图6(d)所示，M-U66-NH2掺杂量低于3wt%时，复合膜的拉伸强度均高于P-N膜，如M/N-3膜的强度达到27 MPa，也高于T/N-3膜(22.3 MPa)。主要原因是UIO-66-NH2具有刚性结构，且—NH2基团与Nafion的—SO3H基团可形成共轭酸碱对，其强相互作用促使Nafion的分子链与UIO-66-NH2发生物理交联，从而提高了复合膜的力学性能[26]。同时，尺寸更小、更规则的M-U66-NH2与Nafion树脂间的分散更充分、交联程度更高，表现出更优的强化作用[1]。当UIO-66-NH2掺杂量超过6wt%时，膜中的孔洞缺陷导致其机械强度显著降低。
2.4 复合膜的钒离子渗透性和离子选择性
本实验选取的UIO-66-NH2的有效孔径为0.52 nm，介于水分子(<0.3 nm)和钒离子(>0.6 nm)之间[14]，可通过筛分效应提高膜的离子选择性。如图7(a)所示，M/N-X系列复合膜的阻钒性更好，如M/N-3膜的钒离子渗透浓度最低，仅为P-N膜的23%左右，而M/N-9膜的阻钒性能最差。当测试时间大于48 h，T/N-3膜的表现略差于M/N-3膜，主要原因是传统水热法所制备UIO-66-NH2的晶体结构不够完善。
![]()
图 7 复合膜的钒离子渗透浓度(a)、钒离子渗透率和离子选择性(b)Figure 7. Vanadium ion permeation concentration (a), vanadium ion permeability and ion selectivity (b) of composite membranes高钒离子渗透性不利于钒电池长期稳定运行，如图7(b)所示，优化条件下所制备的M/N-3膜的钒离子渗透率最低，仅为8.3×10−8 cm2·min−1，且该膜的离子选择性达到15.6×105 S·min·cm−3，约为P-N膜的30倍。当UIO-66-NH2掺入量过低或过高时，膜的离子选择性均较低。另外，与M/N-3膜相比，掺杂T-U66-NH2的复合膜具有接近的离子选择性，这说明两种方法所制备UIO-66-NH2对复合膜离子选择性的影响较小。有研究结果指出增加厚度会提高膜的钒离子渗透率，进而降低膜的离子选择性[7, 27-29]。因此，本实验在充分参考文献数据的基础上，为了平衡氢离子、钒离子的渗透情况，确定膜厚度为40 μm左右。
2.5 复合膜的电池性能
分别将P-N、M/N-X和T/N-3膜组装成模拟电池进行测试，评价不同电池的电压效率(Voltage efficiency，VE)、库伦效率(Coulombic efficiency，CE)和能量效率(EE)。由图8(a)可知，在相同电流密度下，随着M-U66-NH2掺杂量的增加，电池的CE逐渐增大，在100 mA·cm−2电流密度下，M/N-3膜电池的库伦效率达到97.66%，高于P-N膜(95.8%)。因膜内粒子团聚造成了结构缺陷，M/N-6和M/N-9膜电池的CE低于M/N-3膜。图8(b)显示M/N-X复合膜的VE高于P-N膜，且随着电流密度的增加，复合膜所装配电池的VE逐渐减小，该趋势与文献报道相似[5]。能量效率是电池性能的综合体现，由图8(c)可见，在电流密度为100~200 mA·cm−2的范围内，M/N-3膜的电池能量效率均高于P-N膜和N212膜(美国杜邦公司Nafion212商品膜)，最高达到83.8%，说明M-U66-NH2的掺入有效提升了Nafion膜的电池性能。图8(d)~8(f)更清晰地显示了P-N膜、M/N-3膜和T/N-3膜的性能。在测试电流密度范围内，M/N-3膜的库伦效率与T/N-3膜相当，而其电压效率和能量效率均高于其他两种类型膜样品。可见，适宜比例M-U66-NH2的引入有利于改善膜性能，进而提升钒电池的充放电效率。
![]()
图 8 不同膜所装配钒液流电池(VRBs)的库伦效率(CE) ((a), (d))、电压效率(VE) ((b), (e))和能量效率(EE) ((c), (f))Figure 8. Coulombic efficiency (CE) ((a), (d)), voltage efficiency (VE) ((b), (e)) and energy efficiency (EE) ((c), (f)) of vanadium liquid flow battery (VRBs) assembled with different membranes在150 mA·cm−2电流密度下，对不同膜所装配电池充放电200次，比较其循环稳定性。如图9(a)所示，在200次的充放电测试过程中，M/N-3和T/N-3膜的电池CE、VE和EE相对较稳定，无明显降低，说明UIO-66-NH2的引入有效阻碍了钒离子在膜内的渗透，保证了质子在膜中快速、稳定传输。此外，在测试周期内，M/N-3和T/N-3膜的能量效率分别稳定在77.8%和76.5%，无明显衰减，说明复合膜的理化稳定性可以满足钒电池工作环境的需求。但是，测试周期内不同电池的容量衰减情况差异较大。由图9(b)可见，P-N膜的电池容量衰减了81%，而经过同样测试后，掺杂UIO-66-NH2膜的电池容量衰减率仅为45%左右，其中基于M-U66-NH2的复合膜显示出更低的容量衰减率，单次衰减率仅为0.19%，较T/N-3膜(0.24%)提高0.05%，较P-N膜(0.41%)提高0.22%，同时，图9(c)证明了掺杂M-U66-NH2的确会优化VRBs所用质子交换膜的电池性能。
![]()
图 9 150 mA·cm−2电流密度下复合膜所装配电池的循环效率(a)、容量保持率(b)以及与报道性能的对比(c)Figure 9. Cycle efficiency (a) and capacity retention (b) of composite membranes at 150 mA·cm−2, and comparisons with reported performance (c)PBI—Polybenzimidazole; PS—Polystyrene; GO—Graphene oxide; SPEEK—Sulfonated poly(ether ether ketone); 2D-ZMs—Two-dimensional zeolite3. 结 论
(1)与传统水热法相比，微波加热合成UIO-66-NH2的效率更高，耗时仅为前者的1/96，且所制备晶体的结构更完整、更均匀，粒径约为200 nm，在Nafion溶液中分散性良好。
(2) UIO-66-NH2可改善复合膜的理化稳定性，且可提高膜的质子传导性和离子选择性，优化条件下复合膜的质子传导率可达122.18 mS·cm−1，离子选择性可达15.6×105 S·min·cm−3。
(3)优化条件下复合膜体现出良好的电池性能。在150 mA·cm−2电流密度下，电池的能量效率大于77%，200次循环周期内单次容量衰减率为0.19%，较纯树脂膜提高0.22%。
(4)基于微波法的UIO-66-NH2与Nafion形成的复合膜具有良好的理化性质和电池性能，为全钒液流电池用高性能质子交换膜的设计和制备提供了新的策略，具有良好的发展前景。
-
![]()
图 4 LR试件破坏形态
Figure 4. Failure modes of LR specimens
ft,R—Tensile strength of the specimen; fc,R—Compressive strength of the specimen.
![]()
图 8 落叶松试件应变分布
Figure 8. Strain distribution of larch wood
"T" is the time it takes to load
![]()
图 9 落叶松试件应力-应变曲线
Figure 9. Stress-strain curves of specimens of larch wood
"DL" means uniaxial tensile loading, "DY" means uniaxial compressive loading, and "J" means shear loading.
![]()
图 10 落叶松试件应力-应变曲线
Figure 10. Stress-strain curves of specimens of larch wood
"LJ" indicates stretch-shear combined loading, and "LY" indicates compression-shear combined loading.
![]()
图 11 落叶松试件抗剪强度、峰值应变和剪切模量的变化
Figure 11. Variation of shear strengths, peak strains, and shear moduli of larch wood
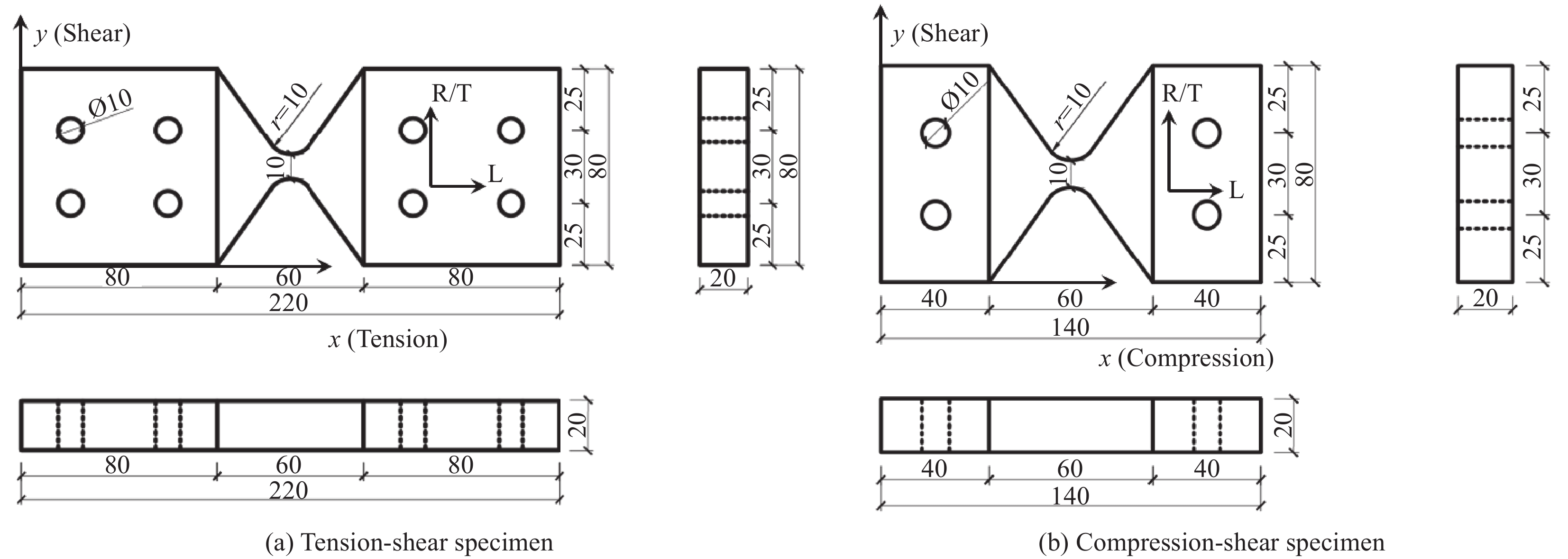
表 1 试件分组
Table 1 Grouping of specimens
Stress Numbers of normal
stress levelsSpecimen type LR LT Uniaxial tension / 3 3 Uniaxial compression / 3 3 Shear / 3 3 Combined
tension-shear4 12 12 Combined
compression -shear3 9 9 Notes: In LR and LT specimens, "L" is the longitudinal direction of wood, "R" is the radial direction of wood, and "T" is the chord direction of wood, where "L" represents the tensile/compressive loading direction, and "R" and "R" represent the shear loading direction. 
下载: 导出CSV
表 2 LR试件材性 (单位：mm)
Table 2 Mechanical properties of LR specimens (unit: mm)
ft,R fc,R fv,R Et Ec G Mean value 60.24 44.67 10.72 48481.33 7809.29 1322.89 Coefficient of variation 7.71% 2.72% 2.85% 27.22% 21.52% 9.08% Notes: “ft,R” is tensile strength, “fc,R” is compressive strength, “fv,R” is shear strength, “Et” is tensile modulus, “Ec” is compression modulus, and “G” is shear modulus.
下载: 导出CSV
表 3 LT试件材性(单位：mm)
Table 3 Mechanical properties of LT specimens (unit: mm)
ft,R fc,R fv,R Et Ec G Mean value 47.17 50.16 12.20 19333.0 15121.60 1279.15 Coefficient of variation 8.34% 1.83% 5.91% 12.83% 18.71% 4.92%
下载: 导出CSV
表 4 木材强度准则强度预测能力评估指标
Table 4 Evaluation index of strength prediction ability of wood strength criteria
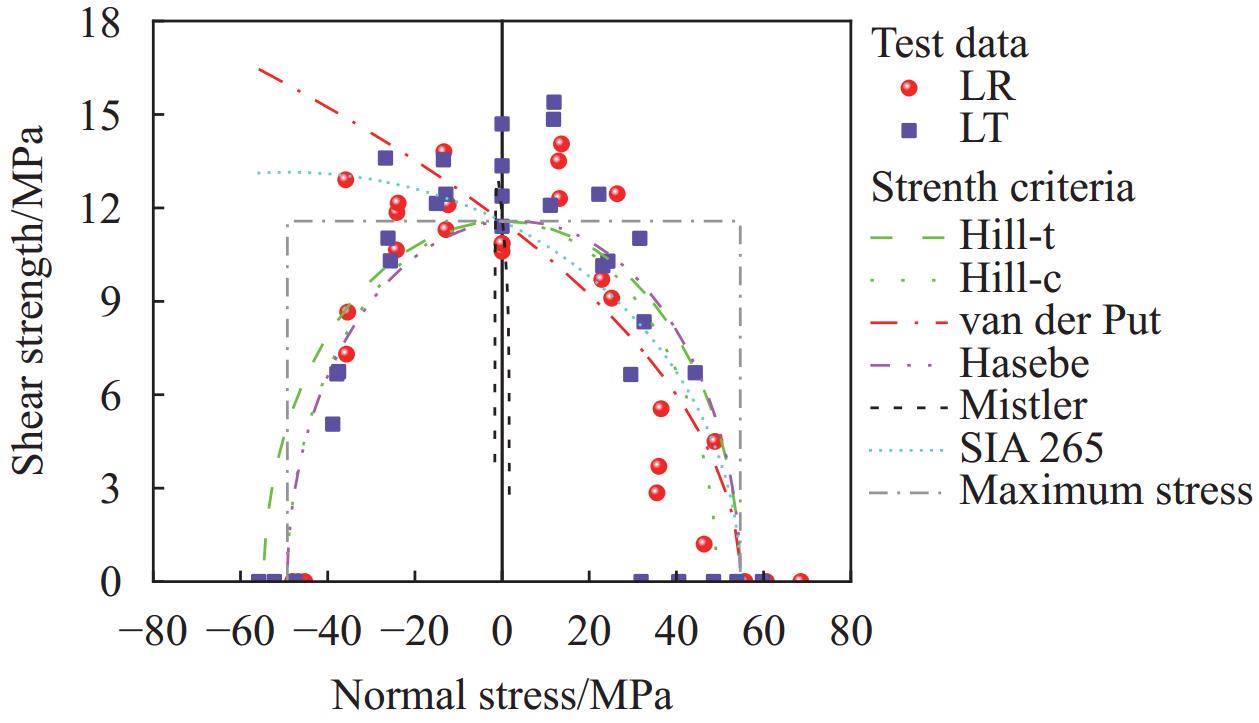
strength criteria k First quadrant Second quadrant Total Hill-t 3.10 12.50 15.59 Hill-c 2.40 9.03 11.43 Van der put 8.72 9.22 17.94 Hasebe 3.19 3.93 7.12 SIA 265 6.44 10.20 16.64 Note: "k" is the sum of the ratio of the absolute residual value of each data point to the test value.
下载: 导出CSV
-
[1] 刘伟庆, 杨会峰. 现代木结构研究进展[J]. 建筑结构学报, 2019, 40(2): 16-43. LIU Weiqing, YANG Huifeng. Research progress of modern wood structures[J]. Journal of Building Structures, 2019, 40(2): 16-43(in Chinese).
[2] 孟宪杰, 陈金永, 师希望, 等. 木材横纹全表面受压性能试验研究[J]. 中国科技论文, 2016, 11(1): 62-65. DOI: 10.3969/j.issn.2095-2783.2016.01.014 MENG Xianjie, CHEN Jinyong, SHI Xixing, et al. Experimental study on full surface compression performance of transverse grain wood[J]. Chinese Science and Technology Papers, 2016, 11(1): 62-65(in Chinese). DOI: 10.3969/j.issn.2095-2783.2016.01.014
[3] 谢启芳, 张利朋, 王龙, 等. 东北落叶松材单向顺纹受拉损伤模型[J]. 湖南大学学报(自然科学版), 2017, 44(11): 109-116. XIE Qifang, ZHANG Lipeng, WANG Long, et al. Longitudinal strain damage model of larix wood in Northeast China[J]. Journal of Hunan University (Natural Science Edition), 2017, 44(11): 109-116(in Chinese).
[4] BILKO P, SKORATKO A, RUTKIEWICZ A, et al. Determination of the shear modulus of pine wood with the arcan test and digital image correlation[J]. Materials, 2021, 14(2): 468-486. DOI: 10.3390/ma14020468
[5] 杨娜, 王忠铖, 常鹏. 藏青杨旧材无疵试样力学性质试验研究[J]. 建筑结构学报, 2022, 43(11): 168-176. YANG Na, WANG Zhongcheng, CHANG Peng. Experimental study on the mechanical properties of undamaged specimens of old Tibetan poplar[J]. Journal of Building Structures, 2022, 43(11): 168-176(in Chinese).
[6] 张利朋, 谢启芳, 刘伊津, 等. 循环荷载作用下木材顺纹受力特性与本构模型研究[J]. 土木工程学报, 2024, 57(3): 42-58. ZHANG Lipeng, XIE Qifang, LIU Yijin, et al. Study on mechanical behavior and constitutive model of wood under cyclic load[J]. Chinese Journal of Civil Engineering, 2024, 57(3): 42-58(in Chinese).
[7] 张利朋, 谢启芳, 吴亚杰, 等. 木材本构模型研究进展[J]. 建筑结构学报, 2023, 44(5): 286-304. ZHANG Lipeng, XIE Qifang, WU Yajie, et al. Research progress of wood constitutive model[J]. Journal of Building Structure, 2023, 44(5): 286-304(in Chinese).
[8] 杨庆山, 伍婷, 王娟. 基于三维力学分析模型与灵敏度方法的古建筑木结构模型修正[J/OL]. 工程力学: 1―11[2024-04-18]. O3.20231123.1808.014.html. YANG Qingshan, WU Ting, WANG Juan. Revision of ancient wooden structure model based on 3D mechanical analysis model and sensitivity method[J/OL]. 1―11 [2024-04-18]. Engineering mechanics: . net/kcms/detail/11.2595.O3.20231123.1808.014.html. (in Chinese)
[9] 2023.0295. (in Chinese) 2023.0295. (in Chinese) 2024-04-18]. https: //doi. org/10.14006/j. jzjgxb. 2023.0295. PAN Yi, AN Renbing, YOU Wenlong. Ancient wood mechanical properties research progress of mortise and tenon joint nodes[J/OL]. Journal of building structures: 1―18 [2024-04-18].
[10] 张利朋, 谢启芳, 吴亚杰, 等. 基于木材弹塑性损伤本构的古建木结构残损梁柱构件损伤非线性分析[J]. 土木与环境工程学报(中英文), 2022, 44(2): 98-106. ZHANG Lipeng, XIE Qifang, WU Yajie, et al. Nonlinear damage analysis of damaged beam and column members of ancient wooden structures based on Wood elastic-plastic damage Constitutive Method[J]. Journal of Civil and Environmental Engineering, 2022, 44(2): 98-106(in Chinese).
[11] MACKENZIE-HELNWEIN P, EBERHARDSTEINER J, MANG H A. A multi-surface plasticity model for clear wood and its application to the finite element analysis of structural details[J]. Computational Mechanics, 2003, 31(1): 204-218.
[12] MACKENZIE-HELNWEIN P, EBERHARDSTEINER J, MANG H A. Rate-independent mechanical behavior of biaxially stressed wood: Experimental observations and constitutive modeling as an orthotropic two-surface elasto-plastic material[J]. Holzforschung, 2005, 59: 311-321. DOI: 10.1515/HF.2005.052
[13] DE MAGISTRIS F, SALMÉN L. Combined shear and compression analysis using the Iosipescu device: analytical and experimental studies of medium density fiberboard[J]. Wood Science and Technology, 2004, 37(6): 509-521. DOI: 10.1007/s00226-003-0217-1
[14] J M CABRERO, C BLANCO, K G GEBREMEDHIN, et al. Assessment of phenomenological failure criteria for wood[J]. Holz als Roh-und Werkstoff, 2012, 70(6): 871-882. DOI: 10.1007/s00107-012-0638-3
[15] 蔡竞. 胶合木材料力学性能研究[D]. 大连: 大连理工大学, 2014. CAI Jing. Study on Mechanical properties of glulam materials[D]. Dalian: Dalian University of Technology, 2014. (in Chinese)
[16] AKTER S T, BADER T K. Experimental assessment of failure criteria for the interaction of normal stress perpendicular to the grain with rolling shear stress in Norway spruce clear wood[J]. European Journal of Wood and Wood Products, 2020, 78: 1105-1123. DOI: 10.1007/s00107-020-01587-w
[17] AKTER S T, SERRANO E, BADER T K. Numerical modelling of wood under combined loading of compression perpendicular to the grain and rolling shear[J]. Engineering Structures, 2021, 244: 112800. DOI: 10.1016/j.engstruct.2021.112800
[18] ZHANG Lipeng, HAN Yonggang, XIE Qifang, et al. Mechanical performance of wood subjected to the interaction of transversal tension/compression and longitudinal shear stresses[J]. Construction and Building Materials, 2024, 420 135637.
[19] GB/T 1927.3-2021, 无疵小试样木材物理力学性质试验方法 第3部分: 生长轮宽度和晚材率测定[S]. 北京: 中国建筑工业出版社. GB/T 1927.3-2021, Methods of test for physical and mechanical properties of undefective small specimens of wood - Part 3: Determination of growth ring width and late wood percentage[S]. Beijing: China Architecture and Architecture Press. (in Chinese)
[20] GB/T 1927.4-2021, 无疵小试样木材物理力学性质试验方法 第4部分: 含水率测定[S]. 北京: 中国建筑工业出版社. GB/T 1927.4-2021, Methods of test for physical and mechanical properties of undefective small samples of wood - Part 4: Determination of moisture content[S]. Beijing: China Architecture and Architecture Press. (in Chinese)
[21] GB/T 1927.5-2021, 无疵小试样木材物理力学性质试验方法 第5部分: 密度测定[S]. 北京: 中国建筑工业出版社. GB/T 1927.5-2021, Methods of test for physical and mechanical properties of undefective small specimens of wood - Part 5: Determination of density[S]. Beijing: China Architecture and Architecture Press. (in Chinese)
[22] GB/T 1927.16-2022, 无疵小试样木材物理力学性质试验方法 第16部分: 顺纹抗剪强度测定[S]. 北京: 中国建筑工业出版社. GB/T 1927.16-2022, Methods of test for physical and mechanical properties of undamaged small samples of wood - Part 16: Determination of shear strength along grain[S]. Beijing: China Architecture and Architecture Press. (in Chinese)
[23] ZHANG Lei, YANG Na. Evaluation of a modified Iosipescu shear test method for determining the shear properties of clear wood[J]. Wood Science and Technology, 2017, 51: 323-343. DOI: 10.1007/s00226-016-0888-z
[24] YANG Na, LI Peng, LAW S S, et al. Experimental research on mechanical properties of timber in ancient Tibetan building[J]. Journal of Materials in Civil Engineering, 2012, 24(6): 635-643. DOI: 10.1061/(ASCE)MT.1943-5533.0000438
[25] 张浩. 纤维增强复合材料剪切试验方法综述[J]. 科技创新导报, 2015, 12(21): 65-66. DOI: 10.3969/j.issn.1674-098X.2015.21.029 ZHANG Hao. Shear test method for fiber reinforced composite material review[J]. Science and technology innovation herald, 2015, 12(21): 65-66. DOI: 10.3969/j.issn.1674-098X.2015.21.029
[26] 徐博瀚, 蔡竞. 木材强度准则的研究进展[J]. 土木工程学报, 2015, 48(1): 64-73. XU Bohan, CAI Jing. Research progress of wood strength criteria[J]. Journal of Civil Engineering, 2015, 48(1): 64-73(in Chinese).
[27] 王明谦, 宋晓滨, 顾祥林. 基于三维弹塑性损伤模型的木材非线性分析[J]. 土木工程学报, 2018, 51(7): 22-28. WANG Mingqian, SONG Xiaobin, GU Xianglin. Nonlinear analysis of wood based on three-dimensional elastic-plastic damage model[J]. Journal of Civil Engineering, 2018, 51(7): 22-28(in Chinese).
[28] HILL R. A theory of the yielding and plastic flow of anisotropic metals[J]. Proceedings of the Royal Society of London. Series A. Mathematical and Physical Sciences, 1948, 193(1033): 281-297.
[29] AZZI V D, TSAI S W. Anisotropic strength of composites: Investigation aimed at developing a theory applicable to laminated as well as unidirectional composites, employing simple material properties derived from unidirectional specimens alone[J]. Experimental mechanics, 1965, 5: 283-288. DOI: 10.1007/BF02326292
[30] HASHIN Z. Failure Criteria for Unidirectional Fiber Composites[J]. Journal of Applied Mechanics, 1980, 47: 329-334. DOI: 10.1115/1.3153664
[31] NORRIS C. Strength of orthotropic materials subjected to combined stresses [R]. Madison: US Department of Agriculture, Forest Products Laboratory, 1962.
[32] VAN DER PUT T A C M. A continuum failure criterion applicable to wood[J]. Journal of Wood Science, 2009, 55: 315-322. DOI: 10.1007/s10086-009-1036-2
[33] TSAI S W, WU E M. A general theory of strength for anisotropic materials[J]. Journal of composite materials, 1971, 5(1): 58-80. DOI: 10.1177/002199837100500106
[34] HASEBE K, USUKI S. Application of orthotropic failure criterion to wood[J]. Journal of engineering mechanics, 1989, 115(4): 867-872. DOI: 10.1061/(ASCE)0733-9399(1989)115:4(867)
[35] HOFFMAN O. The brittle strength of orthotropic materials[J]. Journal of Composite materials, 1967, 1(2): 200-206. DOI: 10.1177/002199836700100210
[36] STEIGER R, GEHRI E. Interaction of shear stresses and stresses perpendicular to the grain. Proc of the CIB-W18 Meeting. Vol. 44. 2011.
[37] 应鹏杰. 古建筑木结构榫卯节点力学性能分析及精细化数值模拟[D]. 西安: 西安建筑科技大学, 2016. YING Pengjie. Mechanical Properties Analysis and Fine Numerical Simulation of mortise and tenon Joints of ancient Wooden Structures[D]. Xi'an: Xi'an University of Architecture & Technology, 2016. (in Chinese)
-
目的
实际木结构中木材大多同时受到顺纹正应力与横向剪应力复合作用，掌握其复合受力性能是木结构受力分析的重要基础。本文利用专门设计的试验加载装置和数字相关技术(DIC)以及单轴试验机进行落叶松木材在拉伸、压缩、剪切、拉伸-剪切以及压缩-剪切五种受力状态下的力学性能研究，并考察Hill、Tsai-Wu等经典正交各向异性强度准则对落叶松材拉/压-剪切复合受力强度的表征效果。
方法研发制作与现有单轴试验机相匹配的用于测试木材拉/压-剪切复合受力性能的杠杆加载装置，基于此开展木材顺纹拉伸/压缩、横纹剪切以及顺纹拉伸/压缩-横向剪切复合加载试验。利用数字相关技术(DIC)进行试件的应变测量，考察不同拉/压-剪切应力复合作用下木材试件的破坏形态、应力-应变曲线、拉/压-剪切复合强度特性，并对几种经典正交各向异性强度准则在木材中的适用性进行分析。
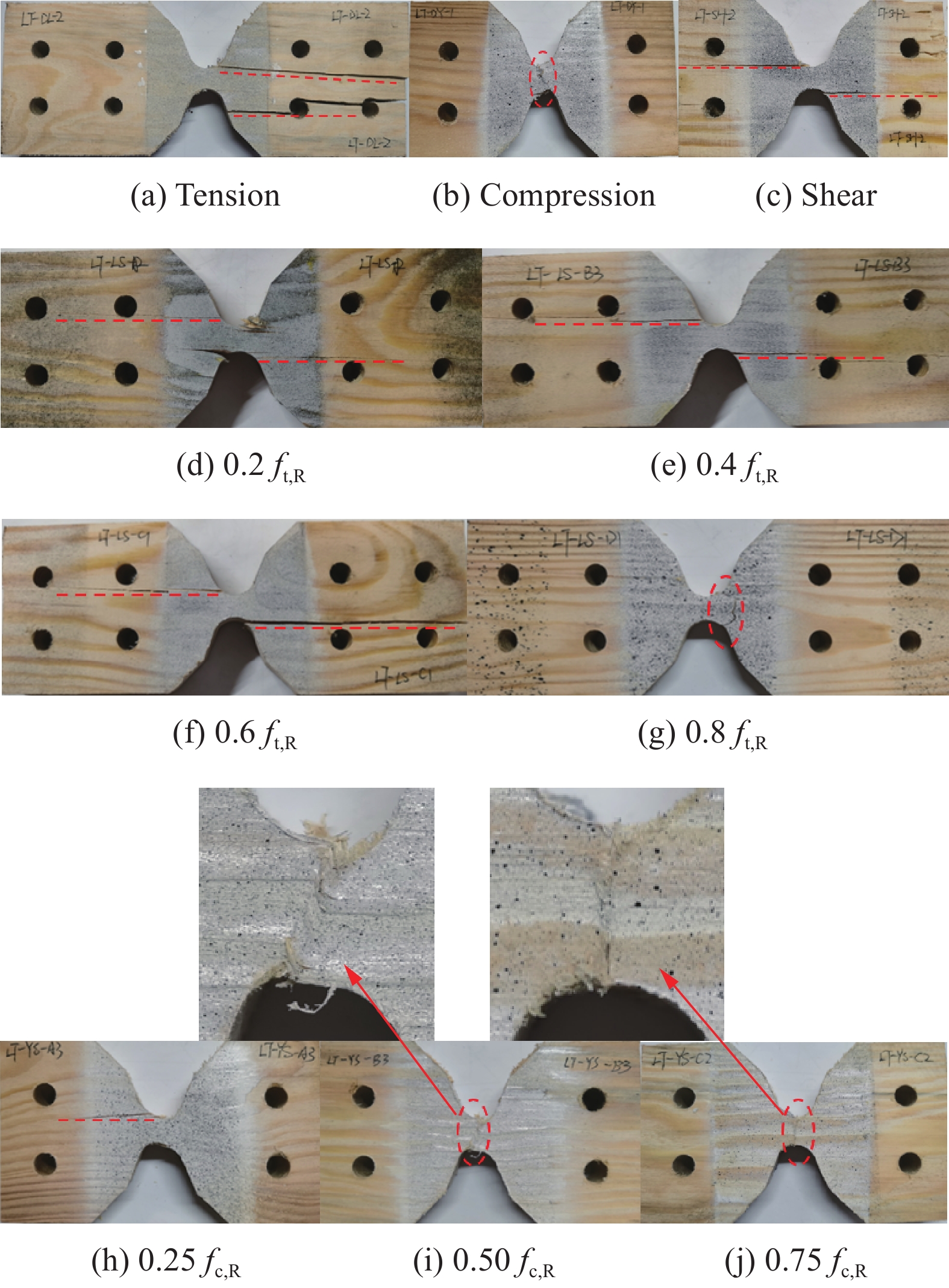
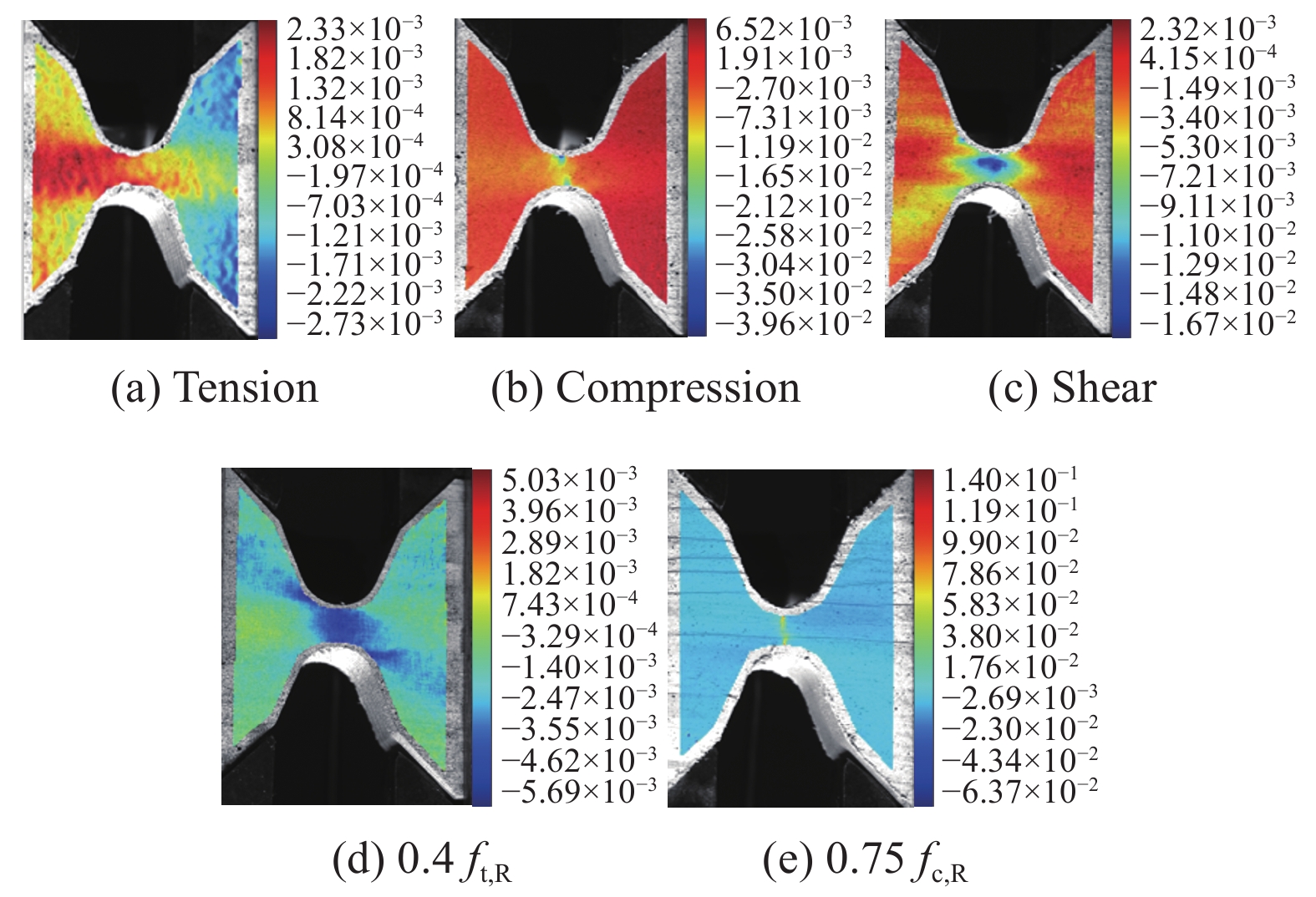
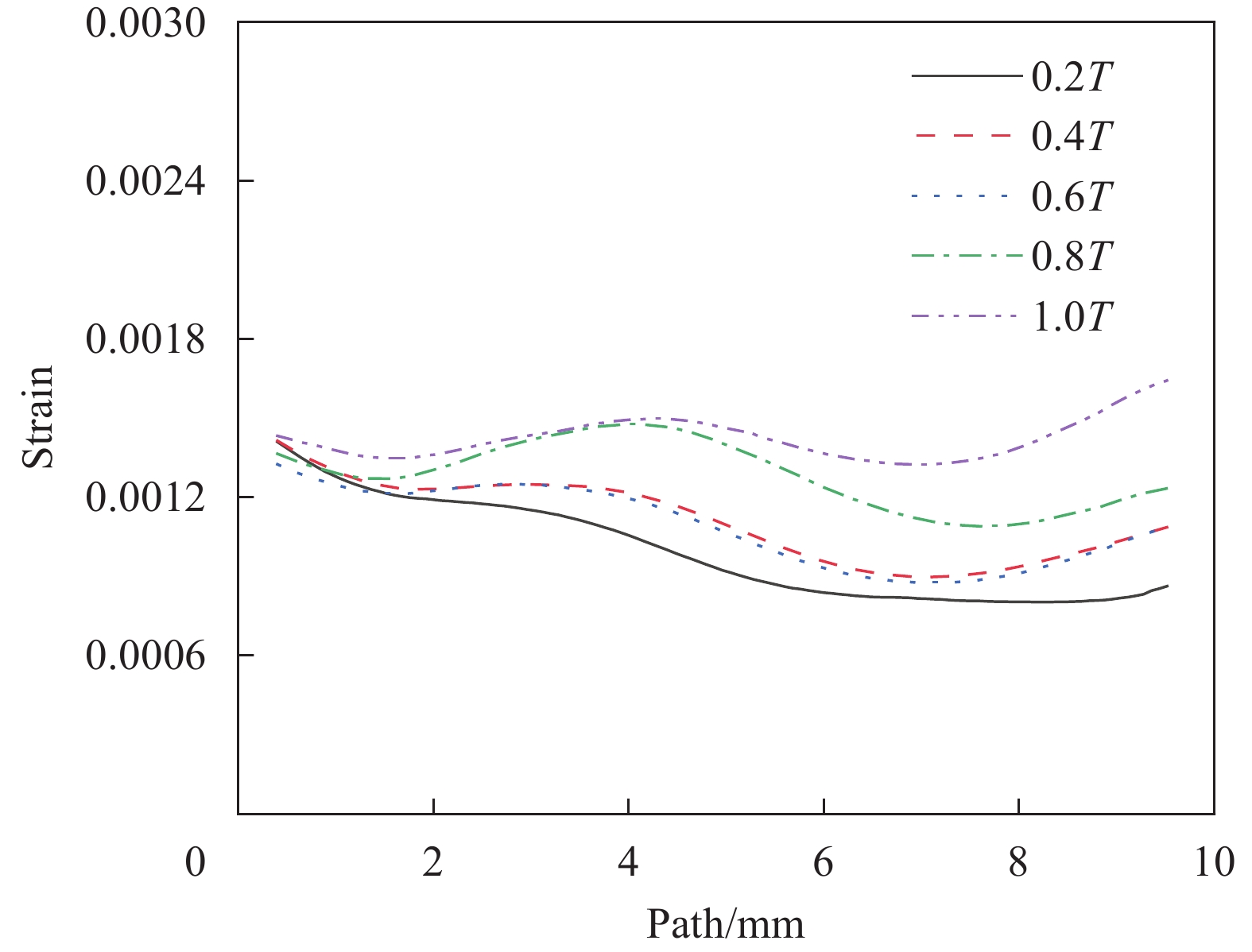
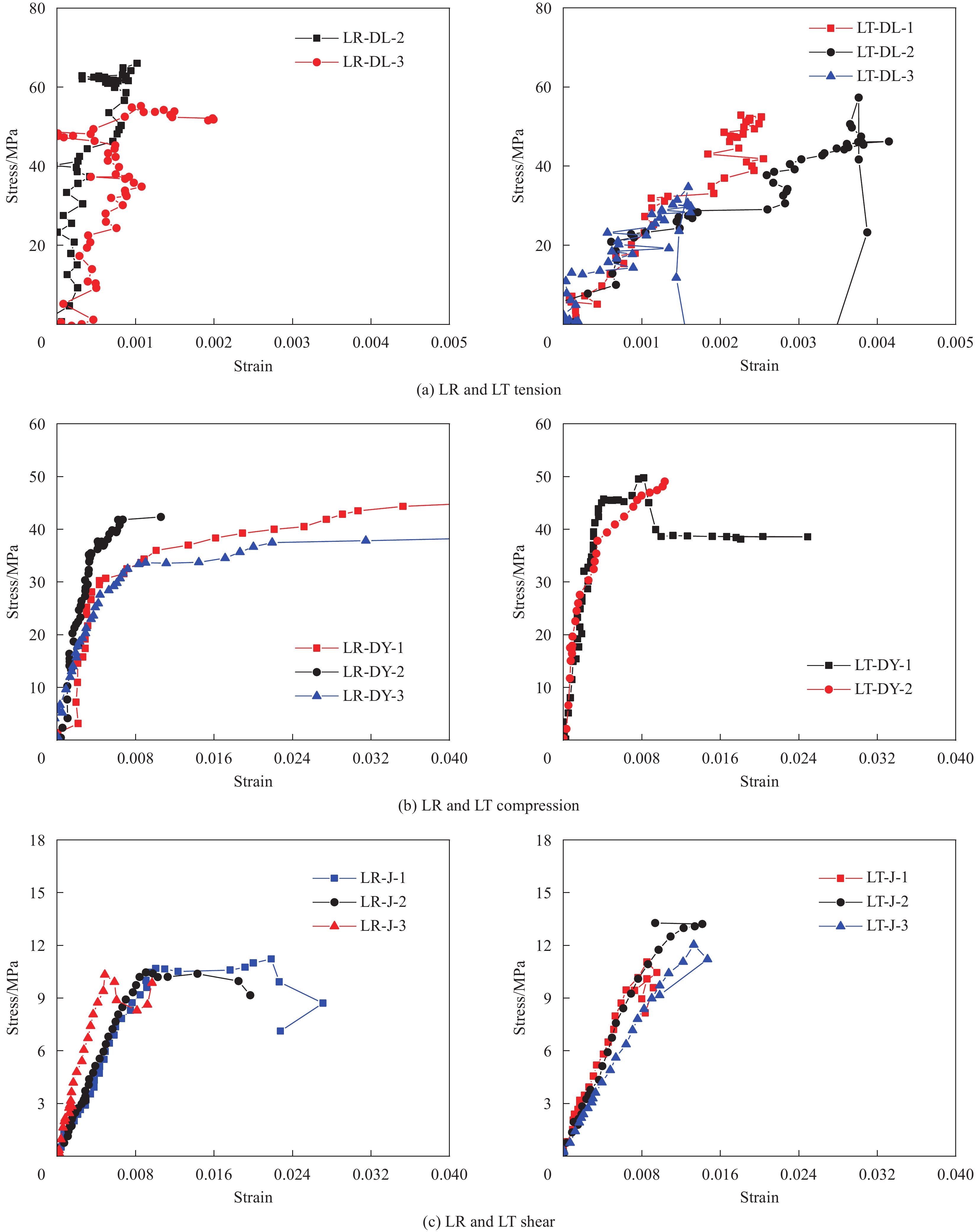
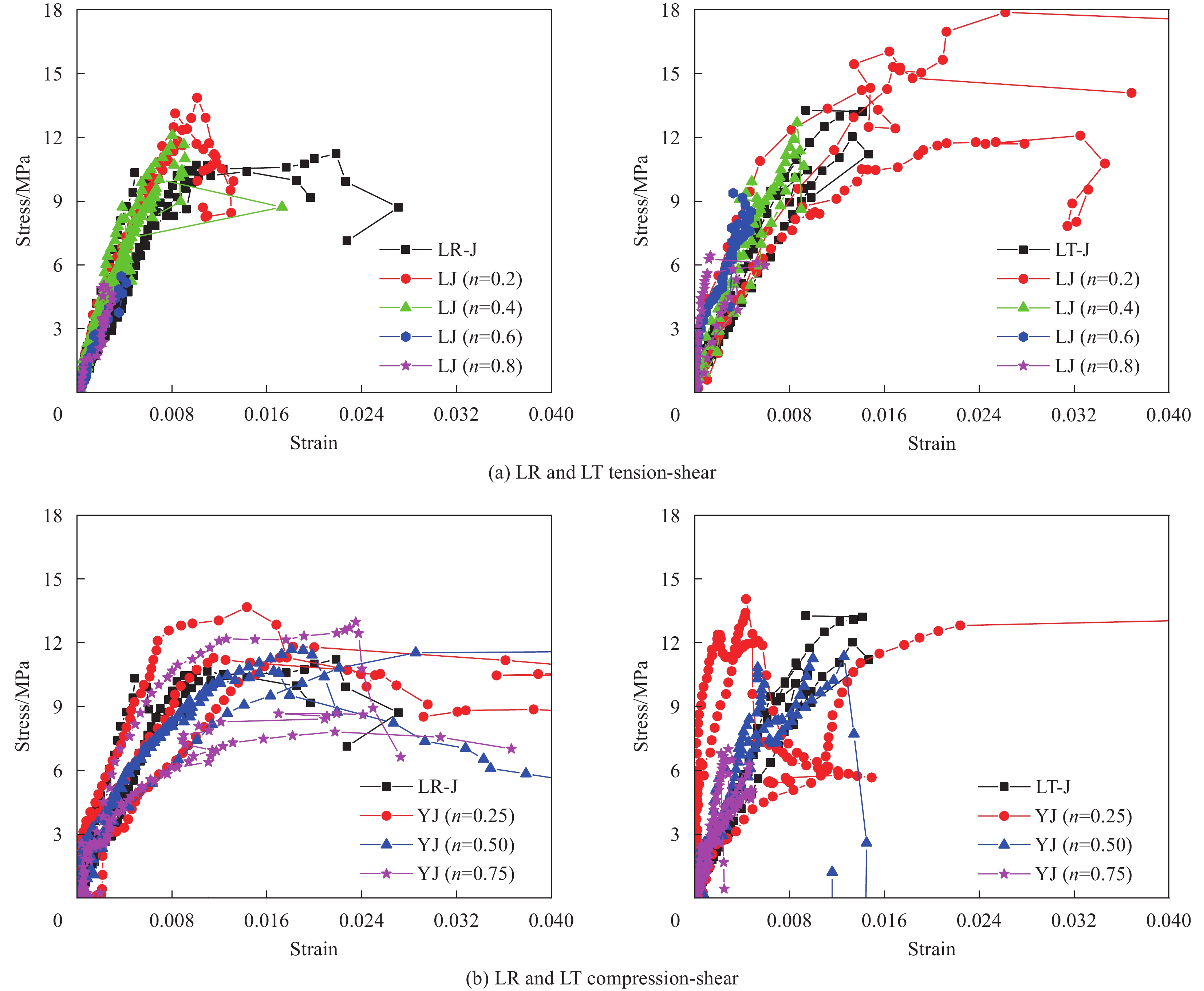
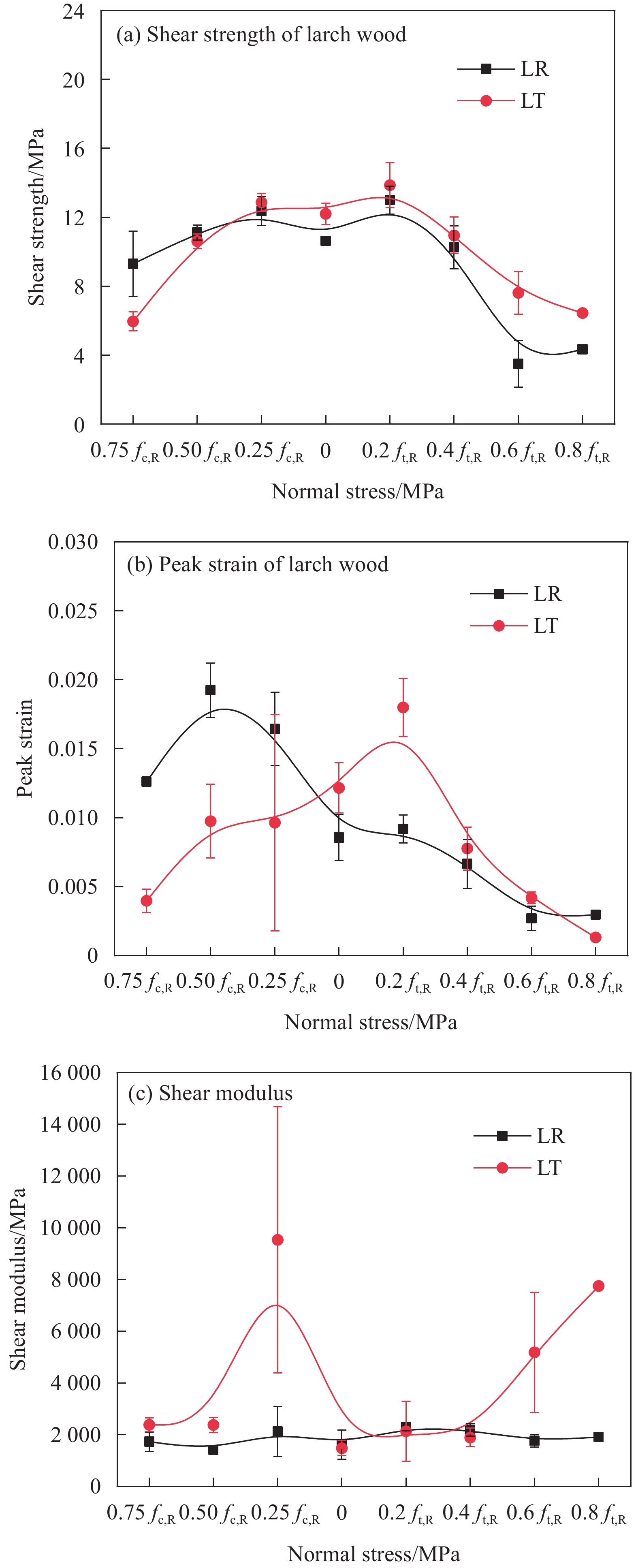
结果落叶松材抗剪强度、刚度与纹理面方向以及正应力的大小有着密切关系。拉应力对LR、LT试件的抗剪强度影响基本相同，随着拉应力的增大抗剪强度不断减小，拉应力增大至0.8 时试件的抗剪强度下降明显，降低约50%。而两者的抗剪刚度受拉应力的影响区别较大，LR试件抗剪刚度基本没有受到拉应力变化的影响，LT试件的抗剪刚度则随着拉应力的增大不断增大。LR试件的刚度整体上不受压应力的影响。LT试件的刚度在压应力较小时(0.25 )明显提高，随着压应力值增大刚度逐渐降低到纯剪切刚度的水平。强度方面，随着压应力值的增大，LR、LT试件抗剪强度都逐渐降低，LT试件强度的降低幅度高达50%。LR试件的剪切加载方向垂直于早晚材纹理面，试件的裂缝基本出现在早材区域，开裂面平行于早材和晚材纹理面，LT试件剪切方向平行于早晚材纹理面，破坏时开裂面同时穿过早材和晚材区域，开裂面和纹理面垂直，可见纹理方向对木材性能有着很大的影响。
结论从受力性能以及强度准则的适用性分析得出以下
结论(1)拉/压-剪切复合受力下LR和LT试件的破坏模式有两种：木材纤维间开裂；压应力较大时的木材纤维受压屈曲。(2)正应力作用下，试件抗剪强度降低。LR试件抗剪强度受到拉应力的影响更大，LT试件受到压应力的影响更大，随着正应力的增大，抗剪强度最大降低幅度可达到20%。(3)LR试件抗剪刚度基本不受正应力的影响，LT试件受到较小压应力(0.25 )，较大拉应力(0.60 t, 0.80 )时刚度明显提升。(4)定量分析了经典强度准则，包括最大应力准则、Hill准则、Tsai-Wu准则、van der Put准则和Hasebe准则等在木材中的适用性，发现Hill、Hasebe准则的预测误差最小。
-
木材具有环保可再生等许多优点，在古建筑和现代建筑结构中都应用较多。但由于木材正交各向异性、并且其生长状况受到环境因素影响很大，使得木材的构造与力学性能比较复杂，这为木材的力学性能研究带来了不小的挑战，进一步影响了古建木结构与现代木结构的保护和发展。
本文研发了一种可以在单轴试验机上进行木材拉/压-剪切复合受力的双轴加载试验装置，基于此进行了木材顺纹拉伸/压缩-横向剪切复合受力的加载试验。考察了不同拉/压-剪应力复合作用下木材试件的破坏形态、应力-应变曲线、拉/压-剪切复合强度特性，进一步考察并发现了Hill、Hasebe两种经典正交各向异性强度准则对木材复合受力强度具有较好的表征效果。
基于单轴试验机进行双轴加载的试验装置
强度准则的对比
计量
- 文章访问数: 92
- HTML全文浏览量: 52
- PDF下载量: 2
