多孔金属陶瓷功能梯度矩形板的低速冲击响应

胡旭初; 付涛

doi:10.13801/j.cnki.fhclxb.20221223.003

多孔金属陶瓷功能梯度矩形板的低速冲击响应

doi: 10.13801/j.cnki.fhclxb.20221223.003

胡旭初,
付涛^,

昆明理工大学　机电工程学院，昆明 650500

基金项目: 国家自然科学基金(52205105)；云南省基础研究专项(202101AU070160；202201AT070145)

详细信息

通讯作者:
付涛，博士，讲师，硕士生导师，研究方向为智能材料与结构　E-mail: ftkmust@126.com

中图分类号: TB333
计量
- 文章访问数: 555
- HTML全文浏览量: 341
- PDF下载量: 37
- 被引次数: 0
出版历程
- 收稿日期: 2022-10-24
- 修回日期: 2022-11-29
- 录用日期: 2022-12-09
- 网络出版日期: 2022-12-26
- 刊出日期: 2023-10-15

Low velocity impact response of porous metal ceramic functionally graded rectangular plate

HU Xuchu,
FU Tao^,

Faculty of Mechanical and Electrical Engineering, Kunming University of Science and Technology, Kunming 650500, China

Funds: National Natural Science Foundation of China (52205105); Yunnan Fundamental Research Projects (202101AU070160; 202201AT070145)

摘要

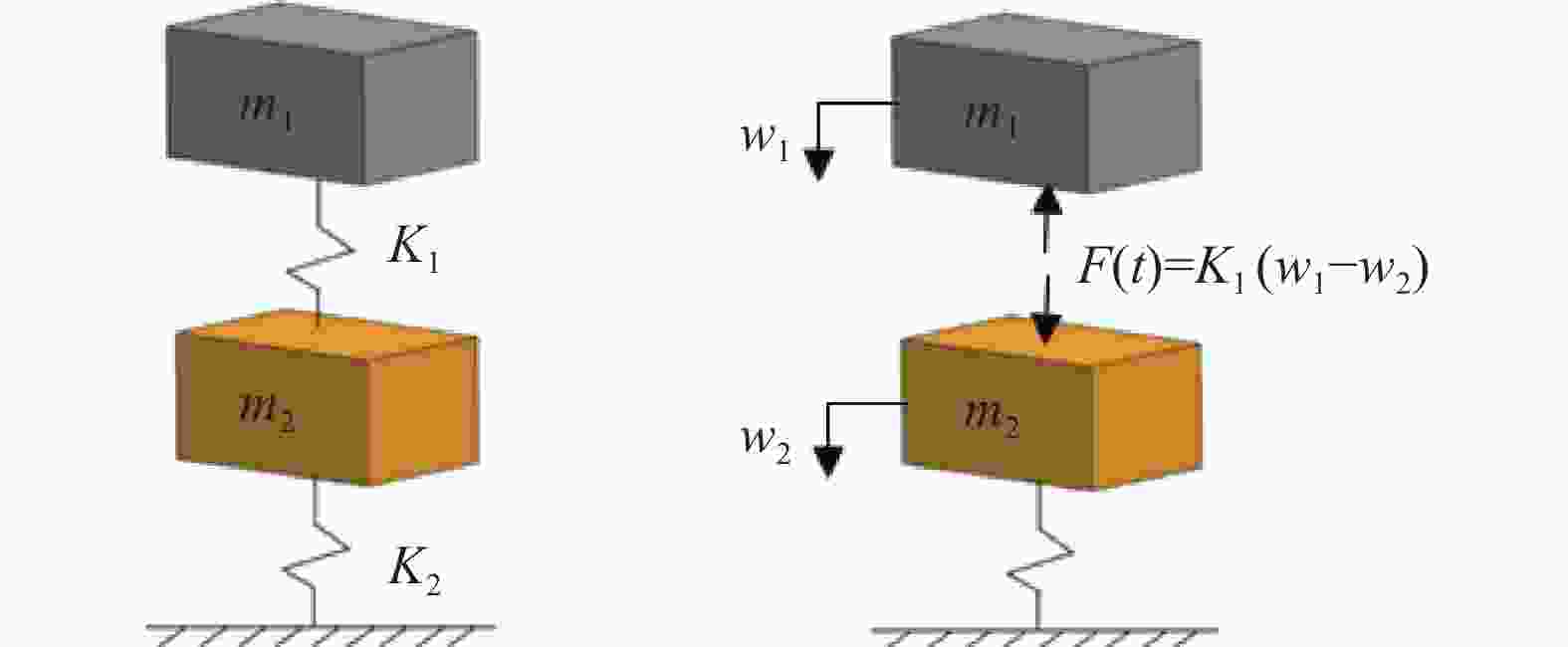
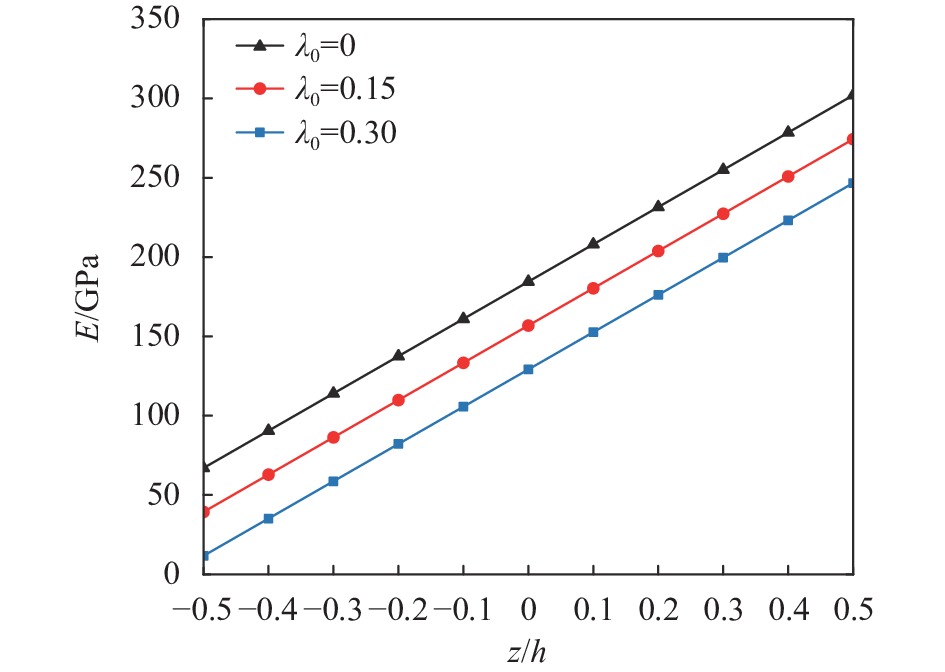
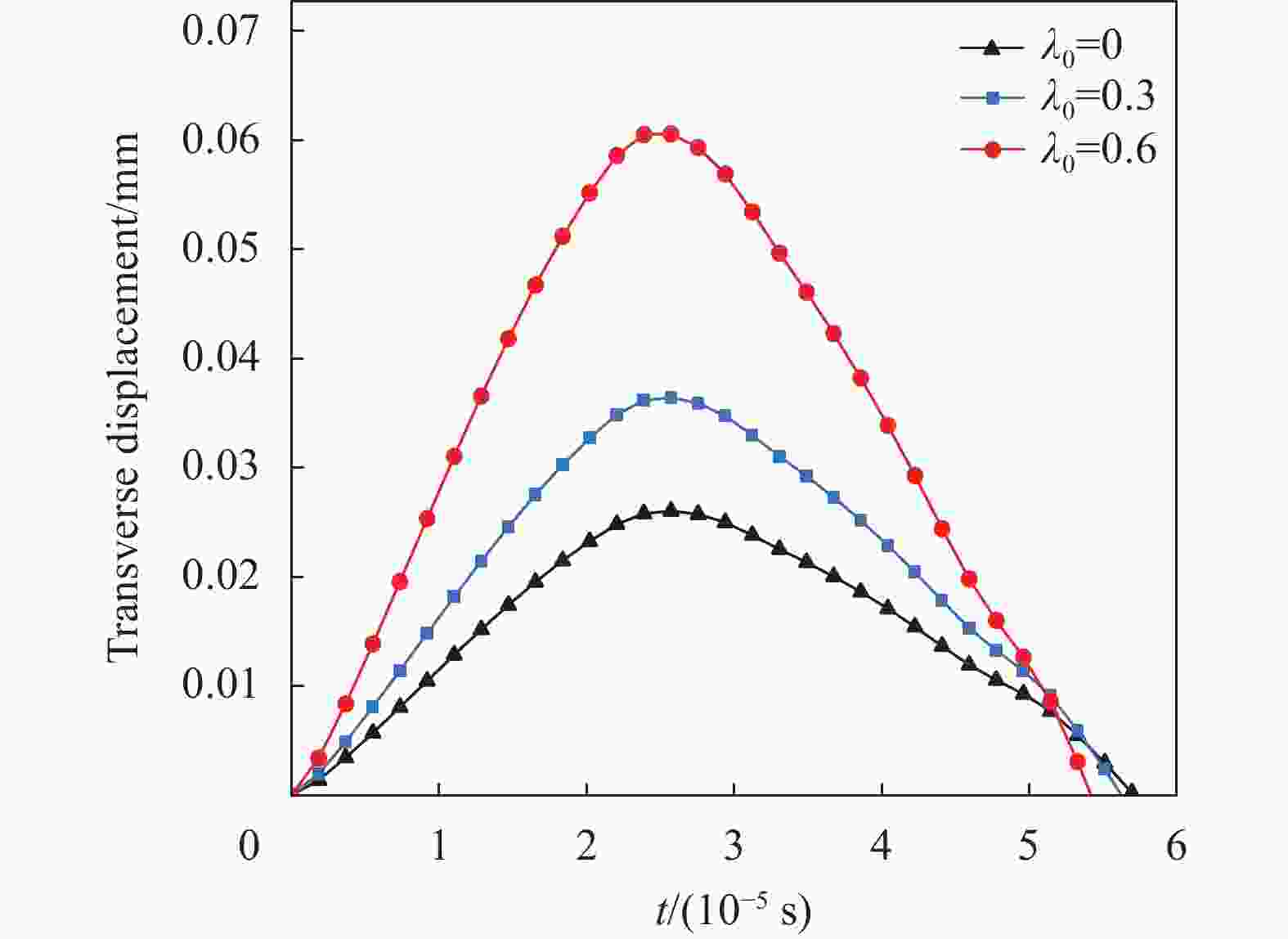
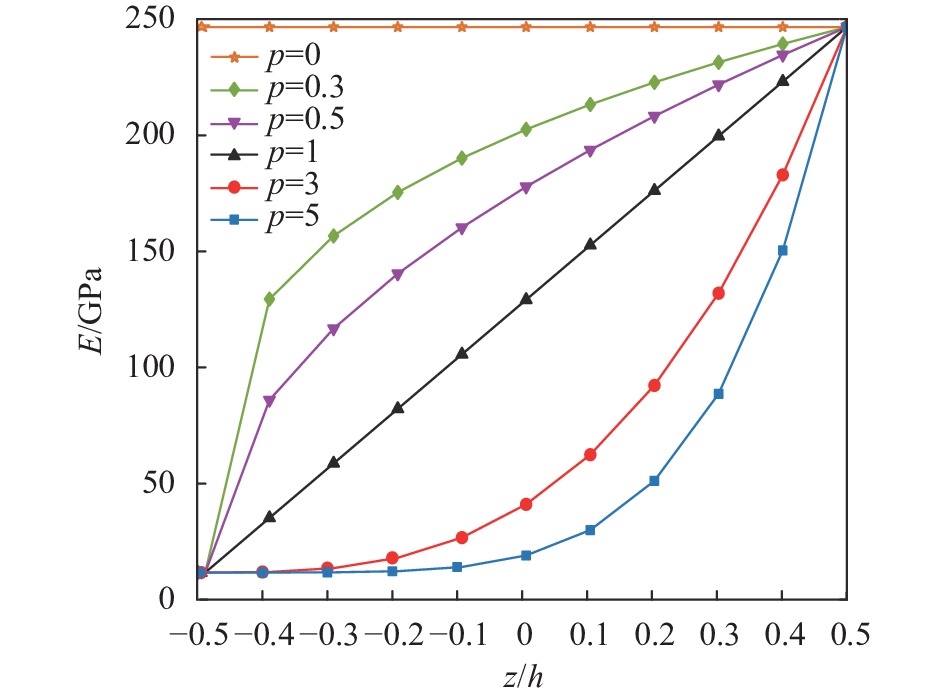
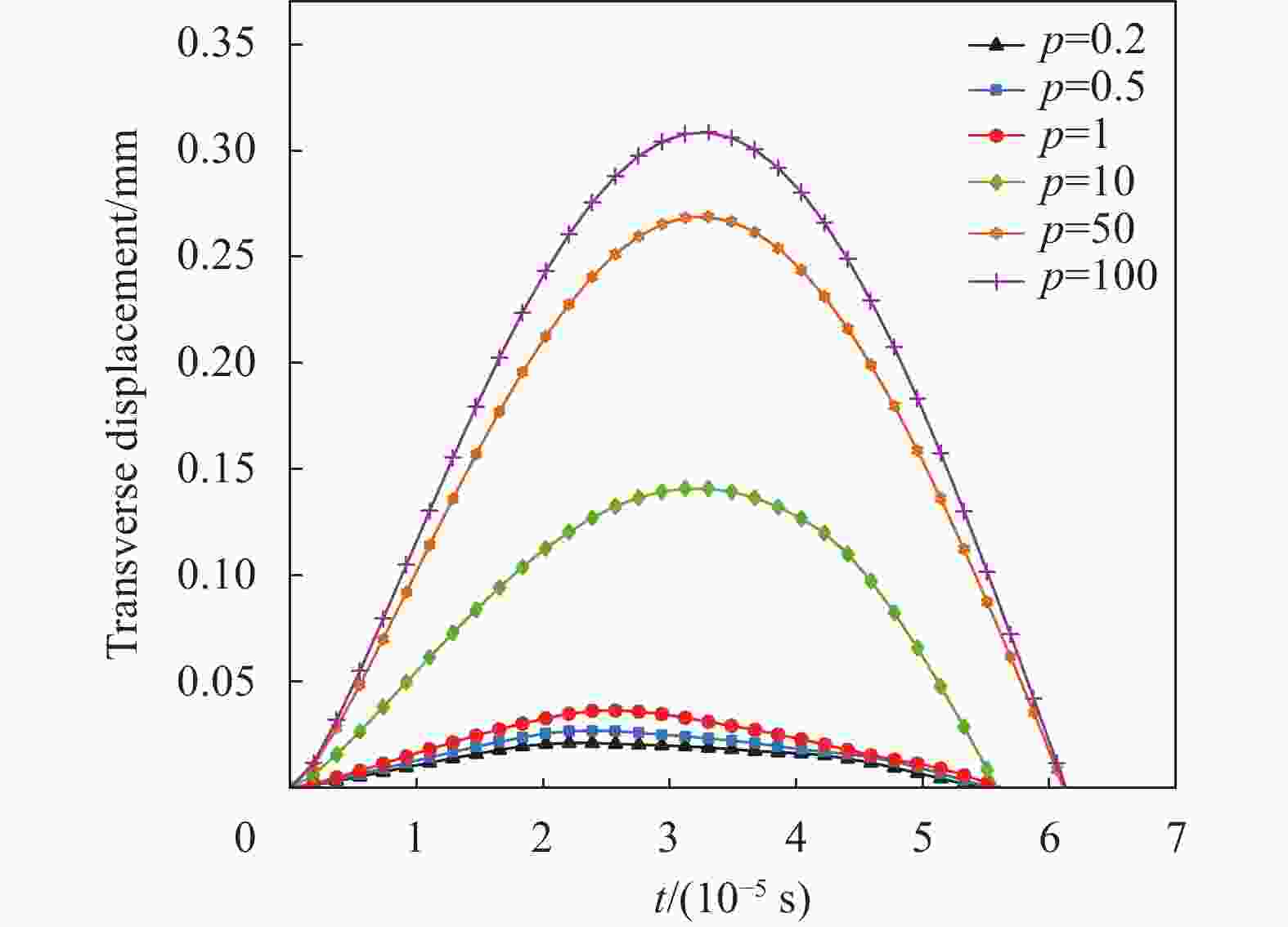
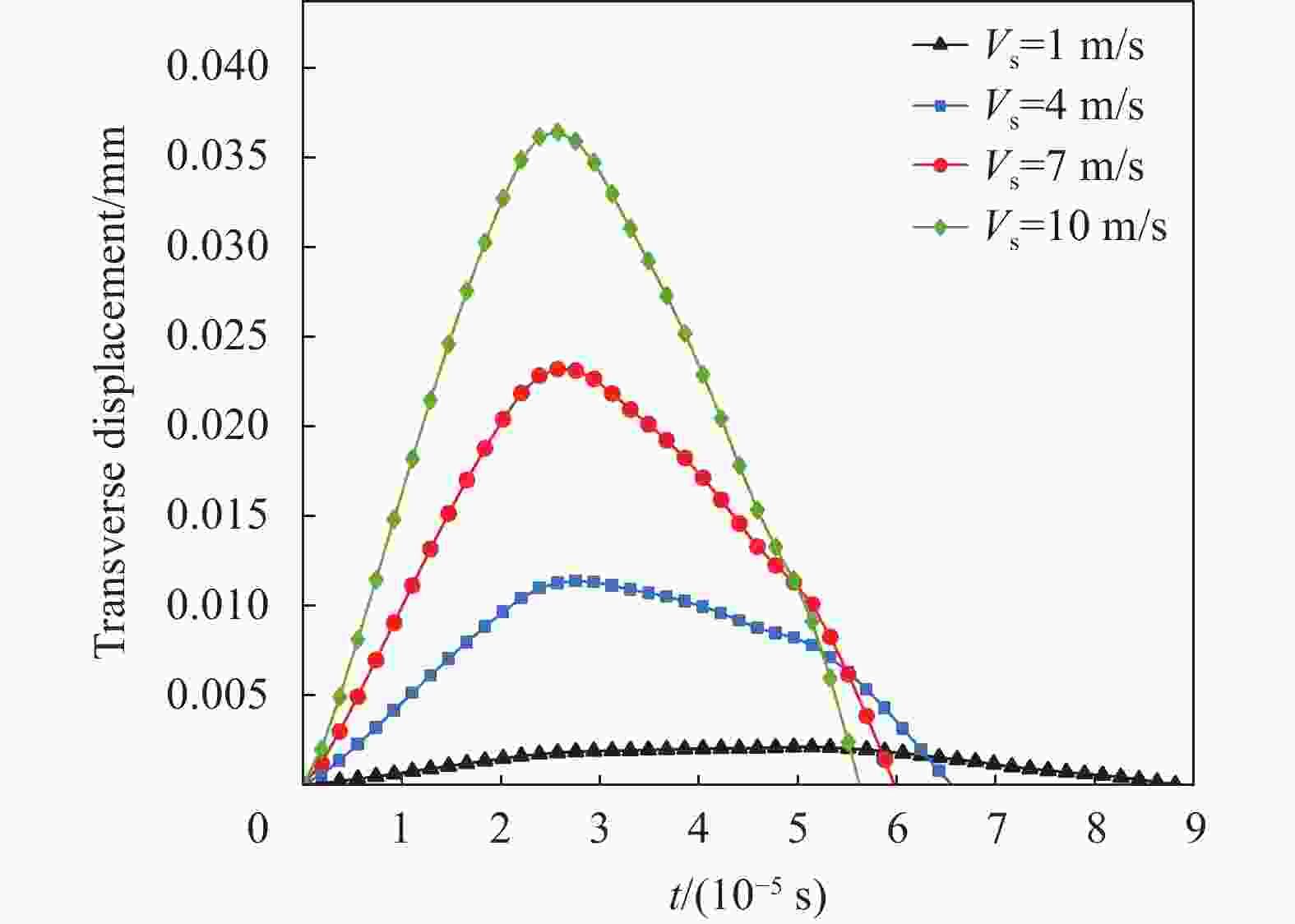
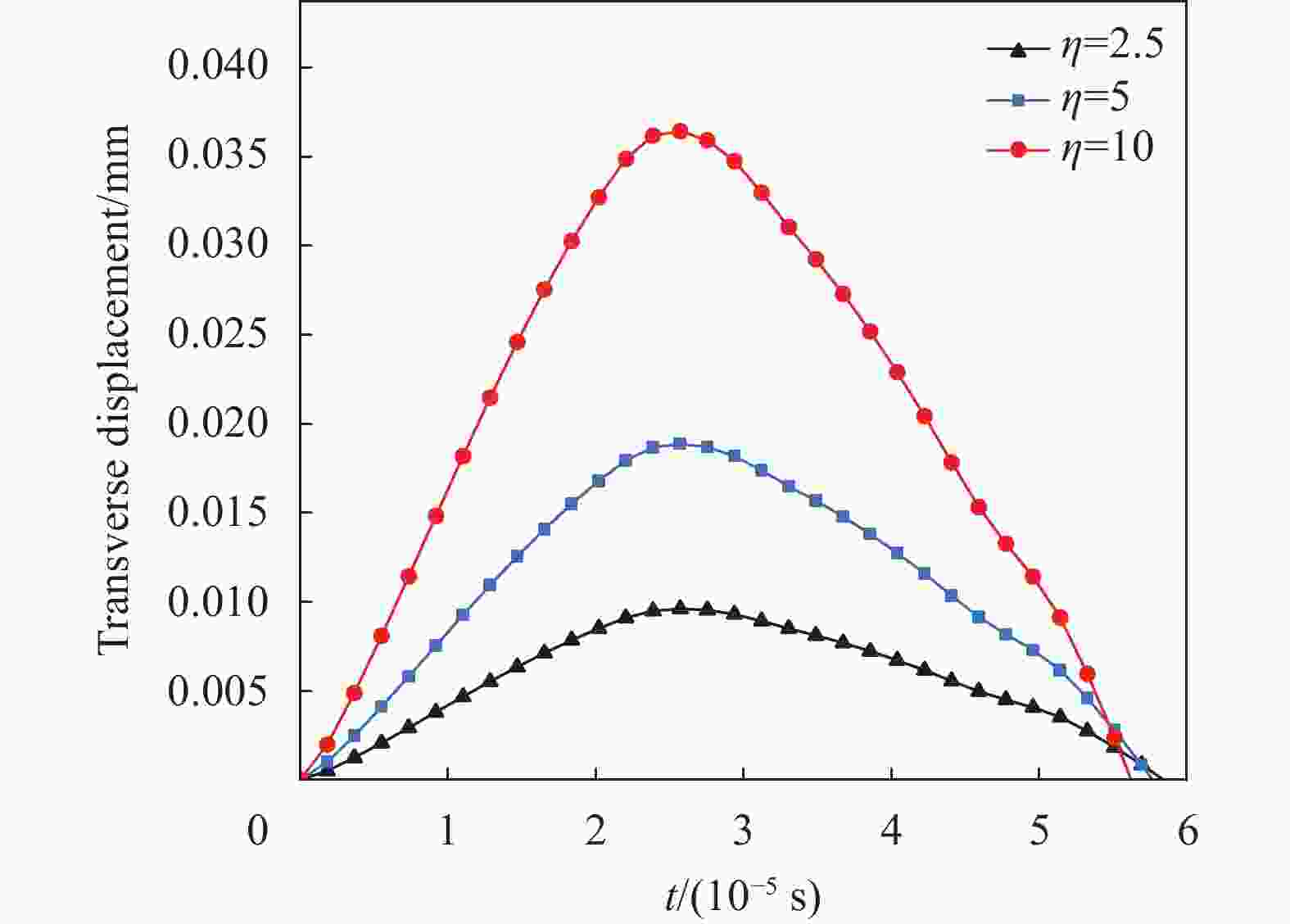
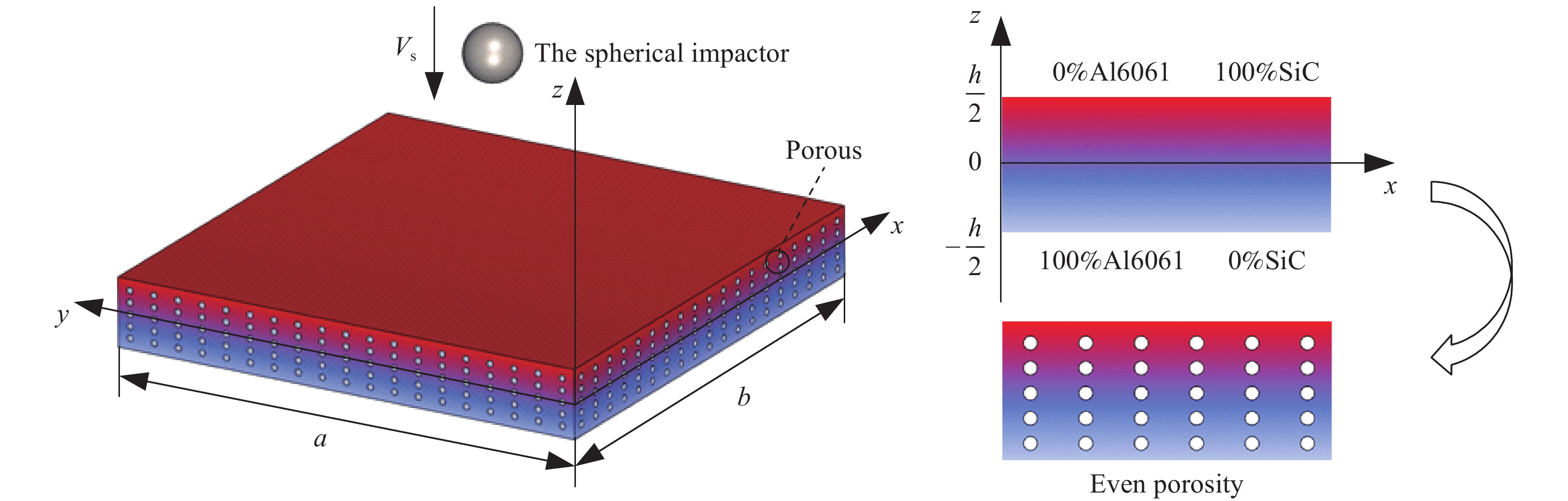
摘要: 为研究多孔金属陶瓷功能梯度矩形板在低速冲击下的动力学响应，提出了一种基于赫兹弹性理论和一阶剪切变形板理论的数值分析模型，获得了低速冲击下多孔金属陶瓷功能梯度矩形板的响应解析解。根据Hamilton原理推导出功能梯度矩形板的运动方程，引入两自由度弹簧-质量(S-M)模型用于获得冲击过程中与时间相关的接触力，利用Duhamel原理和Navier法计算了多孔功能梯度矩形板的横向位移。所得结果与文献数据进行比对，验证了有效性。在此基础上，针对相关参数对功能梯度板抗冲击性能的影响进行了比对分析。结果表明：随着孔隙率、功能梯度指数和宽厚比的减小，最大横向位移减小，功能梯度矩形板的能量吸收和抗冲击性能增强。
- 赫兹弹性理论 /
- 一阶剪切变形板理论 /
- 功能梯度矩形板 /
- 弹簧-质量模型 /
- 低速冲击
Abstract: In order to study the dynamic response of porous metal ceramic functionally graded rectangular plate under low velocity impact, a numerical analysis model based on Hertzian elastic theory and first-order shear deformation plate theory was presented, the analytical solution of response of porous cermet functionally graded rectangular plate under low velocity impact was obtained. According to Hamilton's principle, the equation of motion of functionally graded rectangular plate was derived, a spring-mass (S-M) model with two degrees of freedom was introduced to obtain the time-dependent contact forces during impact, using the Duhamel principle and Navier method to calculate the transverse displacement of porous functionally graded rectangular plate. The results obtained were compared with literature data to verify the validity. On this basis, the influence of related parameters on the impact resistance of functionally graded rectangular plate was compared and analyzed. The results show that with the decrease of porosity, functionally graded index and width to thickness ratio, the maximum transverse displacement of the functionally graded rectangular plate decreases, energy absorption and impact resistance are increased.
- Hertzian elastic theory /
- first-order shear deformation plate theory /
- functionally graded rectangular plate /
- spring-mass model /
- low velocity impact

HTML全文

图 1 多孔功能梯度材料(FGM)矩形板低速冲击模型

Figure 1. Low velocity impact model of porous functionally graded material (FGM) rectangular plate

V_s—Impact velocity of the spherical impactor; a, b and h—Length, width and thickness of the FGM rectangular plate

下载: 全尺寸图片幻灯片

图 2 冲击过程接触力与接触位移示意图

Figure 2. Schematic diagram of contact force and contact displacement during impact

m₁ and m₂—Mass of the spherical impactor and the porous FGM rectangular plate; w₁ and w₂—Displacement response of m₁ and m₂; δ—Contact displacement; F—Contact force

下载: 全尺寸图片幻灯片

图 3 两自由度弹簧-质量模型

Figure 3. Two degree of freedom spring-mass model

$ {K_1} $—Equivalent Hertz spring; $ {K_2} $—Equivalent stiffness spring

下载: 全尺寸图片幻灯片

图 4 均质板的接触力验证

Figure 4. Verification of contact force of homogeneous plate

下载: 全尺寸图片幻灯片

图 5 多孔FGM矩形板不同孔隙率λ₀下的杨氏模量E分布

Figure 5. Young's modulus E distribution of porous FGM rectangular plate with different porosity λ₀

下载: 全尺寸图片幻灯片

图 6 孔隙率对FGM矩形板横向位移的影响

Figure 6. Effect of porosity on transverse displacement of FGM rectangular plate

下载: 全尺寸图片幻灯片

图 7 多孔FGM矩形板不同功能梯度指数p下的E分布

Figure 7. E distribution of porous FGM rectangular plate under different functionally graded index p

下载: 全尺寸图片幻灯片

图 8 功能梯度指数对多孔FGM矩形板横向位移的影响

Figure 8. Effect of functionally graded index on transverse displacement of porous FGM rectangular plate

下载: 全尺寸图片幻灯片

图 9 冲击速度对多孔FGM矩形板横向位移的影响

Figure 9. Effect of impact velocity on transverse displacement of porous FGM rectangular plate

下载: 全尺寸图片幻灯片

图 10 宽厚比η对多孔FGM矩形板横向位移的影响

Figure 10. Effect of width to thickness ratio η on transverse displacement of porous FGM rectangular plate

下载: 全尺寸图片幻灯片

表 1 多孔FGM矩形板各组分材料特性

Table 1. Material properties of components of porous FGM rectangular plate

Material	Young's modulus/GPa	Poisson's ratio	Density/ (kg·m⁻³)
Al6061	67	0.33	2702
SiC	302	0.17	3100

下载: 导出CSV

表 2 含孔隙FGM板的无量纲频率验证

Table 2. Non-dimensional frequencies verification of porous FGM plate

$ \eta $	Method	Porosity	Non-dimensional frequency
$ \eta $	Method	Porosity	$ p = 0 $	$ p = 0.5 $	$ p = 1 $
20	Present	0.2	0.0300	0.0245	0.0210
20	Razaei et al^[27]	0.2	0.0300	0.0245	0.0210
10	Present	0.2	0.1170	0.0959	0.0823
10	Razaei et al^[27]	0.2	0.1170	0.0961	0.0824
5	Present	0.2	0.4284	0.3542	0.3052
5	Razaei et al^[27]	0.2	0.4313	0.3562	0.3068
Notes: $ \eta $—Width to thickness ratio; $ p $—Functionally graded index.

下载: 导出CSV

表 3 不同冲击速度下理想FGM板接触力验证

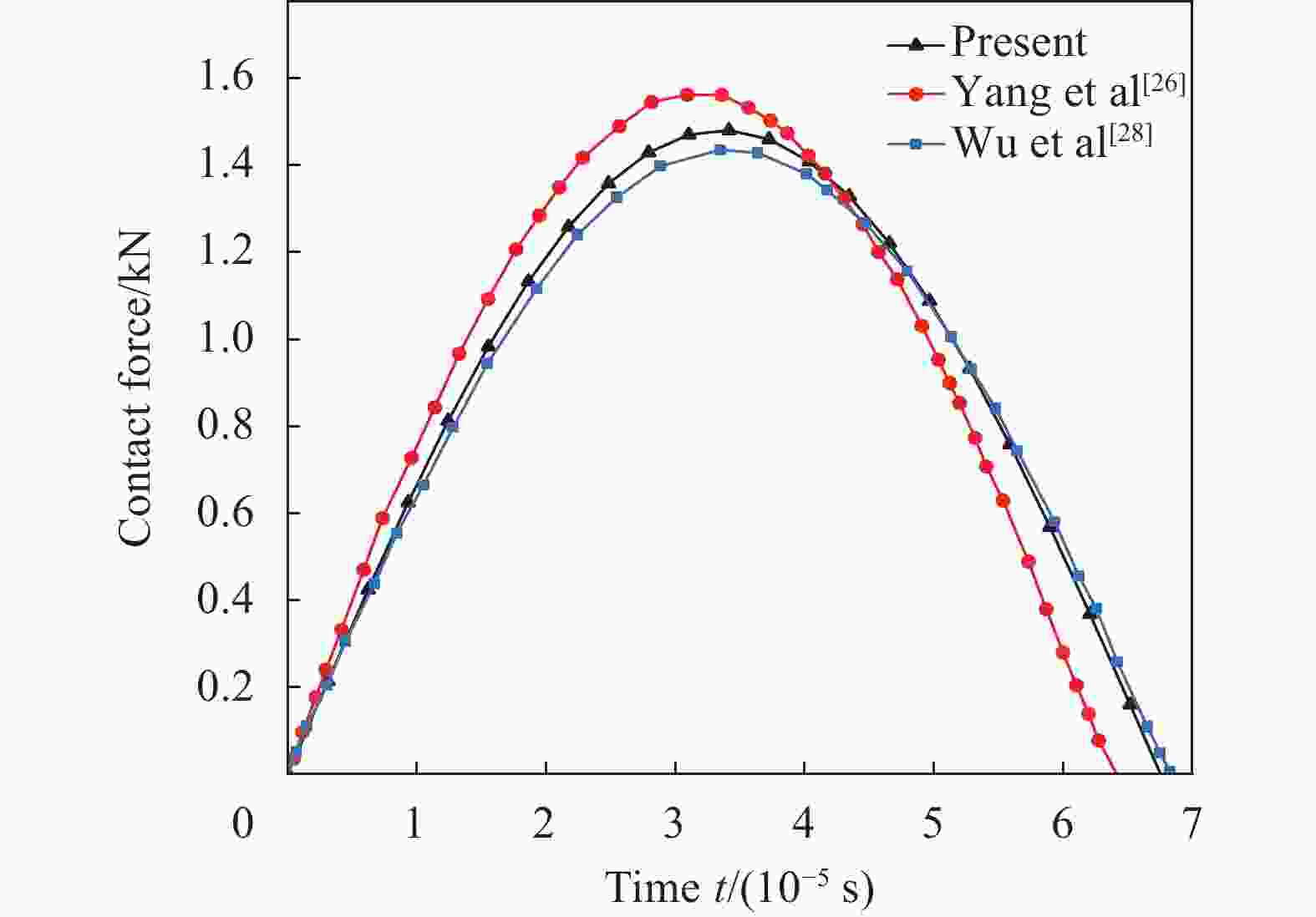
Table 3. Verification of contact force for ideal FGM plate with different impact velocities

V_s/(m·s⁻¹)	Maximum contact force/kN
V_s/(m·s⁻¹)	Khalili et al^[6]	Najafi et al^[29]	Present study	Maximum error/%
1	1.49	1.54	1.50	2.60
4	7.67	8.17	7.92	3.25
7	15.10	16.01	15.49	3.20
10	23.00	24.49	23.76	4.20

下载: 导出CSV

参考文献(29)

[1]	TAN C L, CHEW Y, BI G J, et al. Additive manufacturing of steel-copper functionally graded material with ultrahigh bonding strength[J]. Journal of Materials Science & Technology,2021,72:217-222.
[2]	KUMAR R, DUTTA S C, PANDA S K. Linear and non-linear dynamic instability of functionally graded plate subjected to non-uniform loading[J]. Composite Structures,2016,154:219-230. doi: 10.1016/j.compstruct.2016.07.050
[3]	OKTEM A S, MANTARI J L, SOARES C G. Static response of functionally graded plates and doubly-curved shells based on a higher order shear deformation theory[J]. European Journal of Mechanics A/Solids,2012,36(36):163-172.
[4]	叶仁传, 张志浩, 敖力君, 等. 冲击载荷作用下船用软芯功能梯度夹层板动态响应分析[J]. 船舶力学, 2022, 26(6):933-947. doi: 10.3969/j.issn.1007-7294.2022.06.013 YE Renchuan, ZHANG Zhihao, AO Lijun, et al. Dynamic response of shipbuilding sandwich plates with functionally graded soft core subjected to impulse loading[J]. Journal of Ship Mechanics,2022,26(6):933-947(in Chinese). doi: 10.3969/j.issn.1007-7294.2022.06.013
[5]	ABRATE S. Modeling of impacts on composite structures[J]. Composite Structures,2001,51(2):129-138. doi: 10.1016/S0263-8223(00)00138-0
[6]	KHALILI S M R, MALEKZADEH K, GORGABAD A V. Low velocity transverse impact response of functionally graded plates with temperature dependent properties[J]. Composite Structures,2013,96:64-74. doi: 10.1016/j.compstruct.2012.07.035
[7]	KIRAN M C, KATTIMANI S C, VINYAS M. Porosity influence on structural behaviour of skew functionally graded magneto-electro-elastic plate[J]. Composite Structures,2018,191:36-77. doi: 10.1016/j.compstruct.2018.02.023
[8]	DAIKH A A, ZENKOUR A M. Free vibration and buckling of porous power-law and sigmoid functionally graded sandwich plates using a simple higher-order shear deformation theory[J]. Materials Research Express,2019,6(11):115707. doi: 10.1088/2053-1591/ab48a9
[9]	KITIPORNCHAI S, CHEN D, YANG J. Free vibration and elastic buckling of functionally graded porous beams reinforced by graphene platelets[J]. Materials and Design,2017,116:656-665. doi: 10.1016/j.matdes.2016.12.061
[10]	SHOJAEEFARD M H, SAEIDI GOOGARCHIN H, GHADIRI M, et al. Micro temperature-dependent FG porous plate: Free vibration and thermal buckling analysis using modified couple stress theory with CPT and FSDT[J]. Applied Mathematical Modelling,2017,50:633-655. doi: 10.1016/j.apm.2017.06.022
[11]	WANG Y Q, WAN Y H, ZHANG Y F. Vibrations of longitudinally traveling functionally graded material plates with porosities[J]. European Journal of Mechanics A/Solids,2017,66:55-68. doi: 10.1016/j.euromechsol.2017.06.006
[12]	WANG Y Q, ZU J W. Vibration behaviors of functionally graded rectangular plates with porosities and moving in thermal environment[J]. Aerospace Science and Technology,2017,69:550-562. doi: 10.1016/j.ast.2017.07.023
[13]	ŞAHIN Ö S, GÜNEŞ A, ASLAN A, et al. Low-velocity impact behavior of porous metal matrix composites produced by recycling of bronze and iron chip[J]. Iranian Journal of Science and Technology, Transactions of Mechanical Engineering,2019,43(1):53-60. doi: 10.1007/s40997-017-0139-4
[14]	GUNES R, AYDIN M, KEMAL APALAK M, et al. Experimental and numerical investigations of low velocity impact on functionally graded circular plates[J]. Composites Part B: Engineering,2014,59:21-32. doi: 10.1016/j.compositesb.2013.11.022
[15]	刘涛, 李朝东, 汪超, 等. 基于三阶剪切变形理论的压电功能梯度板静力学等几何分析[J]. 振动与冲击, 2021, 40(1):73-85. doi: 10.13465/j.cnki.jvs.2021.01.011 LIU Tao, LI Chaodong, WANG Chao, et al. Static iso-geometric analysis of piezoelectric functionally graded plate based on third-order shear deformation theory[J]. Journal of Vibration and Shock,2021,40(1):73-85(in Chinese). doi: 10.13465/j.cnki.jvs.2021.01.011
[16]	SUN G Y, WANG E D, WANG H X, et al. Low-velocity impact behaviour of sandwich panels with homogeneous and stepwise graded foam cores[J]. Materials & Design,2018,160:1117-1136.
[17]	高晟耀, 彭德炜, 唐宇航, 等. 基于一阶剪切变形理论的功能梯度球环振动特性[J]. 复合材料学报, 2020, 37(4):935-943. doi: 10.13801/j.cnki.fhclxb.20190909.001 GAO Shengyao, PENG Dewei, TANG Yuhang, et al. Free vibration characteristics of functionally graded spherical torus based on first-order shear deformation theory[J]. Acta Materiae Compositae Sinica,2020,37(4):935-943(in Chinese). doi: 10.13801/j.cnki.fhclxb.20190909.001
[18]	LARSON R A, PALAZOTTO A N, GARDENIER H E. Impact response of titanium and titanium boride monolithic and functionally graded composite plates[J]. American Institute of Aeronautics and Astronautics,2009,47(3):676-691. doi: 10.2514/1.38577
[19]	ICARDI U, FERRERO L. Impact analysis of sandwich composites based on a refined plate element with strain energy updating[J]. Composite Structures,2009,89(1):35-51. doi: 10.1016/j.compstruct.2008.06.018
[20]	MAO Y Q, FU Y M. Nonlinear dynamic response and active vibration control for piezoelectric functionally graded plate[J]. Journal of Sound and Vibration,2010,329(11):2015-2028. doi: 10.1016/j.jsv.2010.01.005
[21]	VLOT A. Impact properties of fibre metal laminates[J]. Composites Engineering,1993,3(10):911-927.
[22]	钟锐, 胡双卫, 秦斌, 等. 功能梯度开孔平行四边形板的等几何振动分析[J]. 哈尔滨工程大学学报, 2022, 43(7):999-1005. doi: 10.11990/jheu.202103048 ZHONG Rui, HU Shuangwei, QIN Bin, et al. Isogeometric vibration analysis of functionally graded perforated parallelogram plates with holes[J]. Journal of Harbin Engineering University,2022,43(7):999-1005(in Chinese). doi: 10.11990/jheu.202103048
[23]	ZHOU C L, ZHAN Z X, ZHANG J, et al. Vibration analysis of FG porous rectangular plates reinforced by graphene platelets[J]. Steel and Composite Structures,2020,34(2):215-226.
[24]	REDDY J N. Mechanics of laminated composite plates and shells: Theory and analysis[M]. Boca Raton: CRC Press, 2004: 575-578.
[25]	TAN T M, SUN C T. Use of statical indentation laws in the impact analysis of laminated composite plates[J]. Journal of Applied Mechanics,1985,52(1):6-12. doi: 10.1115/1.3169029
[26]	YANG F L, WANG Y Q, LIU Y F. Low-velocity impact response of axially moving functionally graded graphene platelet reinforced metal foam plates[J]. Aerospace Science and Technology,2022,123:107496. doi: 10.1016/j.ast.2022.107496
[27]	REZAEI A S, SAIDI A R, ABRISHAMDARI M, et al. Natural frequencies of functionally graded plates with porosities via a simple four variable plate theory: An analytical approach[J]. Thin-Walled Structures,2017,120:366-377. doi: 10.1016/j.tws.2017.08.003
[28]	WU H Y T, CHANG F K. Transient dynamic analysis of laminated composite plates subjected to transverse impact[J]. Computers and Structures,1989,31(3):453-466. doi: 10.1016/0045-7949(89)90393-3
[29]	NAJAFI F, SHOJAEEFARD M H, SAEIDI GOOGARCHIN H. Low-velocity impact response of functionally graded doubly curved panels with Winkler-Pasternak elastic foundation: An analytical approach[J]. Composite Structures,2017,162:351-364. doi: 10.1016/j.compstruct.2016.11.094