基于多尺度模拟的橡胶/钢帘线复合材料辐射散热行为机制研究

周家智; 肖慧萍; 谢小林; 周志嵩; 安林; 李文博

doi:10.13801/j.cnki.fhclxb.20240223.001

基于多尺度模拟的橡胶/钢帘线复合材料辐射散热行为机制研究

周家智¹,
肖慧萍¹,
谢小林¹,
周志嵩²,
安林³,
李文博^{1, 2, ,}

1.
南昌航空大学　材料科学与工程学院，南昌 330000
2.
江苏兴达钢帘线股份有限公司，泰州 225721
3.
青岛科技大学　高分子科学与工程学院，青岛 266000

基金项目: 国家自然科学基金(52063021)；江西省重点研发计划项目(20224BBE51049)

详细信息

通讯作者:
李文博，博士，讲师，研究方向为橡胶制品设计与仿真分析　E-mail: 645504616@qq.com

中图分类号: TB333
计量
- 文章访问数: 310
- HTML全文浏览量: 207
- PDF下载量: 21
出版历程
- 收稿日期: 2023-11-29
- 修回日期: 2024-01-14
- 录用日期: 2024-01-27
- 网络出版日期: 2024-02-25
- 刊出日期: 2024-10-14

Study on the mechanism of radiation heat dissipation behavior of rubber/steel cord composites based on multi-scale simulation

1.
School of Materials Science and Engineering, Nanchang Hangkong University, Nanchang 330000, China
2.
Jiangsu Xingda Steel Cord Co., Ltd., Taizhou 225721, China
3.
School of Polymer Science and Engineering, Qingdao University of Science and Technology, Qingdao 266000, China

Funds: National Natural Science Foundation of China (52063021); Jiangxi Province Key Research and Development Program (20224BBE51049)

摘要

摘要: 橡胶/钢帘线复合材料的传热与温度场分析对橡胶制品的硫化成型、热氧老化、热疲劳寿命研究具有重要意义。本文基于多尺度传热模型对不同钢帘线占比、排列角度和温升工况下的橡胶/钢帘线复合材料传热和散热机制进行研究，并通过实验验证。结果表明，橡胶/钢帘线复合材料呈现明显的各向异性传热行为，传热界面的热流聚集效应会加速热量的层间扩散，使温度分布更均匀。模拟计算得到的辐射散热发射率高达0.95，且随着钢帘线占比增大和温度升高，辐射散热行为越明显。对比串并联传热模型，多尺度传热模型预测误差从10.1%减小到2.5%。
- 橡胶/钢帘线复合材料 /
- 辐射散热 /
- 多尺度模型 /
- 传热机制 /
- 流体动力学模型
Abstract: Heat transfer and temperature field analysis of rubber steel/cord composites are important for the study of vulcanization molding, thermo-oxidative aging, and thermal fatigue life of rubber products. In this paper, the heat transfer and heat dissipation mechanisms of rubber/steel cord composites with different steel cord ratios, laminate angles and temperature rise operating conditions are investigated based on a multi-scale heat transfer model and experimentally verified. The results show that the rubber/steel cord composites exhibit obvious anisotropic heat transfer behavior, and the heat flow aggregation effect at the heat transfer interface accelerates the interlayer diffusion of heat for more uniform temperature distribution. The radiative heat dissipation emissivity obtained from the simulation is as high as 0.95, and the radiative heat dissipation behavior is more pronounced as the percentage of steel cord increases and the temperature rises. Compared to the series-parallel heat transfer model, the prediction error of the multiscale heat transfer model is reduced from 10.1% to 2.5%.
- rubber/steel cord composites /
- radiant heat dissipation /
- multi-scale modeling /
- heat transfer mechanism /
- CFD

HTML全文

空气悬架作为一种可实现高度调节的车辆半主动悬架，越来越受到关注。空气悬架的核心是可通过充放气调节高度和刚度的空气弹簧(下简称空簧)。空簧的有效面积被定义为空簧支承力与内部气体相对压强的比值，其特性对于空气悬架的准确建模与控制设计十分关键。然而，这是一个等效物理量，无法直接测量得到。

在实际中，空簧有效面积主要是空簧高度和内部压强的函数，且存在一定的非线性特征。文献中研究有效面积主要采用有限元仿真^[1-4]、几何图解^[5-14]、实验拟合^[15-20]等手段。成小霞等^[3]、胡德安等^[4]提出的有效面积确定方法结合有限元工具进行了验证。杨泽彪^[7]给出一种几何意义上的有效面积定义，确定了有效面积及其随高度的变化率。其他几何图解方法也沿用了文献[7]中的一些变形规律假设。罗贤光^[10]分析了不同受力状态下的囊式空簧有效面积情况。Li等^[13-14]在对轨道车辆空簧刚度的分析中给出了有效面积变化率的图解公式。不同形式的空簧有效面积变化规律也有差异^[17]。部分空气悬架设计与建模工作采用空簧活塞面积或最大外径面积^[16]代替有效面积，精度不高。采用实验拟合方法确定的有效面积规律一般随高度以线性^[19-20]或多项式^[15]规律变化。

文献中大多仅考虑空簧有效面积与高度的关系^[18-21]，对其随压强变化规律研究相对较少。在不同车辆载荷下，即使空簧处于同一高度，其内部压强也会变化，进而显著影响有效面积和悬架动力学性能。本文结合复合材料力学与几何学分析，提出一种新的车用膜式空簧的有效面积预测方法，探究空簧各参量(尤其是压强)对有效面积变化的影响，并与实验结果进行比对以验证其合理性。

1. 空气弹簧有效面积计算的理论分析

空簧气囊结构一般如图1所示，通过钢丝圈紧箍密闭，以中心帘线-橡胶层为主要承力部位，内外涂以胶层。常用于乘用车的空簧一般为膜式空簧，活塞上下移动时通过气囊壁垂耳在活塞边缘翻卷以改变空簧高度。一般来说，空簧有效面积A_eff与其高度和内部压强相关：

图 1 空簧气囊的典型结构

Figure 1. Structure of air spring rubber reservoir

1—Bead wire; 2—Cord-rubber ply; 3—Outer layer; 4—Inner layer

下载: 全尺寸图片幻灯片

${A_{{\rm{eff}}}}\; = \;{A_{{\rm{eff1}}}}(p)\; + \;{A_{{\rm{eff2}}}}(H)$

(1)

式中：p为气囊内部的相对压强；H为空簧气囊高度。

在气囊工作范围内可视其横截面为圆形，其纵截面如图2所示。这里取远离上下盖板及垂耳处、高度为dy的微元高度横切气囊壁进行分析，横切气囊壁微元段在径向和周向的受力情况如图3、图4所示。

图 2 膜式空簧纵截面示意

Figure 2. Longitudinal section of rolling lobe air spring

R—Radius of cross sectional bellow; R_eff—Radius of effective area

下载: 全尺寸图片幻灯片

图 3 微元高度横切气囊壁的受力分析

Figure 3. Mechanical analysis of cross sectional bellow wall with infinitesimal height

P—Inner pressure of air spring; dT—Vertical force in infinitesimal circumferential length; dθ—Infinitesimal central angle; dy—Infinitesimal height

下载: 全尺寸图片幻灯片

图 4 气囊壁微元体平面内受力状态

Figure 4. Stress diagram of bellow wall microbody in plane

dF—Circumferential force in infinitesimal circumferential length

下载: 全尺寸图片幻灯片

在图3中，dθ为横切气囊壁微元段沿经线方向分割后对应的微元圆心角。由受力平衡，有：

$p{\rm{d}}A\; = \;p{\rm{d}}yR{\rm{d}}\theta \;{\rm{ = }}\;{\rm{2d}}T\sin \frac{{{\rm{d}}\theta }}{2}\; = \;{\rm{d}}T{\rm{d}}\theta$

(2)

式中：dA为微元面积；R为横切面的半径；dT为垂向的微元力。假定横切面在垂向上受到的与有效面积相关的拉力F₀为

${F_0}\; = \;\delta p{\rm{{\text{π}} }}{R_{{\rm{eff}}}}^2$

(3)

式中：δ为形状参数；R_eff为有效面积对应的半径。

进一步进行经线方向和纬线方向的受力分析：

$\left\{ \begin{array}{l} {\rm{d}}T\; = \;pR{\rm{d}}y\;{\rm{ = }}\;T{\rm{d}}y \\ {\rm{d}}F\; = \;\dfrac{{{F_0}}}{{2{\rm{{\text{π}} }}R}}R{\rm{d}}\theta \; = \;\dfrac{{p\delta }}{2}\dfrac{{{R_{{\rm{eff}}}}^2}}{R}R{\rm{d}}\theta \; = \;F R{\rm{d}}\theta \\ \end{array} \right.$

(4)

式中：dF为周向的微元力；T、F分别为垂向与周向单位长度上产生的力。由于取的微元体很小，近似符合平面应力条件，可将圆柱坐标系转化为随体直角坐标系。在该直角坐标系中，忽略内外气压差对垂直于平面方向应变的影响，结合转换关系dx=Rdθ，该微元体在随体坐标系下平面内受力状态如图4所示。

下面对微元体的材料结构进行分析。忽略内外胶层对气囊力学特性的影响，假定完全由帘线-橡胶层承载气囊所受的力。橡胶在大变形情况下具有非线性力学特性，但考虑到气囊是帘线增强的复合材料结构，在力学分析时为简化起见用线弹性材料描述橡胶基体行为。基于此假设，将帘线-橡胶看作正交各向异性复合材料，其中，橡胶作为基体提供支撑，尼龙帘线作为增强体起主要承力作用。

单层帘线-橡胶材料的帘线铺层随体坐标与主方向定义如图5所示。图中的x、y随体坐标轴方向分别对应于前述的空簧气囊的纬线方向与经线方向，1、2分别对应平行与垂直帘线的主方向。

图 5 微元体随体坐标与主方向定义

Figure 5. Definition of local coordinate and principle direction of microbody

H₀—Initial height of air spring; α—Ply angle; L—Length of cords

下载: 全尺寸图片幻灯片

结合上述假设，利用复合材料单层板理论^[22]，单层板1号主方向上的弹性模量E₁、2号主方向的弹性模量E₂、复合材料2-1方向泊松比ν₂₁与1-2方向剪切模量G₁₂的表达式分别为

${E_1}\; = \;{E_{\rm{f}}}{C_{\rm{f}}}\; + \;{E_{\rm{m}}}(1\; - \;{C_{\rm{f}}}{\rm{)}}$

(5)

${E_2}\; = \;\frac{{{E_{\rm{f}}}{E_{\rm{m}}}}}{{{C_{\rm{m}}}{E_{\rm{f}}}\; + \;{C_{\rm{f}}}{E_{\rm{m}}}}}$

(6)

${\nu _{21}}\; = \;{C_{\rm{f}}}{\nu _{\rm{f}}}\; + \;{\nu _{\rm{m}}}(1\; - \;{C_{\rm{f}}})$

(7)

${G_{12}}\; = \;\frac{{{G_{\rm{m}}}{G_{\rm{f}}}}}{{{G_{\rm{m}}}{C_{\rm{f}}}\; + \;{G_{\rm{f}}}(1\; - \;{C_{\rm{f}}})}}$

(8)

式中，E_f、C_f、ν_f和G_f分别表示帘线的弹性模量、相对体积含量、泊松比和剪切模量，与之相对应的m下标四个参量均为橡胶的等效材料参量。采用线弹性小变形的假设，存在如下关系^[23]：

${E_{\rm{m}}}\; = \;\frac{{9KG}}{{3K\; + \;G}}$

(9)

其中，K、G分别为橡胶材料等效的体积模量与剪切模量。值得注意的是，不同理论可对复合材料参数给予不同的预报，这里选择了较通用的一种，暂不研究参数预报方法的准确性。

根据前述假设得到的简化本构方程为

$\left( {\begin{array}{*{20}{c}} {{\varepsilon _1}} \\ {{\varepsilon _2}} \\ {{\gamma _{12}}} \end{array}} \right)\; = \;{\boldsymbol{S}}\left( {\begin{array}{*{20}{c}} {{\sigma _1}} \\ {{\sigma _2}} \\ {{\tau _{12}}} \end{array}} \right)\; = \;\left( {\begin{array}{*{20}{c}} {{S_{11}}}&{{S_{12}}}&0 \\ {{S_{21}}}&{{S_{22}}}&0 \\ 0&0&{{S_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{\sigma _1}} \\ {{\sigma _2}} \\ {{\tau _{12}}} \end{array}} \right)\;$

(10)

其中：σ₁、ε₁、σ₂、ε₂、γ₁₂与τ₁₂分别为面内1方向、2方向和1-2切向的应力与应变；S为柔度矩阵。进一步利用折减刚度矩阵Q表示本构方程：

$\begin{split} &\left( {\begin{array}{*{20}{c}} {{\sigma _1}} \\ {{\sigma _2}} \\ {{\tau _{12}}} \end{array}} \right)\; = \;{\boldsymbol{Q}}\left( {\begin{array}{*{20}{c}} {{\varepsilon _1}} \\ {{\varepsilon _2}} \\ {{\gamma _{12}}} \end{array}} \right)\; =\\ &\;\left( {\begin{array}{*{20}{c}} {{Q_{11}}}&{{Q_{12}}}&0 \\ {{Q_{21}}}&{{Q_{22}}}&0 \\ 0&0&{{Q_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{\varepsilon _1}} \\ {{\varepsilon _2}} \\ {{\gamma _{12}}} \end{array}} \right)\; = \;{{\boldsymbol{S}}^{ - 1}}\left( {\begin{array}{*{20}{c}} {{\varepsilon _1}} \\ {{\varepsilon _2}} \\ {{\gamma _{12}}} \end{array}} \right) \end{split}$

(11)

其中：

$\begin{split} &{Q_{11}}\; = \;\frac{{{E_1}}}{{1\; - \;{\nu _{21}}{\nu _{12}}}},\;\;{Q_{12}}\; = \;{\nu _{12}}{Q_{11}},\\ &{Q_{22}}\; = \;\frac{{{E_2}}}{{1\; - \;{\nu _{21}}{\nu _{12}}}},\;\;{Q_{66}}\; = \;{G_{12}} \end{split}$

(12)

本构方程(11)中存在的四个独立的弹性常数，可利用前述预报公式求得，式(12)中1-2方向的泊松比ν₁₂利用麦克斯韦方程式计算。进一步结合转移矩阵T进行复合材料的转轴转化：

$\begin{split} & {\boldsymbol{T}}{\left( {\begin{array}{*{20}{c}} {{\sigma _x}}&{{\sigma _y}}&{{\tau _{xy}}} \end{array}} \right)^{\rm{T}}}\; = \;{\left( {\begin{array}{*{20}{c}} {{\sigma _1}}&{{\sigma _2}}&{{\tau _{12}}} \end{array}} \right)^{\rm{T}}} \\ & {\boldsymbol{T}}{\left( {\begin{array}{*{20}{c}} {{\varepsilon _x}}&{{\varepsilon _y}}&{\dfrac{1}{2}{\gamma _{xy}}} \end{array}} \right)^{\rm{T}}}\; = \;{\left( {\begin{array}{*{20}{c}} {{\varepsilon _1}}&{{\varepsilon _2}}&{\dfrac{1}{2}{\gamma _{12}}} \end{array}} \right)^{\rm{T}}} \end{split}$

(13)

$\left( {\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {{\sigma _x}} \\ {{\sigma _y}} \end{array}} \\ {{\tau _{xy}}} \end{array}} \right) = \;{{\boldsymbol{T}}^{ - 1}}{\boldsymbol{Q}}{({{\boldsymbol{T}}^{ - 1}})^{\rm{T}}}\left( {\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {{\varepsilon _x}} \\ {{\varepsilon _y}} \end{array}} \\ {{\gamma _{xy}}} \end{array}} \right) = \;{\boldsymbol{\bar Q}}\left( {\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {{\varepsilon _x}} \\ {{\varepsilon _y}} \end{array}} \\ {{\gamma _{xy}}} \end{array}} \right)$

(14)

式中：σ_x、ε_x、σ_y、ε_y、γ_xy与τ_xy分别为面内x方向、y方向和x-y切向的应力与应变； $\bar{\boldsymbol{Q}}$ 为转轴折减刚度矩阵。

下面对多层帘线-橡胶增强复合材料特性进行分析。当前用于乘用车的空簧帘线铺层一般采用偶数反对称铺设，帘线角度铺设形式类似于[+α/…/−α/+α/…/−α]，α为正值帘线铺层角。图6为四层反对称层合板的示意，t为总厚度。根据复合材料宏观力学理论^[22]，层合板边缘单位长度受力向量N为

图 6 四层反对称层合板示意

Figure 6. 4-layer antisymmetric laminate

t—Thickness of the laminate

下载: 全尺寸图片幻灯片

${\boldsymbol{N}}\; = \;\int_{ - \frac{t}{2}}^{\frac{t}{2}} {{\boldsymbol{\sigma }}{\rm{d}}z} \; = \;{\boldsymbol{A}}{{\boldsymbol{\varepsilon }}^0}\; + \;{\boldsymbol{BK}}$

(15)

$\begin{split} & {\boldsymbol{A}}\; = \;\displaystyle\sum\limits_{k\; = \;1}^n {{{{\boldsymbol{\bar Q}}}_k}({z_k}\; - \;{z_{k - 1}})} ,\;\;{\boldsymbol{B}}\; = \;\dfrac{1}{2}\displaystyle\sum\limits_{k\; = \;1}^n {{{{\boldsymbol{\bar Q}}}_k}({z_k}^2\; - \;{z_{k - 1}}^2)} \\ & {{\boldsymbol{\varepsilon }}^0}\; = \;{\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}&{\varepsilon _y^0}&{\gamma _{xy}^0} \end{array}} \right)^{\rm{T}}},\;\;{\boldsymbol{K}}\; = \;{\left( {\begin{array}{*{20}{c}} {{K_x}}&{{K_y}}&{{K_{xy}}} \end{array}} \right)^{\rm{T}}} \end{split}$

(16)

其中：A、B分别为拉伸、耦合刚度矩阵；ε⁰、K分别为中面应变向量、中面曲率向量； $\bar{\boldsymbol{Q}}$ _k为第k层的转轴折减刚度矩阵。偶数层反对称层合板的A、B矩阵有恒零项，且存在拉扭耦合。进一步将式(15)中A、B展开，写为

$\begin{split} {\boldsymbol{N}}\; = \;\left( {\begin{array}{*{20}{c}} {{N_x}} \\ {{N_y}} \\ {{N_{xy}}} \end{array}} \right)\; = &\;\left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&0 \\ {{A_{12}}}&{{A_{22}}}&0 \\ 0&0&{{A_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \\ {\gamma _{xy}^0} \end{array}} \right) + \\ &\left( {\begin{array}{*{20}{c}} 0&0&{{B_{16}}} \\ 0&0&{{B_{26}}} \\ {{B_{16}}}&{{B_{26}}}&0 \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{K_x}} \\ {{K_y}} \\ {{K_{xy}}} \end{array}} \right) \end{split}$

(17)

考虑到本文主要关注气囊x、y方向上的宏观作用力产生的应变，忽略层合板的扭曲率，即K_xy = 0，结合式(4)，简化式(17)为

$\begin{split} \left( {\begin{array}{*{20}{c}} T \\ F \end{array}} \right)\; =& \;\left( {\begin{array}{*{20}{c}} {{N_x}} \\ {{N_y}} \end{array}} \right)\; = \;\left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}} \\ {{A_{12}}}&{{A_{22}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \end{array}} \right)\; = \\ &\frac{t}{n}\left( {\begin{array}{*{20}{c}} {\displaystyle\sum\limits_{k\; = \;1}^n {{{\bar Q}_{11}}} }&{\displaystyle\sum\limits_{k\; = \;1}^n {{{\bar Q}_{12}}} } \\ {\displaystyle\sum\limits_{k\; = \;1}^n {{{\bar Q}_{12}}} }&{\displaystyle\sum\limits_{k\; = \;1}^n {{{\bar Q}_{22}}} } \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \end{array}} \right) \end{split}$

(18)

其中，n为层数。由上文的简化可见，拉扭耦合矩阵B对有效面积计算没有影响。结合层合板的反对称性，式(18)进一步可以简化为

$\begin{split} \left( {\begin{array}{*{20}{c}} T \\ F \end{array}} \right)\; = &\;\frac{t}{n} \times n\left( {\begin{array}{*{20}{c}} {{{\bar Q}_{11}}}&{{{\bar Q}_{12}}} \\ {{{\bar Q}_{12}}}&{{{\bar Q}_{22}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \end{array}} \right)\; = \\ &t\left( {\begin{array}{*{20}{c}} {{{\bar Q}_{11}}}&{{{\bar Q}_{12}}} \\ {{{\bar Q}_{12}}}&{{{\bar Q}_{22}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \end{array}} \right) \end{split}$

(19)

注意到转轴折减刚度矩阵中并非所有项都需要经过计算得出，可得 ${\bar{\boldsymbol{Q}}}_{k}$ 部分项的表达式为

$\left( {\begin{array}{*{20}{c}} {{{\bar Q}_{{\rm{11}}}}} \\ {{{\bar Q}_{{\rm{22}}}}} \\ {{{\bar Q}_{{\rm{12}}}}} \end{array}} \right)\;{\rm{ = }}\;\left( {\begin{array}{*{20}{l}} {{{\cos }^4}\alpha }&{{{\sin }^4}\alpha }&{2{{\cos }^2}\alpha {{\sin }^2}\alpha }&{4{{\cos }^2}\alpha {{\sin }^2}\alpha } \\ {{{\sin }^4}\alpha }&{{{\cos }^4}\alpha }&{2{{\cos }^2}\alpha {{\sin }^2}\alpha }&{4{{\cos }^2}\alpha {{\sin }^2}\alpha } \\ {{{\cos }^2}\alpha {{\sin }^2}\alpha }&{{{\cos }^2}\alpha {{\sin }^2}\alpha }&{{{\sin }^4}\alpha \; + \;{{\cos }^4}\alpha }&{ - 4{{\cos }^2}\alpha {{\sin }^2}\alpha } \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{Q_{11}}} \\ {{Q_{22}}} \\ {{Q_{12}}} \\ {{Q_{66}}} \end{array}} \right)$

(20)

对微元体受力平衡方程式(4)进行转化：

$\left\{ \begin{array}{l} T\; = \;pR \\ F\; = \;\dfrac{{p\delta }}{2}\dfrac{{{R_{{\rm{eff}}}}^2}}{R}\; = \;p{R_\delta },\;\;\;{R_\delta }{\rm{ = }}\dfrac{\delta }{2}\dfrac{{{R_{{\rm{eff}}}}^2}}{R} \\ \end{array} \right.$

(21)

由(19)可得：

$\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0} \\ {\varepsilon _y^0} \end{array}} \right)\; = \;\dfrac{p}{t}{{\boldsymbol{Q}}_{\boldsymbol{0}}}\left( {\begin{array}{*{20}{c}} R \\ {{R_\delta }} \end{array}} \right)\; = \;\dfrac{p}{t}{\left( {\begin{array}{*{20}{c}} {{{\bar Q}_{11}}}&{{{\bar Q}_{12}}} \\ {{{\bar Q}_{12}}}&{{{\bar Q}_{22}}} \end{array}} \right)^{ - 1}}\left( {\begin{array}{*{20}{c}} R \\ {{R_\delta }} \end{array}} \right)$

(22)

此处主要关心 $\varepsilon _x^0$ ，即与气囊半径变化相关的中面横向应变：

$\varepsilon _x^0\; = \;\frac{{R - {R_{\rm{D}}}}}{{{R_{\rm{D}}}}}\; = \;\frac{p}{t}({Q_1}R\; + \;{Q_2}{R_\delta })$

(23)

其中：Q₁、Q₂为式(22)中定义的Q₀矩阵第一行第一列和第二列的元素，材料特性确定后，为α的函数，且有Q₁ > 0、Q₂ < 0；R为压强变化后的气囊半径；R_D为气囊内压强较小时，随压强变化不再发生明显径向形变的初始半径，经实验确定为内部相对压强为0.2 MPa时的气囊外半径。式(23)进一步转化为

$\frac{{R - {R_D}}}{{{R_D}}}\; = \;\frac{p}{t}\left({Q_1}R\; + \;{Q_2}\frac{{\delta {R_{{\rm{eff}}}}^2}}{{2R}}\right)$

(24)

根据式(24)可得有效面积随压强变化部分的表达式为

${A_{{\rm{eff1}}}}\; = \;{\rm{{\text{π}} }}{R_{{\rm{eff}}}}^2\; = \;\frac{{{Q_1}}}{{ - {Q_2}}}\frac{{2{\rm{{\text{π}} }}{R^2}}}{\delta }\; - \;\frac{1}{{ - {Q_2}}}\frac{{2{\rm{{\text{π}} }}R(R - {R_{\rm{D}}})t}}{{{R_{\rm{D}}}\delta p}}$

(25)

这里假设空簧气囊壁在经线方向长度不变，铺层角α不随空簧高度发生变化^[13]。引入新的参数λ、β，并将式(25)进行转化：

$\begin{split} &\lambda \; = \;\dfrac{{2{\rm{{\text{π}} }}{R^2}}}{\delta },\;\;\beta \; = \;\dfrac{{2{\rm{{\text{π}} }}R(R - {R_{\rm{D}}})t}}{{{R_{\rm{D}}}\delta }} \\ & {A_{{\rm{eff1}}}}\; = \;\dfrac{{{Q_1}}}{{ - {Q_2}}}\lambda \; - \;\dfrac{1}{{ - {Q_2}}}\beta \dfrac{1}{p} \end{split}$

(26)

空簧气囊的高度主要由气囊壁垂耳的翻卷改变，前述分析没有考虑到这一高度变化的影响。基于实验^[19]与几何图解^[3]的方法均指出有效面积随高度的近似线性变化规律。这里设定高度对有效面积的影响为线性，由根据实验确定的参量κ表征^[20]。这一部分对有效面积的影响写为

${A_{{\rm{eff2}}}}\; = \;\kappa (H - {H_0})$

(27)

其中，H₀为实验中空簧的初始工作高度。结合式(26)~(27)，进一步定义参数Δ：

$\varDelta \; = \; - \kappa {H_0} + \frac{{{Q_1}}}{{ - {Q_2}}}\lambda$

(28)

最终有效面积预测公式写为

${A_{{\rm{eff}}}}\; = \;\kappa H + \varDelta - \;\frac{1}{{ - {Q_2}}}\beta \lambda \frac{1}{p}$

(29)

根据相应国家标准GB/T 9101—2017^[24]，采用的帘线-橡胶力学参数^[25-26]如表1所示。根据式(5)~(8)计算出的复合材料表观力学参数如表2所示，参数Q₁、Q₂随铺层角α的变化规律如图7所示。

表 1 橡胶和帘线力学参数

Table 1. Mechanical parameters of rubber and cord

Parameter	Value
E_f/MPa	2700
ν_f	0.3
C_f/%	33.2
G_f/MPa	1038
E_m/MPa	2.0
ν_m	0.497
C_m/%	66.8
G_m/MPa	0.667
Notes: E_f, ν_f, C_f and G_f —Young’s modulus, Poisson’s ratio, volume fraction and shear modulus of cord material in composite; E_m, ν_m, C_m and G_m—Equivalent Young’s modulus, Poisson’s ratio, volume fraction and shear modulus of rubber material in composite.

下载: 导出CSV

| 显示表格

表 2 计算得到的帘线-橡胶复合材料表观力学值

Table 2. Apparent mechanical value of cord-rubber composite via computation

Parameter	Value
E₁/MPa	897.7
E₂/MPa	2.993
ν₂₁	0.432
ν₁₂	1.439×10⁻³
G₁₂/MPa	0.998
Q₁₁/MPa	898.3
Q₁₂/MPa	1.293
Q₂₂/MPa	2.995
Q₆₆/MPa	0.998
Notes: E₁, E₂—Elastic modulus of laminate in 1^st and 2^nd principle direction; ν₂₁, ν₁₂—Poisson’s ratios of laminate; G₁₂—Shear modulus of laminate in plane; Q₁₁, Q₁₂, Q₂₁, Q₂₂ and Q₆₆—Elements in matrix Q in Eq.(11).

下载: 导出CSV

| 显示表格

图 7 参数Q₁、Q₂随铺层角α的变化规律

Figure 7. Variation of Q₁ and Q₂ with ply angle α

下载: 全尺寸图片幻灯片

2. 实验对比与验证

在上述理论分析基础上，利用Mechanical Testing & Simulation公司生产的力学性能测试平台对某型号后空簧进行了实验，图8和图9分别为实验台架设备及实验原理。台架下端的液压作动器可产生给定位移，台架上端的力传感器可以测量产生的力并传输至计算机进行采集分析。

图 8 实验台架设备

Figure 8. Test rig

下载: 全尺寸图片幻灯片

图 9 实验原理

Figure 9. Experimental principle

F_AS—Force produced by air spring; z₁—Unsprung displacement

下载: 全尺寸图片幻灯片

在实验中，首先将空簧上端固定，调节下端位置到一定高度并充气至较低的初始压强。保持空簧高度不变，利用减压阀改变空簧内的气体压强，其值通过与管路相连的压强传感器读出。在不同压强下，当力传感器、压强传感器测得的数据基本保持稳定时，记录对应的力与压强。在同一高度下完成不同充气压强的实验测量后，再调整台架至另一实验高度，重复上述过程。最终根据测得的力与压强的比值作为各测量工况下的有效面积。为保证数据的准确性，同一高度的实验中先逐步升压至最大测量压强，再逐步减压至初始压强，每一个压强测量点在升降压过程中均进行数据采集并求平均以减小误差。实验选择了230 mm、250 mm、270 mm和290 mm的空簧高度进行实验，压强变化范围为0.3~0.8 MPa，这基本覆盖了该空簧的实际工况。

根据上节所述的理论分析方法，结合空簧的基本参数，对该空簧的有效面积计算公式进行了拟合，部分参数取值如表3所示，实验数据与理论预测数据如图10所示，理论与实验的误差情况如表4所示。由图10可知，随高度减小、压强增大，有效面积有变大的趋势，此时经线方向上卷耳部分的长度增加，导致空簧气囊整体有变得矮粗的趋势。由表4可以看出，在实验对应的测量范围中，理论预测的有效面积误差都小于1%，这表明上述空簧有效面积的理论分析方法能很好地与实验数据相符合，对进行空簧有效面积的预测具有一定的指导意义。

表 3 用于空气弹簧有效面积计算的参数取值

Table 3. Parameter values in effective area computation for air spring

Parameter	Value
α/(°)	50
R/10⁻²m	7.50
R_D/10⁻²m	7.32
t/10⁻³m	2.00
δ	0.8
κ/10⁻²m	−1.069
Δ/m²	0.01383
Notes: R—Bellow radius after pressure variation; R_D—Initial bellow radius without obvious radial deformation; δ, κ and Δ—Experimental parameters defined in Eq.(27) and Eq.(29).

下载: 导出CSV

| 显示表格

图 10 空气弹簧实验数据与理论预测对比

Figure 10. Comparison of experimental data and theoretical prediction of air spring

下载: 全尺寸图片幻灯片

表 4 空气弹簧理论预测与实验数据的误差

Table 4. Errors between experimental data and theoretical prediction of air spring

Pressure/ 10⁵ Pa	e₂₃/%	e₂₅/%	e₂₇/%	e₂₉/%
3	0.20	0.11	<0.01	0.39
4	0.04	0.56	0.11	0.03
5	0.31	0.51	0.10	0.16
6	0.18	0.35	0.14	0.29
7	0.22	0.25	<0.01	0.38
8	0.23	0.26	<0.01	0.46
Notes: e₂₃, e₂₅, e₂₇ and e₂₉—Errors between experimental data and theoretical prediction when height of air spring are 230 mm, 250 mm, 270 mm and 290 mm, respectively.

下载: 导出CSV

| 显示表格

3. 结论

(1) 提出一种车用膜式空簧的有效面积理论分析与预测方法，给出了与空簧高度和内部压强相关的空簧有效面积理论预测表达式。

(2) 利用实验台架对某型号空簧进行有效面积测量实验，实验与理论预测的结果的对比验证了这种理论分析方法的合理性。

图 1 橡胶/钢帘线复合材料试样制备流程

Figure 1. Specimen preparation process of rubber/steel cord composites

Φ—Diameter

下载: 全尺寸图片幻灯片

图 2 橡胶/钢帘线复合材传热分析的技术路线

Figure 2. Technical route for thermal analysis of rubber/steel cord composites

CFD—Computational fluid dynamics

下载: 全尺寸图片幻灯片

图 3 橡胶/钢帘线复合材料传热实验平台示意图

Figure 3. Schematic diagram of the experimental platform for heat transfer of rubber/steel cord composites

下载: 全尺寸图片幻灯片

图 4 橡胶/钢帘线复合材料热扩散测试结果：(a)测温点；(b)温升历程曲线；(c)顶面和侧面红外热成像云图

Figure 4. Thermal diffusion test results of rubber/steel cord composites: (a) Temperature measurement points; (b) Temperature rise history curves;(c) Infrared thermography cloud maps of top and side surfaces

下载: 全尺寸图片幻灯片

图 5 (a)橡胶/钢帘线复合材料红外热成像测试结果校正；(b)校正结果与热电偶测试结果对比

Figure 5. (a) Calibration of IR thermography test results of rubber/steel cord composites; (b) Comparison of calibration results with thermocouple test results

下载: 全尺寸图片幻灯片

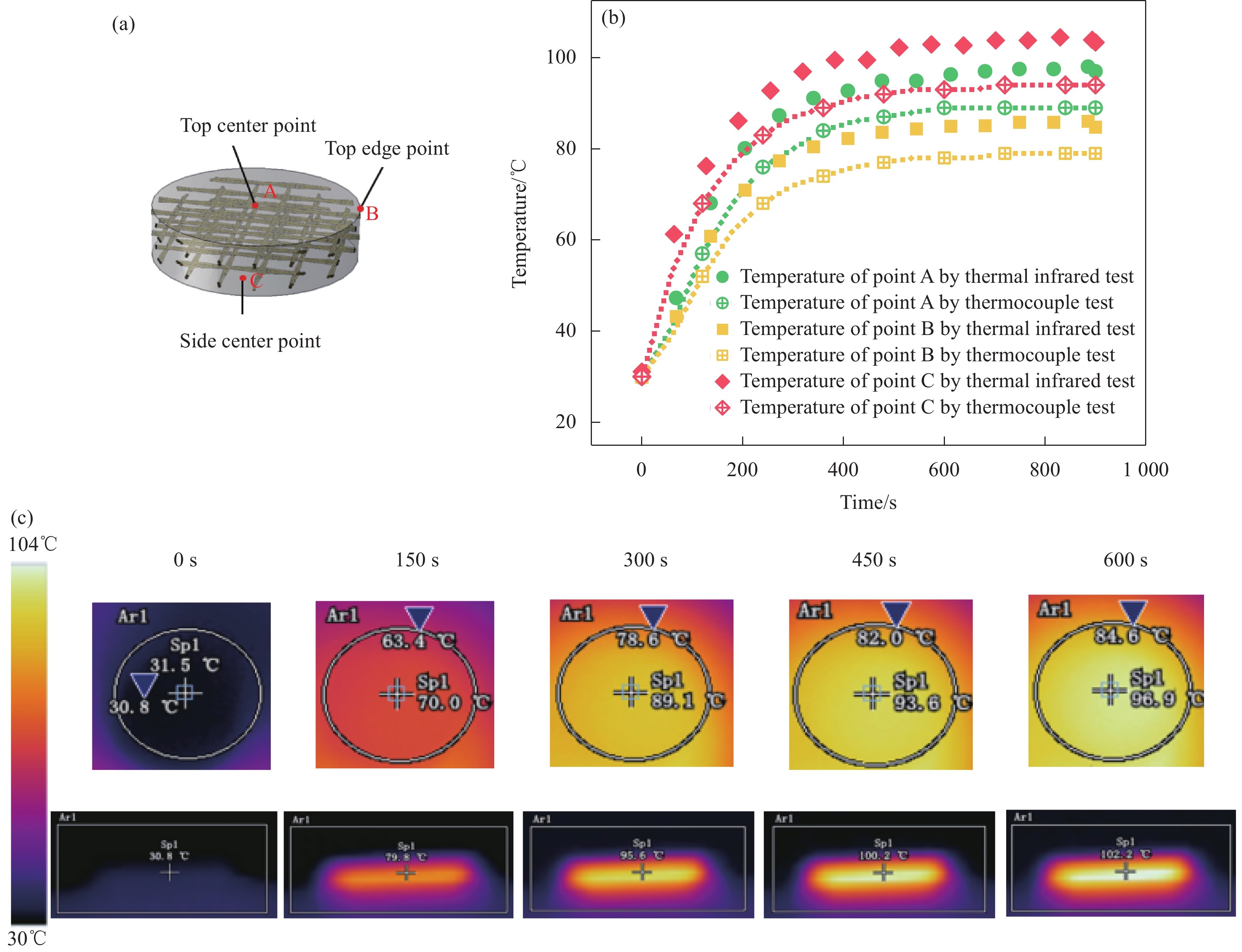
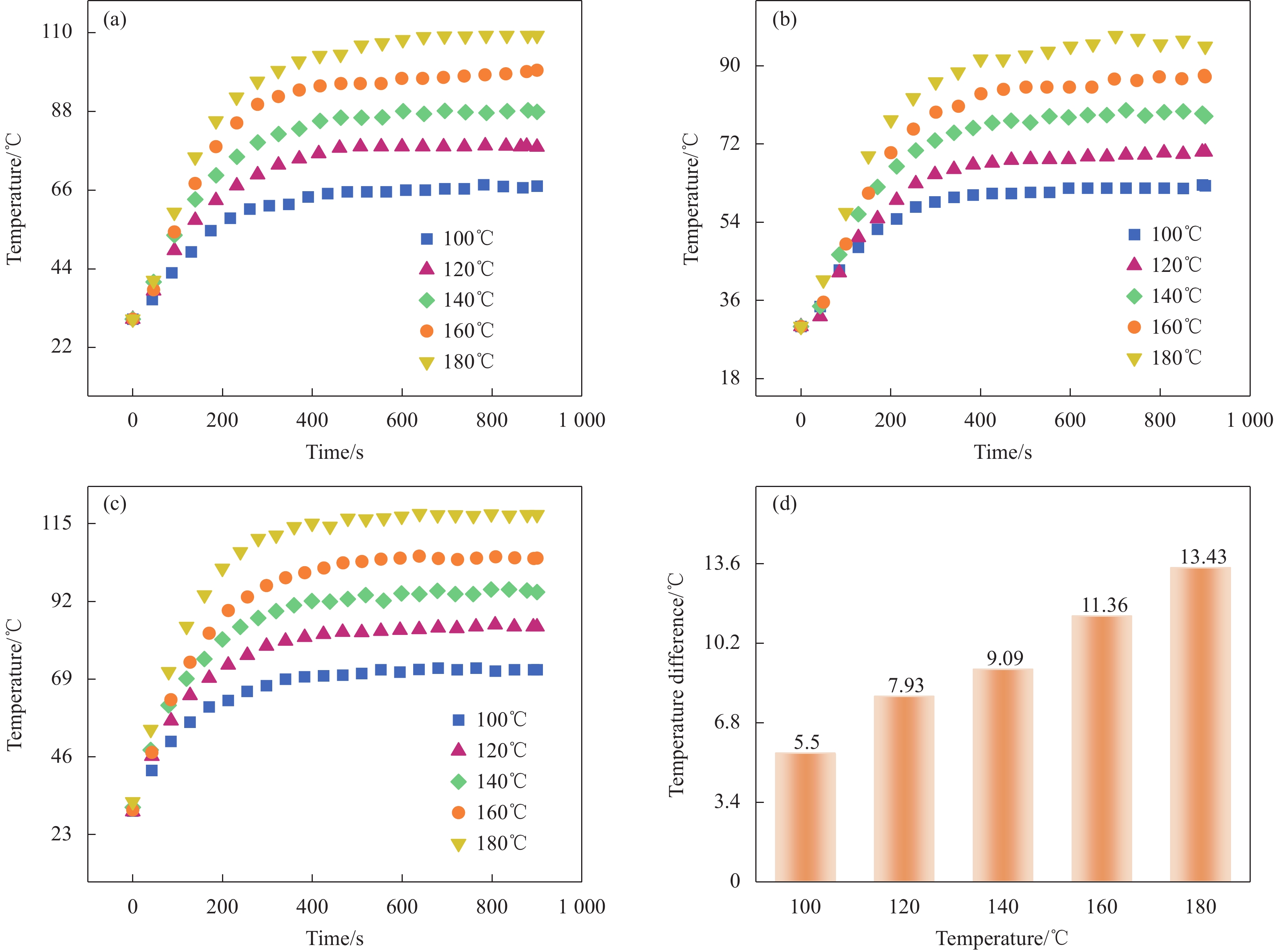
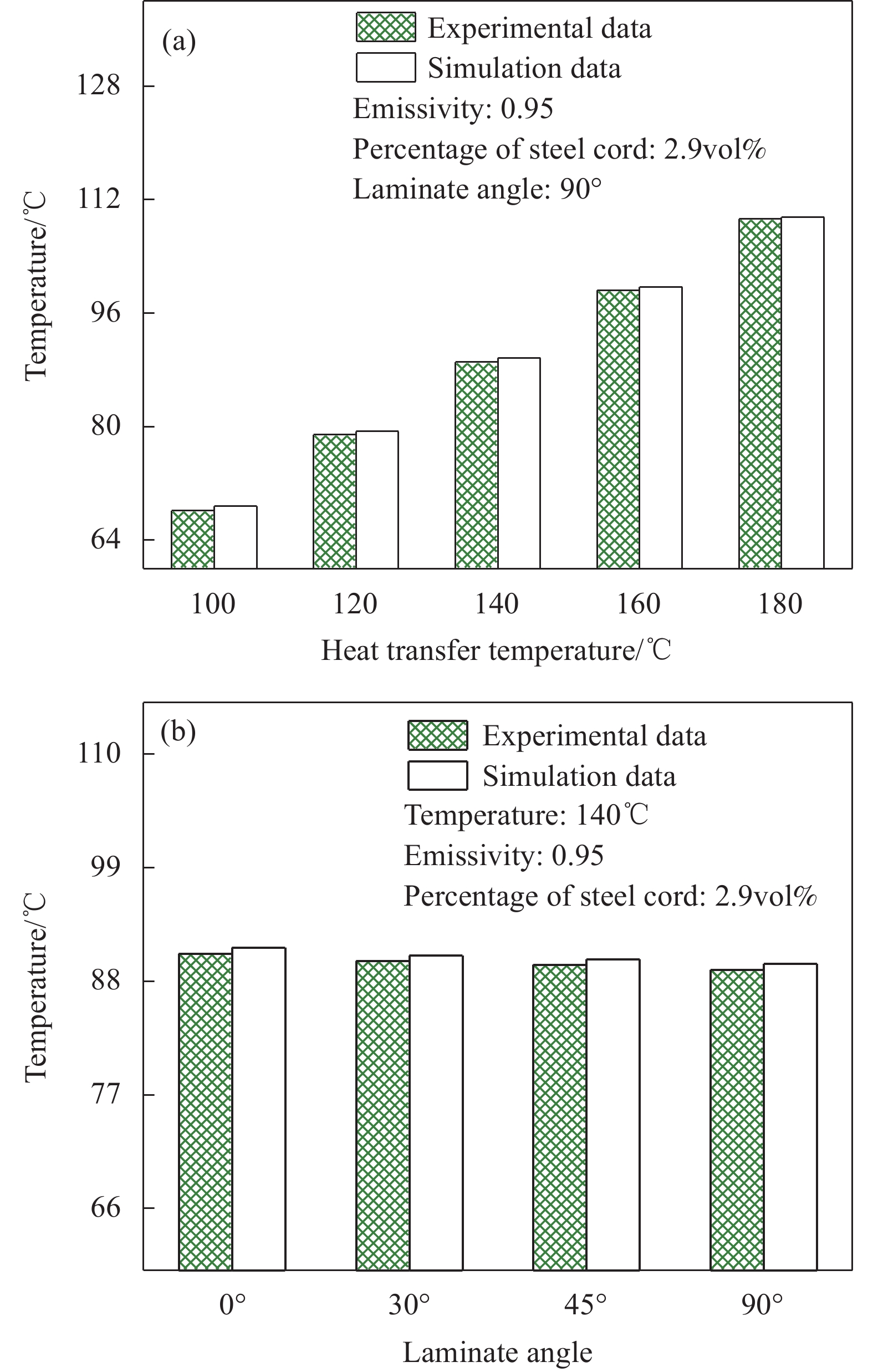
图 6 热台温度对橡胶/钢帘线复合材料温升的影响：(a)顶面中心点温度历程图；(b)顶面边缘点温度历程图；(c)侧面中心点温度历程图；(d)顶部中心点与顶面边缘点温度差值图

Figure 6. Effect of hot bench temperature on temperature rise of rubber/steel cord composites: (a) Plot of temperature history at the top center point; (b) Plot of temperature history at the top edge point; (c) Plot of temperature history at the side center point; (d) Plot of temperature difference between the top center point and the top edge point

下载: 全尺寸图片幻灯片

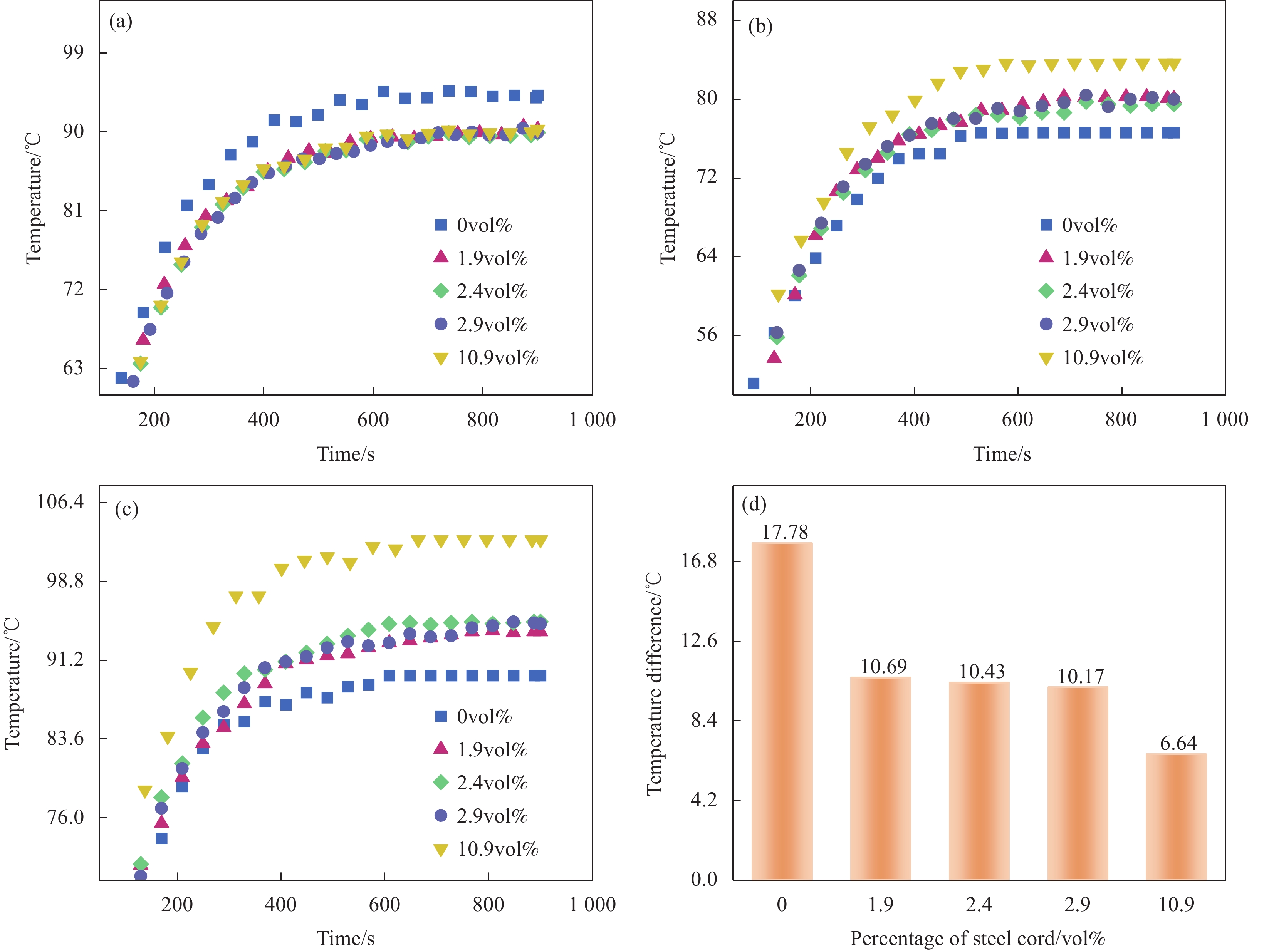
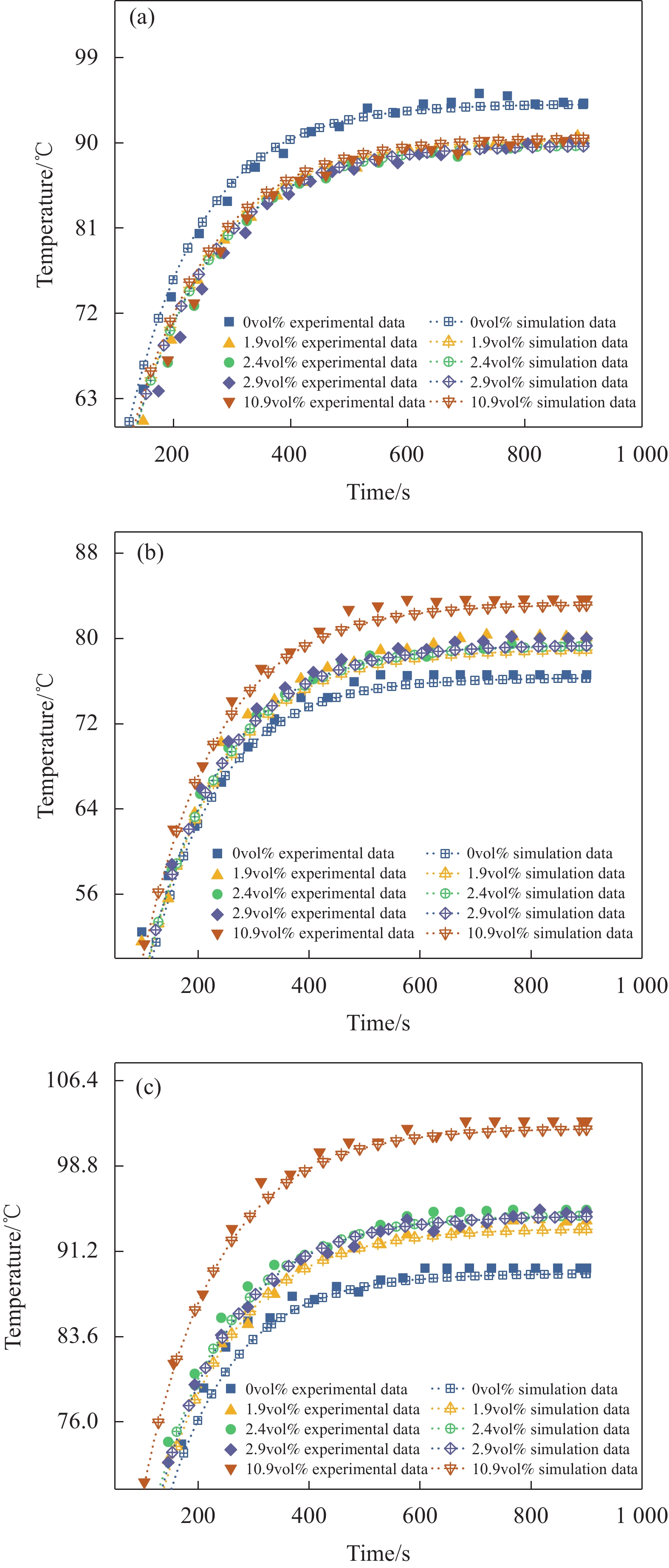
图 7 140℃条件下不同钢帘线占比橡胶/钢帘线复合材料温升影响研究：(a)顶面中心点温度历程图；(b)顶面边缘点温度历程图；(c)侧面中心点温度历程图；(d)顶面中心点与顶面边缘点温度差值图

Figure 7. Study on the effect of temperature rise of rubber/steel cord composites with different steel cord ratios at 140℃: (a) Temperature history of top center point; (b) Temperature history of top edge point; (c) Temperature history of side center point; (d) Temperature difference between top center point and top edge point

下载: 全尺寸图片幻灯片

图 8 不同接触热阻下的橡胶/钢帘线复合材料热导率

Figure 8. Thermal conductivity of rubber/steel cord composites with different contact thermal resistance

下载: 全尺寸图片幻灯片

图 9 橡胶/钢帘线复合材料对流换热CFD模型示意图

Figure 9. Schematic diagram of CFD model for convective heat transfer of rubber/steel cord composites

下载: 全尺寸图片幻灯片

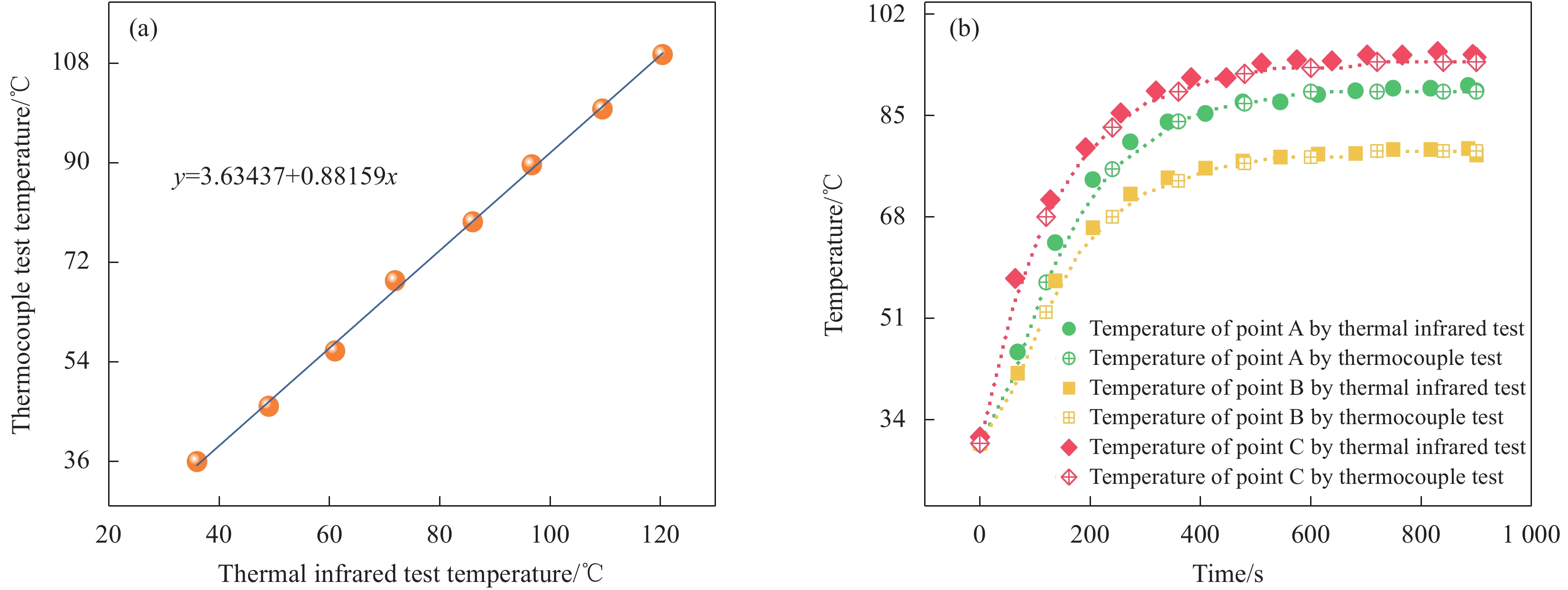
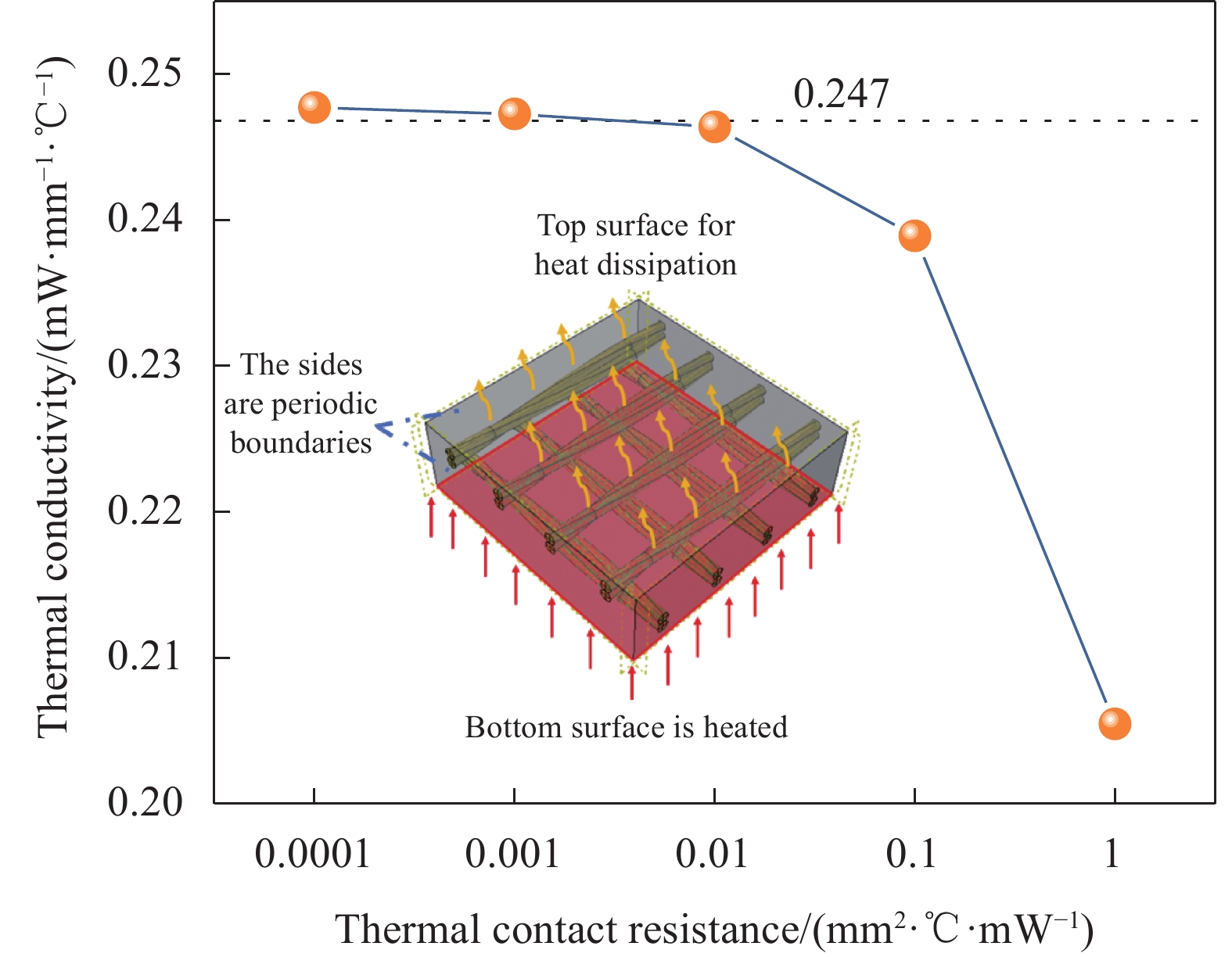
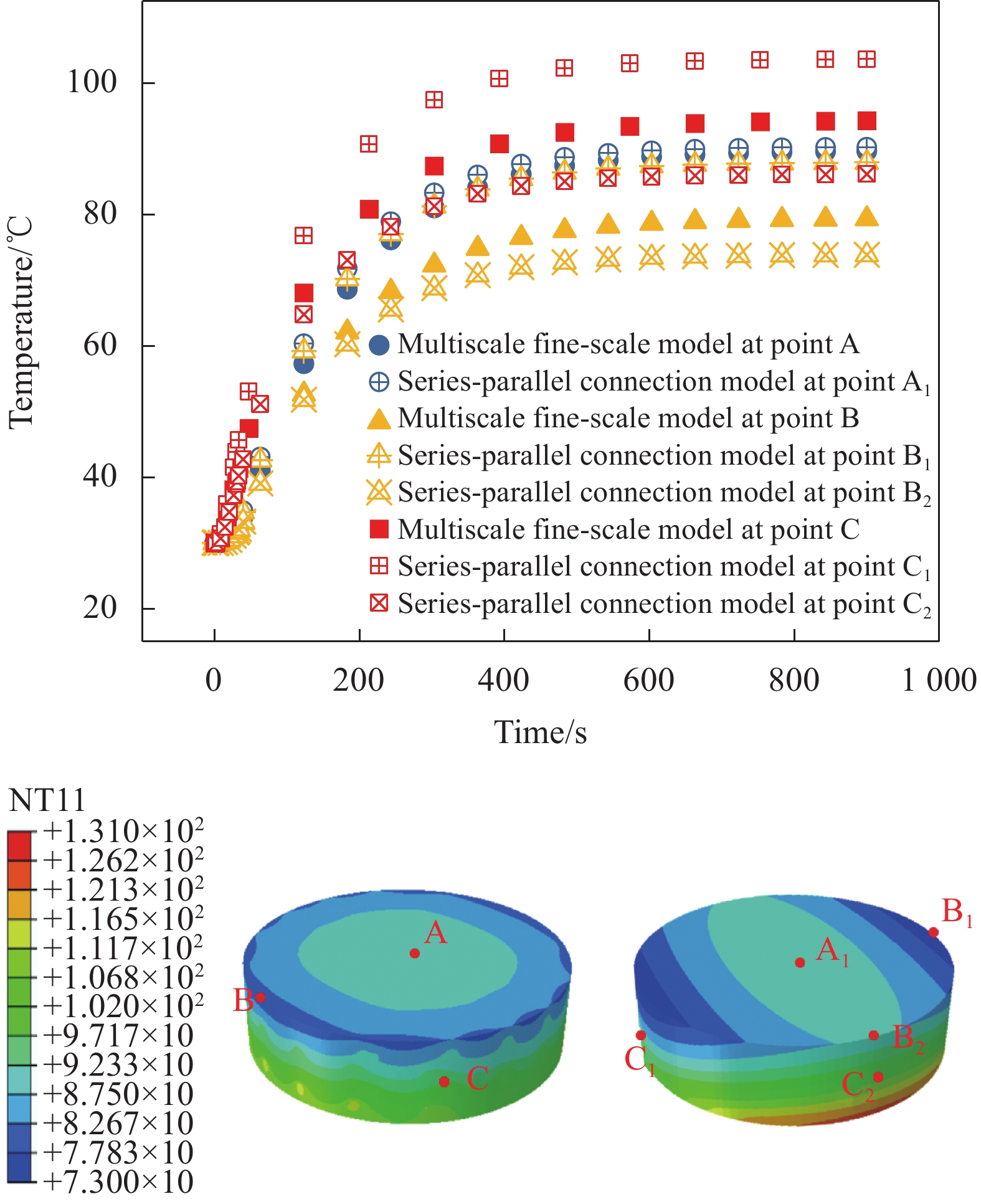
图 10 (a)橡胶/钢帘线复合材料流体动力学(CFD)传热模型与等效热边界传热模型温度历程对比；(b)橡胶/钢帘线复合材料实验与仿真结果温度历程对比

Figure 10. (a) Comparison of CFD heat transfer model and equivalent thermal boundary heat transfer model temperature history for rubber/steel cord composites; (b) Comparison of temperature history between experimental and simulation results for rubber/steel cord composites

下载: 全尺寸图片幻灯片

图 11 橡胶/钢帘线复合材料不同发射率温升历程仿真结果与实验对比：(a)顶面中心点；(b)顶面边缘点；(c)侧面中心点；(d)拟合曲线

Figure 11. Simulation results and experimental comparison of temperature rise history of rubber/steel cord composites with different emissivity: (a) Top center point; (b) Top edge point; (c) Side center point; (d) Fitted curve

下载: 全尺寸图片幻灯片

图 12 0.95发射率时不同钢帘线占比的复合材料温升历程仿真与实验对比：(a)顶面中心点；(b)顶面边缘点；(c)侧面中心

Figure 12. Simulation and experimental comparison of temperature rise histories of composites with different steel cord percentages at 0.95 emissivity: (a) Top center point; (b) Top edge point; (c) Side center

下载: 全尺寸图片幻灯片

图 13 橡胶/钢帘线复合材料顶面中心点稳态温度值：(a)不同加热温度；(b)不同钢帘线排布

Figure 13. Steady state temperature values at the center point of the top surface of rubber/steel cord composites: (a) Different heating temperatures; (b) Different steel cord arrangements

下载: 全尺寸图片幻灯片

图 14 橡胶/钢帘线复合材料多尺度模型与串并联模型对比

Figure 14. Comparison of multiscale model and series-parallel model of rubber/steel cord composites

NT11—Temperature (℃)

下载: 全尺寸图片幻灯片

图 15 橡胶/钢帘线复合材料纵向切面温度云图和热流密度云图

Figure 15. Longitudinal section temperature contour plot and heat flow density contour plot of rubber/steel cord composites

HFL—Heat flow density (mW/mm²)

下载: 全尺寸图片幻灯片

图 16 橡胶/钢帘线复合材料横向切面温度云图和热流密度云图

Figure 16. Temperature contour plot and heat flow density contour plot of rubber/steel cord composites in transverse sections

下载: 全尺寸图片幻灯片

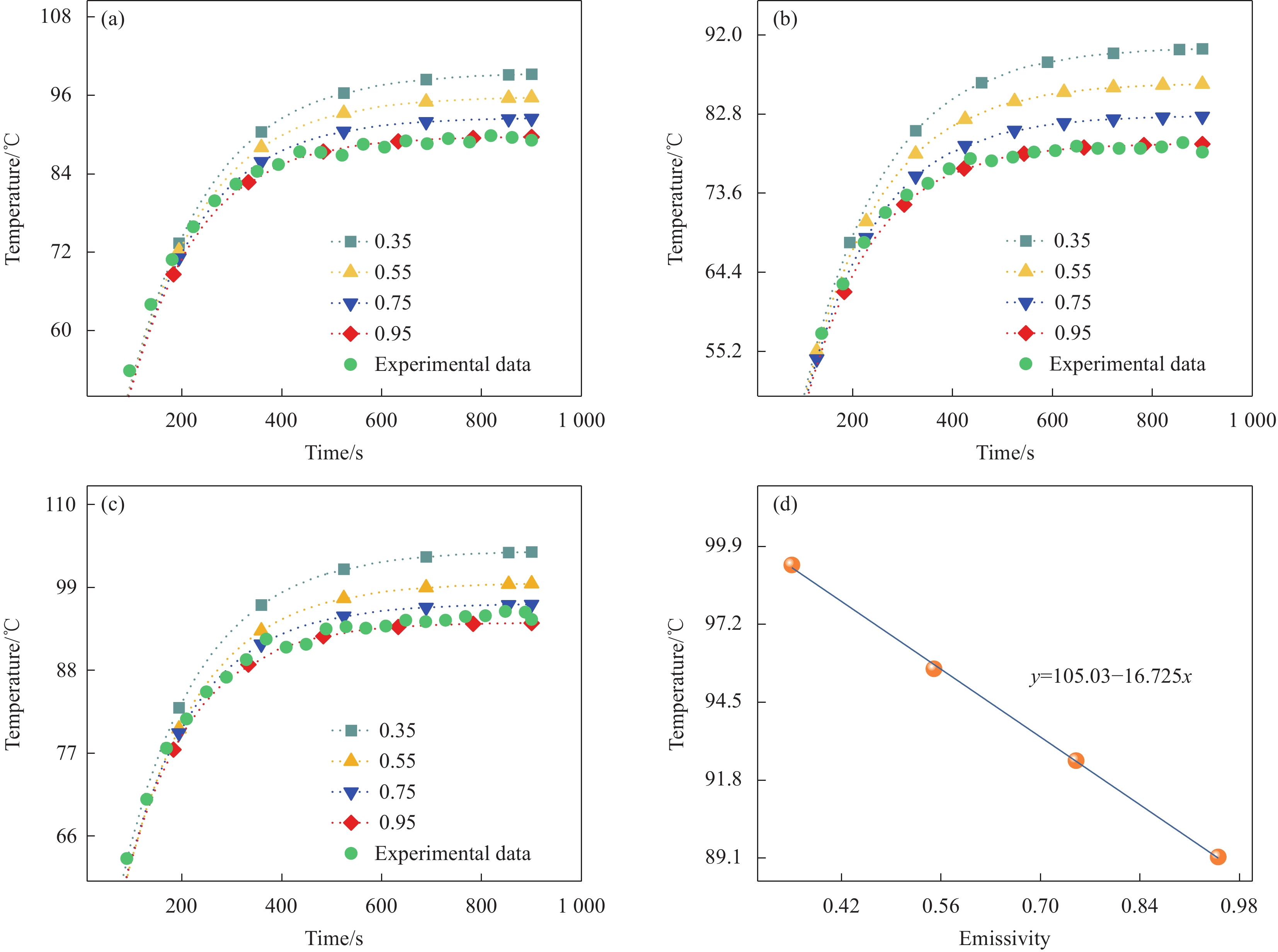
图 17 不同因素对橡胶/钢帘线复合材料辐射散热的影响：(a)发射率的影响；(b)传热时间的影响；(c)传热温度的影响；(d)钢帘线占比的影响

Figure 17. Effect of different factors on radiative heat dissipation of rubber/steel cord composites: (a) Effect of emissivity; (b) Effect of heat transfer time; (c) Effect of heat transfer temperature; (d) Effect of steel cord percentage

下载: 全尺寸图片幻灯片

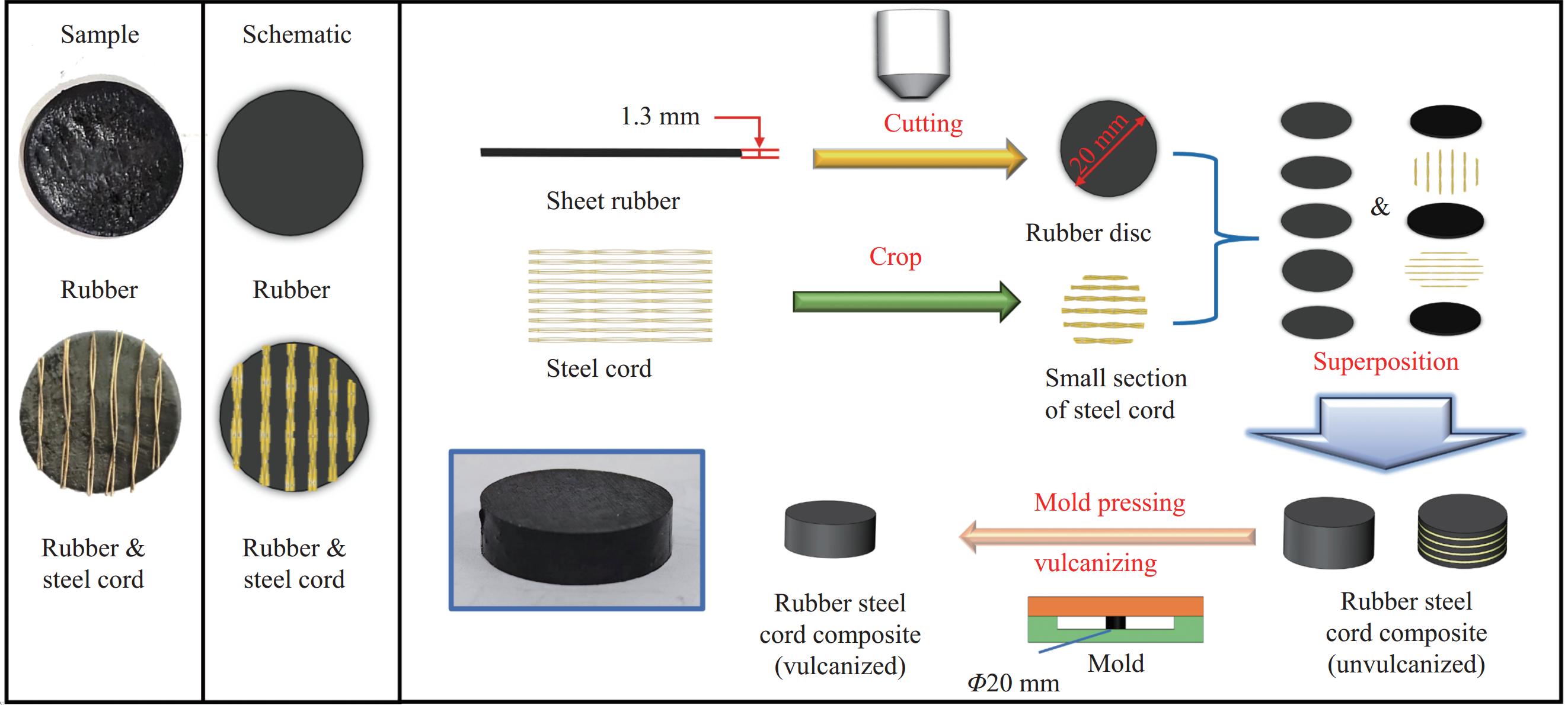
表 1 橡胶配方

Table 1 Rubber formulations

Raw material	Parts per hundreds of rubber
EPDM4045	100
NR	30
ZnO	10
C₁₈H₃₆O₂	1
N220	40
Paraffin oil	14
Coumarone-indene resin	5
DCP	4
C₆H₁₂N₂S₄	0.5
S	0.5
Tackifier	5
Antioxidant RD	1
Notes: EPDM4045—Ethylene propylene diene monomer rubber; NR—Nature rubber; N220—Carbon black; DCP—Dicumyl peroxide crosslinking; RD—Poly 1, 2-dihydro-2, 2, 4-trimethyl-quinoline.

下载: 导出CSV

表 2 橡胶/钢帘线复合材料结构参数

Table 2 Structural parameters of rubber/steel cord composites

Steel cord spacing/mm	Percentage of steel cord/vol%	Laminate angle/(°)
Pure rubber	–	–
5	1.9	0/30/45/90
4	2.4
3.6	2.9
1	10.9

下载: 导出CSV

表 3 橡胶/钢帘线复合材料传热测试实验方案

Table 3 Experimental program for heat transfer testing of rubber/steel cord composites

Steel cord spacing/mm	Percentage of steel cord/vol%	Laminate angle/(°)	Heating pad temperature/℃	Environmental temperature/℃
3.6	2.9	90	100	30
3.6	2.9	90	120	30
3.6	2.9	90	140	30
3.6	2.9	90	160	30
3.6	2.9	90	180	30
Pure rubber	0	–	140	30
4	2.4	90	140	30
5	1.9	90	140	30
1	10.9	90	140	30
3.6	2.9	0	140	30
3.6	2.9	30	140	30
3.6	2.9	45	140	30

下载: 导出CSV

表 4 多尺度模型材料热物性参数

Table 4 Thermo-physical property parameters of materials for multi-scale modeling

Material type	Thermal conductivity/ (mW·(mm·℃)⁻¹)	Density/ (kg·m⁻³)	Specific heat/ (J·(kg·℃)⁻¹)
Rubber	0.21	1200	1503
Steel cord	70	7810	540

下载: 导出CSV

参考文献(25)

[1]	贺婷, 杨长浩, 许宗超, 等. 不同工况下纤维/天然橡胶复合材料界面疲劳失效机制[J]. 弹性体, 2022, 32(5): 13-19. HE Ting, YANG Changhao, XU Zongchao, et al. Fatigue failure mechanism of fiber/natural rubber composite interface under different working conditions[J]. China Elastomerics, 2022, 32(5): 13-19(in Chinese).
[2]	张嘉琦. 高温往复工况下橡胶密封件的密封性能研究[D]. 洛阳: 河南科技大学, 2022. ZHANG Jiaqi. Research on the sealing performance of rubber seals under high temperature reciprocating conditions[D]. Luoyang: Henan University of Science and Technology, 2022(in Chinese).
[3]	LI Z X, KONG Y R, CHEN X F, et al. High-temperature thermo-oxidative aging of vulcanized natural rubber nanocomposites: Evolution of microstructure and mechanical properties[J]. Chinese Journal of Polymer Science, 2023, 41(8): 1287-1297. DOI: 10.1007/s10118-023-2948-9
[4]	ADAM Q, BEHNKE R, KALISKE M. A thermo-mechanical finite element material model for the rubber forming and vulcanization process: From unvulcanized to vulcanized rubber[J]. International Journal of Solids and Structures, 2020, 185-186: 365-379. DOI: 10.1016/j.ijsolstr.2019.08.037
[5]	SALVI W D, DE SANTO C E, MATSUMOTO A, et al. Characterization of thermal-oxidative aging mechanism of commercial tires[J]. Engineering Failure Analysis, 2023, 154: 107631. DOI: 10.1016/j.engfailanal.2023.107631
[6]	NYAABA W, FRIMPONG S, ANANI A. Fatigue damage investigation of ultra-large tire components[J]. International Journal of Fatigue, 2019, 119: 247-260. DOI: 10.1016/j.ijfatigue.2018.07.009
[7]	姜碧羽, 齐俊伟, 刘小林. 预浸料先进拉挤成型的固化传热过程数值模拟[J]. 复合材料学报, 2020, 37(6): 1496-1504. JIANG Biyu, QI Junwei, LIU Xiaolin. Numerical simulation of curing heat transfer process in advanced pultrusion molding of prepreg[J]. Acta Materiae Compositae Sinicia, 2020, 37(6): 1496-1504(in Chinese).
[8]	NAZARI N, ALLAHBAKHSH A, BAHRAMIAN A R. Analytical effective thermal conductivity model for colloidal porous composites and nanocomposites based on novolac/graphene oxide aerogels[J]. International Journal of Energy Research, 2022, 46(12): 16608-16628. DOI: 10.1002/er.8323
[9]	HUI X, XU Y, ZHANG W, et al. Multiscale collaborative optimization for the thermochemical and thermomechanical cure process during composite manufacture[J]. Composites Science and Technology, 2022, 224: 109455. DOI: 10.1016/j.compscitech.2022.109455
[10]	SU B, WU J, CUI Z, et al. Modeling of truck tire curing process by an experimental and numerical method[J]. Iranian Polymer Journal, 2015, 24(7): 583-593. DOI: 10.1007/s13726-015-0349-9
[11]	LI W, ZHANG X, SHANG Y, et al. Investigation of dynamic heat generation and transfer behavior and energy dissipation for nonlinear synchronous belt transmission[J]. Applied Thermal Engineering, 2018, 144: 457-468. DOI: 10.1016/j.applthermaleng.2018.08.080
[12]	ZHOU K, ENOS R, ZHANG D, et al. Uncertainty analysis of curing-induced dimensional variability of composite structures utilizing physics-guided Gaussian process meta-modeling[J]. Composite Structures, 2022, 280: 114816. DOI: 10.1016/j.compstruct.2021.114816
[13]	ZHOU L, SUN X, CHEN M, et al. Multiscale modeling and theoretical prediction for the thermal conductivity of porous plain-woven carbonized silica/phenolic composites[J]. Composite Structures, 2019, 215: 278-288. DOI: 10.1016/j.compstruct.2019.02.053
[14]	杨方沁. 金属/聚合物复合结构对材料导热性能的影响研究[D]. 杭州: 浙江工业大学, 2013. YANG Fangqin. Effects of metal/polymer composite structure on thermal conductivity of materials[D]. Hangzhou: Zhejiang University of Technology, 2013(in Chinese).
[15]	FANG Y, LI L Y, MAWULÉ DASSEKPO J B, et al. Heat transfer modelling of carbon nanotube reinforced composites[J]. Composites Part B: Engineering, 2021, 225: 109280. DOI: 10.1016/j.compositesb.2021.109280
[16]	ZHOU L, YUAN T B, YANG X S, et al. Micro-scale prediction of effective thermal conductivity of CNT/Al composites by finite element method[J]. International Journal of Thermal Sciences, 2022, 171: 107206. DOI: 10.1016/j.ijthermalsci.2021.107206
[17]	XU S, LIU J, ZENG Q. Towards better characterizing thermal conductivity of cement-based materials: The effects of interfacial thermal resistance and inclusion size[J]. Materials & Design, 2018, 157: 105-118.
[18]	贺元骅, 赵逸明, 张俐恒, 等. 辐射对流散热下大尺寸三元锂电池热模型[J]. 中国安全科学学报, 2023, 33(6): 49-55. HE Yuanhua, ZHAO Yiming, ZHANG Liheng, et al. Thermal modeling of large-size lithium ternary batteries under radiative convection heat dissipation[J]. China Safety Science Journal, 2023, 33(6): 49-55(in Chinese).
[19]	YANG Z, WANG Z, YANG Z, et al. Multiscale analysis and computation for coupled conduction, convection and radiation heat transfer problem in porous materials[J]. Applied Mathematics and Computation, 2018, 326: 56-74. DOI: 10.1016/j.amc.2017.12.039
[20]	YANG Z, CUI J, SUN Y, et al. Multiscale computation for transient heat conduction problem with radiation boundary condition in porous materials[J]. Finite Elements in Analysis and Design, 2015, 102-103: 7-18. DOI: 10.1016/j.finel.2015.04.005
[21]	YANG Z, CUI J, MA Q. The second-order two-scale computation for integrated heat transfer problem with conduction, convection and radiation in periodic porous materials[J]. Discrete and Continuous Dynamical Systems: B, 2014, 19(3): 827-848. DOI: 10.3934/dcdsb.2014.19.827
[22]	童自翔, 李明佳, 李冬. 导热-辐射耦合传热的多尺度分析和数值模型[J]. 航空学报, 2021, 42(9): 205-214. TONG Zixiang, LI Mingjia, LI Dong. Multi-scale analysis and numerical modeling of coupled thermal-radiative heat transfer[J]. Acta Aeronautica et Astronautica Sinica, 2021, 42(9): 205-214(in Chinese).
[23]	陈则韶, 钱军, 叶一火. 复合材料等效导热系数的理论推算[J]. 中国科学技术大学学报, 1992(4): 416-424. CHEN Zeshao, QIAN Jun, YE Yihuo. Theoretical derivation of equivalent thermal conductivity of composite materials[J]. Journal of China University of Science and Technology, 1992(4): 416-424(in Chinese).
[24]	石鹏. 活络轮胎模具传热过程仿真建模与分析[D]. 武汉: 华中科技大学, 2021. SHI Peng. Simulation modeling and analysis of heat transfer process in live tire mold[D]. Wuhan: Huazhong University of Science and Technology, 2021(in Chinese).
[25]	张建. 轮胎硫化特性及影响规律研究[D]. 镇江: 江苏大学, 2014. ZHANG Jian. Research on tire curing characteristics and influence law[D]. Zhenjiang: Jiangsu University, 2014(in Chinese).

施引文献(14)

期刊类型引用(6)

1.	付硕，许一虎，孙治博. 汽车空气悬架系统及精准控制技术发展综述. 液压与气动. 2023(04): 107-114 . 百度学术
2.	陈俊杰，谭月玲，康盛，邱光琦. 膜式空气弹簧帘子线铺设角-承载力映射模型研究. 振动与冲击. 2023(21): 291-297 . 百度学术
3.	中国汽车工程学术研究综述·2023. 中国公路学报. 2023(11): 1-192 . 百度学术
4.	王相平，李星，王剑锋，吴少培，丁旺才，李国芳. 中低速磁浮车辆空气弹簧动力学建模及其应用研究. 振动工程学报. 2023(06): 1527-1538 . 百度学术
5.	刘琼，杨纪远，张磊，商涛，谢济兴. 帘线参数对单囊空气弹簧垂向刚度的影响研究. 三明学院学报. 2023(06): 117-124 . 百度学术
6.	孙文，李晨阳，王军年，钱灏喆，张文通. 越野车复合型悬架平顺性的研究. 汽车工程. 2022(01): 105-114+122 . 百度学术

其他类型引用(8)

资源附件(0)

长摘要

目的
橡胶/钢帘线复合材料的传热与温度分析对橡胶制品的硫化成型、热氧老化、热疲劳寿命研究具有重要意义。本文基于多尺度模型对不同钢帘线占比、排列角度和温升工况下的橡胶/钢帘线复合材料传热和散热机制进行研究。

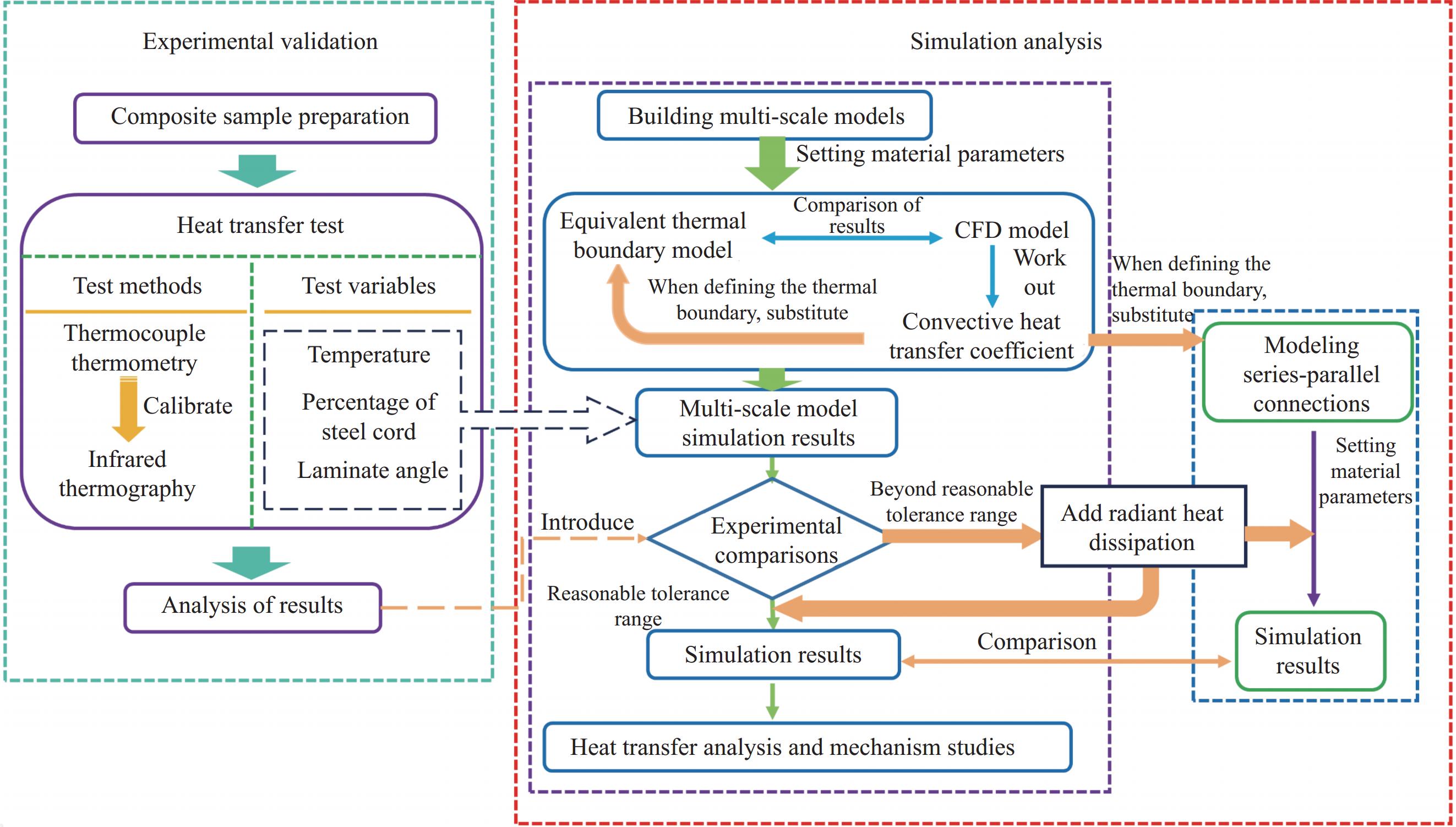
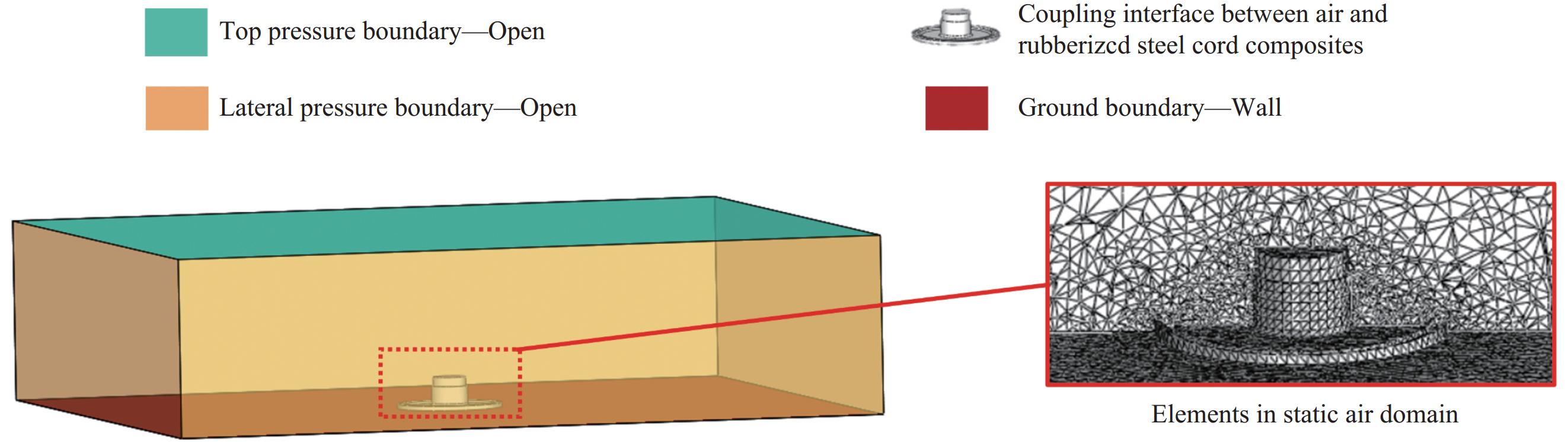
方法
采用多尺度有限元模型对橡胶/钢帘线复合材料传热和散热机制进行研究，并通过实验验证。首先，对橡胶/钢帘线样品进行制备，使用红外热成像仪对不同结构的橡胶/钢帘线复合材料试样温升过程中的温度分布进行监测，并通过热电偶对结果进行校正，研究升温规律。然后，建立多尺度复合材料流体动力学模型(Computational Fluid Dynamics ,CFD)，计算对流换热系数。进一步通过研究不同辐射散热发射率对橡胶钢帘线复合材料温度分布的影响，确定辐射散热发射率。将对流换热系数与辐射散热发射率定义到橡胶/钢帘线复合材料传热仿真模型中，对比多尺度模型和串并联模型的仿真结果，分析误差。模拟橡胶/钢帘线复合材料不同钢帘线占比、排列角度和升温工况下的传热散热行为，分析传热散热机制。

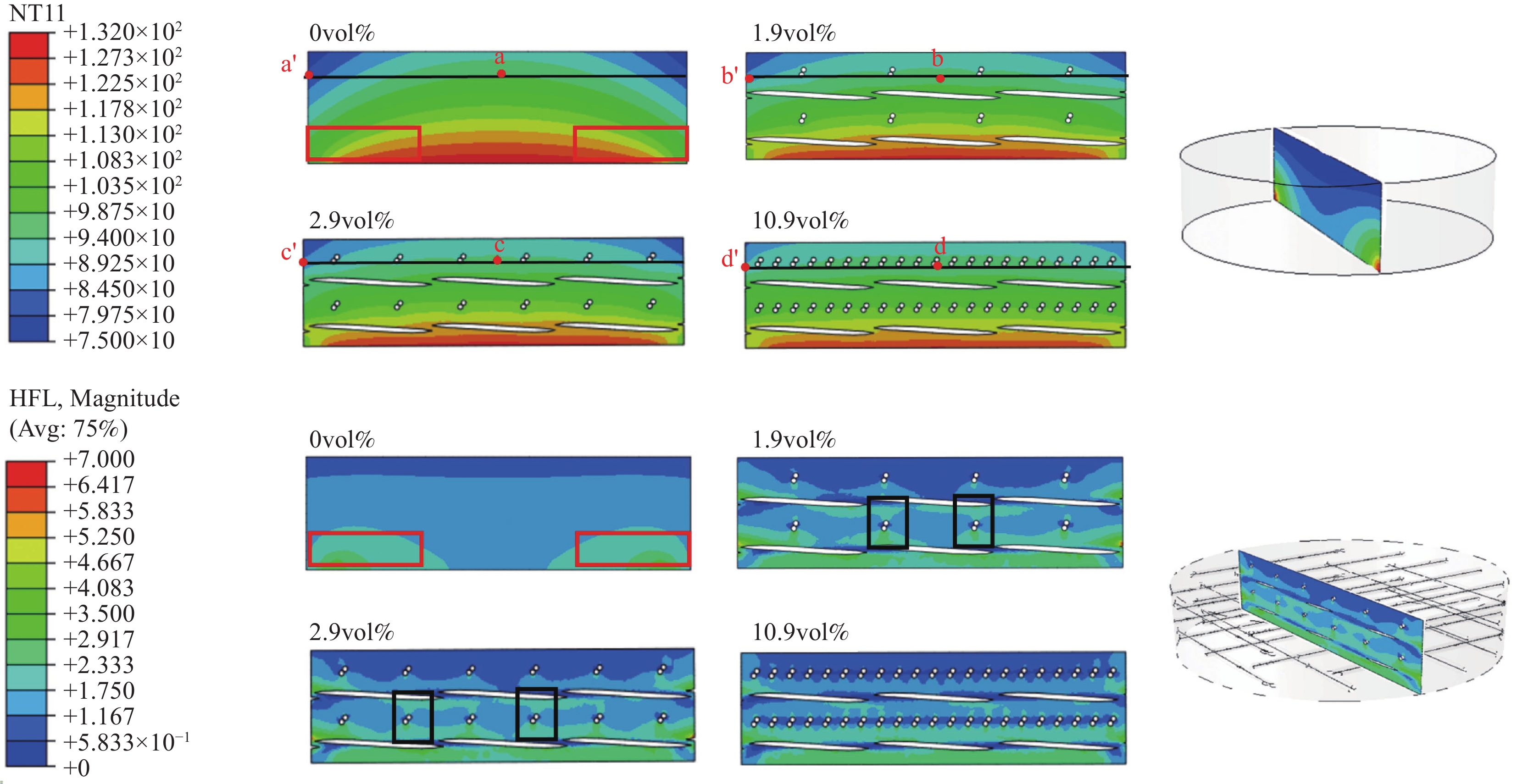
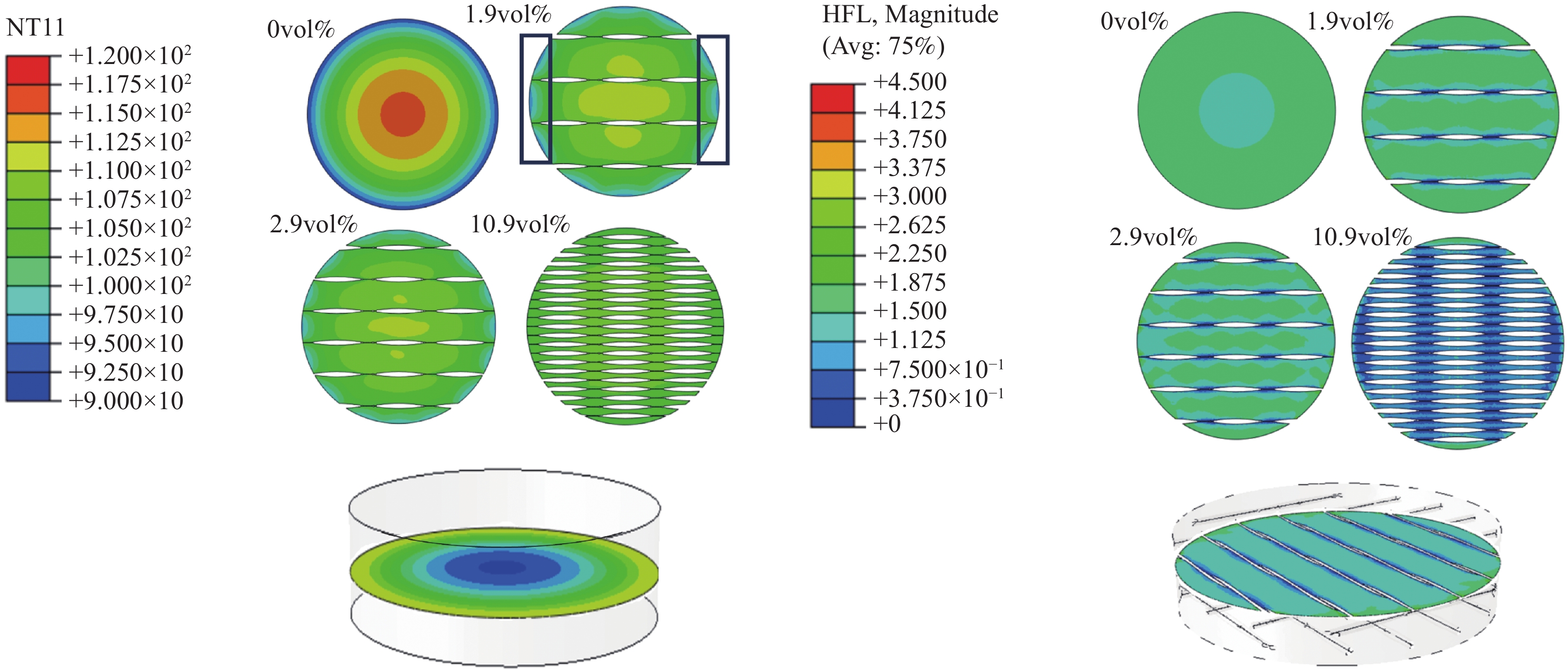
结果
本文对橡胶/钢帘线复合材料的多尺度传热模拟方法，传热机制及辐射散热影响规律展开研究可以得到以下结果①通过仿真计算得到橡胶/钢帘线复合材料的对流换热系数为0.008mW/(mm·℃)，辐射散热发射率为0.95。分析结果表明采用多尺度传热模型计算得到的温度分布与实际结果相比误差为2.5%，远小于串并联传热模型10.1%的计算误差。②通过对橡胶/钢帘线复合材料仿真结果的温度云图和热流密度云图的分析，结果表明，由于钢帘线的高导热特性，钢帘线表现出各向异性的传热行为，钢帘线周围呈现出明显的热流聚集效应，提高了橡胶/钢帘线复合材料层间的热量扩散，当钢帘线占比较低时(1.9%~2.9%)，钢帘线排布不够紧密，使得层间的热流密度分布不均匀。当钢帘线占比较高时(10.9%)，钢帘线排布较紧密，钢帘线之间的热流聚集效应会相互叠加，层间的热流密度分布均匀，温差减小。③通过研究不同因素对橡胶/钢帘线复合材料辐射散热的影响，结果表明，不同的辐射散热发射率对辐射散热在总散热量中的占比影响较大，在稳态时随着辐射散热发射率从0.35升高到0.95，辐射散热占比从27.9%增加到50.3%。在相同加热条件下(140 ℃)，随着加热时间增加，辐射散热占比也随之增加，从30 s的45.4%，提高到900 s的50.3%。当加热温度140 ℃以下时，自然对流散热量较大，随着热台加热温度从100 ℃增加到180 ℃，辐射散热占比从47.7 %提高到52.6%，温度越高，辐射散热占比越大，因此只定义自然对流换热系数，不能达到精确计算橡胶/钢帘线复合材料的温升曲线的目的。纯橡胶试样辐射散热占比较低，约为49.6%，当加入钢帘线后，辐射散热略有提升，且随着钢帘线含量的增加，辐射散热占比也随之增加。在钢帘线含量增加到10.9%时，辐射散热占比提高到50.8%。

结论
橡胶/钢帘线复合材料多尺度传热模型可以全面考虑钢帘线复杂的细观结构以及钢帘线与橡胶基体间的界面传热行为，准确计算分析橡胶与钢帘线接触界面产生热流聚集效应，和层间热扩散行为。整体上橡胶/钢帘线复合材料温度分布更为均匀，且呈现明显的辐射散热行为。随着钢帘线占比的增加，辐射散热在总散热量中的比例也随之增加，当钢帘线含量为10.9 %时，辐射散热占比达到50.8 %。通过对比串并联传热模型，多尺度传热模型计算误差从10.1%降低到2.5%。可进一步应用于橡胶产品的设计分析。

创新点

橡胶/钢帘线复合材料由于高强度，高弹性和高阻尼特性，广泛应用于轮胎、输送带、减震垫块等橡胶制品。这些产品不仅在硫化成型过程中需要经历高温高压作用，在使用中也会受到高温环境的影响而缩短使用寿命。因此橡胶/钢帘线复合材料的温度场分析和传热特性研究十分重要。采用传统的混合模型或串并联模型对橡胶/钢帘线复合材料进行传热模拟仍存在一定误差。其原因是该模型并不能全面考虑到复杂的钢帘线细观结构和界面传热热阻对传热性能的影响。另一方面仅通过对流换热系数定义热边界条件，忽略了辐射散热行为，是导致温度分布计算误差的又一重要原因。本论文通过建立多尺度橡胶/钢帘线复合材料传热模型，充分考虑钢帘线与基体的界面传热效应和高温下辐射散热对热分布的影响。不仅通过流体动力学模型(CFD)计算得到准确的自然对流换热系数，并结合实测温升曲线，进一步推导出合适的辐射散热系数。还进一步研究不同钢帘线占比、不同加热条件、和不同排列角度对橡胶/钢帘线复合材料的辐射散热的影响规律。结果表明，考虑辐射散热后，温度分布预测误差从10.1%减小到2.5%。随着钢帘线含量，温升工况的变化，辐射散热占总散热量的比例从45%提高到51%。
多尺度橡胶/钢帘线复合材料传热模拟(a)测温点(b)温升曲线(c)热流密度云图

图(17) / 表(4)

计量

文章访问数: 310
HTML全文浏览量: 207
PDF下载量: 21
被引次数: 14

1. 空气弹簧有效面积计算的理论分析
2. 实验对比与验证
3. 结论

基于多尺度模拟的橡胶/钢帘线复合材料辐射散热行为机制研究

通讯作者: 李文博，博士，讲师，研究方向为橡胶制品设计与仿真分析 E-mail: 645504616@qq.com

计量

出版历程

Study on the mechanism of radiation heat dissipation behavior of rubber/steel cord composites based on multi-scale simulation

1. 空气弹簧有效面积计算的理论分析

2. 实验对比与验证

3. 结 论

期刊类型引用(6)

其他类型引用(8)

计量

出版历程

目录

1. 空气弹簧有效面积计算的理论分析

2. 实验对比与验证

3. 结 论

通讯作者:
李文博，博士，讲师，研究方向为橡胶制品设计与仿真分析　E-mail: 645504616@qq.com

3. 结论

3. 结论