Effect of degree of aggregation of crystalline dendrites on the uniaxial tensile behavior of metallic glass matrix composites based on molecular dynamics simulations
-
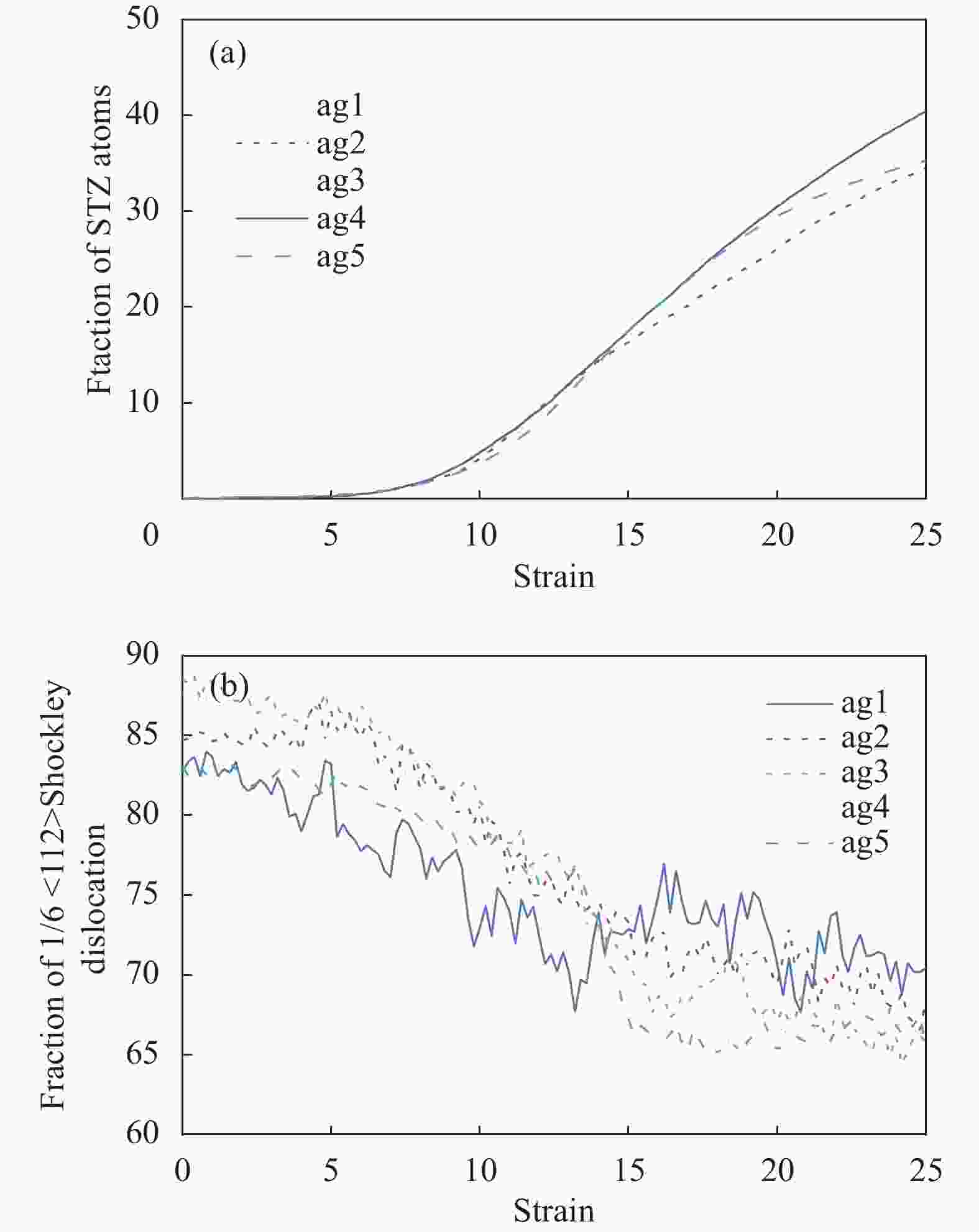
摘要: 引入第二相可以有效地提高金属玻璃基复合材料的塑性。本文通过大规模分子动力学模拟,研究了枝晶弥散分布在基体中的金属玻璃基复合材料试样在单轴拉伸载荷下的力学行为。结果表明,枝晶的聚集程度显著影响材料中剪切带的行为,并导致不同的材料拉伸塑性。枝晶体积分数较小时,金属玻璃基复合材料的塑性变形由STZ原子形成剪切带机制占主导,材料的屈服应力和峰值后应力降总体随枝晶聚集程度的减小而减小;枝晶体积分数较大时,材料塑性变形由枝晶内位错滑移机制占主导,材料的屈服强度和峰值后应力降总体随枝晶聚集程度的减小而增大;在枝晶体积分数中等的情况下,两种机制同时起作用且互相影响,使材料屈服应力和塑性变形能力随枝晶聚集程度变化而呈现复杂非单调的变化趋势。本文工作旨在为具有复杂第二相的金属玻璃基复合材料的设计提供指导。Abstract: Introducing crystalline dendrites can effectively enhance the plasticity of metallic glass matrix composites. In this study, the mechanical behaviors of numerical samples containing dispersed dendrites under uniaxial tensile loading were studied through large-scale molecular dynamics simulations. The results indicate that the degree of aggregation of the dendrites significantly affects the behavior of shear bands in the materials and ultimately leads to different tensile plasticity. When the dendrite volume fraction is relatively low, the plastic deformation of composites is dominated by the mechanism of shear band formation via the initiation of STZ atoms. Conversely, when the dendrite volume fraction is high, the plastic deformation of the material is primarily governed by dislocation slip within the dendrites. In the case of medium dendrite volume fractions, both mechanisms co-exist and interact with each other, resulting in a complex and non-monotonic trend of the yield stress and plastic deformation capability as the degree of dendrite aggregation changes. This work is aimed at providing guidelines for the design of metallic glass matrix composites with complex second phase dendritic structures.
-
Key words:
- shear band /
- mechanical behavior /
- metallic glass /
- molecular dynamics simulations /
- composites
-
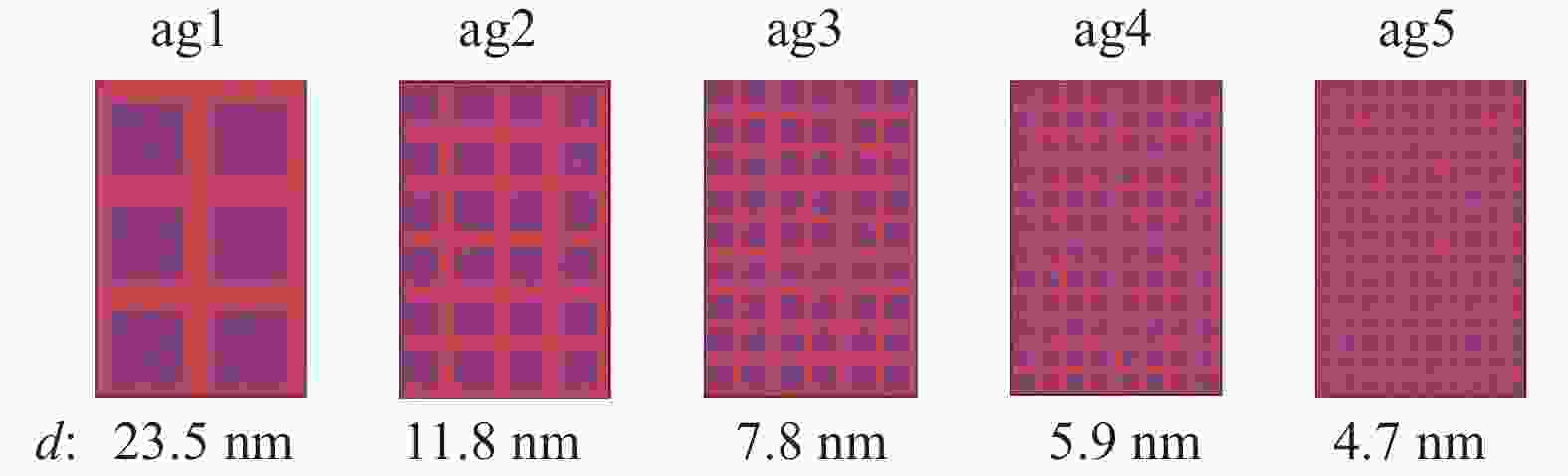
图 1 枝晶聚集程度不同的金属玻璃基复合材料的分子动力学(MD)试样模型。红色区域代表由FCC Cu原子组成的枝晶,其余区域代表金属玻璃基体。
Figure 1. Molecular Dynamics (MD) models of MG-matrix composites employed in tension simulation containing dendrites with different degree of aggregation, with the prominent red regions representing dendrites consisting of FCC Cu atoms and the rest representing the glass matrix.
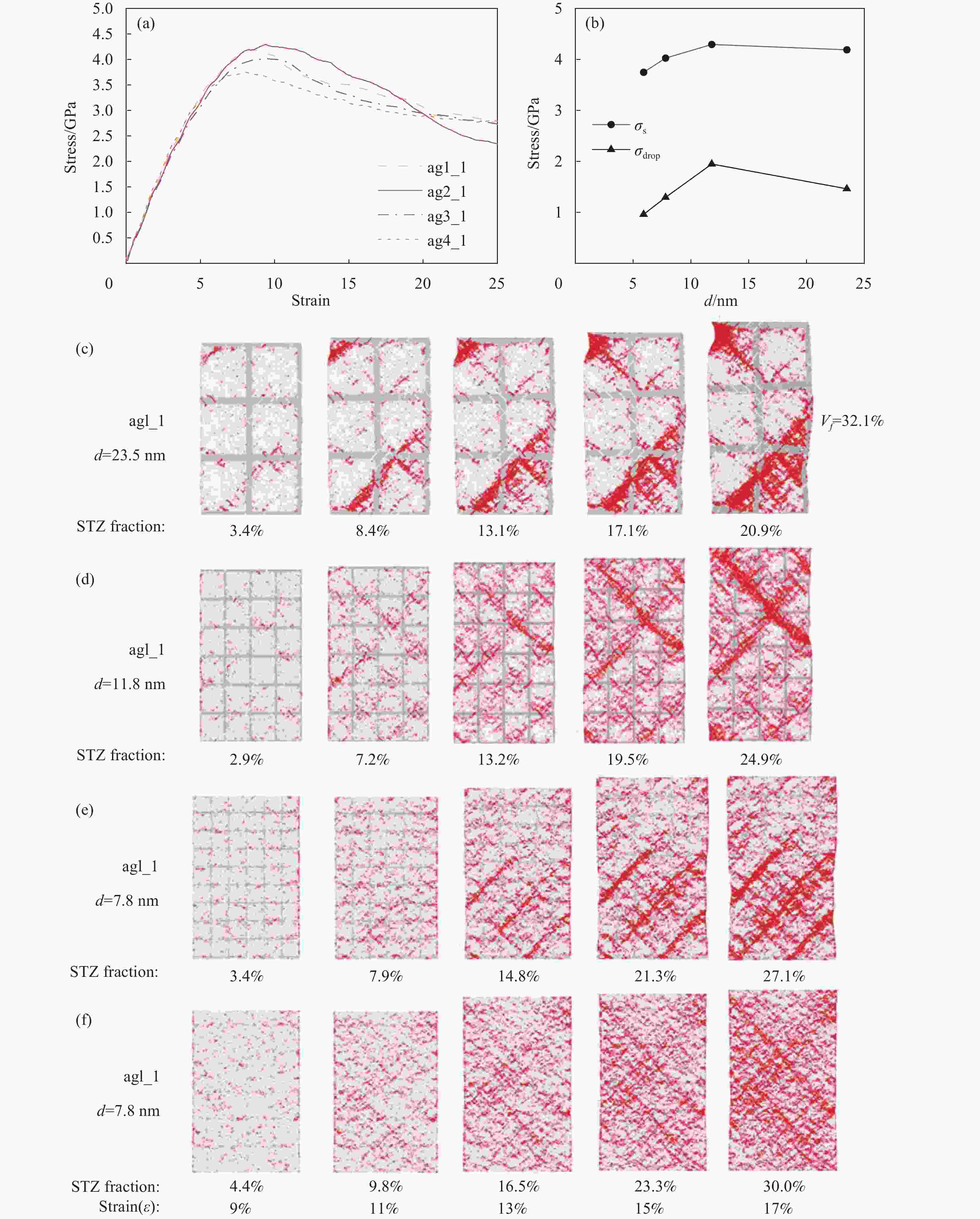
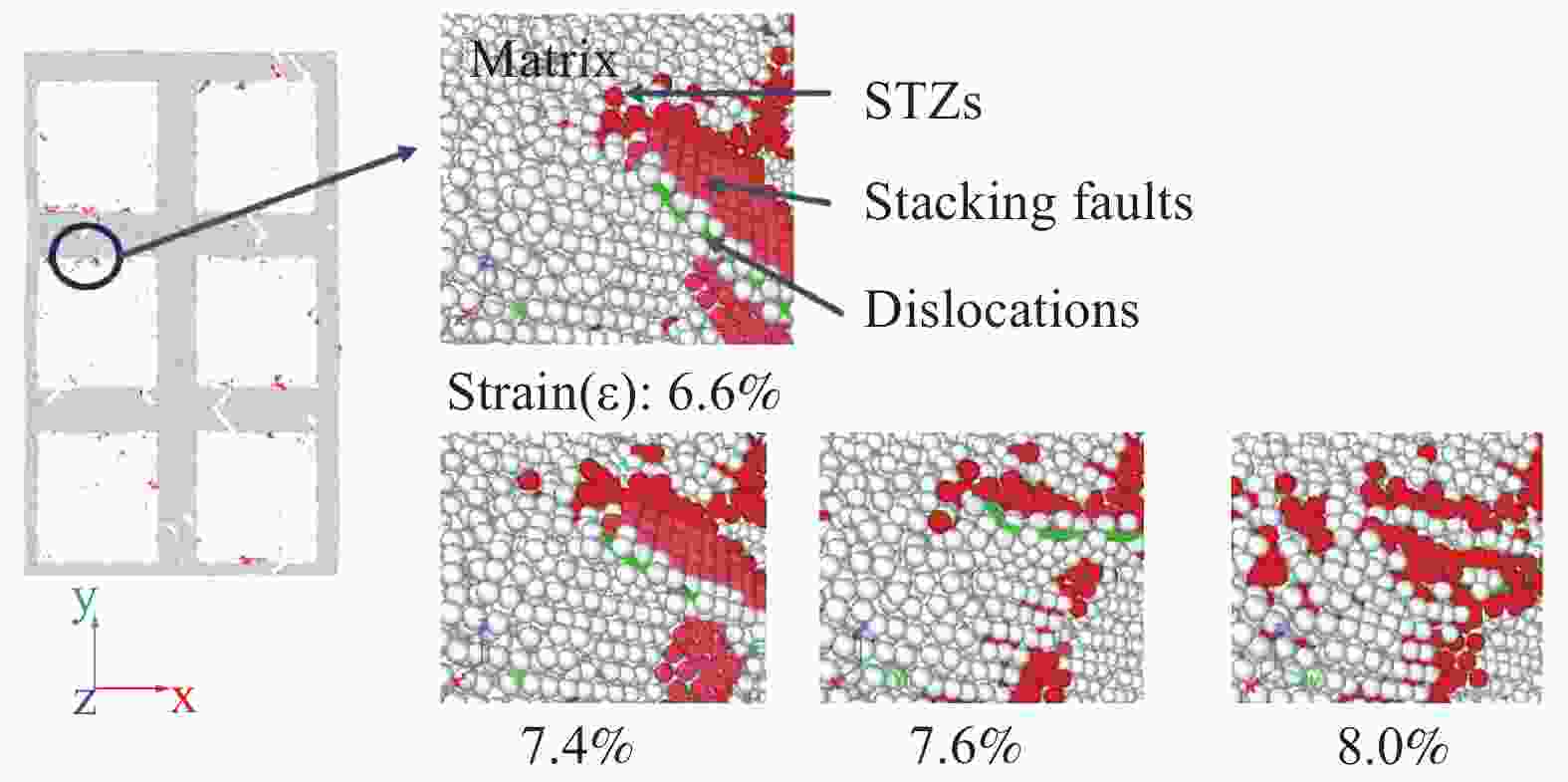
图 2 枝晶体积分数32.1%的金属玻璃基复合材料的力学行为:(a)不同枝晶聚集程度下的拉伸应力-应变曲线,(b)材料屈服应力、应力峰值后应力降与枝晶间间距d之间的关系,(c)-(f)各试样在不同应变下的剪切带演化
Figure 2. Mechanical behavior of MG matrix composites with 32.1% dendrite fraction, (a) stress-strain curves of the simulated samples of with different degree of dendrite aggregation, (b) the yield stress $ {\sigma }_{s} $, the stress drop after the peak stress $ {\sigma }_{drop} $ curves of the simulated samples of with different interdendritic spacing d, (c)-(f) snapshots showing the shear band evolution in the samples at different strains. The prominent red regions represent STZ atoms, the gray regions represent dendrites, and the rest light gray regions represent the glass matrix. The fraction of STZ atoms in the glass matrix, interdendritic spacing d and the volume fraction of dendrites Vf are marked near the snapshots.
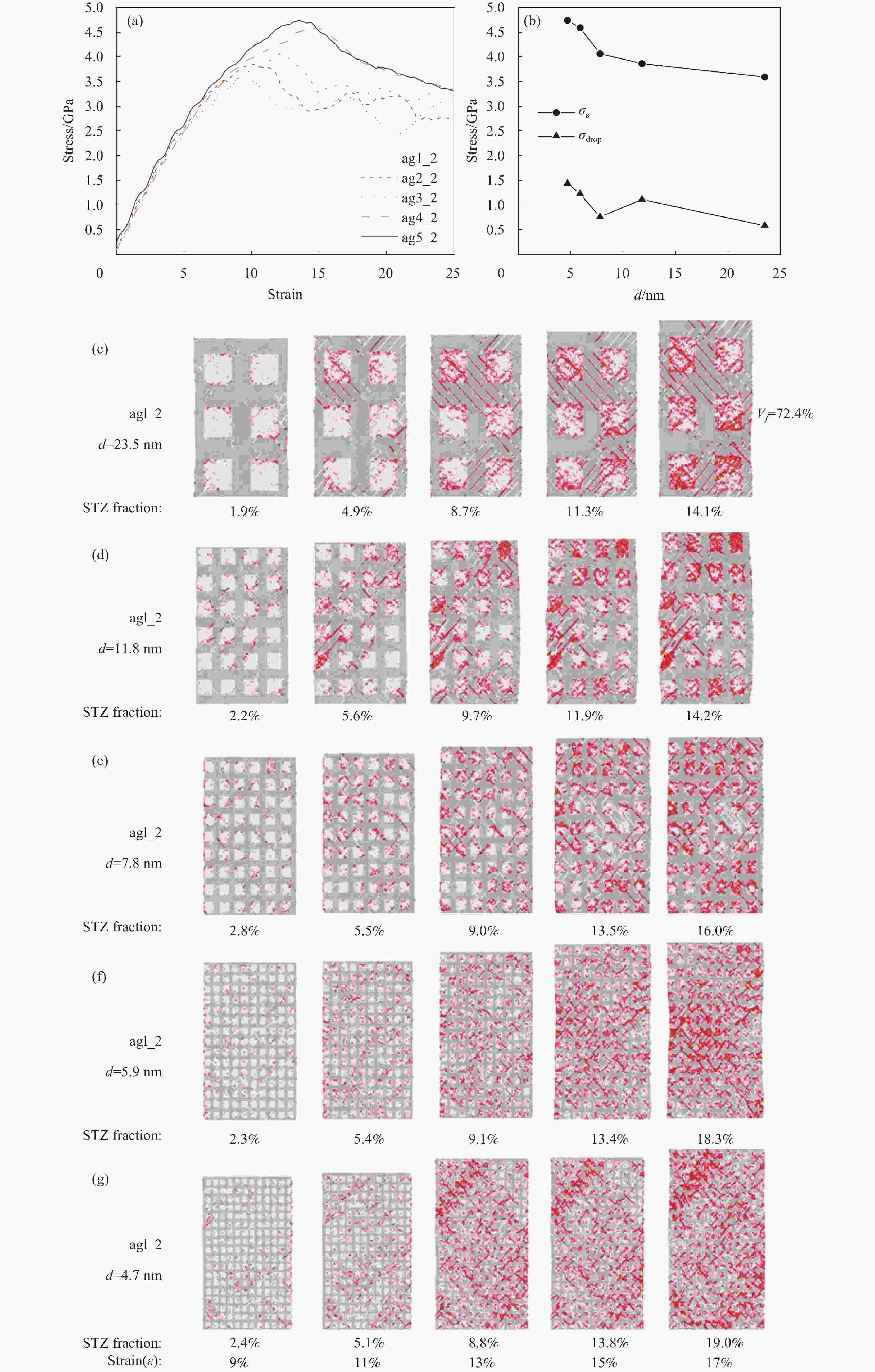
图 3 枝晶体积分数72.4%的金属玻璃基复合材料的力学行为:(a)不同枝晶聚集程度下的拉伸应力-应变曲线,(b)材料屈服应力、应力峰值后应力降与枝晶间间距d之间的关系,(c)-(g)各试样在不同应变下的剪切带演化
Figure 3. Mechanical behavior of MG matrix composites with 72.4% dendrite fraction, (a) stress-strain curves of the simulated samples of with different degree of dendrite aggregation, (b) the yield stress $ {\sigma }_{s} $, the stress drop after the peak stress $ {\sigma }_{drop} $ curves of the simulated samples of with different interdendritic spacing d, (c)-(g) snapshots showing the shear band evolution in the samples at different strains.
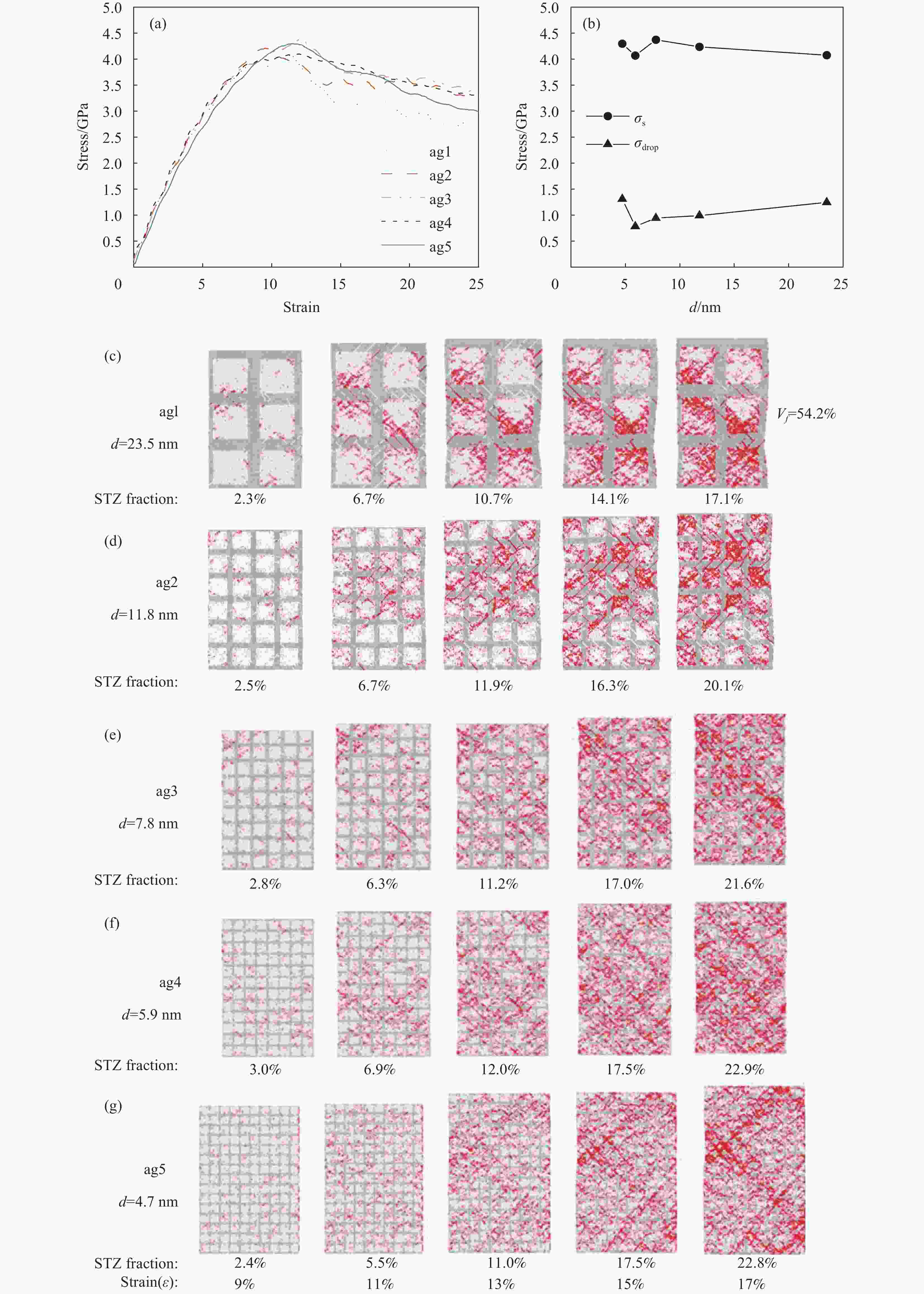
图 4 枝晶体积分数52.4%的金属玻璃基复合材料的力学行为:(a)不同枝晶聚集程度下的拉伸应力-应变曲线,(b) 材料屈服应力、应力峰值后应力降与枝晶间间距d之间的关系,(c)-(g)各试样在不同应变下的剪切带演化。红色区域表示STZ原子,灰色区域表示枝晶,其余浅灰色区域代表玻璃基体。STZ fraction为玻璃基体中STZ原子的占比分数,Vf为枝晶的体积分数。
Figure 4. Mechanical behavior of MG matrix composites with 52.4% dendrite fraction, (a) stress-strain curves of the simulated samples of with different degree of dendrite aggregation, (b) the yield stress $ {\sigma }_{s} $, the stress drop after the peak stress $ {\sigma }_{drop} $ curves of the simulated samples of with different interdendritic spacing d, (c)-(g) snapshots showing the shear band evolution in the samples at different strains.
-
[1] HOFMANN D C, ROBERTS S N. Microgravity metal processing: from undercooled liquids to bulk metallic glasses[J]. npj Microgravity, 2015, 1(1): 1-10. [2] DAVIDSON M, ROBERTS S, CASTRO G, et al. Investigating Amorphous Metal Composite Architectures as Spacecraft Shielding[J]. Advanced Engineering Materials, 2013, 15(1-2): 27-33. doi: 10.1002/adem.201200313 [3] PLUMMER J. Is metallic glass poised to come of age?[J]. Nature Materials, 2015, 14(6): 553-555. doi: 10.1038/nmat4297 [4] CONNER R D, DANDLIKER R B, JOHNSON W L. Mechanical properties of tungsten and steel fiber reinforced Zr41.25Ti13.75Cu12.5Ni10Be22.5 metallic glass matrix composites[J]. Acta Materialia, 1998, 46(17): 6089-6102. doi: 10.1016/S1359-6454(98)00275-4 [5] ZHANG H F, LI H, WANG A M, et al. Synthesis and characteristics of 80 vol. % tungsten (W) fibre/Zr based metallic glass composite[J]. Intermetallics, 2009, 17(12): 1070-1077. doi: 10.1016/j.intermet.2009.05.011 [6] QIAO J W, ZHANG Y, CHEN G L. Fabrication and mechanical characterization of a series of plastic Zr-based bulk metallic glass matrix composites[J]. Materials & Design, 2009, 30(10): 3966-3971. [7] WU F F, ZHANG Z F, MAO S X, et al. Effect of annealing on the mechanical properties and fracture mechanisms of a Zr56.2Ti13.8Nb5.0Cu6.9Ni5.6Be12.5 bulk-metallic-glass composite[J]. Physical Review B, 2007, 75(13): 134201. doi: 10.1103/PhysRevB.75.134201 [8] JIANG Y, QIU K. Computational micromechanics analysis of toughening mechanisms of particle-reinforced bulk metallic glass composites[J]. Materials & Design (1980-2015), 2015, 65: 410-416. [9] JIANG Y. Micromechanics constitutive model for predicting the stress–strain relations of particle toughened bulk metallic glass matrix composites[J]. Intermetallics, 2017, 90: 147-151. doi: 10.1016/j.intermet.2017.07.015 [10] LI J C, CHEN X W, HUANG F L. FEM analysis on the deformation and failure of fiber reinforced metallic glass matrix composite[J]. Materials Science and Engineering: A, 2016, 652: 145-166. doi: 10.1016/j.msea.2015.11.051 [11] LI J C, CHEN X W, HUANG F L. On the mechanical properties of particle reinforced metallic glass matrix composites[J]. Journal of Alloys and Compounds, 2018, 737: 271-294. doi: 10.1016/j.jallcom.2017.12.024 [12] FAN J, QIAO J W, WANG Z H, et al. Twinning-induced plasticity (TWIP) and work hardening in Ti-based metallic glass matrix composites[J]. Scientific Reports, 2017, 7(1): 1877. doi: 10.1038/s41598-017-02100-9 [13] SHETE M K, DUTTA T, SINGH I, et al. Tensile stress-strain response of metallic glass matrix composites reinforced with crystalline dendrites: Role of dendrite morphology[J]. Intermetallics, 2017, 83: 70-82. doi: 10.1016/j.intermet.2016.12.006 [14] THAMBURAJA P. Length scale effects on the shear localization process in metallic glasses: A theoretical and computational study[J]. Journal of the Mechanics and Physics of Solids, 2011, 59(8): 1552-1575. doi: 10.1016/j.jmps.2011.04.018 [15] 邱昆, 姜云鹏, 史雪萍, et al. 新型颗粒增强金属玻璃复合材料的拉伸增韧机制[J]. 复合材料学报, 2018, 35(1): 124-131. [16] ZHOU H, QU S, YANG W. An atomistic investigation of structural evolution in metallic glass matrix composites[J]. International Journal of Plasticity, 2013, 44: 147-160. doi: 10.1016/j.ijplas.2013.01.002 [17] SONG H Y, LI S, ZHANG Y G, et al. Atomic simulations of plastic deformation behavior of Cu50Zr50 metallic glass[J]. Journal of Non-Crystalline Solids, 2017, 471: 312-321. doi: 10.1016/j.jnoncrysol.2017.06.011 [18] SONG H Y, LI S, AN M R, et al. Effect of crystal phase on shear bands initiation and propagation behavior in metallic glass matrix composites[J]. Computational Materials Science, 2018, 150: 42-46. doi: 10.1016/j.commatsci.2018.03.069 [19] FENG S D, LI L, CHAN K C, et al. Control of shear band dynamics in Cu50Zr50 metallic glass by introducing amorphous-crystalline interfaces[J]. Journal of Alloys and Compounds, 2019, 770: 896-905. doi: 10.1016/j.jallcom.2018.08.192 [20] WANG W H, DONG C, SHEK C H. Bulk metallic glasses[J]. Materials Science and Engineering: R: Reports, 2004, 44(2): 45-89. [21] INOUE A, TAKEUCHI A. Recent Progress in Bulk Glassy Alloys[J]. Materials Transactions, 2002, 43(8): 1892-1906. doi: 10.2320/matertrans.43.1892 [22] PARK E S, KIM D H. Design of Bulk metallic glasses with high glass forming ability and enhancement of plasticity in metallic glass matrix composites: A review[J]. Metals and Materials International, 2005, 11(1): 19-27. doi: 10.1007/BF03027480 [23] MENDELEV M I, SORDELET D J, KRAMER M J. Using atomistic computer simulations to analyze x-ray diffraction data from metallic glasses[J]. Journal of Applied Physics, 2007, 102: 043501. doi: 10.1063/1.2769157 [24] CHENG Y Q, SHENG H W, MA E. Relationship between structure, dynamics, and mechanical properties in metallic glass-forming alloys[J]. Physical Review B, 2008, 78(1): 014207. doi: 10.1103/PhysRevB.78.014207 [25] LI M, WANG C Z, HAO S G, et al. Structural heterogeneity and medium-range order in ZrxCu100−x metallic glasses[J]. Physical Review B, 2009, 80(18): 184201. doi: 10.1103/PhysRevB.80.184201 [26] EVANS D J, HOLIAN B L. The Nose–Hoover thermostat[J]. Journal of Applied Physics, 1985, 83: 4069-4074. [27] SHIMIZU F, OGATA S, LI J. Theory of Shear Banding in Metallic Glasses and Molecular Dynamics Calculations[J]. Materials Transactions, 2007, 48(11): 2923-2927. doi: 10.2320/matertrans.MJ200769 [28] STUKOWSKI A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool[J]. Modelling and Simulation in Materials Science and Engineering, 2010, 18(015012)[2023-08-12]. [29] CAO A J, CHENG Y Q, MA E. Structural processes that initiate shear localization in metallic glass[J]. Acta Materialia, 2009, 57(17): 5146-5155. doi: 10.1016/j.actamat.2009.07.016 -

 点击查看大图
点击查看大图
计量
- 文章访问数: 44
- HTML全文浏览量: 31
- 被引次数: 0




 下载:
下载: