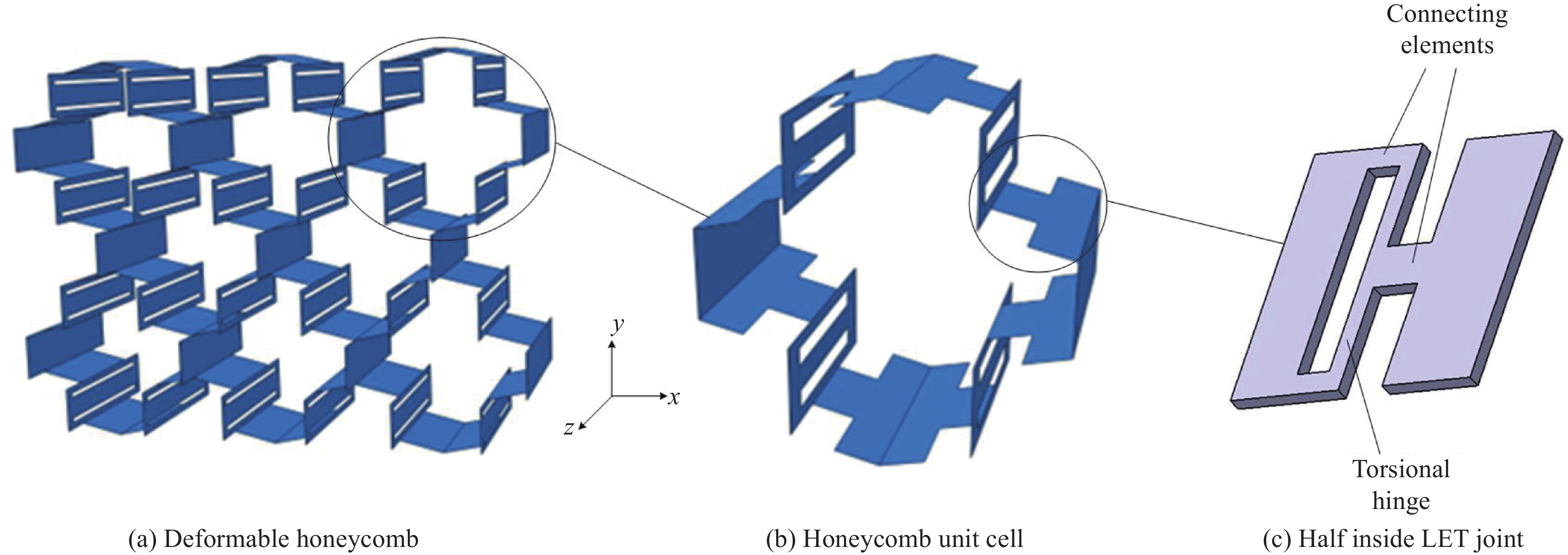
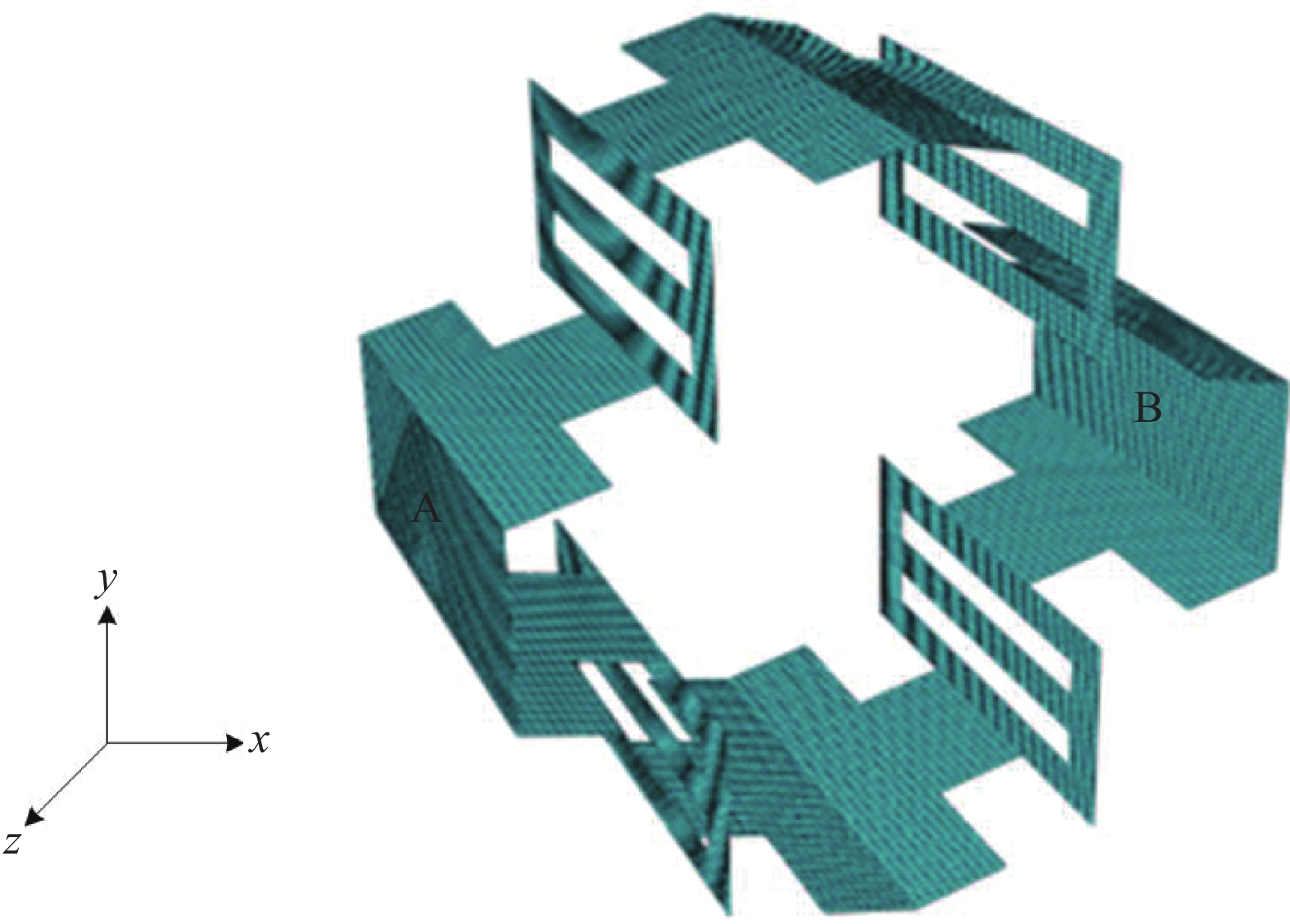
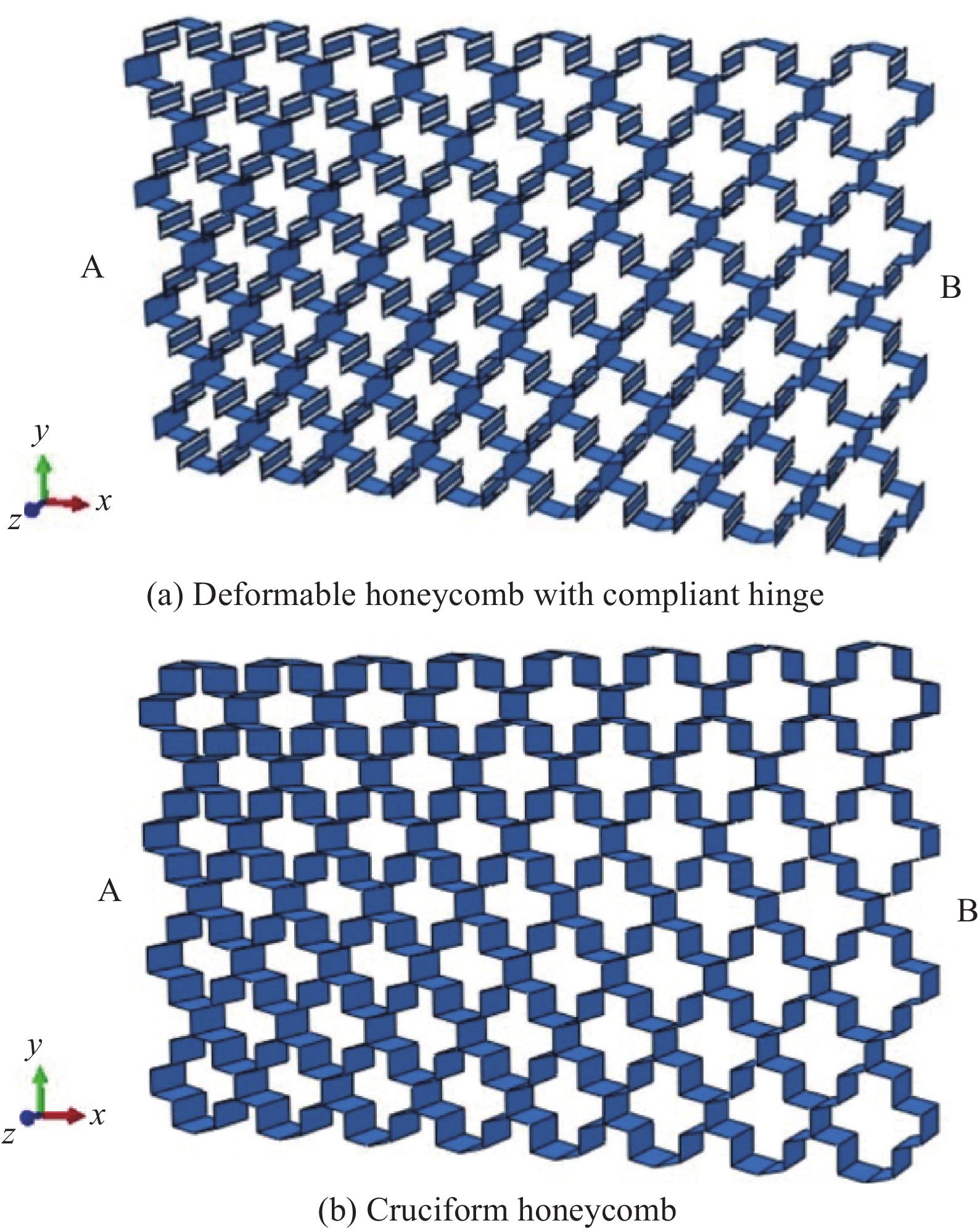
In-plane tensile property of deformable honeycomb structure with compliant hinge
-
摘要:
提出了一种柔性铰可变形蜂窝结构，该结构由十字形蜂窝和内置式平面折展扭转柔性半铰(Half inside lamina emergent torsional joint，内LET半铰)组成，通过降低结构面内刚度提升变形能力，具有质量轻、面内模量低的特点。通过理论分析研究了柔性铰可变形蜂窝结构的面内等效弹性模量，并进行了仿真和实验验证，分析了几何参数对结构等效弹性模量的影响，最后通过仿真和实验比较了柔性铰可变形蜂窝与十字形蜂窝的面内刚度。结果表明：柔性铰可变形蜂窝的等效弹性模量与十字形蜂窝相比降低了80%以上，具有更优秀的面内变形能力，将可变形蜂窝结构与柔性铰链巧妙结合是一种提升蜂窝结构面内变形能力的有效方式。
Abstract:A deformable honeycomb structure with compliant hinge which is composed of the cross-shaped honeycomb and the half inside lamina emergent torsional joint was proposed. By reducing the in-plane stiffness, the deformation ability of the structure is improved, and it has the characteristics of light weight and low in-plane modulus. The in-plane equivalent elastic modulus of the deformable honeycomb structure was studied by theoretical analysis, and simulation and experimental verification were carried out. The influence of geometric parameters on the equivalent elastic modulus of the structure was also analyzed. The in-plane deformation ability of the deformable honeycomb with compliant hinge and the cross-shaped honeycomb was compared through simulation and experiment. The results show that the deformable honeycomb structure with compliant hinge has better in-plane deformable ability. Compared with cross-shaped honeycomb, the equivalent elastic modulus of deformable honeycomb with compliant hinge is reduced by more than 80%. Thus, the combination of compliant hinge and deformable honeycomb structure is an effective way to improve the in-plane deformation ability of honeycomb structure.
-
糖尿病是一种严重威胁人类健康的慢性病。全球糖尿病患者人数从1980年的1.08亿增加到2023年的5.37亿。葡萄糖传感器在糖尿病的诊断和治疗中起着重要作用[1-3]。糖尿病患者要定期检测生理血糖水平，并将血糖水平维持在正常浓度范围内。而且，准确评价食品中的葡萄糖含量对维持糖尿病患者血液中葡萄糖的生理水平至关重要[4-5]。食品和饮料中葡萄糖含量的信息对生产者和消费者都有参考价值。葡萄糖检测在葡萄酒酿造工艺和乳制品工业的发酵过程中是至关重要的[4-5]。迄今为止，检测葡萄糖的方法很多。在各种分析方法中，电化学葡萄糖传感器具有灵敏度高、选择性好、操作简单、成本低等优点，并能实现自我监控和床边血糖检测[5-8]。基于酶的电化学葡萄糖传感器已经商业化并取得了巨大的成功。由于自然酶容易受到环境(温度、湿度、酸碱度等)影响，非酶葡萄糖传感器受到广泛关注[1-3, 8]。
金属-有机框架(Metal-origanic frameworks，MOFs)材料是一类新兴的多孔材料，存在电化学传感器应用潜力[9-12]。它们具有金属活性位点丰富、表面积大、结构多样、孔径可调和功能可调等优点。Li等[13]开发了Co-MOF纳米片阵列构建葡萄糖检测平台，其灵敏度为
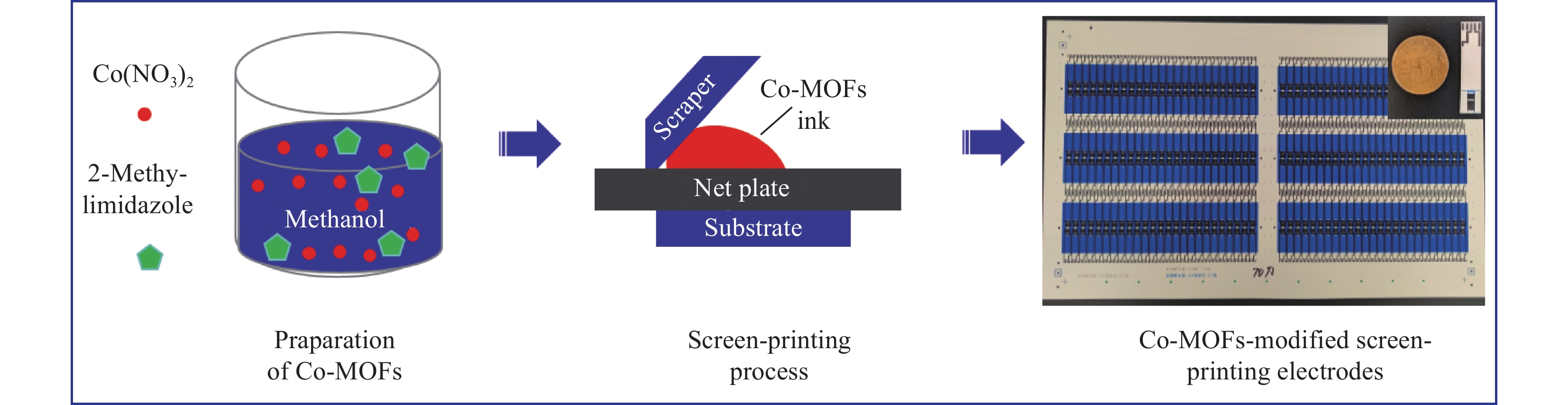
10886 μA·L/(mmol·cm2)，检测极限为1.3 nmol/L。Khan等[14]以MOF-199为前驱体合成 CuO/C复合材料，催化葡萄糖的氧化反应。Cu-MOFs修饰电极在0.06至5 mmol/L的线性范围内，对葡萄糖氧化显示出相对较好的电催化活性，其灵敏度为89 mA·L/(mmol·cm2)，检测限为10.5 nmol/L。在另一份报告中，球形Ni-MOFs 颗粒在单独使用时表现出较差的电化学葡萄糖传感性能[15]。然而，当它们与碳纳米管的杂交后，对葡萄糖检测的灵敏度为13.85 mA·L/(mmol·cm2)，检测极限为0.82 mmol/L，线性范围为1至1.6 mmol/L。此外，Zha等[16]开发了基于NiCo-MOF/C复合材料的无创血糖检测平台，其高灵敏度和检测极限分别为2701.29 μA·L/(mmol·cm2)和0.09 μmol/L。MOFs衍生复合材料在电化学葡糖糖传感器领域得到一定程度的应用。另一方面，随着实时传感设备和护理点设备的发展需要，经济的、可靠的、规模化的电极制备方法受到广泛关注[17]。作为一种商业化电极制备方法，丝网印刷技术具有设备简单、图案设计灵活、操作简单、经济等特点[17]。该技术在生物传感器领域，尤其指尖血糖检测中取得商业成功。Li等[18]实验组通过丝网印刷技术开发了一种具有优化三电极配置的多功能电化学平台，检测葡萄糖浓度。Ji等[19]实验组基于智能手机的循环伏安系统，采用石墨烯修饰的丝网印刷电极检测葡萄糖浓度。因此，本文在室温条件合成Co基MOFs(Co-ZIF-67)，采用丝网印刷技术，制备了Co-ZIF-67修饰的商业银-碳电极，研究其对葡萄糖的传感性能。
1. 实验方法
1.1 原材料与试剂
六水硝酸钴(Co(NO3)2·6H2O，99.5%) 、聚乙烯醇(PVA，92%~94%)、聚乙烯吡咯烷酮(PVP，K23-27)、甲醇(CH2OH，99.5%)、3-(N-吗啉)丙磺酸钠(MOPs-Na，C7H14NO4SNa，99.5%)、羟乙基纤维素(HEC)、丙烯酰胺(C3H5NO，99.0%)、抗坏血酸(C6H6O8，99.0%)、半乳糖(C6H12O6，99.0%)、羧甲基纤维素((C6+2yH7+x+2yO2+x+3yNay)n)、柠檬酸(C6H8O7，99.5%)、葡萄糖(C6H12O6，96%)和葡聚糖(DEAE-Dextran，70 kDa)购自上海阿拉丁生化科技股份有限公司。过硫酸铵(H8N2O8S2，98.5%)购自上海麦克林生化科技有限公司。
1.2 材料表征
采用X-射线衍射仪(Smartlab9kw，Rigaku)对样品的物相和晶体结构进行表征。通过X射线光电子能谱(ESCALAB 250Xi，赛默飞)对样品的元素和表面信息进行分析。采用扫描电子显微镜扫描电镜(SEM，SU8100，日立)和透射电子显微镜(TEM，JEM2100，JEOL)对样品进行形貌表征。通过电化学工作站(CH650E，上海辰华仪器有限公司)评估修饰电极对葡萄糖的电化学传感性能。本研究配制了不同浓度葡萄糖(0.1~0.5 mmol/L)的0.1 mol/L氢氧化钠溶液。
1.3 Co-ZIF-67及Co-ZIF-67修饰电极的制备
Co-ZIF-67纳米材料在室温条件下制备而成。合成过程中，12 mmol/L的Co(NO3)2·6H2O完全溶解于100 mL甲醇中，记为溶液 A；48 mmol/L的2-甲基咪唑溶解于
1000 mL甲醇中，记为溶液 B。溶液B迅速地加入到溶液A中，形成混合液C。该混合液C磁力搅拌10 min后，在室温环境下静置24 h，形成沉淀物。采用甲醇清洗沉淀物，并在60℃干燥过夜，得到紫色Co-ZIF-67粉末。把20 mg Co-ZIF-67在1 mL的超纯水中超声30 min，得到溶液D。0.55 g MOPs 钠盐，0.075 g的羟乙基纤维素，1.75 g丙烯酰胺和0.05 g过硫酸铵分别溶解于25 mL的超纯水中，磁力搅拌2 h后形成混合浆料。将1 mL溶液D与9 mL浆料磁性搅拌1 h后，形成 Co-ZIF-67丝网印刷油墨。
将Co-ZIF-67油墨均匀的丝网印刷在商业银-碳电极的工作区域，经烘干(45℃，15 min)、贴亲水膜、裁剪，制备了便携式一次性条形葡萄糖检测电极。该电极包括一个工作电极，一个对电极。工作电极的表面积为3.78 mm×0.252 mm=
0.9526 mm2。每个电极所分析的溶液量为10 μL。亲水膜的作用是形成流道，吸附检测样品。Co-MOF修饰电极的制备过程见图1。![]()
图 1 Co-ZIF-67修饰电极的制备过程Figure 1. Preparation of Co-ZIF-67 modified electrodesMOFs—Metal-origanic frameworks2. 结果与讨论
2.1 Co-ZIF-67物相分析
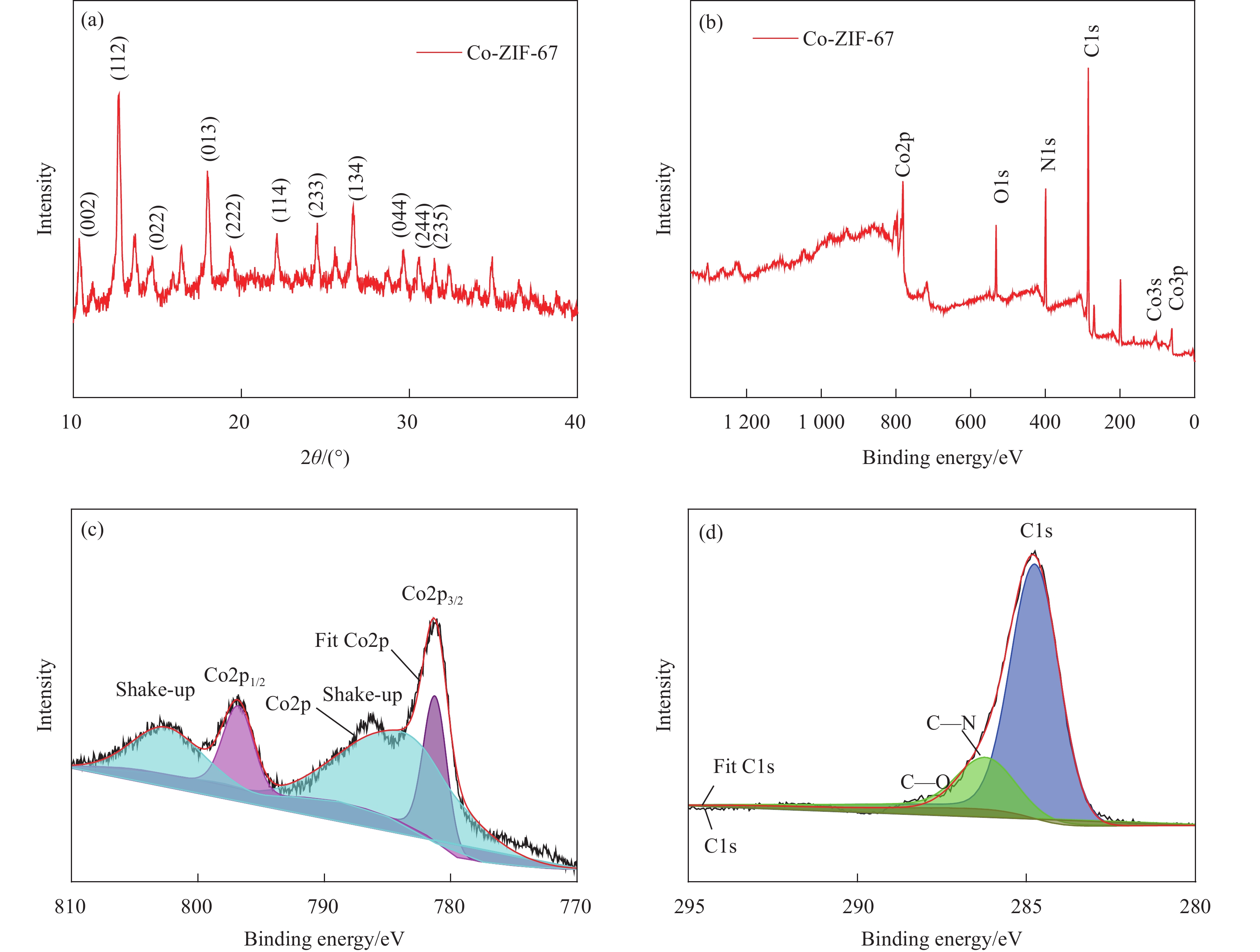
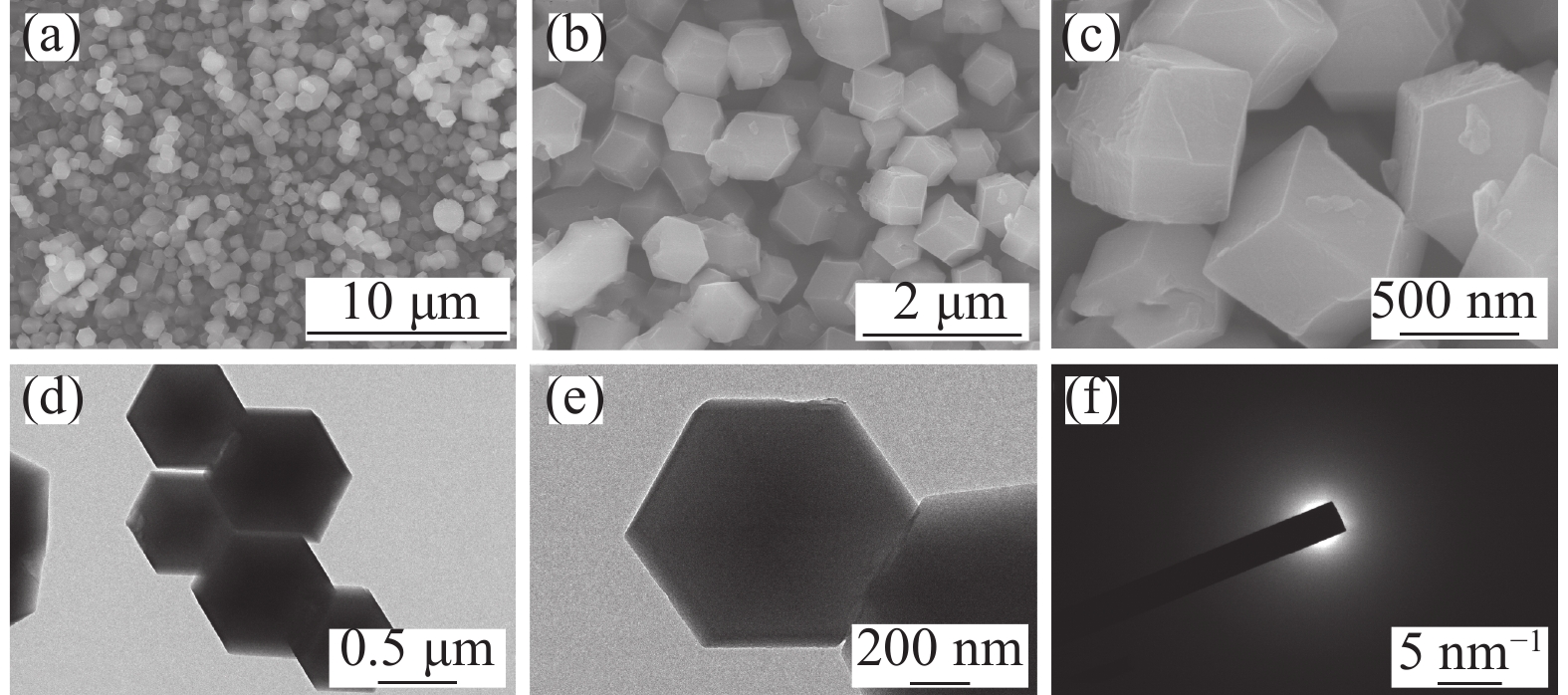
采用XRD技术研究了Co-ZIF-67的晶体结构。从图2(a)可以看出，在2θ=10.4°、12.7°、14.7°、16.4°、18.0°、22.1°、24.4°、26.5°、29.8°、30.5°和32.5°时，分别对应于ZIF-67的(002)、(112)、(022)、(013)、(222)、(114)、(233)、(134)、(044)、(244)、(235)晶面，这与已报道的ZIF-67样品的XRD结果一致[20-22]。采用X射线光电子能谱(XPS)对Co-ZIF-67的表面信息进行了分析。从图2(b)可以看出，样品包含Co2p、O1s、N1s、C1s、Co3s和Co3p核能级区域。Co2p和 C1s的XPS精细谱分别如图2(c)和图2(d)所示。Co2p精细谱含有两个主峰，其中780.1 eV峰来自Co2p3/2；795.3 eV峰来自Co2p1/2。激振峰分别位于785.5和801.7 eV。除了主峰，C1s精细谱还有两个拟合峰。结合能位于286.2和288.1 eV，分别归属于C—N和C—O。上述结果表明，Co-ZIF-67已经被成功制备。采用SEM和TEM研究了样品的形貌。如图3(a)~3(f)所示，Co-ZIF-67呈现多面形，且尺寸分布相对较窄。
![]()
图 2 Co-ZIF-67的XRD图谱(a)、XPS全谱(b)、Co2p (c)和C1s (d)精细谱Figure 2. XRD pattern (a), XPS spectra (b), Co2p (c) and C1s regions (d) of Co-ZIF-67![]()
图 3 Co-ZIF-67在不同放大倍数的SEM和TEM图像Figure 3. SEM and TEM images of Co-ZIF-67 at different magnifications2.2 Co-ZIF-67的电催化性能
采用循环伏安(CV)技术评估了Co-ZIF-67修饰电极的电化学性能。图4(a)是Co-ZIF-67修饰电极在50 mV/s扫速时对0.3 mmol/L葡萄糖在不同pH值溶液中的响应信号。很明显，当pH=13时，Co-ZIF-67表现出对葡萄糖较大的催化活性。当葡萄糖浓度增加时，Co-ZIF-67修饰电极电流信号也随之增强(图4(b))。然而，信号的区分度不大。图4(c)是Co-ZIF-67修饰电极在不同扫速(10、30、50、70、90、110和130 mV/s)下对葡萄糖信号的变化。随着扫速增大，电流信号明显得到加强。将0.5 V电流强度与扫描速度的算术平方根进行拟合，其线性关系为：I (μA/cm2)=0.14v1/2–0.12 (R2=0.988，R2为决定系数)。这说明Co-ZIF-67修饰电极对应的电化学反应是受扩散控制的[23]。
![]()
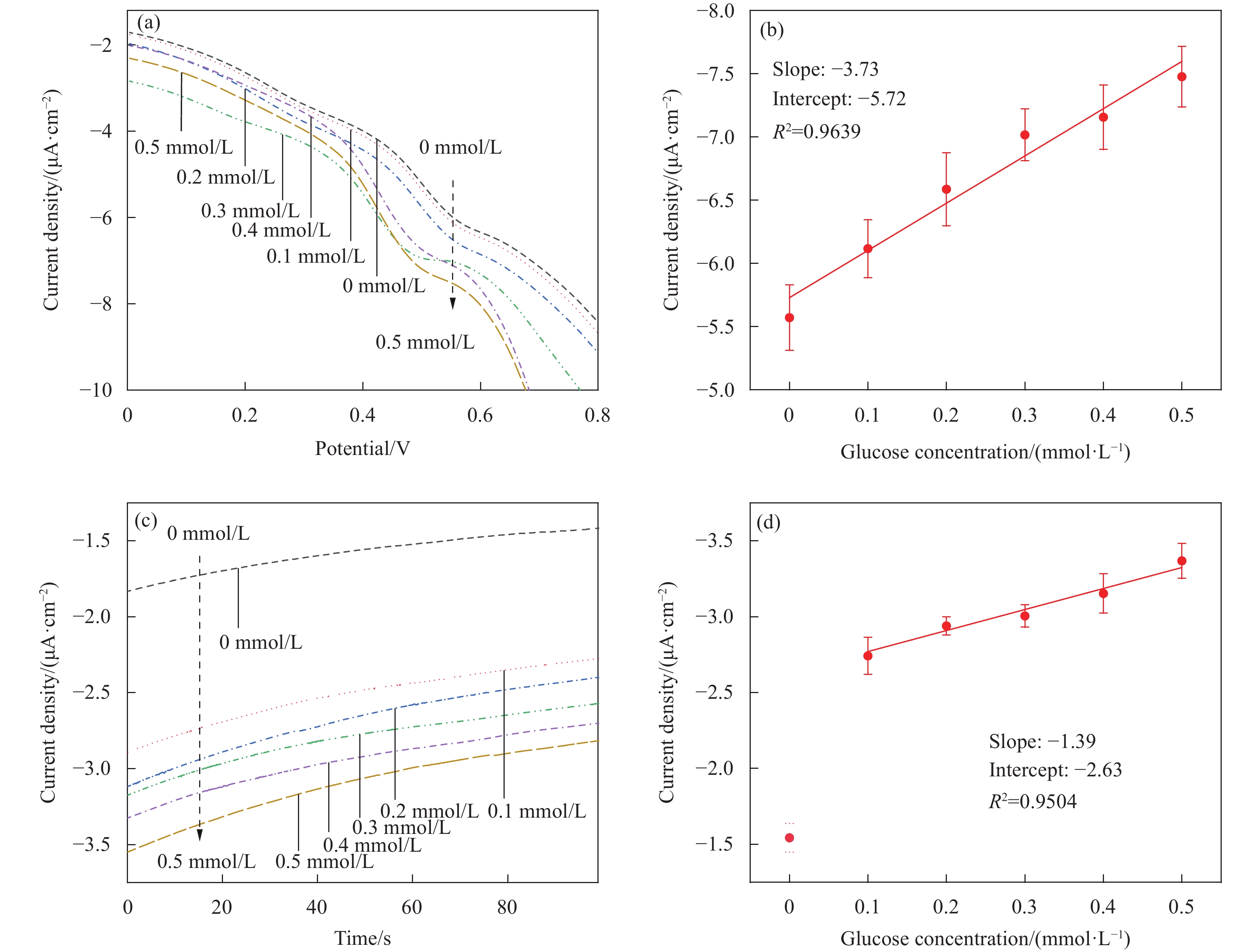
图 4 Co-ZIF-67修饰电极在不同pH值(a)、葡萄糖浓度(b)、扫速(c)对葡萄糖的CV测试曲线；(d)扫描速率(v)的算数平方根与电流(I) (0.5 V)之间的线性关系Figure 4. CV curves of Co-ZIF-67 modified electrodes with different pH (a), glucose concentrations (b), and scan rates (c); (d) Corresponding linear relationship between the arithmetic square root of the scanning rate (v) and current (I, 0.5 V)R2—The coefficient of determination, which determinates the linear relationship of the fit curve采用差分脉冲伏安法(Differential pulse voltammetry，DPV)进一步评估了Co-ZIF-67修饰电极的电化学性能。如图5(a)所示，在0~0.5 mmol/L 葡萄糖溶液中观测了Co-ZIF-67修饰电极表面的氧化和还原反应，其对葡萄糖可能的催化机制为：Co-ZIF-67修饰电极对葡萄糖表现出较CV更强的DPV响应信号，这也表明，Co-ZIF-67对葡萄糖确实存在电催化效果[24-27]。此外，溶液中没有葡萄糖时，Co-ZIF-67修饰电极在0.4~0.6 V有一个不明显的氧化还原峰。随着葡萄糖浓度的增加，该修饰电极的响应信号也随之增强，氧化还原峰变得更加明显，这主要是由于高电位下碱性溶液中Co-ZIF-67中Co2+被氧化为Co3+。此时，Co3+因从葡萄糖得电子(变为Co2+)并不断将葡萄糖氧化为葡萄糖酸从而产生电流信号[28-29]。因此，Co-ZIF-67修饰电极具有较好的电催化性能。如图5(b)所示，Co-ZIF-67修饰电极的电流平均值(0.55 V)与葡萄糖浓度呈线性关系，其线性方程为：I (μA/cm2)=−3.730×C(mmol/L) − 5.720 (R2=
0.9639 )。![]()
图 5 (a) Co-ZIF-67修饰电极在不同葡萄糖浓度中的差分脉冲伏安法(DPV)测试曲线；(b)每5支Co-ZIF-67修饰电极在0.55 V电位对不同浓度葡萄糖的平均电流响应信号；(c) Co-ZIF-67修饰电极对不同葡萄糖浓度的安培响应；(d)每5支电极对不同葡萄糖浓度的平均响应电流(取第15 s数值)Figure 5. (a) Differential pulse voltammetry (DPV) curves of Co-ZIF-67 modified electrodes in the presence of glucose; (b) Linear relationship between average DPV current density response and different glucose concentrations of every five electrodes at 0.55 V; (c) Amperometric response of Co-ZIF-67 modified SPEs to different glucose concentration; (d) Corresponding linear curve of average current density of five electrodes in the 15th s to glucose concentrations采用安培响应技术在Co-ZIF-67修饰电极上对葡萄糖的传感性能做了进一步的评估。图5(c)显示随着电解质溶液中葡萄糖浓度的增加，响应电流随之增强。安培响应电流与葡萄糖浓度之间呈线性关系(图5(d))，其方程为：I (μA/cm2)=−1.390×C(mmol/L)−2.630 (R2=
0.9504 )。经过处理，Co-ZIF-67修饰电极对葡萄糖的检测灵敏度为1390 nA·L/(mmol·cm2)，检测限为0.58 μmol/L (S/N=3)，线性范围为0.1~0.5 mmol/L。值得一提的是，与已报道的电极相比，Co-ZIF-67修饰电极的灵敏度具有较大的优势，如表1所示[27, 29-34]。表 1 Co-ZIF-67修饰电极及其他电极的葡萄糖传感性能Table 1. Glucose sensing performance of Co-ZIF-67-modified electrodes and other previously reported electrodesType of electrode Sensitivity/(μA·L·mmol−1·cm−2) Detection limit/(μmol·L−1) Linear range/(mmol·L−1) Ref. Ag NPs/MOF-74(Ni) 1290 4.7 0.01-4 [27] NF/NiCo2O4 NWs@Co3O4 NPs 8163.2 – 0.001-1.7 [29] CuCo-MOF 6861 0.12 – [30] Ni2Co1-BDC/GCE 3925.3 0.29 0.0005 -2.8995 [31] Ni/Co(HHTP)MOF/CC 3250 0.1 0.0003 -2.312[32] MIL-88A@NiFe-PB 1963.2 0.12 0.005-1 [33] Ni3(HHTP)2/CNT 4774 4.1 0.004-3.9 [34] Co-MOFs/SPEs 1.393 0.58 0.1-0.5 This work Notes: CC—Carbon cloth; BDC—1, 4-benzenedicarboxylic acid; GCE—Glassy carbon electrode; HHTP—2, 3, 6, 7, 10, 11-hexahydroxytriphenylene; MIL—Materials from Institute Lavoisier; PB—Prussian blue; CNT—Carbon nanotubes; NF—Nickel foam; NWs—Nanowires; NPs—Nanoparticles; SPEs—Screen-printing electrodes. 2.3 抗干扰性、稳定性和重现性
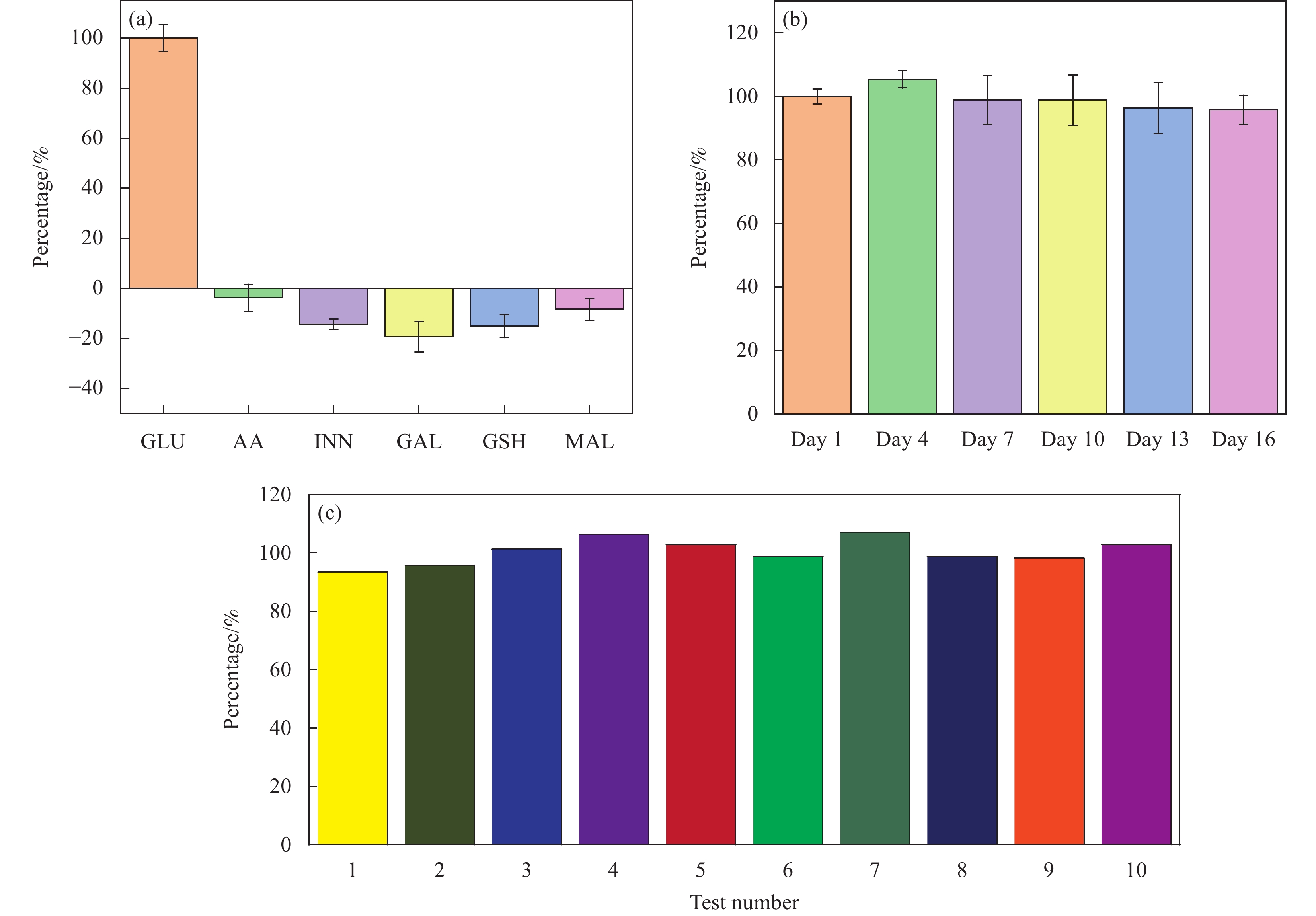
图6(a)描述了Co-ZIF-67修饰电极抗干扰性能。从图上可以看出，干扰物质抗坏血酸(AA，3 mmol/L)、艾考糊精(INN，0.164 mol/L)、半乳糖(GAL，8 mmol/L)、谷胱甘肽(GSH，30 mmol/L)、麦芽糖(MAL，0.584 mol/L)引起的响应电流变化分别为−3.9%、−14.3%、−19.3%、−14.6%和−8.4%。与干扰物质相比，滴加0.1 mmol/L葡萄糖溶液时电流响应的显著变化表明。因此，Co-ZIF-67修饰电极具有较强的抗干扰能力。随后，通过长时间空气存放观察Co-ZIF-67修饰电极对0.1 mmol/L 葡萄糖的电流响应来评估的其稳定性。如图6(b)所示，Co-ZIF-67修饰电极表现出良好的稳定性。16天后，该电极仍然具有96%的初始响应。重现性是对电极的一个重要衡量标准。如图6(c)所示，Co-ZIF-67修饰电极的相对标准方差(Relative standard deviation，RSD)仅为10%，这说明该电极具有较好的重现性。
![]()
图 6 (a)干扰检查：5支Co-ZIF-67修饰电极 对0.1 mmol/L 葡萄糖(GLU)、0.164 mol/L 艾考糊精(INN)、9 mmol/L 半乳糖(GAL)、30 mmol/L谷胱甘肽(GSH)和0.584 mol/L 麦芽糖(MAL) 的平均安培响应；(b)稳定性：每5支Co-ZIF-67修饰电极在第1 d、4 d、7 d、10 d、13 d和16 d内对0.1 mmol/L 葡萄糖的安培响应信号；(c)重现性：10支Co-ZIP-67修饰电极对0.1 mmol/L 葡萄糖的响应Figure 6. (a) Interference examination: Average amperometric responses of five CuO nanomaterials modified SPEs to 0.1 mmol/L glucose (GLU), 0.164 mol/L alcodextrin (INN), 9 mmol/L galactose (GAL), 30 mmol/L glutathione (GSH) and 0.584 mol/L maltose (MAL); (b) Stability of every 5 Co-ZIF-67 modified electrodes to 0.1 mmol/L glucose on the 1st, 4th, 7th, 10th, 13th and 16th days; (c) Reproducibility of Co-ZIF-67 modified electrodes to 0.1 mmol/L glucose2.4 血清测试
为了研究Co-ZIF-67修饰电极在实际样品中检测葡萄糖的性能，我们进行了加标回收实验(拜安进血糖仪(拜安进血糖试纸(葡萄糖脱氢酶)，拜耳公司))。将血清稀释在NaOH溶液中，血清浓度为0.12 mmol/L。如表2所示，葡萄糖的回收率在93.97%~101.5%，RSD小于6.2%。这也表明Co-ZIF-67修饰电极具有潜在应用。
表 2 Co-ZIF-67修饰的Ag-C电极检测血清样品的葡萄糖含量(n=3)Table 2. Glucose detection in human serum samples using Co-ZIF-67 modified Ag-C electrodes (n=3)Sample Serum glucose/(mmol·L−1) Added glucose/(mmol·L−1) Detected glucose/(mmol·L−1) RSD/% Recovery rate/% Human
serum0.12 0.18 0.29 6.20 93.97 0.28 0.39 4.37 101.5 0.36 0.47 3.90 98.97 Note: RSD—Relative standard deviation. 3. 结 论
(1)基于室温合成的Co-ZIF-67，采用丝网印刷技术批量构建了Co-ZIF-67修饰的商业银-碳电极。
(2) Co-ZIF-67修饰电极表现出优异的葡萄糖电催化性能：0.58 μmol/L的检测极限，1.393 μA·L/(mmol·cm2)的灵敏度，高的抗干扰性，96%的空气稳定性。
(3)研究表明，Co-ZIF-67的低能耗合成及其Co-ZIF-67修饰电极的批量化制备为葡萄糖传感器的发展提供一个可参考的方向。
-
![]()
图 1 柔性铰可变形蜂窝几何模型
Figure 1. Geometry model of the deformable honeycomb with compliant hinge
LET—Lamina emergent torsional
![]()
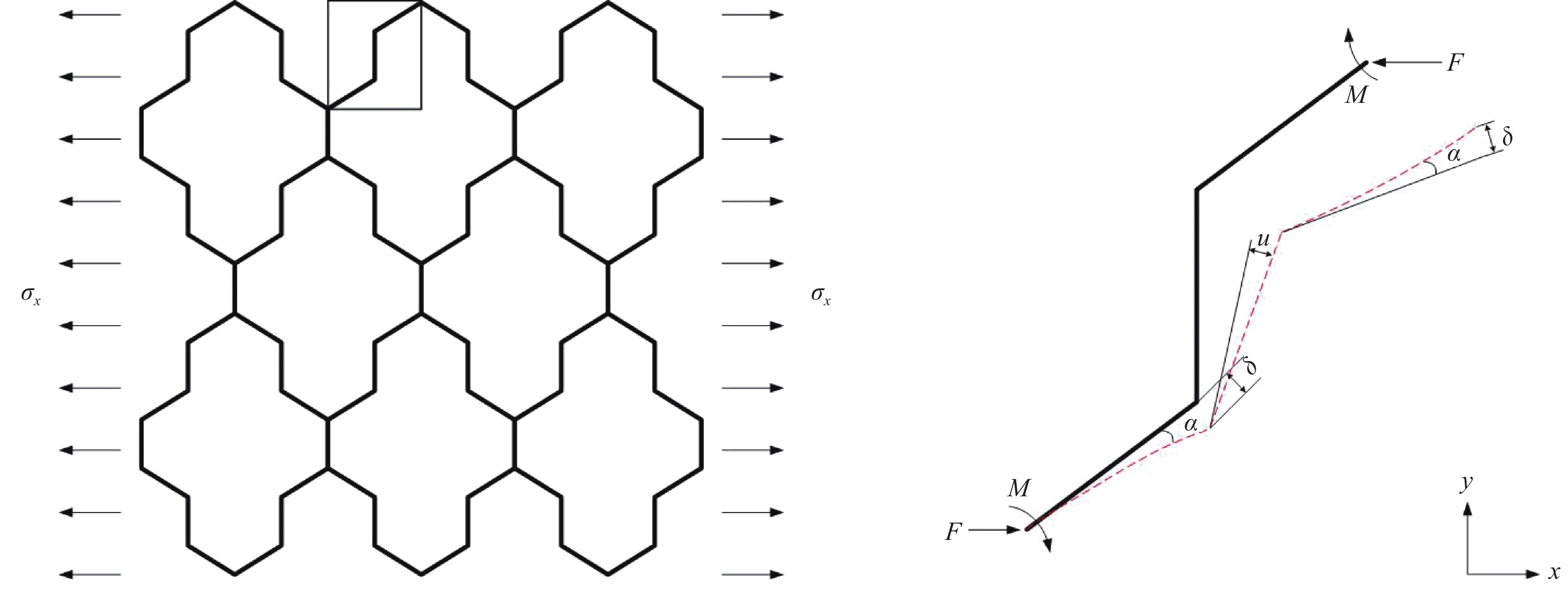
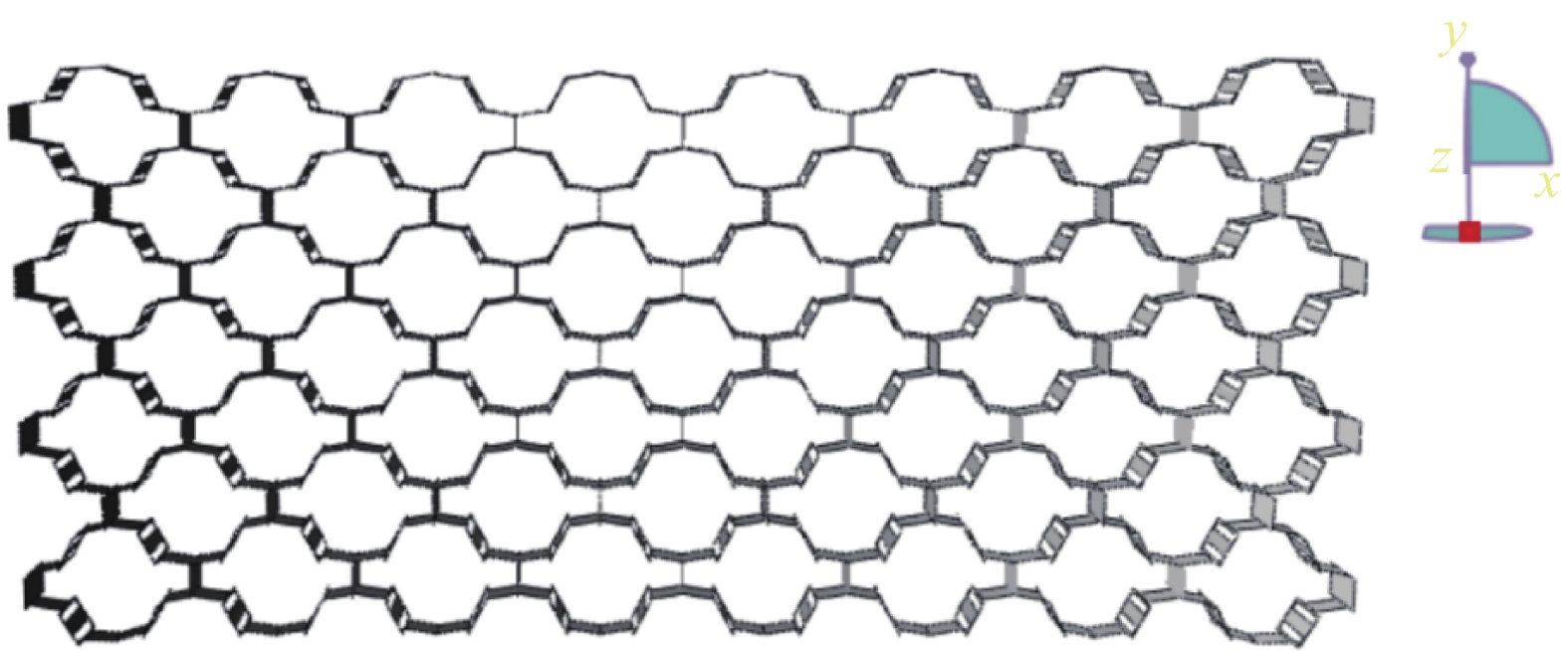
图 2 柔性铰可变形蜂窝单元的几何参数
Figure 2. Geometric parameters of deformable honeycomb with compliant hinge unit cell
l1—Length of the inclined beam; t1—Thickness of the inclined beam; l2—Length of the vertical beam; t2—Thickness of the vertical beam; θ—Honeycomb angle; d1—Width of connecting element in the inclined beam; h1—Length of connecting element in the inclined beam; d2—Width of the connecting element in the vertical beam; h2—Length of the connecting element in the vertical beam; d3—Width of torsional hinge
![]()
图 3 在x方向加载时柔性铰可变形蜂窝受力分析
Figure 3. Force analysis of deformable honeycomb with compliant hinge loaded in x direction
σx—Equivalent stress along the x-direction; F—Tensile load along the x-direction; M—Bending moment of the cross section; δ—Deflection of the inclined beam; α—Rotation angle of the inclined beam; u—Deflection of the vertical beam
![]()
图 4 内LET半铰伪刚体模型
Figure 4. Pseudo-rigid-body model of half inside LET joint
kB1 and kB2—Spring constant of connecting elements in the inclined beam and vertical beam, respectively; kT—Spring constant of torsional hinge
![]()
图 5 柔性铰可变形蜂窝结构的有限元模型
Figure 5. Finite element model of deformable honeycomb structure with compliant hinge
A—Boundary A; B—Boundary B
![]()
图 6 不同d1值下柔性铰可变形蜂窝结构等效弹性模量E*随h1的变化规律
Figure 6. Variation law of equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge with h1 for different d1 values
![]()
图 7 不同d3值下柔性铰可变形蜂窝结构等效弹性模量E*随h1的变化规律
Figure 7. Variation law of equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge with h1 for different d3 values
![]()
图 8 不同h2值下柔性铰可变形蜂窝结构等效弹性模量E*随h1的变化规律
Figure 8. Variation law of equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge with h1 for different h2 values
![]()
图 9 不同d2值下柔性铰可变形蜂窝结构等效弹性模量E*随h1的变化规律
Figure 9. Variation law of equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge with h1 for different d2 values
![]()
图 10 柔性铰可变形蜂窝结构实验件模型和单轴拉伸实验
Figure 10. Test specimen model and uniaxial tensile experiment for the deformable honeycomb structure with compliant hinge
![]()
图 11 4×8构型的柔性铰可变形蜂窝和十字形蜂窝结构模型
Figure 11. Deformable honeycomb with compliant hinge and the cruciform honeycomb structure models with 4×8 configuration
![]()
图 13 十字形蜂窝结构实验件模型和单轴拉伸实验
Figure 13. Test specimen model and uniaxial tensile experiment for the cruciform honeycomb structure
![]()
图 14 柔性铰可变形蜂窝和十字形蜂窝的应力-应变关系
Figure 14. Stress-strain relationship of deformable honeycomb with compliant hinge and cruciform honeycomb
σx—Equivalent stress along the x-direction; εx—Equivalent strain along the x-direction
表 1 柔性铰可变形蜂窝结构等效弹性模量E*理论与仿真值的比较
Table 1 Comparison between the theoretical and finite element method (FEM) results of equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge
Number Parameter of the unit/mm E* (Theoretical value)/MPa E* (FEM value)/MPa Error/% 1 d1=2, d2=2, d3=1, h1=7, h2=1 6.20×10−3 6.13×10−3 1.13 2 d1=4, d2=2, d3=1, h1=7, h2=1 5.90×10−3 5.83×10−3 1.19 3 d1=6, d2=2, d3=1, h1=7, h2=1 5.60×10−3 5.56×10−3 0.71 4 d1=10, d2=2, d3=1, h1=4, h2=1 3.90×10−3 3.79×10−3 2.82 5 d1=10, d2=2, d3=1.5, h1=4, h2=1 4.80×10−3 4.72×10−3 1.67 6 d1=10, d2=2, d3=2, h1=4, h2=1 5.40×10−3 5.49×10−3 1.67 7 d1=10, d2=2, d3=1, h1=3, h2=2 3.80×10−3 3.74×10−3 1.58 8 d1=10, d2=2, d3=1, h1=3, h2=3 4.10×10−3 4.12×10−3 0.49 9 d1=10, d2=2, d3=1, h1=3, h2=4 4.40×10−3 4.49×10−3 2.05 10 d1=10, d2=0.75, d3=1, h1=6, h2=1 5.20×10−3 5.05×10−3 2.88 11 d1=10, d2=1.5, d3=1, h1=6, h2=1 4.90×10−3 4.87×10−3 0.61 12 d1=10, d2=2.25, d3=1, h1=6, h2=1 4.60×10−3 4.75×10−3 3.26 
下载: 导出CSV
表 2 柔性铰可变形蜂窝和十字形蜂窝的结构参数
Table 2 Parameters of the deformable honeycomb with compliant hinge and the cruciform honeycomb
Number Configuration Parameter of the honeycomb unit/mm Parameter of the compliant hinge/mm Parameter of the model/mm 1 4×8 l1= l2=10, t1= t2=0.25, b=25, θ=13° d1=10, d2=2, d3=1, h1=12, h2=1 312×186×25 2 5×10 l1= l2=5, t1= t2=0.1, b=10, θ=13° d1=5, d2=1, d3=0.5, h1=5, h2=0.5 195×118×10 3 6×8 l1= l2=20, t1= t2=1, b=40, θ=13° d1=20, d2=4, d3=3, h1=20, h2=2 624×568×40
下载: 导出CSV
表 3 柔性铰可变形蜂窝结构和十字形蜂窝结构的面内拉伸等效弹性模量E*
Table 3 Equivalent elastic modulus E* of the deformable honeycomb structure with compliant hinge and the cruciform honeycomb
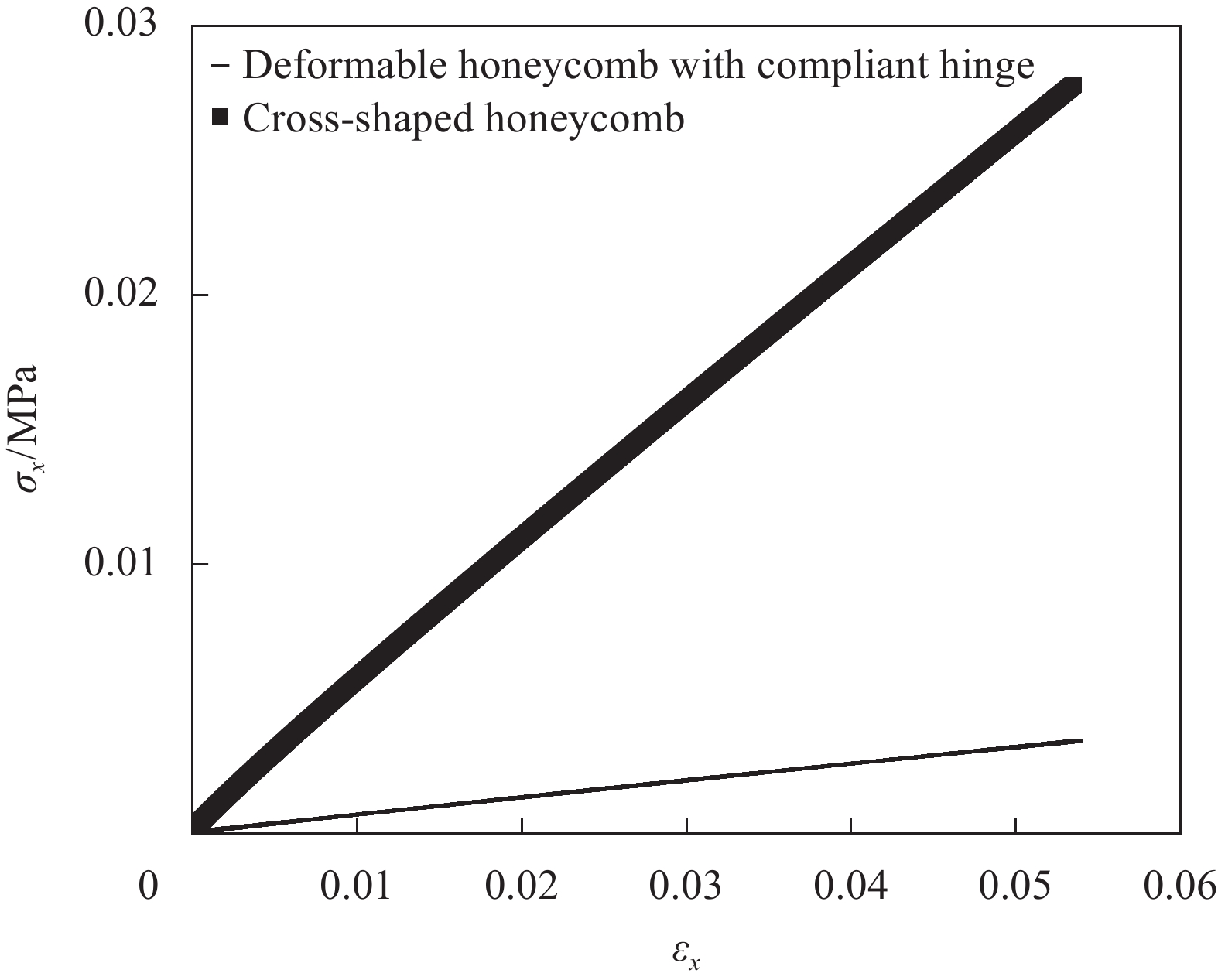
Number Configuration E* (The deformable honeycomb
with compliant hinge)/MPaE* (The cruciform honeycomb)/MPa Difference value/% 1 4×8 0.0064 0.0595 89.2 2 5×10 0.0054 0.0305 82.3 3 6×8 0.0725 0.4515 83.9
下载: 导出CSV
-
[1] LI D, ZHAO S, DA RONCH A, et al. A review of modelling and analysis of morphing wings[J]. Progress in Aerospace Sciences, 2018, 100: 46-62.
[2] LI Y Z, GE W J, ZHOU J, et al. Design and experiment of concentrated flexibility-based variable camber morphing wing[J]. Chinese Journal of Aeronautics, 2022, 35(5): 455-469. DOI: 10.1016/j.cja.2021.04.030
[3] WEISSHAAR T A. Morphing aircraft systems: Historical perspectives and future challenges[J]. Journal of Aircraft, 2013, 50(2): 337-353. DOI: 10.2514/1.C031456
[4] CHU L L, LI Q, GU F, et al. Design, modeling, and control of morphing aircraft: A review[J]. Chinese Journal of Aeronautics, 2022, 35(5): 220-246. DOI: 10.1016/j.cja.2021.09.013
[5] MKHOYAN T, THAKRAR N R, DE BREUKER R, et al. Morphing wing design using integrated and distributed trailing edge morphing[J]. Smart Materials and Structures, 2022, 31(12): 125025. DOI: 10.1088/1361-665X/aca18b
[6] 沈元, 昂海松, 刘卫东. 用于变形机翼夹心式柔性伸缩蒙皮的梯形蜂窝支撑结构[J]. 复合材料学报, 2015, 32(3): 815-822. SHEN Yuan, ANG Haisong, LIU Weidong. Trapezoidal cellular support structure applied to flexible telescope sandwich skin of morphing wing[J]. Acta Materiae Compositae Sinica, 2015, 32(3): 815-822(in Chinese).
[7] 徐云研, 程小全, 张纪奎, 等. 复合材料蜂窝夹层结构T型接头拉伸性能研究[J]. 工程力学, 2015, 32(7): 243-256. DOI: 10.6052/j.issn.1000-4750.2013.12.1236 XU Yunyan, CHENG Xiaoquan, ZHANG Jikui, et al. Study on composite honeycomb sandwich structure formed T-joints under tensile load[J]. Engineering Mechanics, 2015, 32(7): 243-256(in Chinese). DOI: 10.6052/j.issn.1000-4750.2013.12.1236
[8] LIU W D, YANG Z D, DU S, et al. Theoretical, numerical and experimental study on the in-plane elastic behavior of a 2D chiral cellular structure[J]. Composite Structures, 2022, 296: 115889. DOI: 10.1016/j.compstruct.2022.115889
[9] YU X D, ZHANG Q C, KONTOPOULOU A, et al. In-plane elasticity of beetle elytra inspired sandwich cores[J]. Composite Structures, 2022, 300: 116155. DOI: 10.1016/j.compstruct.2022.116155
[10] 沈元, 朱华, 刘卫东, 等. 三种柔性蒙皮支撑结构的力学特性[J]. 振动. 测试与诊断, 2017, 37(1): 18-25. SHEN Yuan, ZHU Hua, LIU Weidong. Mechanical characteristics of three support structures of flexible skin[J]. Journal of Vibration, Measurement & Diagnosis, 2017, 37(1): 18-25(in Chinese).
[11] FENG N, WANG S B, TIE Y H, et al. Elastic deformability and zero Poisson's ratio within a novel structure inspired by the gardenia from nature[J]. Mechanics of Advanced Materials and Structures, 2024, 31(6): 1300-1312.
[12] CHEN J J, SHEN X, LI J F. Zero Poisson’s ratio flexible skin for potential two-dimensional wing morphing[J]. Aerospace Science and Technology, 2015, 45: 228-241. DOI: 10.1016/j.ast.2015.05.011
[13] 郭之熙, 肖俊华. 多弧段曲边内凹蜂窝可调泊松比结构的力学性能研究[J]. 工程力学, 2023, 40(10): 204-212, 236. GUO Zhixi, XIAO Junhua. Mechanical properties of multi-arc concave honeycomb structure with adjustable Poisson's ratio[J]. Engineering Mechanics, 2023, 40(10): 204-212, 236(in Chinese).
[14] SCARPA F, SMITH F C, CHAMBERS B, et al. FE analysis of the in-plane mechanical properties of a novel Voronoi-type lattice with positive and negative Poisson's ratio configurations[J]. International Journal of Solids and Structures, 2010, 107(1069): 175-183.
[15] BOUAKBA M, BEZAZI A, SCARPA F. Mechanical and electromagnetic behaviour of auxetic honeycomb structures[J]. Aeronautical Journal, 2012, 49(18): 2450-2459.
[16] HU L L, LUO Z R, ZHANG Z Y, et al. Mechanical property of re-entrant anti-trichiral honeycombs under large deformation[J]. Composites Part B: Engineering, 2019, 163: 107-120. DOI: 10.1016/j.compositesb.2018.11.010
[17] 尤泽华, 肖俊华, 王美芬. 弧边内凹蜂窝负泊松比结构的力学性能[J]. 复合材料学报, 2022, 39(7): 3570-3580. YOU Zehua, XIAO Junhua, WANG Meifen. Mechanical properties of arc concave honeycomb structure with negative Poisson’s ratio[J]. Acta Materiae Compositae Sinica, 2012, 39(7): 3570-3580(in Chinese).
[18] GRIMA J N, GATT R, FARRUGIA P S. On the properties of auxetic meta-tetrachiral structures[J]. Physica Status Solidi (B)-Basic Solid State Physics, 2008, 245(3): 511-520. DOI: 10.1002/pssb.200777704
[19] FU M H, ZHENG B B, LI W H. A novel chiral three-dimensional material with negative Poisson's ratio and the equivalent elastic parameters[J]. Composite Structures, 2017, 176: 442-448. DOI: 10.1016/j.compstruct.2017.05.027
[20] 王雪松, 刘卫东, 刘典. 新型反四手性蜂窝结构的面内拉伸弹性[J]. 复合材料学报, 2023, 40(8): 4849-4861. WANG Xuesong, LIU Weidong, LIU Dian. In-plane tensile elasticity of a novel anti-tetrachiral cellular structure[J]. Acta Materiae Compositae Sinica, 2023, 40(8): 4849-4861(in Chinese).
[21] QIAO J X, CHEN C Q. Impact resistance of uniform and functionally graded auxetic double arrowhead honeycombs[J]. International Journal of Impact Engineering, 2015, 83: 47-58. DOI: 10.1016/j.ijimpeng.2015.04.005
[22] GAO Q, WANG L M, ZHOU Z, et al. Theoretical, numerical and experimental analysis of three-dimensional double-V honeycomb[J]. Materials & Design, 2018, 139: 380-391.
[23] OLYMPIO K R, GANDHI F. Flexible skins for morphing aircraft using cellular honeycomb core[J]. Journal of Intelligent Material Systems and Structure, 2010, 21(17): 1719-1735. DOI: 10.1177/1045389X09350331
[24] ZHANG P, ZHOU L, QIU T. Design and application of cross-shaped cellular honeycomb for a variable camber wing[J]. Journal of Aircraft, 2012, 49(5): 1451-1459. DOI: 10.2514/1.C031733
[25] 程文杰, 周丽, 张平, 等. 零泊松比十字形混合蜂窝设计分析及其在柔性蒙皮中的应用[J]. 航空学报, 2015, 36(2): 680-690. CHENG Wenjie, ZHOU Li, ZHANG Ping, et al. Design and analysis of a zero Poisson's ratio mixed cruciform honeycomb and its application in flexible skin[J]. Acta Aeronautica et Astronautica Sinica, 2015, 36(2): 680-690(in Chinese).
[26] GONG X B, HUANG J, SCARPA F, et al. Zero Poisson's ratio cellular structure for two-dimensional morphing applications[J]. Composite Structures, 2015, 134: 384-392. DOI: 10.1016/j.compstruct.2015.08.048
[27] JACOBSEN J O, CHEN G M, HOWELL L L, et al. Lamina emergent torsional (LET) joint[J]. Mechanism and Machine Theory, 2009, 44(11): 2098-2109. DOI: 10.1016/j.mechmachtheory.2009.05.015
-
其他相关附件
-
目的
变体飞机可以自适应地改变形状以适应不断变化的飞行条件，使飞机具有多任务适应性。蜂窝结构具有重量轻、面内变形好、面外承载能力强等优良特性，被广泛应用于变体飞机柔性蒙皮结构。为了提升柔性蒙皮蜂窝结构的变形能力，本文提出了一种柔性铰可变形蜂窝结构，通过降低结构面内刚度提升变形能力。
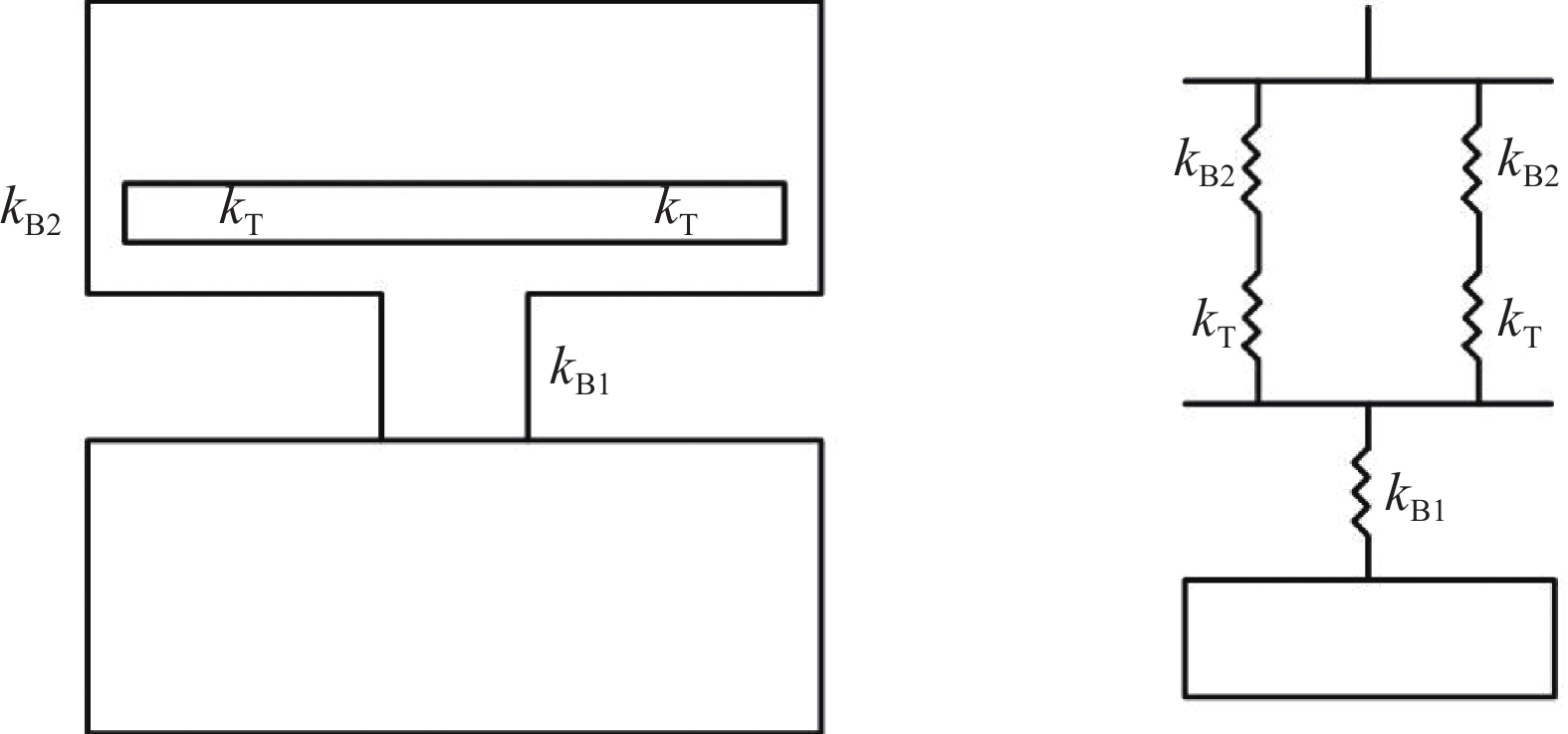
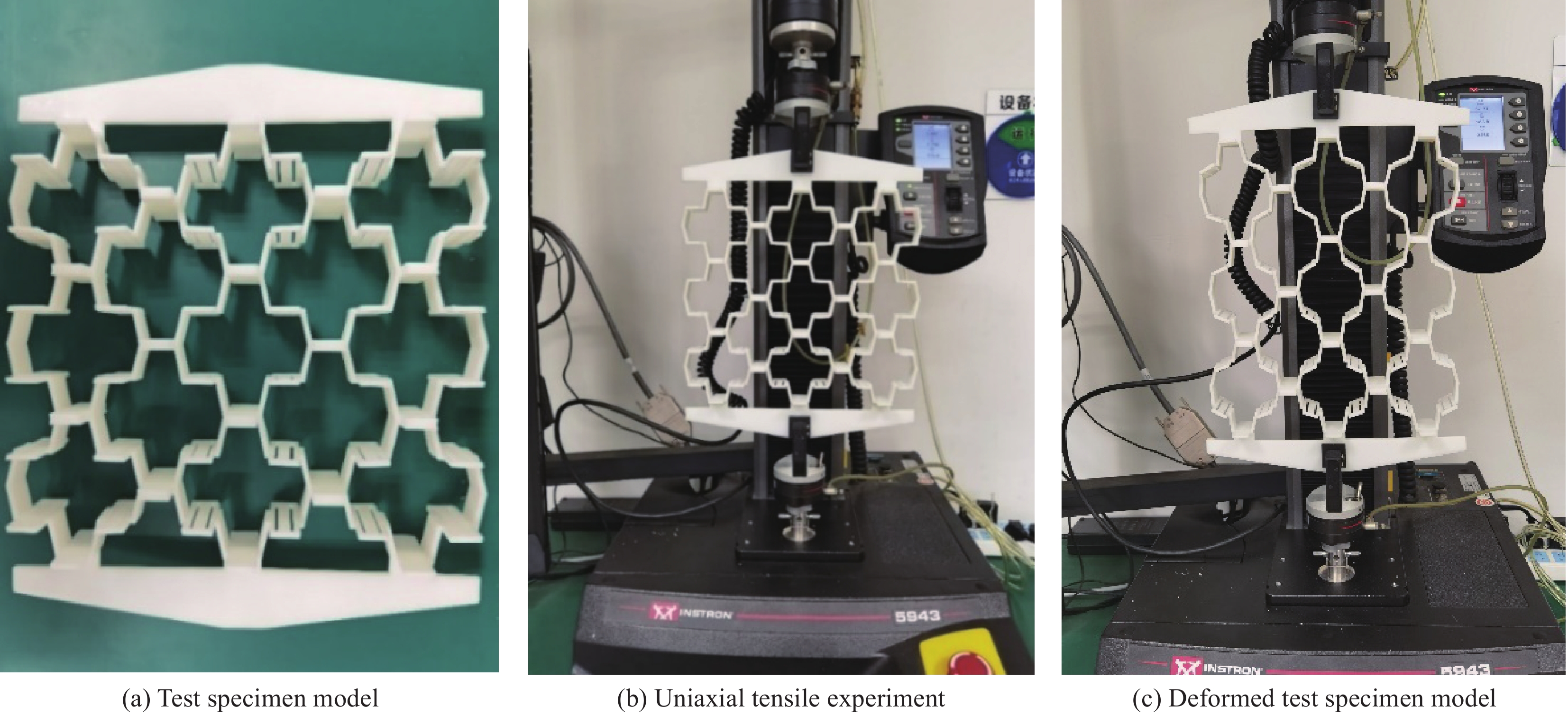
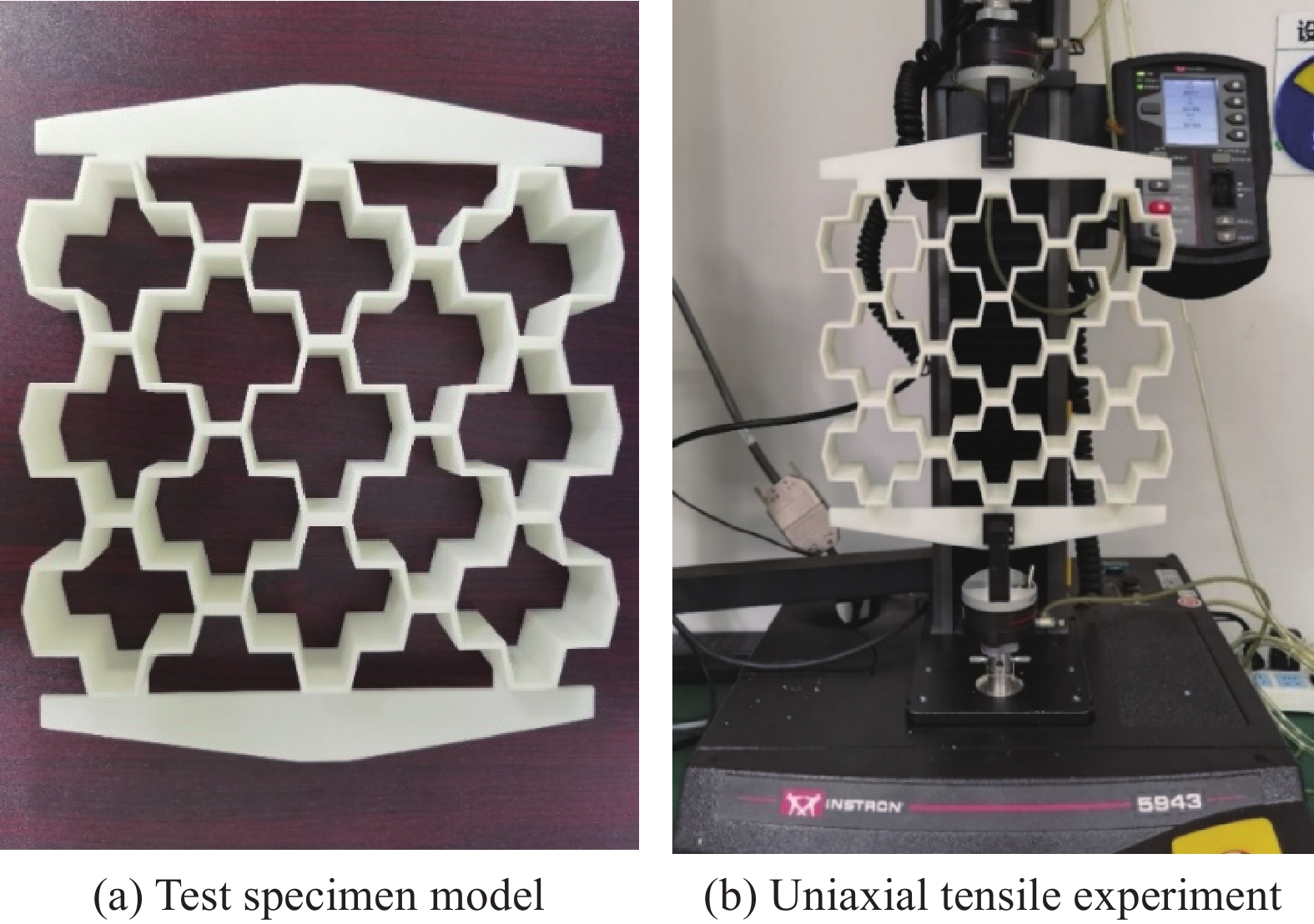
方法柔性铰可变形蜂窝结构由十字形蜂窝和内LET半铰(Half Inside Lamina Emergent Torsional Joint)组成。采用代表体元法，通过理论分析研究柔性铰可变形蜂窝结构的面内等效弹性模量。对柔性铰可变形蜂窝进行仿真分析，选择壳单元(S4R)建立蜂窝单元的有限元模型，分析等效弹性模量随柔性铰可变形蜂窝结构几何参数变化的规律，并对不同结构参数下等效模量的理论值和仿真值行了比较。采用3D打印方法制造了柔性铰可变形蜂窝实验件，在INSTRON 5943电子多功能实验机上进行单轴拉伸实验，验证理论模型和仿真模型的正确性。通过仿真和实验方法比较材料和当量尺寸相同的柔性铰可变形蜂窝和十字形蜂窝的面内刚度。
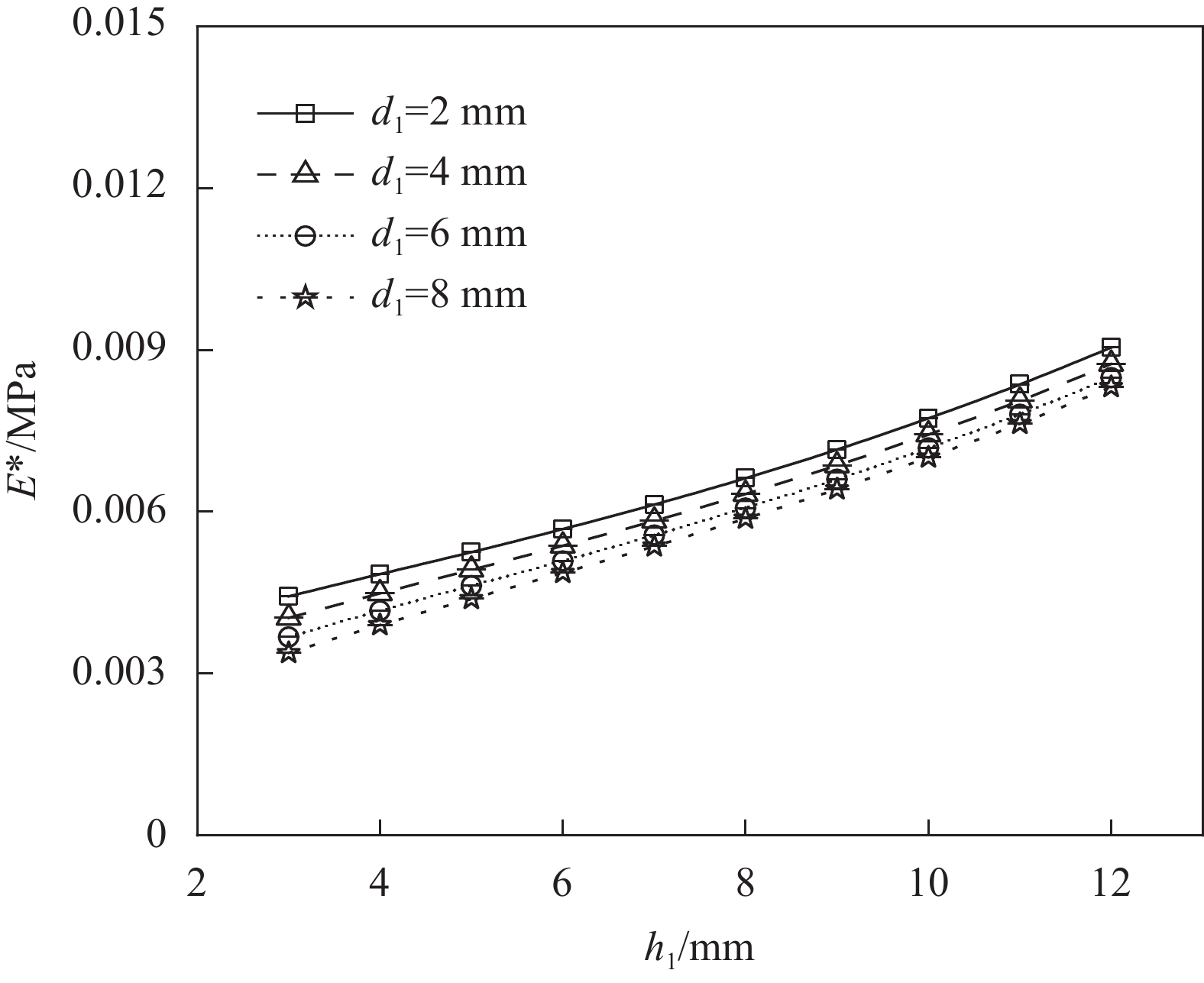
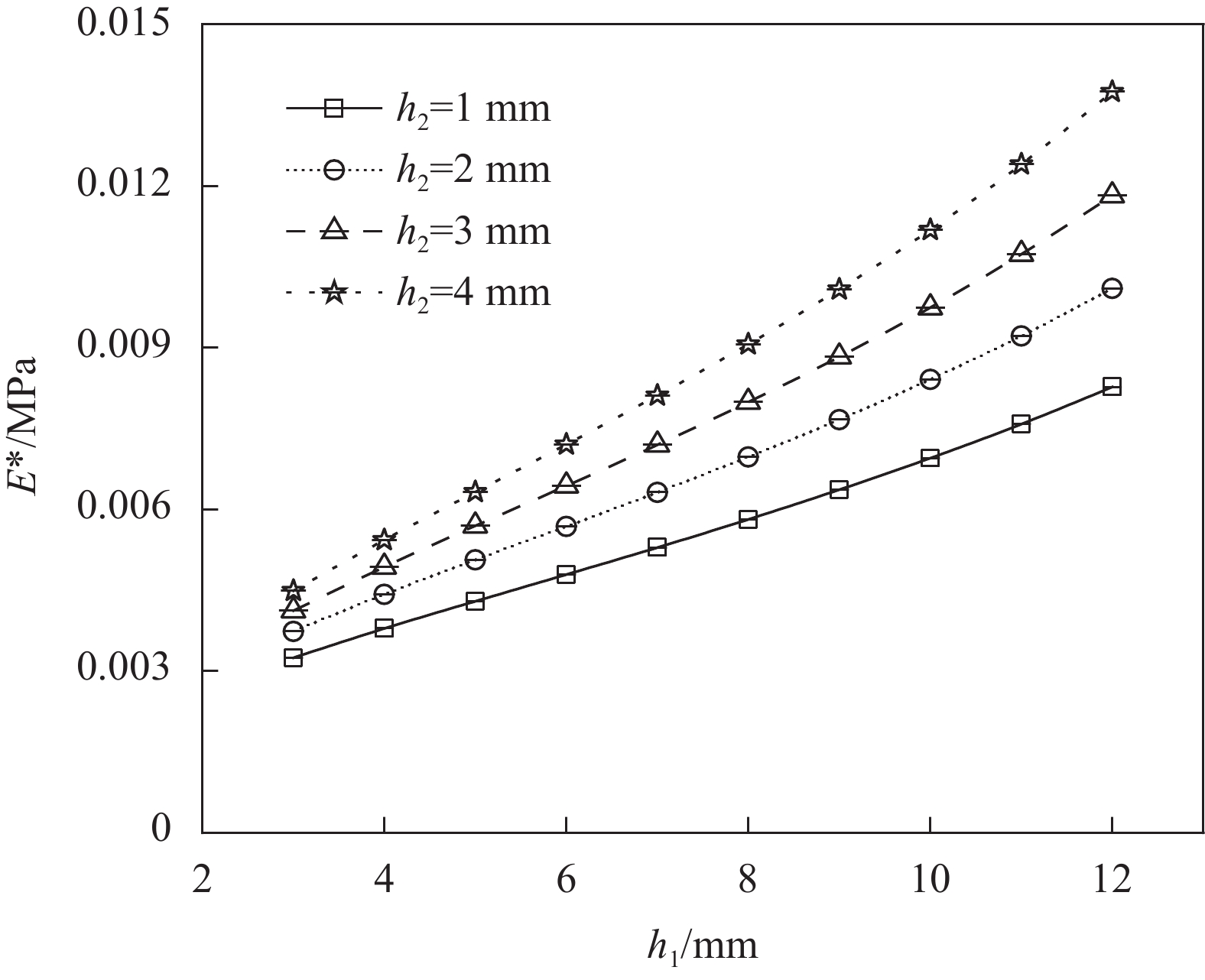
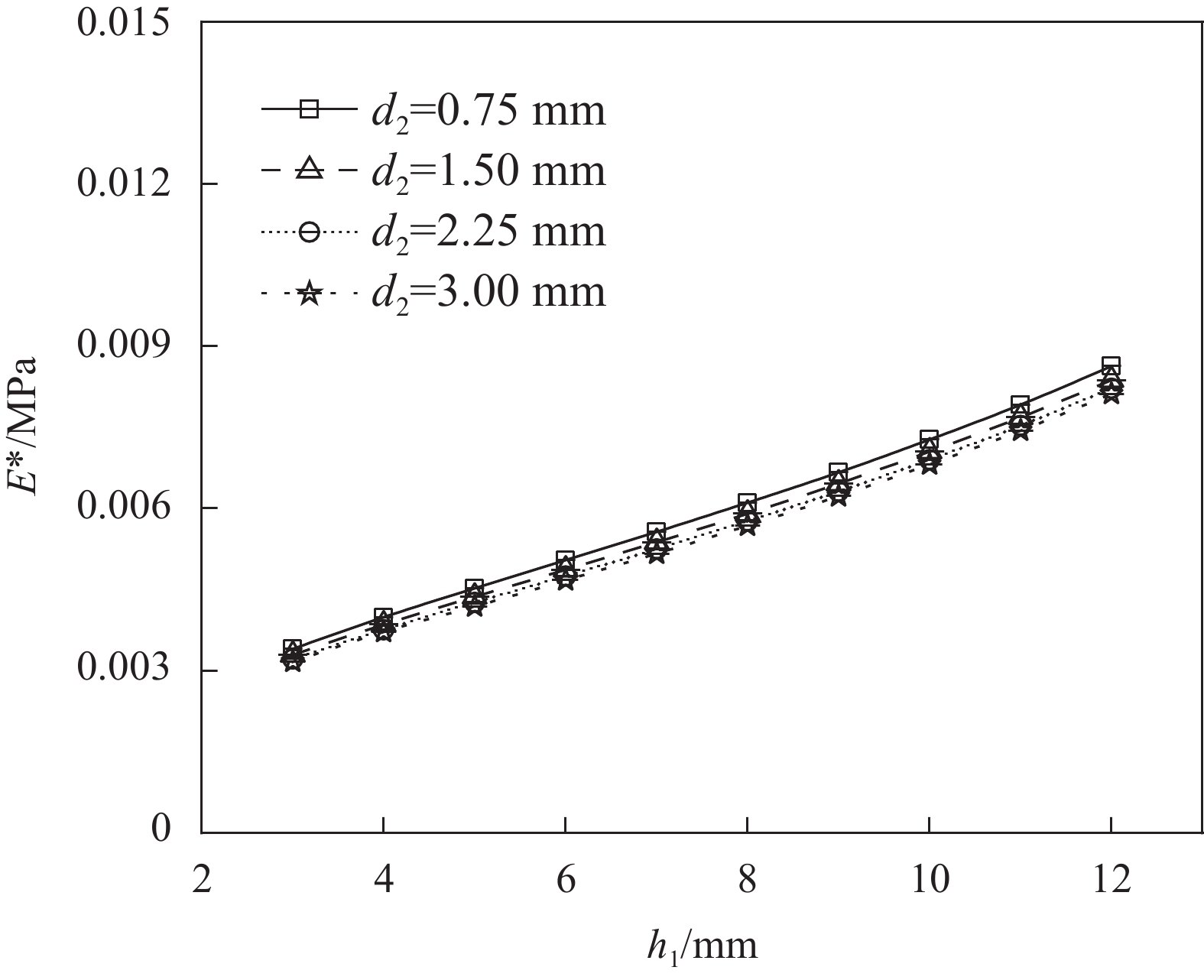
结果1、推导出了柔性铰可变形蜂窝结构面内单向拉伸等效弹性模量的理论表达式，并进行了仿真和实验验证，理论、仿真和实验结果吻合良好。2、等效模量随柔性铰可变形蜂窝结构几何参数变化的规律如下：(1)等效弹性模量随着值的增加而减小。随着的增大，斜壁的弯曲变形增大，从而使等效弹性模量减小；(2)等效弹性模量随着值的增加而增加。值的增加使斜壁的弯曲刚度以及内LET半铰的扭转刚度增大，则等效弹性模量增大；(3)等效弹性模量随着的增大而增大，这是因为值的增大会使内LET半铰的扭转刚度增加。同时，的参数变化对等效模量的影响与相比明显增大，这是因为内LET半铰减小局部刚度主要是源于扭转轴的扭转变形，参数的减小会显著降低扭转轴的扭转刚度，而参数的变化只对斜壁的弯曲刚度有一定的影响，对内LET半铰的扭转变形没有贡献；(4)等效弹性模量随着值的增加而增加，这主要是因为随着值的增加，内LET半铰的扭转刚度增加；(5)值的变化对等效弹性模量的影响很小，当变化时，内LET半铰的扭转变形和蜂窝单元斜壁的弯曲变形都不受其影响。因此，方向上的等效弹性模量主要受参数、和的影响，参数对等效弹性模量也有一定的影响，参数对几乎没有影响，在参数协调的情况下，对于一维变形，、、的值越小，的值越大，结构的变形效果越好。3、通过对柔性铰可变形蜂窝与十字形蜂窝的面内刚度进行比较，可以得出相比于十字形蜂窝，柔性铰可变形蜂窝的面内等效弹性模量降低了80%以上，面内变形能力有了很大提升。
结论柔性铰可变形蜂窝结构由十字形蜂窝和内LET半铰组成，将相邻蜂窝单元壁板的连接处替换为内LET半铰，由此降低可变形蜂窝结构的刚度，提高可变形蜂窝的面内变形能力，因此，将可变形蜂窝结构与柔性铰链巧妙结合是一种提升蜂窝结构面内变形能力的有效方式。
-
变体飞机可以自适应地改变形状以适应不断变化的飞行条件，使飞机具有多任务适应性。蜂窝结构具有重量轻、面内变形好、面外承载能力强等优良特性，被广泛应用于变体飞机柔性蒙皮结构。
为了提升柔性蒙皮蜂窝结构的面内变形能力，本文提出了一种柔性铰可变形蜂窝结构。该结构由十字形蜂窝和内LET半铰(Half Inside Lamina Emergent Torsional Joint)组成，通过降低结构面内刚度提升变形能力，具有重量轻、面内模量低的特点。通过理论分析研究了柔性铰可变形蜂窝结构的面内等效弹性模量，并进行了仿真和实验验证，分析了几何参数对结构等效弹性模量的影响，最后通过仿真和实验比较了柔性铰可变形蜂窝与十字形蜂窝的面内刚度。结果表明：柔性铰可变形蜂窝的等效弹性模量与十字形蜂窝相比降低了80%以上，具有更优秀的面内变形能力，将可变形蜂窝结构与柔性铰链巧妙结合是一种提升蜂窝结构面内变形能力的有效方式。
柔性铰可变形蜂窝几何模型
计量
- 文章访问数: 376
- HTML全文浏览量: 157
- PDF下载量: 33
