Study on plastic yield strength of Gyroid lattice structures based on functional principleson
-
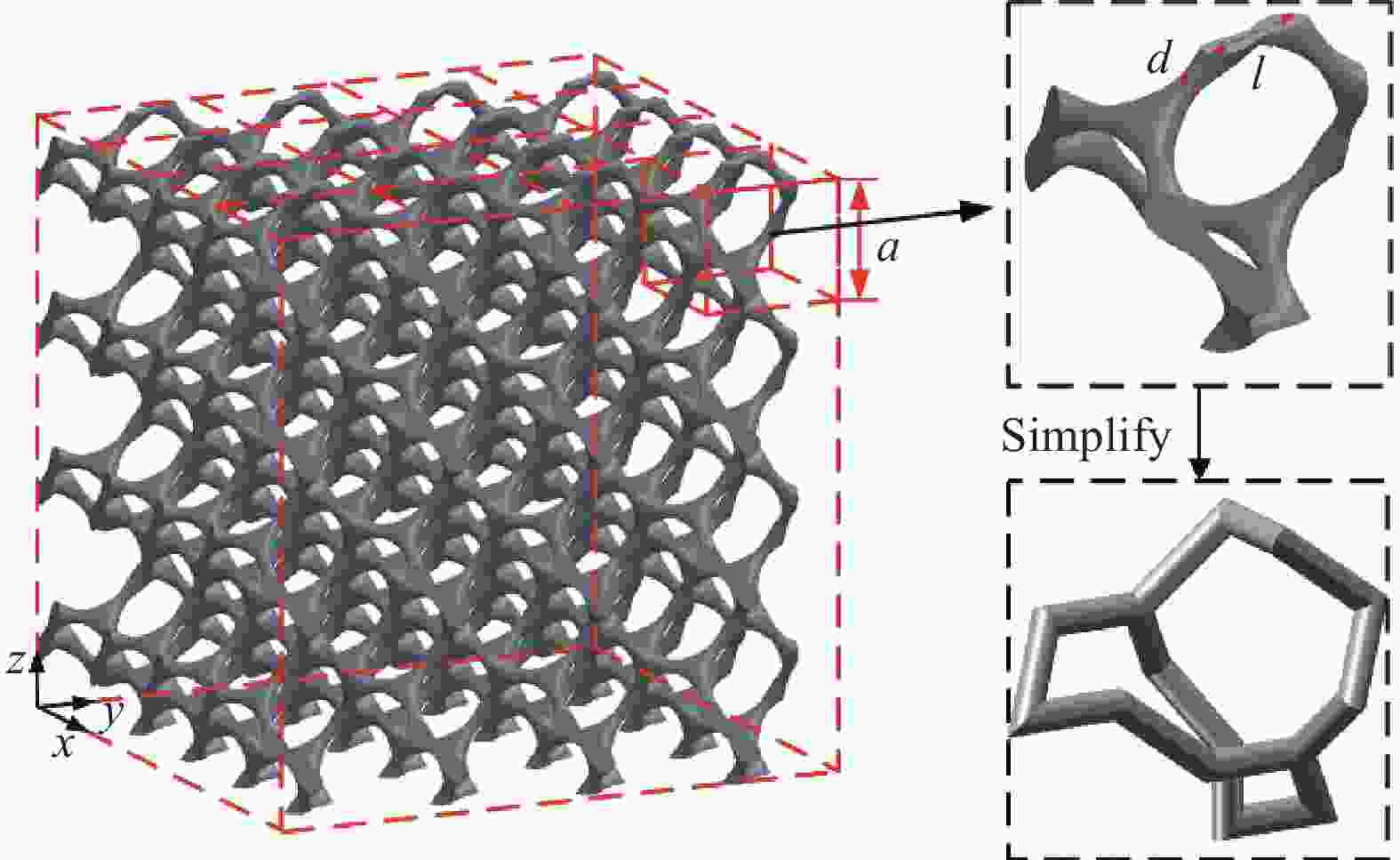
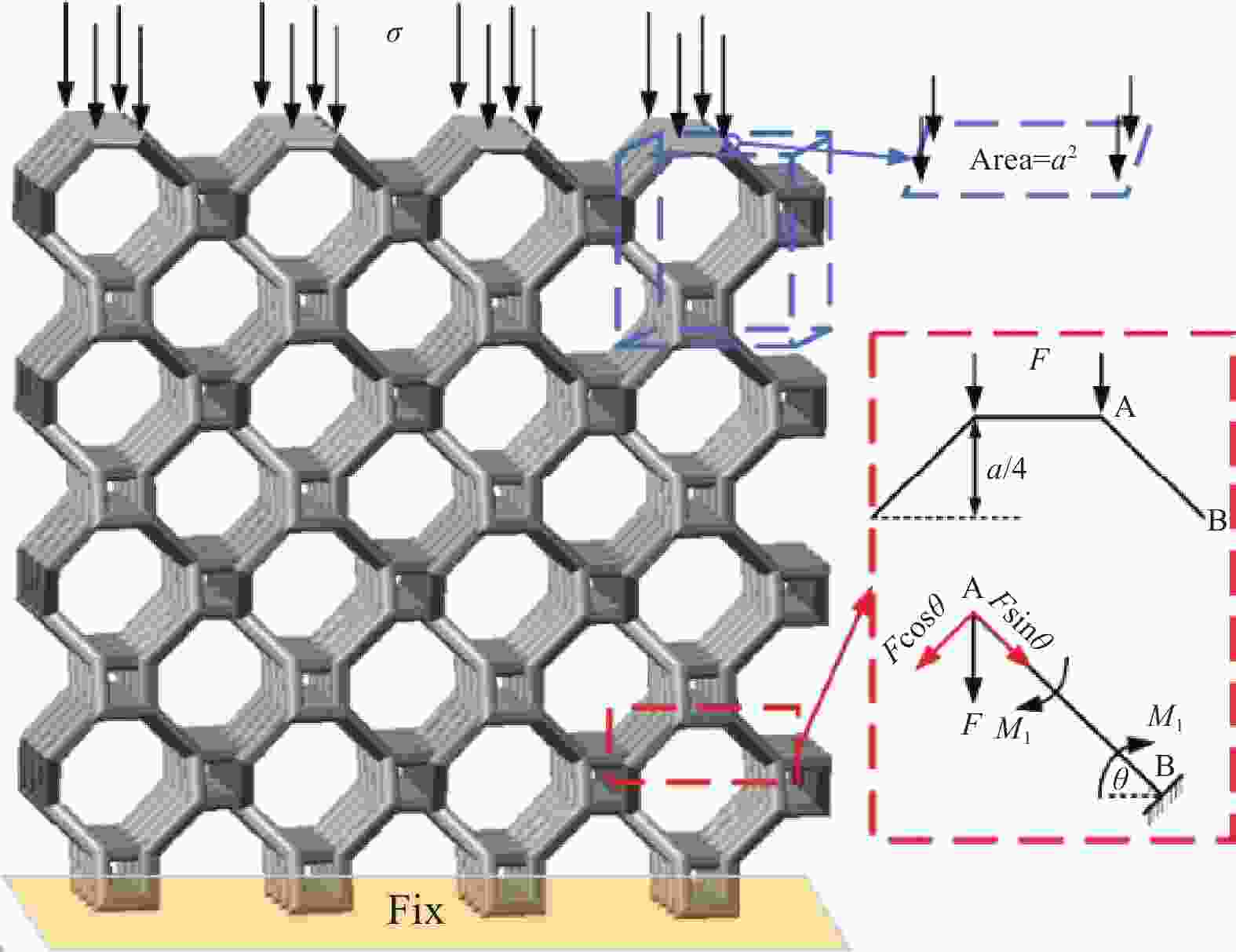
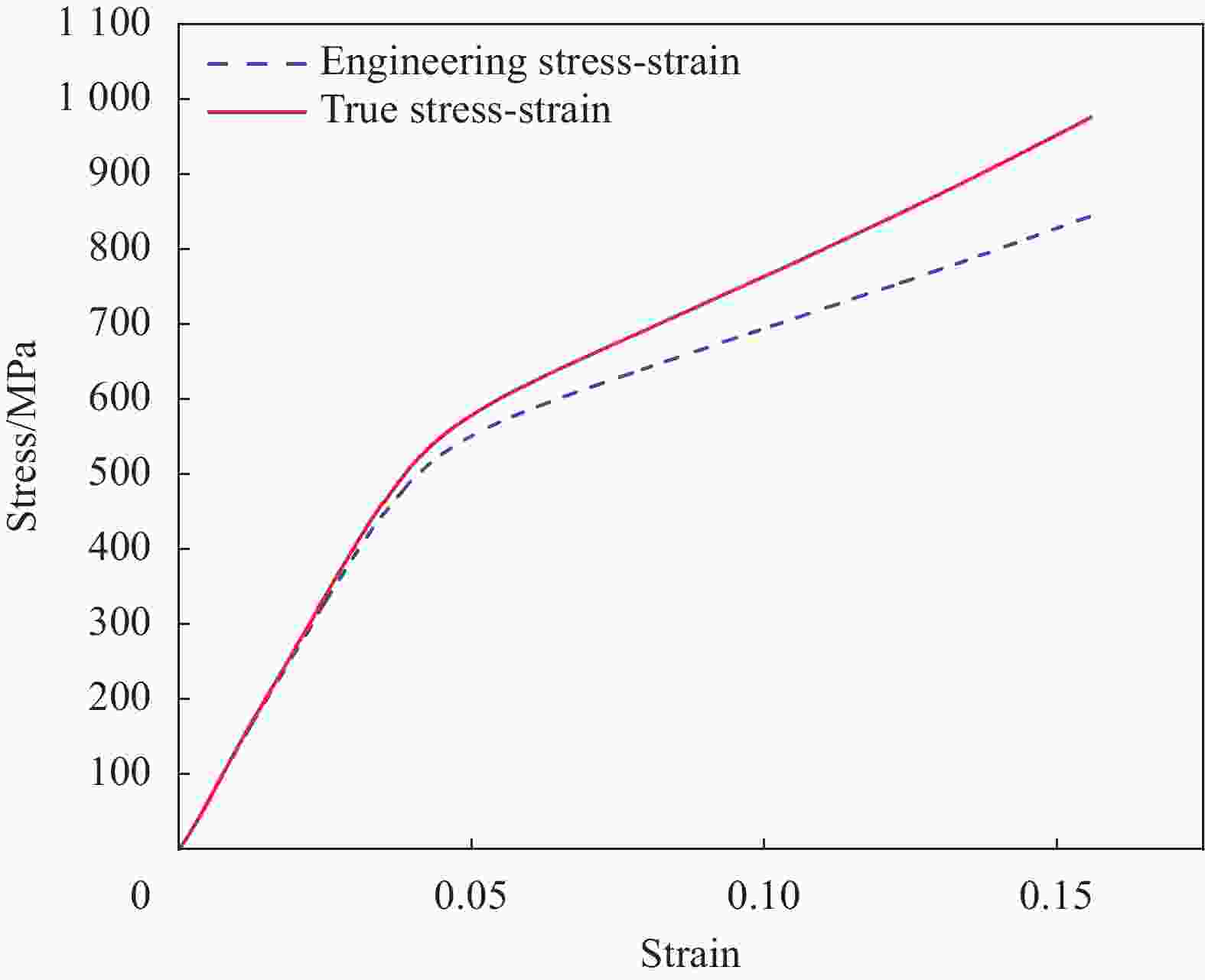
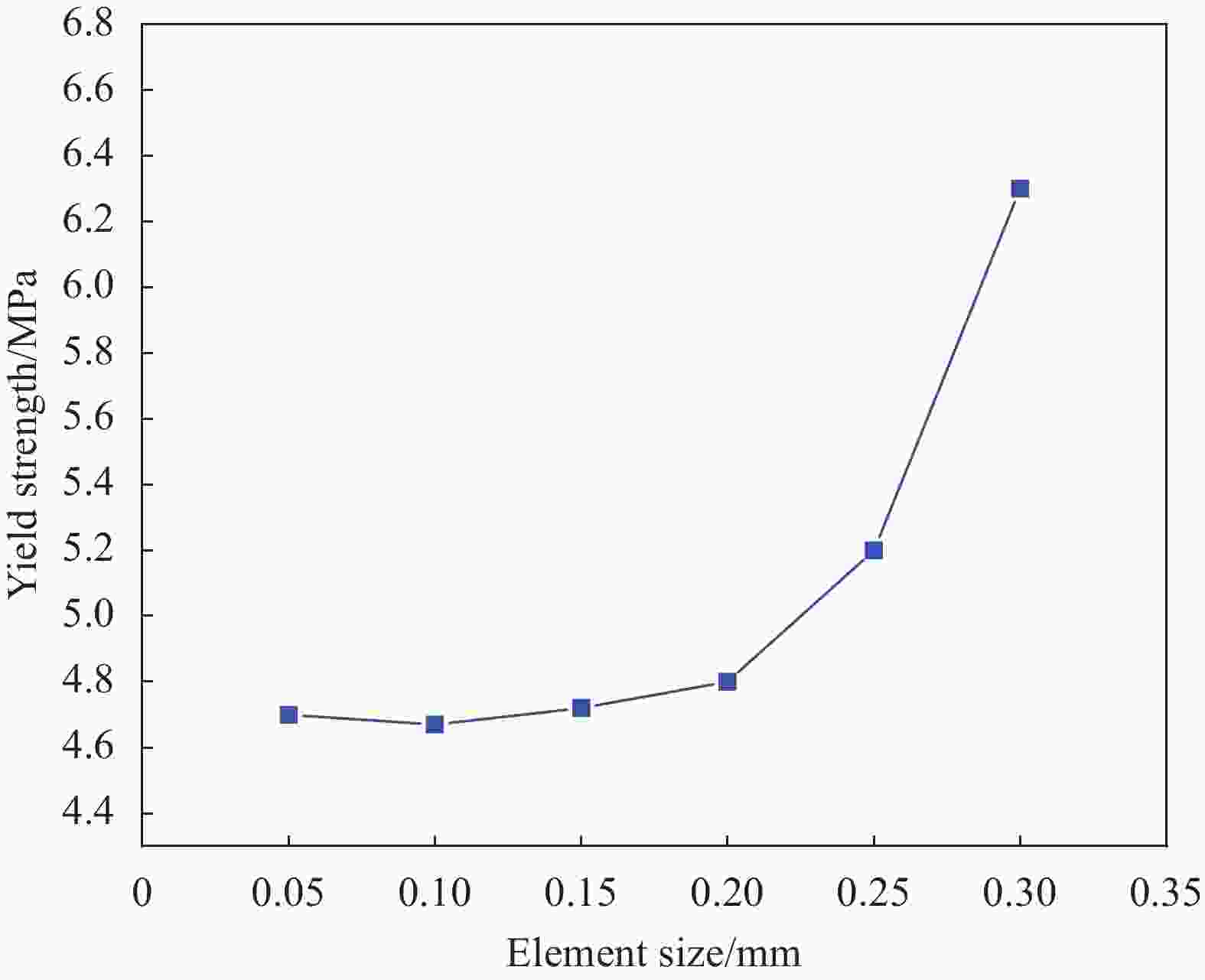
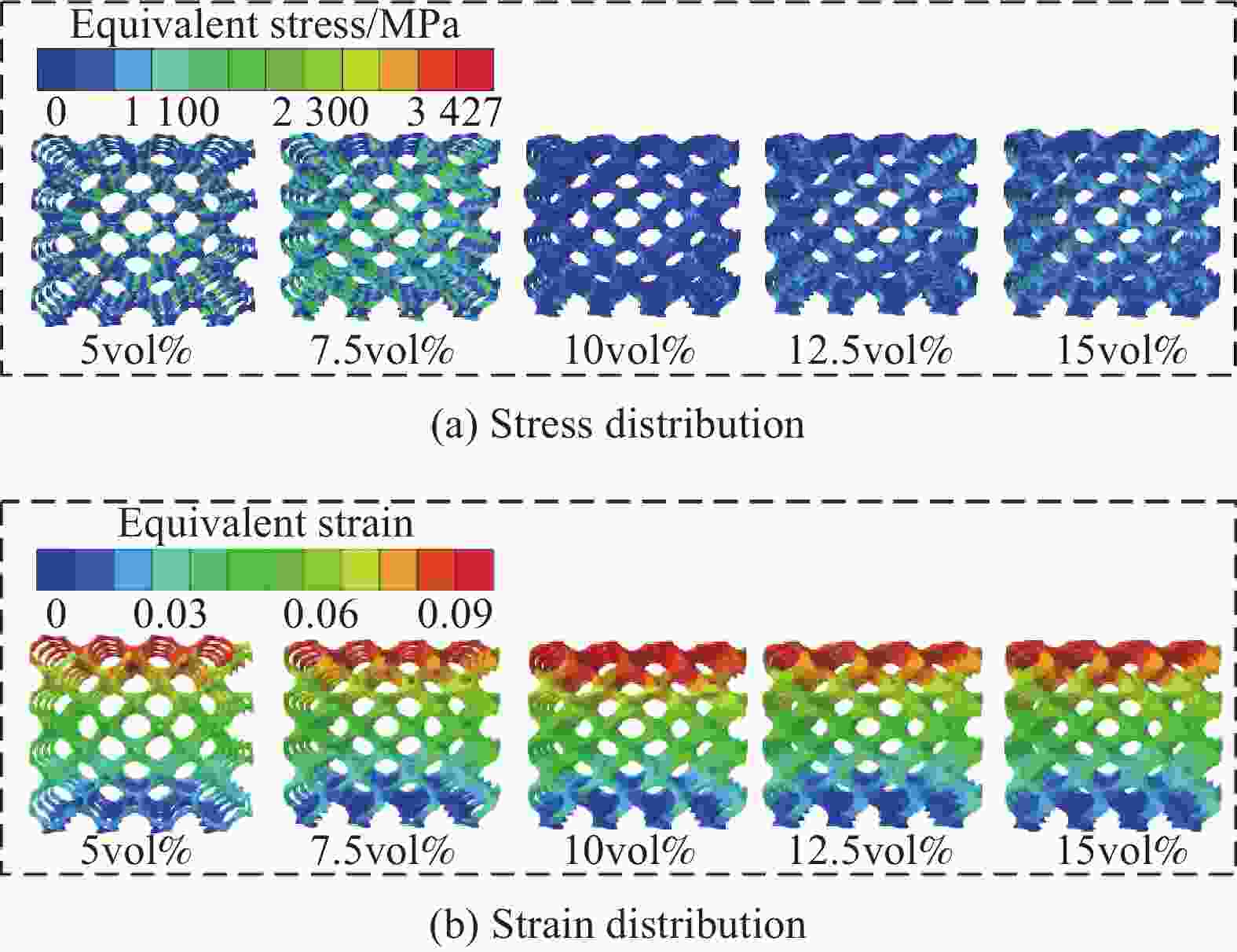
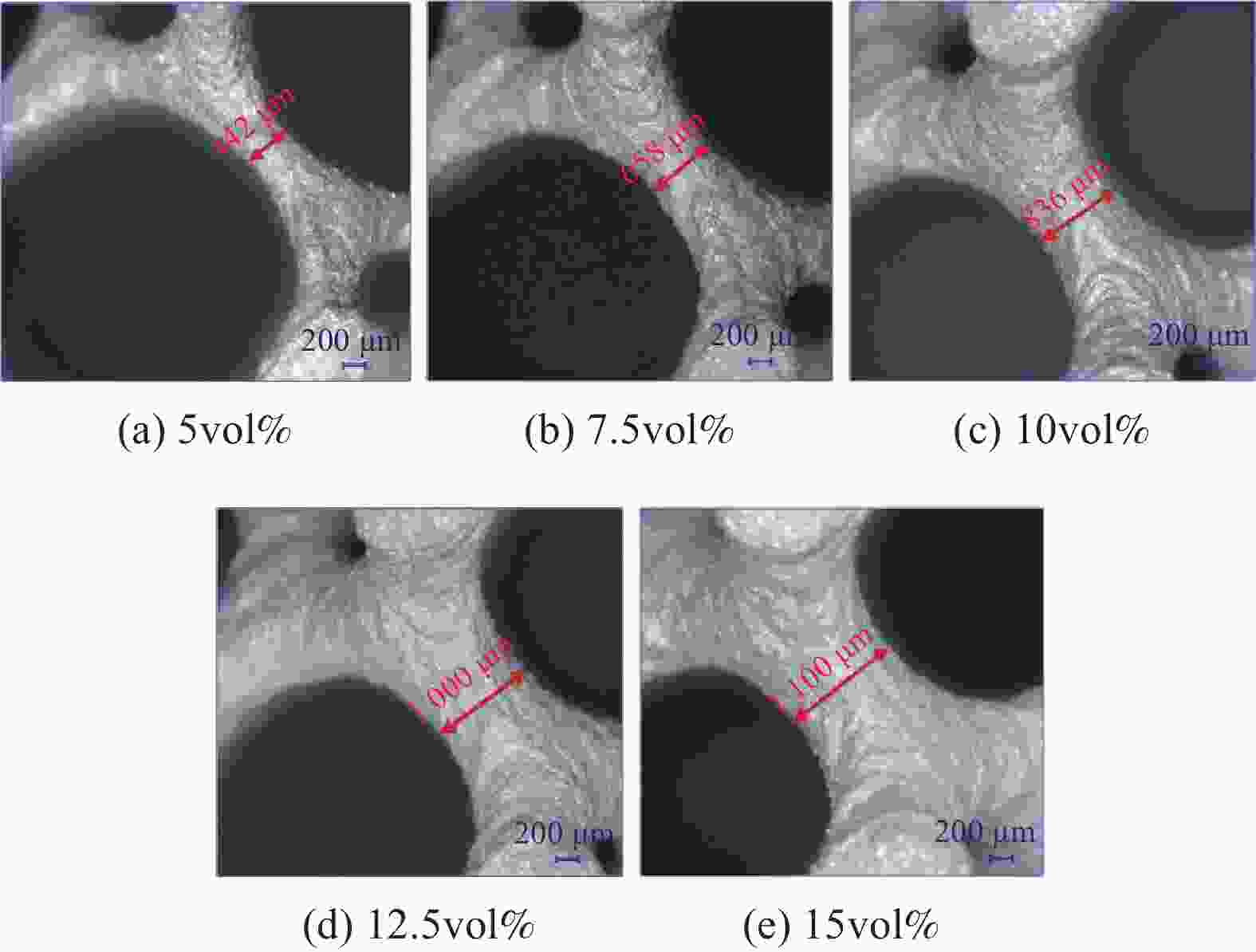
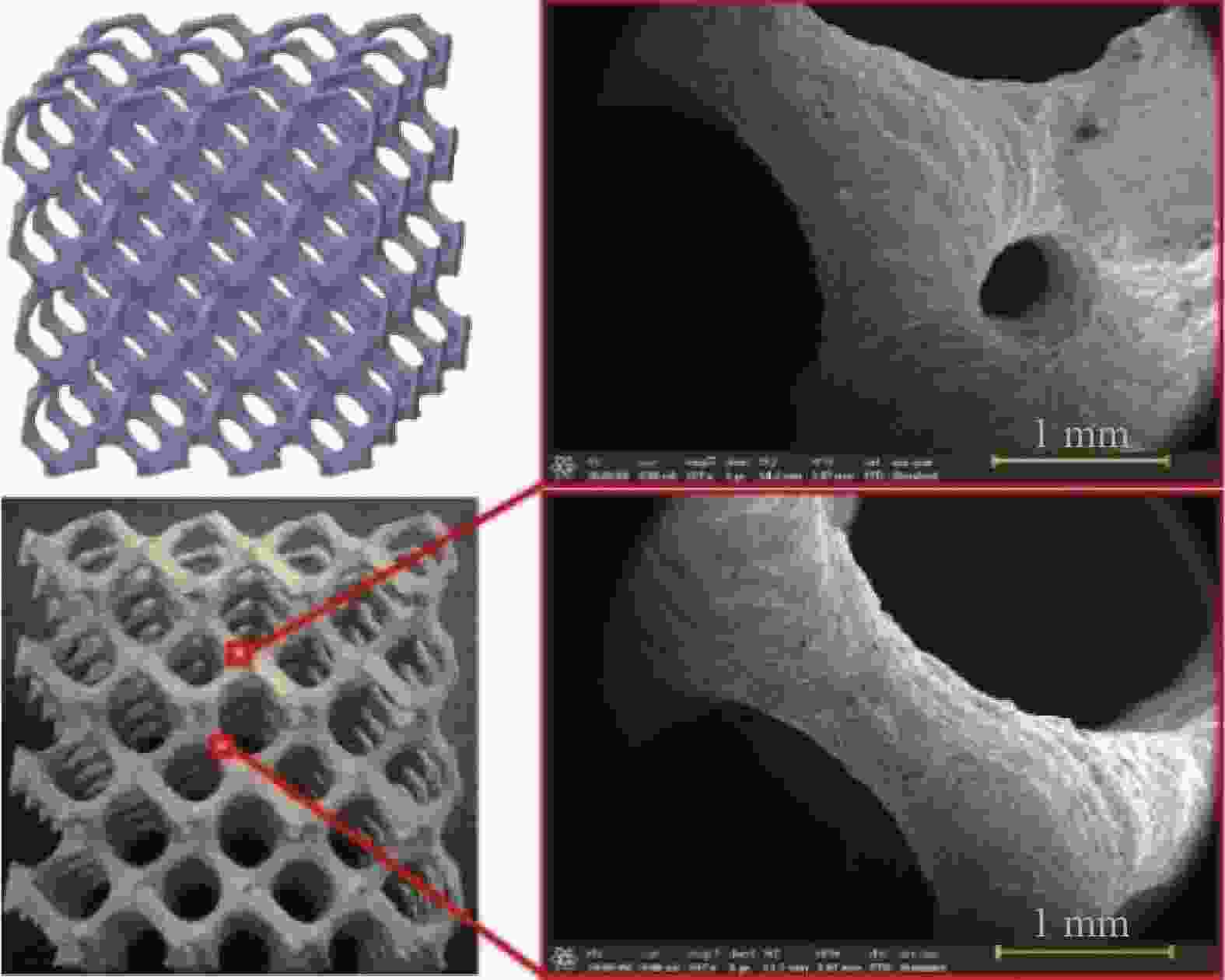
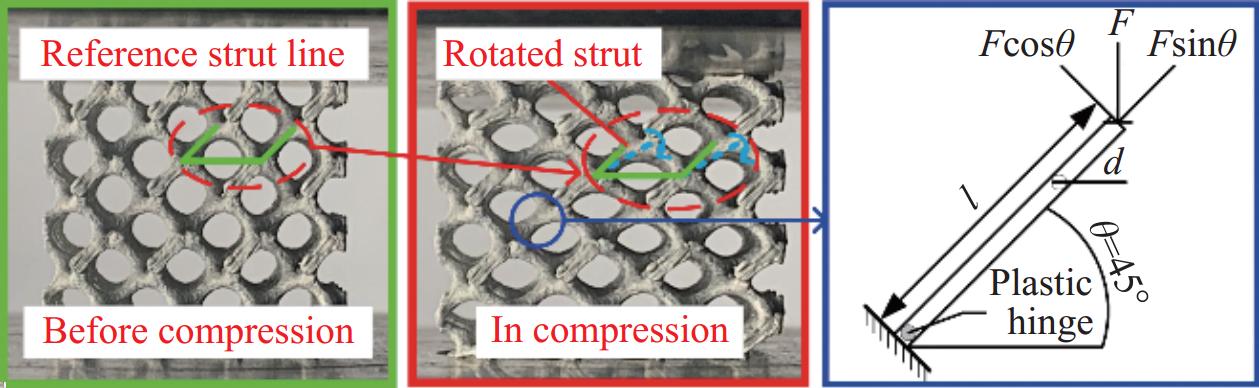
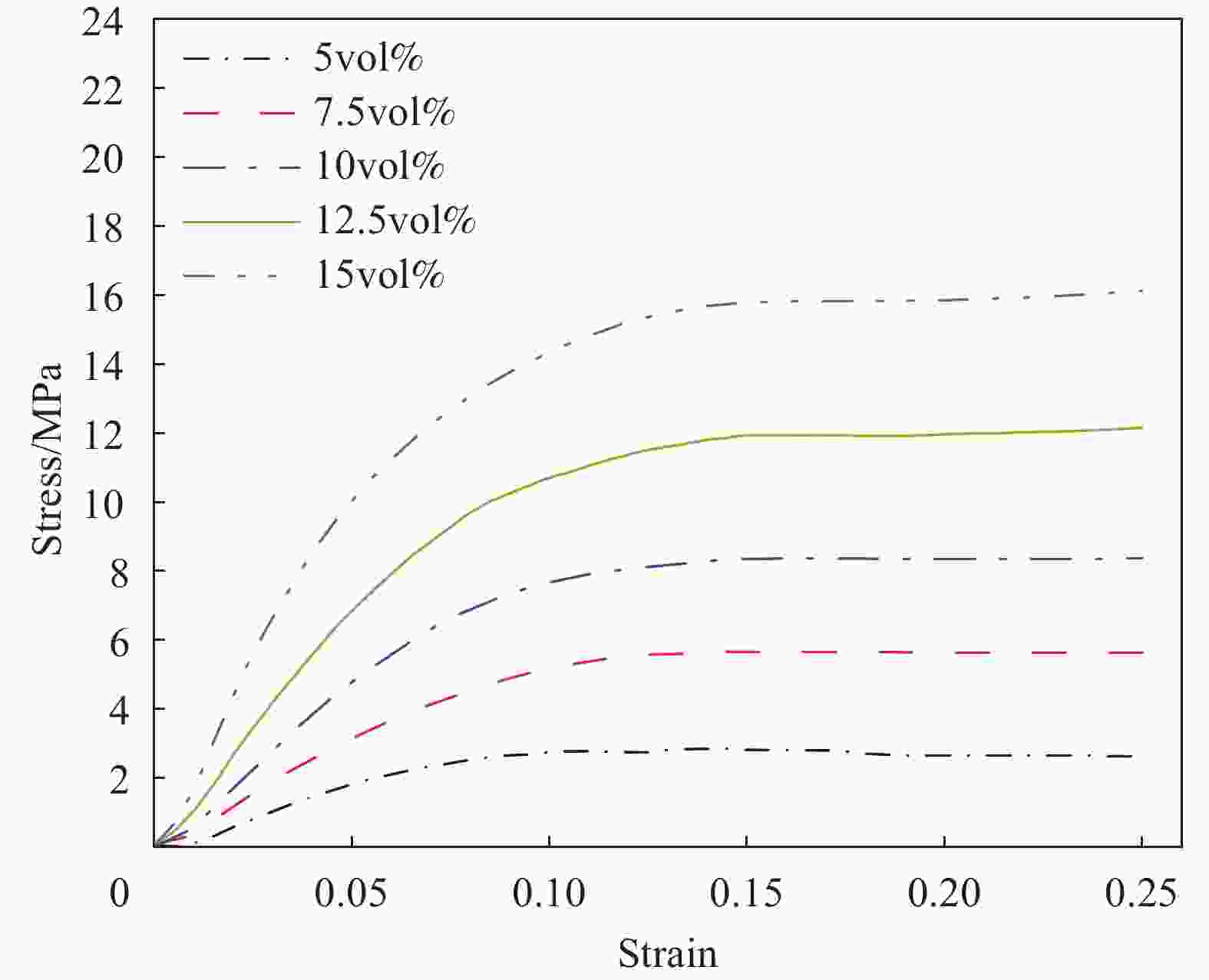
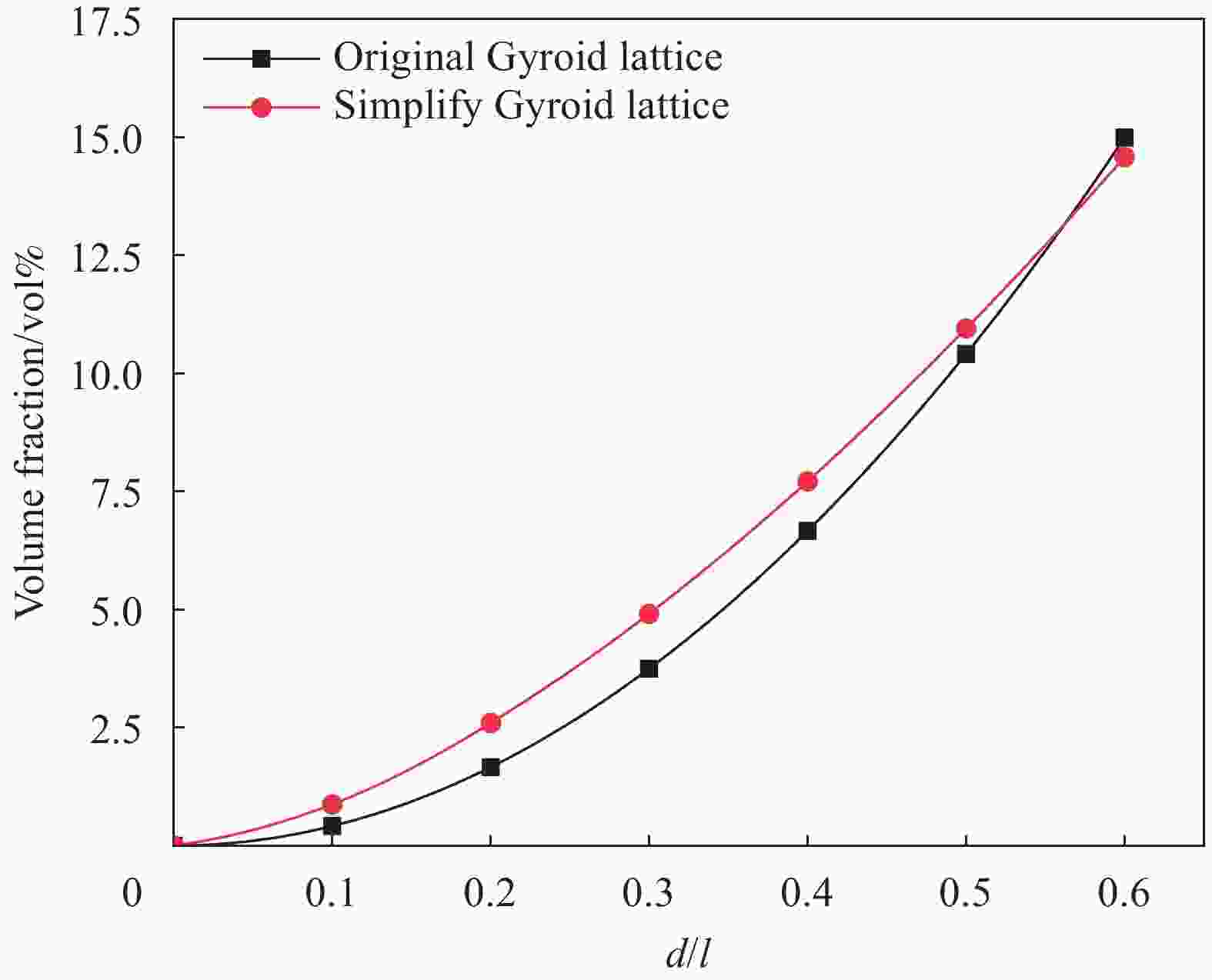
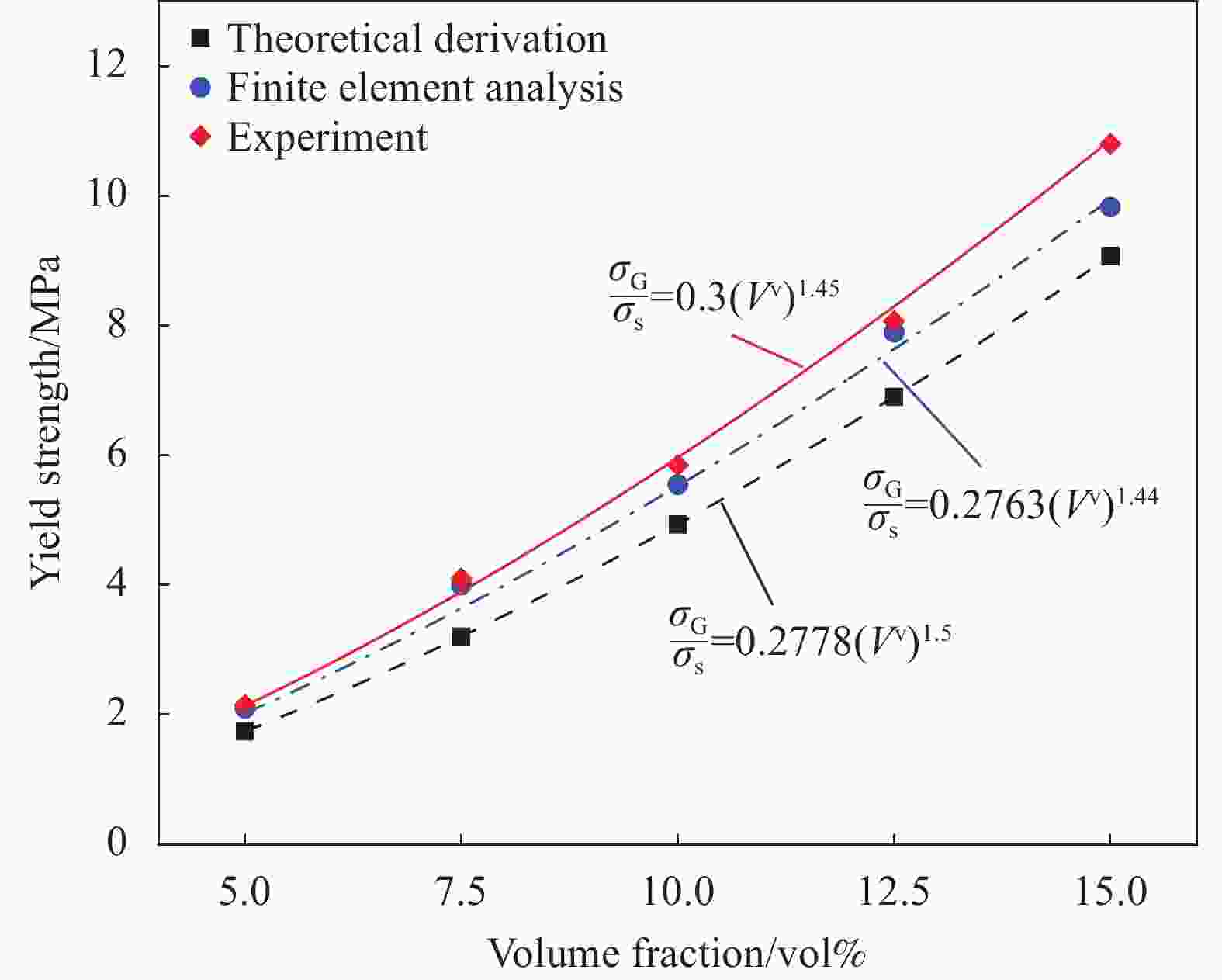
摘要: 点阵结构与密实结构存在的力学性能差异之一表现在塑性屈服响应上,因此,研究其屈服行为可为点阵结构的设计和应用提供重要的理论依据。首先,对Gyroid点阵结构进行简化,并基于变形体功能原理建立其力学模型,得到Gyroid点阵结构塑性屈服强度与体积分数之间的映射关系;然后,基于有限元分析软件Abaqus对Gyroid点阵结构准静态压缩过程开展仿真实验,初步验证理论模型的准确性;最后,通过选择性激光熔化(SLM)制备不同体积分数316L不锈钢Gyroid点阵结构,进行单轴压缩实验,分析其变形机制与力学性能。结果表明:理论推导、有限元仿真结果与实验结果相比,误差在25%以内,且根据3种方法结果拟合得到的Gibson-Ashby模型系数具有较好的一致性,表明本文基于理论推导建立的Gyroid点阵结构塑性屈服强度预测模型的有效性。理论模型的构建方法可以转化到其他复杂类型点阵结构中,为快速核算点阵结构力学性能,并将其应用在工程装备中提供理论依据。Abstract: One of the differences in mechanical properties between lattice structures and dense structures lies in the plastic yield response. Therefore, studying their yield behavior can provide important theoretical basis for the design and application of lattice structures. Firstly, The Gyroid lattice structure was simplified and its mechanical model was established based on the principle of deformable body function, obtaining the mapping relationship between the plastic yield strength and volume fraction of the Gyroid lattice structure. Then, based on the finite element analysis software Abaqus, simulation experiments were conducted on the quasi-static compression process of Gyroid lattice structures to preliminarily verify the accuracy of the theoretical model. Finally, different volume fractions of 316L stainless steel Gyroid lattice structures were prepared by selective laser melting (SLM), and uniaxial compression experiments were conducted to analyze their deformation mechanism and mechanical properties. The results show that the error between theoretical derivation, finite element simulation results and experimental results is within 25%, and the coefficients of the Gibson-Ashby model fitted based on the results of the three methods have good consistency, indicating the effectiveness of the Gyroid lattice structure plastic yield strength prediction model established based on theoretical derivation. The construction method of theoretical models can be transformed into other complex types of lattice structures, providing a theoretical basis for quickly calculating the mechanical properties of lattice structures and applying them in engineering equipment.
-
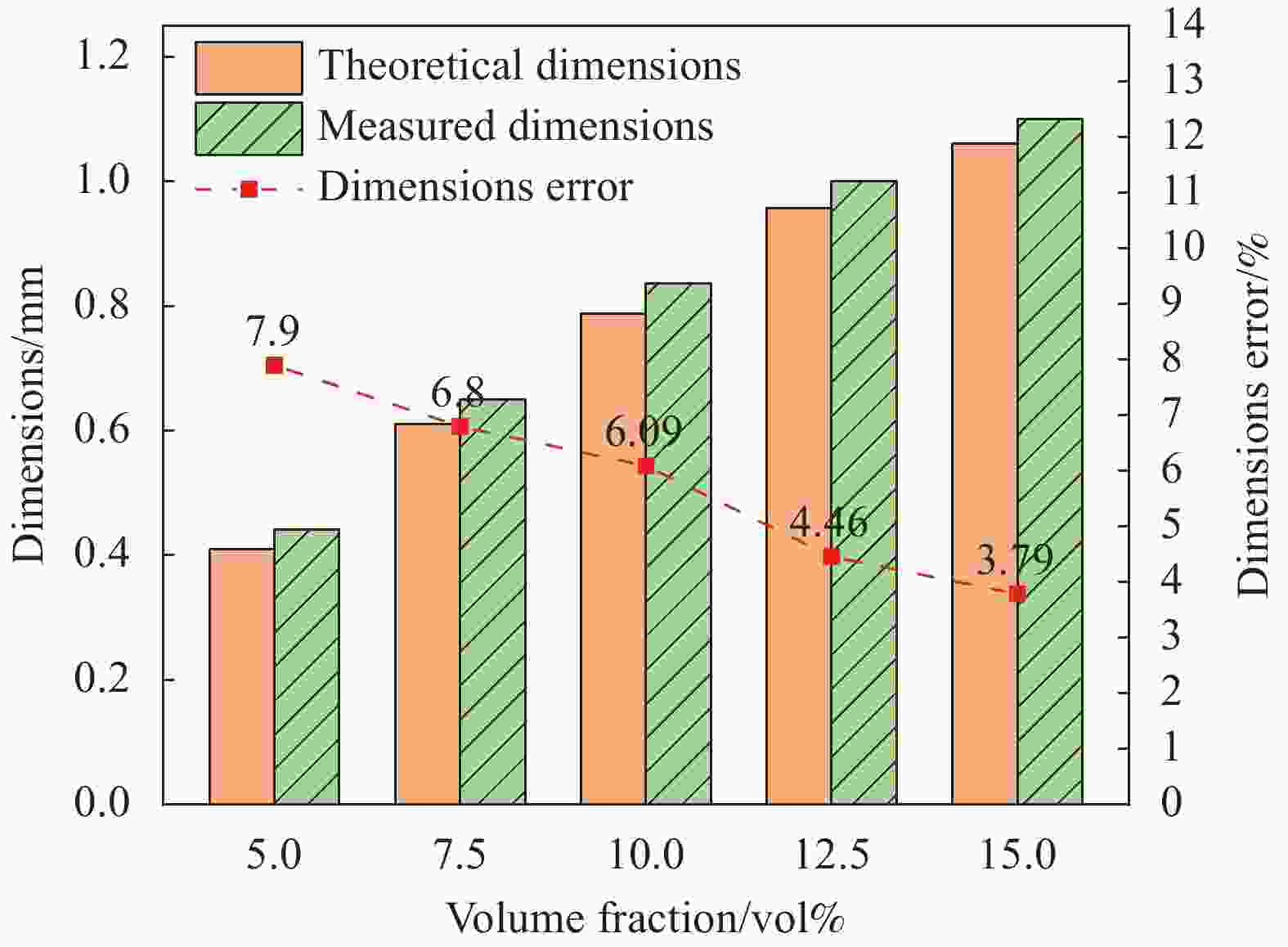
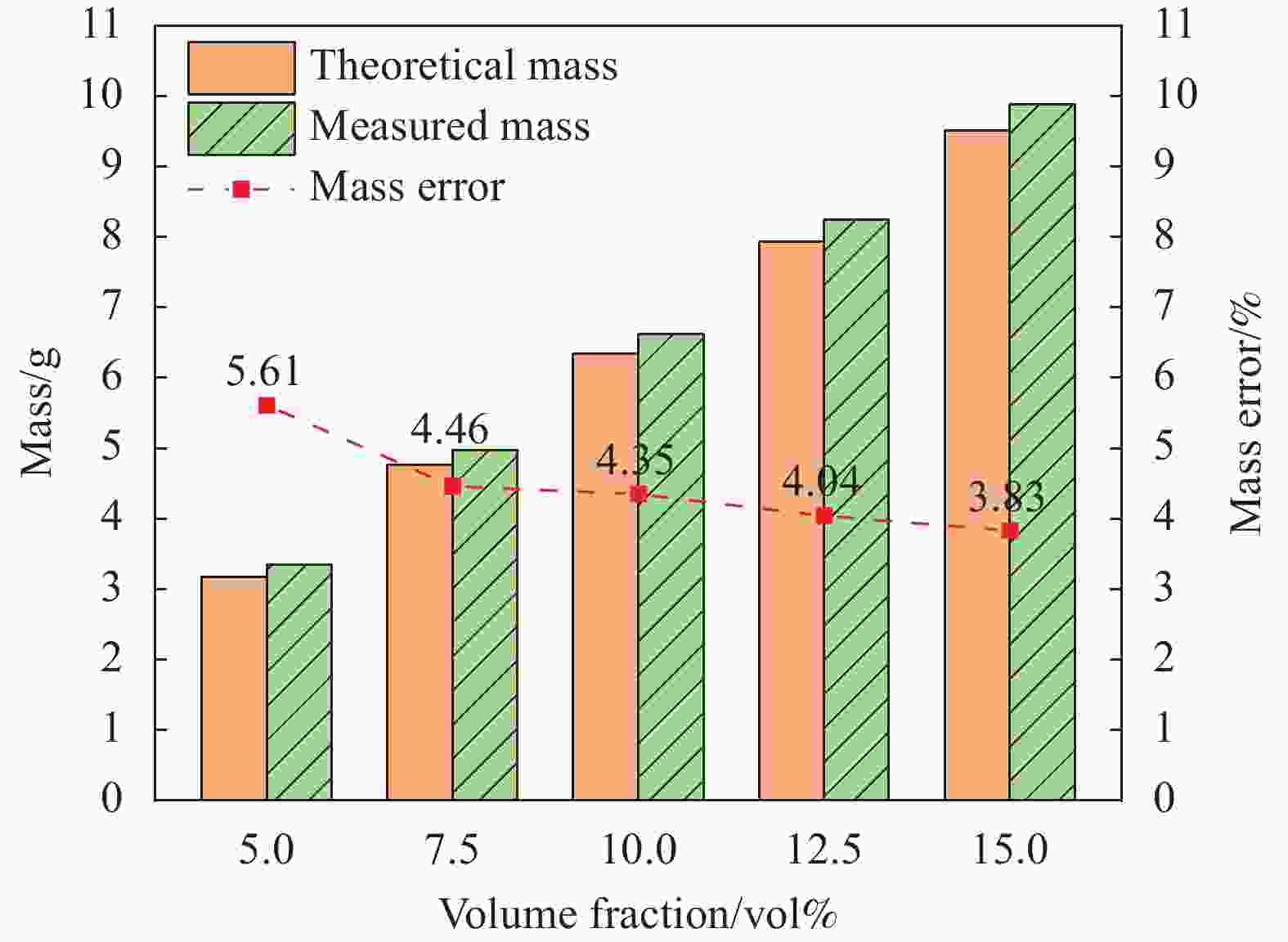
表 1 Gyroid点阵结构理论推导、有限元和实验结果对比
Table 1. Comparison of theoretical derivation, finite element and experimental results of Gyroid lattice structure
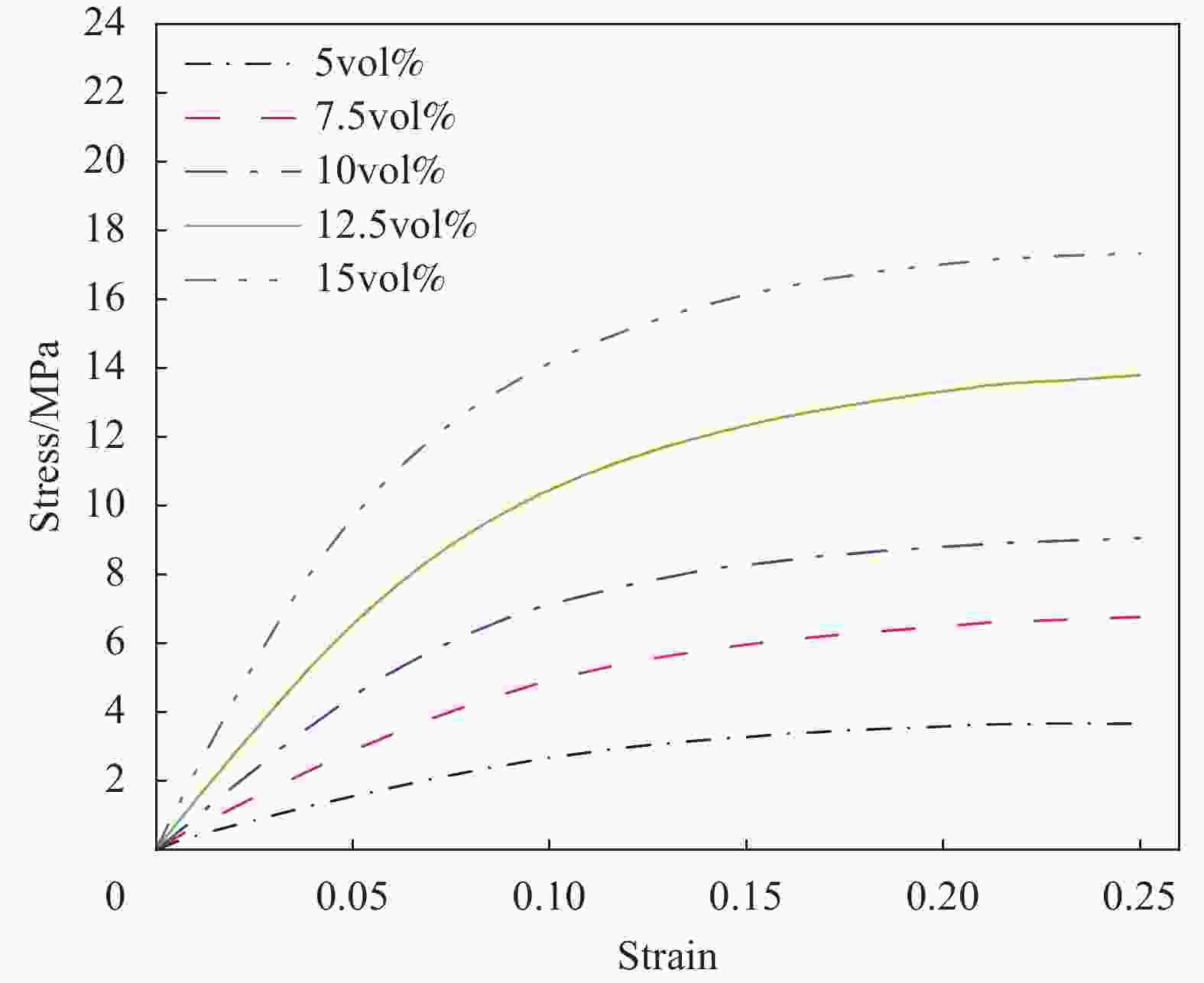
Volume fraction/vol% Plastic yield strength/MPa Theoretical derivation Finite element analysis Experimental 5 1.48 1.78 1.83 7.5 2.72 3.40 3.48 10 4.19 4.72 4.97 12.5 5.85 6.71 6.86 15 7.69 8.36 9.18 -
[1] 程树良, 吴灵杰, 孙帅, 等. X型点阵夹芯结构受局部冲击时动态力学性能试验与数值模拟[J]. 复合材料学报, 2022, 39(7): 3641-3651.CHENG Shuliang, WU Lingjie, SUN Shuai, et al. Experiment and numerical simulation of dynamic mechanical properties of X-lattice sandwich structure under local impact[J]. Acta Materiae Compositae Sinica, 2022, 39(7): 3641-3651(in Chinese). [2] WU F H, LIAN H, PEI G B, et al. Design and optimization of variable-density lattice structure based on load paths[J]. Facta Universitatis-Series Mechanical Engineering, 2023, 21(2): 273-292. doi: 10.22190/FUME220108017W [3] SCHAEDLER T A, JACOBSEN A J, CARTER W B. Toward lighter, stiffer materials[J]. Science, 2013, 341(6151): 1181-1182. doi: 10.1126/science.1243996 [4] 梁栋, 陈伟, 胡勇, 等. 点阵单元类型和排布角度对传热特性的影响[J]. 航空动力学报, 2022, 37(1): 26-35.LIANG Dong, CHEN Wei, HU Yong, et al. Influences of lattice unit type and arrangement orientation on heat transfer characteristics[J]. Journal of Aerospace Power, 2022, 37(1): 26-35(in Chinese). [5] 李永欣, 郭长春, 李凯伦, 等. SLM制备的小长径比四棱锥点阵填充轻量化研究[J]. 机械工程学报, 2021, 57(24): 132-138. doi: 10.3901/JME.2021.24.132LI Yongxin, GUO Changchun, LI Kailun, et al. Filling lightweight research on pyramid lattice with small slenderness ratio fabricated by SLM[J]. Journal of Mechanical Engineering, 2021, 57(24): 132-138(in Chinese). doi: 10.3901/JME.2021.24.132 [6] CHOUHAN G, BALA MURALI G. Designs, advancements, and applications of three-dimensional printed Gyroid structures: A review[J]. Proceedings of the Institution of Mechanical Engineers, Part E: Journal of Process Mechanical Engineering, 2024, 238(2): 965-987. [7] FENG J W, FU J Z, YAO X H, et al. Triply periodic minimal surface (TPMS) porous structures: From multi-scale design, precise additive manufacturing to multidisciplinary applications[J]. International Journal of Extreme Manufacturing, 2022, 4(2): 022001. doi: 10.1088/2631-7990/ac5be6 [8] Al-KETAN O, ROWSHAN R, Al-RUB R K A. Topology-mechanical property relationship of 3D printed strut, skeletal, and sheet based periodic metallic cellular materials[J]. Additive Manufacturing, 2018, 19: 167-183. doi: 10.1016/j.addma.2017.12.006 [9] DEVALK T, LAKES R. Poisson's ratio and modulus of Gyroid lattices[J]. Physica Status Solidi B-Basic Solid State Physics, 2021, 258(12): 2100081. doi: 10.1002/pssb.202100081 [10] 张明康. 隐式曲面梯度多孔结构优化设计及激光选区熔化成形力学性能研究[D]. 广州: 华南理工大学, 2020.ZHANG Mingkang. Optimal design and mechanical properties of implicit surface gradient porous structure manufactured by selective laser melting[D]. Guangzhou: South China University of Technology, 2020(in Chinese). [11] JONES A, LEARY M, BATEMAN S, et al. Parametric design and evaluation of TPMS-like cellular solids[J]. Materials & Design, 2022, 221: 110908. [12] LU Y T, ZHAO W Y, CUI Z T, et al. The anisotropic elastic behavior of the widely-used triply-periodic minimal surface based scaffolds[J]. Journal of the Mechanical Behavior of Biomedical Materials, 2019, 99: 56-65. doi: 10.1016/j.jmbbm.2019.07.012 [13] KHADERI S N, DESHPANDE V S, FLECK N A. The stiffness and strength of the Gyroid lattice[J]. International Journal of Solids and Structures, 2014, 51(23-24): 3866-3877. doi: 10.1016/j.ijsolstr.2014.06.024 [14] MASKERY I, STURM L, AREMU A O, et al. Insights into the mechanical properties of several triply periodic minimal surface lattice structures made by polymer additive manufacturing[J]. Polymer, 2018, 152: 62-71. doi: 10.1016/j.polymer.2017.11.049 [15] XING P, HUANG Q Y, ZHANG G X, et al. Compensating the anisotropic mechanical properties of electron beam melting-based Gyroid scaffolds using structural design[J]. International Journal of Mechanical Sciences, 2022, 226: 107442. doi: 10.1016/j.ijmecsci.2022.107442 [16] KELLY C N, FRANCOVICH J, JULMI S, et al. Fatigue behavior of as-built selective laser melted titanium scaffolds with sheet-based Gyroid microarchitecture for bone tissue engineering[J]. Acta Biomaterialia, 2019, 94: 610-626. doi: 10.1016/j.actbio.2019.05.046 [17] YANG L, YAN C Z, CAO W C, et al. Compression-compression fatigue behaviour of Gyroid-type triply periodic minimal surface porous structures fabricated by selective laser melting[J]. Acta Materialia, 2019, 181: 49-66. doi: 10.1016/j.actamat.2019.09.042 [18] VIET N V, ZAKI W. Free vibration and buckling characteristics of functionally graded beams with triply periodic minimal surface architecture[J]. Composite Structures, 2021, 274: 114342. doi: 10.1016/j.compstruct.2021.114342 [19] USHIJIMA K, CANTWELL W J, MINES R A W, et al. An investigation into the compressive properties of stainless steel micro-lattice structures[J]. Journal of Sandwich Structures & Materials, 2011, 13(3): 303-329. [20] ATAEE A, LI Y C, BRANDT M, et al. Ultrahigh-strength titanium Gyroid scaffolds manufactured by selective laser melting (SLM) for bone implant applications[J]. Acta Materialia, 2018, 158: 354-368. doi: 10.1016/j.actamat.2018.08.005 [21] YANG X, YANG Q, SHI Y S, et al. Effect of volume fraction and unit cell size on manufacturability and compressive behaviors of Ni-Ti triply periodic minimal surface lattices[J]. Additive Manufacturing, 2022, 54: 102737. doi: 10.1016/j.addma.2022.102737 [22] ZADPOOR A A, HEDAYATI R. Analytical relationships for prediction of the mechanical properties of additively manufactured porous biomaterials[J]. Journal of Biomedical Materials Research Part A, 2016, 104(12): 3164-3174. doi: 10.1002/jbm.a.35855 [23] FU J, DING J H, QU S, et al. Improved light-weighting potential of SS316L triply periodic minimal surface shell lattices by micro laser powder bed fusion[J]. Materials & Design, 2022, 222: 111018. [24] YOO D. Computer-aided porous scaffold design for tissue engineering using triply periodic minimal surfaces[J]. International Journal of Precision Engineering and Manufacturing, 2011, 12(1): 61-71. doi: 10.1007/s12541-011-0008-9 [25] PENG X, HUANG Q, ZHANG Y, et al. Elastic response of anisotropic Gyroid cellular structures under compression: Parametric analysis[J]. Materials & Design, 2021, 205: 109706. [26] 杨磊. 增材制造三周期极小曲面点阵结构的力学性能研究[D]. 武汉: 华中科技大学, 2020.YANG Lei. Research on mechanical properties of additive manufactured triply periodic minimal surface lattice structures[D]. Wuhan: Huazhong University of Science and Technology, 2020(in Chinese). [27] 迟静, 李敏, 王淑峰, 等. Ti-C含量对多孔TiC/FeAl复合材料孔型结构和力学性能的影响[J]. 复合材料学报, 2018, 35(9): 2503-2511.CHI Jing, LI Min, WANG Shufeng, et al. Effects of Ti-C contents on the pore structures and mechanical properties of porous TiC/FeAl composites[J]. Acta Materiae Compositae Sinica, 2018, 35(9): 2503-2511(in Chinese). [28] ZHANG L, FEIH S, DAYNES S, et al. Energy absorption characteristics of metallic triply periodic minimal surface sheet structures under compressive loading[J]. Additive Manufacturing, 2018, 23: 505-515. doi: 10.1016/j.addma.2018.08.007 [29] ZHANG C, ZHENG H, YANG L, et al. Mechanical responses of sheet-based Gyroid-type triply periodic minimal surface lattice structures fabricated using selective laser melting[J]. Materials & Design, 2022, 214: 110407. [30] MACONACHIE T, LEARY M, LOZANOVSKI B, et al. SLM lattice structures: Properties, performance, applications and challenges[J]. Materials & Design, 2019, 183: 108137. [31] WANG N Y, MEENASHISUNDARAM G K, CHANG S, et al. A comparative investigation on the mechanical properties and cytotoxicity of cubic, octet, and TPMS Gyroid structures fabricated by selective laser melting of stainless steel 316L[J]. Journal of the Mechanical Behavior of Biomedical Materials, 2022, 129: 015151. [32] RAVICHANDER B B, JAGDALE S H, KUMAR G. Surface morphology, compressive behavior, and energy absorption of graded triply periodic minimal surface 316L steel cellular structures fabricated by laser powder bed fusion[J]. Materials, 2022, 15(23): 8294. doi: 10.3390/ma15238294 [33] YANG K V, ROMETSCH P, JARVIS T, et al. Porosity formation mechanisms and fatigue response in Al-Si-Mg alloys made by selective laser melting[J]. Materials Science and Engineering A-Structural Materials Properties Microstructure and Processing, 2018, 712: 166-174. doi: 10.1016/j.msea.2017.11.078 -





 下载:
下载: