Application of biomass chitosan-based composites for CO2 separation and capture and resource utilization
-
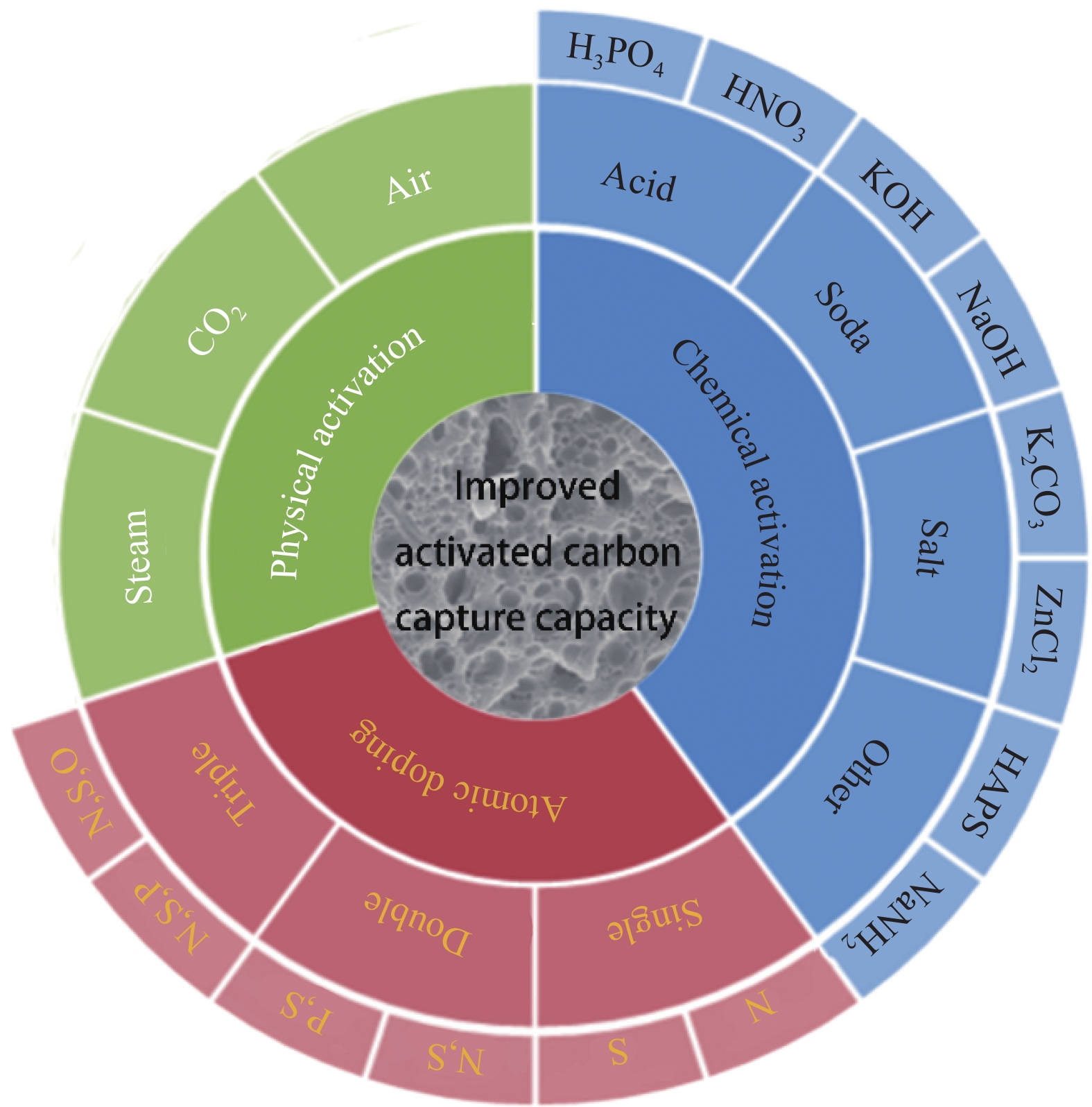
摘要: 二氧化碳过度排放导致的全球变暖、海平面上升和气候恶化等生态环境问题日益显著，亟需探寻新型处理技术和生物质材料来缓解这一问题。本文综述了生物质壳聚糖在二氧化碳分离、捕获和资源化利用领域的研究进展；详细阐述了壳聚糖膜对CO2的分离机制及提高膜分离性能的方法；归纳了增强壳聚糖基活性炭对CO2捕获性能的方法；对利用壳聚糖基催化剂将CO2转化为碳酸酯、甲烷和烯烃等增值品的相关研究进行了总结。最后，对生物质壳聚糖在未来助力“双碳”战略目标实现过程中的发展趋势进行了展望。Abstract: There is an urgent need to explore new treatment technologies and biomass materials to mitigate the growing ecological problems of global warming, sea level rise and climate degradation caused by excessive carbon dioxide emissions. This paper reviews the research progress of biomass chitosan in CO2 separation, capture and resource utilization; details the mechanism of CO2 separation by chitosan membranes and methods to improve membrane separation performance; summarizes the methods to enhance the CO2 capture performance of chitosan-based activated carbon; and summarizes the research on the conversion of CO2 into value-added products such as carbonate, methane and olefin by chitosan-based catalysts. Finally, the future development trend of biomass chitosan in the process of helping to achieve the "double carbon" strategic goal is presented.
-
Keywords:
- carbon peak /
- carbon neutrality /
- biomass materials /
- chitosan /
- carbon dioxide /
- separation /
- capture /
- resource utilization
-
近年来由于航空航天、高铁的飞速发展，航空航天器、高速列车等在高速运行时会产生剧烈的振动，该结构的减振设计将成为技术的关键。波纹夹芯结构作为一种新型的复合材料组合结构，具有高比强度、高比刚度、质量轻、吸能能力强、承载效率高等良好特性，在航空航天、高速列车、汽车、建筑、机械、船舶等领域有着广泛的应用[1-4]。与传统的复合结构材料相比，波纹夹芯板结构很好地克服了夹芯板不连续的问题，具有良好的承载性能。此外，波纹夹芯板芯层间隙具备一定的流通性能，在间隙中穿插泡沫、多孔纤维、玻璃纤维等材料还能起到一定的隔声和隔热作用。因此，对波纹夹芯板的研究具有重要的意义。
由于夹层板拥有广阔的应用前景，近年来国内外学者针对波纹夹芯板各方面特性展开了广泛的研究。Peng等[5] 提出一种无网格伽辽金法来研究非加筋和加筋波纹夹芯板的弹性弯曲问题，将波纹夹芯板视为在两个垂直方向上具有不同挠曲力的正交各项异性板，并推导了梯形、正弦型波纹夹芯板的等效参数。Semenyuk等[6]研究了三种用于纵向波纹圆柱壳稳定性分析的设计方法。Briassoulis等[7]基于正交各向异性壳的拉伸刚度和弯曲刚度的一组解析表达式，对波纹壳进行了数值模拟。Sohrab等[8]提出一种新型的分层波纹复合芯，并通过实验进行了测试。Tian等[9]对波纹夹芯板进行了优化分析。Samanta等[10]首次提出了梯形波纹夹芯板的非线性几何分析，将波纹夹芯板等效成各向异性模型，对梯形波纹夹芯板进行了自由振动分析。Xia等[11]提出了一种基于均质化的波纹夹芯板模型，该模型可用于任何波纹形状。Li 等[12]针对铝制波纹夹芯板开展了空中爆炸试验研究和有限元数值分析，验证了有限元分析技术的可行性。王红霞等[13]和王青伟等[14]推导了考虑波纹拉伸变形的三角形波纹夹芯板的等效弹性参数，后来进行了修正。吴建均等[15]推导了泡沫填充下的三角形波纹夹芯梁的等效弹性常数公式，并且对泡沫波纹夹芯梁的模态进行实验研究和数值计算。还有一些学者对波纹夹芯板的结构性能、力学性能和冲击性能进行了研究[16-18]。
在夹芯板或板结构的研究中，发展了不同的理论，如Reissner板理论、基尔霍夫经典板理论(CLPT)及后来发展的各种剪切变形理论[19-21]等。Talha等[22]基于高阶剪切理论研究了梯度板的静态响应和自由振动。WU等[23]提出了基于RMVT的三阶剪切变形理论(TSDT)。THAI等[24]用正弦剪切变形理论(SSDT)分析了功能梯度板的弯曲、屈曲和振动特性。曹源等[25]用SSDT和修正的欧应力理论研究了功能梯度三明治微梁的静态弯曲和自由振动。但是，用这些理论来研究波纹夹芯板性能的还比较少。
综上所述，波纹夹芯板具有很强的各向异性特性，目前多数文献研究四边简支边界条件下波纹夹芯板的动力学特性，但波纹夹芯板在实际应用中还有其它的边界条件，并且边界条件的影响较显著。鉴于此，本文将采用不同的夹层板理论研究波纹夹芯板在四边简支(SSSS)、四边固支(CCCC)、对边简支和固支(CCSS)、一边固支三边简支(CSSS)四种边界条件下的自由振动，与ABAQUS有限元仿真结果进行对比，验证理论模型的正确性，分析边界条件对波纹夹芯板振动特性的影响。此外，基于指数剪切变形理论（ESDT），讨论波纹夹芯板的材料参数和结构几何参数对系统振动特性的影响。所得结果为轻质波纹夹芯结构的优化设计提供了必要的理论指导。
1. 波纹夹芯板模型
波纹夹芯板的模型如图1(a)所示，由上、下面板及中间的波纹芯层组成。在板中面建立x-y坐标系，
z 轴垂直于x-y面，z>0 的一侧称为下表面，z<0 的一侧称为上表面。波纹夹芯板的长度为a，宽度为b，高度为h，上、下板厚为hf 。图1(b)为波纹芯层的胞元，单胞的底边长为2p ，波纹壁厚为tc ，波纹与面板的夹角为θ，斜边长lc=p/cosθ 芯层厚度hc= ptanθ 。在波纹夹芯板中，上、下面板采用各向同性均质体，中间波纹芯层等效为各向异性均质体，等效示意图如图2所示。仿照文献[13-15]，考虑波纹夹芯板的伸缩变形，波纹芯层的等效参数表达式为
E1(2)=EstcpsinθE2(2)=Estc3cosθhc3[1+(tchc)2cos2θ]G12(2)=Gsptcsinθhc2G13(2)=GstcsinθpG23(2)=Estcsin2θcosθhcv12(2)=vstc2cos2θ(hc2+tc2cos2θ)v21(2)=vsρ(2)=2ρstclcsin2θ (1) 其中：
E1(2) 、E2(2) 、G12(2) 、G13(2) 、G23(2) 、v12(2) 、v21(2) 、ρ(2) 分别表示波纹芯层在各方向上的等效弹性模量、剪切模量、泊松比和密度；Es、Gs和ρs分别为基体材料的弹性模量、剪切模量和密度。![]()
图 1 波纹夹芯板的模型(a)和波纹单胞示意图(b)Figure 1. Model diagrams of corrugated sandwich panel (a) and corrugated cell (b)a—Length of corrugated sandwich panel; b—Width of corrugated sandwich panel; lc—Length of the hypotenuse; hc—Height of core layer; tc—Wall thickness; θ—Corrugation angle; p—Length of bottom side2. 波纹夹芯板振动理论模型
考虑到波纹夹芯板的横向剪切变形效应，位移场可表示为如下形式：
u(x,y,z;t)=u0−z∂w0∂x+f(z)ϕxv(x,y,z;t)=v0−z∂w0∂y+f(z)ϕyw(x,y,z;t)=w0 (2) 其中：
u0 、v0 、w0 分别为波纹夹芯板中面上任意一点的位移；ϕx 和ϕy 分别为波纹夹芯板的直法线沿x轴和y轴的转角；f(z) 是决定横向剪切应力和应变沿厚度分布的形状函数。表1为几种不同剪切形状函数[19, 23-26]。CLPT不考虑横向剪切变形，低估了挠度，高估了固有频率和屈曲载荷，只适用于薄板，而一阶剪切变形理论(FSDT)在此基础上考虑了横向剪切变形，但是在上下表面处不满足面力自由的条件，需要剪切修正因子；ESDT、SSDT和TSDT都考虑了横向剪切变形，且在夹层板的上下表面处满足面力自由的条件，在计算中不需要横向剪切修正因子，很好地克服了CLPT和FSDT的局限性，三者的横向剪切应力和应变沿厚度的形状分别为指数函数型、正弦函数型和曲线型。表 1 不同板理论对应的剪切形状函数Table 1. Shear shape functions corresponding to different plate theoriesShear theory Function f (z) CLPT f(z)=0 FSDT f(z)=z SSDT f(z)=hπsin(πzh) TSDT f(z)=z[1−43(zh)2] ESDT f(z)=ze−2(zh)2 Notes: CLPT—Classical plate theory; FSDT—First-order shear plate theory; SSDT—Sinusoidal shear deformation theory; TSDT—Third-order shear deformation theory; ESDT—Exponential shear deformation theory. 根据小变形假设，位移-应变关系有：
εxx=∂u∂x,εyy=∂v∂y,εzz=∂w∂z,γxz=∂u∂z+∂w∂x,γxy=∂u∂y+∂v∂x,γyz=∂v∂z+∂w∂y (3) 将式(2)代入式(3)得到应变分量为
{εxxεyyγxy}={εxx(0)εyy(0)γxy(0)}+z{εxx(1)εyy(1)γxy(1)}+f{εxx(3)εyy(3)γxy(3)},{γyzγxz}=f′{γyz(2)γxz(2)},εzz=0 (4) 其中，各应变分量的具体表达式为
εxx(0)=∂u0∂x,εxx(1)=−∂2w0∂x2,εxx(3)=∂ϕx∂x,εyy(0)=∂v0∂y,εyy(1)=−∂2w0∂y2,εyy(3)=∂ϕy∂y,γxy(0)=∂u0∂y+∂v0∂x,γxy(1)=−2∂2w0∂x∂y,γxy(3)=∂ϕx∂y+∂ϕy∂x,γyz(2)=ϕy,γxz(2)=ϕx (5) 波纹夹芯板的本构关系可表示为
{σ(k)xxσ(k)yyτ(k)yzτ(k)xzτ(k)xy}=[Q(k)11Q(k)12000Q(k)21Q(k)2200000Q(k)4400000Q(k)5500000Q(k)66]{εxxεyyγyzγxzγxy} (6) 其中，
σ(k)xx 、σ(k)yy 和τ(k)yz 、τ(k)xz 、τ(k)xy 为波纹夹芯板的应力和剪应力。各刚度系数可以表示为
\begin{split} &Q_{11}^{(k)} = \frac{{E_1^{(k)}}}{{1 - \nu_{12}^{(k)}\nu_{21}^{(k)}}},Q_{12}^{(k)} = \frac{{E_1^{(k)}\nu_{21}^{(k)}}}{{1 - \nu_{12}^{(k)}ν_{21}^{(k)}}},Q_{22}^{(k)} = \frac{{E_2^{(k)}}}{{1 - \nu_{12}^{(k)}\nu_{21}^{(k)}}}, \\ &{Q_{66}} = {G_{12}},{Q_{44}} = {G_{23}},{Q_{55}} = {G_{13}},{Q_{21}} = {Q_{12}} \\[-12pt] \end{split} (7) 其中：k=1、2、3 分别代表波纹夹芯板的上、中、下层；E1(k)、E2(k)、G12(k)、G13(k)、G23(k)、ν12(k)、ν21(k)分别表示上、下面板和芯层的弹性模量、剪切模量和泊松比。
波纹夹芯板系统的势能、动能及外力势可以表示为
\begin{split} {\rm{\delta}}U = &\int_0^a {\int_0^b {\int_{ - \frac{h}{2}}^{\frac{h}{2}} {({\sigma _{xx}}{\rm{\delta}}{\varepsilon _{xx}}} } } + {\sigma _{yy}}{\rm{\delta}}{\varepsilon _{yy}} + {\sigma _{{\textit{z}}{\textit{z}}}}{\rm{\delta}}{\varepsilon _{{\textit{z}}{\textit{z}}}} + {\tau _{y{\textit{z}}}}{\rm{\delta}}{\gamma _{y{\textit{z}}}} + \\ &{\tau _{x{\textit{z}}}}{\rm{\delta}}{\gamma _{x{\textit{z}}}} + {\tau _{xy}}{\rm{\delta}}{\gamma _{xy}}){\rm{d}}x{\rm{d}}y{\rm{d}}{\textit{z}} \\[-12pt] \end{split} (8) {\rm{\delta}}K = \int_0^a {\int_0^b {\int_{ - \frac{h}{2}}^{\frac{h}{2}} {\rho (\dot u{\rm{\delta}}\dot u + \dot v{\rm{\delta}}\dot v + \dot w{\rm{\delta}}\dot w)} } } {\rm{d}}x{\rm{d}}y{\rm{d}}{\textit{z}} (9) {\rm{\delta}}V = \int_0^a {\int_0^b {\int_{ - \frac{h}{2}}^{\frac{h}{2}} {q{\rm{\delta}}w{\rm{d}}x{\rm{d}}y{\rm{d}}{\textit{z}}} } } (10) 其中：
\dot u 、\dot v 、\dot w 为波纹夹芯板在x 、y 、{\textit{z}} 方向的运动速度；q为外载荷。根据哈密顿变分原理[27]，得到波纹夹芯板系统的动力学方程为
\begin{split} &\frac{{\partial {N_{xx}}}}{{\partial x}} + \frac{{\partial {N_{xy}}}}{{\partial y}} = {I_0}{{\ddot u}_0} - {I_1}\frac{{\partial {{\ddot w}_0}}}{{\partial x}}{\rm{ + }}{J_1}{{\ddot \phi }_x} \\ &\frac{{\partial {N_{yy}}}}{{\partial y}} + \frac{{\partial {N_{xy}}}}{{\partial x}} = {I_0}{{\ddot v}_0} - {I_1}\frac{{\partial {{\ddot w}_0}}}{{\partial y}}{\rm{ + }}{J_1}{{\ddot \phi }_y} \\ &\frac{{{\partial ^2}{M_{xx}}}}{{\partial {x^2}}} + 2\frac{{{\partial ^2}{M_{xy}}}}{{\partial x\partial y}} + \frac{{{\partial ^2}{M_{yy}}}}{{\partial {y^2}}} + q = {I_0}{{\ddot w}_0}{\rm{ + }}{I_1}\frac{{\partial {{\ddot u}_0}}}{{\partial x}}- \\ &{I_2}\frac{{{\partial ^2}{{\ddot w}_0}}}{{\partial {x^2}}} + {K_2}\frac{{\partial {{\ddot \phi }_x}}}{{\partial x}} + {I_1}\frac{{\partial {{\ddot v}_0}}}{{\partial y}} - {I_2}\frac{{{\partial ^2}{{\ddot w}_0}}}{{\partial {y^2}}} + {K_2}\frac{{\partial {{\ddot \phi }_y}}}{{\partial y}} \\ &\frac{{\partial {H_{xx}}}}{{\partial x}} + \frac{{\partial {H_{xy}}}}{{\partial y}} - {Q_x} = {J_1}{{\ddot u}_0} - {K_2}\frac{{\partial {{\ddot w}_0}}}{{\partial x}} + {J_2}{{\ddot \phi }_x} \\ &\frac{{\partial {H_{yy}}}}{{\partial y}} + \frac{{\partial {H_{xy}}}}{{\partial x}} - {Q_y} = {J_1}{{\ddot v}_0} - {K_2}\frac{{\partial {{\ddot w}_0}}}{{\partial y}} + {J_2}{{\ddot \phi }_y} \\ \end{split} (11) 其中：
\begin{array}{*{20}{l}} {\left[ {\begin{array}{*{20}{c}} {{N_{\xi \eta }}}\\ {{M_{\xi \eta }}}\\ {{H_{\xi \eta }}} \end{array}} \right] = \displaystyle\int_{ - \frac{h}{2}}^{\frac{h}{2}} {{\sigma _{\xi \eta }}} \left[ {\begin{array}{*{20}{c}} 1\\ {\textit{z}}\\ f \end{array}} \right]{\rm{d}}{\textit{z}},{Q_\xi } = \displaystyle\int_{ - \frac{h}{2}}^{\frac{h}{2}} {{\sigma _{\xi {\textit{z}}}}} f'{\rm{d}}{\textit{z}}}\\ {[{I_0},{I_1},{I_2},{J_1},{J_2},{K_2}] = \displaystyle\sum\limits_{k = 1}^3 {\int_{{\zeta _k}}^{{\zeta _{k + 1}}} {{\rho ^{(k)}}[1,{\textit{z}},{{\textit{z}}^2},f,{f^2},{\textit{z}}f]} } {\rm{d}}{\textit{z}}} \end{array} (12) 其中，
\xi 、\eta 可以用x或y表示。将式(4)~(6)、式(12)代入式(11)得到位移形式的运动控制方程为
{E_{i1}}{u_0} + {E_{i2}}{v_0} + {E_{i3}}{w_0} + {E_{i4}}{\phi _x} + {E_{i5}}{\phi _y} = 0,\; i = 1\sim5 (13) 其中：
{E_{11}} = {A_{11}}\frac{{{\partial ^2}}}{{\partial {x^2}}} + {A_{66}}\frac{{{\partial ^2}}}{{\partial {y^2}}} - {I_0}\frac{{{\partial ^2}}}{{\partial {t^2}}} {E_{22}} = {A_{22}}\frac{{{\partial ^2}}}{{\partial {y^2}}} + {A_{66}}\frac{{{\partial ^2}}}{{\partial {x^2}}} - {I_0}\frac{{{\partial ^2}}}{{\partial {t^2}}} {E_{12}}{\rm{ = }}{E_{21}} = ({A_{21}} + {A_{66}})\frac{{{\partial ^2}}}{{\partial x\partial y}} \begin{split} {E_{33}} =& - {G_{11}}\frac{{{\partial ^4}}}{{{x^4}}} - ({G_{12}} + {G_{21}} + 4{G_{66}})\frac{{{\partial ^4}}}{{\partial {x^2}\partial {y^2}}} - \\ &{G_{22}}\frac{{{\partial ^4}}}{{{y^4}}} + {I_2}{\nabla ^2}(\frac{{{\partial ^2}}}{{\partial {t^2}}}) - {I_0}\frac{{{\partial ^2}}}{{\partial {t^2}}} \\ \end{split} {E_{44}} = {R_{11}}\frac{{{\partial ^2}}}{{\partial {x^2}}} + {R_{66}}\frac{{{\partial ^2}}}{{\partial {y^2}}} + {V_{55}} - {J_2}\frac{{{\partial ^2}}}{{{t^2}}} {E_{55}} = {R_{22}}\frac{{{\partial ^2}}}{{\partial {y^2}}} + {R_{66}}\frac{{{\partial ^2}}}{{\partial {x^2}}} + {V_{44}} - {J_2}\frac{{{\partial ^2}}}{{\partial {t^2}}} {E_{13}} = {E_{31}} = {B_{11}}\frac{{{\partial ^3}}}{{{x^3}}} + ({B_{21}} + 2{B_{66}})\frac{{{\partial ^3}}}{{\partial x\partial {y^2}}} - {I_1}\frac{{{\partial ^3}}}{{\partial x\partial {t^2}}} {E_{14}} = {E_{41}} = {D_{11}}\frac{{{\partial ^2}}}{{\partial {x^2}}} + {D_{66}}\frac{{{\partial ^2}}}{{\partial {y^2}}} - {J_1}\frac{{{\partial ^2}}}{{\partial {t^2}}} {E_{15}} = {E_{51}} = {E_{24}} = {E_{42}} = ({D_{21}} + {D_{66}})\frac{{{\partial ^2}}}{{\partial x\partial y}} {E_{23}} = {E_{32}} = - {B_{22}}\frac{{{\partial ^3}}}{{\partial {y^3}}} - ({B_{21}} + 2{B_{66}})\frac{{{\partial ^2}}}{{\partial {x^2}\partial y}} + {I_1}\frac{{{\partial ^3}}}{{\partial y\partial {t^2}}} {E_{25}} = {E_{52}} = {D_{22}}\frac{{{\partial ^2}}}{{\partial {y^2}}} + {D_{66}}\frac{{{\partial ^2}}}{{\partial {x^2}}} - {J_1}\frac{{{\partial ^2}}}{{\partial {t^2}}} {E_{34}} = {E_{43}} = {L_{11}}\frac{{{\partial ^3}}}{{{x^3}}} + ({L_{21}} + 2{B_{66}})\frac{{{\partial ^3}}}{{\partial x\partial {y^2}}} - {K_2}\frac{{{\partial ^3}}}{{\partial x\partial {t^2}}} {E_{35}} = {E_{53}} = ({L_{12}} + 2{L_{66}})\frac{{{\partial ^3}}}{{\partial {x^2}\partial y}} + {L_{22}}\frac{{{\partial ^3}}}{{{y^3}}} - {K_2}\frac{{{\partial ^3}}}{{\partial y\partial {t^2}}} {E_{45}} = {E_{54}} = ({R_{11}} + {R_{66}})\frac{{{\partial ^2}}}{{\partial x\partial y}} \begin{split} &[{A_{ij}},{B_{ij}},{D_{ij}},{G_{ij}},{L_{ij}},{R_{ij}},{V_{ij}}] \\ &= \sum\limits_{k = 1}^3 {\int_{{\zeta _k}}^{{\zeta _{k + 1}}} {{Q^{(k)}}_{ij}[1,{\textit{z}},f,{{\textit{z}}^2},{\textit{z}}f} ,} {f^2},{(f')^2})]{\rm{d}}{\textit{z}} \end{split} 假设位移分量为双三角函数，则
{u_0} 、{v_0} 、{w_0} 、{\phi _x} 、{\phi _y} 可表示为\begin{split} &\left\{ {{u_0},{\phi _x}} \right\} = \displaystyle\sum\limits_{m = 1}^\infty {\displaystyle\sum\limits_{n = 1}^\infty {\left\{ {{U_{mn}},{\Phi _{xmn}}} \right\}\frac{{\partial {X_m}(x)}}{{\partial x}}{Y_n}(y)} } {{\rm{e}}^{{\rm{i}}\omega t}}, \\ &\left\{ {{v_0},{\phi _y}} \right\} = \displaystyle\sum\limits_{m = 1}^\infty {\displaystyle\sum\limits_{n = 1}^\infty {\left\{ {{V_{mn}},{\Phi _{ymn}}} \right\}} } {X_m}(x)\frac{{\partial {Y_n}(y)}}{{\partial y}}{{\rm{e}}^{{\rm{i}}\omega t}}, \\ &\left\{ {{w_0}} \right\} = \displaystyle\sum\limits_{m = 1}^\infty {\displaystyle\sum\limits_{n = 1}^\infty {\left\{ {{W_{0mn}}} \right\}} } {X_m}(x){Y_n}(y){{\rm{e}}^{{\rm{i}}\omega t}} \end{split} (14) 其中：
{\rm{i}} = \sqrt { - 1} ；\omega 为波纹夹芯板系统自由振动下的固有频率。满足波纹夹芯板在4种边界条件下的位移分量形式可以用表2的函数表示。表 2 不同边界条件下的函数Xm(x) 和 Yn(y)Table 2. Functions Xm(x) and Yn(y) for different boundary conditionsBoundary conditions Function Xm(x) Function Yn(y) SSSS \sin \alpha x \sin \beta x CCCC 1 - \cos 2\alpha x 1 - \cos 2\beta x CCSS 1 - \cos 2\alpha x \sin \beta x CSSS \sin \alpha x(\cos \alpha x - 1) \sin \beta x Notes: SSSS—Four sides simply supported; CCCC—Four sides clamped; CCSS—Opposite sides simply supported and clamped; CSSS—One side fixed and three edges clamped; { {\alpha = m{\text{π}}} / a}; { {\beta = n{\text{π}} } / b}; m, n—Half-wave numbers in two orthogonal coordinate directions respectively. 将式(14)代入式(13)可得系统的特征方程，简写为
\left\{ {{{K}} - {\omega ^2}{{M}}} \right\}\left\{ {{\delta}} \right\} = 0 (15) 其中：M和K分别为波纹夹芯板系统的质量矩阵和刚度矩阵；
{\left\{ {\bf{\delta }} \right\}^{\bf{T}}} = \left\{ {{U_{mn}},{V_{mn}},{\Phi _x}_{mn},{\Phi _y}_{mn},{W_{0mn}}} \right\} 为系统的振动幅值。由于它们的取值是任意性的，因此令该特征方程的系数行列式为0，求解该代数方程，即可得到波纹夹芯板自由振动时的固有频率。3. 数值算例及讨论
根据以上理论模型，编写程序计算波纹夹芯板在不同板理论下的固有频率，并且与ABAQUS有限元仿真结果进行对比，验证理论模型的正确性。此外，计算了不同边界条件下波纹夹芯板的基频，分析边界条件对波纹夹芯板振动特性的影响。同时，研究在四种不同边界条件下波纹夹芯板材料参数及结构几何参数的变化对波纹夹芯板基频的影响。
3.1 波纹夹芯板的固有频率
设波纹夹芯板的长a=240 mm，宽b=188.3 mm，上、下面板厚度hf=1 mm，波纹与面板的夹角θ=45°，波纹壁厚tc=1 mm，芯层厚度hc=8 mm。波纹夹芯板三层所采用的基体均为铝材料，其材料参数为：杨氏模量Es=71 GPa，泊松比νs=0.3，剪切模量Gs=Es/2(1+νs)，密度ρs=2 810 kg/m3。
对波纹夹芯板系统进行无量纲化，无量纲化的频率可以定义为
\varpi = \frac{{\omega {a^2}}}{h}\sqrt {\frac{{12{\rho _{\rm{s}}}(1 - \nu_{\rm{s}}^2)}}{{{E_{\rm{s}}}}}} (16) 表3为5种不同板理论所求得的波纹夹芯板在四边简支边界条件下的前五阶固有频率。可以看出，采用SSDT、TSDT、ESDT理论所求得的固有频率与有限元误差较小，全部小于3%，FSDT由于需要剪切修正因子，所求结果误差比以上三个理论略偏大，CLPT由于未考虑横向剪切变形，高估了固有频率，所求结果相比其余4种剪切变形理论，误差要大很多。其中，在基频处，波纹夹芯板的运动和变形相对稳定，剪切应力对板变形的影响相对较小，因此在五种理论中，所求基频与ABAQUS有限元仿真相比误差较小。随着固有频率的增加，波纹夹芯板的变形越来越大，运动也愈加剧烈，因此剪切应力影响变大，考虑剪切变形且满足上下表面处面力自由的SSDT、TSDT和ESDT相对于CLPT和FSDT所求结果更准确。
表 3 四边简支波纹夹芯板在不同理论下的固有频率理论解与有限元仿真结果Table 3. Theoretical solutions and finite element simulation results of natural frequency of simply supported corrugated sandwich plates using different theoriesMode ABAQUS CLPT FSDT SSDT TSDT ESDT Result Error/% Result Error/% Result Error/% Result Error/% Result Error/% (1,1) 31.18 31.72 1.75 31.41 0.77 31.00 −0.57 31.01 −0.54 30.99 −0.58 (2,1) 65.39 69.52 6.32 68.09 4.13 65.82 0.66 65.88 0.75 65.79 0.61 (1,2) 86.48 88.51 2.36 86.23 −0.29 83.74 −3.16 83.78 −3.12 83.74 −3.17 (2,2) 116.13 125.70 8.24 121.21 4.38 115.60 −0.45 115.72 −0.35 115.57 −0.48 (3,1) 118.46 132.06 11.48 127.11 7.30 119.24 0.66 119.45 0.84 119.12 0.56 在四边简支边界条件下，波纹夹芯板前五阶振型模态如图3所示。
![]()
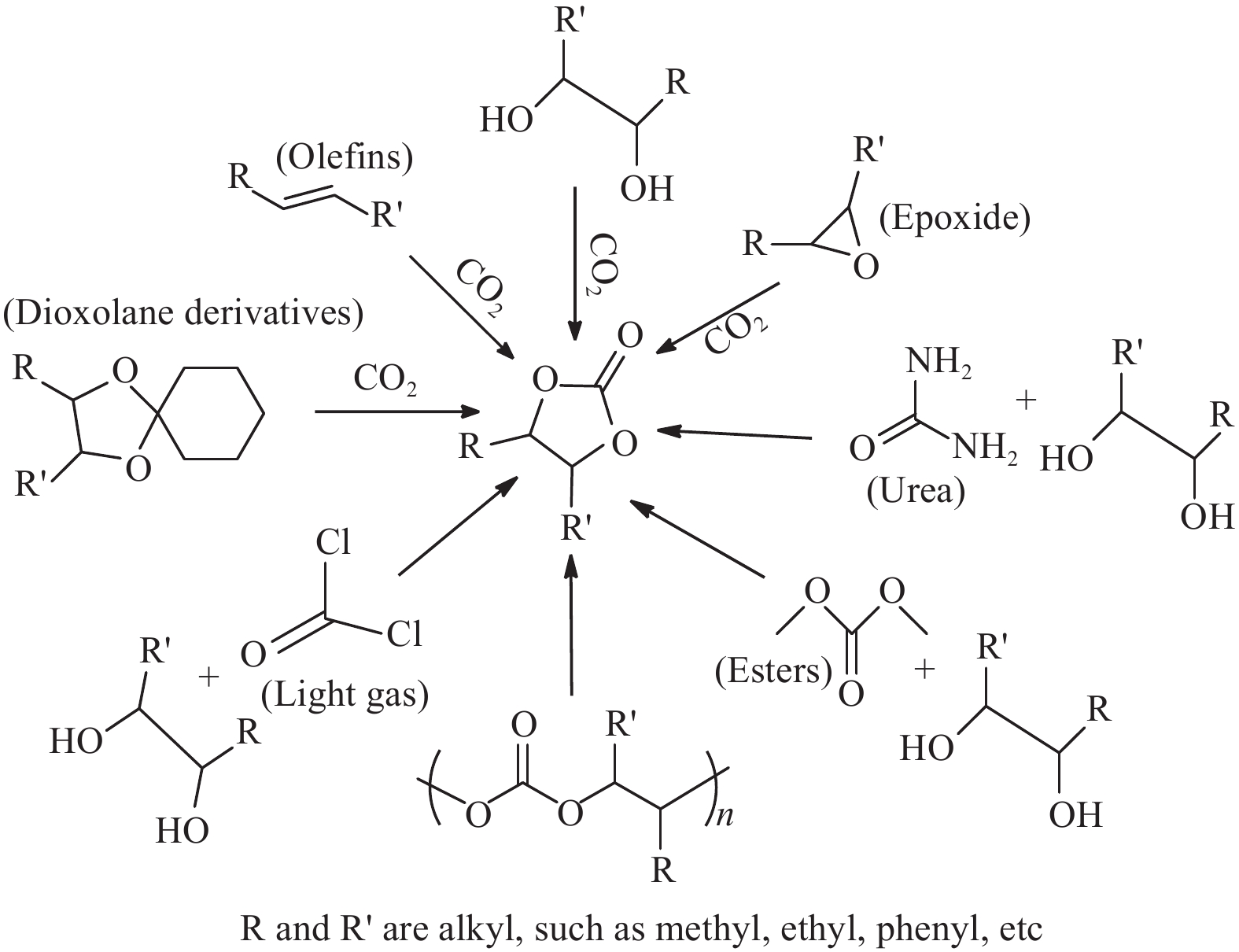
图 3 波纹夹芯板前五阶振型图Figure 3. The first five mode shapes of the corrugated sandwich plates在四种不同边界条件下，由不同板理论计算的波纹夹芯板在自由振动时的基频与有限元仿真结果如表4所示。可以看到，理论解与有限元仿真结果相比，CLPT由于忽略横向剪切应力导致所求结果稍微偏大，考虑横向剪切变形且满足上下表面处面力自由的SSDT、TSDT、ESDT所求结果比FSDT和CLPT的误差小。因为基频处波纹夹芯板的变形相对高频小，剪应力的影响有限，所以5种板理论所求结果误差都在工程允许的误差范围之内，由此也可验证四种边界条件下所假设的位移函数的正确性。比较四种边界条件下所得到的固有频率可以发现，波纹夹芯板在CCCC边界条件下的基频最大，SSSS边界条件下的基频最小，CCCC边界条件下的基频基本可以达到SSSS的1倍左右，CCSS和CSSS边界条件下的固有频率介于上述二者之间，并且两者相差较小，即四种边界条件下波纹夹芯板的基频的关系为CCCC>CCSS>CSSS>SSSS，由此可以得知，固支边界条件会使波纹夹芯板系统在自由振动时的基频增大。同样这也说明了波纹夹芯板边界的约束越多，系统结构整体刚度越大，导致整个系统频率特征值增大。因此，可以通过设置不同的边界条件来调整波纹夹芯板的固有振动频率。
表 4 不同边界条件和板理论下波纹夹芯板的基频理论解与有限元仿真结果Table 4. Theoretical solutions and finite element simulation results of fundamental frequency of corrugated sandwich plates with different boundary conditions and plate theoriesBoundary conditons ABAQUS CLPT FSDT SSDT TSDT ESDT Result Error/% Result Error/% Result Error/% Result Error/% Result Error/% SSSS 31.18 31.72 1.75 31.41 0.77 31.00 −0.57 31.01 −0.54 30.99 −0.58 CCCC 56.42 60.46 7.17 58.90 4.40 56.99 1.01 57.03 1.08 56.98 0.99 CCSS 40.47 43.19 6.71 42.44 4.87 41.23 1.88 41.26 1.96 41.22 1.84 CSSS 39.50 42.14 6.70 41.56 5.22 40.66 2.94 40.68 2.99 40.65 2.91 4种不同边界条件下的波纹夹芯板的一阶振型模态如图4所示。
![]()
图 4 不同边界条件下波纹夹芯板一阶振型图Figure 4. The first mode shapes of corrugated sandwich plates under different boundary conditions3.2 参数变化对波纹夹芯板振动特性的影响
波纹夹芯板的材料参数和结构几何参数对其振动有着重要的影响，通过进一步研究波纹夹芯板的振动特性，为其在工程应用方面提供足够的依据。本节将基于ESDT，研究四种不同边界条件下，波纹夹芯板材料参数和结构几何参数的变化对系统基频的影响。
3.2.1 材料参数变化
波纹夹芯板的上面板、下面板、波纹芯层选用不同的基体材料：Ti和Al，研究不同材料组合对波纹夹芯板固有频率的影响。Al的物理参数在前文已给出，Ti的材料参数为弹性模量Et=177 GPa，泊松比vt = 0.32，密度ρt=4 540 kg/m3。波纹夹芯板的组合方式为Ti-Al-Ti、Ti-Ti-Ti、Al-Al-Al和Al-Ti-Al。板的其它尺寸与上述算例一致，得到四种边界条件下不同材料组合的波纹夹芯板的基频如表5所示。可知，对于每一种边界条件，基于ESDT理论计算所得的四种材料组合下波纹夹芯板的基频大小依次为Ti-Al-Ti> Ti-Ti-Ti> Al-Al-Al> Al-Ti-Al，其中不同材料组合的频率在CCCC边界条件下变化稍大一些，但几种边界条件下的变化趋势基本一致。由式(15)可知，增大材料的弹性模量，即抗变形能力增强，波纹夹芯板结构的刚度增大，系统整体的频率会增大；增大材料的密度，波纹夹芯板的质量增大，波纹夹芯板的频率会降低，但对于波纹夹芯板的上、下面板，弹性模量的影响更显著；对于芯层，密度影响起主导作用。在工程应用中可以选取适当的材料组合以提高波纹夹芯板的固有频率。
表 5 不同材料组合下波纹夹芯板的基频Table 5. Fundamental frequencies of corrugated sandwich panels with different material combinationsBoundary conditon Al-Al-Al Ti-Ti-Ti Al-Ti-Al Ti-Al-Ti SSSS 30.99 38.72 29.07 40.53 CCCC 56.98 71.16 54.31 71.99 CCSS 41.22 51.46 39.96 52.38 CSSS 40.65 50.76 39.03 52.17 3.2.2 结构几何参数变化
作为一种复合结构材料，波纹夹芯板的结构参数对其振动同样有着重要的影响，为了更好地观察波纹夹芯板的基频随结构几何参数的变化趋势，以下计算不再对系统固有频率进行无量纲化，波纹夹芯板各层采用的材料均为铝。
保持波纹夹芯板的基本尺寸和芯层高度及总高度不变，波纹夹芯板在四种边界条件下的基频随波纹与面板夹角θ的变化如图5所示。可以看出，随着波纹与面板夹角θ的增大，在四种边界条件下，波纹夹芯板基频的变化趋势基本接近，都随着夹角θ的增大呈缓慢下降的趋势。由式(1)可知，波纹与面板夹角从
30^\circ 变化到80^\circ 度时，芯层弹性模量E1和剪切模量G13增大，等效密度增大，其它等效参数变化相对较小，波纹夹芯板系统的刚度虽有一定的增大，但等效密度增大更明显，导致波纹夹芯板的基频呈下降趋势。另外，四种边界条件下，CCCC和SSSS边界条件下基频相差较大，且CCCC>SSSS；CSSS和CCSS边界条件下所得到的基频位于CCCC和SSSS之间，CCSS稍大于CSSS，但随着夹角的增大两者对应的波纹夹芯板的基频差值增大。波纹与面板的夹角θ越大，即波纹折皱密度越大，铝制波纹夹芯板越接近实体铝板。由此也可以得知，铝制波纹夹芯板的固有频率大于相同尺寸的实体铝板。![]()
图 5 波纹与面板夹角对波纹夹芯板基频的影响Figure 5. Influence of the angle between corrugation and panel on the fundamental frequency of corrugated sandwich panel波纹芯层高度对波纹夹芯板基频的影响如图6所示。可知，随着芯层高度占比hc/h的增大，即芯层厚度增大，上、下面板厚度减小，4种边界条件下波纹夹芯板基频的变化趋势基本接近。由式(1)可知，随着波纹芯层高度hc的增大，波纹的结构参数(斜边长lc、半底边长p)增大，波纹芯层各个方向的弹性模量和剪切模量减小，导致系统的刚度减小，从而使基频降低，但是随着芯层高度占比hc/h的增大，波纹芯层的等效密度减小，使系统基频增大。因为在hc/h≤0.8时芯层等效密度的影响起主导作用，之后弹性模量和剪切模量的影响起主导作用，所以导致波纹夹芯板的基频先增大后减小。在hc/h=0.8附近，基频达到最大值，在hc/h>0.8时，波纹夹芯板的基频迅速下降。因此可以得知，在实际工程应用中选取适当的芯层占比可以提高波纹夹芯板的固有频率。
![]()
图 6 波纹芯层高度占比对波纹夹芯板基频的影响Figure 6. Influence of corrugated core height ratio on the fundamental frequency of corrugated sandwich panel波纹夹芯板的基频随波纹壁厚的变化如图7所示。由等效参数计算式(1)，随着壁厚的增加，波纹芯层的剪切模量和弹性模量增大，且E12呈直线增大，系统刚度增大，同样芯层的等效密度也呈直线增大，导致系统的基频减小。因为等效密度的影响起主导作用，所以波纹夹芯板的基频持续减小。另外，CCSS和CSSS两种边界条件下的基频的差值略微增大，且4种边界条件下，CCCC边界条件下波纹夹芯板的基频相对于其他3种边界条件在壁厚tc≤3 mm时下降速度略快一些，其余边界条件下，波纹夹芯板基频的下降趋势基本一致。
![]()
图 7 波纹壁厚度对波纹夹芯板基频的影响Figure 7. Influence of corrugated wall thickness on the fundamental frequency of corrugated sandwich panel波纹夹芯板厚度 h对波纹夹芯板基频的影响如图8所示。可见，波纹夹芯板芯层在各方向的弹性模量和剪切模量在h≤30时迅速减小，芯层刚度随之减小，之后几乎保持不变，导致系统的固有频率减小。但是由式(1)可知，芯层的等效密度同样在h≤30时迅速减小，之后几乎保持不变，且等效密度的影响起着主导作用。当波纹夹芯板的总厚度h<30 mm时，四种边界条件下波纹夹芯板系统的基频呈现明显增大的趋势，CCCC边界条件下的基频增长速率最快，CSSS和CCSS边界条件所对应的基频曲线接近重合，在h=15 mm附近CSSS条件下所求的基频开始高于CCSS边界条件下所求的基频。当h>30 mm时，四条曲线都趋于平稳，波纹夹芯板基频变化波动很小，此时板的宽厚比b/h<6，波纹夹芯板由薄板逐渐变为厚板。根据以上分析可以得知，在工程应用中适当提高波纹夹芯板的厚度可以提高系统的固有频率。
![]()
图 8 波纹夹芯板厚度h 对波纹夹芯板基频的影响Figure 8. Influence of corrugated plate thickness on the fundamental frequency of corrugated sandwich panel设k为变化比例因子，将波纹夹芯板结构的所有几何尺寸分别乘以比例因子k，即波纹夹芯板结构整体变大或者缩小，得到的波纹夹芯板基频的变化曲线如图9所示。随着k的变化，波纹夹芯板芯层的弹性模量、剪切模量和密度保持不变。由于波纹夹芯板长和宽的增大，导致整个系统质量增加，因此系统的固有频率将减小。图中所取变化比例因子k为0.2~3，四种边界下波纹夹芯板的基频在
k\!\leqslant\!1 时急剧下降，CCSS和CSSS曲线基本重合；在k \!\geqslant\!1 时，四种边界条件下的波纹夹芯板系统的基频越来越接近。由此可知，当波纹夹芯板尺寸无限大时，四种边界条件下波纹夹芯板系统的基频会接近相等，且波纹夹芯板尺寸越大系统的基频就越小。![]()
图 9 比例因子k 对波纹夹芯板基频的影响Figure 9. Influence of scale factor on the fundamental frequency of corrugated sandwich panel4. 结 论
研究了波纹夹芯板在四种边界条件下的自由振动特性，对比了不同板理论所求得的固有频率，并与有限元仿真结果进行了对比。此外，基于指数剪切变形理论(ESDT)分析了波纹夹芯板材料参数和结构几何参数的变化对系统固有频率的影响。
(1)对于基尔霍夫经典板理论(CLPT)、一阶剪切变形理论(FSDT)、正弦剪切变形理论(SSDT)、三阶剪切变形理论(TSDT)、ESDT五种板理论，其中CLPT由于不考虑板的横向剪切变形，因此求得系统自由振动的固有频率误差最大，FSDT所求固有频率次之，SSDT、TSDT、ESDT所求固有频率相近，且与有限元仿真结果相比，误差最小。
(2)四种边界条件下波纹夹芯板自由振动的基频大小依次为：四边固支(CCCC)>对边简支和固支(CCSS)>一边固支三边简支(CSSS)>四边简支(SSSS)，且CCCC的基频比SSSS的基频约大1倍, 即波纹夹芯板结构的边界约束越多，系统的固有频率越大。
(3)增大波纹夹芯板材料的弹性模量会导致其刚度变大，进而使系统的基频增大；增大材料密度会导致波纹夹芯板的质量增大，进而使系统的基频减小，对于上、下面板，弹性模量的影响较显著，而对于夹芯层，密度的影响起主导作用。
(4)波纹夹芯板的结构几何参数对系统的振动有着重要影响。随着波纹与面板的夹角
\theta 或波纹壁厚tc的增大，波纹夹芯板的基频变小；随着芯层占比hc/h的增大，波纹夹芯板的基频先增大后减小；保持hc/h、面板占比hf/h不变，随着波纹夹芯板厚度h的增大，波纹夹芯板的基频增大明显；随着波纹夹芯板尺寸的增大其基频下降，且四种边界条件下波纹夹芯板的基频趋近相等。 -
![]()
图 2 增强活性炭对CO2捕获性能的方法
HAPS—Hydroquinonesulfonic acid potassium salt
Figure 2. Methods to enhance the CO2 capture performance byactivated carbon
![]()
![]()
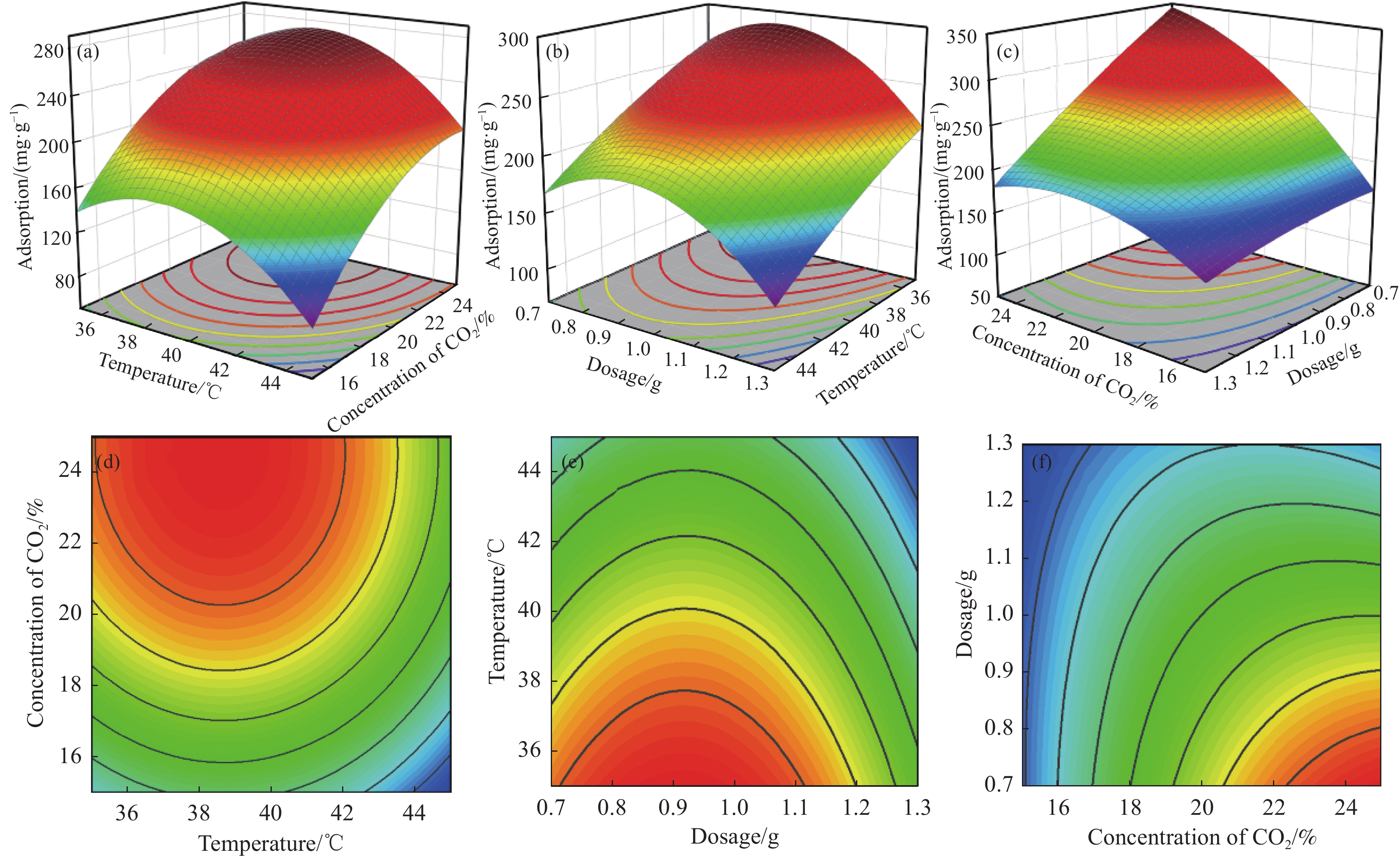
图 4 利用响应曲面法分析不同因素对壳聚糖-漂白土CO2去除率的影响：((a), (d)) 温度和CO2浓度对吸附量的影响；((b), (e))温度和CTS用量对吸附量的影响；((c), (f)) CTS用量和CO2浓度对吸附量的影响[46]
Figure 4. Effect of different factors on CO2 removal from chitosan-bleached soil using response surface methodology: ((a), (d)) Effect of temperature and CO2 concentration on adsorption capacity; ((b), (e)) Effect of temperature and CTS dosage on adsorption capacity; ((c), (f)) Effect of CTS dosage and CO2 concentration on adsorption capacity[46]
表 1 利用壳聚糖(CTS)/羧甲基壳聚糖(CMC)与填料制备混合基质膜
Table 1 Preparation of mixed matrix membrane using chitosan (CTS)/carboxymethyl chitosan (CMC) and filler
Membrane Packing loading rate Penetration rate of CO2 CO2/N2 selectivity Separation condition Literature CMC/PZ PZ，20% 89 GPU 103 80℃, 120 kPa [22] CTS/GO/PVAm HPEI-GO, 2% and 3% 36 GPU (2%) 107 (3%) CO2 : N2 =10 : 90 [23] CTS/ZIF-8/IL ZIF-8，10% (5413±191) Barrer 11.5 50℃, 200 kPa [24] CTS/SF/GNP GNP，0.5% 159 GPU 93 90℃, 200 kPa [25] CMC/HT HT，1% 70 GPU 13 90℃, 200 kPa [26] Notes: PZ—Piperazine; PVAm—Polyvinylamine; HPEI-GO—Hyperbranched polyethyleneimine grafted graphene oxide; ZIF-8—Zinc(2-methylimidazole); IL—Ionic liquid; GNP—Graphene nanoparticles; HT—Hydrotalcite; SF—Silk fibroin. 
下载: 导出CSV
表 2 通过原子掺杂的方法制备壳聚糖基活性炭
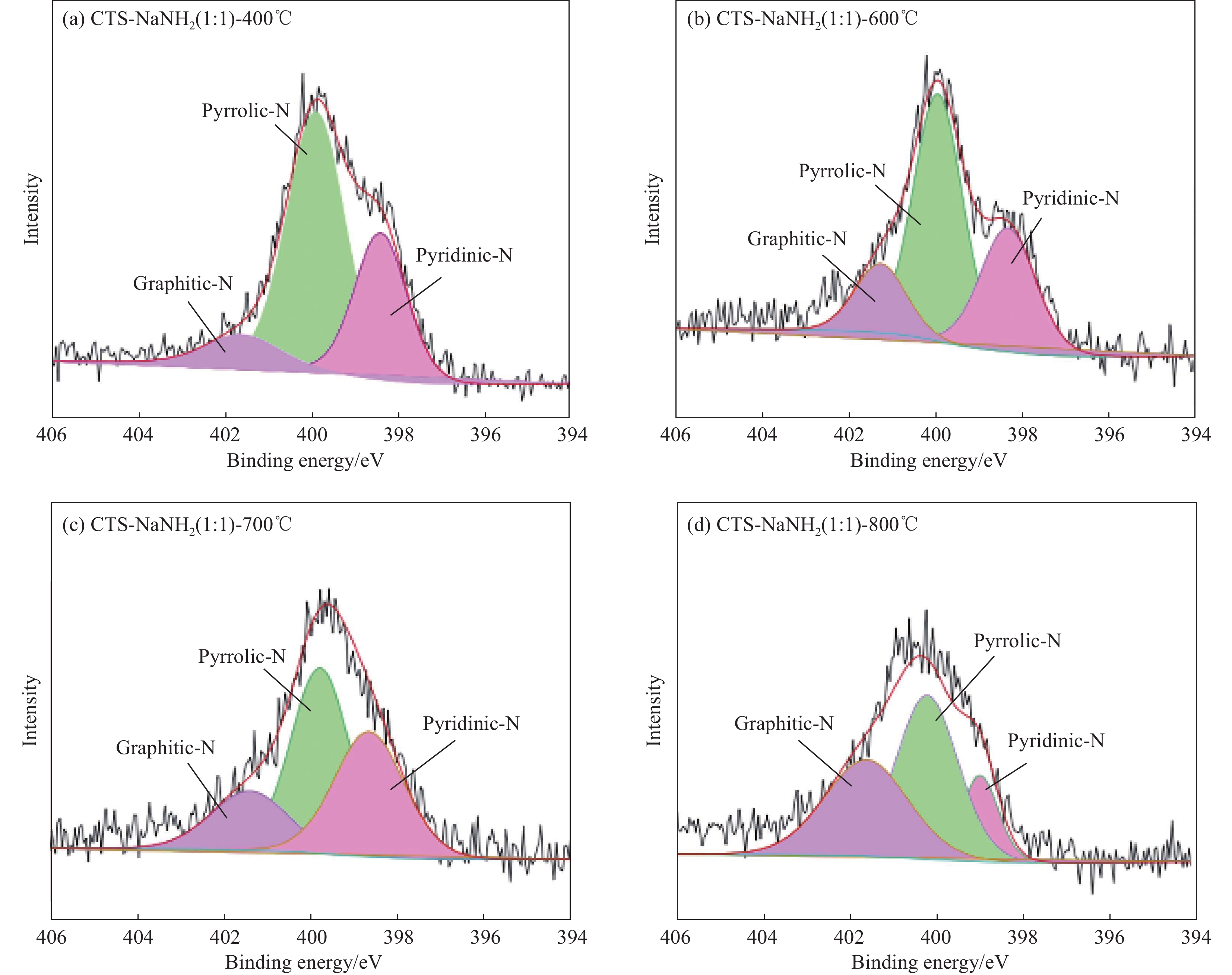
Table 2 Preparation of chitosan-based activated carbon by atomic doping method
Carbon Source Source of heteroatoms Activator Adsorption performance Reusability Literature CTS CTS provides N atoms K2CO3 100 kPa, 25℃, adsorption capacity was 3.86 mmol/g Adsorption capacity unchanged
after five cycles[37] CTS TMP and CTS provide N atoms KOH 0℃, 100 kPa, the maximum CO2 adsorption capacity was 4.74 mmol/g After ten cycles, the adsorption capacity decreased by 8% [38] CTS HMT and CTS provide N atoms ZnCl2 Adsorption capacity was smaller than undoped activated carbon – [39] CTS NaNH2 and CTS provide N atoms NaNH2 0℃, 100 kPa, the adsorption amount of CO2 was 6.33 mmol/g After three cycles, the adsorption
effect remains almost constant
[35]CTS,
SLCTS and SL provide N and S atoms, respectively KOH 0℃, 100 kPa, the adsorption amount of CO2 was 215.2 mg/g After three cycles, the adsorption capacity has decreased by 20% [40] CTS CTS and HAPS provide N and S atoms, respectively HAPS 25℃, 100 kPa, the adsorption amount of CO2 was 2.4 mmol/g – [41] CTS CTS provides the N atoms and phytic acid provides the P atoms NaNO3 100 kPa, the adsorption amount of CO2 was 3.02 mmol/g After twenty cycles, the adsorption capacity remains unchanged [42] Notes: TMP—2, 4, 6-triaminopyrimidine; HMT—Hexamethylenetetramine; SL—Sodium lignosulfonate.
下载: 导出CSV
表 3 改性壳聚糖基吸附剂用于CO2捕获
Table 3 Modified chitosan-based sorbents for CO2 capture
Adsorbent Modification method Modification advantages Introduction of substances Adsorption performance Literature Furfuryl-
imine-CTS
fibersImine formation The introduction of imine groups produced a
highly nucleophilic active surface
20℃, 61 kPa, the adsorption
amount was 0.978 mmol/g[47] Chitosan
based
aerogelGraft Carboxyl, amino, imine, amide and other
groups are introduced
35℃, the adsorption amount
was 5.48 mmol/g[48] CTS/LS hydrogel Cross-link The introduction of Li+ and basic group, enhances the acid-base effect and electrostatic effect 
100 kPa, 25℃, the maximum
adsorption amount
was 67.9 mg/g[49] CTS/MWCNTs Graft Increase the surface area of CTS and introduce hydroxyl and carboxyl groups 
25℃, 100 kPa, the maximum
adsorption amount
was 1.92 cm3/g[50] Notes: MWCNTs—Multi-walled carbon nanotubes; LS—Lithium sulfonate.
下载: 导出CSV
表 4 利用CO2制备其他化学增值品
Table 4 Preparation of other chemical value-added products using CO2
Catalyst Raw materials Reaction condition Product Production efficiency Reusability Literature CTS/KOH/
EnzymesBicarbonate, CaCl2, CO2 pH 8, 35℃ CaCO3 152 mg of CaCO3 per gram of CO2 No change after 7 repetitions [61] K-doped Fe/CTS complexes CO2, H2 330℃, 1.5 MPa Olefins CO2 conversion rate was 41% and
olefin yield was 20.4%– [62] CTS/CA/SiO2 CaCl2, CO2 pH 7.6, 25℃ CaCO3 230 mg CaCO3 were gotten after 10 min Can be reused up to 30 times [63] CTS/PEG Ethanol, CO2, H2 6 MPa, 170℃ Methanol Resulting methanol concentration was 472 mmol/L Can be recycled up to 3 times [64] C/A/PEC CaCl2, CO2 pH 8.2, pH 9.5 CaCO3 1 g of catalyst can catalyze the
synthesis of 253 mg of CaCO3No change after 10 repetitions [65] Cu/TiO2/CTS H2O, CO2 Xenon lamp irradiation Methane Yield of methane was 5.34 μmol/g – [66] Notes: CA—Carbonic anhydrase; PEG—Polyethyleneglycol; C/A/PEC—Chitosan-alginate polyelectrolyte complex.
下载: 导出CSV
-
[1] NAGASE T, MIYAKAWA M, NISHIOKA M, et al. Microwave-assisted green synthesis of mesoporous zeolite adsorbents for direct air capture of CO2[J]. Chemistry Letters, 2022, 51(3): 296-299. DOI: 10.1246/cl.210687
[2] YU S, LI S, HUANG S, et al. Covalently bonded zeolitic imidazolate frameworks and polymers with enhanced compatibility in thin film nanocomposite membranes for gas separation[J]. Journal of Membrane Science, 2017, 540: 155-164. DOI: 10.1016/j.memsci.2017.06.047
[3] CHABAN V V, ANDREEVA N A. Extensively amino-functionalized graphene captures carbon dioxide[J]. Physical Chemistry Chemical Physics, 2022, 24(42): 25801-25815. DOI: 10.1039/D2CP03235J
[4] 汤琦龙, 傅晶依, 窦信, 等. 改性壳聚糖磁性纳米材料的研究进展[J]. 复合材料学报, 2022, 39(3): 1017-1025 TANG Qilong, FU Jingyi, DOU Xin, et al. Research progress of modified chitosan magnetic nanomaterials[J]. Acta Materiae Compositae Sinica, 2022, 39(3): 1017-1025(in Chinese)
[5] RANGNEKAR N, MITTAL N, ELYASSI B, et al. Zeolite membranes-A review and comparison with MOFs[J]. Chemical Society Reviews, 2015, 44(20): 7128-7154. DOI: 10.1039/C5CS00292C
[6] ITO A, SATO M, ANMA T. Permeability of CO2 through chitosan membrane swollen by water vapor in feed gas[J]. Die Angewandte Makromolekulare Chemie: Applied Macromolecular Chemistry and Physics, 1997, 248(1): 85-94. DOI: 10.1002/apmc.1997.052480105
[7] WEINKAUF D H, KIM H D, PAUL D R. Gas transport properties of liquid crystalline poly (p-phenyleneterephthalamide)[J]. Macromolecules, 1992, 25(2): 788-796. DOI: 10.1021/ma00028a044
[8] EL-AZZAMI L A, GRULKE E A. Dual mode model for mixed gas permeation of CO2, H2, and N2 through a dry chitosan membrane[J]. Journal of Polymer Science Part B: Polymer Physics, 2007, 45(18): 2620-2631. DOI: 10.1002/polb.21236
[9] REN J, XUAN H, GE L. Double network self-healing chitosan/dialdehyde starch-polyvinyl alcohol film for gas separation[J]. Applied Surface Science, 2019, 469: 213-219. DOI: 10.1016/j.apsusc.2018.11.001
[10] ZHANG H, TIAN H, ZHANG J, et al. Facilitated transport membranes with an amino acid salt for highly efficient CO2 separation[J]. International Journal of Greenhouse Gas Control, 2018, 78: 85-93. DOI: 10.1016/j.ijggc.2018.07.014
[11] LI N, WANG Z, WANG J. Water-swollen carboxymethyl chitosan (CMC)/polyamide (PA) membranes with octopus-branched nanostructures for CO2 capture[J]. Journal of Membrane Science, 2022, 642: 119946. DOI: 10.1016/j.memsci.2021.119946
[12] DANCKWERTS P V. The reaction of CO2 with ethanolamines[J]. Chemical Engineering Science, 1979, 34(4): 443-446. DOI: 10.1016/0009-2509(79)85087-3
[13] PRASAD B, MANDAL B. CO2 separation performance by chitosan/tetraethylenepentamine/poly(ether sulfone) composite membrane[J]. Journal of Applied Polymer Science, 2017, 134(34): 45206. DOI: 10.1002/app.45206
[14] PRASAD B, MANDAL B. Preparation and characterization of CO2-selective facilitated transport membrane composed of chitosan and poly (allylamine) blend for CO2/N2 separation[J]. Journal of Industrial and Engineering Chemistry, 2018, 66: 419-429. DOI: 10.1016/j.jiec.2018.06.009
[15] PRASAD B, MANDAL B. Moisture responsive and CO2 selective biopolymer membrane containing silk fibroin as a green carrier for facilitated transport of CO2[J]. Journal of Membrane Science, 2018, 550: 416-426. DOI: 10.1016/j.memsci.2017.12.061
[16] DE MORAES M A, NOGUEIRA G M, WESKA R F, et al. Preparation and characterization of insoluble silk fibroin/chitosan blend films[J]. Polymers, 2010, 2(4): 719-727. DOI: 10.3390/polym2040719
[17] EL-AZZAMI L A, GRULKE E A. Carbon dioxide separation from hydrogen and nitrogen: Facilitated transport in arginine salt-chitosan membranes[J]. Journal of Membrane Science, 2009, 328(1-2): 15-22. DOI: 10.1016/j.memsci.2008.08.038
[18] SHEN J N, YU C C, ZENG G N, et al. Preparation of a facilitated transport membrane composed of carboxymethyl chitosan and polyethylenimine for CO2/N2 separation[J]. International Journal of Molecular Sciences, 2013, 14(2): 3621-3638. DOI: 10.3390/ijms14023621
[19] BORGOHAIN R, JAIN N, PRASAD B, et al. Carboxymethyl chitosan/carbon nanotubes mixed matrix membranes for CO2 separation[J]. Reactive and Functional Polymers, 2019, 143: 104331. DOI: 10.1016/j.reactfunctpolym.2019.104331
[20] KATARE A, SHARMA S, MANDAL B. Effects of L-lysine-conjugated-graphene oxide as a nanofiller on the CO2 separation performance of mixed matrix chitosan membrane[J]. Indian Chemical Engineer, 2023, 65(2): 168-179.
[21] BORGOHAIN R, MANDAL B. Thermally stable and moisture responsive carboxymethyl chitosan/dendrimer/hydrotalcite membrane for CO2 separation[J]. Journal of Membrane Science, 2020, 608: 118214. DOI: 10.1016/j.memsci.2020.118214
[22] BORGOHAIN R, PRASAD B, MANDAL B. Synthesis and characterization of water-soluble chitosan membrane blended with a mobile carrier for CO2 separation[J]. Separation and Purification Technology, 2019, 222: 177-187. DOI: 10.1016/j.seppur.2019.04.038
[23] SHEN Y, WANG H, LIU J, et al. Enhanced performance of a novel polyvinyl amine/chitosan/graphene oxide mixed matrix membrane for CO2 capture[J]. ACS Sustainable Chemistry & Engineering, 2015, 3(8): 1819-1829.
[24] CASADO-COTERILLO C, FERNÁNDEZ-BARQUÍN A, ZORNOZA B, et al. Synthesis and characterisation of MOF/ionic liquid/chitosan mixed matrix membranes for CO2/N2 separation[J]. RSC Advances, 2015, 5(124): 102350-102361. DOI: 10.1039/C5RA19331A
[25] PRASAD B, MANDAL B. Graphene-incorporated biopolymeric mixed-matrix membrane for enhanced CO2 separation by regulating the support pore filling[J]. ACS Applied Materials & interfaces, 2018, 10(33): 27810-27820.
[26] BORGOHAIN R, MANDAL B. High-speed CO2 transport channel containing carboxymethyl chitosan/hydrotalcite membrane for CO2 separation[J]. Journal of Applied Polymer Science, 2020, 137(21): 48715. DOI: 10.1002/app.48715
[27] MALINI K, SASI M, MEGHANATH R, et al. Effect of barium activation on chitosan derived carbon: Enhancement of CO2 adsorption capacity[J]. International Journal of Environmental Analytical Chemistry, 2023, 103(18): 7042-7054.
[28] MIYAJIMA N, TAKIZAWA K, SAKANE H. Surface characterization of chitosan and cellulose-derived porous carbons with K2CO3 activation and its application to water and ethene adsorption[J]. Journal of Porous Materials, 2021, 28(6): 1689-1695. DOI: 10.1007/s10934-021-01116-x
[29] NAZIR G, REHMAN A, PARK S J. Valorization of shrimp shell biowaste for environmental remediation: Efficient contender for CO2 adsorption and separation[J]. Journal of Environmental Management, 2021, 299: 113661. DOI: 10.1016/j.jenvman.2021.113661
[30] KAMRAN U, PARK S J. Tuning ratios of KOH and NaOH on acetic acid-mediated chitosan-based porous carbons for improving their textural features and CO2 uptakes[J]. Journal of CO2 Utilization, 2020, 40: 101212. DOI: 10.1016/j.jcou.2020.101212
[31] OTOWA T, NOJIMA Y, MIYAZAKI T. Development of KOH activated high surface area carbon and its application to drinking water purification[J]. Carbon, 1997, 35(9): 1315-1319. DOI: 10.1016/S0008-6223(97)00076-6
[32] LI D, ZHOU J, ZHANG Z, et al. Improving low-pressure CO2 capture performance of N-doped active carbons by adjusting flow rate of protective gas during alkali activation[J]. Carbon, 2017, 114: 496-503. DOI: 10.1016/j.carbon.2016.12.039
[33] LI J, BAO A, CHEN J, et al. A green route to CO2 adsorption on biomass chitosan derived nitrogen-doped micropore-dominated carbon nanosheets by different activators[J]. Journal of Environmental Chemical Engineering, 2022, 10(1): 107021. DOI: 10.1016/j.jece.2021.107021
[34] SINGH G, TIBURCIUS S, RUBAN S M, et al. Pure and strontium carbonate nanoparticles functionalized microporous carbons with high specific surface areas derived from chitosan for CO2 adsorption[J]. Emergent Materials, 2019, 2: 337-349. DOI: 10.1007/s42247-019-00050-8
[35] YANG C, ZHAO T, PAN H, et al. Facile preparation of N-doped porous carbon from chitosan and NaNH2 for CO2 adsorption and conversion[J]. Chemical Engineering Journal, 2022, 432: 134347. DOI: 10.1016/j.cej.2021.134347
[36] SEVILLA M, VALLE-VIGÓN P, FUERTES A B. N-doped polypyrrole-based porous carbons for CO2 capture[J]. Advanced Functional Materials, 2011, 21(14): 2781-2787. DOI: 10.1002/adfm.201100291
[37] FAN X, ZHANG L, ZHANG G, et al. Chitosan derived nitrogen-doped microporous carbons for high performance CO2 capture[J]. Carbon, 2013, 61: 423-430. DOI: 10.1016/j.carbon.2013.05.026
[38] YANG Q, TENG D, QU J, et al. Solvent-free synthesis of N-doped porous carbons from chitosan for an efficient CO2 capture[J]. Industrial & Engineering Chemistry Research, 2021, 60(35): 13023-13030.
[39] MALINI K, SELVAKUMAR D, KUMAR N S. Nitrogen doped activated carbon derived from chitosan/hexamethylenetetramine: Structural and CO2 adsorption properties[J]. Journal of Porous Materials, 2022, 29(5): 1539-1550. DOI: 10.1007/s10934-022-01276-4
[40] SHAO L, WANG L, WANG J, et al. N-doped highly microporous carbon derived from the self-assembled lignin/chitosan composites beads for selective CO2 capture and efficient p-nitrophenol adsorption[J]. Separation and Purification Technology, 2023, 313: 123440. DOI: 10.1016/j.seppur.2023.123440
[41] SHI J, CUI H, XU J, et al. Synthesis of nitrogen and sulfur co-doped carbons with chemical blowing method for CO2 adsorption[J]. Fuel, 2021, 305: 121505. DOI: 10.1016/j.fuel.2021.121505
[42] XIAO J, WANG Y, ZHANG T C, et al. Phytic acid-induced self-assembled chitosan gel-derived N, P–co-doped porous carbon for high-performance CO2 capture and supercapacitor[J]. Journal of Power Sources, 2022, 517: 230727. DOI: 10.1016/j.jpowsour.2021.230727
[43] SEVILLA M, PARRA J B, FUERTES A B. Assessment of the role of micropore size and N-doping in CO2 capture by porous carbons[J]. ACS Applied Materials & Interfaces, 2013, 5(13): 6360-6368.
[44] BABARAO R, DAI S, JIANG D. Nitrogen-doped mesoporous carbon for carbon capture-A molecular simulation study[J]. The Journal of Physical Chemistry C, 2012, 116(12): 7106-7110. DOI: 10.1021/jp301450m
[45] THOTE J A, CHATTI R V, IYER K S, et al. N-doped mesoporous alumina for adsorption of carbon dioxide[J]. Journal of Environmental Sciences, 2012, 24(11): 1979-1984. DOI: 10.1016/S1001-0742(11)61022-X
[46] ISLAM M A, TAN Y L, ISLAM M A, et al. Chitosan-bleaching earth clay composite as an efficient adsorbent for carbon dioxide adsorption: Process optimization[J]. Colloids and Surfaces A: Physicochemical and Engineering Aspects, 2018, 554: 9-15.
[47] MARIN L, DRAGOI B, OLARU N, et al. Nanoporous furfuryl-imine-chitosan fibers as a new pathway towards eco-materials for CO2 adsorption[J]. European Polymer Journal, 2019, 120: 109214. DOI: 10.1016/j.eurpolymj.2019.109214
[48] HSAN N, DUTTA P K, KUMAR S, et al. Arginine containing chitosan-graphene oxide aerogels for highly efficient carbon capture and fixation[J]. Journal of CO2 Utilization, 2022, 59: 101958. DOI: 10.1016/j.jcou.2022.101958
[49] LIU Z, MA R, DU W, et al. Radiation-initiated high strength chitosan/lithium sulfonate double network hydrogel/aerogel with porosity and stability for efficient CO2 capture[J]. RSC Advances, 2021, 11(33): 20486-20497. DOI: 10.1039/D1RA03041H
[50] HSAN N, DUTTA P K, KUMAR S, et al. Capture and chemical fixation of carbon dioxide by chitosan grafted multi-walled carbon nanotubes[J]. Journal of CO2 Utilization, 2020, 41: 101237. DOI: 10.1016/j.jcou.2020.101237
[51] TAMBOLI A H, CHAUGULE A A, KIM H. Highly selective and multifunctional chitosan/ionic liquids catalyst for conversion of CO2 and methanol to dimethyl carbonates at mild reaction conditions[J]. Fuel, 2016, 166: 495-501. DOI: 10.1016/j.fuel.2015.11.023
[52] BESSE V, ILLY N, DAVID G, et al. A chitosan derivative containing both carboxylic acid and quaternary ammonium moieties for the synthesis of cyclic carbonates[J]. ChemSusChem, 2016, 9(16): 2167-2173. DOI: 10.1002/cssc.201600499
[53] XIAO L F, LI F W, XIA C G. An easily recoverable and efficient natural biopolymer-supported zinc chloride catalyst system for the chemical fixation of carbon dioxide to cyclic carbonate[J]. Applied Catalysis A: General, 2005, 279(1-2): 125-129. DOI: 10.1016/j.apcata.2004.10.022
[54] ZHAO Y, TIAN J S, QI X H, et al. Quaternary ammonium salt-functionalized chitosan: An easily recyclable catalyst for efficient synthesis of cyclic carbonates from epoxides and carbon dioxide[J]. Journal of Molecular Catalysis A: Chemical, 2007, 271(1-2): 284-289. DOI: 10.1016/j.molcata.2007.03.047
[55] KUMAR S E, SILVA J A, WANI M Y, et al. Carbon dioxide capture and conversion by an environmentally friendly chitosan based meso-tetrakis (4-sulfonatophenyl) porphyrin[J]. Carbohydrate Polymers, 2017, 175: 575-583. DOI: 10.1016/j.carbpol.2017.08.031
[56] KUMAR S, WANI M Y, KOH J, et al. Carbon dioxide adsorption and cycloaddition reaction of epoxides using chitosan-graphene oxide nanocomposite as a catalyst[J]. Journal of Environmental Sciences, 2018, 69: 77-84. DOI: 10.1016/j.jes.2017.04.013
[57] BORJIAN BOROUJENI M, LAEINI M S, NAZERI M T, et al. A novel and green in situ strategy for the synthesis of metallophthalocyanines on chitosan and investigation their catalytic activity in the CO2 fixation[J]. Catalysis Letters, 2019, 149: 2089-2097. DOI: 10.1007/s10562-019-02740-8
[58] WU Y, ZUO S, ZHAO Y, et al. Biomass-derived metal-organic hybrids for CO2 transformation under ambient conditions[J]. Green Chemistry, 2020, 22(9): 2846-2851. DOI: 10.1039/D0GC00212G
[59] VALENTINI A, CARRENO N L V, PROBST L F D, et al. Ni: CeO2 nanocomposite catalysts prepared by polymeric precursor method[J]. Applied Catalysis A: General, 2006, 310: 174-182. DOI: 10.1016/j.apcata.2006.05.037
[60] SEELAJAROEN H, SPIESS S, HABERBAUER M, et al. Enhanced methane producing microbial electrolysis cells for wastewater treatment using poly (neutral red) and chitosan modified electrodes[J]. Sustainable Energy & Fuels, 2020, 4(8): 4238-4248.
[61] SHARMA A, BHATTACHARYA A, SHRIVASTAVA A. Biomimetic CO2 sequestration using purified carbonic anhydrase from indigenous bacterial strains immobilized on biopolymeric materials[J]. Enzyme and Microbial Technology, 2011, 48(4-5): 416-426. DOI: 10.1016/j.enzmictec.2011.02.001
[62] CHEN B Y, DOBELE G, PLAVNIECE A, et al. Catalytic hydrogenation of CO2 to light olefins by using K-doped FeCx catalysts derived from the Fe-chitosan complex[J]. International Journal of Hydrogen Energy, 2023, 48(11): 4276-4286. DOI: 10.1016/j.ijhydene.2022.11.010
[63] WOO K M, LEE I, HONG S G, et al. Crosslinked chitosan coating on magnetic mesoporous silica with pre-adsorbed carbonic anhydrase for carbon dioxide conversion[J]. Chemical Engineering Journal, 2015, 276: 232-239. DOI: 10.1016/j.cej.2015.04.057
[64] KOTHANDARAMAN J, HELDEBRANT D J. Towards environmentally benign capture and conversion: Heterogeneous metal catalyzed CO2 hydrogenation in CO2 capture solvents[J]. Green Chemistry, 2020, 22(3): 828-834. DOI: 10.1039/C9GC03449H
[65] OVIYA M, SUKUMARAN V, GIRI S S. Immobilization and characterization of carbonic anhydrase purified from E. coli MO1 and its influence on CO2 sequestration[J]. World Journal of Microbiology and Biotechnology, 2013, 29: 1813-1820. DOI: 10.1007/s11274-013-1343-z
[66] SHE H, ZHAO Z, BAI W, et al. Enhanced performance of photocatalytic CO2 reduction via synergistic effect between chitosan and Cu : TiO2[J]. Materials Research Bulletin, 2020, 124: 110758. DOI: 10.1016/j.materresbull.2019.110758
-
期刊类型引用(4)
1. 于丹. 聚氯乙烯/碳纳米管复合材料的制备和性能研究. 塑料科技. 2024(01): 36-39 . 
百度学术
2. 余澎，涂操，郭博森，王闻达，赵航，彭玉婷，罗卫华. 木质素基碳纳米管/炭复合材料的制备及电化学性能研究. 现代化工. 2023(02): 92-97 . 百度学术
3. 冀佳帅，杜佳琪，陈俊琳，张新民，刘伟，宋朝霞. Co-Fe普鲁士蓝/多壁碳纳米管复合材料的超电容性能. 材料科学与工艺. 2023(04): 1-8 . 百度学术
4. 张开砚. 电感耦合等离子体发射光谱法测定普鲁士蓝类正极材料中铁和钠. 化学分析计量. 2022(08): 26-30 . 百度学术
其他类型引用(5)
-
目的
汽车尾气、工厂废气和化石燃料燃烧将大量二氧化碳气体排放到空气中，CO的吸收与释放逐渐失衡，由此导致的全球变暖、臭氧层消耗等现象进一步引发冰川融化、海平面上升等生态环境问题。亟需探寻新型处理技术和生态友好的生物质材料来缓解这一问题。近年，国内外学者制备了大量壳聚糖基复合材料，并将其用于CO分离、捕获和资源化利用等领域，在减少CO排放和缓解全球变暖等问题上取得了重大进展。为推动新型CO处理材料的开发，助力“碳中和”、“碳达峰”战略目标的早日实现，本文对国内外利用壳聚糖基复合材料处理CO的研究进展进行了综述。
方法从壳聚糖基复合材料在CO分离、捕获和资源化利用三个方面的应用进行综述。首先归纳了壳聚糖基复合膜在CO分离中的应用，并分析了增强壳聚糖膜CO分离性能的方法。其次，详细阐述了以壳聚糖基活性炭为代表的吸附剂在CO捕获中的应用，并阐明了不同因素对活性炭吸附性能的影响效果。另外，对将CO转化为碳酸酯、甲醇、甲烷和碳酸钙等化学增值品的研究现状进行总结。最后，讨论了壳聚糖基复合材料在当前CO处理领域所面临的问题与挑战。
结果在CO分离领域，壳聚糖基分离膜对CO的分离机制包括溶解-扩散和促进运输两种。溶解-扩散机制具有选择性差和分离效率低等问题，所以大量研究围绕促进运输机制展开。在促进运输机制中，载体与CO通过可逆反应形成复合物，复合物进一步通过可逆反应实现CO在相邻载体之间的不断“跳跃”，直至到达膜的解吸面完成分离。载体的种类和负载率是决定壳聚糖膜分离性能的关键因素。在CO捕获领域，壳聚糖基活性炭对CO的吸附能力受孔隙率、孔径、内比表面积和孔容的影响。高孔隙率、高内比表面积和小孔径是确保活性炭具备最佳CO吸附能力的前提，但微孔结构的好坏取决于炭化过程中的活化处理措施是否得当。在CO资源化利用领域，通过基于壳聚糖的电催化、生物酶催化和氧化还原反应等方法能够将CO转化为碳酸酯、甲烷、碳酸钙、甲醇和烯烃等增值品，但多数反应需要在高温、高压的苛刻条件下进行，所以制备能够在温和条件下高效催化CO转化为各种化学增值品的催化剂，是未来实现CO高效资源化利用的前提。
结论通过不同的改性方法制备壳聚糖基复合材料，并将其用于CO分离、捕获和资源化利用等领域，可显著改善由CO过度排放引起的生态环境问题。综合国内外利用生物质壳聚糖基复合材料处理CO的研究进展，未来可以从以下几个方面考虑：(1)基于促进运输机制，在壳聚糖膜中引入载体和填料可以显著增强膜的分离性能，未来应通过合理的实验确定不同载体与CO的反应摩尔比，进而确保壳聚糖膜的最适载体负载率，使其具备最佳CO分离性能。(2)在保证壳聚糖基活性炭最佳活化效果的前提下，选择可以同时作为活化剂和原子掺杂剂的无毒材料，确保活性炭具备最佳微孔结构的同时，提高活性炭的碱性位点数量，是未来使活性炭具备最大CO吸附能力的有效手段。(3)制备集分离、捕获和资源化利用功能于一体的壳聚糖基CO处理材料，是未来生物质壳聚糖助力“碳达峰”、“碳中和”快速实现的发展趋势。
计量
- 文章访问数: 729
- HTML全文浏览量: 427
- PDF下载量: 64
- 被引次数: 9
