基于多层次迭代修正的纤维增强复合薄壁截顶圆锥壳振动响应分析

许卓; 许沛尧; 初晨; 姚楠; 李晖; 顾大卫; 李鹤; 闻邦椿

doi:10.13801/j.cnki.fhclxb.20230625.001

基于多层次迭代修正的纤维增强复合薄壁截顶圆锥壳振动响应分析

许卓^{1, 3},
许沛尧¹,
初晨¹,
姚楠¹,
李晖^{2, 3, ,},
顾大卫^{3, 4},
李鹤^{2, 3},
闻邦椿^{2, 3}

1.
东北电力大学　机械工程学院，吉林 132011
2.
东北大学　机械工程与自动化学院，沈阳 110819
3.
东北大学　航空动力装备振动及控制教育部重点实验室，沈阳 110819
4.
浙江工业大学　机械工程学院，杭州 310014

基金项目: 东北电力大学博士科研启动基金(BSJXM-2020221)；东北大学航空动力装备振动及控制教育部重点实验室研究基金(VCAME202204)

详细信息

通讯作者:
李晖，博士，副教授，博士生导师，研究方向为复合结构减振降噪　E-mail: lh200300206@163.com

中图分类号: TB535；TB330.1
计量
- 文章访问数: 566
- HTML全文浏览量: 224
- PDF下载量: 23
出版历程
- 收稿日期: 2023-05-04
- 修回日期: 2023-06-07
- 录用日期: 2023-06-08
- 网络出版日期: 2023-06-24
- 刊出日期: 2024-02-29

Vibration response analysis of fiber reinforced composite thin-walled truncated conical shell based on multilevel iterative correction

XU Zhuo^{1, 3},
XU Peiyao¹,
CHU Chen¹,
YAO Nan¹,
LI Hui^{2, 3, ,},
GU Dawei^{3, 4},
LI He^{2, 3},
WEN Bangchun^{2, 3}

1.
School of Mechanical Engineering, Northeast Electric Power University, Jilin 132011, China
2.
School of Mechanical Engineering and Automation, Northeastern University, Shenyang 110819, China
3.
Key Laboratory of Vibration and Control of Aero-Propulsion System Ministry of Education, Northeastern University, Shenyang 110819, China
4.
School of Mechanical Engineering, Zhejiang University of Technology, Hangzhou 310014, China

Funds: Northeast Electric Power University Doctoral Research Initiation Fund (BSJXM-2020221); Research Fund from the Key Laboratory of Aeroengine Vibration and Control, Ministry of Education, Northeastern University (VCAME202204)

摘要

摘要: 提出了一种纤维增强复合薄壁截锥壳的振动响应分析模型。针对纤维增强复合薄壁截锥壳的结构特点，考虑基础激励载荷方向与母线的夹角、纤维铺层方向与x轴的夹角，利用板壳振动理论、复弹性模量等方法对所研究结构进行了理论建模。利用双向梁函数法表示振型函数，并通过能量法和模态叠加法对其固有特性和振动响应进行求解。为了验证模型的正确性，基于自行搭建的振动测试平台，以TC300/环氧树脂基纤维增强复合薄壁截锥壳为对象，进行了振动特性测试。为减小因样件加工时产生的材料参数误差影响，开发了二分粒子群迭代法对材料参数进行修正。研究发现，测试结果与理论计算获得的共振响应误差最大不超过3.0%，验证了所提出的理论模型与计算方法的正确性和有效性。
- 纤维增强 /
- 圆锥壳 /
- 基础激励 /
- 多层次迭代修正 /
- 振动响应
Abstract: A vibration response analysis model was established for a fiber-reinforced composite thin-walled truncated conical shell. Based on the structural characteristics of the fiber-reinforced composite thin-walled truncated conical shell, the theoretical modeling of the structure was carried out using plate shell vibration theory and complex elastic modulus methods, considering the angle between the basic excitation load direction and the generatrix, the angle between the fiber laying direction and the x-axis. The vibration mode function was expressed using the bi-directional beam function method, and the natural characteristics and vibration response were solved using the energy method and modal superposition method. In order to verify the correctness of the model, vibration characteristic tests were conducted on a TC300/epoxy resin-based fiber-reinforced composite thin-walled truncated cone shell using a self-built vibration test platform. To reduce the influence of material parameter errors caused by sample processing, a dichotomous particle swarm algorithm iteration method was developed to correct the material parameters. The results show that the maximum error between the test results and the theoretically calculated resonance response is within 3.0%, which verifies the correctness and effectiveness of the proposed theoretical model and calculation method.
- fiber reinforced /
- conical shell /
- base excitation /
- multilevel iterative correction /
- vibration response

HTML全文

纤维增强复合材料薄壁截锥壳相对于金属薄壁圆锥壳，具有质量轻、承载能力较好，可靠性高、绝缘性好等多种优点，目前正在被广泛应用于航空航天、海洋船舶、石油化工等重要工程领域^[1-3]。如液体航空发动机所使用的复合材料壳体燃烧室、深海无人探测器的复合材料耐高压壳体、船舶螺旋桨尾部舱段等，在复杂的工程应用环境中，这些壳体很容易受到基础激励载荷的作用，另外，应用结构的复杂性、应用环境的多样性使其振动响应的问题尤为突出^[4-5]。因此，研究基础激励下的纤维增强复合材料薄壁截锥壳的振动响应问题有着重要的工程及学术意义。

长期以来，国内外研究人员针对复合材料薄壁截锥壳在不同激励条件下的振动响应进行了深入的研究，并取得了阶段性的成果。例如：王爱勤^[6]基于二维解析、一维离散的半解析有限元法，计算了一般材料的截锥体(壳)在复杂载荷激励作用下的振动响应。杨绍武^[7]基于一阶剪切变形理论和von Karman几何非线性关系，研究了功能梯度复合材料截锥壳在面内热环境下的振动响应，并对不同温度下的系统非线性振动响应进行分析。Dey等^[8]基于替代模型的方法结合概率有限元模型的方法对复合材料层压截锥壳的固有特性及基础激励响应进行了求解，但此种方法具有概率上的不确定性，因此需要大量实验进行对比拟合。Ansari^[9]基于Hamilton原理与广义微分求积法对功能梯度的碳纳米管复合材料截锥壳的振动特性进行求解，并用变分格式下的数值分析方法求解了其振动响应。Mercan^[10]基于离散奇异卷积的方法，并通过锥壳方程的极限取值对功能梯度复合材料环形板的振动响应。谢坤^[11]基于幂级数半解析计算方法，对一般材料的船舶螺旋桨尾部的加筋圆锥壳舱段进行振动响应分析，并对小型密封式加筋圆锥壳进行横、纵向激励实验。张永强^[12]基于板壳振动理论、弹性力学理论建立了一般材料的薄壁圆锥壳的常规动力学模型，并对模型的固有特性和基础激励响应进行了求解。Lei等^[13]基于场变量修正的傅里叶级数法与Ritz法对一般边界条件下的功能梯度碳纳米管的增强复合材料截锥板进行了自由振动分析，并进行了有限元验证。Li等^[14]基于Rayleigh-Ritz法从理论上对硬质涂层纤维增强复合材料薄壁圆柱壳的振动特性进行分析，并求解了其振动响应。Heidari Soureshjani等^[15]基于一阶剪切变形理论推导了基础激励下的三明治夹层结构的截锥壳的响应方程，并进行了求解。Zhang等^[16]基于简化板理论和二维改进的傅里叶-利兹法对厚度较大的扇形锥板的振动响应问题进行了求解。Kamaloo等^[17]基于能量法与Galerkin方法建立了全周向三明治结构的复合材料截锥壳的振动特性问题的运动学方程，并进行了振动响应求解。Yang等^[18]基于一阶剪切变形理论、von Karman型非线性几何假设建立了截断功能梯度复合材料(FGM)锥壳的非线性振动方程，并采用Hamilton原理和Galerkin方法对其非线性振动响应进行了求解。Safarpour等^[19]基于弹性理论对片状功能梯度石墨烯增强复合材料截锥壳的振动响应公式进行了推导，并进行了自由振动响应的求解。Rezaiee-Pajand等^[20]基于一阶剪切变形理论建立了纳米复合材料锥壳的自由振动方程。并用广义微分求积法来求解Donnell型控制微分方程，从而求解出其振动响应。Maji等^[21]基于高阶剪切变形理论建立了三维编织复合材料截锥壳自由振动方程，并对其振动响应进行求解。Sobhani等^[22]基于一阶剪切变形理论对夹层复合材料圆锥-圆柱-圆锥壳进行了自由振动分析，并根据几个复杂的工程应用实例模拟了不同激励条件下的振动响应。Shi等^[23]基于一阶剪切变形理论，建立了功能梯度材料截锥壳的热力耦合能量方程。通过引入人工弹簧技术，推导了热环境下截锥壳结构的能量表达式。并对振动响应进行求解。

从上述文献可以看出，虽然众多学者已经对截锥壳的振动响应问题进行了深入的研究，但上述研究主要集中在金属和功能梯度材料所制成的构件上，对于纤维增强材料所制成截锥壳的研究较少，且大多都停留在理论分析推导与求解的层面，其理论分析结果并没有经过实验验证。由于纤维增强材料具有各向异性的特点，其本构方程更加复杂，且由于母线与激励方向存在夹角，这导致其振动响应的准确预测更加困难。在上述的一些有理论分析与实验对比的文献中，理论预测结果与实验测试结果出现了较大的对比误差；同时，一些文献中所提出的修正方法计算量较大，迭代过程也过于繁琐。

因此，针对上述问题，本文首先以纤维增强复合薄壁截锥壳(FRCTCS)为研究对象，考虑结构中各向异性的特点，建立所研究结构的本构方程，并通过能量法和拉格朗日方程建立动力学方程，考虑结构母线和激励方向的夹角，将激励分解并求解结构的振动响应。其次，为了验证所提出方法的正确性，搭建了基础激励下的纤维增强复合材料薄壁截顶圆锥壳振动响应的测试系统，进行了实验验证。最后，利用本文提出的二分粒子群迭代法，对理论模型进行修正运算，从而达到较高的计算精度。本文所提出的方法可对该类结构的实际工程应用提供通用性的理论依据和指导。

1. 基础激励下的FRCTCS振动响应求解

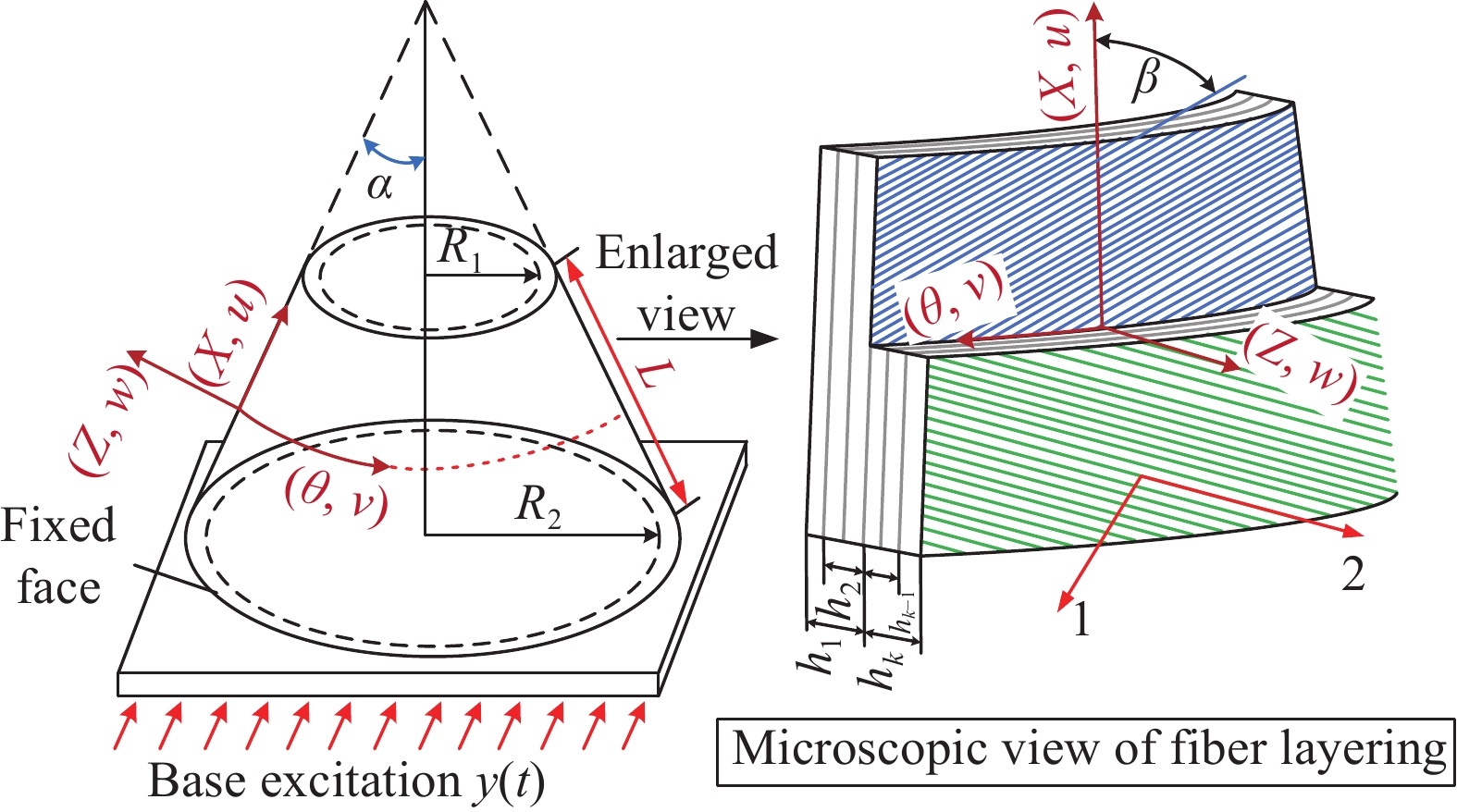
所研究的纤维增强复合薄壁圆锥壳是由N层正交各项异性的纤维材料组合而成的，固定方式为大半径端固定，“O-XθZ”坐标系位于中间平面，所受基础激励方向与“XOZ”面垂直，见图1。

图 1 纤维增强复合薄壁圆锥壳理论模型

Figure 1. Theoretical model for fiber-reinforced composite thin-walled conical shells

u, ν, and w—Displacement functions in the X, θ, and Z directions, respectively; R₂—Major radius; R₁—Minor radius; L—Length of the generatrix; h—Shell thickness; α—Half-cone angle; β—Angle between the fiber direction and the X-axis

下载: 全尺寸图片幻灯片

首先，以其中面为参考平面，纤维增强复合薄壁圆锥壳大半径为R₂，小半径为R₁，母线长为L，壳体厚为h，半锥角为α；其次，材料的每一层位于Z轴较低平面h_k−1与h_k之间，厚度均匀。最后，1代表平行纤维方向，2代表垂直纤维方向。设1-2平面内的剪切弹性模量为G₁₂，1方向的弹性模量为E₁，2方向的弹性模量为E₂，1方向所引起的1-2平面的应变泊松比为μ₁，2方向所引起的1-2平面的应变泊松比为μ₂，密度为ρ。

假设薄壁圆锥壳体受到基础激励载荷的影响，并且给出基础激励的位移表达式为

$y(t) = Y{{{\rm{e}}} ^{{\rm{i}}\omega t}}$

(1)

其中：Y为激励幅值；ω为激励频率；i为复数因子；t为时间。

在考虑层间纤维方向的影响下，基于复模量法，对复合材料的材料参数进行重新定义：

$\begin{split} & E_1^* = E_1^\prime \left( {1 + {\text{i}}{\eta _1}} \right){\text{ }} \\ & E_2^* = E_2^\prime \left( {1 + {\text{i}}{\eta _2}} \right){\text{ }} \\ & G_{12}^* = G_{12}^\prime \left( {1 + {\text{i}}{\eta _{12}}} \right) \end{split}$

(2)

其中：E* ₁、η₁分别代表平行纤维方向的复弹性模量和损耗因子；E* ₂、η₂分别代表垂直纤维方向的复弹性模量和损耗因子；G* ₁₂、η₁₂分别代表1-2平面中的复剪切模量和损耗因子；E' ₁、E' ₂、G' ₁₂分别为复弹性模量的实部及复剪切模量的实部。

基于经典层合理论和Kirchhoff-Love假设，将忽略了剪切变形的纤维增强复合截顶圆锥壳的中面位移分量方程写成如下形式：

$\begin{split} &{u(x,\theta ,t) = A\frac{{\partial \psi (x)}}{{\partial x}}\cos {\omega _0}t\cos n\theta } \\ & {v(x,\theta ,t) = B\psi (x)\cos {\omega _0}t\sin n\theta } \\ & {w(x,\theta ,t) = C\psi (x)\cos {\omega _0}t\cos n\theta } \end{split}$

(3)

其中：A、B、C分别为方向的振动幅值；n为圆锥壳的周向波数；ω₀为复合薄壳的固有圆频率；ψ(x)为不同边界条件下的梁函数。根据文献[1]，梁函数ψ(x)表示为

$\begin{split} \psi (x) =& \cosh \left( {\frac{{{\lambda _m}x}}{L}} \right) - \cos \left( {\frac{{{\lambda _m}x}}{L}} \right) - \\ &{\sigma _m}\left[ {\sinh \left( {\frac{{{\lambda _m}x}}{L}} \right) - \sin \left( {\frac{{{\lambda _m}x}}{L}} \right)} \right] \end{split}$

(4)

其中，m为圆柱壳的轴向波数。由于本章所研究的复合薄壳大半径端为约束端，小半径端及母线端均为自由的状态，因此根据文献[1]，梁函数系数λ_m和σ_m (m=1, 2, 3)的取值如表1所示。

表 1 梁函数系数的取值

Table 1. Values of beam function coefficients

m	λ_m	σ_m
1	1.87510	0.734096
2	4.69409	1.018467
3	7.85476	0.999224
Note: λ_m and σ_m—Coefficients of the beam functions.

下载: 导出CSV

| 显示表格

根据Love壳体理论，纤维增强复合材料薄壁圆锥壳的应力-应变可表示为

${\varepsilon _x} = \frac{{\partial u}}{{\partial x}}$

${\varepsilon _\theta } = \frac{1}{{x \sin \alpha}}\left( {\frac{{\partial v}}{{\partial\theta }} + u\sin \alpha - w\cos \alpha } \right)$

${\gamma _{x\theta }} = \frac{{\partial v}}{{\partial x}} - \frac{v}{x} + \frac{1}{{x \sin \alpha }} \frac{{\partial u}}{{\partial \theta }}$

(5)

${X_x} = - \frac{{{\partial ^2}w}}{{\partial {x^2}}}$

${X_\theta } = \frac{{\cos \alpha \partial v}}{{{x^2}\sin {\alpha ^2}\partial \theta }} - \frac{{{\partial ^2}w}}{{{x^2}\sin \alpha \partial {\theta ^2}}} - \frac{{2\partial w}}{{x\partial x}}$

${X_{x\theta }} = \frac{{\partial v}}{{x\tan \alpha \partial x}} - \frac{{2v}}{{{x^2}\tan \alpha }} - \frac{{2{\partial ^2}w}}{{x\sin \alpha \partial x\partial \theta }} + \frac{{2\partial w}}{{{x^2}\sin \alpha \partial \theta }}$

对于正交各向异性材料，其应力-应变的关系为

${\left[ {\begin{array}{*{20}{c}} {{\sigma _1}}\\ {{\sigma _2}}\\ {{\tau _{12}}} \end{array}} \right]^{(k)}} = {\left[ {\begin{array}{*{20}{c}} {{Q_{11}}}&{{Q_{12}}}&0\\ {{Q_{12}}}&{{Q_{22}}}&0\\ 0&0&{{Q_{66}}} \end{array}} \right]^{(k)}}{\left[ {\begin{array}{*{20}{c}} {{\varepsilon _1}}\\ {{\varepsilon _2}}\\ {{\gamma _{12}}} \end{array}} \right]^{(k)}}$

(6)

其中：σ₁、σ₂、τ₁₂分别为平行于纤维方向的应力、垂直纤维方向的应力和平面内的应力；ε₁、ε₂、γ₁₂分别为平行与纤维方向上的应变、垂直纤维方向的应变和平面内的应变；Q_ij (i=1, 6；j=1, 2, 6)为定义应力-应变关系的利兹参数：

$\begin{split} & {Q_{11}} = \frac{{E_1^*}}{{1 - {\mu _1}{\mu _2}}};{\text{ }}{Q_{12}} = \frac{{{\mu _1}E_2^*}}{{1 - {\mu _1}{\mu _2}}}; \\ & {Q_{22}} = \frac{{E_2^*}}{{1 - {\mu _1}{\mu _2}}};{\text{ }}{Q_{66}} = {G_{12}};{\text{ }}{\mu _2} = {\mu _1}\frac{{E_2^*}}{{E_1^*}} \\ \end{split}$

(7)

其中，由于本文使用的是经典层合理论，利兹参数矩阵省略了Q₁₆、Q₂₆、Q₄₄与剪切变形相关的3个元素。

当纤维材料与主轴x方向有一定的夹角β时，第k层平面的应力-应变关系为

${\left[ {\begin{array}{*{20}{c}} {{\sigma _x}} \\ {{\sigma _\theta }} \\ {{\sigma _{x\theta }}} \end{array}} \right]^{(k)}} = {\left[ {\begin{array}{*{20}{l}} {{{\bar Q}_{11}}}&{{{\bar Q}_{12}}}&{{{\bar Q}_{16}}} \\ {{{\bar Q}_{12}}}&{{{\bar Q}_{22}}}&{{{\bar Q}_{26}}} \\ {{{\bar Q}_{16}}}&{{{\bar Q}_{26}}}&{{{\bar Q}_{66}}} \end{array}} \right]^{(k)}}{\left[ {\begin{array}{*{20}{c}} {{\varepsilon _x}} \\ {{\varepsilon _\theta }} \\ {{\gamma _{x\theta }}} \end{array}} \right]^{(k)}}$

(8)

其中，引入角度β后的参数 ${\bar Q_{ij}}$ 为

$\begin{split} {{\bar Q}_{11}} =& {Q_{11}}{\cos ^4}{\beta _k} + 2\left( {{Q_{12}} + 2{Q_{66}}} \right){\sin ^2}{\beta _k}{\cos ^2}{\beta _k}+ \\ &{\text{ }} {Q_{22}}{\sin ^4}{\beta _k} \\ \end{split} \tag{9a}$

$\begin{split} {{\bar Q}_{12}} = &\left( {{Q_{11}} + {Q_{22}} - 4{Q_{66}}} \right){\sin ^2}{\beta _k}{\cos ^2}{\beta _k}+ \\ &{\text{ }} {Q_{12}}\left( {{{\sin }^4}{\beta _k} + {{\cos }^4}{\beta _k}} \right) \end{split}\tag{9b}$

$\begin{split} {{\bar Q}_{22}} = &{Q_{11}}{\sin ^4}{\beta _k} + 2\left( {{Q_{12}} + 2{Q_{66}}} \right){\sin ^2}{\beta _k}{\cos ^2}{\beta _k}+ \\ & {\text{ }} {Q_{22}}{\cos ^4}{\beta _k} \end{split} \tag{9c}$

$\begin{split} {{\bar Q}_{16}} =& \left( {{Q_{11}} - {Q_{12}} - 2{Q_{66}}} \right)\sin {\beta _k}{\cos ^3}{\beta _k} + \\ & {\text{ }} \left( {{Q_{12}} - {Q_{22}} + 2{Q_{66}}} \right){\sin ^3}{\beta _k}\cos {\beta _k} \end{split} \tag{9d}$

$\begin{split} {{\bar Q}_{26}} =& \left( {{Q_{11}} - {Q_{12}} - 2{Q_{66}}} \right){\sin ^3}{\beta _k}\cos {\beta _k}+ \\ &{\text{ }} \left( {{Q_{12}} - {Q_{22}} + 2{Q_{66}}} \right)\sin {\beta _k}{\cos ^3}{\beta _k} \end{split} \tag{9e}$

$\begin{split} {{\bar Q}_{66}} = & \left( {{Q_{11}} + {Q_{22}} - 2{Q_{12}} - 2{Q_{66}}} \right){\sin ^2}{\beta _k}{\cos ^2}{\beta _k}+ \\ & {Q_{66}}\left( {{{\sin }^4}{\beta _k} + {{\cos }^4}{\beta _k}} \right) \end{split} \tag{9f}$

式中：k表示复合壳的第k层；β_k表示第k层纤维与坐标系x轴的夹角的大小。

为了使理论建模更加方便，将壳体收到的基础激励载荷等效为均匀的惯性力外载荷f (t)，其表达式为

$f(t) = - \rho h\frac{{{{\text{d}}^2}w(t)}}{{{\text{d}}{t^2}}} = \rho hY{\omega ^2}{{\text{e}}^{{\text{i}}\omega t}}$

由基础激励载荷f (t)可以得到激励力所做功W_f为

${W_{\text{f}}} = f(t)\int_0^{2{\text{π }}} {\int_0^L {\left( {{f_u}u + {f_v}v + {f_w}w} \right)} } {\text{d}}x{\text{d}}\theta$

(11)

其中，f_u、f_ν和f_w分别为基础激励在u、ν、w 3个方向的分量系数，由于受力在圆锥壳的径向，因此需要通过半锥角α对母线(L)的斜度进行等效纠正，取值为

${f_u} = \sin \alpha {f_v} = \sin \theta {f_w} = \cos \theta$

(12)

需要说明的是，当不考虑半锥角α与母线(L)的斜度问题时，f_u、f_ν和f_w取1即可。

纤维增强复合薄壁圆锥壳动能T用下式表示：

$T=\frac{\rho h}{2}{\displaystyle {\int }_{0}^{L}{\displaystyle {\int }_{0}^{2{\text{π}} }\left[{\left(\frac{\partial u}{\partial t}\right)}^{2}+{\left(\frac{\partial v}{\partial t}\right)}^{2}+{\left(\frac{\partial w}{\partial t}\right)}^{2}\right]}}{R}_{0} \text{d}\theta \text{d}x$

(13)

其中，R₀=(R₁+R₂)/2。

纤维增强复合薄壁圆锥壳应变能U表示为

$U = \frac{1}{2}\int_0^L {\int_0^{2{\text{π }}} {{{\left[ \varepsilon \right]}^{\rm{T}}}\left[ S \right]} } {\text{ }}\left[ \varepsilon \right]{R_0}{\text{d}}x{\text{d}}\theta$

(14)

其中，[ε]为应变向量，其公式为

$\left[ \varepsilon \right] = \left[ {{\varepsilon _x},{\varepsilon _\theta },{\gamma _{x\theta }},{X_x},{X_\theta },{X_{x\theta }}} \right]$

(15)

S为薄膜刚度矩阵，定义如下：

${\boldsymbol{S}} = \left[ {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}} \\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}} \\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}} \\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}} \\ {{B_{12}}}&{{B_{22}}}&{{B_{26}}}&{{D_{12}}}&{{D_{22}}}&{{D_{26}}} \\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right]$

(16)

其中，矩阵S中的元素计算公式为

$\left\{ \begin{aligned} & {{A_{ij}} = \sum\limits_{k = 1}^N {\bar Q_{ij}^k} \left( {{h_k} - {h_{k + 1}}} \right)} \\ & {{B_{ij}} = \frac{1}{2}\sum\limits_{k = 1}^N {\bar O_{ij}^k} \left( {h_k^2 - h_{k + 1}^2} \right)} \\ & {{D_{ij}} = \frac{1}{3}\sum\limits_{k = 1}^N {\bar O_{ij}^k} \left( {h_k^3 - h_{k + 1}^3} \right)} \end{aligned} \right.$

式中：A_ij、B_ij和D_ij分别为拉伸、耦合与弯曲矩阵；h_k为上下平面到参考平面的距离。

将面位移分量方程式(3)和应力-应变公式(5)代入到外载荷做功公式(11)、动能公式(13)、应变能公式(14)中，可以得到复合薄壳的最大动能、最大应变能和最大外载荷做功分别为T_max、U_max和W_{f max}。

继续推导具有外部激励情况下的拉格朗日能量函数，其表达式为

$\varPi = {T_{\max }} + {W_{{\rm{f}}\max }} - {U_{{\max}}}$

(17)

通过对拉格朗日函数求偏导为0，来计算梁函数参数大小：

$\frac{{\partial \varPi }}{{\partial A}} = \frac{{\partial \varPi }}{{\partial B}} = \frac{{\partial \varPi }}{{\partial C}} = 0$

(18)

对式(18)进行求导，得到圆锥壳频域振动方程：

$\left( {{\boldsymbol{K}} + {\rm{i}}{\boldsymbol{C}} - {\omega ^2}{\boldsymbol{M}}} \right){\boldsymbol{a}} = {\boldsymbol{F}}$

(19)

其中：K、C和M分别为纤维增强复合薄壁圆锥壳的刚度矩阵、阻尼矩阵和质量矩阵；位移向量a=(A, B, C)^T为梁函数系数的具体解；F为激振力向量。令式(1)、阻尼矩阵C和激振力向量F为0时，式(19)变为

$\left( {{\boldsymbol{K}} - {\omega ^2}{\boldsymbol{M}}} \right){\boldsymbol{a}} = 0$

(20)

对式(19)进行求解，即可获得结构的固有频率ω。

通过求解方程(20)中的位移向量a，将其代入式(3)中，可以获得复合薄壳自身的振动响应ω₀。

根据模态叠加法，计算的振动响应结果为结构自身的振动响应ω₀及基础激励y(t)的位移之和。因此，将复合薄壳的振动响应ξ (x, θ, t)可以表示为

$\xi (x,\theta ,t) = y(t) + {w_0}(x,\theta ,t)$

(21)

式(21)给出了基础激励下的纤维增强复合薄壁圆锥壳的绝对振动响应求解的表达式；因此，在确定式(1)和式(3)的情况下，可以进行任意一点的响应进行计算及预测。

2. 基础激励下的FRCTCS振动响应计算原理与流程

2.1 计算原理

由于在实验样件制备的过程中，会产生诸多不可控因素，例如树脂基铺设的不均匀性、纤维布质量的优劣和制作工艺中产生的误差等问题；这些问题都会使结构的材料参数产生偏差。其中，纤维增强复合薄壁圆锥壳的损耗因子与振动响应密切相关。因此，准确确定损耗因子是能否准确分析振动响应的关键。

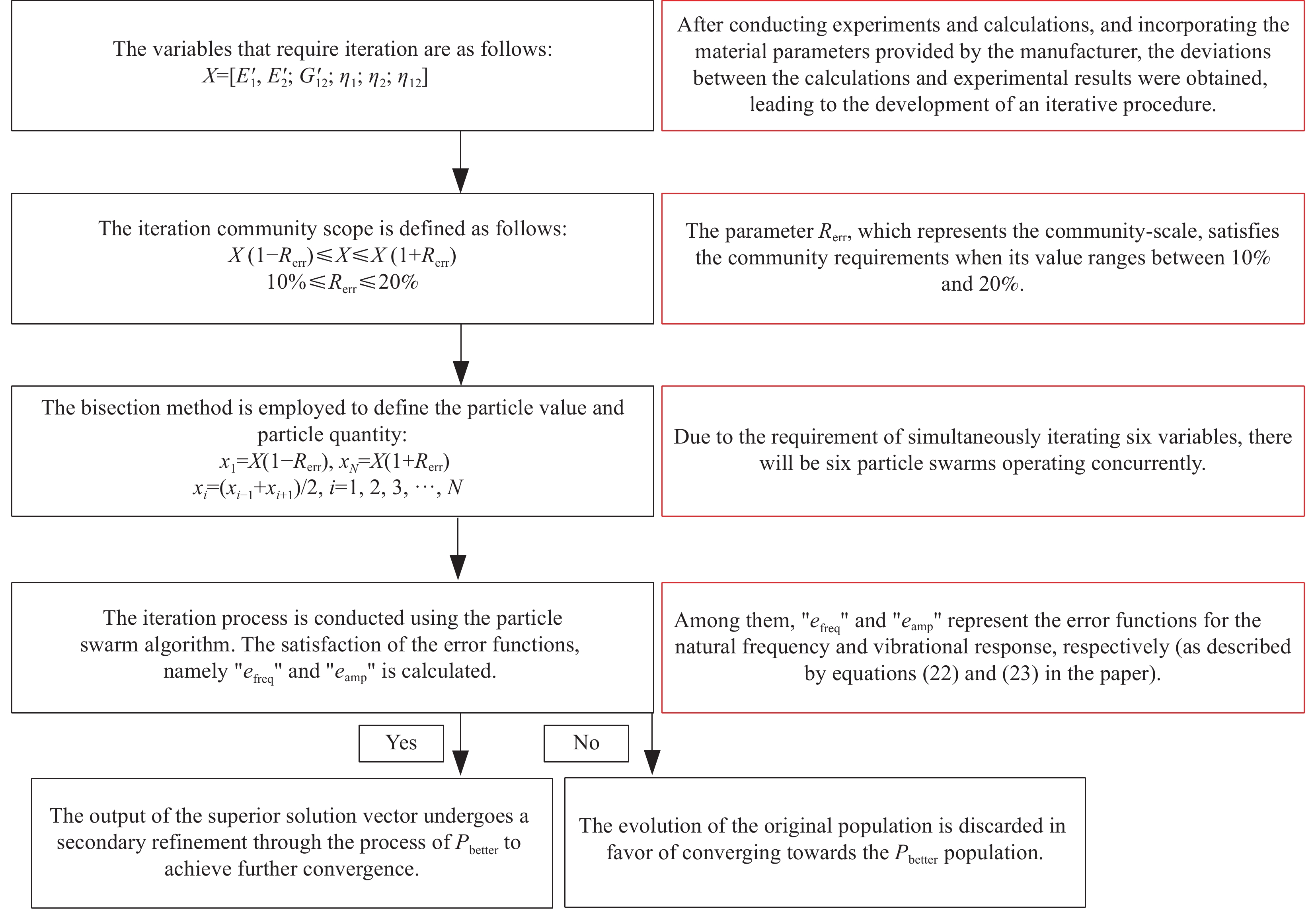
首先，通过理论建模计算结构的各阶固有频率，同时通过模态测试获得相应的固有频率；接着，对理论模型的材料参数进行二分粒子群迭代修正，使固有频率的误差满足误差函数e_freq，其具体的表达式为

${{e} _{{\text{freq}}}} = \sum\limits_{i = 1}^{{R_{{\text{mode}}}}} {\frac{{\left| {\Delta {f_i}} \right|}}{{{f_i}}}}$

(22)

同理，定义振动响应误差函数为e_amp：

${{e}_{{\text{amp}}}}{ = }\sum\limits_{{i = 1}}^{{{R}_{{\text{mode}}}}} {\frac{{\left| {{\Delta }{{M}_{i}}} \right|}}{{{{M}_{i}}}}}$

(23)

其中：Δf_i为计算与测试固有频率之差；f_i为计算的固有频率值；ΔM_i为计算与测试振动响应之差；M_i为计算振动响应值；R_mode为模态阶次。

根据厂家所提供的材料参数值E' ₁、E' ₂、G' ₁₂为基础，定义二分粒子群迭代法的迭代群落范围如下：

$\left\{ \begin{gathered} E_1^0(1 - {R_{{\text{err}}}}) \leqslant E_1' \leqslant E_1^0(1 + {R_{{\text{err}}}}) \\ E_2^0(1 - {R_{{\text{err}}}}) \leqslant E_2' \leqslant E_2^0(1 + {R_{{\text{err}}}}) \\ G_{12}^0(1 - {R_{{\text{err}}}}) \leqslant G_{12}' \leqslant G_{12}^0(1 + {R_{{\text{err}}}}) \\ \end{gathered} \right.$

(24)

其中，R_err为定义粒子群群落范围的参数，其取值在10%~20%之间可以满足粒子群群落范围要求。

在计算固有特性时，为了满足固有特性误差函数(e_freq≤1%~3%)要对材料参数进行二分迭代修正计算。

首先，定义二分粒子群：

$\begin{split} &{X_i} = ({x_1},{x_2},{x_3}, \cdot \cdot \cdot ,{x_i}){\text{ }} \\ & {x_i} = \frac{{({x_{i - 1}} + {x_{i + 1}})}}{2}{\text{ }},i = 1,2,3, \cdot \cdot \cdot ,N \\ & X = ({E_1},{E_2},{G_{12}}) \end{split}$

(25)

其中：x₁=X(1−R_err)、x_N=X(1+R_err)；N为粒子群元素数量且为奇数，一般定义N为9即可获得较高的迭代精度。

其次，将材料参数同时进行迭代计算，将基于二分法形成的粒子群元素代入式(2)进行计算，将满足误差函数e_freq的较优个体极值计为

${P_{{\text{better}}}} = ({p_{i1}},{p_{i2}},{p_{i3}}, \cdot \cdot \cdot ,{p_{iD}}),{\text{ }}i = 1,2,3, \cdot \cdot \cdot ,N$

(26)

其中，P_better是一个D维向量，具体元素数量由满足误差函数e_freq的x_i的数量决定。

最后，当P_better的元素数量大于1时，将定义的误差函数值缩小，当向量P_better中的元素数量唯一时，跳出计算，并定义唯一元素为P_best，即为二分粒子群算法所获得的材料参数最优解。

需要说明的是，由于振动响应与损耗因子密切相关，因此在进行材料参数修正后，需要使用二分粒子群迭代法对式(2)中的损耗因子(η₁, η₂, η₁₂)进行迭代修正；与材料参数不同的是，在定义二分粒子群时，由于损耗因子没有明确的参考值，需要定义η_j ¹ (j=1, 2, 12)为0，并规定最大损耗因子 $\eta _{j\;\max }^N$ 。针对损耗因子所定义的粒子群群落范围为

$\left\{ \begin{aligned} &\eta _1^1 \leqslant {\eta _1} \leqslant \eta _{1\max }^N \\ & \eta _2^1 \leqslant {\eta _2} \leqslant \eta _{2\max }^N \\ & \eta _{12}^1 \leqslant {\eta _{12}} \leqslant \eta _{12\max }^N \end{aligned} \right.$

(27)

针对损耗因子定义的二分粒子群为

$\begin{split} &{Y_i} = ({y_1},{y_2},{y_3}, \cdot \cdot \cdot ,{y_i}){\text{ }} \\ & {y_i} = \frac{{({y_{i - 1}} + {y_{i + 1}})}}{2},{\text{ }}i = 1,2,3, \cdot \cdot \cdot ,N \\ & Y = ({\eta _1},{\eta _2},{\eta _{12}}) \end{split}$

(28)

同上，将定义的二分粒子群(26)代入式(2)进行整个模型的迭代计算，在满足误差函数e_amp后，即可得到二分迭代粒子群算法所获得的损耗因子最优解。

2.2 计算流程

在本部分，利用Matlab软件，编写了相应的计算程序，并提出了纤维增强复合材料圆锥壳的振动响应流程，可分为以下几个关键步骤：

(1) 输入纤维增强复合材料圆锥壳的母线长度L、大半径端R₁、半锥角α和纤维铺层角度β等几何参数后，继续输入纤维纵向弹性模量为E₁、纤维横向弹性模量为E₂、剪切模量G₁₂、泊松比μ₁₂等材料参数；

(2) 将基础激励表达式(1)、基于梁函数法来表示振型函数(3)、应力-应变表达式(5)分别代入激励做功公式(11)、动能表达式(13)、应变能表达式(14)后，令谐波分量为1，即得到激励做功公式W_fmax、最大动能T_max、最大应变能U_max；

(3) 采用Ritz法求解固有频率。将最大动能T_max、最大应变能U_max代入拉格朗日能量函数公式(17)，根据Ritz法，将П对所有参数求偏导，得到了刚度矩阵与质量矩阵的特征方程(19)，令阻尼矩阵C和激振力向量F为0，得到公式(20)，通过对方程(21)进行求解，即可得到纤维增强复合薄壁圆锥壳得固有频率；

(4) 求解纤维增强复合材料薄壁圆锥壳的振动响应。基于复模量法和模态叠加法，在考虑基础激励表达式(1)与表达式(19)中的阻尼矩阵C、激振力向量F的情况下进行求解，得到振动响应ω₀，ω₀与基础激励y(t)进行叠加得到在基础激励下的纤维增强复合材料圆锥壳的振动响应ξ(x, θ, t)；

(5) 进行单点激励多点响应实验，用以测试纤维增强复合材料薄壁圆锥壳的固有频率，并根据固有频率结果定义误差函数(22)；

(6) 根据厂家提供的材料参数来定义材料参数的二分粒子群的迭代群落(24)，选定合适的粒子数量N后，定义二分迭代粒子群(25)，将群落中的粒子代入式(2)进行迭代计算，输出满足误差函数(22)的较优个体向量(26)；最后，通过更改误差函数来完成固有频率的二分粒子群迭代修正并输出材料参数的最优个体P_best；

(7) 进行基础激励多点响应实验，用以测试纤维增强复合薄壁圆锥壳的振动响应，并根据振动响应结果定义误差函数(23)；

(8) 定义损耗因子的二分粒子群的迭代群落(27)，并重复步骤(6)，即可完成对振动响应的二分粒子群迭代修正并输出损耗因子的最优个体。

基于二分粒子群迭代法的修正流程如图2所示，需要说明的是，二分法主要用于规定粒子群落中的粒子数量及取值。

图 2 二分粒子群迭代法的计算流程

Figure 2. Computational process of the binary particle swarm iteration method

E' ₁, E' ₂, G' ₁₂—Elastic modulus in different directions; η₁, η₂, η₁₂—Loss factor in different directions

下载: 全尺寸图片幻灯片

3. 实验验证

3.1 实验对象及测试系统

为了验证本文计算方法的准确性，本文将以TC300碳纤维/环氧树脂基纤维增强复合薄壁圆锥壳为研究对象，搭建相应的测试系统，对其进行模态和基础激励响应测试。实验件母线长度L为150 mm，大半径端R₁为132.5 mm，厚度h为3 mm，半锥角α=30°，密度为ρ=1570 kg/m³，纤维纵向弹性模量为E₁=120 GPa，纤维横向弹性模量为E₂=8.5 GPa，剪切模量G₁₂=4.74 GPa，泊松比μ₁₂=0.3。此类型复合薄壳为对称正交铺设，铺层方式为[+45°/−45°]₁₂，共有24层，并且每一层具有相同的厚度和纤维体积分数。

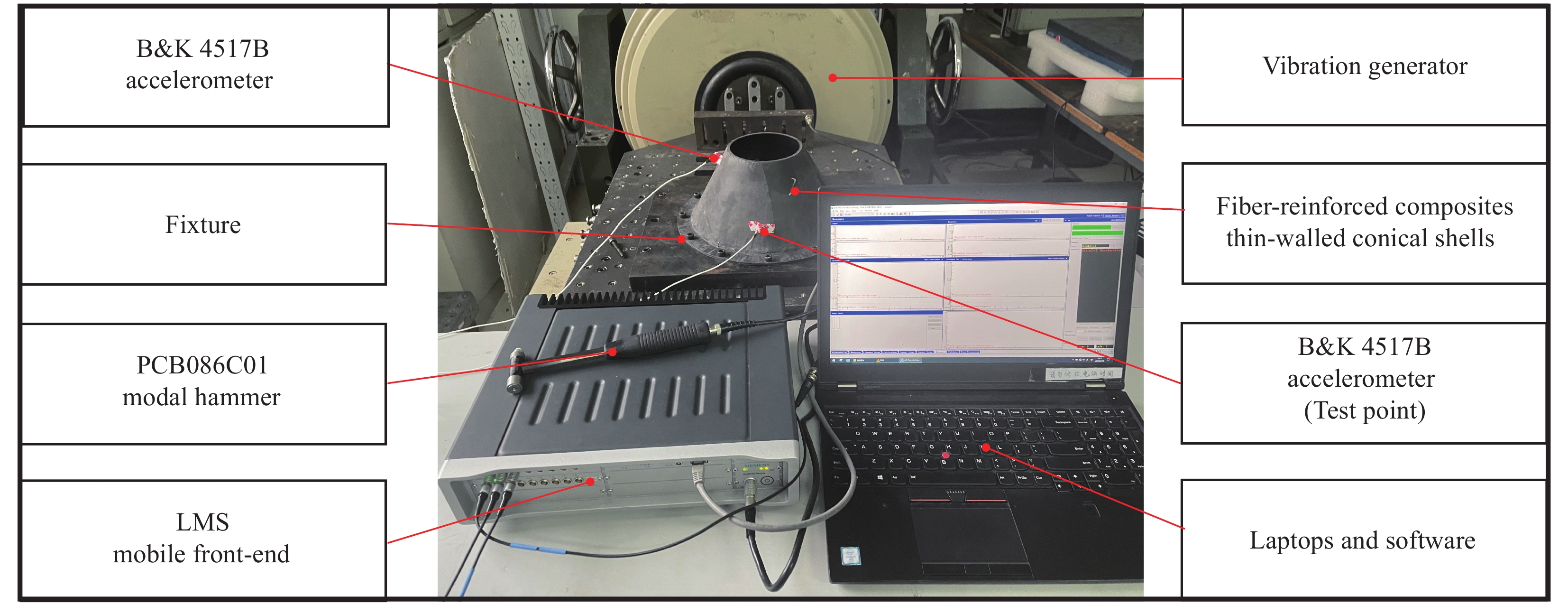
图3为所搭建的基础激励下纤维增强复合薄壁圆锥壳的振动特性测试系统；在测试之前，需要进行如下参数的设置：(I) 频率范围：20~3000 Hz；(II) 频率分辨率：0.1 Hz；(III) 窗函数：Hanning窗；(IV) 基础激励幅度：1 g；(V) 扫频速度：0.5 Hz/s。

图 3 纤维增强复合材料薄壁圆锥壳幅频测试系统

Figure 3. Amplitude frequency test system for thin-walled conical shells of fiber-reinforced composites

下载: 全尺寸图片幻灯片

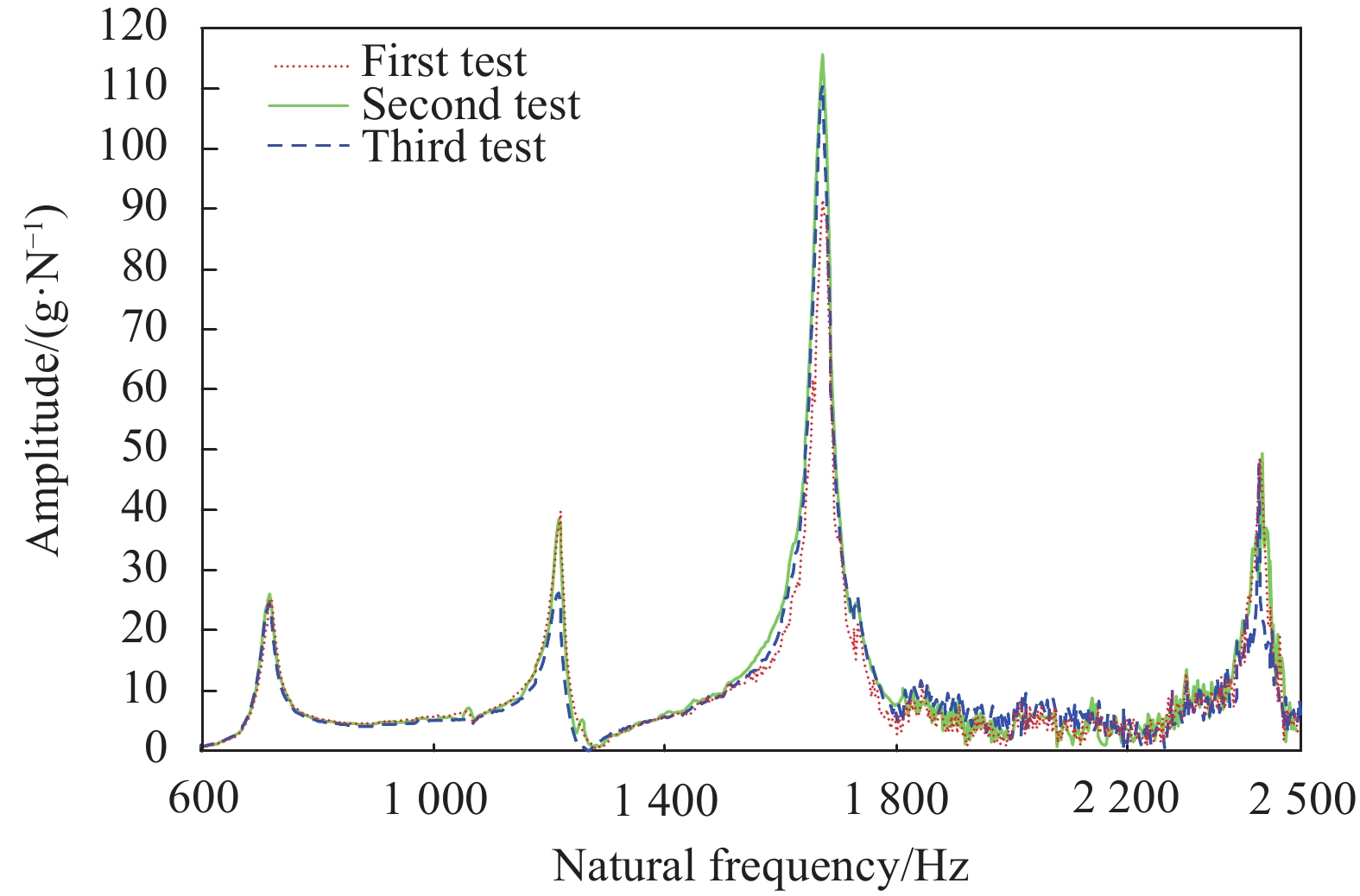
实验时，首先需要通过力锤，对其进行模态测试，以便于确定各阶响应所在的频率区间；模态测试中，本文采用单点激励多点响应的方法，利用两个B&K 4517B加速度传感器来进行对比验证。同时，为了减小误差，进行3次有效锤击测试。

在确定好固有特性与响应点后，开始进行振动响应测试：由金盾EM-1000F电磁振动台提供1 g的基础激励；利用B&K 4517B轻质加速度传感器与LMS16通道便携式数据采集仪进行响应信号的采集，再通过安装有LMS Test.Lab 14A软件的笔记本电脑对数据进行分析和处理，最终获得结构的实验测试结果。另外需要说明的是，被测试的TC300碳纤维/环氧树脂基纤维增强复合薄壁圆锥壳在制造时，其末端预留了安装边和安装孔，可通过多个M8螺栓固定在独立的夹具上，确保其与理论中呈现的大半径端固定、小半径端与沿母线方向自由的边界条件一致。

3.2 测试结果与验证对比

为了验证本文计算方法的正确性，将测试结果与本文提出方法的理论计算结果进行对比，图4给出了模态实验所测得的固有频率-加速度曲线。

图 4 纤维增强复合材料薄壁圆锥壳的固有频率-加速度曲线

Figure 4. Intrinsic frequency-acceleration curves of fiber-reinforced composite conical shells

下载: 全尺寸图片幻灯片

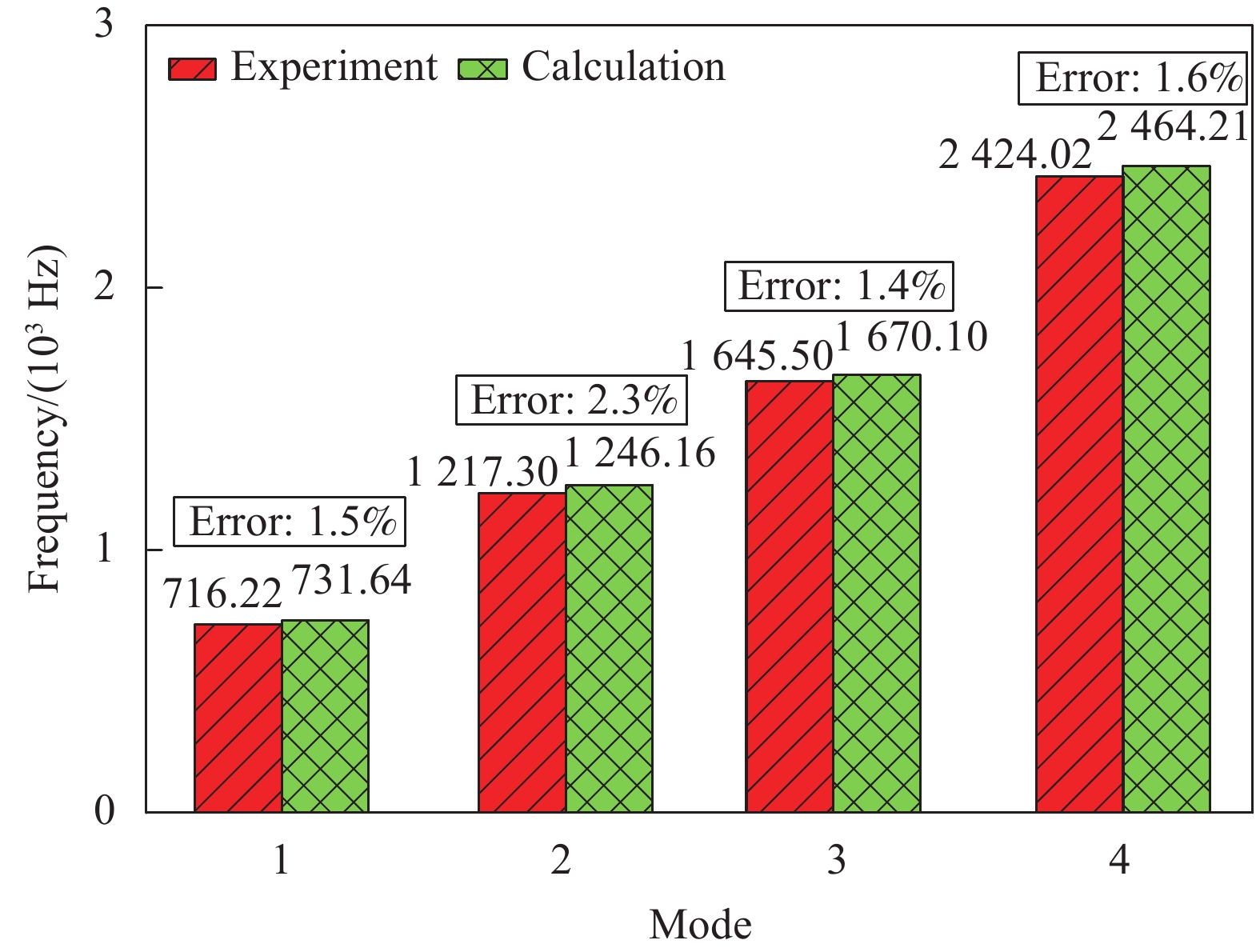
在准确测试获得了纤维增强复合材料薄壁圆锥壳的前4阶固有频率的基础上，设定固有频率误差函数e_freq≤3%，设定群落参数R_err为10%，开始进行材料参数的迭代修正。图5给出了实验测试的固有频率值与经过9次修正后的计算固有频率值及其误差。厂家提供的材料参数、经过二分粒子群迭代法修正计算后的材料参数和损耗因子如表2所示。

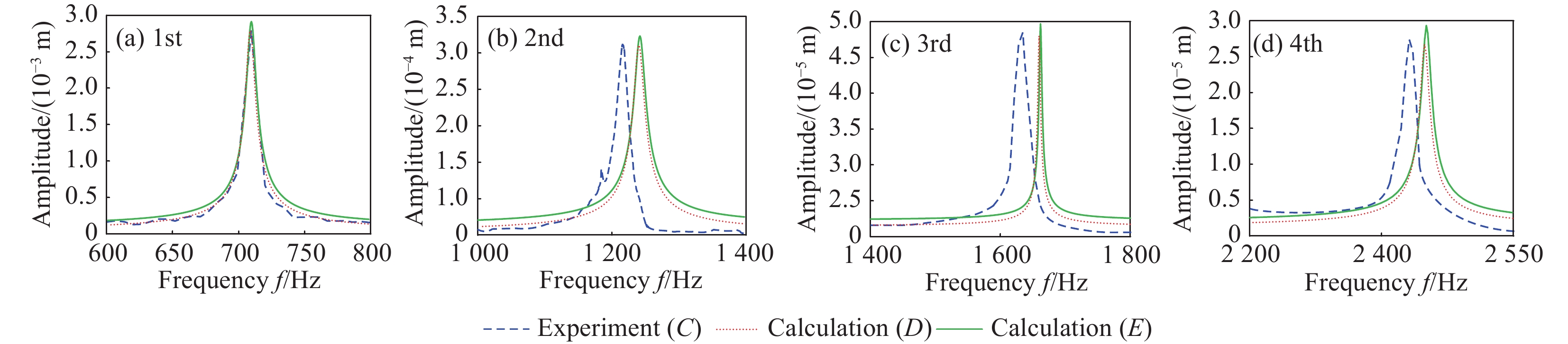
表 2 修正前后的材料参数、损耗因子

Table 2. Material parameters before and after correction, loss factor

Before iterative calculation			After iterative calculation			Loss factor
Material parameters/GPa			Material parameters/GPa			Loss factor
E'₁	E'₂	G'₁₂	E₁	E₂	G₁₂	η₁	η₂	η₁₂
120	8.5	4.74	114.9844351	8.1447308	4.5418851	0.0047909	0.0038328	0.0043119

下载: 导出CSV

| 显示表格

图 5 纤维增强复合材料薄壁圆锥壳的固有频率及计算误差

Figure 5. Natural frequencies and computational inaccuracies of fiber-reinforced composite thin-walled conical shells

下载: 全尺寸图片幻灯片

将材料参数进行准确修正后，结合实验测试结果，可以精确的确定纤维增强复合材料圆锥壳的振动响应扫频范围；在准确测定振动响应后，设定振动响应误差函数e_amp≤3%、损耗因子最大值 $\eta _{j\;\max }^N$ 为0.005，在程序中设定好后，开始对损耗因子进行迭代求解。图6给出了实验测试的频率-响应曲线C、经过迭代计算的频率-响应曲线D、在确定材料参数和损耗因子后，不考虑式(9)进行母线与激励角度偏移修正的频率-响应曲线E。为了使对比结果更加明显，表3给出了实验测试的振动响应值C、经过迭代计算的振动响应结果D、确定损耗因子的计算结果E与误差。

图 6 实验和计算获得的纤维增强复合材料薄壁圆锥壳的前4阶频率-响应曲线

Figure 6. Experimentally and computationally obtained frequency-response curves of the first 4th orders of thin-walled conical shells of fiber-reinforced composites

下载: 全尺寸图片幻灯片

通过表2可以看出，由于制作工艺等不可控误差的存在，实验样件的材料参数与理论的材料参数具有一定的偏差，因此进行材料参数与损耗因子的迭代修正是尤为重要的；通过图5的对比分析可以看出，在经过修正后，实验与计算所获的纤维增强复合材料薄壁圆锥壳固有频率的误差分别在1.4%~2.3%之间，数值变化趋势基本一致，从而验证本文所提出的方法在计算固有特性时的正确性。通过表3可以看出，在经过材料参数与损耗因子修正后，实验振动响应C与计算振动响应D的误差在1.8%~3.0%之间；实验振动响应C与计算振动响应E的误差在2.5%~8.3%之间；因此，在理论建模时考虑母线与基础激励载荷方向存在夹角是尤为重要的。

表 3 实验和计算获得的纤维增强复合材料薄壁圆锥壳的前4阶响应值及误差

Table 3. Experimentally and computationally obtained response values and errors of the first 4th order for thin-walled conical shells of fiber-reinforced composites

Mode	Amplitude/m			Error/%
Mode	Experiment (C)	Calculation (D)	Calculation (E)	\|C−D\|/D	\|C−E\|/E
1	2.71×10⁻³	2.66×10⁻³	2.86×10⁻³	1.8	5.2
2	3.11×10⁻⁴	3.06×10⁻⁴	3.19×10⁻⁴	1.6	2.5
3	4.89×10⁻⁵	4.76×10⁻⁵	4.99×10⁻⁵	2.6	4.3
4	2.73×10⁻⁵	2.65×10⁻⁵	2.98×10⁻⁵	3.0	8.3

下载: 导出CSV

| 显示表格

4. 结论

采用理论与实践相结合的方式，对基础激励下的纤维增强复合材料薄壁圆锥壳的振动响应问题进行了研究，得到的结论如下：

(1) 实验振动响应C与考虑激励载荷与母线夹角的计算振动响应D的误差在1.8%~3.0%之间。实验振动响应C与不考虑激励载荷与母线夹角的计算振动响应E的误差在2.5%~8.3%之间。这主要是由于式(11)中的u、ν、w这3个方向的分量系数f_u、f_ν和f_w值有所变化引起的。由此可见，在理论建模与计算中，需要充分考虑基础激励载荷与圆锥壳母线之间的角度关系；

(2) 由于制作工艺等不可控误差的存在，实验样件材料参数与理论参数具有一定的偏差，因此需要通过迭代计算的到较精确的材料参数与损耗因子；通过修正计算结果与实验，固有特性的误差在1.4%~2.3%之间，振动响应的误差在1.8%~3.0%之间，验证了本文所提出的二分粒子群迭代法具有精度高、通用性强等特点，适用于工程结构的核心部件进行振动特性的研究与材料参数的修正，具有较强的工程实际意义；

(3) 将经典板壳理论、复模量法进行结合，在建立精确的分析模型后，考虑载荷方向与母线的夹角、纤维铺层方向与x轴的夹角，利用能量法与模态叠加法进行振动响应的求解，对于不同材料的圆锥壳结构振动特性的分析与预测具有理论指导意义。

图 1 纤维增强复合薄壁圆锥壳理论模型

Figure 1. Theoretical model for fiber-reinforced composite thin-walled conical shells

下载: 全尺寸图片幻灯片

图 2 二分粒子群迭代法的计算流程

Figure 2. Computational process of the binary particle swarm iteration method

E' ₁, E' ₂, G' ₁₂—Elastic modulus in different directions; η₁, η₂, η₁₂—Loss factor in different directions

下载: 全尺寸图片幻灯片

图 3 纤维增强复合材料薄壁圆锥壳幅频测试系统

Figure 3. Amplitude frequency test system for thin-walled conical shells of fiber-reinforced composites

下载: 全尺寸图片幻灯片

图 4 纤维增强复合材料薄壁圆锥壳的固有频率-加速度曲线

Figure 4. Intrinsic frequency-acceleration curves of fiber-reinforced composite conical shells

下载: 全尺寸图片幻灯片

图 5 纤维增强复合材料薄壁圆锥壳的固有频率及计算误差

Figure 5. Natural frequencies and computational inaccuracies of fiber-reinforced composite thin-walled conical shells

下载: 全尺寸图片幻灯片

图 6 实验和计算获得的纤维增强复合材料薄壁圆锥壳的前4阶频率-响应曲线

Figure 6. Experimentally and computationally obtained frequency-response curves of the first 4th orders of thin-walled conical shells of fiber-reinforced composites

下载: 全尺寸图片幻灯片

表 1 梁函数系数的取值

Table 1 Values of beam function coefficients

m	λ_m	σ_m
1	1.87510	0.734096
2	4.69409	1.018467
3	7.85476	0.999224
Note: λ_m and σ_m—Coefficients of the beam functions.

下载: 导出CSV

表 2 修正前后的材料参数、损耗因子

Table 2 Material parameters before and after correction, loss factor

Before iterative calculation			After iterative calculation			Loss factor
Material parameters/GPa			Material parameters/GPa			Loss factor
E'₁	E'₂	G'₁₂	E₁	E₂	G₁₂	η₁	η₂	η₁₂
120	8.5	4.74	114.9844351	8.1447308	4.5418851	0.0047909	0.0038328	0.0043119

下载: 导出CSV

表 3 实验和计算获得的纤维增强复合材料薄壁圆锥壳的前4阶响应值及误差

Table 3 Experimentally and computationally obtained response values and errors of the first 4th order for thin-walled conical shells of fiber-reinforced composites

Mode	Amplitude/m			Error/%
Mode	Experiment (C)	Calculation (D)	Calculation (E)	\|C−D\|/D	\|C−E\|/E
1	2.71×10⁻³	2.66×10⁻³	2.86×10⁻³	1.8	5.2
2	3.11×10⁻⁴	3.06×10⁻⁴	3.19×10⁻⁴	1.6	2.5
3	4.89×10⁻⁵	4.76×10⁻⁵	4.99×10⁻⁵	2.6	4.3
4	2.73×10⁻⁵	2.65×10⁻⁵	2.98×10⁻⁵	3.0	8.3

下载: 导出CSV

参考文献(23)

[1]	曹志远. 板壳振动理论[M]. 北京: 中国铁道出版社, 1989. CAO Zhiyuan. Vibration theory of plate and shell[M]. Beijing: China Railway Publishing House, 1989(in Chinese).
[2]	QATU M S. Vibration of laminated shells and plates[M]. Amsterdam: Elsevier, 2004.
[3]	邢誉峰, 刘波. 板壳自由振动的精确解[M]. 北京: 科学出版社, 2015. XING Yufeng, LIU Bo. An exact solution for the free vibration of plates and shells[M]. Beijing: Science Press of Beijing, 2015(in Chinese).
[4]	ZOU Y, TONG L, STEVEN G P. Vibration-based model-dependent damage (delamination) identification and health monitoring for composite structures-A review[J]. Journal of Sound and Vibration, 2000, 230(2): 357-378.
[5]	李晖, 孙伟, 许卓, 等. 纤维增强复合薄板振动测试与分析方法[M]. 北京: 机械工业出版社, 2020. LI Hui, SUN Wei, XU Zhuo, et al. Vibration test and analysis method of fiber reinforced composite sheet[M]. Beijing: China Machine Press, 2020(in Chinese).
[6]	王爱勤. 圆锥(柱)体在复杂载荷作用下振动响应的计算[J]. 西安公路交通大学学报, 1998,18(3):66-69. WANG Aiqin. Vibration responses of conical body under complex loads[J]. Journal of Xi'an Highway University,1998,18(3):66-69(in Chinese).
[7]	杨绍武. 复杂载荷作用下功能梯度圆锥壳的非线性动力学研究[D]. 北京: 北京信息科技大学, 2015. YANG Zhaowu. Study on nonlinear dynamics of functionally graded conical shells under complex loads[D]. Beijing: Beijing Information Science and Technology University, 2015(in Chinese).
[8]	DEY S, MUKHOPADHYAY T, KHODAPARAST H H, et al. Stochastic natural frequency of composite conical shells[J]. Acta Mechanica,2015,226(8):2537-2553. DOI: 10.1007/s00707-015-1316-4
[9]	ANSARI R, TORABI J. Numerical study on the buckling and vibration of functionally graded carbon nanotube-reinforced composite conical shells under axial loading[J]. Composites Part B: Engineering,2016,95:196-208. DOI: 10.1016/j.compositesb.2016.03.080
[10]	MERCAN K, BALTACIOGLU A K, CIVALEK Ö. Free vibration of laminated and FGM/CNT composites annular thick plates with shear deformation by discrete singular convolution method[J]. Composite Structures,2018,186:139-153. DOI: 10.1016/j.compstruct.2017.12.008
[11]	谢坤. 纵向激励下桨—轴—艇耦合模型声振响应半解析计算方法及特性研究[D]. 武汉: 华中科技大学, 2018. XIE Kun. Study on semi-analytic methods and vibro-acoustic characteristics of coupled propeller-shaft-hull structures subjected to longitudinal excitations[D]. Wuhan: Huazhong University of Science and Technology, 2018(in Chinese).
[12]	张永强. 薄壁圆锥壳构件的动力学相似设计方法研究[D]. 沈阳: 东北大学, 2020. ZHANG Yongqiang. Research on dynamic similarity design method for thin-walled conical shell structures[D]. Shenyang: Northeastern University, 2020(in Chinese).
[13]	LEI Z X, LIEW K M, YU J L. Free vibration analysis of functionally graded carbon nanotube-reinforced composite plates using the element-free kp-Ritz method in thermal environment[J]. Composite Structures,2013,106:128-138. DOI: 10.1016/j.compstruct.2013.06.003
[14]	LI H, ZHOU Z X, SUN H, et al. Theoretical study on the influence of hard coating on vibration characteristics of fiber-reinforced composite thin shell[J]. Coatings,2018,8(3):87. DOI: 10.3390/coatings8030087
[15]	HEIDARI SOURESHJANI A, TALEBITOOTI R, TALEBITOOTI M. A semi-analytical approach on the effect of external lateral pressure on free vibration of joined sandwich aerospace composite conical-conical shells[J]. Aerospace Science and Technology,2020,99:105559. DOI: 10.1016/j.ast.2019.105559
[16]	ZHANG H, ZHU R P, SHI D Y, et al. A simplified plate theory for vibration analysis of composite laminated sector, annular and circular plate[J]. Thin-Walled Structures,2019,143:106252. DOI: 10.1016/j.tws.2019.106252
[17]	KAMALOO A, JABBARI M, TOOSKI M Y, et al. Nonlinear free vibrations analysis of delaminated composite conical shells[J]. International Journal of Structural Stability and Dynamics,2020,20(1):2050010. DOI: 10.1142/S0219455420500108
[18]	YANG S W, ZHANG W, HAO Y X, et al. Nonlinear vibrations of FGM truncated conical shell under aerodynamics and in-plane force along meridian near internal resonances[J]. Thin-Walled Structures,2019,142:369-391. DOI: 10.1016/j.tws.2019.04.024
[19]	SAFARPOUR M, RAHIMI A R, ALIBEIGLOO A. Static and free vibration analysis of graphene platelets reinforced composite truncated conical shell, cylindrical shell, and annular plate using theory of elasticity and DQM[J]. Mechanics Based Design of Structures and Machines,2020,48(4):496-524. DOI: 10.1080/15397734.2019.1646137
[20]	REZAIEE-PAJAND M, SOBHANI E, MASOODI A R. Free vibration analysis of functionally graded hybrid matrix/fiber nanocomposite conical shells using multiscale method[J]. Aerospace Science and Technology,2020,105:105998. DOI: 10.1016/j.ast.2020.105998
[21]	MAJI P, SINGH B N. Free vibration responses of 3D braided rotating cylindrical shells based on third-order shear deformation[J]. Composite Structures,2021,260:113255. DOI: 10.1016/j.compstruct.2020.113255
[22]	SOBHANI E, MASOODI A R, AHMADI-PARI A R. Vibration of FG-CNT and FG-GNP sandwich composite coupled conical-cylindrical-conical shell[J]. Composite Structures,2021,273:114281. DOI: 10.1016/j.compstruct.2021.114281
[23]	SHI X J, ZUO P, ZHONG R, et al. Vibration analysis of combined functionally graded cylindrical-conical shells coupled with annular plates in thermal environment[J]. Composite Structures, 2022, 294: 115738.

施引文献

资源附件(0)

长摘要

目的
纤维增强复合材料薄壁截锥壳相对于金属薄壁圆锥壳，具有质量轻、承载能力较好，可靠性高、绝缘性好等多种优点，目前正在被广泛应用于航空航天、海洋船舶、石油化工等重要工程领域。如液体航空发动机所使用的复合材料壳体燃烧室、深海无人探测器的复合材料耐高压壳体、船舶螺旋桨尾部舱段等，在复杂的工程应用环境中，这些壳体很容易受到基础激励载荷的作用，另外，应用结构的复杂性、应用环境的多样性使其振动响应的问题尤为突出。因此，研究基础激励下的纤维增强复合材料薄壁截锥壳的振动响应问题有着重要的工程及学术意义。
方法
针对纤维增强复合薄壁截锥壳的结构特点，考虑基础激励载荷方向与母线的夹角、纤维铺层方向与轴的夹角，利用板壳振动理论、复弹性模量等方法对所研究结构进行了理论建模。利用双向梁函数法表示振型函数，并通过能量法和模态叠加法对其固有特性和振动响应进行求解。为了验证模型的正确性，基于自行搭建的振动测试平台，以TC300/环氧树脂基纤维增强复合薄壁截锥壳为对象，进行了振动特性测试；通过实验测试结果与理论计算结果进行对比，来验证本文计算方法的准确性。
结果
在经过迭代计算修正后，实验与计算所获的纤维增强复合材料薄壁圆锥壳固有频率的最低误差为1.4%，数值变化趋势基本一致；在经过材料参数与损耗因子修正后，实验振动响应与计算振动响应的最低误差为1.8%。
结论
本文采用理论与实践相结合的方式，对基础激励下的纤维增强复合材料薄壁圆锥壳的振动响应问题进行了研究；得到的结论如下：(1) 以本文为例：实验振动响应与考虑激励载荷与母线夹角的计算振动响应的误差在1.8~3.0%之间；实验振动响应与不考虑激励载荷与母线夹角的计算振动响应的误差在2.5~8.3%之间；这主要是由于，，三个方向的分量系数，和值有所变化引起的。由此可见，在理论建模与计算中，需要充分考虑基础激励载荷与圆锥壳母线之间的角度关系。(2) 由于制作工艺等不可控误差的存在，实验样件材料参数与理论参数具有一定的偏差，因此需要通过迭代计算的到较为精确的材料参数与损耗因子；通过修正计算结果与实验，固有特性的误差在1.4~2.3%之间，振动响应的误差在1.8~3.0%之间，验证了本文所提出的二分粒子群迭代法具有精度高、通用性强等特点，适用于工程结构的核心部件进行振动特性的研究与材料参数的修正，具有较强的工程实际意义。(3) 本文将经典板壳理论、复模量法进行结合，在建立精确的分析模型后，考虑载荷方向与母线的夹角、纤维铺层方向与轴的夹角，利用能量法与模态叠加法进行振动响应的求解，对于不同材料的圆锥壳结构振动特性的分析与预测具有理论指导意义。

创新点

纤维增强复合薄壁截顶圆锥壳相比较于普通金属薄壁截顶圆锥壳，具有质量轻、承载压力大、抗冲击性能好、抗疲劳性能突出、绝缘性好等多种特点，目前正在被越来越多的应用于航空航天、船舶、海洋工程等重要领域。工程实际中存在大量该类型材料制成的典型薄壁圆锥壳构件，如航空发动机的复合材料机匣、海底深潜器用的复合材料耐压壳等，由于工作环境的复杂、工作环境越来越苛刻，其振动响应问题也越来越突出，由于振动响应超标而引发的碰撞、摩擦等问题也越来越受到人们的关注。因此，研究基础激励下纤维增强复合薄壁截顶圆锥壳的振动响应问题具有重要的工程学术意义。
本文将理论分析与实验测试相结合，针对纤维增强复合薄壁截锥壳的结构特点，考虑基础激励载荷方向与母线的夹角、纤维铺层方向与x轴的夹角，利用板壳振动理论、复弹性模量等方法对所研究的结构进行了理论建模。利用双向梁函数法表示振型函数，并通过能量法和模态叠加法对其固有特性和振动响应进行求解；在经过实验对比后，为了降低理论测试结果误差，开发了二分粒子群算法进行迭代修正，结果表明迭代修正后的理论模型结果更精准，两者的最大误差不超过3.0%，验证了本文理论模型与迭代方法的准确性；实验系统如图1所示；实验测试结果C、迭代修正计算结果D、未经母线夹角修正与迭代修正的结果E一并在图2中给出。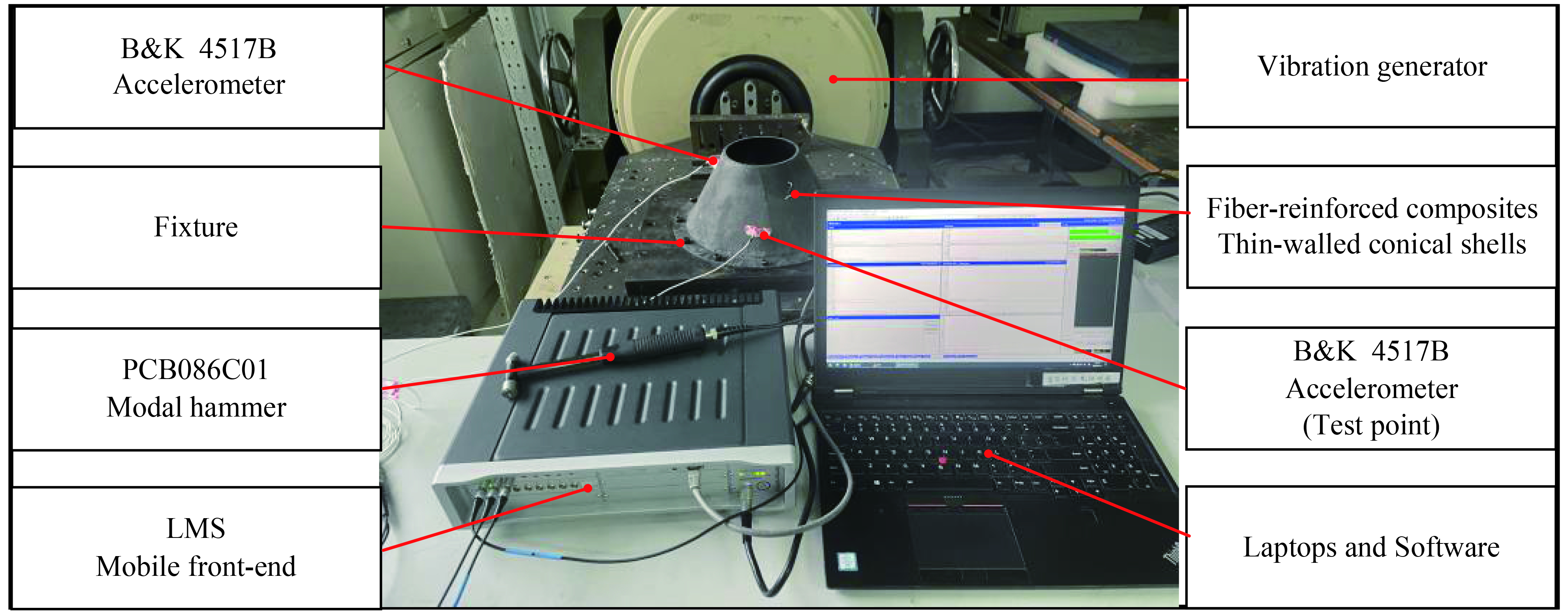
Amplitude frequency test system for thin-walled conical shells of fiber-reinforced composites
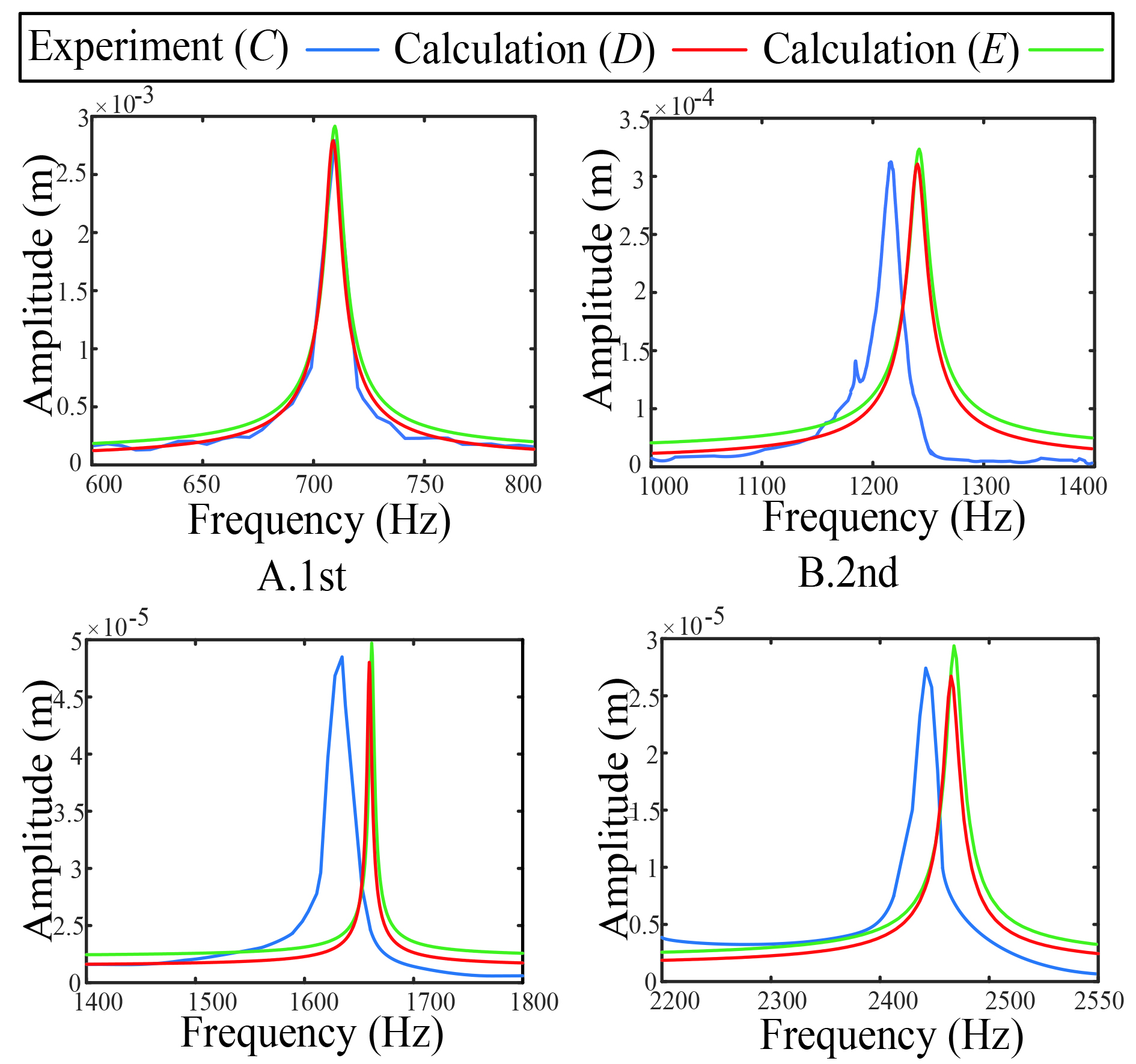
Experimentally and computationally obtained frequency-response curves of the first 4th orders of thin-walled conical shells of fiber-reinforced composites

图(6) / 表(3)

计量

文章访问数: 566
HTML全文浏览量: 224
PDF下载量: 23
被引次数: 0

1. 基础激励下的FRCTCS振动响应求解
2. 基础激励下的FRCTCS振动响应计算原理与流程
2.1 计算原理
2.2 计算流程
3. 实验验证
3.1 实验对象及测试系统
3.2 测试结果与验证对比
4. 结论

基于多层次迭代修正的纤维增强复合薄壁截顶圆锥壳振动响应分析

通讯作者: 李晖，博士，副教授，博士生导师，研究方向为复合结构减振降噪 E-mail: lh200300206@163.com

计量

出版历程

Vibration response analysis of fiber reinforced composite thin-walled truncated conical shell based on multilevel iterative correction

1. 基础激励下的FRCTCS振动响应求解

2. 基础激励下的FRCTCS振动响应计算原理与流程

2.1 计算原理

2.2 计算流程

3. 实验验证

3.1 实验对象及测试系统

3.2 测试结果与验证对比

4. 结 论

计量

出版历程

目录

1. 基础激励下的FRCTCS振动响应求解

2. 基础激励下的FRCTCS振动响应计算原理与流程

2.1 计算原理

2.2 计算流程

3. 实验验证

3.1 实验对象及测试系统

3.2 测试结果与验证对比

4. 结 论

通讯作者:
李晖，博士，副教授，博士生导师，研究方向为复合结构减振降噪　E-mail: lh200300206@163.com

4. 结论

4. 结论