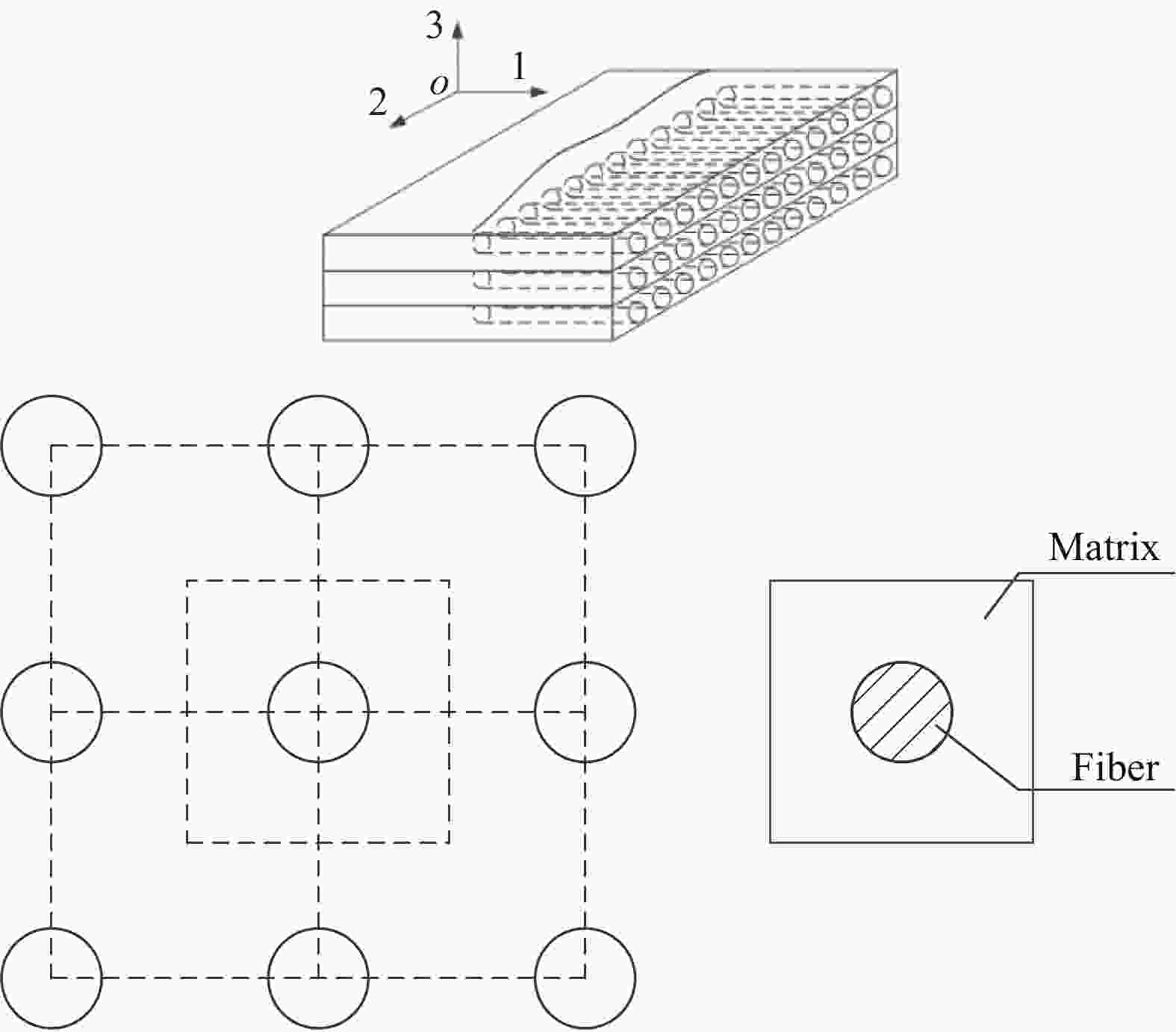
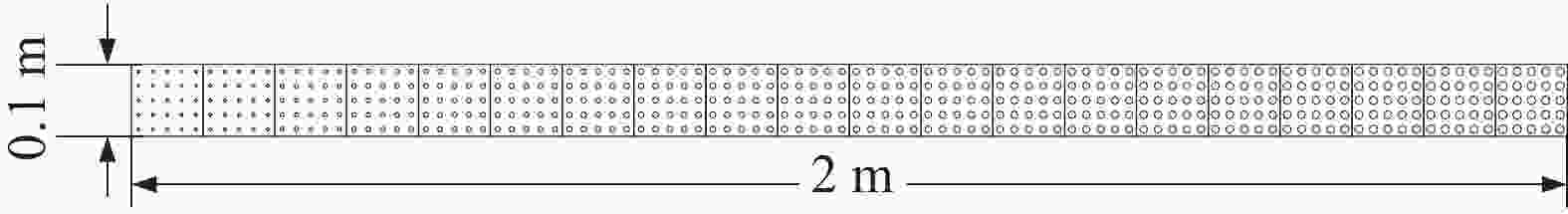
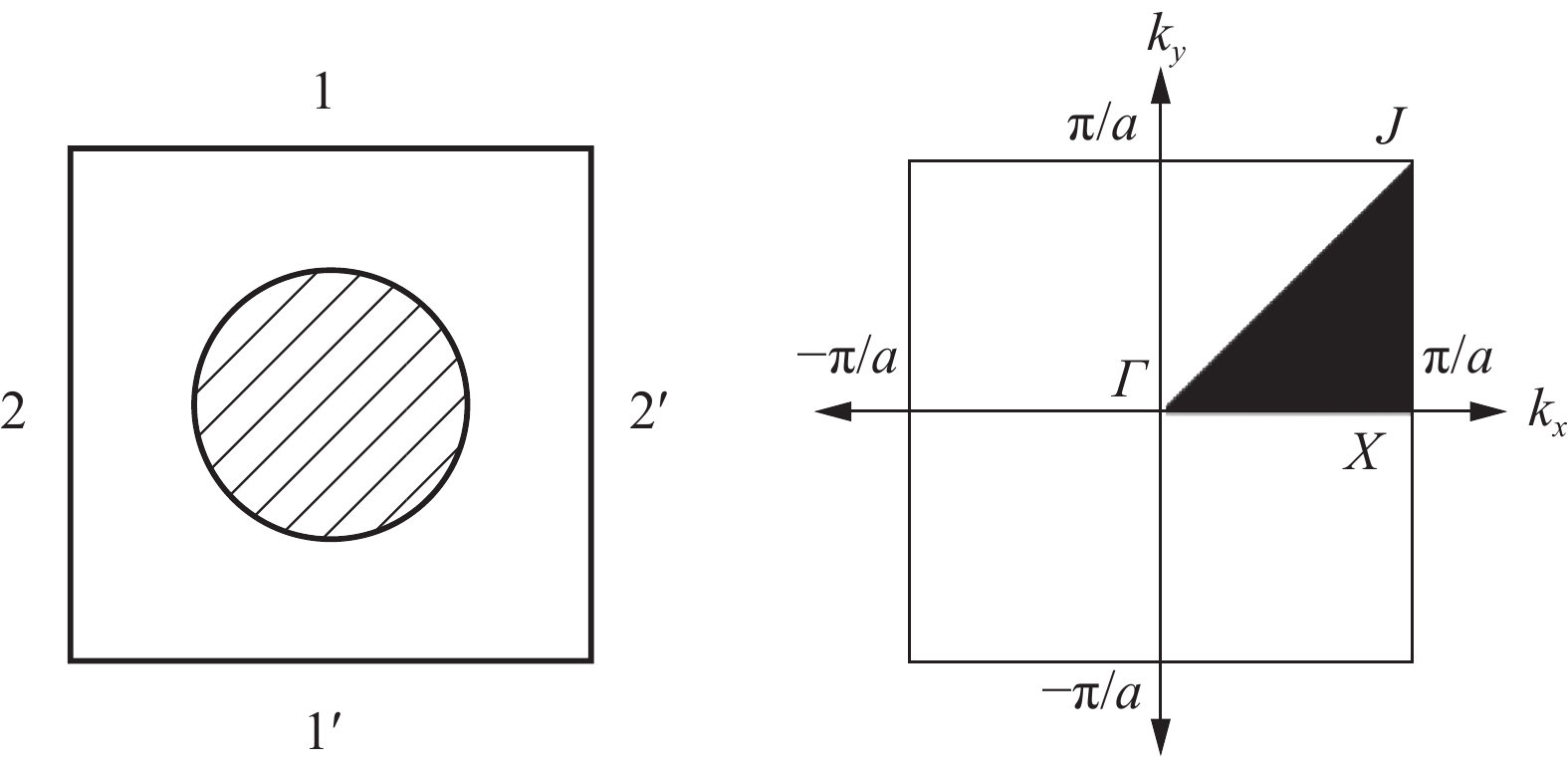
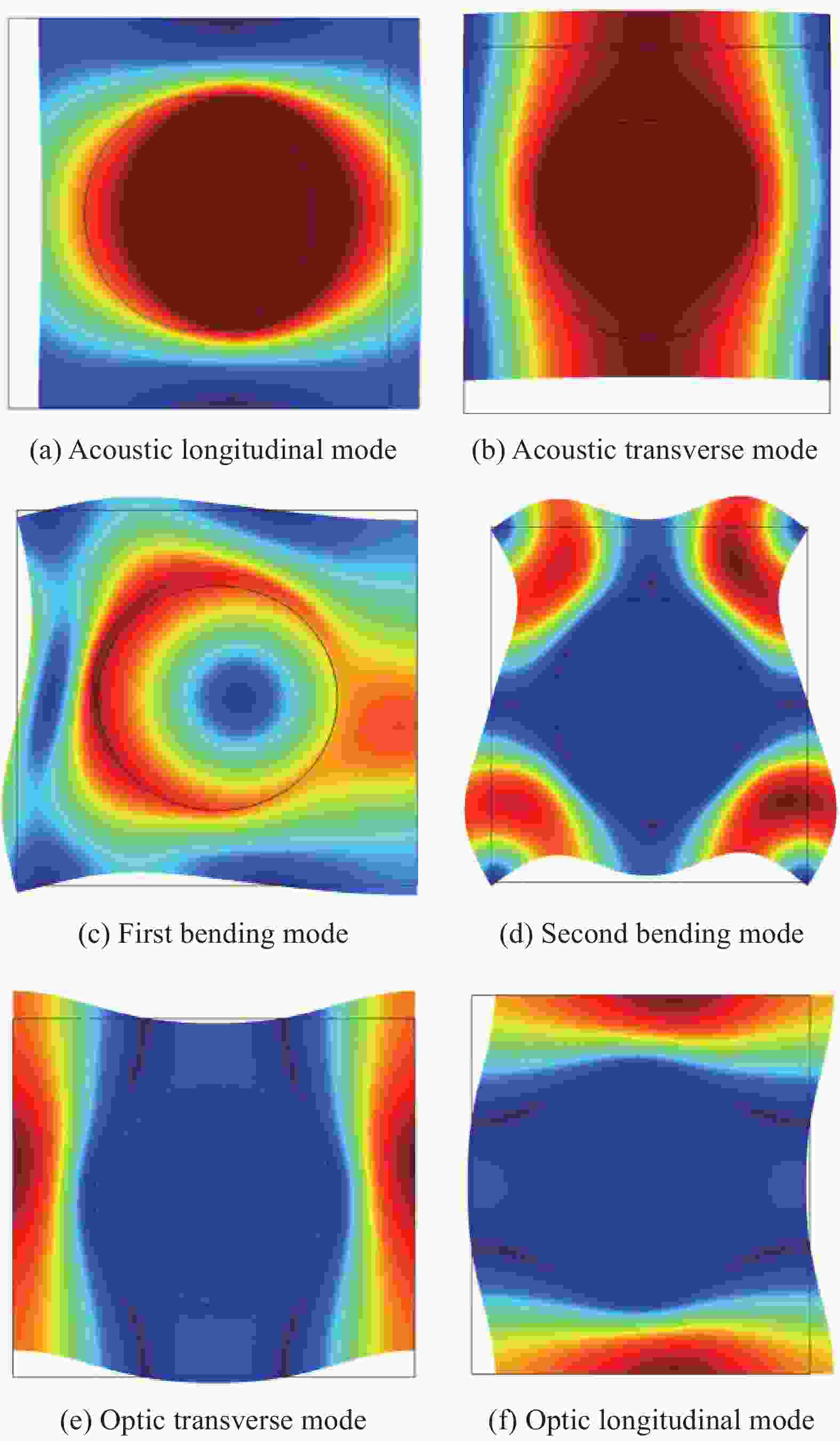
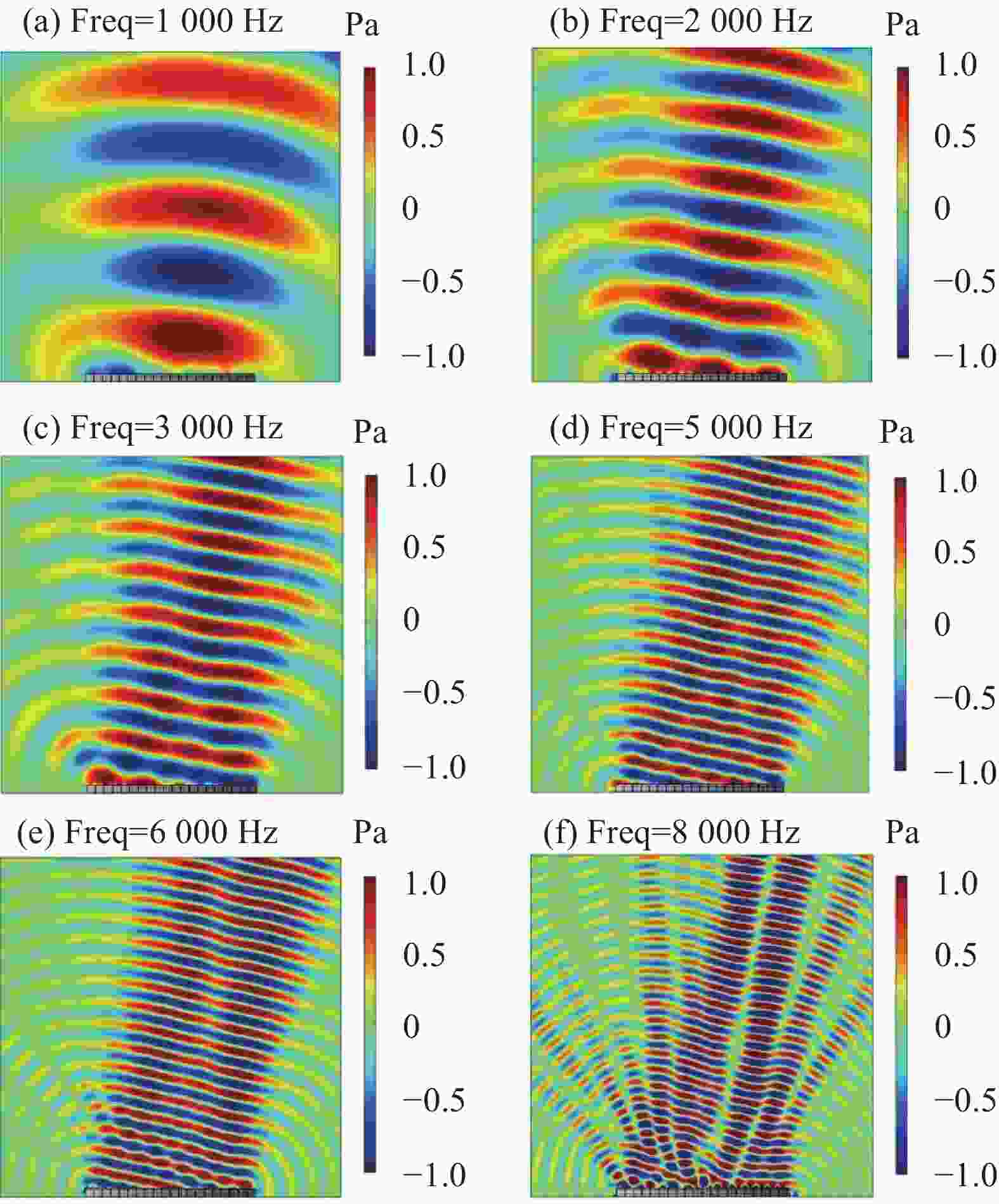
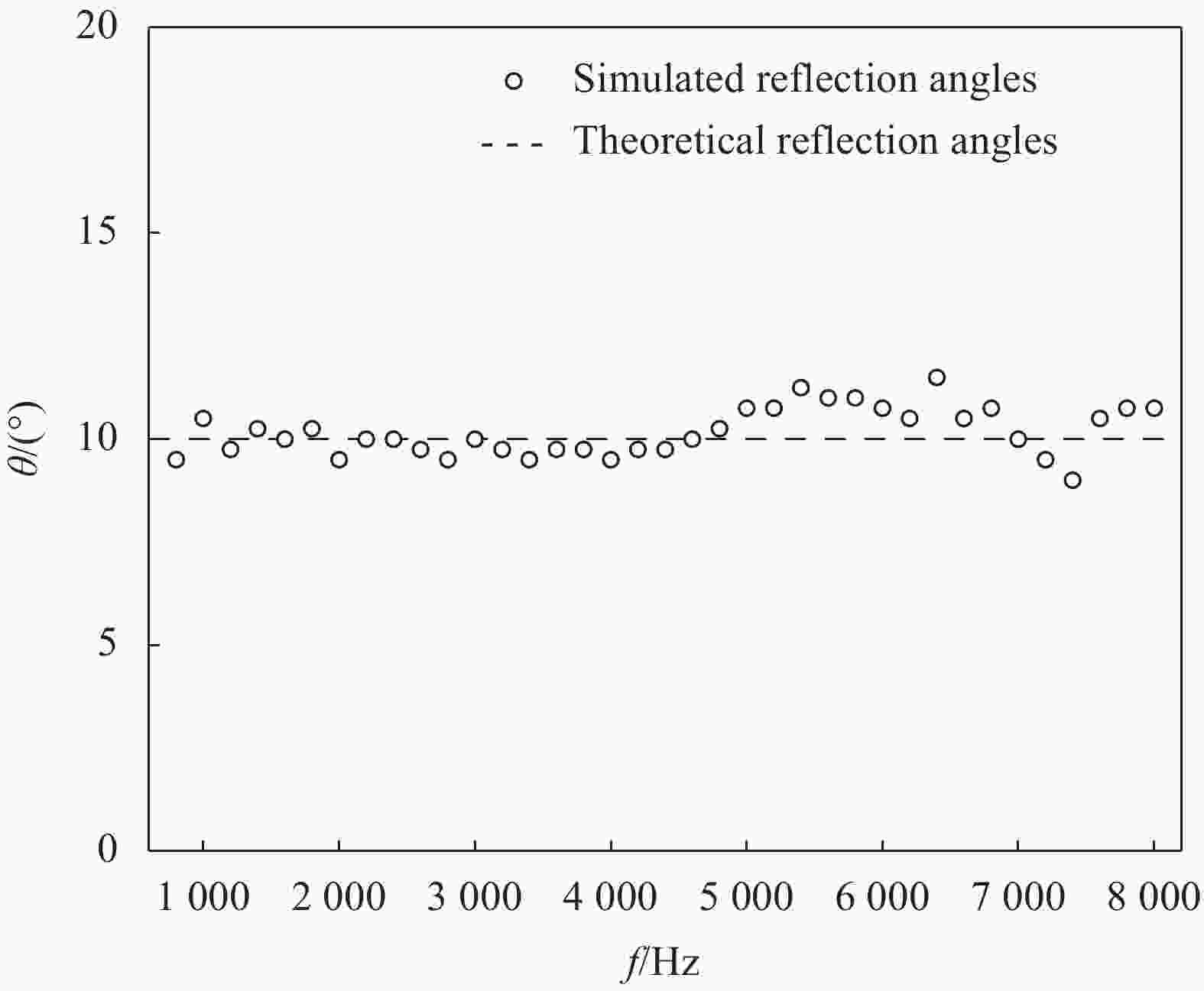
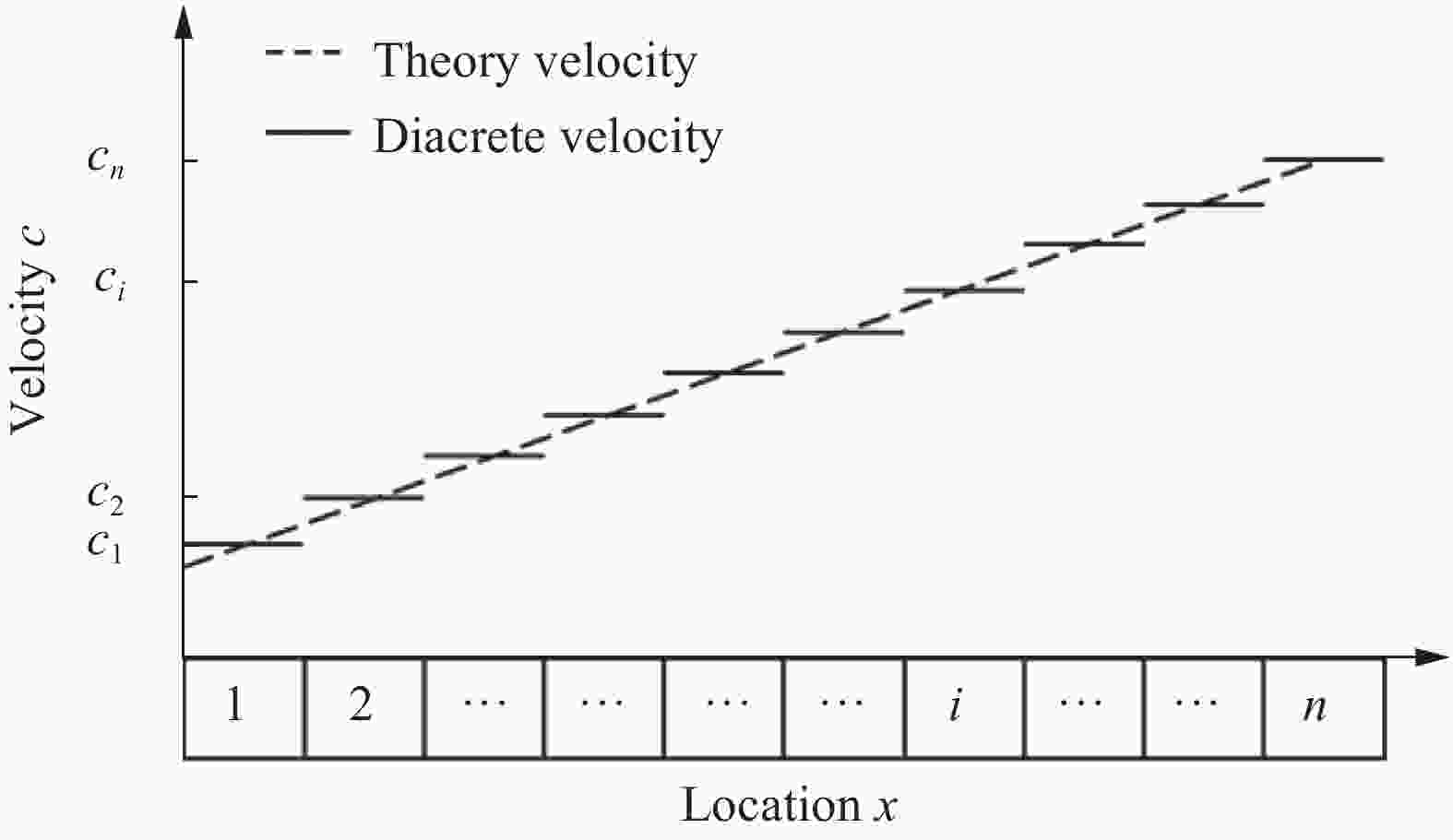
An acoustic metasurface composed by unidirectional fiber composite materials
-
摘要: 声学超表面是一种能够调节声波反射、透射和吸收特性的超薄人工结构，对于空间受限的应用领域具有重要价值，目前声学超表面主要借助超构材料来实现。提出一种非超构材料的新型声学超表面，采用单向纤维周期复合材料对声波进行调制，实现了声波的定向反射调控。借助复合材料细观力学方法，采用均匀化理论和优化方法设计周期复合材料单胞的组分，使单胞具有特定的等效力学性能与声学性能，并满足特性阻抗匹配，从而形成超表面所需的声速梯度分布。通过能带分析获得了单胞纵波波速与频率的关系，显示出复合材料超表面的宽频特性。定向反射仿真展示了复合材料超表面操控声波的有效性，并验证了对于垂直入射声波纵波是影响波控性能的主要因素。研究工作为声学超表面及其他声学波控装置的设计提供了一种新途径。Abstract: Acoustic metasurfaces are artificial materials of subwavelength thickness capable of manipulating reflection, transmission and absorption of acoustic waves. Acoustic metasurfaces have important values for space limi-ted application fields. At present, the strategy for the design of acoustic metasurfaces depends on the metamaterials. Therefore, we proposed a scheme by designing a kind of non-metamaterials acoustic metasurface to manipulate the wavefont in fluids. Based on this idea, a new type of reflection acoustic metasurface composed by the unidirectional fiber composites with periodicity was studied. Through homogenization theory and optimization method of micromechanics the fractions of composites unit cells were designed in order to obtain the effective mechani-cal and acoustics prosperities of unit cells to satisfy the densities and longitudinal velocities of discrete metasurfaces needed, as well as the impedance matching condition. Finally, the gradient longitudinal velocity of the acoustic metasurfaces needed was achieved. Using Bloch-Floquet analysis, the relationship between longitudinal velocity and frequency was studied. The band diagrams exhibit the broadband characteristics of the designed metasurfaces. Simulations of reflection control were studied for the designed metasurfaces with normally incident plan wave. Excellent wavefont manipulation effects were observed in broadband frequencies. Accordingly, it is verified that longitudinal modes are the most important factors for wave manipulation under normally incident waves. The research work provides a novel idea and potential method for the design and physical implementation of the acoustic metasurfaces as well as the other acoustic wave manipulation devices.
-
图 1 单向纤维复合材料超表面离散示意图
Figure 1. Schematic diagram of discrete metasurfaces composed by unidirectional fiber composite materials
图 2 单向纤维复合材料与单胞示意图
Figure 2. Schematic diagram of the unidirectional fiber composites and unit cell
图 3 单向钢纤维聚乙烯(PE)基复合材料超表面仿真模型
Figure 3. Simulation model diagram of metasurface composed by unidirectional steel fibers and polyethylene (PE) matrix composites
图 4 单向纤维复合材料超表面的正方晶格周期性边界条件和第一布里渊区
Figure 4. Periodic boundary conditions and the first brillouin zone of square unit cell composed by unidirectional fiber composite materials
图 5 单向纤维复合材料超表面的1号与20号单胞ΓX和ΓJ方向能带图
Figure 5. Band diagrams of ΓX and ΓJ directions in the first and the twentieth unit cells of metasurfaces composed by unidirectional fiber composite materials
图 6 单向纤维复合材料超表面的单胞沿ΓX方向的六阶振动模态
Figure 6. Six vibration modes of unit cell from the ΓX direction composed by unidirectional fiber composite materials
图 7 单向纤维复合材料超表面对水下1 kHz至8 kHz频率垂直入射平面波的10°定向反射声压场
Figure 7. Simulation acoustic field maps of metasurfaces composed by unidirectional fiber composite materials of 10° reflection for a plane wave normally incident from the top side with frequencies 1 kHz to 8 kHz under water
图 8 单向纤维复合材料超表面的仿真定向反射角与理论反射角度对比
Figure 8. Comparison of simulated directional reflection angles with theoretical reflection angles of metasurfaces composed by unidirectional fiber composite materials
表 1 PE基体和钢纤维力学性能
Table 1. Mechanical properties of steel fiber and PE matrix
Mechanical property PE matrix Steel fiber Young’s modulus/GPa 0.15-1.00 210.00 Poisson’s ratio 0.46 0.30 Density/(kg·m−3) 940 7850 
下载: 导出CSV
表 2 单向纤维复合材料超表面的单胞基体杨氏模量和体积分数优化结果
Table 2. Optimization results of the Young’s modulus of matrix and fiber volume fraction of unit cells of metasurfaces composed by unidirectional fiber composite materials
Unit cell Young’s modulus
of matrix/MPaFiber volume
fraction/vol%1 371.7 4.4 2 342.2 5.7 3 316.4 6.9 4 293.5 8.1 5 273.1 9.4 6 254.8 10.7 7 238.3 11.9 8 223.3 13.2 9 209.7 14.4 10 197.2 15.7 11 185.8 17.0 12 175.3 18.2 13 165.5 19.5 14 156.5 20.7 15 148.1 22.0 16 140.3 23.2 17 133.0 24.5 18 126.2 25.8 19 119.8 27.0 20 113.7 28.3
下载: 导出CSV
表 3 单向纤维复合材料超表面的单胞等效声速
${\bar c^i}$ 与等效密度${\bar \rho ^i}$ Table 3. Effective velocity
${\bar c^i}$ and effective density${\bar \rho ^i}$ of metasurface unit cells composed by unidirectional fiber composite materialsUnit cell Theory velocity
/(m·s−1)Effective velocity
/(m·s−1)Theory density
/(kg·m−3)Effective density
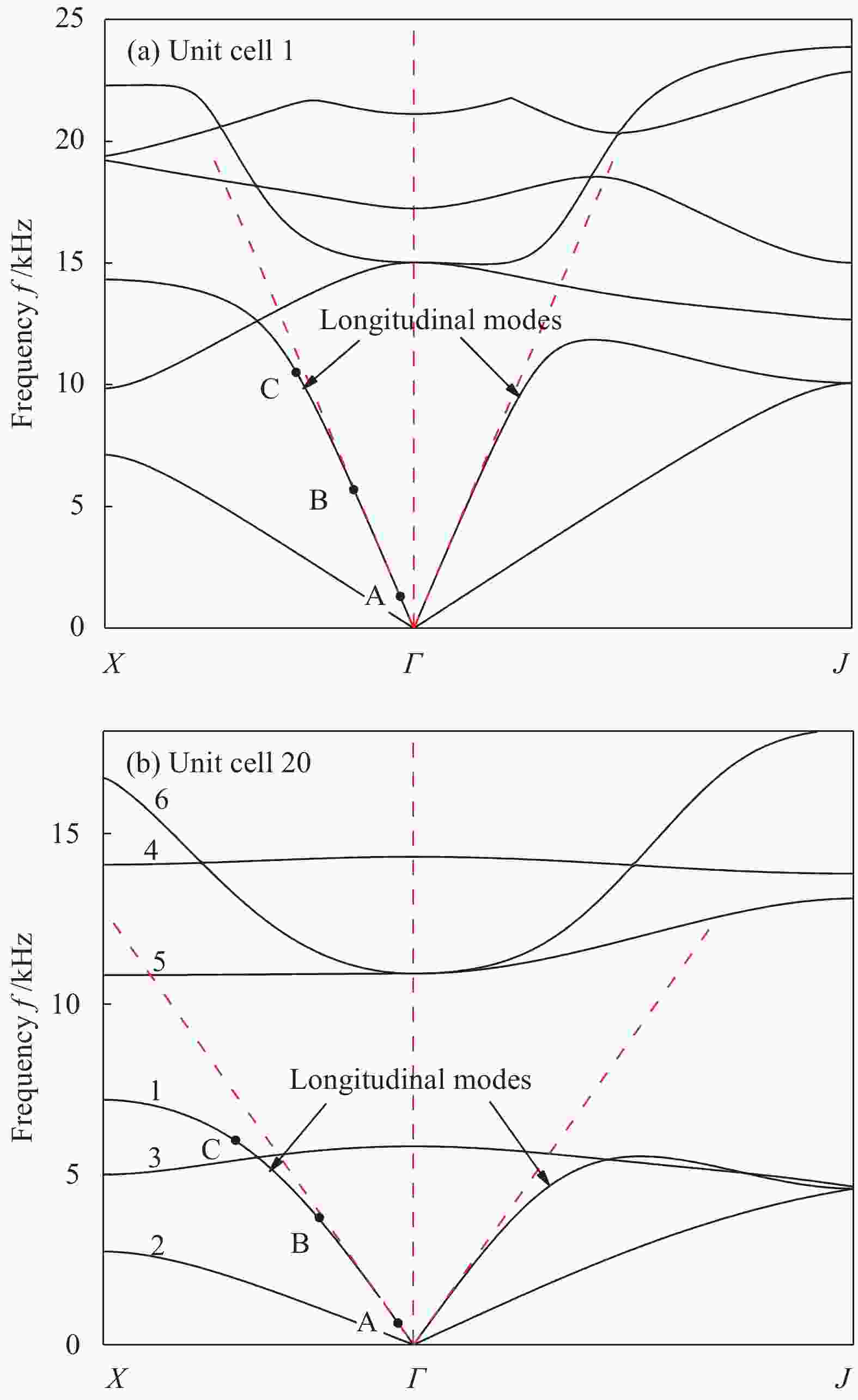
/(kg·m−3)1 1206 1204 1243 1243 2 1128 1127 1330 1330 3 1059 1057 1417 1417 4 997 992 1504 1503 5 943 939 1591 1590 6 894 894 1678 1677 7 850 852 1764 1764 8 810 815 1 851 1 851 9 774 774 1 938 1 937 10 741 741 2 025 2 024 11 710 710 2112 2111 12 682 681 2198 2198 13 656 657 2285 2284 14 632 632 2372 2371 15 610 612 2459 2458 16 589 587 2546 2545 17 570 568 2633 2631 18 552 551 2719 2718 19 535 535 2806 2805 20 518 519 2893 2892
下载: 导出CSV
表 4 单向纤维复合材料超表面的单胞沿ΓX方向等效纵波波速
${\bar c^i}$ 误差分析Table 4. Errors of longitudinal velocities
${\bar c^i}$ in unit cells from ΓX direction of metasurfaces composed by unidirectional fiber composite materialsUnit cell No. A point B point C point ${\bar c^i}$/(m·s−1) Error
/%f
/kHz${\bar c^i}$/(m·s−1) Error
/%f
/kHz${\bar c^i}$/(m·s−1) Error
/%f
/kHz1 1202 0.3 2.11 1188 1.5 5.97 1087 9.9 11.42 5 938 0.5 2.11 919 2.6 4.60 897 4.9 6.06 10 734 0.9 2.02 704 5.0 4.58 665 10.3 6.15 15 602 1.3 2.11 573 6.1 4.30 522 14.4 6.01 20 516 0.4 1.16 489 5.6 3.79 424 18.1 5.94
下载: 导出CSV
-
[1] ZHAO J J, LI B W, CHEN Z N, et al. Redirection of sound waves using acoustic metasurface[J]. Applied Physics Letters,2013,103(15):151604. doi: 10.1063/1.4824758 [2] ZHAO J J, LI B W, CHEN Z N, et al. Manipulating acoustic wavefront by inhomogeneous impedance and steerable extraordinary reflection[J]. Scientific Reports,2013,3:2537. doi: 10.1038/srep02537 [3] LI Y, LIANG B, ZHONG M G, et al. Reflected wavefront manipulation based on ultrathin planar acoustic metasurfaces[J]. Scientific Reports,2013,3:2546. doi: 10.1038/srep02546 [4] YU N F, GENEVET P, KATS M A, et al. Light propagation with phase discontinuities: generalized laws of reflection and refraction[J]. Science,2011,334(6054):333-337. doi: 10.1126/science.1210713 [5] LI Y, JIANG X, LI R Q, et al. Experimental realization of full control of reflected waves with subwavelength acoustic metasurfaces[J]. Physical Review Applied,2014,2(6):064002. doi: 10.1103/PhysRevApplied.2.064002 [6] MEI J, WU Y. Controllable transmission and total refection through an impedance -matched acoustic metasurface[J]. New Journal of Physics,2014,16:123007. doi: 10.1088/1367-2630/16/12/123007 [7] 李勇. 声学超构表面[J]. 物理, 2017, 46(11):721-730. doi: 10.7693/wl20171102LI Yong. Acoustic metasurface[J]. Physics,2017,46(11):721-730(in Chinese). doi: 10.7693/wl20171102 [8] ASSOUAR B, LIANG B, WU Y, et al. Acoustic metasurfaces[J]. Nature Reviews Materials,2018,3:460-472. doi: 10.1038/s41578-018-0061-4 [9] 朱一凡, 梁彬, 程建春. 广义斯奈尔定律与声超表面[J]. 应用声学, 2018, 37(1):53-62. doi: 10.11684/j.issn.1000-310X.2018.01.008ZHU Yifan, LIANG Bin, CHENG Jianchun. The generalized Snell’s law and acoustic metasurfaces[J]. Journal of Applied Acoustics,2018,37(1):53-62(in Chinese). doi: 10.11684/j.issn.1000-310X.2018.01.008 [10] 温激鸿, 蔡力, 郁殿龙, 等. 声学超材料基础理论与应用[M]. 北京: 科学出版社, 2018: 34−37.WEN Jihong, CAI Li, YU Dianlong, et al. Theory and applica-tions of acoustics metamaterials[M]. Beijing: Science Press, 2018: 34−37(in Chinese). [11] 田源, 葛浩, 卢明辉, 等. 声学超构材料及其物理效应的研究进展[J]. 物理学报, 2019, 68(19):194301-12. doi: 10.7498/aps.68.20190850TIAN Yuan, GE Hao, LU Minghui, et al. Research advances in acoustic metamaterials[J]. Acta hysica Sinica,2019,68(19):194301-12(in Chinese). doi: 10.7498/aps.68.20190850 [12] XIE Y B, WANG W Q, CHEN H Y, et al. Wavefront modulation and subwavelength diffractive acoustics with an acoustic metasurface[J]. Nature Communications,2014,5:5553. doi: 10.1038/ncomms6553 [13] LI Y, BADREDDINE M A. Acoustic metasurface-based perfect absorber with deep subwavelength thickness[J]. Applied Physics Letters,2016,108:063502. doi: 10.1063/1.4941338 [14] MA G C, YANG M, XIAO S W, et al. Acoustic metasurface with hybrid resonances[J]. Nature Materials,2014,13:873-878. doi: 10.1038/nmat3994 [15] ZHU X H, LI J F, SHEN C, et al. Non-reciprocal acoustic transmission via space-time modulated membranes[J]. Applied Physics Letters,2020,116(3):034101. doi: 10.1063/1.5132699 [16] TIAN Y, WEI Q, CHENG Y, et al. Broadband manipulation of acoustic wavefronts by pentamode metasurface[J]. Applied Physics Letters,2015,107(22):221906. doi: 10.1063/1.4936762 [17] CHEN Y, HU G K. Broadband and high-transmission metasurface for converting underwater cylindrical waves to plane waves[J]. Physical Review Applied,2019,12(4):044046. doi: 10.1103/PhysRevApplied.12.044046 [18] LIU Y, LI Y F, LIU X Z. Manipulation of acoustic wavefront by transmissive metasurface based on pentamode metamaterials[J]. Chinese Physics B,2019,28(2):306-312. [19] CHU Y Y, WANG Z H, XU Z. Broadband high-efficiency controllable asymmetric propagation by pentamode acoustic metasurface[J]. Physics Letters A,2020,384(11):26230. [20] ZHANG X D, CHEN H, ZHAO Z G, et al. Experimental demonstration of a broadband waterborne acoustic meta-surface for shifting reflected waves[J]. Journal of Applied Physics,2020,127(17):174902. doi: 10.1063/1.5139008 [21] SU X S, NORRIS A N, CUSHING C W, et al. Broadband focusing of underwater sound using a transparent pentamode lens[J]. The Journal of the Acoustical Society of America,2017,14(6):4408. [22] 陈毅, 刘晓宁, 向平, 等. 五模材料及其水声调控研究[J]. 力学进展, 2016, 46:382.CHEN Yi, LIU Xiaoning, XIANG Ping, et al. Pentamode material for underwater acoustic wave control[J]. Advances in Mechanics,2016,46:382(in Chinese). [23] ZHU Y F, FAN X D, LIANG B, et al. Multi-frequency acoustic metasurface for extraordinary reflection and sound focusing[J]. AIP Advances,2016,6(12):121702. doi: 10.1063/1.4968607 [24] MILTON G W, CHERKAEV A V. Which elasticity tensors are realizable?[J]. Journal of Engineering Materials and Technology,1995,117:483-493. doi: 10.1115/1.2804743 [25] DONG H W, ZHAO S D, MIAO X B, et al. Customized broadband pentamode metamaterials by topology optimization[J]. Journal of the Mechanics and Physics of Solids,2021,152:104407. doi: 10.1016/j.jmps.2021.104407 [26] CAI X, Wang L, ZHAO Z G, et al. The mechanical and acoustic properties of two-dimensional pentamode metamaterials with different structural parameters[J]. Applied Phy-sics Letters,2016,109(13):131904. doi: 10.1063/1.4963818 [27] 郝潇潇, 王真, 赵志高. 五模声学超表面理论分析与定向反射声学仿真[J]. 应用声学, 2021, 40(6): 904−910. HAO Xiaoxiao, WANG Zhen, ZHAO Zhigao. Theory analysis and acoustics simulation of reflection pentamode acoustic metasurface[J]. Journal of Applied Acoustics, 2021, 40(6): 904−910(in Chinese). [28] 程建春. 声学原理[M]. 北京: 科学出版社, 2019: 89.CHENG Jianchun. Acoustics theory[M]. Beijing: Science Press, 2019: 89(in Chinese). [29] 杜功焕. 声学基础[M]. 南京: 南京大学出版社, 2012: 520 − 526.DU Gonghuan. Acoustics Theory[M]. Nanjing: Nanjing University Press, 2012: 520 − 526(in Chinese). [30] 陈烈民, 杨宝宁. 复合材料的力学分析[M]. 北京: 中国科学技术出版社, 2006: 88−91.CHEN Liemin, YANG Baoning. Mechanics analysis of composite materials[M]. Beijing: China Science and Technology Press, 2006: 88−91(in Chinese). [31] 黄富华. 周期性复合材料有效性能的均匀化计算[D]. 哈尔滨: 哈尔滨工业大学, 2010: 26.HUANG Fuhua. Homogenizated numeration of effective properties for composite material with periodicity[D]. Harbin: Harbin Institute of Technology, 2010: 26(in Chinese). [32] 顾伯洪, 孙宝忠. 纺织复合材料设计[M]. 上海: 东华大学出版社, 2018: 132−133.GU Bohong, SUN Baozhong. Design of textile composites[M]. Shanghai: Donghua University Press, 2018: 132−133(in Chinese). [33] UGRAY Z, LASDON L, PLUMMER J, et al. Scatter search and local NLP solvers: a multistart framework for global optimization[J]. INFORMS Journal on Computing. 2007, 19(3): 328−340. [34] 梁军, 方国东. 三维编织复合材料力学性能分析方法[M]. 哈尔滨: 哈尔滨工业大学出版社, 2013: 17−24.LIANG Jun, FANG Guodong. Mechanical analysis method of 3d braided composites[M]. Harbin: Harbin Institute of Thechnology Press, 2013: 17−24(in Chinese). -
下载:
点击查看大图
计量
- 文章访问数: 1003
- HTML全文浏览量: 438
- PDF下载量: 88
- 被引次数: 0
