Fatigue delamination analysis of composite double cantilever beams based on discrete damage zone model
-
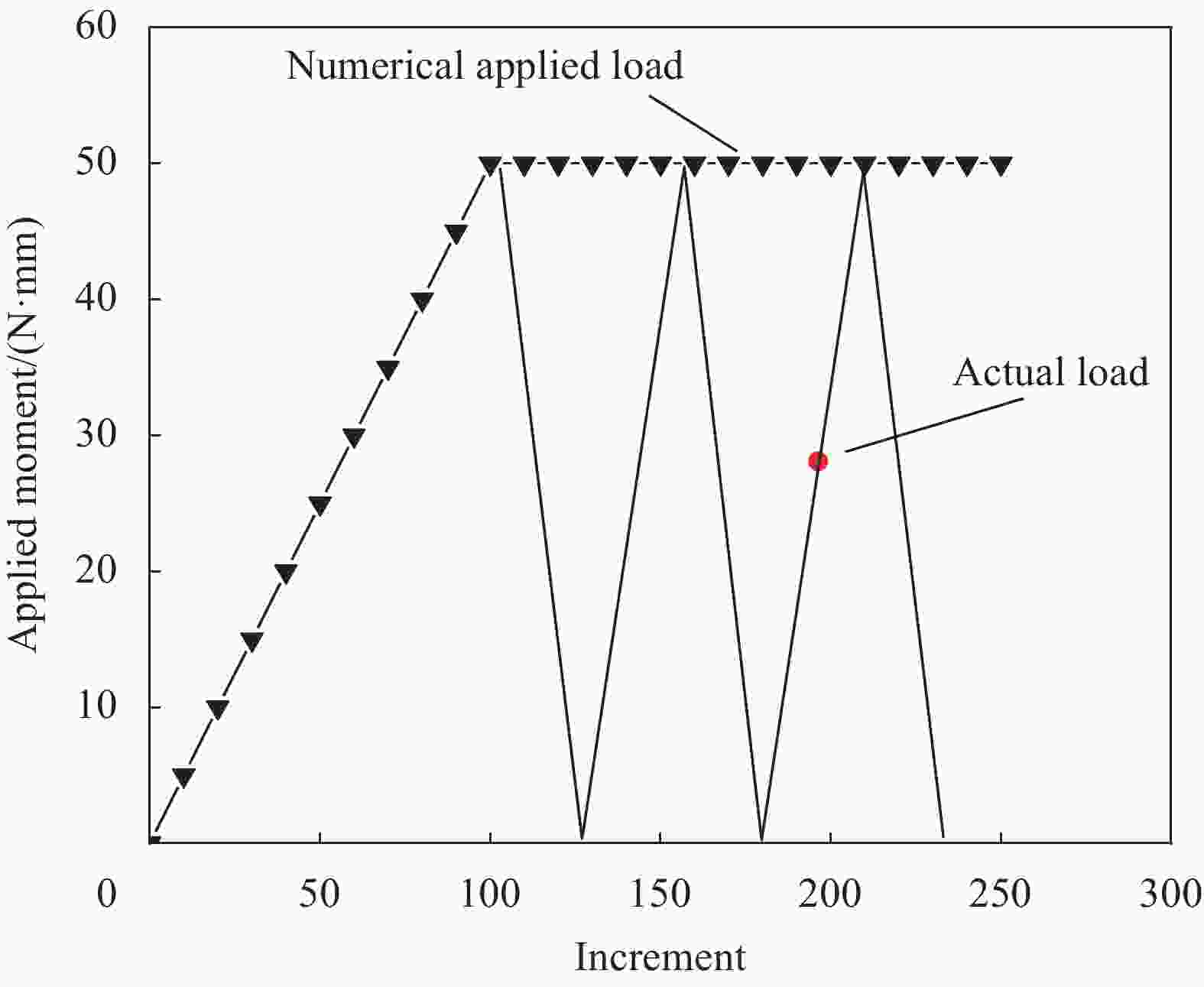
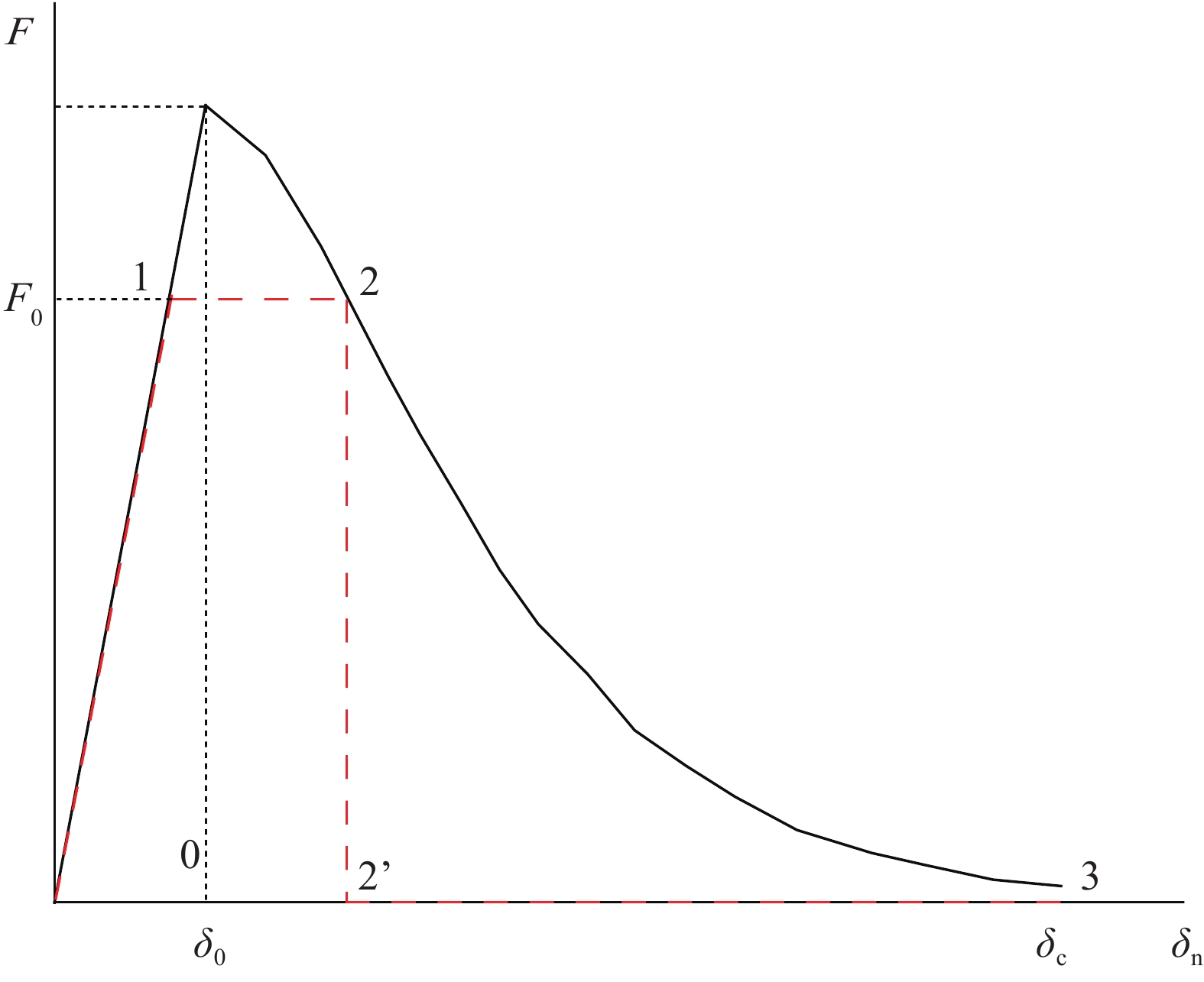
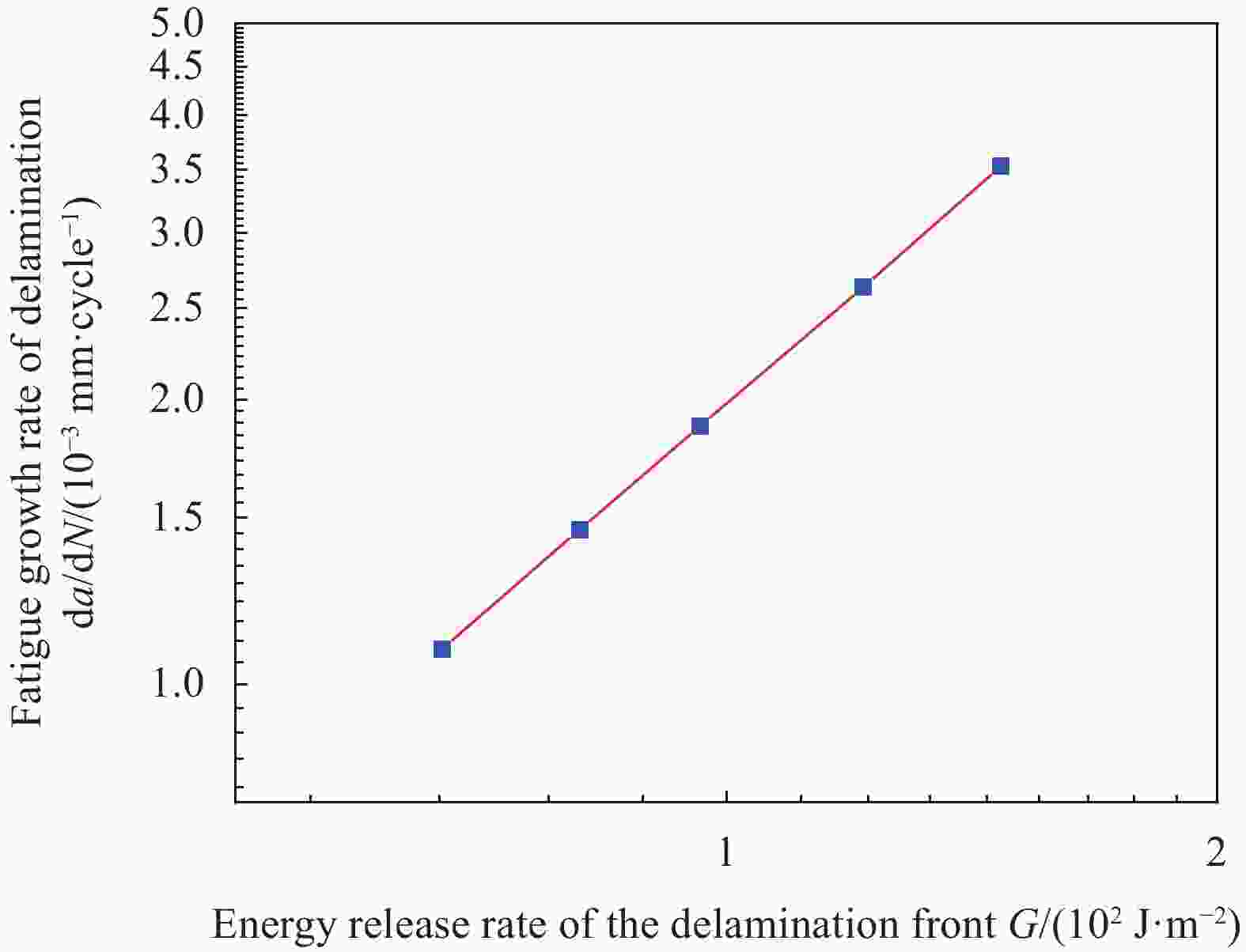
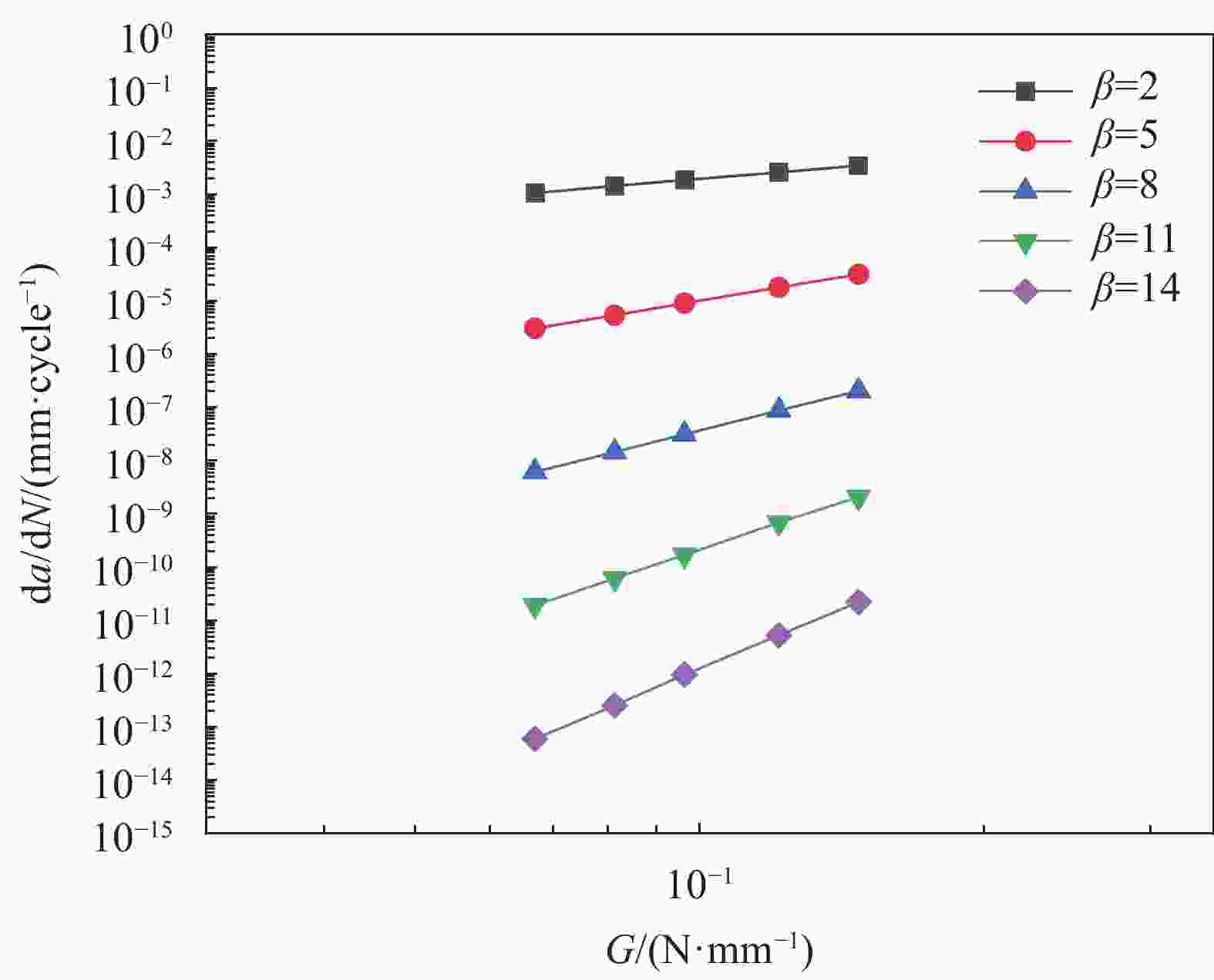
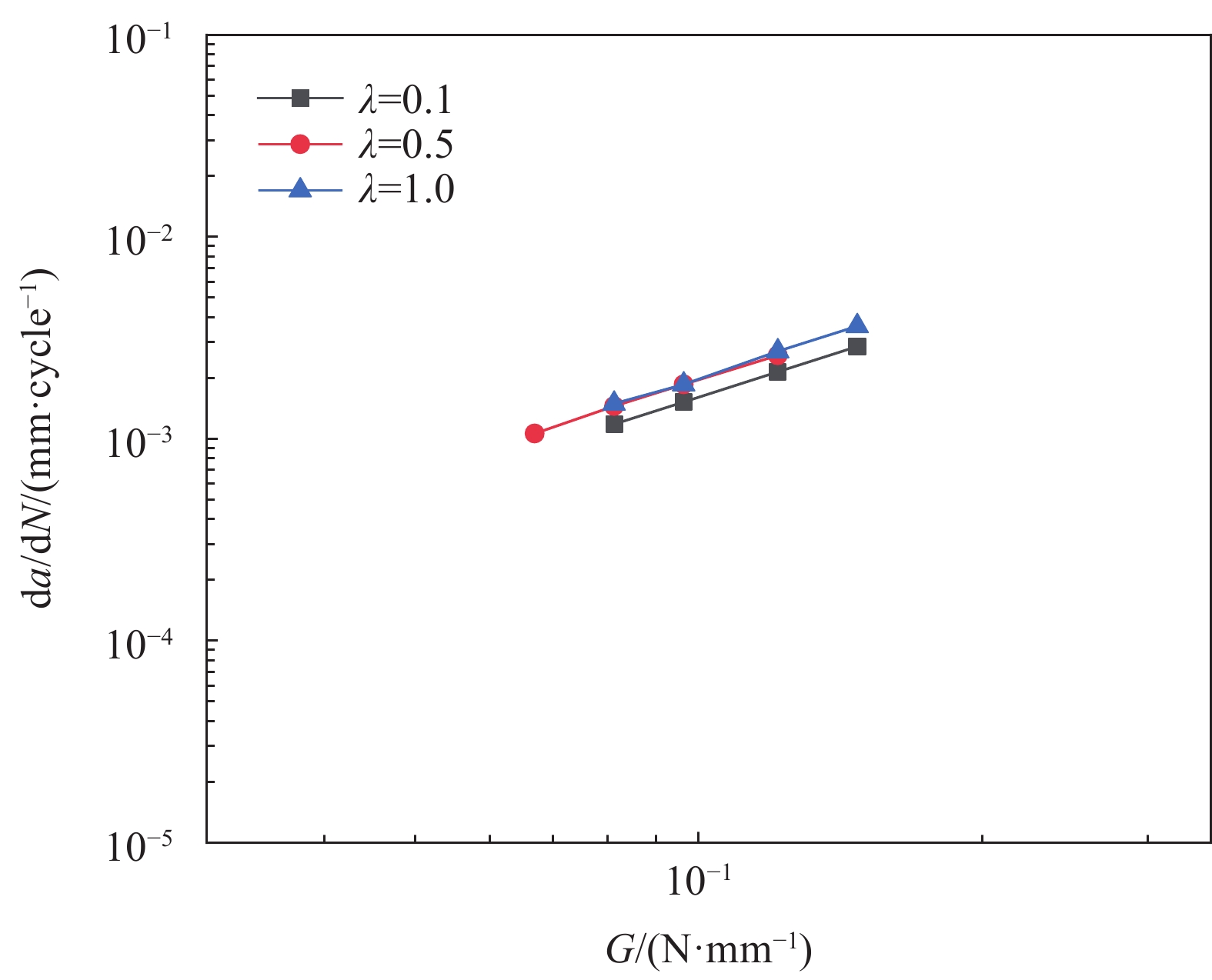
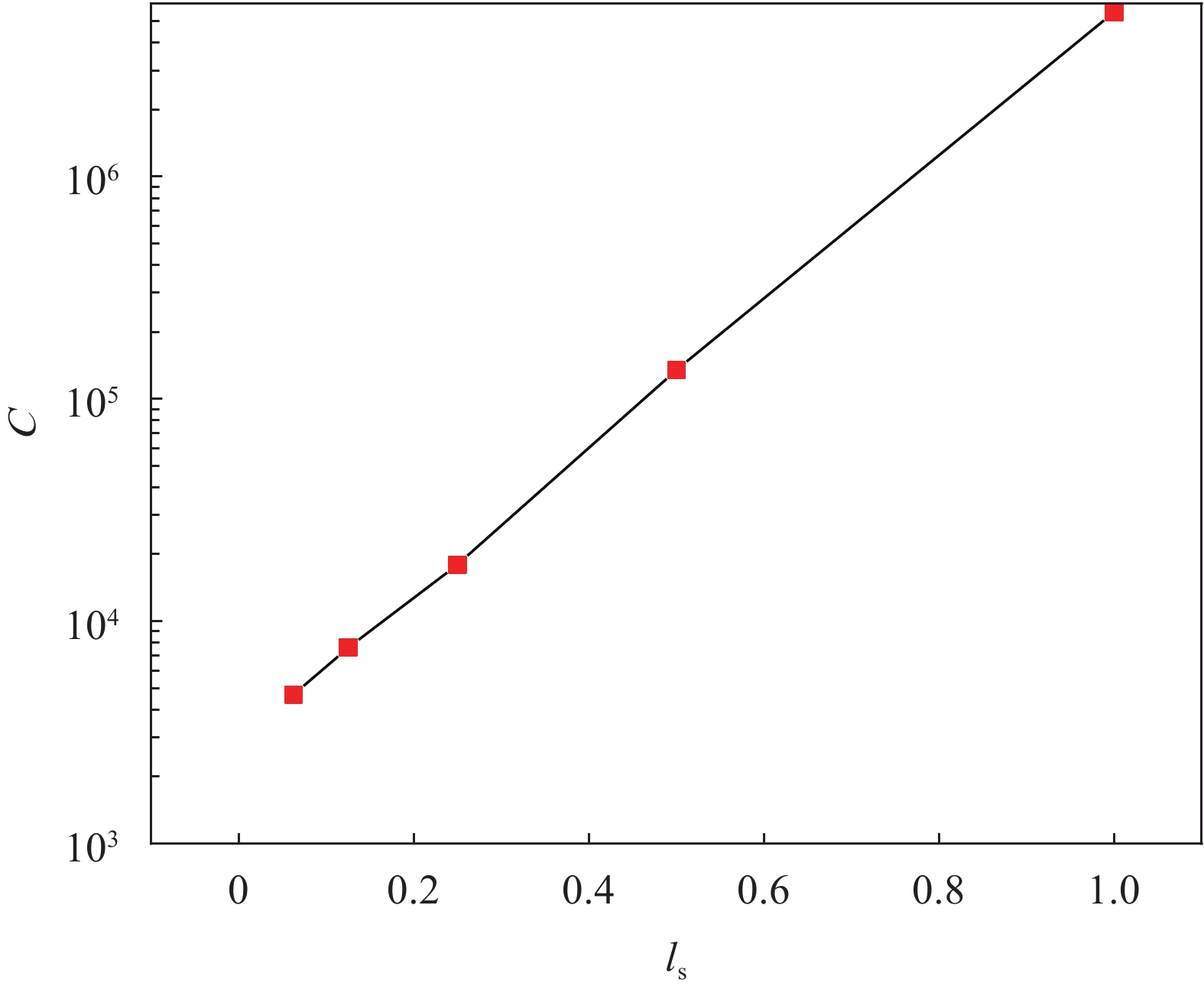
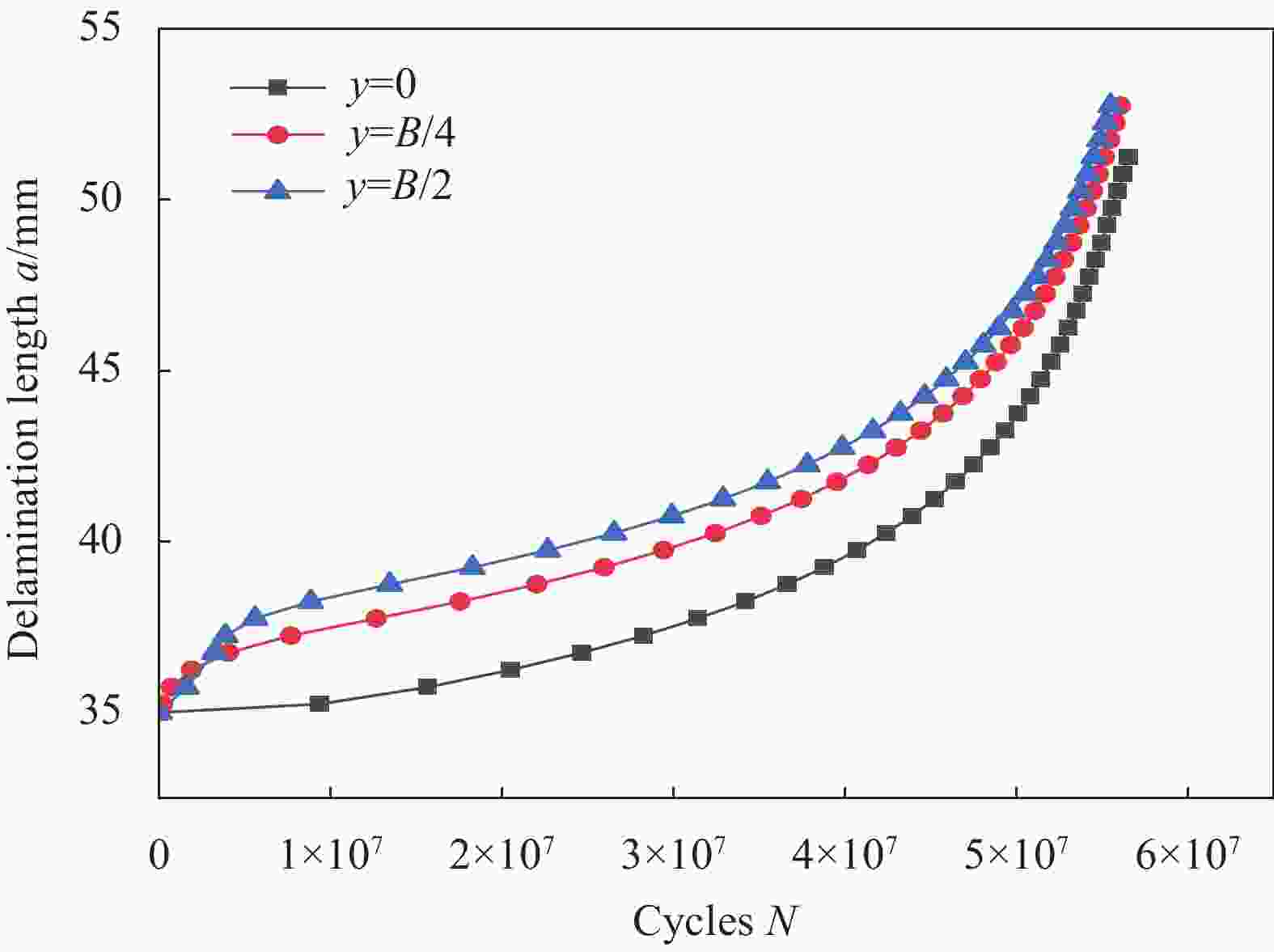
摘要: 基于扩展逐层理论(Extended layerwise method,XLWM)、离散损伤模型(Discrete damage zone model,DDZM)和Peerlings损伤定律,建立了可以分析复合材料结构分层损伤疲劳扩展的疲劳渐进扩展逐层方法(Fatigue progressive extended layerwise method,FPXLWM)。首先对HTC/6736A碳纤维双悬臂梁进行分析,施加恒定弯矩,验证该方法的正确性;然后通过计算多组Paris曲线,确定模型的疲劳参数,对HTC/6736A碳纤维双悬臂梁分层疲劳扩展机制进行深入探讨。
-
关键词:
- 离散损伤模型 /
- 扩展逐层方法 /
- 分层疲劳扩展 /
- HTC/6736A碳纤维双悬臂梁 /
- Paris曲线
Abstract: Based on extended layerwise method (XLWM), discrete damage zone model (DDZM) and Peerlings damage law, a fatigue progressive extended layerwise method (FPXLWM) was established to analyze the delamination fatigue growth of composites. Firstly, a HTC/6736A carbon fiber double cantilever beam subjected to a constant bending moment was analyzed to verify the proposed method in numerical examples. Then, the fatigue parameters of the proposed model were determined by several groups of Paris curves; the fatigue growth mechanisms of delamination in the HTC/6736A carbon fiber double cantilever beam were investigated. -
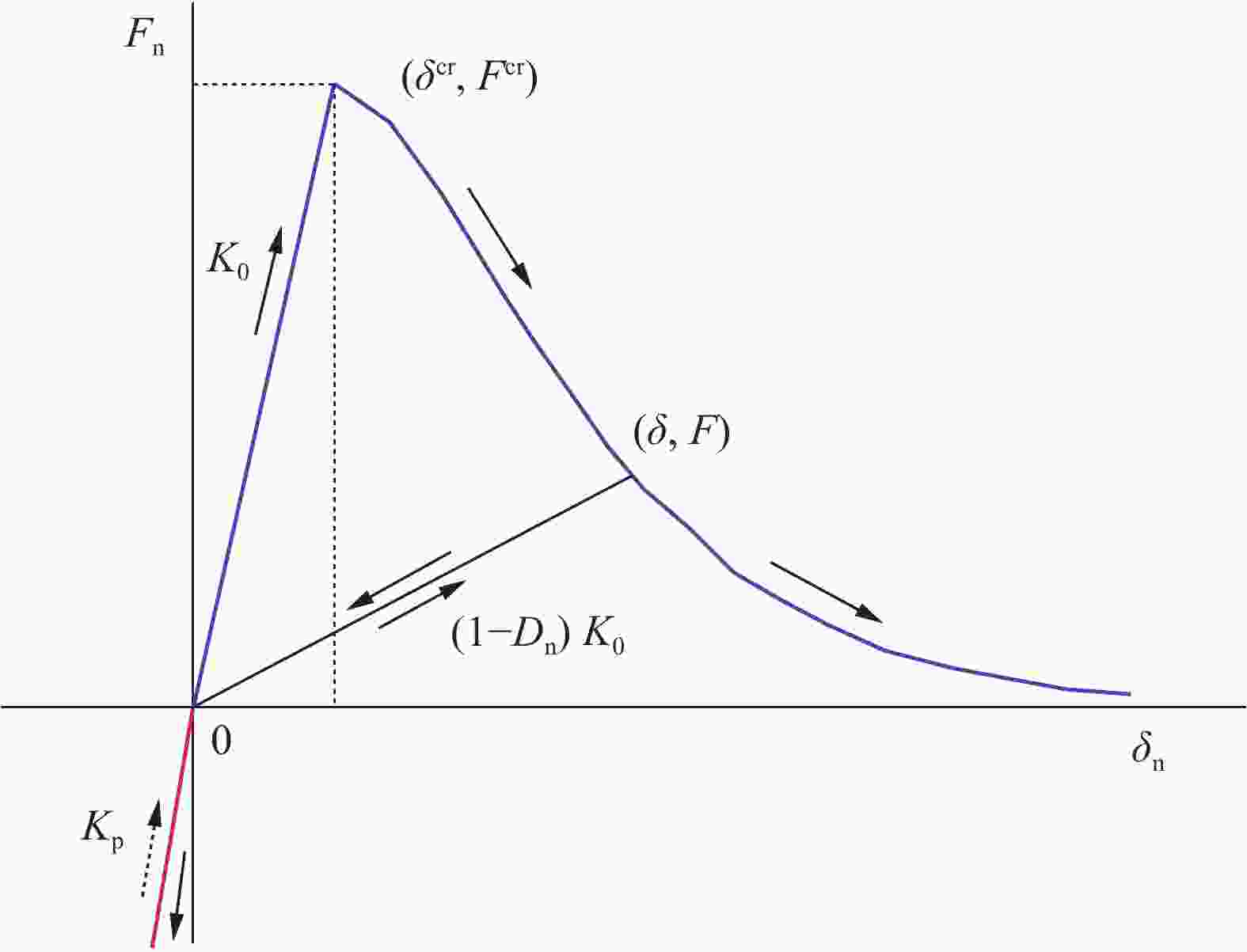
图 1 含离散损伤模型的求解区域Ω0
Figure 1. A solve domain Ω0 with discrete damage zone model
$\bar t $—Load; $\bar u $—Displacement ; $\varGamma_{\rm{t}} $—Load boundary ; $\varGamma_{\rm{tf}} $—Surface force region; $ \varGamma_{\rm{c}}$—Damage region; $\varGamma_{\rm{coh}} $—Cohesion region; $\varGamma_{\rm{u}} $—Displacement boundary
图 5 疲劳渐进扩展逐层方法的位移场
Figure 5. Displacement field for fatigue progressive extended layerwise method
hk—Thickness of layer k; zk—Coordinate in thickness direction of layering interfaces of layers k and (k−1); The numbers on the left represent the mathematical layer interpolation nodes of the displacement field along the thickness direction, and the numbers on the right represent the interfaces between the layers
-
[1] JOSHI S P, SUN C T. Impact induced fracture in a laminated composite[J]. Journal of Composite Materials,1985,19(1):51-66. doi: 10.1177/002199838501900104 [2] LAWS N, DVORAK G J. Progressive transverse cracking in composite laminates[J]. Journal of Composite Materials,1988,22(10):900-916. doi: 10.1177/002199838802201001 [3] ABOUDI J. Stiffness reduction of cracked solids[J]. Engineering Fracture Mechanics,1987,26(5):637-650. doi: 10.1016/0013-7944(87)90129-9 [4] GUDMUNDSON P, ZANG W L. An analytic model for thermoelastic properties of composite laminates containing transverse matrix cracks[J]. International Journal of Solids & Structures,1993,30(23):3211-3231. [5] CHOI H Y, CHANG F K. A model for predicting damage in graphite/epoxy laminated composites resulting from low-velocity point impact[J]. Journal of Composite Materials,1992,26(14):2134-2169. doi: 10.1177/002199839202601408 [6] DUGDALE D S. Yielding of steel sheets containing slits[J]. Journal of the Mechanics and Physics of Solids,1960,8(2):100-104. doi: 10.1016/0022-5096(60)90013-2 [7] BARENBLATT G I. The mechanical theory of equilibrium cracks in brittle fracture[J]. Advances in Applied Mechanics,1962,7:55-129. [8] NEEDLEMAN A. A continuum model for void nucleation by inclusion debonding[J]. Journal of Applied Mechanics,1987,54(3):525. doi: 10.1115/1.3173064 [9] NEEDLEMAN A. An analysis of decohesion along an imperfect interface[J]. International Journal of Fracture,1990,42(1):21-40. doi: 10.1007/BF00018611 [10] HUTCHINSON J W, EVANS A G. Mechanics of materials: Top-down approaches to fracture[J]. Acta Materialia,2000,48(1):125-135. doi: 10.1016/S1359-6454(99)00291-8 [11] ELICES M, GUINEA G, GÓMEZ SÁNCHEZ F J, et al. The cohesive zone model: Advantages, limitations and challenges[J]. Engineering Fracture Mechanics,2002,69(2):137-163. doi: 10.1016/S0013-7944(01)00083-2 [12] 李西宁, 王悦舜, 周新房. 复合材料层合板分层损伤数值模拟方法研究现状[J]. 复合材料学报, 2021, 38(4):1076-1086.LI Xining, WANG Yueshun, ZHOU Xinfang. Research status of numerical simulation methods for delamination of composite laminates[J]. Acta Materiae Compositae Sinica,2021,38(4):1076-1086(in Chinese). [13] SHAHVERDI M, VASSILOPOULOS A P, KELLER T. Experimental investigation of R-ratio effects on fatigue crack growth of adhesively-bonded pultruded GFRP DCB joints under CA loading[J]. Composites Part A: Applied Science and Manufacturing,2012,43(10):1689-1697. doi: 10.1016/j.compositesa.2011.10.018 [14] MURRI G B. Effect of data reduction and fiber-bridging on mode I delamination characterization of unidirectional composites[J]. Journal of Composite Materials,2014,48(19):2413-2424. doi: 10.1177/0021998313498791 [15] ANDERSONS J, MOJO M, OCHIAI S. Empirical model for stress ratio effect on fatigue delamination growth rate in composite laminates[J]. International Journal of Fatigue,2004,26(6):597-604. [16] BEGHINI M, BERTINI L, FORTE P. Experimental investigation on the influence of crack front to fiber orientation on fatigue delamination growth rate under mode II[J]. Composites Science & Technology,2006,66(2):240-247. [17] PASCOE J A, ALDERLIESTEN R C, BENEDICTUS R. Methods for the prediction of fatigue delamination growth in composites and adhesive bonds-A critical review[J]. Engineering Fracture Mechanics,2013,112-113:72-96. [18] TAO C, MUKHOPADHYAY S, ZHANG B, et al. An improved delamination fatigue cohesive interface model for complex three-dimensional multi-interface cases[J]. Composites Part A: Applied Science and Manufacturing,2018,107:633-646. doi: 10.1016/j.compositesa.2018.02.008 [19] REMACLE J F, LAMBRECHTS J, SENY B, et al. Blossom-Quad: A non-uniform quadrilateral mesh generator using a minimum-cost perfect-matching algorithm[J]. International Journal for Numerical Methods in Engineering,2012,89(9):1102-1119. [20] ROBINSON P, GALVANETTO U, TUMINO D, et al. Numerical simulation of fatigue-driven delamination using interface elements[J]. International Journal for Numerical Methods in Engineering,2005,63(13):1824-1848. doi: 10.1002/nme.1338 [21] MUNOZ J J, GALVANETTO U, ROBINSON P. On the numerical simulation of fatigue driven delamination with interface elements[J]. International Journal of Fatigue,2006(28):1136-1146. doi: 10.1016/j.ijfatigue.2006.02.003 [22] TUMINO D, CAPPELLO F. Simulation of fatigue delamination growth in composites with different mode mixtures[J]. Journal of Composite Materials,2007,41(20):2415-2441. doi: 10.1177/0021998307075439 [23] MAY M, HALLETT S R. A combined model for initiation and propagation of damage under fatigue loading for cohesive interface elements[J]. Composites Part A: Applied Science & Manufacturing,2010,41(12):1787-1796. [24] EKLIND A, WALANDER T, CARLBERGER T, et al. High cycle fatigue crack growth in Mode I of adhesive layers: modelling, simulation and experiments[J]. International Journal of Fracture,2014,190(1-2):125-146. doi: 10.1007/s10704-014-9979-8 [25] 邵洪. 层合复合材料低速冲击后疲劳损伤数值模拟[D]. 南京: 南京航空航天大学, 2018.SHAO Hong. Numerical simulation of fatigue damage in low-velocity impact laminated composites[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2018(in Chinese). [26] 廖兴升, 梁智洪, 傅继阳, 等. 基于频率变化预测玻璃纤维增强树脂复合材料层合板的剩余疲劳寿命[J]. 复合材料学报, 2021, 38(10): 3323-3337.LIAO Xingsheng, LIANG Zhihong, FU Jiyang, et al. Prediction of remaining fatigue life of glass fiber reinforced polymer laminates based on frequency change[J]. Acta Materiae Compositae Sinica, 2021, 38(10): 3323-3337 (in Chinese). [27] 姚思远, 陈秀华. 三维机织复合材料在拉压循环载荷下的疲劳性能[J]. 复合材料学报, 2018, 35(10):112-120.YAO Siyuan, CHEN Xiuhua. Fatigue behaviors of 3D woven composites under tension-compression cyclic loading[J]. Acta Materiae Compositae Sinica,2018,35(10):112-120(in Chinese). [28] 胡殿印, 曾雨琪, 来亮, 等. 复合材料高周弯曲疲劳试验与寿命预测[J]. 复合材料学报, 2018, 35(12):3407-3414.HU Dianyin, ZENG Yuqi, LAI Liang, et al. High-cycle bending fatigue and life prediction of composite material[J]. Acta Materiae Compositae Sinica,2018,35(12):3407-3414(in Chinese). [29] 任鹏, 夏峰. 复合材料层合板冲击后疲劳寿命试验研究与数值模拟[J]. 工程与试验, 2019, 59(2):7-9, 11.REN Peng, XIA Feng. Experimental study and numerical simulation of fatigue life of composite laminates after impact[J]. Engineering and Testing,2019,59(2):7-9, 11(in Chinese). [30] 丁京龙. 复合材料孔板结构渐进疲劳损伤分析[J]. 江苏科技信息, 2017, 4(17):35-39. doi: 10.3969/j.issn.1004-7530.2017.17.013DING Jinlong. Progressive fatigue damage analysis of composite orifice plate structures[J]. Jiangsu Science and Technology Information,2017,4(17):35-39(in Chinese). doi: 10.3969/j.issn.1004-7530.2017.17.013 [31] 刘英芝. 复合材料层合板疲劳行为研究[D]. 哈尔滨: 哈尔滨工业大学, 2015.LIU Yingzhi. Research on fatigue failure of property composites laminates[D]. Harbin: Harbin Institute of Technology, 2015(in Chinese). [32] 张晋华. 基于疲劳主曲线的复合材料层合疲劳寿命研究[D]. 哈尔滨: 哈尔滨工业大学, 2019.ZHANG Jinhua. Fatigue life prediction of composite laminates based on fatigue master curve[D]. Harbin: Harbin Institute of Technology, 2019(in Chinese). [33] PEERLINGS R H J, BREKELAMNS W A M, BORST R D, et al. Gradient-enhanced damage modelling of high-cycle fatigue[J]. International Journal for Numerical Methods in Engineering,2000,49(12):1547-1569. doi: 10.1002/1097-0207(20001230)49:12<1547::AID-NME16>3.0.CO;2-D [34] PAAS M H J W, SCHREURS P J G, BREKELAMNS W A M. A continuum approach to brittle and fatigue damage: Theory and numerical procedures[J]. International Journal of Solids and Structures,1993,30(4):579-599. doi: 10.1016/0020-7683(93)90189-E -






 下载:
下载: