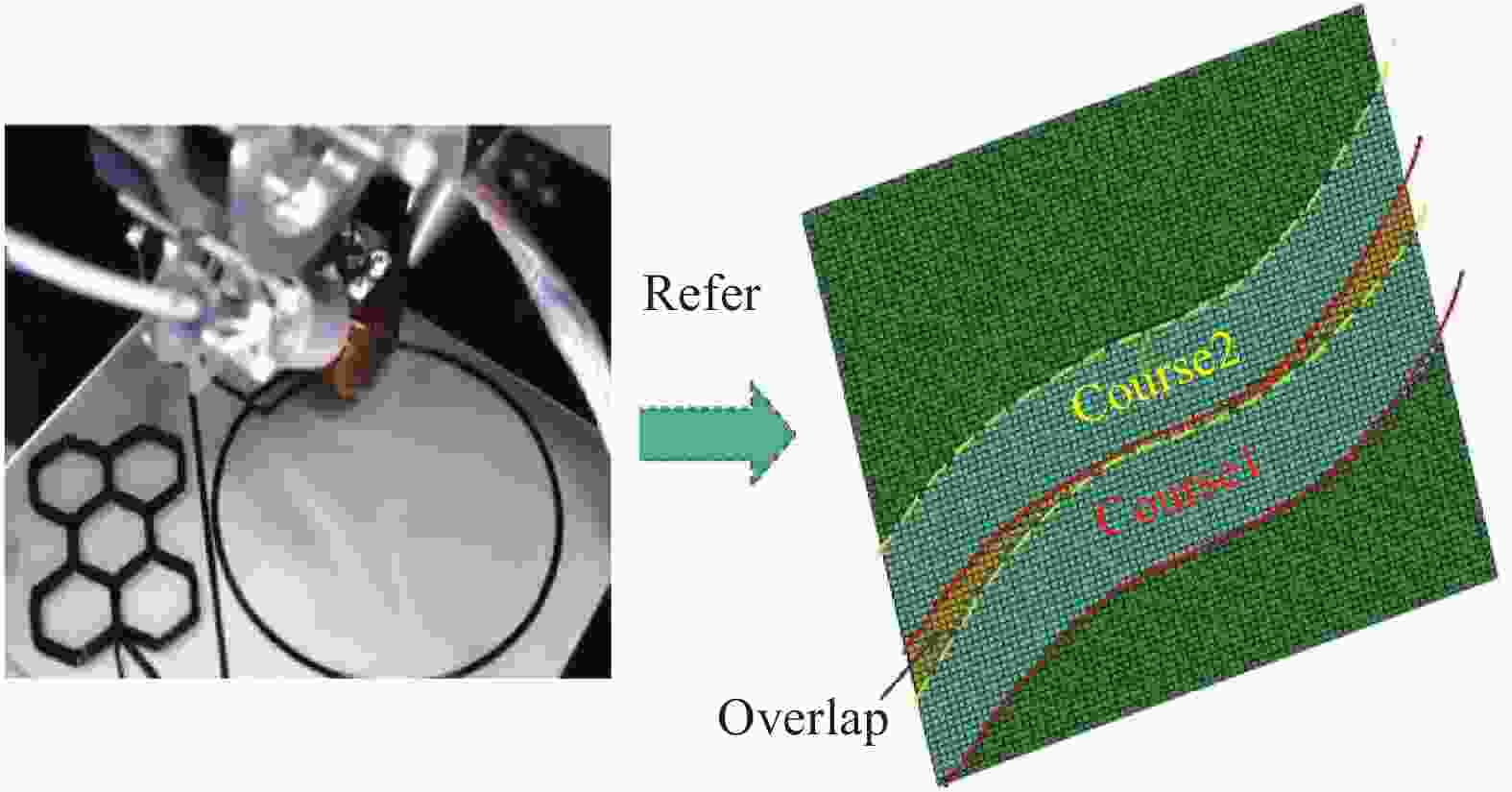
Process-induced deformation characteristics of variable stiffness composite laminates based on automatic placement technology
-
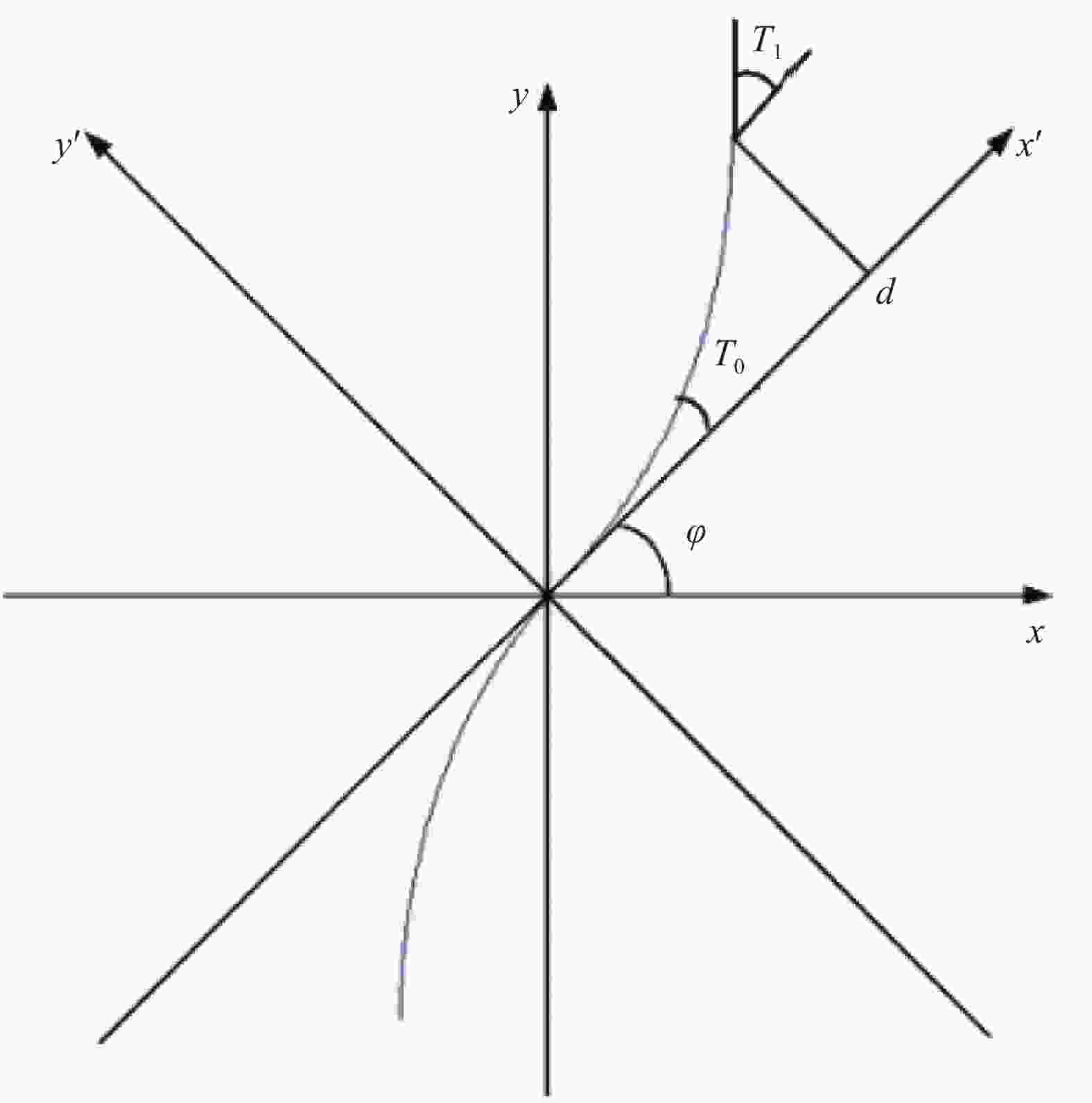
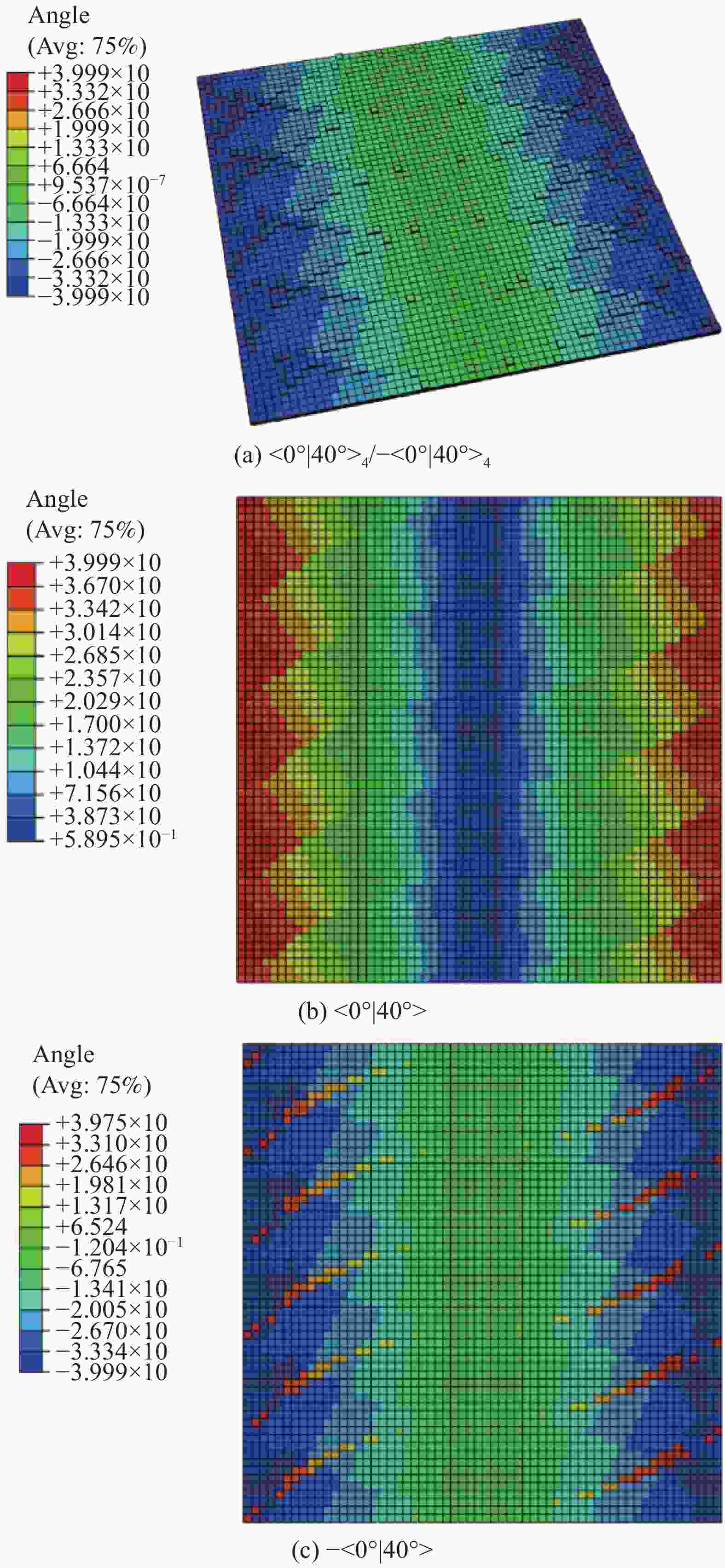
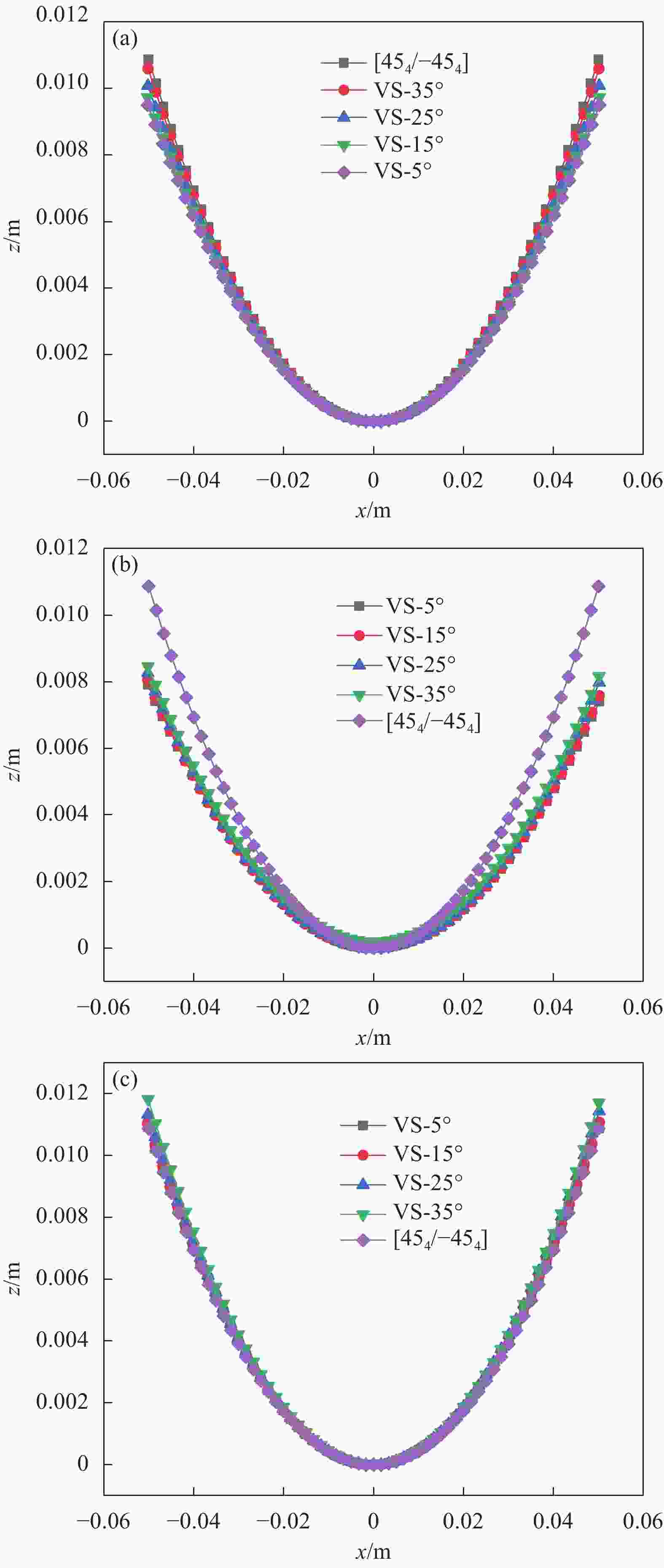
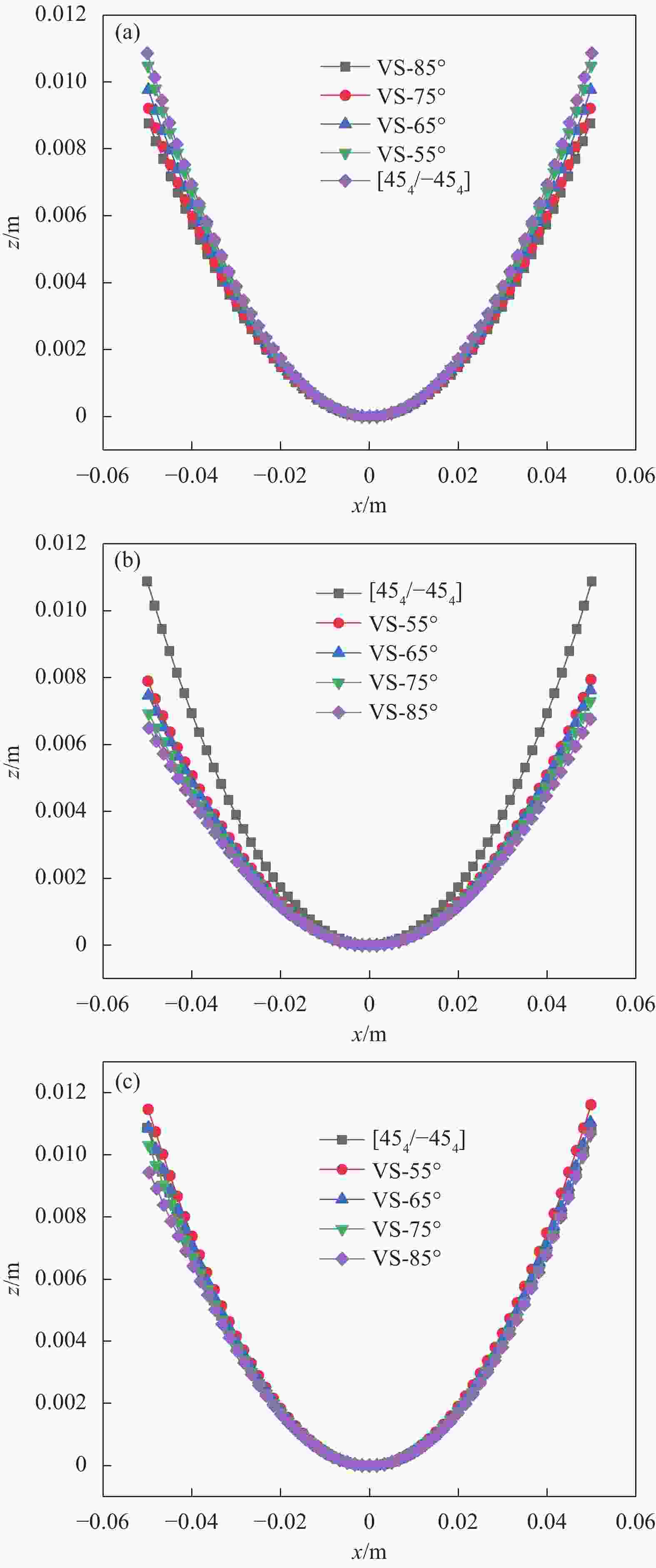
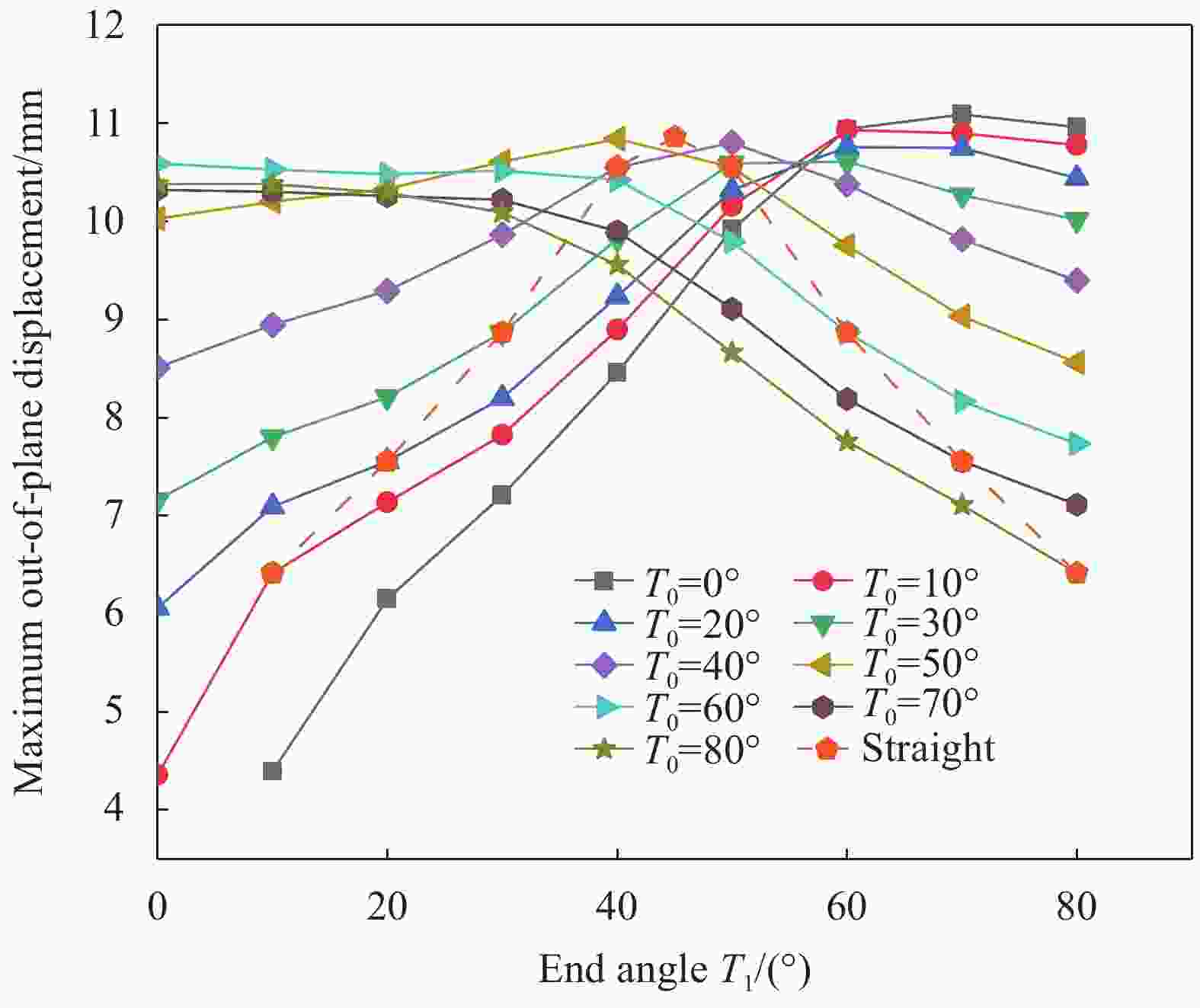
摘要: 准确预测和控制变刚度结构的固化变形是合理进行变刚度设计的关键环节，复合材料结构的固化变形不仅会影响结构的刚度强度特性、同时会对结构的装配性能产生影响。基于自动铺放技术，提出面向过程的丝束-路径-面板多级三维变刚度有限元模型算法。结合Kamal自催化固化动力学模型和广义Maxwell黏弹性本构模型进行热-化学-力多场耦合分析，计算固化过程中结构内部温度场、固化度场和残余应力场的变化，最终得到变刚度层合板的固化变形特性。通过参数化分析，研究了自动铺放过程中轨迹控制参数T0、T1和覆盖法则对结构固化变形产生的影响。研究结果表明：T0=45°时，当T1<T0变刚度结构的固化变形随着T1的增大而增大，当T1>T0，变刚度结构的固化变形随着
$ {T_1}$ 的增大而减小。100%覆盖法则有效减小结构的固化变形，而0%覆盖法则使固化变形增大。本文提出的方法可以有效预测工艺参数对变刚度结构固化变形的影响。Abstract: Accurately predicting and controlling the process-induced deformation of a variable stiffness structure is a key to obtain a reasonable variable stiffness design, the process-induced deformation of a composite structure will not only affect the stiffness and strength of the structure, but also affect the assembly performance of the structure. Based on the automatic placement technology, a process-oriented tow-course-panel multi-level three-dimensional variable stiffness finite element model algorithm was proposed. Combining the Kamal autocatalytic reaction curing kinetic model and the generalized Maxwell viscoelastic constitutive model for thermo-chemical-mechanical multi-field coupling analysis, the changes in the internal temperature field, curing degree field and residual stress field of the structure during the curing process were calculated, and the curing deformation of the variable stiffness structure was finally obtained. The results show that: when T0=45°, T1<T0, the curing deformation of the variable stiffness structure increases with the increase of T1. When T1>T0, the curing deformation of the variable stiffness structure decreases with the increase of T1. The 100% coverage rule effectively reduces the curing deformation of the structure, while the 0% coverage rule increases the curing deformation. The method proposed in this paper can effectively predict the effect of process parameters on the process-induced deformation of variable stiffness structures. -
图 2 面向铺放过程的变刚度复合材料层合板三维有限元模型建模方法
Figure 2. Three-dimensional finite element model modeling method for AFP of variable stiffness composite laminates
图 3 变刚度复合材料层合板纤维角度线性变化
Figure 3. Fiber angle linear variation of variable stiffness composite laminates
T1—End fiber angle; T0—Initial fiber angle; d—Characteristic length; φ—Reference coordinate system deflection angle
图 4 变刚度复合材料层合板丝束铺放路径模型
Figure 4. Course model of variable stiffness composite laminate
A'—A point on the centerline of the fiber course; A—A point on the course centerline normal; φA'—Fiber angle at point; φA—Fiber angle at point A
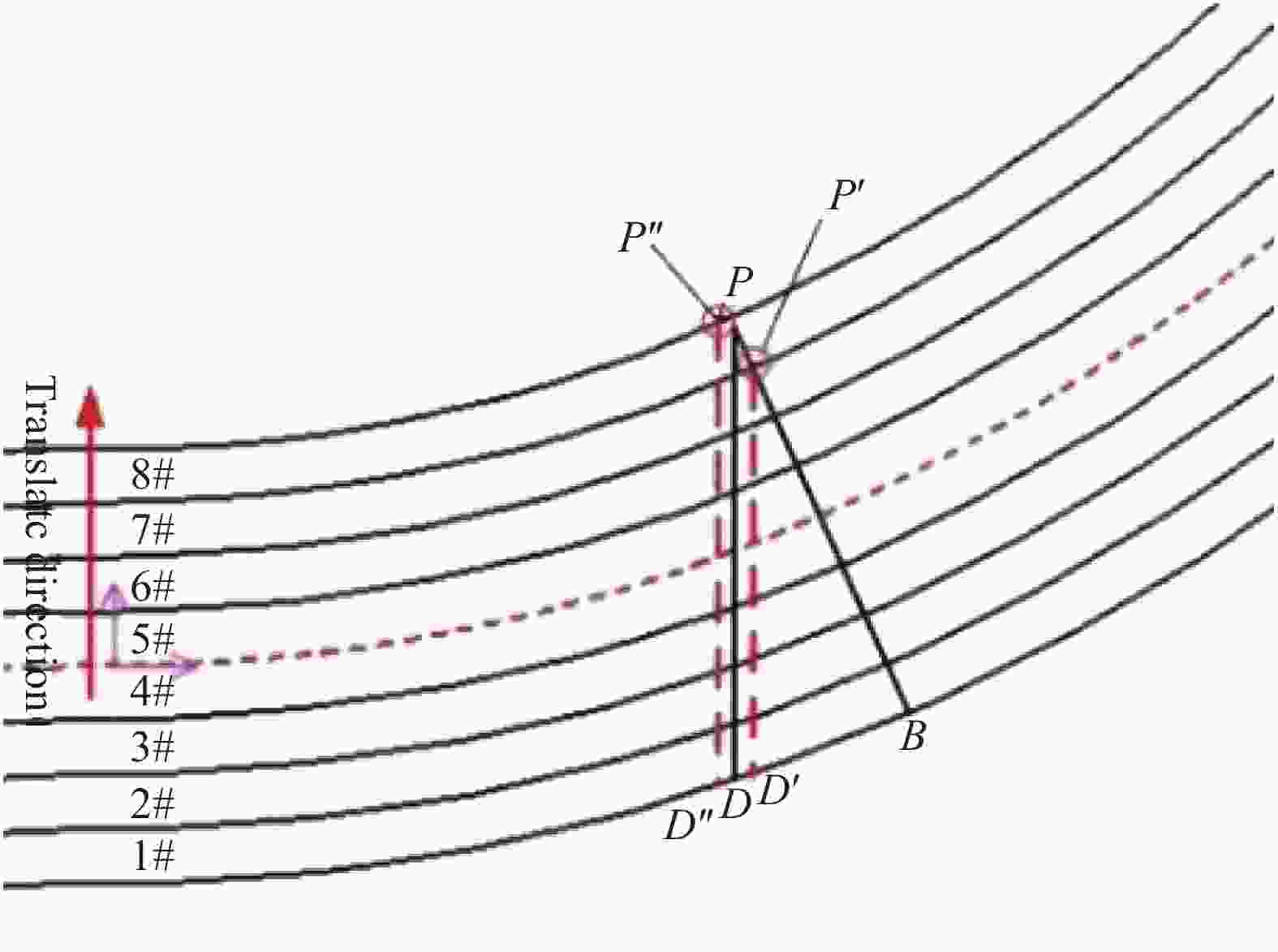
图 6 变刚度复合材料层合板丝束剪切区域计算模型
Figure 6. Tow cutting area calculation model of variable stiffness composite laminates
P—A point in the course; P', P''—Lower and upper boundary of the tow where point P is located; B—Intersection of the course normal where point P is located and the lower boundary of the course; D, D', D''—P, P', P'' corresponds to the point on the lower boundary of the course in the translation direction
图 7 三维变刚度层合板有限元模型
Figure 7. Three-dimensional finite element model of variable stiffness composite laminates
图 8 本文理论模型计算AS4/3501-6复合材料层合板固化度场和温度场有效性验证
Figure 8. Validation verification of theoretical model for calculating curing degree field and temperature field of AS4/3501-6 composite laminates
图 9 本文理论模型计算AS4/3501-6复合材料层合板残余应力有效性验证
Figure 9. Verification of the validity of the theoretical model in the calculation of residual stress of AS4/3501-6 composite laminates
y—y-axis; a—Length of side
图 10 AS4/3501-6变刚度层合板对角截面的面外位移：(a) 理想模型；(b) 100%覆盖法则；(c) 0%覆盖法则(
${T_0} > {T_1}$ )Figure 10. Out-of-plane displacement of diagonal section: (a) Ideal model; (b) 100% covering rule; (c) 0% covering rule (
${T_0} > {T_1}$ ) of AS4/3501-6 variable stiffness laminates
图 11 AS4/3501-6变刚度层合板对角截面的面外位移：(a) 理想模型；(b) 100%覆盖法则；(c) 0%覆盖法则(
${T_0} < {T_1}$ )Figure 11. Out-of-plane displacement of diagonal section: (a) Ideal model; (b) 100% covering rule; (c) 0% covering rule (
${T_0} < {T_1}$ ) of AS4/3501-6 variable stiffness laminates
图 12 纤维轨迹参数对AS4/3501-6变刚度层合板固化变形影响的参数化研究
Figure 12. Parameterized study of fiber trajectory parameters and effects on curing deformation of AS4/3501-6 variable stiffness composite laminates
表 1 AS4/3501-6碳纤维/环氧树脂预浸料热化学参数[17]
Table 1. Thermochemical parameters for the AS4/3501-6 carbon/epoxy prepreg[17]
Constant Value $\rho /\left( {{\rm{kg}} \cdot {{\rm{m}}^{{\rm{ - 3}}}}} \right)$ 1578 ${c_{\rm{p}}}/\left( {{\rm{J}} \cdot {{\left( {{\rm{kg}} \cdot {\rm{K}}} \right)}^{{\rm{ - 1}}}}} \right)$ 862 ${k_x}/\left( {{\rm{W}} \cdot {{\left( {{\rm{m}} \cdot {\rm{K}}} \right)}^{{\rm{ - 1}}}}} \right)$ 12.83 ${k_y} = {k_z}/\left( {{\rm{W}} \cdot {{\left( {{\rm{m}} \cdot {\rm{K}}} \right)}^{{\rm{ - 1}}}}} \right)$ 0.4135 ${H_{\rm{T}}}/\left( {{\rm{J}} \cdot {\rm{k}}{{\rm{g}}^{{\rm{ - 1}}}}} \right)$ $198.6 \times {10^3}$ ${{{A_1}} /{{\rm{mi}}{{\rm{n}}^{{\rm{ - 1}}}}}}$ $2.101 \times {10^9}$ ${{{A_2}} /{{\rm{mi}}{{\rm{n}}^{{\rm{ - 1}}}}}}$ $ - 2.014 \times {10^9}$ ${{{A_3}} /{{\rm{mi}}{{\rm{n}}^{{\rm{ - 1}}}}}}$ $1.96 \times {10^5}$ ${{\Delta {E_1}} /{\left( {{\rm{J}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}} \right)}}$ $8.07 \times {10^4}$ ${{\Delta {E_2}} / {\left( {{\rm{J}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}} \right)}}$ $7.78 \times {10^4}$ ${{\Delta {E_3}}/ {\left( {{\rm{J}} \cdot {\rm{mo}}{{\rm{l}}^{{\rm{ - 1}}}}} \right)}}$ $5.66 \times {10^4}$ Notes: $\rho $—Density of material; Cp—Specific heat of composite; kx and ky—Heat conduction coefficient of prepreg along x direction and y direction; HT—Total heat of the curing of unit mass; A1, A2, A3—Frequency factors of autocatalysis model; $\Delta {E_1}$,$\Delta {E_2}$,$\Delta {E_3}$—Activation energy of autocatalysis model. 
下载: 导出CSV
表 2 AS4/3501-6碳纤维/环氧树脂预浸料参考固化度下的松弛时间和权重因子[19]
Table 2. Relaxation times and weighting factors at the reference degree of cure of AS4/3501-6 carbon/epoxy prepreg[19]
m ${\tau _m}/\min $ ${W_m}$ 1 $2.922 \times {10^1}$ 0.059 2 $2.921 \times {10^3}$ 0.066 3 $1.824 \times {10^5}$ 0.083 4 $1.103 \times {10^7}$ 0.112 5 $2.831 \times {10^8}$ 0.154 6 $7.943 \times {10^9}$ 0.262 7 $1.953 \times {10^{11}}$ 0.184 8 $3.315 \times {10^{12}}$ 0.049 9 $4.917 \times {10^{14}}$ 0.025 Notes: ${\tau _m}$—Discrete stress relaxation time for the ${m{{\rm{th}}}}$ Maxwell element; ${W_m}$—Mass factor for the ${m{{\rm{th}}}}$ Maxwell element.
下载: 导出CSV
表 3 AS4/3501-6预浸料未松弛状态下热力学参数
Table 3. Thermomechanical parameters for the unrelaxed AS4/3501-6 prepreg
Parameter Value Elastic modulus ${E_1}/{\rm{MPa}}$ 126000 Elastic modulus ${E_2} = {E_3}/{\rm{MPa}}$ 8300 Shear modulus ${G_{12}} = {G_{13}}/{\rm{MPa}}$ 4100 Shear modulus ${G_{23}}/{\rm{MPa}}$ 2800 Thermal expansion coefficient ${\alpha _1}/\left( {{\rm{1}}{{\rm{0}}^{{\rm{ - 6}}}} \cdot {}^{\circ} {{\rm{C}}^{ - 1}}} \right)$ 0.5 Thermal expansion coefficient ${\alpha _2} = {\alpha _3}/\left( {{\rm{1}}{{\rm{0}}^{{\rm{ - 6}}}} \cdot {}^{\circ}{{\rm{C}}^{ - 1}}} \right)$ $35.3$ Chemical shrinkage coefficient ${\varPhi _1}/{\rm{1}}{{\rm{0}}^{{\rm{ - 6}}}}$ −167 Chemical shrinkage coefficient ${\varPhi _2} = {\varPhi _3}/{\rm{1}}{{\rm{0}}^{{\rm{ - 6}}}}$ −8810
下载: 导出CSV
-
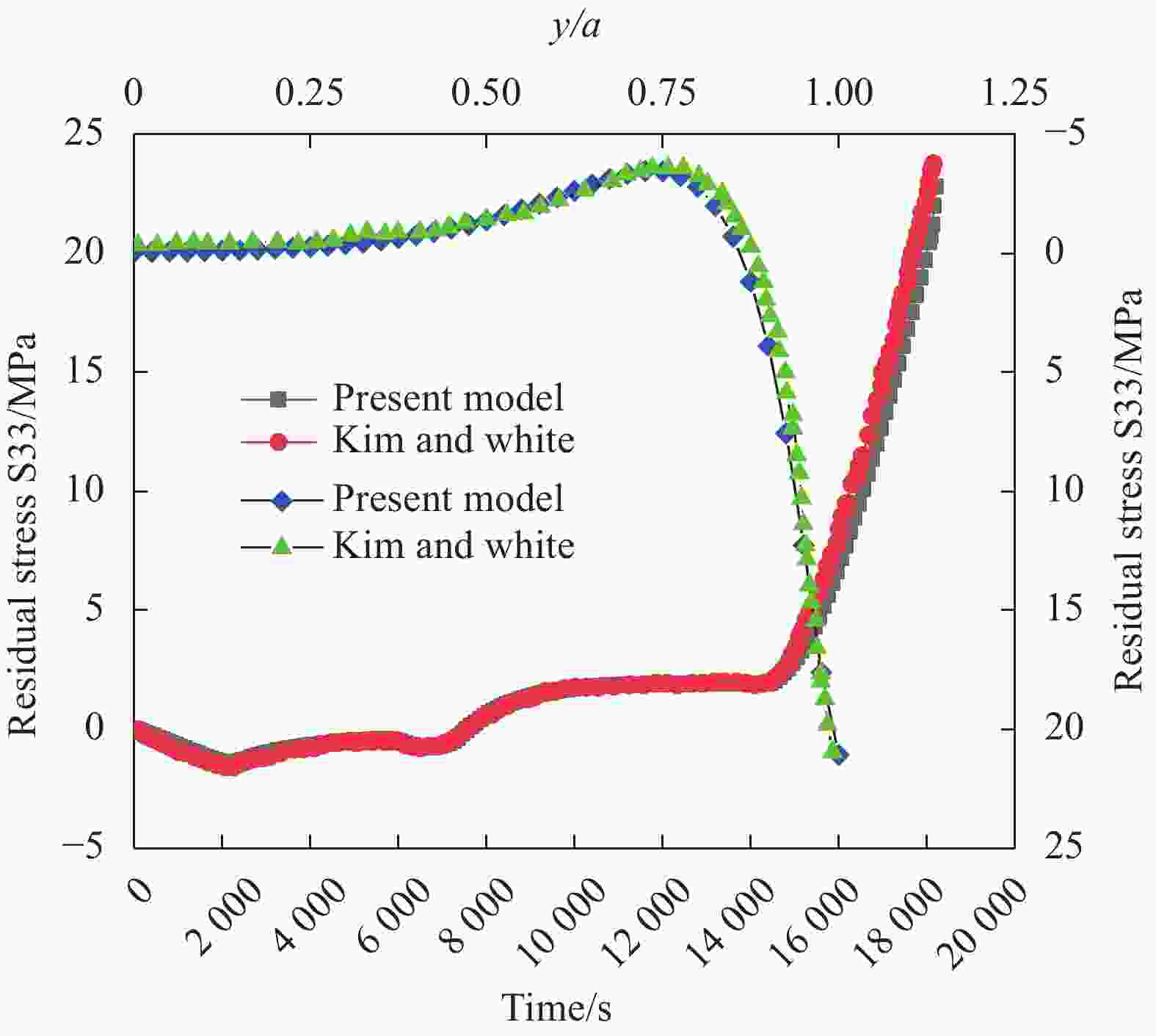
[1] GÜRDAL Z, OLMEDO R. In-plane response of laminates with spatially varying fiber orientations-Variable stiffness concept[J]. AIAA Journal,1993,31(4):751-758. doi: 10.2514/3.11613 [2] GÜRDAL Z, TATTING B F, WU C K. Variable stiffness composite panels: Effects of stiffness variation on the in-plane and buckling response[J]. Composites Part A: Applied Science and Manufacturing,2008,39(5):911-922. doi: 10.1016/j.compositesa.2007.11.015 [3] LOPES C S, GÜRDAL Z, CAMANHO P P. Variable-stiffness composite panels: Buckling and first-ply failure improvements over straight-fibre laminates[J]. Computers & Structures,2008,86(9):897-907. [4] 富宏亚, 曹忠亮, 杜霖, 等. Bezier曲线变角度层合板设计及屈曲特性分析[J]. 复合材料学报, 2017, 34(8):1729-1735.FU Hongya, CAO Zhongliang, DU Lin, et al. Design of Bezier curve variable angle laminates and analysis on buckling property[J]. Acta Materiae Composite Sinica,2017,34(8):1729-1735(in Chinese). [5] 卫宇璇, 张明, 刘佳, 等. 基于自动铺放技术的高精度变刚度复合材料层合板屈曲性能[J]. 复合材料学报, 2020, 37(11):2807-2815.WEI Yuxuan, ZHANG Ming, LIU Jia, et al. Buckling performance of high-precision variable stiffness composites laminate based on automatic placement technology[J]. Acta Materiae Compositae Sinica,2020,37(11):2807-2815(in Chinese). [6] HAO P, LIU C, YUAN X, et al. Buckling optimization of variable-stiffness composite panels based on flow field function[J]. Composite Structures,2017,181:240-255. doi: 10.1016/j.compstruct.2017.08.081 [7] 秦永利, 祝颖丹, 范欣愉, 等. 纤维曲线铺放制备变刚度复合材料层合板的研究进展[J]. 玻璃钢/复合材料, 2012(1):61-66.QIN Yongli, ZHU Yindan, FAN Xinyu, et al. Research progress on fabrication of variable stiffness composite la-minates by fiber curve laying[J]. Fiber Reinforced Plastics/Composites,2012(1):61-66(in Chinese). [8] DONG C. Development of a model for predicting the tran-sverse coefficients of thermal expansion of unidirectional carbon fibre reinforced composites[J]. Applied Compo-site Materials,2008,15(3):171-182. doi: 10.1007/s10443-008-9065-3 [9] 张纪奎, 郦正能, 关志东, 等. 固化度与固化收缩对非对称复合材料层合板固化变形的影响[J]. 复合材料学报, 2007, 24(2):120-124. doi: 10.3321/j.issn:1000-3851.2007.02.021ZHANG Jikui, LI Zhengneng, GUAN Zhidong, et al. In-fluence of degree of cure and cure shrinkage on the finial deformation of the unsymmetric composite laminates[J]. Acta Materiae Compositae Sinica,2007,24(2):120-124(in Chinese). doi: 10.3321/j.issn:1000-3851.2007.02.021 [10] 元振毅, 王永军, 王俊彪, 等. 基于模具-制件相互作用的复合材料制件固化变形数值模型[J]. 复合材料学报, 2016, 33(4):902-909.YUAN Zhenyi, WANG Yongjun, WANG Junbiao, et al. Numerical model on curing deformation of composite part based on tool-part interaction[J]. Acta Materiae Compositae Sinica,2016,33(4):902-909(in Chinese). [11] ZENG X, RAGHAVAN J. Role of tool-part interaction in process-induced warpage of autoclave-manufactured compo-site structures[J]. Composites Part A: Applied Science and Manufacturing,2010,41(9):1174-1183. doi: 10.1016/j.compositesa.2010.04.017 [12] KAUSHIK V, RAGHAVAN J. Experimental study of tool-part interaction during autoclave processing of thermoset polymer composite structures[J]. Composites Part A: Applied Science and Manufacturing,2010,41(9):1210-1218. doi: 10.1016/j.compositesa.2010.05.003 [13] FERNLUND G, RAHMAN N, COURDJI R, et al. Experimental and numerical study of the effect of cure cycle, tool surface, geometry, and lay-up on the dimensional fidelity of autoclave-processed composite parts[J]. Composites Part A: Applied Science and Manufacturing,2002,33(3):341-351. doi: 10.1016/S1359-835X(01)00123-3 [14] ZHANG G, WANG J, NI A, et al. Process-induced residual stress of variable-stiffness composite laminates during cure[J]. Composite Structures,2018,204:12-21. doi: 10.1016/j.compstruct.2018.07.040 [15] ZHANG G, WANG J, NI A, et al. Process-induced deformation of L-shaped variable-stiffness composite structures during cure[J]. Composite Structures,2019,230:111461. doi: 10.1016/j.compstruct.2019.111461 [16] ZHANG G, WANG J, NI A. Process-induced stress and deformation of variable-stiffness composite cylinders during curing[J]. Materials,2019,12(2):259-272. doi: 10.3390/ma12020259 [17] ZHU Q, GEUBELLE P H, LI M, et al. Dimensional accuracy of thermoset composites: simulation of process-induced residual stresses[J]. Journal of Composite Materials,2015,35(24):2171-2205. [18] 闵荣, 元振毅, 王永军, 等. 基于黏弹性本构模型的热固性树脂基复合材料固化变形数值仿真模型[J]. 复合材料学报, 2017, 34(10):2254-2262.MIN Rong, YUAN Zhenyi, WANG Yongjun, et al. Nu-merical simulation for curing deformation of resin matrix thermosetting composite using viscoelastic constitutive model[J]. Acta Materiae Compositae Sinica,2017,34(10):2254-2262(in Chinese). [19] Kim Y K, WHITE S R. Stress relaxation behavior of 3501-6 epoxy resin during cure[J]. Polymer Engineering & Science,1996,36(23):2852-2862. [20] WHITE S R, KIM Y K. Process-induced residual stress analysis of AS4/3501-6 composite material[J]. Mechanics of Composite Materials and Structures,1998,5(2):153-186. doi: 10.1080/10759419808945897 -
下载:
点击查看大图
计量
- 文章访问数: 1104
- HTML全文浏览量: 593
- PDF下载量: 85
- 被引次数: 0
