平纹编织碳纤维增强树脂复合材料离散电导率建模方法

张荣华; 史可宇; 李硕; 张一帆

doi:10.13801/j.cnki.fhclxb.20200327.001

平纹编织碳纤维增强树脂复合材料离散电导率建模方法

doi: 10.13801/j.cnki.fhclxb.20200327.001

张荣华^1, ,,
史可宇^2,,
李硕^2,,
张一帆³

1.
天津工业大学　人工智能学院，天津 300387
2.
天津工业大学　电气工程及自动化学院，天津 300387
3.
天津工业大学　复合材料研究所先进纺织复合材料教育部重点实验室，天津 300387

基金项目: 国家自然科学基金(61872269)

详细信息

通讯作者:
张荣华，博士，副教授，硕士生导师，研究方向为电磁涡流无损检测及其相关理论　E-mail：rh_zhang_2005@163.com

中图分类号: TH701
计量
- 文章访问数: 1363
- HTML全文浏览量: 535
- PDF下载量: 98
- 被引次数: 0
出版历程
- 收稿日期: 2020-02-04
- 录用日期: 2020-03-16
- 网络出版日期: 2020-03-27
- 刊出日期: 2020-12-15

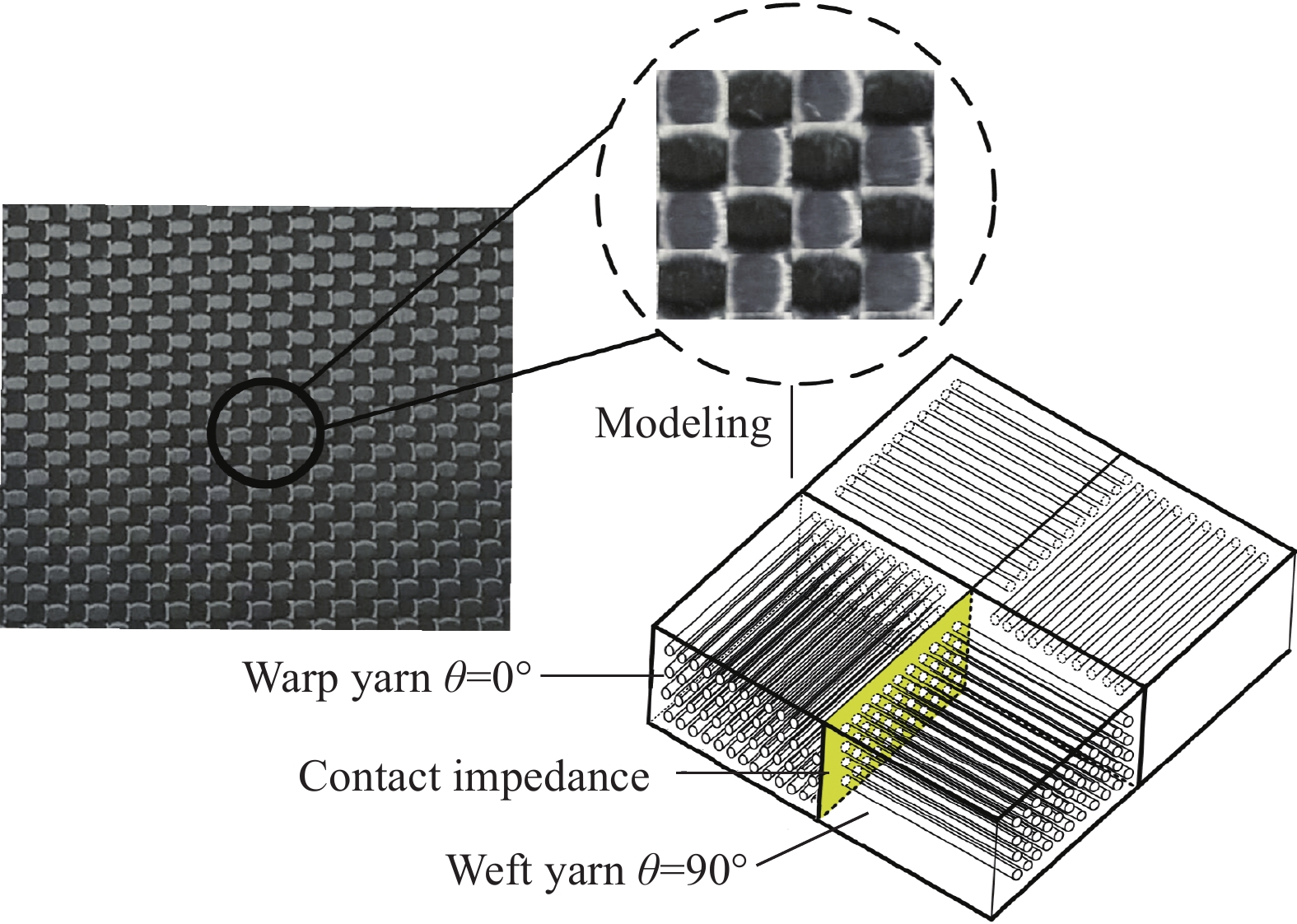
Discrete conductivity modeling method for plain weave carbon fiber reinforced resin composites

ZHANG Ronghua^{1
, ,},
SHI Keyu^2
,,
LI Shuo^2
,,
ZHANG Yifan³

1.
School of Artificial Intelligence, Tiangong University, Tianjin 300387, China
2.
School of Electrical Engineering and Automation, Tiangong University, Tianjin 300387, China
3.
Ministry of Education Key Laboratory of Advanced Textile Composite Materials, Institute of Textile Composites, Tiangong University, Tianjin 300387, China

摘要

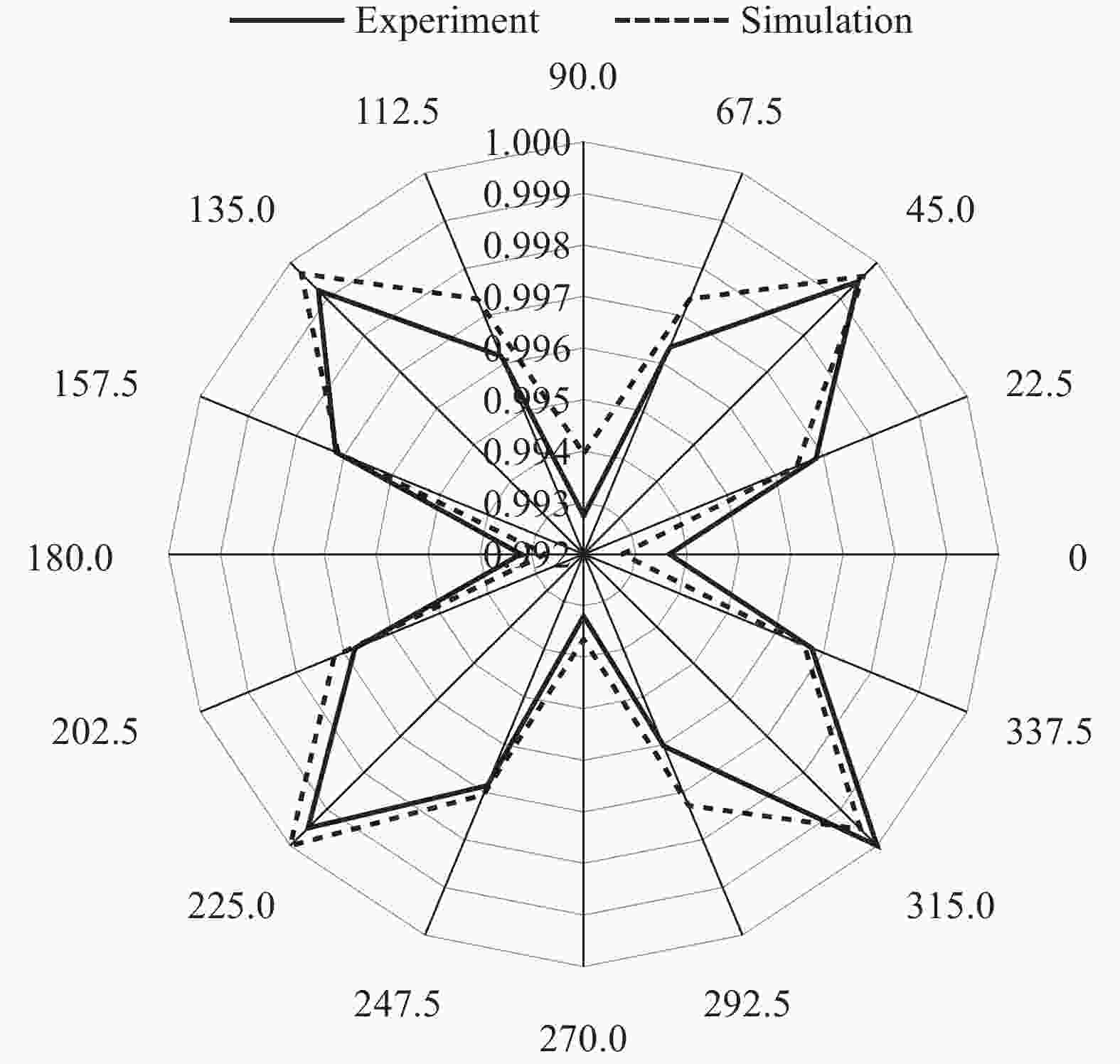
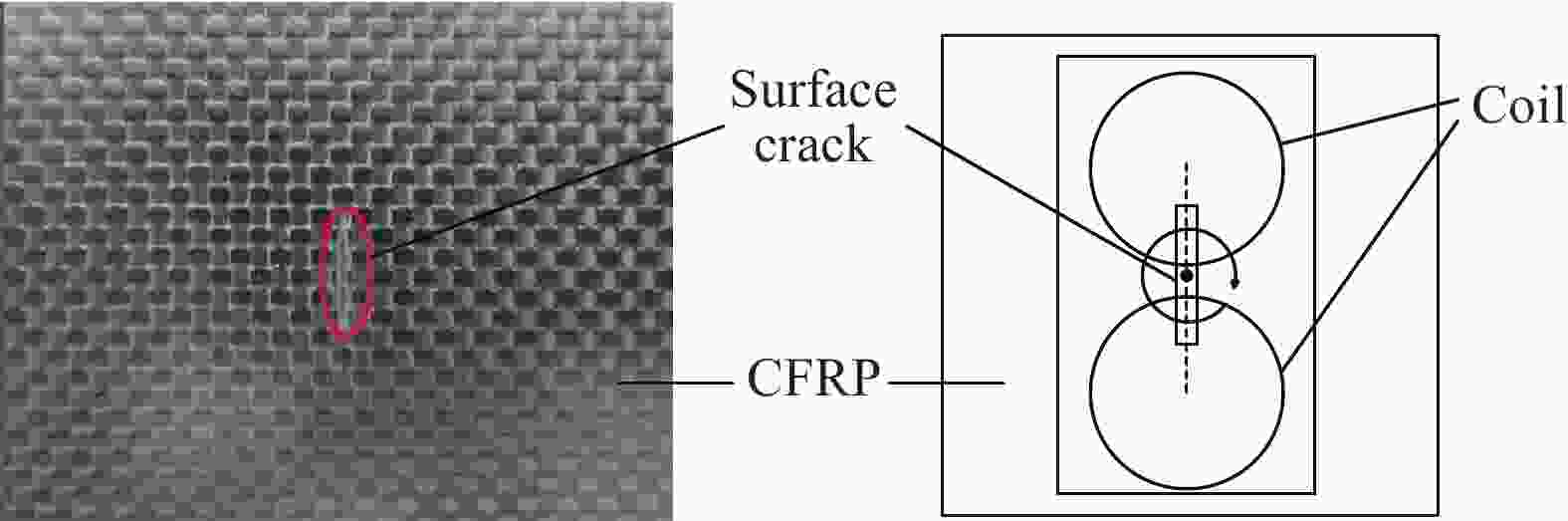
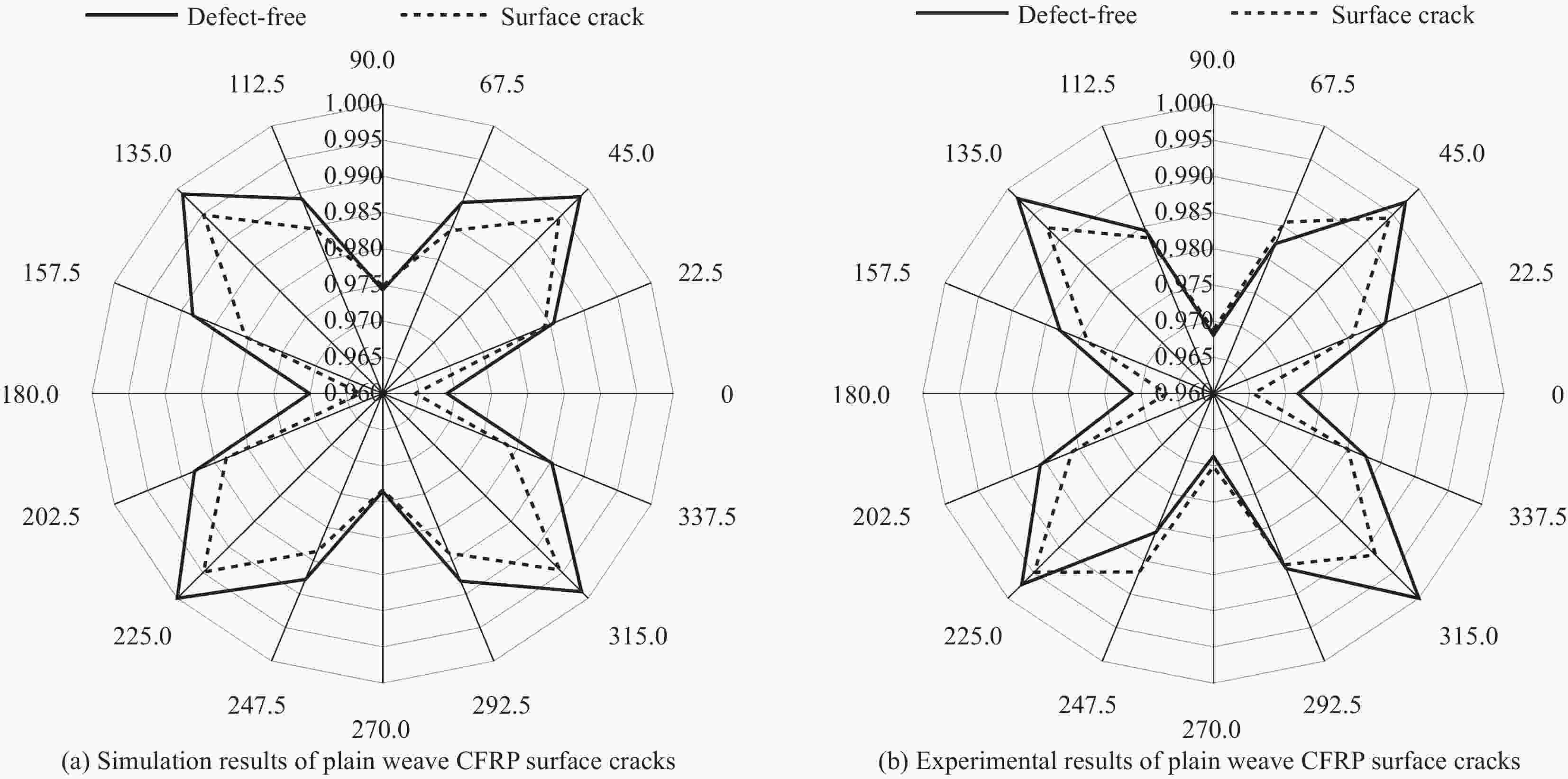
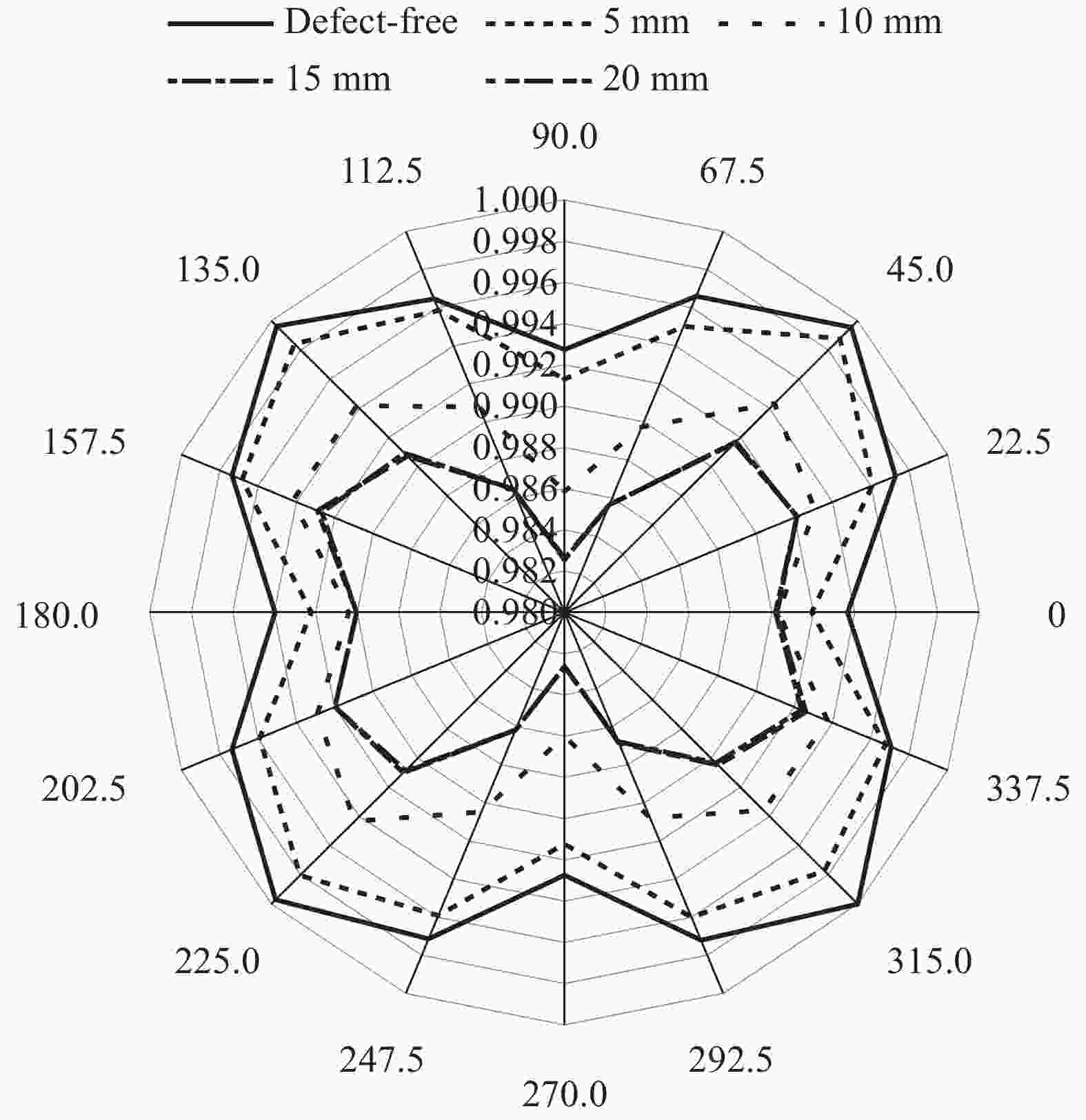
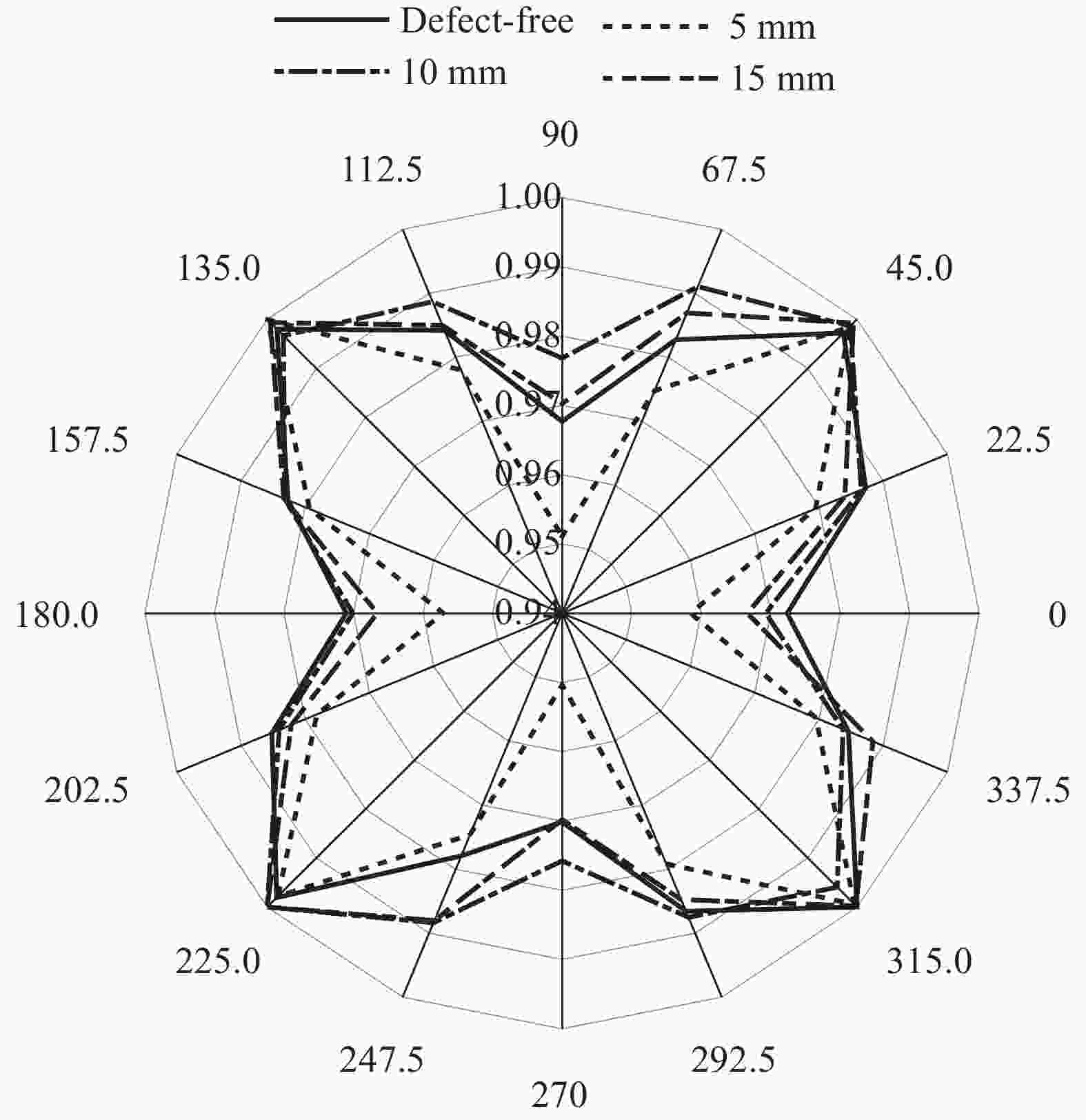
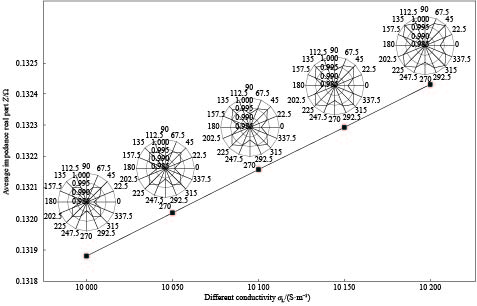
摘要: 编织碳纤维增强树脂复合材料(CFRP)的电阻抗分布具有各向异性、异质性、几何结构复杂等特点。建立电阻抗分布模型是利用电磁涡流无损检测技术获取编织CFRP缺陷及疲劳损伤信息的关键关节。基于电阻抗张量建模理论，采用多层编织结构CFRP二维平面的分块均化电学特性表征方法，建立编织结构CFRP的简化电阻抗分布模型，从而实现编织结构CFRP电磁特性的精确、快速有限元分析。在有限元仿真基础上，通过设计双空气旋转线圈电磁传感器对平纹编织CFRP进行电磁无损检测，选用阻抗的极坐标图描述被测材料沿不同方向的阻抗变化趋势，通过实验验证有限元建模的正确性。最后利用所提出的建模方法模拟了双空气旋转线圈传感器对平纹编织CFRP的结构缺陷及循环载荷疲劳的检测效果。
- 涡流检测 /
- 有限元法 /
- 平纹编织 /
- CFRP /
- 缺陷检测
Abstract: The electrical impedance distribution of carbon fiber reinforced resin composites (CFRP) with weaving technology has the characteristics of anisotropy, heterogeneity and complex geometric structure. Establishing the electrical impedance distribution model is the key joint to obtain the defect and fatigue damage information of CFRP in the braiding process by using electromagnetic eddy current nondestructive testing technology. Based on the theory of electrical impedance tensor modeling, the method of characterizing the electrical characteristics of the multi-layer braided structure CFRP two-dimensional plane was established, and a simplified electrical impedance distribution model of the braided CFRP was established, thereby realizing the accurate and fast finite element analysis of the electromagnetic properties of the braided CFRP. Based on the finite element simulation, the electromagnetic nondestructive testing of plain weave CFRP was designed by designing a dual-air rotating coil electromagnetic sensor. The polar coordinate chart of the impedance was used to describe the impedance change trend of the measured material in different directions. The correctness of the finite element modeling was proved by experiment. Finally, the proposed modeling method was used to simulate the detection effect of the double air rotating coil sensor on the structural defects and cyclic load fatigue of the plain weave CFRP.
- eddy current testing /
- finite element method /
- plain weave /
- CFRP /
- defect detection

HTML全文

图 1 平纹编织碳纤维增强树脂复合材料(CFPR)离散化电导率模型

Figure 1. Plain weave carbon fiber reinforced polymer (CFPR)discretization conductivity model

下载: 全尺寸图片幻灯片

图 2 平纹编织CFRP涡流检测系统的仿真模型

Figure 2. Simulation model of plain weave CFRP eddy current detection system

下载: 全尺寸图片幻灯片

图 3 双线圈传感器产生的涡流分布

Figure 3. Distribution of the eddy currents created by the double-coils-rotatable sensor

下载: 全尺寸图片幻灯片

图 4 双线圈旋转传感器不同旋转角的平纹编织CFRP归一化阻抗变化实部极坐标的仿真结果

Figure 4. Simulation results of normalized impedance change of plain weave CFRP with different rotation angles of double-coils-rotatable sensor

下载: 全尺寸图片幻灯片

图 5 平纹编织CFRP涡流检测实验系统

Figure 5. Eddy current detection experiment system for plain weave CFRP

下载: 全尺寸图片幻灯片

图 6 传感器的相-频响应曲线

Figure 6. Phase-frequency curve of the sensor

下载: 全尺寸图片幻灯片

图 7 使用双圆旋转线圈检测平纹编织CFRP的阻抗特性实验和仿真极坐标图对比

Figure 7. Comparison of experimental and simulated polar coordinate diagrams of impedance characteristics of plain woven CFRP using double-coils-rotatable coils

下载: 全尺寸图片幻灯片

图 8 含缺陷平纹编织CFRP试样

Figure 8. Plain weave CFRP specimen with defect

下载: 全尺寸图片幻灯片

图 9 使用双圆旋转线圈检测的含表面裂纹（长为10 mm）的平纹编织CFRP的归一化阻抗变化实验和仿真结果

Figure 9. Experimental and simulation results of normalized impedance variation of plain weave CFRP with surface cracks (10 mm length) detected by double-coils-rotatable coils

下载: 全尺寸图片幻灯片

图 10 含表面裂纹的平纹编织CFRP使用双圆旋转线圈检测的仿真模型

Figure 10. Simulation model of plain weave CFRP with surface cracks using double-coils-rotatable coils detection

下载: 全尺寸图片幻灯片

图 11 使用双圆旋转线圈检测的含不同长度表面裂纹的平纹编织CFRP的归一化阻抗变化仿真结果

Figure 11. Simulation results of normalized impedance variation of plain weave CFRP with different lengths surface cracks detected by double-coils-rotatable coils

下载: 全尺寸图片幻灯片

图 12 使用双圆旋转线圈检测的含不同长度表面裂纹的平纹编织CFRP的归一化阻抗变化实验结果

Figure 12. Experimental results of normalized impedance variation of plain weave CFRP with different lengths surface cracks detected by double-coils-rotatable coils

下载: 全尺寸图片幻灯片

图 13 使用双圆旋转线圈检测的平纹编织CFRP拉-拉疲劳的仿真结果

Figure 13. Simulation results of pull-pull fatigue of plain-weave CFRP detected by double-coils-rotatable coils

下载: 全尺寸图片幻灯片

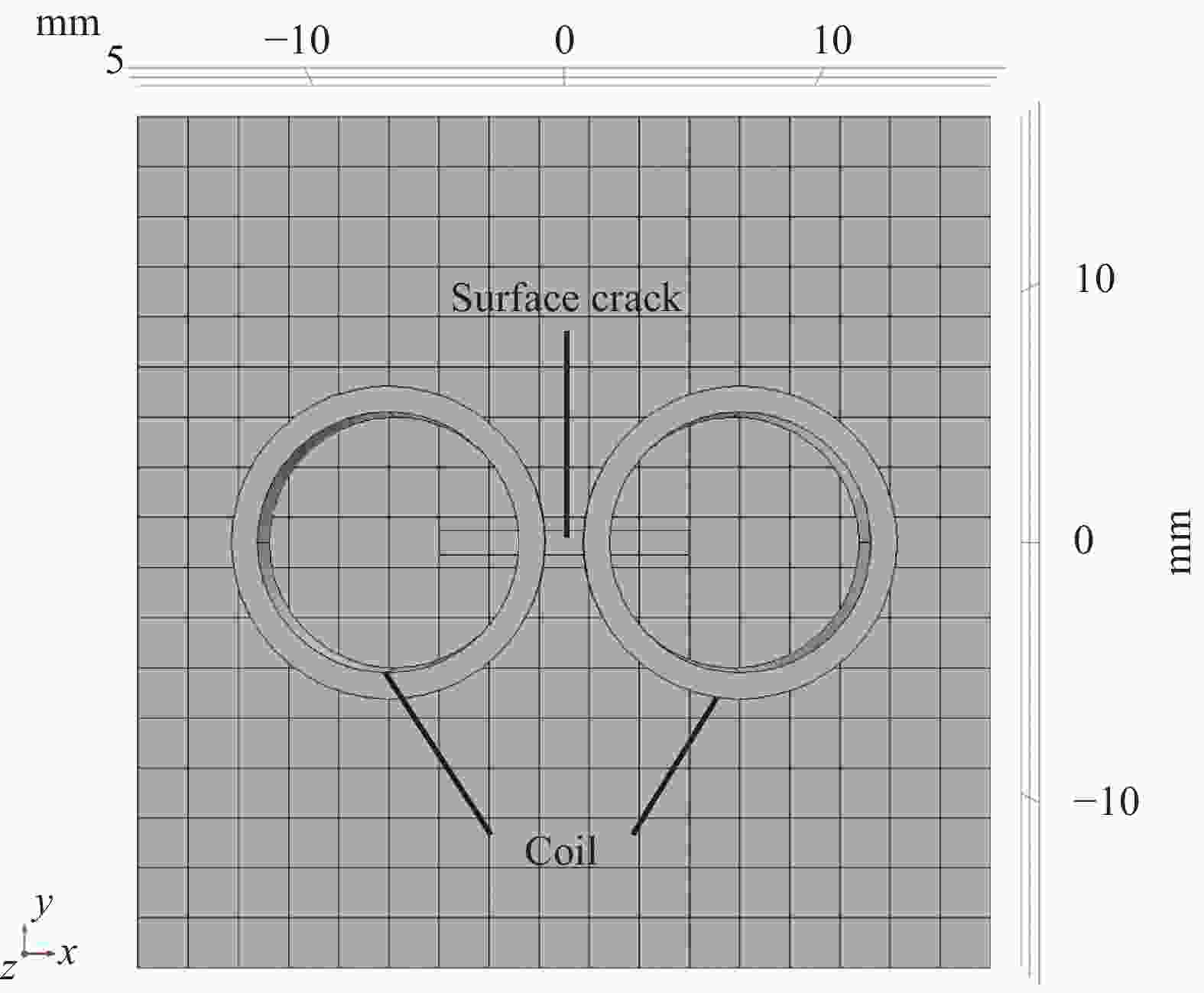
表 1 平纹编织CFRP涡流检测系统的几何和物理参数

Table 1. Geometric and physical parameters of plain weave CFRP eddy current detection system

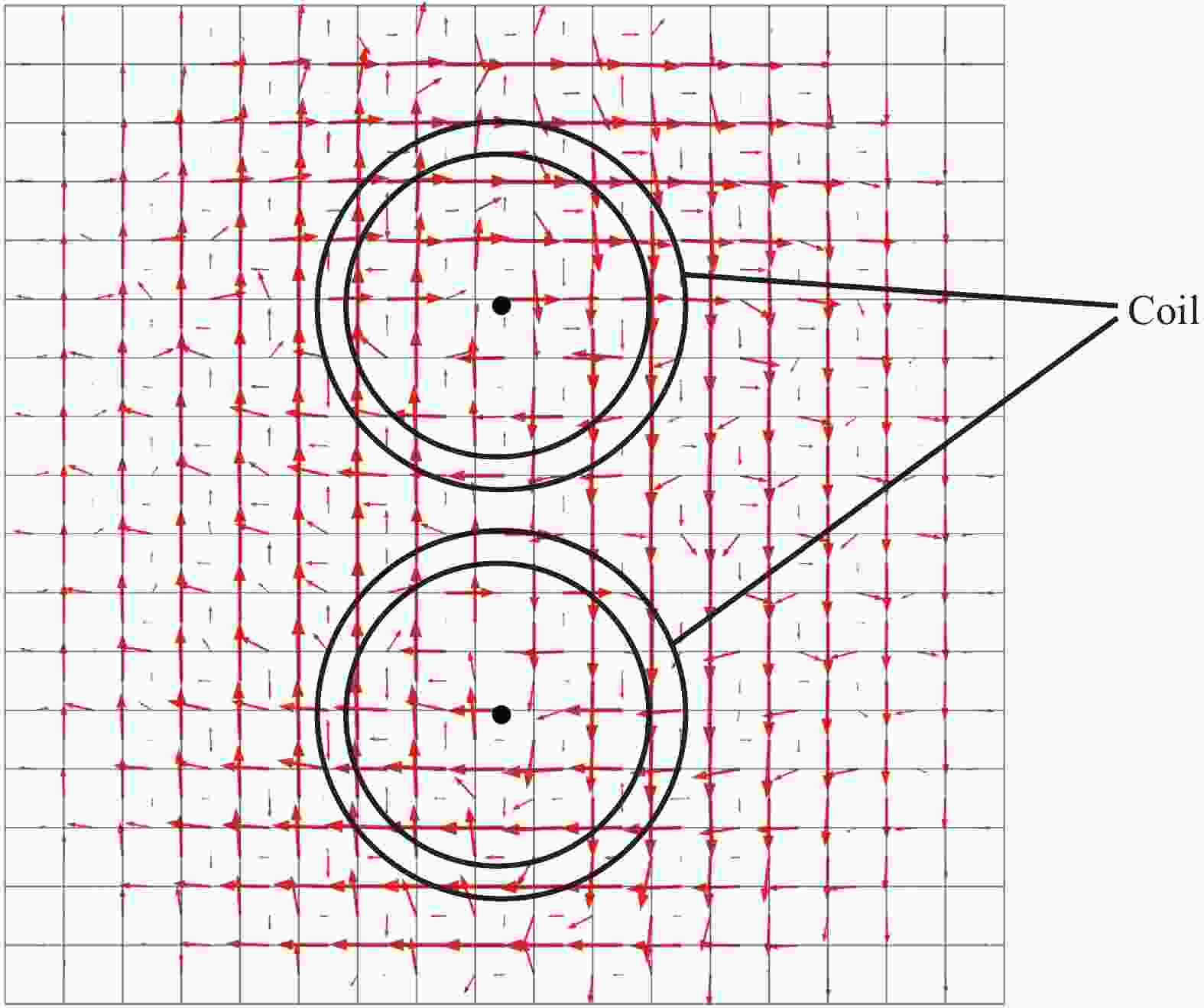
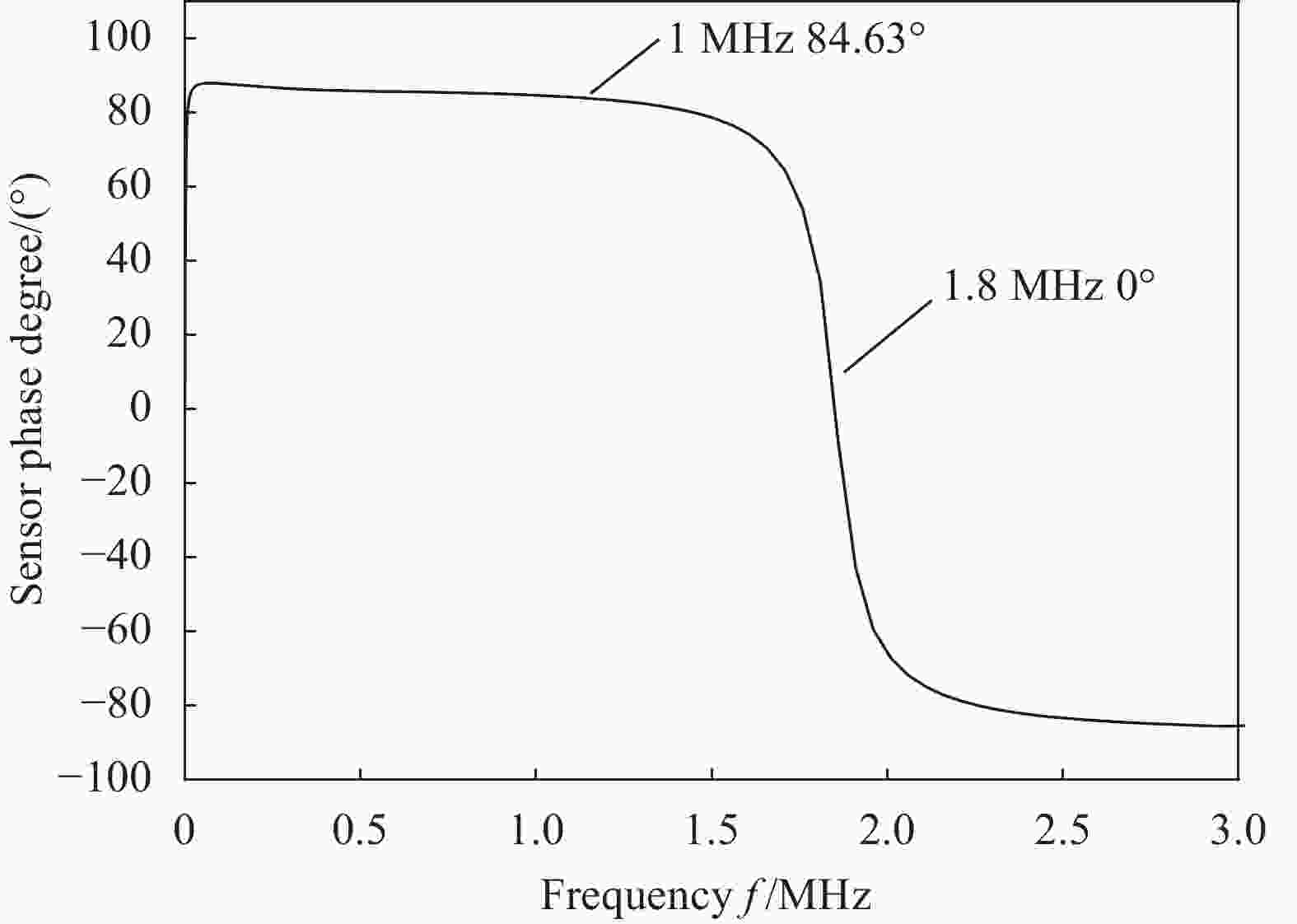
Coil	Parameter
Number of turns	150
Inner radius/mm	10
Outer radius/mm	12
Coil spacing d/mm	13.5
Current intensity ${I_{{\rm{source}}}}$/A	0.02
Frequency/MHz	1
Lift-off/mm	0.5
Angular step of the rotation α/(°)	22.5
Warp yarn conductivity/(S·m^–1)	(10 000, 10, 0)
Weft yarn conductivity/(S·m^–1)	(10, 10 000, 0)

下载: 导出CSV

参考文献(26)

[1]	韩帅, 段跃新, 李超, 等. 不同针织结构经编碳纤维复合材料弯曲性能[J]. 复合材料学报, 2011, 28(5):52-57. HAN Shuai, DUAN Yuexin, LI Chao, et al. Bending properties of non-crimp stitched carbon fabric reinforced composites of different knit patte[J]. Acta Materiae Compositae Sinica,2011,28(5):52-57(in Chinese).
[2]	吴良义. 先进复合材料的应用扩展: 航空、航天和民用航空先进复合材料应用技术和市场预测[J]. 化工新型材料, 2012, 40(1):4-9, 91. doi: 10.3969/j.issn.1006-3536.2012.01.002 WU Liangyi. The applcation extend of advanced compo-site materials: Technology markets of ACM application in aeronutics, astrnautics and civil aviation[J]. New Chem-ical Materials,2012,40(1):4-9, 91(in Chinese). doi: 10.3969/j.issn.1006-3536.2012.01.002
[3]	王冰佳, 黄强, 呼慧. 复合材料及碳纤维在风力机叶片中的应用现状[J]. 电站系统工程, 2019, 35(3):43-45. WANG Bingjia, HUANG Qiang, HU Hui. Application status and development of wind power blade carbon fiber composites[J]. Power System Engineering,2019,35(3):43-45(in Chinese).
[4]	罗栋. 碳纤维复合材料在汽车、体育用品领域的应用[J]. 合成材料老化与应用, 2016, 45(2):91-94. doi: 10.3969/j.issn.1671-5381.2016.02.021 LUO Dong. Application of carbon fiber com-posite material in the field of automotive and sports goods[J]. Synthetic Material Aging and Applation,2016,45(2):91-94(in Chinese). doi: 10.3969/j.issn.1671-5381.2016.02.021
[5]	林刚. 2018全球碳纤维复合材料市场报告[J]. 纺织科学研究, 2019(7):52-71. LIN Gang. Global carbon fiber composites market report 2018[J]. Textile Science Research,2019(7):52-71(in Chinese).
[6]	孙旋, 童明波, 陈智, 等. 碳纤维织物布层压复合材料湿热环境疲劳后剩余压缩强度[J]. 复合材料学报, 2016, 33(3):535-544. SUN Xuan, TONG Mingbo, CHEN Zhi, et al. Residual compressive strength after fatigue of carbon fiber fabric composite laminates in hydrotherm[J]. Acta Materiae Compo-sitae Sinica,2016,33(3):535-544(in Chinese).
[7]	GARNIER C, PASTOR M L, EYMA F, et al. The detection of aeronautical defects in situ on composite structures using non destructive testing[J]. Composite structures,2011,93(5):1328-1336. doi: 10.1016/j.compstruct.2010.10.017
[8]	LANGE R, MOOK G. Structural analysis of CFRP using eddy current methods[J]. NDT & E International,1994,27(5):241-248.
[9]	MENANA H, FÉLIACHI M. Modeling the response of a rotating eddy current sensor for the characterization of carbon fiber reinforced composites[J]. The European Physical Journal-Applied Physics,2010,52(2):1-9.
[10]	MENANA H, FELIACHI M. An integro-differential model for 3-D eddy current computation in carbon fiber reinforced polymer composites[J]. IEEE Transactions on Magnetics,2010,47(4):756-763.
[11]	CAO M S, WANG X X, ZHANG M, et al. Electromagnetic response and energy conversion for functions and devices in low-dimensional materials[J]. Advanced Functional Materials,2019,29(25):1807398. doi: 10.1002/adfm.201807398
[12]	CAO M S, SONG W L, HOU Z L, et al. The effects of temperature and frequency on the dielectric properties, electromagnetic interference shielding and microwave-absorption of short carbon fiber/silica composites[J]. Carbon,2010,48(3):788-796. doi: 10.1016/j.carbon.2009.10.028
[13]	蔡永珠, 何朋, 疏金成, 等. 二维过渡金属碳化物的结构、电磁特性及微波吸收性能[J]. 黑龙江大学自然科学学报, 2019, 36(1):47-60. CAI Yongzhu, HE Peng, SHU Jincheng, et al. Structure, electromagnetic properties and microwave absorption performance of two-dimensional transition metal carbides[J]. Journal of Natural Science of Heilongjiang University,2019,36(1):47-60(in Chinese).
[14]	CHENG J, JI H, QIU J, et al. Role of interlaminar interface on bulk conductivity and electrical anisotropy of CFRP laminates measured by eddy current method[J]. NDT & E International,2014,68:1-12.
[15]	YIN W, WITHERS P J, SHARMA U, et al. Noncontact characterization of carbon-fiber-reinforced plastics using multifrequency eddy current sensors[J]. IEEE Transactions on Instrumentation and Measurement,2008,58(3):738-743.
[16]	BENSAID S, TRICHET D, FOULADGAR J. Optimal design of a rotating eddy-current probe—Application to characterization of anisotropic conductive materials[J]. IEEE Transactions on Magnetics,2015,51(3):1-4.
[17]	程军, 杨继全, 裘进浩, 等. 基于涡流成像的碳纤维增强树脂基复合材料细观结构可视化[J]. 复合材料学报, 2018, 35(8):2074-2083. CHENG Jun, YANG Jiquan, QIU Jinhao, et al. Visualization of meso-structure of carbon fiber reinforced polymer based on eddy current imaging[J]. Acta Materiae Compo-sitae Sinica,2018,35(8):2074-2083(in Chinese).
[18]	MEGALI G, PELLICANO D, CACCIOLA M, et al. EC modelling and enhancement signals in CFRP inspection[J]. Progress in Electromagnetics Research,2010,14:45-60.
[19]	PRATAP B, WELDON W. Eddy currents in anisotropic composites applied to pulsed machinery[J]. IEEE Transactions on Magnetics,1996,32(2):437-444. doi: 10.1109/20.486530
[20]	XU X, JI H, QIU J, et al. Interlaminar contact resistivity and its influence on eddy currents in carbon fiber reinforced polymer laminates[J]. NDT & E International,2018,94:79-91.
[21]	杨光猛, 万小朋, 侯赤. 纤维束波动效应对平纹编织复合材料损伤行为的影响[J]. 复合材料学报, 2020, 37(1):132-139. YANG Guangmeng, WAN Xiaopeng, HOU chi. Damage behavior of plain woven composites considering the undulation effect of fiber bundles[J]. Acta Materiae Compositae Sinica,2020,37(1):132-139(in Chinese).
[22]	邵兵. 大丝束碳纤维平纹编织复合材料孔边应力细观分析[D]. 南昌: 南昌大学, 2018. SHAO Bing. The mesomechanical analysis of stresses near central hole in big carbon tow plain-woven composite[D]. Nanchang: Nanchang University, 2018(in Chinese).
[23]	HIVET G, BOISSE P. Consistent 3D geometrical model of fabric elementary cell. Application to a meshing preprocessor for 3D finite element analysis[J]. Finite Elements in Analysis and Design,2005,42(1):25-49. doi: 10.1016/j.finel.2005.05.001
[24]	DUCHENE P, CHAKI S, AYADI A, et al. A review of non-destructive techniques used for mechanical damage assessment in polymer composites[J]. Journal of Materials Science,2018,53(11):7915-7938. doi: 10.1007/s10853-018-2045-6
[25]	PENG T, LIU Y, SAXENA A, et al. In-situ fatigue life prognosis for composite laminates based on stiffness degradation[J]. Composite Structures,2015,132:155-165. doi: 10.1016/j.compstruct.2015.05.006
[26]	NISHIO Y, TODOROKI A, MIZUTANI Y, et al. Piezoresistive effect of plain-weave CFRP fabric subjected to cyclic loading[J]. Advanced Composite Materials,2017,26(3):229-243. doi: 10.1080/09243046.2016.1239354