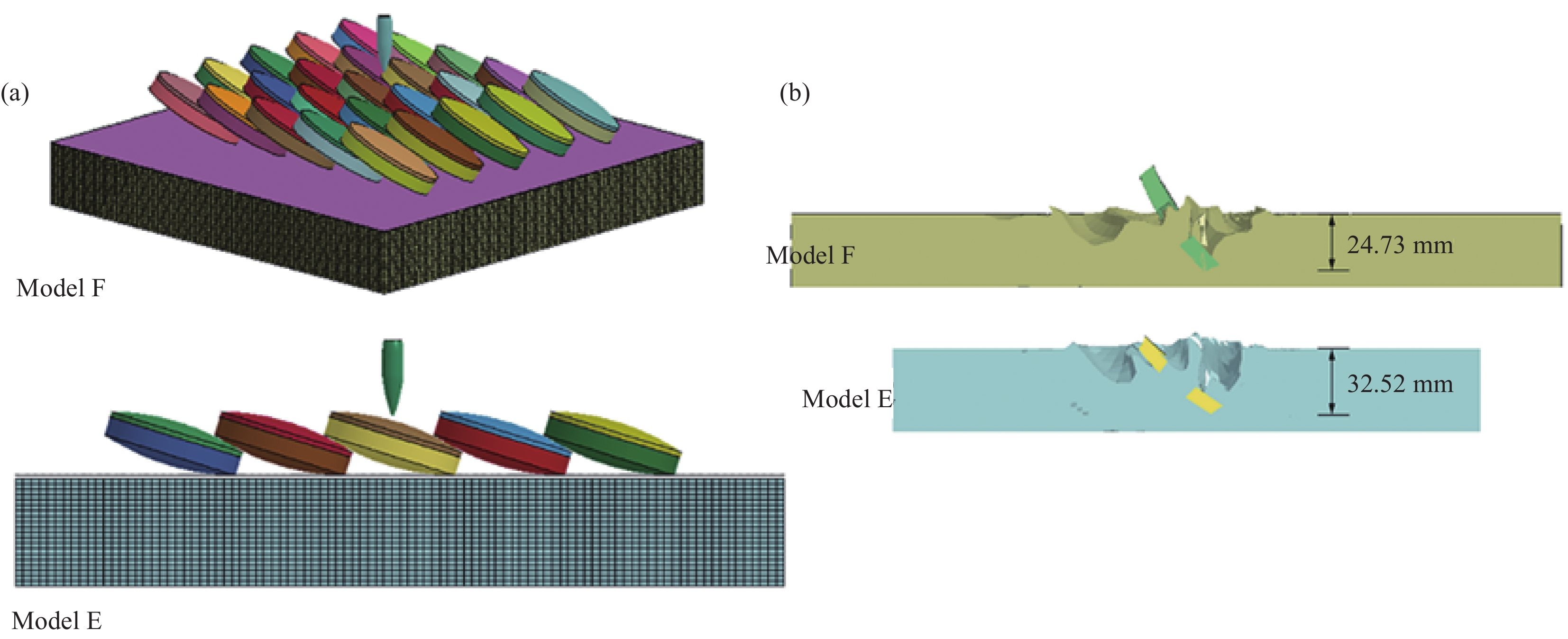
Simulation and experiment of key influencing factors on ballistic performance of SiC-ultra-high molecular weight polyethylene biomimetic flexible laminated structure
-
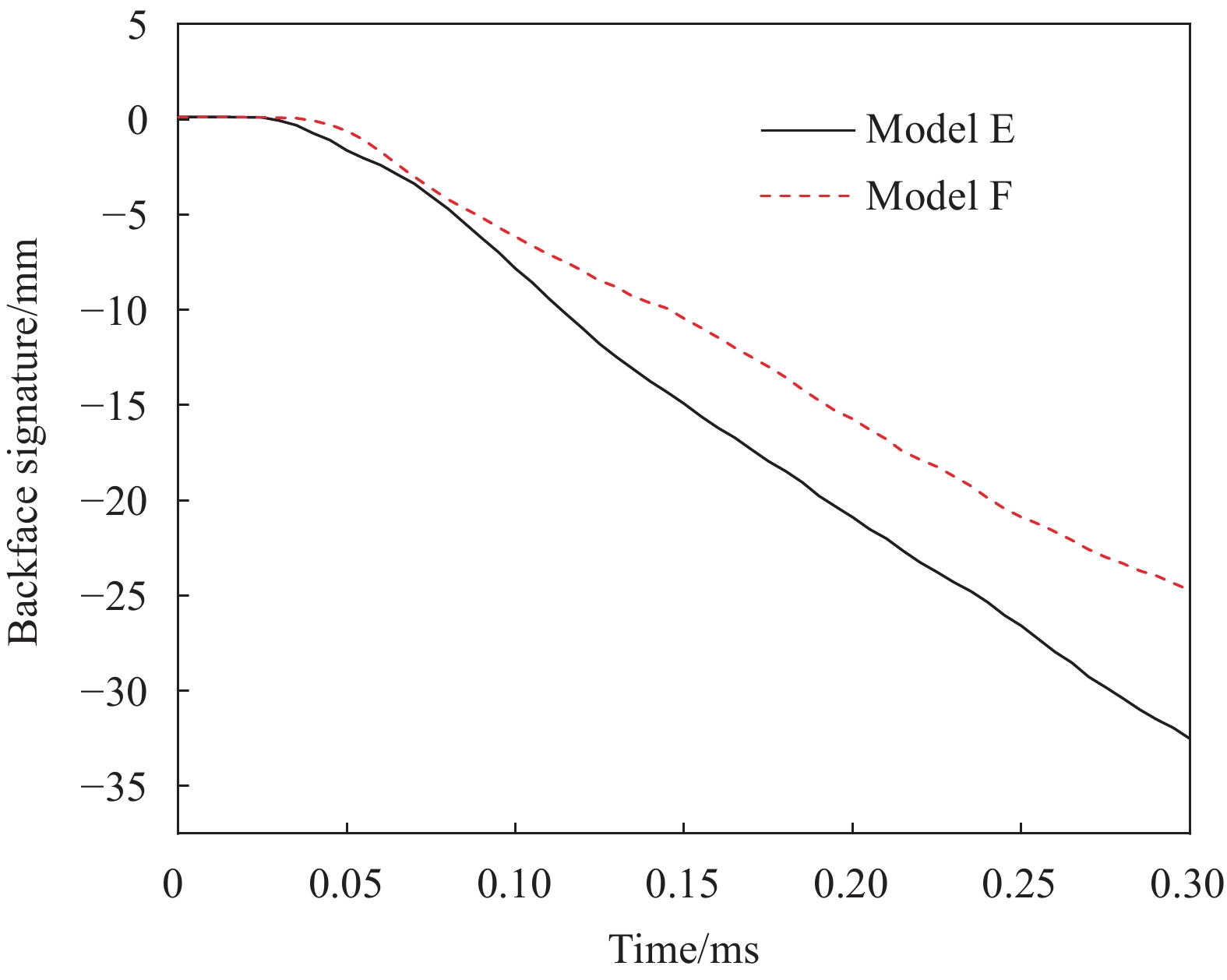
摘要: 基于仿生学原理构建一种鱼鳞状的柔性叠层防护装具，仿生鳞片为中间厚边缘薄的双层复合结构，上下层分别为SiC陶瓷和超高分子量聚乙烯(UHMWPE)。采用ANSYS LS-DYNA软件的显式分析方法模拟了SiC-UHMWPE柔性叠层结构的防弹性能，主要从装具变形量、应力传递规律、能量耗散机制和子弹残余速度展开分析，重点研究了支撑点数量、曲率半径及覆盖角对防护性能的影响。鳞片单排与多排排列时背面垫料的凹陷深度仿真结果分别为32.52 mm和24.73 mm。本文依据NIJ标准Ⅲ级要求对柔性防护装具进行实弹测试。结果表明，试件在多发子弹侵彻后，出现局部两点支撑的不利情形。该成果将对新型柔性防护装具的设计和制备具有重要意义。Abstract: Based on the principle of bionics, a fish scale like flexible laminated protective device was built. The bionic scale is a double-layer composite with thick central region and thin edges. The upper and lower layers of the bionic scale were made of SiC ceramic and ultra-high molecular weight polyethylene (UHMWPE), respectively. The explicit analysis method in the ANSYS LS-DYNA software was used to simulate the ballistic performance of the SiC-UHMWPE flexible laminated structure. The analysis included the deformation, stress transfer pattern and energy dissipation mechanism of the device and the residual velocity of bullet, and mainly focused on the effects of the number of support points, the radius of curvature and the overlapping angle. The simulated backface signatures for the protective device with one row scales and multiple rows scales are 32.52 mm and 24.73 mm, respectively. Ballistic tests were conducted on the bio-inspired flexible protection device according to the requirements of level Ⅲ of the NIJ standard. The results show that there is an unfavorable mode of two-point support at the local area after multiple impacts of bullets. This study will be valuable for the design and manufacture of new flexible protective devices.
-
由于可穿戴电子设备在个人健康监测领域的广泛应用，近年来引起了人们极大关注，但商用电池的寿命问题极大限制可穿戴电子设备的发展[1]，同时电池电量有限所导致的频繁充电也极大地影响了可穿戴电子的使用体验[2]；因此自供能电子器件成为当下的热点研究方向。利用机电转换原理的纳米发电机在机械能收集及电能转换方向得到广泛关注，有望为小型可穿戴电子设备进行供能，近年来成为自供能领域的研究热点[3-5]。
纳米发电机主要包括压电纳米发电机(PNGs)、摩擦电纳米发电机(TNGs)及复合纳米发电机；其中PNGs利用压电效应将机械能转化为电能，TNGs利用摩擦起电和静电感应完成机械能向电能的转化[6-8]，而复合纳米发电机则是能集机械能、光能、热能等多种能源转化为一体的纳米发电微系统[9]。PNGs具有较高的灵敏度和稳定性但其输出性能较低，TNGs则具有相对较高的输出性能但其输出的不稳定，因此两者的应用场景都受到了不同的限制。将二者进行集成的压电-摩擦电复合纳米发电机在提升电输出性能的同时，兼具两类纳米发电机的优势，为低功耗可穿戴电子产品的供电提供了一个良好的解决方案[10]。
苯乙烯-丁二烯-苯乙烯嵌段共聚物(SBS)作为摩擦材料中一种常用的正极材料[11]，因其突出的介电性能与弹性，可广泛应用于传感器、能量收集等领域[12]。以SBS为基体制作的柔性传感器件在工作室可以承受高达600%的应变[13]，可有效提高器件的可靠性和寿命，同时SBS还被认为是制备可调共聚物静电纺丝纤维的最佳选择[14]。而SBS作为为摩擦材料中的正极材料，将其用作摩擦纳米发电机的研究尚未见公开报道。聚偏二氟乙烯(PVDF)是摩擦材料中常用的负极材料，同时具有较高压电系数、良好生物相容性及机械稳定性的重要压电聚合物[15]，其黏附力较强，适合与多种材料进行混合纺丝。因此，将二者复合，有望提高压电-摩擦电复合纳米发电机的电输出性能。
静电纺丝技术具有装置简单、成本低、工艺可控等优势，所制备的纳米纤维薄膜具有较高的比表面积和孔隙率，可有效增加摩擦时的接触面积，提高摩擦电荷密度[16-17]；同时静电纺丝工艺在纺丝过程中即完成对PVDF的极化，实现压电-摩擦电复合纳米发电机输出性能的提升。本文以PVDF颗粒为填料采用静电纺丝工艺将其与SBS复合，制备PVDF/SBS复合纤维薄膜，再利用复合纤维薄膜制备柔性压电-摩擦电复合纳米发电机。利用PVDF与SBS之间摩擦起电与静电感应效应，对外产生摩擦电压、电流信号。同时借助PVDF的压电性能，由压电、摩擦电两种效应共同作用提高机械能-电能转换效率及器件的电输出性能。实验探究了不同PVDF掺入量对复合纳米发电机的电学输出性能的影响，同时测试了器件在人体运动监测和能量收集方面的相关应用。
1. 实验材料及方法
1.1 复合纤维薄膜制备
实验采用静电纺丝方法制备PVDF/SBS复合纳米纤维薄膜。实验首先取10 mL二氯乙烷，之后以质量比5∶1称取SBS备用，再以SBS质量为基准称取质量分数为0wt%、5wt%、10wt%、15wt%、20wt%及30wt%的PVDF颗粒。将PVDF加入二氯乙烷中，超声30 min使PVDF颗粒均匀分散，加入SBS后磁力搅拌直至得到均匀的灰白色溶液，即为静电纺丝方法制备PVDF/SBS复合纳米纤维的前驱液。将所配制的前驱液装入注射器中，设置注推速度为2 mL/h。使用接地的平面电极板收集PVDF/SBS纤维，加有12 kV正电压的针尖距离平面电极板15 cm；在纺丝过程中保持环境湿度相对湿度(RH)<30%，以保证溶剂完全挥发。待纺丝过程结束之后即在接收电极板得到平整的PVDF/SBS复合纤维薄膜。
1.2 复合纳米发电机制备
将静电纺丝所得的复合纤维薄膜剪裁成2 cm×3 cm的长方形薄膜，再将两片长方形铝膜粘贴到PVDF/SBS薄膜的两侧作为上下电极，形成夹层结构；再利用两条5 cm铜箔将上下电极引出，最后用两片3 cm×4 cm的聚酰亚胺(PI)对夹层器件进行封装得到PVDF/SBS复合纳米发电机。
1.3 测试与表征
实验采用场发射扫描电子显微镜(FESEM，JSM-7100F，日本JEOL)、傅里叶变换红外光谱仪(FT-IR，Vertex，德国Bruker)测试和分析复合纤维薄膜的形貌与结构。复合纳米发电机的电学输出性能测试由信号发生器(UTG2025A)、功率放大器(SA-PA010)、激振器(SA1804B)、数字示波器(MDO 3024)、数据采集卡(USB-6210)、数字源表(Keithley 2611B)和电荷放大器(SA1804B)组成。测试方式主要通过敲击方式进行测试，具体过程为：将一块硬质聚四氟乙烯平板夹在样品固定架上，同时将所制器件贴在平板的正面，调节样品架高度使其能够刚好与激振器的击锤相接触；在测试时通过信号发生器产生正弦信号，正弦信号经功率放大器放大后传递至激振器，激振器在接受到正弦信号后不断敲击器件，器件产生电压信号和电流信号，最后通过数字示波器和2611B源表对器件的电压、电流输出信号进行采集记录。
2. 结果与讨论
2.1 PVDF/SBS复合纤维薄膜的微观形貌与结构
图1为PVDF/SBS复合纤维薄膜的截面SEM图像。可以看出通过静电纺丝制备的复合纤维薄膜厚度约为260 μm。
![]()
图 1 聚偏二氟乙烯/苯乙烯-丁二烯-苯乙烯嵌段共聚物(PVDF/SBS)复合纤维薄膜的SEM截面图像Figure 1. SEM section image of polyvinyl fluoride/styrene butadiene styrene block copolymer (PVDF/SBS) composite fiber图2为不同质量分数PVDF的PVDF/SBS复合纤维薄膜的表面SEM图像。可以看出，纯SBS纤维直径为5~10 μm，纤维表面光滑且直径相对均匀。随着复合纤维中PVDF质量分数不断增加，单根纤维表面的粗糙程度变大。当PVDF质量分数为15wt%时，纤维表面开始出现较明显的凹陷(图2(d))；当掺入质量分数达30wt%时，复合纤维表面出现明显孔洞，同时在孔洞处可以观察到较为明显的颗粒团聚现象(图2(f)及局部放大图)。PVDF/SBS复合纤维表面的形貌与掺入的PVDF颗粒质量分数有关，当掺入的PVDF质量分数较少时，PVDF颗粒在前驱体溶液中分散较均匀，复合纤维中的溶剂挥发后，PVDF颗粒分散在纤维中使纤维表面粗糙度增加；当掺入的PVDF质量分数较大时，前驱体溶液中容易出现PVDF颗粒的团聚，纺丝后在接收板上得到的SBS纤维中PVDF仍然团聚在一起，因此溶剂挥发后会在SBS纤维表面形成孔洞。SBS纤维表面的孔洞使PVDF颗粒与SBS摩擦层分离，在受力时两种摩擦材料不能有效接触，因此无法产生摩擦电荷，从而影响SBS/PVDF压电-摩擦电纳米发电机的电输出性能。
![]()
图 2 不同PVDF含量的PVDF/SBS复合纤维的SEM图像：(a) 0wt%；(b) 5wt%；(c) 10wt%；(d) 15wt%；(e) 20wt%；(f) 30wt%及局部放大图Figure 2. SEM images of PVDF/SBS composite fiber with different PVDF contents: (a) 0wt%; (b) 5wt%; (c) 10wt%; (d) 15wt%; (e) 20wt%; (f) 30wt% and partially enlarged image为了进一步探究薄膜的组成和结构，对不同质量分数的PVDF/SBS复合纤维薄膜进行了FTIR测试，结果如图3所示。图中699 cm−1和966 cm−1处的吸收峰对应为SBS中聚苯乙烯和聚丁二烯的吸收特征峰[18]；878 cm−1和1403 cm−1处的吸收峰对应为PVDF中C—F和C—H的吸收特征峰[19]。同时，536 cm−1和615 cm−1处特征峰对应PVDF的非极性α相，510 cm−1、840 cm−1和1278 cm−1处特征峰对应PVDF的β相[20]。FTIR测试结果表明静电纺丝所得复合纤维薄膜中PVDF聚合物既有α相，又存在β相，且随着PVDF复合量的增加，复合纤维中β相含量也相应增加。PVDF中极性β相含量的增加将提高PVDF的压电性能，从而有助于增强压电-摩擦电复合纳米发电机的电输出信号强度。
![]()
图 3 不同质量分数PVDF/SBS复合纤维薄膜的FTIR图谱Figure 3. FTIR spectra of PVDF/SBS composite fiber films with different mass fractions of PVDF2.2 PVDF/SBS复合纳米发电机的性能测试及分析
PVDF/SBS压电-摩擦电复合纳米发电机及PVDF/SBS复合纤维薄膜的光学照片如图4所示，可以看出PVDF/SBS复合纤维薄膜表面均匀平整，所制备的器件具有良好的柔性。
![]()
图 4 (a) PVDF/SBS压电-摩擦电复合纳米发电机；(b) PVDF/SBS复合纤维薄膜Figure 4. Optical photos of PVDF/SBS piezoelectric/triboelectric composite nanogenerator (a) and PVDF/SBS composite fiber film (b)实验测试了不同质量分数PVDF/SBS压电-摩擦电复合纳米发电机的电输出性能，结果如图5所示。可以看出，敲击纯SBS纤维薄膜器件可产生20 V的输出电压及0.056 μA的电流。在SBS中复合PVDF压电聚合物后，器件的输出电压与输出电流均有明显增强。随着复合薄膜中PVDF质量分数的增加，器件的输出电压、电流均呈现出先显著增加再下降的趋势。
![]()
图 5 不同质量分数PVDF/SBS压电-摩擦电复合纳米发电机的输出响应：(a) 开路电压；(b) 短路电流Figure 5. PVDF/SBS piezoelectric/triboelectric composite nanogenerator with different mass fraction of PVDF: (a) Open-circuit voltage;(b) short- circuit current当加入20wt%PVDF时，复合纤维薄膜的输出电压、电流均达到最大值，分别为108 V和0.34 μA，是纯SBS器件输出电压的5倍、输出电流的6倍。上述测试表明在SBS纤维中引入具有压电性的PVDF后可显著提高器件的电输出性能，是压电-摩擦电两种效应共同作用的结果。
PVDF/SBS压电-摩擦电复合纳米发电机的工作原理可用如图6所示的原理图解释。当无PVDF复合时，由纯SBS纤维组成的器件中仅由SBS纤维与电极之间相互摩擦产生静电电荷，在敲击过程中电极与纯SBS纤维发生相对移动，电极两端的电势发生变化，对外产生摩擦电电压、电流。
![]()
图 6 PVDF/SBS压电-摩擦电复合纳米发电机工作原理图Figure 6. Working principle diagram of PVDF/SBS piezoelectric/triboelectric composite nanogeneratorIT—Triboelectric current; IP—Piezoelectric current当纤维中复合了PVDF压电聚合物后，PVDF被静电纺丝过程中的强电场极化，静电纺丝结束后，纤维中的PVDF颗粒仍存在剩余极化。在初始不受力的情况下，由于复合纤维内部PVDF颗粒存在剩余极化，复合纤维的上、下表面都分别产生负、正束缚电荷以保持电荷中性[21]，内部PVDF颗粒与SBS摩擦产生的摩擦电荷也在剩余极化作用下分布于复合纤维的上、下表面，复合纤维薄膜的表面电荷密度得以提升，同时在上下电极分别感应产生极性相对的静电电荷，整体处于静电平衡状态，如图6(a)所示。受力后，电极与纤维薄膜相互靠近，电极两端产生电势差，下电极内部电子在电场的驱动下通过外电路流向上电极，产生向下的摩擦电流IT，如图6(b)所示。当电极与复合纤维薄膜相接触时，复合纤维内部的PVDF颗粒受到挤压，自身发生形变，沿自身极化方向又产生额外的偶极矩，因此在PVDF颗粒的相对表面再次产生极性相反的电荷，电极表面感应出极性相对的电荷，上下电极形成的电场驱动电子通过下电极经外电路流向上电极，产生向下的压电电流IP，此时IP方向与先前IT方向一致，如图6(c)所示。当外力逐渐释放时，PVDF颗粒的形变逐渐恢复，因此产生相反的压电势，对外产生反向的压电电流IP，外力继续释放，纤维薄膜与电极逐渐分离，上下电极产生相反的电势差驱动电子从上电极流向下电极，对外产生反向的摩擦电流IT，如图6(d)、6(e)所示。由此表明PVDF压电聚合物与SBS复合后，PVDF颗粒的剩余极化及压电效应有利于复合纳米发电机输出性能的提升。复合纤维薄膜中PVDF质量分数的提高有利于增加由剩余极化产生的束缚电荷从而提升表面电荷密度，同时也有利于增强受力时复合纤维薄膜的压电极化，提高复合纳米发电机的输出性能。而当掺入PVDF颗粒的含量过大(＞20wt%)时PVDF明显团聚(如图2(f)所示)，受外力作用时PVDF颗粒的极化存在相互抵消的现象，因此复合纳米发电机输出性能反而下降。
2.3 PVDF/SBS复合纳米发电机的实际应用
为了探究复合纳米发电机对于人日常活动时产生的机械能的收集与传感特性，实验将PVDF含量20wt%的PVDF/SBS复合纤维器件分别固定在手掌内侧与鞋子底部，记录日常肢体运动时器件的电压输出信号，结果见图7。当双手拍打，完成鼓掌动作时，器件的电压输出见图7(a)。可以看出，复合纳米发电机可以有效收集鼓掌时的机械能，产生输出电压约为2.5 V。截取其中一次鼓掌动作的输出电压响应信号，可以观察到器件的响应时间为2 ms (图7(b))，表明PVDF/SBS器件对外部作用力的响应速度快。将器件安装在鞋底，收集成年人走路、小跑、蹦跳时的电输出信号，如图7(c)所示。从图中可以看出，成年人走路时，器件可产生1.3 V的输出电压；小跑时，复合纳米发电机能产生2.9 V的输出电压；当跳起离地面约5 cm时，器件能产生11.6 V的输出电压。由上述可以看出，PVDF/SBS复合纤维薄膜能够有效收集人体运动的机械能并进行传感，在人体运动状态监测领域有着巨大的发展前景。
![]()
图 7 PVDF/SBS压电-摩擦电复合纳米发电机对人体运动的传感：(a) 拍手时的电输出响应；(b) 拍手时输出响应时间；(c) 人体走路、小跑、蹦跳时的电输出响应Figure 7. PVDF/SBS piezoelectric/triboelectric composite nanogenerator for human motion sensing: (a) Electrical output response when clapping; (b) Response time when clapping; (c) Electrical output response when walking, trotting and jumping为了验证复合纳米纤维薄膜在小型便携式电子元件自供电领域的应用前景，实验将器件与整流桥及有“HUBU”图案的64个商用蓝色LED灯串联，通过手掌拍打器件可将图案中所有LED灯珠点亮，结果如图8所示。上述结果表明，PVDF/SBS复合纳米发电机在生物力学采集与便携式电子元器件的自供电领域具有良好的应用前景。
![]()
图 8 PVDF/SBS压电-摩擦电复合纳米发电机点亮64个蓝色LEDFigure 8. PVDF/SBS piezoelectric/triboelectric composite nanogenerator lights up 64 blue LEDs实验将PVDF含量 20wt%的PVDF/SBS复合纤维纳米发电机用于检测重物自由掉落时的压力测试。将一个网球从5、7、10、12、15、17、20、22、25及27 cm共10个不同高度落至器件表面，采集相应的电压输出信号。同时采用东莞市智取精密仪器有限公司的DS2-50 N-XD数显压力测试仪获取网球从不同高度下落至器件表面对应的作用力为0.42~1.77 N，由此得到器件受不同作用力后的电压输出信号，结果如图9(a)~9(f)所示。可以看到，复合纳米发电机的输出电压随受力的增加而明显增强，可产生0.2~2.5 V的电压，并能检测到网球落至器件表面多次回弹的信号。如图9(g)、图9(h)所示，器件可在14 ms内检测到0.42 N的作用力，在35 ms内检测到1.77 N的作用力。实验进一步分析了PVDF/SBS复合纤维薄膜对外力作用的灵敏度，结果如图9(i)所示。器件呈现出两个线性响应区，在低压力(0.42~1.11 N)作用范围内，器件灵敏度SL1为0.871 V·N−1；在高压力(1.2~1.77 N)作用力范围内，器件灵敏度SL2为3.685 V·N−1；相比于文献报道的基于ZnO@C/PVDF的压电-摩擦电传感器[22]，实验所制备的PVDF/SBS复合纤维薄膜的灵敏度具有一定优势。器件在较大作用力下灵敏度增加是由于较大的作用力使复合薄膜中PVDF的压电极化增强，因此复合纳米发电机的电输出明显增强，器件的灵敏度显著增加。该测试结果表明，PVDF/SBS复合纳米发电机不仅为低功率柔性电子器件的续航提供技术支持，在微小压力检测领域也具有良好的应用前景。
![]()
图 9 PVDF 含量 20wt% 的PVDF/SBS压电-摩擦电复合纳米发电机对不同外力的电压输出信号及灵敏度特性：(a) 0.42 N；(b) 0.56 N；(c) 0.72 N；(d) 1.11 N；(e) 1.57 N；(f) 1.77 N；(g) 0.42 N下响应时间；1.77 N下响应时间 (h) 及器件灵敏度 (i)Figure 9. Voltage output signals and sensitivity characteristics of PVDF/SBS piezoelectric/triboelectric composite nanogenerator with PVDF content 20wt% to different forces: (a) 0.42 N; (b) 0.56 N; (c) 0.72 N; (d) 1.11 N; (e) 1.57 N; (f) 1.77 N; (g) Response time in 0.42 N; Response time (h) and the sensitivity (i) of the device in 1.77 NSL1—Sensitivity of the low-pressure region; SL2—Sensitivity of the high-pressure region3. 结 论
采用静电纺丝制备了聚偏二氟乙烯(PVDF)/苯乙烯-丁二烯-苯乙烯嵌段共聚物(SBS)复合纳米纤维薄膜，得到了PVDF/SBS压电-摩擦电复合纳米发电机，研究了PVDF颗粒含量对复合纤维薄膜的结构、复合纳米发电机电气输出性能的影响。具体结论如下：
(1) 适量PVDF颗粒与SBS复合后，可提供额外的束缚电荷，提升表面电荷密度，从而增加PVDF/SBS摩擦电输出性能。同时掺入PVDF压电聚合物后，在外力作用下PVDF可提供压电输出，与摩擦电效应协同作用，有效了提高PVDF/SBS复合纤维薄膜的电输出性能。PVDF掺入质量分数为20wt%时复合纤维薄膜的输出电压达到最大值108 V、输出电流达到最大值0.34 μA，分别为纯SBS纤维样品的5倍和6倍；
(2) 将PVDF 含量20wt%的PVDF/SBS复合纤维薄膜组装成的压电-摩擦电复合纳米发电机可有效收集人们日常生活肢体运动产生的机械能；器件可将手掌拍打器件的能量转换为电能，点亮64个商用蓝色LED灯珠；
(3) 将PVDF/SBS复合纤维薄膜用于微小外力的实时检测，测试结果表明器件响应速度快，对0.42 N作用力的响应时间仅为14 ms，器件对微小外力的灵敏度可达3.685 V·N−1。
-
![]()
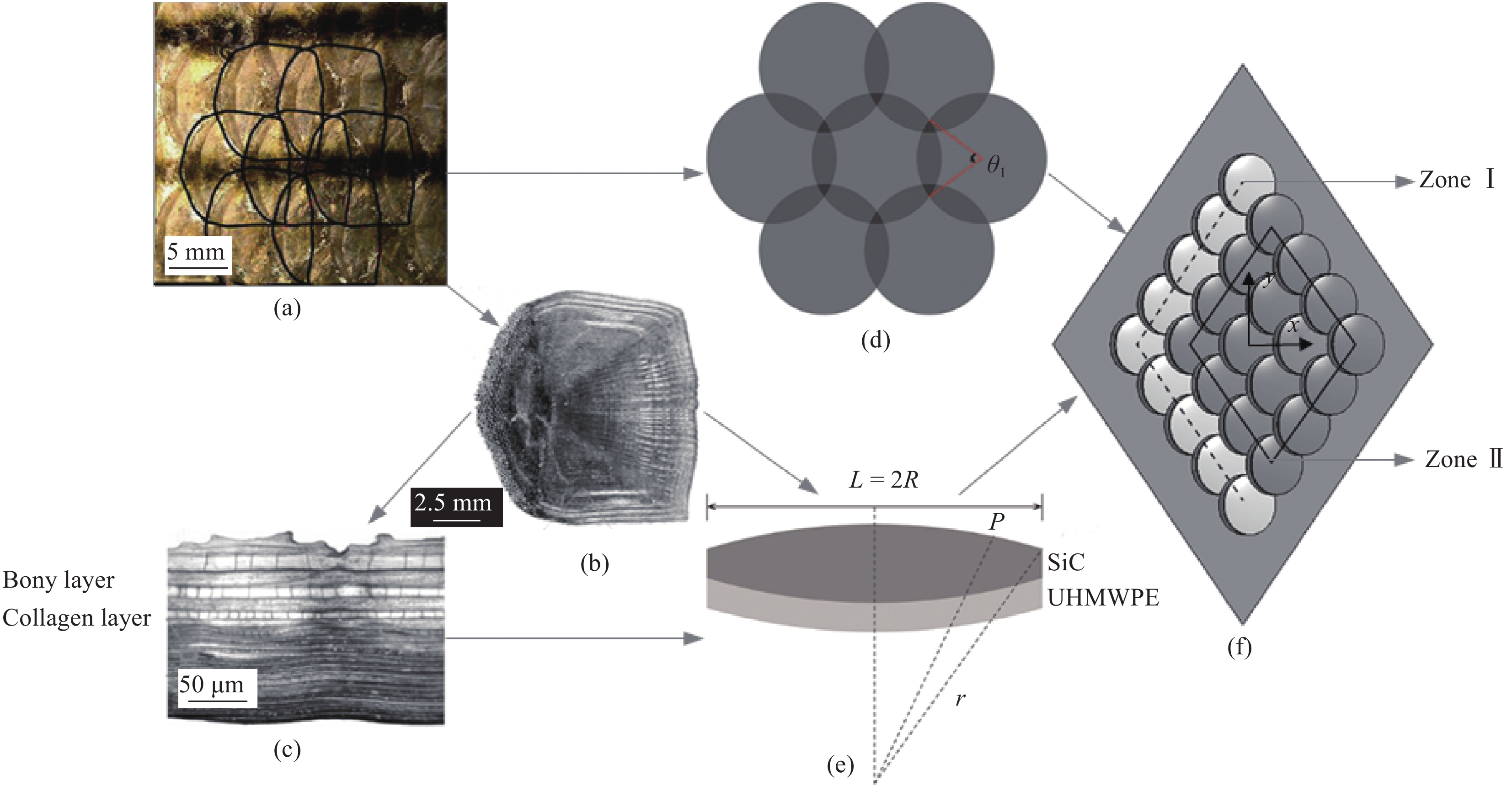
图 1 SiC-超高分子量聚乙烯(UHMWPE)仿生柔性防护装具结构设计示意图：(a)真实鱼鳞片排列模式; (b)单个鱼鳞片; (c)鳞片截面; (d)仿生鳞片排列; (e)仿生复合鳞片截面; (f)仿生防护装具
Figure 1. Schematic diagram of structural design of SiC-ultra-high molecular weight polyethylene (UHMWPE) bionic flexible protection device: (a) Arrangement structure of the real fish scale; (b) Demonstration of an individual scale; (c) Cross-section of a scale; (d) Bionic scale arrangement pattern; (e) Cross-section of a bionic composite scale; (f) Bionic protection device
θ1—Overlapping angle; L—Bionic scale diameter; R—Bionic scale radius; P—Contact point of bionic scale; r—Radius of arc of bionic scale
![]()
图 2 防护装具旋转角示意图
Figure 2. Diagram of rotation angle of protection device
α—Rotation angle
![]()
图 3 SiC-UHMWPE柔性防护装具的有限元模型及网格划分示意图
Figure 3. Demonstration of finite element model and meshing of SiC-UHMWPE protection device
![]()
图 4 SiC-UHMWPE柔性防护装具各模型随时间的动力响应曲线
Figure 4. Dynamic response curves of each model over time of SiC-UHMWPE protection device
![]()
图 5 模型A和模型C靶鳞片UHMWPE层和子弹变形图: (a)靶鳞片UHMWPE层(t=50 μs); (b)子弹(t=20 μs)
Figure 5. Simulated deformation patterns of target scale with UHMWPE layer and bullet in model A and model C: (a) Target scale with UHMWPE layer (t=50 μs); (b) Bullet (t=20 μs)
![]()
图 6 SiC-UHMWPE 各模型靶鳞片应力分布(t=50 μs): (a)模型A; (b)模型B; (c)模型C; (d)模型D
Figure 6. Von Mises stress distribution of target scale in each model of SiC-UHMWPE (t=50 μs): (a) Model A; (b) Model B; (c) Model C; (d) Model D
![]()
图 7 SiC-UHMWPE 模型C和模型A的应力分布: (a) Kevlar层; (b)整个防护装具
Figure 7. Von Mises stress distribution of model C and model A for SiC-UHMWPE : (a) Kevlar layer; (b) Whole protection device
![]()
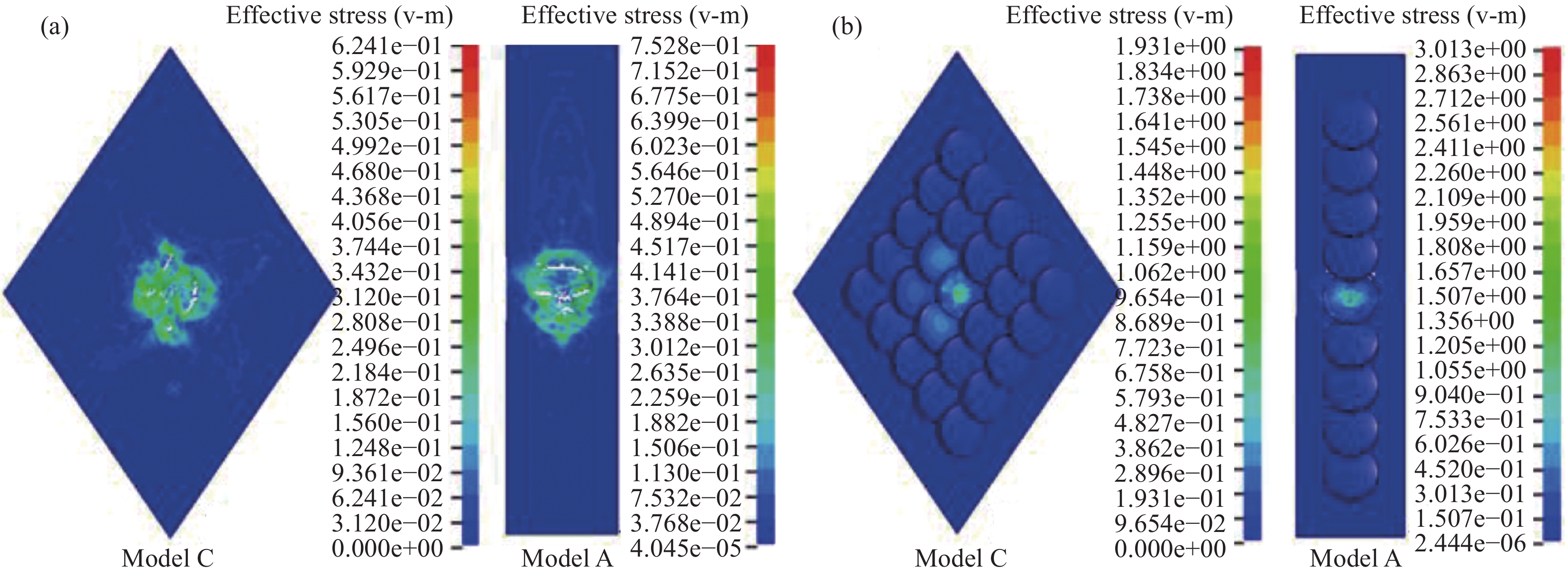
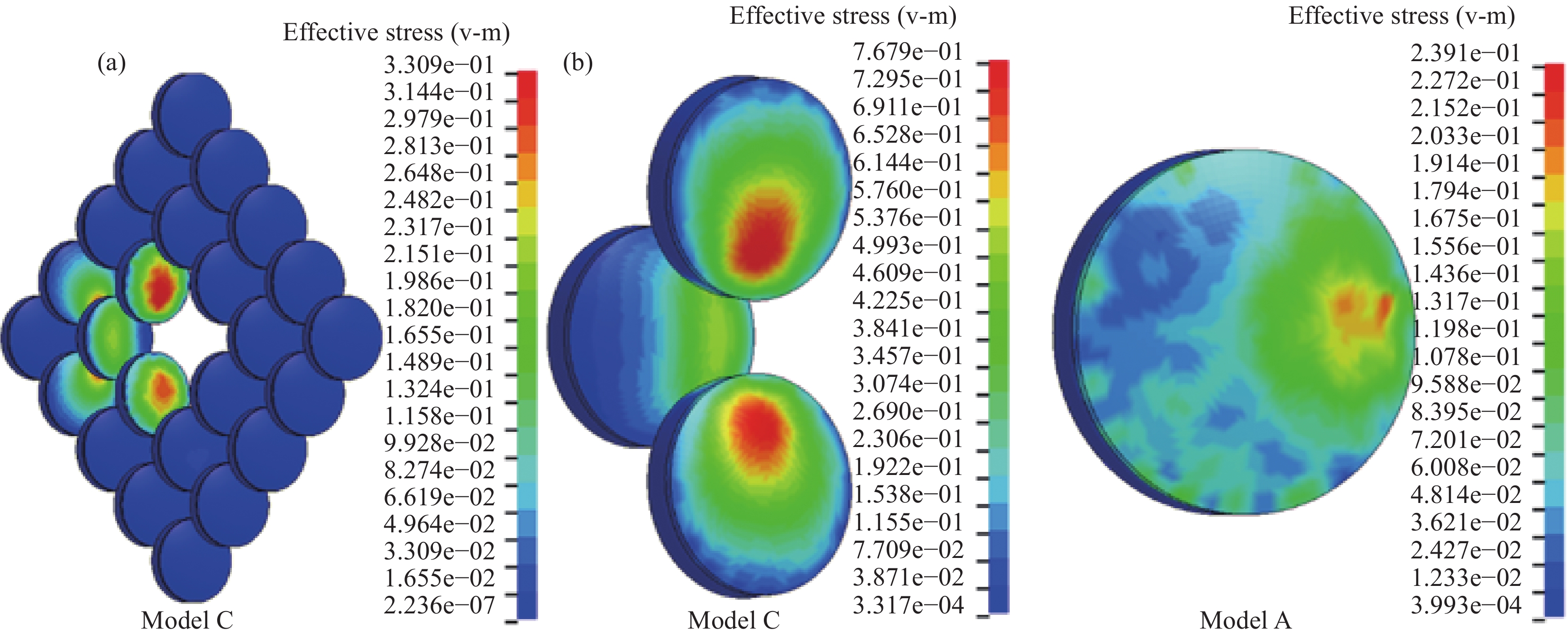
图 8 SiC-UHMWPE 模型C与模型A的应力传递规律: (a) 模型C应力影响区域图; (b)子弹残余速度为0时模型C和模型A支撑鳞片等效应力图
Figure 8. Stress transfer patterns of model C and model A for SiC-UHMWPE: (a) Stress affected region in model C; (b) Von Mises stress contour of supporting scales in model C and model A when bullet’s residual velocity is zero
![]()
图 9 SiC-UHMWPE 模型B和模型D直接支撑鳞片的应力分布
Figure 9. Effective stress distribution of direct supporting scale in model B and model D of SiC-UHMWPE
![]()
图 10 SiC-UHMWPE模型E和模型F的有限元模型及仿真结果: (a)有限元模型; (b)凹陷深度
Figure 10. Finite element model and simulation results of model E and model F for SiC-UHMWPE: (a) Finite element model; (b) Backface signature
![]()
图 11 试验样件及局部区域凹陷外貌: (a)试验前后防护装具X光照片; (b)试验和仿真的靶鳞片下背面垫料凹陷外貌
Figure 11. Test sample and concave appearance of local area: (a) X-ray pictures of armor sample before and after test; (b) Signature of backing material under target scale obtained by test and simulation
![]()
图 12 模型E和模型F靶鳞片UHMWPE层位移-时间曲线
Figure 12. Displacement-time curves of UHMWPE layer of target scale in model E and model F
表 1 有限元模型命名
Table 1 Finite element model naming
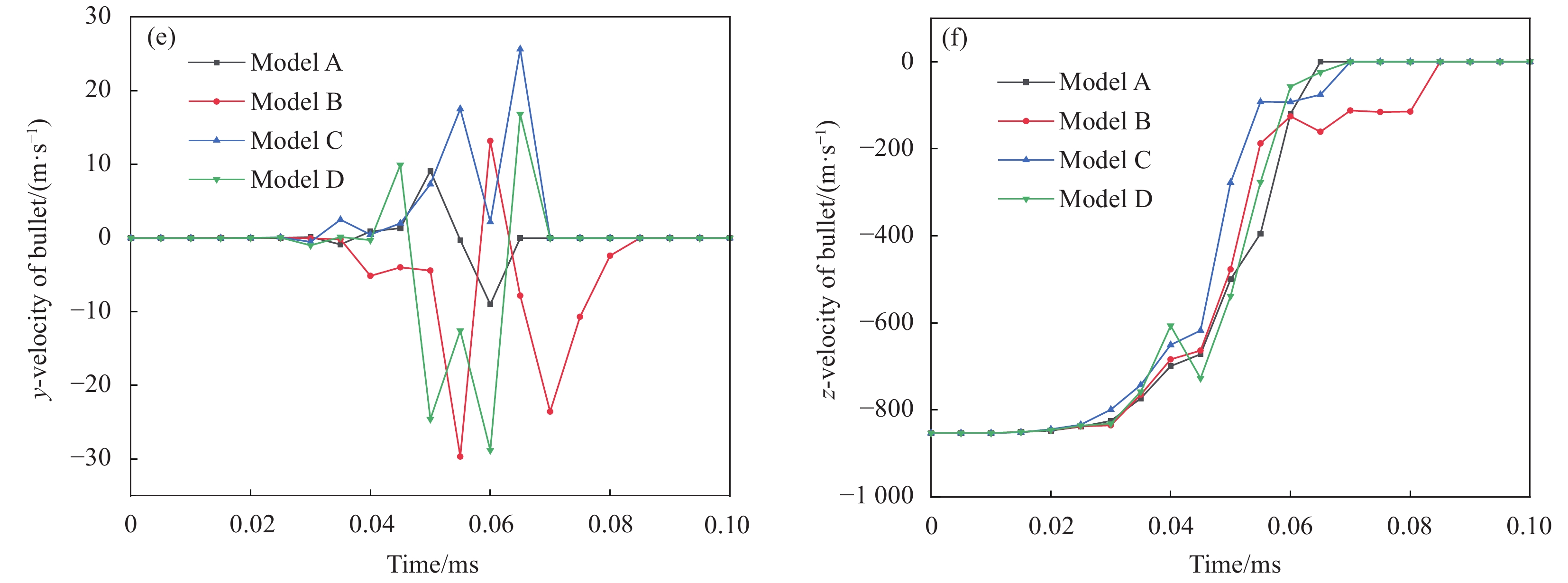
Mark Name Named annotation Model A a0c80 Flat model of single-row arrangement of scales with rotation angle α=0° and coverage angle θ1=80°. Model B a0c100 Flat model of single-row arrangement of scales with rotation angle α=0° and coverage angle θ1=100°. Model C 5x5a0c80 Flat model of 5 scales in the horizontal and vertical directions with the rotation angle α=0°, coverage angle θ1=80°. Model D a4c100 Curved model of single-row arrangement of scales with rotation angle α=4° and coverage angle θ1=100°. Model E a0c80gel Flat model of single-row arrangement of scales with rotation angle α=0°, coverage angle θ1=80°, and backing materials (gel) at bottom. Model F 5x5a0c80gel Flat model of 5 scales in the horizontal and vertical directions with rotation angle α=0°, coverage angle θ1=80°, and backing materials (gel) at bottom. 
下载: 导出CSV
表 2 SiC陶瓷材料模型参数
Table 2 Material model parameters of SiC
ρ/(g·cm−3) G/GPa A B C M N 3.163 127 0.96 0.35 0 1.0 0.65 EPSI Tensile strength /
GPaNormalized fracture strength HEL/GPa HEL pressure/GPa HEL vol. strain HEL strength/GPa 1.0 0.37 0.8 14.567 5.9 — 13.0 D1 D2 K1/GPa K2/GPa K3/GPa Beta PSFAIL 0.48 0.48 204.785 0 0 1.0 — Notes: ρ—Density; G—Shear modulus; A—Intact normalized strength parameter; B—Fractured normalized strength parameter; C—Strength parameter (for strain rate dependence); M—Fractured strength parameter (pressure exponent); N—Intact strength parameter (pressure exponent); EPSI—Reference strain rate; HEL—Hugoniot elastic limit; vol.—Volumetric; D1—Parameter for plastic strain to fracture; D2—Parameter for plastic strain to fracture (exponent); K1—First pressure coefficient (equivalent to the bulk modulus); K2—Second pressure coefficient; K3—Elastic constants; Beta—Fraction of elastic energy loss converted to hydrostatic energy; PSFAIL—Effective plastic strain at failure.
下载: 导出CSV
表 3 超高分子量聚乙烯(UHMWPE)材料特性参数
Table 3 Material model parameters of ultra-high molecular weight polyethylene(UHMWPE)
ρ/(g·cm−3) P1 P2 P3 P4 P5 P6 0.97 5.796 5.796 6.12561 0.025 3.58889 0.41368 P7 P8 P9 P10 P11 P12 P13 0.25 3.709 2.884 1 0.05 6.6939 0.05 P14 P15 P16 P17 P18 P19 P20 2.29 2.29 0.025 0.2645 0 0.185 1.3328 P21 P22 P23 P24 P25 P26 P27 0.28285 4.63 0.28285 4.63 0.28285 2.25 −0.005 Notes: P1, P2, P3—Moduli of elasticity in x, y and z directions; P4—Stretching poisson’s ratio in xy plane; P5—Shear modulus in xy plane; P6—Yield stress in xy plane; P7—Failure strain in xy direction; P8, P9—Linear buckling parameters; P10—Unloading modulus factor in xy plane; P11—xy plane; P12—Unloading modulus in z direction; P13—Tensile modulus factor in z direction; P14, P15, P16—Shear moduli in yz, zx and xy planes; P17—Compression failure strain in z direction; P18—Tensile failure strain in z direction; P19—Local strain of area 1 in z direction; P20—Modulus of elasticity of area 1 in z direction; P21, P22—C, P parameters of area 2; P23, P24—C, P parameters of area 1; P25, P26—C, P parameters in xy plane; P27—Parameter of strain rate.
下载: 导出CSV
表 4 凯夫拉(Kevlar)材料模型参数
Table 4 Material model parameters of Kevlar
ρ/(g·cm−3) Ea/GPa Eb/GPa Gab1/GPa Gab2/GPa Gab3/GPa Gbc/GPa 0.8 32.69 32.69 0.004 0.042 0.349 0.349 Gca/GPa Gamab1 Gamab2 Ea,crimpfac Eb,crimpfac εa,crimp εb,crimp 0.349 0.25 0.35 0.06 0.20 0.007 0.0025 Ea,softfac Eb,softfac Eunloadfac Ecompfac εa,max εb,max σpost/GPa −2.20 −5.60 1.5 0.005 0.023 0.02 0.01 CCE PCE CSE dfac εmax εa,fail εb,fail 0.005 40.00 0.005 0.30 0.035 0.20 0.20 Notes: Ea, Eb—Moduli of elasticity in the longitudinal and transverse directions; Gab1, Gab2, Gab3—Shear moduli Gabi correspond to the slope of the ith segment; Gbc, Gca—Shear moduli in bc and ca directions; Gamab1, Gamab2—Shear strains Gamabi correspond to the slope of the ith segment; Ea,crimpfac, Eb,crimpfac—Factors for crimp region modulus of elasticity in longitudinal and transverse directions; εa,crimp, εb,crimp—Crimp strains in longitudinal and transverse directions; Ea,softfac, Eb,softfac—Factors for post-peak region modulus of elasticity in longitudinal and transverse directions; Eunloadfac, Ecompfac—Factors for unloading and compression zone modulus of elasticity; εa,max, εb,max—Strains at peak stress in longitudinal and transverse directions; σpost—Stress value in post-peak region at which nonlinear behavior begins; CCE, PCE, CSE—Cowper-Symonds factors; dfac—Damage factor; εmax—Erosion strain of element; εa,fail, εb,fail—Erosion strains in longitudinal and transverse directions.
下载: 导出CSV
表 5 弹壳及弹芯材料模型参数
Table 5 Material model parameters of bullet jacket and core
Material parameter Density/
(g·cm−3)Young’s modulus/
GPaPoisson’s ratio Yield stress/
GPaTagent modulus/
GPaHardening parameter Strain rate parameter (SRC) Strain rate parameter (SRP) Failure strain Bullet jacket 8.858 117 0.4 0.345 0 0 0 0 1.0 Bullet core 11.270 17 0.4 0.008 0.015 0.2 0.6 3.0 3.0
下载: 导出CSV
-
[1] ABTEW M A, BOUSSU F, BRUNIAUX P, et al. Ballistic impact mechanisms: A review on textiles and fibre-reinforced composites impact responses[J]. Composite Structures,2019,223:110966. DOI: 10.1016/j.compstruct.2019.110966
[2] CROUCH I G. Body armour: New materials, new systems[J]. Defence Technology,2019,15(3):241-253. DOI: 10.1016/j.dt.2019.02.002
[3] WHITE Z W, VERNEREY F J. Armours for soft bodies: How far can bioinspiration take us[J]. Bioinspiration & Biomimetics,2018,13(4):041004.
[4] ZHU D, SZEWCIW L, VERNEREY F, et al. Puncture resistance of the scaled skin from striped bass: Collective mechanisms and inspiration for new flexible armor designs[J]. Journal of the Mechanical Behavior of Biomedical Materials,2013,24:30-40. DOI: 10.1016/j.jmbbm.2013.04.011
[5] ZHU D, ORTEGA C F, MOTAMEDI R, et al. Structure and mechanical performance of a “Modern” fish scale[J]. Advanced Engineering Materials,2012,14(4):185-194. DOI: 10.1002/adem.201180057
[6] YANG W, SHERMAN V R, GLUDOVATZ B, et al. Protective role of Arapaima gigas fish scales: Structure and mechanical behavior[J]. Acta Biomaterialia,2014,10(8):3599-3614. DOI: 10.1016/j.actbio.2014.04.009
[7] VERNEREY F J, BARTHELAT F. Skin and scales of teleost fish: Simple structure but high performance and multiple functions[J]. Journal of the Mechanics and Physics of Solids,2014,68:66-76. DOI: 10.1016/j.jmps.2014.01.005
[8] VERNEREY F J, BARTHELAT F. On the mechanics of fishscale structures[J]. International Journal of Solids and Structures,2010,47(17):2268-2275. DOI: 10.1016/j.ijsolstr.2010.04.018
[9] FUNK N, VERA M, SZEWCIW L J, et al. Bioinspired fabrication and characterization of a synthetic fish skin for the protection of soft materials[J]. ACS Applied Materials & Interfaces,2015,7(10):5972-5983.
[10] WHITE Z, SHEN T, VOLK E M, et al. The role of surface properties on the penetration resistance of scaled skins[J]. Mechanics Research Communications,2019,98:1-8. DOI: 10.1016/j.mechrescom.2019.05.001
[11] 刘鹏, 汪俊文, 朱德举. 草鱼鳞片的多级结构及力学性能[J]. 复合材料学报, 2016, 33(3):657-665. LIU P, WANG J W, ZHU D J. Hierarchical structure and mechenical properties of scales from grass carp[J]. Acta Materiae Compositae Sinica,2016,33(3):657-665(in Chinese).
[12] LIU P, ZHU D, YAO Y, et al. Numerical simulation of ballistic impact behavior of bio-inspired scale-like protection system[J]. Materials & Design,2016,99:201-210.
[13] SHEN Z, HU D, YANG G, et al. Ballistic reliability study on SiC/UHMWPE composite armor against armor-piercing bullet[J]. Composite Structures,2019,213:209-219. DOI: 10.1016/j.compstruct.2019.01.078
[14] HU D, ZHANG Y, SHEN Z, et al. Investigation on the ballistic behavior of mosaic SiC/UHMWPE composite armor systems[J]. Ceramics International,2017,43(13):10368-10376. DOI: 10.1016/j.ceramint.2017.05.071
[15] 刘鹏. 鳞片多级结构、力学性能及其仿生研究[D]. 长沙: 湖南大学, 2017. LIU Peng. The research on hierarchical structure mechanical behavior and biomimetic of fish scales[D]. Changsha: Hunan University, 2017(in Chinese).
[16] FLORES-JOHNSON E A, SHEN L, GUIAMATSIA I, et al. Numerical investigation of the impact behaviour of bioinspired nacre-like aluminium composite plates[J]. Composites Science and Technology,2014,96:13-22. DOI: 10.1016/j.compscitech.2014.03.001
[17] DHANDAPANI K. Experimental investigation and development of a constitutive model for ultra high molecular weight polyethylene materials[D]. Phoenix: Arizona State University, 2009.
[18] AUDIBERT C, ANDREANI A S, LAINÉ É, et al. Mechanical characterization and damage mechanism of a new flax-Kevlar hybrid/epoxy composite[J]. Composite Structures,2018,195:126-135.
[19] KRISHNAN K, SOCKALINGAM S, BANSAL S, et al. Numerical simulation of ceramic composite armor subjected to ballistic impact[J]. Composites Part B: Engineering,2010,41(8):583-593. DOI: 10.1016/j.compositesb.2010.10.001
[20] 孙素杰, 赵宝荣, 王军, 等. 不同背板对陶瓷复合装甲抗弹性能影响的研究[J]. 兵器材料科学与工程, 2006, 29(2):70-72. DOI: 10.3969/j.issn.1004-244X.2006.02.019 SUN Sujie, ZHAO Baorong, WANG Jun, et al. Study on the penetration performance of ceramic armors with different backing plate[J]. Ordnance Material Science and Engineering,2006,29(2):70-72(in Chinese). DOI: 10.3969/j.issn.1004-244X.2006.02.019
[21] 朱德举, 赵波. 仿生柔性防护装具的设计及防弹性能测试[J]. 复合材料学报, 2020, 37(6):1411-1417. ZHU Deju, ZHAO Bo. Design and ballistic performance testing of bio-inspired flexible protection devices[J]. Acta Materiae Compositae Sinica,2020,37(6):1411-1417(in Chinese).
[22] SHEN W, NIU Y, BYKANOVA L, et al. Characterizing the interaction among bullet, body armor, and human and surrogate targets[J]. Journal of Biomechanical Engineering,2010,132(12):121001. DOI: 10.1115/1.4002699
-
期刊类型引用(2)
1. 罗敬之，金育安，孔浩宇，李光勇，张明华，杜建科. 基于微纳米纤维的多层次微结构设计制备及其传感应用. 复合材料学报. 2024(01): 207-218 . 
本站查看
2. 张松通，顾昊，胡海良，明海. 军用便携式机械能发电技术. 国防科技. 2024(04): 34-42 . 百度学术
其他类型引用(5)
-
计量
- 文章访问数: 3543
- HTML全文浏览量: 760
- PDF下载量: 140
- 被引次数: 7
