复合材料由于具有比刚度高，比强度高，性能可设计等优点，正广泛应用于航空、航天、汽车和船舶等领域^[1-3]。虽然有易于结构一体化的特点，可以大大降低零件数量，但仍然不可避免地存在多钉连接，复合材料多钉连接钉载分配分析是航空航天飞行器，特别是飞机上复合材料用量进一步提升的关键问题^[4-7]。

目前广泛采用的一种方法是有限元法^[8,9]，但是有限元法存在的缺点是计算量大，当螺栓数量成百上千后，即使采用二维单元进行简化，也依然难以满足计算量要求。另一种计算量极小的方法是刚度法^[10-15]，起源于上世纪40年代^[10]，目前，刚度法假设多排多列螺栓连接在拉伸载荷作用下，钉载分配在不同列上可以单独计算，对多个多排单列连接进行钉载分配分析，即可得到所有螺栓的钉载结果；对多排单列连接采用平面内弹簧-梁模型推导简单的刚度矩阵即可实现钉载分配计算^[12]。在发展了复杂的螺栓刚度模型后^[10-15]，刚度法已经可以模拟间隙、拧紧力矩和复合材料失效等对钉载分配的影响，可以满足一定条件下的工程应用。但是因为间隙随机分布之后，同一排上不同列的螺栓钉载将不再相同，连接板变形也不再相同，本方法的一个重要的假设，即不同列的钉载相互不影响，单列的钉载分配可以单独计算，已不再成立，采用本方法计算将会导致计算结果与实际结构的真实钉载存在很大的误差。

为了解决上述问题，本文提出一种考虑列间载荷传递的刚度方法，使得刚度方法可以考虑不同列螺栓孔间隙不同时对钉载分配的影响，高精度进行钉载分配计算。

1.   现有刚度法

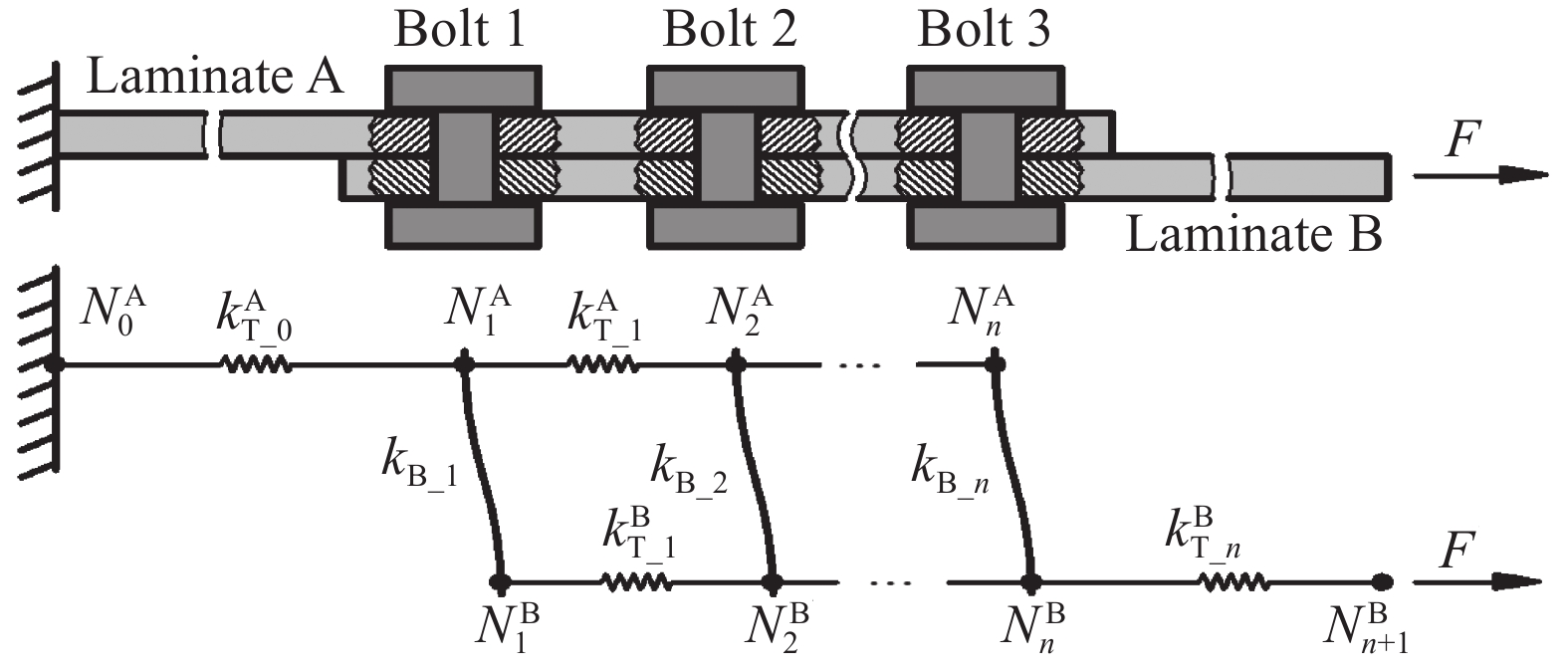
以如图1所示n排单列单剪连接结构为例，传统刚度法将连接结构简化为的一组弹簧-梁模型，并采用单参数迭代方法^[13,16]或者矩阵方法^[17,18]求解模型中螺栓传递的载荷。

弹簧-梁模型中的节点为螺栓与层合板的连接点以及层合板加载端点，用$N_i^{\text{A}}$和$N_i^{\text{B}}$(i=0,1,2,…,n+1)表示，其中上标“A”和“B”分别表示层合板A和层合板B。定义节点处变形为$\delta _i^{\text{A}}$和$\delta _i^{\text{B}}$，节点处载荷为$F_i^{\text{A}}$和$F_i^{\text{B}}$。单元有两种，分别是模拟层合板的弹簧，其刚度矩阵分别用${\boldsymbol{K}}_{{\text{T}}\_i}^{\text{A}}$和${\boldsymbol{K}}_{{\text{T}}\_i}^{\text{B}}$(i=1,2,…,n)表示，以及模拟螺栓的梁，其刚度矩阵用${\boldsymbol{K}}_{{\text{B}}\_i}^{}$(i=1,2,…,n)表示。

图  1  多排单列螺栓连接结构弹簧-梁模型

Figure  1.  Spring-beam model of multi-row single-column bolted joint structures

$N_i^{\text{A}}$-The i-th node on laminate A; $N_i^{\text{B}}$-The i-th node on laminate B; $k_{{\text{T}}\_i}^{\text{A}}$- Stiffness of laminate A in the i-th segment; $k_{{\text{T}}\_i}^{\text{B}}$- Stiffness of laminate B in the i-th segment; $k_{{\text{B}}\_i}^{}$-Stiffness of the i-th bolt

下载: 全尺寸图片 幻灯片

弹簧单元和梁单元均只考虑沿载荷方向的变形和刚度，每个单元有两个节点，刚度矩阵${{\boldsymbol{K}}_{\text{T}}}$和${\boldsymbol{K}}_{\text{B}}^{}$计算公式为

其中，刚度系数$k_{\text{T}}^{}$计算公式为

式中，E_L为连接板在载荷方向的等效刚度，w为板宽，t为板厚，L为两钉间距离。螺栓刚度系数$k_{\text{B}}^{}$采用试验进行测试^[12]。

一个单元的两个节点的载荷向量${\boldsymbol{F}}$包含两部分，分别是外载荷${{\boldsymbol{F}}_{\text{L}}}$和螺栓上载荷${{\boldsymbol{F}}_{\text{B}}}$，计算公式为

式中，F_L为施加在节点的外载荷，只有位于加载端处的节点(图1中的节点$N_0^{\text{A}}$和节点$N_{n + 1}^{\text{B}}$)上施加有外载荷，图1中的外载荷为F。${F_{\text{B}}}$为螺栓上等效外载荷，当存在间隙时，需将等效的外载荷施加在螺栓上，间隙为c_i时，等效载荷大小为$k_{{\text{B\_1}}}^{}{c_i}$。

根据广义胡克定律，弹簧-梁模型的载荷向量可用整体刚度矩阵与变形向量相乘计算：

其中，${\boldsymbol{K}}$是整体刚度矩阵，${\boldsymbol{\delta}}$是该弹簧-梁模型中节点的变形向量，${\boldsymbol{F}}$是节点载荷的向量。

对于图1中的弹簧-梁模型，当螺栓连接结构有3个钉时，模型中将包含8个节点、6个弹簧单元和3个梁单元。由于节点$N_0^{\text{A}}$处施加了固支边界条件，其位移为零，可以不写入整体刚度矩阵。因此三钉连接结构的弹簧-梁模型的整体刚度矩阵为7维矩阵。节点顺序为$N_1^{\text{A}},N_1^{\text{B}},$$N_2^{\text{A}},$$N_2^{\text{B}},$$N_3^{\text{A}},$$N_3^{\text{B}},$$N_4^{\text{B}}$时，节点变形向量为$\delta {\text{ = [}}\delta _1^{\text{A}},\delta _1^{\text{B}},\delta _2^{\text{A}},\delta _2^{\text{B}},\delta _3^{\text{A}},\delta _3^{\text{B}},\delta _4^{\text{B}}{]^{\text{T}}}$，整体刚度矩阵$K$计算公式为

载荷向量$F$计算公式为：

采用式(5)计算得到各节点变形后，可以计算得到螺栓载荷：

螺栓载荷除以总载荷F，即可得到钉载系数η。

2.   考虑列间剪切作用的刚度法

2.1   考虑列间剪切作用的刚度法模型

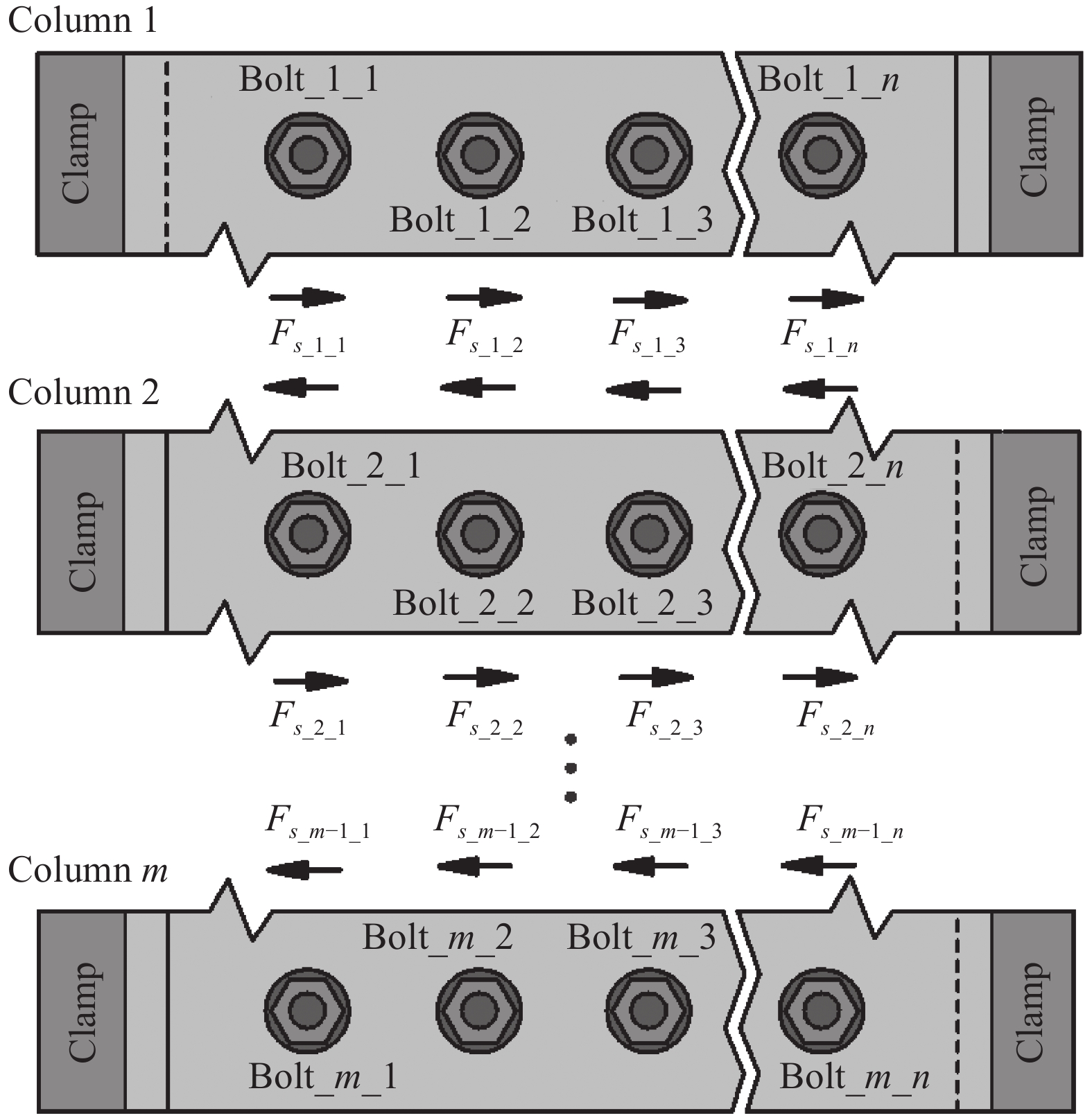
当同一排不同列的螺栓孔存在不同间隙时，这一列螺栓间的连接板会存在剪切变形，导致剪切力F_s，如图2(a)所示。因此，对于多排多列螺栓连接结构，不能再单独计算各列的钉载分配。

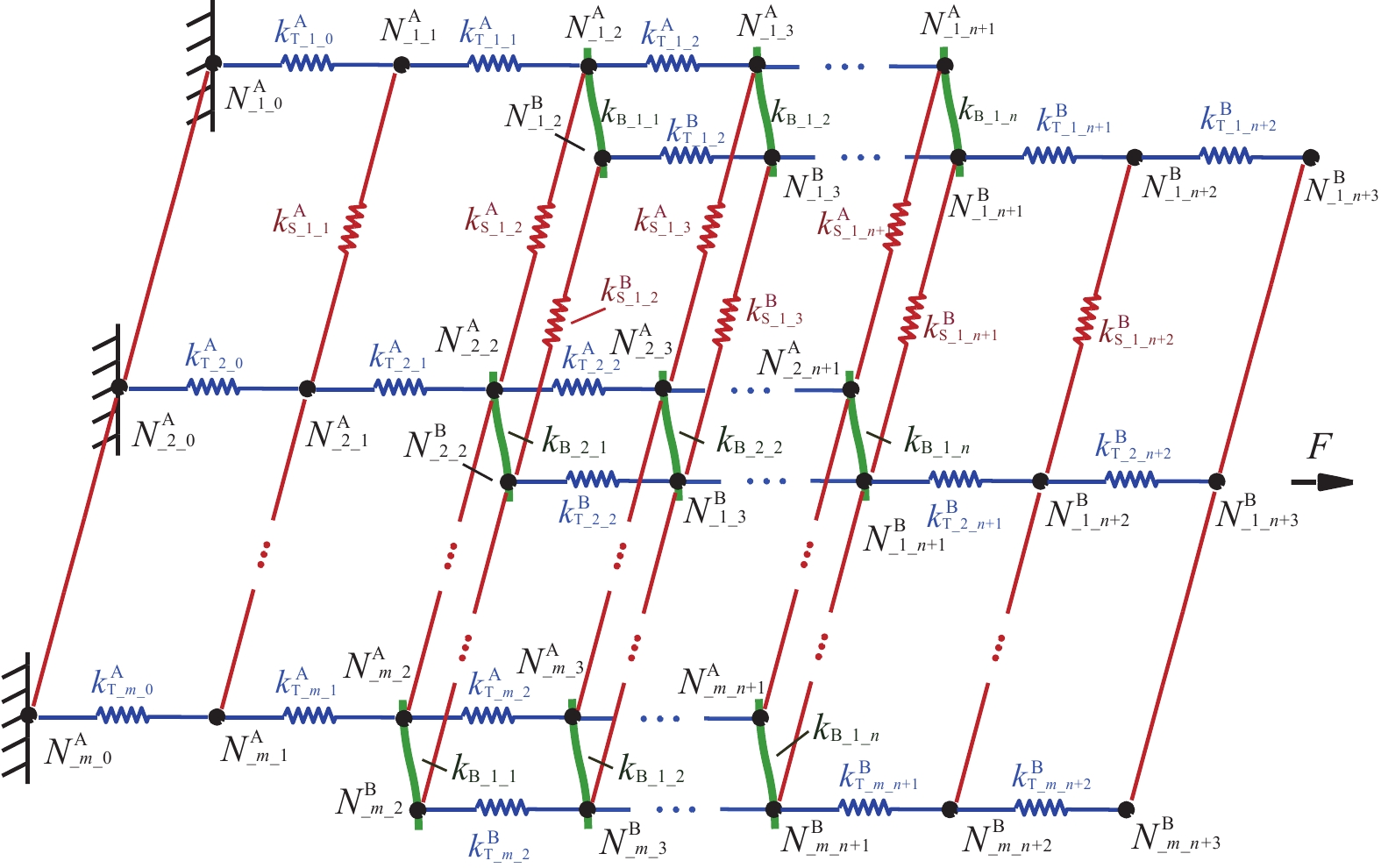
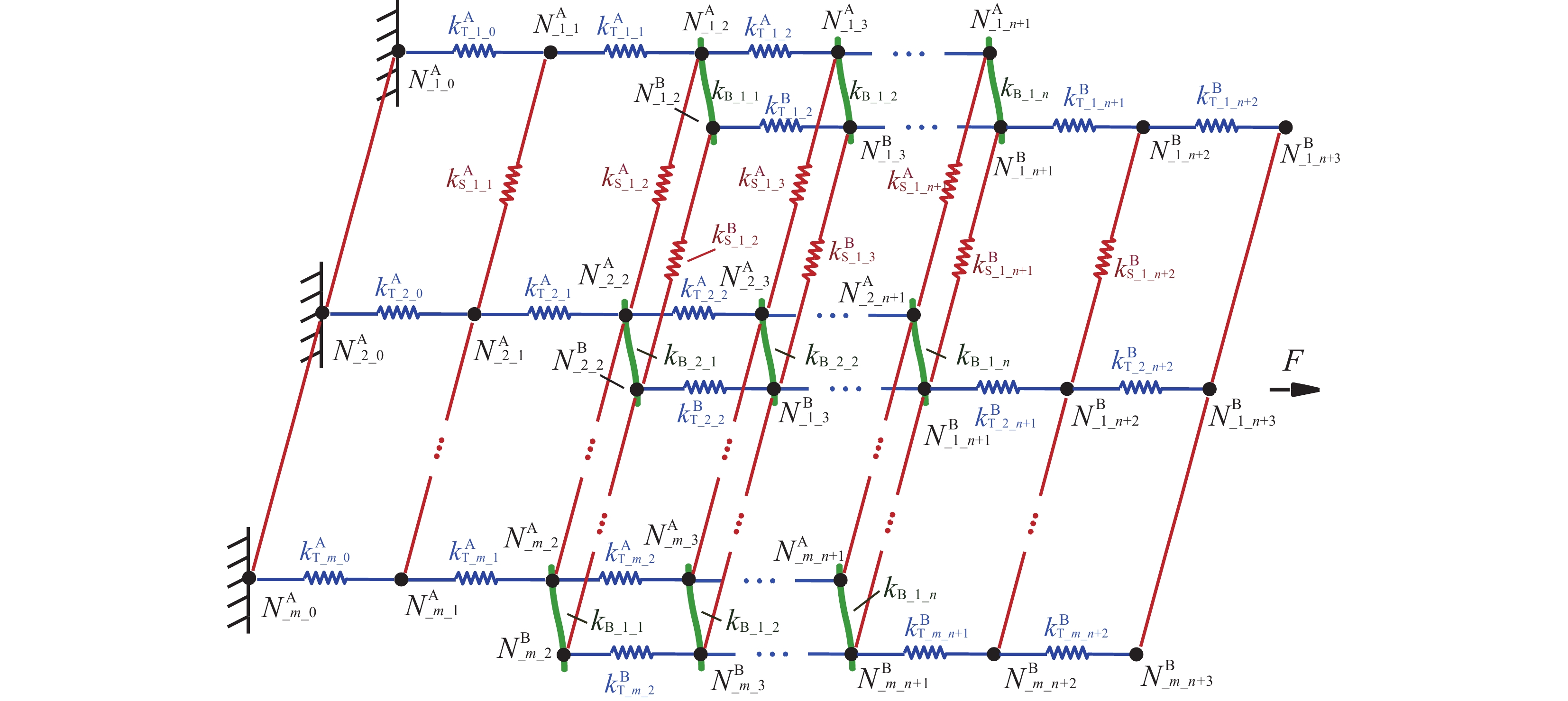
为了考虑多排多列螺栓连接结构各列之间的剪切相互作用，对图1的刚度法模型进行改进。首先将n排m列的结构简化为m组弹簧-梁模型，此处的弹簧是模拟连接板拉伸刚度的；然后，为了考虑这m组弹簧-梁模型之间的剪切相互制约关系，在m组模型间增加列间弹簧，模拟剪切行为，如图3所示，节点编号为$N_{\_i\_j}^{\text{A}}$、$N_{\_i\_j}^{\text{B}}$，拉伸弹簧、梁和剪切弹簧编号为：$k_{{\text{T}}\_i\_j}^{\text{A}}$和$k_{{\text{T}}\_i\_j}^{\text{B}}$，$k_{{\text{B}}\_i\_j}^{}$和$k_{{\text{S}}\_i\_j}^{\text{A}}$以及$k_{{\text{S}}\_i\_j}^{\text{B}}$。除此之外，图3中的弹簧-梁模型在层合板各列夹持段中间位置增加节点$N_{\_i\_1}^{\text{A}}$和$N_{\_i\_n + 2}^{\text{B}}$，用刚度系数为$k_{{\text{S}}\_i\_1}^{\text{A}}$和$k_{{\text{S}}\_i\_n + 2}^{\text{B}}$的弹簧连接各相邻列间增加的节点，用来模拟层合板各列夹持段间的剪切变形行为。位于层合板夹持段最边缘的节点$N_{\_i\_0}^{\text{A}}$和$N_{\_i\_n + 3}^{\text{B}}$之间采用刚性元件连接，用来模拟多排多列螺栓连接结构各列总变形相同的特征。把图3和图1对比可见，由图1的平面弹簧-梁模型变成了图3的三维空间模型。

图  2  多列连接结构列间载荷传递行为

Figure  2.  Load transfer behavior between columns of multi-column joint structures

${F_{s\_m\_n}}$-Shear force between the bolt in column m, row n and the bolt in column m+1, row n

下载: 全尺寸图片 幻灯片

图  3  计算多排多列连接结构钉载分配的三维弹簧-梁模型

Figure  3.  A Three-dimensional spring-beam model for calculation of load distribution in multi-row and multi-column bolted joint structures

$N_{\_m\_n}^{\text{A}}$- Node in the m-th column and n-th row of the laminate A; $N_{\_m\_n}^{\text{B}}$- Node in the m-th column and n-th row of the laminate B; $k_{{\text{T}}\_m\_n}^{\text{A}}$- Stiffness of the laminate A between the node in column m, row n and the node in column m, row n+1; $k_{{\text{T}}\_m\_n}^{\text{B}}$- Stiffness of the laminate B between the node in column m, row n and the node in column m, row n+1; $k_{S\_m\_n}^{\text{A}}$- Shear stiffness of the laminate A between the node in column m, row n and the node in column m+1, row n; $k_{S\_m\_n}^{\text{B}}$-Shear stiffness of the laminate B between the node in column m, row n and the node in column m+1, row n; $k_{{\text{B}}\_m\_n}^{}$-Stiffness of the bolt in column m, row n; F- External force

下载: 全尺寸图片 幻灯片

2.2   考虑剪切刚度的整体刚度矩阵

三维弹簧-梁模型的整体刚度矩阵相对于平面弹簧-梁模型需增加剪切刚度矩阵，比如，点$N_{{\text{\_1\_2}}}^{\text{A}}$和点$N_{{\text{\_2\_2}}}^{\text{A}}$之间的弹簧单元刚度矩阵${\boldsymbol{K}}_{{\text{S\_1\_2}}}^{\text{A}}$为

其中，刚度系数$k_{{\text{S\_1\_2}}}^{\text{A}}$代表了两个钉孔间发生沿载荷方向的相对位移时，相对位移和剪切载荷之间的关系，后续将采用有限元方法计算。

对于图3中的多排多列连接，当螺栓为两排两列时，三维弹簧-梁模型中将包含16个节点、12个模拟层合板拉伸变形行为的弹簧单元、6个模拟层合板剪切变形行为的弹簧单元和4个梁单元。由于节点$N_{{\text{\_1\_0}}}^{\text{A}}$和点$N_{{\text{\_2\_0}}}^{\text{A}}$处施加了固支边界条件，其位移为零，所以在整体刚度矩阵中可以不包含该节点的刚度，以降低计算量；则该两排两列连接结构的弹簧-梁模型的整体刚度矩阵为14维矩阵。设置节点顺序为$N_{{\text{\_1\_1}}}^{\text{A}}$、$N_{{\text{\_1\_2}}}^{\text{A}}$、$N_{{\text{\_1\_2}}}^{\text{B}}$、$N_{{\text{\_1\_3}}}^{\text{A}}$、$N_{{\text{\_1\_3}}}^{\text{B}}$、$N_{{\text{\_1\_4}}}^{\text{B}}$、$N_{{\text{\_1\_5}}}^{\text{B}}$、$N_{{\text{\_2\_1}}}^{\text{A}}$、$N_{{\text{\_2\_2}}}^{\text{A}}$、$N_{{\text{\_2\_2}}}^{\text{B}}$、$N_{{\text{\_2\_3}}}^{\text{A}}$、$N_{{\text{\_2\_3}}}^{\text{B}}$、$N_{{\text{\_2\_4}}}^{\text{B}}$和$N_{{\text{\_2\_5}}}^{\text{B}}$时，节点变形向量为

载荷向量为

整体刚度矩阵$K$为

2.3   剪切刚度计算方法

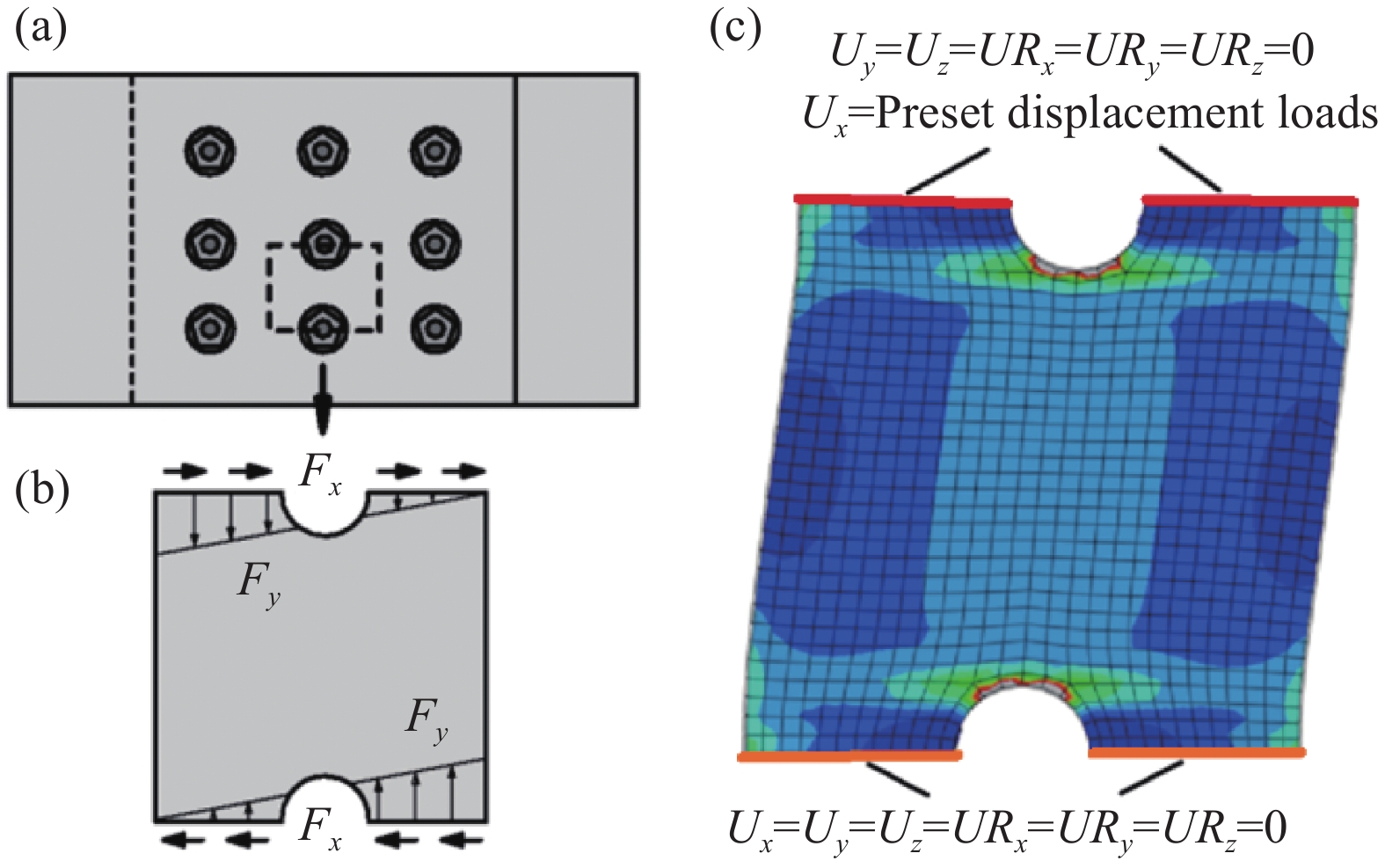
同一排相邻两列螺栓间的连接板(如图4(a)中虚线框所示)在钉载作用下变形时，其变形和边界上载荷分布受相邻连接板影响，具体形式非常复杂，如图4(b)所示。

图  4  层合板剪切刚度计算方法图

Figure  4.  Schematic diagram of calculation of shear stiffness of laminates

U_x- Translational degrees of freedom along the load direction; U_y- Translational degrees of freedom perpendicular to the load direction in the plane of laminates; U_z-Translational degrees of freedom perpendicular to the plane of the laminates; UR_x-Rotational degrees of freedom around the direction of the load; UR_y- Rotational degrees of freedom around the direction perpendicular to the load in the plane of laminates; UR_z- Rotational degrees of freedom around the direction perpendicular to the plane of the laminates

下载: 全尺寸图片 幻灯片

本方法为了得到连接板近似的剪切刚度，假设平行于载荷方向的两个边界上仅存在沿载荷方向的相对位移，这两个边界上点的其他平动和转动自由度均固定；假设垂直于加载方向上的边为自由边；然后采用有限元方法进行求解。取出的局部结构的有限元模型如图4(c)所示，定义载荷方向为x向，则设置图4(c)中红色线所示边界U_x为非零数，其他位移为0，橘黄色下边界上节点6个位移均为0，垂直于载荷方向的边不约束。则剪切刚度$k_{\text{S}}^{}$为x方向支反力和x向相对位移的U_x的比值。

3.   考虑列间剪切作用的刚度法验证

为了验证预测同一排不同列螺栓孔配合间隙不同时复合材料三排三列连接结构钉载分配时，本文三维弹簧-梁模型相对于平面弹簧-梁模型的优越性，本节设计了多种间隙分布的复合材料三排三列螺栓连接结构。采用两种方法开展钉载分配预测，并将预测结果与有限元分析结果进行比较。

3.1   复合材料三排三列螺栓连接及其有限元模型

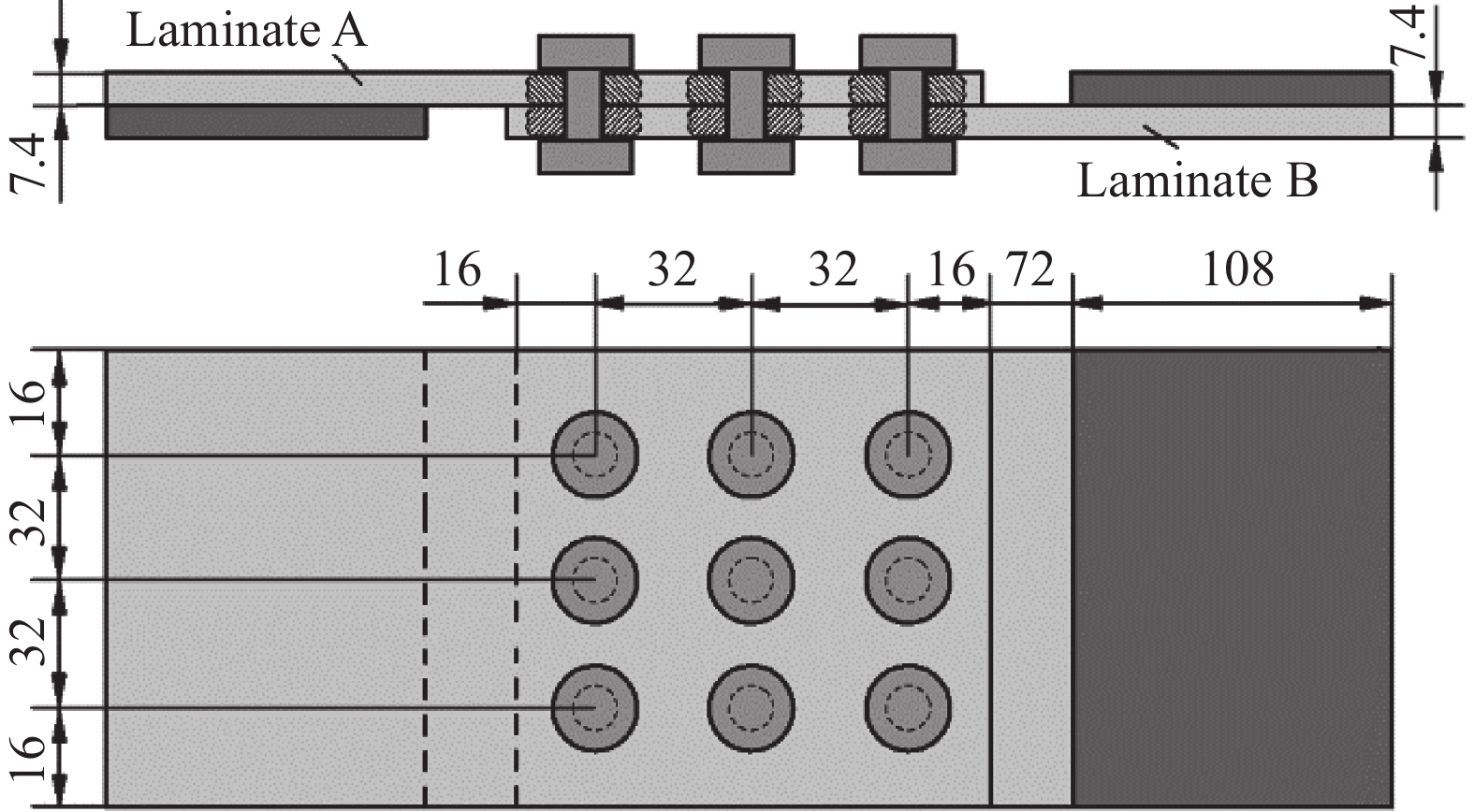
参考文献^[13]和^[16]中的三排单列螺栓连接设计了三排三列螺栓连接结构，其结构形式和几何尺寸如图5所示。层合板材料为T800复合材料，铺层顺序为：[45/0/−45/0/90/0/45/0/−45/0]_s2，单层厚度为0.185 mm。碳纤维增强聚合物(Carbon Fiber Reinforced Polymer, CFRP)单向板的材料参数：E₁₁=195 GPa，E₂₂=8.58 GPa，G₁₂=4.57 GPa，v₁₂=0.33。螺栓和螺母分别是直径为7.94 mm的HST12-10高锁螺栓^[19]和配套的HST1078-10螺母^[20]。设计了4种具有不同装配间隙的连接，其装配间隙如表1所示。

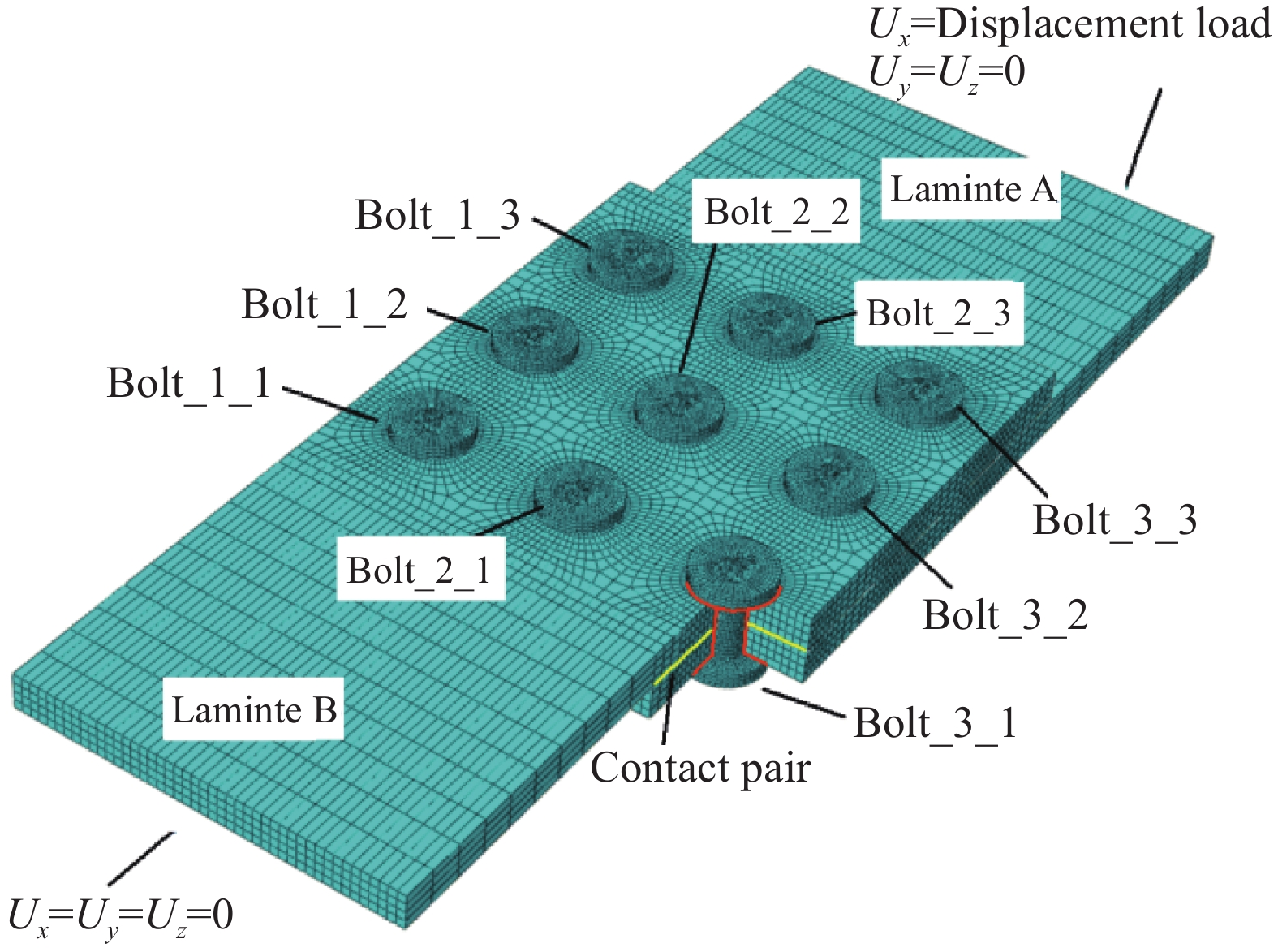
基于文献[16]中针对CFRP单剪螺栓连接结构的建模方法，建立复合材料三排三列螺栓连接结构的有限元模型，如图6所示。文献[16]中的有限元建模方法已经经过试验测试结果的验证。层合板和螺栓/螺母装配体采用实体单元C3 D8 R建立。为了得到螺栓孔周围更为准确的应力分布计算结果，在靠近螺栓和螺母的区域划分较为密集的网格。为了控制计算精度，每10个复合材料单层采用1层单元模拟，即将连接结构中的复合材料层合板沿厚度方向划分为4层单元。将模型中层合板A左端面三个方向的位移设置为零；层合板B右端面沿载荷方向施加非零位移，并将其余两个方向上的位移设置为零。层合板与层合板之间、层合板与螺栓之间定义接触单元，摩擦力采用传统的“库伦摩擦”模型模拟，层合板与层合板之间、层合板与螺栓之间的摩擦系数分别设置为0.55和0.1。

图  5  三排三列连接结构的几何形式及尺寸(单位：mm)

Figure  5.  Geometric forms and dimensions of multi-row and multi-column joint structures (Units: mm)

下载: 全尺寸图片 幻灯片

表  1  4种三排三列连接结构装配间隙方案(单位：mm)

Table  1.  Clearance of three-row and double-column joint structure and three-row and three-column joint structure (Units: mm)

No. of bolts	Without clearance	Clearance case 1	Clearance case 2	Clearance case 3
B_1_1	0	0.16	0.16	0.16
B_1_2	0	0	0	0
B_1_3	0	0	0	0
B_2_1	0	0.16	0.16	0.16
B_2_2	0	0	0.16	0.16
B_2_3	0	0	0	0
B_3_1	0	0	0	0
B_3_2	0	0	0	0
B_3_3	0	0	0	0.16

下载: 导出CSV 

| 显示表格

图  6  三排三列螺栓连接结构的有限元模型

Figure  6.  Finite element model of the three-row and three-column bolted joint structure

下载: 全尺寸图片 幻灯片

3.2   基于有限元方法的本文方法优越性验证

对于本文的三排三列螺栓连接结构，本文方法和现有方法中5种刚度($k_{{\text{T}}\_i\_j}^{\text{A}}$，$k_{{\text{T}}\_i\_j}^{\text{B}}$，$k_{{\text{B}}\_i\_j}^{}$，$k_{{\text{S}}\_i\_j}^{\text{A}}$和$k_{{\text{S}}\_i\_j}^{\text{B}}$)取值如下：A板拉伸刚度$k_{{\text{T}}\_i\_j}^{\text{A}}$(i=1,2,3)(j=0,1,2,3)和B板拉伸刚度$k_{{\text{T}}\_i\_j}^{\text{B}}$(i=1,2,3)(j=2,3,4,5)均取相同值，采用公式(3)计算得471.28 kN/mm。A板剪切刚度$k_{{\text{S}}\_i\_j}^{\text{A}}$(i=1,2,3)(j=1,2,3,4)和B板剪切刚度$k_{{\text{S}}\_i\_j}^{\text{B}}$(i=1,2,3)(j=2,3,4,5)均取相同值，采用2.3节方法进行计算，结果为450.70 kN/mm。螺栓刚度$k_{{\text{B}}\_i\_j}^{}$(i=1,2,3)(j=1,2,3)均相同，采用文献[13]的试验结果，数值为23.92 kN/mm。当间隙为0时螺栓上等效外载荷为0，当间隙为0.16 mm时螺栓上等效外载荷大小为3.83 kN。

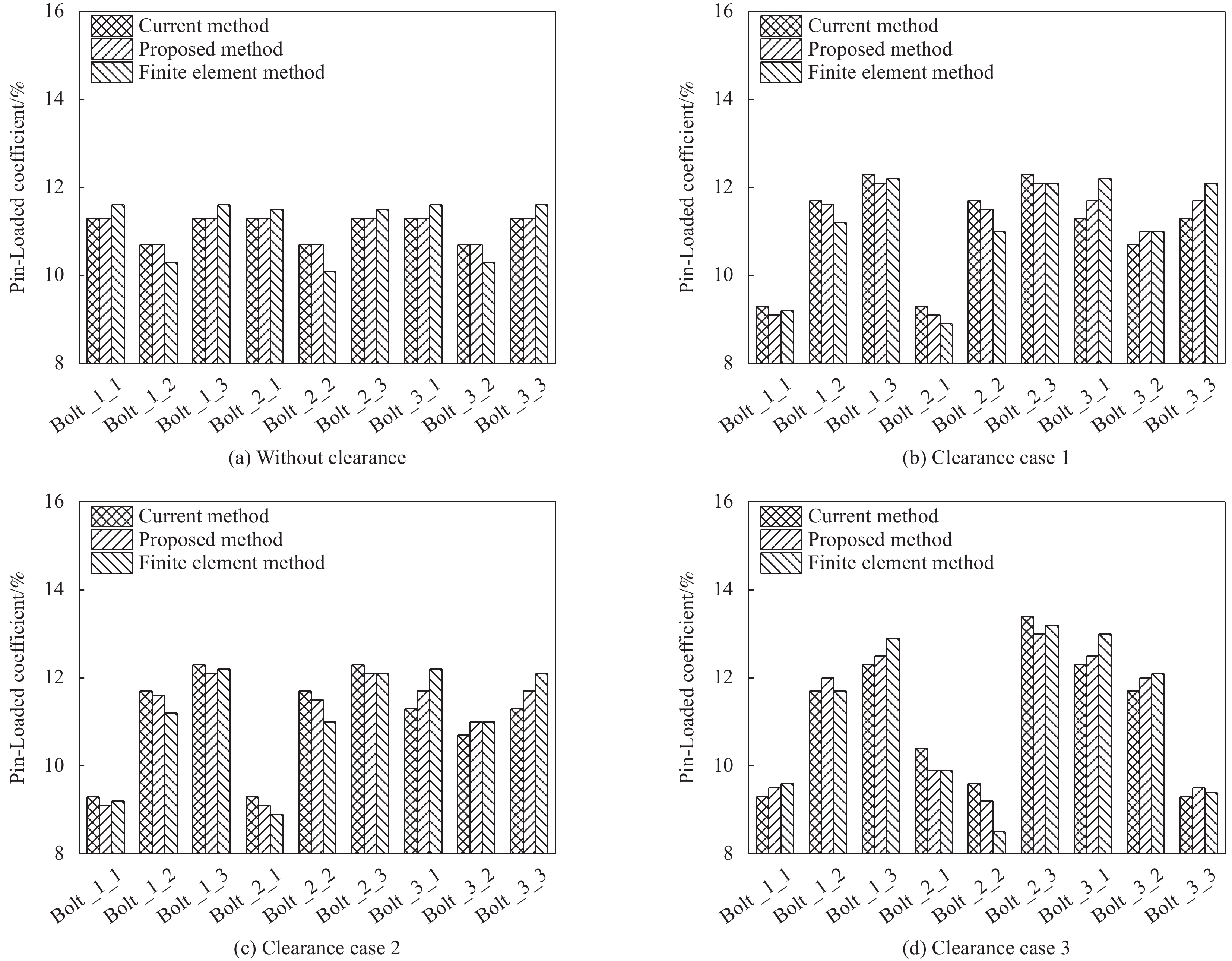
4种间隙方案时，本文方法、现有方法的钉载系数预测结果与有限元法结果比较情况如图7所示。

图  7  三排三列螺栓连接结构钉载分配预测结果

Figure  7.  Prediction results of pin load distribution of the three-row by three-column bolt joint structure

下载: 全尺寸图片 幻灯片

由图7可见，连接结构无间隙时，现有方法和本文方法预测结果相同，两种方法和有限元方法的钉载分配预测结果具有相同的分布趋势：第一排和第三排螺栓传递的载荷近乎相等，并略大于第二排螺栓传递的载荷，呈现出对称分布的形式；同一排不同列螺栓传递的载荷相同。

当连接结构中存在装配间隙时，本文方法和有限元方法的钉载分配预测结果中，装配间隙的存在不仅导致该处螺栓传递的载荷明显减小，同时也使得与其相邻的螺栓(与存在间隙的螺栓位于同一排或者同一列的螺栓)传递的载荷显著提高。而在现有方法的预测结果中，装配间隙仅对存在间隙的螺栓和同列的螺栓传递的载荷产生影响，对同排螺栓传递的载荷无影响，这体现了现有方法对复合材料多排多列连接结构钉载预测的局限性。

为了从数值上对比现有方法和本文方法对多排多列结构钉载系数的预测偏差，表2给出4种不同装配间隙下三排三列连接结构的钉载系数结果，其中包括采用有限元方法预测的钉载系数η_FE、采用现有刚度方法预测的钉载系数η_s、采用本文刚度方法预测的钉载系数η_m、以及η_s和η_m对η_FE的相对误差ε_s和ε_m。

表  2  三种方法预测的三排三列螺栓连接结构钉载系数结果及其对比

Table  2.  Results of bolt-load coefficient for three-row and three-column bolted joint structures predicted with three methods

Bolts		B_1_1	B_1_2	B_1_3	B_2_1	B_2_2	B_2_3	B_3_1	B_3_2	B_3_3
Without clearance	ηs	0.113	0.107	0.113	0.113	0.107	0.113	0.113	0.107	0.113
	ηm	0.113	0.107	0.113	0.113	0.107	0.113	0.113	0.107	0.113
	ηFE	0.116	0.103	0.116	0.115	0.101	0.115	0.116	0.103	0.116
	εs/%	−1.99	3.23	−1.99	−1.25	5.32	−1.25	−1.99	3.23	−1.99
	εm/%	−1.99	3.23	−1.99	−1.25	5.32	−1.25	−1.99	3.23	−1.99
Clearance case 1	ηs	0.093	0.117	0.123	0.093	0.117	0.123	0.113	0.107	0.113
	ηm	0.091	0.116	0.121	0.091	0.115	0.121	0.117	0.110	0.117
	ηFE	0.092	0.112	0.122	0.089	0.110	0.121	0.122	0.110	0.121
	εs/%	1.05	4.37	0.81	3.85	6.37	1.64	−7.13	−2.80	−6.24
	εm/%	−0.64	3.01	−0.50	1.34	4.39	−0.17	−3.95	0.52	−3.13
Clearance case 2	ηs	0.093	0.117	0.123	0.104	0.096	0.134	0.113	0.107	0.113
	ηm	0.093	0.118	0.124	0.097	0.089	0.127	0.119	0.112	0.119
	ηFE	0.094	0.115	0.126	0.097	0.082	0.129	0.124	0.111	0.123
	εs/%	−0.74	1.76	−2.35	7.04	16.83	3.64	−8.55	−4.10	−7.75
	εm/%	−0.14	2.30	−1.87	0.25	9.15	−1.59	−3.64	1.10	−2.90
Clearance case 3	ηs	0.093	0.117	0.123	0.104	0.096	0.134	0.123	0.117	0.093
	ηm	0.095	0.120	0.125	0.099	0.092	0.130	0.125	0.120	0.095
	ηFE	0.096	0.117	0.129	0.099	0.085	0.132	0.130	0.121	0.094
	εs/%	−2.74	0.12	−4.43	4.87	12.62	1.21	−5.23	−3.16	−1.62
	εm/%	−0.54	1.97	−2.69	0.39	7.71	−2.13	−3.41	−1.37	0.48
Notes: ηs, ηm, and ηFE are the bolt load factors predicted by the finite element method, the existing stiffness method and the proposed stiffness method, respectively; εs and εm are the relative errors of ηs and ηm with respect to ηFE.

下载: 导出CSV 

| 显示表格

由表2可见，无间隙时现有方法和本文方法预测结果相同，同一螺栓的|ε_s|和|ε_m|相同。在间隙方案1中，现有方法时螺栓_3_1的|ε_s|最大达到7.13%，本文方法时所有螺栓处的|ε_m|均不超过4.4%。在间隙方案2中，现有方法时螺栓_2_2的|ε_s|最大达到16.83%，螺栓_3_3和螺栓_2_1的|ε_s|也分别达到了7.75%和7.04%，本文方法时螺栓_2_2的|ε_m|最大，且不超过9.2%，其余螺栓处的|ε_m|均不超过3.7%。在间隙方案3中，现有方法时螺栓_2_2的|ε_s|最大达到12.62%，螺栓_3_1的|ε_s|也达到了5.23%，本文方法时螺栓_2_2的|ε_m|最大，且不超过7.8%，其余螺栓处的|ε_m|均不超过3.5%。验证了本文方法的优越性。

在计算效率方面，现有方法计算钉载分配时，对三个三排单列连接的7维整体刚度矩阵进行求逆计算，另外还需刚度等参数的计算和代数计算等，采用matlab进行计算，1 s内可以完成；本文方法对1个14维矩阵进行求逆，加上其他的刚度等参数计算和代数计算，也能在1 s内完成。但是本文方法需要单独进行层合板剪切刚度计算，需要建立有限元模型、求解和进行数据处理，需要时间以小时计。所以从计算效率看本文方法有所降低。但是因为剪切刚度计算比较简单，并且经过一次计算之后，对不同间隙的结构进行计算时，不需要重新计算剪切刚度，所以计算时间仍可接受。

需要说明的是，在一些情况下可将多排多列螺栓连接简化成多个多排单列连接，可以采用现有方法进行钉载分配计算，比如当螺栓连接的间隙为零时，两种方法计算结果相同；当不同列同一排的间隙相同时，不同列同一排钉孔变形会相同，可以忽略剪切变形，两种方法也能得到相同的结果；本文中对于8 mm直径的螺栓，间隙值采用了0.16 mm，这是航空航天工程中常用值中的较大值，当实际间隙小于这个值时，两种方法计算结果差别会变小，间隙非常小时，两种方法计算结果的差别可以忽略，可将多排多列螺栓连接简化成多个多排单列连接。

4.   结 论

(1)提出了一种考虑列间剪切效应，可以对同一排不同列螺栓孔配合间隙不同时，复合材料多排多列螺栓连接进行钉载分配预测的刚度法。

(2)通过将本文方法和现有方法预测的不同间隙工况的三排三列螺栓连接的钉载分配结果与有限元模型分析结果进行对比，验证了本文方法的优越性。

(3)本文方法预测结果表明，装配间隙的存在不仅导致该处螺栓传递的载荷明显减小，同时也使得与其相邻的螺栓(与存在间隙的螺栓位于同一排或者同一列的螺栓)传递的载荷显著提高。