碳纤维复合材料电导特性和力电耦合行为研究进展

韩朝锋; 薛有松; 张东生; 冯向伟; 陈莉娜; 朱晓伟; 吴海宏; 苏玉恒

doi:10.13801/j.cnki.fhclxb.20230119.004

碳纤维复合材料电导特性和力电耦合行为研究进展

韩朝锋^{1, 3, 5,},
薛有松²,
张东生³,
冯向伟¹,
陈莉娜¹,
朱晓伟⁴,
吴海宏^5, ,,
苏玉恒¹

1.
河南工程学院　纺织工程学院，郑州 450000
2.
东华大学　纺织学院，上海 201620
3.
巩义市泛锐熠辉复合材料有限公司，郑州 450000
4.
河南工业大学　土木建筑学院，郑州 450000
5.
河南工业大学　机电工程学院，郑州 450000

基金项目: 国家自然科学基金委-河南省联合基金重点项目(U1604253)；国家重点研发计划(2016YFB0101602)

详细信息

通讯作者:
吴海宏，博士，教授，博士生导师，研究方向为碳纤维复合材料结构-功能一体化　E-mail：hhwu@haut.edu.cn

中图分类号: TB332
计量
- 文章访问数: 1783
- HTML全文浏览量: 988
- PDF下载量: 293
出版历程
- 收稿日期: 2022-11-24
- 修回日期: 2023-01-05
- 录用日期: 2023-01-18
- 网络出版日期: 2023-01-18
- 刊出日期: 2023-06-14

Research progress on electrical property and electromechanical coupling behaviors of carbon fiber composites

1.
School of Textiles Engineering, Henan University of Engineering, Zhengzhou 450000, China
2.
College of Textiles, Donghua University, Shanghai 201620, China
3.
Gongyi Van-Research Innovation Composite Material CO., LTD., Zhengzhou 450000, China
4.
College of Civil Engineering and Architecture, Henan University of Technology, Zhengzhou 450000, China
5.
School of Mechanical and Electrical Engineering, Henan University of Technology, Zhengzhou 450000, China

Funds: National Natural Science Foundation of China-Henan Province Joint Fund Key Project (U1604253); Supported by the National Key Research and Development Program of China (2016YFB0101602)

摘要

摘要: 碳纤维复合材料具有良好的导电性能，但其作为增强体的树脂基复合材料的导电性能与增强体结构显著相关，且在加载条件下的结构变形触发碳纤维复合材料的导电行为发生改变，这一特性在智能传感器方面具有重要的应用。本文从复合材料电阻法结构健康监测应用出发，着重概述了不同维度增强体碳纤维树脂基复合材料的导电性能和预测模型、表面电势场分布和主要测量技术及力电耦合行为和本构模型，以期为碳纤维复合材料“材料-结构-功能”一体化集成系统的设计提供新方向和新途径。
- 碳纤维 /
- 电导率 /
- 电势场分布 /
- 力电耦合行为
Abstract: Carbon fiber composites have good electrical conductivity, however, the electrical conductivity of the resin matrix composites is significantly related to reinforcement architectures. The structural deformation under external load triggers the modification in the conductive behavior of carbon fiber composites, which has an important application in smart sensors. Based on the utilization of electrical resistance method in structural health monitoring for composites, this paper focuses on the overview of electrical conductivity and predictive models, surface potential field distribution and main measurement technologies, as well as electromechanical coupling behavior and constitutive models of carbon fiber reinforced resin matrix composites with different dimensional architectures. It is expected to provide a new direction and path to design "material-structure-performance" integrated system in carbon fiber composites.
- carbon fiber /
- electrical conductivity /
- potential distribution /
- electromechanical coupling behaviors

HTML全文

连续碳化硅纤维增韧碳化硅陶瓷基复合材料(SiC/SiC)具备高强度、耐高温、低密度等特点，可作为新型航空发动机的优选材料之一^[1-5]。随着SiC/SiC复合材料应用范围愈加广泛，不仅应用于航空领域，在化工领域中也是制造密封环的理想材料，在核聚变反应堆中可用于包层结构、包层流道内衬和转换器等方面^[6]，这些尖端领域均对复合材料的稳定性提出要求。化学气相渗透法(CVI)工艺导致陶瓷基复合材料(CMC)内部普遍存在一些缺陷(束内外孔隙、分层、预制体铺层错位和基体开裂等)，故其力学性能具有较大的离散性，强度作为结构设计的一项重要参数势必会受到其离散性的影响，从而限制结构的高可靠性设计。

CMC成型编织和致密化工艺复杂，易形成损伤源，有必要综合考虑制备工艺中的损伤对复合材料力学性能的影响，建立概率论可靠性模型^[7]。大量研究表明CMC拉伸强度服从Weibull分布^[8-13]。陶永强等^[14]通过渐进损伤的简化剪滞理论得到了各层应力分布及90°层裂纹密度与施加应力之间的关系；Curtin等^[15]基于基体裂纹随机开裂、纤维随机破坏理论，对单向增强CMC的应力-应变行为进行了模拟；孟志新等^[16]探究了不同纤维束下SiC CMC的拉伸行为；Vagaggini等^[17]计算了界面性能参数对复合材料拉伸行为的影响，表明增大界面脱粘能和界面滑移应力利于提高拉伸强度。目前CMC已实现工程化应用，评估工艺放大对复合材料性能分散性的影响成为主要的技术问题，分析单向纤维束复合材料拉伸强度的可靠性，评估结构单元和微结构特征对分散性的影响，也是该材料由小试走向中试的重要环节，小试指实验室开发优化阶段，中试指在小试的基础上继续放大、量产化。关于各组元对CMC性能影响的研究已有很多报道，但是关于小试炉与中试炉对CMC力学性能及其各组元影响的研究鲜有报道。

本文采用CVI技术分别在小试炉与中试炉中制备了单向纤维束SiC/SiC复合材料(Mini-SiC/SiC)的两批试样，探究工艺放大制备对复合材料力学性能离散的影响，并讨论造成复合材料力学性能离散的来源。其中小试炉为实验室制备，一台CVI沉积炉，中试为90台沉积炉，小试炉膛直径为600 mm，中试炉膛直径为1200 mm。通过双参数Weibull分布模型对Mini-SiC/SiC复合材料强度分布和可靠性进行分析，并基于双参数Weibull分布损伤模型建立拉伸载荷-位移关系，最终采用ORS Dragonfly软件分割各组元，结合基体间距裂纹公式分析分散性对可靠性的影响。

1. 材料制备与测试分析

1.1 Mini-SiC/SiC复合材料的制备

采用CVI法制备Mini-SiC/SiC复合材料，见图1。首先，均匀地将SiC纤维束缠绕到石墨框上，获得纤维预制体。然后，在纤维预制体表面沉积BN界面层，其中以BCl₃作为硼源，NH₃作为氨源，Ar为稀释气体，沉积温度600℃^[18]。最后，将沉积BN界面层的SiC纤维预制体在化学气相沉积炉中浸渗SiC基体，选择三氯甲基硅烷(MTS)为SiC基体的源物质，以H₂为载气，Ar为稀释气体，MTS与H₂摩尔比1∶10，沉积温度1150℃。Mini-SiC/SiC复合材料制备工艺参数如表1所示，Mini-SiC/SiC A为小试炉制备(炉膛直径为600 mm)，Mini-SiC/SiC B为中试炉制备(炉膛直径为1200 mm)，小试炉制备复合材料平均密度为3.09 g/cm²，中试炉制备复合材料平均密度为3.07 g/cm²。SiC纤维耐温为1350℃，故制备温度对其影响不大。

图 1 单向纤维束SiC/SiC (Mini-SiC/SiC)复合材料制备示意图

Figure 1. Schematic diagram of preparation of unidirectional fiber bundle SiC/SiC (Mini-SiC/SiC) composites

MTS—Methyltrichlorosilane

下载: 全尺寸图片幻灯片

表 1 Mini-SiC/SiC复合材料的制备工艺参数

Table 1. Process parameters of Mini-SiC/SiC composites

Material name	Deposition time/h		Inside diameter/mm
Material name	BN interface	SiC matrix	Inside diameter/mm
Mini-SiC/SiC A	100	160	600
Mini-SiC/SiC B	100	160	1200
Notes: Mini-SiC/SiC A—Preparation in a small test furnace (Furnace diameter of 600 mm); Mini-SiC/SiC B—Preparation in a pilot test furnace (Furnace diameter of 1200 mm).

下载: 导出CSV

| 显示表格

1.2 力学测试与分析方法

拉伸测试在美特斯(MTS)公司生产的CMT6203型微机控制电子万能试验机上进行。实验执行标准为GB/T 1040.5—2008^[19]，标距50 mm，加载速度3 mm/min。其拉伸测试特定夹具组件见图2。

图 2 拉伸测试特定夹具组件

Figure 2. Stretch test specific fixture assembly

F—Force

下载: 全尺寸图片幻灯片

Mini-SiC/SiC复合材料性能分析，采用以概率论为根据的结构可靠性模型，基于双参数Weibull分布模型对Mini-SiC/SiC复合材料的强度分布和可靠性进行分析。

1.3 表征与分析

采用扫描电子显微镜(SEM，SU3800，日本日立)观察Mini-SiC/SiC复合材料的显微结构和微观形貌。ORS Dragonfly软件在阈值分割的基础上，对Mini-SiC/SiC复合材料断层扫描(CT，AX-2000，宁波奥影检测)图像使用深度学习工具分割出灰度值相近的部分进行处理^[20]。

2. 结果与讨论

2.1 Mini-SiC/SiC复合材料非线性拉伸行为

图3为典型Mini-SiC/SiC复合材料载荷-位移曲线。该图由两组曲线组成，图3(a)、3(b)分别为小试炉与中试炉制备，可见曲线规律一致，故各选取其中一条曲线分析，曲线分三阶段：第一阶段加载初期，复合材料整体发生弹性变形，拉伸曲线斜率保持不变；第二阶段曲线呈非线性，且斜率逐渐减小，SiC基体产生新裂纹，随应力增加而增多；当达到最大载荷时曲线进入第三阶段，该阶段曲线未表现出韧性现象，呈现当基体裂纹达到饱和时，直接发生脆性断裂，其断口主要以纤维单丝形式拔出，未出现纤维簇形式拔出。

图 3 Mini-SiC/SiC复合材料典型的拉伸载荷-位移曲线

Figure 3. Typical tensile load-displacement curves of Mini-SiC/SiC composites

下载: 全尺寸图片幻灯片

2.2 Mini-SiC/SiC复合材料拉伸强度统计分布规律

采用中位估计法获取材料强度分布，计算每个强度所对应的失效概率，该失效概率为试验估计值。中位估计法失效概率估计值F_n可表示为

${F}_{\mathrm{n}}\left(\sigma \right)=\frac{i-0.5}{N}$

(1)

式中：i为强度从小到大排列的序数，即强度σ所对应的序号，i的最大值为 N；N为样本总量。

双参数Weibull分布模型累积失效分布函数F(σ) 数学表达式为

$F\left(\sigma \right)=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{\sigma }{{\sigma }_{0}}\right)}^{m}\right]$

(2)

式中： ${\sigma }_{0}$ 为尺度参数，当 σ₀=σ时，Weibull函数取最大值；m为形状参数，表示材料强度的分布规则，m越大，强度分散性越小。

将式(2)整理得：

$\mathrm{ln}\left\{\mathrm{ln}\left[\frac{1}{1-F\left(\sigma \right)}\right]\right\}=m\mathrm{ln}\sigma -m\mathrm{ln}{\sigma }_{0}$

(3)

由式(3)将 $\mathrm{ln}\left\{\mathrm{ln}\left[{1}/({1-F\left(\sigma \right)})\right]\right\}$ 作为y轴，lnσ作为x轴，可得：

$y=mx-m\mathrm{ln}{\sigma }_{0}$

(4)

将式(4)进行作图，并线性拟合分析，由此可得m和σ₀值。

2.2.1 参数估计方法

Weibull分布参数求解及检验主要数据(见附录表4)，线性拟合如图4所示。以Mini-SiC/SiC A复合材料为例，进行双参数Weibull分布模型的参数估计。

图 4 Mini-SiC/SiC复合材料拉伸强度Weibull分布的线性拟合图

Figure 4. Linear fitting diagram of Weibull distribution of tensile strength of Mini-SiC/SiC composites

F_n(σ)—Failure probability; σ—Strength value

下载: 全尺寸图片幻灯片

由图4(a)可得，Mini-SiC/SiC A线性拟合曲线函数为

$y=20.59x-122.03$

(5)

由此可知，m=20.59，σ₀=374.79 MPa。同理可得，Mini-SiC/SiC B的m=5.01，σ₀=400.74 MPa，前者m值大于后者，表明小试炉制备稳定性更优。此外，Weibull分布参数和线性相关系数如表2所示。由表可得，线性相关系数r都接近于1，变量之间存在高度相关性，线性拟合合理，因此m与σ₀接近真实值。

表 2 两种Mini-SiC/SiC复合材料拉伸强度双参数Weibull分布的参数和线性相关系数

Table 2. Parameters and linear correlation coefficient of two-parameter Weibull distribution for tensile strength of two Mini-SiC/SiC composites

Name	Shape parameter b	Scale parameter a/mm	r
Mini-SiC/SiC A	20.59	374.79	0.94
Mini-SiC/SiC B	5.01	400.74	0.98

下载: 导出CSV

| 显示表格

将上述得到的m和σ₀代入式(2)，Mini-SiC/SiC A复合材料的双参数Weibull累积失效分布函数可表示为

$F\left(\sigma \right)=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{\sigma }{374.79}\right)}^{20.59}\right]$

(6)

同理可得，Mini-SiC/SiC B的双参数Weibull累积失效分布函数可表示为

$F\left(\sigma \right)=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{\sigma }{400.74}\right)}^{5.01}\right]$

(7)

图5为两种Mini-SiC/SiC复合材料双参数Weibull累积失效分布函数曲线与中位估计数据曲线对比图。分析可知，分别采用式(6)、式(7)计算的曲线与中位估计法得到的曲线能够较好吻合。因此，拉伸强度分布规律能够采用双参数Weibull分布模型表征，且效果较好。

图 5 Mini-SiC/SiC复合材料Weibull累积分布函数曲线与中位估计数据的对比

Figure 5. Weibull cumulative distribution function curve compared with the median estimated data for Mini-SiC/SiC composites

下载: 全尺寸图片幻灯片

为检验Mini-SiC/SiC A复合材料拉伸强度与双参数Weibull分布的拟合优度。本文引入Kolmogorov-Smirnov(K-S)检验，可通过样本观测值的失效概率估计值F_n(σ)与假设的双参数Weibull累积失效概率分布F(σ)的差值进行对比分析，从而确定该失效概率的分布类型。

F_n(σ)与F(σ)的最大差值D_N由下式得出：

${D}_{\mathrm{N}}=\mathrm{m}\mathrm{a}\mathrm{x}\left|{F}_{\mathrm{n}}\left(\sigma \right)-F\left(\sigma \right)\right|$

(8)

F_n(σ)和F(σ)在上文中已完成计算，前者由中位估计法获得，后者则通过双参数Weibull分布函数计算得到，都代表材料在强度达到σ时发生失效的概率。D_N数据见附录表4。

工程上一般采取显著水平α＝0.05或α=0.01。经查阅D_N临界值表，当N大于35，D_N临界值等于D₃₅与 $\sqrt{N}$ 的比值，如附录表1所示。

当复合材料Mini-SiC/SiC A拉伸强度为356.48 MPa时(附录表4)，Kolmogorov距离取最大值D_N=0.1252<D_60,0.05=0.1756<D_60,0.01=0.2104，可知D_N小于显著水平α=0.05和α=0.01的D_N临界标准值。

采用相同方法可检验Mini-SiC/SiC B拉伸强度是否服从双参数Weibull分布，检验结果数据如附录表2所示。由表可知，D_N分别为0.0995均小于D_60,0.01和D_60,0.05，故拉伸强度均服从双参数Weibull分布，且拟合优度良好。

根据K-S检验可知，D_N小于显著水平α＝0.05和α=0.01的D_N临界标准值。故拉伸强度均服从双参数Weibull分布，且拟合优度良好。

可靠度R是度量可靠性的标准，其与失效概率关系可表示为

$R\left(\sigma \right)=1-F\left(\sigma \right)$

(9)

该式意味着给定任意强度，都可计算出其可靠度为多大，并随着强度增大，失效概率会增大，可靠度会减小。由于给出任意强度数据便可计算其可靠度，因此对于每个给定的可靠度，也可以计算出相应的可靠拉伸强度。联立式(2)和式(9)并求反函数，可获得给定可靠度下的可靠拉伸强度σ如下式：

$\sigma \left(R\right)={\sigma }_{0}{\left(\mathrm{ln}\frac{1}{R}\right)}^{\frac{1}{m}}$

(10)

由此就可获得任意可靠度所对应的可靠强度值。在工程上，一般将5%、25%、50%、75%、95%可靠度作为可靠质量标准。5个可靠度下的可靠拉伸强度值如附录表3所示。同时，不同可靠度下的可靠拉伸强度如附录图1所示。

研究表明，可靠度为50%时的可靠拉伸强度与平均拉伸强度较为接近，表3给出了可靠度为50%的可靠拉伸强度与平均拉伸强度。由表中数据通过计算绝对偏差和相对偏差可知：最大绝对偏差仅4.69 MPa，最大相对偏差仅1.2%。因此，采用平均拉伸强度可准确预测50%可靠度所对应的可靠强度，且误差较小。

表 3 两种Mini-SiC/SiC复合材料在可靠度50%时的可靠拉伸强度与平均拉伸强度

Table 3. Reliable tensile strength and average tensile strength of two Mini-SiC/SiC composites at 50% reliability

Name	Mean tensile strength/MPa	Tensile strength of 50% reliability/MPa	Absolute deviation/MPa	Relative deviation/%
Mini-SiC/SiC A	365.08	368.18	3.10	0.8
Mini-SiC/SiC B	367.78	372.47	4.69	1.2

下载: 导出CSV

| 显示表格

2.3 Mini-SiC/SiC复合材料拉伸载荷与位移关系的确定

复合材料拉伸应力σ与载荷P关系为

$\sigma =\frac{P}{S}$

(11)

复合材料拉伸应变ε与伸长量∆l关系为

$\varepsilon =\frac{\Delta l}{{l}_{0}}$

(12)

式中：S为材料截面积； ${l}_{0}$ 为标距，值为50 mm。

材料宏观损伤变量服从双参数Weibull分布，由式(2)与拉伸强度建立联系。在拉伸加载时，损伤贯穿整个过程，故累积损伤量和位移x同样服从双参数Weibull分布。因此累积损伤因子D_a随着位移x变化的分布函数可表示为类似于式(2)形式，如下所示：

${D}_{\mathrm{a}}=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{a}\right)}^{b}\right]$

(13)

由于SiC/SiC复合材料为脆性材料，为便于计算参数a、b，获取Weibull分布模型，因此将x视为断裂位移x_max。

将式(13)整理得：

$\mathrm{ln}\left\{\mathrm{ln}\left[\frac{1}{1-{D}_{\mathrm{a}}}\right]\right\}=b\mathrm{ln}{x}_{\mathrm{m}\mathrm{a}\mathrm{x}}-b\mathrm{ln}a$

(14)

可将 $\mathrm{ln}\left\{\mathrm{ln}\left[{1}/({1-{D}_{\mathrm{a}}})\right]\right\}$ 作为y轴，lnx_max作为x轴，得：

$y=b{x}_{\mathrm{m}\mathrm{a}\mathrm{x}}-b\mathrm{ln}a$

(15)

SiC/SiC复合材料中，SiC纤维和SiC基体均为脆性材料，各组分材料达到破坏极限前几乎未发生塑性变形。因此，基于双参数Weibull分布的弹性损伤模型表征Mini-SiC/SiC复合材料拉伸加载过程：

$P=k{\left(1-{D}_{\mathrm{a}}\right)}_{x}$

(16)

式中，k为抗拉刚度。

2.3.1 双参数Weibull分布模型参数估计

Weibull分布参数求解及检验的详细数据见附录表5，线性拟合如图6所示。以Mini-SiC/SiC A复合材料为例，进行双参数Weibull分布模型参数估计。

图 6 Mini-SiC/SiC复合材料断裂位移Weibull分布线性拟合

Figure 6. Linear fitting of Weibull distribution for fracture displacement of Mini-SiC/SiC composites

D_a—Cumulative damage factor

下载: 全尺寸图片幻灯片

Mini-SiC/SiC A复合材料拟合曲线函数为

$y=3.09x+0.42$

(17)

由此可得，b=3.09，a=0.87 mm。同理可得，Mini-SiC/SiC B的b=2.50，a=1.16 mm。此外，Weibull分布参数和线性相关系数r如表4所示。可知，线性相关系数都接近于1，变量之间存在高度相关关系，线性拟合合理，a与b接近真实数据。

表 4 两种Mini-SiC/SiC复合材料断裂位移双参数Weibull分布参数、线性相关系数

Table 4. Weibull distribution parameters and linear correlation coefficients of two Mini-SiC/SiC composites with two-parameter fracture displacement

Name	Shape parameter b	Scale parameter a/mm	r
Mini-SiC/SiC A	3.09	0.87	0.97
Mini-SiC/SiC B	2.50	1.16	0.99

下载: 导出CSV

| 显示表格

代入参数后，双参数Weibull累积损失因子损伤函数可分别表示为

${D}_{\mathrm{a}}=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{0.87}\right)}^{3.09}\right]$

(18)

${D}_{\mathrm{a}}=1-\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{1.16}\right)}^{2.50}\right]$

(19)

图7为拉伸强度的Weibull累积损伤因子函数拟合结果与试验结果对比图。分析可知，中位估计数据分别和式(18)、式(19)函数曲线吻合较好，因此，双参数Weibull模型能较好地表征累积损伤因子分布规律。

图 7 Mini-SiC/SiC复合材料Weibull累积损伤因子分布函数曲线与中位估计数据的对比

Figure 7. Weibull cumulative damage factor distribution function curves for Mini-SiC/SiC composites compared with median estimated data

下载: 全尺寸图片幻灯片

D_N为Kolmogorov距离；D_60,0.01为样本总量为60，显著水平α取0.01；D_60,0.05为样本总量为60，显著水平α取0.05。

采用K-S检验进行拟合优度分析，计算估计值D和D_a最大差异值可获得D_N。如表5所示，经比对发现，两种材料D_N值均小于D_60,0.01、D_60,0.05，故断裂位移均服从双参数Weibull分布，且拟合优度良好。

表 5 Mini SiC/SiC复合材料的D_N与D_60,0.01、D_60,0.05

Table 5. D_N and D_60,0.01, D_60,0.05 of Mini SiC/SiC composites

Name	D_N	D_60,0.01	D_60,0.05
Mini-SiC/SiC A	0.1139	0.2104	0.1756
Mini-SiC/SiC B	0.1036	0.2104	0.1756
Notes: D_N—Kolmogorov distance; D_60,0.01—Total sample size is 60 and the significance level α is taken as 0.01; D_60,0.05—Total sample size is 60 and α is taken as 0.05.

下载: 导出CSV

| 显示表格

2.3.2 拉伸载荷与位移关系

将式(13)代入式(16)得拉伸载荷-位移关系模型：

$P=k\left(\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{a}\right)}^{b}\right]\right)x$

(20)

为求解k值，假设拉伸载荷-位移为线性关系。将每个试样断裂位移和最大载荷数据代入式(20)，可得每个试样值，取平均值，即为k值。经计算，两种材料k值分别为370.89 N/mm、251.09 N/mm。将a、b、k值代入式(20)，得Mini-SiC/SiC A、Mini-SiC/SiC B拉伸载荷-位移模型分别为

$P=370.89\left(\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{0.87}\right)}^{3.09}\right]\right)x$

(21)

$P=251.09\left(\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left(\frac{x}{1.16}\right)}^{2.50}\right]\right)x$

(22)

将拉伸应力和载荷关系(式(11))与拉伸应变和加载位移之间的关系(式(12))及拉伸载荷-位移模型(式(20))联立进行求解，得拉伸应力-应变关系如下式：

$\sigma =\frac{k\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left({\varepsilon {l}_{0}}/{a}\right)}^{b}\right]\varepsilon {l}_{0}}{S}$

(23)

代入相关数据得上述两种材料拉伸应力-应变关系分别如下式所示：

$\sigma =\frac{370.89\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left({ 50\varepsilon }/{0.87}\right)}^{3.09}\right] 50\varepsilon}{S}$

(24)

$\sigma =\frac{251.09\mathrm{e}\mathrm{x}\mathrm{p}\left[-{\left({ 50\varepsilon }/{1.16}\right)}^{2.50}\right] 50\varepsilon }{S}$

(25)

为验证拉伸应力-应变关系合理性，抽取拉伸强度接近于平均值的试样进行检验。Mini-SiC/SiC A中选取最大载荷128.79 N，断裂位移0.83 mm的试样。Mini-SiC/SiC B中选取最大载荷132.60 N，断裂位移为1.03 mm的试样。求出试样断裂应变ε_max后，将该值分别代入式(24)、式(25)可获得采用拉伸应力-应变关系计算强度值(表6)。比对发现，与真实强度误差不超过7%。因此，该关系建立合理，可预测应力-应变关系。

表 6 Mini-SiC/SiC复合材料拉伸应力-应变关系检验过程主要数据

Table 6. Main data of the test process of tensile stress-strain relationship of Mini-SiC/SiC composites

Name	Mini-SiC/ SiC A	Mini-SiC/ SiC B
Maximum load P/N	128.79	132.60
Fracture displacement x_max/mm	0.83	1.03
True strength value σ/MPa	368.72	393.48
Fracture strain ε_max	0.0166	0.0205
Strength calculation value of con- stitutive model/MPa	373.50	366.40
Error between calculated value and real value	+1.2%	–6.8%

下载: 导出CSV

| 显示表格

2.4 基体对复合材料强度分散性的影响

源于CVI工艺的复杂性，故复合材料内部存在缺陷，这些缺陷导致复合材料宏观力学性能具有分散性。图8为Mini-SiC/SiC复合材料初始态与断口SEM图像。图8(a)为初始态单向纤维，可看出纤维束表面沉积基体较粗糙，存在参差不齐菜花状β-SiC，表明基体沉积状态具有不稳定性。由图8(b)、8(c)可见，Mini-SiC/SiC复合材料断口纤维呈纤维单丝形式拔出，基体裂纹未出现大范围偏转，表现瞬间断裂。当纤维束表面存在缺陷时，拉伸过程中会由于应力集中而成为断裂源，造成纤维早期破环，故降低纤维表面缺陷可提高纤维束强度，从而降低强度的分散性。

图 8 Mini-SiC/SiC复合材料初始态与断口SEM图像

Figure 8. SEM images of initial state and fracture of Mini-SiC/SiC composites

下载: 全尺寸图片幻灯片

图9采用ORS Dragonfly软件对Mini-SiC/SiC复合材料拉断CT图进行处理，图9(a)为初始状态下的正视断口，在阈值分割基础上采用深度学习方法提取拉伸断口裂纹分布(图9(b))情况，其中近似垂直于纤维且相互平行的线条为复合材料周期性裂纹。接着提取SiC纤维(图9(c))，可看出纤维分布并不均匀，纤维少的地方为基体与孔隙填充。随后，对断口俯视角度也进行学习处理(图9(d))，先提取SiC基体(图9(e))，可清楚看到纤维与基体分布状况，证实纤维少的地方由基体填充。最后，提取纤维表面BN界面相(图9(f))，可以看出界面相均匀覆盖纤维表面，形成一根根圆柱管。

图 9 ORS Dragonfly软件处理Mini-SiC/SiC复合材料断层扫描图像

Figure 9. ORS Dragonfly software for processing Mini-SiC/SiC composite tomography images

下载: 全尺寸图片幻灯片

由图8和图9从Mini-SiC/SiC复合材料宏观表面和分离复合材料内部各组元来看，制备态表面状态与复合材料内部纤维分布情况，会影响界面相与基体分布状态，导致复合材料分散性大，影响其可靠性。

复合材料拉伸断裂强度σ_u如下式所示：

${\sigma }_{\mathrm{u}}={\sigma }_{\mathrm{f}\mathrm{u}}{V}_{\mathrm{f}}$

(26)

式中： ${\sigma }_{\mathrm{f}\mathrm{u}}$ 为纤维断裂强度； ${V}_{\mathrm{f}}$ 为纤维体积分数。

由式(26)求得理论拉伸强度为499.45 MPa，但由于单向复合材料断裂时，连续纤维(l>30l_c)并非同时断裂，下式中载荷传递因子β为0.7，其纤维承载效率大于99%：

${\sigma }_{\mathrm{f}\mathrm{u}}={\sigma }_{\mathrm{f}\mathrm{u}}^{*}\left(1-\frac{1-\beta }{l/{l}_{\mathrm{c}}}\right)$

(27)

式中： ${\sigma }_{\mathrm{f}\mathrm{u}}^{*}$ 为纤维的原位断裂强度； $l/{l}_{\mathrm{c}}$ 为比长度；l为纤维长度；l_c为临界纤维长度。

此时求得理论拉伸强度为349.61 MPa，该值与实测拉伸强度365.08 MPa相差仅4.24%。表明纤维基本全部承载，纤维对复合材料分散性影响较小。

图10对Mini-SiC/SiC复合材料拉伸产生的周期性裂纹间距进行测量，最小裂纹间距为83.2 μm，最大裂纹为107.8 μm。根据图9(f)可知界面相均匀分布，故视界面滑移应力(τ)为定值，不影响复合材料的分散性。由式(28)可计算出(附录表8、表9) Mini-SiC/SiC复合材料基体裂纹宽度为89.43 μm，该宽度处于实际裂纹宽度范围内。验证了该式可以较好地描述Mini-SiC/SiC复合材料力学断裂行为。

图 10 ORS Dragonfly软件提取Mini-SiC/SiC复合材料周期性裂纹间距图

Figure 10. Extraction of periodic crack spacing diagram of Mini-SiC/SiC composites by ORS Dragonfly software

下载: 全尺寸图片幻灯片

将最小与最大裂纹间距代入式(25)，分别求出其对应拉伸强度为376.16 MPa和406.14 MPa，该值同样处于该批试样实际拉伸强度(331.02 MPa，407.82 MPa)内，表明基体裂纹间距具有离散性，基体是影响Mini-SiC/SiC复合材料可靠性的主要来源。

$\; \varsigma =\frac{{{b}_{2}{(1-{a}_{1}V_{\rm{f}})}^{2}R{{\sigma }_{\mathrm{p}}}^{2}}}{{4d{\tau }_{0}{E}_{\mathrm{m}}{V_{\rm{f}}}^{2} }}$

(28)

式中：V_f为纤维体积分数； ${\sigma }_{\rm{p}}$ 为峰值应力；R为纤维半径；d为裂纹间距；E_m为基体模量。

通过双参数Weibull分布模型可知，小试炉与中试炉拟合良好，故裂纹间距仍选用图10。此时中试炉试样拉伸强度(附录表6)范围为(161.09 MPa，540.95 MPa)，该范围大于小试炉试样拉伸强度范围，这是由于中试炉沉积基体工艺的放大化所导致，这种现象与m值对应，表明中试炉具有更大离散性(图3)。最大和最小裂纹间距对应的拉伸强度仍处于该范围内。

图11为基体影响复合材料形状参数m与力学性能离散的机制图。根据图9(f)与式(26)、式(27)可知，纤维与界面对复合材料性能离散的影响不大，故基体为影响复合材料可靠性的重要原因。m值影响应力-应变曲线非线性段的平稳性^[21]，m越大非线性段越平稳，表明复合材料在达到基体最大开裂后曲线越规律，离散性越小。图3与表2可明显看出小试炉制备的复合材料更加稳定，这是由于在小试炉中CVI沉积复合材料基体更加均匀，复合材料孔隙分布集中在内部中心区域(图11(a))，该区域的形成主要原因是基体在沉积时会形成沉积梯度，靠近表层区域的部分复合材料密度大、孔隙被充分填充，致使复合材料内部孔隙无法填充。反观中试炉制备，CVI工艺得到放大，同批次制备试样大大增多，造成复合材料内部孔隙发生不均匀分布现象(图11(b))，进而导致制备出的试样离散性增大、m值减小，影响其可靠性。

图 11 基体对Mini-SiC/SiC复合材料形状参数m与力学性能离散的影响

Figure 11. Matrix effects on the shape parameter m and mechanical property dispersion of Mini-SiC/SiC composites

下载: 全尺寸图片幻灯片

3. 结论

(1) 双参数Weibull分布模型可准确表征小试炉制备单向纤维束 SiC/SiC (Mini-SiC/SiC A)、中试炉制备Mini-SiC/SiC B拉伸强度分布，其拉伸强度分别为365.08 MPa和367.78 MPa，形状参数分别为20.59和5.01。

(2) 基于双参数Weibull分布模型建立的可靠度分析模型可准确预测不同可靠度下的可靠强度。其中，当可靠度为50%时，可靠强度与拉伸强度最大误差为1.2%，故在工程中可采用材料的平均拉伸强度预测50%可靠拉伸强度。

(3) 基于双参数Weibull分布模型建立的拉伸载荷-位移关系可准确预测拉伸应力-应变关系，与真实强度误差不超过7%。

(4) 复合材料内部界面相均匀包裹纤维，界面相滑移应力视为定值；连续纤维承载大于99%，纤维对复合材料性能离散性影响较小；通过基体裂纹间距公式可知，最小和最大裂纹间距所对应的拉伸强度为376.16 MPa和406.14 MPa，该值均处于Mini-SiC/SiC A复合材料拉伸强度(331.02 MPa，407.82 MPa)和Mini-SiC/SiC B复合材料拉伸强度(161.09 MPa，540.95 MPa)范围内。然而，Mini-SiC/SiC B复合材料拉伸强度范围大于Mini-SiC/SiC A，且前者 Weibull模数5.01比后者 Weibull模数 20.59低75.7%，中试分散性更大是由于中试炉尺寸放大所致，基体非均匀性是影响复合材料可靠性的主要原因。

图 1 碳纤维复合材料在结构健康监测(SHM)中的应用：(a) 力电耦合行为；(b) 导电机制；(c) 飞机机翼监测；(d) 桥梁承载监测

Figure 1. Application of carbon fiber composites in structural health monitoring (SHM): (a) Electromechanical coupling behavior; (b) Conductive mechanism; (c) Aircraft wing monitoring; (d) Bridge bearing monitoring

ΔR—Relative resistance change; R₀—Initial resistance; σ₁—Stress; ε₁—Strain

下载: 全尺寸图片幻灯片

图 2 复合材料不同维度增强体结构

Figure 2. Different dimensional reinforcement structure of composites

下载: 全尺寸图片幻灯片

图 3 复合材料内部电流传导路径示意图

Figure 3. Schematic diagram of internal current conduction path of composites

下载: 全尺寸图片幻灯片

图 4 碳纤维复合材料内部三维导电网络示意图^[23]

Figure 4. Schematic diagram of three-dimensional conductive network inside carbon fiber composite^[23]

下载: 全尺寸图片幻灯片

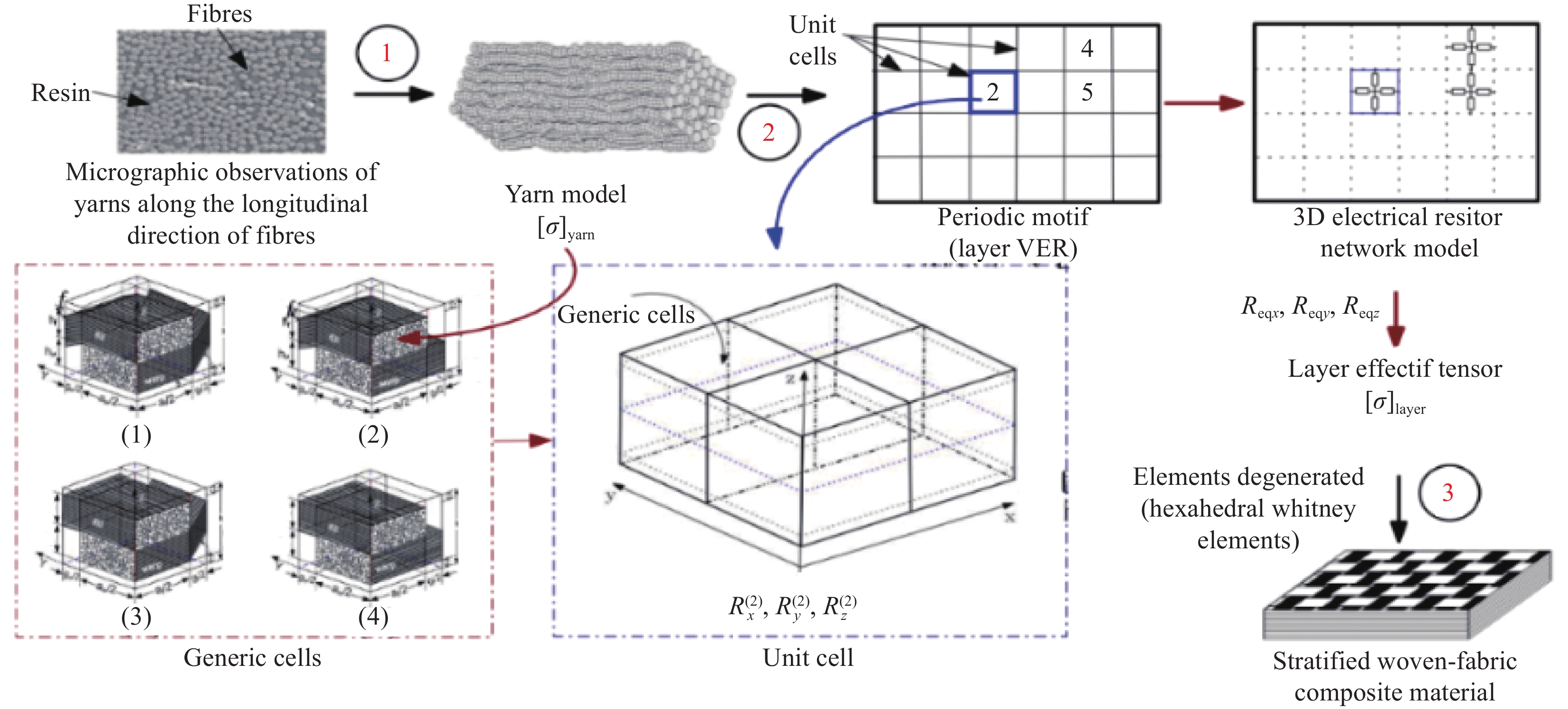
图 5 缎纹机织复合材料层压板电导率多尺度均质模型分析^[40]

Figure 5. Multi-scale homogeneous model analysis of electrical conductivity of satin woven composite laminates^[40]

[σ]_yarn—Yarn conductivity; R_x, R_y, R_z—Resistance in the x, y and z directions of Unit 2; R_eq_x, R_eq_y, R_eq_z—Equivalent resistance of single-layer composite materials in the x, y and z directions

下载: 全尺寸图片幻灯片

图 6 不同铺层结构碳纤维复合材料层压板电势场和温度场分布^[47]

Figure 6. Distribution of electric potential field and temperature field of carbon fiber composite laminates with different laminate structures^[47]

J—Electrical current; φ^*—Electrical potential field; E^*—Electrical field component; Q^*—Heat flux; T^*—Temperature; T^*_image—Thermal imaging temperature

下载: 全尺寸图片幻灯片

图 7 单对电极加载下平纹机织复合材料层压板表面电势场分布^[42]

Figure 7. Surface potential field distributions of plain woven composite laminates under single pair of electrodes^[42]

CFRP—Carbon fiber reinforced plastic; DC—Direct current

下载: 全尺寸图片幻灯片

图 8 两对电极加载下平纹机织复合材料层压板表面电势场分布^[42]

Figure 8. Surface potential field distributions of plain woven composite laminate under two pairs of electrodes^[42]

下载: 全尺寸图片幻灯片

图 9 3D机织角连锁(AI)织物结构

Figure 9. Architecture of 3D woven angle-interlock (AI) fabric

下载: 全尺寸图片幻灯片

图 10 单对电极加载下3D机织AI复合材料表面电势场分布^[56]

Figure 10. Surface potential field distributions of 3D woven AI composites under single pair of electrodes^[56]

AIW—Angle-interlock woven

下载: 全尺寸图片幻灯片

图 11 两对电极加载下3D机织AI复合材料表面电势场分布^[56]

Figure 11. Surface potential field distribution of 3D woven AI composites under two pairs of electrodes^[56]

下载: 全尺寸图片幻灯片

图 12 应变电压变化与循环次数曲线^[64]

Figure 12. Strain and Potential vs. number of loading cycles curve^[64]

CH 1—Channel 1; CH 2—Channel 2

下载: 全尺寸图片幻灯片

图 13 单节点处电阻网路和等效电阻模型^[68]

Figure 13. Electrical network and equivalent electrical circuit around a single node^[68]

δ—Electrical ineffective length; R_i_,_j—Node resistance; R_c—Contact resistance; V_i_,_j—Node voltage

下载: 全尺寸图片幻灯片

图 14 等效电阻模型^[70]

Figure 14. Equivalent electrical circuit^[70]

R_L(i)—Longitudinal resistance of the ith lamina; R_Γ(i)—Thickness resistance associated with half of the ith lamina; A—Voltage contact

下载: 全尺寸图片幻灯片

图 15 (a) 循环压缩实验；(b) 灵敏度系数与压缩应变曲线^[72]

Figure 15. (a) Cycle compressive test; (b) Gage factor vs compressive strain curve^[72]

下载: 全尺寸图片幻灯片

图 16 循环载荷与最大剪切载荷比和相对电阻变化关系^[11]

Figure 16. Relationship between the ratio of cycle load to shear strength and relative resistance change^[11]

下载: 全尺寸图片幻灯片

图 17 电阻-循环次数曲线^[14]

Figure 17. Resistance-number of loading cycles curve^[14]

下载: 全尺寸图片幻灯片

表 1 单向复合材料层压板电导率预测模型

Table 1 Prediction model for electrical conductivity of unidirectional composite laminates

Model	Electrical conductivity prediction model			Ref.
Model	Longitudinal direction	Transverse direction	Thickness direction	Ref.
Haider model (Composites containing conductive particles)	${\sigma _{{{xx}}}} = {\sigma _{\text{f}}}{V_{\rm{f}}} + {\sigma _{\rm{m}}}(1 - {V_{\rm{f}}})$	${\sigma _{{{yy}}}} = {\sigma _{zz}}{\text{ = }}{\sigma _{\text{m}}}\dfrac{{{\sigma _{\rm{f}}}(1 + {V_{\rm{f}}}) + {\sigma _{\text{m}}}(1 - {V_{\rm{f}}})}}{{{\sigma _{\rm{f}}}(1 - {V_{\rm{f}}}) + {\sigma _{\text{m}}}(1 + {V_{\rm{f}}})}}$		[34]
Penetration model(Unidirectional composite)	${\sigma _{{{zz}}}}{\text{ = }}{\sigma _{\rm{f}}}{\left( {{V_{\rm{f}}} - {V_{\rm{c}}}} \right)^{{p}}}$			[35]
Bueche model(Composites containing conductive particles)	${\sigma _{{{xx}}}} = \dfrac{{{{(1 - {V_{\rm{f}}})} /{{\sigma _{\rm{f}}} + {{{V_{\rm{f}}}{w_{\rm{g}}}} / {{\sigma _{\rm{m}}}}}}}}}{{{1 /{{\sigma _{\rm{m}}}{\sigma _{\rm{f}}}}}}}$			[36]
Fiber contact model(Multidirectional composites containing angle layers)	${\sigma _{ { {xx} } } } = \dfrac{ { {\text{π} } {d^2}X} }{ {4{ \sigma_{\text{f} } }{V_{\rm{p} } }{d_{\rm{c} } }l{\cos^2}\theta } }$	${\sigma _{{\text{yy}}}} = \dfrac{{{\text{π}} {d^2}X}}{{4{\sigma _{{\rm{f}}}}{V_{\rm{p}}}{d_{\rm{c}}}l{\text{si}}{{\text{n}}^2}\theta }}$		[37]
Fiber inclination model(Multidirectional composites containing angle layers)	${\sigma _{ { {xx} } } } = {\sigma _{\rm{f} } }{V_{\text{f} } }\cos{^2}\theta \left(1 - \dfrac{ {L\tan \theta } }{W}\right)$	${\sigma _{ { {yy} } } } = {\sigma _{\rm{f} } }{V_{\text{f} } }{\text{si} }{ {\text{n} }^2}\theta \left(1 - \dfrac{ {L\cot \theta } }{W}\right)$		[37]
Effective medium model(Unidirectional composite)	${\sigma _{{{xx}}}} = {\sigma _{\rm{m}}}\left[ {1 + \dfrac{{{V_{\rm{f}}}({\sigma _{\rm{f}}} - {\sigma _{\rm{m}}})\left[ {({\sigma _{\rm{f}}} - {\sigma _{\rm{m}}})\left( {{S_{11}} + {S_{33}}} \right) + 2{\sigma _{\rm{m}}}} \right]}}{{2{{({\sigma _{\rm{f}}} - {\sigma _{\rm{m}}})}^2}(1 - {V_{\rm{f}}}){S_{11}}{S_{33}} + {\sigma _{\rm{m}}}({\sigma _{\rm{f}}} - {\sigma _{\rm{m}}})(2 - {V_{\rm{f}}})({S_{11}} - {S_{33}})}}} \right]$			[38]
McCullough model(Composites containing conductive particles)	${\sigma _{ { {ii} } } } = {V_{\rm{f} } }{\sigma _{\rm{f} } } + {V_{\rm{m} } }{\sigma _{\rm{m} } } - \dfrac{ { {\lambda _i}{V_{\rm{f} } }{V_{\rm{m} } }{ {({\sigma _{\rm{f} } } - {\sigma _{\rm{m} } })}^2} } }{ { {V_{ { {{\rm{f}}i} } } }{\sigma _{\rm{f} } } + {V_{ { {{\rm{m}}i} } } }{\sigma _{\rm{m} } } } }$ ${V_{{{\rm{f}}}}}_{{i}} = (1 - {\lambda _i}){V_{\text{f}}} + {\lambda _i}{V_{\text{m}}}$ , ${V_{\text{m}}}_{{i}} = (1 - {\lambda _i}){V_{\text{m}}} + {\lambda _i}{V_{\text{f}}}$ , i=1, 2, 3			[39]
Notes: ${\sigma _{\text{f}}}$ —Fiber conductivity; ${\sigma _{\text{m}}}$ —Resin conductivity; ${V_{\text{f}}}$ —Fiber volume fraction; ${V_{\text{c} } }$ —Critical fiber volume fraction; p—Critical exponent; ${w_{\text{g} } }$ —Conductive particle content; d—Fiber diameter; ${d_{\text{c}}}$ —Fiber contact circle diameter; X—Fiber contact coefficient; l—Fiber length; ${V_{\text{p}}}$ —Contact fiber volume; L—Sample length; W—Sample width; $\theta$ —Fiber inclination angle; S—Shape parameter; x, y, z—Coordinate direction; 1, 2, 3—Principal axis direction; ${\lambda _{{{{i}}} } }$ —Fiber contact factor; σ_xx, σ_yy, σ_zz—Electrical conductivity in the x, y and z directions; σ_ii—Conductivity component.

下载: 导出CSV

表 2 单/多向复合材料层压板力电耦合本构模型

Table 2 Electromechanical coupling constitutive model of unidirectional/multi-directional composite laminates

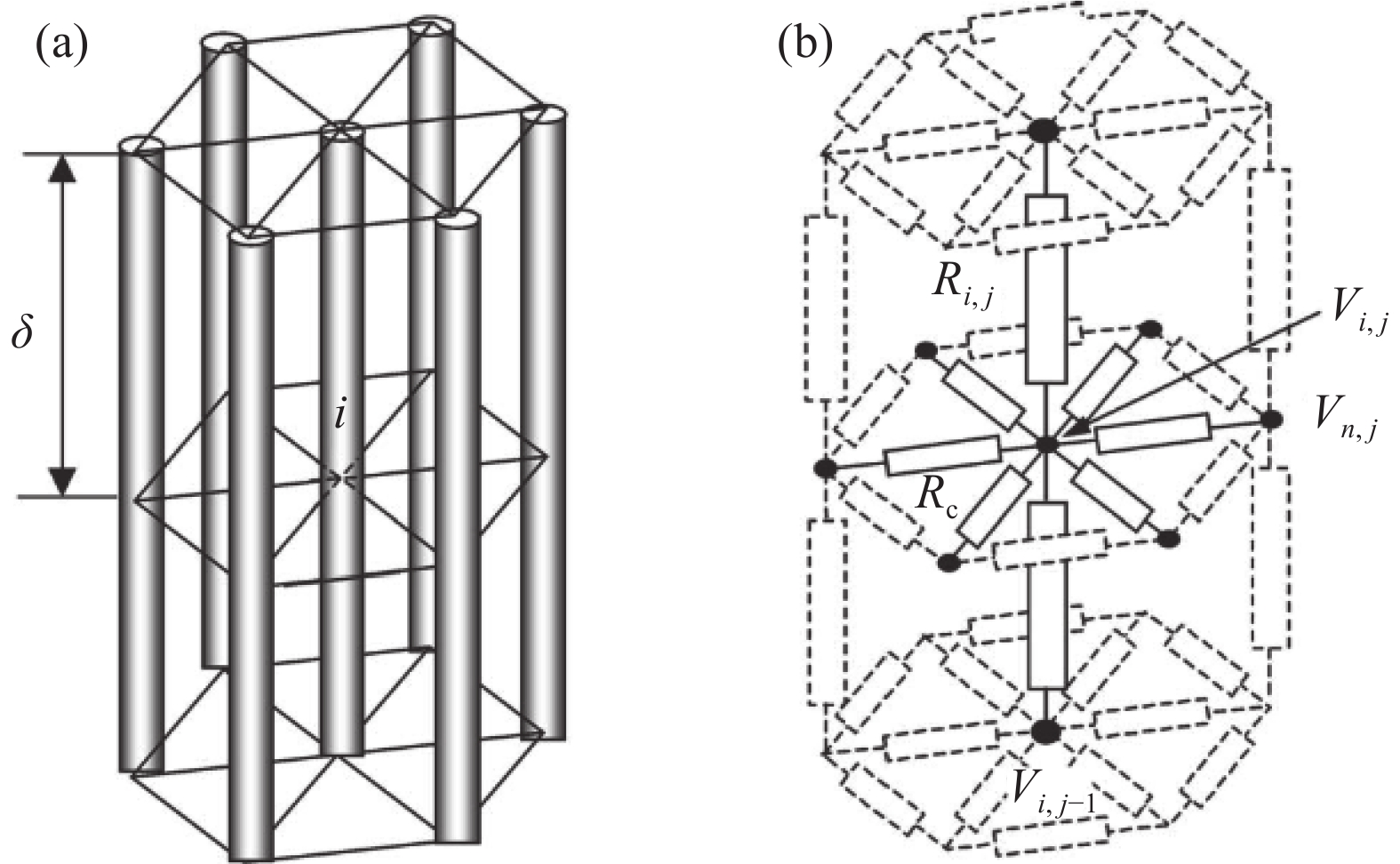
Dimension	Resistance model	Electromechanical coupling constitutive model	Ref.
1D	Considering fiber contact resistance, the basic resistance unit is the electrical ineffective length, which is suitable for unidirectional laminates	$\dfrac{ {\Delta R} }{R} = \dfrac{ {(1 + \alpha \varepsilon )} }{ {\exp\left[ { - \left(\dfrac{ { {\delta _{\rm ec} } } }{ { {L_{\rm{O}}} } }\right)\left(\dfrac{ { {E_{\rm{f} } }\varepsilon } }{ { {\sigma _0} } }\right)} \right]} }{ { - } }1$	[67]
2D	Considering fiber contact resistance, the basic resistance unit is 1/2 of the ineffective length, suitable for unidirectional laminates	$\begin{gathered} \dfrac{{\Delta {R_{11}}}}{{{R_{11}}}} = \dfrac{{1 + K{}_{11}{\varepsilon _{11}}}}{{{{\left( {1 - {F_1}} \right)}^2}}} - 1(0^\circ ) \\ \dfrac{{\Delta {R_{22}}}}{{{R_{22}}}} = \dfrac{{1 + K{}_{22}{\varepsilon _{22}}}}{{1 - {F_2}}} - 1(90^\circ ) \\ \end{gathered}$	[69]
1D	Considering the fiber contact resistance, it follows Weibull statistical distribution; Conductivity, which follows a linear change before fiber fracture and an exponential change after fiber fracture, suitable for multi-directional laminates	$\begin{gathered} \dfrac{ {\Delta R} }{R} = \dfrac{ { {\text{1 + e} } } }{ { {\sigma / { {\sigma _{\rm 0} } } } } } - 1(\varepsilon \leqslant 10\% ) \\ \dfrac{ {\Delta R} }{R} = \dfrac{ { {\text{1 + e} } } }{ {b{ {\rm e}^{ - { {(S - {S_0})}^t} } } }} - 1(\varepsilon \leqslant 10\% ) \\ \end{gathered}$	[71]
2D	Considering Poisson effect, strain effect, and damage effect, suitable for multi-directional laminates	$\begin{gathered}\upsilon = - \dfrac{{{\varepsilon _{11}}}}{{{\varepsilon _{33}}}},{\left( {\dfrac{{\Delta \rho }}{\rho }} \right)_{{\text{reversible}}}} = \alpha {\varepsilon _{33}} \\ \dfrac{{\Delta {R_{33}}}}{{{R_{33}}}} = {\varepsilon _{33}}(1 + \alpha + 2\upsilon ) \\ \dfrac{{\Delta {R_{11}}}}{{{R_{11}}}} = \dfrac{{\Delta {\rho _{11}}}}{{{\rho _{11}}}} - \dfrac{{{{\Delta {R_{33}}} \mathord{\left/ {\vphantom {{\Delta {R_{33}}} {{R_{33}}}}} \right. } {{R_{33}}}}}}{{1 + \alpha + 2\upsilon }} \\ {\left( {\dfrac{{\Delta \rho }}{\rho }} \right)_{{\text{irreversible}}}} = \beta {\varepsilon _{33}} \\ \end{gathered}$	[72]
Notes: $\Delta R$ —Resistance change rate; R—Resistance; $\alpha$ —Gage factor; $\varepsilon$ —Strain; ${\delta _{{\text{ec}}}}$ —Slip length; ${E_{\text{f}}}$ —Tensile modulus; L—Sample length; ${L_{\text{O}}}$ —Reference length; ${\sigma _{\text{0}}}$ —Weibull scale factor; K—Strain coefficient; ${F_{\text{1}}}$ —Failure probability of series resistor components; ${F_{\text{2}}}$ —Probability of failure of parallel resistor components; S—Loading stress; ${S_{\text{0}}}$ —Reference stress; $\Delta \rho$ —Resistivity change rate; $\rho$ —Resistivity; υ—Poisson's ratio; b—Constant, 0.973; t—Constant,8.67; β—Damage effect coefficient.

下载: 导出CSV

参考文献(77)

[1]	LLORCA J, GONZALEZ C, MOLINA-ALDAREGUIA J M, et al. Multiscale modeling of composite materials: A roadmap towards virtual testing[J]. ChemInform, 2011, 23: 5130-5147.
[2]	王晓旭, 张典堂, 钱坤, 等. 深海纤维增强树脂复合材料圆柱耐压壳力学性能的研究进展[J]. 复合材料学报, 2020, 37(1): 16-26. WANG X X, ZHANG D T, QIAN K, et al. Research progress on mechanical properties of deep-seafiber reinforced resin composite cylindrical pressureshells[J]. Acta Materiae Compositae Sinica, 2020, 37(1): 16-26(in Chinese).
[3]	HUANG Z M, LI P. Prediction of laminate delamination with no iteration[J]. Engineering Fracture Mechanics, 2020, 238: 107248.
[4]	GE L, LI H, ZHONG J, et al. Micro-CT based trans-scale damage analysis of 3D braided composites with pore defects[J]. Composites Science and Technology, 2021, 211: 108830.
[5]	KE Y, HUANG S, GUO J, et al. Effects of thermo-oxidative aging on 3-D deformation field and mechanical behaviors of 3-D angle-interlock woven composites[J]. Composite Structures, 2022, 281: 115116.
[6]	IRFAN M S, KHAN T, HUSSAIN T, et al. Carbon coated piezoresistive fiber sensors: From process monitoring to structural health monitoring of composites—A review[J]. Composites Part A: Applied Science and Manufacturing, 2021, 141: 106236.
[7]	GONZÁLEZ C, VILATELA J J, MOLINA-ALDAREGUÍA J M, et al. Structural composites for multifunctional applications: Current challenges and future trends[J]. Progress in Materials Science, 2017, 89: 194-251.
[8]	CHUNG D D L. A review of multifunctional polymer-matrix structural composites[J]. Composites Part B: Engineering, 2019, 160: 644-660.
[9]	ROH H D, OH S Y, PARK Y B. Self-sensing impact damage in and non-destructive evaluation of carbon fiber-reinforced polymers using electrical resistance and the corresponding electrical route models[J]. Sensors and Actuators A: Physical, 2021, 332: 112762.
[10]	MATSUZAKI R, YAMAMOTO K, TODOROKI A. Delamination detection in carbon fiber reinforced plastic cross-ply laminates using crack swarm inspection: Experimental verification[J]. Composite Structures, 2017, 173: 127-135.
[11]	HAN C, HUANG S, SUN B, et al. Electrical resistance changes of 3D carbon fiber/epoxy woven composites under short beam shear loading along different orientations[J]. Composite Structures, 2021, 276: 114549.
[12]	CAN-ORTIZ A, ABOT J L, AVILÉS F. Electrical characterization of carbon-based fibers and their application for sensing relaxation-induced piezoresistivity in polymer composites[J]. Carbon, 2019, 145: 119-130.
[13]	SALEH M N, YUDHANTO A, LUBINEAU G, et al. The effect of z-binding yarns on the electrical properties of 3D woven composites[J]. Composite Structures, 2017, 182: 606-616.
[14]	薛有松, 薛凌明, 孙宝忠, 等. 碳纤维三维角联锁机织复合材料弯曲作用下力阻响应[J]. 复合材料学报, 2023, 40(3): 1468-1476. XUE Y S, XUE L M, SUN B Z, et al. Piezoresistive effect of carbon fiber 3D angle-interlock woven composites under bending[J]. Acta Materiae Compositae Sinica, 2023, 40(3): 1468-1476(in Chinese).
[15]	SELVAKUMARAN L, LONG Q, PRUDHOMME S, et al. On the detectability of transverse cracks in laminated composites using electrical potential change measurements[J]. Composite Structures, 2015, 121: 237-246.
[16]	BOUSSU F, CRISTIAN I, NAUMAN S. General definition of 3D warp interlock fabric architecture[J]. Composites Part B: Engineering, 2015, 81: 171-188.
[17]	YU H, SUN J, HEIDER D, et al. Experimental investigation of through-thickness resistivity of unidirectional carbon fiber tows[J]. Journal of Composite Materials, 2019, 53: 2993-3003.
[18]	JIANG M, CONG X, YI X, et al. A stochastic overlap network model of electrical properties for conductive weft yarn composites and their experimental study[J]. Composites Science and Technology, 2022, 217: 109075.
[19]	WINTIBA B, VASIUKOV D, PANIER S, et al. Automated reconstruction and conformal discretization of 3D woven composite CT scans with local fiber volume fraction control[J]. Composite Structures, 2020, 248: 112438.
[20]	ISAAC-MEDINA B, ALONZO-GARCÍA A, AVILÉS F. Electrical self-sensing of impact damage in multiscale hierarchical composites with tailored location of carbon nanotube networks[J]. Structural Health Monitoring, 2019, 18: 806-818.
[21]	YU H, HEIDER D, ADVANI S. A 3D microstructure based resistor network model for the electrical resistivity of unidirectional carbon composites[J]. Composite Structures, 2015, 134: 740-749.
[22]	LOUIS M, JOSHI S P, BROCKMANN W. An experimental investigation of through-thickness electrical resistivity of CFRP laminates[J]. Composites Science and Technology, 2001, 61: 911-919.
[23]	HAN C, SUN B, GU B. Electric conductivity and surface potential distributions in carbon fiber reinforced composites with different ply orientations[J]. Textile Research Journal, 2022, 92: 1147-1160.
[24]	ABRY J C, BOCHARD S, CHATEAUMINOIS A, et al. In situ detection of damage in CFRP laminates by electrical resistance measurements[J]. Composites Science and Technology, 1999, 59: 925-935.
[25]	TODOROKI A, TANAKA M, SHIMAMURA Y. Measurement of orthotropic electric conductance of CFRP laminates and analysis of the effect on delamination monitoring with an electric resistance change method[J]. Composites Science and Technology, 2002, 62: 619-628.
[26]	WASSELYNCK G, TRICHET D, FOULADGAR J. Determination of the electrical conductivity tensor of a CFRP composite using a 3-D percolation model[J]. IEEE Transactions on Magnetics, 2013, 49: 1825-1828.
[27]	KANE B, PIERQUIN A, WASSELYNCK G, et al. Coupled numerical and experimental identification of geometrical parameters for predicting the electrical conductivity of CFRP layers[J]. IEEE Transactions on Magnetics, 2020, 56: 6701604.
[28]	XIAO J, LI Y, FAN W X. A laminate theory of piezoresistance for composite laminates[J]. Composites Science and Technology, 1999, 59: 1369-1373.
[29]	TODOROKI A. Electric current analysis for thick laminated CFRP composites[J]. Transactions of the Japan Society for Aeronautical and Space Sciences, 2012, 55: 237-243.
[30]	YAMANE T, TODOROKI A. Analysis of electric current density in carbon fiber reinforced plastic laminated plates with angled plies[J]. Composite Structures, 2017, 166: 268-276.
[31]	ATHANASOPOULOS N, KOSTOPOULOS V. A comprehensive study on the equivalent electrical conductivity tensor validity for thin multidirectional carbon fibre reinforced plastics[J]. Composites Part B: Engineering, 2014, 67: 244-255.
[32]	BENSAID S, TRICHET D, FOULADGAR J. Electrical conductivity identification of composite materials using a 3-D anisotropic shell element model[J]. IEEE Transactions on Magnetics, 2009, 45: 1859-1862.
[33]	CHENG J, JI H, TAKAGI T, et al. Role of interlaminar interface on bulk conductivity and electrical anisotropy of CFRP Laminates measured by eddy current method[J]. NDT & E International, 2014, 68: 1-12.
[34]	HAIDER M F, HAIDER M M, YASMEEN F. Micromechanics model for predicting anisotropic electrical conductivity of carbon fiber composite materials[J]. AIP Conference Proceedings, 2016, 1754: 030011.
[35]	VILČÁKOVÁ J, SÁHA P, QUADRAT O. Electrical conductivity of carbon fibres/polyester resin composites in the percolation threshold region[J]. European Polymer Journal, 2002, 38: 2343-2347.
[36]	AHARONI S M. Electrical resistivity of a composite of conducting particles in an insulating matrix[J]. Journal of Applied Physics, 1972, 43: 2463-2465.
[37]	WEBER M, KAMAL M R. Estimation of the volume resistivity of electrically conductive composites[J]. Polymer Composites, 1997, 18: 711-725.
[38]	TAYA M, UEDA N. Prediction of the in-plane electrical conductivity of a misoriented short fiber composite: Fiber percolation model versus effective medium theory[J]. Journal of Engineering Materials and Technology-Transactions of the Asme, 1987, 109: 252-256.
[39]	BERGER M A, MCCULLOUGH R L. Characterization and analysis of the electrical properties of a metal-filled polymer[J]. Composites Science and Technology, 1985, 22: 81-106.
[40]	SENGHOR F, WASSELYNCK G, HUU KIEN B, et al. Electrical conductivity tensor modeling of stratified woven-fabric carbon fiber reinforced polymer composite materials[J]. IEEE Transactions on Magnetics, 2017, 53: 9401604.
[41]	BALTOPOULOS A, POLYDORIDES N, PAMBAGUIAN L, et al. Damage identification in carbon fiber reinforced polymer plates using electrical resistance tomography mapping[J]. Journal of Composite Materials, 2013, 47: 3285-3301.
[42]	HAN C, SUN B, GU B. Electric potential distributions in carbon fiber/epoxy plain-woven laminates with different current directions[J]. Composite Structures, 2021, 270: 114059.
[43]	石荣荣, 武宝林. 三维编织碳纤维复合材料电阻率的估算[J]. 复合材料学报, 2016, 33(12): 2775-2780. SHI R R, WU B L. Resistivity estimation of 3D braided carbon fiber composites[J]. Acta Materiae Compositae Sinica, 2016, 33(12): 2775-2780(in Chinese).
[44]	WANG D, CHUNG D D L. Comparative evaluation of the electrical configurations for the two-dimensional electric potential method of damage monitoring in carbon fiber polymer-matrix composite[J]. Smart Materials and Structures, 2006, 15: 1332-1344.
[45]	ANGELIDIS N, KHEMIRI N, IRVING P E. Experimental and finite element study of the electrical potential technique for damage detection in CFRP laminates[J]. Smart Materials and Structures, 2004, 14: 147-154.
[46]	ANGELIDIS N, IRVING P E. Detection of impact damage in CFRP laminates by means of electrical potential techniques[J]. Composites Science and Technology, 2007, 67: 594-604.
[47]	ATHANASOPOULOS N, KOSTOPOULOS V. Resistive heating of multidirectional and unidirectional dry carbon fibre preforms[J]. Composites Science and Technology, 2012, 72: 1273-1282.
[48]	BUSCH R, RIES G, WERTHNER H, et al. New aspects of the mixed state from six-terminal measurements on Bi₂Sr₂CaCu₂O_x single crystals[J]. Physical Review Letters, 1992, 69: 522-525.
[49]	PARK J B, OKABE T, TAKEDA N. New concept for modeling the electromechanical behavior of unidirectional carbon-fiber-reinforced plastic under tensile loading[J]. Smart Materials and Structures, 2003, 12: 105-114.
[50]	TODOROKI A. Electric current analysis of CFRP using perfect fluid potential flow[J]. Journal of The Japan Society for Aeronautical and Space Sciences, 2012, 55: 183-190.
[51]	YAMANE T, TODOROKI A. Electric potential function of oblique current in laminated carbon fiber reinforced polymer composite beam[J]. Composite Structures, 2016, 148: 74-84.
[52]	YAMANE T, TODOROKI A, FUJITA H, et al. Electric current distribution of carbon fiber reinforced polymer beam: Analysis and experimental measurements[J]. Advanced Composite Materials, 2016, 25: 497-513.
[53]	LEE I Y, ROH H D, PARK Y B. Novel structural health monitoring method for CFRPs using electrical resistance based probabilistic sensing cloud[J]. Composites Science and Technology, 2021 ,213: 108812.
[54]	KIKUNAGA K, TERASAKI N. Characterization of electrical conductivity of carbon fiber reinforced plastic using surface potential distribution[J]. Japanese Journal of Applied Physics, 2018, 57: 04 FC02.
[55]	GAIER J R, YODERVANDENBERG Y, BERKEBILE S, et al. The electrical and thermal conductivity of woven pristine and intercalated graphite fiber-polymer composites[J]. Carbon, 2003, 41: 2187-2193.
[56]	韩朝锋. 碳纤维复合材料电导率、电势分布及力电耦合行为[D]. 上海: 东华大学, 2022. HAN C F. Electrical conductivity, potential distributions and electromechanical coupling behavior of carbon fiber composites[D]. Shanghai: Donghua University, 2022(in Chinese).
[57]	CHUNG D D L. A critical review of piezoresistivity and its application in electrical-resistance-based strain sensing[J]. Journal of Materials Science, 2020, 55: 15367-15396.
[58]	WANG S, CHUNG D, CHUNG J. Self-sensing of damage in carbon fiber polymer-matrix composite by measurement of the electrical resistance or potential away from the damaged region[J]. Journal of Materials Science, 2005, 40: 6463-6472.
[59]	WANG S, CHUNG D D L, CHUNG J H. Impact damage of carbon fiber polymer–matrix composites, studied by electrical resistance measurement[J]. Composites Part A: Applied Science and Manufacturing, 2005, 36: 1707-1715.
[60]	WANG S, CHUNG D D L, CHUNG J H. Self-sensing of damage in carbon fiber polymer-matrix composite cylinder by electrical resistance measurement[J]. Journal of Intelligent Material Systems and Structures, 2006, 17: 57-62.
[61]	LEONG C K, WANG S, CHUNG D D L. Effect of through-thickness compression on the microstructure of carbon fiber polymer-matrix composites, as studied by electrical resistance measurement[J]. Journal of Materials Science, 2006, 41: 2877-2884.
[62]	WANG D, WANG S, CHUNG D D L, et al. Comparison of the electrical resistance and potential techniques for the self-sensing of damage in carbon fiber polymer-matrix composites[J]. Journal of Intelligent Material Systems and Structures, 2006, 17: 853-861.
[63]	WANG D, CHUNG D D L. Through-thickness stress sensing of a carbon fiber polymer-matrix composite by electrical resistance measurement[J]. Smart Materials and Structures, 2007, 16: 1320-1330.
[64]	ANGELIDIS N, WEI C Y, IRVING P E. The electrical resistance response of continuous carbon fibre composite laminates to mechanical strain[J]. Composites Part A: Applied Science and Manufacturing, 2004, 35: 1135-1147.
[65]	WEN S, CHUNG D D L. Electrical-resistance-based damage self-sensing in carbon fiber reinforced cement[J]. Carbon, 2007, 45: 710-716.
[66]	KWON D J, SHIN P S, KIM J H, et al. Detection of damage in cylindrical parts of carbon fiber/epoxy composites using electrical resistance (ER) measurements[J]. Composites Part B: Engineering, 2016, 99: 528-532.
[67]	PARK JB, OKABE T, TAKEDA N, et al. Electromechanical modeling of unidirectional CFRP composites under tensile loading condition[J]. Composites Part A: Applied Science and Manufacturing, 2002, 33: 267-275.
[68]	XIA Z, OKABE T, PARK J B, et al. Quantitative damage detection in CFRP composites: Coupled mechanical and electrical models[J]. Composites Science and Technology, 2003, 63: 1411-1422.
[69]	OGI K, TAKAO Y. Characterization of piezoresistance behavior in a CFRP unidirectional laminate[J]. Composites Science and Technology, 2005, 65: 231-239.
[70]	ZHU S, CHUNG D D L. Analytical model of piezoresistivity for strain sensing in carbon fiber polymer-matrix structural composite under flexure[J]. Carbon, 2007, 45: 1606-1613.
[71]	SEVKAT E, LI J, LIAW B, et al. A statistical model of electrical resistance of carbon fiber reinforced composites under tensile loading[J]. Composites Science and Technology, 2008, 68: 2214-2219.
[72]	WANG D, CHUNG D D L. Through-thickness piezoresistivity in a carbon fiber polymer-matrix structural composite for electrical-resistance-based through-thickness strain sensing[J]. Carbon, 2013, 60: 129-138.
[73]	NISHIO Y, TODOROKI A, MIZUTANI Y, et al. Piezoresistive effect of plain-weave CFRP fabric subjected to cyclic loading[J]. Advanced Composite Materials, 2017, 26: 229-243.
[74]	ROH H D, LEE S Y, JO E, et al. Deformation and interlaminar crack propagation sensing in carbon fiber composites using electrical resistance measurement[J]. Composite Structures, 2019, 216: 142-150.
[75]	ALSAADI A, MEREDITH J, SWAIT T, et al. Structural health monitoring for woven fabric CFRP laminates[J]. Composites Part B: Engineering, 2019, 174: 107048.
[76]	WAN Z K, WANG Z G. Electrical characteristics of composite material under loading based on SQUID technology[J]. Journal of Textile Research, 2010, 31: 58-63.
[77]	CHENG X, ZHOU H, WU Z, et al. An investigation into self-sensing property of hat-shaped 3D orthogonal woven composite under bending test[J]. Journal of Reinforced Plastics and Composites, 2019, 38: 149-166.

施引文献(2)

期刊类型引用(1)

李新娅，王宁，卢佳浩，张鹏，夏兆鹏，侯耒. 基于改进Weibull模型的高强缝合锚钉缝线强度预测. 现代纺织技术. 2024(06): 52-60 .

百度学术

其他类型引用(1)

资源附件(0)

长摘要

目的
碳纤维及其复合材料作为国家战略新兴材料，因其轻质高强、高冲击损伤容限和多重增强结构混杂制造等特性，广泛应用在航空航天、远洋船舶、轨道交通和新能源汽车等高精尖技术领域。碳纤维复合材料除优异的损伤容限和热传导性能以外，突出的电导特性和压阻效应等多功能属性近年来逐步受到重视。碳纤维复合材料在外部承载下结构变形诱发电阻发生相应变化，本身可作为智能传感器件记录损伤演变过程，在实现结构材料承载的同时发挥功能材料自我感应的作用，在结构健康监测应用中具有巨大的潜在价值。因此研究和分析碳纤维树脂基复合材料不同维度增强体的电导特性与结构效应，揭示碳纤维复合材料的导电机理和力电耦合行为，对实现碳纤维复合材料在结构健康监测中的导电结构优化和高效配置，拓展碳纤维复合材料的多功能用途，具有重要的经济效益和应用价值。
方法
电阻法利用碳纤维树脂基复合材料自身的压阻效应实现自我智能监测，无需依赖复杂的监测仪器和数据处理系统便可实现材料自身健康状态的原位监测，从而为复合材料损伤起始和积累过程提供精确实时的电信号信息。在复合材料增强体结构中，碳纤维纱线无序接触、穿插交织和屈曲变形，导致内部的电流传导路径随机分布，在复合材料中形成复杂的导电路径，从而对复合材料的电导性能产生重要影响。同时碳纤维复合材料的非均质特性，造成内部导电机理尚不清楚。本文从复合材料电阻法结构健康监测应用出发，着重概述了不同维度增强体碳纤维树脂基复合材料的导电性能和预测模型、表面电势场分布和主要测量技术，以及力电耦合行为和本构模型，以期为碳纤维复合材料“材料-结构-功能”一体化集成系统的设计提供新方向和新途径。
结果
目前复合材料电导率的研究对象主要集中在传统层压复合材料，忽略铺层角度和层间界面电导率对导电性能的影响；对于三维增强结构复合材料的电导率仅限于实验测试，并未揭示复合材料内部的导电机理以及建立有效的导电模型。复合材料电势分布的研究主要局限于薄层复合材料层压板的表面，并未考虑电势沿厚度方向衰减的影响；而且对三维增强结构复合材料的电势分布研究较少，缺少空间电势分布的有效预测模型。现有对复合材料力电耦合行为的研究多处于实验分析和唯象描述，而且已建立的力电耦合解析模型只适用于单向复合材料的预测，对分析预测不同维度增强结构复合材料的力电耦合行为适用性较差，缺少系统地分析。
结论
当前国内外对碳纤维树脂基复合材料的电导率、电势场分布和力电耦合行为的研究还处于探索阶段，缺乏行之有效的预测模型分析碳纤维复合材料内部的导电机理。尤其是在三维增强结构复合材料中，纱线相互交织成为一个整体，而且内部的纱线截面形态和路径变化更加复杂，研究难度进一步增加。因此下一阶段的主要任务和努力方向包括：(1)深入研究不同增强结构碳纤维树脂基复合材料的电导率、电势场分布和力电耦合行为，系统揭示复合材料导电机理的结构效应和传导路径差异；(2)结合电阻抗成像技术、电网络理论和机器学习等新技术，为研究三维增强结构碳纤维树脂基复合材料的电导特性和力电耦合行为提供新思路和新方案；(3)在构建碳纤维树脂基复合材料力电耦合本构模型时，应准确表征碳纤维复合材料在加载条件下电阻响应和刚度退化之间的定量关系，明晰导电网络的变形、破坏和重组等变化过程，最终实现碳纤维复合材料导电结构的优化设计和智能监测的有效利用。

创新点

碳纤维及其复合材料作为国家战略新兴材料，因其轻质高强、高冲击损伤容限和多重增强结构混杂制造等特性，广泛应用在航空航天、远洋船舶、轨道交通和新能源汽车等高精尖技术领域。碳纤维复合材料除优异的损伤容限和热传导性能以外，突出的电导特性和压阻效应等多功能属性近年来逐步受到重视。碳纤维复合材料在外部承载下结构变形诱发电阻发生相应变化，本身可作为智能传感器件记录损伤演变过程，在实现结构材料承载的同时发挥功能材料自我感应的作用，在结构健康监测(Structural health monitoring，SHM)应用中具有巨大的潜在价值。
本文从复合材料电阻法结构健康监测应用出发，着重概述了不同维度增强体碳纤维树脂基复合材料的导电性能和预测模型、表面电势场分布和主要测量技术，以及力电耦合行为和本构模型，以期为碳纤维复合材料“材料-结构-功能”一体化集成系统的设计提供新方向和新途径。针对现有研究现状，指出下一阶段的主要任务和努力方向包括：(1)深入研究不同增强结构碳纤维树脂基复合材料的电导率、电势场分布和力电耦合行为，系统揭示复合材料导电机理的结构效应和传导路径差异；(2)结合电阻抗成像技术、电网络理论和机器学习等新技术，为研究三维增强结构碳纤维树脂基复合材料的电导特性和力电耦合行为提供新思路和新方案；(3)在构建碳纤维树脂基复合材料力电耦合本构模型时，应准确表征碳纤维复合材料在加载条件下电阻响应和刚度退化之间的定量关系，明晰导电网络的变形、破坏和重组等变化过程，最终实现碳纤维复合材料导电结构的优化设计和智能监测的有效利用。
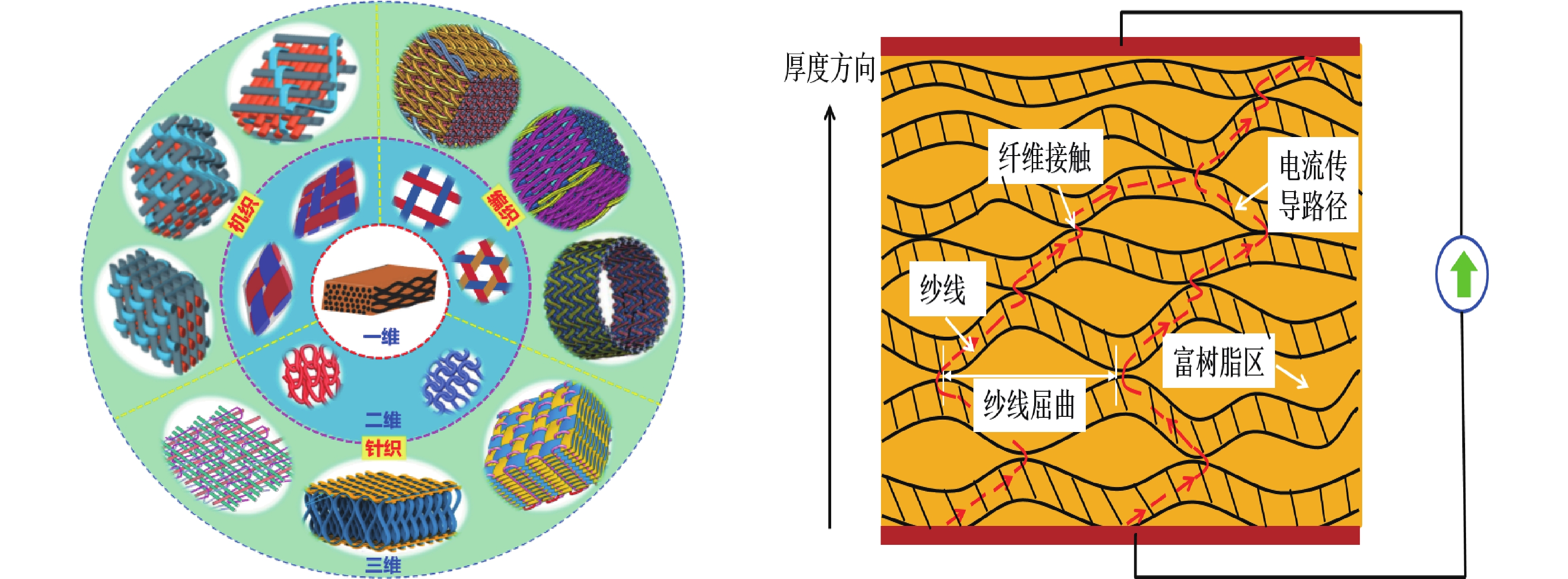
碳纤维复合材料(a)不同维度增强体结构和(b)内部电流传导路径示意图