基于相场法的复合材料失效分析研究进展

彭帆; 马玉娥; 黄玮; 陈鹏程; 马维力

doi:10.13801/j.cnki.fhclxb.20220818.001

基于相场法的复合材料失效分析研究进展

1.
长安大学　理学院，西安 710064
2.
西北工业大学　航空学院，西安 710072

基金项目: 国家自然科学基金(91860128)

详细信息

通讯作者:
马玉娥，博士，教授，博士生导师，研究方向为复合材料结构力学　E-mail: ma.yu.e@nwpu.edu.cn

中图分类号: TB332
计量
- 文章访问数: 1765
- HTML全文浏览量: 859
- PDF下载量: 278
出版历程
- 收稿日期: 2022-06-21
- 修回日期: 2022-07-22
- 录用日期: 2022-08-03
- 网络出版日期: 2022-08-17
- 刊出日期: 2023-05-14

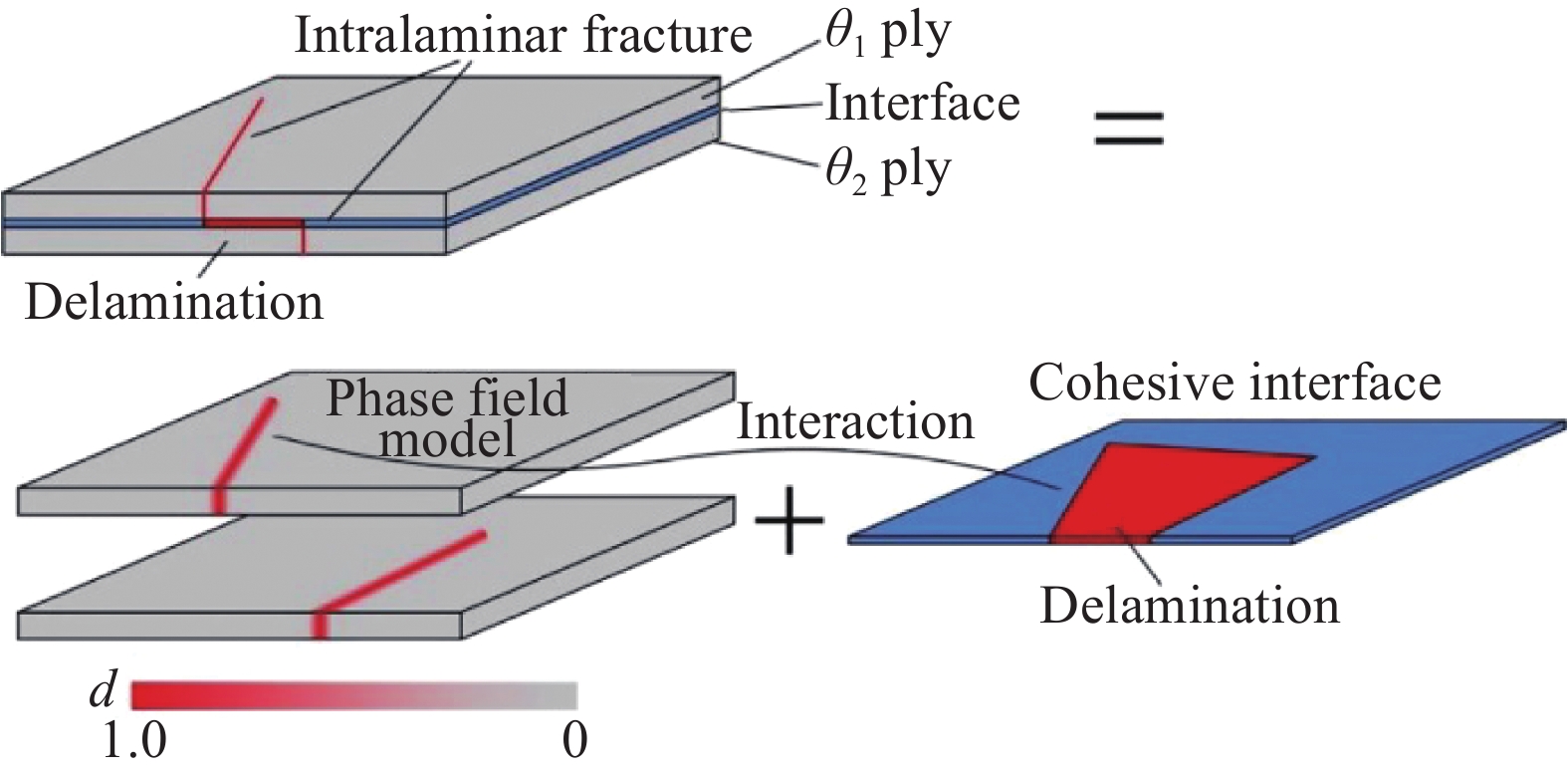
Failure analysis of composite materials based on phase field method: A review

1.
College of Science, Chang'an University, Xi'an 710064, China
2.
School of Aeronautics, Northwestern Polytechnical University, Xi'an 710072, China

Funds: National Natural Science Foundation of China (91860128)

摘要

摘要: 预测复合材料的失效行为，对复合材料结构设计具有重要意义。由于其失效模式和失效机制较复杂，传统的计算断裂力学方法和基于损伤力学的数值方法在对其进行失效分析存在一定困难。相场法结合了断裂力学和损伤力学的优点，无需额外的判据便可精确捕捉裂纹的萌生、扩展和扭结行为，近年来被广泛地应用于复合材料的失效分析。本文首先简要介绍相场法的基本理论，给出了基本的断裂能模型和控制方程。然后着重介绍了基于相场法的复合材料失效分析的研究进展，梳理了相场法在复合材料领域的应用范围。最后，对相场法模拟复合材料在疲劳、疲劳湿热环境下和冲击下的损伤进行了展望。
- 相场法 /
- 复合材料 /
- 失效分析 /
- 内聚力模型 /
- 分层
Abstract: Predicting the failure behavior of composite materials is of great significance to the design of composite structures. Due to the complexity of its failure mode and failure mechanism, the traditional computational fracture mechanics method and the numerical method based on damage mechanics are difficulty to model modeling its failure behavior. The phase field method combines the advantages of fracture mechanics and damage mechanics. It can accurately capture the crack initiation, propagation and kink behavior without additional criteria. Recently, it has been widely used in the failure analysis of composite materials. In this paper, the basic theory of phase field method was briefly introduced, and the fundamental fracture energy model and governing equations were given. Following that, the review focused on the research progress of composite failure analysis based on phase field method. The application ranges of phase field method on composite material field were reviewed. Finally, the damage simulations of composites under fatigue, hygrothermal environment and impact by using phase field method were discussed.
- phase field method /
- composite material /
- failure analysis /
- cohesive zone model /
- delamination

HTML全文

近年来，随着经济的快速发展，工业化污染严重导致各类细菌滋生和传播，由细菌感染引发的伤口感染、人体炎症、伤寒等症状一直威胁着人类健康^[1]。致病性细菌感染可通过飞沫、接触及气溶胶进行快速传播，存在很大的感染风险。目前，由革兰氏菌引起的传染病是全球几大健康问题之一^[2-3]，但使用抗生素治疗细菌感染存在诸多不足，例如，细菌耐药性及多药外排泵作用等^[4-5]。为克服细菌耐药性等缺点，多种新型抑菌材料不断被开发出来，但多数材料的抑菌效果不佳^[6]，某些材料还需要复杂的表面改性才能实现抑菌功能^[7-8]，因此，开发高性能的抑菌材料并研究其抑菌机制，解决细菌耐药性具有重要的应用价值。通过研究发现，金属有机骨架材料与细菌之间可通过范德华力、静电相互作用导致细菌死亡，也不会使细菌基因发生突变而产生耐药性，表现出良好的抑菌性能^[9]。

金属-有机骨架(Metal-organic framework，MOF)是由金属离子或离子簇与有机配体配位组成的多孔纳米材料^[10]，在吸附分离^[11]、气体储存^[12]、药物分子输送^[13]和化学催化^[14]等方面备受青睐。通过有机配体与作为节点的金属离子或离子簇配位，MOF材料可以制备具有预期的结构，并实现其结构的功能化，同时也因其缓慢的释放能力、高比表面积和大孔隙率等优势逐渐成为一种新的载体^[15]。近年来，基于MOF结构为载体的材料已经成为抑菌应用的一个热点^[16-19]。UiO-66-NH₂是以Zr₆O₄(OH)₄簇为金属节点，2-氨基对苯二甲酸为配体，形成的具有三维立体骨架八面体结构的材料^[20-23]。UiO-66-NH₂材料不仅具有低毒性、高耐水性和良好的结构稳定性^[24]，而且结构中的氨基可以作为反应位点进一步功能化^[25]，因此可以将UiO-66-NH₂材料作为理想的载体，进一步功能化改性后，用于抗菌方面的研究。

基于此，本文通过水热法合成了锆基MOF材料UiO-66-NH₂，将多孔UiO-66-NH₂作为可再生载体，以亚氯酸钠为活性氯来源，通过后合成改性的方法，使活性氯原子与骨架中的氨基官能团形成一种氯胺基团，合成了UiO-66-NHCl新型复合抑菌材料，采用XRD、FTIR、SEM、TEM、EDS和XPS等手段对UiO-66-NHCl复合材料进行表征，同时探索了不同负载工艺对氯负载量的影响，并研究了UiO-66-NHCl复合材料的抑菌性能。结果显示，UiO-66-NHCl对革兰氏阳性菌和革兰氏阴性菌的广谱灭活具有快速而有效的作用，在抑菌方面表现出优异的性能。

1. 实验材料及方法

1.1 原材料

四氯化锆(ZrCl₄)、2-氨基-1, 4-苯二甲酸(NH₂-BDC)、亚氯酸钠，上海麦克林生化科技有限公司；碘化钾，天津市科密欧化学试剂有限公司；硫代硫酸钠、淀粉、N, N-二甲基甲酰胺(DMF)、无水甲醇，天津市永大化学试剂有限公司；重铬酸钾，莱阳市康德化工有限公司；大肠杆菌、金黄色葡萄球菌，北京生物保藏生物科技有限公司；蛋白胨、牛肉膏、琼脂粉，天津市英博生化试剂有限公司。

1.2 实验方法

1.2.1 UiO-66-NH₂的合成

将ZrCl₄ (4.5 mmol)和NH₂-BDC (6.4 mmol)溶于DMF (40 mL)，超声搅拌20 min，随后将冰醋酸(HAc，0.3 mmol)加到上述悬浮液，继续超声搅拌20 min。将搅拌后的悬浮液转移到不锈钢高压釜(100 mL)，在135℃下反应24 h。将产物冷却至室温，再用DMF和无水甲醇洗涤离心数次，最后将产物在80℃真空干燥8 h。

1.2.2 UiO-66-NHCl的合成

将一定质量的UiO-66-NH₂材料，置于亚氯酸钠溶液(pH=5)中，避光搅拌4 h，抽滤，收集固体产物，产物用无水乙醇洗涤3次，以去除氯化后材料表面的杂质，产物在40℃下真空干燥12 h，得到UiO-66-NHCl复合材料。在保证其他条件不变的情况下，采用单一因素法设置不同氯负载比例(质量比m(UiO-66-NH₂)∶m(NaClO₂))和氯化时间(0.5 h、2 h、4 h、6 h)，浸渍抽滤后，滤液用碘量滴定法测定有效氯的含量。氯负载量的计算公式如下所示：

$\mathrm{氯}\mathrm{负}\mathrm{载}\mathrm{量}=\frac{{m}_{0}-{m}_{{\rm{f}}}}{{m}_{{\rm{ads}}}}\times 100\%$

(1)

式中：m₀为初始亚氯酸钠的质量(g)；m_f为上清液中亚氯酸钠的质量(g)；m_ads为UiO-66-NH₂的质量(g)。

1.2.3 碘钾氧化实验

采用亚氯酸钠溶液浸渍改性合成了UiO-66-NHCl新型复合抑菌材料，通过碘钾氧化实验验证UiO-66-NHCl复合材料中活性氯的存在，用滴定法加入硫代硫酸钠溶液滴定来计算氯的含量。有效氯含量计算公式如下所示：

$\mathrm{C}\mathrm{l}\left(\mathrm{\%}\right)=\frac{{C V\times 35.45\times 100}}{{W}} \times 2$

(2)

式中：C为硫代硫酸钠滴定液浓度(0.01 mol/L)；V为消耗硫代硫酸钠滴定液体积(mL)；W为MOF复合材料的质量(g)。

1.2.4 UiO-66-NHCl的稳定性能

在储存过程中，氯的释放会降低材料的杀菌效果，因此探究UiO-66-NHCl复合材料在高温、高湿和强光等条件下密封储存30天后的稳定性，通过碘量滴定法测UiO-66-NHCl复合材料中活性氯的含量，通过抑菌实验直观表现UiO-66-NHCl的杀菌效果。

高温实验是设定条件温度为60℃，每5 天取样，测量30天内UiO-66-NHCl材料中活性氯的含量。高湿和强光实验分别设定条件温度为25℃，湿度为75%；光照强度为4500 lx，其他步骤同上。

1.2.5 UiO-66-NHCl的抑菌性能

根据《消毒技术规范》(2002年版)^[26]配制Luria-Bertani (LB)液体培养基、LB固体培养基和相应的菌悬液。采用抑菌圈法对UiO-66-NHCl的抑菌性能进行评价。配制不同浓度的UiO-66-NHCl复合材料，将菌悬液均匀涂布在含有培养基的平板上。用移液枪吸取20 μL不同浓度的样品，滴加到灭菌后滤纸片的表面，对照滤纸片滴加20 μL浓度为0.9%的生理盐水，将平板倒置在37℃电热恒温培养箱(DPH 9402，上海一恒科学仪器有限公司)培养12~16 h后，观察结果，记录抑菌圈直径的大小。

1.2.6 皮肤刺激性实验

根据《消毒技术规范》(2002年版)^[26]对选用的新西兰兔进行完整和破损皮肤刺激性实验，采用同体左右侧自身对比法，即每只新西兰兔左侧去毛区域涂抹生理盐水做对照，右侧去毛区域涂抹UiO-66-NHCl抑菌材料。给药4 h后清洁，每天对新西兰兔给药1次，连续给药7天。观察新西兰兔是否正常，并记录是否出现红斑和水肿情况。表1为皮肤刺激性反应评分标准。

表 1 皮肤刺激性反应评分标准

Table 1. Skin irritation response scoring criteria

Erythema	Score	Edema	Score
No	0	No	0
Mildly (barely visible)	1	Mildly (barely visible)	1
Moderately (clearly visible)	2	Moderately (visible bulge)	2
Severely	3	Severely (skin augmentation of 1 mm, clear contours)	3

下载: 导出CSV

| 显示表格

2. 结果与讨论

2.1 UiO-66-NHCl复合材料的制备及优化

采用浸渍键合的方法合成UiO-66-NHCl材料，探究不同的氯负载比例与氯化时间对UiO-66-NHCl材料合成的影响，并计算氯负载量。如表2所示，在氯负载比例为1∶5时，氯负载量最高，氯负载比例为1∶9时次之。图1(a)为不同氯负载比例的UiO-66-NHCl的XRD图谱(D/MAX-2500 型X 射线衍射仪，日本Rigaku公司)，可知，1-3组材料的XRD图谱与模拟的UiO-66-NH₂的XRD图谱基本一致，说明UiO-66-NHCl的晶体结构并未发生改变，而当氯负载比例为1∶9 (4组)时，其XRD图谱中并未出现材料的特征峰，表明材料未成功合成。选取氯负载比例为1∶5的条件下对氯化时间进行探究，当氯化时间为4 h时，材料的氯负载量最高，且随着氯化时间的增加，材料的结晶度下降，如图1(b)所示。

表 2 活性氯负载比例与氯化时间对氯负载量的影响

Table 2. Effect of active chlorine loading ratio and chlorination time on chlorine loading

No.	Chlorine load ratio	Chlorination time/h	Chlorine loading/wt%
1	1∶3	4	6.65
2	1∶5	4	9.35
3	1∶7	4	7.12
4	1∶9	4	9.04
5	1∶5	0.5	4.85
6	1∶5	2	8.40
7	1∶5	4	9.11
8	1∶5	6	8.54

下载: 导出CSV

| 显示表格

采用S-4800-I 型场发射扫描电子显微镜(日本HITACHI 公司)对不同氯负载比例和氯化时间所制备材料进行形貌分析，其SEM图像如图2所示，氯负载比例在1∶7范围内，材料均表现为均匀的颗粒，呈现出八面体结构，氯负载比例在1∶9时，材料发生团聚，结晶度低，此时八面体结构已被破坏，这点可以从图1(a)样品的XRD图谱中看出；而随着氯化时间的增加，材料逐渐开始团聚，但颗粒形态仍保持八面体结构。

图 1 UiO-66-NH₂和UiO-66-NHCl的XRD图谱：(a) 不同氯负载比例；(b) 不同氯化时间

Figure 1. XRD patterns of UiO-66-NH₂和UiO-66-NHCl: (a) Different chlorine loading ratios; (b) Different chlorination time

下载: 全尺寸图片幻灯片

图 2 UiO-66-NH₂/NaClO₂的SEM图像：((a)~(d)) 负载比例分别为1∶3、1∶5、1∶7、1∶9；((e)~(h)) 氯化时间分别为0.5 h、2 h、4 h、6 h

Figure 2. SEM images of UiO-66-NH₂/NaClO₂: ((a)-(d)) Loading ratios of 1∶3, 1∶5, 1∶7, 1∶9; ((e)-(h)) Chlorination time of 0.5 h, 2 h, 4 h, 6 h, respectively

下载: 全尺寸图片幻灯片

2.2 UiO-66-NHCl复合材料的结构表征

2.2.1 碘钾氧化实验结果

通过碘量滴定法测定UiO-66-NHCl中有效氯的含量。从图3中(左)可以看出，未经氯化处理的 UiO-66-NH₂材料在加入碘化钾溶液后，溶液没有发生任何颜色变化，而将UiO-66-NH-Cl复合材料与碘化钾溶液混合后，清澈的无色溶液立即变成棕色，这是由于溶液中碘离子被活性氯胺(N—Cl)氧化，碘被释放出来，颜色变为棕色，证明所制备的UiO-66-NHCl复合材料中含有N—Cl结构。反应式如式(3)所示。

图 3 UiO-66-NH₂加KI溶液(左)；UiO-66-NHCl加KI溶液(中)；UiO-66-NHCl加KI溶液后滴定(右)

Figure 3. KI solution added to UiO-66-NH₂ (left); KI solution added to UiO-66-NHCl (middle); KI solution added to UiO-66-NHCl after titration (right)

下载: 全尺寸图片幻灯片

$\mathrm{N}-\mathrm{C}\mathrm{l}+2{\mathrm{I}}^-+{\mathrm{H}}^+\to \mathrm{N}-\mathrm{H}+{\mathrm{I}}_{2}+{\mathrm{C}\mathrm{l}}^-$

(3)

${\mathrm{I}}_{2}+2{\mathrm{S}}_{2}{\mathrm{O}}_{3}^{2-}\to {2\mathrm{I}}^-+{\mathrm{S}}_{4}{\mathrm{O}}_{6}^{2-}$

(4)

通过碘量滴定法测定UiO-66-NHCl中有效氯的含量，从图3中(右)可以看出，滴定硫代硫酸钠溶液后，颜色由棕色变为无色，这是由于所生成的碘单质与硫代硫酸根离子反应，再变为碘离子，反应式如式(4)所示。

2.2.2 晶相结构分析

图4为UiO-66-NH₂和UiO-66-NHCl的XRD图谱，可见，在2θ=7.4°、8.5°、25.7°等处出现的衍射峰，与模拟UiO-66-NH₂的出峰位置相吻合，表明成功合成了UiO-66-NH₂^[27]；同时，UiO-66-NHCl的衍射峰和UiO-66-NH₂的基本保持一致，表明UiO-66-NH₂的晶体结构在引入活性氯后未发生变化，结构保持较好。

图 4 UiO-66-NH₂和UiO-66-NHCl的XRD图谱

Figure 4. XRD patterns of UiO-66-NH₂ and UiO-66-NHCl

下载: 全尺寸图片幻灯片

采用FTS-65A1896型傅里叶红外光谱(美国铂金埃尔默公司)对UiO-66-NH₂和UiO-66-NHCl进行红外光谱分析，如图5所示。在660~680 cm⁻¹和460~480 cm⁻¹处分别为μ₃—O和μ₃—OH基团的拉伸振动^[28]，UiO-66-NH₂存在553 cm⁻¹处的峰对应Zr—(OC)的不对称拉伸振动，1610~1550 cm⁻¹和1420~1335 cm⁻¹分别对应有机配体中O—C—O的对称和不对称伸缩振动。此外，1255 cm⁻¹处表现为芳香胺特有的C—N键拉伸振动，1507 cm⁻¹处的峰是由H₂-BDC中C=C键振动引起的，而1661 cm⁻¹处的峰是由于材料孔内残余的DMF或丙酮的C=O键的拉伸振动。在3270 cm⁻¹和3309 cm⁻¹处为—NH₂官能团特有的伯胺基团的双吸收峰，表明UiO-66-NH₂材料成功合成^[29]。UiO-66-NHCl复合材料红外图谱显示，负载活性氯后，在3290 cm⁻¹处出现仲胺基团的单峰，原始材料的氨基官能团的双峰消失^[30]，表明UiO-66-NH₂材料氨基上的氢已成功被活性氯取代，成功制备出UiO-66-NHCl复合材料。

2.2.3 官能团分析

图 5 UiO-66-NH₂和UiO-66-NHCl的FTIR图谱

Figure 5. FTIR spectra of UiO-66-NH₂ and UiO-66-NHCl

下载: 全尺寸图片幻灯片

(1) 微观形貌分析

原始UiO-66-NH₂材料的SEM图像如图6(a)~6(c)所示，可以看出所合成的UiO-66-NH₂呈规则的八面体结构，这与文献[31]中所制备材料的形貌相符，表明材料成功合成，且UiO-66-NH₂材料粒径大小较均一，粒径大约为300 nm。而通过活性氯改性的UiO-66-NHCl材料在负载活性氯后，材料的形貌没有发生太大变化，说明经活性氯改性后，不会影响UiO-66-NH₂的结构(图6(d)~6(f))。采用JEM-2100型透射电子显微镜(德国电子有限公司)对UiO-66-NH₂和UiO-66-NHCl进行形貌分析，其TEM图像如图7所示，所制备的UiO-66-NH₂内部为实心结构，且在经活性氯改性后，UiO-66-NHCl材料的内部结构状态没有发生改变，表明氯的掺杂没有影响材料的晶体结构。

图 6 UiO-66-NH₂ ((a)~(c))和UiO-66-NHCl ((d)~(f))的SEM图像

Figure 6. SEM images of UiO-66-NH₂ ((a)-(c)) and UiO-66-NHCl ((d)-(f))

下载: 全尺寸图片幻灯片

图 7 UiO-66-NH₂ ((a)~(c))和UiO-66-NHCl ((d)~(f))的TEM图像

Figure 7. TEM images of UiO-66-NH₂ ((a)-(c)) and UiO-66-NHCl ((d)-(f))

下载: 全尺寸图片幻灯片

(2)元素含量分析

采用S-4800-I型X 射线能谱仪(日本HITACHI公司)对UiO-66-NH₂和 UiO-66-NHCl进行元素分析，如图8所示，原始UiO-66-NH₂材料含有C、N、O、Zr元素，表明材料已成功合成；经活性氯改性后，UiO-66-NHCl材料上有Cl元素的存在，进一步证明了活性氯已成功的被引入到UiO-66-NHCl材料中。

(3) 元素价态分析

图 8 UiO-66-NH₂和UiO-66-NHCl的EDS元素分析((a), (b))；UiO-66-NHCl的元素分布图像((c)~(f))

Figure 8. EDS elemental analysis of UiO-66-NH₂ and UiO-66-NHCl ((a), (b)); Elemental distribution images of UiO-66-NHCl ((c)-(f))

下载: 全尺寸图片幻灯片

通过X射线光电子能谱仪(ESCALAB 250Xi，赛默飞世尔科技有限公司)分析UiO-66-NHCl中各个元素的元素组成和化学状态。图9(a)为UiO-66-NH₂和UiO-66-NHCl的XPS总光谱对比图，可以看出，氯化后，在200 eV左右出现了明显的属于Cl元素的峰，399.8 eV处也出现了N元素的峰，表明Cl成功被引入到UiO-66-NH₂中，与C、O和Zr元素的峰相比，Cl元素的峰较弱，也说明Cl的含量明显低于其他元素，这与EDS结果一致。在C1s图谱中(图9(b))：284.1、285.5和288.04 eV处的峰分别对应于：C—C键、C—O键和OH—C=O键，这些键归属于H₂-BDC。O1图谱(图9(c))可分为3个峰：530.1 eV处的峰为Zr—O键，531.3 eV处的峰为表面活性氧物种C=O，而在532.6 eV处的峰可归因于H—O的化学吸附氧物种。如图9(d)所示，可以观察到Zr3d_5/2和Zr3d_3/2结合能的峰归属于Zr—O键，表明锆氧簇中Zr⁴⁺的存在^[32]。在N1s高分辨图谱中(图9(e))，398.2、399.8和400.8 eV处的3个峰分别对应于吡啶氮、吡咯氮和石墨氮^[33]。对Cl2p图谱(图9(f))分析，发现了Cl2p_3/2 (199.8 eV)和Cl2p_1/2(201.3 eV)的自旋-轨道分裂双态，这可能与N—Cl键的存在有很大的关系^[34]。

图 9 (a) UiO-66-NH₂和UiO-66-NHCl的XPS全谱图；(b) C1s；(c) O1s；(d) Zr3d；(e) N1s；(f) Cl2p

Figure 9. (a) Total XPS spectra of UiO-66-NH₂ and UiO-66-NHCl; (b) C1s; (c) O1s; (d) Zr3d; (e) N1s; (f) Cl2p

下载: 全尺寸图片幻灯片

2.3 UiO-66-NHCl的稳定性结果

由于储存过程中氯的释放会降低材料的抑菌效果，因此研究了UiO-66-NHCl复合材料在高温、高湿和强光等条件下的稳定性。如图10所示，在高温60℃、高湿75%和强光4500 lx的因素下，30天后，测定了材料所负载的活性氯含量分别为原始氯负载量的82%、89.3%和81.2%。因此，UiO-66-NHCl复合材料在高温、高湿和强光条件下仍能保持较好的稳定性。表3为UiO-66-NHCl复合材料对金黄色葡萄球菌和大肠杆菌的抑菌圈直径。图11和图12分别为UiO-66-NHCl复合材料对金黄色葡萄球菌和大肠杆菌的抑菌效果图。可以看出，随着稳定性实验天数的增加，抑菌圈直径虽在减小，但仍大于7 mm，表明在经过高温、高湿和强光实验后，材料仍能保持较好的抑菌效果。

图 10 UiO-66-NHCl在不同条件下的稳定性实验结果

Figure 10. Experimental results on the stability of UiO-66-NHCl under different conditions

下载: 全尺寸图片幻灯片

表 3 UiO-66-NHCl复合材料在高温、高湿和强光条件下的抑菌圈直径的影响(标准差，±SD mm)

Table 3. Effect of UiO-66-NHCl composites on the diameter of the inhibition circle under high temperature, high humidity and strong light conditions (Standard deviation, ±SD mm)

Strains	Factors	Diameter/mm
Strains	Factors	0 d	5 d	10 d	15 d	20 d	25 d	30 d
Staphylococcus aureus	High temperature	10.12±0.31	9.57±0.29	9.13±0.37	8.76±0.34	8.04±0.41	7.69±0.29	7.25±0.38
	High humidity	10.33±0.28	9.88±0.34	9.32±0.14	8.94±0.25	8.25±0.18	7.91±0.43	7.63±0.36
	Bright light	10.14±0.19	9.62±0.16	9.17±0.21	8.82±0.32	8.15±0.28	7.83±0.37	7.33±0.45
Escherichia coli	High temperature	10.07±0.27	9.61±0.37	9.05±0.35	8.62±0.28	8.01±0.32	7.52±0.47	7.16±0.39
	High humidity	10.21±0.24	9.82±0.29	9.14±0.36	8.77±0.35	8.19±0.27	7.74±0.42	7.33±0.32
	Bright light	10.11±0.32	9.67±0.26	9.09±0.42	8.53±0.29	8.08±0.36	7.67±0.51	7.25±0.43

下载: 导出CSV

| 显示表格

图 11 UiO-66-NHCl复合材料对金黄色葡萄球菌的抑菌效果

Figure 11. Bacterial inhibition effect of UiO-66-NHCl composite against Staphylococcus aureus

下载: 全尺寸图片幻灯片

图 12 UiO-66-NHCl复合材料对大肠杆菌的抑菌效果

Figure 12. Bacterial inhibition effect of UiO-66-NHCl composites on Escherichia coli

下载: 全尺寸图片幻灯片

2.4 UiO-66-NHCl的抑菌性能

图13和图14为不同浓度下UiO-66-NH₂材料改性前后对革兰氏阳性菌(金黄色葡萄球菌)和革兰氏阴性菌(大肠杆菌)的抑菌结果，表4为不同浓度下，UiO-66-NH₂和UiO-66-NHCl对金黄色葡萄球菌和大肠杆菌抑菌圈直径。可以看出，氯化后的UiO-66-NHCl复合材料对金黄色葡萄球菌和大肠杆菌均有抑制作用；随着样品浓度的增加，抑菌圈直径也随之增加，抑菌作用也随之增强。相比之下，未氯化的UiO-66-NH₂材料抑菌圈直径为0，没有表现出抑菌效果。

图 13 UiO-66-NH₂材料改性前后对金黄色葡萄球菌的抑菌结果影响

Figure 13. Effect of inhibition results of UiO-66-NH₂ material on Staphylococcus aureus before and after modification

下载: 全尺寸图片幻灯片

图 14 UiO-66-NH₂材料改性前后对大肠杆菌的抑菌结果影响

Figure 14. Effect of inhibition results of UiO-66-NH₂ material on Escherichia coli before and after modification

下载: 全尺寸图片幻灯片

表 4 浓度对UiO-66-NHCl和UiO-66-NH₂材料抑菌圈直径的影响对比(±SD mm)

Table 4. Comparison of the effect of concentration on the diameter of the inhibition circle of UiO-66-NHCl and UiO-66-NH₂

Strains	Sample	Diameter/mm
Strains	Sample	200 mg/L	300 mg/L	400 mg/L	500 mg/L	600 mg/L
Staphylococcus aureus	UiO-66-NHCl	7.88±0.48	8.55±0.55	8.96±0.32	9.58±0.32	10.03±0.41
Staphylococcus aureus	UiO-66-NH₂	0	0	0	0	0
Escherichia coli	UiO-66-NHCl	7.94±0.51	8.27±0.43	8.73±0.23	9.21±0.18	9.98±0.34
Escherichia coli	UiO-66-NH₂	0	0	0	0	0

下载: 导出CSV

| 显示表格

2.5 皮肤刺激性实验结果

表5为UiO-66-NHCl复合材料多次给药皮肤刺激反应实验结果。结果表明，在连续多次给药后，实验组和对照组的皮肤给药部位均未出现红斑、水肿等情况，两者平均反应均值均为0分，因此该材料表现为无刺激性。

表 5 UiO-66-NHCl多次给药皮肤刺激反应实验结果

Table 5. Experimental results of skin irritation response to multiple doses of UiO-66-NHCl

Dosing time/d	Complete skin group (Score)		Damaged skin group (Score)
Dosing time/d	UiO-66-NHCl	Water	UiO-66-NHCl	Water
1	0	0	0	0
2	0	0	0	0
3	0	0	0	0
4	0	0	0	0
5	0	0	0	0
6	0	0	0	0
7	0	0	0	0

下载: 导出CSV

| 显示表格

3. 结论

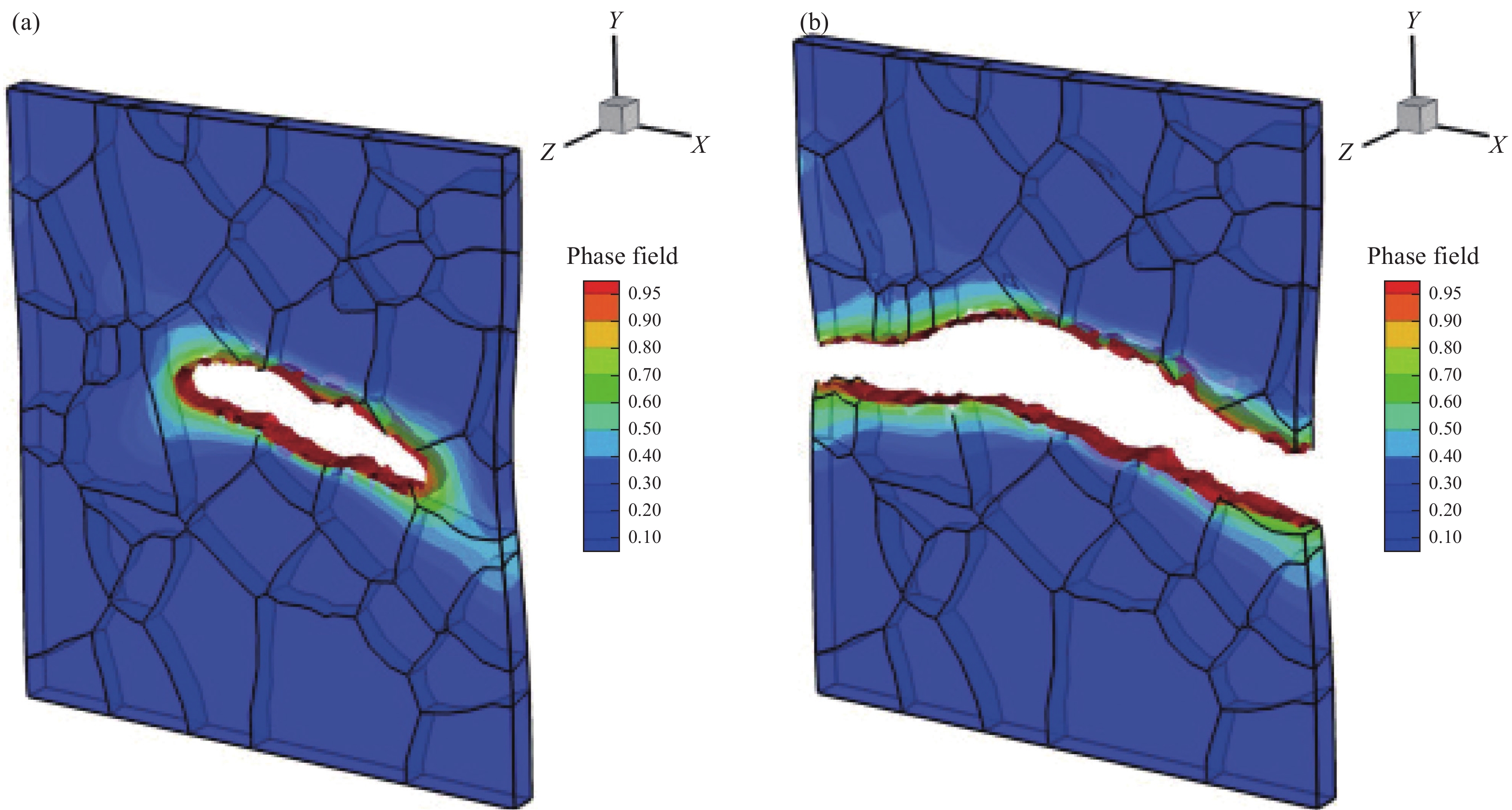
采用亚氯酸钠溶液对锆基金属-有机骨架材料UiO-66-NH₂进行后合成改性，通过碘钾氧化实验、XRD、SEM、TEM、EDS和XPS等表征分析结果可知，成功制备了UiO-66-NHCl复合抑菌材料，稳定性、抑菌性和皮肤刺激性实验结论如下：

(1) 在高温、高湿和强光条件下，UiO-66-NHCl的氯负载量分别为原始材料的82%、89.3%和81.2%，均能保持较好的稳定性；

(2) 抑菌实验表明，与未氯化的UiO-66-NH₂材料相比，氯化后的UiO-66-NHCl材料对大肠杆菌和金黄色葡萄球菌均有抑制作用，这是由于活性氯将位于UiO-66-NH₂材料上的氨基官能团转化为活性N—Cl结构所导致；

(3) 皮肤刺激性实验表明，氯化后的UiO-66-NHCl复合抑菌材料对皮肤无刺激性，可用于纺织纤维等防护服的应用。

图 1 断裂面的光滑过渡

l₀—Length; $\phi$ —Phase field variable

Figure 1. Smooth transition of fracture surface

下载: 全尺寸图片幻灯片

图 2 相场变量的分布函数

Figure 2. Distribution function of phase field variable

下载: 全尺寸图片幻灯片

图 3 铺层角α=30° (a), α=45° (b), α=60° (c)和α=90° (d)铺层复合材料层合板的数值结果(i)^[13]和试验结果(ii)^[14]

Figure 3. Numerically results (i)^[13] and experimentally (ii)^[14] obtained crack patterns for ply angle α=30° (a), α=45° (b), α=60° (c) and α=90° (d)

下载: 全尺寸图片幻灯片

图 4 含螺旋状纤维的圆柱形管在拉伸下的断裂行为^[18]： (a) 缠绕角θ=0°；(b) 缠绕角θ=60°

Figure 4. Fracture in a cylindrical tube with helix-type fiber structure under tension crack patterns for helix angles^[18]: (a) Wrap angle θ=0°; (b) Wrap angle θ=60°

下载: 全尺寸图片幻灯片

图 5 复合材料层合板失效行为的模拟策略^[23]

θ₁ and θ₂—Ply angle; d—Phase field

Figure 5. Modelling strategy about failure composite laminates^[23]

下载: 全尺寸图片幻灯片

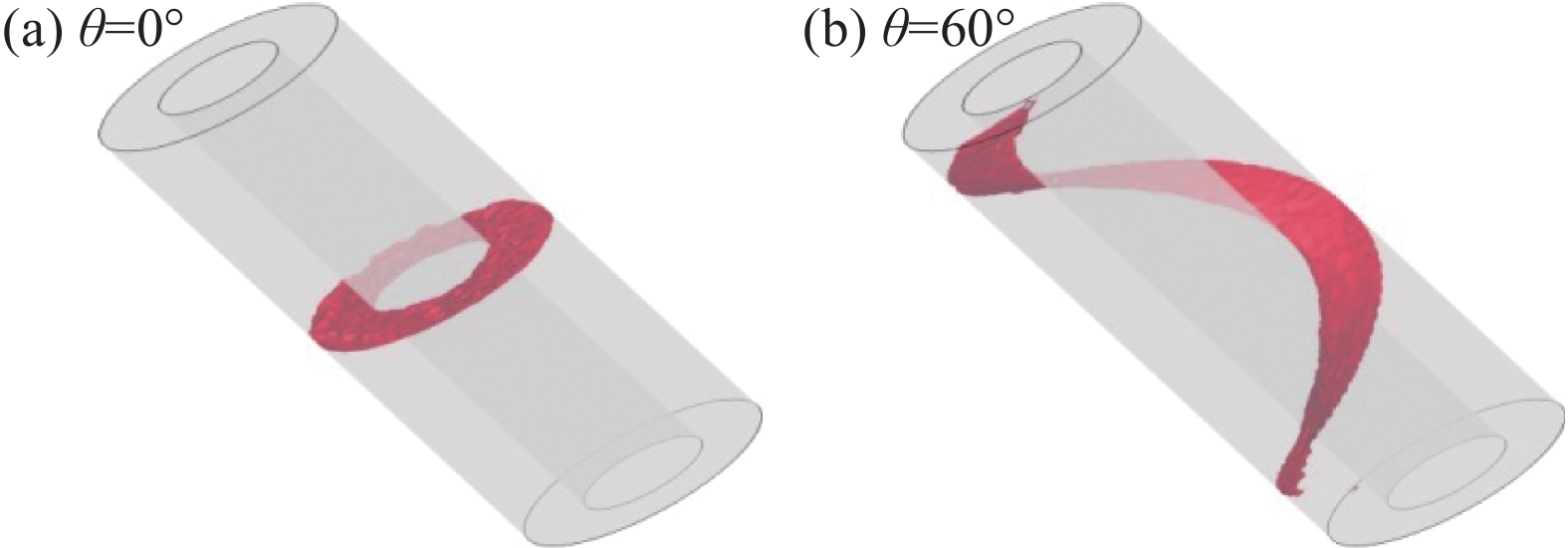
图 6 纤维桥联模型的机制和桥联区长度^[28]

L—Length; h—Height; a_t—Crack length; a_e—Effective crack length; l_c—Fracture process zone length; l_b—Bridging zone length; ${\delta _{\rm{n}}}$ —Opening displacement of the bridging ligament; $\delta _{\rm{n}}^{\rm{f}}$ —Opening displacement of the cracked fiber

Figure 6. Sketch of the fibre bridging mechanism and the bridging zone length^[28]

下载: 全尺寸图片幻灯片

图 7 相场法与其他数值方法的结合

Figure 7. Combination of phase field method and other numerical methods

下载: 全尺寸图片幻灯片

图 8 相场法与其他理论的结合

Figure 8. Combination of phase field method and other theories

下载: 全尺寸图片幻灯片

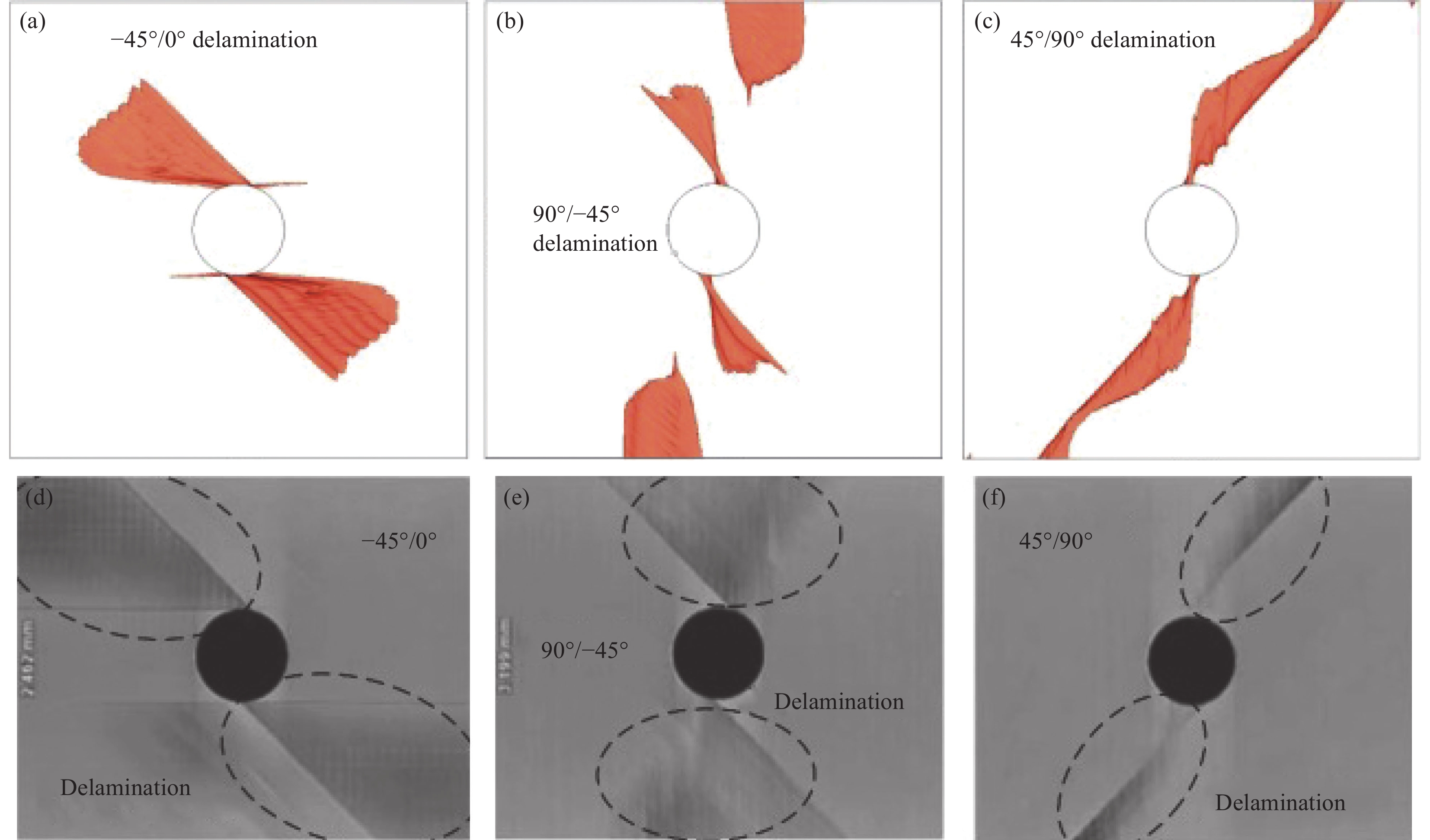
图 9 模拟^[39]和测量^[40]得到的纤维增强复合材料的载荷-裂纹口张开位移(CMOD)曲线(a)和裂纹扩展路径(b)

FE—Finite element simulation; Exp—Experimental results; P—Loading; Δ—Crack mouth opening displacement

Figure 9. Predicted^[39] and measured^[40] of load-crack opening displacement (CMOD) curves (a) and crack propagation path (b) for fibre-reinforced composites

下载: 全尺寸图片幻灯片

图 10 纤维增强复合材料：((a)~(c)) 损伤演化阶段不同铺层之间的分层^[3] ；((d)~(f)) 试验结果的X光扫描的分层图像^[43]

Figure 10. Fibre reinforced composites: ((a)-(c)) Delamination between different plies at the damage evolution stage^[3]; ((d)-(f)) Experimental X-ray images of delamination^[43]

下载: 全尺寸图片幻灯片

图 11 大变形拉伸导致的炭黑增强天然橡胶复杂裂纹扩展拓扑^[50]

Δu—Displacement loading; λ—Elongation

Figure 11. Snapshots showing the complex crack propagation topology of natural rubber filled with stiff/very stiff carbon black particles due to large deformation stretching^[50]

下载: 全尺寸图片幻灯片

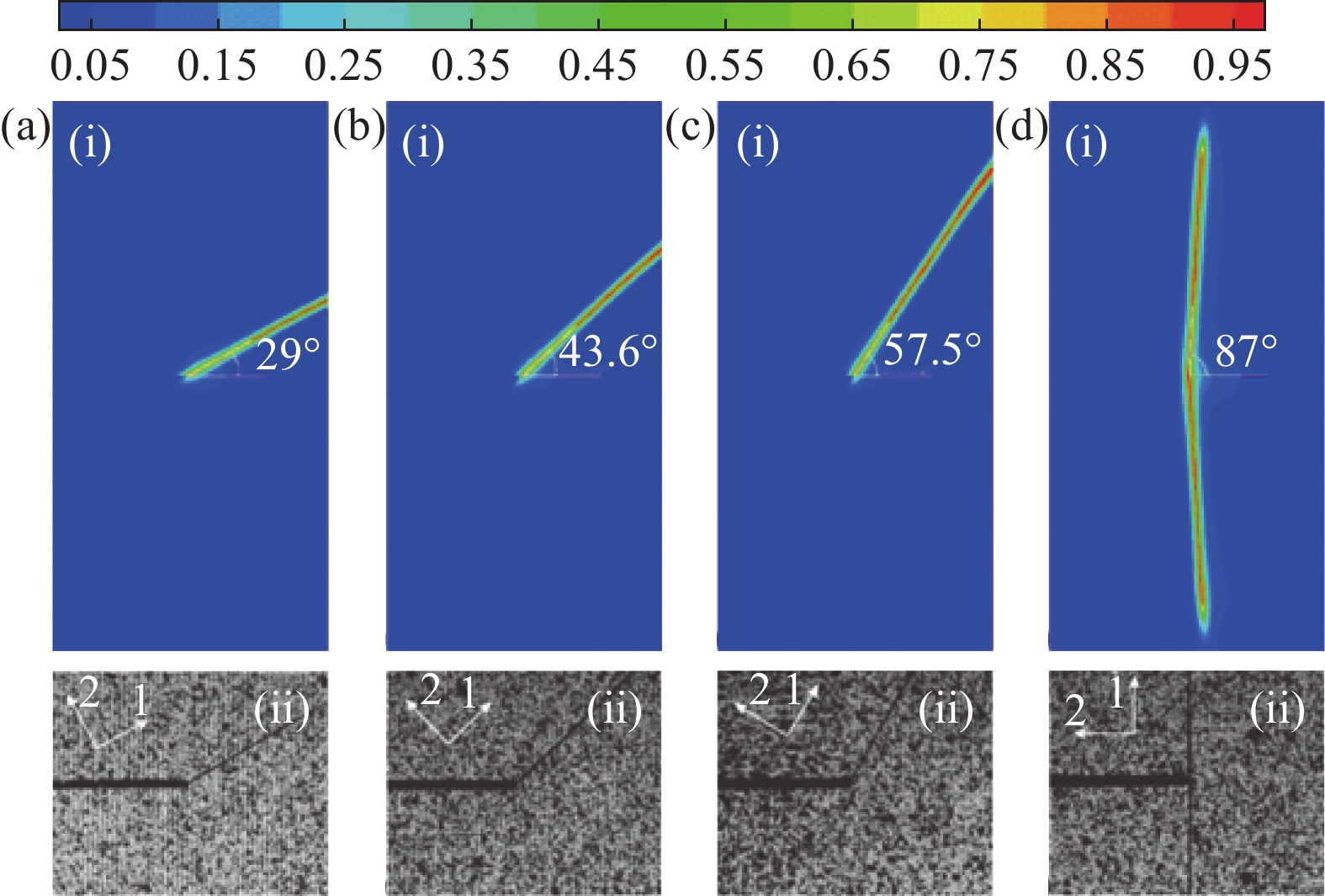
图 12 3D打印聚合物复合材料在不同伸长下的裂纹萌生序列^[52]：((a), (c), (e), (g), (i)) 数值结果；((b), (d), (f), (h), (j))实验结果

Figure 12. Crack initiation sequence of 3D-printed hyperelastic composites at different values of global stretch^[52]: ((a), (c), (e), (g), (i)) Numerical results; ((b), (d), (f), (h), (j)) Experimental results

下载: 全尺寸图片幻灯片

图 13 Ti-6V-4Al合金微结构韧性裂纹扩展在7.6%体积平均真实应变加载(a)和13.0%体积平均真实应变加载下(b)的相场云图^[62]

Figure 13. Contour plots of the phase field for Ti-6V-4Al microstructure in the deformed configuration from ductile crack propagation for 7.6% volume-averaged true strain (a) and 13.0% volume-averaged true strain (b)^[62]

下载: 全尺寸图片幻灯片

表 1 复合材料的相场断裂能模型

Table 1 Phase field fracture energy model of composite material

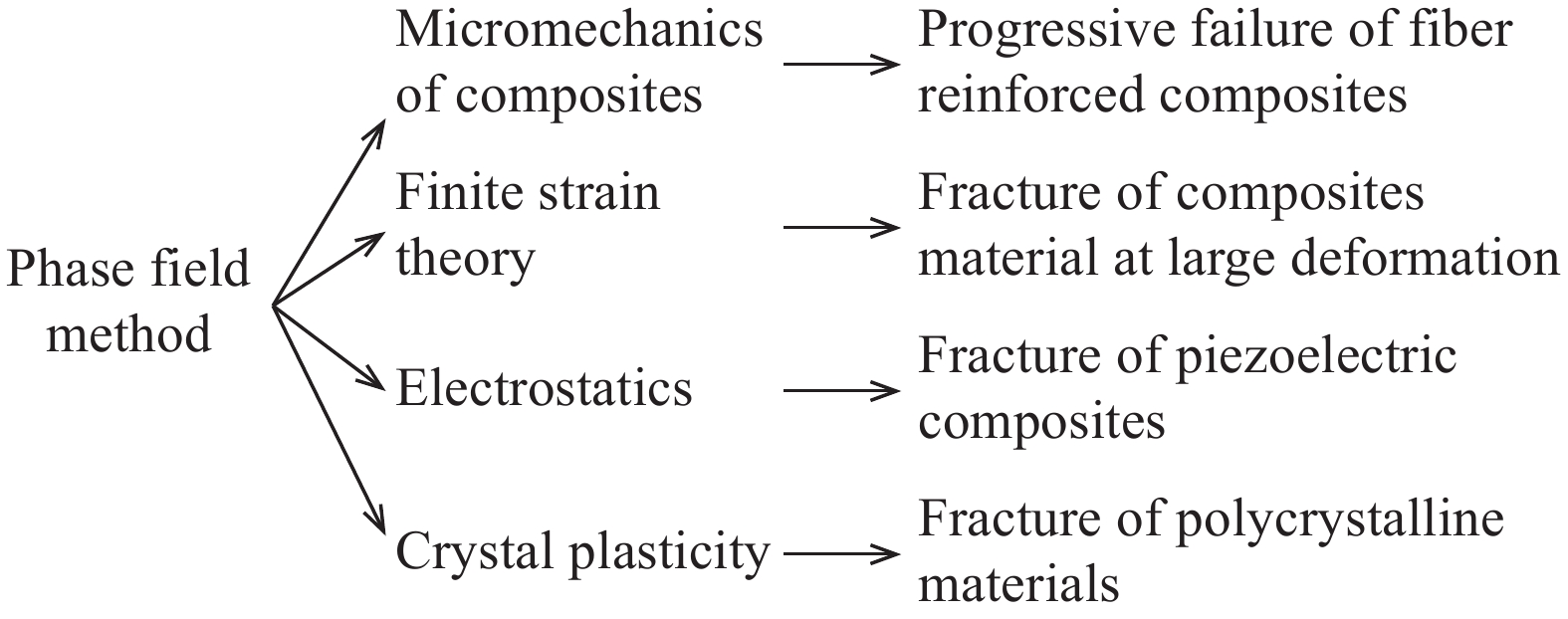
Model	Mathematical model	Ref.
Second-order anisotropic model	$\dfrac{1}{2}\left[ {\dfrac{1}{ { {l_0} } }{\phi ^2} + {l_0}\left( {\nabla \phi {{A} } \nabla \phi } \right)} \right]$ ${A_{ij}} = {\delta _{ij}} + \gamma {M_{ij}}$ ${M_{ij}}{\text{ = }}{N_i}{N_j}$	[13]
Double isotropic model for different crack mode	$\displaystyle\int_\varOmega {\dfrac{{{G_{{\rm{cI}}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}\phi _1^2 + {l_0}\left( {\nabla {\phi _1} \cdot \nabla {\phi _1}} \right)} \right]} {\rm{d}}V + \displaystyle\int_\varOmega {\dfrac{{{G_{{\rm{cII}}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}\phi _2^2 + {l_0}\left( {\nabla {\phi _2} \cdot \nabla {\phi _2}} \right)} \right]} {\rm{d}}V$	[15]
Double isotropic model for different component	$\displaystyle\int_\varOmega {\dfrac{{{G_{\rm{f}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}\phi _{\rm{f}}^2 + {l_0}\left( {\nabla {\phi _{\rm{f}}} \cdot \nabla {\phi _{\rm{f}}}} \right)} \right]} {\rm{d}}V + \displaystyle\int_\varOmega {\dfrac{{{G_{\rm{m}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}\phi _{\rm{m}}^2 + {l_0}\left( {\nabla {\phi _{\rm{m}}} \cdot \nabla {\phi _{\rm{m}}}} \right)} \right]} {\rm{d}}V$	[16]
Double second-order anisotropic model	$\displaystyle\int_\varOmega {\dfrac{{{G_{{\rm{cI}}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}{\phi ^2} + {l_0}{{{A}}_1}:\left( {\nabla \phi \otimes \nabla \phi } \right)} \right]} {\rm{d}}V + \displaystyle\int_\varOmega {\dfrac{{{G_{{\rm{cII}}}}}}{2}\left[ {\dfrac{1}{{{l_0}}}{\phi ^2} + {l_0}{{{A}}_2}:\left( {\nabla \phi \otimes \nabla \phi } \right)} \right]} {\rm{d}}V$	[17]
Fourth-order transverse isotropic model	$\begin{array}{l}{\displaystyle {\int }_{\varOmega }\dfrac{1}{2}\left[\dfrac{1}{{l}_{0}}{\phi }^{2}+\dfrac{{l}_{0}}{2}A:\left(\nabla \phi \otimes \nabla \phi \right)+\dfrac{{l}_{0}^{3}}{16}\left(\nabla \nabla \phi :\mathbb{A}:\nabla \nabla \phi \right)\right]}{\rm{d}}V，{A}_{ij}={\delta }_{ij}+\gamma {M}_{ij}，{M}_{ij}\text{= }{N}_{i}{N}_{j}\\ {\mathbb{A}}_{ijkl}={\delta }_{ij}{\delta }_{kl}+{\rm{sym}}\left({\beta }_{1}{M}_{ij}{M}_{kl}+{\beta }_{2}{\delta }_{ij}{M}_{kl}\right)+\dfrac{{\beta }_{3}}{2}\left({\delta }_{ik}{M}_{jl}+{M}_{ik}{\delta }_{jl}+{\delta }_{il}{M}_{jk}+{M}_{il}{\delta }_{jk}\right)\end{array}$	[18]
Fourth-order orthotropic model	$\begin{array}{l}{\displaystyle {\int }_{\varOmega }\dfrac{1}{2}\left[\dfrac{1}{{l}_{0}}{\phi }^{2}+\dfrac{{l}_{0}}{2}A:\left(\nabla \phi \otimes \nabla \phi \right)+\dfrac{{l}_{0}^{3}}{16}\left(\nabla \nabla \phi :\mathbb{A}:\nabla \nabla \phi \right)\right]}{\rm{d}}V\\ {A}_{ij}={\delta }_{ij}+{\gamma }_{1}{M}_{ij}^{1}+{\gamma }_{2}{M}_{ij}^{2}，{M}_{ij}^{1}\text{= }{N}_{i}^{1}{N}_{j}^{1}，{M}_{ij}^{2}\text{= }{N}_{i}^{2}{N}_{j}^{2}\\ {\mathbb{A}}_{ijkl}=\dfrac{1}{2}\left({\delta }_{ij}{\delta }_{kl}+{\delta }_{il}{\delta }_{kj}\right)+{\rm{sym}}[{\displaystyle \sum _{s=1}^{2}\left({\alpha }_{1}^{s}{M}_{ij}^{s}{M}_{kl}^{s}+{\alpha }_{2}^{s}{\delta }_{ij}{M}_{kl}^{s}\right)}+{\alpha }_{7}{M}_{ij}^{1}{M}_{kl}^{2}+\\ \text{ }{\displaystyle \sum _{s=1}^{2}\dfrac{{\alpha }_{3}^{s}}{2}\left({\delta }_{ik}{M}_{jl}^{s}+{M}_{ik}^{s}{\delta }_{jl}+{\delta }_{il}{M}_{jk}^{s}+{M}_{il}^{s}{\delta }_{jk}\right)}]\end{array}$	[18]
Fourth-order cubic symmetric model	$\begin{array}{l}{\displaystyle {\int }_{\varOmega }\dfrac{1}{2}\left[\dfrac{1}{{l}_{0}}{\phi }^{2}+\dfrac{{l}_{0}}{2}\left(\nabla \phi \cdot \nabla \phi \right)+\dfrac{{l}_{0}^{3}}{16}\left(\nabla \nabla \phi :\mathbb{A}:\nabla \nabla \phi \right)\right]}{\rm{d}}V\\ {\mathbb{A}}_{ijkl}=\dfrac{1}{2}\left({\delta }_{ij}{\delta }_{kl}+{\delta }_{il}{\delta }_{kj}\right)+{\displaystyle \sum _{m=1}^{2}{\displaystyle \sum _{n=1}^{2}\left[\alpha {\delta }_{mn}+\dfrac{\beta }{2}\left(1-{\delta }_{mn}\right)\right]}}{M}_{ij}^{m}{M}_{kl}^{m}\end{array}$	[18]
Notes: A and ${A_{ij}}$ —Second-order structure tensor and its component form; ${\delta _{ij}}$ —Component of the second-order indentiy tensor; $\gamma$ —Penalty parameter; ${N_i}$ —Component of unit vector along the fiber direction; ${G_{{\rm{cI}}}}$ and ${G_{{\rm{cII}}}}$ —Crtical energy release rate for mode I and mode II crack; ${\phi _1}$ and ${\phi _2}$ —Phase field variables for mode I and mode II crack ; ${G_{\rm{f}}}$ and ${G_{\rm{m}}}$ —Crtical energy release rate of fiber and matrix; ${\phi _{\rm{f}}}$ and ${\phi _{\rm{m}}}$ —Phase field variables of fiber and matrix; ${{{A}}_1}$ and ${{{A}}_2}$ —Second-order structure tensor for mode I and mode II crack ; $\mathbb{A}$ —Fourth-order structure tensor; $N_i^1$ and $N_i^2$ —Components of two orthonomal basis; ${\beta _1}$ , ${\beta _2}$ , ${\beta _3}$ , ${\gamma _1}$ , ${\gamma _2}$ , $\alpha _1^s$ , $\alpha _2^s$ , $\alpha _3^s$ , ${\alpha _7}$ , $\alpha$ and $\beta$ —Material parameters.

下载: 导出CSV

表 2 复合材料的长度尺度参数模型

Table 2 Length scale parameter model of composite material

Model	Mathematical model	Ref.
Zhang's model	$l_0^{{\rm{ani}}}\left( {{\varphi _1}} \right) = l_0^{{\rm{iso}}}\sqrt {1 + \beta {{\cos }^2}{\varphi _1}}$	[13]
Transverse isotropic model	$l_0^{{\rm{tran}}}\left( {\varphi ,\theta } \right) = {l_0}\left[ {1 + \alpha {{\sin }^2}\left( {\varphi - \theta } \right)} \right]$	[18]
Orthotropic model	$l_0^{{\rm{orth}}}\left( {\varphi ,\theta } \right) = {l_0}\left[ {1 + {\alpha ^1}{{\sin }^2}\left( {\varphi - \theta } \right) + {\alpha ^2}{{\cos }^2}\left( {\varphi - \theta } \right)} \right]$	[18]
Cubic symmetric model	$\begin{gathered} l_0^{\rm cubic}\left( {\varphi ,\theta } \right) = \gamma {\left\{ {1 + \eta \cos \left[ {4\left( {\varphi - \theta } \right)} \right]} \right\}^{1/3}} \\ \gamma = {l_0}{\left( {\frac{{8 + 6\alpha + \beta }}{8}} \right)^{1/3}},\quad \eta = \left( {\frac{{8 + 6\alpha + \beta }}{{2\alpha - \beta }}} \right) \\ \end{gathered}$	[18]
Notes: ${\varphi _1}$ —Angle between the direction of gradient of phase field and the weak failure direction; ${l_0}$ —Length scale parameter of isotropic phase field fracture model; $\varphi$ —Angle between the horizontal axis and the tangent of the crack at position; $\theta$ —Angle between the horizontal axis and the direction of the fiber; $\alpha$ , ${\alpha ^1}$ , ${\alpha ^2}$ , $\gamma$ , $\eta$ , $\beta$ —Material parameter.

下载: 导出CSV

参考文献(63)

[1]	杜善义, 关志东. 我国大型客机先进复合材料技术应对策略思考[J]. 复合材料学报, 2008, 25(1):1-10. DOI: 10.3321/j.issn:1000-3851.2008.01.001 DU Shanyi, GUAN Zhidong. Strategic considerations for development of advanced composite technology for large commercial aircraft in China[J]. Acta Materiae Compositae Sinaca,2008,25(1):1-10(in Chinese). DOI: 10.3321/j.issn:1000-3851.2008.01.001
[2]	李西宁, 王悦舜, 周新房. 复合材料层合板分层损伤数值模拟方法研究现状[J]. 复合材料学报, 2021, 38(4):1076-1086. DOI: 10.13801/j.cnki.fhclxb.20210302.006 LI Xi'ning, WANG Yueshun, ZHOU Xinfang. Status of numerical simulation methods for delamination damage of composite laminates[J]. Acta Materiae Compositae Sinica,2021,38(4):1076-1086(in Chinese). DOI: 10.13801/j.cnki.fhclxb.20210302.006
[3]	ZHANG P, YAO W, HU X, et al. An explicit phase field model for progressive tensile failure of composites[J]. Engineering Fracture Mechanics,2021,241:107371. DOI: 10.1016/j.engfracmech.2020.107371
[4]	PRANAVI D, RAJAGOPAL A, REDDY J N. Interaction of anisotropic crack phase field with interface cohesive zone model for fiber reinforced composites[J]. Composite Structures,2021,270:114038. DOI: 10.1016/j.compstruct.2021.114038
[5]	BUI T Q, HU X. A review of phase-field models, fundamentals and their applications to composite laminates[J]. Engineering Fracture Mechanics,2021,248:107705. DOI: 10.1016/j.engfracmech.2021.107705
[6]	ZHUANG X, ZHOU S, HUYNH G D, et al. Phase field modelling and computer implementation: A review[J]. Engineering Fracture Mechanics,2022 , 262:108234. DOI: 10.1016/j.engfracmech.2022.108234
[7]	FRANCFORT G A, MARIGO J J. Revisiting brittle fracture as an energy minimization problem[J]. Journal of the Mechanics and Physics of Solids,1998,46(8):1319-1342. DOI: 10.1016/S0022-5096(98)00034-9
[8]	吴建营. 固体结构损伤破坏统一相场理论、算法和应用[J]. 力学学报, 2021, 53(2):301-329. DOI: 10.6052/0459-1879-20-295 WU Jianying. On the theoretical and numerical aspects of the unified phase-field theory for damage and failure in solids and structures[J]. Chinese Journal of Theoretical and Applied Mechanics,2021,53(2):301-329(in Chinese). DOI: 10.6052/0459-1879-20-295
[9]	WU J Y, NGUYEN V P, NGUYEN C T, et al. Phase-field modeling of fracture[J]. Advances in Applied Mechanics,2020,53:1-183.
[10]	BOURDIN B, FRANCFORT G A, MARIGO J J. Numerical experiments in revisited brittle fracture[J]. Journal of the Mechanics and Physics of Solids,2000,48(4):797-826.
[11]	BOURDIN B, FRANCFORT G A, MARIGO J J. The variational approach to fracture[J]. Journal of Elasticity,2008,91(1):5-148.
[12]	AMOR H, MARIGO J J, MAURINI C. Regularized formulation of the variational brittle fracture with unilateral contact: Numerical experiments[J]. Journal of the Mechanics and Physics of Solids,2009,57(8):1209-1229. DOI: 10.1016/j.jmps.2009.04.011
[13]	ZHANG P, HU X, BUI T Q, et al. Phase field modeling of fracture in fiber reinforced composite laminate[J]. International Journal of Mechanical Sciences,2019,161:105008.
[14]	CAHILL L M A, NATARAJAN S, BORDAS S P A, et al. An experimental/numerical investigation into the main driving force for crack propagation in uni-directional fibre-reinforced composite laminae[J]. Composite Structures,2014,107:119-130. DOI: 10.1016/j.compstruct.2013.05.039
[15]	BLEYER J, ALESSI R. Phase-field modeling of anisotropic brittle fracture including several damage mechanisms[J]. Computer Methods in Applied Mechanics and Engineering,2018,336:213-236. DOI: 10.1016/j.cma.2018.03.012
[16]	NGUYEN T T, RÉTHORÉ J, BAIETTO M C. Phase field modelling of anisotropic crack propagation[J]. European Journal of Mechanics-A/Solids,2017,65:279-288.
[17]	ZHANG P, TAN S, HU X, et al. A double-phase field model for multiple failures in composites[J]. Composite Structures,2022,293:115730. DOI: 10.1016/j.compstruct.2022.115730
[18]	TEICHTMEISTER S, KIENLE D, ALDAKHEEL F, et al. Phase field modeling of fracture in anisotropic brittle solids[J]. International Journal of Non-Linear Mechanics,2017,97:1-21.
[19]	MIEHE C, WELSCHINGER F, HOFACKER M. Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations[J]. International Journal for Numerical Methods in Engineering,2010,83:1273-1311. DOI: 10.1002/nme.2861
[20]	ASUR VIJAYA KUMAR P K, DEAN A, REINOSO J, et al. A multi phase-field-cohesive zone model for laminated composites: Application to delamination migration[J]. Composite Structures,2021,276:114471. DOI: 10.1016/j.compstruct.2021.114471
[21]	PILLAI U, TRIANTAFYLLOU S P, ESSA Y, et al. An anisotropic cohesive phase field model for quasi-brittle fractures in thin fibre-reinforced composites[J]. Composite Structures,2020,252:112635.
[22]	CAROLLO V, REINOSO J, PAGGI M. A 3D finite strain model for intralayer and interlayer crack simulation coupling the phase field approach and cohesive zone model[J]. Composite Structures,2017,182:636-651. DOI: 10.1016/j.compstruct.2017.08.095
[23]	ZHANG P, YAO W, HU X, et al. Phase field modelling of progressive failure in composites combined with cohesive element with an explicit scheme[J]. Composite Structures,2021,262:113353. DOI: 10.1016/j.compstruct.2020.113353
[24]	ZHANG P, FENG Y, BUI T Q, et al. Modelling distinct failure mechanisms in composite materials by a combined phase field method[J]. Composite Structures,2020,232:111551. DOI: 10.1016/j.compstruct.2019.111551
[25]	RAO S, BUDZIK M K, DIAS M A. On microscopic analysis of fracture in unidirectional composite material using phase field modelling[J]. Composites Science and Technology,2022,220:109242. DOI: 10.1016/j.compscitech.2021.109242
[26]	GARCÍA-MACÍAS E, D'ALESSANDRO A, CASTRO-TRIGUERO R, et al. Micromechanics modeling of the electrical conductivity of carbon nanotube cement-matrix composites[J]. Composites Part B: Engineering,2017,108:451-469. DOI: 10.1016/j.compositesb.2016.10.025
[27]	QUINTEROS L, GARCÍA-MACÍAS E, MARTÍNEZ-PAÑEDAE. Micromechanics-based phase field fracture modeling of CNT composites[J]. Composites Part B: Engineering,2022,236:109788. DOI: 10.1016/j.compositesb.2022.109788
[28]	TAN W, MARTÍNEZ-PAÑEDA E. Phase field fracture predictions of microscopic bridging behaviour of composite materials[J]. Composite Structures,2022,286:115242. DOI: 10.1016/j.compstruct.2022.115242
[29]	GUILLÉN-HERNÁNDEZ T, QUINTANA-COROMINAS A, GARCIA I G, et al. In-situ strength effects in long fibre reinforced composites: A micro-mechanical analysis using the phase field approach of fracture[J]. Theoretical and Applied Fracture Mechanics,2020,108:102621. DOI: 10.1016/j.tafmec.2020.102621
[30]	LI X, XU Y. Phase field modeling scheme with mesostructure for crack propagation in concrete composite[J]. International Journal of Solids and Structures,2022,234:111259.
[31]	XIA Y, WU W, YANG Y, et al. Mesoscopic study of concrete with random aggregate model using phase field method[J]. Construction and Building Materials,2021,310:125199. DOI: 10.1016/j.conbuildmat.2021.125199
[32]	PAGGI M, CORRADO M, REINOSO J. Fracture of solar-grade anisotropic polycrystalline silicon: A combined phase field-cohesive zone model approach[J]. Computer Methods in Applied Mechanics and Engineering,2018,330:123-148.
[33]	HEISTER T, WHEELER M F, WICK T. A primal-dual active set method and predictor-corrector mesh adaptivity for computing fracture propagation using a phase-field approach[J]. Computer Methods in Applied Mechanics and Engineering,2015,290:466-495. DOI: 10.1016/j.cma.2015.03.009
[34]	NGUYEN-THANH N, LI W, HUANG J, et al. Multi phase-field modeling of anisotropic crack propagation in 3D fiber-reinforced composites based on an adaptive isogeometric meshfree collocation method[J]. Computer Methods in Applied Mechanics and Engineering,2022,393:114794. DOI: 10.1016/j.cma.2022.114794
[35]	SHAO Y, DUAN Q, QIU S. Adaptive analysis for phase-field model of brittle fracture of functionally graded materials[J]. Engineering Fracture Mechanics,2021,251:107783. DOI: 10.1016/j.engfracmech.2021.107783
[36]	CHEN Y, GÉLÉBART L, MARANO A, et al. FFT phase-field model combined with cohesive composite voxels for fracture of composite materials with interfaces[J]. Computational Mechanics,2021,68(2):433-457.
[37]	CAO Y J, SHEN W Q, SHAO J F, et al. A novel FFT-based phase field model for damage and cracking behavior of heterogeneous materials[J]. International Journal of Plasticity,2020,133:102786. DOI: 10.1016/j.ijplas.2020.102786
[38]	ROY P, DEEPU S P, PATHRIKAR A, et al. Phase field based peridynamics damage model for delamination of compo-site structures[J]. Composite Structures,2017,180:972-993. DOI: 10.1016/j.compstruct.2017.08.071
[39]	TAN W, MARTÍNEZ-PAÑEDA E. Phase field predictions of microscopic fracture and R-curve behaviour of fibre-reinforced composites[J]. Composites Science and Technology,2021,202:108539. DOI: 10.1016/j.compscitech.2020.108539
[40]	CANAL L P, GONZÁLEZ C, SEGURADO J, et al. Intraply fracture of fiber-reinforced composites: Microscopic mechanisms and modeling[J]. Composites Science and Technology,2012,72:1223-1232. DOI: 10.1016/j.compscitech.2012.04.008
[41]	ESPADAS-ESCALANTE J J, VAN DIJK N P, ISAKSSON P. A phase-field model for strength and fracture analyses of fiber-reinforced composites[J]. Composites Science and Technology,2019,174:58-67. DOI: 10.1016/j.compscitech.2018.10.031
[42]	QUINTANAS-COROMINAS A, REINOSO J, CASONI E, et al. A phase field approach to simulate intralaminar and translaminar fracture in long fiber composite materials[J]. Composite Structures,2019,220:899-911. DOI: 10.1016/j.compstruct.2019.02.007
[43]	SONG K, LI Y, ROSE C. Continuum damage mechanics models for the analysis of progressive failure in open-hole tension laminates[J]. Archives of Biochemistry & Biophysics, 1957, 69: 634-643.
[44]	LI G, YIN B B, ZHANG L W, et al. Modeling microfracture evolution in heterogeneous composites: A coupled cohesive phase-field model[J]. Journal of the Mechanics and Physics of Solids,2020,142:103968. DOI: 10.1016/j.jmps.2020.103968
[45]	LI G, YIN B B, ZHANG L W, et al. A framework for phase-field modeling of interfacial debonding and frictional slipping in heterogeneous composites[J]. Computer Methods in Applied Mechanics and Engineering,2021,382:113872.
[46]	ALESSI R, FREDDI F. Phase-field modelling of failure in hybrid laminates[J]. Composite Structures,2017,181:9-25. DOI: 10.1016/j.compstruct.2017.08.073
[47]	ALESSI R, FREDDI F. Failure and complex crack patterns in hybrid laminates: A phase-field approach[J]. Composites Part B: Engineering,2019,179:107256. DOI: 10.1016/j.compositesb.2019.107256
[48]	PAN Z Z, ZHANG L W, LIEW K M. A phase-field framework for failure modeling of variable stiffness composite lami-nate[J]. Computer Methods in Applied Mechanics and Engineering,2022,388:114192.
[49]	NATARAJAN S, ANNABATTULA R K. Modeling crack propagation in variable stiffness composite laminates using the phase field method[J]. Composite Structures,2019,209:424-433. DOI: 10.1016/j.compstruct.2018.10.083
[50]	WU J, MCAULIFFE C, WAISMAN H, et al. Stochastic analysis of polymer composites rupture at large deformations modeled by a phase field method[J]. Computer Methods in Applied Mechanics and Engineering,2016,312:596-634. DOI: 10.1016/j.cma.2016.06.010
[51]	WANG X, JIANG M, ZHOU Z, et al. 3D printing of polymer matrix composites: A review and prospective[J]. Compo-sites Part B: Engineering,2017,110:442-458. DOI: 10.1016/j.compositesb.2016.11.034
[52]	RUSS J, SLESARENKO V, RUDYKH S, et al. Rupture of 3D-printed hyperelastic composites: Experiments and phase field fracture modeling[J]. Journal of the Mechanics and Physics of Solids,2020,140:103941. DOI: 10.1016/j.jmps.2020.103941
[53]	MANDAL T K, NGUYEN V P, WU J Y. A length scale insensitive anisotropic phase field fracture model for hyperelastic composites[J]. International Journal of Mechani-cal Sciences,2020,188:105941.
[54]	BHARDWAJ G, SINGH I, MISHRA B, et al. Numerical simulation of functionally graded cracked plates using NURBS based XIGA under different loads and boundary conditions[J]. Composite Structures,2015,126:347-359. DOI: 10.1016/j.compstruct.2015.02.066
[55]	DUC H, TINH Q, NGUYEN D, et al. Hybrid phase field simulation of dynamic crack propagation in functionally graded glass-filled epoxy[J]. Composites Part B: Engineering,2016,99:266-276. DOI: 10.1016/j.compositesb.2016.06.016
[56]	THOM V, DUC H, NGUYEN D, et al. Phase-field thermal buckling analysis for cracked functionally graded compo-site plates considering neutral surface[J]. Composite Structures,2017,182:542-548. DOI: 10.1016/j.compstruct.2017.09.059
[57]	PHUC P, NGUYEN D. The effect of cracks on the stability of the functionally graded plates with variable-thickness using HSDT and phase-field theory[J]. Composites Part B: Engineering,2019,175:107086.
[58]	HIRSHIKESH, NATARAJAN S, ANNABATTULA R K, et al. Phase field modelling of crack propagation in functionally graded materials[J]. Composites Part B: Engineering,2019,169:239-248. DOI: 10.1016/j.compositesb.2019.04.003
[59]	TAN Y, HE Y, LI X. Phase field fracture modeling of transversely isotropic piezoelectric material with anisotropic fracture toughness[J]. International Journal of Solids and Structures,2022,248:111615. DOI: 10.1016/j.ijsolstr.2022.111615
[60]	DAN S, TARAFDER P, GHOSH S. Adaptive wavelet-enhanced cohesive zone phase-field FE model for crack evolution in piezoelectric composites[J]. Computer Methods in Applied Mechanics and Engineering,2022,392:114636. DOI: 10.1016/j.cma.2022.114636
[61]	SOUKAINA R, DIDIER B, JULIEN R. Unified phase field model to simulate both intergranular and transgranular failure in polycrystalline aggregates[J]. Finite Elements in Analysis and Design,2021,194:103555. DOI: 10.1016/j.finel.2021.103555
[62]	CHENG J, TU X, GHOSH S. Wavelet-enriched adaptive hierarchical FE model for coupled crystal plasticity-phase field modeling of crack propagation in polycrystalline microstructures[J]. Computer Methods in Applied Mechanics and Engineering,2020,361:112757. DOI: 10.1016/j.cma.2019.112757
[63]	EMDADI A, ZAEEM M A. Phase-field modeling of crack propagation in polycrystalline materials[J]. Computational Materials Science,2021,186:110057. DOI: 10.1016/j.commatsci.2020.110057