CFRP薄壁结构多尺度建模及耐撞性分析

朱国华; 竺森森; 胡珀; 王振; 赵轩

doi:10.13801/j.cnki.fhclxb.20220720.002

CFRP薄壁结构多尺度建模及耐撞性分析

长安大学　汽车学院，西安 710064

基金项目: 国家重点研发计划(2021 YFB2501705)；国家自然科学基金 (51905042)；长安大学中央高校基础研究基金 (300102221201)National Key R&D Program of China (2021YFB2501705); National Natural Science Foundation of China (51905042); The Fundamental Research Funds for the Central Universities, CHD (300102221201)

详细信息

通讯作者:
朱国华，博士，副教授，硕士生导师，研究方向为汽车轻量化　 E-mail: guohuazhu@chd.edu.cn

中图分类号: TB332
计量
- 文章访问数: 1072
- HTML全文浏览量: 727
- PDF下载量: 148
出版历程
- 收稿日期: 2022-06-06
- 修回日期: 2022-06-22
- 录用日期: 2022-07-06
- 网络出版日期: 2022-07-21
- 刊出日期: 2023-06-14

Multi-scale modeling and crashworthiness analysis of CFRP thin-walled structures

School of Automobile, Chang'an University, Xi'an 710064, China

摘要

摘要: 碳纤维增强树脂基复合材料(CFRP)具有较高的比强度、比刚度及显著的轻量化效果，因此CFRP薄壁结构被作为能量吸收装置广泛应用于工程领域。以单向碳纤维复合材料为研究对象，利用扫描电镜获取其微观胞元结构参数及纤维体积分数，构建能够准确反映其微观形态的代表性体积单元(Representative volume element，RVE)，通过加载周期性边界条件及单位载荷，获取材料宏观等效弹性参数，并开展实验验证。随后，开发基于微观力学的失效准则及损伤演化方程，并结合材料力学特点，构建CFRP宏观损伤模型，最终形成一套基于微观失效的多尺度损伤模型。在此基础上，对CFRP薄壁圆管在轴向准静态载荷下的压溃性能进行数值仿真，并与实验结果进行对比，验证了多尺度模型的仿真精度。最后，基于验证后的多尺度有限元模型，研究了碳纤维铺层角度及碳纤维体积分数对CFRP薄壁结构耐撞性的影响。结果表明：铺层角度和碳纤维体积分数对CFRP圆管耐撞性能具有较大影响。
- 碳纤维增强树脂基复合材料 /
- 耐撞性 /
- 多尺度分析 /
- 多尺度损伤模型 /
- 微观失效准则
Abstract: Carbon fiber reinforced plastics (CFRP) are of high specific strength, specific stiffness and significant lightweight effect. Therefore, CFRP thin-walled structures are widely used as energy-absorbing devices in engineering fields. This paper took unidirectional CFRP as research object. The micro-scale structural parameters and fiber volume fraction were obtained by using scanning electron microscope. Then, a representative volume element (RVE) was established, which was capable to accurately reflect its micro morphology. By applying periodic boundary conditions and unit load, the macro equivalent elastic parameters were acquired and then verified by experimental tests. Subsequently, the failure criterion and damage evolution equation based on micromechanics were developed. Combined with the mechanical characteristics of unidirectional CFRP, the macro damage model was developed, and finally forming a set of multi-scale damage model based on micro failure. On this basis, the crashworthiness performance of CFRP thin-walled circular tube under axial quasi-static load was numerically explored, and the numerical results were verified through the crushing test. Based on the verified multi-scale finite element model, the effects of carbon fiber ply angle and carbon fiber volume fraction on the crashworthiness were investi-gated. The results show that the ply angle and carbon fiber volume fraction have great impact on the crashworthiness characteristics of CFRP thin-walled structures.
- carbon fiber reinforced plastics /
- crashworthiness /
- multi-scale analysis /
- multi-scale damage model /
- micromechanics of failure

HTML全文

图 1 碳纤维增强树脂基复合材料(CFRP)薄壁管制造工艺流程图

Figure 1. Manufacturing process of carbon fiber reinforced plastics (CFRP) thin-walled tubes

下载: 全尺寸图片幻灯片

图 2 试验现场布置图

Figure 2. Experimental setup

下载: 全尺寸图片幻灯片

图 3 CFRP的截面SEM图像

Figure 3. SEM images of cross section for CFRP

下载: 全尺寸图片幻灯片

图 4 (a) 单向碳纤维复合材料六面体代表性体积单元(RVE)模型；(b) RVE参考点

Figure 4. (a) Hexagonal representative volume element (RVE) model of UD-CFRP; (b) Reference points at RVE

F, M—Fiber and matrix, respectively; Number—Selection order of reference points; a=c=1; b= $\sqrt 3$

下载: 全尺寸图片幻灯片

图 5 多尺度分析框架

Figure 5. Multiscale analysis framework

下载: 全尺寸图片幻灯片

图 6 多尺度模型的数值算法流程

Figure 6. Numerical calculation procedure for the multi-scale model

SAFs—Stress amplification factors; RVEs—Representative volume elements

下载: 全尺寸图片幻灯片

图 7 CFRP薄壁结构单位应力施加

Figure 7. Application of unit macro stress for CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 8 CFRP薄壁结构RVE模型在宏观六工况下的微观应力分布

Figure 8. Microscopic stress distribution for RVEs of CFRP thin-walled structure under six loading cases

下载: 全尺寸图片幻灯片

图 9 CFRP薄壁结构0°拉伸试件试验结果

Figure 9. Experimental results of 0° samples of CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 10 CFRP薄壁结构90°拉伸试件试验结果

Figure 10. Experimental results of 90 ° samples of CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 11 CFRP薄壁结构有限元模型

Figure 11. Finite element model of CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 12 网格大小敏感性分析

Figure 12. Mesh size sensitivity analysis

下载: 全尺寸图片幻灯片

图 13 CFRP薄壁结构实验与仿真载荷-位移对比曲线

Figure 13. Comparison of load-displacement curves between experiment and simulation for CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 14 CFRP薄壁结构变形结果对比

Figure 14. Comparison of deformation modes for CFRP thin-walled structure

下载: 全尺寸图片幻灯片

图 15 不同铺层角度的CFRP圆管载荷-位移曲线

Figure 15. Comparison of load-displacement curves for CFRP tubes with different fiber orientations

下载: 全尺寸图片幻灯片

图 16 不同铺层角度CFRP薄壁圆管轴向压溃后的变形结果

Figure 16. Crushing process of CFRP tubes with different fiber orientations

下载: 全尺寸图片幻灯片

图 17 不同体积分数的CFRP薄壁圆管载荷-位移曲线

Figure 17. Comparison of load-displacement curves for CFRP tubes with different fiber volume fractions

下载: 全尺寸图片幻灯片

图 18 不同纤维体积分数CFRP薄壁圆管的变形过程

Figure 18. Deformation process of CFRP thin-walled circular tubes with different fiber volume fractions

下载: 全尺寸图片幻灯片

表 1 T300碳纤维力学性能

Table 1 Mechanical properties of T300 carbon fiber

Mechanical property	Value
$E_{\text{f}\text{1}}$ /GPa	185
$E_{\text{f}\text{2}}\text{=}E_{\text{f}\text{3}}$ /GPa	13
${G}_{\text{f}\text{12}}\text{=}{G}_{\text{f}\text{13}}$ /GPa	15
${G}_{\text{f}\text{23}}$ /GPa	9
${\nu}_{\text{f}\text{12}}\text{=}{\nu}_{\text{f}\text{13}}$	0.28
${\nu}_{\text{f}\text{23}}$	0.35
Notes: E_f1, E_f2, E_f3, G_f12, G_f13, and G_{—Elastic moduli of T300 carbon fiber fibers in the 1, 2, 3, 12, 13, and 23 directions, respectively; v_f12, v_f13, and v_f23—Poisson's ratios in the 12, 13, and 23 directions, respectively.}

下载: 导出CSV

表 2 基体力学性能

Table 2 Mechanical properties of matrix

Mechanical property	Value
${E}_{\mathrm{m}}$ /GPa	2.6
$\nu$	0.33
Note: E_m and v—Micro elastic modulus and Poisson's ratio of the matrix, respectively.

下载: 导出CSV

表 3 CFRP薄壁结构宏观单位应力加载

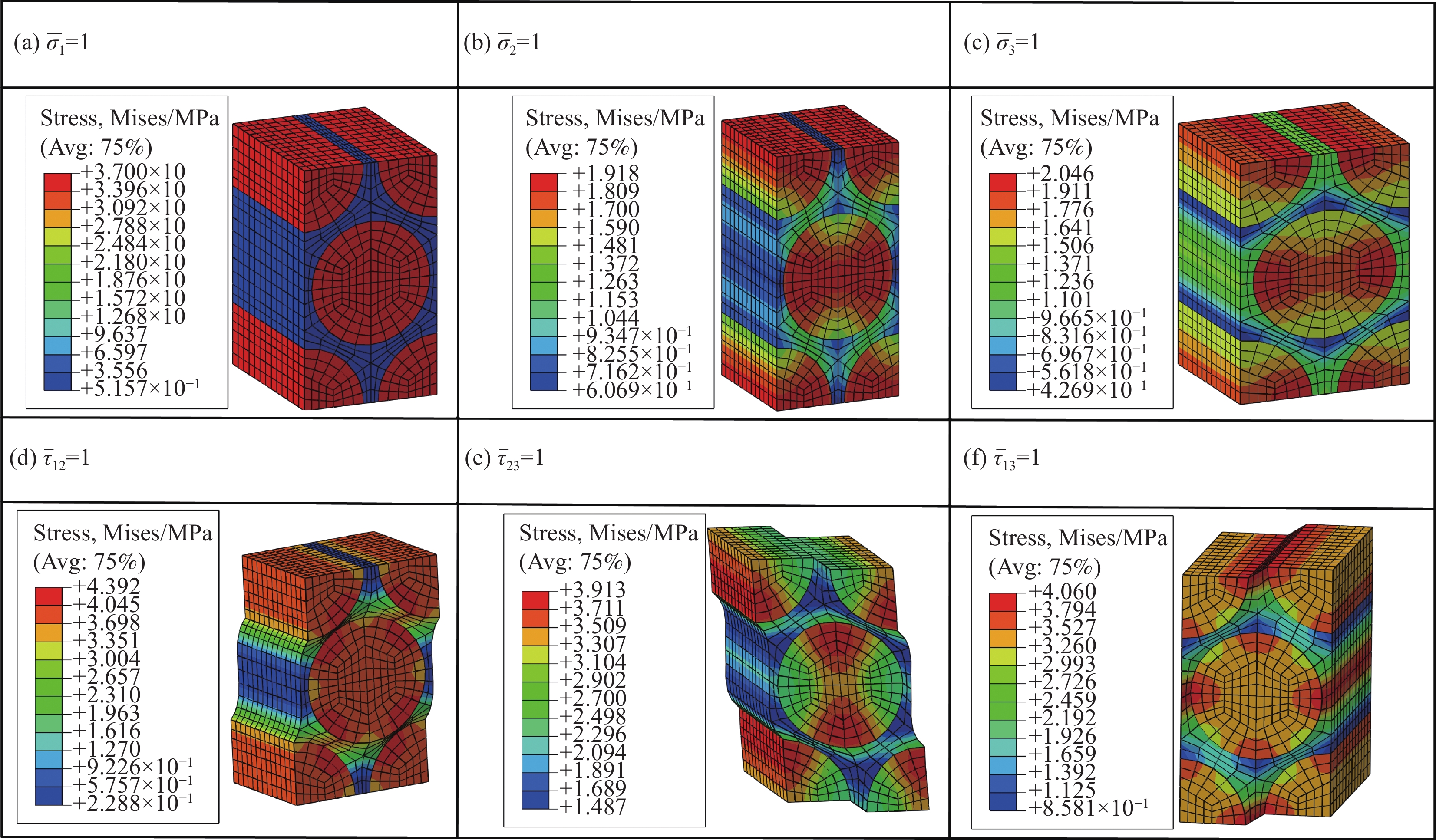
Table 3 Appling macro unit stress of CFRP thin-walled structure

n	${\overline{\sigma} }_{\text{1}}$	${\overline{\sigma} }_{\text{2}}$	${\overline{\sigma} }_{\text{3}}$	${\overline{\tau} }_{\text{12}}$	${\overline{\tau} }_{\text{23}}$	${\overline{\tau} }_{\text{13}}$
1	1	0	0	0	0	0
2	0	1	0	0	0	0
3	0	0	1	0	0	0
4	0	0	0	1	0	0
5	0	0	0	0	1	0
6	0	0	0	0	0	1
Notes: ${\overline{\sigma} }_{\text{1}}$ , ${\overline{\sigma} }_{\text{2}}$ and ${\overline{\sigma} }_{\text{3}}$ —Macro-stress in the 1, 2 and 3 direction of CFRP, respectively; ${\overline{\tau} }_{\text{12}}$ , ${\overline{\tau} }_{\text{23}}$ , ${\overline{\tau} }_{\text{13}}$ —Macro shear stress in the 12, 23 and 13 direction of CFRP, respectively.

下载: 导出CSV

表 4 CFRP薄壁结构宏观弹性参数计算方法

Table 4 Calculation method of macro elastic parameters for CFRP thin-walled structure

Loading case	Loading condition	Calculation formula
1	${\overline{\sigma }}_{{1}}$ =1	${{E}}_{{1}}=\dfrac{{\overline{\sigma }}_{{1}}}{{}{\bar{{ \varepsilon }}}_{{1}}} ， {\upsilon }_{{12}} =−\dfrac{{\bar{{ \varepsilon }}}_{{2}}}{{}{\bar{{ \varepsilon }}}_{{1}}} ， {\upsilon }_{{13}} =−\dfrac{{\bar{{ \varepsilon }}}_{{3}}}{{}{\bar{{ \varepsilon }}}_{{1}}}$
2	${\overline{\sigma }}_{{2}}$ =1	${{E}}_{{2}}=\dfrac{{\overline{\sigma }}_{{2}}}{{\bar{{ \varepsilon }}}_{{2}}} ， {\upsilon }_{{21}} =−\dfrac{{\bar{{ \varepsilon }}}_{{1}}}{{}{\bar{{ \varepsilon }}}_{{2}}} ， {\upsilon }_{{23}} =− \dfrac{{\bar{{ \varepsilon }}}_{{3}}}{{}{\bar{{ \varepsilon }}}_{{2}}}$
3	${\overline{\sigma }}_{{3}}$ =1	${{E}}_{{3}} =\dfrac{{\overline{\sigma }}_{{3}}}{{}{\bar{{ \varepsilon }}}_{{3}}}，{\upsilon }_{{31}} =− \dfrac{{}{\bar{{ \varepsilon }}}_{{1}}}{{}{\bar{{ \varepsilon }}}_{{3}}} ，{\upsilon }_{{32}}=− \dfrac{{\bar{{ \varepsilon }}}_{{2}}}{{}{\bar{{ \varepsilon }}}_{{3}}}$
4	${\overline{\tau }}_{{12}}$ =1	${{G}}_{{12}}=\dfrac{{\overline{\tau }}_{{12}}}{{\bar{\gamma }}_{{12}}}$
5	${\overline{\tau }}_{{23}}$ =1	${{G}}_{{23}}=\dfrac{{\overline{\tau }}_{{23}}}{{\bar{\gamma }}_{{23}}}$
6	${\overline{\tau }}_{{13}}=1$	${{G}}_{{13}} = \dfrac{{\overline{\tau }}_{{13}}}{{\bar{\gamma }}_{{13}}}$
Notes: E₁, E₂ and E₃—Macroscopic elastic modulus in the 1, 2 and 3 direction of CFRP, respectively; G₁₂, G₂₃ and G₁₃—Macroscopic shear modulus in the 12, 23 and 13 direction of CFRP, respectively; ${\bar \upsilon} _{ij}$ (i, j=1, 2, 3)—Poisson's ratio of CFRP in the ij direction; ${\bar\varepsilon} _i$ (i=1, 2, 3)—Strain of CFRP in the i direction; ${\bar \gamma} _{ij}$ (i, j=1, 2, 3)—Strain of CFRP in the ij direction.

下载: 导出CSV

表 5 CFRP弹性参数预测结果与试验结果对比

Table 5 Comparison of CFRP elastic parameters between numerical and experimental results

Property	${ {E} }_{ {1} }$ /GPa	${ {E} }_{ {2} }$ = ${ {E} }_{ {3} }$ /GPa	${{G}}_{{12}}={{G}}_{{13}}$ /GPa	${{G}}_{{23}}$ /GPa	${\upsilon }_{{12}}{=}{\upsilon }_{{13}}$	${\upsilon }_{{23}}$
Test	131.5	7.6	—	—	0.29	—
RVE	132.6	7.5	4.3	3.5	0.29	0.39
Error/%	2.13	9.21	—	—	0	—

下载: 导出CSV

表 6 CFRP多尺度损伤模型输入参数

Table 6 Input parameters of CFRP multi-scale damage model

Parameter	Value	Parameter	Value
${E}_{\text{1} }$ /MPa	132600	${E}_{\text{f}\text{1}}$ /MPa	185000
${E}_{\text{2} }$ /MPa	7500	${E}_{\text{f}\text{2}}$ /MPa	13000
${E}_{\text{3} }$ /MPa	7500	${\text{X}}_{\text{f}}^{\text{0,}\text{T}}$ /MPa	3470
${\nu}_{\text{12}}$	0.29	${\text{X}}_{\text{f}}^{\text{0,}\text{C}}$ /MPa	2100
${\nu}_{\text{13}}$	0.29	${E}_{\text{m}}$ /MPa	2600
${\nu}_{\text{23}}$	0.39	${\text{Y}}_{\text{m}}^{\text{0,}\text{T}}$ /MPa	77
${G}_{\text{12}}$ /MPa	4300	${\text{Y}}_{\text{m}}^{\text{0,}\text{C}}$ /MPa	121
${G}_{\text{13}}$ /MPa	4300	$\text{γ}$	1.5
${G}_{\text{23}}$ /MPa	3500	$\rho$ /(t·mm⁻³)	1.4×10⁻⁹
Notes: $\rm X_f^{0,T}$ and $\rm X_f^{0,C}$ —Longitudinal tensile strength and compressive strength of T300 fiber, respectively; $\rm Y_m^{0,T}$ and $\rm Y_m^{0,C}$ —Final tensile strength and compressive strength of matrix, respectively; $\gamma$ —Damage shape parameter of matrix; p—Beta damping parameter of CFRP.

下载: 导出CSV

表 7 CFRP层间失效模型输入参数

Table 7 Input parameters of CFRP inter-laminar failure model

Description	Variable	Value
Damage initiation/MPa	${{t}}_{\text{n}}^{\text{0}}$	49.2
	${{t}}_{\text{s}}^{\text{0}}$	59.5
	${{t}}_{\text{t}}^{\text{0}}$	59.5
Fracture energies/(J·m⁻²)	${{G}}_{\text{n}}^{\text{C}}$	490
	${{G}}_{\text{s}}^{\text{C}}$	1060
	${{G}}_{\text{t}}^{\text{C}}$	1060
BK	${\eta}$	2.284
Notes: ${{t}}_{\text{n}}^{\text{0}}$ —Maximum nominal stress in the normal-only mode; ${{t}}_{\text{s}}^{\text{0}}$ —Maximum nominal stress in the first shear direction (for a mode that involves separation only in this direction); ${{t}}_{\text{t}}^{\text{0}}$ —Maximum nominal stress in the second shear direction (for a mode that involves separation only in this direction); ${{G}}_{\text{n}}^{\text{C}}$ —Ttype I critical fracture energies in n direction; ${{G}}_{\text{s}}^{\text{C}}$ — Type II critical fracture energies in n direction; ${{G}}_{\text{t}}^{\text{C}}$ —Critical fracture energies in t direction; $\eta$ —A bonding attribute parameter.

下载: 导出CSV

表 8 CFRP薄壁结构耐撞性能指标结果对比

Table 8 Comparison of crashworthiness index results for CFRP thin-walled structure

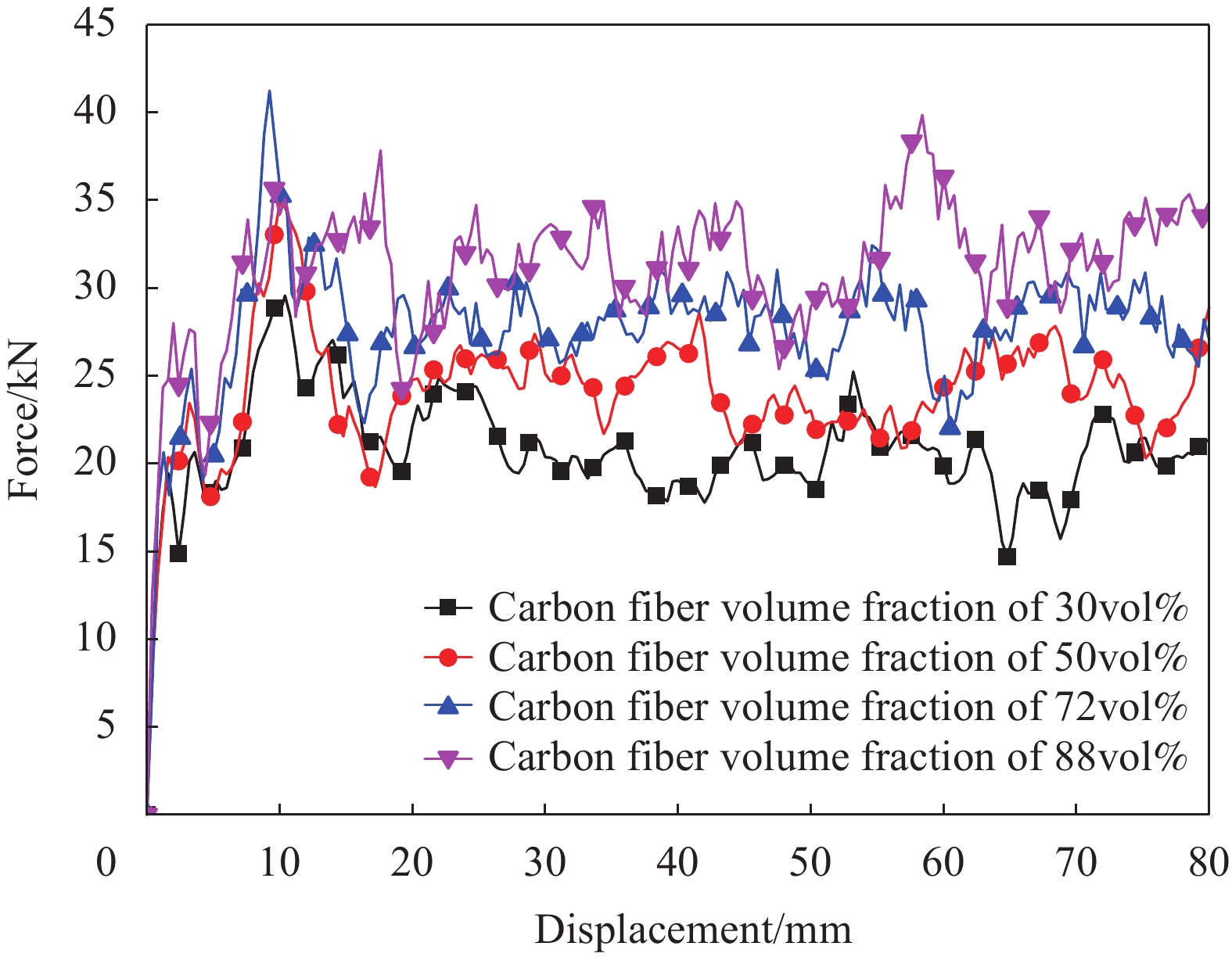
Property	F_p/kN	W_e/kJ	W_s/(J·g⁻¹)	F_m/kN	E_c
Experimental	35.0	2.230	48.02	27.88	0.80
Simulation	37.8	2.218	47.76	27.73	0.73
Error/%	8	0.54	0.54	0.5	7.9
Notes: F_p—Maximum peak force that occurs during the entire crushing process; W_e—Total energy absorbed by the structural component during the impact process; W_s—Amount of energy absorbed by energy absorbing structure per unit mass; F_m—Average force is the energy absorbed during the impact process per unit distance; E_c—Ratio of the average force to the maximum peak force.

下载: 导出CSV

表 9 不同铺层角度的CFRP薄壁圆管耐撞性能指标结果

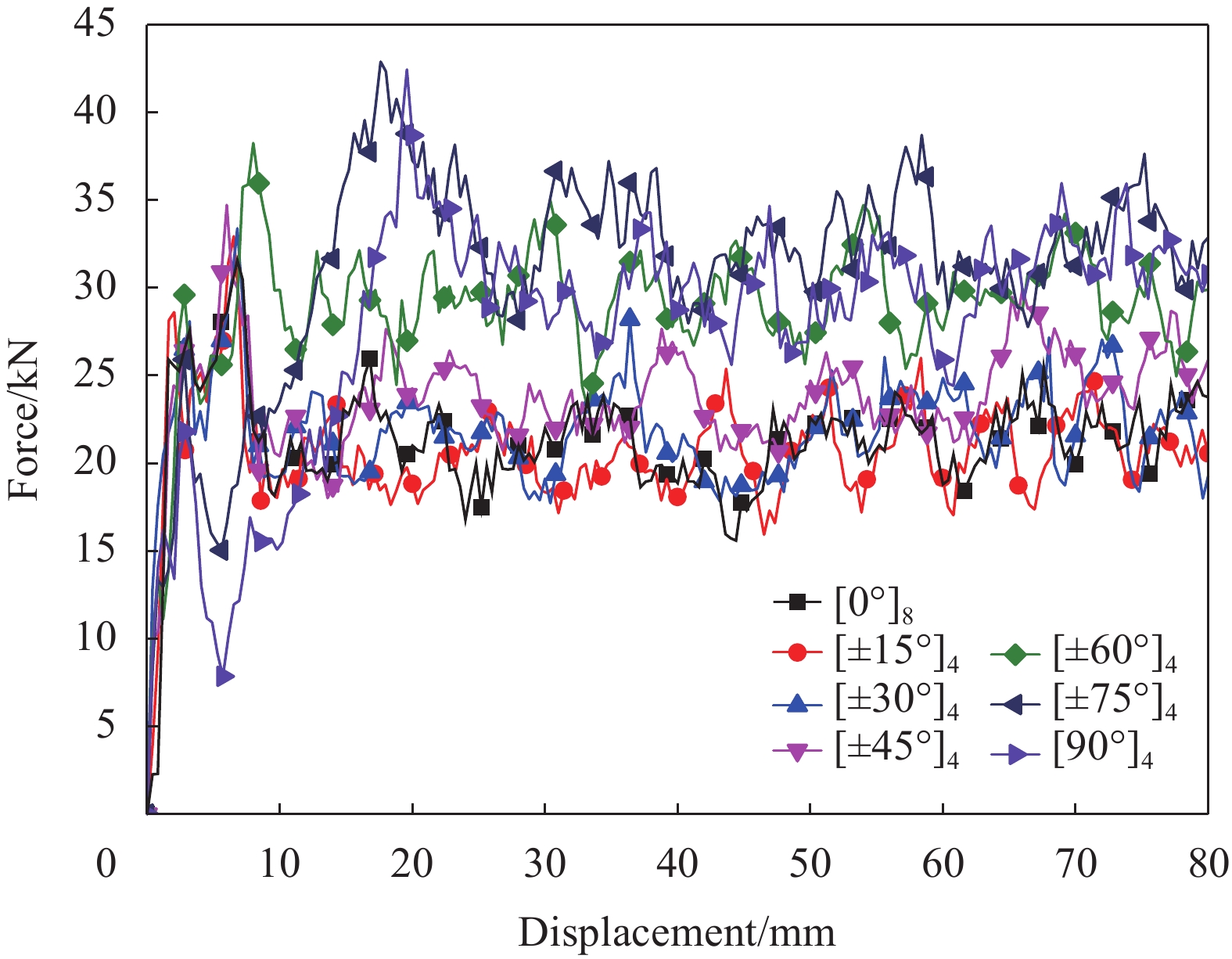
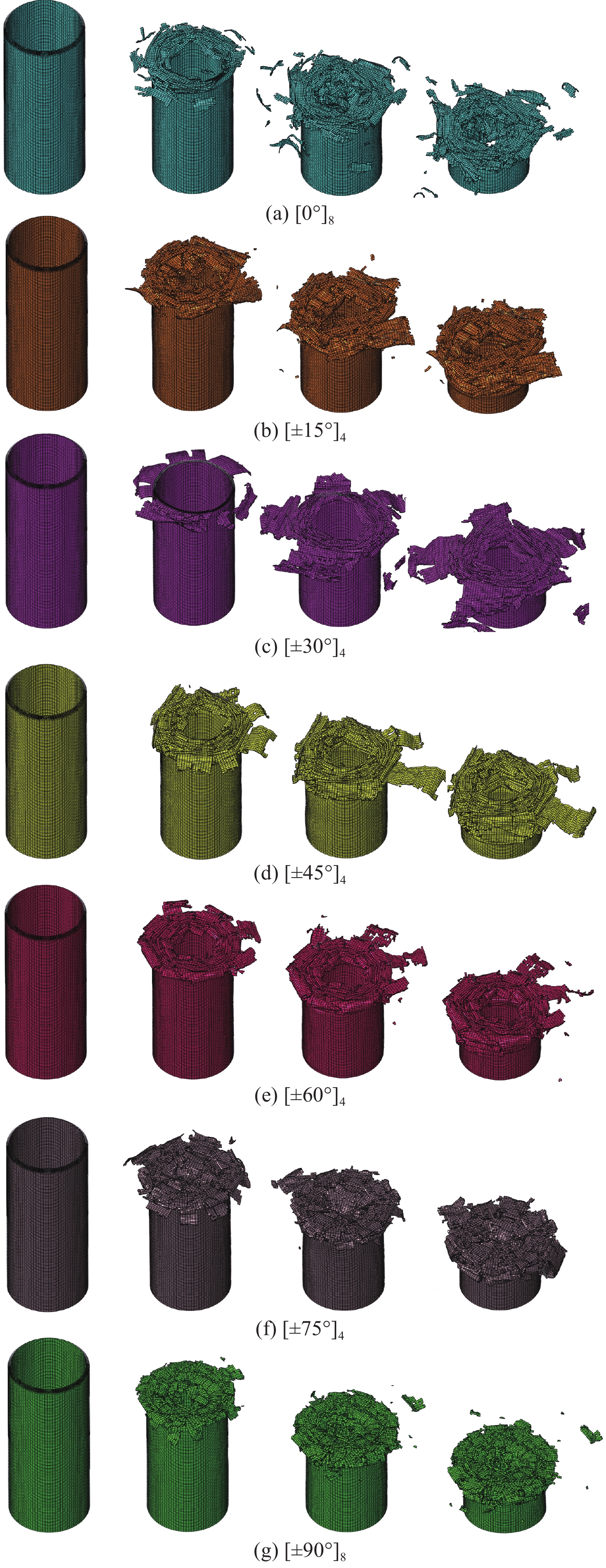
Table 9 Crashworthiness index results of CFRP tubes with different fiber orientations

Ply angle	F_p/kN	W_e/J	W_s/(J·g⁻¹)	F_m/kN	E_c
[0°]₈	31.6	1703	36.7	21.3	0.674
[±15°]₄	32.9	1653	35.6	20.7	0.629
[±30°]₄	33.4	1754	37.8	21.9	0.656
[±45°]₄	34.7	1899	40.9	23.7	0.683
[±60°]₄	38.3	2316	49.9	29.0	0.757
[±75°]₄	42.9	2496	53.7	31.2	0.727
[90°]₈	42.4	2236	48.1	28.0	0.660

下载: 导出CSV

表 10 不同体积分数的CFRP薄壁圆管耐撞性能指标结果

Table 10 Crashworthiness indicators of CFRP tubes with different volume fractions

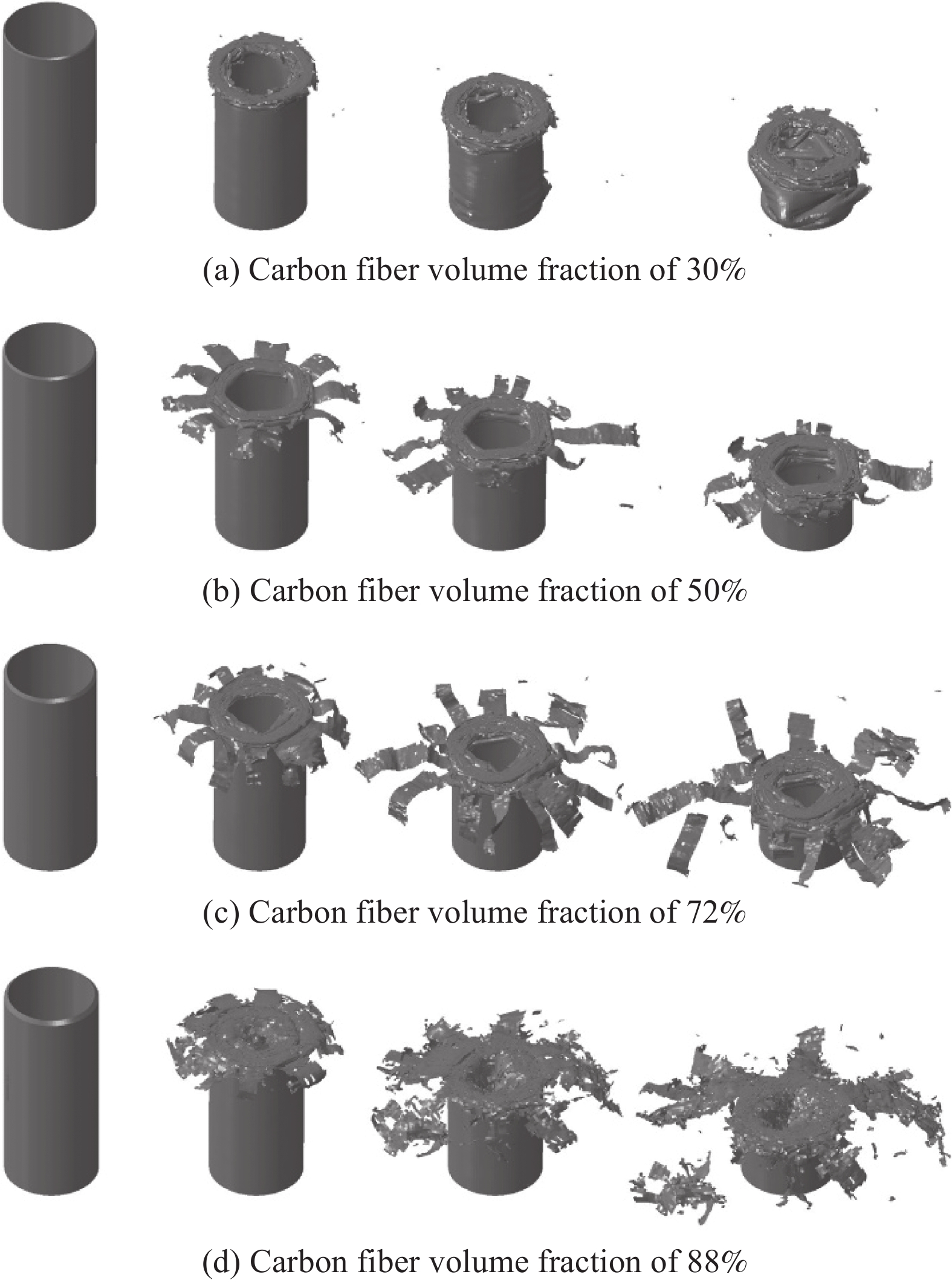
Volume fraction/vol%	m/g	F_p/kN	W_e/J	W_s/(J·g⁻¹)	F_m $/$ kN	E_c
30	38.95	29.8	1649	42.3	20.6	0.692
50	42.52	34.8	1933	45.5	24.2	0.694
72	46.44	37.8	2218	47.8	27.7	0.733
88	49.56	39.9	2494	50.3	31.2	0.781
Note: m—Mass of carbon fiber.

下载: 导出CSV

参考文献(26)

[1]	HA N S, LU G. A review of recent research on bio-inspired structures and materials for energy absorption applications[J]. Composites Part B: Engineering,2020,181:107496. DOI: 10.1016/j.compositesb.2019.107496
[2]	HA N S, LU G. Thin-walled corrugated structures: A review of crashworthiness designs and energy absorption characteristics[J]. Thin-Walled Structures,2020,157:106995. DOI: 10.1016/j.tws.2020.106995
[3]	ZHU G, LIAO J, SUN G, et al. Comparative study on metal/CFRP hybrid structures under static and dynamic loading[J]. International Journal of Impact Engineering,2020,141:103509.
[4]	MAMALIS A G, MANOLAKOS D E, IOANNIDIS M B, et al. On the response of thin-walled CFRP composite tubular components subjected to static and dynamic axial compressive loading: Experimental[J]. Composite Structures,2005,69(4):407-420. DOI: 10.1016/j.compstruct.2004.07.021
[5]	ZHU G, SUN G, LI G, et al. Modeling for CFRP structures subjected to quasi-static crushing[J]. Composite Structures,2018,184:41-55. DOI: 10.1016/j.compstruct.2017.09.001
[6]	LIU Q, OU Z, MO Z, et al. Experimental investigation into dynamic axial impact responses of double hat shaped CFRP tubes[J]. Composites Part B: Engineering,2015,79(15):494-504.
[7]	GRECO F, LUCIANO R. A theoretical and numerical stability analysis for composite micro-structures by using homogenization theory[J]. Composites Part B: Engineering,2011,42(3):382-401. DOI: 10.1016/j.compositesb.2010.12.006
[8]	ZHAO Z, DANG H, ZHANG C, et al. A multi-scale modeling framework for impact damage simulation of triaxially braided composites[J]. Composites Part A: Applied Science and Manufacturing,2018,110:113-125. DOI: 10.1016/j.compositesa.2018.04.020
[9]	MELRO A R, CAMANHO P P, ANDRADE P F M, et al. Micromechanical analysis of polymer composites reinforced by unidirectional fibres: Part I–Constitutive modelling[J]. International Journal of Solids and Structures,2013,50:1897-1905. DOI: 10.1016/j.ijsolstr.2013.02.009
[10]	GE L, LI H, LIU B, et al. Multi-scale elastic property prediction of 3D five-directional braided composites considering pore defects[J]. Composite Structures,2020,244:112287. DOI: 10.1016/j.compstruct.2020.112287
[11]	XIONG X, HUA L, MIAO M, et al. Multi-scale constitutive modeling of natural fiber fabric reinforced composites[J]. Composites Part A: Applied Science & Manufacturing,2018,115:383-396.
[12]	CALNERYTE D, BARAUSKAS R. Multi-scale evaluation of the linear elastic and failure parameters of the unidirectional laminated textiles with application to transverse impact simulation[J]. Composite Structures,2016,142:325-334. DOI: 10.1016/j.compstruct.2016.01.104
[13]	TAO W, LIU Z, ZHU P, et al. Multi-scale design of three dimensional woven composite automobile fender using modified particle swarm optimization algorithm[J]. Composite Structures,2017,181:73-83. DOI: 10.1016/j.compstruct.2017.08.065
[14]	HA S K, JIN K K, HUANG Y. Micro-mechanics of failure (MMF) for continuous fiber reinforced composites[J]. Journal of Composite Materials,2008,42(18):1873-1895. DOI: 10.1177/0021998308093911
[15]	JIN K K, HWAN Y C, LEE Y H, et al. Distribution of micro stresses and interfacial tractions in unidirectional compo-sites[J]. Journal of Composite Materials,2008,42(18):1825-1849. DOI: 10.1177/0021998308093909
[16]	LIAO B, TAN H, ZHOU J, et al. Multi-scale modelling of dynamic progressive failure in composite laminates subjected to low velocity impact[J]. Thin-Walled Structures,2018,131:695-707. DOI: 10.1016/j.tws.2018.07.047
[17]	LANGSETH M, HOPPERSTAD O S. Static and dynamic axial crushing of square thin-walled aluminium extrusions[J]. International Journal of Impact Engineering,1996,18(7-8):949-968. DOI: 10.1016/S0734-743X(96)00025-5
[18]	ASTM. Standard test method for tensile properties of polymer matrix composite materials: ASTM D3039[S]. West Conshohcken: ASTM, 2000.
[19]	李星, 关志东, 刘璐, 等. 复合材料跨尺度失效准则及其损伤演化[J]. 复合材料学报, 2013, 30(2):152-158. DOI: 10.13801/j.cnki.fhclxb.2013.02.003 LI Xing, GUAN Zhidong, LIU Lu, et al. Composite multiscale failure criteria and damage evolution[J]. Acta Materiae Compositae Sinica,2013,30(2):152-158(in Chinese). DOI: 10.13801/j.cnki.fhclxb.2013.02.003
[20]	LI S, WONGSTO A. Unit cells for micromechanical analyses of particle-reinforced composites[J]. Mechanics of Materials,2004,36(7):543-572. DOI: 10.1016/S0167-6636(03)00062-0
[21]	赖卫清, 王秀梅, 辛亮亮, 等. 基于RVE方法的二维机织复合材料弹性性能预测[J]. 玻璃钢/复合材料, 2019(6):64-72. LAI Weiqing, WANG Xiumei, XIN Liangliang, et al. Prediction of elastic properties of 2D woven composites based on RVE method[J]. Composites Science and Engineering,2019(6):64-72(in Chinese).
[22]	王新峰. 机织复合材料多尺度渐进损伤研究[D]. 南京: 南京航空航天大学, 2007. WANG Xinfeng. Multi-scale analyses of damage evolution in woven composite materials[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2007(in Chinese).
[23]	HUANG Y, XU L, HA S K. Prediction of three-dimensional composite laminate response using micromechanics of failure[J]. Journal of Composite Materials,2012,46(19-20):2431-2442. DOI: 10.1177/0021998312449888
[24]	XU L, JIN C Z, HA S K. Ultimate strength prediction of braided textile composites using a multi-scale approach[J]. Journal of Composite Materials,2015,49(4):477-494. DOI: 10.1177/0021998314521062
[25]	WANG L, WANG B, WEI S, et al. Prediction of long-term fatigue life of CFRP composite hydrogen storage vessel based on micromechanics of failure[J]. Composites Part B: Engineering,2016,97:274-281.
[26]	薛亚红, 陈继刚, 闫世程, 等. 二维机织复合材料力学分析中的周期性边界条件研究[J]. 纺织学报, 2016, 37(9):70-77. DOI: 10.13475/j.fzxb.20150800808 XUE Yahong, CHEN Jigang, YAN Shicheng, et al. Periodic boundary conditions for mechanical property analysis of 2D woven fabric composite[J]. Journal of Textile Research,2016,37(9):70-77(in Chinese). DOI: 10.13475/j.fzxb.20150800808

施引文献(7)

期刊类型引用(2)

1.	柯俊，李志虎，秦玉林. 金属主簧-复合材料副簧复合刚度计算模型. 汽车实用技术. 2021(05): 39-43 . 百度学术
2.	曾婷，王咏莉，尹忠旺，苏婷慧，师志峰，饶猛. 端面凸轮下压机构支承轴承冲击失效分析及优化（英文）. 机床与液压. 2017(24): 37-42 . 百度学术