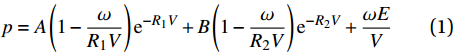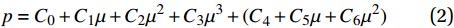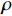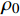纤维织物袋的内爆响应与抗爆性能

解江; 高斌元; 蒋逸伦; 潘汉源; 冯振宇

doi:10.13801/j.cnki.fhclxb.20220630.003

纤维织物袋的内爆响应与抗爆性能

解江^{1, 2, ,},
高斌元^{1, 2},
蒋逸伦^{1, 2},
潘汉源^{1, 2},
冯振宇^{1, 2}

1.
中国民航大学　安全科学与工程学院，天津 300300
2.
民航航空器适航审定技术重点实验室，天津 300300

详细信息

通讯作者:
解江，博士，副研究员，硕士生导师，研究方向为复合材料冲击动力学　E-mail: xiejiang5@126.com

中图分类号: O383
计量
- 文章访问数: 1128
- HTML全文浏览量: 618
- PDF下载量: 44
出版历程
- 收稿日期: 2022-04-25
- 修回日期: 2022-06-11
- 录用日期: 2022-06-17
- 网络出版日期: 2022-06-30
- 刊出日期: 2023-04-14

Implosion response and anti-explosion performance of fabric bags

XIE Jiang^{1, 2, ,},
GAO Binyuan^{1, 2},
JIANG Yilun^{1, 2},
PAN Hanyuan^{1, 2},
FENG Zhenyu^{1, 2}

1.
College of Safety Science and Engineering, Civil Aviation University of China, Tianjin 300300, China
2.
Key Laboratory of Civil Aircraft Airworthiness Technology, CAAC, Tianjin 300300, China

HTML全文

摘要

摘要: 针对小型简易爆炸装置，设计了芳纶和超高分子量聚乙烯(UHMWPE)纤维织物防爆袋，开展了织物袋内爆试验，同时建立了织物袋内爆数值分析模型。从外部超压和临界厚度两个角度对比了两种材料织物袋的抗爆能力，分析了初始内爆距离和织物袋厚度对织物袋抗爆性能的影响。结果表明：内爆载荷下织物袋的主要失效模式为中心区域破孔和封口拉链失效。在20~100 g药量范围内，织物袋的临界厚度与药量近似呈线性增长关系。同一药量下，芳纶织物袋的临界厚度明显大于UHMWPE织物袋，在不考虑爆炸火球对织物烧蚀的影响时，UHMWPE织物袋具有更好的抗爆效果。厚度相同时，芳纶织物袋外部的超压峰值更小，表明芳纶织物袋的超压衰减能力更强。随着织物袋初始内爆距离的增大，织物袋的临界厚度减小。织物袋外部一定范围内存在高于人体能够承受的超压，以30 g TNT、3 mm厚芳纶织物袋为例，在距织物袋中心665 mm处的超压为34.2 kPa，超过鼓膜损伤阈值。
- 纤维织物袋 /
- 内爆载荷 /
- 动态响应 /
- 冲击波超压 /
- 有限元模型
Abstract: Aramid and ultra-high molecular weight polyethylene (UHMWPE) fiber fabric bags were designed for the disposal of improvised explosive device (IED). The implosion test was carried out, and a finite element (FE) model of fabric bag implosion was established. The anti-explosion abilities of the two fabric bags were compared from the perspectives of external overpressure and critical thickness, and the effects of the initial implosion distance and the thickness of the fabric bag on the anti-explosion ability were analyzed. The results show that the main failure modes of the fabric bag under implosion load are broken hole in the center area and the failure of the sealing zipper. The critical thickness of the fabric bag increases approximately linearly with the charge in the range of 20-100 g TNT charge. The critical thickness of the aramid fabric bag is significantly larger than that of the UHMWPE fabric bag when the charges are the same. The UHMWPE fabric bag has a better anti-explosion ability without considering the influence of the explosion fireball. When the thicknesses are the same, the overpressure outside the aramid fabric bag is smaller, indicating that the overpressure attenuation ability of the aramid fabric bag is better. As the initial implosion distance of the fabric bag increases, the critical thickness decreases. There is an overpressure higher than the human body which can withstand within a certain range outside the fabric bag. Taking the 30 g TNT, 3 mm aramid fabric bag condition as an example, the overpressure at 665 mm from the center of the fabric bag is 34.2 kPa, exceeding the eardrum damage threshold.
- fiber fabric bag /
- implosion load /
- dynamic response /
- shock wave overpressure /
- FEM

HTML全文

近年来，国际上发生多起民航客机遭遇劫炸机事件。炸弹在飞机内部爆炸危害性极大，轻则造成机体和乘客受伤，重则导致飞机解体。FAA AC 25.795-6^[1]标准中给出了一种建议的符合性方法，在客舱内发现疑似爆炸物时可以采用炸弹包容装置来减小爆炸对飞机的影响。

现有的以金属材料为主的抗爆容器因其庞大的体积和质量，会为民机带来巨大经济负担，不适用于民用飞机。随着材料科学的发展，轻质高强的纤维复合材料在抗爆领域得到应用。Fallah等^[2]对超高分子量聚乙烯(Ultra-high molecular weight polyethylene，UHMWPE)和少量树脂制成的Dyneema HB26板进行了爆炸冲击试验研究，发现Dyneema板出现了永久变形、边缘屈曲、分层、迎爆面熔化和边缘收缩等失效行为。Gargano等^[3]对碳纤维和玻璃纤维为增强体，聚酯和乙烯基酯为基体的层合板进行了爆炸冲击试验，结果表明玻璃纤维层合板具有更好的抗分层和断裂能力，乙烯基酯的层合板抗损伤能力更强。Zhang等^[4-5]通过试验与仿真分析研究了UHMWPE纤维柔性防爆墙的爆炸响应与失效行为，结果表明柔性墙能够有效抵抗小当量爆炸物，防爆墙背爆面存在绕射波和透射波，墙后压力与墙的高度、厚度及比例距离相关。赵敏等^[6]通过试验研究了内部含有碳纤维增强复合材料(CFRP)片材的多层UHMWPE织物的抗爆性能，发现嵌入CFRP片能够明显提升织物对雷管破片的阻挡效果。前期研究^[7-9]对芳纶、碳纤和UHMWPE纤维机织物进行了爆炸冲击试验和数值分析，获得了爆炸载荷下纤维织物的失效模式及影响织物抗爆性能的因素。

在织物材料防爆结构方面，Chun等^[10]研究了空气冲击波作用下柔性帐篷结构的动态响应，模拟了冲击波在柔性结构内部的传播过程，并与试验进行了对比，结果表明基于计算流体力学和结构动力学的流固耦合方法能够用来分析内爆载荷下柔性结构的动态响应过程。Markert等^[11]、Zangani等^[12]、Giovanelle^[13]采用高性能纤维织物研发了一种名为“飞行包裹”的柔性防爆袋，并通过内爆试验验证了其防护效果，这种抗爆袋能够完全包容爆炸产生的冲击波和高速破片，在超当量防护时，抗爆结构自身不会产生破片，已在民用飞机上得到应用。Polak等^[14-15]对聚乙烯和对位芳纶制成的防爆围栏进行了冲击波和破片联合冲击试验，并在中间增加了金属、橡胶和塑料内衬，研究内衬对防爆围栏抗爆能力的加强作用，结果表明金属内衬能够明显提升防爆围栏的抗爆性能。Zhu等^[16]对水障/UHMWPE纤维层的圆柱形抗爆结构进行了内爆试验，结果表明水障和UHMWPE纤维层均能有效地降低爆炸破片的速度。Masi等^[17]在飞机客舱内饰中增加了Kevlar织物层，并进行了爆炸冲击仿真分析，发现Kevlar织物的加入提高了机身的抗爆能力。蔡军锋等^[18-19]将聚氨酯泡沫材料和UHMWPE板材作为内衬，薄钢板作为主体框架，设计了一种防爆箱，试验表明防爆箱具有较好的抗爆效果。

目前，有关柔性纤维织物抗爆容器的研究主要通过开展爆炸试验，并且集中在以削弱冲击波和破片威力为目的的抗爆墙、防爆围栏和防爆罐方面，缺少对包容冲击波和破片的柔性抗爆结构的研究。

针对小当量简易爆炸装置，提出一种柔性纤维织物防爆袋，利用比强度、比模量高的芳纶和UHMWPE织物制成的织物袋来包容爆轰产物和冲击波，通过开展织物袋内爆试验和数值模拟，研究内爆载荷下柔性纤维织物袋的动态响应和失效行为，为织物防爆袋的设计提供支持。

1. 纤维织物袋内爆试验

1.1 试件制备

织物袋由芳纶纤维织物或UHMWPE纤维织物制成，织物的主要物理参数见表1。织物袋一端通过加捻的芳纶纤维缝合进行封口，另一端通过两条拉链封口，并采用两个安全带扣对封口进一步加固，如图1所示。

表 1 各织物的主要物理参数

Table 1. Main physical parameters of fabrics

Material	Aramid	UHMWPE
Grade	F-268	ZTZ 24
Yarn fineness/tex	166	126±10
Yarn body density/(g·cm⁻³)	1.44	0.97
Yarn breaking elongation/%	≥3.2	3-3.5
Yarn tensile modulus/GPa	≥125	105-110
Fabric thickness/mm	0.3	0.55
Weave count/(yarns·(10 cm)⁻¹)	65×65	87×87
Areal density/(g·m⁻²)	210-220	235-245
Note: UHMWPE—Ultra-high molecular weight polyethylene.

下载: 导出CSV

| 显示表格

图 1 织物袋实物图

Figure 1. Fabric bag

下载: 全尺寸图片幻灯片

1.2 试验方案

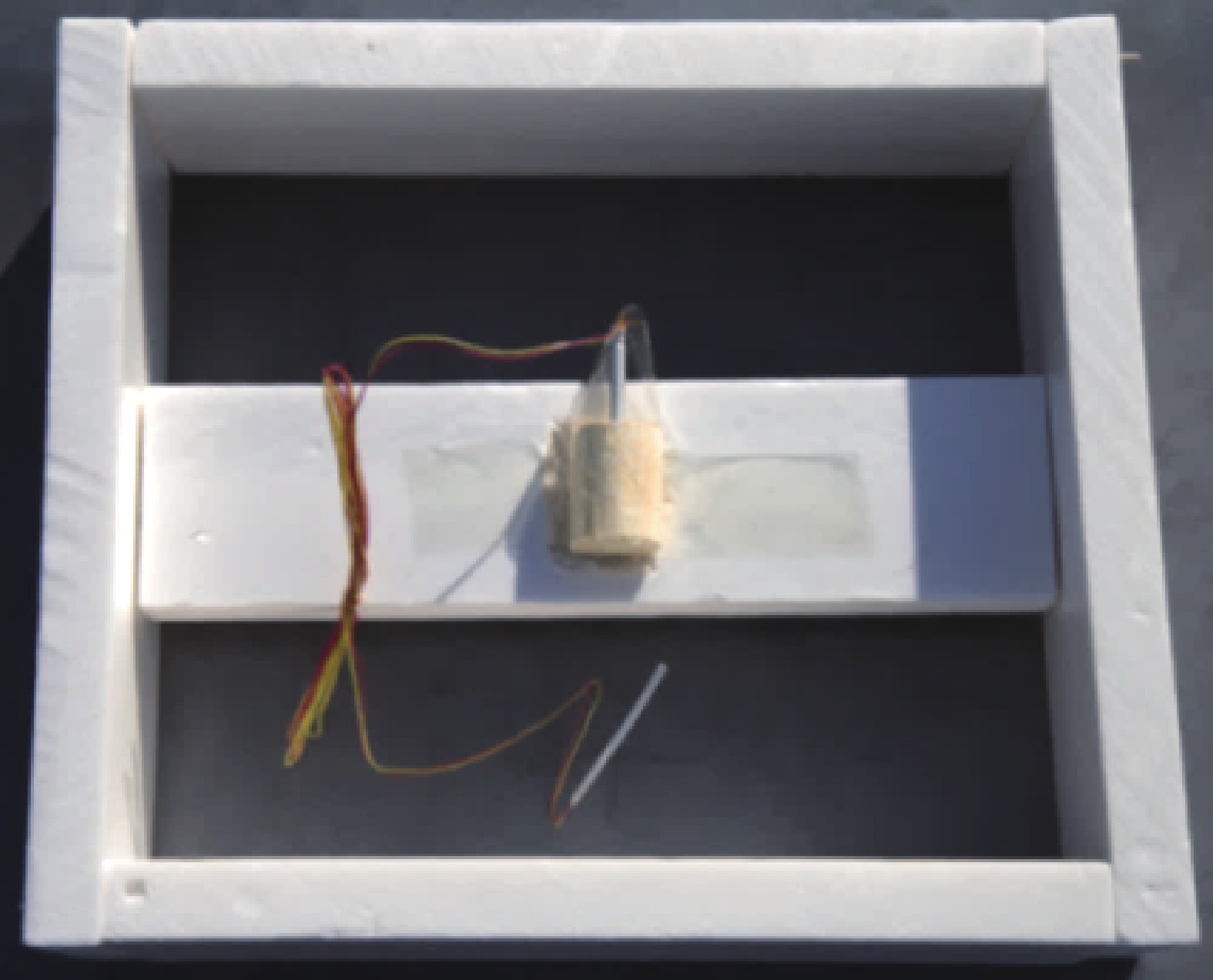
试验中将织物袋悬挂在钢架上，为了使炸药置于织物袋中心，用泡沫板制做了如图2所示药托，药托的长宽高分别为40 cm、40 cm和10 cm。泡沫板选用厚度3 cm的低密度泡沫，爆炸过程中泡沫板迅速熔化、解体，对内部冲击波的影响极小，可忽略不计。采用3个超压传感器测量织物袋外部不同位置处的超压，传感器厂商为PCB，型号为PCB137 B24 B，量程为0~1725 kPa，编号分别为S1、S2和S3。采用1台Phatron SA5高速摄像捕获织物袋的动态响应过程，试验系统如图3所示。

图 2 三硝基甲苯(TNT)放置方式

Figure 2. Placement of tri-nitro-toluene (TNT)

下载: 全尺寸图片幻灯片

图 3 试验系统

Figure 3. Experimental setup

下载: 全尺寸图片幻灯片

1.3 试验结果

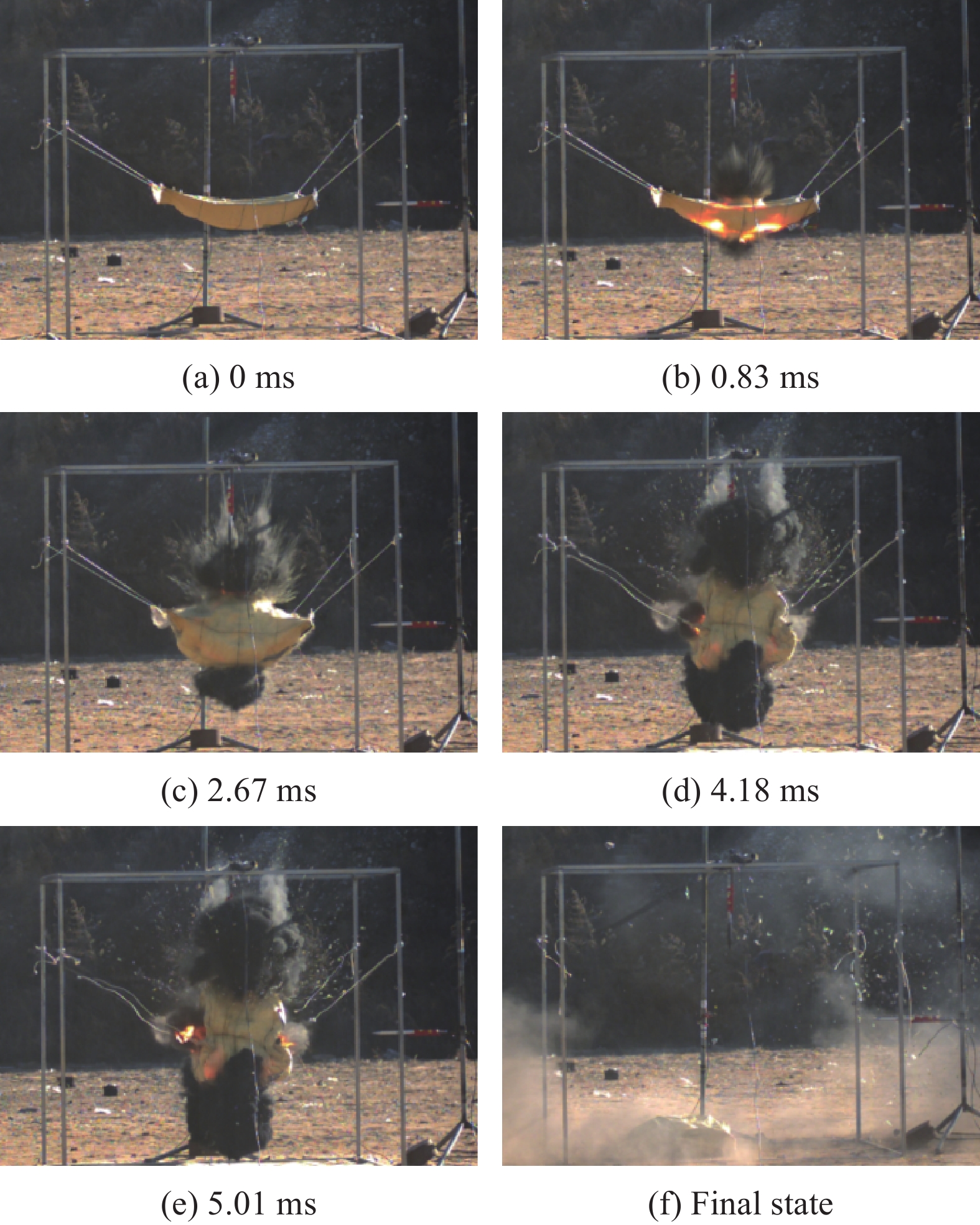
图4所示为20 g三硝基甲苯(TNT，梯恩梯炸药)内爆载荷作用下，1.2 mm厚芳纶织物袋的动态响应过程。以能够观察到织物袋变形的前一帧时刻点作为起爆零时刻点，0.83 ms时刻，在冲击波和爆轰产物作用下织物袋中心出现破孔，爆轰产物从破孔中冲出，织物袋上方出现大量浓烟；此后随着冲击波在织物袋内部传播，织物袋不断膨胀；4.18 ms时刻，织物袋底部缝合线断裂，出现开口；5.01 ms时刻，织物袋拉链失效，火焰从开口处冒出，连接织物袋的细绳断裂，最终织物袋落在地面。

图 4 20 g TNT作用下，1.2 mm厚芳纶织物袋的动态响应过程

Figure 4. Dynamic response process of 1.2 mm aramid fabric bag under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

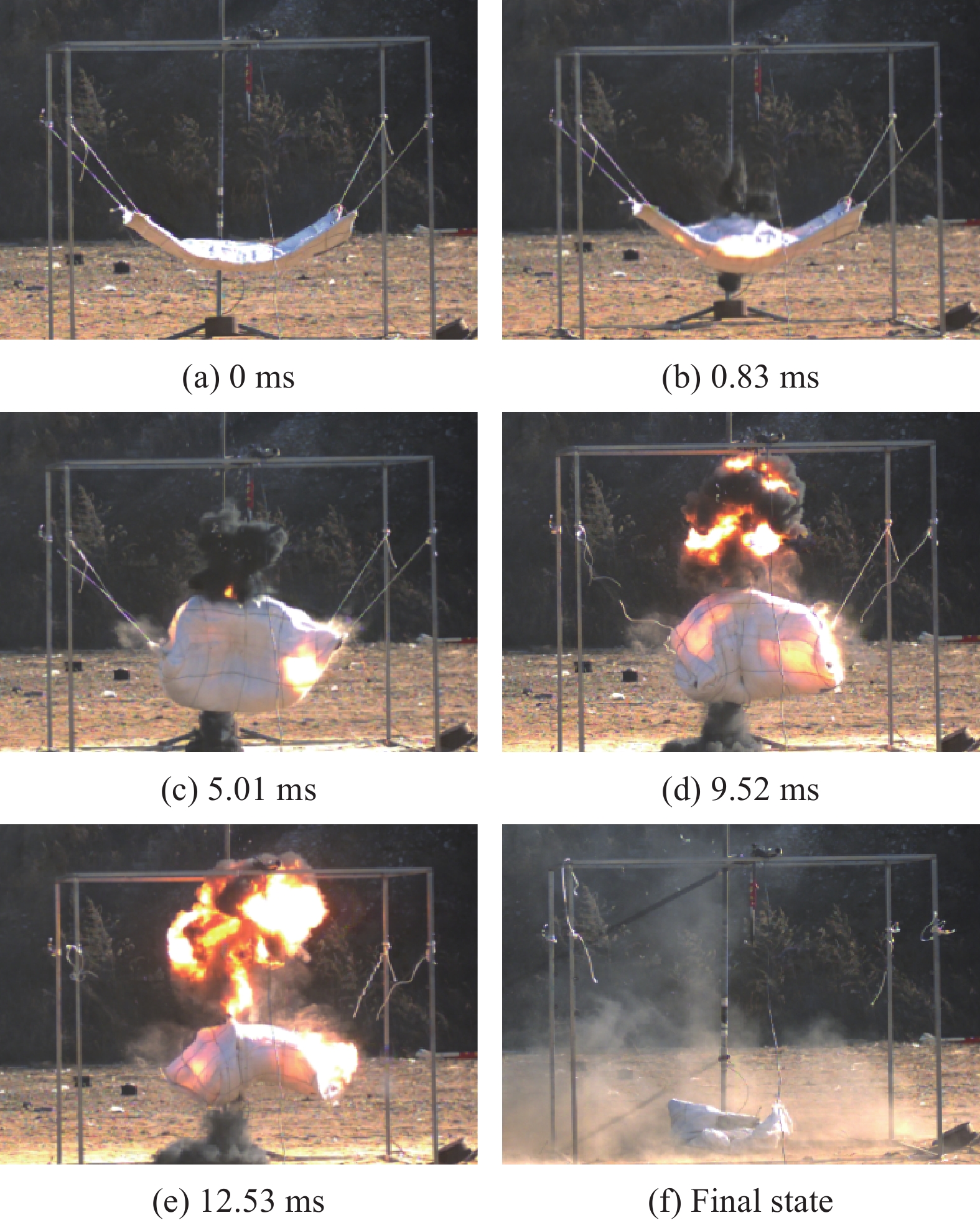
图5为20 g TNT内爆载荷作用下，2.2 mm厚UHMWPE织物袋的动态响应过程。0.83 ms时刻，在冲击波和爆轰产物作用下织物袋中心出现破孔，爆轰产物从破孔中冲出，织物袋上方出现大量浓烟；此后随着冲击波在织物袋内部传播，织物袋不断膨胀，从外面可以明显地看到内部的爆炸火球；在爆炸火球烧蚀作用下，破孔尺寸不断增大，9.52 ms时刻，织物袋膨胀到最大体积，破孔处伴随有火焰冒出。12.53 ms时刻，织物袋封口拉链完全失效，火焰和爆轰产物从封口处喷出，随着内部压力的外泄，织物袋形状趋于扁平状，最终掉落在地上。

图 5 20 g TNT作用下，2.2 mm厚UHMWPE织物袋的动态响应过程

Figure 5. Dynamic response process of 2.2 mm UHMWPE fabric bag under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

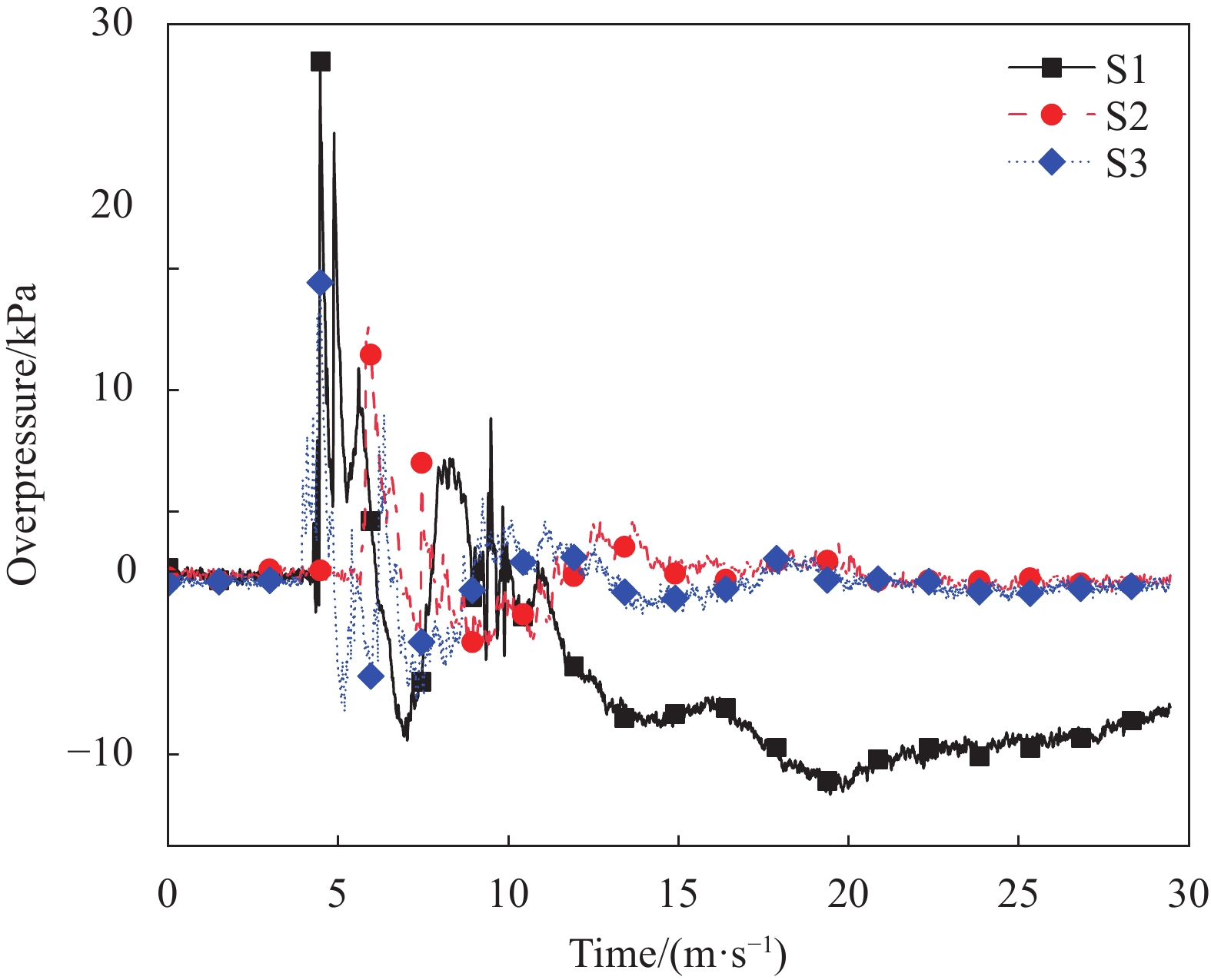
试验中用3个超压传感器测量了织物袋外部不同位置处的冲击波超压，其中S1位于织物袋中心正上方700 mm处，S2位于织物袋封口一侧距中心1200 mm处，S3位于织物袋侧面距中心600 mm处。图6为超压传感器测得的织物袋外部3个测点的超压曲线。试验中传感器与雷管同时触发，其中雷管触发至起爆需要一段时间，起爆时刻晚于传感器记录开始时刻。由于试验中没使用触发探针，未考虑零时刻点，本文只关注织物袋外部的超压峰值。

图 6 织物袋外部3个测点超压随时间的变化曲线

Figure 6. Variation curves of overpressure with time at three measuring points outside the fabric bag

下载: 全尺寸图片幻灯片

从上述试验发现，织物袋的失效模式主要为中心区域破孔和封口拉链失效。在织物防爆袋的设计中应重点关注其厚度和封口强度。

2. 织物袋内爆数值分析模型的建立与验证

2.1 织物袋数值模型的建立

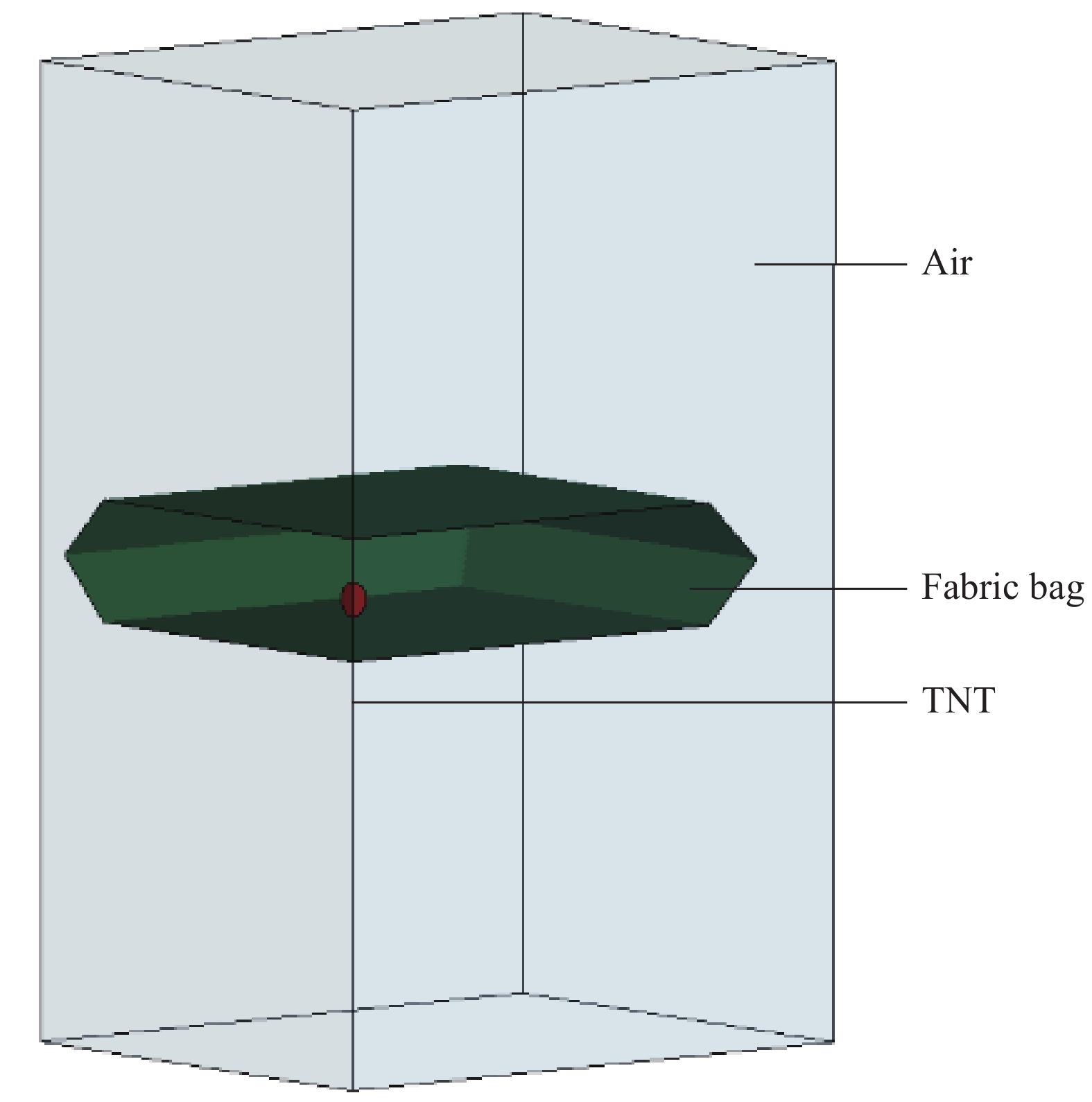
不考虑缝合线和拉链，将织物袋简化为各方向等强度，参考试验设计，给织物袋赋予一定形状，为了提高计算效率，建立了如图7所示的1/4有限元模型。织物袋在平铺状态下的尺寸为900 mm×800 mm，在赋予形状后，织物袋上下两个面之间的距离为100 mm。

图 7 织物袋有限元模型

Figure 7. Finite element model of fabric bag

下载: 全尺寸图片幻灯片

在计算分析中，空气和TNT采用ALE算法，织物采用LAGRANGE算法，ALE材料和LAGRANGE材料之间建立流固耦合实现压力在介质中的传播。炸药采用高能炸药材料模型，爆轰产物的状态方程采用JWL方程，其形式为

$p=A\left(1-\frac{\omega }{{R}_{1}V}\right){\mathrm{e}}^{-{R}_{1}V}+B\left(1-\frac{\omega }{{R}_{2}V}\right){\mathrm{e}}^{-{R}_{2}V}+\frac{\omega E}{V}$

(1)

式中：p为压力；系数A、B、R₁、R₂、ω为表征炸药特性的常数；E为单位体积的内能；V为相对体积。TNT相关参数见表2。

表 2 TNT参数

Table 2. Parameters of TNT

Parameter	Value
Density/(kg·cm⁻³)	1.63×10³
Detonation velocity/(m·s⁻¹)	6930
Detonation pressure/GPa	21
A/GPa	374
B/GPa	3.74
R₁	4.15
R₂	1.4
ω	0.35
E₀/(MJ·m⁻³)	7×10³
Notes: A, B, R₁, R₂, ω—Constants characterizing TNT properties; E₀—Detonation energy per unit volume.

下载: 导出CSV

| 显示表格

空气采用空材料模型和线性多项式状态方程描述。状态方程为

$p={C}_{0}+{C}_{1}\mu +{C}_{2}{\mu }^{2}+{C}_{3}{\mu }^{3}+{(C}_{4}+{C}_{5}\mu +{C}_{6}{\mu }^{2})$

(2)

$\mu =\frac{\rho }{{\rho }_{0}}-1$

(3)

式中：μ为空气密度相关函数；C₀~C₆为常数； $\rho$ 为当前密度； ${\rho }_{0}$ 为参考密度。各相关系数值见表3。

表 3 空气参数

Table 3. Parameters of air

Parameter	Value
Density/(kg·m⁻³)	1.29
C₄	0.4
C₅	0.4
C₁, C₂, C₃, C₆	0
E₀/(MJ·m⁻³)	0.25
Notes: C₀-C₆—Polynomial equation coefficients.

下载: 导出CSV

| 显示表格

采用LS-DYNA中的干布模型模拟织物，该模型将织物等效为正交各向异性连续体，采用最大应变失效准则，即单元的失效应变达到给定的失效应变时，单元删除。前期研究^[19]对两种织物进行了力学性能试验，图8为两种织物的应力-应变曲线。何泽侃^[20]的研究表明，可以用一层壳单元模拟多层织物，而Zhang等^[4]的研究发现使用膜单元模拟织物更为准确。织物袋实际由多层织物组成，但多层织物模型建模较复杂且计算效率低，因此本研究中用一层膜单元表示织物袋的整个厚度。

图 8 两种织物的应力-应变曲线

Figure 8. Stress-strain curves of the two fabrics

下载: 全尺寸图片幻灯片

2.2 织物袋动态响应过程对比

图9为20 g TNT、1.2 mm厚芳纶织物袋工况下，试验和数值模拟得到的织物袋封口失效前几个典型时刻织物袋的形貌图。可以看出，数值模拟中织物袋的变形过程与试验相似。0.83 ms时刻，在冲击波作用下织物袋中心区域首先鼓起并发生破孔失效；随着冲击波在织物袋内部传播，在1.67 ms时刻织物袋整体呈现鼓胀的状态；此后，冲击波在内部发生反射汇聚到织物袋中心，并从破孔处向外传播；2.5 ms时刻，织物袋整体呈现方形，负压作用下织物袋4个角变瘪并向内收缩。总体来看，数值模拟较好地还原了炸药在织物袋内部爆炸的过程，与试验不同的是数值模拟中未对拉链和安全带扣进行建模，未模拟出拉链失效后冲击波从封口泄漏的现象。

图 9 20 g TNT作用下试验和数值模拟得到的1.2 mm厚芳纶织物袋的动态响应过程对比

Figure 9. Comparison of dynamic response process of 1.2 mm aramid fabric bag by experiment and simulation under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

2.3 织物袋失效模式对比

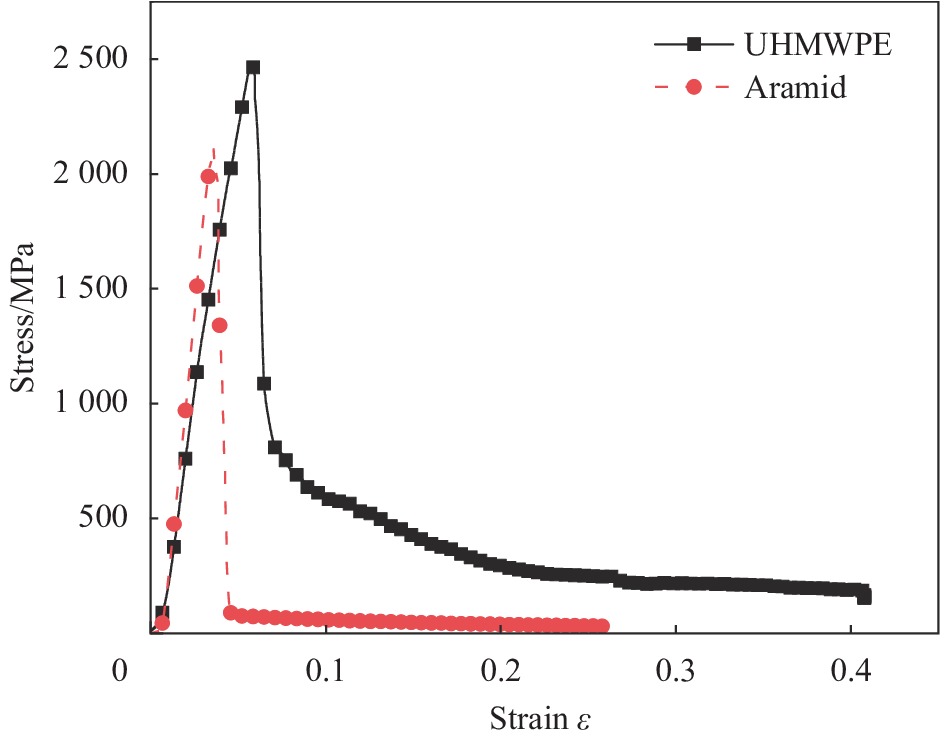
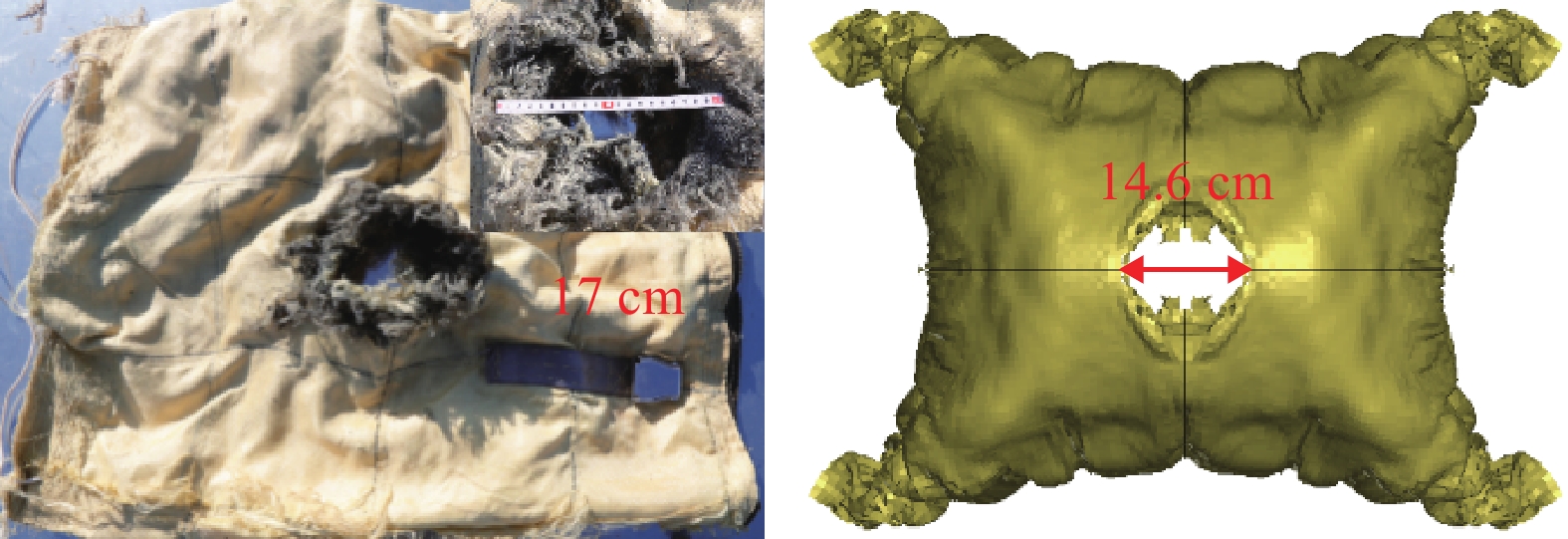
图10为20 g TNT作用下，1.2 mm厚芳纶织物袋的失效模式。可以看出数值模拟和试验出现相同的中心破孔失效模式，破孔尺寸分别为14.6 cm和17 cm，误差为14.1%。与试验不同的是数值模拟中未对拉链、安全带扣和缝合线进行建模，未模拟出封口失效。

图 10 20 g TNT作用下，试验和数值模拟得到的1.2 mm厚芳纶织物袋的失效模式

Figure 10. Failure modes of 1.2 mm aramid fabric bag obtained by experiment and simulation under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

结合芳纶织物袋的动态响应和失效模式可以看出，数值模拟较好地复现了内爆载荷下织物袋的响应行为，所建立的模型能够用于分析织物袋的内爆过程。

3. 两种材料织物袋抗爆性能对比

3.1 两种材料织物袋外部测点超压对比

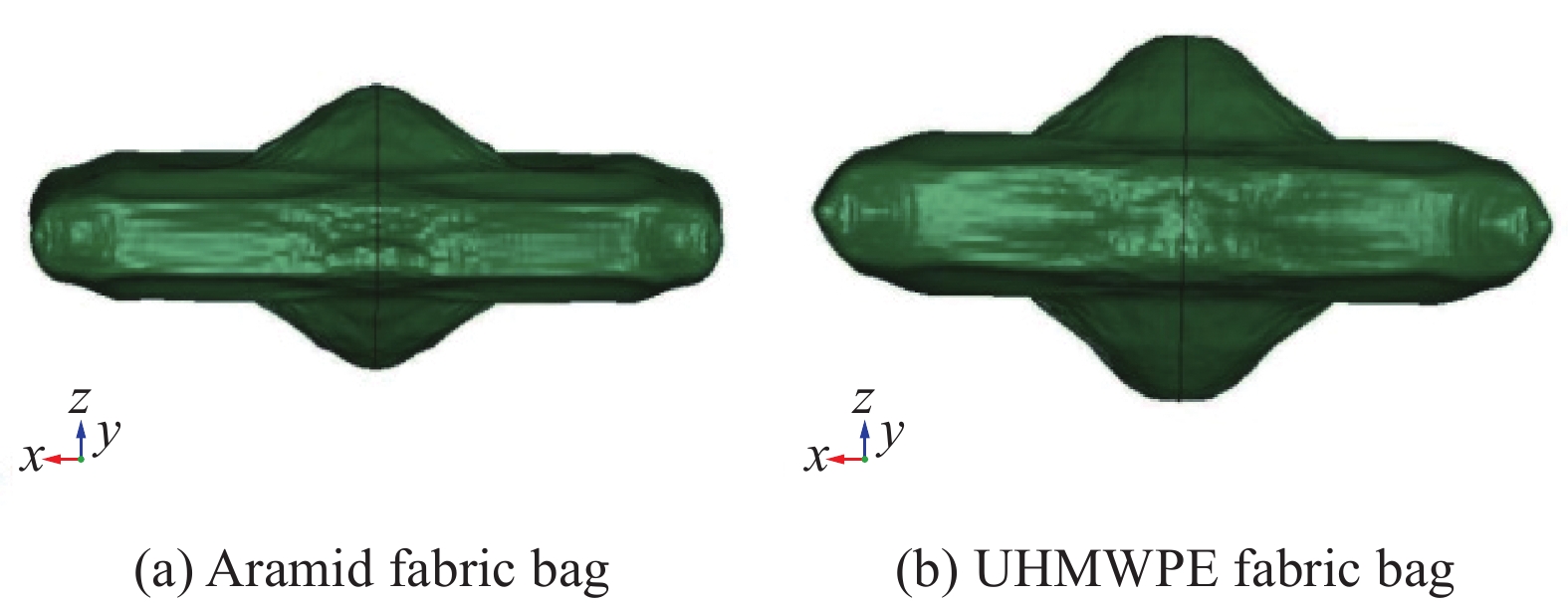
在20 g TNT、2 mm厚织物袋工况下，提取了织物袋正上方距炸药0.5 m处的超压随时间的变化曲线，如图11所示。其中，芳纶织物袋外部超压峰值为43.5 kPa，UHMWPE织物袋外部超压峰值为62.3 kPa，是芳纶织物袋的1.43倍。可以看出同一工况下芳纶纤维织物袋外部的超压小于UHMWPE纤维织物袋，表明在厚度相同时，芳纶织物袋对冲击波超压的衰减作用强于UHMWPE织物袋，主要原因是UHMWPE纤维织物的模量较小，织物的变形能力更强，在内部冲击波和爆轰产物的推动下，UHMWPE织物袋迅速向外扩展，压缩外部空气。图12为超压峰值时刻对应的两种织物袋的形状。可以看出，UHMWPE织物袋的变形明显大于芳纶织物袋。

图 11 织物袋正上方距炸药0.5 m处压力

Figure 11. Pressure above fabric bag 0.5 m from explosive

下载: 全尺寸图片幻灯片

图 12 压力峰值时刻两种织物袋的形状

Figure 12. Shapes of two fabric bags at peak pressure

下载: 全尺寸图片幻灯片

3.2 两种材料织物袋不同药量临界厚度

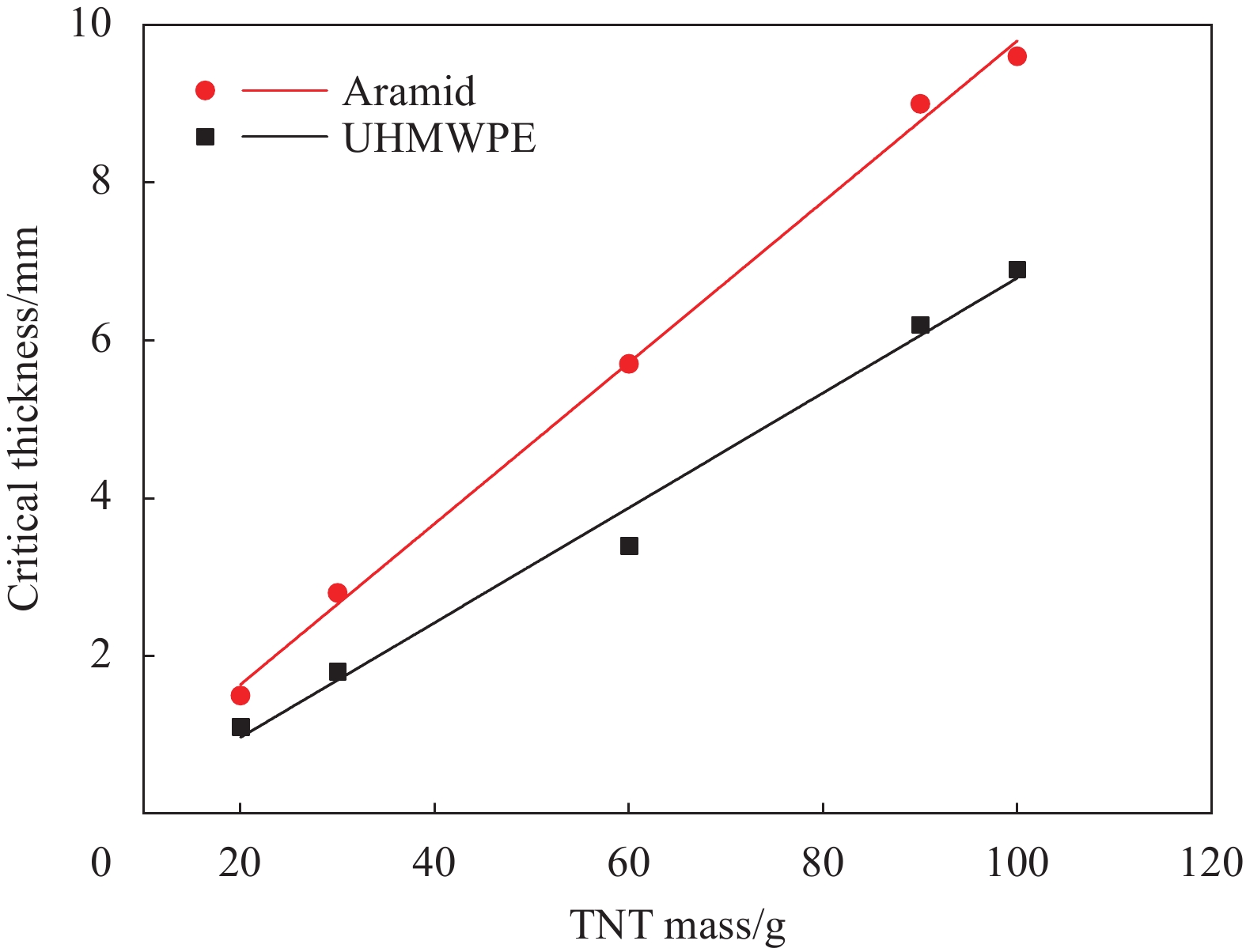
在同一药量不同织物袋厚度下进行数值分析，将织物袋刚好未发生失效的厚度定义为织物袋的临界厚度，计算得到不同药量下尺寸为900 mm×800 mm的两种织物袋的临界厚度如表4所示。图13为拟合得到的织物袋临界厚度随TNT质量的变化关系曲线。可以看出，在20~100 g药量范围内，织物袋的临界厚度与药量呈线性增长关系。

表 4 不同药量下织物袋的临界厚度

Table 4. Critical thickness of fabric bags at different charges

Charge/g	Critical thickness/mm
Charge/g	Aramid fiber	UHMWPE
20	1.5	1.1
30	2.8	1.8
60	5.7	3.4
90	9.0	6.2
100	9.6	6.9

下载: 导出CSV

| 显示表格

图 13 织物袋临界厚度随药量的变化规律

Figure 13. Variation of critical thickness of fabric bags with TNT charge

下载: 全尺寸图片幻灯片

由表4可知，芳纶织物袋的临界厚度明显大于UHMWPE织物袋的临界厚度，说明包容相同当量的TNT需要的芳纶织物袋更厚，且芳纶织物的面密度比UHMWPE织物大，由此可见，UHMWPE织物袋具有更好的抗爆性能。

4. 织物袋抗爆性能影响因素

4.1 初始内爆距离对织物袋抗爆性能的影响

自然状态下织物袋上下两个面紧贴在一起，从文献[9]的研究结果可以看出，爆距对织物的抗爆性能有较大的影响，爆炸物与织物袋接触更容易出现破孔失效的情况。为识别爆炸物与织物袋的相对位置对其抗爆性能的影响，对同一尺寸不同初始内爆距离的织物袋进行内爆数值分析，通过对比临界厚度来说明初始内爆距离对其抗爆性能的影响。

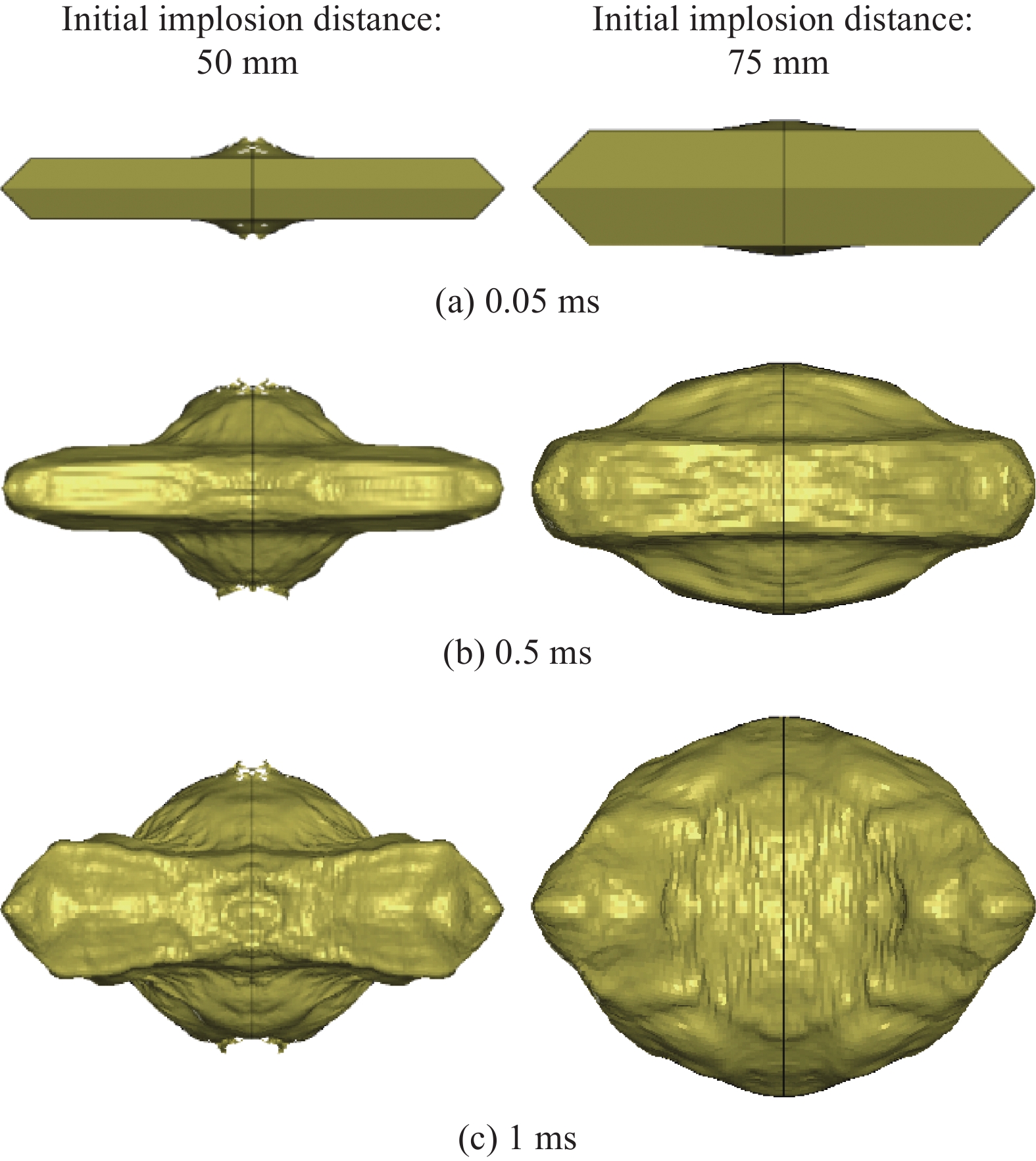
对平铺状态下相同尺寸、相同厚度的芳纶织物袋在不同初始内爆距离下进行内爆数值模拟，研究不同初始内爆距离下织物袋的内爆响应与失效模式。图14为30 g TNT作用下尺寸为900 mm×800 mm、厚度为2 mm的芳纶织物袋在50 mm和75 mm两个初始内爆距离下不同时刻的形状。可以看出，初始内爆距离为50 mm的织物袋在爆炸起始时刻便发生破孔失效，失去抗爆能力，未能起到包容冲击波的作用；初始内爆距离为75 mm的织物袋发生在冲击波和爆轰产物作用下不断膨胀，最终呈现气囊状态。由此可见，对于同一个织物袋，初始内爆距离越大抗爆效果越好。

图 14 不同初始内爆距离下织物袋在不同时刻的形状

Figure 14. Shapes of the fabric bags with different initial implosion distances at different time

下载: 全尺寸图片幻灯片

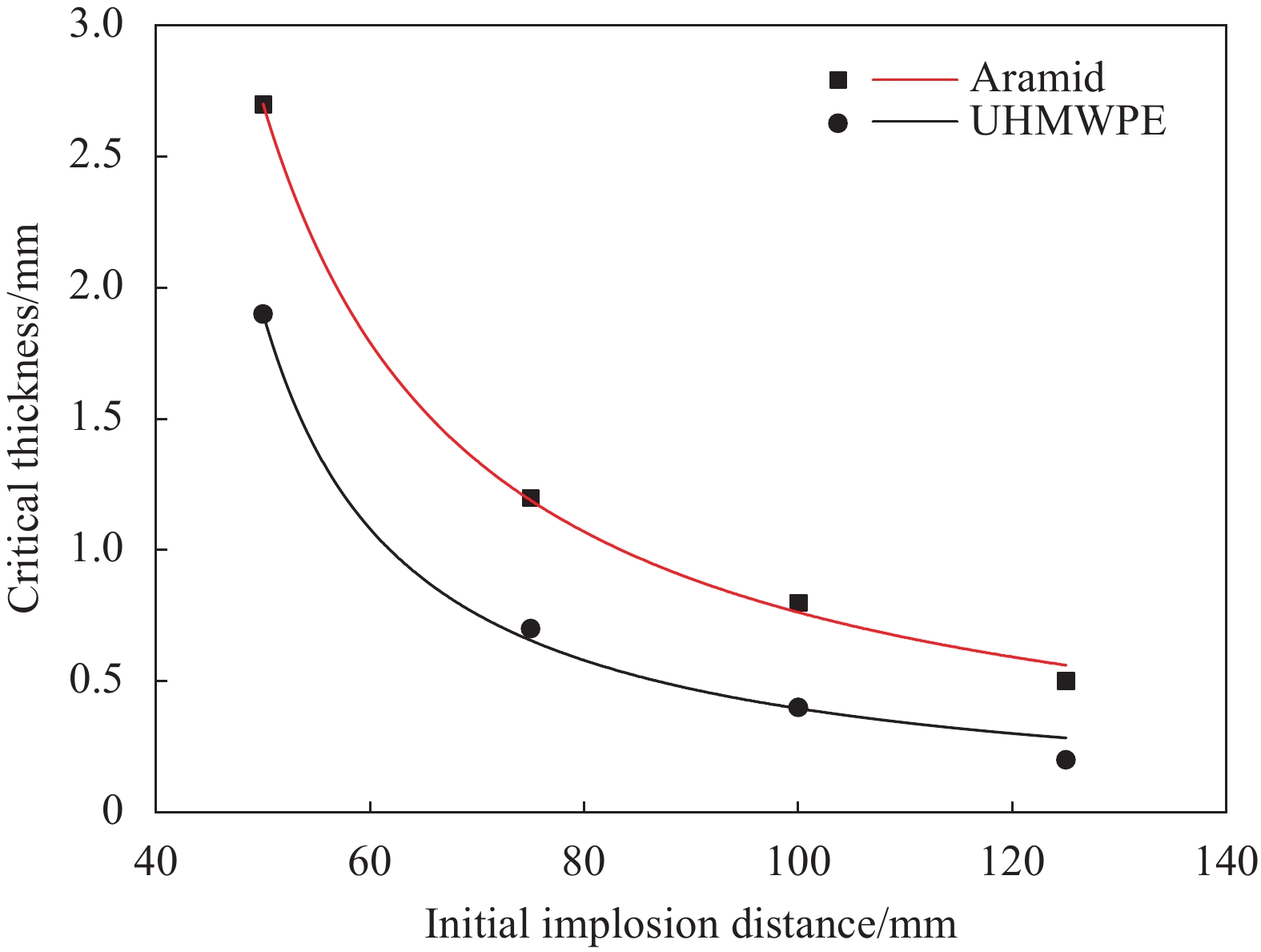
在30 TNT下，分别对尺寸为900 mm×800 mm的芳纶和UHMWPE织物袋在50、75、100和125 mm 4个初始距离下进行内爆数值模拟得到不同初始内爆距离下织物袋的临界厚度如表5所示，图15为拟合得到的织物袋临界厚度随高度变化的趋势。

表 5 30 g TNT作用下，不同初始内爆距离的织物袋的临界厚度

Table 5. Critical thickness of fabric bags with different initial implosion distances under the condition of 30 g TNT

Size/mm	Initial implosion distance/mm	Charge/g	Critical thickness/mm
Size/mm	Initial implosion distance/mm	Charge/g	Aramid	UHMWPE
900×800	50	30	2.8	1.9
	75		1.2	0.7
	100		0.8	0.4
	125		0.5	0.2

下载: 导出CSV

| 显示表格

图 15 织物袋临界厚度随初始内爆距离的变化规律

Figure 15. Variation of critical thickness of fabric bags with initial implosion distances

下载: 全尺寸图片幻灯片

从图15可以看出，随着织物袋上下两个面之间的距离增大，织物袋的临界厚度减小，因此，在织物袋的设计和使用中，可以在织物袋内爆填充其他物质，使炸药尽可能远离织物袋，以提高织物袋的抗爆能力。

4.2 厚度对织物袋外部超压的影响

不同于刚性抗爆容器，织物袋在内爆载荷下会发生大的变形，推动周围空气，在其附近形成超压。文献[21]通过分析冲击波超压对人的鼓膜、肺和消化道等的损伤规律，将鼓膜损伤的阈值34 kPa定为对人体相对安全的冲击波超压临界上限值，即超压大于34 kPa时会对人体造成伤害，因此在柔性织物抗爆容器的设计中需要考虑外部压力水平。

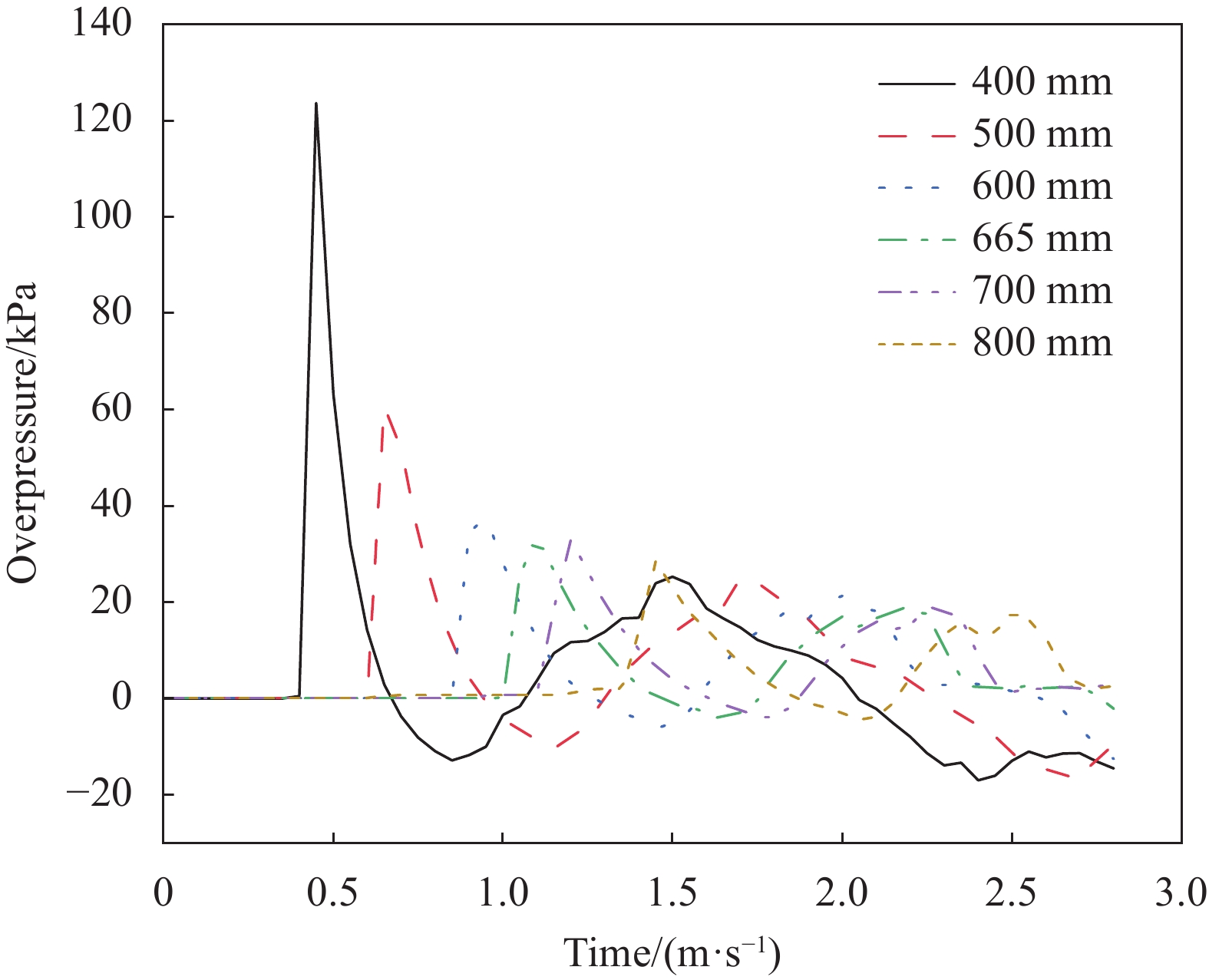
在3 mm厚芳纶织物袋、30 g TNT工况下测量得到了织物袋外部不同位置处的超压峰值，如表6所示。可以看出，随着测点到炸药的距离不断增大，超压峰值迅速下降，距离为665 mm时超压峰值降为34.2 kPa，略高于人体鼓膜损伤阈值34 kPa，距离为700 mm时的超压峰值为32.8 kPa。图16为不同位置处超压随时间的变化曲线。

表 6 30 g TNT作用下3 mm厚芳纶织物袋外部不同位置处的超压峰值

Table 6. Overpressure peaks at different positions outside the 3 mm aramid fabric bag under the condition of 30 g TNT

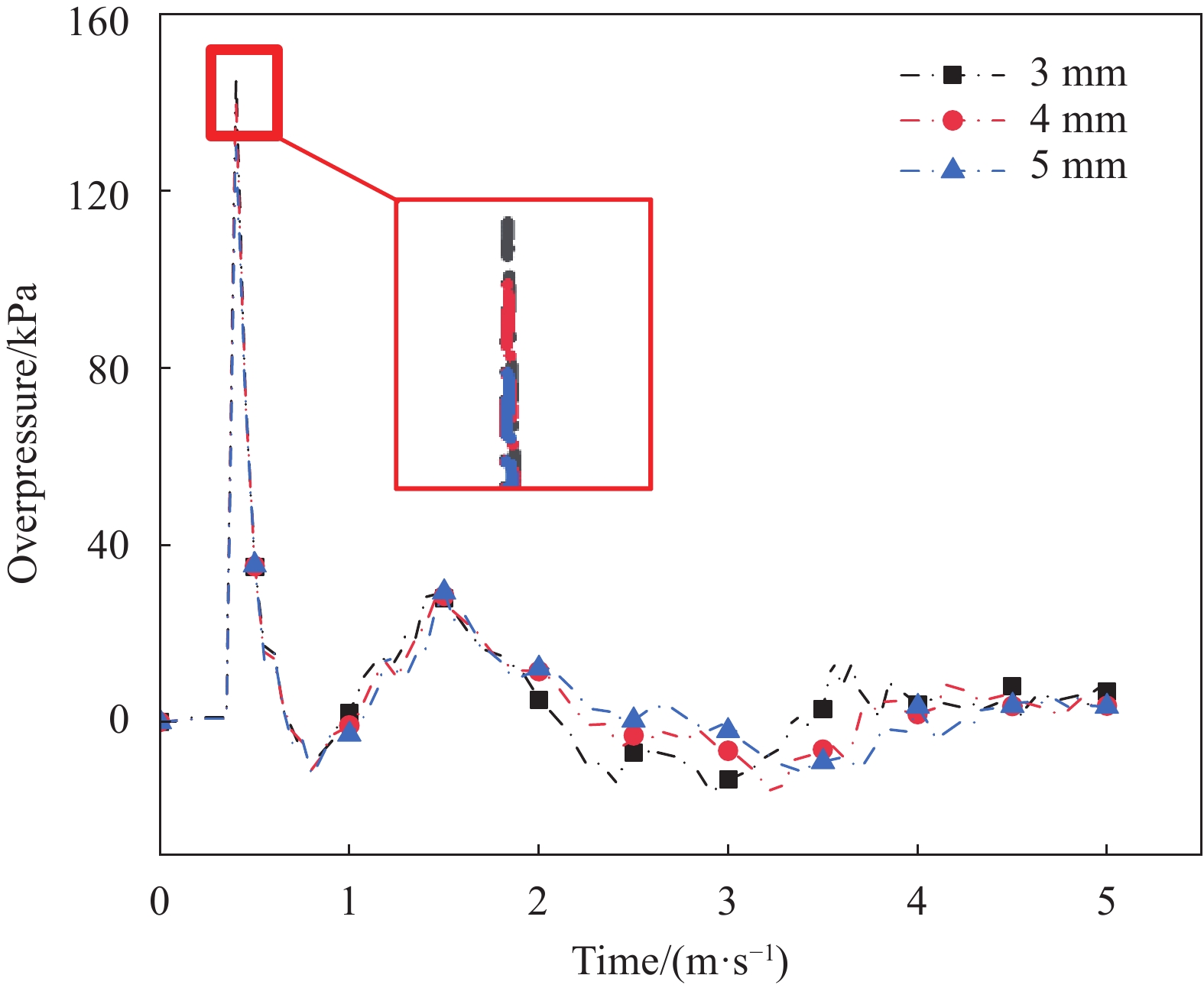
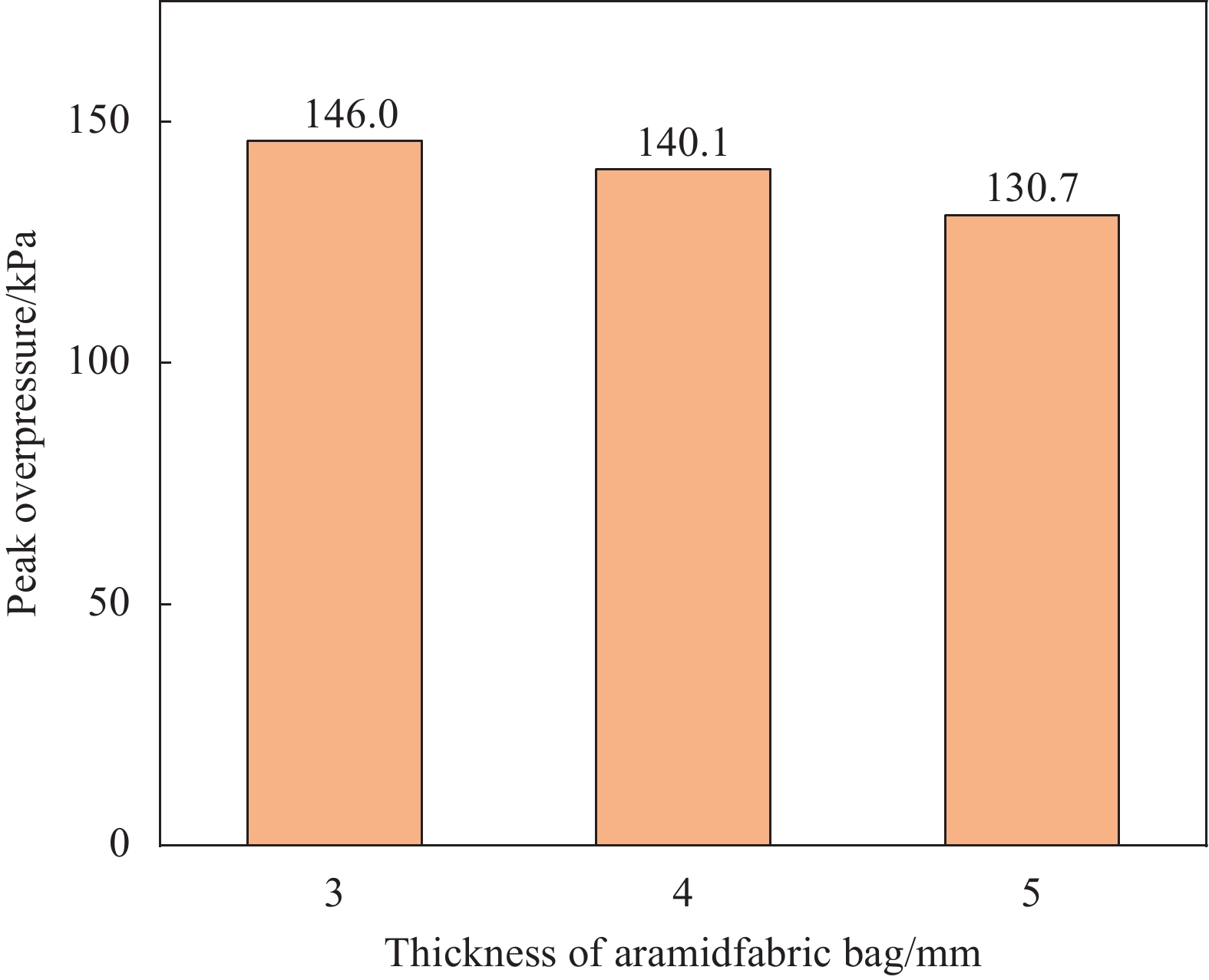
Distance from measuring point to explosive/mm	Charge/g	Overpressure peak/kPa
400	30	123.9
500		60.7
600		37.7
665		34.2
700		32.8
800		28.6

下载: 导出CSV

| 显示表格

图 16 30 g TNT作用下，3 mm厚芳纶织物袋外部不同距离处冲击波超压随时间的变化曲线

Figure 16. Variation curves of shock wave overpressure with time at different positions outside the 3 mm aramid fabric bag under the condition of 30 g TNT

下载: 全尺寸图片幻灯片

控制织物袋的尺寸和形状不变，在同一TNT当量下改变织物袋厚度，提取同一测点处的空气压力，研究厚度对织物袋外部超压的影响。图17为30 g TNT作用下，不同厚度织物袋外部距炸药中心370 mm测点处的超压随时间的变化曲线，在整个爆炸过程中该位置始终处于织物袋外部。可以看出，不同厚度工况下，测点处达到超压峰值的时间几乎一致，随着织物袋厚度的增加，测点处的超压峰值在不断减小，如图18所示，3个厚度下测点处的超压峰值分别为146.0、140.1和130.7 kPa，织物袋厚度对外部超压的影响较小，增加织物袋的厚度一定程度上能够减小外部超压，但效果不太明显。

图 17 不同厚度芳纶织物袋外部测点超压随时间变化规律

Figure 17. Variation of external measuring point overpressure of different thickness aramid fabric bags with time

下载: 全尺寸图片幻灯片

图 18 不同厚度芳纶织物袋外部测点超压峰值对比

Figure 18. Comparison of overpressure peaks at measuring points of aramid fabric bags with different thickness

下载: 全尺寸图片幻灯片

5. 结论

(1) 内爆载荷下织物袋的主要失效模式为中心区域破孔和封口拉链失效；数值模拟中织物袋的动态响应过程和失效模式与试验结果较为接近，所建立的模型能够用于分析纤维织物袋的内爆过程。

(2) 厚度相同时，芳纶织物袋外部的超压峰值更小，表明芳纶织物袋的超压衰减能力更强。

(3) 在20~100 g 药量范围内，织物袋的临界厚度与药量近似呈线性增长关系。对于100 g TNT而言，芳纶织物袋的临界厚度为9.6 mm，超高分子量聚乙烯(UHMWPE)织物袋的临界厚度为6.9 mm。同一当量下，芳纶织物袋的临界厚度明显大于UHMWPE织物袋，在不考虑爆炸火球对材料的影响时，UHMWPE织物袋具有更好的抗爆效果。

(4) 随着初始内爆距离的增大，织物袋的临界厚度减小，因此，在织物袋的设计和使用中，可以在织物袋内爆填充其他物体，使炸药尽可能远离织物袋，以提高织物袋的抗爆能力。

(5) 织物袋外部一定范围内存在高于人体能够承受的超压，即使织物袋未失效也可能对人体造成损伤，以30 g TNT、3 mm厚芳纶织物袋工况为例，距织物袋中心665 mm处的超压峰值为34.2 kPa，超过人体鼓膜损伤阈值34 kPa。织物袋厚度对外部超压的影响较小。

图 1 织物袋实物图

Figure 1. Fabric bag

下载: 全尺寸图片幻灯片

图 2 三硝基甲苯(TNT)放置方式

Figure 2. Placement of tri-nitro-toluene (TNT)

下载: 全尺寸图片幻灯片

图 3 试验系统

Figure 3. Experimental setup

下载: 全尺寸图片幻灯片

图 4 20 g TNT作用下，1.2 mm厚芳纶织物袋的动态响应过程

Figure 4. Dynamic response process of 1.2 mm aramid fabric bag under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

图 5 20 g TNT作用下，2.2 mm厚UHMWPE织物袋的动态响应过程

Figure 5. Dynamic response process of 2.2 mm UHMWPE fabric bag under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

图 6 织物袋外部3个测点超压随时间的变化曲线

Figure 6. Variation curves of overpressure with time at three measuring points outside the fabric bag

下载: 全尺寸图片幻灯片

图 7 织物袋有限元模型

Figure 7. Finite element model of fabric bag

下载: 全尺寸图片幻灯片

图 8 两种织物的应力-应变曲线

Figure 8. Stress-strain curves of the two fabrics

下载: 全尺寸图片幻灯片

图 9 20 g TNT作用下试验和数值模拟得到的1.2 mm厚芳纶织物袋的动态响应过程对比

Figure 9. Comparison of dynamic response process of 1.2 mm aramid fabric bag by experiment and simulation under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

图 10 20 g TNT作用下，试验和数值模拟得到的1.2 mm厚芳纶织物袋的失效模式

Figure 10. Failure modes of 1.2 mm aramid fabric bag obtained by experiment and simulation under the condition of 20 g TNT

下载: 全尺寸图片幻灯片

图 11 织物袋正上方距炸药0.5 m处压力

Figure 11. Pressure above fabric bag 0.5 m from explosive

下载: 全尺寸图片幻灯片

图 12 压力峰值时刻两种织物袋的形状

Figure 12. Shapes of two fabric bags at peak pressure

下载: 全尺寸图片幻灯片

图 13 织物袋临界厚度随药量的变化规律

Figure 13. Variation of critical thickness of fabric bags with TNT charge

下载: 全尺寸图片幻灯片

图 14 不同初始内爆距离下织物袋在不同时刻的形状

Figure 14. Shapes of the fabric bags with different initial implosion distances at different time

下载: 全尺寸图片幻灯片

图 15 织物袋临界厚度随初始内爆距离的变化规律

Figure 15. Variation of critical thickness of fabric bags with initial implosion distances

下载: 全尺寸图片幻灯片

图 16 30 g TNT作用下，3 mm厚芳纶织物袋外部不同距离处冲击波超压随时间的变化曲线

Figure 16. Variation curves of shock wave overpressure with time at different positions outside the 3 mm aramid fabric bag under the condition of 30 g TNT

下载: 全尺寸图片幻灯片

图 17 不同厚度芳纶织物袋外部测点超压随时间变化规律

Figure 17. Variation of external measuring point overpressure of different thickness aramid fabric bags with time

下载: 全尺寸图片幻灯片

图 18 不同厚度芳纶织物袋外部测点超压峰值对比

Figure 18. Comparison of overpressure peaks at measuring points of aramid fabric bags with different thickness

下载: 全尺寸图片幻灯片

表 1 各织物的主要物理参数

Table 1 Main physical parameters of fabrics

Material	Aramid	UHMWPE
Grade	F-268	ZTZ 24
Yarn fineness/tex	166	126±10
Yarn body density/(g·cm⁻³)	1.44	0.97
Yarn breaking elongation/%	≥3.2	3-3.5
Yarn tensile modulus/GPa	≥125	105-110
Fabric thickness/mm	0.3	0.55
Weave count/(yarns·(10 cm)⁻¹)	65×65	87×87
Areal density/(g·m⁻²)	210-220	235-245
Note: UHMWPE—Ultra-high molecular weight polyethylene.

下载: 导出CSV

表 2 TNT参数

Table 2 Parameters of TNT

Parameter	Value
Density/(kg·cm⁻³)	1.63×10³
Detonation velocity/(m·s⁻¹)	6930
Detonation pressure/GPa	21
A/GPa	374
B/GPa	3.74
R₁	4.15
R₂	1.4
ω	0.35
E₀/(MJ·m⁻³)	7×10³
Notes: A, B, R₁, R₂, ω—Constants characterizing TNT properties; E₀—Detonation energy per unit volume.

下载: 导出CSV

表 3 空气参数

Table 3 Parameters of air

Parameter	Value
Density/(kg·m⁻³)	1.29
C₄	0.4
C₅	0.4
C₁, C₂, C₃, C₆	0
E₀/(MJ·m⁻³)	0.25
Notes: C₀-C₆—Polynomial equation coefficients.

下载: 导出CSV

表 4 不同药量下织物袋的临界厚度

Table 4 Critical thickness of fabric bags at different charges

Charge/g	Critical thickness/mm
Charge/g	Aramid fiber	UHMWPE
20	1.5	1.1
30	2.8	1.8
60	5.7	3.4
90	9.0	6.2
100	9.6	6.9

下载: 导出CSV

表 5 30 g TNT作用下，不同初始内爆距离的织物袋的临界厚度

Table 5 Critical thickness of fabric bags with different initial implosion distances under the condition of 30 g TNT

Size/mm	Initial implosion distance/mm	Charge/g	Critical thickness/mm
Size/mm	Initial implosion distance/mm	Charge/g	Aramid	UHMWPE
900×800	50	30	2.8	1.9
	75		1.2	0.7
	100		0.8	0.4
	125		0.5	0.2

下载: 导出CSV

表 6 30 g TNT作用下3 mm厚芳纶织物袋外部不同位置处的超压峰值

Table 6 Overpressure peaks at different positions outside the 3 mm aramid fabric bag under the condition of 30 g TNT

Distance from measuring point to explosive/mm	Charge/g	Overpressure peak/kPa
400	30	123.9
500		60.7
600		37.7
665		34.2
700		32.8
800		28.6

下载: 导出CSV

参考文献(21)

[1]	Federal Aviation Administration. Least risk bomb location: FAA. AC 25.795-6[S]. Washington: Federal Aviation Administration, 2008.
[2]	FALLAH A S, MICALLEF K, LANGDON G S, et al. Dynamic response of Dyneema HB26 plates to localised blast loading[J]. International Journal of Impact Engineering,2014,73(11):91-100.
[3]	GARGANO A, PINGKARAWAT K, BLACKLOCK M. Comparative assessment of the explosive blast performance of carbon and glass fibre-polymer composites used in naval ship structures[J]. Composite Structures,2017,171(1):306-316.
[4]	ZHANG Y, YAN D J, NIAN X Z, et al. Numerical analysis of rigid and flexible reflection of air shock wave[J]. Applied Mechanics & Materials,2014,638-640:2010-2014.
[5]	ZHANG B, NIAN X Z, JIN F, et al. Failure analyses of flexible ultra-high molecular weight polyethylene (UHMWPE) fiber reinforced anti-blast wall under explosion[J]. Composite Structures,2018,184:759-774. DOI: 10.1016/j.compstruct.2017.10.037
[6]	赵敏, 赵睿昕, 沈永明, 等. CFR嵌片对超高分子质量聚乙烯多层复合织物防爆性能的影响[J]. 纺织学报, 2014, 35(3):37-40. ZHAO Min, ZHAO Ruixin, SHEN Yongming, et al. The effect of CFR inserts on the explosion-proof properties of ultra-high molecular weight polyethylene multilayer composite fabrics[J]. Journal of Textile Research,2014,35(3):37-40(in Chinese).
[7]	解江, 姜超, 高斌元, 等. 高性能纤维织物抗爆性能试验研究[J]. 应用力学学报, 2022, 39(1):35-43. XIE Jiang, JIANG Chao, GAO Binyuan, et al. Experimental study on anti-explosion performance of high-performance fiber fabrics[J]. Chinese Journal of Applied Mechanics,2022,39(1):35-43(in Chinese).
[8]	冯振宇, 姜超, 高斌元, 等. 芳纶纤维平纹织布在爆炸载荷下的动态响应与失效行为的数值分析[J]. 应用数学和力学, 2021, 42(11):1113-1125. FENG Zhenyu, JIANG Chao, GAO Binyuan, et al. Numerical analysis of dynamic response and failure behavior of aramid fiber plain weave under blast load[J]. Applied Mathematics and Mechanics,2021,42(11):1113-1125(in Chinese).
[9]	解江, 高斌元, 甄婷婷, 等. 爆炸载荷下机织物的动态响应与失效行为[J]. 复合材料学报, 2022, 39(10): 4949-4960. XIE Jiang, GAO Binyuan, ZHEN Tingting, et al. Dynamic response and failure behaviors of woven fabrics under blast load[J]. Acta Materiae Compositae Sinica, 2022, 39(10): 4949-4960(in Chinese).
[10]	CHUN S, KAPOOR H, KAPANIA R, et al. Nonlinear fluid-structure interaction in a flexible shelter under blast loading[C]//46th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Materials Conference. Virginia, 2005.
[11]	MARKERT F, THOMMESEN J. Blast worthy textile-based luggage containers for aviation safety[J]. Safety and Security Analysis,2011,40:213557.
[12]	ZANGANI D, AMBROSETTI S, BOZZOLO A, et al. Textile-based luggage containers for onboard blast protection[J]. Sae International Journal of Aerospace,2011,4(2):690-698. DOI: 10.4271/2011-01-2517
[13]	GIOVANELLE M L. Advanced technologies for bombproof cargo containers and blast containment units for the retrofitting of passenger airplanes[J]. International Journal of Aviation Systems, Operations and Training, 2015, 2(1): 33-47.
[14]	POLAK J, REDLICH G, WITCZAK E. The reaction of cylinder fibrous cover to explosion of charge with fragments[J]. Central European Journal of Energetic Materials,2009,6(2):137-148.
[15]	POLAK J, ROMEK R, WIŚNIEWSKI A. Lightweight covers for attenuating the explosion of the charges and the methodology of their protective performance assay[J]. Problemy Techniki Uzbrojenia,2008,37(107):15-24.
[16]	ZHU W, HUANG G Y, LIU H, et al. Experimental and numerical investigation of a hollow cylindrical water based barrier against internal blast induced fragment loading[J]. International Journal of Impact Engineering,2020,138:103503. DOI: 10.1016/j.ijimpeng.2020.103503
[17]	MASI F, MARIA M P, VANNUCCI P. Blast actions in aircrafts: An integrated methodology for designing protection devices[J]. Engineering Structures,2018,175:895-911. DOI: 10.1016/j.engstruct.2018.08.082
[18]	蔡军锋, 易建政. 新型危险品防爆运输箱设计及抗爆试验研究[J]. 包装工程, 2012, 33(5):6-8, 55. CAI Junfeng, YI Jianzheng. Design and anti-explosion test of new explosion-proof transport case for dangerous article[J]. Packaging Engineering,2012,33(5):6-8, 55(in Chinese).
[19]	蔡军锋, 傅孝忠, 易建政. 超高分子量聚乙烯-聚氨酯泡沫复合材料的抗爆实验与数值模拟[J]. 高分子材料科学与工程, 2013, 29(11):79-83. DOI: 10.16865/j.cnki.1000-7555.2013.11.019 CAI Junfeng, FU Xiaozhong, YI Jianzheng. Anti-explosion experiment and numerical simulation of ultra-high molecular weight polyethylene-polyurethane foam composites[J]. Polymer Materials Science and Engineering,2013,29(11):79-83(in Chinese). DOI: 10.16865/j.cnki.1000-7555.2013.11.019
[20]	何泽侃. 芳纶纤维布缠绕增强软壁机匣包容性研究[D]. 杭州: 浙江大学, 2018. HE Zekan. Research on the containment of soft wall casing wrapped with aramid fiber fabric[D]. Hangzhou: Zhejiang University, 2018(in Chinese).
[21]	孙艳馥, 王欣. 爆炸冲击波对人体损伤与防护分析[J]. 火炸药学报, 2008, 31(4):50-53. DOI: 10.3969/j.issn.1007-7812.2008.04.013 SUN Yanfu, WANG Xin. Analysis of human body injury and protection by explosion shock wave[J]. Journal of Explosives,2008,31(4):50-53(in Chinese). DOI: 10.3969/j.issn.1007-7812.2008.04.013