Status of numerical simulation methods for delamination damage of composite laminates
-
摘要: 复合材料层合板在航空航天等领域受到广泛应用,分层损伤作为复合材料层合板主要的损伤形式,对复合材料结构的强度和刚度有显著的影响,是限制其重大工程应用的热点问题之一。通过实验的方法对复合材料结构进行研究,往往需要耗费大量的时间和成本,成熟的有限元数值模拟技术可以较低成本实现复合材料结构的分层行为模拟,成为分层损伤研究的重要手段。本文从数值模拟角度总结了国内外在纤维增强复合材料分层损伤取得的研究成果,并对目前主要的方法虚拟裂纹闭合技术(VCCT)、内聚力模型(CZM)及扩展有限元法(XFEM)进行阐述。最后,对其发展方向进行了展望。Abstract: Composite laminates are widely used in aerospace and other fields. Delamination is the main damage form in composite laminates, which has a significant impact on the strength and stiffness of composite structures. It’s one of the hot issues which is limited in major engineering applications. It often takes a lot of time and cost to study composite structure by experimental methods. Mature finite element numerical simulation technology can realize the layered behavior simulation of composite structures at a low cost and become an important means for delamination damage research. In this paper, from the perspective of numerical simulation, the research results on the interface damage of fiber reinforced composite materials at home and abroad are summarized, and the current main methods are virtual crack closure technology (VCCT), cohesive force model (CZM) and extended finite element method (XFEM). Finally, the text makes a prospect for the future work.
-
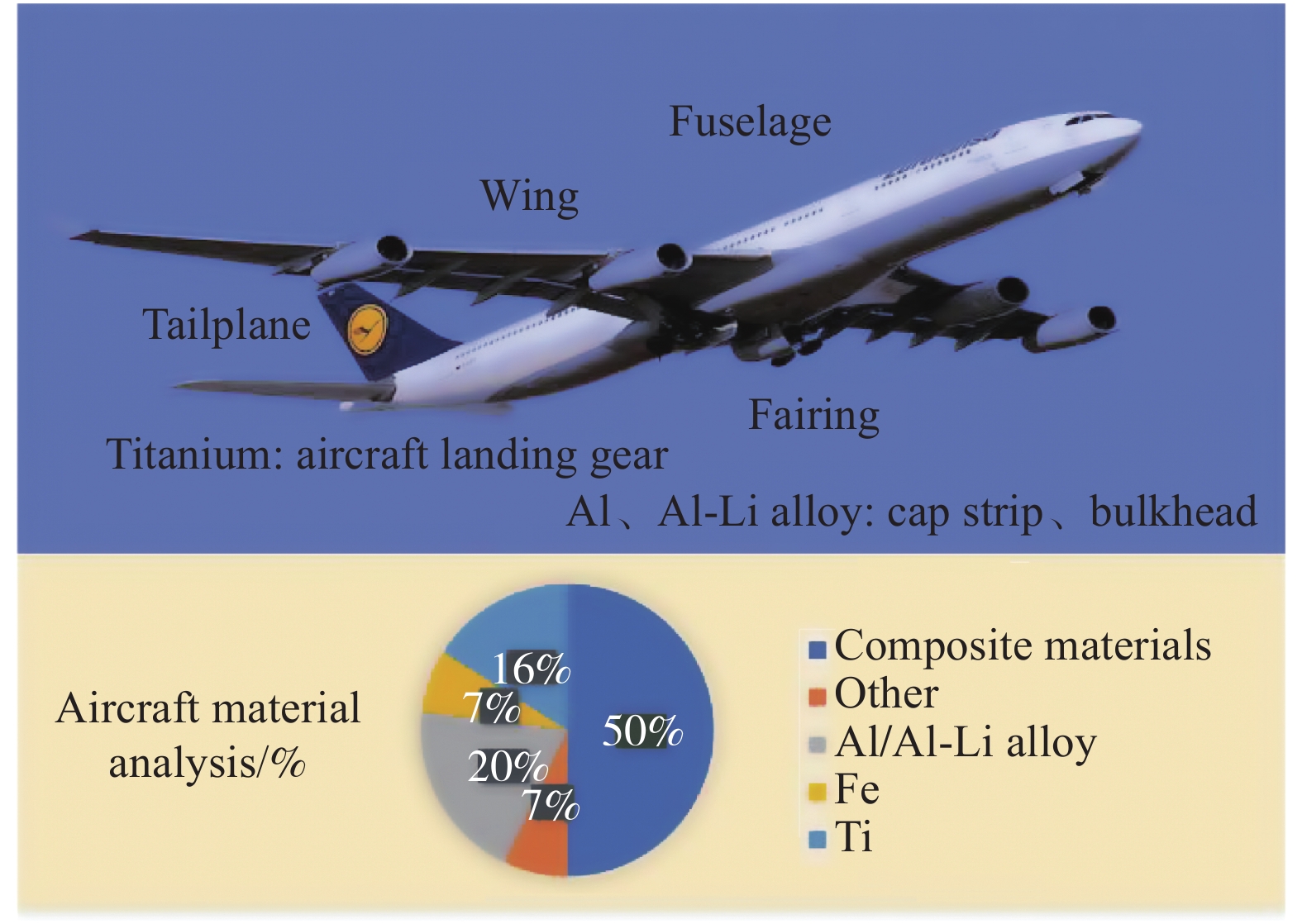
纤维增强树脂复合材料拥有高强、轻质、耐腐蚀、抗疲劳、吸波隐身等优异性能,在航空航天、军工、汽车、化工及电子等先进工业领域得到广泛应用[1-4]。按树脂基体性质可以将纤维增强树脂复合材料分为两大类,即热固性树脂基复合材料和热塑性树脂基复合材料;相较而言,热塑性树脂基复合材料具有:(1)韧性好,疲劳强度高,冲击损伤容限高;(2)预浸料和热塑性树脂存储没有期限要求;(3)热成型工艺性好,成型周期短,生产效率高;(4)边角料或废料可再熔融成型或回收利用,环境友好[5]。因此,热塑性树脂基复合材料随着工业技术发展越来越受到青睐。以聚醚醚酮(PEEK)、聚醚酮酮(PEKK)、聚苯硫醚(PPS)和聚醚酰亚胺(PEI)等为代表的高性能热塑性树脂基复合材料的出现,为航空航天结构材料选材提供了富有竞争性的解决方案,且随着产品线的延伸和技术成熟度的不断提高,高性能热塑性树脂基复合材料在航空航天结构材料体系中的地位越来越重要,并逐渐打破以环氧树脂及双马来酰亚胺树脂等为代表的传统热固性树脂基复合材料的垄断地位。
航空航天结构大而复杂,在制造和装配时需要通过连接技术将不同的部件连接在一起来实现,因此需要可靠、自动化和经济的连接技术。机械连接是目前航空结构的主要连接方法,具有制造工艺简单、厚度方向起到增强作用和便于拆装等优点;但也引入许多问题,特别是钻孔存在应力集中、孔边分层、纤维损伤、重量增加和热膨胀系数不匹配等问题[6-7]。胶接连接使连接应力集中最小化,具有优越的抗疲劳性能,但胶接需要严格的表面处理,对污染和环境非常敏感(如粉尘、水分和加工油污等),胶粘剂有存储寿命、胶接工艺复杂和周期长等问题[8]。热塑性树脂基复合材料具有可焊性,其焊接工艺已被认为是热塑性树脂基复合材料连接的有效替代技术,利用热塑性树脂基复合材料焊接可以在很大程度上消除这些问题。最具潜力的焊接方法主要有超声波焊接[9]、感应焊[10]、电阻焊[11]和激光焊[12]等。
热塑性树脂基复合材料焊接在国外已经有较长的研究历程,且相应成果已经成功应用于民用客机及战斗机结构中[2],而国内热塑性树脂基复合材料的制造及相应技术尚处于起步阶段[13]。因此,本文针对航空结构用碳纤维/聚苯硫醚(CF/PPS)热塑性复合材料,采用CF/PPS复合材料混编织物电阻元件对其织物层压板进行电阻焊接;电流、压力和加热时间是电阻焊接的重要工艺参数,采用正交实验、Taguchi方法和方差分析(ANOVA)研究分析CF/PPS热塑性复合材料电阻焊接最佳工艺参数和其对接头强度的影响,同时对其焊接接头单搭接剪切强度和断口进行测试分析,获取其失效机制。该方法也是热塑性树脂基复合材料焊接技术的有益探索。
1. 实验材料及方法
1.1 原材料
碳纤维/聚苯硫醚(CF/PPS)复合材料层压板是利用加拿大Barrday公司提供的AS4 3K 5HS CF织物/PPS树脂预浸料在XLB-660.660.3型平板硫化机上热压成型;热压工艺为:温度为320℃±10℃,保温时间为30 min,压力为2 MPa,冷却速率为5℃/min,压制过程排气两次;试板铺层结构为[0]8,试板厚度为2 mm,CF体积分数约为54vol%。AS4 3K 5HS CF/PPS复合材料混编织物的混编方式如图1所示,PPS树脂薄膜由日本东丽公司提供,PPS树脂膜厚度为50 μm。
1.2 电阻焊接装置
采用中国民航大学自主研制的复合材料焊接设备(CAUC-CW100),如图2所示,该设备能进行多种形式电载荷施加(直流、交流和脉冲电流),采用伺服电子压力缸进行多段恒位移或压力压装控制,数据采集器可进行焊接件的焊接接头温度、压力和位移变化的信息实时采集等功能。
电阻焊接:利用CF电热特性,在试样的焊接表面放置CF混编织物,并在焊接件上下焊接表面放置PPS树脂薄膜,CF在电热作用时产生焦耳热,熔融被焊接的焊接表面及PPS树脂薄膜,并在压力作用下形成焊接接头。在焊缝中间布置K型伯劳焊点热电偶,对焊缝温度检测,焊接示意图及尺寸如图3所示。
1.3 Taguchi方法和实验设计
Taguchi方法是以正交表为基础来获取可得到CF/PPS复合材料层板焊接质量的优化工艺方案,与传统正交实验相比,该方法利用信噪比(S/N)来衡量指标的波动[14-16]。本研究质量特性为CF/PPS复合材料层板电阻焊接单搭接的剪切强度,3个关键焊接工艺参数即电流、压力和焊接时间作为因素,基于相同比例给出水平变化,如表1所示;因此优化目标即是获得最大的信噪比。CF/PPS复合材料层板电阻焊接剪切强度属于越大越优的质量指标,其信噪比公式为[11]
表 1 因素和水平Table 1. Factors and levelsParameters Levels 1 2 3 4 Factor A(Current/A) 8 10 12 14 Factor B(Pressure/MPa) 0.5 1.0 1.5 2.0 Factor C(Time/min) 10 20 30 40 η=−10lg[1nn∑i=11Xi2] (1) 式中:η是CF/PPS复合材料层板电阻焊接质量特性,即信噪比(dB);Xi是第i个CF/PPS复合材料层板电阻焊接试样剪切强度(MPa);n是每组试验中的重复数(本研究n=6)。
设计L16实验,得到各次CF/PPS复合材料层板电阻焊接实验的焊接剪切强度,然后利用式(1)计算出各次实验的信噪比,结果如表2所示。
表 2 CF/PPS复合材料层板的正交实验参数及结果Table 2. Input parameters of orthogonal arrays and performance characteristics of CF/PPS composite laminatesExpt.run Factor Performance Current
A/APressure
B/MPaTime
C/minLSS/MPa η 1 8 0.5 10 3.68 11.32 2 8 1.0 20 5.34 14.55 3 8 1.5 30 6.86 16.73 4 8 2.0 40 5.37 14.60 5 10 0.5 20 9.02 19.10 6 10 1.0 10 8.65 18.74 7 10 1.5 40 12.46 21.91 8 10 2.0 30 10.34 20.29 9 12 0.5 30 13.05 22.31 10 12 1.0 40 16.78 24.50 11 12 1.5 10 14.28 23.09 12 12 2.0 20 12.15 21.69 13 14 0.5 40 7.49 17.49 14 14 1.0 30 9.65 19.69 15 14 1.5 20 9.84 19.86 16 14 2.0 10 7.51 17.51 Average η 18.96 Notes: LSS—Lap shear strength; η—Signal to noise ratio. 同时,采用方差分析(ANOVA)来获取CF/PPS热塑性复合材料层板电阻焊接各个工艺参数对其剪切强度影响的贡献度。
1.4 焊接接头力学性能测试
参考ASTM D1002—10标准[17],采用Instron5982电子万能材料试验机对CF/PPS复合材料层板焊接的单搭接剪切强度(LSS)进行测试,试验件尺寸如图4所示。
剪切强度τ(MPa)
计算如下: τ=Fmax (2) 式中:L为搭接长度(mm);b为搭接宽度(mm);Fmax为最大拉伸力(N)。
1.5 剪切断口分析
采用激光共聚焦显微镜(OLYMPUS 4100)和SEM(Quanta FEG250)对Taguchi方法优化最佳焊接参数的焊接试样(记为H-LSS)和本实验方案中较低剪切强度试样(记为L-LSS)的截面形貌和剪切测试断口形貌进行观察,分析焊缝结构、铺展形态及其失效形式和机制。
2. 结果与讨论
2.1 CF/PPS复合材料的焊接
图5为CF/PPS复合材料电阻焊接控制过程。可知,CF/PPS复合材料电阻焊接控制过程主要为五个阶段。第Ⅰ阶段:通电,以一定速率增加电流强度,CF/PPS复合材料混编织物电热作用产生的焦耳热也随之增加,PPS膜及焊接件表面受热软化变形,达到PPS树脂热熔温度(Tm);第Ⅱ阶段:初始压紧(预压力Ps),释放焊接件焊接界面与CF/PPS复合材料混编织物间的间隙,保证受热均匀;第Ⅲ阶段:增加焊接压力(达到额定压力Pm),保持恒温恒压,焊接件的焊接界面及PPS膜热熔,在压力作用下热塑性树脂流动,分子链热运动和扩散缠结;第Ⅳ阶段:调节电流以一定冷却速率(Tf,本实验Tf≈5℃/min)冷却,固化;第Ⅴ阶段:采用压缩空气冷却,焊接接头热收缩,完成焊接。在第Ⅱ和第Ⅲ阶段施加压力时会出现温度小幅度迅速升高后降低,这是由于施加压力使焊缝紧密,焦耳热集聚增加,随后在热传导和热扩散作用下又缓慢减小,同时压力也增大,这是由于高温使焊接接头自由体积膨胀。依据表2焊接参数完成试样焊接,如图6所示,CF/PPS复合材料层板焊接接头无明显变形。
![]() 图 5 CF/PPS复合材料电阻焊接控制过程Figure 5. Resistance welding control process of CF/PPS composites (Tm—PPS hot melt temperature; Ps—Pre-pressure during welding; Pm—Rated pressure during welding; Tf—Cooling rate; RT—Room temperature; t1—Temperature rise time of resistance welding; t2—Pre-pressure time; t 3—Constant temperature and pressure time; t4—Cooling time at Tf rate; t5—Natural cooling time)
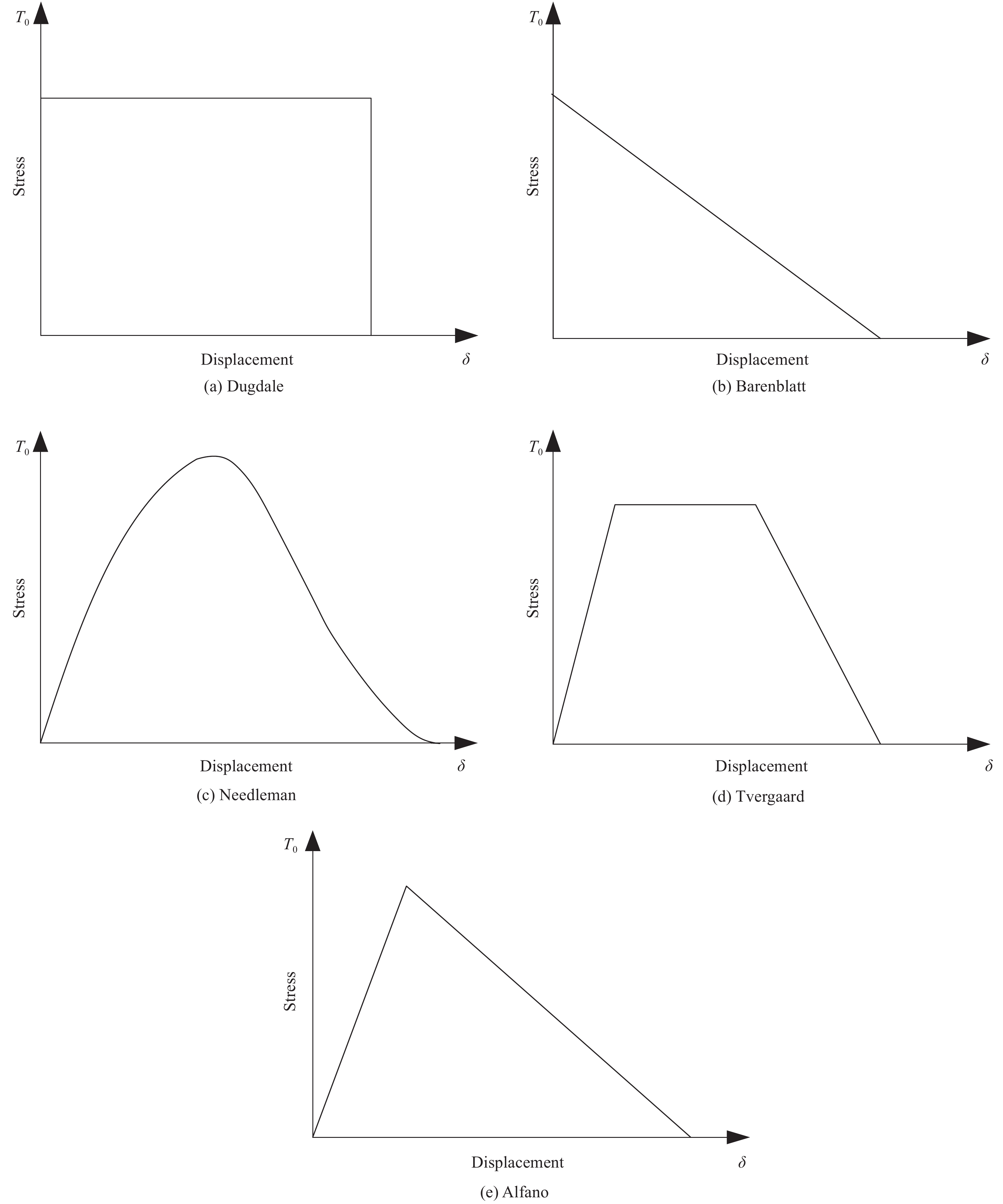
图 5 CF/PPS复合材料电阻焊接控制过程Figure 5. Resistance welding control process of CF/PPS composites (Tm—PPS hot melt temperature; Ps—Pre-pressure during welding; Pm—Rated pressure during welding; Tf—Cooling rate; RT—Room temperature; t1—Temperature rise time of resistance welding; t2—Pre-pressure time; t 3—Constant temperature and pressure time; t4—Cooling time at Tf rate; t5—Natural cooling time)2.2 CF/PPS复合材料的工艺参数优化与性能预测
预测CF/PPS复合材料层板电阻焊接工艺参数最优组合需要计算各个因素的信噪比均值,计算如下:
{m_{fi}} = \frac{1}{N}\sum\limits_{i = 1}^n {{\eta _i}} (3) 式中:f是因素;i是水平;N是实验次数;η是信噪比。
利用式(3)计算得到各因素的信噪比,比较得到该因素下的最大值是该因素的主效应,所有因素都取主效应即是预测的最优组合。CF/PPS复合材料电阻焊接工艺参数主效应图如图7所示。
可以看出,随着焊接电流、压力和焊接时间的增加,CF/PPS复合材料层板电阻焊接单搭接剪切强度均呈先增大后减小的变化趋势,获得最优焊接参数水平为A3、B3、C3 (即电流为12 A,压力为1.5 MPa,时间为30 min),其信噪比为[11]
{\eta _{\rm A3B3C3}} = m + ({m_{\rm A3}} - m) + ({m_{\rm B3}} - m) + ({m_{\rm C3}} - m) (4) 式中:ηA3B3C3为优化因素水平的预测信噪比;m为总体信噪比均值;mA3、mB3、mC3分别为因素的主效应。
由式(1)、式(3)、式(4)可得,预测最优因素水平焊接参数的CF/PPS复合材料层板电阻焊接单搭接剪切强度为18.07 MPa,同时用实验对预测结果进行验证,采用预测最优化焊接参数焊接CF/PPS复合材料单搭接试样,并测试了其剪切强度,如表3所示,可以看出,CF/PPS复合材料单搭接试样剪切强度均值为17.88 MPa,Taguchi方法预测剪切强度和实验测试剪切强度非常接近,表明Taguchi方法可有效应用到热塑性树脂基复合材料焊接工艺参数优化中。
表 3 CF/PPS复合材料Taguchi方法预测最优因子水平、焊接接头剪切强度和实验验证结果Table 3. Taguchi method for predicting optimal factor level, weld joint shear strength and experimental verification results of CF/PPS compositesFactor A Factor B Factor C Optimal parameter 12 1.5 30 Taguchi method predicted optimum shear strength/MPa 18.07 Verification experiment results shear strength/MPa 17.88 为了更准确地研究各焊接工艺参数对CF/PPS热塑性复合材料层压板焊接接头质量影响的大小,对表2中信噪比(S/N)进行方差分析,如表4所示。可以看出,各参数方差均值和贡献分数表明焊接电流强度为CF/PPS热塑性复合材料焊接剪切强度的最主要影响因素,其贡献值为83.37%,压力其次,贡献值为9.55%,时间贡献值为6.02%。同时所有参数均具有90%以上的置信度。
表 4 CF/PPS复合材料层压板焊接接头信噪比方差分析Table 4. Analysis of variance of signal-to-noise ratio of CF/PPS composite laminate welded jointsANOVA-Signal-to-noise ratio values Process parameters Level mean value SS df Variance F Sig. P Level 1 Level 2 Level 3 Level 4 Factor A(Current) 14.30 20.01 22.90 18.64 153.704 3 51.235 156.957 0 83.37 Factor B(Pressure) 17.56 19.37 20.40 18.52 17.600 3 5.867 17.972 0.02 9.55 Factor C(Time) 17.67 18.80 19.75 19.62 11.107 3 3.702 11.343 0.05 6.02 Errors – – – – 1.959 6 0.326 – – 1.06 Total – – – – 184.370 15 – – – 100.00 Notes: SS—Sum of squares of mean deviation; df—Degrees of freedom; F—F statistics; Sig.—Significance; P—Probability. 2.3 CF/PPS复合材料电阻焊接剪切断口的微观形貌
图8和图9为H-LSS和L-LSS两类试样的截面形貌和剪切断口形貌。
从图8可以看出,最佳焊接工艺参数焊接的H-LSS试样焊接接头中CF/PPS复合材料混编织物、PPS膜及接层板紧密结合,焊缝中无空隙及脱黏区域等缺陷,CF/PPS复合材料混编织物很好地植入到焊接层板结合界面,且PPS膜和层板PPS基体很好地融合、扩渗及结合。而L-LSS试样的焊缝中存在空隙,CF/PPS复合材料混编织物中CF和PPS树脂的浸润较差,有大量裸纤维存在,表明其焊缝结合较差。
由图9 可以看出,植入的CF/PPS复合材料混编织物断裂,焊接层板的焊接界面也有纤维拔出和断裂,表明焊接接头在剪切力作用时,CF/PPS复合材料混编织物起到了焊缝增强相作用,且与焊接层板很好地结合,是典型复合材料层板层间失效断裂破坏模式,即焊接层板和CF/PPS复合材料混编织物都受损失效。H-LSS试样中植入的CF/PPS复合材料混编织物的纤维断裂平齐,纤维上黏附大量树脂,与之对应黏结的层板表面树脂基体在纤维拔出后出现明显破碎和塑性变形,证明CF/PPS复合材料混编织物、PPS膜及层板焊接界面间树脂与纤维充分浸润、扩渗链接和结合,保证了力的有效传递。而 L-LSS试样在剪切力作用下焊接接头失效形式为CF/PPS复合材料混编织物与焊接层板界面脱黏失效,即CF/PPS复合材料混编织物和被焊接层板的焊接界面胶层脱黏。这是由于在该工艺参数下树脂和纤维的浸润差,焊缝区域边缘处有部分PPS膜未完全熔化,焊缝区域存在大量空隙和裸纤维(如图8 中L-LSS试样),在剪切力作用下诱发裂纹产生,导致接头性能较低;由图9 也可看出,L-LSS试样中CF/PPS复合材料混编织物的纤维与树脂的浸润和包裹较差,拔出纤维上树脂附着很少且不均匀,与之黏接的层板树脂基体上有明显纤维压痕,在剪切力作用下,CF/PPS复合材料混编织物中的纤维拔出,而层板树脂无明显形变,表明其结合强度较弱,剪切强度低。
由上述实验结果及分析可知,CF/PPS复合材料层板电阻焊接工艺参数(电流、压力和时间)的变化直接影响着焊接接头的性能。随着电流强度的增加,焊接接头剪切强度呈先增大后减小的趋势,这是由于在较低电流时,CF混编织物电阻元件电热作用产生的焦耳热不足以充分软化和熔化PPS树脂,使焊缝处树脂扩渗和黏接不够充分,导致焊接接头剪切强度低;在较大电流时,CF电阻元件产生足够的焦耳热,使被焊接件的焊接界面充分均匀受热,PPS树脂充分受热软化、流动、扩渗和熔融胶接,使焊接接头剪切强度增大。但电流强度过大,产生热量过大,会使PPS树脂产生分子链断裂、热降解和烧蚀变性等不良影响[7],使焊接接头性能下降。CF/PPS复合材料层板电阻焊接需要一定的压力,由于熔融态PPS黏度较大,且流动困难,其流动是若干分子链段运动推动另一部分分子链段再运动并形成整个大分子重心移动[18],因此焊接压力是热塑性树脂基复合材料焊接的重要因素之一,压力过低时,不足以使受热软化和熔融的树脂充分流动与纤维浸润,无法排除焊缝中的气体,会增加焊缝中空隙等缺陷[19-20];而压力过高,会使焊缝软化和热熔树脂挤压排出,使焊缝的树脂含量过低,从而影响焊缝的有效黏接,因此,焊接压力过高或过低都会导致其剪切强度减小。焊接时间为电流流经CF混编织物电阻元件产生热量持续的过程,保证焊接接头所需热量,其对焊接接头性能的影响与焊接压力类似,焊接时间短,会引起树脂流动、扩渗和浸润不充分;焊接时间过长会导致焊接件的焊接界面树脂热解和烧蚀,同时引起焊接接头整体软化及纤维排布变形,使剪切强度减小。
3. 结 论
对航空结构用碳纤维/聚苯硫醚(CF/PPS)热塑性复合材料层压板,通过植入CF/PPS复合材料混编织物电阻元件进行电阻焊接技术研究。
(1)采用植入CF/PPS复合材料混编织物电阻元件对CF/PPS复合材料层板进行电阻焊接,工艺简单,设备成本低,焊接接头无异质材料引入,无明显应力集中。
(2) Taguchi方法可用于CF/PPS复合材料电阻焊接工艺参数设计和优化分析中,优化预测得到最佳焊接工艺参数为:电流为12 A,压力为1.5 MPa,时间为30 min,剪切强度为18.07 MPa;采用该参数验证CF/PPS复合材料层板焊接剪切强度为17.88 MPa,与其预测结果相近。方差分析得到CF/PPS复合材料层压板电阻焊接工艺参数电流贡献率最高,为83.37%,其次是压力,为9.55%,时间贡献率为6.02%。
(3) CF/PPS复合材料层压板电阻焊接接头横截面和剪切断口观察分析表明,最佳参数焊接试样(H-LSS)焊接接头界面结合良好,CF/PPS复合材料混编织物很好融合进焊缝,主要剪切失效形式为层间失效。较低剪切强度试样(L-LSS)焊接接头失效形式主要为界面脱黏失效。
(4)电阻焊接技术可以推广于商业和工业任何类型热塑性树脂基复合材料的连接。
-
-
[1] 杜善义, 关志东. 我国大型客机先进复合材料技术应对策略思考[J]. 复合材料学报, 2008, 25(1):1-10. DOI: 10.3321/j.issn:1000-3851.2008.01.001 DU Shanyi, GAUN Zhidong. Strategic considerations for development of advances composite technology for large commercial aircraft in china[J]. Acta Materiae Compo-sitae Sinica,2008,25(1):1-10(in Chinese). DOI: 10.3321/j.issn:1000-3851.2008.01.001
[2] 赵丽滨, 龚愉, 张建宇. 纤维增强复合材料层合板分层行为研究进展[J]. 航空学报, 2019, 40(1):166-194. ZHAO Libin, GONG Yu, ZHANG Jianyu. A survey on delamination growth behavior in fiber reinforced compo-site laminates[J]. Acta Aeronautica et Astronautica Sinica,2019,40(1):166-194(in Chinese).
[3] 庄蔚敏, 王楠, 吴迪, 等. 碳纤维复合材料层合板三点弯曲损伤仿真研究[J]. 机械工程学报, 2019, 55(10):109-114. DOI: 10.3901/JME.2019.10.109 ZHUANG Weimin, WANG Nan, WU Di, et al. Simulation and analysis of the damage of carbon fiber composite laminates under three point bending load[J]. Journal of Mechanical Engineering,2019,55(10):109-114(in Chinese). DOI: 10.3901/JME.2019.10.109
[4] HILLERBORG A, MODEER M, PETERSSON P. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements[J]. Cement and Concrete Research,1976,6(6):773-782. DOI: 10.1016/0008-8846(76)90007-7
[5] CARTER B, WAWRZYNEK P, INGRAFFEA A. Automated 3-D crack growth simulation[J]. International Journal for Numerical Methods in Engineering,2000,47(3):229-258.
[6] KIM J, SIMONE A, DUARTE C. Mesh refinement strategies without mapping of nonlinear solutions for the generalized and standard FEM analysis of 3-D cohesive fractures[J]. International Journal for Numerical Methods in Engineering,2017,109(2):235-258. DOI: 10.1002/nme.5286
[7] KRUEGER R. Virtual crack closure technique: History, approach, and applications[J]. Applied Mechanics Reviews,2004,57(2):109-143. DOI: 10.1115/1.1595677
[8] HEIDARI-RARANI M, SAYEDAIN M. Finite element modeling strategies for 2D and 3D delamination propagation in composite DCB specimens using VCCT, CZM and XFEM approaches[J]. Theoretical and Applied Fracture Mechanics,2019,103(3):102246.
[9] OKINEN J, KANERVA M. Simulation of delamination growth at CFRP-tungsten aerospace laminates using VCCT and CZM modelling techniques[J]. Applied Composite Materials,2019,26(7):709-721.
[10] RYBICKI E F, KANNINEN M F. A finite element calculation of stress intensity factors by a modified crack closure integral[J]. Engineering Fracture Mechanics,1997,9(4):931-938.
[11] SHIVAKUMAR K N, TAN P W, NEWMAN J J. A virtual crack-closure technique for calculating stress intensity factors for cracked three dimensional bodies[J]. International Journal of Facture,1988,36(1):43-50.
[12] RAJU I S, SISTLA R, KRISHNAMURTHY T. Fracture mechanics analyses for skin-stiffener debondingt[J]. Engineering Fracture Mechanics,1996,54(3):371-385. DOI: 10.1016/0013-7944(95)00184-0
[13] XIE D, SHERRILL B, BIGGERS J. Strain energy release rate calculation for a moving delamination front of arbitrary shape based on the VCCT: Part I and Part II[J]. Engineering Fracture Mechanics,2006,73(6):771-785. DOI: 10.1016/j.engfracmech.2005.07.013
[14] FAWAZ S A. Application of virtual crack closure technique to calculate stress intensity factors for through cracks with an elliptical crack front[J]. Engineering Fracture Mechanics,1998,59(3):327-342. DOI: 10.1016/S0013-7944(97)00126-4
[15] IRWIN G R. One set fast crack propagation in high steel and aluminum alloys[C]. Sagamore Research Conference Proceedings, 1956, 2(32): 289-305.
[16] 王自强, 陈少华. 高等断裂力学(精)[M]. 北京: 科学出版社, 2009: 124-140. WANG Ziqiang, CHEN Shaohua. Higher fracture mechanics[M]. Beijing: Science Publishing, 2009: 124-140(in Chinese).
[17] GRIFFITH A A. The phenomena of rupture and flow in solids[J]. Philosophical Transactions of the Royal Society of London A,1921,221(6):163-198.
[18] PIETROPAOLI Elisa, RICCIO Aniello. Formulation and assessment of an enhanced finite elementprocedure for the analysis of delamination growth phenomena in composite structures[J]. Composites Science and Technology,2011,71(6):836-846. DOI: 10.1016/j.compscitech.2011.01.026
[19] RAIMONDO A, RICCIO A. Inter-laminar and intra-laminar damage evolution in composite panels with skin-stringer debonding under compression[J]. Composites Part B: Engineering,2016,94(12):139-151.
[20] LI H C H, DHARMAWAN F, HERSZBERG I, et al. Fracture behaviour of composite maritime T-joints[J]. Composite Structures,2006,75(1):339-350.
[21] TAWK I, NAVARRO P, FERRERO J F, et al. Composite delamination modelling using a multi-layered solid element[J]. Composites Science and Technology,2010,70(2):207-214. DOI: 10.1016/j.compscitech.2009.10.008
[22] PANIGRAHI S K, PRADHAN B. Three dimensional failure analysis and damage propagation behavior of, adhesively bonded single lap joints in laminated FRP composites[J]. Journal of Reinforced Plastics and Composites,2007,26(2):183-201. DOI: 10.1177/0731684407070026
[23] 周立明, 孟广伟, 王晖, 等. 基于光滑有限元的含裂纹复合材料的虚拟裂纹闭合法[J]. 湖南大学学报(自然科学版), 2014, 41(8):36-40. ZHOU Liming, MENG Guangwei, WANG Hui, et al. Virtual crack closure technique based on smoothed finite element method for composite materials with cracks[J]. Journal of Hunan University (Natural Science),2014,41(8):36-40(in Chinese).
[24] 陈玉良, 程宸, 万水, 等. 基于虚拟裂纹闭合法计算组合材料的应变能释放率[J]. 玻璃钢/复合材料, 2013, 4(3):8-12. CHEN Yuliang, CHENG Chen, WAN Shui, et al. Based on virtual crack close technique the calculation of strain energy release rete for composite material[J]. Journal of FRP/Composites,2013,4(3):8-12(in Chinese).
[25] 孟令兵, 陈普会. 层压复合材料分层扩展分析的虚拟裂纹闭合技术及其应用[J]. 复合材料学报, 2010, 27(1):190-195. MENG Lingbing, CHEN Puhui. Virtual crack closure technique for delamination growth analysis of laminated composites and its application[J]. Acta Materiae Compositae Sinica,2010,27(1):190-195(in Chinese).
[26] MAGI F, MAIO D D, SEVER I. Validation of initial crack propagation under vibration fatigue by Finite Element analysis[J]. International Journal of Fatigue,2017,104(6):183-194.
[27] CARVALHO N V D, KRUEGER R, MABSON G E, et al. Combining progressive nodal release with the virtual crack closure technique to model fatigue delamination growth without re-meshing[C]//2018 AIAA/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference. 2018.
[28] 杜洪雨, 奚晓波, 孟力华, 等. 含分层损伤的复合材料层压板后屈曲及低周疲劳分层扩展有限元模拟研究[J]. 玻璃钢/复合材料, 2018(6):39-43. DU Hongyu, XI Xiaobo, MENG Lihua, et al. Finite element of post-buckled delamination of composite laminate with preliminary debond subjected to static and fatigue loads[J]. Journal of FRP/Composites,2018(6):39-43(in Chinese).
[29] 孙益军, 林智育. 含分层损伤复合材料层板压缩分层疲劳扩展研究[J]. 航空制造技术, 2015(9):77-81. SUN Yijun, LIN Zhiyu. Progressive delamination growth in composites laminates under compressive fatigue load[J]. Aeronautical Manufacturing Technology,2015(9):77-81(in Chinese).
[30] 熊伟华. 含分层损伤复合材料层合板疲劳分层扩展研究[D]. 南京: 南京航空航天大学, 2015. XIONG Weihua. Delamination growth in composite laminates[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2015(in Chinese).
[31] DUGDALE D S. Yielding of steel sheets containing slits[J]. Journal of Mechanics & Physics of Solid,1960,8(2):100-104.
[32] BARENBLATT G I. The mathematical theory of equilibrium cracks in brittle fracture[J]. Advances in Applied Mechanics,1962,7(8):55-129.
[33] NEEDLEMAN A. A continuum model for void nucleation by inclusion debonding[J]. Journal of Applied Mechanics,1987,54(3):525-531. DOI: 10.1115/1.3173064
[34] NEEDLEMAN A. An analysis of decohesion along an imperfect interface[J]. International Journal of Fracture,1990,42(5):21-40.
[35] LIU P F, ISLAM M M. A nonlinear cohesive model for mixed-mode delamination of composite laminates[J]. Composite Structures,2013,106(6):47-56.
[36] TVERGAARD V, HUTCHINSON J W. The relation between crack growth resistance and fracture process parameters in elastic-plastic solids[J]. Journal of the Mechanics and Physics of Solids,1992,40(6):1377-1397. DOI: 10.1016/0022-5096(92)90020-3
[37] MI Y, CRISFIELD M A, DAVIES G A O, et al. Progressive delamination using interface elements[J]. Journal of Composite Materials,1998,32(14):1246-1272. DOI: 10.1177/002199839803201401
[38] ALLIX O, LADEVÉZE P, CORIGLIANO A. Damage analysis of interlaminar fracture specimens[J]. Composite Structures,1995,31(1):61-74. DOI: 10.1016/0263-8223(95)00002-X
[39] ALLIX O, CORIGLIANO A. Modeling and simulation of crack propagation in mixed-modes interlaminar fracture specimens[J]. International Journal of Fracture,1996,77(2):111-140. DOI: 10.1007/BF00037233
[40] CHAMPANEY L, VALOROSO N. Evaluation of interface models for the analysis of non-linear behaviour of adhesively bonded joints[C]. Proceedings of the European Conference on Computational Mechanics ECCM-2001, Cracow, 2001.
[41] 卢子兴. 复合材料界面的内聚力模型及其应用[J]. 固体力学学报, 2015, 36(S1):85-94. LU Zixing. A simple review for cohesive zone models of composite interface and their applications[J]. Chinese Journal of Solid Mechanics,2015,36(S1):85-94(in Chinese).
[42] RICHEFEU V, CHRYSOCHOOS A, HUON V, et al. Toward local identification of cohesive zone models using digital image correlation[J]. European Journal of Mechanics, A/Solids,2012,34(27):38-51.
[43] J. REINOSO, M. PAGGI, A. BLAZQUEZ. A nonlinear finite thickness cohesive interface element for modelig delamination in fibre-reinforced composite laminates[J]. Compo-sites Part B: 2017, 40(5): 116-128.
[44] HAN T S, URAL A, CHEN C S, et al. Delamination buckling and propagation analysis of honeycomb panels using a cohesive element approach[J]. International Journal of Fracture,2002,115(2):101-123. DOI: 10.1023/A:1016333709040
[45] RIDHA M, TAN V B C, TAY T E. Traction separation laws for progressive failure of bonded scarf repair of composite panel[J]. Composite Structures,2011,93(4):1239-1245. DOI: 10.1016/j.compstruct.2010.10.015
[46] 张鹏, 胡小飞, 姚伟岸. 内聚力模型裂纹问题分析的解析奇异单元[J]. 固体力学学报, 2017, 38(2):68-75. ZHANG Peng, HU Xiaofei, YAO Weian. An analytical singular element to study the cohesive zone model for cracks[J]. Chinese Journal of Solid Mechanics,2017,38(2):68-75(in Chinese).
[47] 王振清, 雷红帅, 周博, 等. 基于内聚力模型的形状记忆合金短纤维增强树脂基复合材料的模拟分析[J]. 复合材料学报, 2012, 24(5):236-243. WANG Zhenqing, LEI Hongshuai, ZHOU Bo. Simulation and analysis on short-cut shape memory alloy reinforced epoxy composite based on cohesive zone model[J]. Acta Materiae Compositae Sinica,2012,24(5):236-243(in Chinese).
[48] WAAS V D, HIDAYAT M I P, NOEROCHIM L. Finite element simulation of delamination in carbon fiber/epoxy laminate using cohesive zone model: Effect of meshing variation[J]. Materials Science Forum,2019,964(7):257-262.
[49] SADIQ M A, ZAFAR N, BUTT H S U, et al. An efficient approach to the design of damage tolerant CFRP stiffened panels[C] 2017 14th International Bhurban Conference on Applied Sciences and Technology (IBCAST). IEEE, 2017: 58-65.
[50] NIAN G, LI Q, XU Q, et al. A cohesive zone model incorporating a Coulomb friction law for fiber-reinforced compo-sites[J]. Composites Science and Technology,2018,34(157):195-201.
[51] 关志东, 刘德博, 李星, 等. 基于界面单元的复合材料层间损伤分析方法[J]. 复合材料学报, 2012, 29(2):136-140. GAUN Zhidong, LIU Debo, LI Xing, et al. Composite interlaminar damage analysis based on cohesive element[J]. Composites Science and Technology,2012,29(2):136-140(in Chinese).
[52] 尹涵彬. 薄膜/基底界面撕脱全过程力学行为的定量预测[D]. 北京: 中国科学院大学, 2018. YIN Hanbin. Quantitative prediction of mechanical behavior in the whole process of film/substrate interface avulsion[D]. Beijing: Chinese Academy of Sciences, 2018(in Chinese).
[53] 孙中雷, 赵美英, 万小朋. 含分层复合材料层合板剪切稳定性数值模拟研究[J]. 机械强度, 2013, 35(5):641-645. SUN Zhonglei, ZHAO Meiying, WAN Xiaopeng. A numerical study on shear behavior of composite plate with a circular delamination[J]. Journal of Mechanical Strength,2013,35(5):641-645(in Chinese).
[54] 尹世豪, 张建宇, 龚愉, 等. 新型三线性本构内聚力模型的界面参数研究[J]. 重庆大学学报, 2020, 43(2):1-8. DOI: 10.11835/j.issn.1005-2909.2020.02.001 YIN Shihao, ZHANG Jianyu, GONG Yu, et al. Studies on the interfacial parameters in a novel three-linear cohesive zone model[J]. Journal of Chongqing University,2020,43(2):1-8(in Chinese). DOI: 10.11835/j.issn.1005-2909.2020.02.001
[55] GUTKIN R, LAFFAN M L, PINHO S T, et al. Modeling the R-curve effect and its specimen-dependence[J]. International Journal of Solids and Structures,2011,48(11):1767-1777.
[56] 段红燕, 王智明, 桑元成. III型裂纹裂尖应力场的内聚力模型[J]. 上海交通大学学报(自然版), 2017, 51(1):113-118. DUAN Hongyan, WANG Zhiming, SHAN Yuancheng. Cohesive zone modeling for model III crack based on near tip stress field[J]. Journal of Shanghai Jiao Tong University (Nature Edition),2017,51(1):113-118(in Chinese).
[57] 刘斌, 徐绯, 菊池正纪, 等. 斜胶接CFRP的冲击损伤容限研究[J]. 固体火箭技术, 2015, 38(6):870-876. LIU Bin, XU Fei, KIKUCHI Masanori, et al. Study on impact damage tolerance of scarf bonded CFRP[J]. Journal of Solid Rocket Technology,2015,38(6):870-876(in Chinese).
[58] 朱炜垚, 许希武. 复合材料层合板低速冲击损伤的有限元模拟[J]. 复合材料学报, 2010, 27(6):200-207. ZHU Weiyao, XU Xiwu. Finite element simulation of low velocity impact damage on composite laminates[J]. Acta Materiae Compositae Sinica,2010,27(6):200-207(in Chinese).
[59] FAGGIANI A, FALZON B G. Predicting low-velocity impact damage on a stiffened composite panel[J]. Compo-sites: Part A, 2010, 41(6): 737-749.
[60] 于飞. 弹塑性断裂过程与复合材料层板低速冲击损伤的数值模拟[D]. 南京: 南京航空航天大学, 2012. YU Fei. Numerical study on elastoplastic fracture process and low-velocity impact damage of laminated composites[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2012(in Chinese).
[61] LI S, THOULESS M D, WAAS A M, et al. Mixed-mode cohesive-zone models for fracture of an adhesively bonded polymer-matrix composite[J]. Engineering Fracture Mechanics,2006,73(1):64-78. DOI: 10.1016/j.engfracmech.2005.07.004
[62] LI S, THOULESS M D, WAAS A M, et al. Use of a cohesive-zone model to analyze the fracture of a fiber-reinforced polymer-matrix composite[J]. Composites Science & Technology,2005,65(3-4):537-549.
[63] BORG R, NILSSON L, SIMONSSON K. Simulating DCB, ENF and MMB experiments using shell elements and a cohesive zone model[J]. Composites Science and Technology,2004,64(2):269-278. DOI: 10.1016/S0266-3538(03)00255-0
[64] BORG R, NILSSON L, SIMONSSON K. Modeling of delamination using a discretized cohesive zone and damage formulation[J]. Composites Science and Technology,2002,62(10):1299-1314.
[65] RICE J R, BELTZ G E. The activation-energy for dislocation nucleation at a crack[J]. Journal of the Mechanics and Physics of Solids, 1994, 42(6): 333-360.
[66] TURON A, DÁVILA C G, CAMANHO P P, et al. An engineering solution for mesh size effects in the simulation of delamination using cohesive zone models[J]. Engineering Fracture Mechanics,2007,74(10):1665-1682. DOI: 10.1016/j.engfracmech.2006.08.025
[67] 郭历伦, 陈忠富, 罗景润, 等. 扩展有限方法即应用综述[J]. 力学季刊, 2011, 32(4):144-157. GUO Lilun, CHEN Zhongfu, LUO Jingrun, et al. A review of the extended finite element method and its applications[J]. Chinese Quarterly of Mechanics,2011,32(4):144-157(in Chinese).
[68] 丁晶. 扩展有限元在断裂力学中的应用[D]. 南京: 河海大学, 2007. DING Jing. Application of the extend finite element method to fracture mechanics[D]. Nanjing: Hohai University, 2007(in Chinese).
[69] 王哲成, 张云, 于军, 等. 基于扩展有限元的应力强度因子计算精度研究[J]. 南京大学学报, 2019, 55(3):361-369. WANG Zhecheng, ZHANG Yun, YU Jun, et al. Calculation of stress intensity factor based on the extended finite element method[J]. Journal of Nanjing University,2019,55(3):361-369(in Chinese).
[70] BELYTSCHKO T, CHEN H, XU J, et al. Dynamic crack propagation based on loss if hyperbolicity and a new discontinuous enrichment[J]. International Journal for Numerical Methods in Engineerinf,2003,58(12):1873-1905. DOI: 10.1002/nme.941
[71] FRIES T P. A corrected XFEM approximation with out problems in blending elements[J]. International Journal for Numerical Methods in Engineering,2008,75(5):503-532. DOI: 10.1002/nme.2259
[72] MOES N, CLOIREC M, CARTRAUD P, et al. A computational approach to handle complex microstructure geometries[J]. Computer Methods in Applied Mechanics & Engineering,2003,192(28):3163-3177.
[73] 林铁军, 练章华, 曾晓健, 等. 应用XFEM模拟研究钻杆裂纹扩展过程[J]. 重庆大学学报, 2010, 33(7):123-128. LIN Tiejun, LIAN Zhanghua, ZENG Xiaojian, et al. Simulation on crack growth of drill pipe with XFEM[J]. Journal of Chongqing University,2010,33(7):123-128(in Chinese).
[74] OSHER S, SETHIAN J. Fronts propagating with curvature dependent speed: Algorithms based on Hamilton Jacobi formulations[J]. Journal of Computational Physics,1988,79(1):12-49. DOI: 10.1016/0021-9991(88)90002-2
[75] GROGAN D M, BRDALGH C, LEEN S B. A combined XFEM and cohesive zone model for composite laminate microcracking and permeability[J]. Composite Structures,2015,120(7):246-261.
[76] GROGAN D M, BRDALGH C, MCGARRY J P, et al. Damage and permeability in tape-laid thermoplastic composite cryogenic tanks[J]. Composites Part A: Applied Science and Manufacturing,2015,78(4):390-402.
[77] NAGASHIMA T, SUEMASU H. XFEM analysis of a thin-walled composite shell structure with a delamination[J]. Computers & Structures, 2010, 88(9): 549-557.
[78] CURIEL SOSA J, KARAPURATH N. Delamination modelling of GLARE using the extended finite element method[J]. Composites Science and Technology, 2012, 72(7): 788-791.
[79] JIN C F, ZHU Q Z, SHAO J F. A numerical analysis of interface damage effect on mechanical properties of composite materials[J]. Mechanics Research Communications,2014,62(4):18-24.
[80] ZHAO L B, ZHI J, ZHANG J Y, at al. XFEM simulation of delamination in composite laminates[J]. Composites Part A: Applied Science and Manufacturing,2016,80(7):61-71.
[81] ZHAO L B, ZHI J, ZHANG J Y, at al. XFEM based model for simulating zigzag delamination growth in laminated composites under mode I loading[J]. Composite Structures,2017,160(7):1155-1162.
[82] 段青枫. 基于LaRC05和XFEM的复材构件失效行为数值研究[D]. 武汉: 武汉理工大学, 2019. DUAN Qingfeng. Numerical study on failure behavior of composite structure components based on LaRC05 and XFEM[D]. Wuhan: Wuhan University of Technology, 2019(in Chinese).
[83] 周立明, 孟广伟, 李峰, 等. 含裂纹复合材料的Cell-based光滑扩展有限元法[J]. 东北大学学报: 自然科学版, 2016, 8(37):1127-1132. ZHOU Liming, MENG Guangwei, LI Feng, et al. Cell-based smoothed extended finite element method for composite materials with cracks[J]. Journal of Northeastern University: Natural Science,2016,8(37):1127-1132(in Chinese).
[84] CHANG Y E, SHI J, GARY J. An extended finite element study on the effect of reinforcing particles on the crack propagation behavior in a metal matrix composite[J]. International Journal of Fatigue, 2012, 44(1): 151-156.
[85] 杨宇宙, 钱林方, 徐亚栋, 等. 复合材料身管的疲劳裂纹扩展分析[J]. 弹道学报, 2013, 25(2):100-105. DOI: 10.3969/j.issn.1004-499X.2013.02.020 YANG Yuzhou, QIAN Linfang, XU Yadong, et al. Analysis of fatigue crack growth of composite barrel[J]. Journal of Ballistics,2013,25(2):100-105(in Chinese). DOI: 10.3969/j.issn.1004-499X.2013.02.020
[86] 王志勇, 马力, 吴林志, 等. 基于扩展有限元法的颗粒增强复合材料静态及动态断裂行为研究[J]. 固体力学学报, 2011, 32(6):566-573. WANG Zhiyong, MA Li, WU Linzhi, et al. Investigation of static and dynamic fracture behavior of particle-reinforced composite materials by the extended finite element method[J]. Chinese Journal of Solid Mechanics,2011,32(6):566-573(in Chinese).
[87] 屈琼. 基于实验的复合材料层合板的界面损伤分析及界面力学性能研究[D]. 福州: 福州大学, 2010. QU Qiong. Experiment-based analysis of interfacial damage in laminates and study on interfacial mechanical properties[D]. Fuzhou: Fuzhou University, 2010(in Chinese).
[88] 胡振东, 黄海, 贾光辉. 超高速撞击条件下铝合金材料参数识别方法[J]. 北京航空航天大学学报, 2008, 34(9):99-102. HU Zhendong, HUANG Hai, JIA Guanghui. Identification method for the material parameters of alloy under the condition of hypervelocity impact[J]. Journal of Beijing University of Aeronautics and Astronautics,2008,34(9):99-102(in Chinese).
[89] BOLZON G, GHILOTTI D, MAIER G. Strength of periodic elastic-brittle composites evaluated through homogenization and parameter identification[J]. European Journal of Mechanics A/Soilds, 2002, 21(7): 355-378.
-
期刊类型引用(7)
1. 钱小琴. 基于3D打印的热塑性聚氨酯防护材料性能分析及应用. 粘接. 2025(03): 111-114 .  百度学术
百度学术
2. 李世雄,侯琳,蔡普宁,刘泱,樊威. 纺织用X射线防护材料的研究进展. 棉纺织技术. 2024(03): 89-94 .  百度学术
百度学术
3. 李昊,吴承承,王志新,王亚平,廖学品,石碧. 钆-钡/天然皮革复合可穿戴X射线屏蔽材料的制备及性能. 皮革科学与工程. 2024(03): 9-16 .  百度学术
百度学术
4. 邱淑秀,马杨,汤新颖. 一种屏蔽X射线的新型纳米复材性能测试及应用效果研究. 粘接. 2024(06): 115-118 .  百度学术
百度学术
5. 雷超,许维星,曾运航,石碧. 硅烷改性胶原纤维/聚氯乙烯复合材料的界面相容性及其高弹抗蠕变性研究. 复合材料学报. 2024(06): 2923-2934 .  本站查看
本站查看
6. 王博宇,张栋栋,袁继龙,陆地,王琦,刘洋. 无铅柔性高分子复合材料X射线屏蔽性能研究. 辐射防护. 2024(06): 695-704 .  百度学术
百度学术
7. 冯斯涵,韩刘佳,陈长洁,王新厚. 纤维基轻质高效X射线防护材料的制备及性能探究. 产业用纺织品. 2024(12): 20-26 .  百度学术
百度学术
其他类型引用(0)
-





 下载:
下载: