基于高Q腔法测试氮化硅纤维的介电性能

李庆辉; 孔维纳; 李喆; 王少敏; 王绍凯; 顾轶卓; 李敏

doi:10.13801/j.cnki.fhclxb.20200115.003

基于高Q腔法测试氮化硅纤维的介电性能

北京航空航天大学　材料科学与工程学院，北京 100191

详细信息

通讯作者:
李敏，教授，博士生导师，研究方向为先进树脂基复合材料　E-mail：leemy@buaa.edu.cn

中图分类号: TB332
计量
- 文章访问数: 1233
- HTML全文浏览量: 475
- PDF下载量: 65
出版历程
- 收稿日期: 2019-10-15
- 录用日期: 2020-01-13
- 网络出版日期: 2020-01-15
- 刊出日期: 2020-09-14

Dielectric properties measurements on silicon nitride fiber based on high-Q cavity method

School of Materials Science and Engineering, Beihang University, Beijing 100191, China

HTML全文

摘要

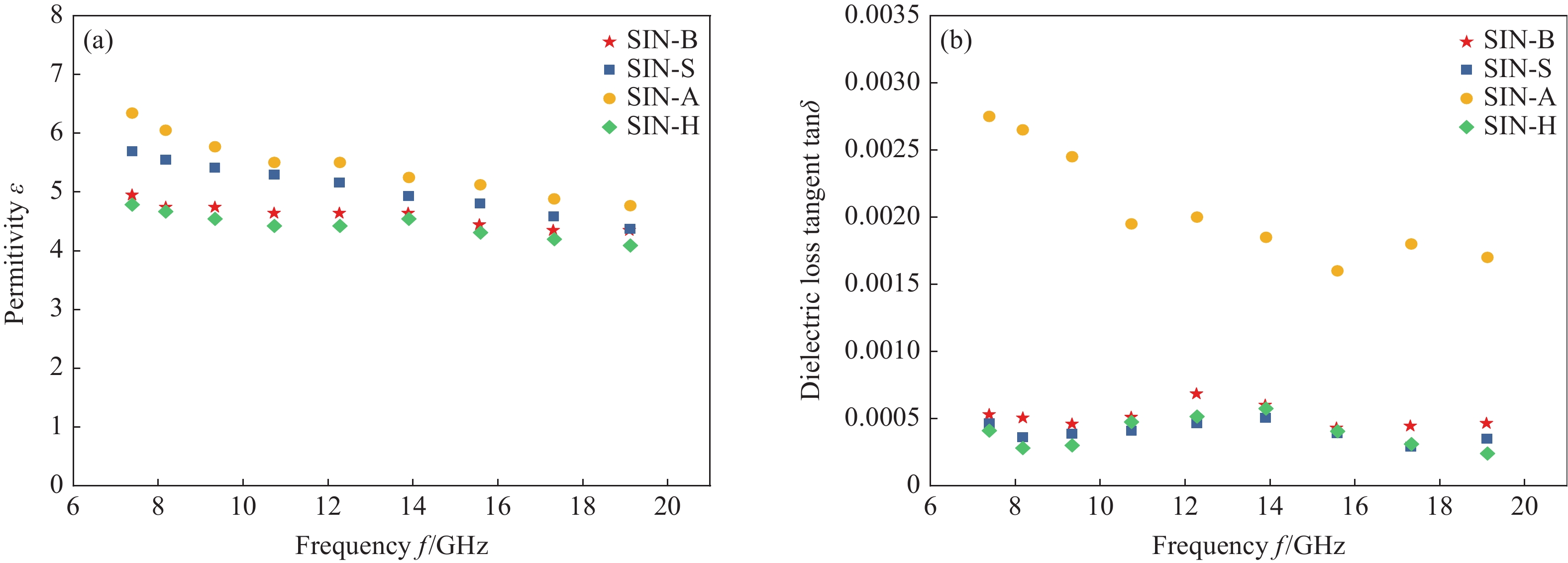
摘要: 氮化硅纤维具有优异的耐高温性能和透波能力，是理想的高温透波增强材料。本文对高Q腔法测试陶瓷纤维介电性能的样品制备和测试方法进行了研究和优化。研究发现，介电测试试样中纤维含量应不低于20wt%，含量过低易导致计算所得的纤维介电常数偏低；同时短切纤维的长度主要影响介电测试数据的离散性，由长度不大于1.0 mm的短纤维所制试样质量高，介电测试结果稳定性更佳。对比讨论了Lichtenecker、Bruggeman和Looyenga三种介电混合模型的适用性，最终基于Lichtenecker介电常数对数混合法则计算得到石英纤维的相对介电常数与文献报道数据较一致。分析表明，氮化硅纤维在10 GHz频率下的相对介电常数为4.4，损耗角正切值为0.0005，是优异的低介电高透波材料。同时，表面上浆剂通过改变纤维表面极性特征，对氮化硅纤维介电性能尤其介电损耗产生显著影响。
- 陶瓷纤维 /
- 介电性能 /
- 高透波材料 /
- 高Q腔法 /
- 表面极性
Abstract: The silicon nitride fiber has excellent high temperature resistance and wave transmission capability, which is an ideal reinforcement using for high-temperature wave-transparent composites. In this paper, preparation and test methods for the dielectric properties of ceramic fibers tested by high-Q cavity method were studied and optimized for continuous ceramic fibers. The study shows that the fiber content in the dielectric test sample should be no less than 20wt%, and less fiber content tended to result in a relatively smaller value of the fiber dielectric constant. Meanwhile, the length of chopped fiber has effect on the repeatability of the fiber dielectric properties. The samples made by the chopped fiber with the length no more than 1.0 mm are of high quality and the resulted fiber dielectric data are more reliable. In terms of data processing, the applicability of three dielectric mixing models of Lichtenecker, Bruggeman and Looyenga was discussed in comparison. Finally, the permittivity of quartz fibers was calculated based on Lichtenecker dielectric constant logarithmic mixing rule, which was consistent with the reported data. It has been found that the silicon nitride fiber has a permittivity of 4.4 and a loss tangent of 0.0005 at 10 GHz, demonstrating excellent low dielectric. Furthermore, it shows that the surface sizing agent has a significant effect on the dielectric properties particularly the loss tangent of the silicon nitride fiber, which is associated with its surface polarity characteristics of the silicon nitride fiber.
- ceramic fiber /
- dielectric properties /
- high wave-transmissivity materials /
- high-Q cavity method /
- surface polarity

HTML全文

碳化钨颗粒增强钢铁基(WC_P/Fe)复合材料因兼顾金属基体的良好韧性与陶瓷增强颗粒的高强度、高硬度、高模量而广泛应用于机械制造、能源开发、交通运输等领域，但基体与增强颗粒间热物理性能差异过大使复合材料在激冷激热环境下服役时产生热应力，诱发界面处裂纹的萌生与扩展^[1-4]。而将WC充分溶解，W在熔体中均匀扩散形成合金化复合层能有效解决该问题，故研究WC/Fe复合材料中W扩散均匀性十分必要^[5-7]。

蜂窝结构因形状连续、比强度高等特性而对复合材料的综合性能产生重要影响^[8-10]。Magotteaux公司发明X-win蜂窝结构ZTA_P/Fe复合材料技术，制造的磨辊使用寿命提高两倍以上^[11]；WU等^[12]从模拟与实验角度出发，揭示预制体孔径与孔距作为蜂窝结构重要参数对复合材料力学性能的影响；SONG等^[13]成功制备蜂窝结构还原氧化石墨烯增强环氧树脂(rGH/EP)复合材料，电磁屏蔽效能与导电性明显提升。但对蜂窝预制体结构与元素扩散均匀性间的关联机制仍研究较少。

本文采用真空消失模铸渗(V-EPC)工艺制备WC/Fe复合材料^[14-15]，选取孔径孔距比相同孔径不同的蜂窝预制体，并将W质量分数最高与最低的预制体原孔壁与原孔心处W质量分数作为W扩散均匀性的判断条件^[16-17]。表征复合层的显微组织、物相组成、元素分布，并检测预制体原孔壁与原孔心处W质量分数、硬度及复合层耐磨性，揭示其孔径对W质量分数分布的影响规律。通过求解非稳态扩散方程得解析解，对预制体孔内熔体凝固时的热物理场进行有限元模拟，并通过二次开发程序对其原孔内W质量分数分布进行数值模拟，揭示其孔径对W扩散均匀性的影响机制；提出W扩散均匀性与复合层耐磨性间的关联机制，为工程应用提供理论依据。

1. 实验材料与方法

为避免铸渗时W粉因粒径过小而大量烧损，选取铸造WC颗粒(WC/W₂C_P)为合金化提供W；表1为WC/Fe复合材料中预制体的成分组成，配置预制体粉末300 g，与5wt%水玻璃粘结剂均匀混合；表2为WC/Fe复合材料中预制体的结构参数，填充到预制体孔径孔距比相同孔径不同的蜂窝模具内，其轮廓为50 mm×100 mm×6 mm；采用CO₂硬化与微波烧结方法，得最终预制体。

表3为WC/Fe复合材料中基体的成分组成。配置基体并采用中频感应炉熔炼20 kg。图1为WC/Fe复合材料的制备过程，采用V-EPC工艺成型，浇注温度为1 500℃，型腔负压为0.05 MPa。

表 1 WC/Fe复合材料中预制体的成分

Table 1. Composition of preform in WC/Fe composites

Composition	Mass fraction/wt%	Size/μm
WC	40	150-200
Ni₆₀	30	60-90
FeCr₅₅C_6.0	30	150-200

下载: 导出CSV

| 显示表格

表 2 WC/Fe复合材料中预制体的结构参数

Table 2. Structure parameters of preform in WC/Fe composites

Diameter R/mm	Distance d/mm	Number n
3	6	63
6	12	16
9	18	7

下载: 导出CSV

| 显示表格

表 3 WC/Fe复合材料中基体的成分

Table 3. Composition of matrix in WC/Fe composites

Composition	C	Cr	Mn	Si	Fe
Mass fraction/wt%	1.2-1.3	18.0-20.0	0.4-0.6	1.0-1.2	Balance

下载: 导出CSV

| 显示表格

图 1 WC/Fe复合材料的制备过程

Figure 1. Preparation process of WC/Fe composites ((a)V-EPC (Vacuum-expendable pattern casting); (b)Honeycomb preform; (c)Transformation between preform and layer)

下载: 全尺寸图片幻灯片

采用尼康MA200型OM表征复合层显微组织，并统计预制体原孔壁与原孔心处平均晶粒尺寸分布。采用岛津7000S/L型XRD、牛津仪器Ultim Extreme型EDS面扫描表征复合层物相组成、元素分布。采用牛津仪器Ultim Extreme型EDS点扫描、上海光学仪器厂HX1000型显微硬度计表征复合层预制体原孔壁与原孔心处元素质量分数、硬度。采用广州试验仪器厂MS-5E型三体磨料磨损机表征复合层耐磨性，载荷为2 kg、转速为40 r/min、预磨时间为30 min、磨粒粒径为200~550 μm，并采用蔡司EVO18型SEM表征预制体原孔心处磨损形貌。采用COMSOL Multiphysics 5.4有限元模拟预制体孔内熔体凝固时的热物理场。采用MATLAB R2015b通过二次开发程序数值模拟预制体原孔内W质量分数分布。

2. 结果与分析

2.1 WC/Fe复合材料复合层的显微组织与元素分布

图2为不同预制体孔径下WC/Fe复合材料复合层的显微组织。熔体填充预制体孔洞，WC高温下分解，W由其孔壁扩散至孔心处，形成复合层。图3为不同预制体孔径下WC/Fe复合材料复合层原孔内的平均晶粒尺寸分布，随预制体孔径增加，其原孔壁处晶粒尺寸基本不变，而在其原孔心处先减小后增大。

图 3 不同预制体孔径下WC/Fe复合材料复合层原孔内的平均晶粒尺寸分布

Figure 3. Average grain size distribution into initial hole of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

图4为不同预制体孔径下WC/Fe复合材料复合层原孔内的平均晶粒尺寸分布。表明复合层中均形成W₂C、WC、Ni₁₇W₃、Fe₃W₃C、(Fe,Cr)₃C。根据W-C相图，WC/W₂C_P中WC分解形成W₂C、C，而C扩散到熔体中使(Fe,Cr)₃C增多；根据Fe-W-C相图，熔体与W₂C发生包晶反应形成Fe₃W₃C；WC、Ni₆₀分解使W、Ni质量分数增加，形成镍钨化合物Ni₁₇W₃。

图 2 不同预制体孔径下WC/Fe复合材料复合层的显微组织

Figure 2. Microstructure of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

图 4 不同预制体孔径下WC/Fe复合材料复合层的物相组成

Figure 4. Phase composition of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

根据Fe-Cr-C相图^[18-19]，推测熔体(Cr：18.0wt%~20.0wt%，C：1.2wt%~1.3wt%)为典型亚共晶成分合金(Cr：11.0wt%~30.0wt%，C：<2.8wt%)，凝固时先析出一次奥氏体枝晶，待温度降至共晶点发生共晶转变形成共晶奥氏体与二次碳化物的混合共晶组织，而复合层为网状形貌的M₃C型碳化物^[7,20]。图5为不同预制体孔径下WC/Fe复合材料复合层的元素分布。Cr分布在共晶奥氏体与二次碳化物中，W、Ni弥散分布在一次奥氏体枝晶内。

图 5 不同预制体孔径下WC/Fe复合材料复合层的元素分布

Figure 5. Element distribution of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

图6为不同预制体孔径下WC/Fe复合材料复合层原孔内的W质量分数分布。其原孔壁处W质量分数较高，而在其原孔心处较低。与晶粒尺寸的变化相反，随预制体孔径增加，其原孔壁处W质量分数基本不变，而在其原孔心处先增大后减小。为进一步表征W扩散均匀性，通过计算得不同预制体孔径下W扩散均匀性分别为84.1%、88.7%、86.9%，表明W扩散均匀性随其孔径增加而先增大后减小，W扩散均匀性的表达式为

图 6 不同预制体孔径下WC/Fe复合材料复合层原孔内的W质量分数分布

Figure 6. W mass fraction distribution into initial hole of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

$\delta = 1 - \frac{{2\sqrt 3 }}{{{\rm{{\text{π}} }}{R^2}}}\frac{{C\left( {0,{t_{\max }}} \right) - C\left( {{R / 2},{t_{\max }}} \right)}}{{C\left( {0,0} \right)}}$

(1)

式中：δ为W扩散均匀性；C(0,t_max)、C(R/2,t_max)分别为预制体原孔壁、原孔心处W质量分数。

2.2 WC/Fe复合材料复合层的硬度分布与耐磨性

图7为不同预制体孔径下WC/Fe复合材料复合层原孔内的硬度分布。其原孔壁处硬度较高，而在其原孔心处较低。与W质量分数的变化相同，随预制体孔径增加，其原孔壁处硬度基本不变，而在其原孔心处先增大后减小。图8为不同预制体孔径下WC/Fe复合材料复合层原孔心处的磨损形貌。R=3 mm时其原孔心处犁沟最明显，R=9 mm时次之，R=6 mm时最不明显。图9为不同预制体孔径下WC/Fe复合材料复合层的磨损量。表明复合层耐磨性随其孔径增加而先增大后减小。

图 7 不同预制体孔径下WC/Fe复合材料复合层原孔内的硬度分布

Figure 7. Hardness distribution into initial hole of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

图 8 不同预制体孔径下WC/Fe复合材料复合层原孔心处的磨损形貌

Figure 8. Wear morphologies at initial hole center of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

图 9 不同预制体孔径下WC/Fe复合材料复合层的磨损量

Figure 9. Wear amount of layer in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

2.3 WC/Fe复合材料复合层的W扩散均匀性机制

为进一步探究影响W扩散均匀性的因素，对预制体原孔内W质量分数分布进行数值模拟。将W扩散区看作受固液界面移动驱动的半无限大物体，且扩散时间近似为预制体孔内熔体凝固时间^[21-22]。下式为W扩散区边界条件的表达式：

$\left\{ \begin{array}{l} C\left( {x,t} \right) = {C_{{\rm{SL}}}},x = x\left( t \right) \\ C\left( {x,t} \right) = {C_{{\rm{LS}}}},x < x\left( t \right) \end{array}\right.$

(2)

式中，C_SL、C_LS分别为固液界面固、液相侧W质量分数。根据Arrhenius方程，W质量分数分布为

$\left\{ \begin{array}{l} C\left( {x,t} \right) = \dfrac{{{C_{{\rm{SL}}}}}}{{{\rm{erf}}\left( k \right) - 1}}\left( {{\rm{erf}}\left( {\dfrac{x}{{2\sqrt {Dt} }}} \right) + 1} \right) \\ D = {D_0}{\rm{exp}}\left( { - \dfrac{{{E_{\rm{A}}}}}{{{k_{\rm{B}}}T}}} \right) \end{array} \right.$

(3)

式中：x为W扩散距离；t为W扩散时间；T为W扩散温度；D为W扩散系数；k为常数；E_A为W元素扩散激活能；k_B为玻尔兹曼常数^[22]。W扩散总时间为

${t_{{\rm{max}}}} = \frac{{{R^2}}}{{64{k^2}D}}$

(4)

通过解析解得W扩散过程同时受温度与固液界面移动影响。故先采用有限元模拟软件COMSOL Multiphysics 5.4模拟预制体孔内熔体凝固时的温度场与相场，表4为预制体孔内熔体凝固时热物理场模拟的参数设置。图10为预制体孔内熔体凝固时热物理场的有限元模拟。发现固液界面明显存在，且其左侧温度较高，熔体为液相，而其右侧温度较低，熔体为固相，即其孔壁处熔体先凝固，且固液界面移动驱动W扩散。此外，随预制体孔径增加，其孔内熔体高温区增多，使其平均温度增高，W扩散系数受W扩散温度影响，扩散温度越高扩散系数越大，其原孔内W质量分数分布曲线斜率绝对值越大；图11为不同预制体孔径下WC/Fe复合材料复合层原孔内W质量分数分布的数值模拟。再将该有限元模拟结果代入数学分析软件MATLAB R2015b的二次开发程序中进行数值模拟，最终得其原孔内W质量分数分布曲线，发现其原孔内W质量分数为W扩散距离的单调递减函数。因预制体孔径孔距比相同且W扩散区为受固液界面移动驱动的半无限大物体，故设置W初始质量分数相同。随预制体孔径增加，其原孔内W质量分数分布曲线斜率绝对值增大，故R=3 mm时其原孔心处W质量分数较R=6 mm时低，但R=6 mm时W扩散距离较R=9 mm时短，故其原孔心处W质量分数R=6 mm时最高，R=9 mm时次之，R=3 mm时最低，即该数值模拟与实验结果相符。

表 4 预制体孔内熔体凝固时热物理场模拟的参数设置

Table 4. Parameters setting of thermal physical field simulation when internal matrix of preform solidifies

Phase	Density/(kg·m⁻³)	Thermal conductivity/(W·m⁻¹·K⁻¹)	Heat capacity/(J·kg⁻¹·K⁻¹)
Fe(s)	8 500	200	400
Fe(l)	7 800	450	550
Inlet temperature/°C	Melting temperature/°C	Temperature transition half width/K	Surface emissivity
1 500	1 100	50	0.8
Specific heat/(J·kg⁻¹·K⁻¹)		Solidification latent heat/(kJ·kg⁻¹)	Heat transfer coefficient/(W·m⁻²·K⁻¹)
60		200	800

下载: 导出CSV

| 显示表格

图 10 不同预制体孔径下孔内熔体凝固时热物理场的有限元模拟

Figure 10. Finite element simulation of thermal physical field when internal matrix solidifies with different hole diameters of preform

下载: 全尺寸图片幻灯片

图 11 不同预制体孔径下WC/Fe复合材料复合层原孔内W质量分数分布的数值模拟

Figure 11. Numerical simulation of W mass fraction distribution in initial hole in WC/Fe composites with different hole diameters of preform

下载: 全尺寸图片幻灯片

预制体孔径较大时，其孔内熔体较多，温度也较高。一方面扩散时间较长，有利于W扩散；另一方面扩散距离较长，不利于W扩散均匀，使预制体原孔心处W质量分数降低。同理，预制体孔径较小时，扩散距离虽短，但扩散时间较短，使W扩散不充分，其原孔心处W质量分数较低，故W扩散均匀性也较低；故预制体孔径适中时，因兼顾扩散距离与扩散时间而使W扩散均匀性最高。综上所述，W扩散过程同时受扩散距离与扩散时间的影响。

2.4 WC/Fe复合材料复合层的耐磨性与W扩散均匀性间关联机制

亚共晶Fe-Cr-C系合金中含大量低硬度、高韧性的一次奥氏体，硬度、耐磨性较低，而W弥散分布在一次奥氏体枝晶内形成M₆C型碳化物Fe₃W₃C，细化晶粒使复合层冲击韧性未明显降低，且引入硬质相使其硬度明显提高^[20,23]，一定范围内也提高其耐磨性^[24]。W扩散均匀性越高，预制体原孔心处W质量分数越高，形成硬质相越多，硬度也越高，最终提高复合层耐磨性。

3. 结论

采用真空消失模铸渗(V-EPC)工艺制备WC/Fe复合材料，选取预制体孔径孔距比相同孔径不同的蜂窝预制体，并将其原孔壁与原孔心处W质量分数作为W扩散均匀性的判断条件，得如下结论。

(1) WC高温下分解，W由预制体孔壁至孔心处扩散，形成弥散分布的硬质相Fe₃W₃C。

(2)预制体原孔壁与原孔心处W质量分数与硬度相差随孔径增加而先增大后减小，复合层耐磨性的变化亦然。

(3) W扩散均匀性同时受扩散距离与扩散时间的影响。预制体孔径较小时，扩散距离虽短，但扩散时间较短，不利于W扩散；预制体孔径较大时，扩散时间虽长，但扩散距离增长，仍不利于W扩散；预制体孔径适中时，因兼顾扩散距离与扩散时间，利于W扩散。

(4)耐磨性与W扩散均匀性间存在关联，W扩散均匀性越高，预制体原孔心处W质量分数越高，形成硬质相越多，硬度也越高，一定范围内复合层耐磨性也越高。

图 1 不同方式去剂前后氮化硅纤维表面的SEM图像

SIN-B—Bare fibers; SIN-S—As-received fibers; SIN-A—Fibers removed sizing agent with acetone; SIN-H—Fibers removed sizing agent with water

Figure 1. SEM images of surface of silicon nitride fibers before and after sizing removal in different ways (((a), (b)) SIN-B; ((c), (d)) SIN-S; ((e), (f)) SIN-A; ((g), (h)) SIN-H)

下载: 全尺寸图片幻灯片

图 2 不同方法制备的氮化硅纤维/石蜡复合材料介电测试试样

Figure 2. Dielectric test samples of silicon nitride fiber/paraffin wax composites prepared by different methods

下载: 全尺寸图片幻灯片

图 3 不同纤维含量的氮化硅纤维/石蜡复合材料依据三种混合模型估算所得氮化硅纤维的相对介电常数

Figure 3. Permittivities of silicon nitride fiber evaluated by three dielectric mixture models according to silicon nitride fiber/paraffin wax composites with different fiber contents

下载: 全尺寸图片幻灯片

图 4 不同切割长度的氮化硅纤维/石蜡复合材料的相对介电常数

Figure 4. Relative permittivity of silicon nitride fiber/paraffin wax composites with different chopped lengths of silicon nitride fiber

下载: 全尺寸图片幻灯片

图 5 不同切割长度的氮化硅纤维/石蜡复合材料的照片

Figure 5. Photographs of silicon nitride fiber/paraffin wax composites with different chopped lengths of silicon nitride fiber

下载: 全尺寸图片幻灯片

图 6 同一氮化硅纤维/石蜡复合材料不同时间相对介电常数测试结果

Figure 6. Permitvities of silicon nitride fiber/paraffin wax composites sample obtained at different times

下载: 全尺寸图片幻灯片

图 7 高Q腔法测试得到的石英纤维的相对介电常数

Figure 7. Permittivities of quartz fiber based on high-Q cavity method

下载: 全尺寸图片幻灯片

图 8 不同方式去剂的氮化硅纤维的相对介电常数及损耗角正切

Figure 8. Permittivities and dielectric loss tangent of silicon nitride fibers after sizing removal by different methods

下载: 全尺寸图片幻灯片

表 1 表面能测试试剂相关参数

Table 1 Parameters of surface energy test reagent

Reagent	Surface energy/(mJ·m^–²)
Reagent	$\gamma _{\rm{L} }^{}$	$\gamma _{\rm{L}}^{\rm{d}}$	$\gamma _{\rm{L}}^{\rm{p}}$
H₂O	72.8	21.8	51.0
Formamide	58.2	39.5	18.7
Methylene iodide	50.8	48.5	2.3
Notes： ${\gamma _{\rm{L} }^{} }$ , $\gamma _{\rm{L}}^{\rm{d}}$ , $\gamma _{\rm{L}}^{\rm{p}}$ —Solid-liquid surface tension, dispersion and polarity property, respectively.

下载: 导出CSV

表 2 不同方法制备的氮化硅纤维/石蜡复合材料及反演的氮化硅纤维的介电性能

Table 2 Dielectric properties of silicon nitride fiber/paraffin wax composites and silicon nitride fiber prepared by different methods

Type	Frequency/ MHz	Permittivity by test			Permittivity of fiber calculated by Lichtenecker formula
Type	Frequency/ MHz	ε₁	ε₂	Average	ε₁	ε₂	Difference	Average
UB	7 382	2.33	2.34	2.34	2.33	3.23	0.90	2.74
	8 173	2.33	2.35	2.34	2.33	4.47	2.14	3.23
	9 335	2.33	2.35	2.34	2.33	4.47	2.14	3.23
	10 736	2.33	2.35	2.34	2.33	4.47	2.14	3.23
	12 283	2.33	2.35	2.34	2.33	4.47	2.14	3.23
	13 914	2.34	2.35	2.35	3.23	4.47	1.24	3.80
	15 607	2.33	2.35	2.34	2.33	4.47	2.14	3.23
	17 348	2.33	2.34	2.34	2.33	3.23	0.90	2.74
FMB	7 382	2.49	2.49	2.49	4.75	4.75	0	4.75
	8 173	2.49	2.50	2.50	4.75	4.95	0.20	4.85
	9 334	2.49	2.50	2.50	4.75	4.95	0.20	4.85
	10 733	2.49	2.49	2.49	4.75	4.75	0	4.75
	12 277	2.47	2.49	2.48	4.38	4.75	0.37	4.56
	13 905	2.47	2.49	2.48	4.38	4.75	0.37	4.56
	15 592	2.47	2.48	2.48	4.38	4.56	0.18	4.47
	17 331	2.46	2.48	2.47	4.20	4.56	0.36	4.38
Notes：ε₁, ε₂—Permittivity of the front and back of the samples or fibers; Difference and average are the differences and averages of ε₁ and ε₂.

下载: 导出CSV

表 3 氮化硅纤维上浆剂薄膜丙酮处理前后的表面能及极性/色散比

Table 3 Surface energy and ratio of polarity to dispersion of silicon nitride fibers’ sizing film before and after treated by acetone

Sample	Surface energy
Sample	$\gamma _{\rm{S} }^{}$ / (mJ·m^–²)	$\gamma_{\rm{S} }^{\rm{p} }$ / (mJ·m^–²)	$\gamma_{\rm{S} }^{\rm{d} }$ / (mJ·m^–²)	$\gamma_{\rm{S} }^{\rm{p} }$ / $\gamma_{\rm{S} }^{\rm{d} }$
Sizing film	63.6	22.3	41.4	0.54
Acetone treated sizing film	67.2	34.1	33.1	1.03
Notes: ${\gamma _{\rm{S} }^{} }$ , $\gamma _{\rm{S}}^{\rm{d}}$ , $\gamma _{\rm{S}}^{\rm{p}}$ —Solid surface energy, dispersion and polarity property, respectively.

下载: 导出CSV

参考文献(40)

[1]	TAKI T, INUI M, OKAMURA K, et al. A study of nitridation process of polycarbosilane fibers by solid-state high-resolution NMR[J]. Applied Magnetic Resonance,1991,2(1):61-68. DOI: 10.1007/BF03166267
[2]	PETZOW G, HERRMANN M. Silicon nitride ceramics[J]. Structure and Bonding,2009,102:47-167.
[3]	TAKI T, OKAMURA K, SATO M, et al. A study on the electron irradiation curing mechanism of polycarbosilane fibres by solid-state<sup>29</sup>Si high-resolution nuclear magnetic resonance spectroscopy[J]. Journal of Materials Science Letters,1988,7(3):209-211. DOI: 10.1007/BF01730172
[4]	邹春荣, 张长瑞, 肖永栋, 等. 高性能透波陶瓷纤维的研究现状和展望[J]. 硅酸盐通报, 2013, 32(2):274-279. ZOU Chunrong, ZHANG Changrui, XIAO Yongdong, et al. Progress and prospect of high performance wave-transparent ceramic fibers[J]. Bulletin of the Chinese Ceramic Society,2013,32(2):274-279(in Chinese).
[5]	李端, 张长瑞, 李斌, 等. 氮化硅高温透波材料的研究现状和展望[J]. 宇航材料工艺, 2011, 41(6):4-9. DOI: 10.3969/j.issn.1007-2330.2011.06.002 LI Duan, ZHANG Changrui, LI Bin, et al. High temperature wave-transparent silicon nitride materials[J]. Aerospace Materials <italic>&</italic> Technology,2011,41(6):4-9(in Chinese). DOI: 10.3969/j.issn.1007-2330.2011.06.002
[6]	ZHOU J, YE F, CUI X, et al. Mechanical and dielectric properties of two types of Si<sub>3</sub>N<sub>4</sub> fibers annealed at elevated temperatures[J]. Materials,2018,11(9):1498.
[7]	赵晓明, 李卫斌, 赵家琪, 等. 涤纶纤维介电性能研究[J]. 成都纺织高等专科学校学报, 2016, 33(1):89-93. ZHAO Xiaoming, LI Weibin, ZHAO Jiaqi, et al. Research on dielectric properties of polyester fiber[J]. Journal of Chengdu Textile College,2016,33(1):89-93(in Chinese).
[8]	伏金刚, 朱冬梅, 罗发, 等. 短切碳纤维/环氧树脂复合材料的介电性能研究[J]. 材料导报, 2012, 26(18):58-60. DOI: 10.3969/j.issn.1005-023X.2012.18.016 FU Jingang, ZHU Dongmei, LUO Fa, et al. Dielectric properties of short carbon fiber filled epoxy resins composites[J]. Materials Review,2012,26(18):58-60(in Chinese). DOI: 10.3969/j.issn.1005-023X.2012.18.016
[9]	陈平, 张显友, 陈辉. 纤维含量及排列方向对单向纤维复合材料介电性能的影响[J]. 复合材料学报, 1993, 10(2):61-67. CHEN Ping, ZHANG Xianyou, CHEN Hui. Influence of content and orientation of fiber on the electrical properties of unidirectional composites[J]. Acta Materiae Compositae Sinica,1993,10(2):61-67(in Chinese).
[10]	伏金刚, 朱冬梅, 周万城, 等. 定向分布碳纤维复合材料介电性能研究[J]. 无机材料学报, 2012, 27(11):1223-1227. FU Jingang, ZHU Dongmei, ZHOU Wancheng, et al. Anisotropic dielectric properties of short carbon fiber composites[J]. Journal of Inorganic Materials,2012,27(11):1223-1227(in Chinese).
[11]	张灵林. 碳纤维增强树脂基复合材料微波固化机理[D]. 南京: 南京航空航天大学, 2018. ZHANG Linglin. Microwave curing mechanism of carbon fiber reinforced polymer composites[D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2018(in Chinese).
[12]	高正平, 王晓红. 多层吸波材料的电磁参数反演[J]. 材料工程, 2006(7):19-22, 27. GAO Zhengping, WANG Xiaohong. Inverse for EM parameters of multilayer absorbing material[J]. Journal of Materials Engineering,2006(7):19-22, 27(in Chinese).
[13]	饶克谨, 高正平. 碳纤维多向铺层板的雷达反射特性[J]. 电子科技大学学报, 1998, 27(4):397-402. RAO Kejin, GAO Zhengping. Radar reflection characteristics of multi-plies carbon fiber[J]. Journal of University Electronic Science and Technology of China,1998,27(4):397-402(in Chinese).
[14]	GAO H, LUO F, WEN Q, et al. Effect of preparation conditions on mechanical, dielectric and microwave absorption properties of SiC fiber/mullite matrix composite[J]. Ceramics International,2019,45(9):11625-11632. DOI: 10.1016/j.ceramint.2019.03.034
[15]	OMRI M A, SANJAY M R, TRIKI A, et al. Dielectric properties and interfacial adhesion of jute, kenaf and E-glass fabrics reinforcing epoxy composites[J]. Polymer Composites,2019,40(6):2142-2153. DOI: 10.1002/pc.25001
[16]	KUANG J, HOU X, XIAO T, et al. Enhanced dielectric permittivity and microwave absorbing properties of Au-decorated SiC nanowires[J]. Japanese Journal of Applied Physics,2019,58(6):060916.
[17]	曾娟娟. 碳纳米纤维/聚合物基复合材料的结晶行为和介电性能研究[D]. 北京: 北京化工大学, 2013. ZENG Juanjuan. The crystallization behavior and dielectric properties of carbon nanofibers/polymer composites[D]. Beijing: Beijing University of Chemical Technology, 2013(in Chinese).
[18]	WESTPHAL W B, IGLESIAS J. Dielectric measurements on high-temperature materials: AFML TR70-138[R]. Cambridge: Massachusetts Institute of Technology Cambridge, 1970.
[19]	黎义, 李建保, 何小瓦. 采用谐振腔法研究透波材料的高温介电性能[J]. 红外与毫米波学报, 2004, 23(2):157-160. DOI: 10.3321/j.issn:1001-9014.2004.02.017 LI Yi, LI Jianbao, HE Xiaowa, et al. Study on high temperature dielectric properties of magnetic window materials by cavity resonator method[J]. Journal of Infrared and Millimeter Waves,2004,23(2):157-160(in Chinese). DOI: 10.3321/j.issn:1001-9014.2004.02.017
[20]	LI E, LI Z, NIE Z, et al. Measurement of complex permittivity of dielectrics at high temperatures by using cylindrical cavity[C]//2008 China-Japan Joint Microwave Conference. Shanghai: IEEE, 2008: 707-709.
[21]	李军奇, 周万城, 罗发, 等. 晶相组成对Si<sub>3</sub>N<sub>4</sub>陶瓷介电性能的影响[J]. 稀有金属材料与工程, 2009, 38(s2):384-386. LI Junqi, ZHOU Wancheng, LUO Fa, et al. Influence of crystal phase on dielectric properties of silicon nitride ceramics[J]. Rare Metal Materials and Engineering,2009,38(s2):384-386(in Chinese).
[22]	李巍. 低耗介质介电参数的圆柱腔测量方法研究[D]. 西安: 西北工业大学, 2006. LI Wei. Research on cylindrical cavity measurement method for low-consumption dielectric parameters[D]. Xi’an: Northwestern Polytechnical University, 2006(in Chinese).
[23]	胡暄, 纪小宇, 邵长伟, 等. 连续氮化硅陶瓷纤维的组成结构与性能研究[J]. 功能材料, 2016, 47(s1):123-126. HU Xuan, JI Xiaoyu, SHAO Changwei, et al. Composition, structure and properties of continuous silicon nitride fibers[J]. Journal of Functional Materials,2016,47(s1):123-126(in Chinese).
[24]	LICHTENECKER K. Die Dielektrizitätskonstante natürlicher und künstlicher mischkörper[J]. Physikalische Zeitschrift,1926,27:115-158.
[25]	LICHTENECKER K, ROTHER K. Die herleitung des logarithmischen mischungs-gesetzes aus allegemeinen prinzipien der stationaren stromung[J]. Physikalische Zeitschrift,1931,32:255-260.
[26]	张娟, 周明星, 张敬义, 等. 透波氮化硅纤维的综合性能评价表征研究[J]. 宇航材料工艺, 2019, 49(4):72-75, 94. DOI: 10.12044/j.issn.1007-2330.2019.04.014 ZHANG Juan, ZHOU Mingxing, ZHANG Jingyi, et al. Characterization and properties of new silicon nitride fibers for wave-transmitting[J]. Aerospace Materials <italic>&</italic> Technology,2019,49(4):72-75, 94(in Chinese). DOI: 10.12044/j.issn.1007-2330.2019.04.014
[27]	OWENS D K, WENDT R C. Estimation of the surface free energy of polymers[J]. Journal of Applied Polymer Science,1969,13(8):1741-1747.
[28]	中国国家标准化管理委员会. 固体电介质微波复介电常数的测试方法: GB/T 5597—1999[S]. 北京: 中国标准出版社, 2009. Standardization Administration of the People’s Republic of China. Test method for complex permittivity of solid dielectric materials at microwave frequencies: GB/T 5597—1999[S]. Beijing: China Standards Press, 2009(in Chinese).
[29]	BRUGGEMAN D A G. Berechnung verschiedener physikalischer Konstanten von heterogenen Substanzen Ⅱ: Dielektrizitätskonstanten und Leitfähigkeiten von Vielkristallen der nichtregulären Systeme[J]. Annalen der Physik,1936,417(7):645-672. DOI: 10.1002/andp.19364170706
[30]	LOOYENGA H. Dielectric constants of homogeneous mixture[J]. Molecular Physics,1965,9(6):501-511. DOI: 10.1080/00268976500100671
[31]	SIMPKIN R. Derivation of Lichtenecker’s logarithmic mixture formula from Maxwell’s equations[J]. IEEE Transactions on Microwave Theory and Techniques,2010,58(3):545-550. DOI: 10.1109/TMTT.2010.2040406
[32]	TUNCER E. The Landau-Lifshitz/Looyenga dielectric mixture expression and its self-similar fractal nature[EB/OL]. (2005-03-31) [2019-10-16]. Website: arxiv.org/abs/cond-mat/0503750.
[33]	MARQUARDT P, NIMTZ G. Size-governed electromagnetic absorption by metal particles[J]. Physical Review B,1989,40(11):7996-7998. DOI: 10.1103/PhysRevB.40.7996
[34]	GONCHARENKO A V, LOZOVSKI V Z, VENGER E F. Lichtenecker’s equation: Applicability and limitations[J]. Optics Communications,2000,174(1-4):19-32.
[35]	MACKAY T G. Bruggeman formalism versus “Bruggeman formalism”: Particulate composite materials comprising oriented ellipsoidal particles[J]. Journal of Nanophotonics,2012,6(1):069501. DOI: 10.1117/1.JNP.6.069501
[36]	MACKAY T G, LAKHTAKIA A. A limitation of the Bruggeman formalism for homogenization[J]. Optics Communications,2004,234(1-6):35-42.
[37]	KAMPIA R D, LAKHTAKIA A. Bruggeman model for chiral particulate composites[J]. Journal of Physics D: Applied Physics,1992,25(10):1390-1394. DOI: 10.1088/0022-3727/25/10/002
[38]	STAICOPOLUS D N. The computation of surface tension and of contact angle by the sessile-drop method[J]. Journal of Colloid Science,1962,17(5):439-447. DOI: 10.1016/0095-8522(62)90055-7
[39]	FOWKES F M. Determination of interfacial tensions, contact angles, and dispersion forces in surfaces by assuming additivity of intermolecular interactions in surfaces[J]. Journal of Physical Chemistry,1962,66(2):382.
[40]	李刚, 欧书方, 赵敏健. 石英玻璃纤维的性能和用途[J]. 玻璃纤维, 2007(4):9-13, 16. LI Gang, OU Shufang, ZHAO Minjian. Properties and uses of quartz glass fiber[J]. Fiber Glass,2007(4):9-13, 16(in Chinese).